Experimental Analysis of the Effect of Limescale on the Wettability of Indirect Evaporative Cooling System Plates
Abstract
1. Introduction
2. Qualitative Analyses, Materials, and Methods
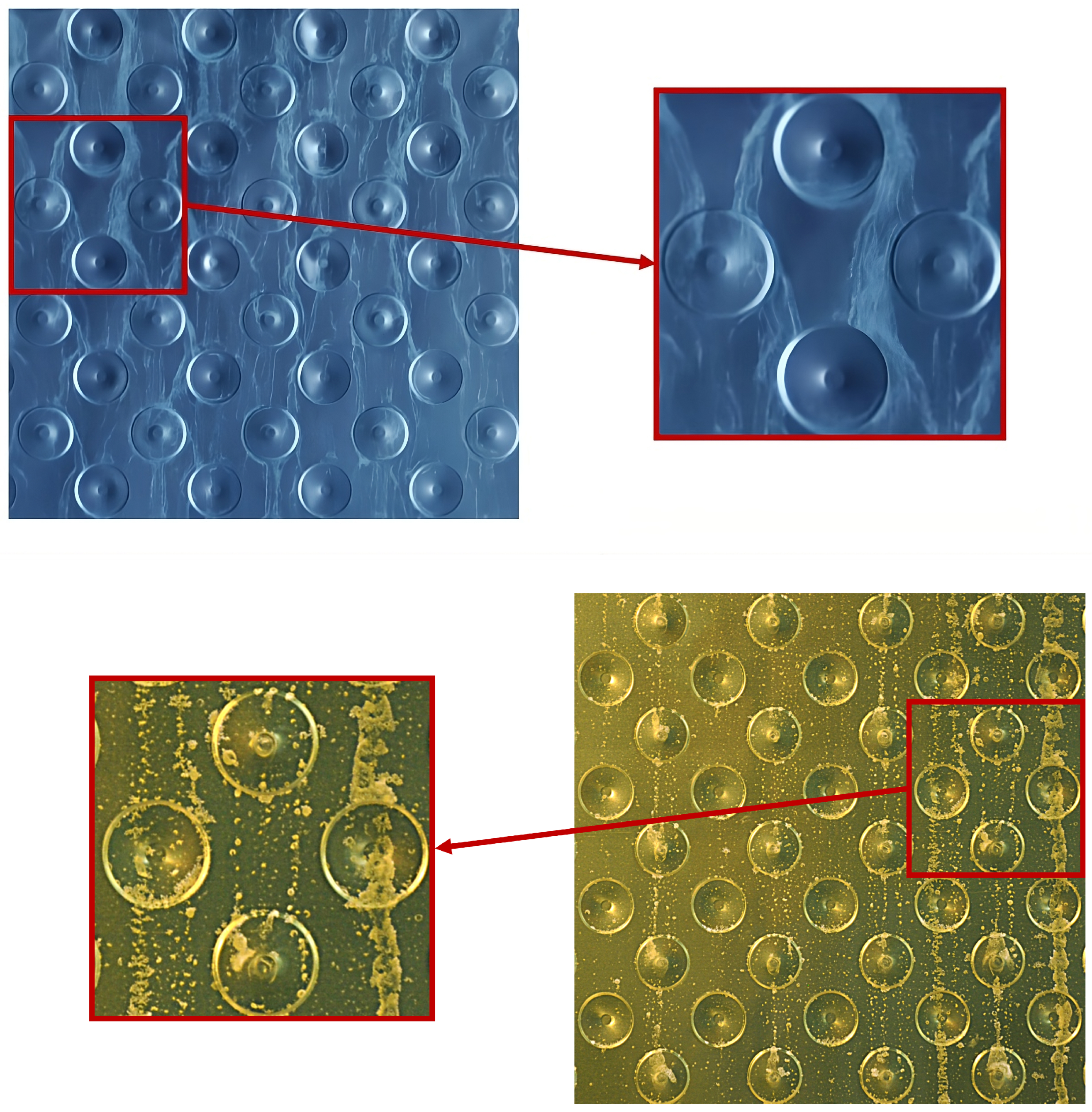
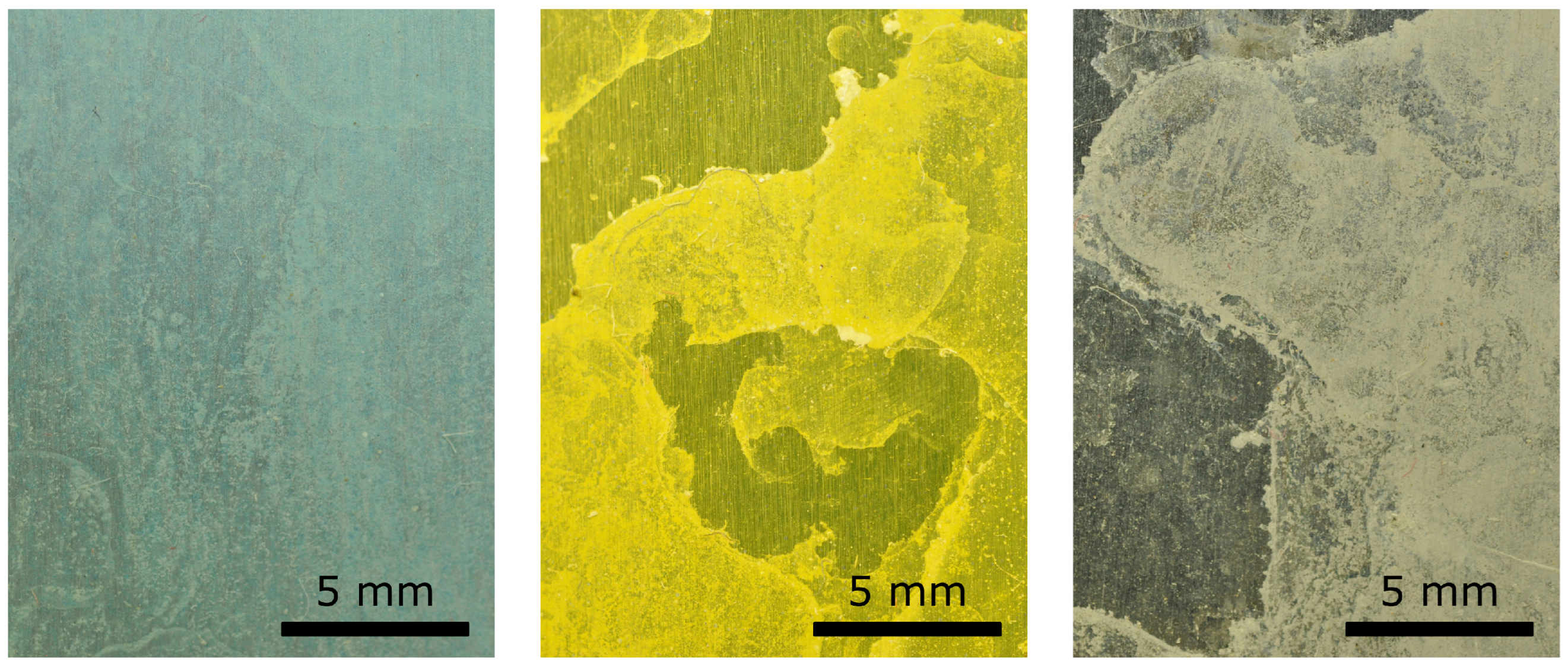
2.1. Qualitative Analyses
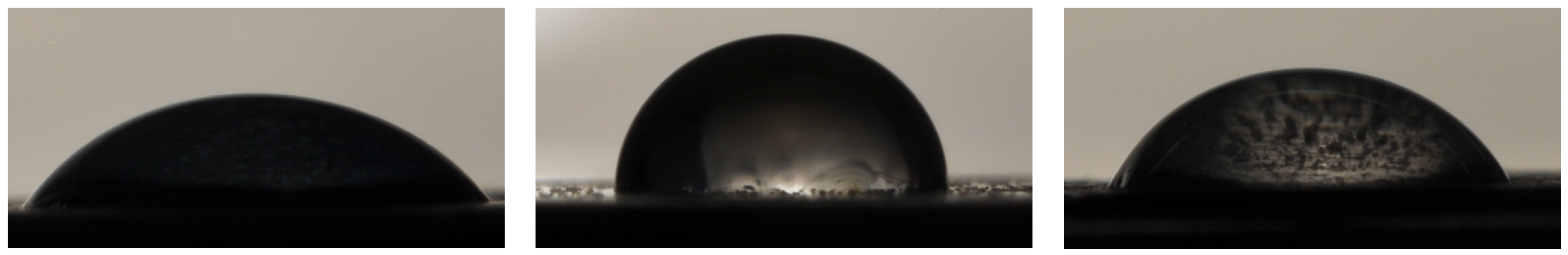
2.2. Static Contact Angle
2.3. Spreading Factor and Reversible Work of Adhesion
3. Results and Discussion
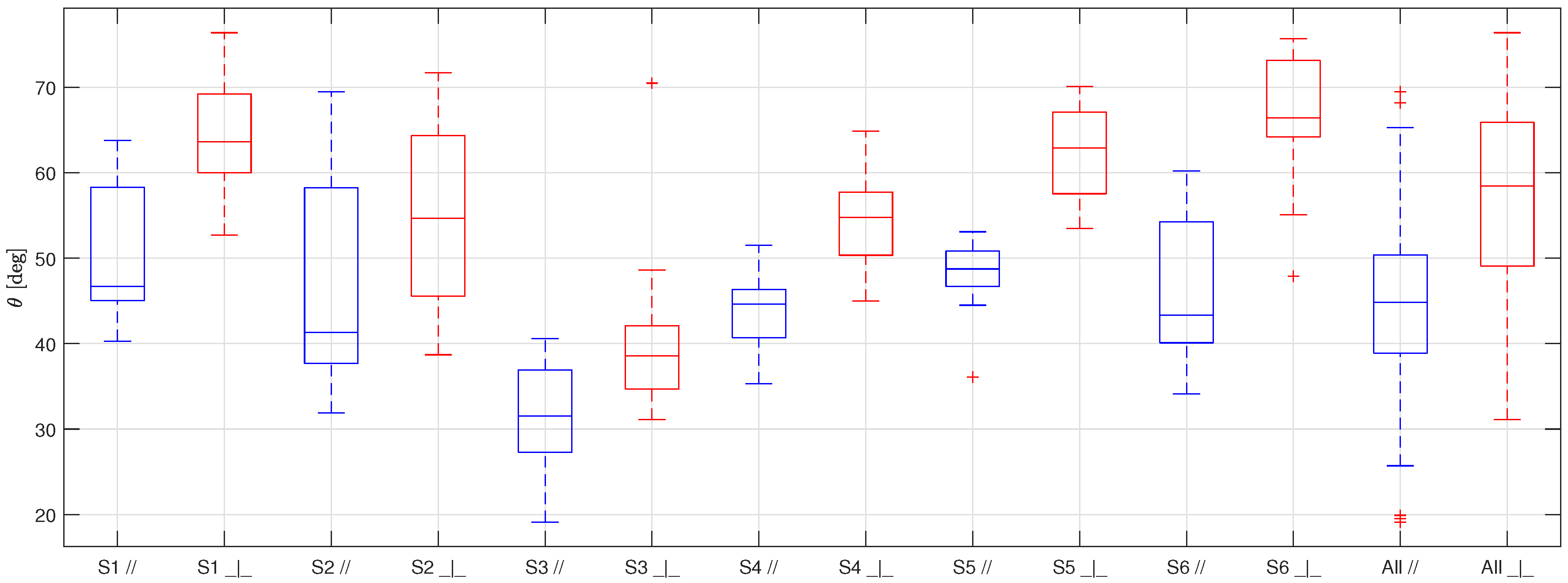
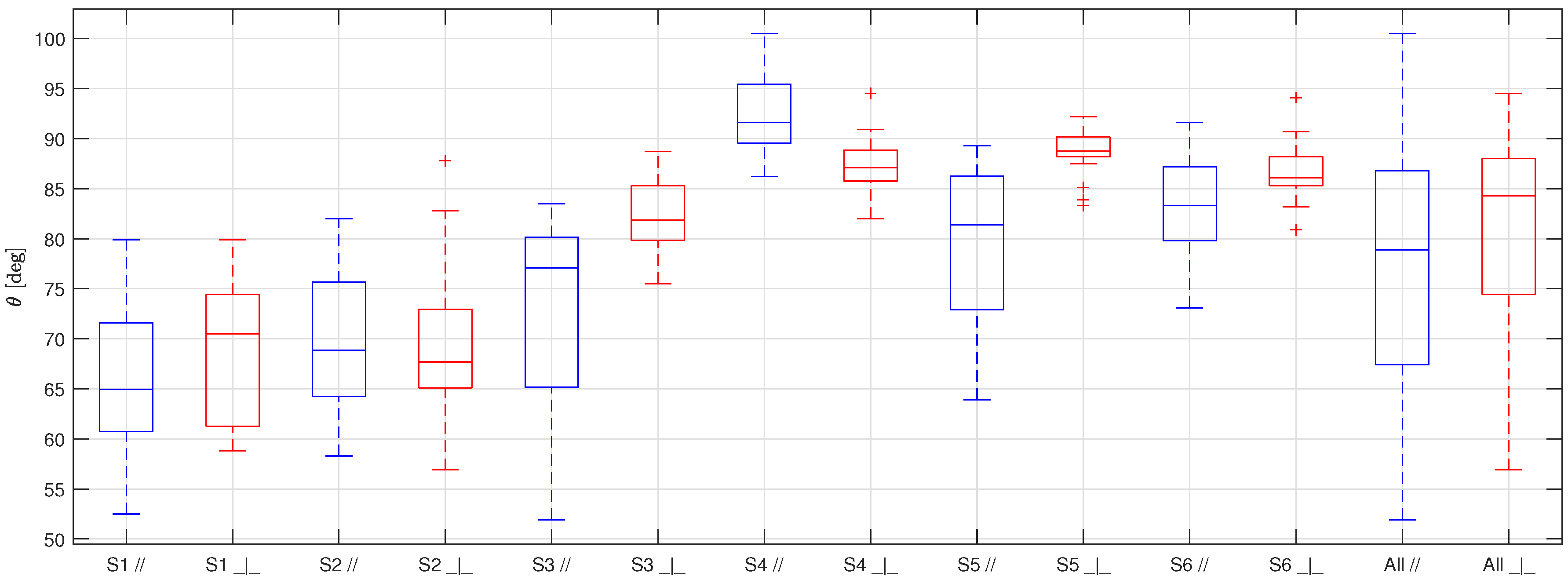
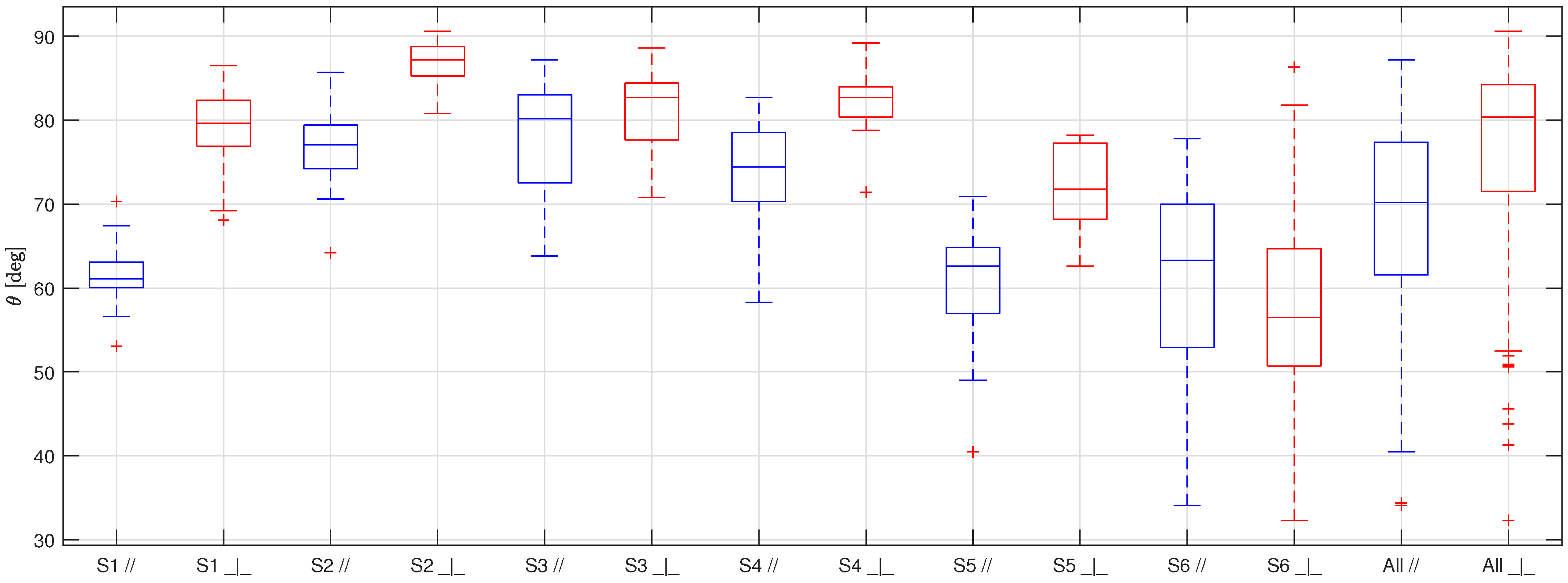
3.1. Static Contact Angle
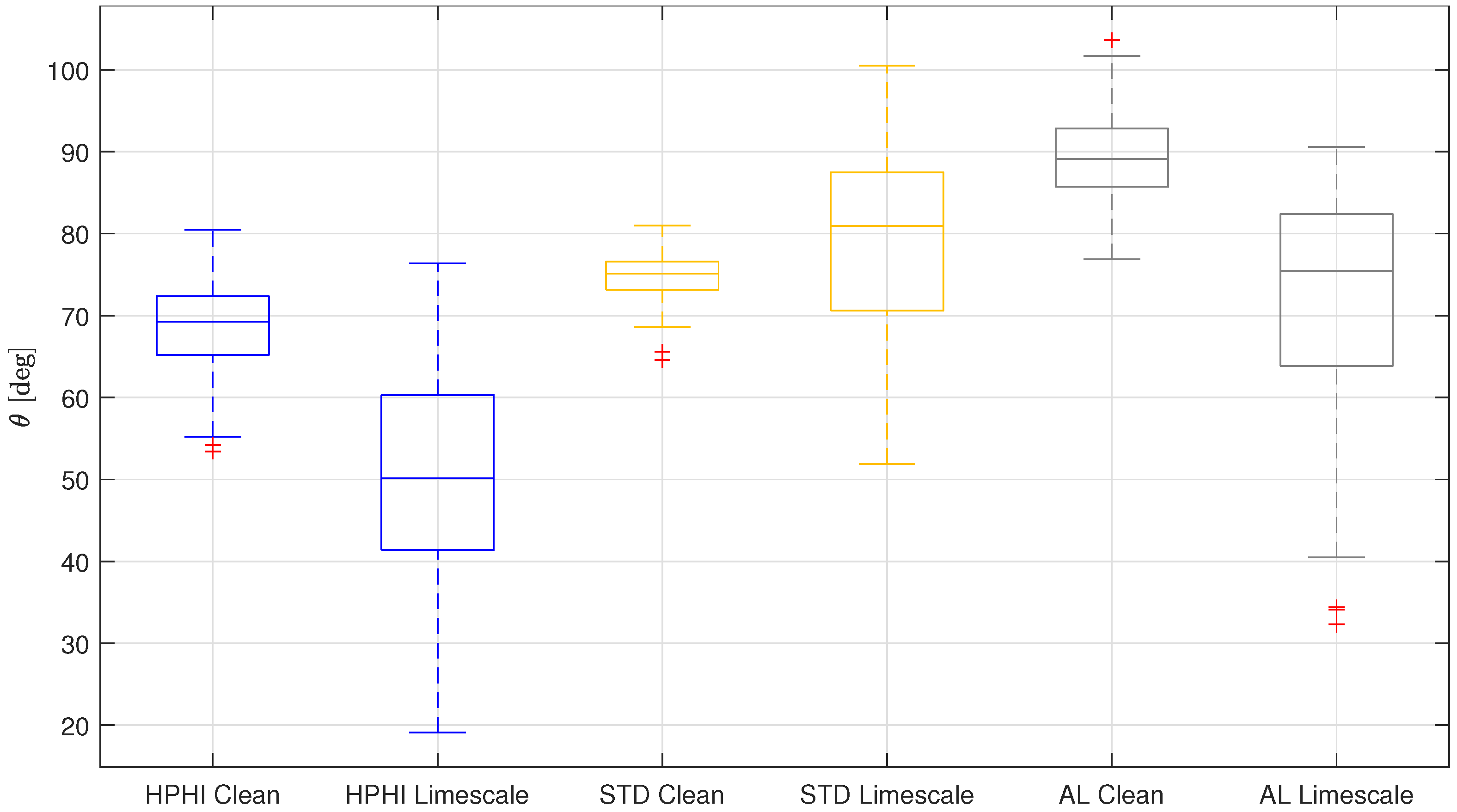
3.2. Comparison between Clean and Limescale Surfaces
3.3. Spreading Factor and Reversible Work of Adhesion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| ADSA | Axisymmetric drop shape analysis |
| AL | Aluminum uncoated surface |
| HPHI | Aluminum surface coated with a hydrophilic lacquer |
| IEC | Indirect evaporative cooling |
| STD | Aluminum surface covered with a standard epoxy coating |
| S | Spreading factor |
| Reversible work of adhesion | |
| Interface energy between the solid surface and the gaseous phase | |
| Interface energy between the solid surface and the liquid phase | |
| Interface energy between the liquid and gaseous phases | |
| Static contact angle |
References
- Yang, H.; Shi, W.; Chen, Y.; Min, Y. Research development of indirect evaporative cooling technology: An updated review. Renew. Sustain. Energy Rev. 2021, 145, 111082. [Google Scholar] [CrossRef]
- Duan, Z.; Zhan, C.; Zhang, X.; Mustafa, M.; Zhao, X.; Alimohammadisagvand, B.; Hasan, A. Indirect evaporative cooling: Past, present and future potentials. Renew. Sustain. Energy Rev. 2012, 16, 6823–6850. [Google Scholar] [CrossRef]
- Amer, O.; Boukhanouf, R.; Ibrahim, H.G. A review of evaporative cooling technologies. Int. J. Environ. Sci. Dev. 2015, 6, 111. [Google Scholar] [CrossRef]
- Hussain, I.; Bibi, F.; Bhat, S.A.; Sajjad, U.; Sultan, M.; Ali, H.M.; Azam, M.W.; Kaushal, S.K.; Hussain, S.; Yan, W.M. Evaluating the parameters affecting the direct and indirect evaporative cooling systems. Eng. Anal. Bound. Elem. 2022, 145, 211–223. [Google Scholar] [CrossRef]
- Porumb, B.; Ungureşan, P.; Tutunaru, L.F.; Şerban, A.; Bălan, M. A review of indirect evaporative cooling operating conditions and performances. Energy Procedia 2016, 85, 452–460. [Google Scholar] [CrossRef]
- Chua, K.; Xu, J.; Cui, X.; Ng, K.; Islam, M. Numerical heat and mass transfer analysis of a cross-flow indirect evaporative cooler with plates and flat tubes. Heat Mass Transf. 2016, 52, 1765–1777. [Google Scholar] [CrossRef]
- De Antonellis, S.; Joppolo, C.M.; Liberati, P.; Milani, S.; Romano, F. Modeling and experimental study of an indirect evaporative cooler. Energy Build. 2017, 142, 147–157. [Google Scholar] [CrossRef]
- Guilizzoni, M.; Milani, S.; Liberati, P.; De Antonellis, S. Effect of plates coating on performance of an indirect evaporative cooling system. Int. J. Refrig. 2019, 104, 367–375. [Google Scholar] [CrossRef]
- Caruana, R.; De Antonellis, S.; Marocco, L.; Guilizzoni, M. Modeling of Indirect Evaporative Cooling Systems: A Review. Fluids 2023, 8, 303. [Google Scholar] [CrossRef]
- Caruana, R.; De Antonellis, S.; Marocco, L.; Liberati, P.; Guilizzoni, M. Experimental Characterization of the Wettability of Coated and Uncoated Plates for Indirect Evaporative Cooling Systems. Fluids 2023, 8, 122. [Google Scholar] [CrossRef]
- Orkoula, M.G.; Koutsoukos, P.G.; Robin, M.; Vizika, O.; Cuiec, L. Wettability of CaCO3 surfaces. Colloids Surf. A Physicochem. Eng. Asp. 1999, 157, 333–340. [Google Scholar] [CrossRef]
- Hu, B.; Fraunholz, N.; Rem, P. Wetting technologies for high-accuracy sink-float separations in water-based media. Open Waste Manag. J. 2010, 3, 71–80. [Google Scholar] [CrossRef]
- Jin, H.Q.; Athreya, H.; Wang, S.; Nawaz, K. Experimental study of crystallization fouling by calcium carbonate: Effects of surface structure and material. Desalination 2022, 532, 115754. [Google Scholar] [CrossRef]
- Liu, Y.; Jiang, T.; Zhou, Y.; Zhang, Z.; Wang, Z.; Tong, H.; Shen, X.; Wang, Y. Evaluation of the attachment, proliferation, and differentiation of osteoblast on a calcium carbonate coating on titanium surface. Mater. Sci. Eng. C 2011, 31, 1055–1061. [Google Scholar] [CrossRef]
- Cruz, M.A.E.; Ruiz, G.C.; Faria, A.N.; Zancanela, D.C.; Pereira, L.S.; Ciancaglini, P.; Ramos, A.P. Calcium carbonate hybrid coating promotes the formation of biomimetic hydroxyapatite on titanium surfaces. Appl. Surf. Sci. 2016, 370, 459–468. [Google Scholar] [CrossRef]
- Marmur, A. Soft contact: Measurement and interpretation of contact angles. Soft Matter 2006, 2, 12–17. [Google Scholar] [CrossRef]
- Ponomar, M.; Krasnyuk, E.; Butylskii, D.; Nikonenko, V.; Wang, Y.; Jiang, C.; Xu, T.; Pismenskaya, N. Sessile drop method: Critical analysis and optimization for measuring the contact angle of an ion-exchange membrane surface. Membranes 2022, 12, 765. [Google Scholar] [CrossRef]
- Tadmor, R. Approaches in wetting phenomena. Soft Matter 2011, 7, 1577–1580. [Google Scholar] [CrossRef]
- Tsoumpas, Y.; Dehaeck, S.; Galvagno, M.; Rednikov, A.; Ottevaere, H.; Thiele, U.; Colinet, P. Nonequilibrium Gibbs’ criterion for completely wetting volatile liquids. Langmuir 2014, 30, 11847–11852. [Google Scholar] [CrossRef] [PubMed]
- Wenzel, R.N. Resistance of solid surfaces to wetting by water. Ind. Eng. Chem. 1936, 28, 988–994. [Google Scholar] [CrossRef]
- Cassie, A.; Baxter, S. Wettability of porous surfaces. Trans. Faraday Soc. 1944, 40, 546–551. [Google Scholar] [CrossRef]
- Feng, L.; Zhang, Y.; Xi, J.; Zhu, Y.; Wang, N.; Xia, F.; Jiang, L. Petal effect: A superhydrophobic state with high adhesive force. Langmuir 2008, 24, 4114–4119. [Google Scholar] [CrossRef]
- Hejazi, V.; Moghadam, A.D.; Rohatgi, P.; Nosonovsky, M. Beyond Wenzel and Cassie–Baxter: Second-order effects on the wetting of rough surfaces. Langmuir 2014, 30, 9423–9429. [Google Scholar] [CrossRef]
- Li, J.; Jing, Z.; Yang, Y.; Wang, Q.; Lei, Z. From Cassie state to Gecko state: A facile hydrothermal process for the fabrication of superhydrophobic surfaces with controlled sliding angles on zinc substrates. Surf. Coat. Technol. 2014, 258, 973–978. [Google Scholar] [CrossRef]
- Tadmor, R.; Yadav, P.S. As-placed contact angles for sessile drops. J. Colloid Interface Sci. 2008, 317, 241–246. [Google Scholar] [CrossRef] [PubMed]
- Del Rıo, O.; Neumann, A. Axisymmetric drop shape analysis: Computational methods for the measurement of interfacial properties from the shape and dimensions of pendant and sessile drops. J. Colloid Interface Sci. 1997, 196, 136–147. [Google Scholar]
- Guilizzoni, M. Drop shape visualization and contact angle measurement on curved surfaces. J. Colloid Interface Sci. 2011, 364, 230–236. [Google Scholar] [CrossRef]
- Hoorfar, M.; Neumann, A. Recent progress in axisymmetric drop shape analysis (ADSA). Adv. Colloid Interface Sci. 2006, 121, 25–49. [Google Scholar] [CrossRef]
- Guilizzoni, M.; Sapienza, J.; Caruana, R.; Basso Peressut, A.S.; Di Virgilio, M.; Latorrata, S. Wettability of sulfonated graphene oxide membranes for proton exchange membrane fuel cells. Colloids Surf. A Physicochem. Eng. Asp. 2024, 684, 133151. [Google Scholar] [CrossRef]
- Zisman, W.A. Relation of the Equilibrium Contact Angle to Liquid and Solid Constitution; ACS Publications: Washington, DC, USA, 1964. [Google Scholar]
- Ayyad, A.H. Thermodynamic derivation of the Young–Dupré form equations for the case of two immiscible liquid drops resting on a solid substrate. J. Colloid Interface Sci. 2010, 346, 483–485. [Google Scholar] [CrossRef]


| Material and Orientation | Mean | Median | Standard Deviation |
|---|---|---|---|
| HPHI Parallel | 45° | 45° | 10° |
| HPHI Perpendicular | 57° | 58° | 12° |
| STD Parallel | 77° | 81° | 11° |
| STD Perpendicular | 79° | 84° | 10° |
| AL Parallel | 69° | 70° | 11° |
| AL Perpendicular | 77° | 80° | 12° |
| Material | Contact Angle Median | Spreading Factor | Reversible Work of Adhesion |
|---|---|---|---|
| HPHI | 50° | ||
| STD | 81° | ||
| AL | 75° |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Caruana, R.; Marocco, L.; Liberati, P.; Guilizzoni, M. Experimental Analysis of the Effect of Limescale on the Wettability of Indirect Evaporative Cooling System Plates. Fluids 2024, 9, 76. https://doi.org/10.3390/fluids9030076
Caruana R, Marocco L, Liberati P, Guilizzoni M. Experimental Analysis of the Effect of Limescale on the Wettability of Indirect Evaporative Cooling System Plates. Fluids. 2024; 9(3):76. https://doi.org/10.3390/fluids9030076
Chicago/Turabian StyleCaruana, Roberta, Luca Marocco, Paolo Liberati, and Manfredo Guilizzoni. 2024. "Experimental Analysis of the Effect of Limescale on the Wettability of Indirect Evaporative Cooling System Plates" Fluids 9, no. 3: 76. https://doi.org/10.3390/fluids9030076
APA StyleCaruana, R., Marocco, L., Liberati, P., & Guilizzoni, M. (2024). Experimental Analysis of the Effect of Limescale on the Wettability of Indirect Evaporative Cooling System Plates. Fluids, 9(3), 76. https://doi.org/10.3390/fluids9030076







