Hydromechanical Transmission IC2OC: Component Sizing and Optimization †
Abstract
1. Introduction
2. Materials and Methods
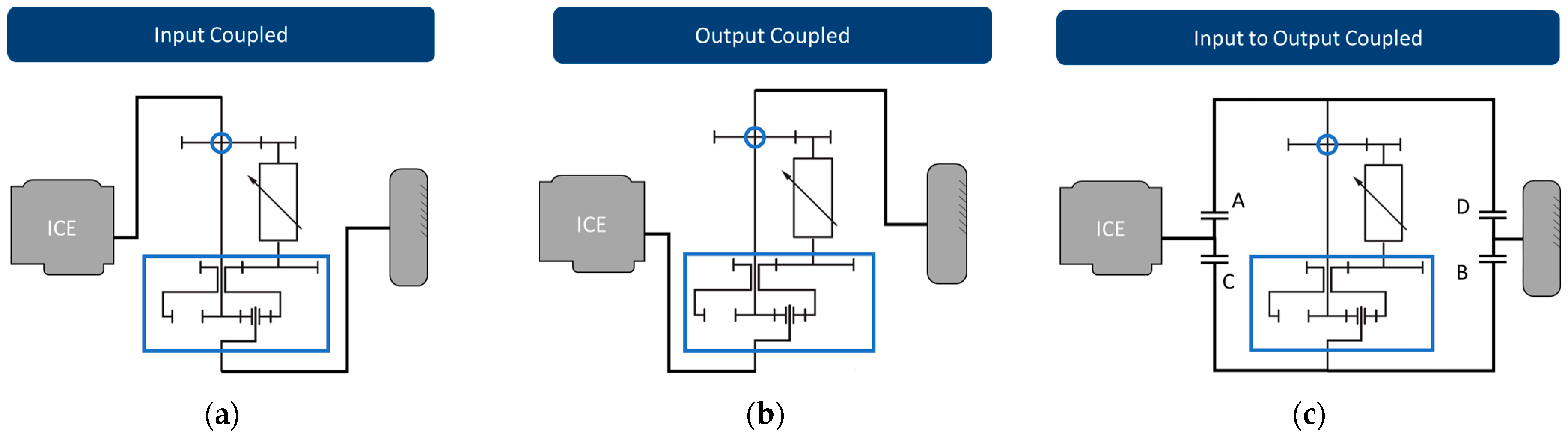
2.1. IC2OC Transmission
2.2. Transmission Design
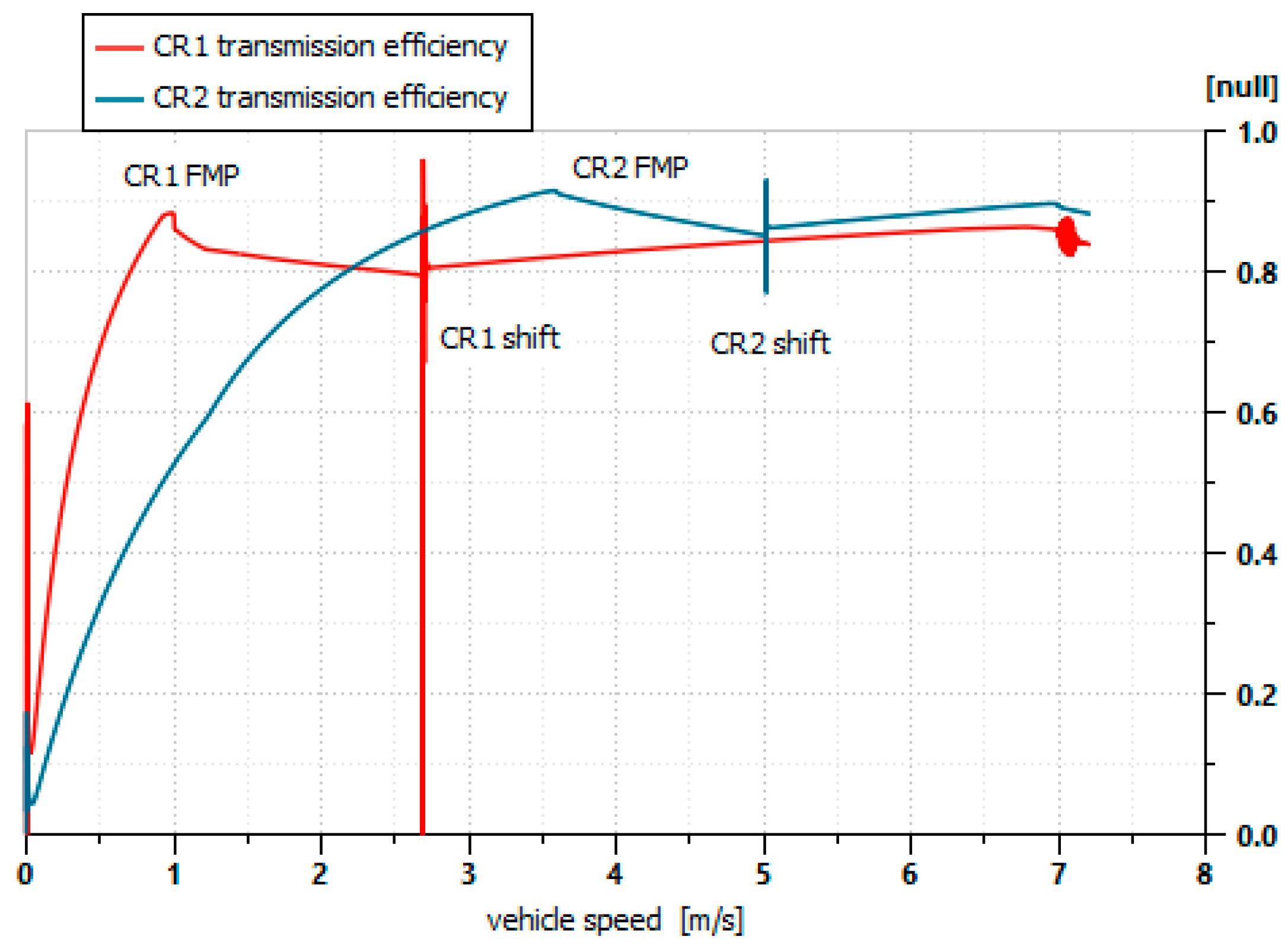
2.2.1. FMP Speeds
- First criterion
- Second criterion
2.2.2. Functional Approach
- Vehicle still, maximum pulling force, IC mode;
- Shifting speed, maximum power, OC mode.
2.2.3. Optimal Approach
- engine maximum power and speed,
- maximum vehicle speed,
- maximum wheel pulling force,
- wheel radius.
- maximum pressure of hydraulic machines,
- maximum speed of hydraulic machines as a function of displacement.
2.3. Case Study
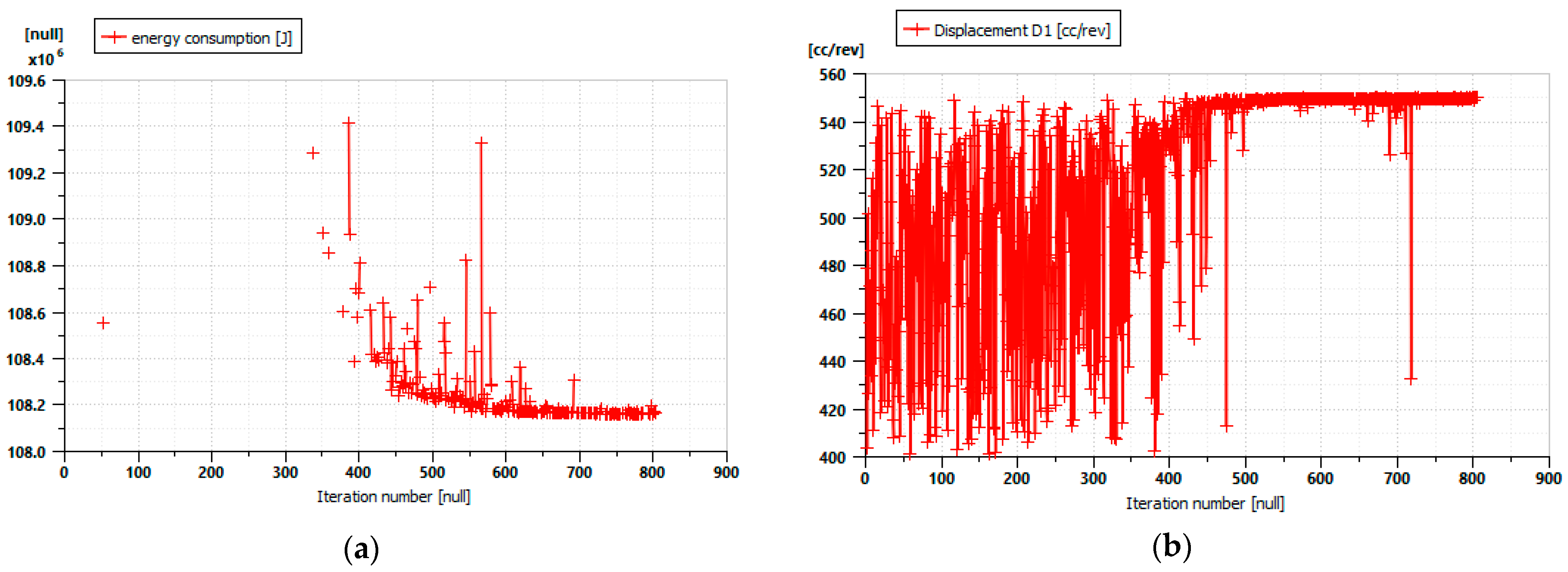
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Jarchow, F. Leistungsverzweigte Getriebe (Power Split Transmissions). VDI-Z 1964, 106, 196–205. [Google Scholar]
- Kress, J.H. Hydrostatic Power-Splitting Transmissions for Wheeled Vehicles. Classification and Theory of Operation; SAE Paper No. 680549; SAE International: Warrendale, PA, USA, 1968. [Google Scholar]
- Renius, K.T.; Resch, R. Continuously Variable Tractor Transmissions; ASAE Distinguished Lecture Series Tractor Design. 29; American Society of Agricultrual Engineers: St. Joseph, MI, USA, 2005. [Google Scholar]
- Blake, C.; Ivantysynova, M.; Williams, K. Comparison of operational characteristics in power split continuously variable transmissions. In Proceedings of the SAE 2006 Commercial Vehicle Engineering Congress & Exhibition, Rosemont/Chicago, IL, USA, 31 October–2 November 2006. [Google Scholar]
- Linares, P.; Mendez, V.; Catalan, H. Design parameters for continuously variable power-split transmissions using planetaries with 3 active shafts. J. Terramechanics 2010, 47, 323–335. [Google Scholar] [CrossRef]
- Casoli, P.; Vacca, A.; Berta, G.; Meleti, S.; Vescovini, M. A numerical model for the simulation of Diesel/CVT power split transmission. In Proceedings of the SAE-NA ICE2007, Napoli, Italy, 17–20 September 2007; SAE Paper n. 2007-24-137. SAE International: Warrendale, PA, USA, 2007. ISBN 978-88-900399-3-0. [Google Scholar] [CrossRef]
- Xiong, S.; Wilfong, G.; Lumkes, J., Jr. Components sizing, and performance analysis of hydro-mechanical power split transmission applied to a wheel loader. Energies 2019, 12, 1613. [Google Scholar] [CrossRef]
- Wan, L.; Dai, H.; Zeng, Q.; Sun, Z.; Tian, M. Characteristic Analysis and Co-Validation of Hydro-Mechanical Continuously Variable Transmission Based on the Wheel Loader. Appl. Sci. 2020, 10, 5900. [Google Scholar] [CrossRef]
- Wei, W.; Junlin, L.; Chunhui, W.; Hui, L.; Shihua, Y. Design and control of a hydro-mechanical transmission for all-terrain vehicle. Mech. Mach. Theory 2020, 154, 104052. [Google Scholar] [CrossRef]
- Pettersson, K.; Krus, K. Design Optimization of Complex Hydro-mechanical Transmissions. J. Mech. Des. 2013, 135, 091005. [Google Scholar] [CrossRef]
- Xia, X.; Sun, D. Characteristic analysis on a new hydro-mechanical continuously variable transmission system. Mech. Mach. Theory 2018, 126, 457–467. [Google Scholar] [CrossRef]
- Xia, Y.; Sun, D.; Qin, D.; Zhou, X. Optimisation of the power-cycle hydro-mechanical parameters in a continuously variable transmission designed for agricultural tractors. Biosyst. Eng. 2020, 193, 12–24. [Google Scholar] [CrossRef]
- Liu, F.X.; Wu, W.; Hu, J.B.; Yuan, S.H. Design of multi-range hydro-mechanical transmission using modular method. Mech. Syst. Signal. Proc. 2019, 126, 1–20. [Google Scholar] [CrossRef]
- Guo, Z.; Sun, D.; Xu, L.; Xu, R. Research on Continuous Power Shift Process of Hydro-Mechanical Continuously Variable Transmission. IOP Conf. Ser. Mater. Sci. Eng. 2020, 790, 012172. [Google Scholar] [CrossRef]
- Zhao, Y.; Chen, X.; Song, Y.; Wang, G.; Zhai, Z. Energy and Fuel Consumption of a New Concept of Hydro-Mechanical Tractor Transmission. Sustainability 2023, 15, 10809. [Google Scholar] [CrossRef]
- Rossetti, A.; Macor, A. Continuous formulation of the layout of a hydromechanical transmission. Mech. Mach. Theory 2019, 133, 545–558. [Google Scholar] [CrossRef]
- Ahn, S.; Choi, J.; Kim, S.; Lee, J.; Choi, C.; Kim, H. Development of an integrated engine-hydro-mechanical transmission control algorithm for a tractor. Adv. Mech. Eng. 2015, 7, 1687814015593870. [Google Scholar] [CrossRef]
- Rossetti, A.; Andretta, N.; Macor, A. On the use of the Disability-adjusted Life Year (DALY) estimator as a metric to optimally manage ICE emissions. Energies 2022, 15, 4386. [Google Scholar] [CrossRef]
- Zhu, Z.; Yang, Y.; Wang, D.; Cai, Y.; Lai, L. Energy Saving Performance of Agricultural Tractor Equipped with Mechanic-Electronic-Hydraulic Powertrain. Syst. Agric. 2022, 12, 436. [Google Scholar] [CrossRef]
- Ramdan, M.I.; Stelson, K.A. Optimal design of a power-split hybrid hydraulic bus. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2016, 230, 1699–1718. [Google Scholar] [CrossRef]
- Andretta, N. Design Methods for Hybrid Hydro-Mechanical Transmissions of Heavy-Duty Vehicles. Ph.D. Thesis, Padua University, Padova, Italy, 2022. [Google Scholar]
- Mercati, S.; Panizzolo, F.; Profumo, G. Power split hydro-mechanical variable transmission (HVT) for off-highway application. In Proceedings of the 10th International Fluid Power Conference, Dresden, Germany, 8–10 March 2016. [Google Scholar]
- Legner, J.; Rebholz, W.; Morrison, R. ZF cPower—Hydrostatic-Mechanical Powersplit Transmission for Construction and Forest Machinery. In Proceedings of the 10th International Fluid Power Conference, Dresden, Germany, 8–10 March 2016. [Google Scholar]
- Rossetti, A.; Andretta, N.; Macor, A. Study of a dual layout Input-to-Output Coupled hydromechanical transmission. In Proceedings of the 2022 IEEE Global Fluid Power Society—PhD Symposium, Napoli, Italy, 12–14 October 2022. [Google Scholar]
- Rossetti, A.; Andretta, N.; Macor, A. Design considerations about the hydromechanical transmission IC2OC. J. Phys. Conf. Ser. 2023, 2648, 012054. [Google Scholar] [CrossRef]
- AMESim; Simcenter Amesim Version 2210; Siemens Industry Software NV: Plano, TX, USA, 2021.



| Engine Data | Unit | |
|---|---|---|
| Engine model | TAD-1150-VE | - |
| Max power @ speed | 235 @ 1450–2000 | kW@rpm |
| Max torque @ speed | 1581 @ 950–1450 | Nm@rpm |
| Vehicle Data | ||
| Maximum Load | 112,400 | kg |
| Wheel radius | 0.825 | m |
| Maximum Torque at Wheel | 128,000 | Nm |
| Maximum Vehicle Speed | 26 | km/h |
| Gradeability at rated load | 17 | % |
| CR1 | CR2 | ||||
| nICE | [rpm] | 2000 | 2000 | ||
| nHymax1, nHymax2 | [rpm] | 2100/2900 | 2100/2900 | ||
| Δpmax | [bar] | 465 | 465 | ||
| Vmax | [km/h] | 26 | 26 | ||
| VfmpIC | [km/h] | 3.2 | 13 | ||
| VfmpOC | [km/h] | 26 | 26 | ||
| Vshift | [km/h] | 9 | 18 | ||
| τ0 | / | −1/1.65 | −1/0.4 | ||
| τout | / | 63 | 34 | ||
| functional | optimal | functional | optimal | ||
| D1 | [cm3] | 428 | 550 | 519 | 550 |
| D2 | [cm3] | 115 | 296 | 261 | 471 |
| τ1 | / | 0.45 | 0.38 | 0.73 | 0.63 |
| τ2 | / | 1.51 | 0.63 | 0.572 | 0.31 |
| CR1 | CR2 | Δ CR2-CR1 | |
|---|---|---|---|
| Functional | 53.1 | 59.24 | +11.6% |
| Optimal | 53.06 | 57.41 | +8.2% |
| Δ Optimal--Functional | −0.1% | −3.1% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Andretta, N.; Rossetti, A.; Macor, A. Hydromechanical Transmission IC2OC: Component Sizing and Optimization. Fluids 2024, 9, 73. https://doi.org/10.3390/fluids9030073
Andretta N, Rossetti A, Macor A. Hydromechanical Transmission IC2OC: Component Sizing and Optimization. Fluids. 2024; 9(3):73. https://doi.org/10.3390/fluids9030073
Chicago/Turabian StyleAndretta, Nicola, Antonio Rossetti, and Alarico Macor. 2024. "Hydromechanical Transmission IC2OC: Component Sizing and Optimization" Fluids 9, no. 3: 73. https://doi.org/10.3390/fluids9030073
APA StyleAndretta, N., Rossetti, A., & Macor, A. (2024). Hydromechanical Transmission IC2OC: Component Sizing and Optimization. Fluids, 9(3), 73. https://doi.org/10.3390/fluids9030073









