Numerical Simulations of Impact River Morphology Evolution Mechanism Under the Influence of Floodplain Vegetation
Abstract
1. Introduction
2. Methodology and Model Validation
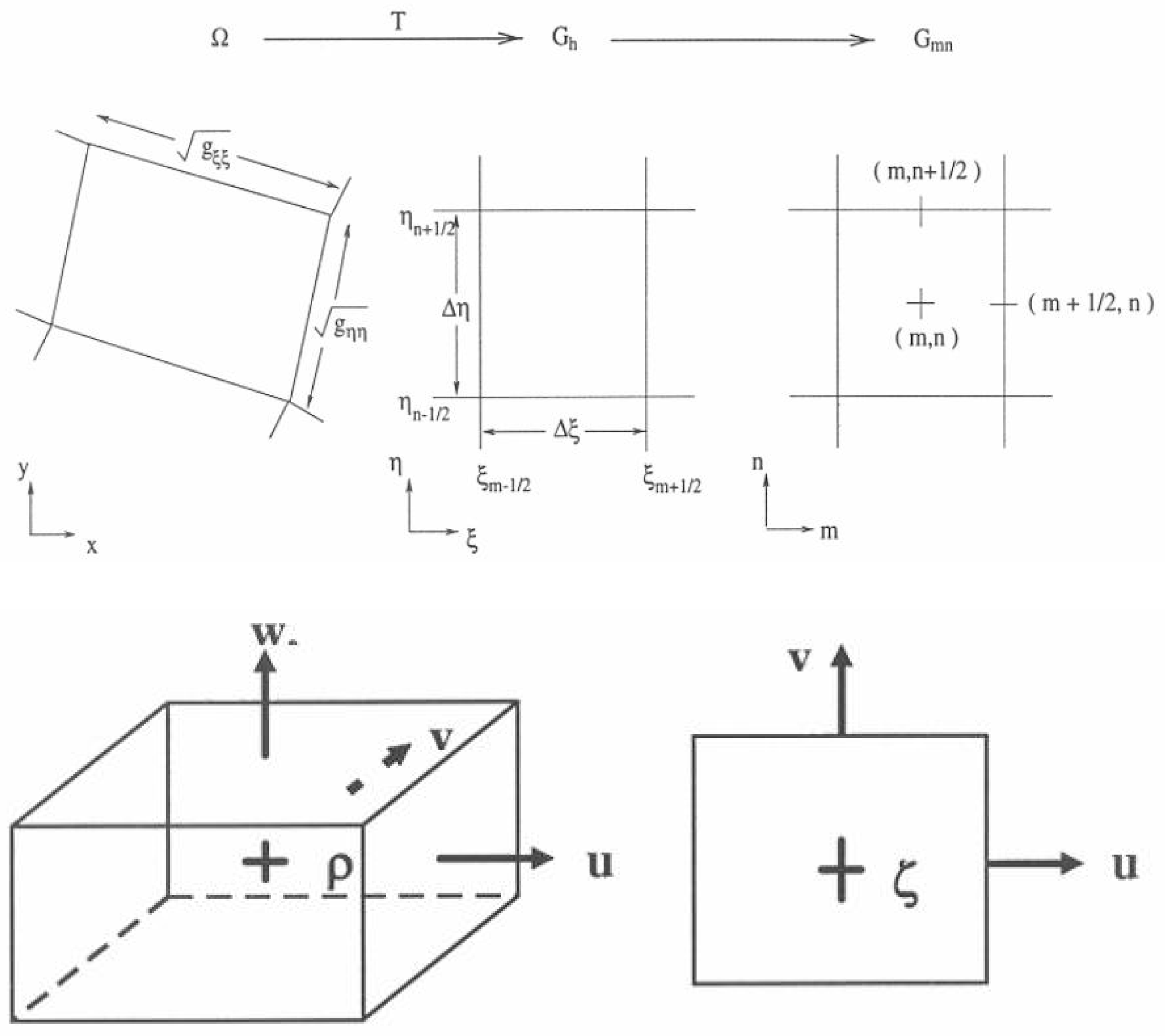
2.1. Numerical Methods
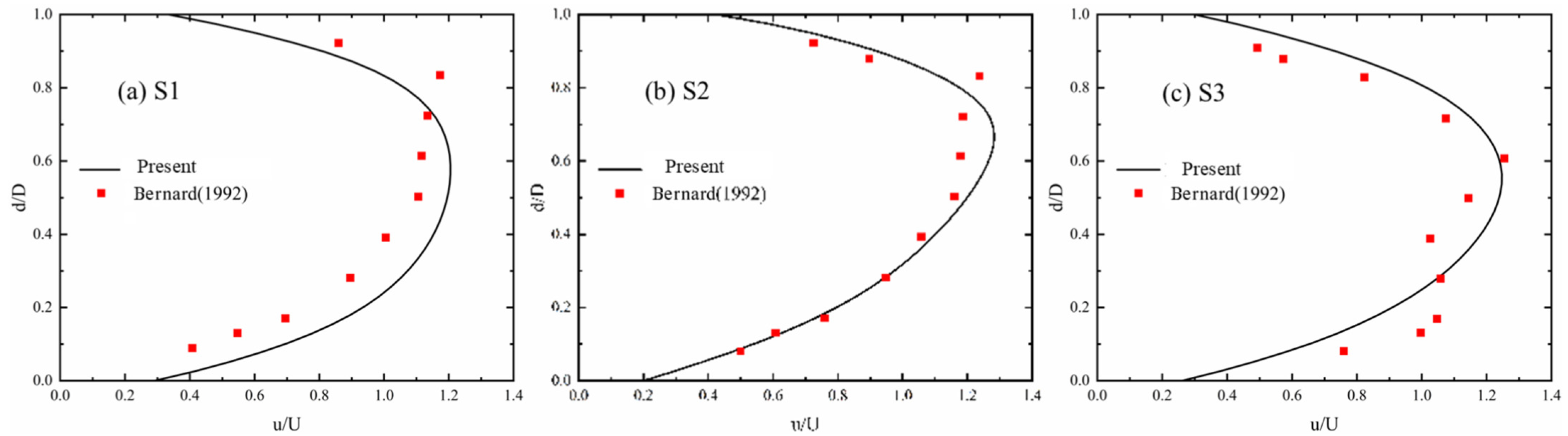
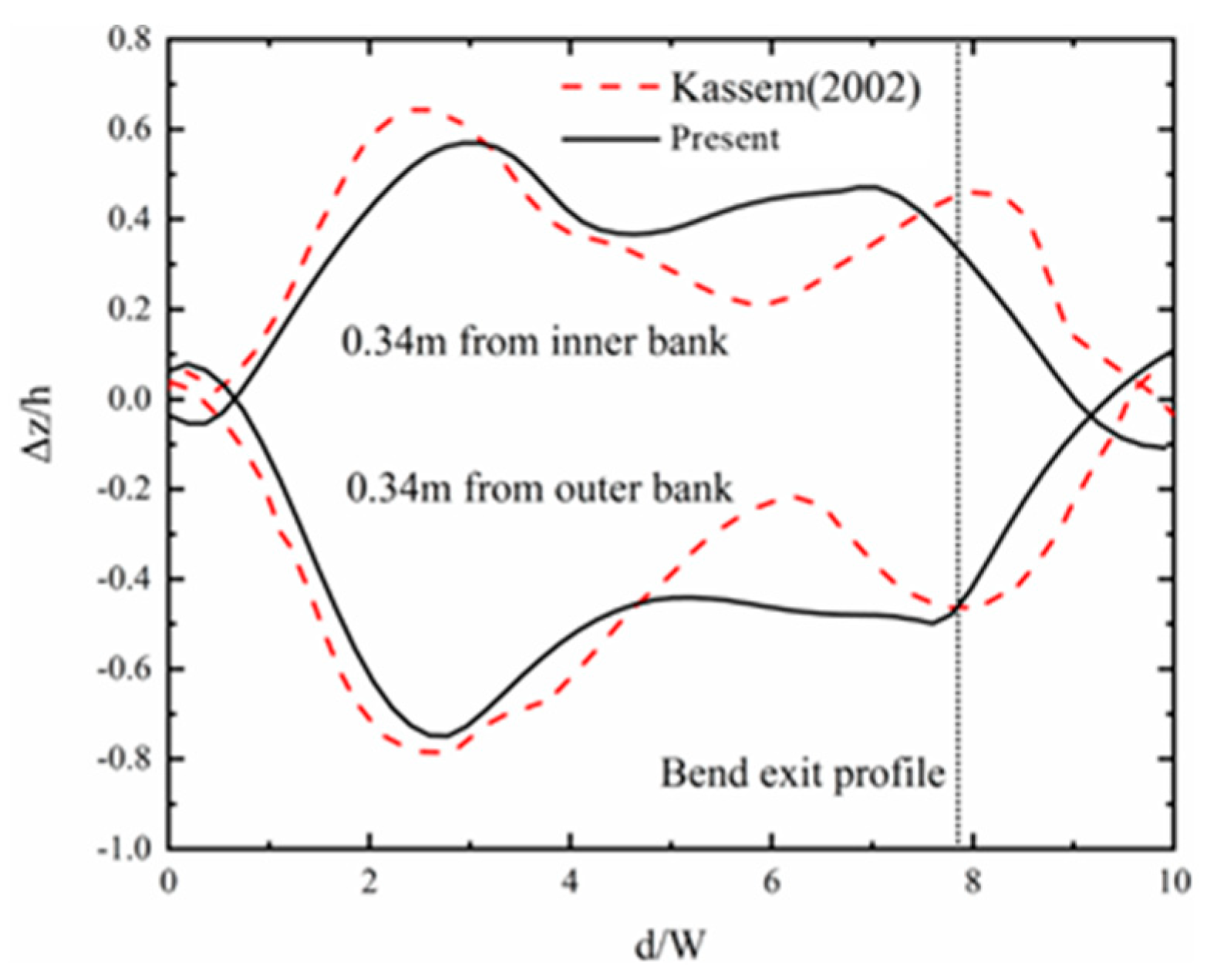
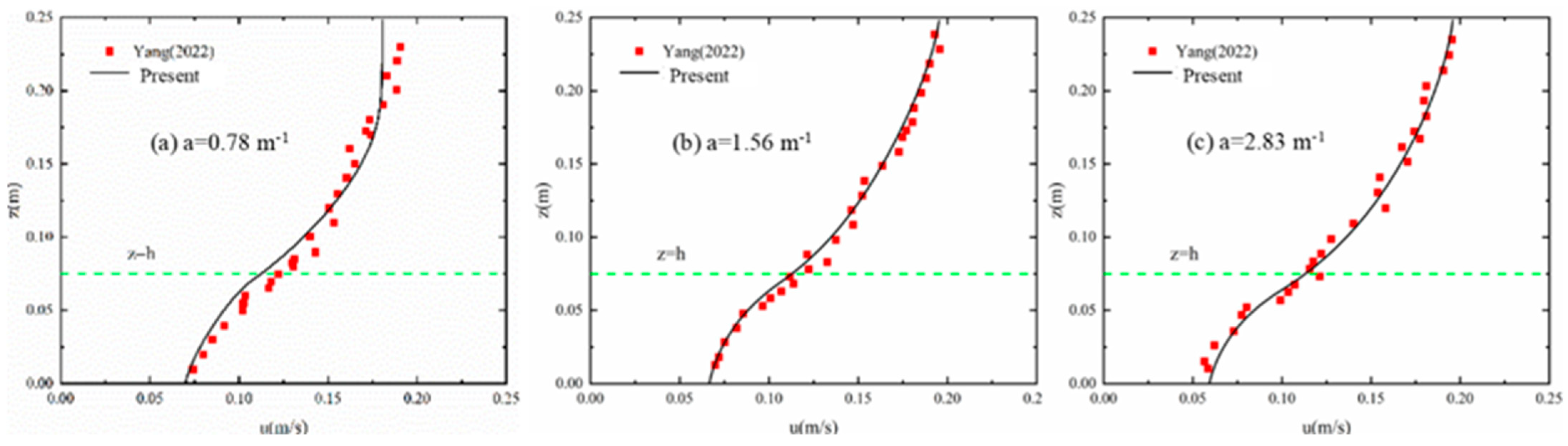
2.2. Model Validation
2.3. Simulation Settings
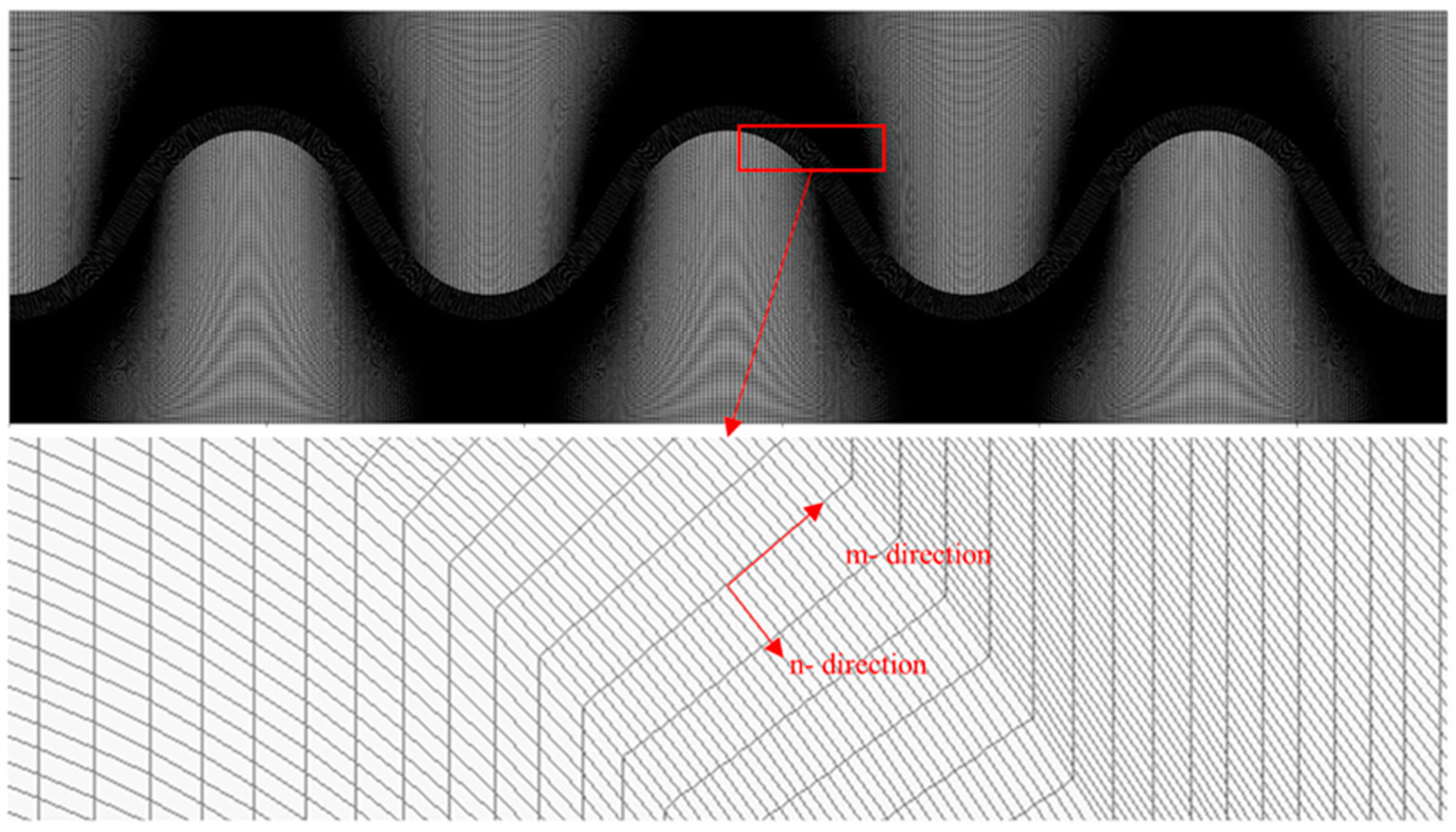
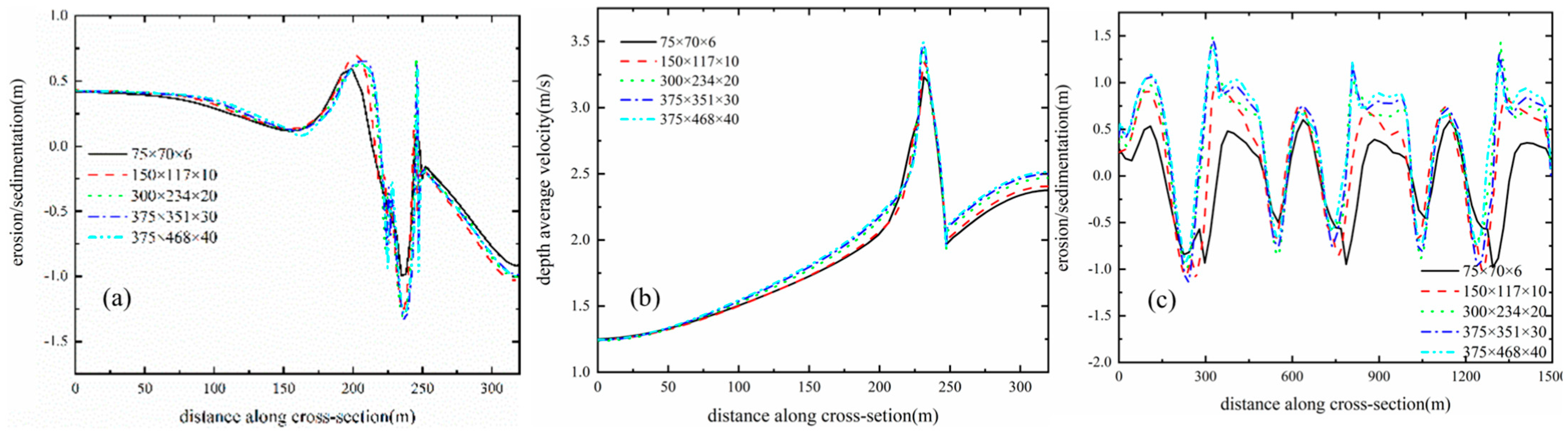
2.4. Mesh Convergence Study
3. Results and Discussions
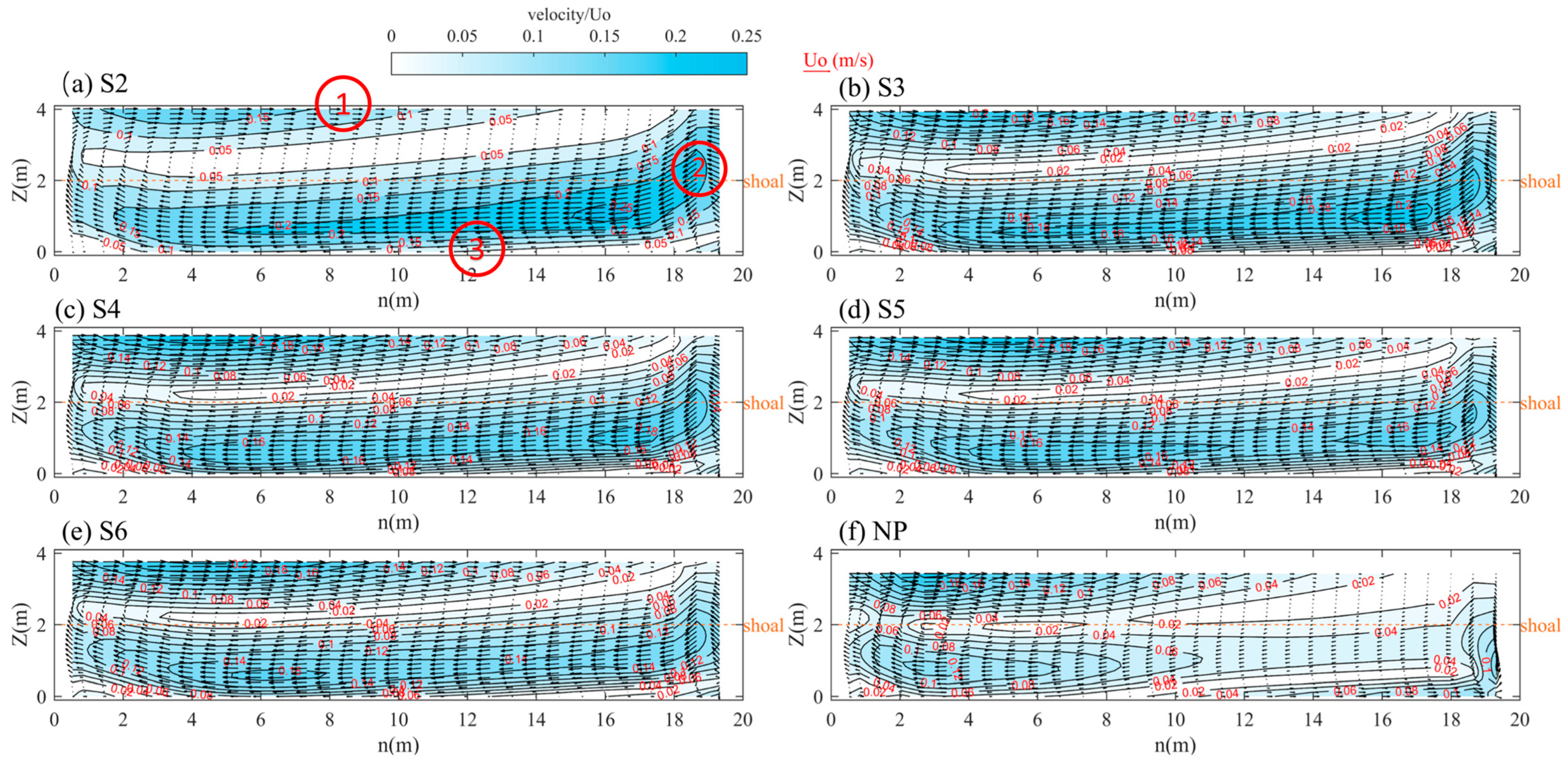
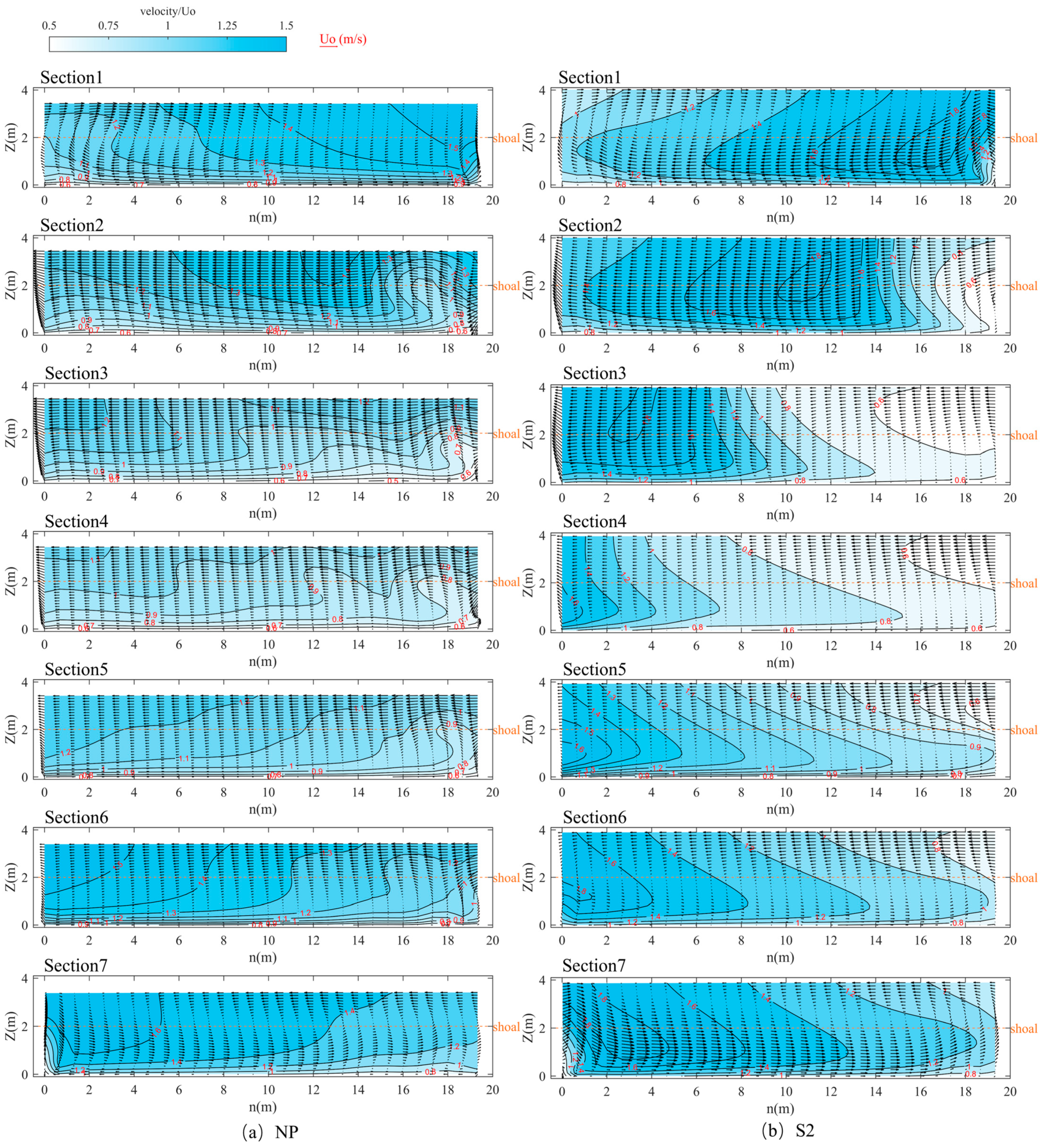
3.1. Flow Structure
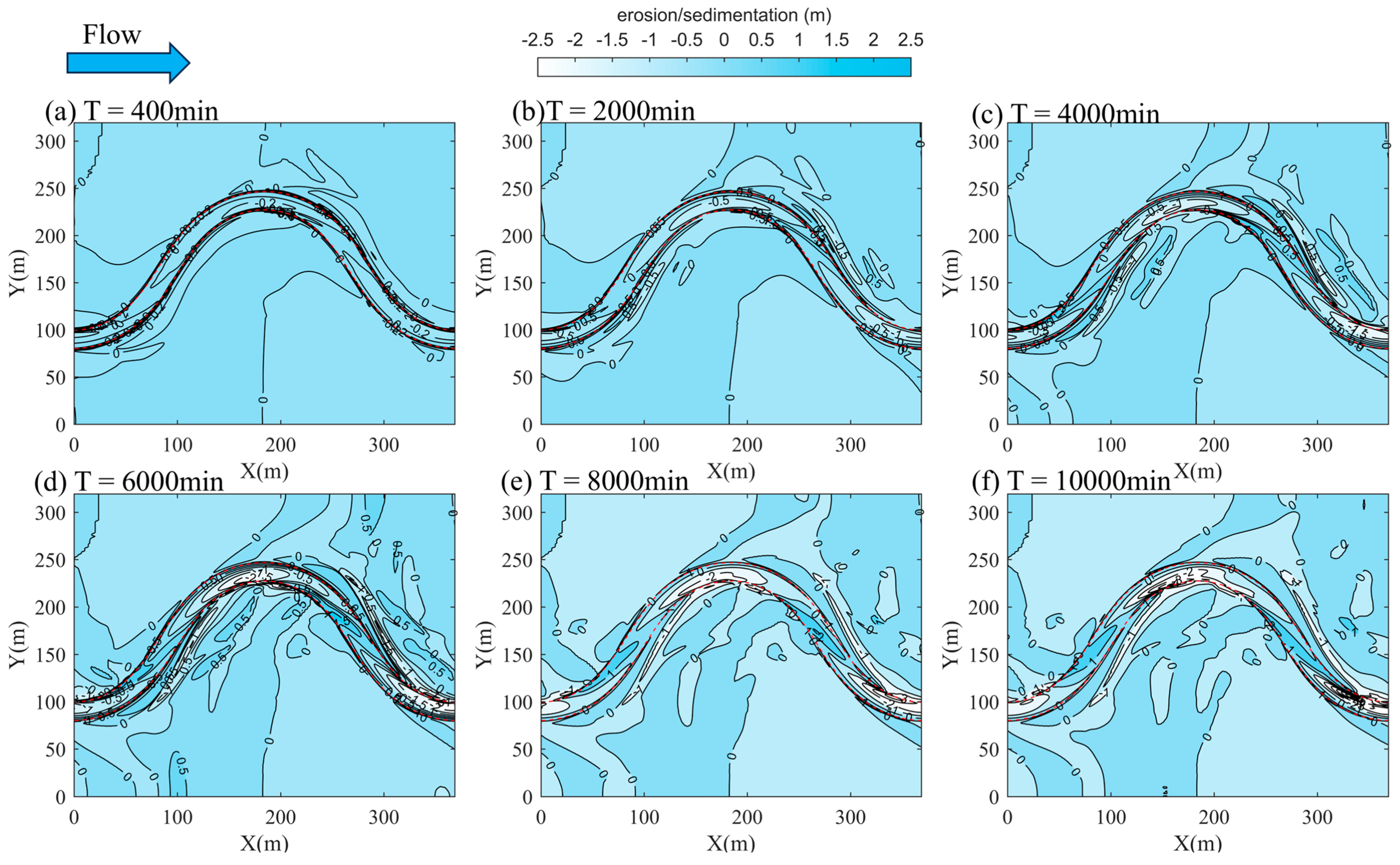
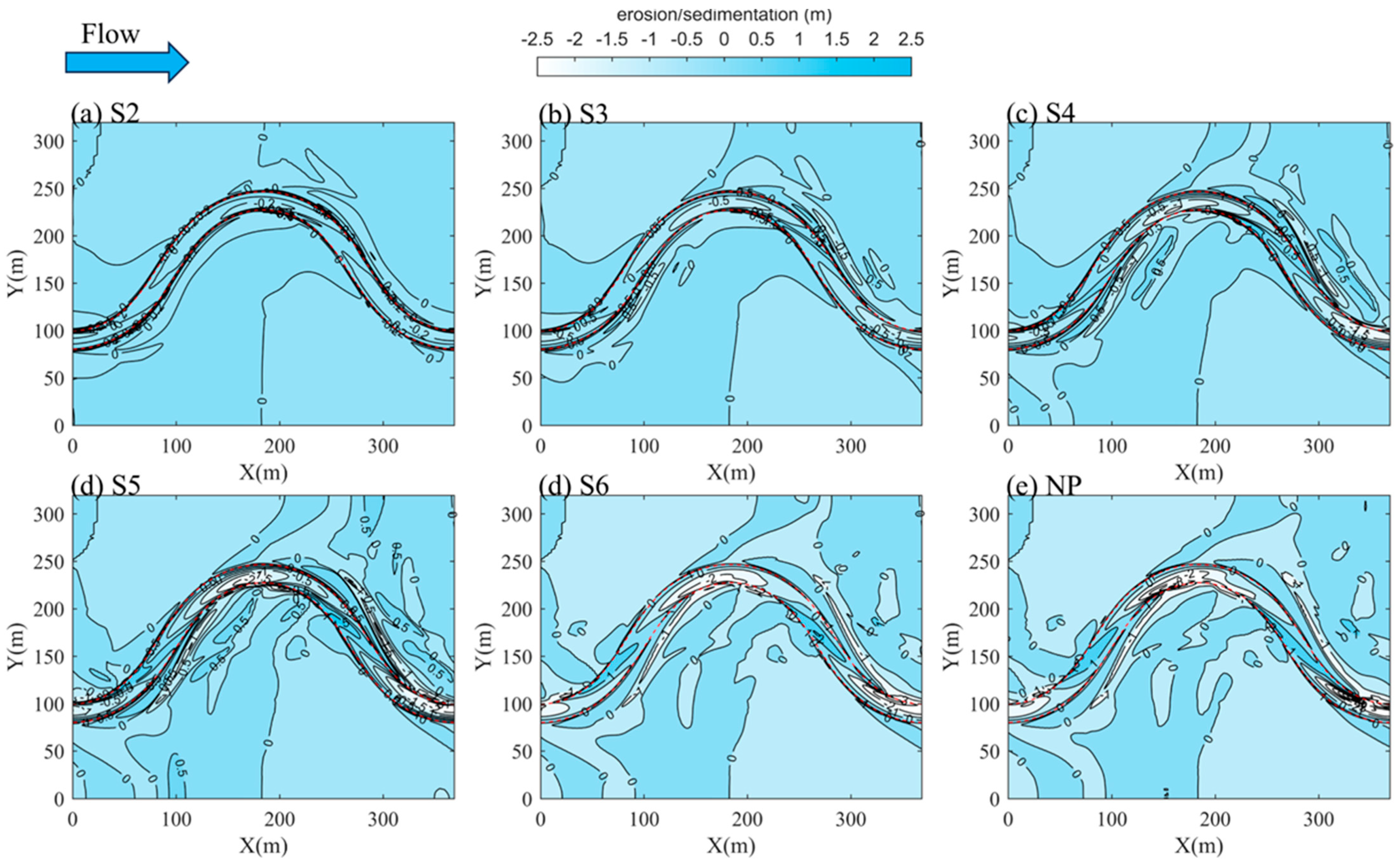
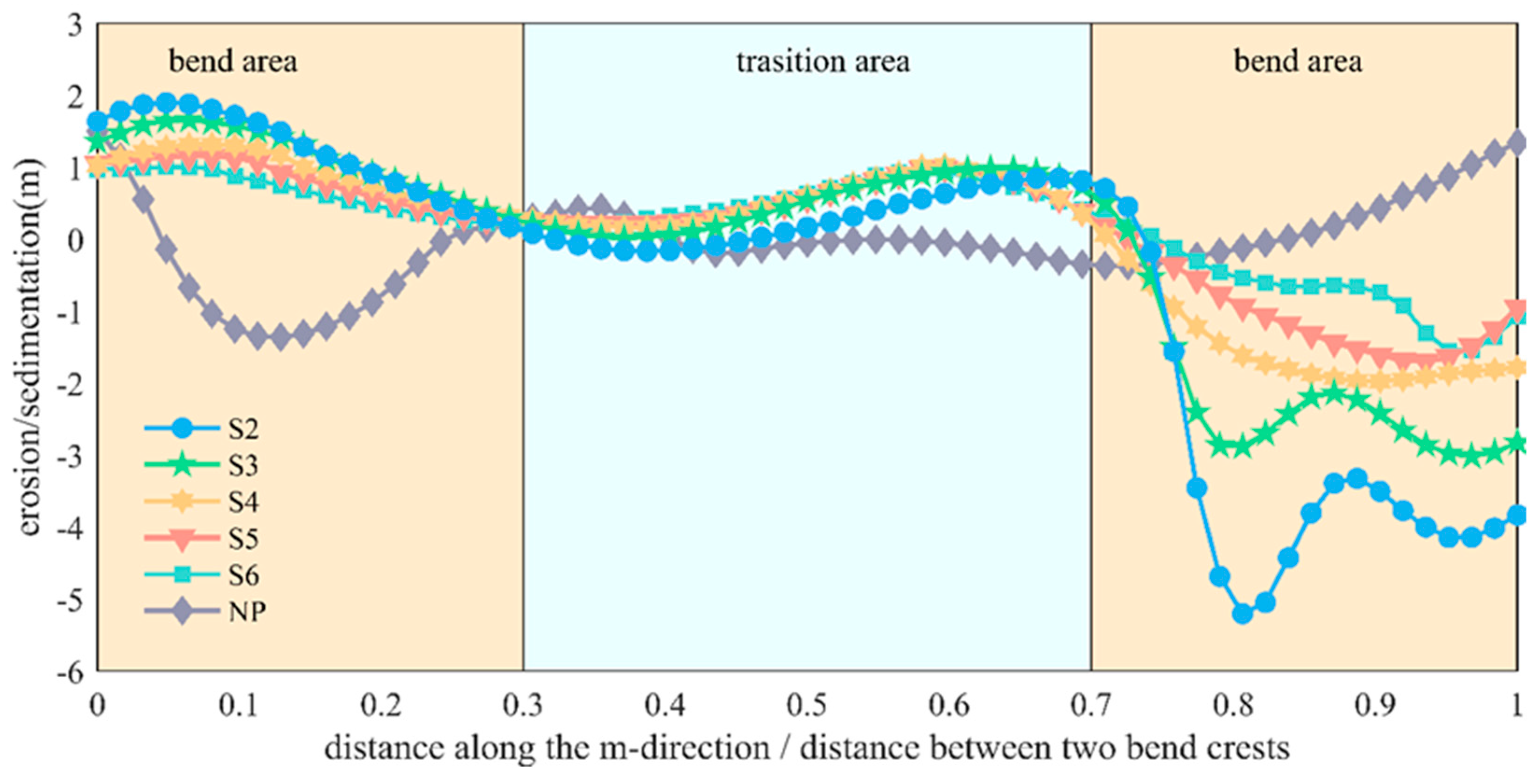
3.2. River Channel Evolution
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| the maximum bend angle of the river meander curve | |
| unit water-facing area of the vegetation [m−1] | |
| the solid volume fraction | |
| the diameter of the vegetation element [m] | |
| the average spacing of the vegetation element [m2] | |
| the drag force coefficient of the water flow under the action of vegetation | |
| the strength of the circulation | |
| the average flow velocity in the river | |
| the main channel width [m] |
References
- Ali, S.Z.; Dey, S. Hydrodynamic instability of meandering channels. Phys. Fluids 2017, 29, 125107. [Google Scholar] [CrossRef]
- Engel, F.L.; Rhoads, B.L. Three-dimensional flow structure and patterns of bed shear stress in an evolving compound meander bend. Earth Surf. Process. Landf. 2016, 41, 1211–1226. [Google Scholar] [CrossRef]
- Li, J.; Zhang, M.; Jiang, E.; Pan, L.; Wang, A.; Wang, Y.; Jian, S. Influence of floodplain flooding on channel siltation adjustment under the effect of vegetation on a meandering riverine beach. Water 2021, 13, 1402. [Google Scholar] [CrossRef]
- Chatelain, M.; Proust, S. Open-channel flows through emergent rigid vegetation: Effects of bed roughness and shallowness on the flow structure and surface waves. Phys. Fluids 2021, 33, 106602. [Google Scholar] [CrossRef]
- Tominaga, A.; Nezu, I. Turbulent Structure in Compound Open-Channel Flows. J. Hydraul. Eng. 1991, 117, 21–41. [Google Scholar] [CrossRef]
- Wormleaton, P.R.; Allen, J.; Hadjipanos, P. Discharge Assessment in Compound Channel Flow. Am. Soc. Civ. Eng. 1982, 108, 975–994. [Google Scholar] [CrossRef]
- Sanjou, M.; Nezu, I. Turbulence structure and coherent motion in meandering compound open-channel flows. J. Hydraul. Res. 2009, 47, 598–610. [Google Scholar] [CrossRef]
- Zhang, H.T.; Dai, W.H.; da Silva, A.M.F.; Tang, H.-W. Numerical study on resistance to flow in meandering channels. J. Hydraul. Eng. 2022, 148, 04021059. [Google Scholar] [CrossRef]
- Guan, D.; Zhou, Y.; Shahin, M.A.; Tirkolaei, H.K.; Cheng, L. Assessment of urease enzyme extraction for superior and economic bio-cementation of granular materials using enzyme-induced carbonate precipitation. Acta Geotech. 2023, 18, 2263–2279. [Google Scholar] [CrossRef]
- Tang, Z.; Melville, B.; Shamseldin, A.; Guan, D.; Singhal, N.; Yao, Z. Experimental study of collar protection for local scour reduction around offshore wind turbine monopile foundations. Coast. Eng. 2023, 183, 104324. [Google Scholar] [CrossRef]
- Wang, M.; Yu, Q.; Xu, Y.; Li, N.; Wang, J.; Cao, B.; Wang, L.; Avital, E.J. A Numerical Study on the Influence of Riparian Vegetation Patch on the Transportation of Suspended Sediment in a U-Bend Channel Flow. Fluids 2024, 9, 109. [Google Scholar] [CrossRef]
- Yang, K.J.; Liu, X.N.; Cao, S.Y.; Zhang, Z.X. Flow turbulence characteristics of compound river channel with floodplain under vegetation effect. J. Hydraul. Eng. 2005, 36, 1263–1268. (In Chinese) [Google Scholar]
- Zhang, M.W. Study on Flow Characteristics of Compound River Channel with Vegetated Floodplains; Tsinghua University: Beijing, China, 2011. (In Chinese) [Google Scholar]
- Stone, B.M.; Shen, H.T. Hydraulic resistance of flow in channels with cylindrical roughness. J. Hydraul. Eng. 2002, 128, 500–506. [Google Scholar] [CrossRef]
- Stoesser, T.; Kim, S.J.; Diplas, P. Turbulent flow through idealized emergent vegetation. J. Hydraul. Eng. 2010, 136, 1003–1017. [Google Scholar] [CrossRef]
- Rao, P.L.; Prasad, B.S.S.; Sharma, A.; Khatua, K.K. Experimental and numerical analysis of velocity distribution in a compound meandering channel with double layered rigid vegetated flood plains. Flow Meas. Instrum. 2022, 83, 102111. [Google Scholar]
- Lyness, J.F.; Myers WR, C.; O’Sullivan, J.J. Hydraulic characteristics of meandering mobile bed compound channels. Proc. Inst. Civ. Eng. -Water Marit. Energy 1998, 130, 179–188. [Google Scholar] [CrossRef]
- Liu, C.; Shan, Y.; Liu, X.; Yang, K.; Liao, H. The effect of floodplain grass on the flow characteristics of meandering compound channels. J. Hydrol. 2016, 542, 233. [Google Scholar] [CrossRef]
- Wang, Y.; Yang, Z.; Liu, M.; Yu, M. Numerical study of flow characteristics in compound meandering channels with vegetated floodplains. Phys. Fluids 2022, 34, 115107. [Google Scholar] [CrossRef]
- Gran, K.; Paola, C. Riparian vegetation controls on braided stream dynamics. Water Resour. Res. 2001, 37, 3275–3283. [Google Scholar] [CrossRef]
- Inoue, T.; Mishra, J.; Parker, G. Numerical Simulations of Meanders Migrating Laterally as They Incise into Bedrock. J. Geophys. Res. Earth Surf. 2021, 126, e2020JF005645. [Google Scholar] [CrossRef]
- Braudrick, C.A.; Dietrich, W.E.; Leverich, G.T.; Sklar, L.S. Experimental evidence for the conditions necessary to sustain meandering in coarse-bedded rivers. Proc. Natl. Acad. Sci. USA 2001, 106, 16936–16941. [Google Scholar] [CrossRef] [PubMed]
- Tal, M.; Paola, C. Dynamic single-thread channels maintained by the interaction of flow and vegetation. Geology 2007, 35, 347–350. [Google Scholar] [CrossRef]
- Tal, M.; Paola, C. Effects of vegetation on channel morphodynamics: Results and insights from laboratory experiments. Earth Surf. Process. Landf. 2010, 35, 1014–1028. [Google Scholar] [CrossRef]
- Le Bouteiller, C.; Venditti, J.G. Vegetation-driven morphodynamic adjustments of a sand bed. Geophys. Res. Lett. 2014, 41, 3876–3883. [Google Scholar] [CrossRef]
- van Dijk, W.M.; Teske, R.; van de Lageweg, W.I.; Kleinhans, M.G. Effects of vegetation distribution on experimental river channel dynamics. Water Resour. Res. 2013, 49, 7558–7574. [Google Scholar] [CrossRef]
- Vargas-Luna, A.; Duró, G.; Crosato, A.; Uijttewaal, W. Morpho logical adaptation of river channels to vegetation establishment: A lab oratory study. J. Geophys. Res. Earth Surf. 2019, 124, 1981–1995. [Google Scholar] [CrossRef]
- Fernandez, R.L.; McLelland, S.; Parsons, D.R.; Bodewes, B. Riparian vegetation life stages control the impact of flood sequencing on braided river morphodynamics. Earth Surf. Process. Landforms 2021, 46, 2315–2329. [Google Scholar] [CrossRef]
- Kyuka, T.; Yamaguchi, S.; Inoue, Y.; Ferrel, K.R.A.; Kon, H.; Shimizu, Y. Morphodynamic effects of vegetation life stage on experimental meandering channels. Earth Surf. Process. Landforms 2021, 46, 1225–1237. [Google Scholar] [CrossRef]
- Wang, W.J.; Peng, W.Q.; Huai, W.X.; Katul, G.G.; Liu, X.-B.; Qu, X.-D.; Dong, F. Friction factor for turbulent open Channel flow covered by vegetation. Sci. Rep. 2019, 9, 5178. [Google Scholar] [CrossRef]
- Bernard, R.S.; Schneider, M.L. Depth-Averaged Numerical Modeling for Curved Channels; USAE Waterways Experiment Station, Hydraulics Laboratory: Warren County, MS, USA, 1992.
- Kassem, A.A.; Chaudhry, M.H. Numerical Modeling of Bed Evolution in Channel Bends. J. Hydraul. Eng. 2002, 128, 507–514. [Google Scholar] [CrossRef]
- Yang, Z.H.; Guo, M.; Li, D. Theoretical model of suspended sediment transport capacity in submerged vegetation flow. J. Hydrol. 2022, 609, 127761. [Google Scholar] [CrossRef]
- Kinoshita. Investigation of Channel Deformation in Ishikari River. In Report of Bureau of Resources; Department of Science and Technology: Tokyo, Japan, 1961. [Google Scholar]
- Ghisalberti, M.; Nepf, H.M. Mixing layers and coherent structures in vegetated aquatic flows. J. Geophys. Res. Ocean. 2002, 107, 3-1–3-11. [Google Scholar] [CrossRef]
- Langbein, W.B.; Leopold, L.B. River Meanders—Theory of Minimum Variance. In Rivers and River Terraces; U.S. Geological Survey Professional Paper; Palgrave Macmillan: London, UK, 1966; pp. H1–H14. [Google Scholar]
- Niu, C.; Li, D.; Zhao, L.; Han, S.; Quan, L.; Li, X.; Hu, C. Analysis of the Evolution Law and Influencing Factors of River Bed Roughness in the Wide Beach Area of the Lower Yellow River in the Past 20 Years. J. Appl. Basic Eng. Sci. 2024, 32, 984–999. (In Chinese) [Google Scholar] [CrossRef]
- Tinoco, R.O.; Coco, G. A laboratory study on sediment resuspension within arrays of rigid cylinders. Adv. Water Resour. 2016, 92, 1–9. [Google Scholar] [CrossRef]
- Shukry, A. Flow around bends in an open flume. Trans. Am. Soc. Civ. Eng. 1950, 115, 751–779. [Google Scholar] [CrossRef]
- Moncho-Esteve, I.J.; Folke, F.; García-Villalba, M.; Palau-Salvador, G. Influence of the secondary motions on pollutant mixing in a meandering open channel flow. Environ. Fluid Mech. 2017, 17, 695–714. [Google Scholar] [CrossRef]



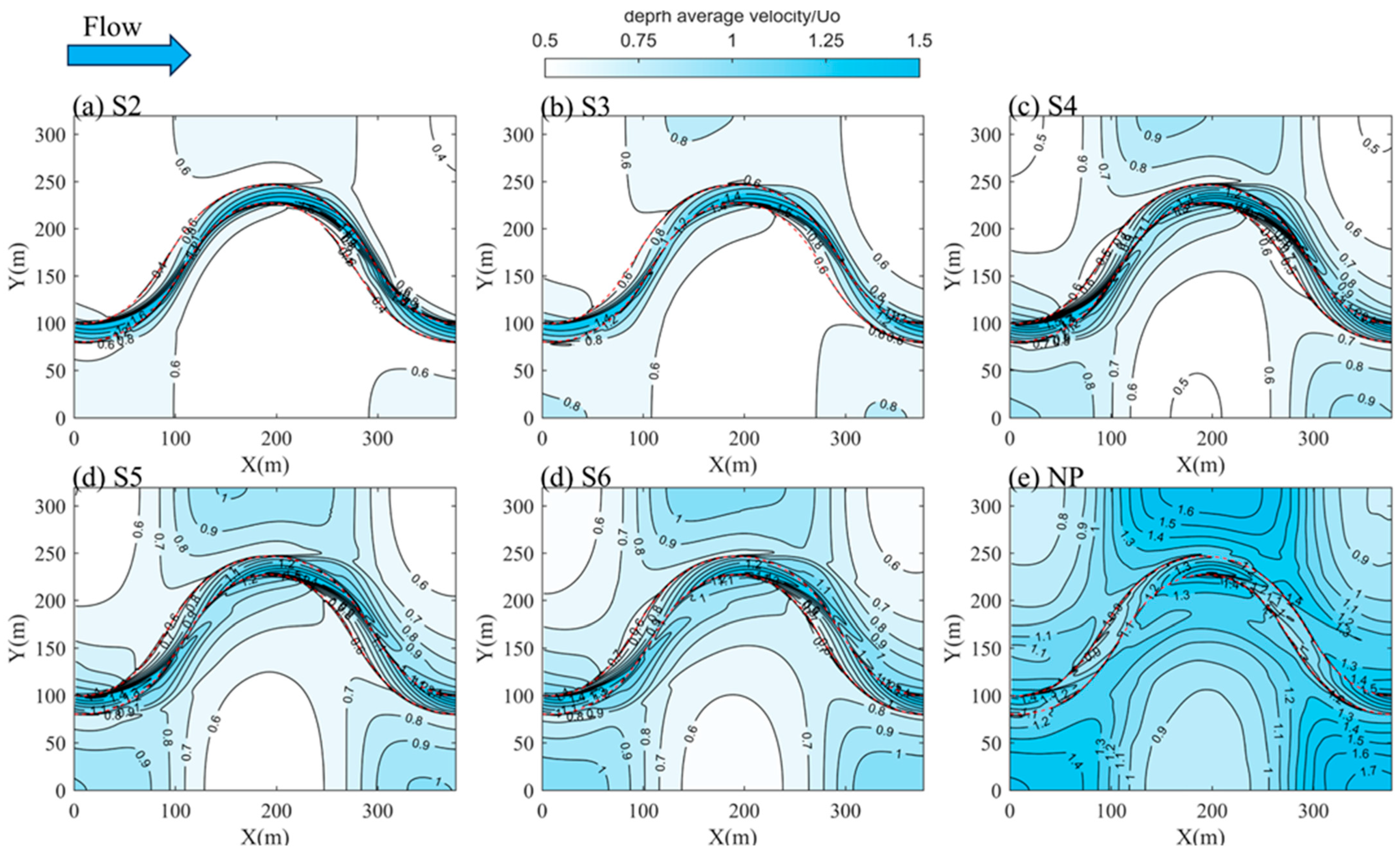
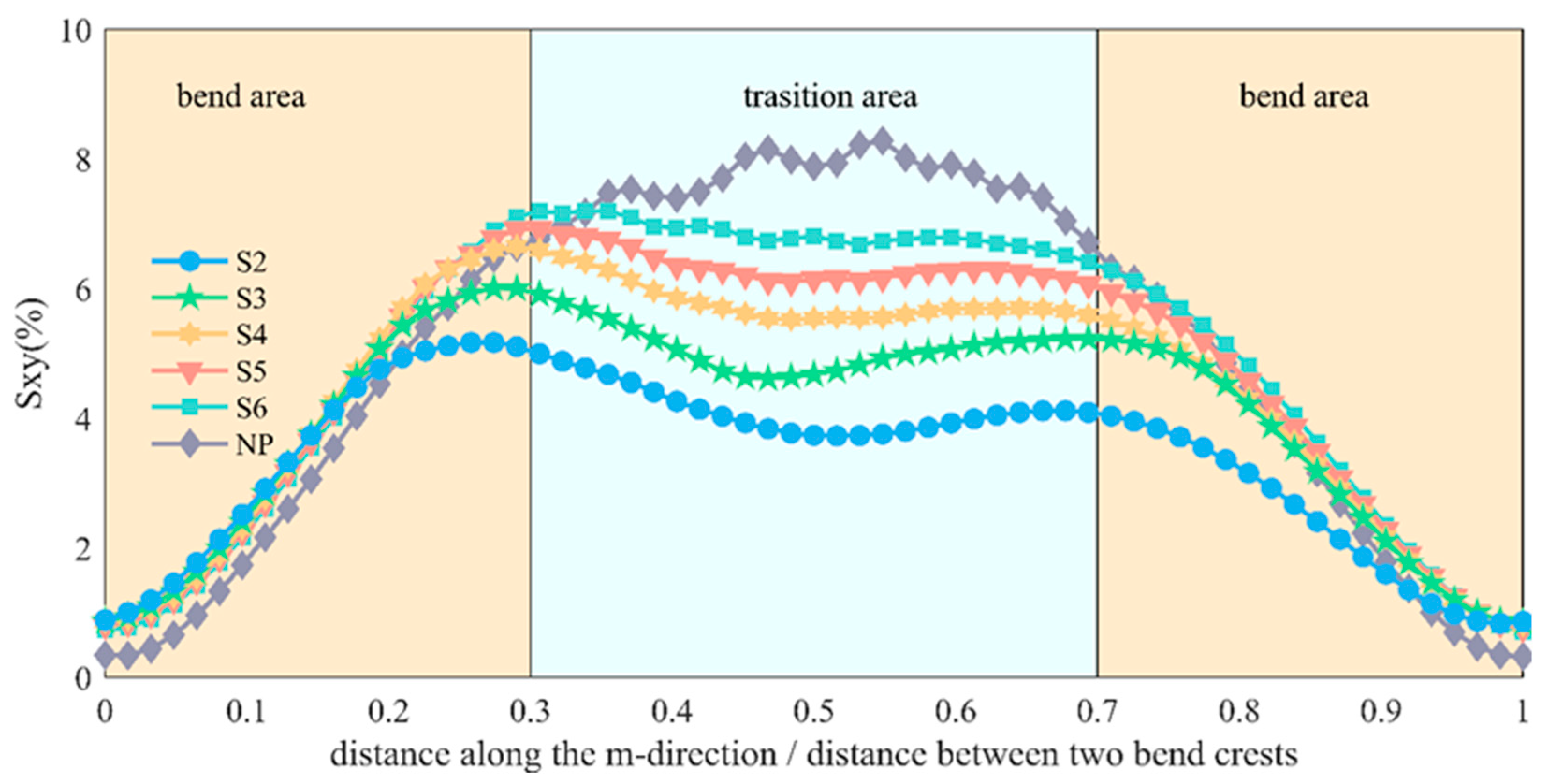





| Case Number | ) | Vegetation Distribution | |||
|---|---|---|---|---|---|
| NP | π/3 | None | \ | 0.00 | \ |
| S6 | Both Sides | 0.6 | 0.10 | 0.82239 | |
| S5 | Both Sides | 0.5 | 0.14 | 0.82307 | |
| S4 | Both Sides | 0.4 | 0.21 | 0.82410 | |
| S3 | Both Sides | 0.3 | 0.40 | 0.82585 | |
| S2 | Both Sides | 0.2 | 0.90 | 0.82949 |
| Grid | m-Grid Number | n-Grid Number | z-Grid Number | Total Grids |
|---|---|---|---|---|
| G1 | 75 | 70 | 6 | 31,500 |
| G2 | 150 | 117 | 10 | 175,500 |
| G3 | 300 | 234 | 20 | 1,404,000 |
| G4 | 375 | 351 | 30 | 3,948,750 |
| G5 | 375 | 408 | 40 | 6,120,000 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xiang, H.; Zhang, Z.; Ji, C.; Xu, D.; Chen, X.; Tang, L.; Wang, Y. Numerical Simulations of Impact River Morphology Evolution Mechanism Under the Influence of Floodplain Vegetation. Fluids 2024, 9, 243. https://doi.org/10.3390/fluids9100243
Xiang H, Zhang Z, Ji C, Xu D, Chen X, Tang L, Wang Y. Numerical Simulations of Impact River Morphology Evolution Mechanism Under the Influence of Floodplain Vegetation. Fluids. 2024; 9(10):243. https://doi.org/10.3390/fluids9100243
Chicago/Turabian StyleXiang, Heng, Zhimeng Zhang, Chunning Ji, Dong Xu, Xincong Chen, Lian Tang, and Yuelei Wang. 2024. "Numerical Simulations of Impact River Morphology Evolution Mechanism Under the Influence of Floodplain Vegetation" Fluids 9, no. 10: 243. https://doi.org/10.3390/fluids9100243
APA StyleXiang, H., Zhang, Z., Ji, C., Xu, D., Chen, X., Tang, L., & Wang, Y. (2024). Numerical Simulations of Impact River Morphology Evolution Mechanism Under the Influence of Floodplain Vegetation. Fluids, 9(10), 243. https://doi.org/10.3390/fluids9100243








