Experimental Analysis of a Cracked Cardan Shaft System under the Influence of Viscous Hydrodynamic Forces
Abstract
:1. Introduction
2. Experimental Description of the Coupled Shaft System
2.1. Description of the Cardan Shaft Setup
2.2. Measurement and Structural Adjustments
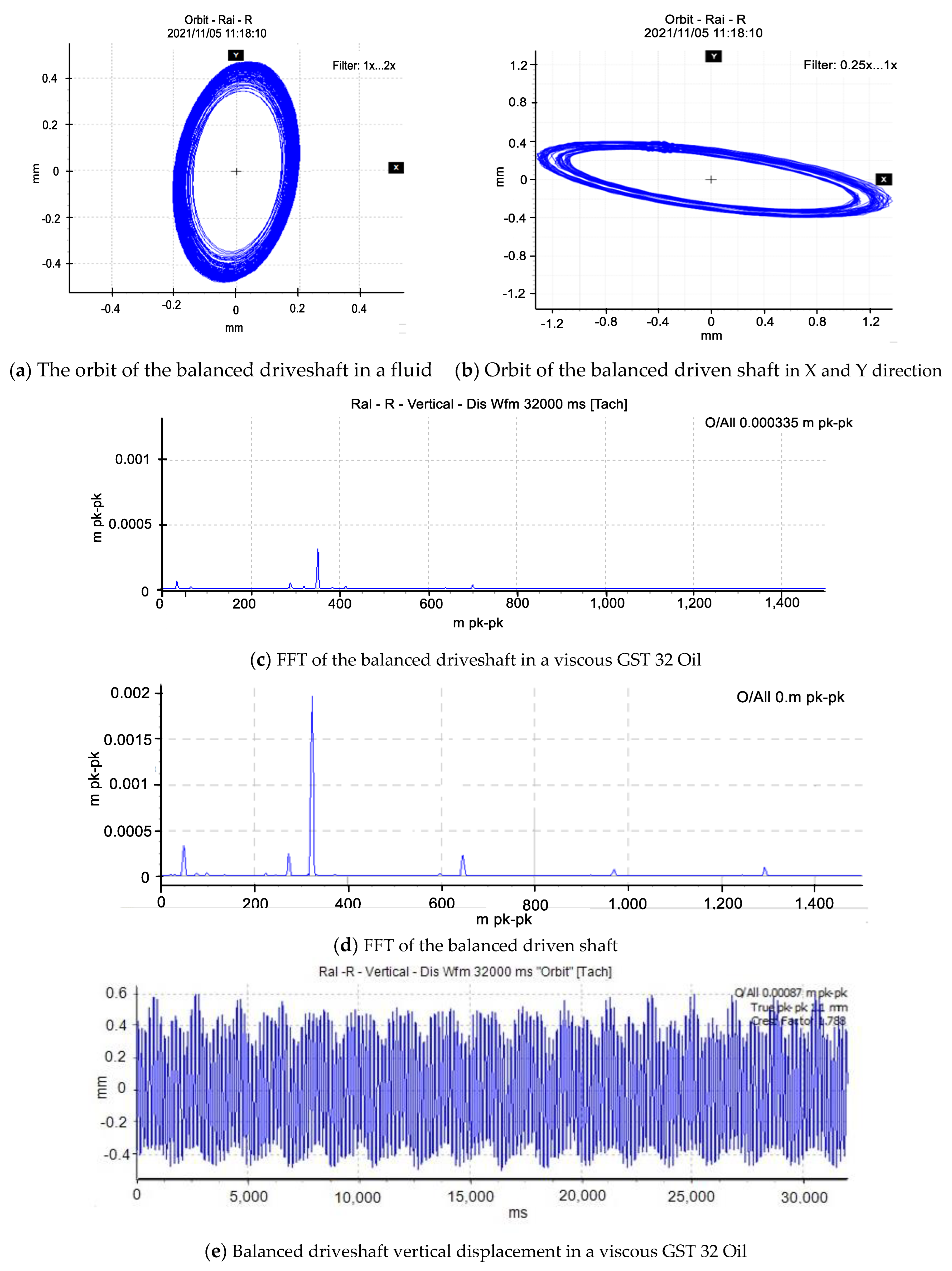
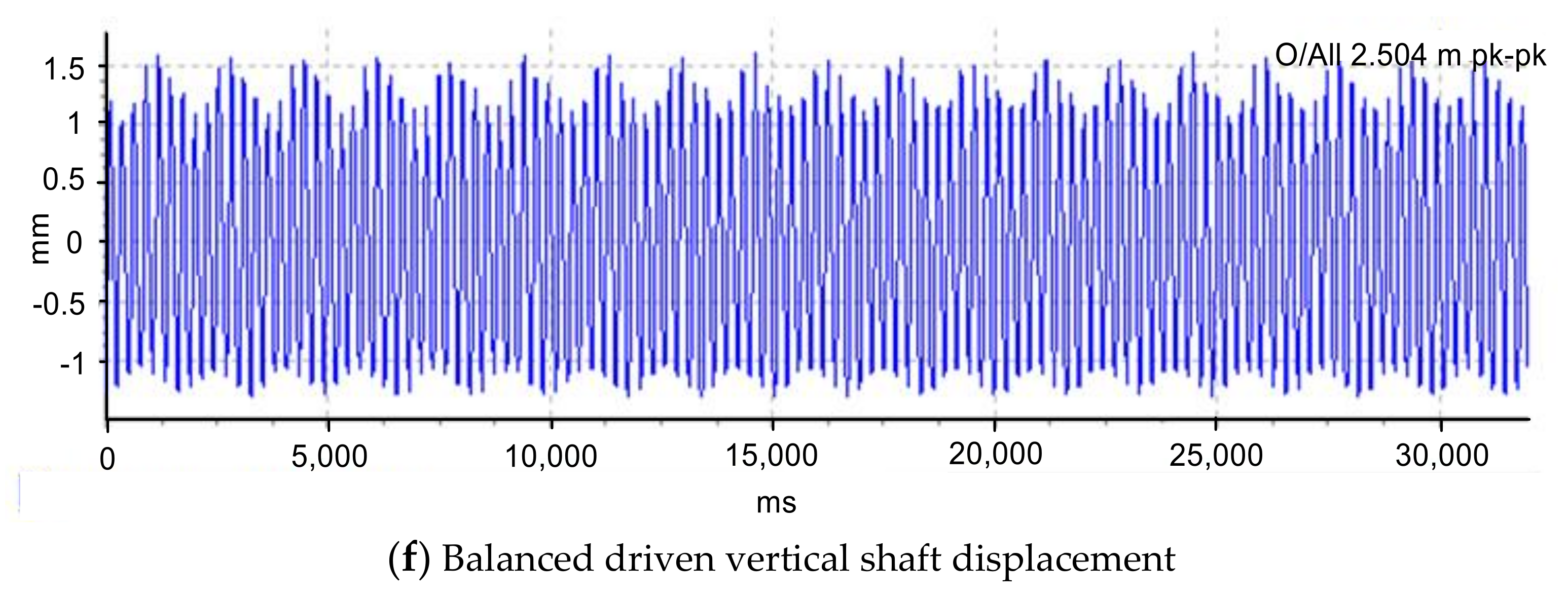
3. Results and Discussion on the Balanced Rotor System
3.1. Baseline Test Results
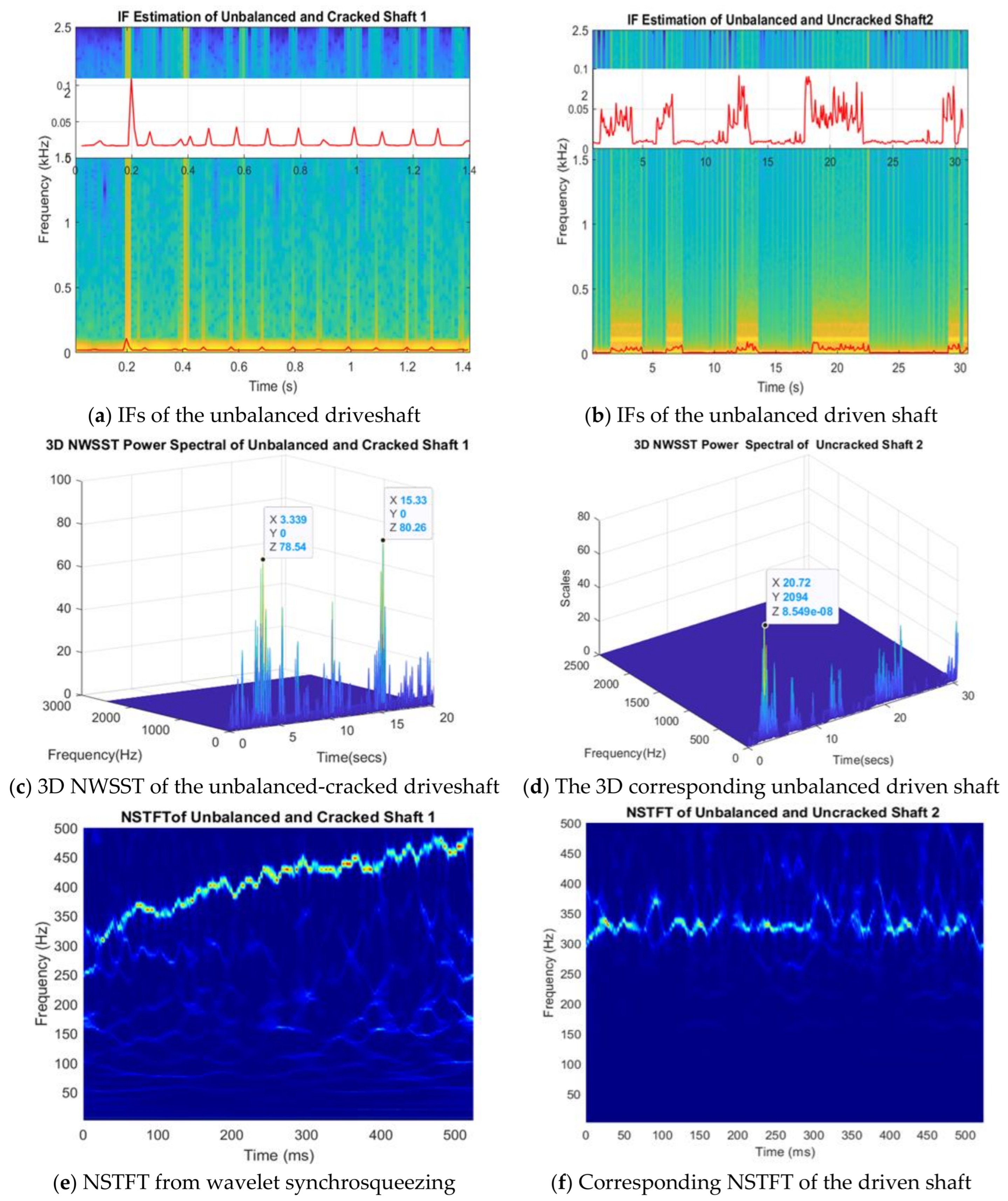
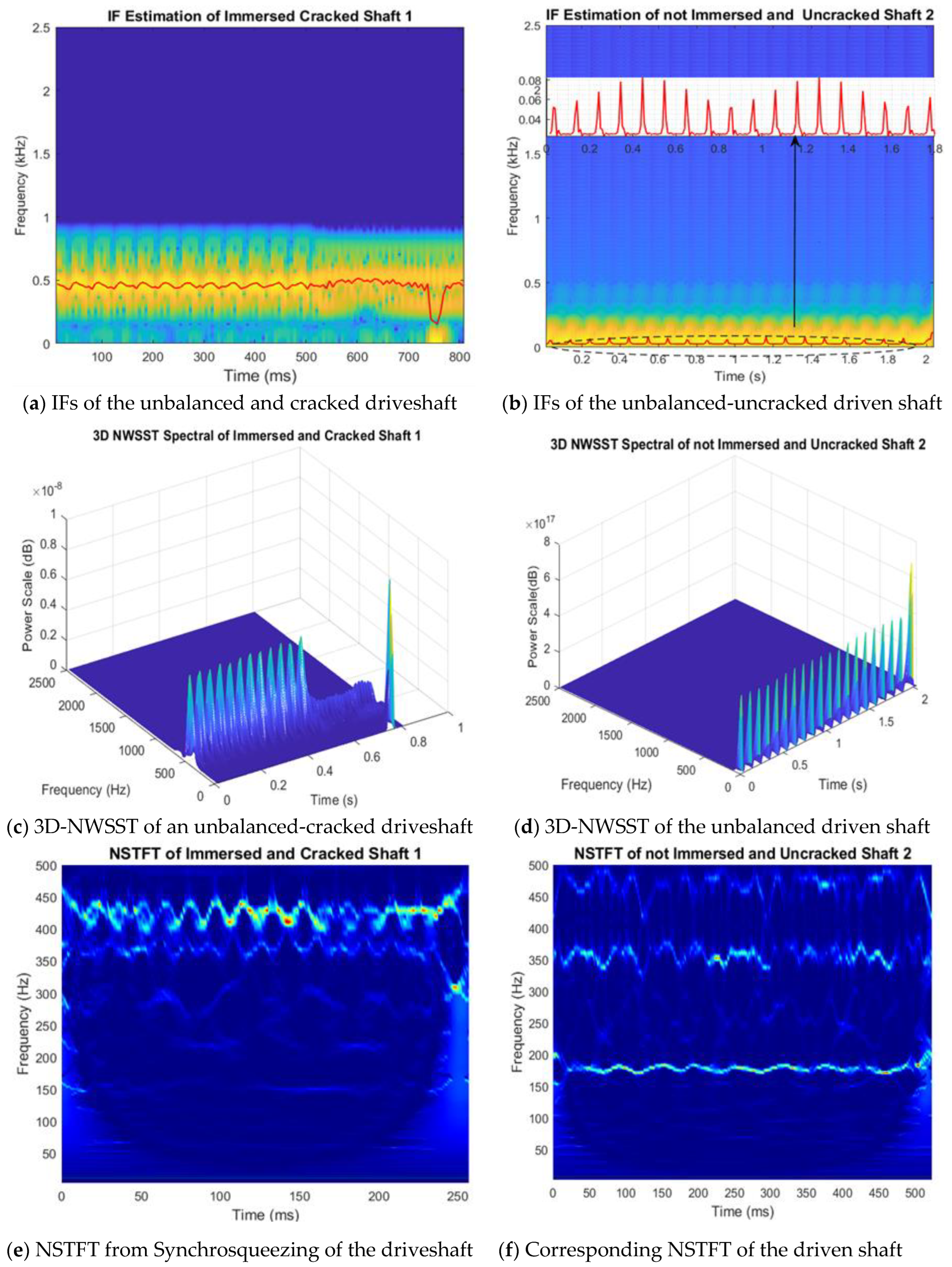
3.2. Crack Feature Extraction and Fault Identification
4. Application of the Wavelet Synchrosqueezing for Fault Detection
Mathematical Theory of the Wavelet Synchrosqueezing for Crack Detection
- First, the continuous wavelet transform (CWT) of a function g(t) with respect to a chosen parent wavelet function ψ(t) is calculated as:where a is the scale parameter and b is the translation parameter. Morlet’s mother wavelet was chosen for the present study because of its concentrated use of time and frequency space. To reduce or eliminate coefficients with small magnitudes or noise-like properties. Denoised coefficients Wg(a, b) are produced by transforming the thresholded coefficients Wsgtresh(a, τ) using the inverse synchrosqueezing transform.where the time-frequency plane is represented by . In order to rebuild the denoised version gs(t) of the original signal g(t), the denoised coefficients Wg(a, b) are combined with the inverse wavelet transform as follows:
- The wavelet transform coefficients are then used to estimate the instantaneous frequency (IF) of gs(t) at each time point t as follows:where d/dt stands for the derivative with respect to time and is the wavelet transform phase Wgs(a, b).
- Normalizing the mother wavelet causes the wavelet function to have unit energy at each scale. The energy of the wavelet coefficients is therefore redistributed to the corresponding instantaneous frequencies using the nonlinear wavelet synchronization transformation. The modified wavelet transform coefficients can be represented as follows:where K(.) is a windowing function. The inverse continuous wavelet transform (ICWT) is then applied to the modified coefficients Wgs(a, τ) to finally obtain the final version of the improved nonlinear squeezing time-frequency transform (NSTFT) representation of the original signal g(t), with improved localization of its content energy.
5. Crack Characteristics and Fault Identification Based on Synchrosqueezing
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yang, Y.; Zhang, Y.; Tan, X. Review on vibration-based structural health monitoring techniques and technical codes. Symmetry 2021, 13, 1998. [Google Scholar] [CrossRef]
- Alugongo, A.A. A nonlinear torsional vibration model of a propeller shaft with a crack-induced parametric excitation and a Hooke’s joint type of kinematic constraint. In IEEE Africon’11; IEEE: Piscataway, NJ, USA, 2011; pp. 1–7. [Google Scholar]
- Asonja, A.; Desnica, E. Reliability of agriculture universal joint shafts based on temperature measuring in universal joint bearing assemblies. Span. J. Agric. Res. 2015, 13, e0201. [Google Scholar] [CrossRef] [Green Version]
- Desnica, E.; Ašonja, A.; Kljajin, M.; Glavaš, H.; Pastukhov, A. Analysis of Bearing Assemblies Refit in Agricultural PTO Shafts. Teh. Vjesn. 2023, 30, 872–881. [Google Scholar]
- Wahab, A.M.; Rasid, Z.A.; Mohd Rudin, N.F.; Abu, A. Dynamic stability of shaft interconnected through joint. ARPN J. Eng. Appl. Sci. 2015, 10, 6310–6318. [Google Scholar]
- Al-Hussain, K.M.; Redmond, I. Dynamic response of two rotors connected by rigid mechanical coupling with parallel misalignment. J. Sound Vib. 2002, 249, 483–498. [Google Scholar] [CrossRef]
- Dupal, J.; Zajíček, M. Analytical periodic solution and stability assessment of 1 DOF parametric systems with time varying stiffness. Appl. Math. Comput. 2014, 243, 138–151. [Google Scholar] [CrossRef]
- Yi, C.; Lin, J.; Ruan, T.; Li, Y. Real time Cardan shaft state estimation of high-speed train based on ensemble empirical mode decomposition. Shock. Vib. 2015, 2015, 912483. [Google Scholar] [CrossRef] [Green Version]
- Patel, T.H.; Darpe, A.K. Experimental investigations on vibration response of misaligned rotors. Mech. Syst. Signal Process. 2009, 23, 2236–2252. [Google Scholar] [CrossRef]
- Ludwicki, M.; Awrejcewicz, J.; Kudra, G. Spatial double physical pendulum with axial excitation: Computer simulation and experimental set-up. Int. J. Dyn. Control 2015, 3, 1–8. [Google Scholar] [CrossRef] [Green Version]
- Zhang, G.; Du, J.; To, S. Study of the workspace of a class of universal joints. Mech. Mach. Theory 2014, 73, 244–258. [Google Scholar] [CrossRef]
- Golafshan, R.; Dascaliuc, C.; Jacobs, G.; Roth, D.; Berroth, J.; Neumann, S. Damage diagnosis of Cardan shafts in mobile mining machines using vibration analysis. In Proceedings of the IOP Conference Series: Materials Science and Engineering, Sanya, Chian, 12–14 November 2021; IOP Publishing: Bristol, UK, 2021; Volume 1097, p. 012019. [Google Scholar]
- Tchomeni, B.X.; Sozinando, D.F.; Alugongo, A. Influences of hydrodynamic forces on the identification of the rotor-stator-rubbing fault in a rotating machinery. Int. J. Rotating Mach. 2020, 2020, 8816191. [Google Scholar] [CrossRef]
- Tchomeni, B.X.; Alugongo, A. Modelling and Dynamic Analysis of an Unbalanced and Cracked Cardan Shaft for Vehicle Propeller Shaft Systems. Appl. Sci. 2021, 11, 8132. [Google Scholar] [CrossRef]
- Tchomeni Kouejou, B.X.; Alugongo, A.A. Dynamic Analysis of a Rotating Cardan Shaft under the Influence of a Breathing Crack and Dense Fluid Force. Shock. Vib. 2022, 2022, 5021488. [Google Scholar] [CrossRef]
- Sabnavis, G.; Kirk, R.G.; Kasarda, M.; Quinn, D. Cracked shaft detection and diagnostics: A literature review. Shock. Vib. Dig. 2004, 36, 287–296. [Google Scholar] [CrossRef]
- Wang, S.; Chen, X.; Wang, Y.; Cai, G.; Ding, B.; Zhang, X. Nonlinear squeezing time–frequency transform for weak signal detection. Signal Process. 2015, 113, 195–210. [Google Scholar] [CrossRef]
- Jiang, Q.; Suter, B.W. Instantaneous frequency estimation based on synchrosqueezing wavelet transform. Signal Process. 2017, 138, 167–181. [Google Scholar] [CrossRef]

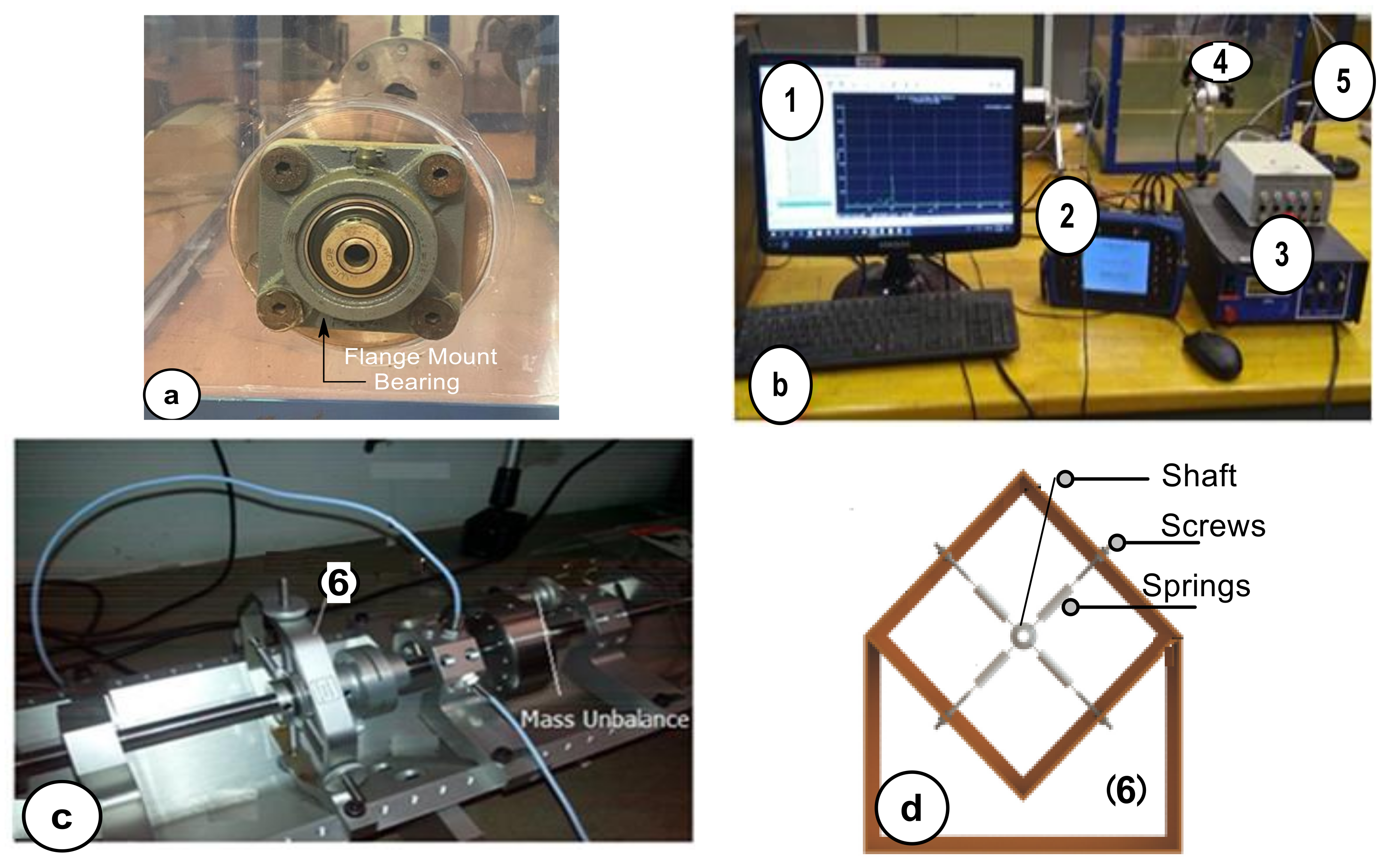




| Shaft Parameters | Value and Units | Bearing Stiffness | Value and Units |
|---|---|---|---|
| Length of the shaft (L) | 570 mm | Left bearing stiffness | 7.35 × 105 Nm−1 |
| Shaft Diameter (D) | 10 mm | Right bearing stiffness | 7.35 × 105 Nm−1 |
| Outer Diameter (Dout) | 75 mm | Damper | 200 Ns/m |
| Inner Diameter (Din) | 10 mm | Discs | Value and Units |
| Density of the material () | 7800 kg m−3 | Mass (M) | 16.845 kg |
| Modulus of elasticity (E) | 2.11 × 1011 Pa | Eccentricity mass (mu) | 0.25 kg |
| Viscous damping ratio | 0.8 × 10−6 s−1 | Mass eccentricity (e) | 10 mm |
| Hooke’s joint angle () | 7° | Disc thickness (Ddisc) | 25 m |
| Characteristics of the Oil | |
|---|---|
| Oil type GST Oil 32 (CHEVRON) | GST Oil 32 (CHEVRON) |
| Colour | Colourless to yellow |
| Physical State | Liquid |
| Vapour Pressure (at 22 °C) | <0.01 mmHg@ 22 °C |
| Viscosity of the oil (at 22 °C) | 145 mPa·s |
| Density of the oil | 866 kg/m3 |
| Depth of oil bath | 4 mm |
| Frequency of oscillations | 0.666 à 30 Hz |
| Amplitudes of oscillations | 0.36 et 0.4 mm |
| Initial thicknesses of oil film | 0.4 à 0.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tchomeni Kouejou, B.X.; Alugongo, A.A. Experimental Analysis of a Cracked Cardan Shaft System under the Influence of Viscous Hydrodynamic Forces. Fluids 2023, 8, 211. https://doi.org/10.3390/fluids8070211
Tchomeni Kouejou BX, Alugongo AA. Experimental Analysis of a Cracked Cardan Shaft System under the Influence of Viscous Hydrodynamic Forces. Fluids. 2023; 8(7):211. https://doi.org/10.3390/fluids8070211
Chicago/Turabian StyleTchomeni Kouejou, Bernard Xavier, and Alfayo Anyika Alugongo. 2023. "Experimental Analysis of a Cracked Cardan Shaft System under the Influence of Viscous Hydrodynamic Forces" Fluids 8, no. 7: 211. https://doi.org/10.3390/fluids8070211
APA StyleTchomeni Kouejou, B. X., & Alugongo, A. A. (2023). Experimental Analysis of a Cracked Cardan Shaft System under the Influence of Viscous Hydrodynamic Forces. Fluids, 8(7), 211. https://doi.org/10.3390/fluids8070211







