High Power Output Augmented Vertical Axis Wind Turbine
Abstract
:1. Introduction
2. Materials and Methods
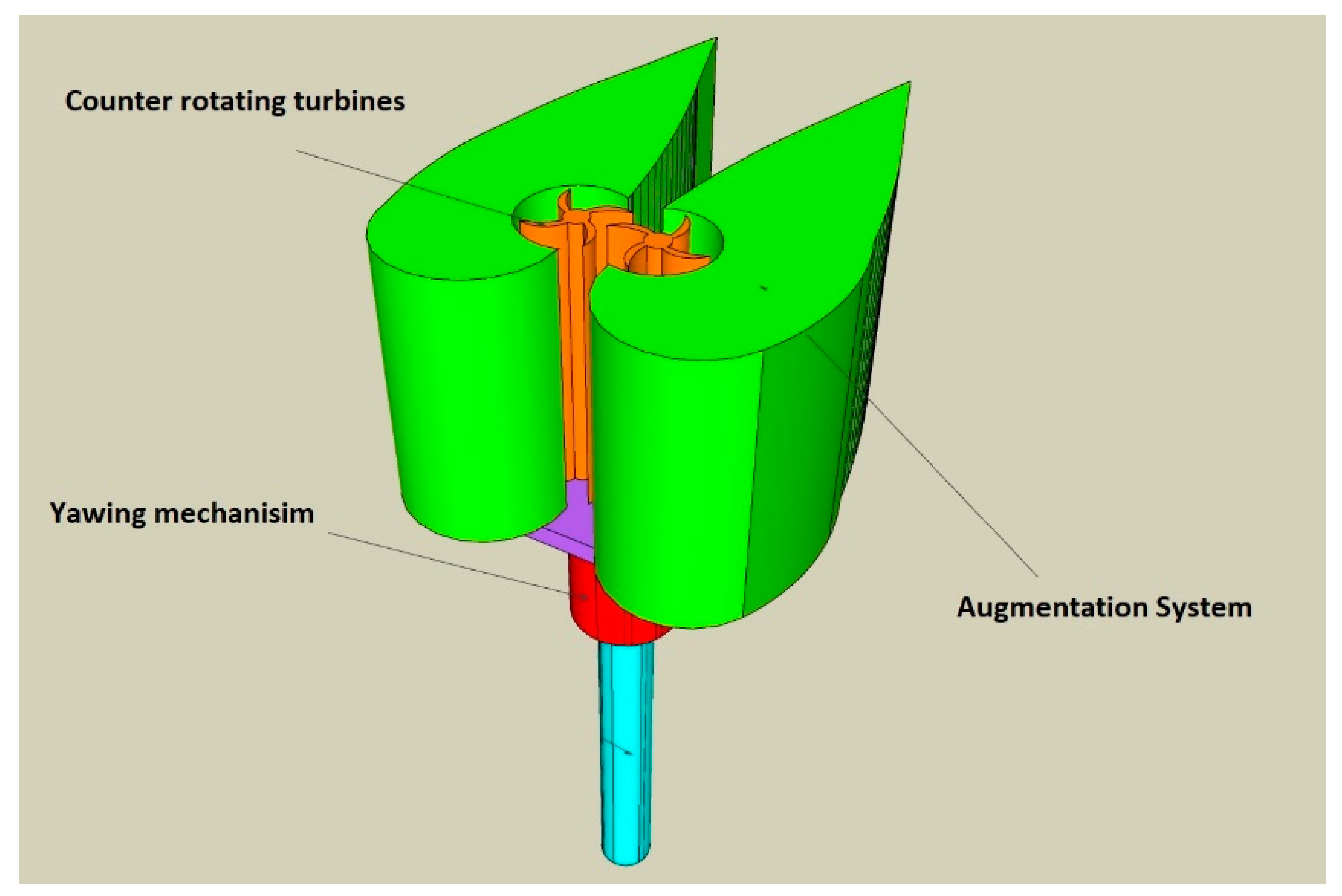
2.1. Computational Model
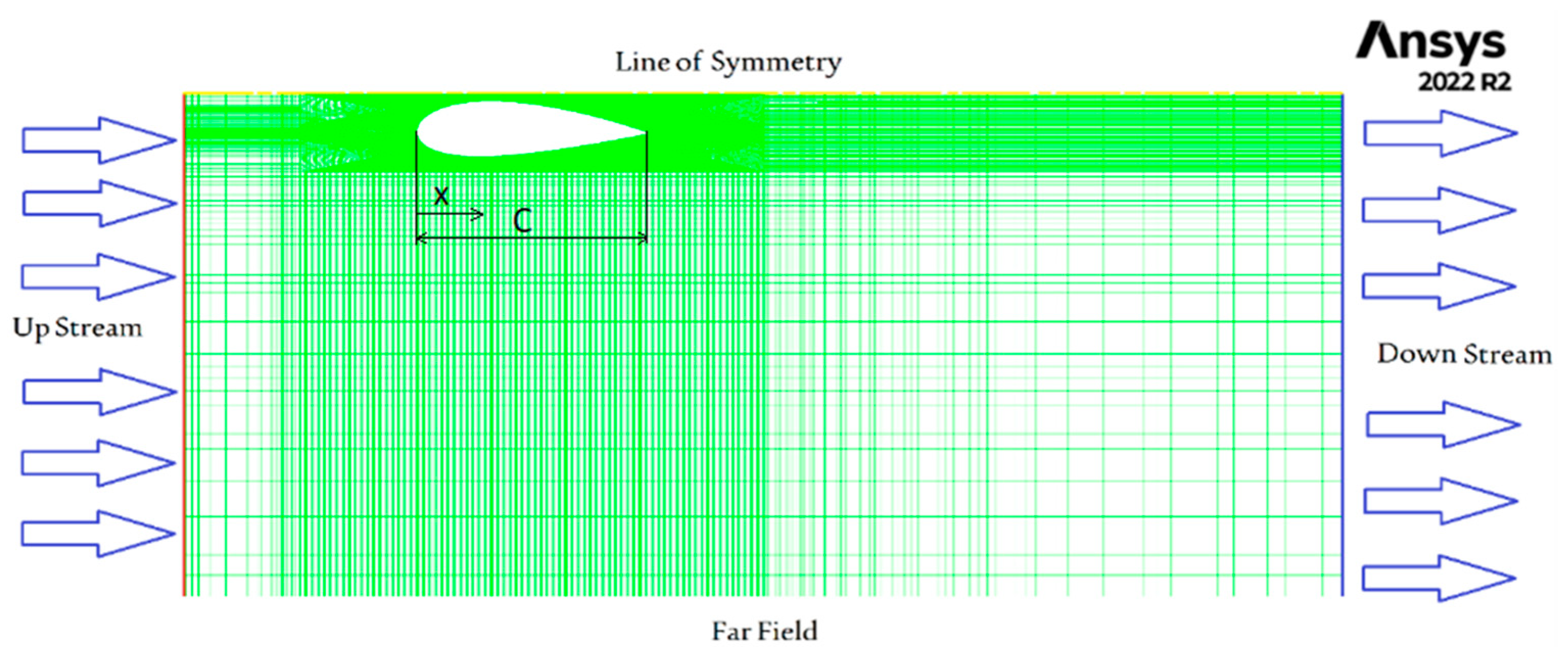
2.2. Governing Equations and Boundary Conditions
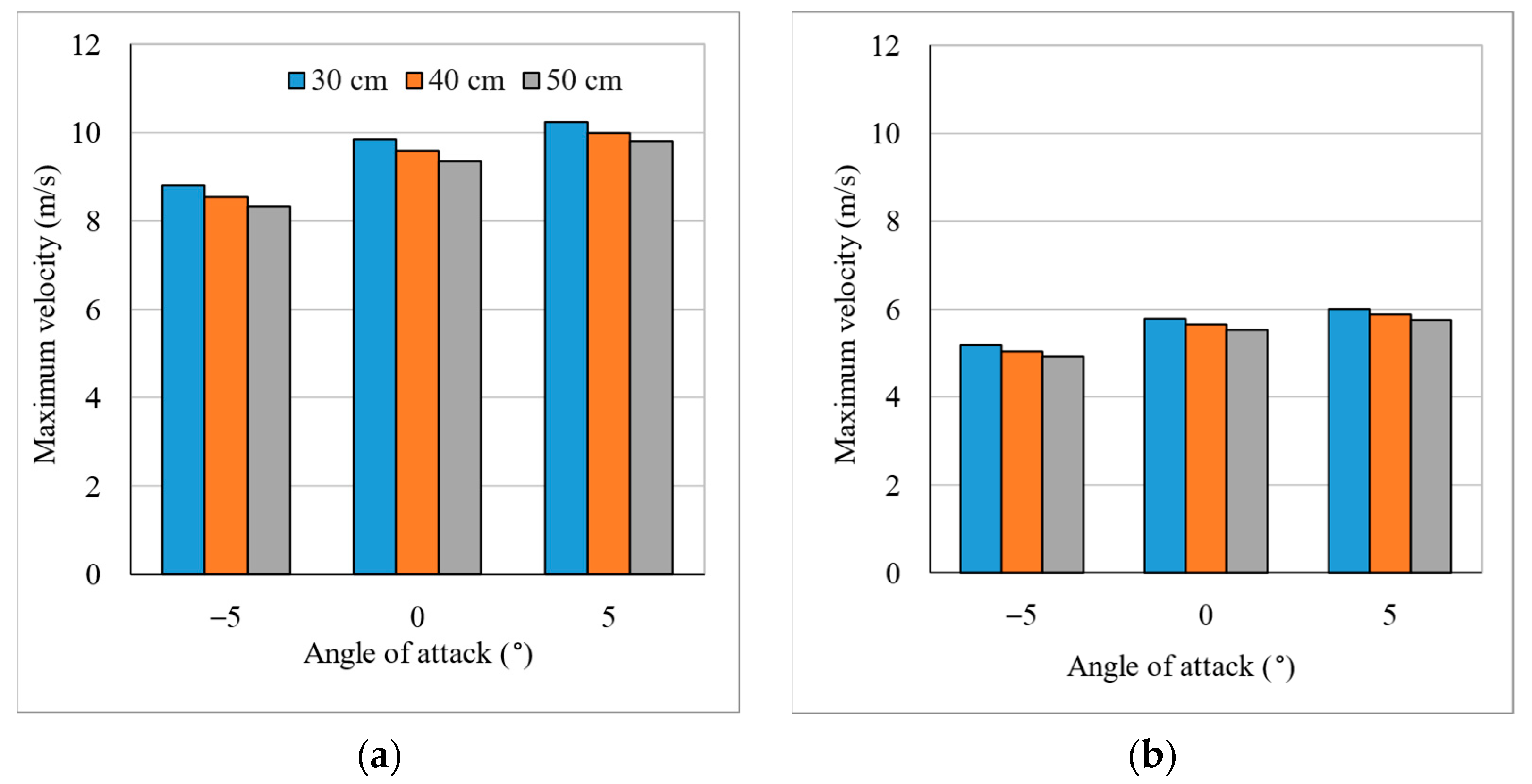
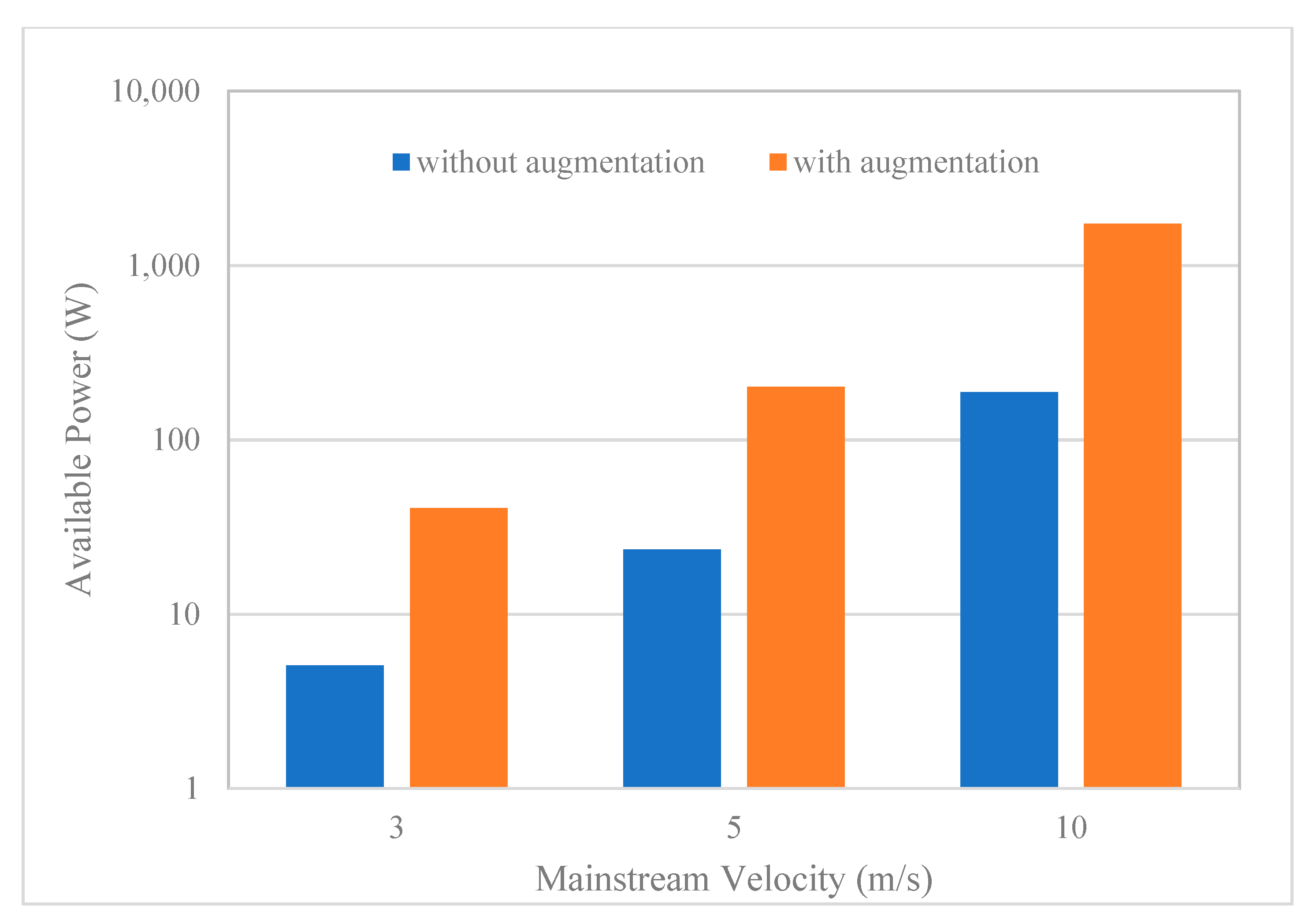
3. Results
4. Conclusions
5. Patents
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Burton, T.; Sharpe, D.; Jenkins, N.; Bossanyi, E. Wind Energy Handbook; John Wiley & Sons, Ltd.: New York, NY, USA, 2001. [Google Scholar]
- Zilberman, M. Optimization of Small, Low Cost, Vertical Axis Wind Turbine for Private and Institutional Use. ACE Res. Propos. 2017, 9, 043302. [Google Scholar] [CrossRef]
- Global Wind Report 2022. Available online: https://gwec.net/global-wind-report-2022/ (accessed on 9 December 2022).
- Betz, A. Introduction to the Theory of Flow Machines; Pergamon Press: Oxford, UK, 1966. [Google Scholar]
- Mohammed, A.; Ouakad, H.; Sahin, A.; Bahaidarah, H. Vertical axis wind turbine aerodynamics: Summary and review of momentum models. J Energy Resour. Technol. 2019, 141, 050801. [Google Scholar] [CrossRef]
- Muratoglu, A.; Yuce, M.I. Design of a River Hydrokinetic Turbine Using Optimization and CFD Simulations. J. Energy Eng. 2017, 143, 04017009. [Google Scholar] [CrossRef]
- Sagharichi, A.; Zamani, M.; Ghasemi, A. Effect of solidity on the performance of variable-pitch vertical axis wind turbine. Energy 2018, 161, 753–775. [Google Scholar] [CrossRef]
- Akwa, J.; Vielmo, H.; Petry, A. A review on the performance of Savonius wind turbines. Renew Sustain. Energy Rev. 2012, 16, 3054–3064. [Google Scholar] [CrossRef]
- Jin, X.; Zhao, G.; Gao, K.; Ju, W. Darrieus vertical axis wind turbine: Basic research methods. Renew Sustain. Energy Rev. 2015, 42, 212–225. [Google Scholar] [CrossRef]
- Wong, K.; Chong, W.; Sukiman, N.; Poh, S.; Shiah, Y.-C.; Wang, C.-T. Performance enhancements on vertical axis wind turbines using flow augmentation systems: A review. Renew Sustain. Energy Rev. 2017, 73, 904–921. [Google Scholar] [CrossRef]
- Karmakar, S.; Chattopadhyay, H. A review of augmentation methods to enhance the performance of vertical axis wind turbine. Sustain. Energy Technol. Assess. 2022, 53, 102469. [Google Scholar] [CrossRef]
- Jamieson, P. Innovation in Wind Turbine Design, 2nd ed.; Wiley: Hoboken, NJ, USA, 2018; Chapter 4; ISBN 978-1119137900. [Google Scholar]
- Wilson, R. Wind-turbine aerodynamic. J. Ind. Aerodyn. 1980, 5, 357–372. [Google Scholar] [CrossRef]
- Menter, F. Review of the shear-stress transport turbulence model experience from an industrial perspective. Int. J. Comput. Fluid Dyn. 2009, 23, 305–316. [Google Scholar] [CrossRef]
- National Weather Service. Available online: https://www.weather.gov/mfl/beaufort (accessed on 9 December 2022).
- Roache, P.J. Perspective: A method for uniform reporting of grid refinement studies. Trans. Am. Soc. Mech. Eng. J. Fluids Eng. 1994, 116, 405–413. [Google Scholar] [CrossRef]
- American Institute of Aeronautics and Astronautics. Guide for the Verification and Validation of Computational Fluid Dynamics Simulations; AIAA: Reston, VA, USA, 1998; ISBN 978-1563472855. [Google Scholar]
- Morris, A.S.; Langari, R. Measurement and Instrumentation Theory and Application; Third Edition 2020 Copyright ©; Elsevier: Amsterdam, The Netherlands, 2021. [Google Scholar]
- Hajaali, A.; Stoesser, T. Flow Separation Dynamics in Three-Dimensional Asymmetric Diffusers Flow. Turbul. Combust. 2022, 108, 973–999. [Google Scholar] [CrossRef]
- Hashem, I.; Mohamed, M.H. Aerodynamic performance enhancements of H-rotor Darrieus wind turbine. Energy 2018, 142, 531–545. [Google Scholar] [CrossRef]
- Ohya, Y.; Karasudani, T.; Sakurai, A.; Abe, K.; Inoue, M. Development of a shrouded wind turbine with a flanged diffuser. J. Wind. Eng. Ind. Aerodyn. 2008, 96, 524–539. [Google Scholar] [CrossRef]
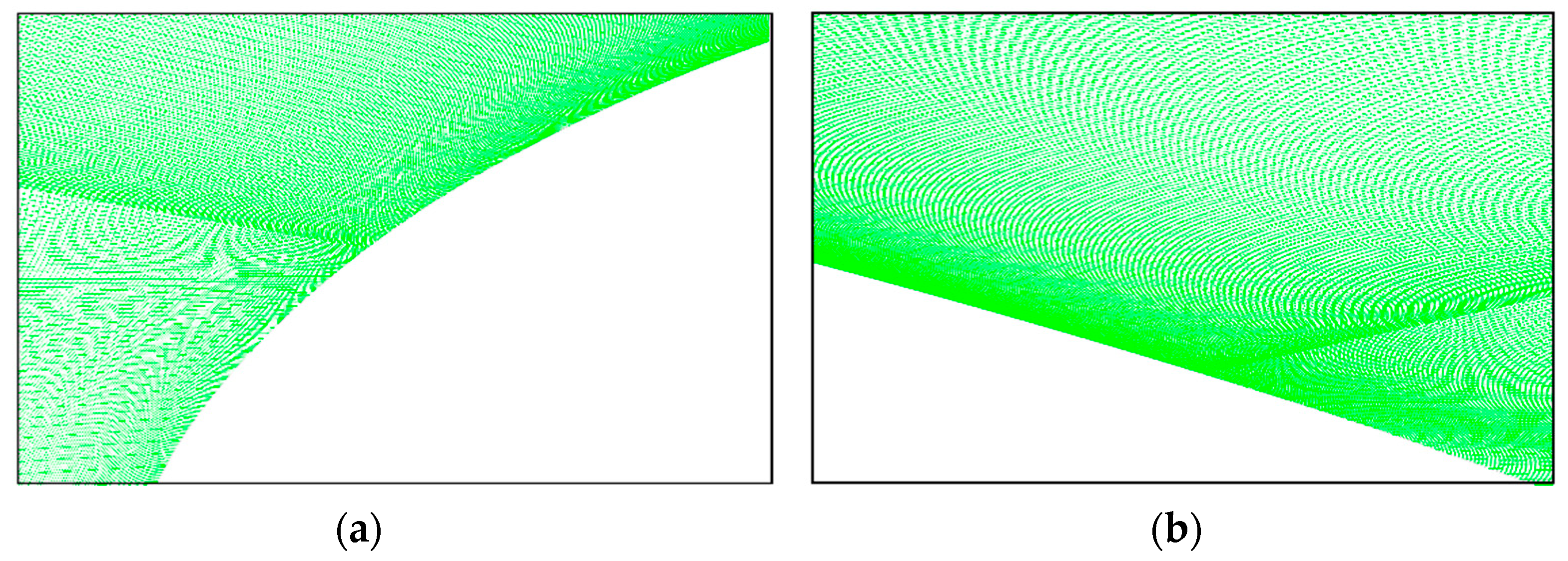

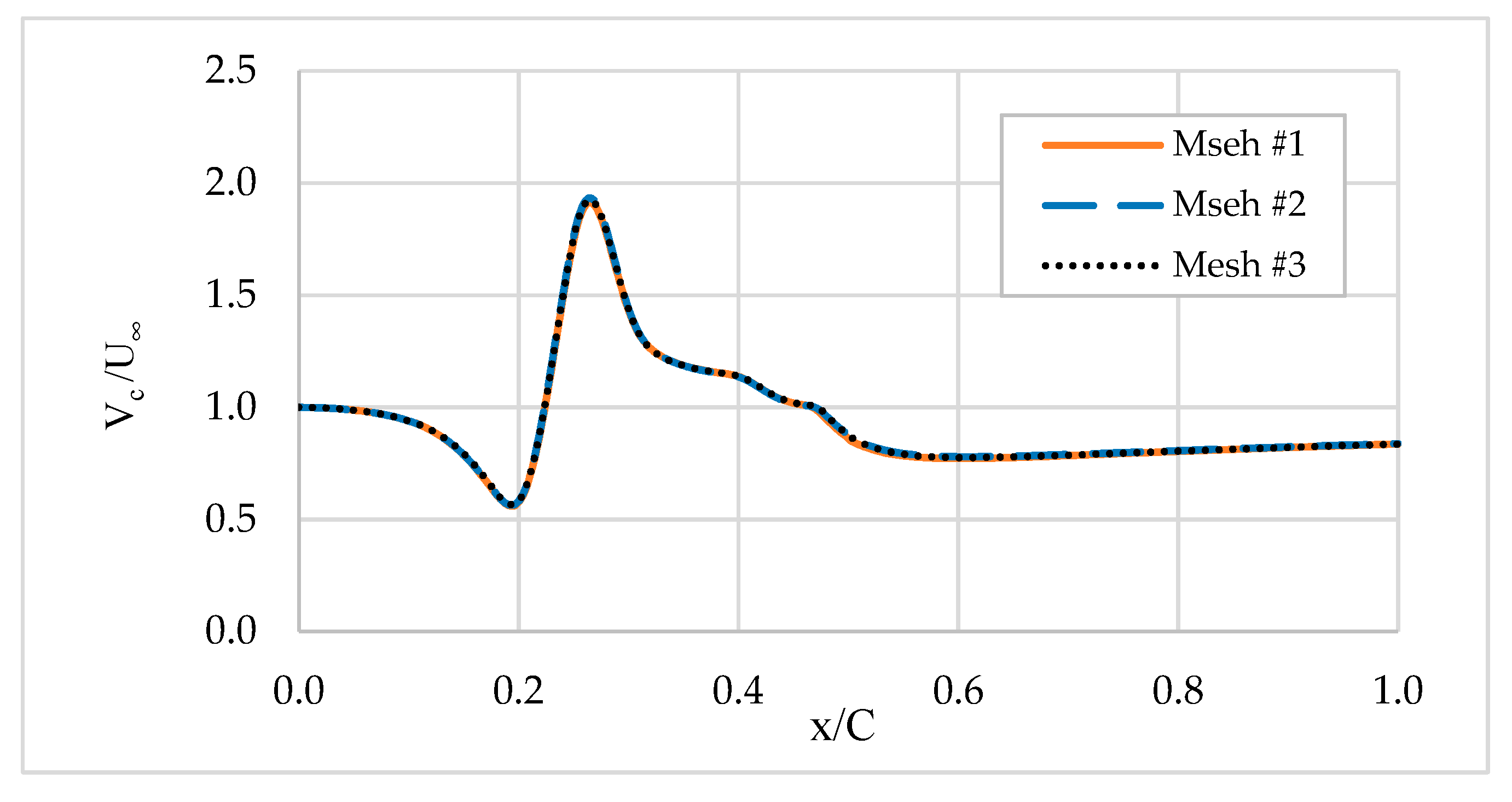

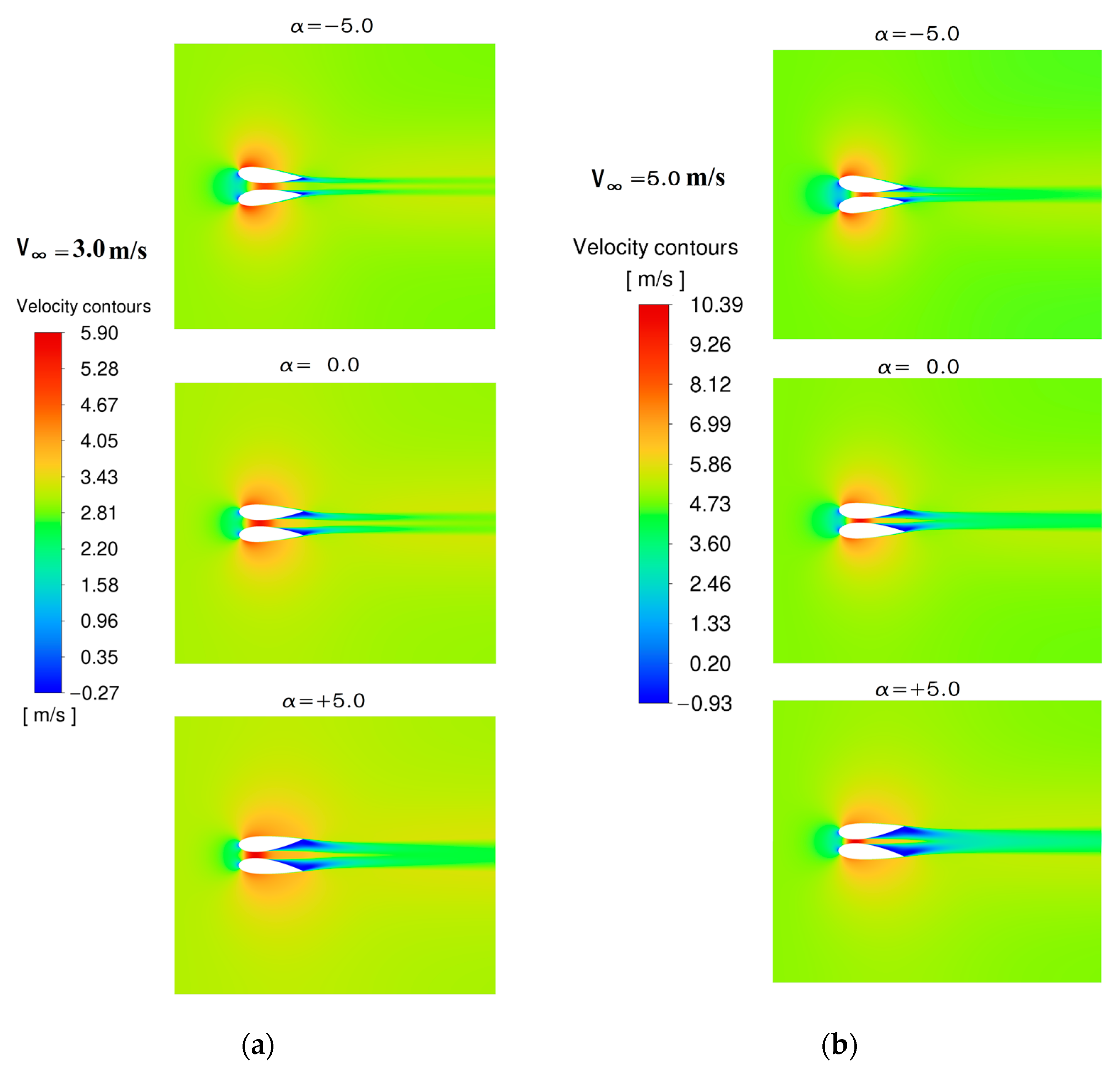


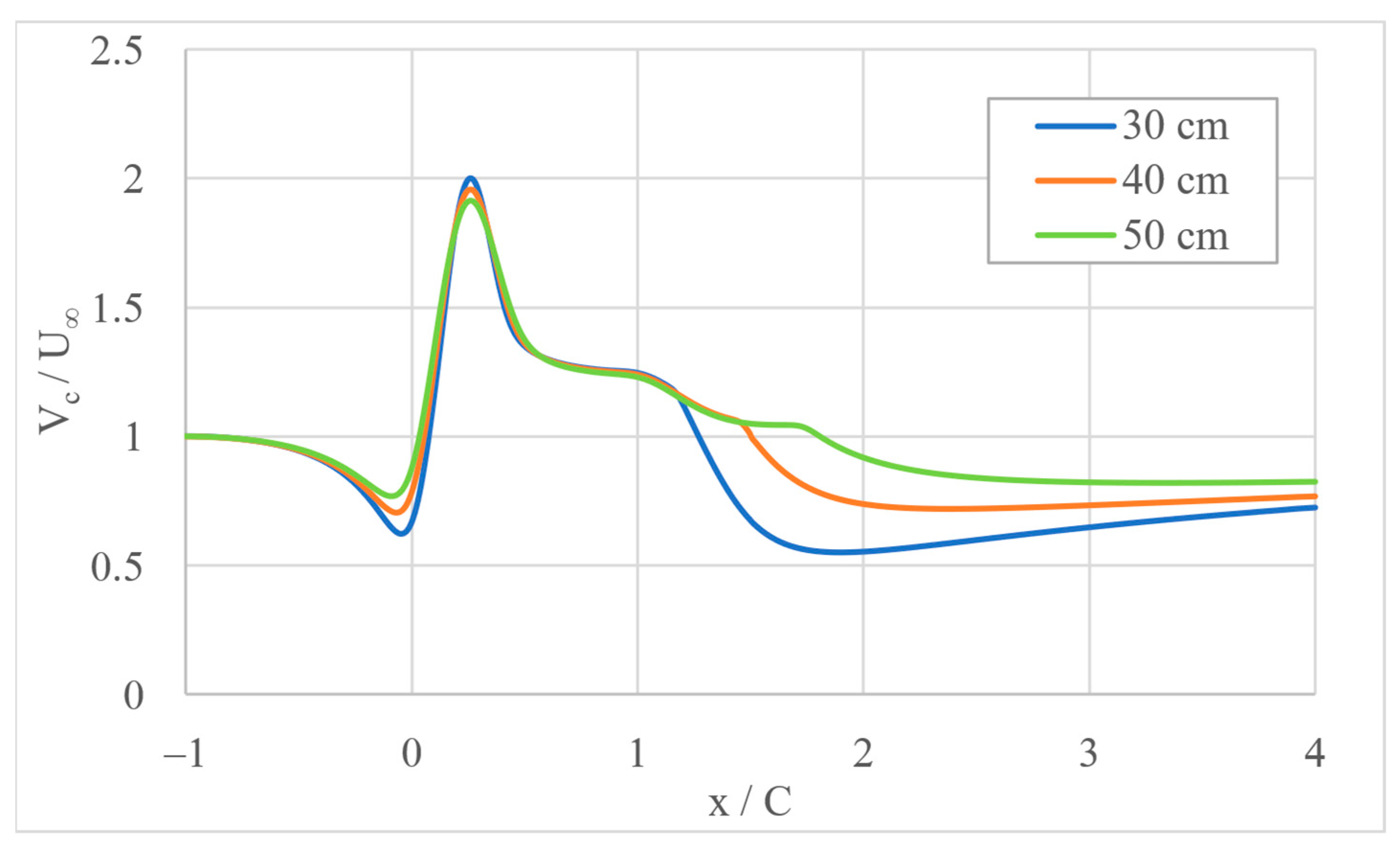
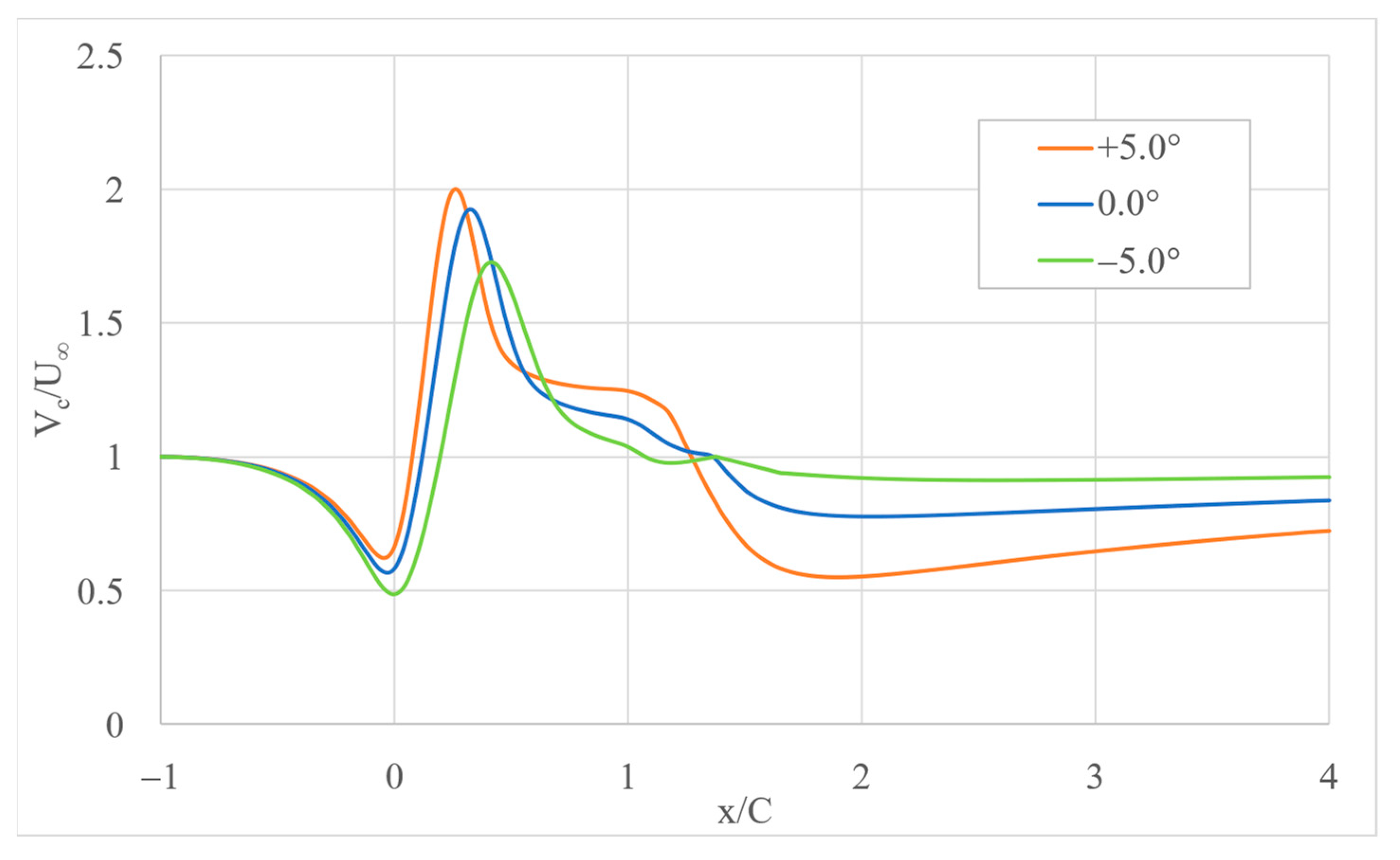
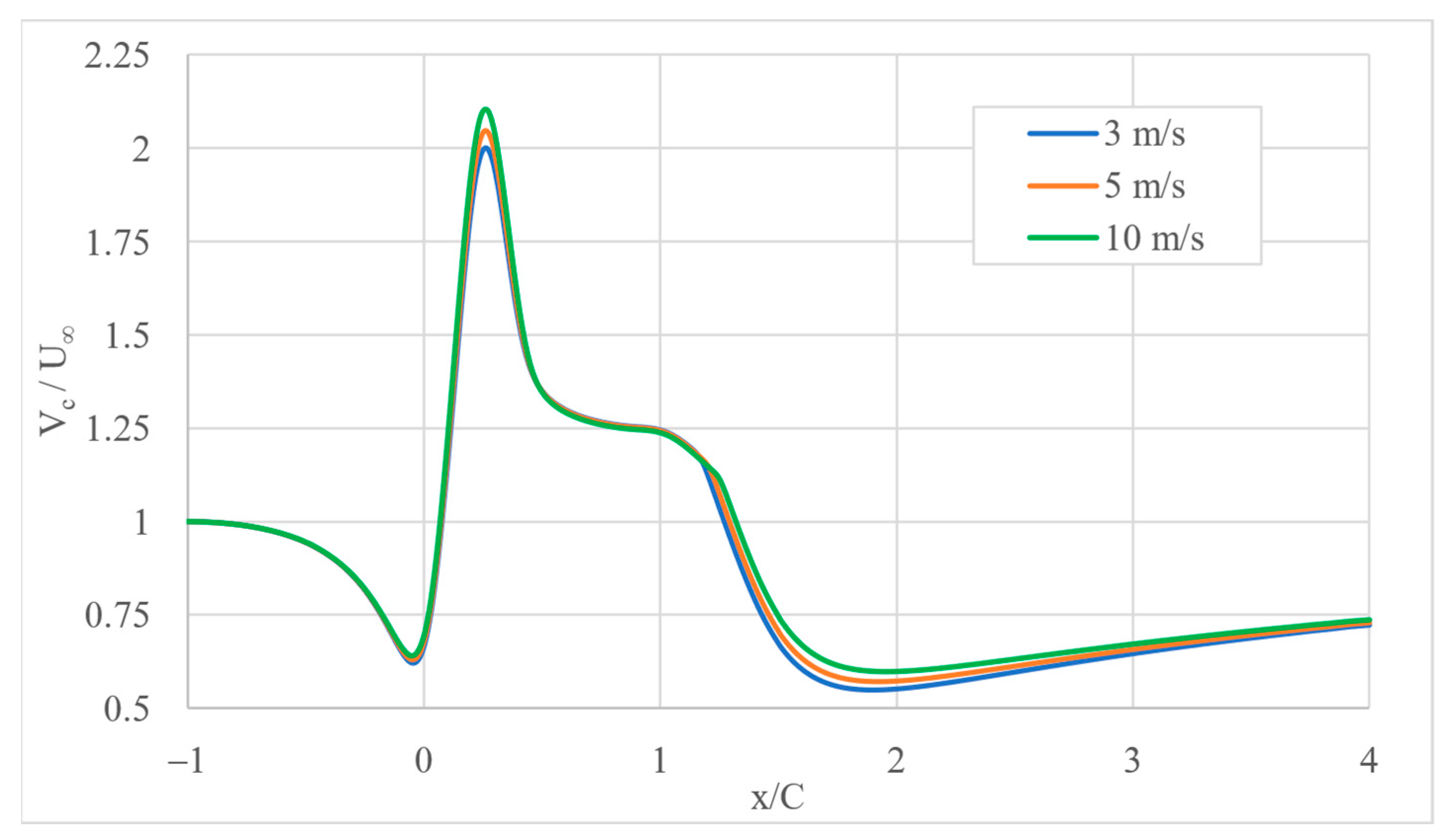
| Mesh # | Mesh Size (Cells) | Increase in Mesh Size (%) |
|---|---|---|
| Mesh 1 | 113,545 | - |
| Mesh 2 | 177,875 | 57 |
| Mesh 3 | 268,107 | 51 |
| Run # | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Gap (cm) | 30 | 40 | 50 | 30 | |||||||||||||||
| U∞ (m/s) | 3 | 5 | 3 | 5 | 3 | 5 | 10 | ||||||||||||
| α (o) | −5 | 0 | 5 | −5 | 0 | 5 | −5 | 0 | 5 | −5 | 0 | 5 | −5 | 0 | 5 | −5 | 0 | 5 | 5 |
| x/Chord | Vc Exp (m/s) | Vc CFD (m/s) | Error (%) |
|---|---|---|---|
| 0 | 7.9 | 7.4 | 6.33 |
| 0.25 | 18 | 16.7 | 7.22 |
| 0.5 | 15.5 | 14.4 | 7.1 |
| 1 | 11 | 10.8 | 1.82 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Salem, H.; Mohammedredha, A.; Alawadhi, A. High Power Output Augmented Vertical Axis Wind Turbine. Fluids 2023, 8, 70. https://doi.org/10.3390/fluids8020070
Salem H, Mohammedredha A, Alawadhi A. High Power Output Augmented Vertical Axis Wind Turbine. Fluids. 2023; 8(2):70. https://doi.org/10.3390/fluids8020070
Chicago/Turabian StyleSalem, Hayder, Adel Mohammedredha, and Abdullah Alawadhi. 2023. "High Power Output Augmented Vertical Axis Wind Turbine" Fluids 8, no. 2: 70. https://doi.org/10.3390/fluids8020070
APA StyleSalem, H., Mohammedredha, A., & Alawadhi, A. (2023). High Power Output Augmented Vertical Axis Wind Turbine. Fluids, 8(2), 70. https://doi.org/10.3390/fluids8020070







