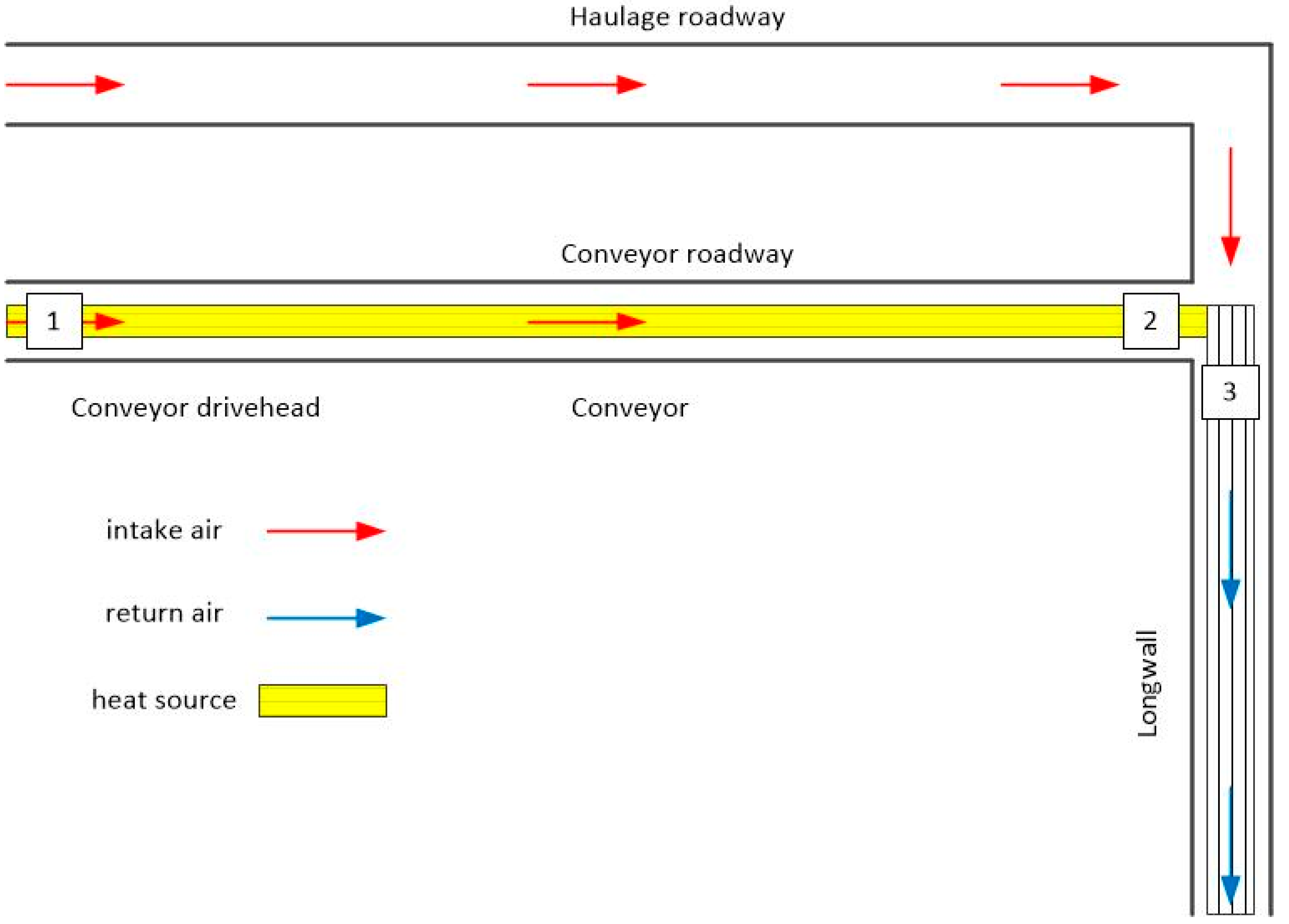
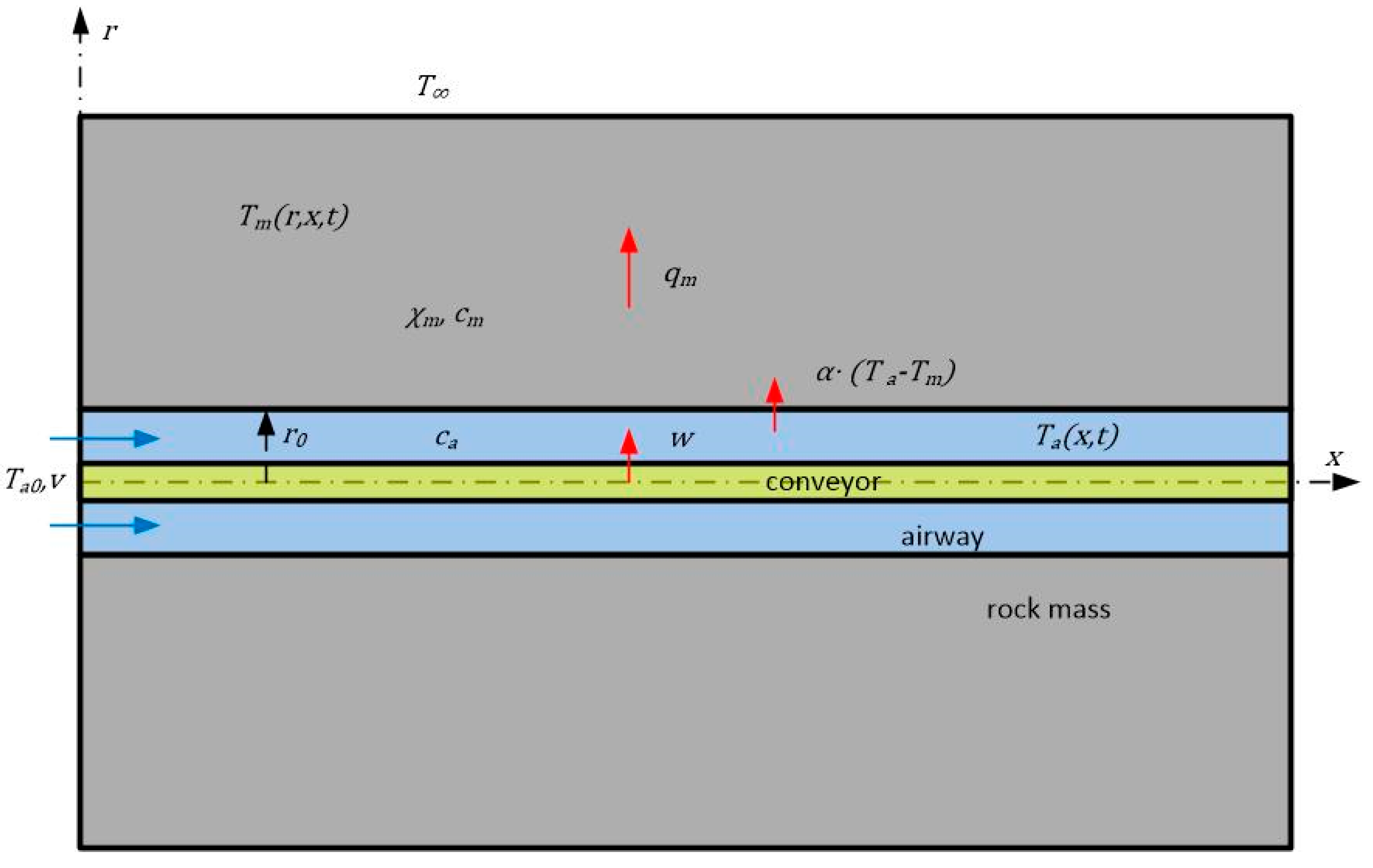
1. Introduction
The air temperature in mine excavation depends on many factors, which can be divided into two types—the heat exchange of air with the rock mass and the heat exchange due to heat sources/sinks. The nature of the problem under study determines how detailed the factors of the first type should be. The simplest approach is to set boundary conditions of the first, second, or third kind on the excavation wall without considering the heat propagation in the rock mass. Boundary conditions of the first kind are also called Dirichlet conditions, boundary conditions of the second kind are also called Neumann conditions, and boundary conditions of the third kind can be called mixed or convective conditions. A more complex approach considers heat transfer in both the air and surrounding rock mass in a coupled formulation [
1,
2].
The simplest approach is appropriate and has an insignificant error in limiting cases for short and long simulation times. In the first case, when thermal contact between air and the rock mass is short (on the order of several hours), the rock mass at the air boundary does not have time to change temperature significantly. For this reason, the heat exchange of air with the rock mass is specified using the convective boundary condition of the third kind, and the heat transfer in the rock mass is not investigated. With thermal insulation near the mine excavation, a boundary condition of the second kind is set to a zero value for the heat flux on the wall. In another limiting case, when—after a long time with a nearly unchanged air temperature distribution along the length of the mine excavation—temperature changes propagate deep into the rock mass, the boundary condition of the first kind with the equality of air and rock mass temperatures is acceptable.
If the specifics of the problem do not correspond to the limiting cases above, then the problem should be solved in a coupled formulation, considering the variation of the temperature field in the rock mass. It is also possible to use a simplified model based on the “nonstationary heat transfer coefficient”. This coefficient implicitly considers the change in temperature of the rock mass near the mine excavation with time [
3]. At the same time, the excavation wall’s temperature at the initial time is presented in the boundary condition of the heat balance between the air and the rock mass.
To predict temperature changes in ventilation air as it moves through the systems of mine excavations, it is also necessary to know the factors of the second type associated with heat sources and sinks. After the air is supplied to the mine through the air supply shaft, the temperature begins to change—both due to heat exchange with the rock mass and incoming heat of natural and artificial origin. Natural processes include the heating and cooling of air in the vertical mine shaft due to hydrostatic compression and expansion, evaporation and condensation of moisture, and heat release during oxidative processes and combustion. The artificial heat sources include heating and air conditioning units and mining equipment that generates heat.
Accounting for artificial sources of heat release is especially important if they are located along the air path near the working areas since, in this case, they can affect the temperature of the air supplied to ventilate the working areas [
4]. Most often, this temperature is determined by the natural temperature of the rock mass, which it approaches due to heat transfer. Local sources of heat release affect the air parameters only at small distances since local air heating is quickly leveled by the heat exchange process. However, the situation changes in the presence of distributed heat sources such as conveyor systems. The results of the experimental measurements given below show a significant influence of this factor on air temperature.
The novelty of the study lies in solving the problem of coupled heat transfer in the mine air and rock mass in the presence of a distributed heat source. Traditionally, the problem of heat transfer is limited by the influence of point sources [
2,
4,
5]. This usually comes down to setting the appropriate boundary conditions for the incoming air temperature; then the air temperature changes due to heat and mass transfer processes. In this study, the final air temperature at short and long times was of interest under the simultaneous action of both factors.
3. Mathematical Model
This section describes a heat transfer model in the atmosphere of the conveyor roadway. The conveyor belt is considered a uniformly distributed heat source along the excavation length. The conveyor operating time is assumed to be arbitrary. Therefore, a simplified heat transfer analysis without considering the heat distribution in the rock mass is not acceptable since it does not allow an accurate assessment of the change in heat removal intensity in the rock mass. The approach based on the nonstationary heat transfer coefficient [
5] is also not acceptable in this case because it has a limited time range of applicability and leads to overestimated results in relation to the heat removal intensity. The dependence of the heat transfer coefficient between the mine air and rock mass on their geometric parameters and air velocity is well studied [
6]. Therefore, the heat transfer problem can be reduced to the statement of the boundary conditions of the fourth kind without simulating heat transfer through the boundary layer [
7]. Here, we understand the boundary condition of the fourth kind as the equality of temperature and heat fluxes at the boundary of two heat-conducting media (rock mass and air) at each moment of time.
The solution was determined based on the model presented in [
8,
9,
10] since this heat transfer model, unlike approximate analytical models [
11,
12,
13,
14,
15,
16] and numerical models [
17,
18,
19], does not have strong assumptions regarding the limitation of the computational domain or the use of approximate solutions.
The problem of heat exchange between mine air and rock mass with heat sources distributed along the length of a horizontal conveyor roadway is modeled assuming cylindrical symmetry of the roadway with two spatial coordinates—radial
(m) and horizontal
(m) [
6]. The values of volumetric heat capacity
(J/(m
3·°C)) and thermal diffusivity
(m
2/s) of a rock mass are considered constant. Air with volumetric heat capacity
(J/(m
3·°C)) and constant temperature
(°C) is supplied to the entry of the roadway (
x = 0) with radius
(m). Assuming insignificant changes in air density
(kg/m
3) and velocity
(m/s), these values are assumed to be constant and correspond to the average air density. It is assumed that at the initial moment of time before the heat source is turned on, the entire rock mass and all the air in the excavation at
x > 0 have the temperature of the “undisturbed” rock mass
(°C). Because the turbulent thermal conductivity of air is much greater than the molecular thermal conductivity of rock, it can be assumed that the air temperature along the excavation cross section equalizes instantly. Meanwhile, the diffusive heat transfer in the air movement direction is insignificant compared to convective heat transfer; therefore, the longitudinal thermal conductivity in air can be set to zero—i.e., heat transfer along the excavation is only convective air movement. A similar simplification is made since the air velocity is much higher than the intensity of heat propagation in the rock mass; i.e., temperature differences along the
x axis and in the radial direction in the rock mass will be of different orders: in
, meters, in
, centimeters. This makes it possible to neglect the propagation of heat in the direction of the
x axis in the rock mass. Thus, only radial thermal conductivity takes place in both the rock mass and air, while the longitudinal thermal conductivity is considered insignificant and is not considered in the model. Another important characteristic of the air flow is its relative humidity. However, in this study, we did not analyze its distribution for two reasons—due to the absence of significant sources of moisture in the potash mine, and also due to the fact that the regulatory requirements place restrictions only on the dry bulb temperature.
The computational scheme for the problem is shown in
Figure 2.
To simplify the mathematical notation of the problem, dimensionless coordinates are introduced:
Air temperature (°C) and rock mass temperature (°C) are counted from the value . These parameters remain dimensional.
Dimensionless variables are written with a prime. The equation of heat conduction in the rock mass in cylindrical coordinates has the following form:
The density of the heat flux from the air to the rock mass (J/(m2·s)) should be equal to the density of the heat flux (J/(m2·s)) incoming through the excavation wall = 1 to the rock mass. It is necessary to write an equation for the balance of heat (J) in an elementary volume of air (m3) with a cross-section area (m2) and thickness (m) with the heat exchange surface of volume (m2).
Since the thermal conductivity of air in the radial direction in the model is assumed to be infinite, the heat balance is reduced to the equality of the total heat flux through
and the change in heat content in a volume of air
(in dimensional form):
where
is the volume source of heat, J/(m
3·s).
Equation (2) differs from the model presented in [
8] by the presence of an additional term
, which specifies the intensity of heat release from a source (conveyor belt) uniformly distributed along the length of the excavation. A similar approach to heat transfer modeling was used in [
9] to describe air heating due to its hydrostatic compression while moving along an air supply shaft.
The problem is solved analytically to obtain a definite integral, for the calculation of which the numerical method of trapezoids is used; the solution is implemented in a self-written program in the Pascal language.
This makes it possible to obtain an exact solution of the heat transfer problem without introducing restrictions on the computational area of the array and to use the most physical boundary condition at an infinite radius value. In addition, the proposed solution allows one to quickly calculate the air temperature even at long heat transfer times.
The condition at the boundary of the air with the rock mass in dimensionless form takes the following form:
The second boundary condition contains information about the value of the heat transfer coefficient and determines the temperature difference between the air and the rock mass at the boundary (excavation wall):
Since condition (3) contains a derivative concerning the coordinate, it must be supplemented with the value
(°C) of the air temperature at the beginning of the excavation; thus,
The whole system of equations is supplemented with the initial condition for temperature fields:
The unsteady cylindrical problem (1)–(6) is solved using the Laplace transformation [
20]. The functions of air and rock mass temperatures are associated with their images.
Here, is a complex parameter with domain .
The partial differential Equation (1) for the original
is reduced to an ordinary differential equation for the image
:
with the following boundary and initial conditions:
which are obtained from conditions (3)–(5), respectively.
Equation (7) is the Bessel equation, the solution of which is
where
and
are the zero-order Bessel and Neumann functions, and the coefficients
and
are to be determined [
21]. The connection between them sets the condition at infinity:
The ratio between the coefficients
and
should be such that when
and
,
Based on the asymptotic expansions of the functions
and
as
[
9], we can conclude that in (12),
for
and
for
. The function
, where
is a complex number, is two-valued, and only one of its values should appear in the calculation. This value can be specified by the condition
. After representing
in exponential form
, it is easy to verify that the condition
corresponds to the condition
and vice versa; the condition
corresponds to the condition
.
Laplace transforms allow one to separate the variables
and
and reduce the dimension of the problem. Now, taking
= 1, only the dependence on
remains and, after a transition to the original, on
. Further, the
coordinate is omitted, which means
= 1. After substituting (11) into (9), the coefficient
, taking into account (7), is expressed in terms of
:
Now, after substituting (13) into (11) and (11) into (8), we obtain a differential equation with one unknown function,
:
Solution (14), taking into account (10), gives the dependence
which coincides with the formula obtained in [
8] for heat transfer without heat release sources. Restoring the original,
where the integration is carried out along any straight line with a real coordinate
greater than the growth rate of the function
.
To determine the heat transfer coefficient, which determines the parameters
and
, the dependence [
6] can be used:
This dependence was obtained for cylindrical channels with airflow at Reynolds numbers over 104.
The obtained Formulas (15) and (16) make it possible to calculate the air temperature as a function of time and longitudinal coordinates. The numerical integration of expression (16) was done using the trapezoidal rule.
4. Results and Discussion
In accordance with the developed mathematical model of heat transfer in an excavation with distributed heat sources, a numerical assessment was made of air heating by a moving conveyor belt after a long period (after 1 year) of usage. The purpose of the calculation was also to optimize the distribution of the given airflow in two parallel air supply excavations (the conveyor and haulage roadways) according to the criteria for minimizing the air temperature of the mixed air streams at the outlet. An additional limitation was not exceeding the maximum allowable air temperature in the conveyor roadway. The calculation parameters in dimensional form were as follows. The cross-section area of two parallel excavations was assumed to be 11 m2, and the length was 3000 m. The total air flow rate along two excavations was 10 m3/s. The average intensity of heat release in the conveyor roadway was 100 W/m, taking into account the cyclic operation of the conveyor and the fact that the conveyor operates only 50% of the total shift time. The temperature of the rock mass was +21 °C, and the initial temperature of the air entering the excavations was +18 °C. The thermal conductivity of the rock mass was 5 × 10−6 m2/s, and the specific heat capacity of the rock mass was 838 J/(kg∙°C). The density of the rock mass was 2.1 kg/m3. The equivalent diameter of the mine excavation was 3.7 m. In addition, when solving the problem, there was an additional limitation—the air temperature in the conveyor roadway should not exceed +40 °C. Safety rules restrict people from staying in the mine at temperatures >26 °C but still make it possible to operate the electrical conveyor equipment. However, at temperatures >+40 °C, electrical equipment must be turned off.
Table 2 shows the results of calculating the final air temperature after mixing the airflows from haulage and conveyor roadways and the temperature at the end of the conveyor roadway.
The calculated data presented in
Table 1 show that the optimal ratio of air distribution along the roadways under the given conditions is 20% (2 m
3/s) in the conveyor roadway and 80% (8 m
3/s) in the haulage roadway. At the same time, the minimum temperature of the mixed air is +22 °C, and the temperature in the conveyor drift does not exceed +40 °C (the limiting temperature of the electrical equipment).
It should be noted that the heat exchange time (1 year) from the beginning of the conveyor operation is not large in the sense of reaching the maximum possible temperature
(°C) at the outlet of the conveyor roadway, which, after an infinitely long time, should correspond to the adiabatic solution:
where
is the air flow rate, m
3/s.
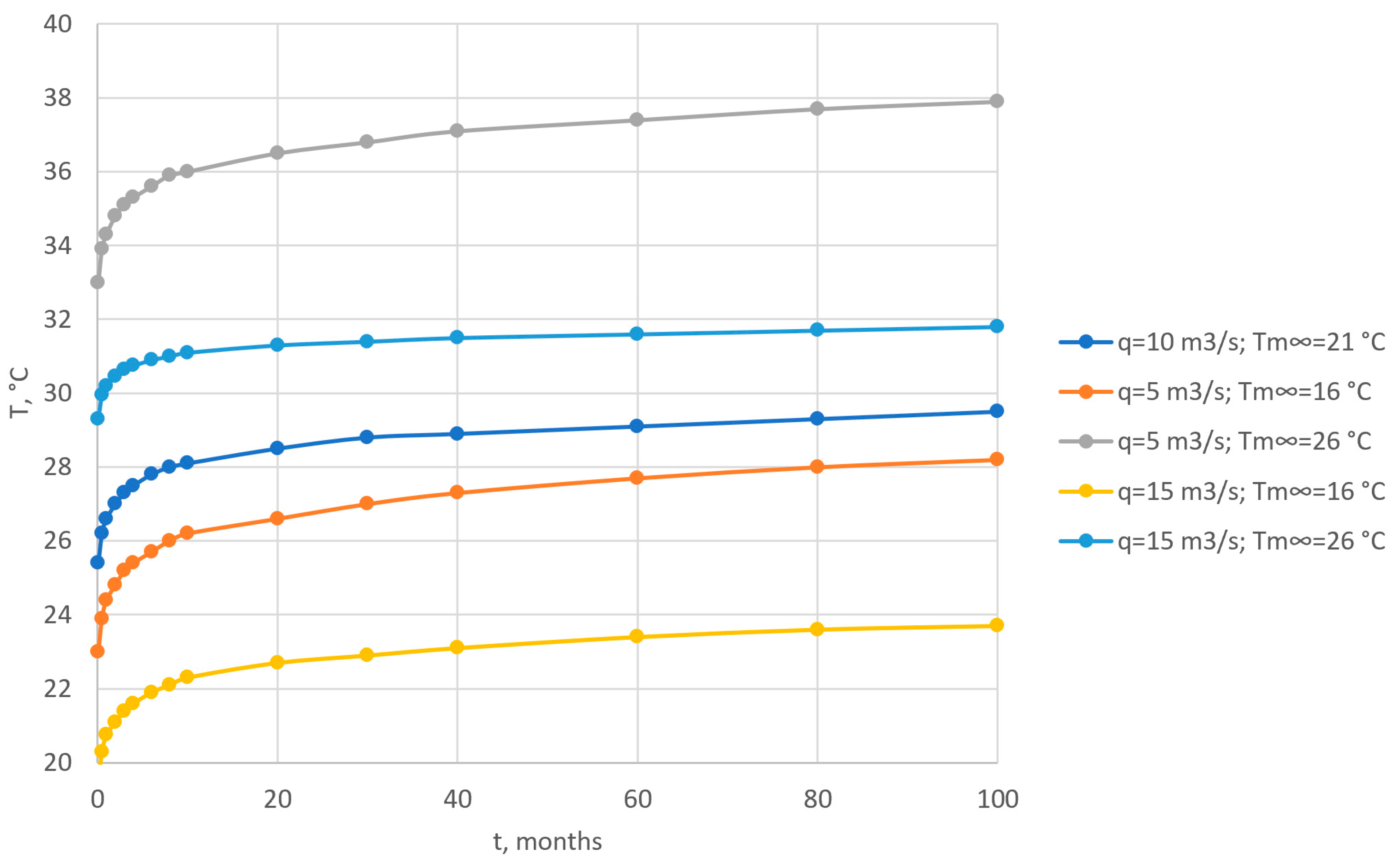
With the given parameters of the problem, when all the air goes along the conveyor roadway, its outlet temperature tends to +43 °C. This value is much higher than the outlet air temperature of +28.2 °C obtained in the calculation. Thus, the temperature in the excavation will continue to increase; however, the rate of increase will decrease yearly, as shown in
Figure 3. This is true for various ventilation parameters (
q) and thermal conditions (
).
Judging by the calculated data, even after 100 months from the beginning of mining operations in the longwall and the beginning of heat exchange, the air temperature at the end of the conveyor belt will not exceed 30 °C, which leads to the conclusion that in real-time intervals the heat exchange process is unsteady, the temperature increases at a decreasing rate, and the asymptotic solution will never be reached. In the first month from the beginning of heat exchange, the air temperature at the outlet of the conveyor drift is 27 °C; by the end of the year, it increases to 28 °C; after 4 years, up to 29 °C; after 8 years, it increases by another 0.5 °C, etc.