A New Rheological Model for Phosphate Slurry Flows
Abstract
:1. Introduction
2. Materials and Methods
2.1. The New Rheological Model
2.2. Numerical Modelling
2.2.1. Governing Equations
2.2.2. Turbulence Equations
3. Results and Discussions
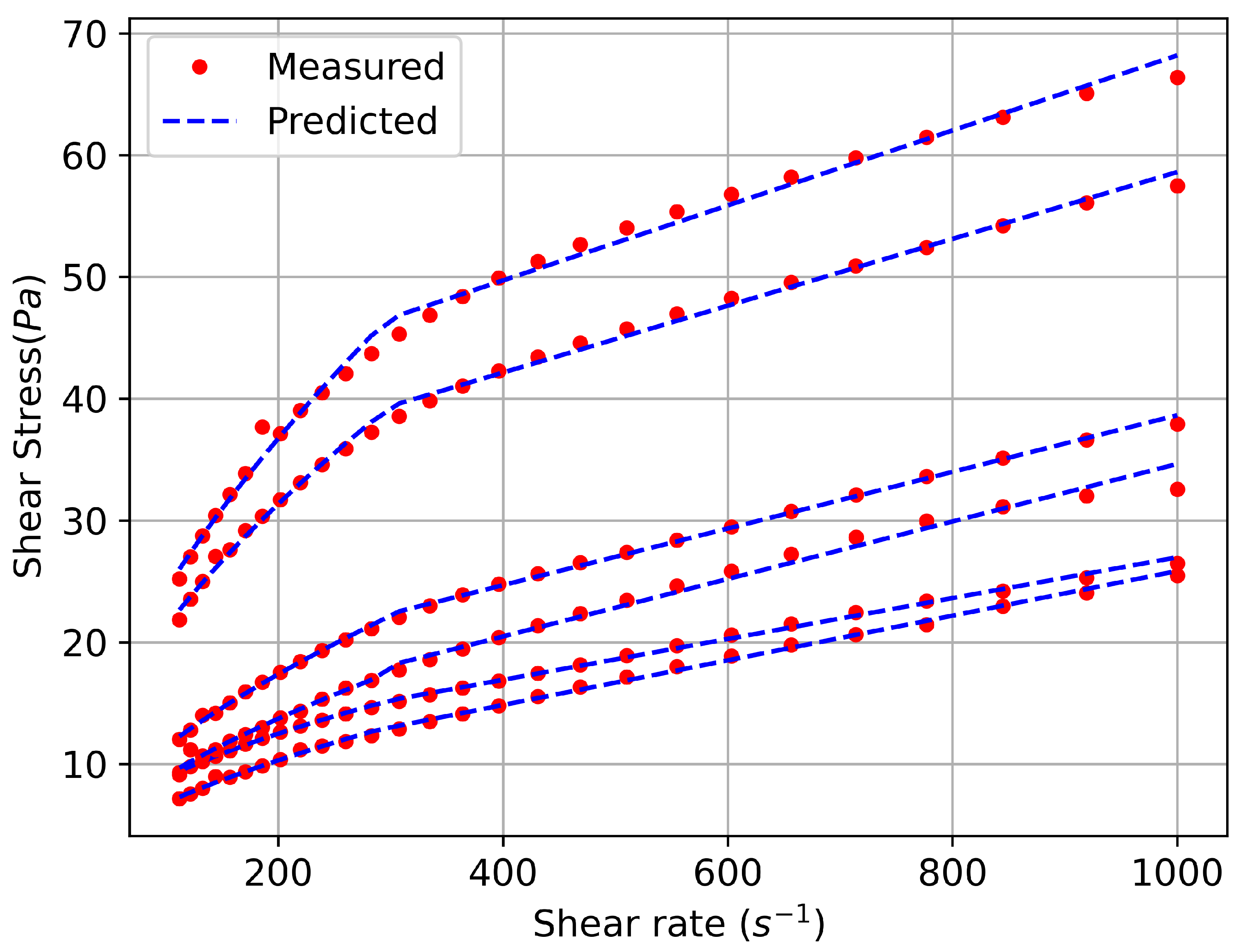
3.1. Rheological Evaluation of the New Model
3.2. Numerical Results
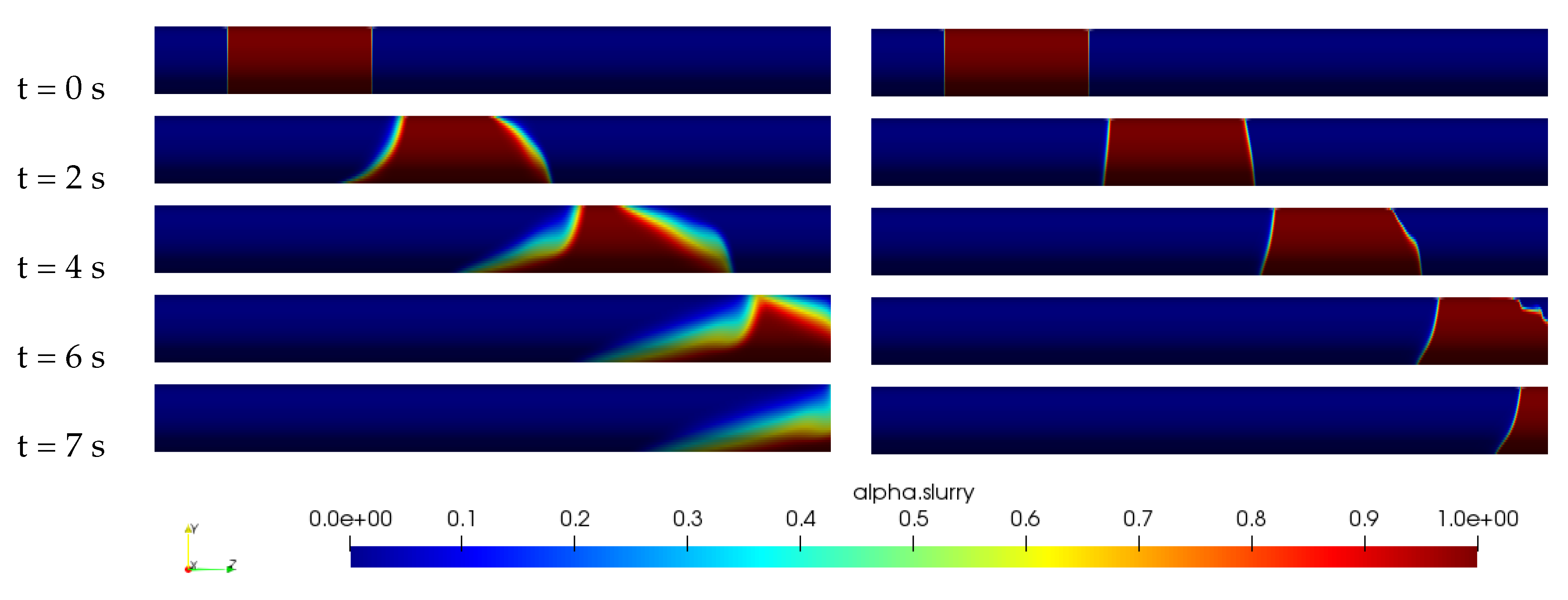
3.2.1. Model Implementation
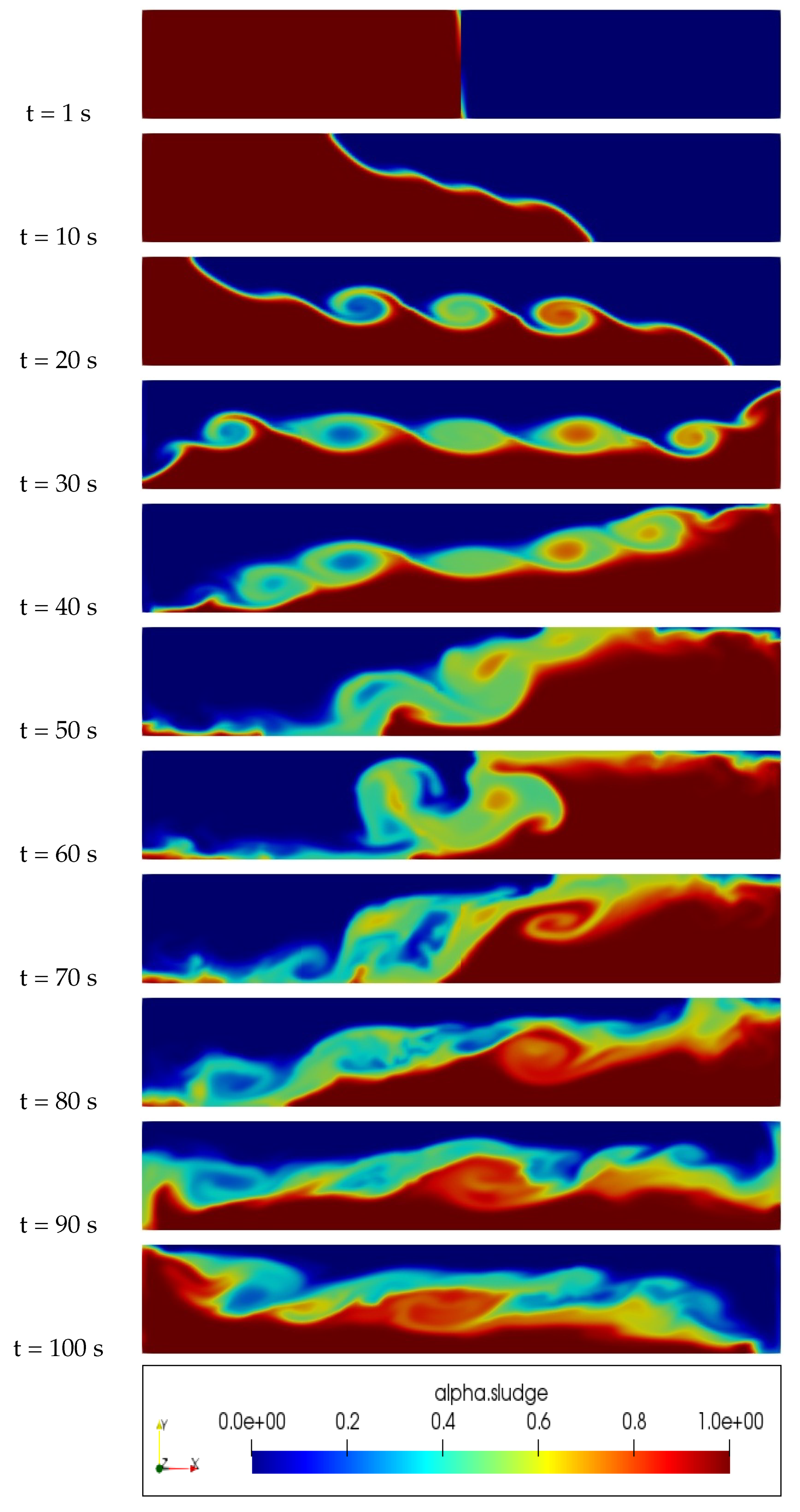
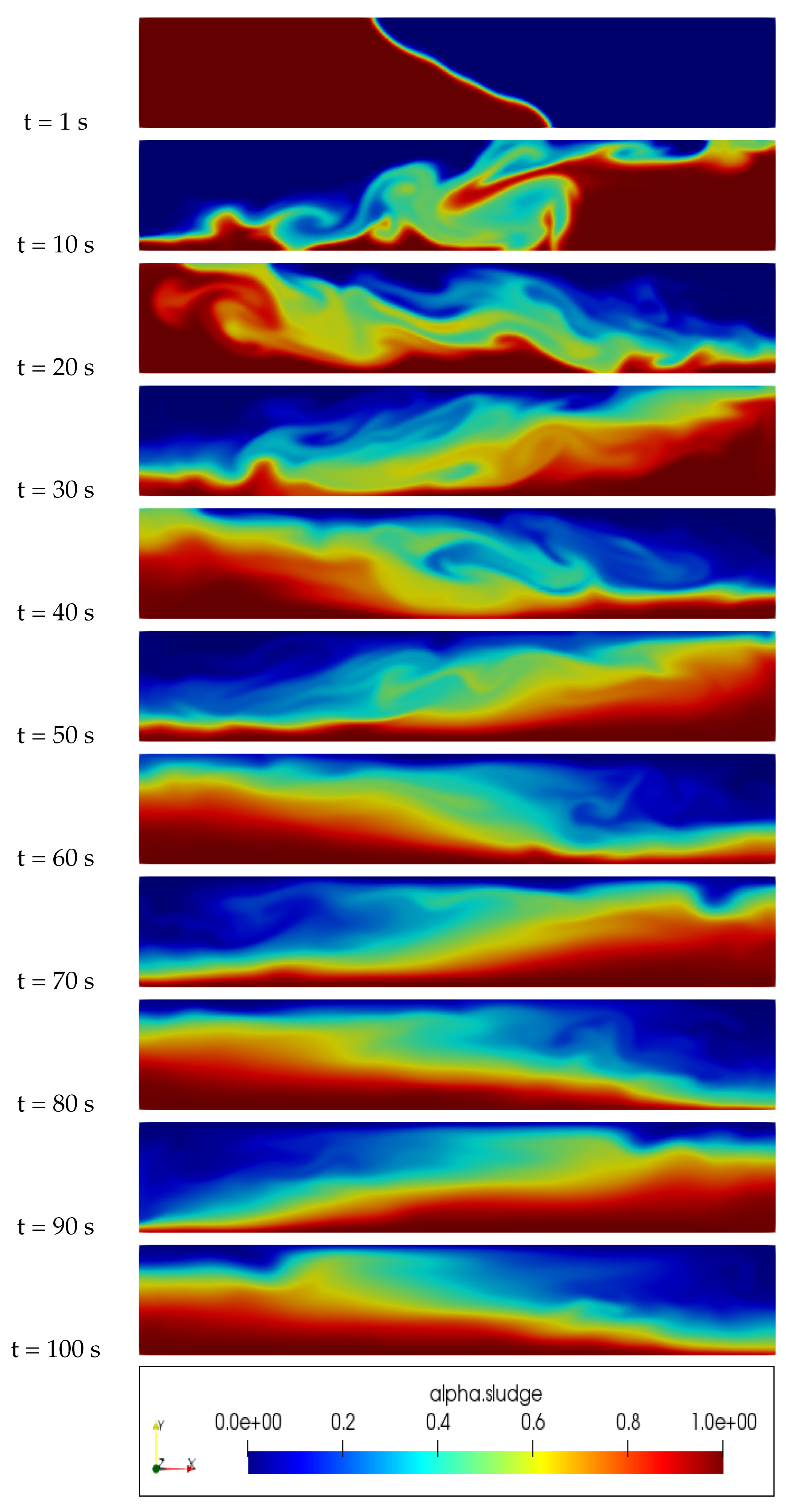
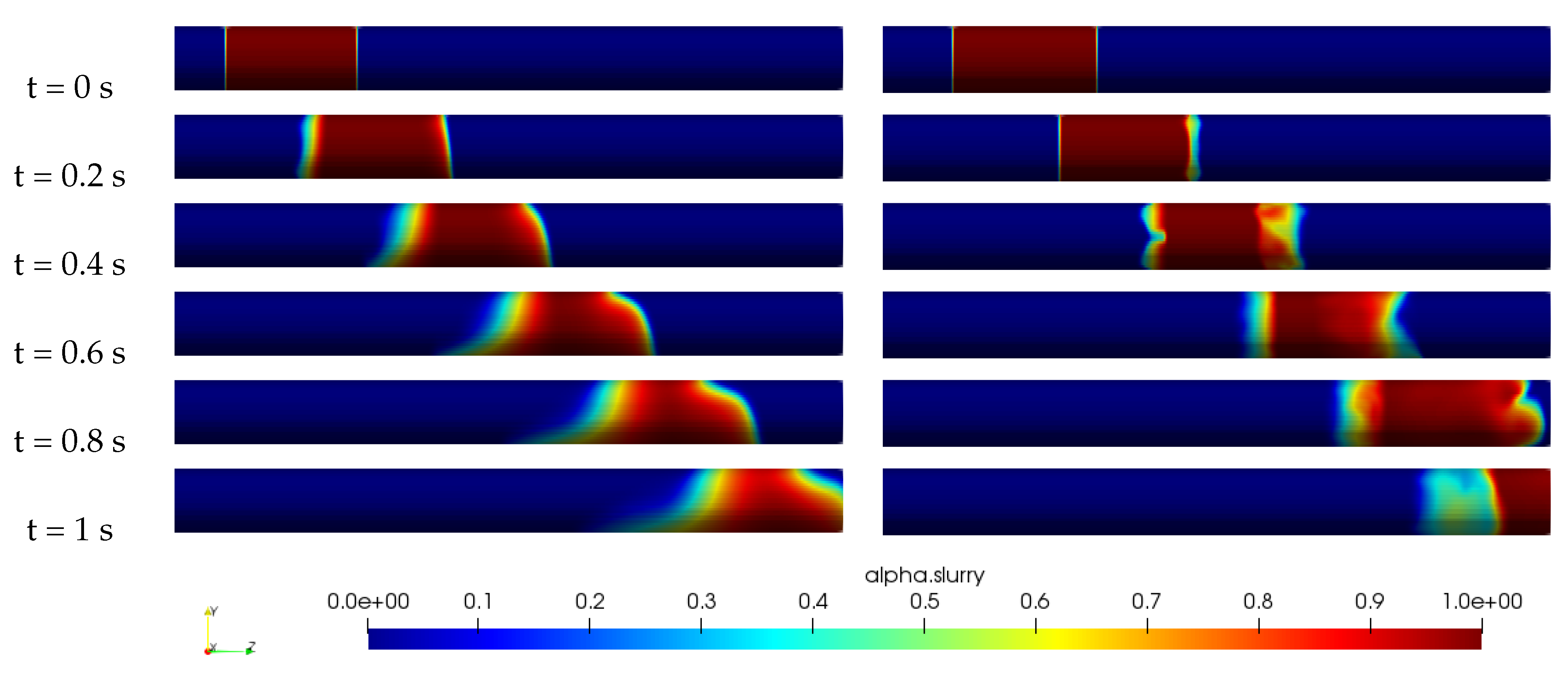
3.2.2. Two-Phase Pipe Flow
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bureš, L.; Sato, Y. Direct numerical simulation of evaporation and condensation with the geometric VOF method and a sharp-interface phase-change model. Int. J. Heat Mass Transf. 2021, 173, 121233. [Google Scholar] [CrossRef]
- Zhang, C.; Tan, J.; Ning, D. Machine learning strategy for viscous calibration of fully-nonlinear liquid sloshing simulation in FLNG tanks. Appl. Ocean. Res. 2021, 114, 102737. [Google Scholar] [CrossRef]
- Saghi, R.; Hirdaris, S.; Saghi, H. The influence of flexible fluid structure interactions on sway induced tank sloshing dynamics. Eng. Anal. Bound. Elem. 2021, 131, 206217. [Google Scholar] [CrossRef]
- Silvi, L.D.; Chandraker, D.K.; Ghosh, S.; Das, A.K. Understanding dry-out mechanism in rod bundles of boiling water reactor. Int. J. Heat Mass Transf. 2021, 177, 121534. [Google Scholar] [CrossRef]
- Vångö, M.; Pirker, S.; Lichtenegger, T. Unresolved CFD–DEM modeling of multiphase flow in densely packed particle beds. Appl. Math. Model. 2018, 56, 501–516. [Google Scholar] [CrossRef]
- Giussani, F.; Piscaglia, F.; Saez-Mischlich, G.; Helie, J. A three-phase VOF solver for the simulation of in-nozzle cavitation effects on liquid atomization. J. Comput. Phys. 2020, 406, 109068. [Google Scholar] [CrossRef]
- Di Iorio, S.; Catapano, F.; Magno, A.; Sementa, P.; Vaglieco, B.M. Investigation on sub-23 nm particles and their volatile organic fraction (VOF) in PFI/DI spark ignition engine fueled with gasoline, ethanol and a 30% v/v ethanol blend. J. Aerosol Sci. 2021, 153, 105723. [Google Scholar] [CrossRef]
- Sussman, M.; Smereka, P.; Osher, S. A level set approach for computing solutions to incompressible two-phase flow. J. Comput. Phys. 1994, 114, 146–159. [Google Scholar] [CrossRef]
- Hirt, C.W.; Nichols, B.D. Volume of fluid (VOF) method for the dynamics of free boundaries. J. Comput. Phys. 1981, 39, 201–225. [Google Scholar] [CrossRef]
- Kraposhin, M.V.; Banholzer, M.; Pfitzner, M.; Marchevsky, I. A hybrid pressure-based solver for nonideal single-phase fluid flows at all speeds. Int. J. Numer. Methods Fluids 2018, 88, 79–99. [Google Scholar] [CrossRef]
- Yu, C.-H.; Wen, H.L.; Gu, Z.H.; An, R.D. Numerical simulation of dam-break flow impacting a stationary obstacle by a CLSVOF/IB method. Commun. Nonlinear Sci. Numer. Simul. 2019, 79, 104934. [Google Scholar] [CrossRef]
- Jafari, E.; Namin, M.M.; Badiei, P. Numerical simulation of wave interaction with porous structures. Appl. Ocean. Res. 2021, 108, 102522. [Google Scholar] [CrossRef]
- Garoosi, F.; Mellado-Cusicahua, A.N.; Shademani, M.; Shakibaeinia, A. Experimental and numerical investigations of dam break flow over dry and wet beds. Int. J. Mech. Sci. 2022, 215, 106946. [Google Scholar] [CrossRef]
- Zhang, W.; Wang, J.; Yang, S.; Li, B.; Yu, K.; Wang, D.; Yongphet, P.; Xu, H. Dynamics of bubble formation on submerged capillaries in a non-uniform direct current electric field. Colloids Surfaces A Physicochem. Eng. Asp. 2020, 606, 125512. [Google Scholar] [CrossRef]
- Li, T.; Wang, S.; Li, S.; Zhang, A.-M. Numerical investigation of an underwater explosion bubble based on FVM and VOF. Appl. Ocean. Res. 2018, 74, 49–58. [Google Scholar] [CrossRef]
- Das, S.; Weerasiri, L.D.; Yang, W. Influence of surface tension on bubble nucleation, formation and onset of sliding. Colloids Surfaces A Physicochem. Eng. Asp. 2017, 516, 23–31. [Google Scholar] [CrossRef]
- Yang, C.; Cao, W.; Yang, Z. Study on dynamic behavior of water droplet impacting on super-hydrophobic surface with micro-pillar structures by VOF method. Colloids Surfaces A Physicochem. Eng. Asp. 2021, 603, 127634. [Google Scholar] [CrossRef]
- Hanene, Z.; Alla, H.; Abdelouahab, M.; Roques-Carmes, T. A numerical model of an immiscible surfactant drop spreading over thin liquid layers using CFD/VOF approach. Colloids Surfaces A Physicochem. Eng. Asp. 2020, 600, 124953. [Google Scholar] [CrossRef]
- de Lima, B.S.; Meira, L.d.; Souza, F.J.d. Numerical simulation of a water droplet splash: Comparison between PLIC and HRIC schemes for the VoF transport equation. Eur. J. -Mech.-B/Fluids 2020, 84, 63–70. [Google Scholar] [CrossRef]
- Yun, S. Ellipsoidal drop impact on a single-ridge superhydrophobic surface. Int. J. Mech. Sci. 2021, 208, 106677. [Google Scholar] [CrossRef]
- Kalifa, R.B.; Hamza, S.B.; Said, N.M.; Bournot, H. Fluid flow phenomena in metals processing operations: Numerical description of the fluid flow field by an impinging gas jet on a liquid surface. Int. J. Mech. Sci. 2020, 165, 105220. [Google Scholar] [CrossRef]
- Frigaard, I. Simple yield stress fluids. Curr. Opin. Colloid Interface Sci. 2019, 43, 80–93. [Google Scholar] [CrossRef]
- Bingham, E.C. Fluidity and Plasticity; McGraw-Hill: New York, NY, USA, 1922. [Google Scholar]
- Herschel, W.H.; Bulkley, R. Konsistenzmessungen von gummi-benzollösungen. Kolloid-Zeitschrift 1926, 39, 291–300. [Google Scholar] [CrossRef]
- Casson, N. A flow equation for pigment-oil suspensions of the printing ink type. Rheol. Disperse Syst. 1959, 84–104. [Google Scholar]
- Tanner, R.I. Rheology of noncolloidal suspensions with non-Newtonian matrices. J. Rheol. 2019, 63, 705–717. [Google Scholar] [CrossRef]
- Zhang, Z.; Ye, S.; Yin, B.; Song, X.; Wang, Y.; Huang, C.; Chen, Y. A semi-implicit discrepancy model of Reynolds stress in a higher-order tensor basis framework for Reynolds-averaged Navier–Stokes simulations. AIP Adv. 2021, 11, 045025. [Google Scholar] [CrossRef]
- Dai, S.-C.; Bertevas, E.; Qi, F.; Tanner, R.I. Viscometric functions for noncolloidal sphere suspensions with Newtonian matrices. J. Rheol. 2013, 57, 493–510. [Google Scholar] [CrossRef]
- Belbsir, H.; El-Hami, K.; Soufi, A. Study of the rheological behavior of the phosphate-water slurry and search for a suitable model to describe its rheological behavior. Int. J. Mech. Mechatron. Eng. IJMME-IJENS 2018, 18, 73–81. [Google Scholar]
- Zhang, S.; Jiang, B.; Law, A.W.; Zhao, B. Large eddy simulations of 45 inclined dense jets. Environ. Fluid Mech. 2016, 16, 101–121. [Google Scholar] [CrossRef]
- Krpan, R.; Končar, B. Simulation of turbulent wake at mixing of two confined horizontal flows. Sci. Technol. Nucl. Install. 2018, 2018, 5240361. [Google Scholar] [CrossRef]
- Jasak, H.; Jemcov, A.; Tukovic, Z. OpenFOAM: A C++ library for complex physics simulations. In Proceedings of the International Workshop on Coupled Methods in Numerical Dynamics, IUC, Dubrovnik, Croatia, 19–21 September 2007. [Google Scholar]
- Palmore, J., Jr.; Desjardins, O. A volume of fluid framework for interface-resolved simulations of vaporizing liquid-gas flows. J. Comput. Phys. 2019, 399, 108954. [Google Scholar] [CrossRef]
- Brackbill, J.U.; Kothe, D.B.; Zemach, C. A continuum method for modeling surface tension. J. Comput. Phys. 1992, 100, 335–354. [Google Scholar] [CrossRef]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Launder, B.E.; Spalding, D.B. The numerical computation of turbulent flows. In Numerical Prediction of Flow, Heat Transfer, Turbulence and Combustion; Elsevier: Amsterdam, The Netherlands, 1983; pp. 96–116. [Google Scholar]
- Henkes, R.A.W.M.; Van Der Vlugt, F.F.; Hoogendoorn, C.J. Natural-convection flow in a square cavity calculated with low-Reynolds-number turbulence models. Int. J. Heat Mass Transf. 1991, 34, 377–388. [Google Scholar] [CrossRef]
- Yakhot, V.; Smith, L.M. The renormalization group, the ϵ-expansion and derivation of turbulence models. J. Sci. Comput. 1992, 7, 35–61. [Google Scholar] [CrossRef]
- Menter, F.R. Turbulence Modeling for Engineering Flows; Ansys, Inc.: Canonsburg, PA, USA, 2011. [Google Scholar]
- Maazioui, S.; Maazouz, A.; Benkhaldoun, F.; Ouazar, D.; Lamnawar, K. Rheological Characterization of a Concentrated Phosphate Slurry. Fluids 2021, 6, 178. [Google Scholar] [CrossRef]
- Maazioui, S.; Kissami, I.; Benkhaldoun, F.; Ouazar, D. Numerical Study of Viscoplastic Flows Using a Multigrid Initialization Algorithm. Algorithms 2023, 16, 50. [Google Scholar] [CrossRef]
- Guadagni, S.; Palade, L.I.; Fusi, L.; Farina, A. On a Casson fluid motion: Nonuniform width symmetric channel and peristaltic flows. Fluids 2021, 6, 356. [Google Scholar] [CrossRef]




| Material | Density | Kinematic Viscosity | Rheological Model |
|---|---|---|---|
| (kg/m) | (m/s) | ||
| Water | 990 | Newtonian | |
| Sludge | 1000 | Newtonian | |
| non-constant | Herschel–Bulkley, Casson and New Model |
| Term | Details |
|---|---|
| Name of solver | twoLiquidMixingFoam |
| Type of solver | Density-based, segregated solver |
| Time dependency | Transient |
| Pressure-velocity coupling | Pimple |
| nCorrectors | 3 |
| nNonOrthogonalCorrector | 0 |
| Model | Phosphate Slurry Samples | |||||
|---|---|---|---|---|---|---|
| Parameters | S1 | S2 | S3 | S4 | S5 | S6 |
| a [Pa·s] | 0.93 | 0.44 | 0.72 | 0.57 | 1.60 | 1.56 |
| [mPa·s] | 16.77 | 18.33 | 23.26 | 23.6 | 27.45 | 30.8 |
| b [-] | 0.49 | 0.59 | 0.60 | 0.57 | 0.56 | 0.59 |
| [Pa] | 10.20 | 7.51 | 15.38 | 11.03 | 31.16 | 37.39 |
| [1/s] | 12.54 | 6.22 | 2.42 | 22.78 | 18.46 | 15.26 |
| Parameters | Ranges | Unit |
|---|---|---|
| Pipe diameter | 0.054–0.9 | m |
| Pipe Length | 3.3–50 | m |
| Solid concentration by mass | 38–56 | wt% |
| Water density | 1000 | kg/m |
| Water viscosity | Pa·s | |
| Velocity | 2–5 | m/s |
| Water Reynolds Number | > | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ghoudi, Z.; Maazioui, S.; Benkhaldoun, F.; Hajjaji, N. A New Rheological Model for Phosphate Slurry Flows. Fluids 2023, 8, 57. https://doi.org/10.3390/fluids8020057
Ghoudi Z, Maazioui S, Benkhaldoun F, Hajjaji N. A New Rheological Model for Phosphate Slurry Flows. Fluids. 2023; 8(2):57. https://doi.org/10.3390/fluids8020057
Chicago/Turabian StyleGhoudi, Zeineb, Souhail Maazioui, Fayssal Benkhaldoun, and Noureddine Hajjaji. 2023. "A New Rheological Model for Phosphate Slurry Flows" Fluids 8, no. 2: 57. https://doi.org/10.3390/fluids8020057
APA StyleGhoudi, Z., Maazioui, S., Benkhaldoun, F., & Hajjaji, N. (2023). A New Rheological Model for Phosphate Slurry Flows. Fluids, 8(2), 57. https://doi.org/10.3390/fluids8020057








