Modeling of Indirect Evaporative Cooling Systems: A Review
Abstract
:1. Introduction
2. IEC Systems
2.1. Analytical Models
2.2. Numerical Models
3. DIEC Systems
3.1. Analytical Models
3.2. Numerical Models
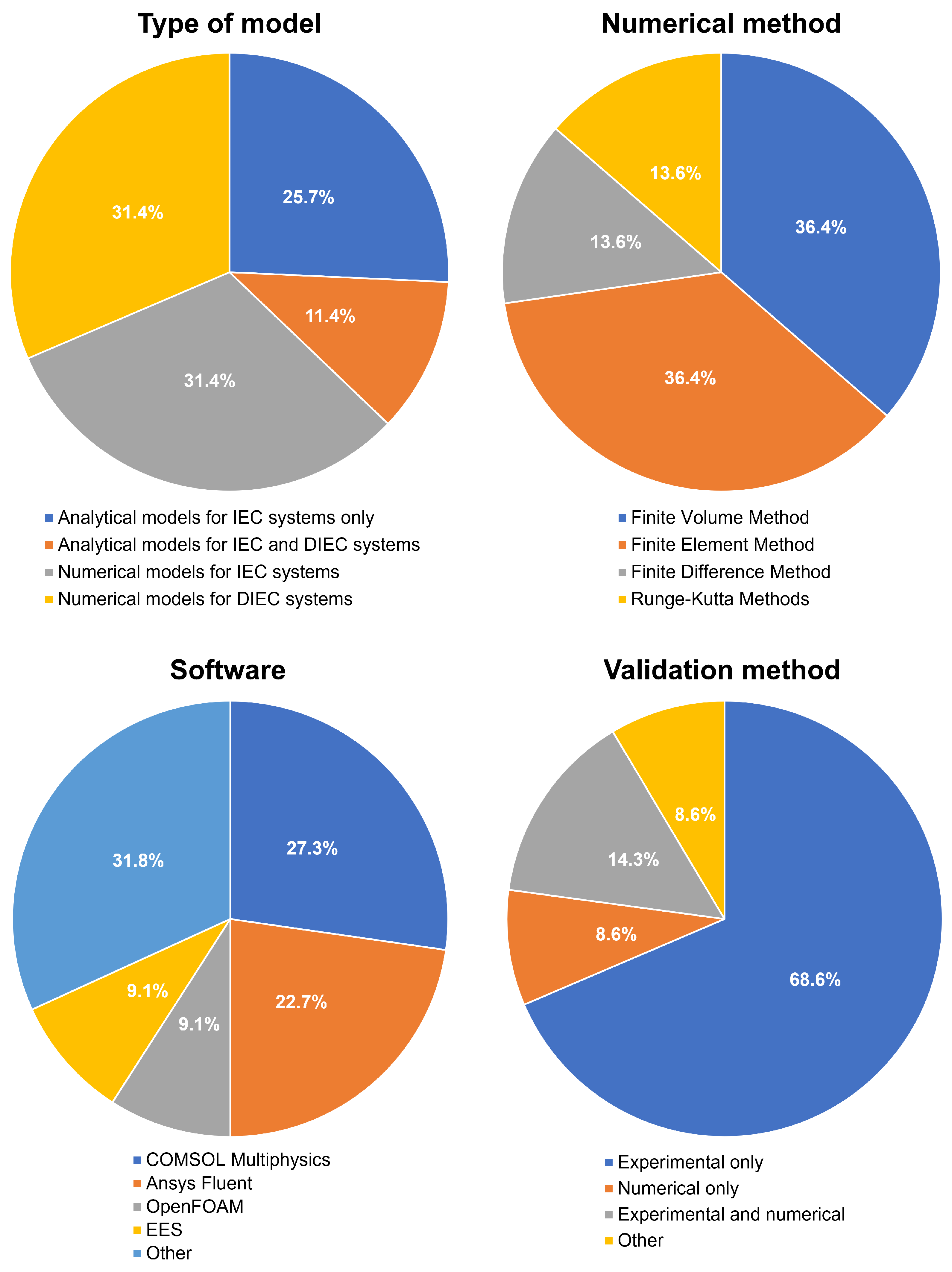
4. Results and Discussion
- Analytical models for IEC systems have been developed since the late 1990s, while numerical models have started to spread in recent years, as they require more computational power;
- Approximately of the models analyzed are analytical and were developed for IEC systems. About of these can also be used for DIEC systems;
- More than half of the analytical models considered can be used for all types of IEC systems, in any configuration;
- Half of the numerical models were developed specifically for IEC systems, the other half for DIEC systems;
- Numerical models for IEC heat exchangers are mainly developed for cross-flow systems, while the great majority of the numerical models for DIEC heat exchangers are developed for counter-flow systems;
- The favorite discretization methods for numerical models were the FVM ( of the cases) and the FEM (), followed by the FDM () and the RK ();
- The most common software tools used for the numerical simulations were COMSOL Multiphysics (about of the cases) and Ansys Fluent (about );
- Most of the numerical models presented were developed for steady-state conditions, with a majority of 2-D models, but a significant presence of 3-D models as well;
- Almost all the models were validated against experimental data and/or numerical results, which were acquired from literature or by the authors;
- The majority of the analyzed models are able to predict the performance parameters with an error within ;
- The validations were performed by comparison with numerical and/or experimental data in a few test cases, so in real applications it might be difficult to associate the models boundary conditions and the heat exchangers operating conditions;
- Another common limitation concerns the modeling of the wettability factor, which is often assumed to be unitary, and of the air density, which is often assumed to be constant, thus potentially reducing the accuracy of the results in some cases.
5. Conclusions and Future Developments
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| 1-D | One-dimensional |
| 2-D | Two-dimensional |
| 3-D | Three-dimensional |
| CFD | Computational Fluid Dynamics |
| DEC | Direct Evaporative Cooling |
| DIEC | Dew-point Indirect Evaporative Cooling |
| DM | Detailed Model |
| EES | Engineering Equation Solver |
| FDM | Finite Difference Method |
| FEM | Finite Element Method |
| FVM | Finite Volume Method |
| HVAC | Heating, Ventilation, and Air Conditioning |
| IEC | Indirect Evaporative Cooling |
| LMTD | Logarithmic Mean Temperature Difference |
| M-cycle | Maisotsenko cycle |
| NTU | Number of Transfer Units |
| RK | Runge-Kutta methods |
| RK4 | Forth-order Runge-Kutta method |
| RKM | Modified Runge-Kutta method |
| SM | Simplified Model |
| Specific heat at constant pressure of the primary air | |
| Coefficient Of Performance | |
| Primary air mass flow rate | |
| Cooling capacity | |
| Primary air inlet temperature | |
| Primary air outlet temperature | |
| Dew-point temperature of the secondary air at the inlet | |
| Wet-bulb temperature of the secondary air at the inlet | |
| Mechanical power | |
| Effectiveness | |
| Dew-point effectiveness | |
| Wet-bulb effectiveness |
References
- Sajjad, U.; Abbas, N.; Hamid, K.; Abbas, S.; Hussain, I.; Ammar, S.M.; Sultan, M.; Ali, H.M.; Hussain, M.; Wang, C.C.; et al. A review of recent advances in indirect evaporative cooling technology. Int. Commun. Heat Mass Transf. 2021, 122, 105–140. [Google Scholar] [CrossRef]
- Caruana, R.; De Antonellis, S.; Marocco, L.; Liberati, P.; Guilizzoni, M. Experimental Characterization of the Wettability of Coated and Uncoated Plates for Indirect Evaporative Cooling Systems. Fluids 2023, 8, 122. [Google Scholar] [CrossRef]
- Porumb, B.; Ungureşan, P.; Tutunaru, L.F.; Şerban, A.; Bălan, M. A review of indirect evaporative cooling technology. Energy Procedia 2016, 85, 461–471. [Google Scholar] [CrossRef]
- Zhao, X.; Liu, S.; Riffat, S.B. Comparative study of heat and mass exchanging materials for indirect evaporative cooling systems. Build. Environ. 2008, 43, 1902–1911. [Google Scholar] [CrossRef]
- Hussain, I.; Bibi, F.; Bhat, S.A.; Sajjad, U.; Sultan, M.; Ali, H.M.; Azam, W.; Kaushal, S.K.; Hussain, S.; Yan, W.M. Evaluating the parameters affecting the direct and indirect evaporative cooling systems. Eng. Anal. Bound. Elem. 2022, 145, 211–223. [Google Scholar] [CrossRef]
- Costelloe, B.; Finn, D. Indirect evaporative cooling potential in air–water systems in temperate climates. Energy Build. 2003, 35, 573–591. [Google Scholar] [CrossRef]
- Raza, H.M.; Sultan, M.; Bahrami, M.; Khan, A.A. Experimental investigation of evaporative cooling systems for agricultural storage and livestock air-conditioning in Pakistan. Build. Simul. 2021, 14, 617–631. [Google Scholar] [CrossRef]
- Misra, D.; Ghosh, S. Evaporative cooling technologies for greenhouses: A comprehensive review. Agric. Eng. Int. Cigr J. 2018, 20, 1–15. [Google Scholar]
- Testa, C.J.; Shvedova, K.; Hu, C.; Wu, W.; Born, S.C.; Takizawa, B.; Mascia, S. Heterogeneous crystallization as a process intensification technology in an integrated continuous manufacturing process for pharmaceuticals. Org. Process Res. Dev. 2021, 25, 225–238. [Google Scholar] [CrossRef]
- Lv, J.; Xu, H.; Zhu, M.; Dai, Y.; Liu, H.; Li, Z. The performance and model of porous materials in the indirect evaporative cooling system: A review. J. Build. Eng. 2021, 41, 102741. [Google Scholar] [CrossRef]
- Glanville, P.; Kozlov, A.; Maisotsenko, V. Dew point evaporative cooling: Technology review and fundamentals. Ashrae Trans. 2011, 117, 117–125. [Google Scholar]
- Duan, Z.; Zhan, C.; Zhang, X.; Mustafa, M.; Zhao, X.; Alimohammadisagvand, B.; Hasan, A. Indirect evaporative cooling: Past, present and future potentials. Renew. Sustain. Energy Rev. 2012, 16, 6823–6850. [Google Scholar] [CrossRef]
- Wani, C.; Ghodke, S.; Shrivastava, C. A review on potential of Maisotsenko cycle in energy saving applications using evaporative cooling. Int. J. Adv. Res. Sci. Eng. Technol. 2012, 1, 15–20. [Google Scholar]
- Amer, O.; Boukhanouf, R.; Ibrahim, H.G. A review of evaporative cooling technologies. Int. J. Environ. Sci. Dev. 2015, 6, 111. [Google Scholar] [CrossRef]
- Cuce, P.M.; Riffat, S. A state of the art review of evaporative cooling systems for building applications. Renew. Sustain. Energy Rev. 2016, 54, 1240–1249. [Google Scholar] [CrossRef]
- Mahmood, M.H.; Sultan, M.; Miyazaki, T.; Koyama, S.; Maisotsenko, V.S. Overview of the Maisotsenko cycle—A way towards dew point evaporative cooling. Renew. Sustain. Energy Rev. 2016, 66, 537–555. [Google Scholar] [CrossRef]
- Yang, Y.; Cui, G.; Lan, C.Q. Developments in evaporative cooling and enhanced evaporative cooling—A review. Renew. Sustain. Energy Rev. 2019, 113, 109230. [Google Scholar] [CrossRef]
- Sofia, E.; Putra, N. Evaporative cooling innovations—A review. AIP Conf. Proc. 2020, 2255, 020036. [Google Scholar]
- Pacak, A.; Worek, W. Review of dew point evaporative cooling technology for air conditioning applications. Appl. Sci. 2021, 11, 934. [Google Scholar] [CrossRef]
- Yang, H.; Shi, W.; Chen, Y.; Min, Y. Research development of indirect evaporative cooling technology: An updated review. Renew. Sustain. Energy Rev. 2021, 145, 111082. [Google Scholar] [CrossRef]
- Zhu, G.; Wen, T.; Wang, Q.; Xu, X. A review of dew-point evaporative cooling: Recent advances and future development. Appl. Energy 2022, 312, 118785. [Google Scholar] [CrossRef]
- Kapilan, N.; Isloor, A.M.; Karinka, S. A comprehensive review on evaporative cooling systems. Results Eng. 2023, 18, 101059. [Google Scholar] [CrossRef]
- Pandelidis, D.; Cichoń, A.; Pacak, A.; Anisimov, S.; Drąg, P. Performance comparison between counter-and cross-flow indirect evaporative coolers for heat recovery in air conditioning systems in the presence of condensation in the product air channels. Int. J. Heat Mass Transf. 2019, 130, 757–777. [Google Scholar] [CrossRef]
- Vali, A.; Simonson, C.J.; Besant, R.W.; Mahmood, G. Numerical model and effectiveness correlations for a run-around heat recovery system with combined counter and cross flow exchangers. Int. J. Heat Mass Transf. 2009, 52, 5827–5840. [Google Scholar] [CrossRef]
- Alonso, J.S.J.; Martinez, F.R.; Gomez, E.V.; Plasencia, M.A.G. Simulation model of an indirect evaporative cooler. Energy Build. 1998, 29, 23–27. [Google Scholar] [CrossRef]
- Stoitchkov, N.; Dimitrov, G. Effectiveness of crossflow plate heat exchanger for indirect evaporative cooling: Efficacité des échangeurs thermiques à plaques, à courants croises pour refroidissement indirect évaporatif. Int. J. Refrig. 1998, 21, 463–471. [Google Scholar] [CrossRef]
- Maclaine-Cross, I.; Banks, P. A general theory of wet surface heat exchangers and its application to regenerative evaporative cooling. J. Heat Transf. 1981, 103, 579–585. [Google Scholar] [CrossRef]
- Ren, C.; Yang, H. An analytical model for the heat and mass transfer processes in indirect evaporative cooling with parallel/counter flow configurations. Int. J. Heat Mass Transf. 2006, 49, 617–627. [Google Scholar]
- Heidarinejad, G.; Bozorgmehr, M. Modelling of indirect evaporative air coolers. In Proceedings of the 2nd PALENC Conference and 28th AIVC Conference on Building Low Energy Cooling and Advanced Ventilation Technologies in the 21st Century, Crete Island, Greece, 27–29 September 2007; Citeseer: University Park, PA, USA, 2007. [Google Scholar]
- Hasan, A. Going below the wet-bulb temperature by indirect evaporative cooling: Analysis using a modified ε-NTU method. Appl. Energy 2012, 89, 237–245. [Google Scholar] [CrossRef]
- Liu, Z.; Allen, W.; Modera, M. Simplified thermal modeling of indirect evaporative heat exchangers. HVAC&R Res. 2013, 19, 257–267. [Google Scholar]
- Cui, X.; Chua, K.; Islam, M.; Yang, W. Fundamental formulation of a modified LMTD method to study indirect evaporative heat exchangers. Energy Convers. Manag. 2014, 88, 372–381. [Google Scholar] [CrossRef]
- Chen, Y.; Luo, Y.; Yang, H. A simplified analytical model for indirect evaporative cooling considering condensation from fresh air: Development and application. Energy Build. 2015, 108, 387–400. [Google Scholar] [CrossRef]
- Heidarinejad, G.; Moshari, S. Novel modeling of an indirect evaporative cooling system with cross-flow configuration. Energy Build. 2015, 92, 351–362. [Google Scholar] [CrossRef]
- De Antonellis, S.; Joppolo, C.M.; Liberati, P.; Milani, S.; Romano, F. Modeling and experimental study of an indirect evaporative cooler. Energy Build. 2017, 142, 147–157. [Google Scholar] [CrossRef]
- Liberati, P.; De Antonellis, S.; Leone, C.; Joppolo, C.M.; Bawa, Y. Indirect Evaporative cooling systems: Modelling and performance analysis. Energy Procedia 2017, 140, 475–485. [Google Scholar] [CrossRef]
- Moshari, S.; Heidarinejad, G. Analytical estimation of pressure drop in indirect evaporative coolers for power reduction. Energy Build. 2017, 150, 149–162. [Google Scholar] [CrossRef]
- Comino, F.; Milani, S.; De Antonellis, S.; Joppolo, C.M.; de Adana, M.R. Simplified performance correlation of an indirect evaporative cooling system: Development and validation. Int. J. Refrig. 2018, 88, 307–317. [Google Scholar] [CrossRef]
- Zheng, B.; Guo, C.; Chen, T.; Shi, Q.; Lv, J.; You, Y. Development of an experimental validated model of cross-flow indirect evaporative cooler with condensation. Appl. Energy 2019, 252, 113438. [Google Scholar] [CrossRef]
- Wan, Y.; Ren, C.; Xing, L. An approach to the analysis of heat and mass transfer characteristics in indirect evaporative cooling with counter flow configurations. Int. J. Heat Mass Transf. 2017, 108, 1750–1763. [Google Scholar] [CrossRef]
- You, Y.; Jiang, H.; Lv, J. Analysis of influence of IEC heat exchanger based on CFD method. Energy Procedia 2019, 158, 5759–5764. [Google Scholar] [CrossRef]
- Min, Y.; Chen, Y.; Yang, H. Numerical study on indirect evaporative coolers considering condensation: A thorough comparison between cross flow and counter flow. Int. J. Heat Mass Transf. 2019, 131, 472–486. [Google Scholar] [CrossRef]
- Wan, Y.; Soh, A.; Shao, Y.; Cui, X.; Tang, Y.; Chua, K.J. Numerical study and correlations for heat and mass transfer coefficients in indirect evaporative coolers with condensation based on orthogonal test and CFD approach. Int. J. Heat Mass Transf. 2020, 153, 119580. [Google Scholar] [CrossRef]
- Guo, C.; Liu, Q.; Zheng, B.; You, Y.; Li, Y. Development of model based on condensation area ratio and effect on heat transfer capacity of indirect evaporative cooling. Appl. Therm. Eng. 2020, 164, 114557. [Google Scholar] [CrossRef]
- Adam, A.; Han, D.; He, W.; Chen, J. Numerical analysis of cross-flow plate type indirect evaporative cooler: Modeling and parametric analysis. Appl. Therm. Eng. 2021, 185, 116379. [Google Scholar] [CrossRef]
- Shi, W.; Min, Y.; Chen, Y.; Yang, H. Development of a three-dimensional numerical model of indirect evaporative cooler incorporating with air dehumidification. Int. J. Heat Mass Transf. 2022, 185, 122316. [Google Scholar] [CrossRef]
- Adam, A.; Han, D.; He, W.; Amidpour, M.; Zhong, H. Numerical investigation of the heat and mass transfer process within a cross-flow indirect evaporative cooling system for hot and humid climates. J. Build. Eng. 2022, 45, 103499. [Google Scholar] [CrossRef]
- Pacak, A.; Baran, B.; Sierpowski, K.; Malecha, Z.; Pandelidis, D. Application of computational fluid dynamics (CFD) methods to analyze energy efficiency of indirect evaporative coolers. Int. Commun. Heat Mass Transf. 2023, 143, 106727. [Google Scholar] [CrossRef]
- Adam, A.; Han, D.; He, W.; Shi, Q.; Chen, J.; Zhong, H. The influences of the plate shape on the performance of the indirect evaporative cooler based on the CFD approach. J. Braz. Soc. Mech. Sci. Eng. 2023, 45, 123. [Google Scholar] [CrossRef]
- Ma, X.; Shi, W.; Yang, H. Improving the performance of indirect evaporative cooler for energy recovery from the perspective of nozzle configuration: A CFD model analysis. J. Build. Eng. 2023, 76, 107195. [Google Scholar] [CrossRef]
- Rogdakis, E.D.; Tertipis, D.N. Maisotsenko cycle: Technology overview and energy-saving potential in cooling systems. Energy Emiss. Control Technol. 2015, 3, 15–22. [Google Scholar]
- Caliskan, H.; Hepbasli, A.; Dincer, I.; Maisotsenko, V. Thermodynamic performance assessment of a novel air cooling cycle: Maisotsenko cycle. Int. J. Refrig. 2011, 34, 980–990. [Google Scholar] [CrossRef]
- Anisimov, S.; Pandelidis, D.; Jedlikowski, A.; Polushkin, V. Performance investigation of a M (Maisotsenko)-cycle cross-flow heat exchanger used for indirect evaporative cooling. Energy 2014, 76, 593–606. [Google Scholar] [CrossRef]
- Zanchini, E.; Naldi, C. Energy saving obtainable by applying a commercially available M-cycle evaporative cooling system to the air conditioning of an office building in North Italy. Energy 2019, 179, 975–988. [Google Scholar] [CrossRef]
- Riangvilaikul, B.; Kumar, S. An experimental study of a novel dew point evaporative cooling system. Energy Build. 2010, 42, 637–644. [Google Scholar] [CrossRef]
- Bruno, F. On-site experimental testing of a novel dew point evaporative cooler. Energy Build. 2011, 43, 3475–3483. [Google Scholar] [CrossRef]
- Xu, P.; Ma, X.; Zhao, X.; Fancey, K. Experimental investigation of a super performance dew point air cooler. Appl. Energy 2017, 203, 761–777. [Google Scholar] [CrossRef]
- Chu, J.; Xu, W.; Fu, Y.; Huo, H. Experimental research on the cooling performance of a new regenerative dew point indirect evaporative cooler. J. Build. Eng. 2021, 43, 102921. [Google Scholar] [CrossRef]
- Yang, Y.; Ren, C.; Yang, C.; Tu, M.; Luo, B.; Fu, J. Energy and exergy performance comparison of conventional, dew point and new external-cooling indirect evaporative coolers. Energy Convers. Manag. 2021, 230, 113824. [Google Scholar] [CrossRef]
- Sun, T.; Huang, X.; Liang, C.; Liu, R.; Huang, X. Prediction and analysis of dew point indirect evaporative cooler performance by artificial neural network method. Energies 2022, 15, 4673. [Google Scholar] [CrossRef]
- Jia, L.; Liu, J.; Wang, C.; Cao, X.; Zhang, Z. Study of the thermal performance of a novel dew point evaporative cooler. Appl. Therm. Eng. 2019, 160, 114069. [Google Scholar] [CrossRef]
- Ali, M.; Ahmad, W.; Sheikh, N.A.; Ali, H.; Kousar, R.; ur Rashid, T. Performance enhancement of a cross flow dew point indirect evaporative cooler with circular finned channel geometry. J. Build. Eng. 2021, 35, 101980. [Google Scholar] [CrossRef]
- Kousar, R.; Ali, M.; Sheikh, N.A.; Gilani, S.I.U.H.; Khushnood, S. Holistic integration of multi-stage dew point counter flow indirect evaporative cooler with the solar-assisted desiccant cooling system: A techno-economic evaluation. Energy Sustain. Dev. 2021, 62, 163–174. [Google Scholar] [CrossRef]
- Güzelel, Y.E.; Olmuş, U.; Büyükalaca, O. Simulation of a desiccant air-conditioning system integrated with dew-point indirect evaporative cooler for a school building. Appl. Therm. Eng. 2022, 217, 119233. [Google Scholar] [CrossRef]
- Zhao, X.; Li, J.; Riffat, S. Numerical study of a novel counter-flow heat and mass exchanger for dew point evaporative cooling. Appl. Therm. Eng. 2008, 28, 1942–1951. [Google Scholar] [CrossRef]
- Riangvilaikul, B.; Kumar, S. Numerical study of a novel dew point evaporative cooling system. Energy Build. 2010, 42, 2241–2250. [Google Scholar] [CrossRef]
- Zhan, C.; Zhao, X.; Smith, S.; Riffat, S. Numerical study of a M-cycle cross-flow heat exchanger for indirect evaporative cooling. Build. Environ. 2011, 46, 657–668. [Google Scholar] [CrossRef]
- Cui, X.; Chua, K.; Yang, W. Numerical simulation of a novel energy-efficient dew-point evaporative air cooler. Appl. Energy 2014, 136, 979–988. [Google Scholar] [CrossRef]
- Anisimov, S.; Pandelidis, D. Numerical study of the Maisotsenko cycle heat and mass exchanger. Int. J. Heat Mass Transf. 2014, 75, 75–96. [Google Scholar] [CrossRef]
- Anisimov, S.; Pandelidis, D.; Danielewicz, J. Numerical analysis of selected evaporative exchangers with the Maisotsenko cycle. Energy Convers. Manag. 2014, 88, 426–441. [Google Scholar] [CrossRef]
- Pandelidis, D.; Anisimov, S.; Worek, W.M. Performance study of the Maisotsenko Cycle heat exchangers in different air-conditioning applications. Int. J. Heat Mass Transf. 2015, 81, 207–221. [Google Scholar] [CrossRef]
- Pandelidis, D.; Anisimov, S.; Worek, W.M.; Drąg, P. Numerical analysis of a desiccant system with cross-flow Maisotsenko cycle heat and mass exchanger. Energy Build. 2016, 123, 136–150. [Google Scholar] [CrossRef]
- Anisimov, S.; Pandelidis, D.; Maisotsenko, V. Numerical study of heat and mass transfer process in the Maisotsenko cycle for indirect evaporative air cooling. Heat Transf. Eng. 2016, 37, 1455–1465. [Google Scholar] [CrossRef]
- Jafarian, H.; Sayyaadi, H.; Torabi, F. A numerical model for a dew-point counter-flow indirect evaporative cooler using a modified boundary condition and considering effects of entrance regions. Int. J. Refrig. 2017, 84, 36–51. [Google Scholar] [CrossRef]
- Wan, Y.; Lin, J.; Chua, K.J.; Ren, C. A new method for prediction and analysis of heat and mass transfer in the counter-flow dew point evaporative cooler under diverse climatic, operating and geometric conditions. Int. J. Heat Mass Transf. 2018, 127, 1147–1160. [Google Scholar] [CrossRef]
- Wan, Y.; Lin, J.; Chua, K.J.; Ren, C. Similarity analysis and comparative study on the performance of counter-flow dew point evaporative coolers with experimental validation. Energy Convers. Manag. 2018, 169, 97–110. [Google Scholar] [CrossRef]
- Lin, J.; Bui, D.T.; Wang, R.; Chua, K.J. On the fundamental heat and mass transfer analysis of the counter-flow dew point evaporative cooler. Appl. Energy 2018, 217, 126–142. [Google Scholar] [CrossRef]
- Pakari, A.; Ghani, S. Comparison of 1D and 3D heat and mass transfer models of a counter flow dew point evaporative cooling system: Numerical and experimental study. Int. J. Refrig. 2019, 99, 114–125. [Google Scholar] [CrossRef]
- Pakari, A.; Ghani, S. Regression models for performance prediction of counter flow dew point evaporative cooling systems. Energy Convers. Manag. 2019, 185, 562–573. [Google Scholar] [CrossRef]
- Liu, Y.; Li, J.M.; Yang, X.; Zhao, X. Two-dimensional numerical study of a heat and mass exchanger for a dew-point evaporative cooler. Energy 2019, 168, 975–988. [Google Scholar] [CrossRef]
- Comino, F.; Romero-Lara, M.J.; de Adana, M.R. Experimental and numerical study of dew-point indirect evaporative coolers to optimize performance and design. Int. J. Refrig. 2022, 142, 92–102. [Google Scholar] [CrossRef]
- Zhu, G.; Chen, W.; Zhang, D.; Wen, T. Performance evaluation of counter flow dew-point evaporative cooler with a three-dimensional numerical model. Appl. Therm. Eng. 2023, 219, 119483. [Google Scholar] [CrossRef]
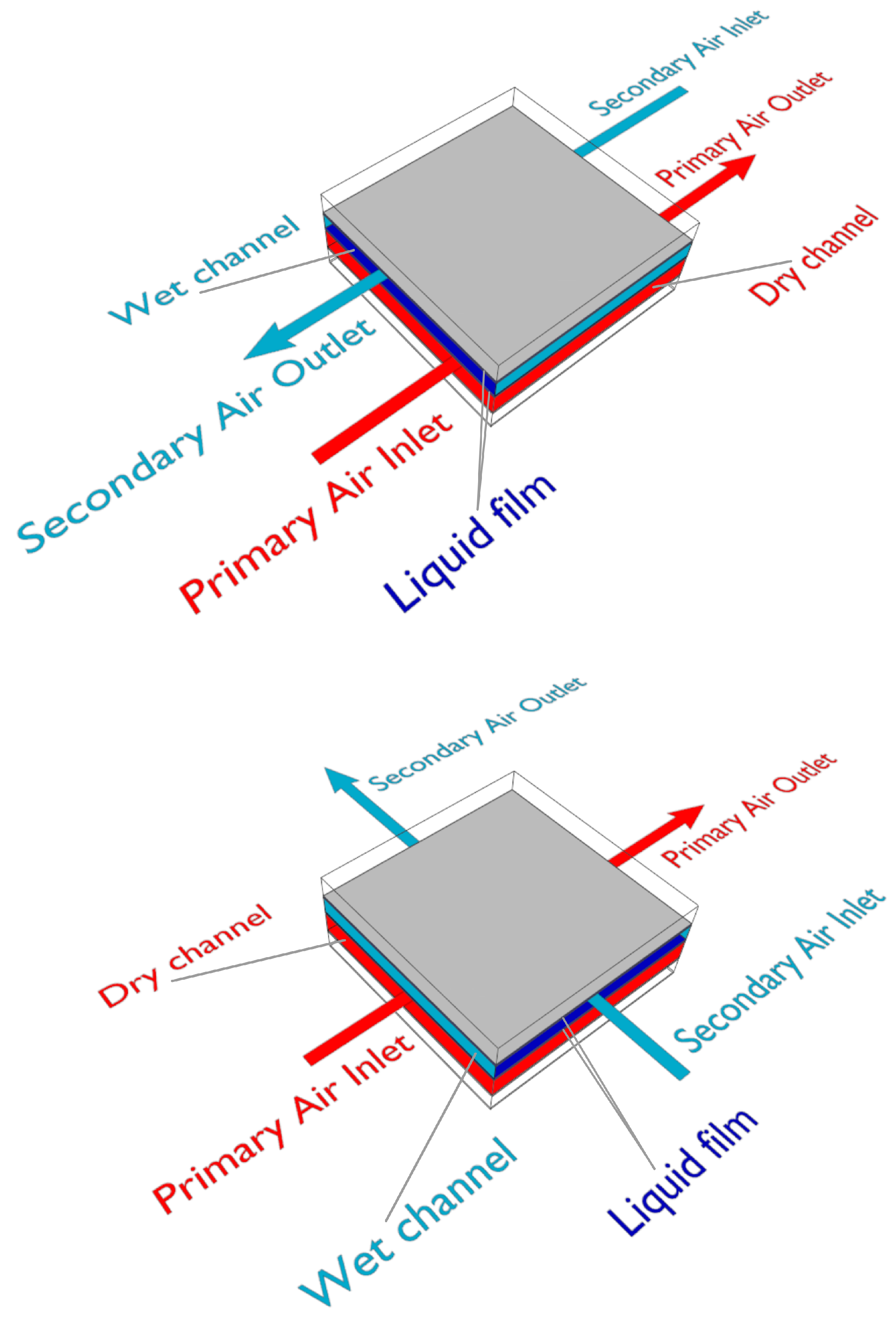
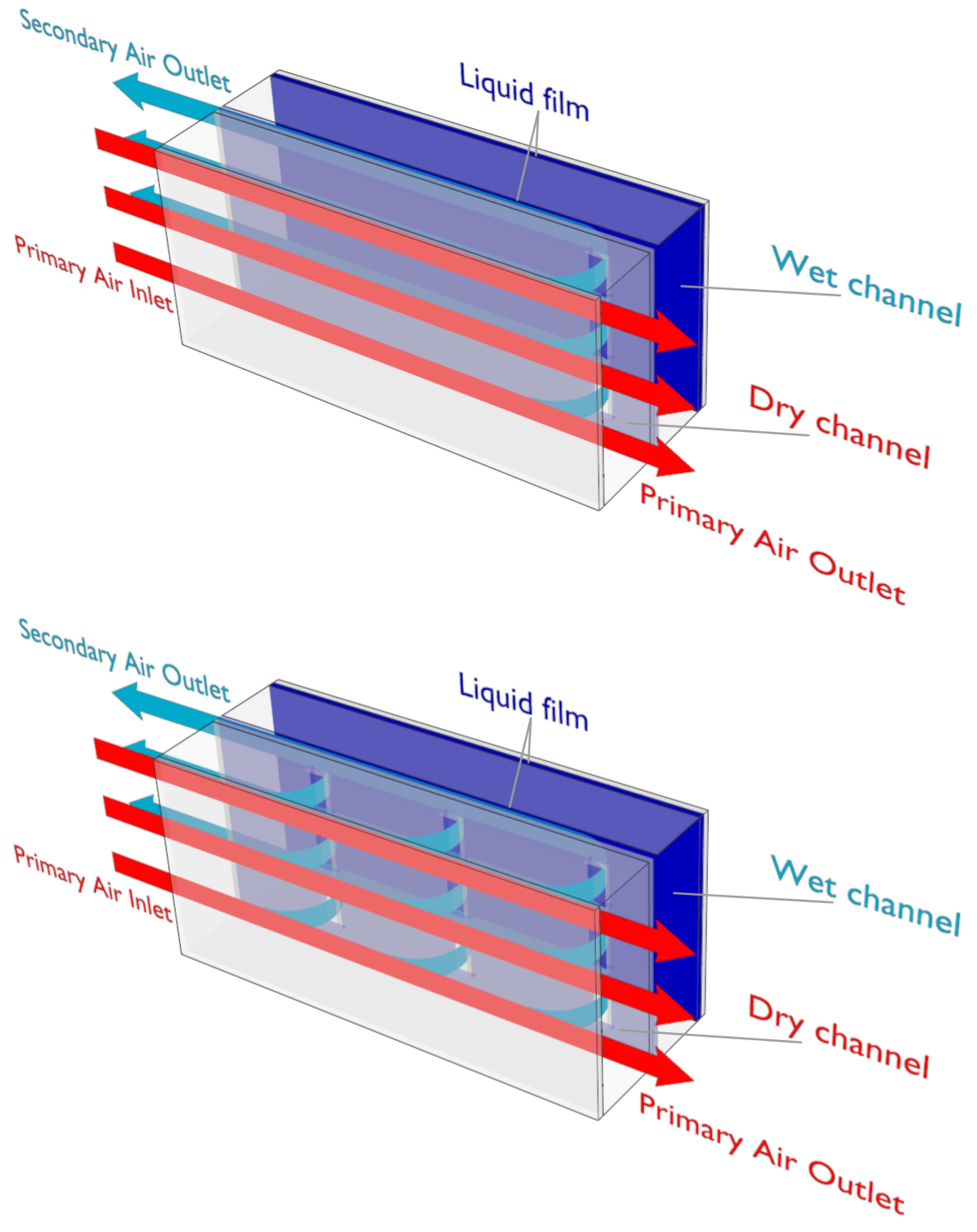
| Paper | Type of IEC System | Innovations and Advantages | Validation | Possible Limitations |
|---|---|---|---|---|
| Alonso et al. (1998) [25] | All | Equivalence between an infinitesimal element of a IEC system and a complete heat exchanger; definition of an equivalent temperature | Experimental from literature | Assumptions of steady flow with constant air density, and surface wettability factor equal to one |
| Stoitchkov and Dimitrov (1998) [26] | Cross-flow | Estimation of the mean surface water temperature; consideration of the barometric pressure | Numerical from the authors | Model suitable only for cross-flow IEC systems with a flowing down water film; assumption of uniform flow velocities |
| Ren and Yang (2006) [28] | Parallel-flow and Counter-flow | Non-unitary Lewis number and wettability factor; consideration of spray water evaporation, temperature changes, and enthalpy variations | Numerical from the authors | Not suitable for cross-flow IEC systems |
| Heidarinejad and Bozorgmehr (2007) [29] | All | Simplification assuming isothermal plate wall, water film, and air/water interface | Experimental from literature | Implicit assumption of uniformly wetted surface |
| Hasan (2012) [30] | Parallel-flow and Counter-flow | Linear saturation temperature-enthalpy relation; redefinition of potential gradients, heat capacity rate parameters, and transfer coefficient | Experimental from literature | Assumption of unitary wettability factor; non-consideration of cross-flow IEC systems |
| Liu et al. (2013) [31] | All | Iterative estimation of the enthalpy change/temperature change ratio in the wet channels | Experimental from literature | Assumptions of steady flows with constant air density, and unitary wettability factor |
| Cui et al. (2014) [32] | All | Introduction of enthalpy/wet bulb temperature ratio, of a modified overall heat transfer coefficient, and of a driving-force based on the wet-bulb temperature | Experimental from literature | Assumption of unitary wettability factor |
| Chen et al. (2015) [33] | Counter-flow | Consideration of the possible condensation in the dry channels | Numerical and experimental from literature | Assumption of unitary wettability factor; non-consideration of parallel-flow and cross-flow configurations |
| Heidarinejad and Moshari (2015) [34] | All | Consideration of longitudinal heat conduction and the change of water temperature along the plates surfaces in cross-flow configuration | Experimental from literature | Assumption of constant air density |
| De Antonellis et al. (2017) [35,36] | All | Consideration of the adiabatic cooling of the working air in the inlet plenum and of the wettability of the plates | Experimental from the authors | Same heat exchanger and similar working conditions for calibration and validation |
| Moshari and Heidarinejad (2017) [37] | Counter-flow and Cross-flow | Evaluation of the impact of different fin heights on the pressure drops and, consequently, on the power consumption | Experimental from literature | Assumption of unitary wettability factor |
| Comino et al. (2018) [38] | All | Consideration of the effects of variable water flow rate | Experimental from the authors | Same heat exchanger and similar working conditions for calibration and validation |
| Zheng et al. (2019) [39] | Cross-flow | Consideration of the thermal resistance of the condensate film | Experimental from the authors | Assumption of unitary wettability factor |
| Paper | Type of IEC System | Numerical Method | Innovations and Advantages | Validation | Possible Limitations |
|---|---|---|---|---|---|
| Wan et al. (2017) [40] | Counter-flow | FVM | Use of a 2-D numerical CFD model to improve the accuracy of a 1-D analytical model | Numerical from literature | Assumptions of constant air density and unitary wettability factor; non-consideration of parallel-flow and cross-flow configurations |
| You et al. (2019) [41] | Cross-flow | FVM | Use of a CFD model to evaluate the influence of some design parameters on the performance of cross-flow IEC systems | Experimental from the authors | Assumptions of constant air density and unitary wettability factor; non-consideration of parallel-flow and counter-flow configurations |
| Min et al. (2019) [42] | Cross-flow | FDM | Use of a numerical model for a performance comparison between counter-flow and cross-flow IEC systems and an optimization of the geometrical parameters | Numerical and experimental from literature | Assumptions of constant air density, and unitary wettability factor; non-consideration of parallel-flow and counter flow configurations |
| Wan et al. (2020) [43] | Counter-flow | FEM | Use of a CFD model to conduct a single-factor and a multiple-factor analysis on the design parameters to improve the correlations for the mean heat and mass transfer coefficients | Experimental from literature | Assumptions of constant air density and unitary wettability factor; non-consideration of parallel-flow and cross-flow configurations |
| Guo et al. (2020) [44] | Cross-flow | FDM | Evaluation of the effect of the condensation area ratio on the IEC system performance | Experimental from the authors | Assumption of unitary wettability factor; non-consideration of parallel-flow and counter-flow configurations |
| Adam et al. (2021) [45] | Cross-flow | RK4 | Use of a numerical model to evaluate the effects of the wettability factor on the IEC system effectiveness | Experimental from literature | Non-consideration of parallel-flow and counter-flow configurations; wettability factor calculation based on the results of an empirical model |
| Shi et al. (2022) [46] | Cross-flow | FEM | Consideration of the non-uniformity in temperature and humidity along the channel width | Numerical and experimental from literature | Assumptions of constant air density and unitary wettability factor; non-consideration of parallel-flow and counter-flow configurations |
| Adam et al. (2022) [47] | Cross-flow | RK4 | Use of a numerical model to evaluate the effects of different condensation states in a cross-flow IEC system | Numerical and experimental from literature | Not specified wettability factor in the validation; non-consideration of parallel-flow and counter-flow configurations |
| Pacak et al. (2023) [48] | Counter-flow | FVM | Use of a CFD model to evaluate the pressure drop along the dry channel of a counter-flow IEC system | Experimental from the manufacturer | Implicit assumption of unitary wettability factor; non-consideration of parallel-flow and cross-flow configurations |
| Adam et al. (2023) [49] | Counter-flow | FVM | Use of an unsteady 2-D CFD model to analyze the influence of the plates shape on the performance of counter-flow IEC systems | Nusselt film empirical formula | Validation of water film thickness only; non-consideration of parallel-flow and cross-flow configurations |
| Ma et al. (2023) [50] | Cross-flow | FVM | Consideration of water spray configuration and water film distribution in the performance analysis | Experimental from literature, Nusselt film empirical formula, and experimental from the authors | Non-consideration of parallel flow and counter-flow configurations |
| Paper | Type of DIEC System | Numerical Method | Innovations and Advantages | Validation | Possible Limitations |
|---|---|---|---|---|---|
| Zhao et al. (2008) [65] | Counter-flow | FVM | Use of a numerical model for the evaluation of the suitability of DIEC systems in hot and dry regions | Not performed | Assumptions of unitary wettability factor and constant air density; absence of validation |
| Riangvilaikul and Kumar (2010) [66] | Counter-flow | FDM | Use of a numerical model to evaluate the influence of the inlet air temperature and humidity on the dew-point effectiveness of a DIEC system | Experimental from the authors and from literature | Assumption of unitary wettability factor |
| Zhan et al. (2011) [67] | Cross-flow | FEM | Use of a numerical model for the performance analysis of a cross-flow DIEC system | Experimental from literature | Assumption of unitary wettability factor and constant air density; not-suitable for counter-flow configuration in this form |
| Cui et al. (2014) [68] | Counter-flow | FVM | Use of a CFD model to evaluate the influence of the design parameters on the performance of a new type of DIEC system with separate product air and working air | Experimental from literature | Assumptions of unitary wettability factor and constant air density |
| Anisimov et al. (2014–2016) [69,70,71,72,73] | All | RKM | Description of the air streams mixing process and of the complete conduction equations for the finned surface | Experimental from literature | Assumptions of constant air density and unitary wettability factor |
| Jafarian et al. (2017) [74] | Counter-flow | FVM | Introduction of a new thermal boundary condition which better reflects reality | Experimental from literature | Assumptions of constant air density and unitary wettability factor |
| Wan et al. (2018) [75,76,77] | Counter-flow | FEM | Use of a CFD model for the evaluation of the heat and mass transfer coefficients and dimensionless numbers in a DIEC system | Experimental from the authors | Assumptions of constant air density and unitary wettability factor |
| Pakari and Ghani (2019) [78,79] | Counter-flow | FEM | Use of CFD regression models for the performance prediction of DIEC systems | Experimental from the authors | Assumptions of constant air density and unitary wettability factor |
| Liu et al. (2019) [80] | Counter-flow | FEM | Coupling of the heat and mass transfer equations with the energy equation | Experimental from literature | Assumption of unitary wettability factor |
| Comino et al. (2022) [81] | Counter-flow | FEM | Use of a modified -NTU numerical model for the optimization of a DIEC system | Experimental from the authors | Assumption of constant wettability factor |
| Zhu et al. (2023) [82] | Counter-flow | FEM | Evaluation of the effects of a non-uniform water distribution along the DIEC system plates | Numerical and experimental from literature | Assumption of unitary wettability factor |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Caruana, R.; De Antonellis, S.; Marocco, L.; Guilizzoni, M. Modeling of Indirect Evaporative Cooling Systems: A Review. Fluids 2023, 8, 303. https://doi.org/10.3390/fluids8110303
Caruana R, De Antonellis S, Marocco L, Guilizzoni M. Modeling of Indirect Evaporative Cooling Systems: A Review. Fluids. 2023; 8(11):303. https://doi.org/10.3390/fluids8110303
Chicago/Turabian StyleCaruana, Roberta, Stefano De Antonellis, Luca Marocco, and Manfredo Guilizzoni. 2023. "Modeling of Indirect Evaporative Cooling Systems: A Review" Fluids 8, no. 11: 303. https://doi.org/10.3390/fluids8110303
APA StyleCaruana, R., De Antonellis, S., Marocco, L., & Guilizzoni, M. (2023). Modeling of Indirect Evaporative Cooling Systems: A Review. Fluids, 8(11), 303. https://doi.org/10.3390/fluids8110303







