Fluid–Structure Interaction Aortic Valve Surgery Simulation: A Review
Abstract
1. Introduction
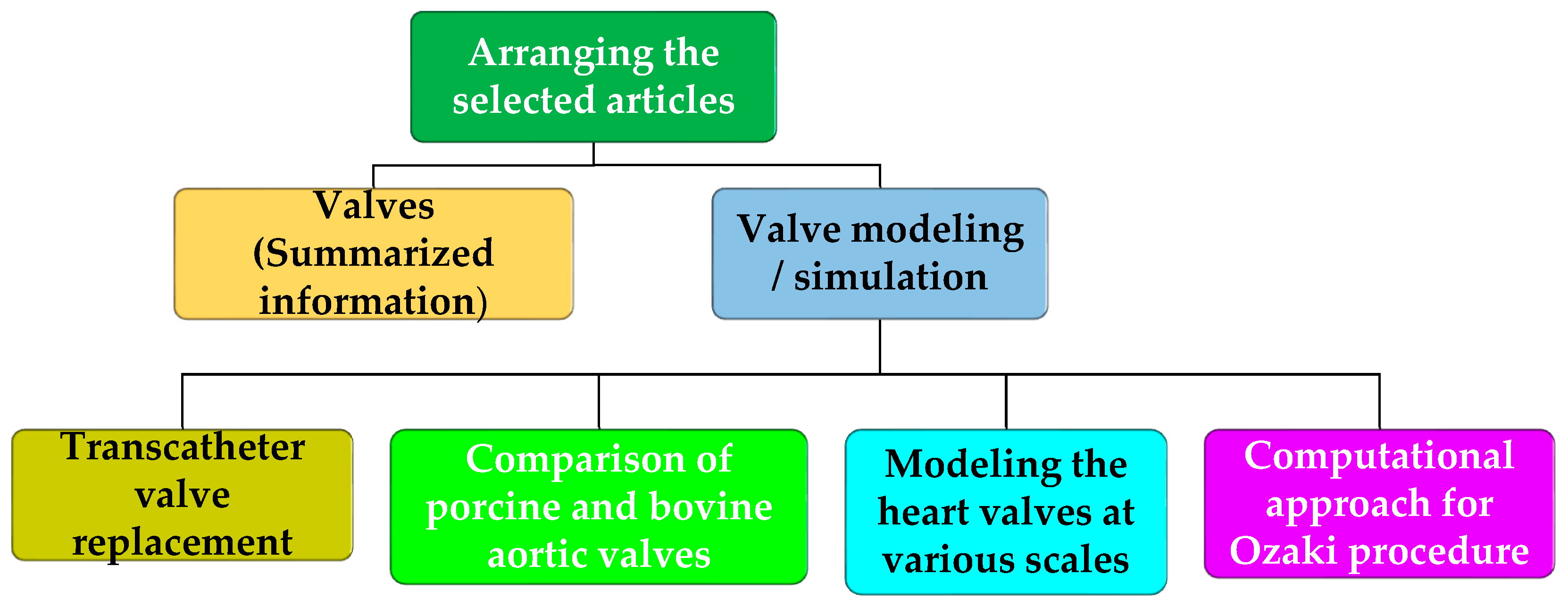
2. Materials and Methods
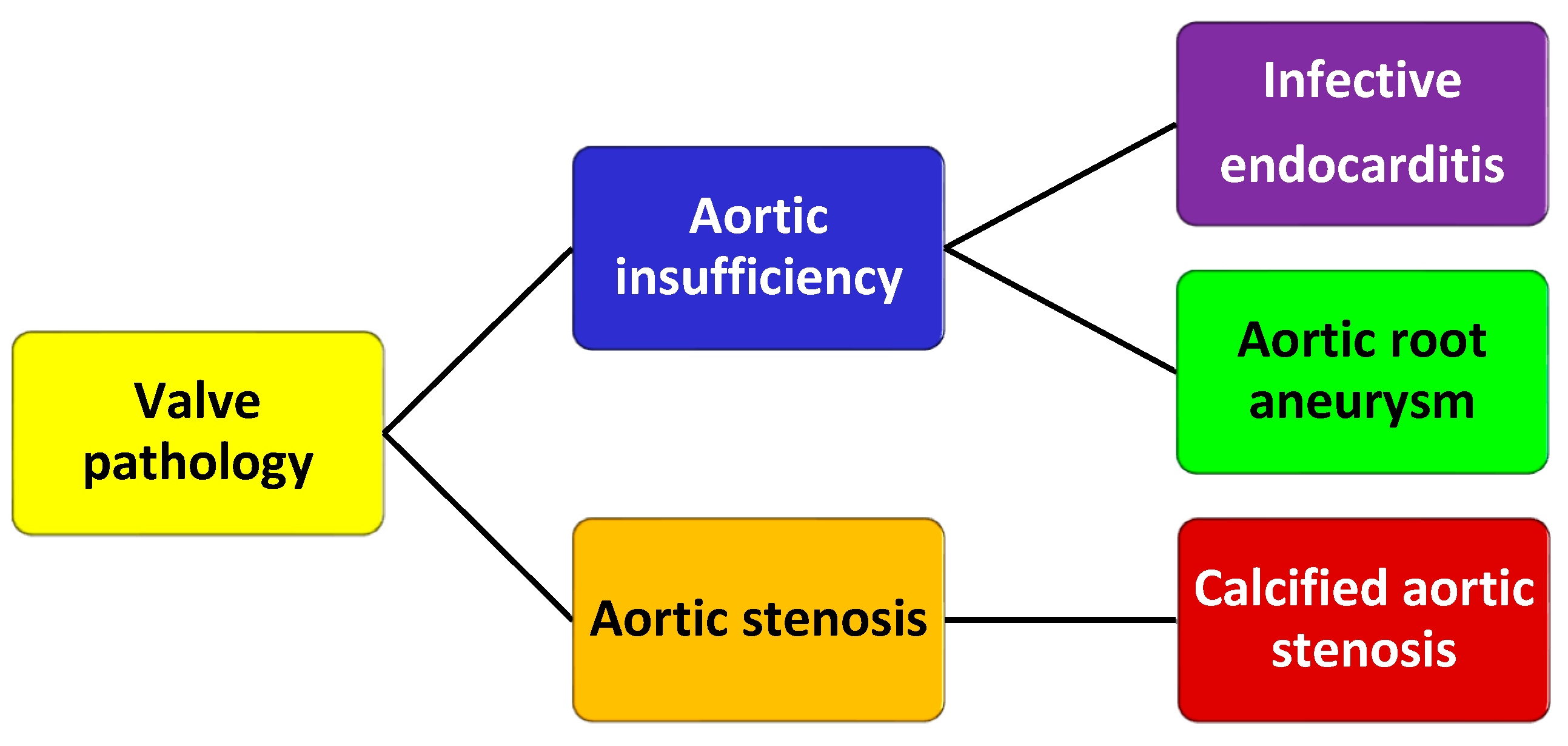
2.1. Aortic Valves
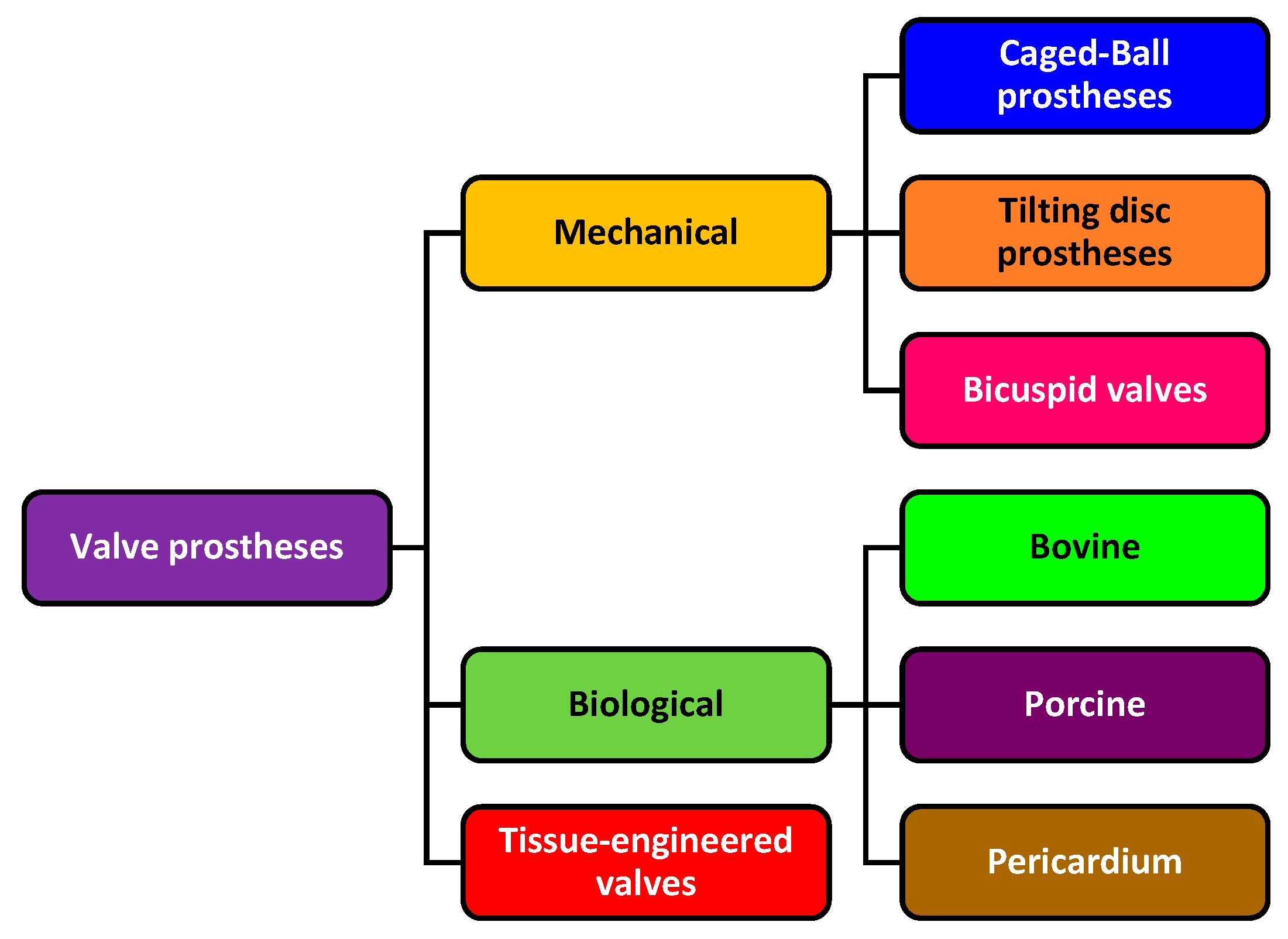
2.2. Mechanical Valve Prostheses
2.3. Biological Valve Prostheses
2.4. Advanced Developments in the Field of Heart Valves
3. Numerical Simulation
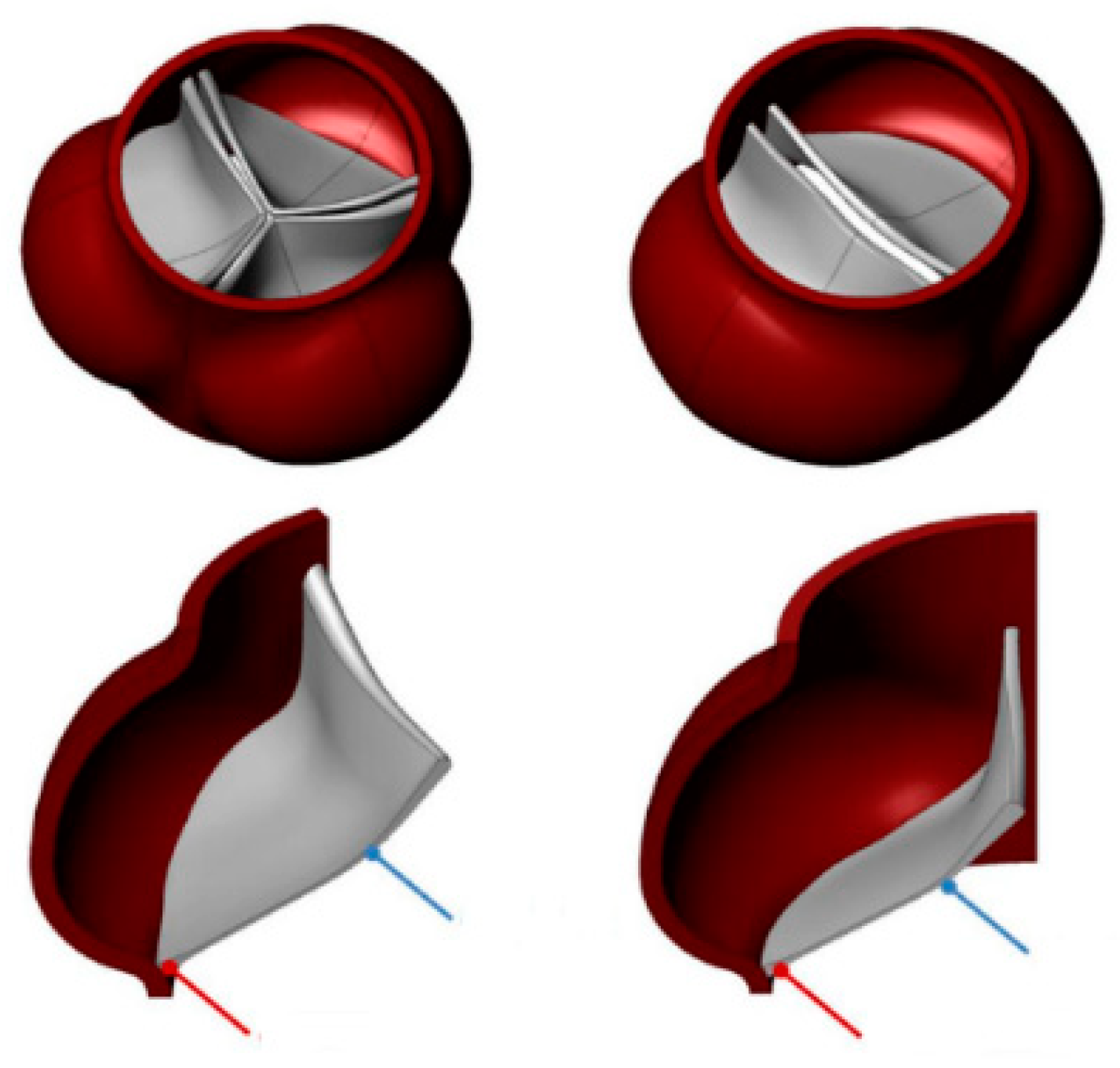
3.1. Valve Modeling
3.2. Numerical Simulation of Transcatheter Valve Replacement
3.3. Numerical Modeling for Comparison of Porcine and Bovine Aortic Valves
3.4. Multi-Scale Modeling
3.5. Ozaki Procedure Modeling
3.6. Numerical Modeling of a Bileaflet Mechanical Valve
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Marom, G. Numerical Methods for Fluid–Structure Interaction Models of Aortic Valves. Arch. Comput. Methods Eng. 2015, 22, 595–620. [Google Scholar] [CrossRef]
- Yacoub, M.H.; Takkenberg, J.J.M. Will Heart Valve Tissue Engineering Change the World? Nat. Clin. Pract. Cardiovasc. Med. 2005, 2, 60–61. [Google Scholar] [CrossRef]
- Li, K.Y.C. Bioprosthetic Heart Valves: Upgrading a 50-Year Old Technology. Front. Cardiovasc. Med. 2019, 6, 47. [Google Scholar] [CrossRef]
- Iung, B.; Vahanian, A. Epidemiology of Valvular Heart Disease in the Adult. Nat. Rev. Cardiol. 2011, 8, 162–172. [Google Scholar] [CrossRef] [PubMed]
- Dangas, G.D.; Weitz, J.I.; Giustino, G.; Makkar, R.; Mehran, R. Prosthetic Heart Valve Thrombosis. J. Am. Coll. Cardiol. 2016, 68, 2670–2689. [Google Scholar] [CrossRef]
- Pogosova, G.V.; Oganov, R.G.; Saner, H. Positive Trends in Cardiovascular Mortality in Russia and Moscow: Potential Confounders. Eur. Heart J. 2016, 37, 3184–3185. [Google Scholar] [CrossRef][Green Version]
- Lakunchykova, O.; Averina, M.; Wilsgaard, T.; Watkins, H.; Malyutina, S.; Ragino, Y.; Keogh, R.H.; Kudryavtsev, A.V.; Govorun, V.; Cook, S.; et al. Why Does Russia Have Such High Cardiovascular Mortality Rates? Comparisons of Blood-Based Biomarkers with Norway Implicate Non-Ischaemic Cardiac Damage. J. Epidemiol. Community Health 2020, 74, 698–704. [Google Scholar] [CrossRef]
- Han, L.; Zhao, S.; Li, S.; Gu, S.; Deng, X.; Yang, L.; Ran, J. Excess Cardiovascular Mortality across Multiple COVID-19 Waves in the United States from March 2020 to March 2022. Nat. Cardiovasc. Res. 2023, 2, 322–333. [Google Scholar] [CrossRef]
- Kamaltdinov, M.R.; Kuchumov, A.G. Application of a mathematical model of systemic circulation for determination of blood flow parameters after modified blalock-taussig shunt operation in newborns. Russ. J. Biomech. 2021, 25, 268–284. [Google Scholar]
- Kamaltdinov, M.; Trusov, P.; Zaitseva, N. A Mathematical Model of the Multiphase Flow in the Antroduodenum: Consideration of the Digestive Enzymes and Regulation Processes. Ser. Biomech. 2018, 32, 36–42. [Google Scholar]
- Kamaltdinov, M.; Trusov, P.; Zaitseva, N. A Multiphase Flow in the Antroduodenum: Some Results of the Mathematical Modelling and Computational Simulation. MATEC Web Conf. 2018, 145, 04002. [Google Scholar] [CrossRef]
- Kuchumov, A.; Tuktamyshev, V.; Kamaltdinov, M. Peristaltic Flow of Lithogenic Bile in the Vateri’s Papilla as Non-Newtonian Fluid in the Finite-Length Tube: Analytical and Numerical Results for Reflux Study and Optimization. Lek. A Technol. 2017, 47, 35–42. [Google Scholar]
- Kuchumov, A.G.; Kamaltdinov, M.; Selyaninov, A.; Samartsev, V. Numerical Simulation of Biliary Stent Clogging. Ser. Biomech. 2019, 33, 3–15. [Google Scholar]
- Mantskava, M.M.; Nyashin, Y.I.; Lokhov, V.A. The Study of Blood Circulation at Experimental Tumur Paraneoplasticism. Russ. J. Biomech. 2020, 23, 505–509. [Google Scholar]
- Nowak, M.; Divo, E.; Adamczyk, W.P. Fluid–Structure Interaction Methods for the Progressive Anatomical and Artificial Aortic Valve Stenosis. Int. J. Mech. Sci. 2022, 227, 107410. [Google Scholar] [CrossRef]
- Kuchumov, A.G.; Selyaninov, A. Application of Computational Fluid Dynamics in Biofluids Simulation to Solve Actual Surgery Tasks. Adv. Intell. Syst. Comput. 2020, 1018, 576–580. [Google Scholar] [CrossRef]
- Kuchumov, A. Patient-Specific Bile Flow Simulation to Evaluate Cholecystectomy Outcome. IOP Conf. Ser. Mater. Sci. Eng. 2019, 581, 012022. [Google Scholar] [CrossRef]
- Kuchumov, A. Biomechanical Modelling of Bile Flow in the Biliary System. In Proceedings of the MATEC Web of Conferences, 9 January 2018; Vassilev, V.M., Nikolov, S.G., Datcheva, M.D., Ivanova, Y.P., Eds.; EDP Sciences: Les Ulis, France, 2018; Volume 145, p. 04004. [Google Scholar]
- Kuchumov, A.G. Mathematical Modeling of the Peristaltic Lithogenic Bile Flow through the Duct at Papillary Stenosis as a Tapered Finite-Length Tube. Russ. J. Biomech. 2016, 20, 77–96. [Google Scholar]
- Kuchumov, A.G. Biomechanical Model of Bile Flow in the Biliary System. Russ. J. Biomech. 2019, 23, 224–248. [Google Scholar]
- Wu, M.C.H.; Muchowski, H.M.; Johnson, E.L.; Rajanna, M.R.; Hsu, M.C. Immersogeometric Fluid–Structure Interaction Modeling and Simulation of Transcatheter Aortic Valve Replacement. Comput. Methods Appl. Mech. Eng. 2019, 357, 112556. [Google Scholar] [CrossRef]
- Bosi, G.M.; Capelli, C.; Cheang, M.H.; Delahunty, N.; Mullen, M.; Taylor, A.M.; Schievano, S. Population-Specific Material Properties of the Implantation Site for Transcatheter Aortic Valve Replacement Finite Element Simulations. J. Biomech. 2018, 71, 236–244. [Google Scholar] [CrossRef]
- Amindari, A.; Kırkköprü, K.; Saltık, İ.L.; Sünbüloğlu, E. Effect of Non-Linear Leaflet Material Properties on Aortic Valve Dynamics-a Coupled Fluid-Structure Approach. Eng. Solid Mech. 2021, 9, 123–136. [Google Scholar] [CrossRef]
- Mao, W.; Wang, Q.; Kodali, S.; Sun, W. Numerical Parametric Study of Paravalvular Leak Following a Transcatheter Aortic Valve Deployment into a Patient-Specific Aortic Root. J. Biomech. Eng. 2018, 140, 101007. [Google Scholar] [CrossRef]
- Sturla, F.; Votta, E.; Stevanella, M.; Conti, C.A.; Redaelli, A. Impact of modeling fluid–structure interaction in the computational analysis of aortic root biomechanics. Med. Eng. Phys. 2013, 35, 1721–1730. [Google Scholar] [CrossRef]
- Wu, W.; Pott, D.; Mazza, B.; Sironi, T.; Dordoni, E.; Chiastra, C.; Petrini, L.; Pennati, G.; Dubini, G.; Steinseifer, U.; et al. Fluidstructure interaction model of a percutaneous aortic valve: Comparison with an in vitro test and feasibility study in a patient-specific case. Ann. Biomed. Eng. 2016, 44, 590–603. [Google Scholar] [CrossRef]
- Kalyana Sundaram, G.B.; Balakrishnan, K.R.; Kumar, R.K. Aortic Valve Dynamics Using a Fluid Structure Interaction Model-The Physiology of Opening and Closing. J. Biomech. 2015, 48, 1737–1744. [Google Scholar] [CrossRef]
- Tzamtzis, S.; Viquerat, J.; Yap, J.; Mullen, M.J.; Burriesci, G. Numerical Analysis of the Radial Force Produced by the Medtronic-CoreValve and Edwards-SAPIEN after Transcatheter Aortic Valve Implantation (TAVI). Med. Eng. Phys. 2013, 35, 125–130. [Google Scholar] [CrossRef] [PubMed]
- Bosmans, B.; Famaey, N.; Verhoelst, E.; Bosmans, J.; Vander Sloten, J. A Validated Methodology for Patient Specific Computational Modeling of Self-Expandable Transcatheter Aortic Valve Implantation. J. Biomech. 2016, 49, 2824–2830. [Google Scholar] [CrossRef] [PubMed]
- Finotello, A.; Morganti, S.; Auricchio, F. Finite Element Analysis of TAVI: Impact of Native Aortic Root Computational Modeling Strategies on Simulation Outcomes. Med. Eng. Phys. 2017, 47, 2–12. [Google Scholar] [CrossRef] [PubMed]
- Wang, Q.; Kodali, S.; Primiano, C.; Sun, W. Simulations of Transcatheter Aortic Valve Implantation: Implications for Aortic Root Rupture. Biomech. Model. Mechanobiol. 2015, 14, 29–38. [Google Scholar] [CrossRef]
- Dowling, C.; Bavo, A.M.; El Faquir, N.; Mortier, P.; De Jaegere, P.; De Backer, O.; Sondergaard, L.; Ruile, P.; Mylotte, D.; McConkey, H.; et al. Patient-Specific Computer Simulation of Transcatheter Aortic Valve Replacement in Bicuspid Aortic Valve Morphology. Circ. Cardiovasc. Imaging 2019, 12, e009178. [Google Scholar] [CrossRef] [PubMed]
- Bianchi, M.; Marom, G.; Ghosh, R.P.; Rotman, O.M.; Parikh, P.; Gruberg, L.; Bluestein, D. Patient-Specific Simulation of Transcatheter Aortic Valve Replacement: Impact of Deployment Options on Paravalvular Leakage. Biomech. Model. Mechanobiol. 2019, 18, 435–451. [Google Scholar] [CrossRef] [PubMed]
- Whelan, A.; Duffy, J.; Gaul, R.T.; O’Reilly, D.; Nolan, D.R.; Gunning, P.; Lally, C.; Murphy, B.P. Collagen fibre orientation and dispersion govern ultimate tensile strength, stiffness and the fatigue performance of bovine pericardium. J. Mech. Behav Biomed Mater. 2019, 90, 54–60. [Google Scholar] [CrossRef]
- Travaglino, S.; Murdock, K.; Tran, A.; Martin, C.; Liang, L.; Wang, Y.; Sun, W. Computational Optimization Study of Transcatheter Aortic Valve Leaflet Design Using Porcine and Bovine Leaflets. J. Biomech. Eng. 2020, 142, 011007. [Google Scholar] [CrossRef]
- van Loon, R.; Anderson, P.D.; de Hart, J.; Baaijens, F.P.T. A Combined Fictitious Domain/Adaptive Meshing Method for Fluid-Structure Interaction in Heart Valves. Int. J. Numer. Methods Fluids 2004, 46, 533–544. [Google Scholar] [CrossRef]
- Li, C.; Tang, D.; Yao, J.; Shao, Y.; Sun, H.; Hammer, P.; Gong, C.; Ma, L.; Zhang, Y.; Wang, L.; et al. Porcine and Bovine Aortic Valve Comparison for Surgical Optimization: A Fluid-Structure Interaction Modeling Study. Int. J. Cardiol. 2021, 334, 88–95. [Google Scholar] [CrossRef]
- Weinberg, E.J.; Kaazempur Mofrad, M.R. A Multiscale Computational Comparison of the Bicuspid and Tricuspid Aortic Valves in Relation to Calcific Aortic Stenosis. J. Biomech. 2008, 41, 3482–3487. [Google Scholar] [CrossRef]
- De Hart, J.; Peters, G.W.M.; Schreurs, P.J.G.; Baaijens, F.P.T. A Three-Dimensional Computational Analysis of Fluid-Structure Interaction in the Aortic Valve. J. Biomech. 2003, 36, 103–112. [Google Scholar] [CrossRef]
- Nicosia, M.A.; Cochran, R.P.; Einstein, D.R.; Rutland, C.J.; Kunzelman, K.S. A coupled fluid-structure finite element model of the aortic valve and root. J. Heart Valve Dis. 2003, 12, 781–789. [Google Scholar]
- Weinberg, E.J.; Kaazempur-Mofrad, M.R. On the Constitutive Models for Heart Valve Leaflet Mechanics. Cardiovasc. Eng. 2005, 5, 37–43. [Google Scholar] [CrossRef]
- Stella, J.A.; Sacks, M.S. On the Biaxial Mechanical Properties of the Layers of the Aortic Valve Leaflet. J. Biomech. Eng. 2007, 129, 757–766. [Google Scholar] [CrossRef]
- Weinberg, E.J.; Kaazempur Mofrad, M.R. A Finite Shell Element for Heart Mitral Valve Leaflet Mechanics, with Large Deformations and 3D Constitutive Material Model. J. Biomech. 2007, 40, 705–711. [Google Scholar] [CrossRef] [PubMed]
- Liu, X.; Zhang, W.; Ye, P.; Luo, Q.; Chang, Z. Fluid-Structure Interaction Analysis on the Influence of the Aortic Valve Stent Leaflet Structure in Hemodynamics. Front. Physiol. 2022, 13, 904453. [Google Scholar] [CrossRef]
- Pil, N.; Kuchumov, A.G.; Kadyraliev, B.; Arutunyan, V. Influence of Aortic Valve Leaflet Material Model on Hemodynamic Features in Healthy and Pathological States. Mathematics 2023, 11, 428. [Google Scholar] [CrossRef]
- Qashqaie Abdi, S.; Hassani, K. The Study of the Relationship between Unicuspid Aortic Valve Insufficiency and Heart Disease by Fluid-Structure Interaction Modeling. Biomed. Eng. Adv. 2023, 5, 100079. [Google Scholar] [CrossRef]
- Bosi, G.M.; Capelli, C.; Cheang, M.H.; Delahunty, N.; Mullen, M.; Taylor, A.M.; Schievano, S. A validated computational framework to predict outcomes in TAVI. Sci. Rep. 2020, 10, 9906. [Google Scholar] [CrossRef]
- Morany, A.; Lavon, K.; Gomez Bardon, R.; Kovarovic, B.; Hamdan, A.; Bluestein, D.; Haj-Ali, R. Fluid–Structure Interaction Modeling of Compliant Aortic Valves Using the Lattice Boltzmann CFD and FEM Methods. Biomech. Model. Mechanobiol. 2023, 22, 837–850. [Google Scholar] [CrossRef]
- Hinton, R.B.; Yutzey, K.E. Heart Valve Structure and Function in Development and Disease. Annu. Rev. Physiol. 2011, 73, 29–46. [Google Scholar] [CrossRef]
- Zamorano, J.L.; Gonçalves, A.; Lang, R. Imaging to Select and Guide Transcatheter Aortic Valve Implantation. Eur. Heart J. 2014, 35, 1578–1587. [Google Scholar] [CrossRef]
- Paiocchi, V.L.; Faletra, F.F.; Ferrari, E.; Schlossbauer, S.A.; Leo, L.A.; Maisano, F. Multimodality Imaging of the Anatomy of the Aortic Root. J. Cardiovasc. Dev. Dis. 2021, 8, 51. [Google Scholar] [CrossRef]
- Loukas, M.; Bilinsky, E.; Bilinsky, S.; Blaak, C.; Tubbs, R.S.; Anderson, R.H. The Anatomy of the Aortic Root. Clin. Anat. 2014, 27, 748–756. [Google Scholar] [CrossRef] [PubMed]
- Edwards, J.E. Pathology of left ventricular outflow tract obstruction. Circulation 1965, 31, 586–599. [Google Scholar] [CrossRef] [PubMed]
- Redel, D.A. Blood Flow Velocity Patterns in Heart Disease. In Color Blood Flow Imaging of the Heart; Springer: Berlin, Heidelberg, 1988. [Google Scholar]
- Suvorov, V.V.; Fedotova, E.P.; Zaitsev, V.V.; Dolgova, E.V.; Popova, L.L.; Glazunova, A.E.; Novak, M.U.; Nasyrov, R.A. A Rare Case of Diagnosed Absent Aortic Valve and Severely Hypoplastic Pulmonary Valve with Double Outlet Right Ventricle: A Case Report. Heliyon 2023, 9, e17373. [Google Scholar] [CrossRef] [PubMed]
- Naeije, R.; Tello, K.; D’Alto, M. Tricuspid Regurgitation: Right Ventricular Volume Versus Pressure Load. Curr. Heart. Fail. Rep. 2023, 20, 208–217. [Google Scholar] [CrossRef]
- Prodromo, J.; D’Ancona, G.; Amaducci, A.; Pilato, M. Aortic Valve Repair for Aortic Insufficiency: A Review. J. Cardiothorac. Vasc. Anesth. 2012, 26, 923–932. [Google Scholar] [CrossRef]
- Reed, A.; Bajwa, S.; Schuh, S.; Mikhael, M. Incidental Perforation of Aortic Valve Leaflet Found on Presentation of Cardiogenic Shock. Cureus 2023, 15, e39476. [Google Scholar] [CrossRef]
- Sassis, L.; Kefala-Karli, P.; Cucchi, I.; Kouremenos, I.; Demosthenous, M.; Diplaris, K. Valve Repair in Aortic Insufficiency: A State-of-the-Art Review. Curr. Cardiol. Rev. 2022, 19, 21–31. [Google Scholar] [CrossRef]
- Ziganshin, B.A.; Kargin, N.; Zafar, M.A.; Elefteriades, J.A. The Natural History of Aortic Root Aneurysms. Ann. Cardiothorac. Surg. 2023, 12, 213–224. [Google Scholar] [CrossRef]
- Chung, J.C.-Y. Pathology and Pathophysiology of the Aortic Root. Ann. Cardiothorac. Surg. 2023, 12, 159–167. [Google Scholar] [CrossRef]
- Masri, A.; Kalahasti, V.; Alkharabsheh, S.; Svensson, L.G.; Sabik, J.F.; Roselli, E.E.; Hammer, D.; Johnston, D.R.; Collier, P.; Rodriguez, L.L.; et al. Characteristics and Long-Term Outcomes of Contemporary Patients with Bicuspid Aortic Valves. J. Thorac. Cardiovasc. Surg. 2016, 151, 1650–1659.e1. [Google Scholar] [CrossRef]
- Hoffman, J.I.E.; Kaplan, S. The Incidence of Congenital Heart Disease. J. Am. Coll. Cardiol. 2002, 39, 1890–1900. [Google Scholar] [CrossRef]
- Cripe, L.; Andelfinger, G.; Martin, L.J.; Shooner, K.; Benson, D.W. Bicuspid Aortic Valve Is Heritable. J. Am. Coll. Cardiol. 2004, 44, 138–143. [Google Scholar] [CrossRef]
- Ellison, J.W.; Yagubyan, M.; Majumdar, R.; Sarkar, G.; Bolander, M.E.; Atkinson, E.J.; Sarano, M.E.; Sundt, T.M. Evidence of Genetic Locus Heterogeneity for Familial Bicuspid Aortic Valve. J. Surg. Res. 2007, 142, 28–31. [Google Scholar] [CrossRef] [PubMed]
- Huntington, K.; Hunter, A.G.W.; Chan, K.L. A Prospective Study to Assess the Frequency of Familial Clustering of Congenital Bicuspid Aortic Valve. J. Am. Coll. Cardiol. 1997, 30, 1809–1812. [Google Scholar] [CrossRef] [PubMed]
- Mcdonald, K.; Maurer, B.J. Familial Aortic Valve Disease: Evidence for a Genetic Influence? Eur. Heart J. 1989, 10, 676–677. [Google Scholar] [CrossRef] [PubMed]
- Glick, B.N.; Roberts, W.C. Congenitally Bicuspid Aortic Valve in Multiple Family Members. Am. J. Cardiol. 1994, 73, 400–404. [Google Scholar] [CrossRef]
- Della Corte, A.; Bancone, C.; Quarto, C.; Dialetto, G.; Covino, F.E.; Scardone, M.; Caianiello, G.; Cotrufo, M. Predictors of Ascending Aortic Dilatation with Bicuspid Aortic Valve: A Wide Spectrum of Disease Expression. Eur. J. Cardio-Thorac. Surg. 2007, 31, 397–405. [Google Scholar] [CrossRef]
- Emanuel, R.; Withers, R.; O’Brien, K.; Ross, P.; Feizi, Ö. Congenitally Bicuspid Aortic Valves. Clinicogenetic Study of 41 Families. Heart 1978, 40, 1402–1407. [Google Scholar] [CrossRef] [PubMed]
- Otto, C.M. Calcification of Bicuspid Aortic Valves. Heart 2002, 88, 321–322. [Google Scholar] [CrossRef]
- Lewin, M.B.; Otto, C.M. The Bicuspid Aortic Valve: Adverse Outcomes from Infancy to Old Age. Circulation 2005, 111, 832–834. [Google Scholar] [CrossRef]
- Slama, M.; Tribouilloy, C.; Maizel, J. Left Ventricular Outflow Tract Obstruction in ICU Patients. Curr. Opin. Crit. Care 2016, 22, 260–266. [Google Scholar] [CrossRef] [PubMed]
- Joseph, J.; Naqvi, S.Y.; Giri, J.; Goldberg, S. Aortic Stenosis: Pathophysiology, Diagnosis, and Therapy. Am. J. Med. 2017, 130, 253–263. [Google Scholar] [CrossRef] [PubMed]
- Mas-Peiro, S.; Fichtlscherer, S.; Walther, C.; Vasa-Nicotera, M. Current Issues in Transcatheter Aortic Valve Replacement. J. Thorac. Dis. 2020, 12, 1665–1680. [Google Scholar] [CrossRef]
- Nathaniel, S. Aortic Stenosis: An Update. World J. Cardiol. 2010, 2, 135–139. [Google Scholar] [CrossRef]
- Boskovski, M.T.; Gleason, T.G. Current Therapeutic Options in Aortic Stenosis. Circ. Res. 2021, 128, 1398–1417. [Google Scholar] [CrossRef] [PubMed]
- Marijon, E.; Mirabel, M.; Celermajer, D.S.; Jouven, X. Rheumatic Heart Disease. Lancet 2012, 379, 953–964. [Google Scholar] [CrossRef] [PubMed]
- Choudhary, S.K.; Talwar, S.; Airan, B. Choice of Prosthetic Heart Valve in a Developing Country. Heart Asia 2016, 8, 65–72. [Google Scholar] [CrossRef]
- Rasheed, N.F.; Stonebraker, C.; Li, Z.; Siddiqi, U.; Lee, A.C.H.; Li, W.; Lupo, S.; Cruz, J.; Cohen, W.G.; Staub, C.; et al. Figure of Eight Suture Technique in Aortic Valve Replacement Decreases Prosthesis-Patient Mismatch. J. Cardiothorac. Surg. 2023, 18, 117. [Google Scholar] [CrossRef] [PubMed]
- Head, S.J.; Çelik, M.; Kappetein, A.P. Mechanical versus Bioprosthetic Aortic Valve Replacement. Eur. Heart J. 2017, 38, 2183–2191. [Google Scholar] [CrossRef]
- Pibarot, P.; Dumesnil, J.G. Prosthetic Heart Valves: Selection of the Optimal Prosthesis and Long-Term Management. Circulation 2009, 119, 1034–1048. [Google Scholar] [CrossRef]
- Ascione, G.; Denti, P. Transcatheter Mitral Valve Replacement and Thrombosis: A Review. Front. Cardiovasc. Med. 2021, 8, 621258. [Google Scholar] [CrossRef]
- Babur Guler, G.; Memic Sancar, K.; Corekcioglu, B.; Topel, C.; Erturk, M. Early Valve Thrombosis Management after Successful Transcatheter Tricuspid Valve-in-Valve Implantation. JACC Case Rep. 2023, 5, 101584. [Google Scholar] [CrossRef]
- Couper, G.S. Surgical Aspects of Prosthetic Valve Selection. In Overview of Cardiac Surgery for the Cardiologist; Springer: New York, NY, USA, 1994. [Google Scholar]
- Russo, M.; Taramasso, M.; Guidotti, A.; Pozzoli, A.; Nietilspach, F.; von Segesser, L.K.; Maisano, F. The Evolution of Surgical Valves. Cardiovasc. Med. 2017, 20, 285–292. [Google Scholar] [CrossRef]
- Lund, O.; Nielsen, S.L.; Arildsen, H.; Ilkjaer, L.B.; Pilegaard, H.K. Standard Aortic St. Jude Valve at 18 Years: Performance Profile and Determinants of Outcome. Ann. Thorac. Surg. 2000, 69, 1459–1465. [Google Scholar] [CrossRef] [PubMed]
- Sacks, M.S.; Yoganathan, A.P. Heart Valve Function: A Biomechanical Perspective. Philos. Trans. R. Soc. B Biol. Sci. 2007, 362, 1369–1391. [Google Scholar] [CrossRef] [PubMed]
- Ashour, Z.A.; Shawky, H.A.F.; Hussein, M.H. Outcome of Pregnancy in Women with Mechanical Valves. Tex. Heart Inst. J. 2000, 27, 240–245. [Google Scholar] [PubMed]
- Ng, A.P.; Verma, A.; Sanaiha, Y.; Williamson, C.G.; Afshar, Y.; Benharash, P. Maternal and Fetal Outcomes in Pregnant Patients with Mechanical and Bioprosthetic Heart Valves. J. Am. Heart Assoc. 2023, 12, e028653. [Google Scholar] [CrossRef] [PubMed]
- Jaffer, I.H.; Whitlock, R.P. A Mechanical Heart Valve Is the Best Choice. Heart Asia 2016, 8, 62–64. [Google Scholar] [CrossRef] [PubMed]
- Siddiqui, R.F.; Abraham, J.R.; Butany, J. Bioprosthetic Heart Valves: Modes of Failure. Histopathology 2009, 55, 135–144. [Google Scholar] [CrossRef]
- Blum, K.M.; Drews, J.D.; Breuer, C.K. Tissue-Engineered Heart Valves: A Call for Mechanistic Studies. Tissue Eng. Part B Rev. 2018, 24, 240–253. [Google Scholar] [CrossRef] [PubMed]
- Meuris, B.; De Praetere, H.; Strasly, M.; Trabucco, P.; Lai, J.C.; Verbrugghe, P.; Herijgers, P. A Novel Tissue Treatment to Reduce Mineralization of Bovine Pericardial Heart Valves. J. Thorac. Cardiovasc. Surg. 2018, 156, 197–206. [Google Scholar] [CrossRef] [PubMed]
- Williams, D.F.; Bezuidenhout, D.; de Villiers, J.; Human, P.; Zilla, P. Long-Term Stability and Biocompatibility of Pericardial Bioprosthetic Heart Valves. Front. Cardiovasc. Med. 2021, 8, 728577. [Google Scholar] [CrossRef]
- Campion, G.; Hershberger, K.; Whelan, A.; Conroy, J.; Lally, C.; Murphy, B.P. A Biomechanical and Microstructural Analysis of Bovine and Porcine Pericardium for Use in Bioprosthetic Heart Valves. Struct. Heart 2021, 5, 486–496. [Google Scholar] [CrossRef]
- Soares, J.S.; Feaver, K.R.; Zhang, W.; Kamensky, D.; Aggarwal, A.; Sacks, M.S. Biomechanical Behavior of Bioprosthetic Heart Valve Heterograft Tissues: Characterization, Simulation, and Performance. Cardiovasc. Eng. Technol. 2016, 7, 309–351. [Google Scholar] [CrossRef] [PubMed]
- Kostyunin, A.E.; Yuzhalin, A.E.; Rezvova, M.A.; Ovcharenko, E.A.; Glushkova, T.V.; Kutikhin, A.G. Degeneration of Bioprosthetic Heart Valves: Update 2020. J. Am. Heart Assoc. 2020, 9, e018506. [Google Scholar] [CrossRef] [PubMed]
- Ciolacu, D.E.; Nicu, R.; Ciolacu, F. Natural Polymers in Heart Valve Tissue Engineering: Strategies, Advances and Challenges. Biomedicines 2022, 10, 1095. [Google Scholar] [CrossRef]
- Kalogeropoulos, A.S.; Redwood, S.R.; Allen, C.J.; Hurrell, H.; Chehab, O.; Rajani, R.; Prendergast, B.; Patterson, T. A 20-Year Journey in Transcatheter Aortic Valve Implantation: Evolution to Current Eminence. Front. Cardiovasc. Med. 2022, 9, 971762. [Google Scholar] [CrossRef]
- Nappi, F.; Singh, S.S.A.; Nappi, P.; Fiore, A. Biomechanics of Transcatheter Aortic Valve Implant. Bioengineering 2022, 9, 299. [Google Scholar] [CrossRef]
- Rotman, O.M.; Bianchi, M.; Ghosh, R.P.; Kovarovic, B.; Bluestein, D. Principles of TAVR Valve Design, Modelling, and Testing. Expert Rev. Med. Devices 2018, 15, 771–791. [Google Scholar] [CrossRef]
- Kilic, T.; Yilmaz, I. Transcatheter Aortic Valve Implantation: A Revolution in the Therapy of Elderly and High-Risk Patients with Severe Aortic Stenosis. J. Geriatr. Cardiol. 2017, 14, 204–217. [Google Scholar]
- Tamburino, C.; Valvo, R.; Crioscione, E.; Reddavid, C.; Picci, A.; Costa, G.; Barbanti, M. The Path of Transcatheter Aortic Valve Implantation: From Compassionate to Low-Risk Cases. Eur. Heart J. Suppl. 2020, 22, L140–L145. [Google Scholar] [CrossRef] [PubMed]
- Mitsis, A.; Yuan, X.; Eftychiou, C.; Avraamides, P.; Nienaber, C.A. Personalised Treatment in Aortic Stenosis: A Patient-Tailored Transcatheter Aortic Valve Implantation Approach. J. Cardiovasc. Dev. Dis. 2022, 9, 407. [Google Scholar] [CrossRef]
- Luraghi, G.; Rodriguez Matas, J.F.; Migliavacca, F. In Silico Approaches for Transcatheter Aortic Valve Replacement Inspection. Expert Rev. Cardiovasc. Ther. 2021, 19, 61–70. [Google Scholar] [CrossRef] [PubMed]
- Manji, R.A.; Lee, W.; Cooper, D.K.C. Xenograft Bioprosthetic Heart Valves: Past, Present and Future. Int. J. Surg. 2015, 23, 280–284. [Google Scholar] [CrossRef]
- Ruel, M.; Kulik, A.; Lam, B.; Rubens, F.; Hendry, P.; Masters, R.; Bedard, P.; Mesana, T. Long-Term Outcomes of Valve Replacement with Modern Prostheses in Young Adults. Eur. J. Cardio-Thorac. Surg. 2005, 27, 425–433. [Google Scholar] [CrossRef] [PubMed]
- Sulejmani, F.; Caballero, A.; Martin, C.; Pham, T.; Sun, W. Evaluation of Transcatheter Heart Valve Biomaterials: Computational Modeling Using Bovine and Porcine Pericardium. J. Mech. Behav. Biomed. Mater. 2019, 97, 159–170. [Google Scholar] [CrossRef] [PubMed]
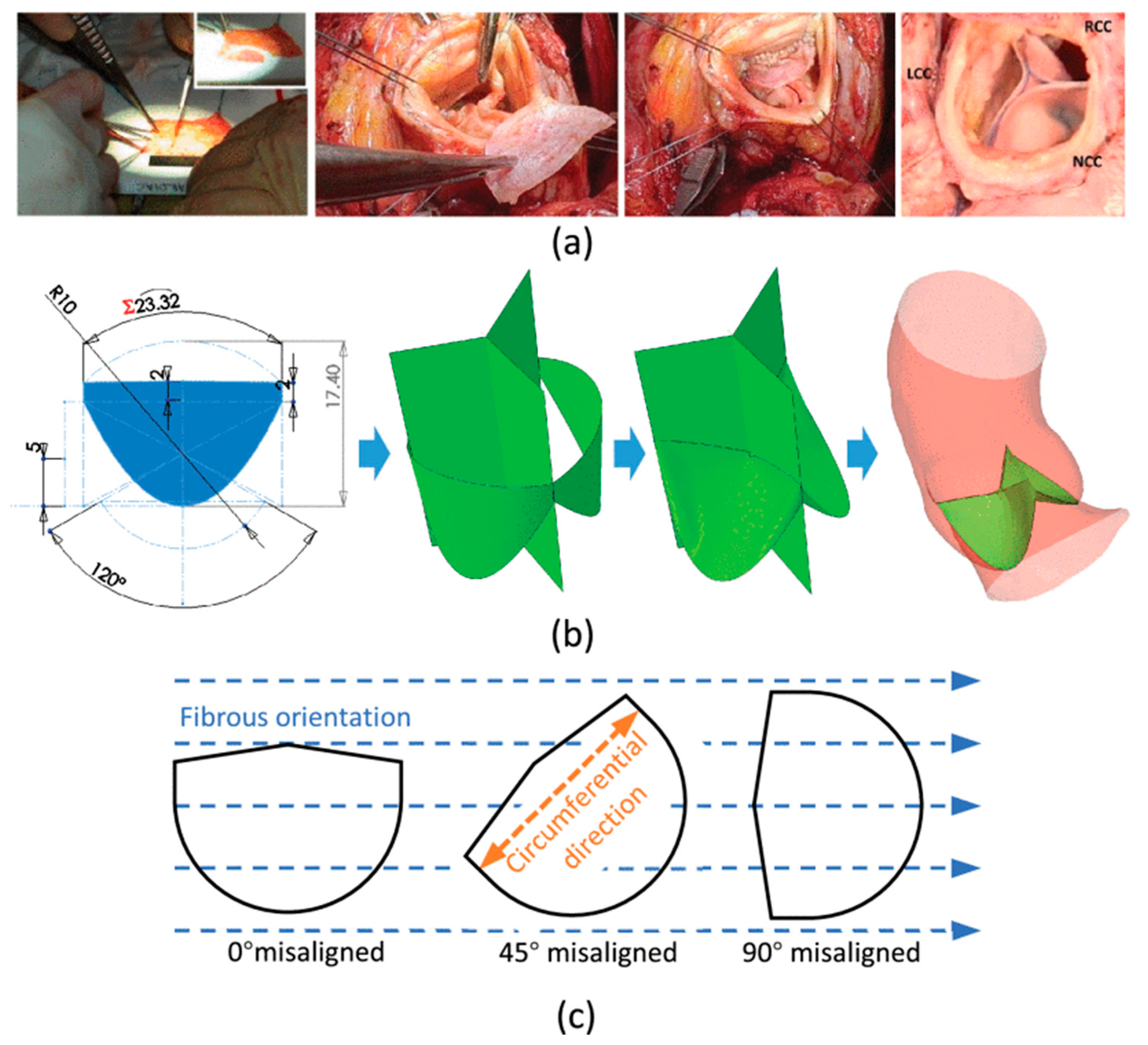
- Ozaki, S.; Kawase, I.; Yamashita, H.; Uchida, S.; Takatoh, M.; Kiyohara, N. Midterm Outcomes after Aortic Valve Neocuspidization with Glutaraldehyde-Treated Autologous Pericardium. J. Thorac. Cardiovasc. Surg. 2018, 155, 2379–2387. [Google Scholar] [CrossRef]
- Ozaki, S.; Kawase, I.; Yamashita, H.; Uchida, S.; Takatoh, M.; Hagiwara, S.; Kiyohara, N. Aortic Valve Reconstruction Using Autologous Pericardium for Aortic Stenosis. Circ. J. 2015, 79, 1504–1510. [Google Scholar] [CrossRef]
- Ozaki, S.; Kawase, I.; Yamashita, H.; Uchida, S.; Nozawa, Y.; Takatoh, M.; Hagiwara, S. A Total of 404 Cases of Aortic Valve Reconstruction with Glutaraldehyde-Treated Autologous Pericardium. J. Thorac. Cardiovasc. Surg. 2014, 147, 301–306. [Google Scholar] [CrossRef]
- Saisho, H.; Scharfschwerdt, M.; Schaller, T.; Sadat, N.; Aboud, A.; Ensminger, S.; Fujita, B. Ex Vivo Evaluation of the Ozaki Procedure in Comparison with the Native Aortic Valve and Prosthetic Valves. Interact. Cardiovasc. Thorac. Surg. 2022, 35, ivac199. [Google Scholar] [CrossRef]
- Theodoridis, K.; Müller, J.; Ramm, R.; Findeisen, K.; Andrée, B.; Korossis, S.; Haverich, A.; Hilfiker, A. Effects of Combined Cryopreservation and Decellularization on the Biomechanical, Structural and Biochemical Properties of Porcine Pulmonary Heart Valves. Acta Biomater. 2016, 43, 71–77. [Google Scholar] [CrossRef]
- Hu, X.; Li, S.; Peng, P.; Wang, B.; Liu, W.; Dong, X.; Yang, X.; Karabaliev, M.; Yu, Q.; Gao, C. Prosthetic Heart Valves for Transcatheter Aortic Valve Replacement. BMEMat 2023, 1, e12026. [Google Scholar] [CrossRef]
- Nistal, F.; Garcia-Martinez, V.; Arbe, E.; Fernandez, D.; Artinano, E.; Mazorra, F.; Gallo, I. In Vivo Experimental Assessment of Polytetrafluoroethylene Trileaflet Heart Valve Prosthesis. J. Thorac. Cardiovasc. Surg. 1990, 99, 1074–1081. [Google Scholar] [CrossRef]
- Kütting, M.; Roggenkamp, J.; Urban, U.; Schmitz-Rode, T.; Steinseifer, U. Polyurethane Heart Valves: Past, Present and Future. Expert Rev. Med. Devices 2011, 8, 227–233. [Google Scholar] [CrossRef]
- Singh, S.K.; Kachel, M.; Castillero, E.; Xue, Y.; Kalfa, D.; Ferrari, G.; George, I. Polymeric Prosthetic Heart Valves: A Review of Current Technologies and Future Directions. Front. Cardiovasc. Med. 2023, 10, 1137827. [Google Scholar] [CrossRef] [PubMed]
- Li, R.L.; Russ, J.; Paschalides, C.; Ferrari, G.; Waisman, H.; Kysar, J.W.; Kalfa, D. Mechanical Considerations for Polymeric Heart Valve Development: Biomechanics, Materials, Design and Manufacturing. Biomaterials 2019, 225, 119493. [Google Scholar] [CrossRef] [PubMed]
- Kuchumov, A.G.; Nyashin, Y.I.; Samartsev, V.A. Modelling of Peristaltic Bile Flow in the Papilla Ampoule with Stone and in the Papillary Stenosis Case: Application to Reflux Investigation. IFMBE Proc. 2015, 52, 158–161. [Google Scholar]
- Kuchumov, A.G.; Nyashin, Y.I.; Samarcev, V.A.; Gavrilov, V.A. Modelling of the Pathological Bile Flow in the Duct with a Calculus. Acta Bioeng. Biomech. 2013, 15, 9–17. [Google Scholar] [CrossRef] [PubMed]
- Kuchumov, A.G.; Gilev, V.; Popov, V.; Samartsev, V.; Gavrilov, V. Non-Newtonian Flow of Pathological Bile in the Biliary System: Experimental Investigation and CFD Simulations. Korea Aust. Rheol. J. 2014, 26, 81–90. [Google Scholar] [CrossRef]
- Kuchumov, A.G.; Khairulin, A.; Shmurak, M.; Porodikov, A.; Merzlyakov, A. The Effects of the Mechanical Properties of Vascular Grafts and an Anisotropic Hyperelastic Aortic Model on Local Hemodynamics during Modified Blalock–Taussig Shunt Operation, Assessed Using FSI Simulation. Materials 2022, 15, 2719. [Google Scholar] [CrossRef]
- Kuchumov, A.G.; Vedeneev, V.; Samartsev, V.; Khairulin, A.; Ivanov, O. Patient-Specific Fluid–Structure Interaction Model of Bile Flow: Comparison between 1-Way and 2-Way Algorithms. Comput. Methods Biomech. Biomed. Eng. 2021, 24, 1693–1717. [Google Scholar] [CrossRef] [PubMed]
- Zakerzadeh, R.; Hsu, M.C.; Sacks, M.S. Computational Methods for the Aortic Heart Valve and Its Replacements. Expert Rev. Med. Devices 2017, 14, 849–866. [Google Scholar] [CrossRef]
- Tahir, A.M.; Mutlu, O.; Bensaali, F.; Ward, R.; Ghareeb, A.N.; Helmy, S.M.H.A.; Othman, K.T.; Al-Hashemi, M.A.; Abujalala, S.; Chowdhury, M.E.H.; et al. Latest Developments in Adapting Deep Learning for Assessing TAVR Procedures and Outcomes. J. Clin. Med. 2023, 12, 4774. [Google Scholar] [CrossRef]
- McLoone, M.; Quinlan, N.J. Coupling of the Meshless Finite Volume Particle Method and the Finite Element Method for Fluid–Structure Interaction with Thin Elastic Structures. Eur. J. Mech. B Fluids 2022, 92, 117–131. [Google Scholar] [CrossRef]
- Diniz dos Santos, N.; Gerbeau, J.F.; Bourgat, J.F. A Partitioned Fluid-Structure Algorithm for Elastic Thin Valves with Contact. Comput. Methods Appl. Mech. Eng. 2008, 197, 1750–1761. [Google Scholar] [CrossRef]
- Feng, Y.; Cao, Y.; Wang, W.; Zhang, H.; Wei, L.; Jia, B.; Wang, S. Computational Modeling for Surgical Reconstruction of Aortic Valve by Using Autologous Pericardium. IEEE Access 2020, 8, 97343–97352. [Google Scholar] [CrossRef]
- Fernández, M.A.; Moubachir, M. A Newton Method Using Exact Jacobians for Solving Fluid-Structure Coupling. Comput. Struct. 2005, 83, 127–142. [Google Scholar] [CrossRef]
- Shadden, S.C.; Astorino, M.; Gerbeau, J.F. Computational Analysis of an Aortic Valve Jet with Lagrangian Coherent Structures. Chaos 2010, 20, 017512. [Google Scholar] [CrossRef]
- Cheng, R.; Lai, Y.G.; Chandran, K.B. Three-Dimensional Fluid-Structure Interaction Simulation of Bileaflet Mechanical Heart Valve Flow Dynamics. Ann. Biomed. Eng. 2004, 32, 1471–1483. [Google Scholar] [CrossRef]
- Dumont, K.; Vierendeels, J.; Kaminsky, R.; Van Nooten, G.; Verdonck, P.; Bluestein, D. Comparison of the Hemodynamic and Thrombogenic Performance of Two Bileaflet Mechanical Heart Valves Using a CFD/FSI Model. J. Biomech. Eng. 2007, 129, 558–565. [Google Scholar] [CrossRef]
- Kandail, H.S.; Trivedi, S.D.; Shaikh, A.C.; Bajwa, T.K.; O’Hair, D.P.; Jahangir, A.; LaDisa, J.F. Impact of Annular and Supra-Annular CoreValve Deployment Locations on Aortic and Coronary Artery Hemodynamics. J. Mech. Behav. Biomed. Mater. 2018, 86, 131–142. [Google Scholar] [CrossRef]
- Abbas, S.S.; Nasif, M.S.; Al-Waked, R. State-of-the-Art Numerical Fluid–Structure Interaction Methods for Aortic and Mitral Heart Valves Simulations: A Review. Simulation 2022, 98, 3–34. [Google Scholar] [CrossRef]
- Nejadmalayeri, A.; Hoffmann, K.A.; Dietiker, J.F. Numerical Simulation of Pulsatile Blood Flow across a Tilting-Disk Valve. In Proceedings of the Collection of Technical Papers-37th AIAA Fluid Dynamics Conference, Miami, FL, USA, 25–28 June 2007; Volume 3. [Google Scholar]
- De Vita, F.; de Tullio, M.D.; Verzicco, R. Numerical Simulation of the Non-Newtonian Blood Flow through a Mechanical Aortic Valve: Non-Newtonian Blood Flow in the Aortic Root. Theor. Comput. Fluid Dyn. 2016, 30, 129–138. [Google Scholar] [CrossRef]
- Hedayat, M.; Asgharzadeh, H.; Borazjani, I. Platelet Activation of Mechanical versus Bioprosthetic Heart Valves during Systole. J. Biomech. 2017, 56, 111–116. [Google Scholar] [CrossRef] [PubMed]
- Li, W.-Q.; Gao, Z.-X.; Jin, Z.-J.; Qian, J.-Y. Transient Study of Flow and Cavitation inside a Bileaflet Mechanical Heart Valve. Appl. Sci. 2020, 10, 2548. [Google Scholar] [CrossRef]
- Kuan, Y.H.; Kabinejadian, F.; Nguyen, V.T.; Su, B.; Yoganathan, A.P.; Leo, H.L. Comparison of Hinge Microflow Fields of Bileaflet Mechanical Heart Valves Implanted in Different Sinus Shape and Downstream Geometry. Comput. Methods Biomech. Biomed. Eng. 2015, 18, 1785–1796. [Google Scholar] [CrossRef]
- Yun, B.M.; Wu, J.; Simon, H.A.; Arjunon, S.; Sotiropoulos, F.; Aidun, C.K.; Yoganathan, A.P. A Numerical Investigation of Blood Damage in the Hinge Area of Aortic Bileaflet Mechanical Heart Valves during the Leakage Phase. Ann. Biomed. Eng. 2012, 40, 1468–1485. [Google Scholar] [CrossRef]
- Simon, H.A.; Ge, L.; Sotiropoulos, F.; Yoganathan, A.P. Numerical Investigation of the Performance of Three Hinge Designs of Bileaflet Mechanical Heart Valves. Ann. Biomed. Eng. 2010, 38, 3295–3310. [Google Scholar] [CrossRef][Green Version]
- Abbas, S.S.; Nasif, M.S.; Said, M.A.M.; Al-Waked, R. Numerical Simulation of the Non-Newtonian Blood Flow through Aortic Bileaflet Mechanical Heart Valve Using Fluid-Structure Interaction Approach. In Proceedings of the AIP Conference Proceedings, Kuala Lumpur, Malaysia, 13–14 August 2018; Volume 2035. [Google Scholar]
- Dasi, L.P.; Sucosky, P.; De Zelicourt, D.; Sundareswaran, K.; Jimenez, J.; Yoganathan, A.P. Advances in Cardiovascular Fluid Mechanics: Bench to Bedside. Ann. N. Y. Acad. Sci. 2009, 1161, 1–25. [Google Scholar] [CrossRef]
- Khellaf, B.; Boussad, B. Computational Hemodynamic Investigation of a New Bileaflet Mechanical Heart Valve. Simulation 2020, 96, 459–469. [Google Scholar] [CrossRef]
- Hanafizadeh, P.; Mirkhani, N.; Davoudi, M.R.; Masouminia, M.; Sadeghy, K. Non-Newtonian Blood Flow Simulation of Diastolic Phase in Bileaflet Mechanical Heart Valve Implanted in a Realistic Aortic Root Containing Coronary Arteries. Artif. Organs 2016, 40, E179–E191. [Google Scholar] [CrossRef]
- Simon, H.A.; Dasi, L.P.; Leo, H.L.; Yoganathan, A.P. Spatio-Temporal Flow Analysis in Bileaflet Heart Valve Hinge Regions: Potential Analysis for Blood Element Damage. Ann. Biomed. Eng. 2007, 35, 1333–1346. [Google Scholar] [CrossRef] [PubMed]
- Mohammadi, H.; Ahmadian, M.T.; Wan, W.K. Time-Dependent Analysis of Leaflets in Mechanical Aortic Bileaflet Heart Valves in Closing Phase Using the Finite Strip Method. Med. Eng. Phys. 2006, 28, 122–133. [Google Scholar] [CrossRef] [PubMed]
- Amiri Delouei, A.; Nazari, M.; Kayhani, M.H.; Succi, S. Non-Newtonian Unconfined Flow and Heat Transfer over a Heated Cylinder Using the Direct-Forcing Immersed Boundary-Thermal Lattice Boltzmann Method. Phys. Rev. E Stat. Nonlin Soft Matter. Phys. 2014, 89, 053312. [Google Scholar] [CrossRef] [PubMed]
- Afra, B.; Delouei, A.A.; Tarokh, A. Flow-Induced Locomotion of a Flexible Filament in the Wake of a Cylinder in Non-Newtonian Flows. Int. J. Mech. Sci. 2022, 234, 107693. [Google Scholar] [CrossRef]
- Afra, B.; Karimnejad, S.; Amiri Delouei, A.; Tarokh, A. Flow Control of Two Tandem Cylinders by a Highly Flexible Filament: Lattice Spring IB-LBM. Ocean Eng. 2022, 250, 111025. [Google Scholar] [CrossRef]
- Gilmanov, A.; Barker, A.; Stolarski, H.; Sotiropoulos, F. Image-Guided Fluid-Structure Interaction Simulation of Transvalvular Hemodynamics: Quantifying the Effects of Varying Aortic Valve Leaflet Thickness. Fluids 2019, 4, 119. [Google Scholar] [CrossRef]
- Gilmanov, A.; Stolarski, H.; Sotiropoulos, F. Non-Linear Rotation-Free Shell Finite-Element Models for Aortic Heart Valves. J. Biomech. 2017, 50, 56–62. [Google Scholar] [CrossRef]
- Gilmanov, A.; Stolarski, H.; Sotiropoulos, F. Flow-Structure Interaction Simulations of the Aortic Heart Valve at Physiologic Conditions: The Role of Tissue Constitutive Model. J. Biomech. Eng. 2018, 140, 041003. [Google Scholar] [CrossRef]
- Chen, Y.; Luo, H. A Computational Study of the Three-Dimensional Fluid–Structure Interaction of Aortic Valve. J. Fluids Struct. 2018, 80, 332–349. [Google Scholar] [CrossRef]
- Laadhari, A.; Székely, G. Eulerian Finite Element Method for the Numerical Modeling of Fluid Dynamics of Natural and Pathological Aortic Valves. J. Comput. Appl. Math. 2017, 319, 236–261. [Google Scholar] [CrossRef]
- Soltany Sadrabadi, M.; Hedayat, M.; Borazjani, I.; Arzani, A. Fluid-Structure Coupled Biotransport Processes in Aortic Valve Disease. J. Biomech. 2021, 117, 110239. [Google Scholar] [CrossRef]
- Torrado, A. Analysis of Hemodynamic Indicators in Bicuspid Aortic Valves Using a Computational Mathematical Model. Ph.D. Thesis, Instituto Superior Técnico, Lisboa, Portugal, 2015. [Google Scholar]
- Luraghi, G.; Migliavacca, F.; García-González, A.; Chiastra, C.; Rossi, A.; Cao, D.; Stefanini, G.; Rodriguez Matas, J.F. On the Modeling of Patient-Specific Transcatheter Aortic Valve Replacement: A Fluid–Structure Interaction Approach. Cardiovasc. Eng. Technol. 2019, 10, 437–455. [Google Scholar] [CrossRef] [PubMed]
- Luraghi, G.; Wu, W.; De Gaetano, F.; Rodriguez Matas, J.F.; Moggridge, G.D.; Serrani, M.; Stasiak, J.; Costantino, M.L.; Migliavacca, F. Evaluation of an Aortic Valve Prosthesis: Fluid-Structure Interaction or Structural Simulation? J. Biomech. 2017, 58, 45–51. [Google Scholar] [CrossRef] [PubMed]
- Johnson, N.P.; Zelis, J.M.; Tonino, P.A.L.; Houthuizen, P.; Bouwman, R.A.; Brueren, G.R.G.; Johnson, D.T.; Koolen, J.J.; Korsten, H.H.M.; Wijnbergen, I.F.; et al. Pressure Gradient vs. Flow Relationships to Characterize the Physiology of a Severely Stenotic Aortic Valve before and after Transcatheter Valve Implantation. Eur. Heart J. 2018, 39, 2646–2655. [Google Scholar] [CrossRef] [PubMed]
- Zelis, J.M.; Tonino, P.A.L.; Johnson, D.T.; Balan, P.; Brueren, G.R.G.; Wijnbergen, I.; Kirkeeide, R.L.; Pijls, N.H.J.; Gould, K.L.; Johnson, N.P. Stress Aortic Valve Index (SAVI) with Dobutamine for Low-Gradient Aortic Stenosis: A Pilot Study. Struct. Heart 2020, 4, 53–61. [Google Scholar] [CrossRef]
- Biffi, B.; Bosi, G.M.; Lintas, V.; Jones, R.; Tzamtzis, S.; Burriesci, G.; Migliavacca, F.; Taylor, A.M.; Schievano, S.; Biglino, G. Numerical Model of a Valvuloplasty Balloon: In Vitro Validation in a Rapid-Prototyped Phantom. Biomed. Eng. Online 2016, 15, 37. [Google Scholar] [CrossRef]
- Luraghi, G.; Migliavacca, F.; Chiastra, C.; Rossi, A.; Reimers, B.; Stefanini, G.G.; Rodriguez Matas, J.F. Does Clinical Data Quality Affect Fluid-Structure Interaction Simulations of Patient-Specific Stenotic Aortic Valve Models? J. Biomech. 2019, 94, 202–210. [Google Scholar] [CrossRef]
- Martin, C.; Sun, W. Transcatheter Valve Underexpansion Limits Leaflet Durability: Implications for Valve-in-Valve Procedures. Ann. Biomed. Eng. 2017, 45, 394–404. [Google Scholar] [CrossRef]
- Dasi, L.P.; Hatoum, H.; Kheradvar, A.; Zareian, R.; Alavi, S.H.; Sun, W.; Martin, C.; Pham, T.; Wang, Q.; Midha, P.A.; et al. On the Mechanics of Transcatheter Aortic Valve Replacement. Ann. Biomed. Eng. 2017, 45, 310–331. [Google Scholar] [CrossRef]
- Pasta, S.; Cannata, S.; Gentile, G.; Di Giuseppe, M.; Cosentino, F.; Pasta, F.; Agnese, V.; Bellavia, D.; Raffa, G.M.; Pilato, M.; et al. Simulation Study of Transcatheter Heart Valve Implantation in Patients with Stenotic Bicuspid Aortic Valve. Med. Biol. Eng. Comput. 2020, 58, 815–829. [Google Scholar] [CrossRef] [PubMed]
- Pasta, S.; Gandolfo, C. Computational Analysis of Self-Expanding and Balloon-Expandable Transcatheter Heart Valves. Biomechanics 2021, 1, 43–52. [Google Scholar] [CrossRef]
- Van Aswegen, K.H.J.; Smuts, A.N.; Scheffer, C.; Weich, H.S.V.; Doubell, A.F. Investigation of Leaflet Geometry in a Percutaneous Aortic Valve with the Use of Fluid-Structure Interaction Simulation. J. Mech. Med. Biol. 2012, 12, 1250003. [Google Scholar] [CrossRef]
- Govindarajan, V.; Kolanjiyil, A.; Johnson, N.P.; Kim, H.; Chandran, K.B.; McPherson, D.D. Improving Transcatheter Aortic Valve Interventional Predictability via Fluid-Structure Interaction Modelling Using Patient-Specific Anatomy. R Soc. Open Sci. 2022, 9, 211694. [Google Scholar] [CrossRef] [PubMed]
- Weinberg, E.J.; Mofrad, M.R.K. Three-Dimensional, Multiscale Simulations of the Human Aortic Valve. Cardiovasc. Eng. 2007, 7, 140–155. [Google Scholar] [CrossRef] [PubMed]
- Conti, C.A.; Votta, E.; Della Corte, A.; Del Viscovo, L.; Bancone, C.; Cotrufo, M.; Redaelli, A. Dynamic Finite Element Analysis of the Aortic Root from MRI-Derived Parameters. Med. Eng. Phys. 2010, 32, 212–221. [Google Scholar] [CrossRef]
- Bianchi, D.; Monaldo, E.; Gizzi, A.; Marino, M.; Filippi, S.; Vairo, G. A FSI Computational Framework for Vascular Physiopathology: A Novel Flow-Tissue Multiscale Strategy. Med. Eng. Phys. 2017, 47, 25–37. [Google Scholar] [CrossRef]
- Pasta, S.; Cannata, S.; Gentile, G.; Agnese, V.; Raffa, G.M.; Pilato, M.; Gandolfo, C. Transcatheter Heart Valve Implantation in Bicuspid Patients with Self-Expanding Device. Bioengineering 2021, 8, 91. [Google Scholar] [CrossRef]
- Colombo, A.; Latib, A. Bicuspid Aortic Valve: Any Room for TAVR? J. Am. Coll Cardiol. 2014, 64, 2340–2342. [Google Scholar] [CrossRef][Green Version]
- Guyton, R.A.; Padala, M. Transcatheter Aortic Valve Replacement in Bicuspid Aortic Stenosis Early Success but Concerning Red Flags. JACC Cardiovasc. Interv. 2016, 9, 825–827. [Google Scholar] [CrossRef]
- Makkar, R.; Chakravarty, T.; Jilaihawi, H. Transcatheter Aortic Valve Replacement for Bicuspid Aortic Stenosis: Are We Ready for the Challenge? J. Am. Coll Cardiol. 2016, 68, 1206–1208. [Google Scholar] [CrossRef]
- Esmailie, F.; Razavi, A.; Yeats, B.; Sivakumar, S.K.; Chen, H.; Samaee, M.; Shah, I.A.; Veneziani, A.; Yadav, P.; Thourani, V.H.; et al. Biomechanics of Transcatheter Aortic Valve Replacement Complications and Computational Predictive Modeling. Struct. Heart 2022, 6, 100032. [Google Scholar] [CrossRef]
- Dalmau, M.J.; González-Santos, J.M.; Blázquez, J.A.; Sastre, J.A.; López-Rodríguez, J.; Bueno, M.; Castaño, M.; Arribas, A. Hemodynamic Performance of the Medtronic Mosaic and Perimount Magna Aortic Bioprostheses: Five-Year Results of a Prospectively Randomized Study. Eur. J. Cardio-Thorac. Surg. 2011, 39, 844–852. [Google Scholar] [CrossRef]
- Andreas, M.; Wallner, S.; Ruetzler, K.; Wiedemann, D.; Ehrlich, M.; Heinze, G.; Binder, T.; Moritz, A.; Hiesmayr, M.J.; Kocher, A.; et al. Comparable Long-Term Results for Porcine and Pericardial Prostheses after Isolated Aortic Valve Replacement. Eur. J. Cardio-Thorac. Surg. 2015, 48, 557–561. [Google Scholar] [CrossRef]
- Wagner, I.M.; Eichinger, W.B.; Bleiziffer, S.; Botzenhardt, F.; Gebauer, I.; Guenzinger, R.; Bauernschmitt, R.; Lange, R. Influence of Completely Supra-Annular Placement of Bioprostheses on Exercise Hemodynamics in Patients with a Small Aortic Annulus. J. Thorac. Cardiovasc. Surg. 2007, 133, 1234–1241. [Google Scholar] [CrossRef]
- Yap, K.H.; Murphy, R.; Devbhandari, M.; Venkateswaran, R. Aortic Valve Replacement: Is Porcine or Bovine Valve Better? Interact. Cardiovasc. Thorac. Surg. 2013, 16, 361–373. [Google Scholar] [CrossRef]
- Borger, M.A.; Nette, A.F.; Maganti, M.; Feindel, C.M. Carpentier-Edwards Perimount Magna Valve Versus Medtronic Hancock II: A Matched Hemodynamic Comparison. Ann. Thorac. Surg. 2007, 83, 2054–2058. [Google Scholar] [CrossRef]
- Marn, J.; Iljaž, J.; Žunič, Z.; Ternik, P. Non-Newtonian Blood Flow around Healthy and Regurgitated Aortic Valve with Coronary Blood Flow Involved. Stroj. Vestn. J. Mech. Eng. 2012, 58, 482–491. [Google Scholar] [CrossRef]
- Wong, I.; Sondergaard, L.; De Backer, O. Computational Simulation Models to Test Bioprosthetic Aortic Valves: A Valuable Alternative or Addition to Bench Testing? Int. J. Cardiol. 2021, 340, 66–67. [Google Scholar] [CrossRef]
- Chan, V.; Kulik, A.; Tran, A.; Hendry, P.; Masters, R.; Mesana, T.G.; Ruel, M. Long-Term Clinical and Hemodynamic Performance of the Hancock II versus the Perimount Aortic Bioprostheses. Circulation 2010, 122, S10–S16. [Google Scholar] [CrossRef]
- Hickey, G.L.; Grant, S.W.; Bridgewater, B.; Kendall, S.; Bryan, A.J.; Kuo, J.; Dunning, J. A Comparison of Outcomes between Bovine Pericardial and Porcine Valves in 38 040 Patients in England and Wales over 10 Years. Eur. J. Cardio-Thorac. Surg. 2014, 47, 1067–1074. [Google Scholar] [CrossRef]
- Einstein, D.R.; Kunzelman, K.S.; Reinhall, P.G.; Nicosia, M.A.; Cochran, R.P. Non-Linear Fluid-Coupled Computational Model of the Mitral Valve. J. Heart Valve Dis. 2005, 14, 376–385. [Google Scholar] [PubMed]
- Huang, H.S. Micromechanical Simulations of Heart Valve Tissues. Ph.D. Thesis, University of Pittsburgh, Pittsburgh, PA, USA, 2004. [Google Scholar]
- Khang, A.; Buchanan, R.M.; Ayoub, S.; Rego, B.V.; Lee, C.H.; Ferrari, G.; Anseth, K.S.; Sacks, M.S. Mechanobiology of the Heart Valve Interstitial Cell: Simulation, Experiment, and Discovery. In Mechanobiology in Health and Disease; Academic Press: Cambridge, MA, USA, 2018; pp. 249–283. ISBN 9780128129524. [Google Scholar] [CrossRef]
- Nowak, M.; Divo, E.; Adamczyk, W.P. Multiscale Model for Blood Flow after a Bileaflet Artificial Aortic Valve Implantation. Comput. Biol. Med. 2023, 158, 106805. [Google Scholar] [CrossRef]
- Borazjani, I.; Sotiropoulos, F. The Effect of Implantation Orientation of a Bileaflet Mechanical Heart Valve on Kinematics and Hemodynamics in an Anatomic Aorta. J. Biomech. Eng. 2010, 132, 111005. [Google Scholar] [CrossRef]
- Allen, P.; Robertson, R. The Significance of Intermittent Regurgitation in Aortic Valve Prostheses. J. Thorac. Cardiovasc. Surg. 1967, 54, 549–552. [Google Scholar] [CrossRef]
- Khalili, F.; Gamage, P.P.T.; Sandler, R.H.; Mansy, H.A. Adverse Hemodynamic Conditions Associated with Mechanical Heart Valve Leaflet Immobility. Bioengineering 2018, 5, 74. [Google Scholar] [CrossRef]
- Le, T.B.; Usta, M.; Aidun, C.; Yoganathan, A.; Sotiropoulos, F. Computational Methods for Fluid-Structure Interaction Simulation of Heart Valves in Patient-Specific Left Heart Anatomies. Fluids 2022, 7, 94. [Google Scholar] [CrossRef]
- Mourato, A.; Valente, R.; Xavier, J.; Brito, M.; Avril, S.; de Sá, J.C.; Tomás, A.; Fragata, J. Computational Modelling and Simulation of Fluid Structure Interaction in Aortic Aneurysms: A Systematic Review and Discussion of the Clinical Potential. Appl. Sci. 2022, 12, 8049. [Google Scholar] [CrossRef]
- Kuchumov, A.G.; Nyashin, Y.I.; Samartsev, V.A.; Tuktamyshev, V.S.; Lokhov, V.A.; Shestakov, A.P. Mathematical Modelling of Shape Memory Stent Placing at Endobiliary Interventions. Russ. J. Biomech. 2017, 21, 394–404. [Google Scholar]
- Sinelnikov, Y.S.; Arutunyan, V.B.; Porodikov, A.A.; Biyanov, A.N.; Tuktamyshev, V.S.; Shmurak, M.I.; Khairulin, A.R.; Kuchumov, A.G. Application of Mathematical Modelling for the Evaluation of the Results of Systemic-Pulmonary Shunts Formation. Patol. Krovoobrashcheniya I Kardiokhirurgiya 2020, 24, 45–61. [Google Scholar] [CrossRef]
- Kuchumov, A.G.; Kamaltdinov, M.R.; Samartsev, V.A.; Khairulin, A.R.; Ivashova, Y.A.; Taiar, R. Patient-Specific Simulation of a Gallbladder Refilling Based on MRI and Ultrasound in Vivo Measurements. AIP Conf. Proc. 2020, 2216, 060004. [Google Scholar]
- Kuchumov, A.G.; Khairulin, A.R.; Biyanov, A.N.; Porodikov, A.A.; Arutyunyan, V.B.; Sinelnikov, Y.S. Effectiveness of Blalock-Taussig Shunt Performance in the Congenital Heart Disease Children. Russ. J. Biomech. 2020, 24, 65–83. [Google Scholar]
- Bailoor, S.; Seo, J.H.; Dasi, L.P.; Schena, S.; Mittal, R. A Computational Study of the Hemodynamics of Bioprosthetic Aortic Valves with Reduced Leaflet Motion. J. Biomech. 2021, 120, 110350. [Google Scholar] [CrossRef] [PubMed]
- Lone, T.; Alday, A.; Zakerzadeh, R. Numerical Analysis of Stenoses Severity and Aortic Wall Mechanics in Patients with Supravalvular Aortic Stenosis. Comput. Biol. Med. 2021, 135, 104573. [Google Scholar] [CrossRef] [PubMed]
- Sigüenza, J.; Pott, D.; Mendez, S.; Sonntag, S.J.; Kaufmann, T.A.S.; Steinseifer, U.; Nicoud, F. Fluid-Structure Interaction of a Pulsatile Flow with an Aortic Valve Model: A Combined Experimental and Numerical Study. Int. J. Numer. Method Biomed. Eng. 2018, 34, e2945. [Google Scholar] [CrossRef]
- Bavo, A.M.; Rocatello, G.; Iannaccone, F.; Degroote, J.; Vierendeels, J.; Segers, P. Fluid-Structure Interaction Simulation of Prosthetic Aortic Valves: Comparison between Immersed Boundary and Arbitrary Lagrangian-Eulerian Techniques for the Mesh Representation. PLoS ONE 2016, 11, e0154517. [Google Scholar] [CrossRef] [PubMed]
- Carmody, C.J.; Burriesci, G.; Howard, I.C.; Patterson, E.A. An Approach to the Simulation of Fluid-Structure Interaction in the Aortic Valve. J. Biomech. 2006, 39, 158–169. [Google Scholar] [CrossRef]
- Bucelli, M.; Zingaro, A.; Africa, P.C.; Fumagalli, I.; Dede’, L.; Quarteroni, A. A Mathematical Model That Integrates Cardiac Electrophysiology, Mechanics, and Fluid Dynamics: Application to the Human Left Heart. Int. J. Numer. Method Biomed. Eng. 2023, 39, e3678. [Google Scholar] [CrossRef] [PubMed]
- Engel, M.; Griebel, M. Flow Simulation on Moving Boundary-Fitted Grids and Application to Fluid-Structure Interaction Problems. Int. J. Numer. Methods Fluids 2006, 50, 437–468. [Google Scholar] [CrossRef]
- Tango, A.M.; Salmonsmith, J.; Ducci, A.; Burriesci, G. Validation and Extension of a Fluid–Structure Interaction Model of the Healthy Aortic Valve. Cardiovasc. Eng. Technol. 2018, 9, 739–751. [Google Scholar] [CrossRef]








| Article + Year | Application | Revised Model | Personalized Model | Analyzed Characteristics |
|---|---|---|---|---|
| Michael et al. 2019 [21] | TAVR | FSI | No | Radial force |
| Bosi et al. 2018 [22] | TAVR | Matlab, Abaqus | Yes | Influence of chosen material on valve diameter |
| Amindari et al. 2021 [23] | TAVR | FSI | No | Aortic ring size, valve area, jet velocity |
| Mao et al. 2018 [24] | TAVR | CFD | Yes | Deployment height |
| Sturla et al. 2013 [25] | Aortic root | FSI | No | Tissue deformation and stress |
| Wu et al. 2016 [26] | TAVR | FSI | Yes | Pressure and velocities |
| Kalyana et al. 2015 [27] | Aortic valve | FSI | No | Pressure and flow |
| Tzamtzis et al. 2013 [28] | TAVR | MSC | No | Radial force |
| Bosmans et al. 2016 [29] | TAVR | FEM | Yes | Influence of chosen material on valve diameter, leakage |
| Finotello et al. 2017 [30] | TAVI | Matlab, Abaqus | Yes | Material properties, discretization |
| Wang et al. 2015 [31] | TAVI | FSI | No | Stress |
| Dowling et al. 2019 [32] | TAVR | CFD | Yes | Size and depth of implantation |
| Bianchi et al. 2019 [33] | TAVR | CFD | Yes | Depth of implantation, stress, pressure, velocities, contact force, contact surface |
| Whelan et al. 2018 [34] | Bovine valve | Uniaxial tension | No | Tensile strength, strength at break, fatigue performance |
| Travaglino et al. 2020 [35] | Transcatheter aortic valve | Bayesian optimization | No | Stress, contact surface |
| Loon et al. 2004 [36] | Aortic valve | FSI | No | Pressure, shear stress, velocity |
| Li et al. 2021 [37] | Porcine and bovine valves | FSI | No | Blood flow velocity, systolic transverse pressure gradient, geometric surface, flux shear stress, strain/stress |
| Weinberg et al. 2008 [38] | Bicuspid and tricuspid valves | FSI | No | Deformation, dynamic tension, dependence of fluid velocity on radial position, surface curvature, dynamic proportions of cells |
| Hart et al. 2003 [39] | Aortic valve | FSI | No | Flow velocity, pressure |
| Nicosia et al. 2003 [40] | Aortic valve, aortic root | FEM LS-Dyna | No | Flow velocity, material |
| Weinberg et al. 2005 [41] | Valve cusps | FEM | No | Tissue deformation |
| Stella et al. 2007 [42] | Valve cusps | Biaxial tension | No | Radial stress, thickness |
| Weinberg et al. 2006 [43] | Valve cusps | FEM | No | Wall shear stress, strain |
| Liu et al. 2022 [44] | TAVI | FSI | No | Flow velocity, maximum principal stress distribution |
| Pil et al. 2023 [45] | Valve cusps | FSI | No | Wall shear stress, OSI, TAWSS |
| Abdi et al. 2023 [46] | Aortic root, aortic valve | FSI | No | Wall shear stress, OSI, TAWSS |
| Bosi et al. 2020 [47] | TAVI | FSI | Yes | max principal strain, prosthesis deployment |
| Morany et al. 2023 [48] | Aortic valve, aortic root | FSI | No | Velocity, maximum principal stress distribution, wall shear stress |
| Section | Summary |
|---|---|
| Numerical modeling during transplant valve implantation |
|
| Numerical modeling for comparing porcine and bovine aortic valves |
|
| Modeling at different scales of an organ |
|
| Ozaki procedure modeling |
|
| Numerical modeling of a bileaflet mechanical valve |
|
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kuchumov, A.G.; Makashova, A.; Vladimirov, S.; Borodin, V.; Dokuchaeva, A. Fluid–Structure Interaction Aortic Valve Surgery Simulation: A Review. Fluids 2023, 8, 295. https://doi.org/10.3390/fluids8110295
Kuchumov AG, Makashova A, Vladimirov S, Borodin V, Dokuchaeva A. Fluid–Structure Interaction Aortic Valve Surgery Simulation: A Review. Fluids. 2023; 8(11):295. https://doi.org/10.3390/fluids8110295
Chicago/Turabian StyleKuchumov, Alex G., Anastasiya Makashova, Sergey Vladimirov, Vsevolod Borodin, and Anna Dokuchaeva. 2023. "Fluid–Structure Interaction Aortic Valve Surgery Simulation: A Review" Fluids 8, no. 11: 295. https://doi.org/10.3390/fluids8110295
APA StyleKuchumov, A. G., Makashova, A., Vladimirov, S., Borodin, V., & Dokuchaeva, A. (2023). Fluid–Structure Interaction Aortic Valve Surgery Simulation: A Review. Fluids, 8(11), 295. https://doi.org/10.3390/fluids8110295








