Design of a Cryogenic Duplex Pressure-Swirl Atomizer through CFDs for the Cold Conservation of Marine Products
Abstract
:1. Introduction
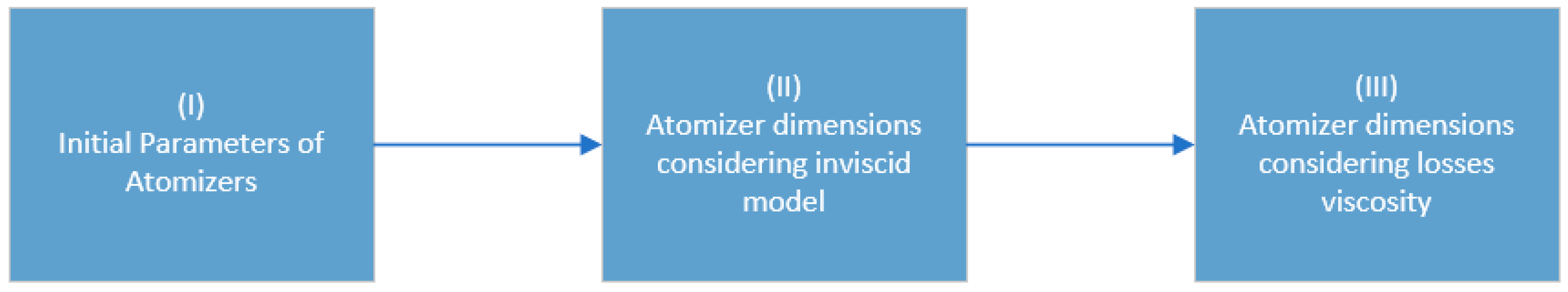
2. Mathematical Model
3. Numerical Simulation
- η: Volumetric fraction of fluid.
- Φ: Scalar.
- ρ: Density.
- : Velocity component in the i direction.
- : Diffusion coefficient for a scalar Φ.
- : Source term for a scalar Φ.
- : Nú Total number of phases.
- : Mass transfer coefficient between phases m and n.
- : Mass variation per unit volume of phase m to phase n.
- -
- Geometry design: CFDs enables the evaluation of different atomizer designs prior to physical fabrication.
- -
- Fluid-structure interaction: CFDs can be used to assess how the flow of a liquid and gas affects the atomizer’s structure and vice versa.
- -
- Virtual experiment design: CFDs enables virtual experiments under different operating conditions before physical implementation.
- -
- Interaction analysis with the environment: CFDs can simulate how atomized droplets interact with surfaces, air currents, or other fluids in the environment.
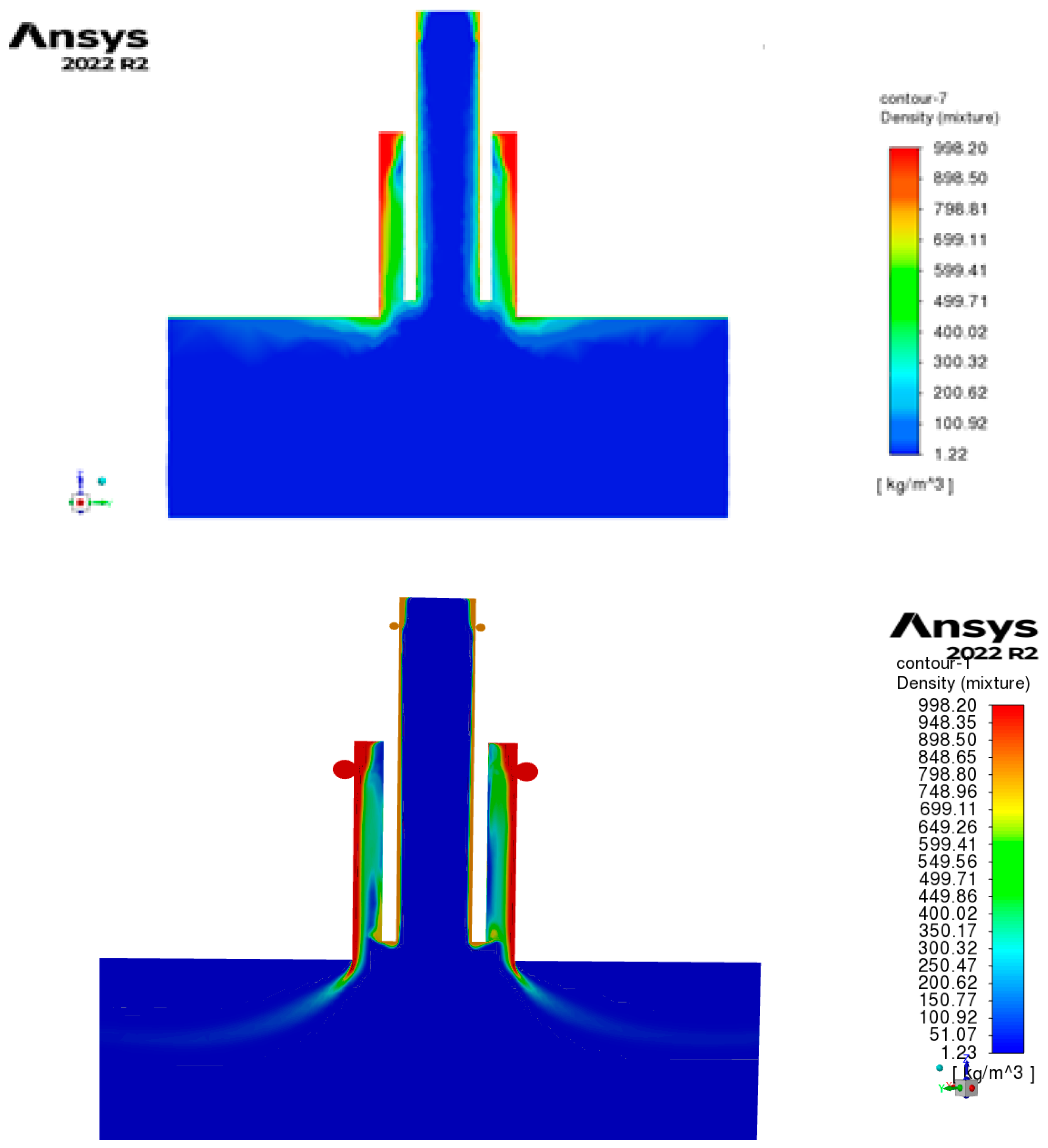
4. Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| A | geometrical characteristics parameter of pressure swirl atomizer of tangential inlets |
| AE | equivalent geometrical characteristics parameter due to the viscosity of swirl atomizers |
| Ac | geometrical characteristics parameter of conical pressure swirl atomizer |
| Cd | discharge coefficient |
| fp | cross-sectional area of inlet port |
| K | coefficient of loss due to liquid viscosity |
| n | number of inlet channels |
| mass flow rate | |
| ΔP | differential pressure |
| Re | Reynolds number |
| Rs | swirl chamber radius |
| Rinj | radius to axis inlet channel |
| ra | air core radius |
| ro | outlet orifice radius |
| u | vectorial velocity |
| Uin | inlet entrance velocity |
| U,W | velocities |
| Greek Symbols | |
| α | half-spray angle |
| ξ | losses Coefficient |
| η | volume fraction of fluid |
| φ | film flow area coefficient |
| Φ | scalar |
| λ | resistance coefficient of Blasius |
| μ | liquid absolute viscosity |
| ρ | liquid density |
| σ | liquid surface tension |
| v | liquid kinemátic viscosity |
| Subscripts | |
| a | air core |
| eq | equivalent parameter due to viscosity |
| inj | parameters related to inlet channels. |
| liq | liquid |
| r | radial component |
| s | swirl chamber. |
| tot | total |
| θ | tangential component |
| z | axial component |
References
- Sociedad Nacional de Pesquería. Industria Pesquera: Contribución a la Economía Peruana. 2018. Available online: https://www.snp.org.pe/relevancia-economica/ (accessed on 1 May 2023).
- Rodriguez, H. Propiedades del Nitrógeno (N). 2022. Available online: https://www.nationalgeographic.com.es/ciencia/propiedades-nitrogeno-n_18217 (accessed on 2 May 2023).
- Rivas, J.; Pimienta, A.; Rivas, G. Development of a mathematical model and 3D numerical simulation of the internal flow in a conical swirl atomizer. J. At. Sprays 2014, 24, 97–114. [Google Scholar] [CrossRef]
- Rivas, J.R.; Pimenta, A.P.; Salcedo, S.G.; Rivas, G.R.; Suazo, M.G. Study of internal flow of a bipropellant swirl injector of a rocket engine. J. Braz. Soc. Mech. Sci. Eng. 2018, 40, 289. [Google Scholar] [CrossRef]
- Çengel, Y.A.; Cimbala, J.M. Fluid Mechanics: Fundamentals and Applications; McGraw-Hill Education: New York, NY, USA, 2014. [Google Scholar]
- Lefebvre, A.H.; McDonell, V.G. Atomization and Sprays; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar]
- Elger, D.F.; Williams, D.C.; Crowe, C.T.; Roberson, J.A. Engineering Fluid Mechanics; John Wiley & Sons: Hoboken, NJ, USA, 2015. [Google Scholar]
- Fox, R.W.; McDonald, A.T.; Pritchard, P.J. Introduction to Fluid Mechanics; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Aminjan, K.K.; Kundu, B.; Ganji, D.D. Study of pressure swirl atomizer with tangential input at design point and outside of design point. Phys. Fluids 2020, 32, 127113. [Google Scholar] [CrossRef]
- Bayvel, L.; Orzechowski, T. Liquid Atomization; CRC Press: Boca Raton, FL, USA, 2006. [Google Scholar]
- Rivas, J.R.R. Modelo Matemático e Simulação Numérica do Spray em Injetores de Uso Aeroespacial. Ph.D. Dissertation, Instituto Tecnológico de Aeronáutica, São José dos Campos, SP, Brazil, 2015. [Google Scholar]
- Pimenta, A.P.; Lima, A.R. Analytical model for conical injector flows. In Proceedings of the International Congress Mechanical Engineering ABCM, Brasilia, Brazil, 5–9 November 2007. [Google Scholar]
- Fu, Q.-F.; Yang, L.-J.; Zhang, W.; Cui, K.-D. Spray Characteristics of an Open-End Swirl Injector. At. Sprays 2012, 22, 431–445. [Google Scholar] [CrossRef]
- Ronceros, J.; Porto, A.; Sumara, J.; Ronceros, G. An improved theoretical formulation for sauter mean diameter of pressure-swirl atomizers using geometrical parameter of atomization. Propuls. Power Res. 2022, 11, 240–252. [Google Scholar] [CrossRef]
- Reddy, K.U.; Mishra, D.P. Studies on spray behavior of pressure swirl atomizer in transition regime. J. Propul. Power 2008, 24, 74–80. [Google Scholar] [CrossRef]
- Savonov, R.I. Análise Numérica e Teórica de Injetores Tipo “Swirl” Empregados em Motores-Foguete a Propelente Líquido. Ph.D. Dissertation, Instituto Tecnológico de Aeronáutica, São José Dos Campos, SP, Brazil, 2011. [Google Scholar]
- Vehi Josep, M. Mallado de Geometrías Complejas Mediante CFD; Escuela Tecnica Superior de Ingenieria Industrial del Barcelona: Barcelona, Spain, 2016. [Google Scholar]
- Ochowiak, M.; Janecki, D.; Krupińska, A.; Włodarczak, S.; Wilk, T.; Olszewski, R. Conical Two-Phase Swirl Flow Atomizers—Numerical and Experimental Study. Energies 2021, 14, 1745. [Google Scholar] [CrossRef]
- Ronceros, G.A.R. Simulação Numérica da Convecção Forçada Turbulenta Acoplada à Condução de Calor em Dutos Retangulares. Ph.D. Dissertation, ITA—Instituto Tecnológico de Aeronáutica, São Jose dos Campos, SP, Brazil, 2010. [Google Scholar]
- ANSYS FLUENT Theory Guide Release 15.0; ANSYS Inc.: Canonsburg, PA, USA, 2013; Available online: http://www.pmt.usp.br/academic/martoran/notasmodelosgrad/ANSYS%20Fluent%20Theory%20Guide%2015.pdf (accessed on 20 May 2023).
- Hirt, C.W.; Nichols, B.D. Volume of fluid (VOF) Method for the Dynamics of Free Boundarys. J. Comput. Phys. 1981, 39, 201–225. [Google Scholar] [CrossRef]
- Natarajan, V.; Unnikrishnan, U.; Hwang, W.-S.; Choi, J.-Y.; Yang, V. Numerical study of two-phase flow dynamics and atomization in an open-type liquid swirl injector. Int. J. Multiph. Flow 2021, 143, 103702. [Google Scholar] [CrossRef]
- Roshdi, S.; Kasiri, N.; Rahbar-Kelishami, A. VOF simulation of single rising drops in three liquid-liquid extraction systems using csf and css interfacial force models. Braz. J. Chem. Eng. 2018, 35, 1315–1331. [Google Scholar] [CrossRef]
- Rajesh, V.M.; Vivek, B. Volume Of Fluid Simulations of Gas-Liquid—Liquid flow in minichannels. Chem. Eng. J. 2018, 345, 688–705. [Google Scholar] [CrossRef]
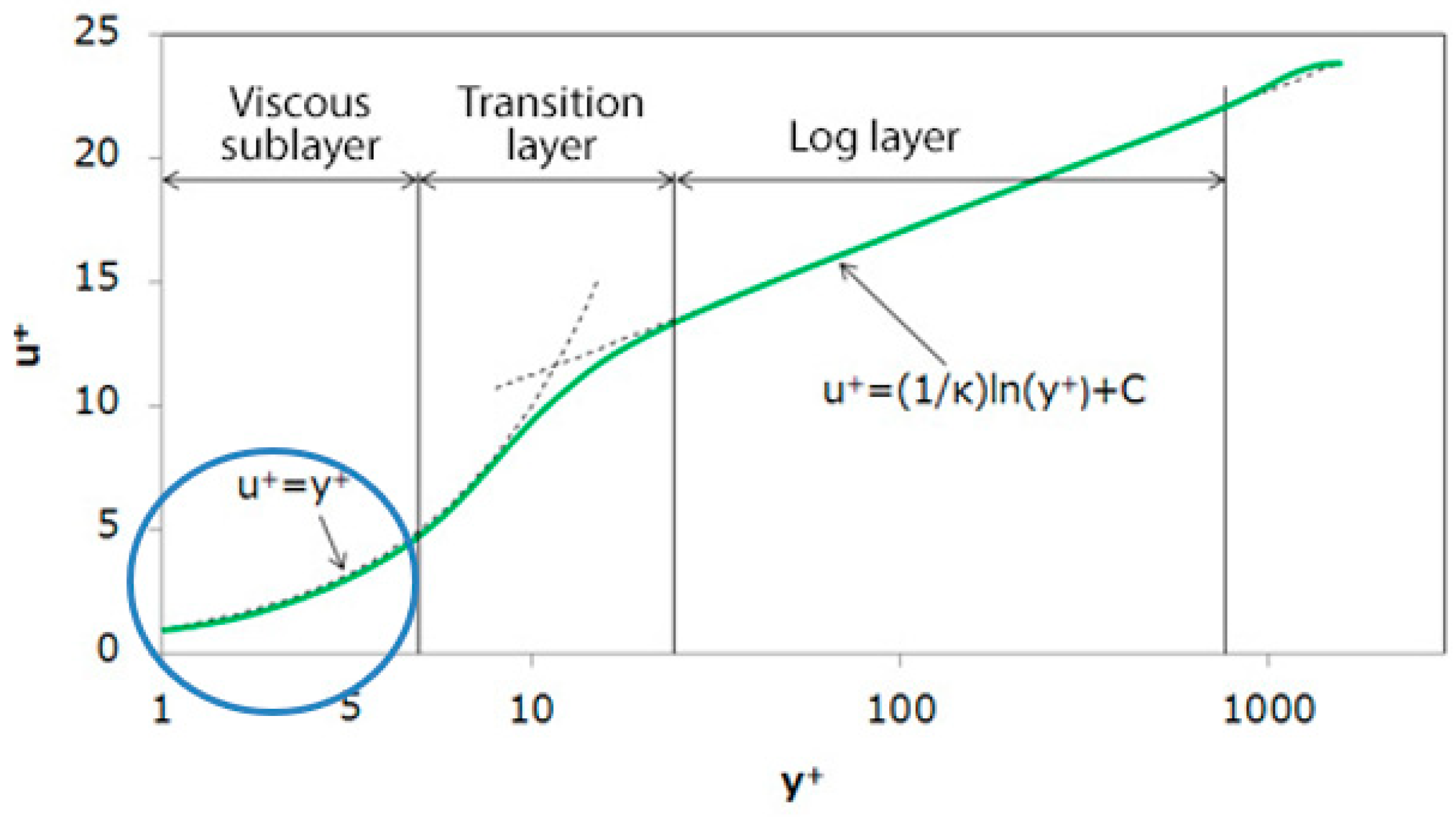
- Basic Course on Turbulence and Turbulent Flow Modeling 10: 10.1 Wall Condition of RANS Model, 10.2 Wall Function, 10.3 Turbulence Models and Wall Condition. 2019. Available online: https://www.cradle-cfd.com/media/column/a163 (accessed on 25 May 2023).
- ANSYS Inc. ANSYS Meshing User’s Guide; Release 13.0; ANSYS, Inc.: Canonsburg, PA, USA.
- Turbulence Modeling for CFD. pp. 87–90. Available online: http://ci.nii.ac.jp/ncid/BA21991687 (accessed on 30 May 2023).
- White, F.M. Fluid Mechanics; McGraw-Hill Education: New York, NY, USA, 2011. [Google Scholar]
- Weaver, D.S.; Miskovic, S. A study of RANS turbulence models in fully Turbulent jets: A perspective for CFD-DEM Simulations. Fluids 2021, 6, 271. [Google Scholar] [CrossRef]
- Blazek, J. Computational Fluid Dynamics: Principles and Applications, 2nd ed.; Elsevier Science: Amsterdam, The Netherlands, 2006. [Google Scholar]
- Ghaib, K. Introduction to Computational Fluid Dynamics; Springer: Berlin/Heidelberg, Germany, 2023. [Google Scholar]
- Liu, X.; Xue, R.; Ruan, Y.; Chen, L.; Zhang, X.; Hou, Y. Flow characteristics of liquid nitrogen through solid-cone pressure swirl nozzles. Appl. Therm. Eng. 2017, 110, 290297. [Google Scholar] [CrossRef]
- Zong, N.; Yang, V. Cryogenic fluid dynamics of pressure swirl injectors at supercritical conditions. Phys. Fluids 2008, 20, 056103. [Google Scholar] [CrossRef]
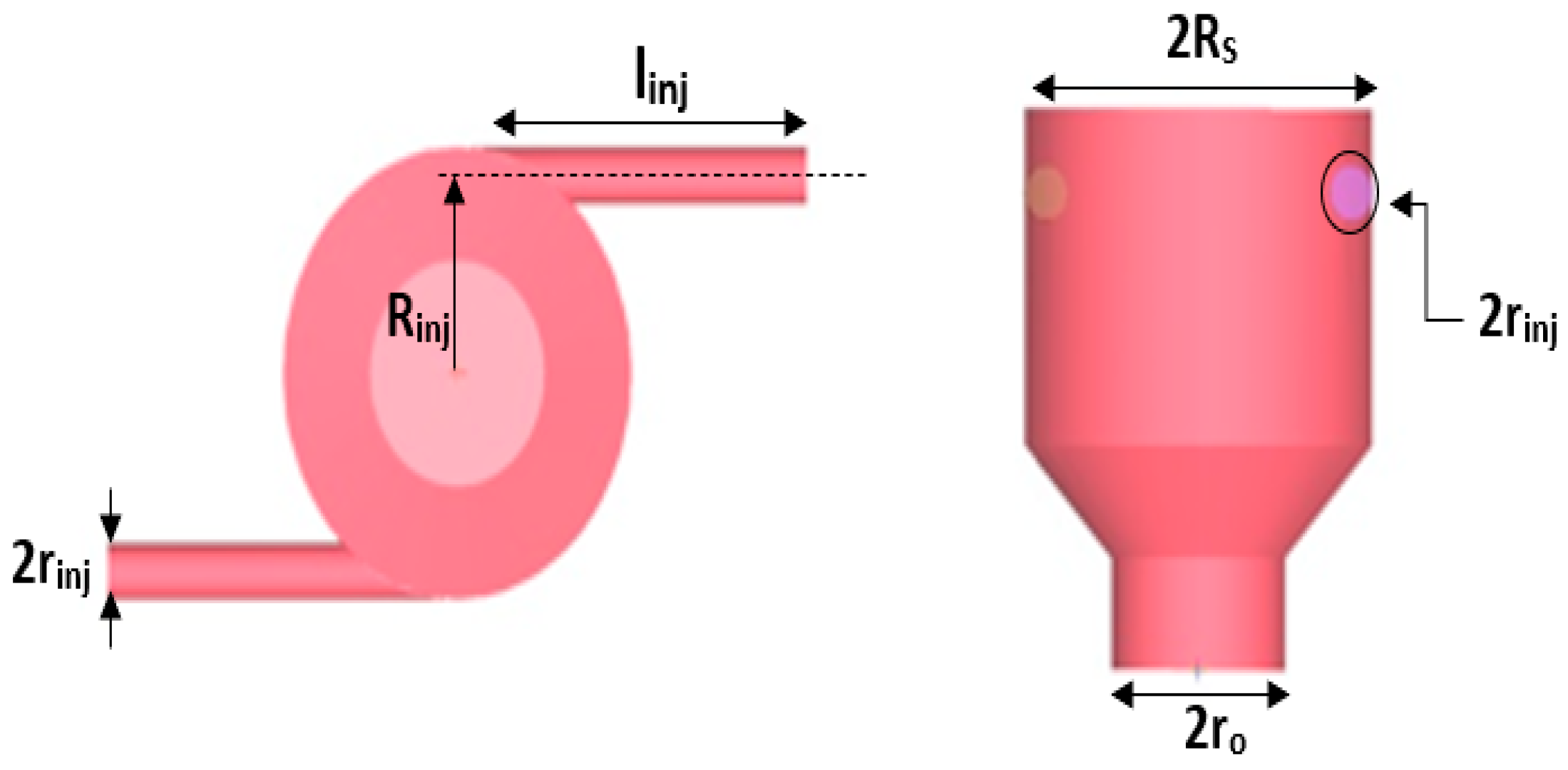
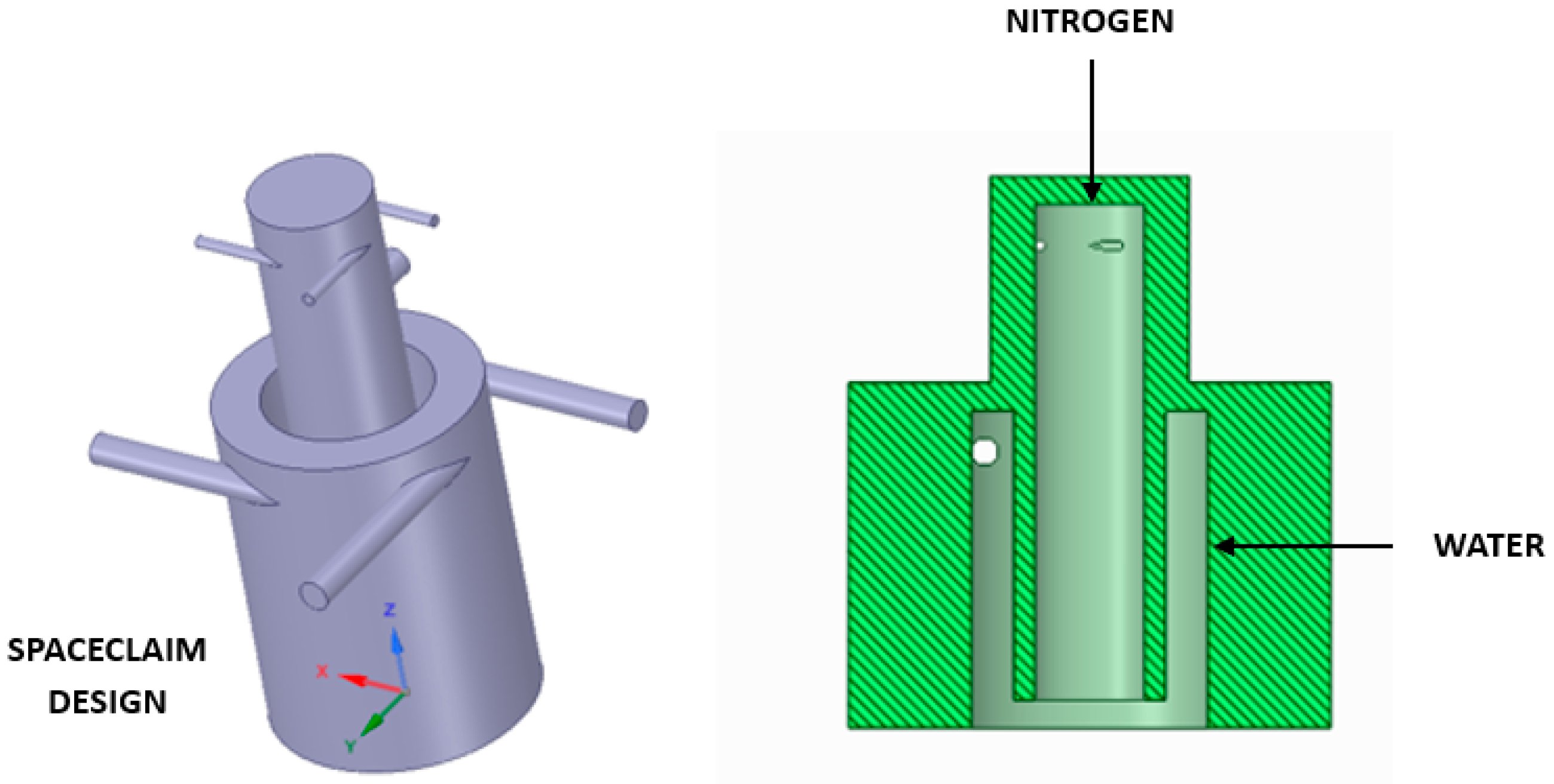

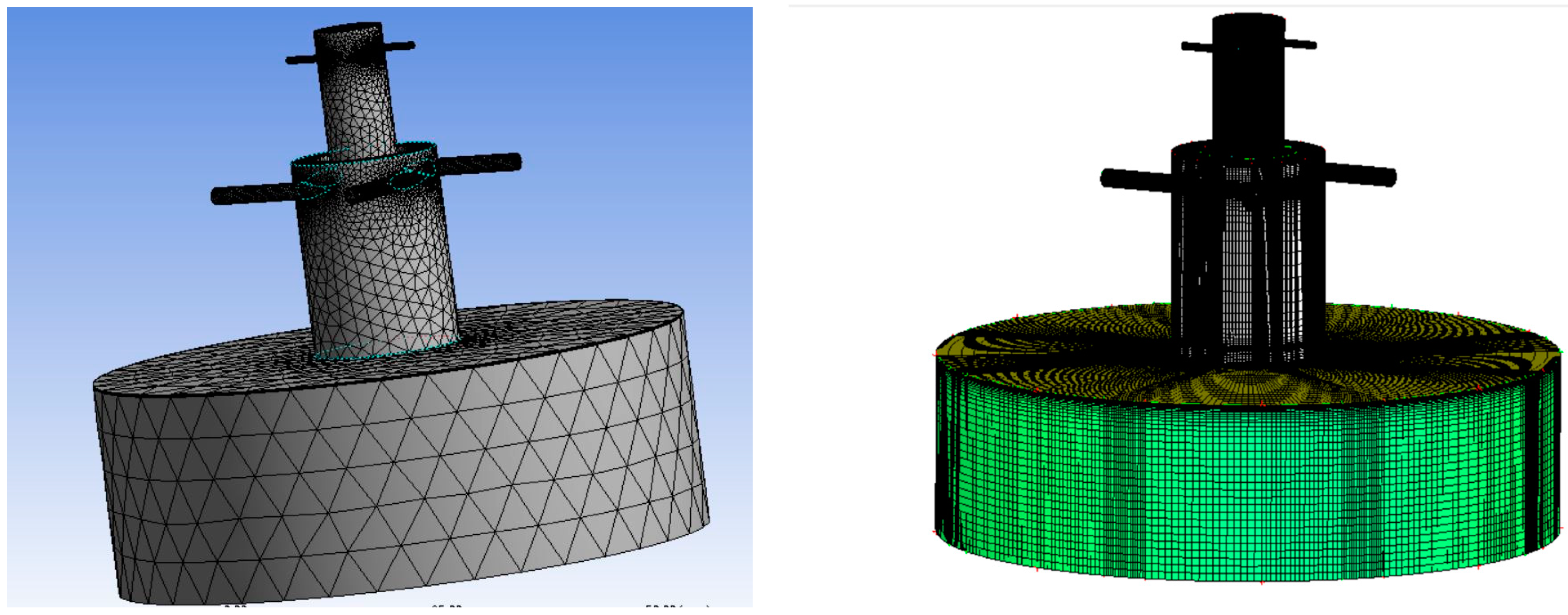

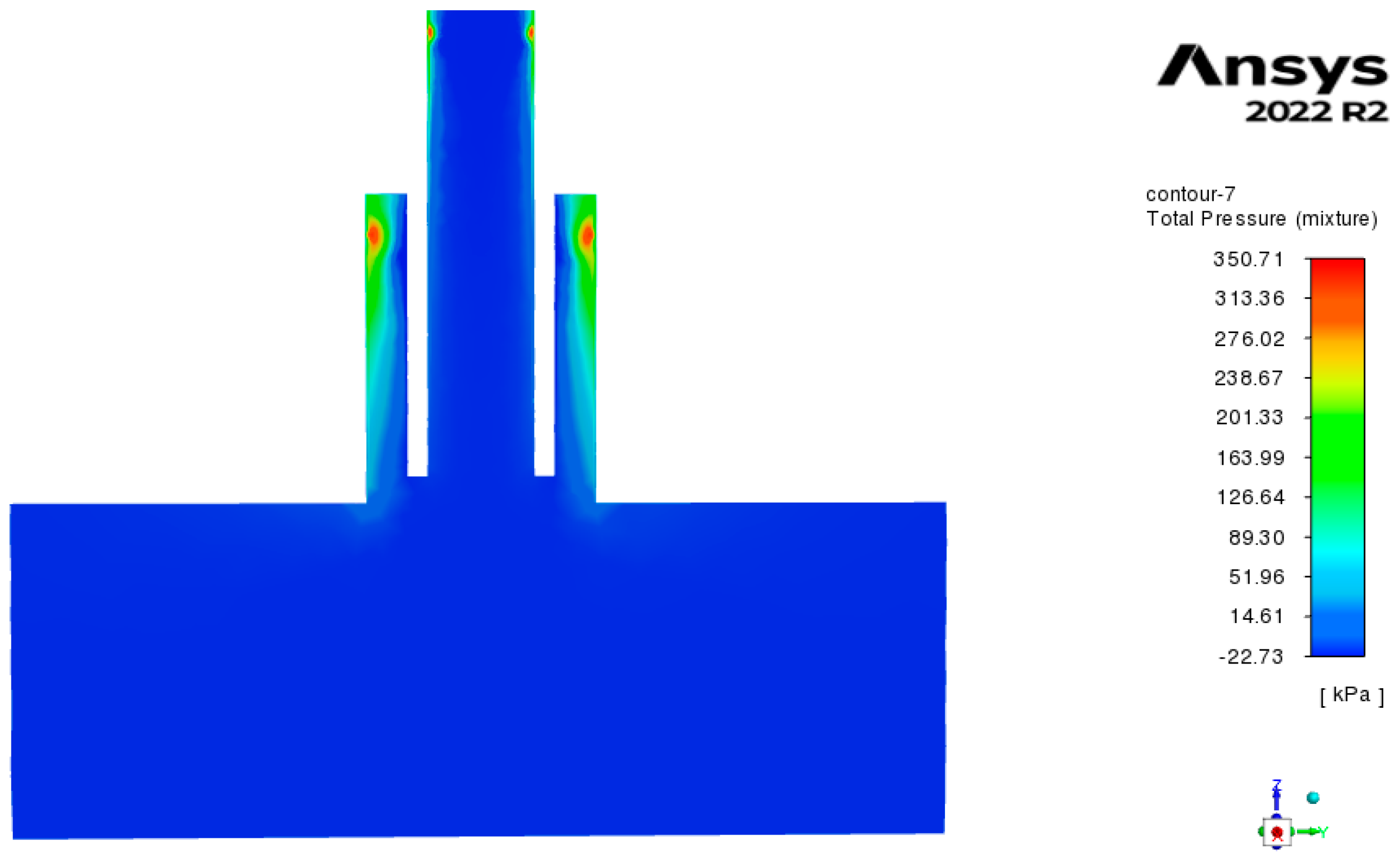

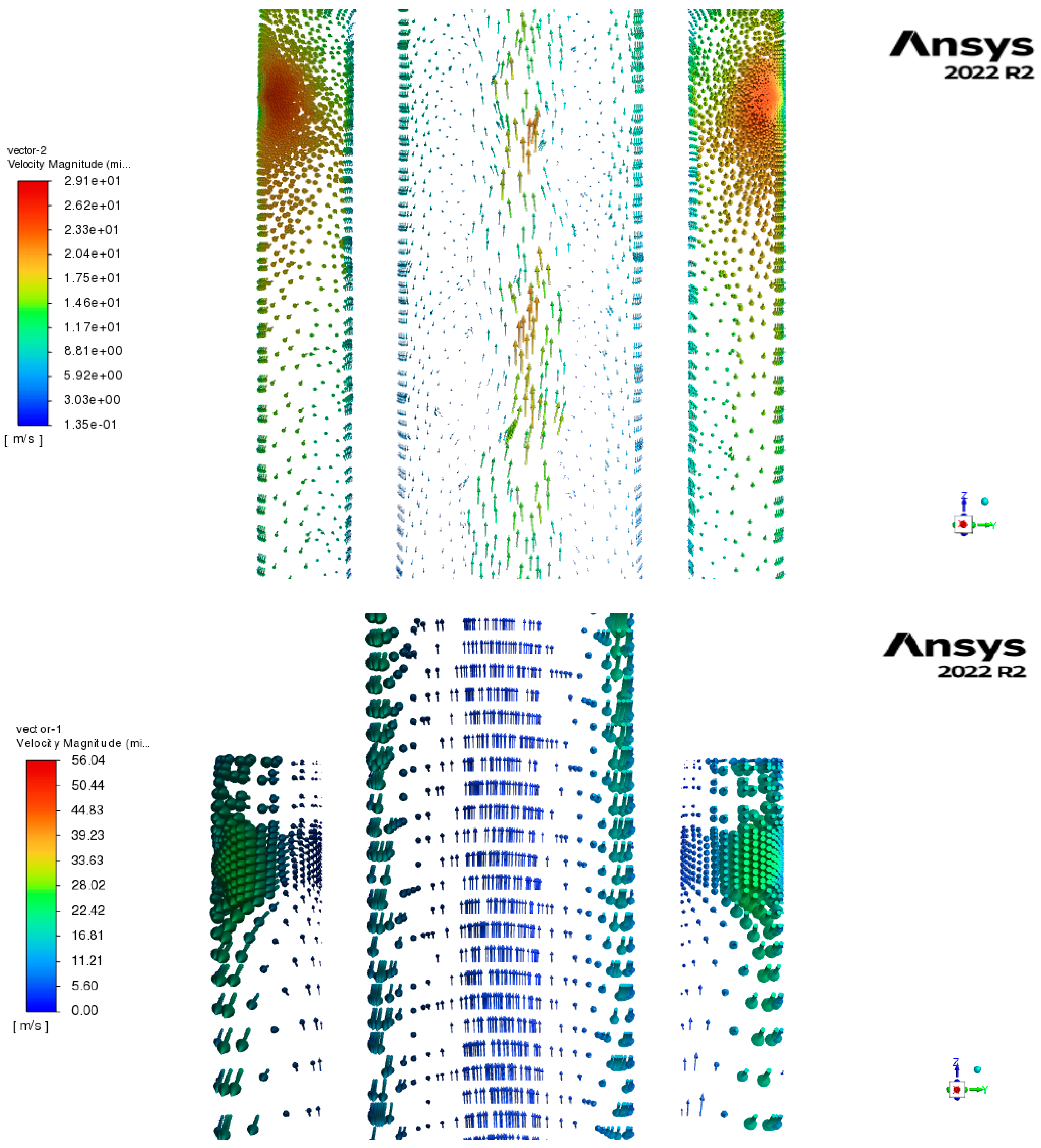
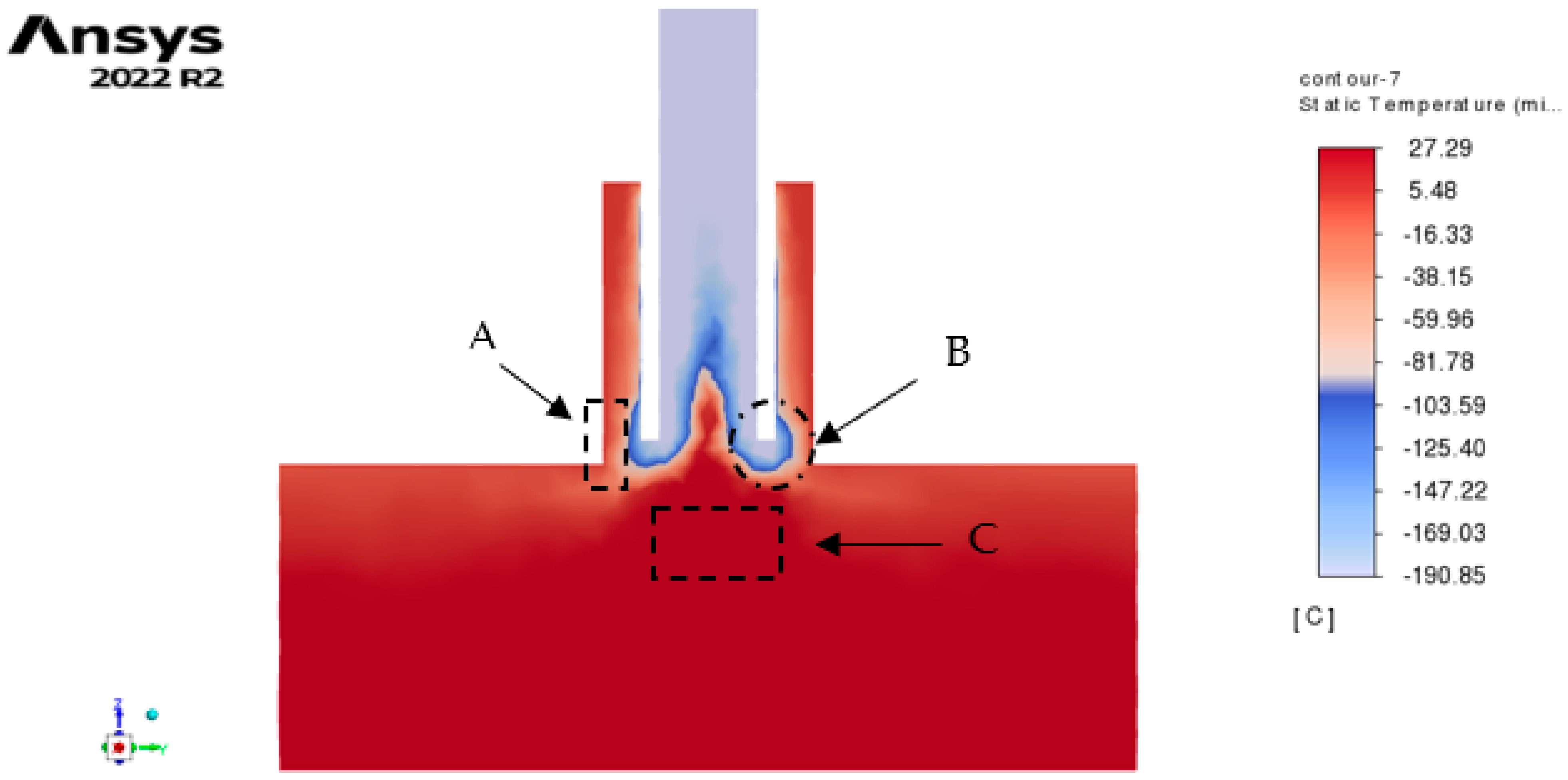

| Initial Parameters | Water | LN2 |
|---|---|---|
| Spray Angle, 2α (°) | 130 | 135 |
| Mass Flow Rate, m (kg. s−1) | 0.245 | 0.035 |
| Pressure differential, ΔP (kPa) | 350 | 350 |
| Number of channels, “n” | 4 | 4 |
| Physics Properties of Fluid | Water | LN2 |
|---|---|---|
| Density, ρ, (kg.m−3) | 1000 | 806.08 |
| Kinematic viscosity, ν (m2.s−1) | 10−6 | 19 × 10−8 |
| Absolut viscosity, μ (kg. (m.-s)−1) | 1.003 × 10−3 | 160.65 × 10−6 |
| Surface tension, σ, (N. m−1) | 0.072 | 0.0263 |
| Parameters | Ideal Liquid | Losses Viscosity |
|---|---|---|
| φ | 0.125 | 0.125 |
| A | 27.85 | 27.85 |
| cd | 0.0324 | 0.0237 |
| Rs | 3.802 | 4.45 |
| ro | 3.802 | 4.45 |
| 0.477 | 0.552 | |
| 4.765 | 5.574 |
| Parameters | Ideal Liquid | Losses Viscosity |
|---|---|---|
| φ | 0.173 | 0.173 |
| A | 16.258 | 16.258 |
| cd | 0.05323 | 0.0392 |
| Rs | 7.445 | 8.672 |
| ro | 7.445 | 8.672 |
| 1.196 | 1.393 | |
| 11.962 | 13.932 |
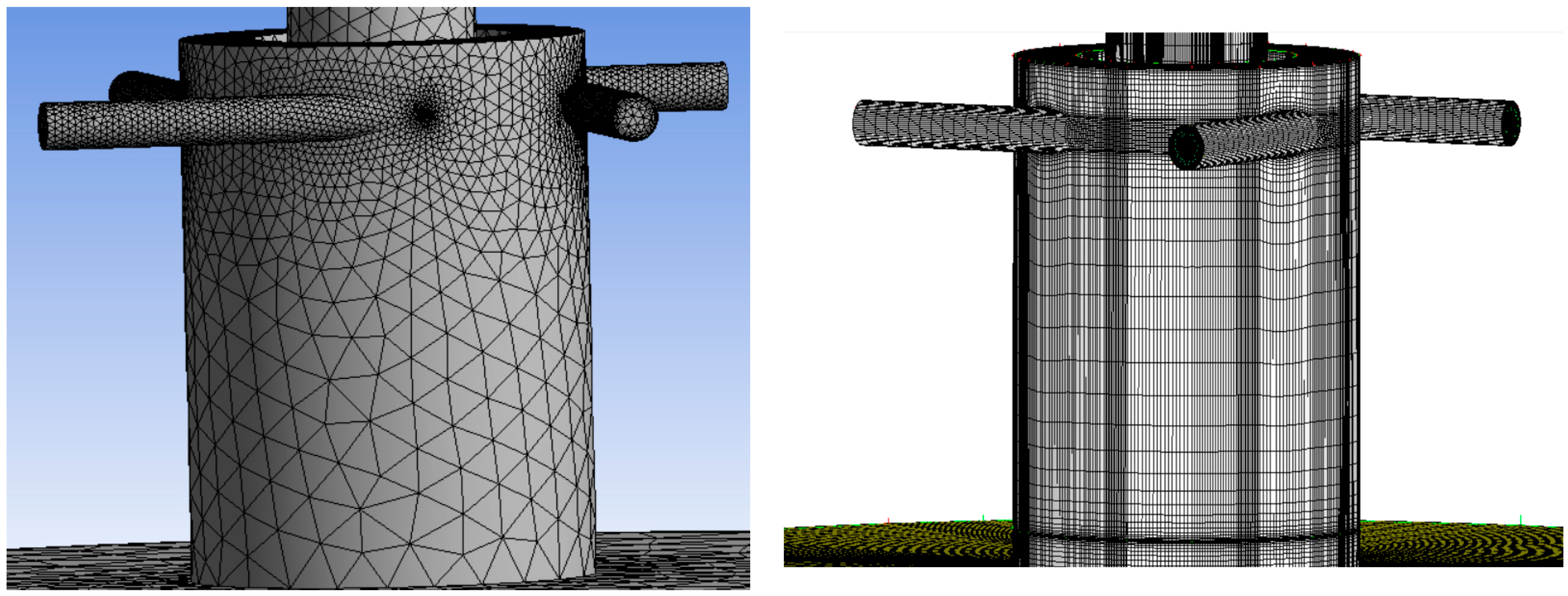
| Characteristics | Tetrahedral | Hexahedral |
|---|---|---|
| y (mm) | 3.2 × 10−3 | 4 × 10−3 |
| y+ | 3.002 | 3.655 |
| Cells | 266,119 | 6,048,104 |
| Faces | 617,024 | 18,265,032 |
| Nodes | 99,037 | 6,169,490 |
| 10,000 Iterations Time (hours) | 20 | 42 |
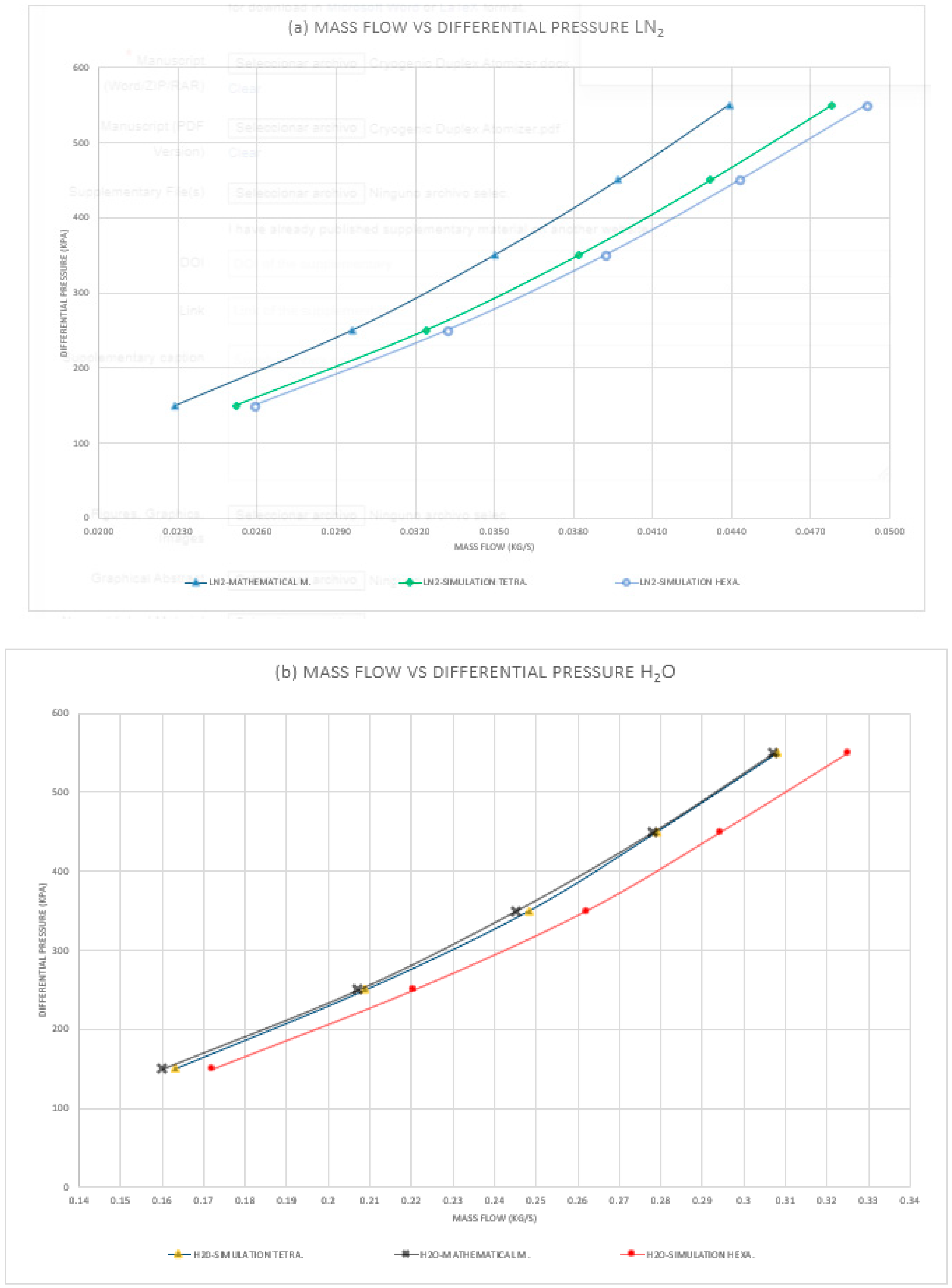
| LIQUID NITROGEN | |||||||
|---|---|---|---|---|---|---|---|
| Differential Pressure, (ΔP/kPa) | Mass Flow, (kg/s) | Heat Transfer Rate (KW) Tetrahedral | Heat Transfer Rate (KW) Hexahedral | ||||
| Mathematical Model | Simulation Tetrahedral | Deviation Mathematical Model Respect to Mesh Tetrahedral (%) | Simulation Hexahedral | Deviation Mathematical Model Respect to Mesh Hexahedral (%) | |||
| 150 | 0.0229 | 0.0252 | −10.0437 | 0.0259 | −13.1004 | −11.076 | −11.373 |
| 250 | 0.0296 | 0.0324 | −9.4595 | 0.0332 | −12.1622 | −14.241 | −14.578 |
| 350 | 0.035 | 0.0382 | −9.1429 | 0.0392 | −12.0000 | −16.791 | −17.213 |
| 450 | 0.0397 | 0.0432 | −8.8161 | 0.0443 | −11.5869 | −18.988 | −19.453 |
| 550 | 0.0439 | 0.0478 | −8.8838 | 0.0491 | −11.8451 | −21.011 | −21.560 |
| WATER | |||||||
|---|---|---|---|---|---|---|---|
| Differential Pressure, (ΔP/kPa) | Mass Flow, (kg/s) | Heat Transfer Rate (KW) Tetrahedral | Heat Transfer Rate (KW) Hexahedral | ||||
| Mathematical Model | Simulation Tetrahedral | Deviation Mathematical Model Respect to Mesh Tetrahedral (%) | Simulation Hexahedral | Deviation Mathematical Model Respect to Mesh Hexahedral (%) | |||
| 150 | 0.1600 | 0.1631 | −1.9375 | 0.1721 | −7.5625 | −13.557 | −14.395 |
| 250 | 0.2070 | 0.2089 | −0.9179 | 0.2205 | −6.5217 | −17.364 | −18.444 |
| 350 | 0.2450 | 0.2482 | −1.3061 | 0.262 | −6.9388 | −20.631 | −21.915 |
| 450 | 0.2780 | 0.2789 | −0.3237 | 0.2944 | −5.8993 | −23.183 | −24.625 |
| 550 | 0.3070 | 0.3079 | −0.2932 | 0.325 | −5.8632 | −25.594 | −27.184 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ayala, E.; Rivera, D.; Ronceros, J.; Vinces, N.; Ronceros, G. Design of a Cryogenic Duplex Pressure-Swirl Atomizer through CFDs for the Cold Conservation of Marine Products. Fluids 2023, 8, 271. https://doi.org/10.3390/fluids8100271
Ayala E, Rivera D, Ronceros J, Vinces N, Ronceros G. Design of a Cryogenic Duplex Pressure-Swirl Atomizer through CFDs for the Cold Conservation of Marine Products. Fluids. 2023; 8(10):271. https://doi.org/10.3390/fluids8100271
Chicago/Turabian StyleAyala, Eduardo, Diego Rivera, Julio Ronceros, Nikolai Vinces, and Gustavo Ronceros. 2023. "Design of a Cryogenic Duplex Pressure-Swirl Atomizer through CFDs for the Cold Conservation of Marine Products" Fluids 8, no. 10: 271. https://doi.org/10.3390/fluids8100271
APA StyleAyala, E., Rivera, D., Ronceros, J., Vinces, N., & Ronceros, G. (2023). Design of a Cryogenic Duplex Pressure-Swirl Atomizer through CFDs for the Cold Conservation of Marine Products. Fluids, 8(10), 271. https://doi.org/10.3390/fluids8100271







