CFD Simulation of a Hybrid Solar/Electric Reactor for Hydrogen and Carbon Production from Methane Cracking
Abstract
1. Introduction
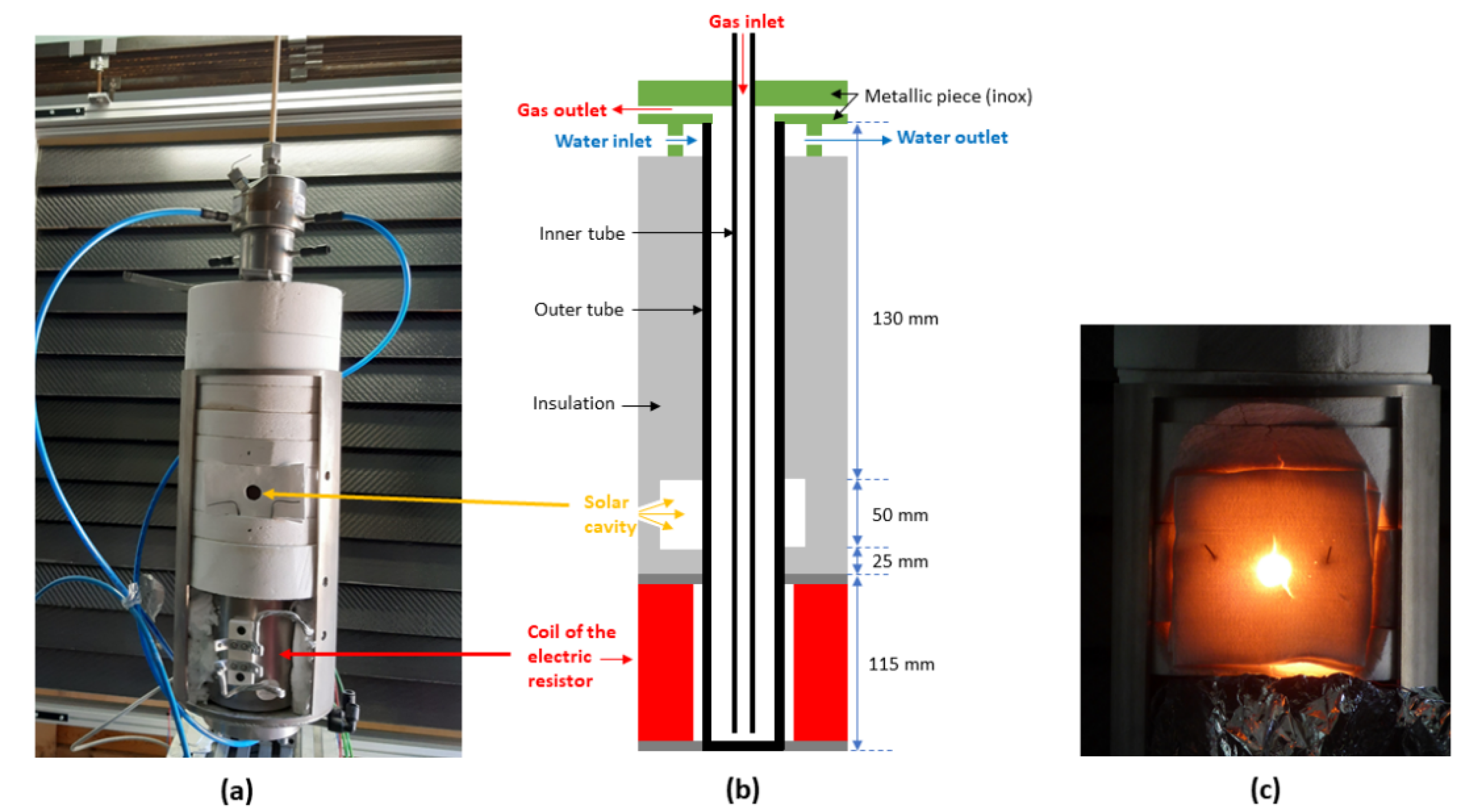
2. Design of the Hybrid Reactor
3. Modeling and Simulation Methods
3.1. System Geometry
3.2. Modelling Methods
3.2.1. Species, Models, and Boundary Conditions
3.2.2. Parametric Study
4. Results and Discussion
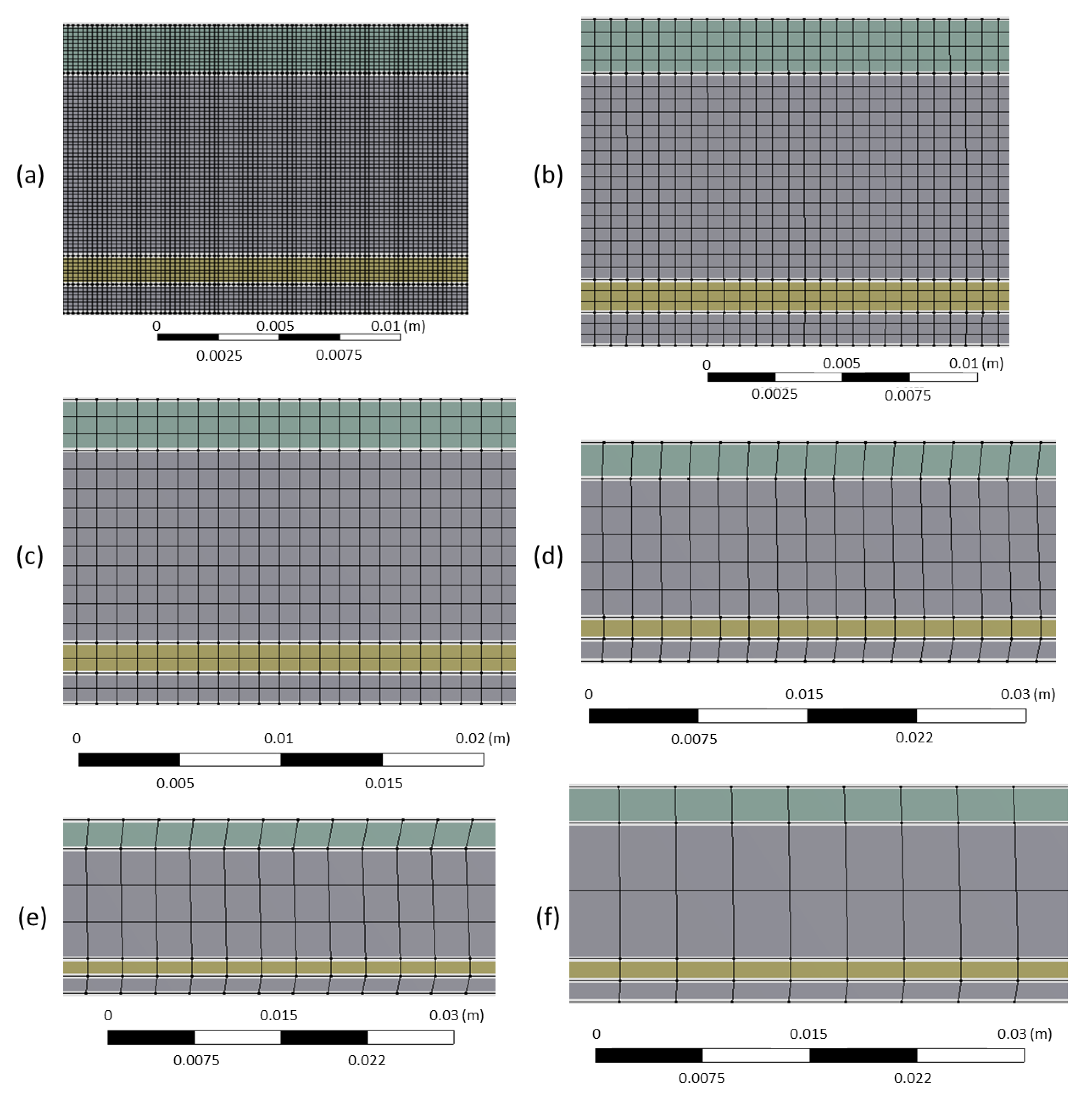
4.1. Effect of Mesh Size (Grid Independence Study)
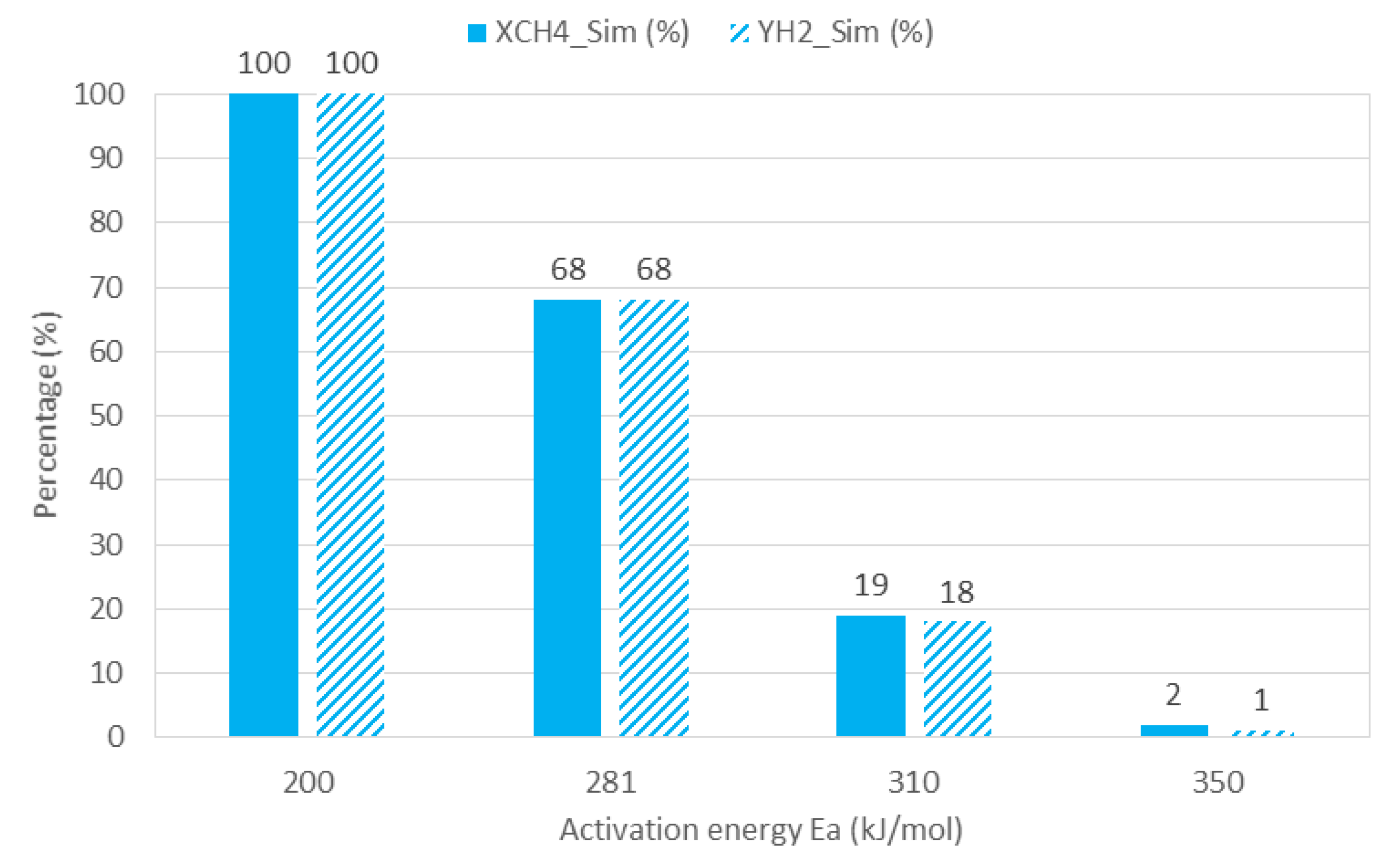
4.2. Model Sensitivity to Activation Energy
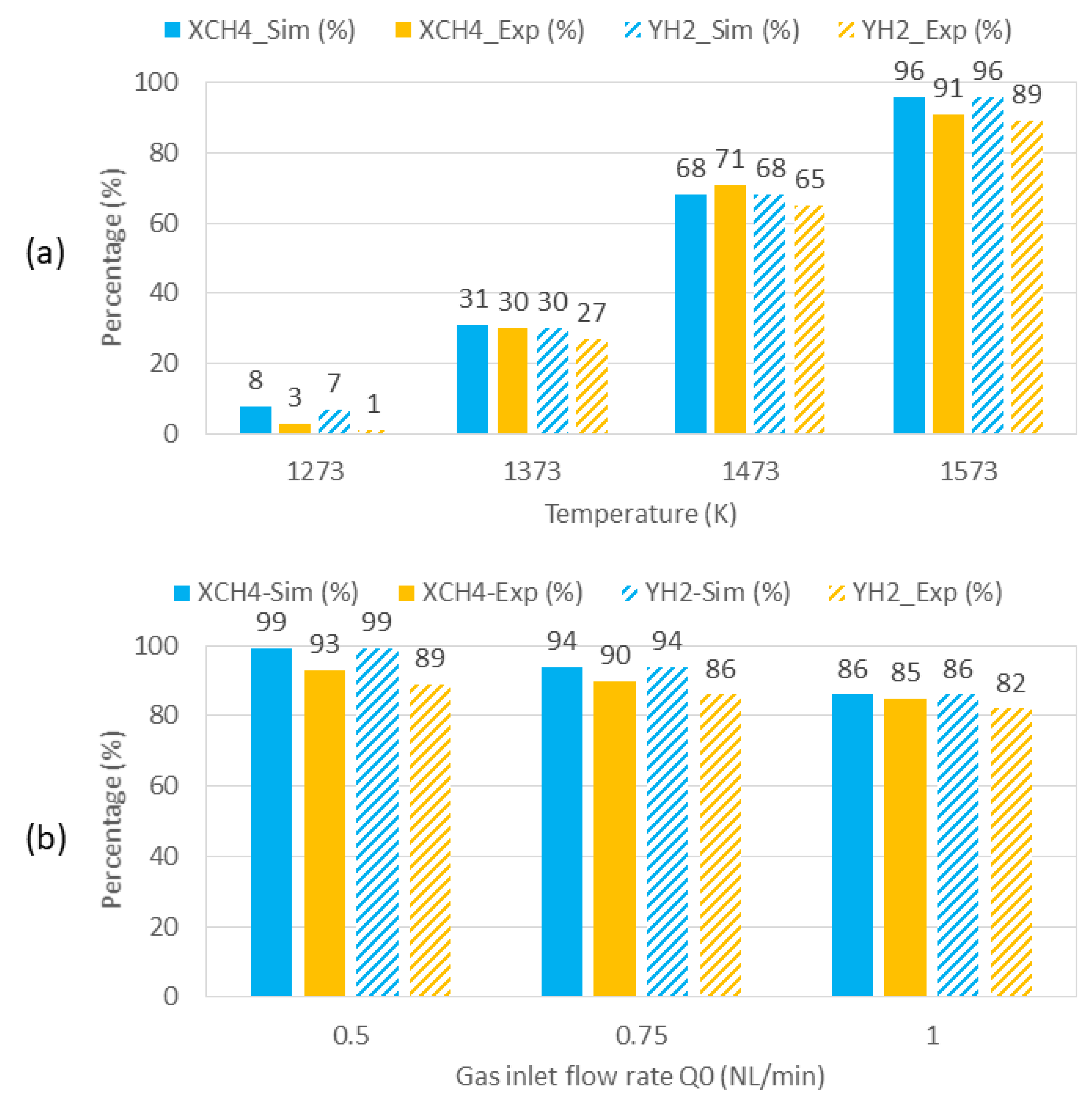
4.3. Experimental Validation of the Model
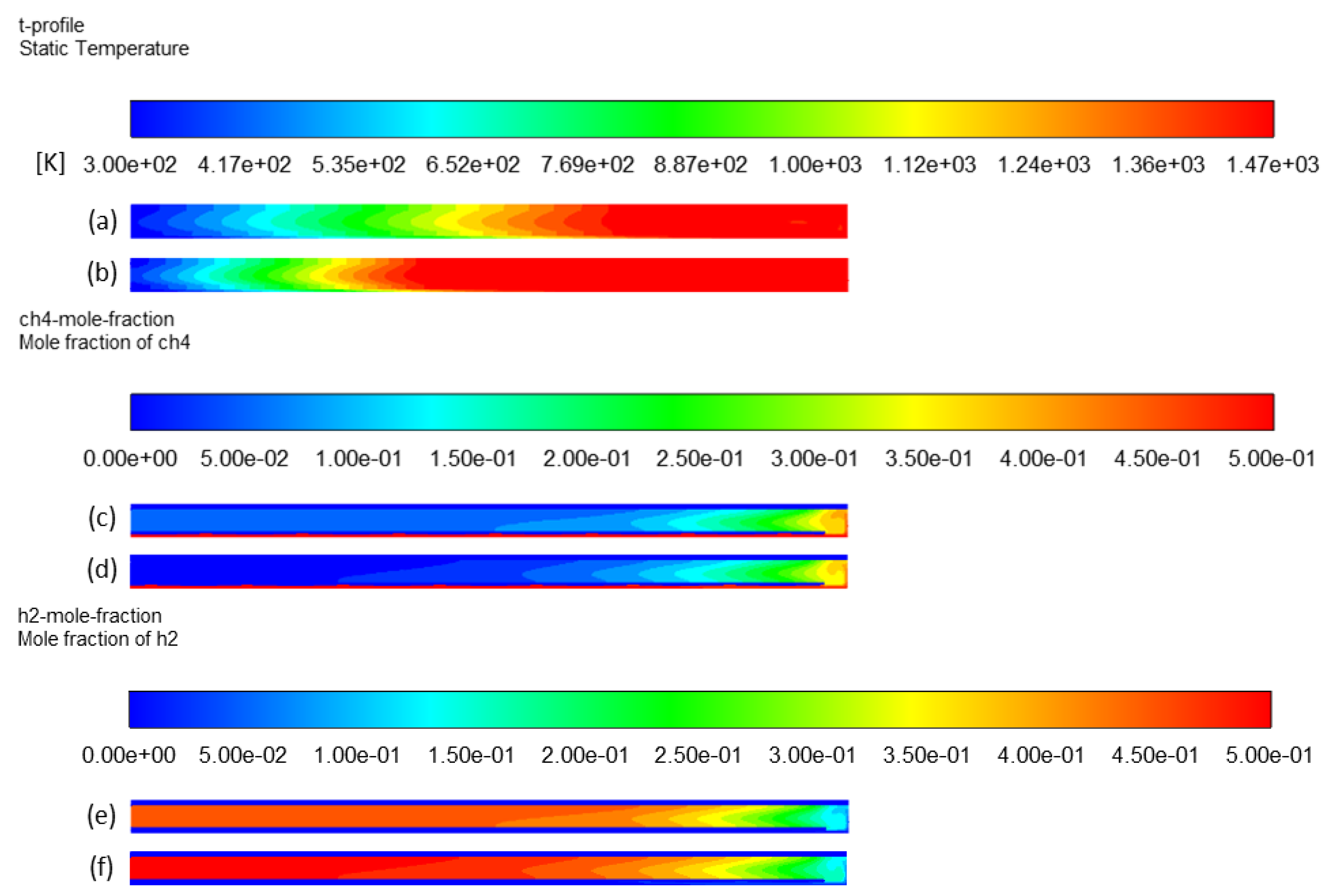
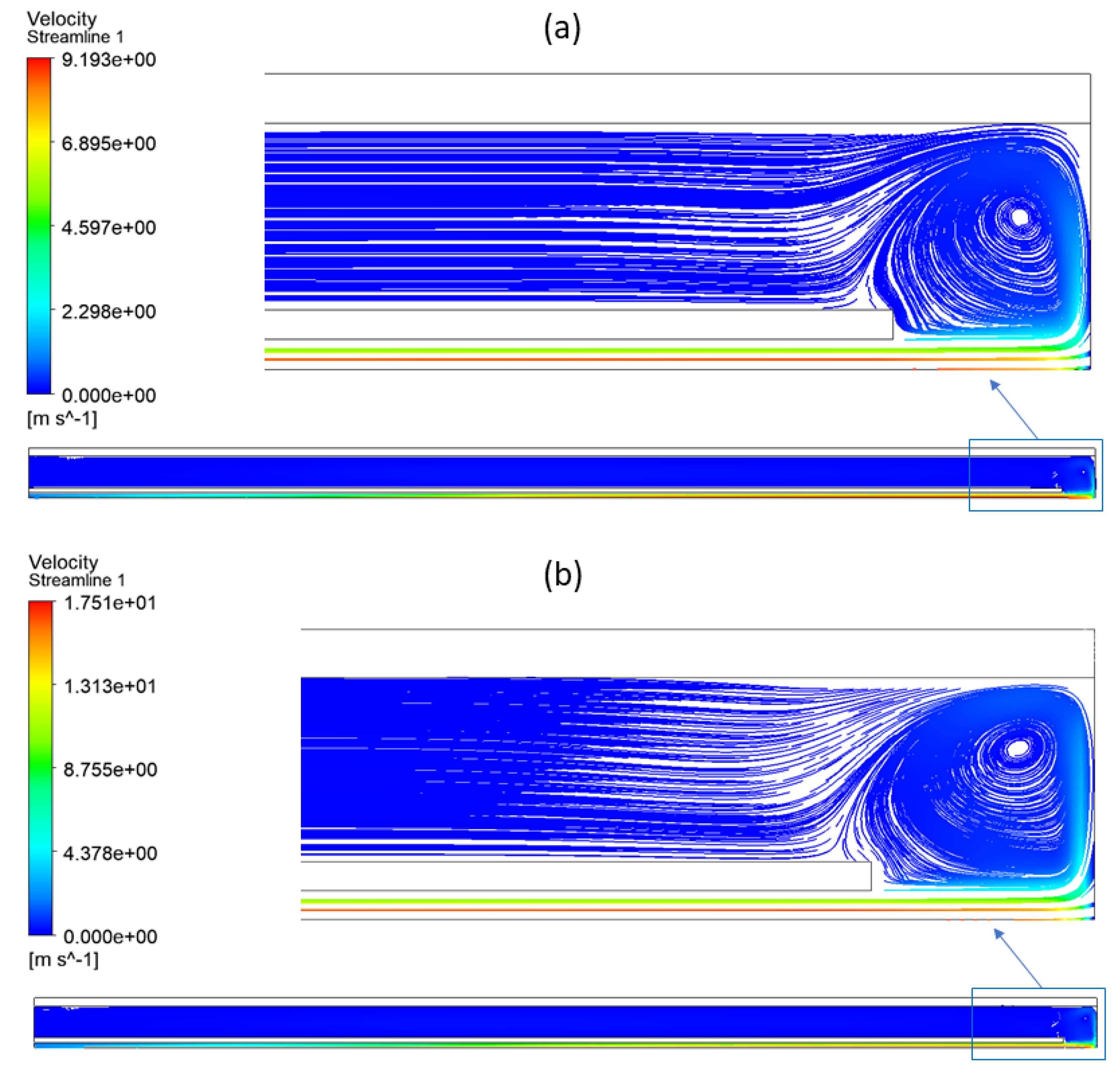
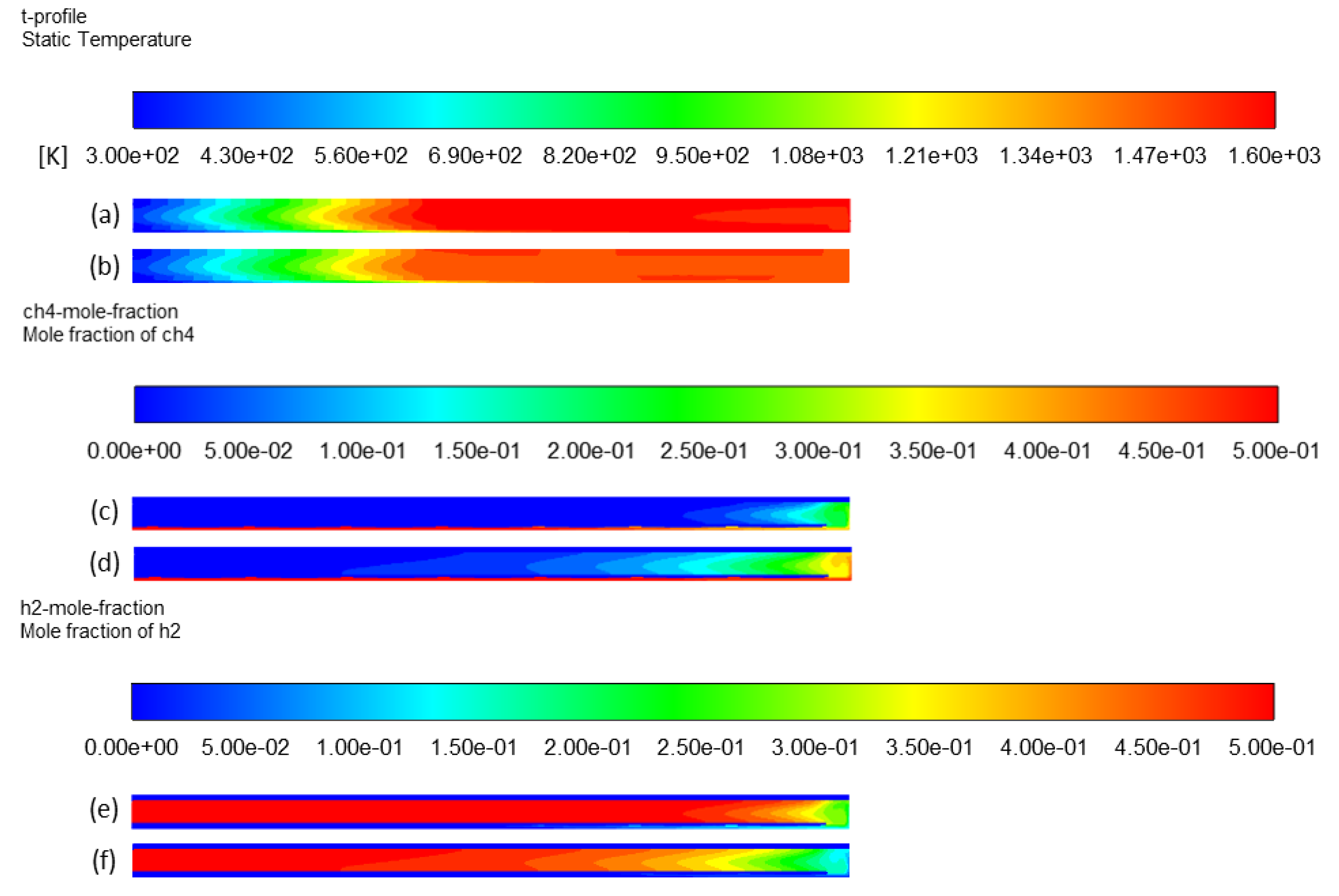
4.4. Effect of Hybridization
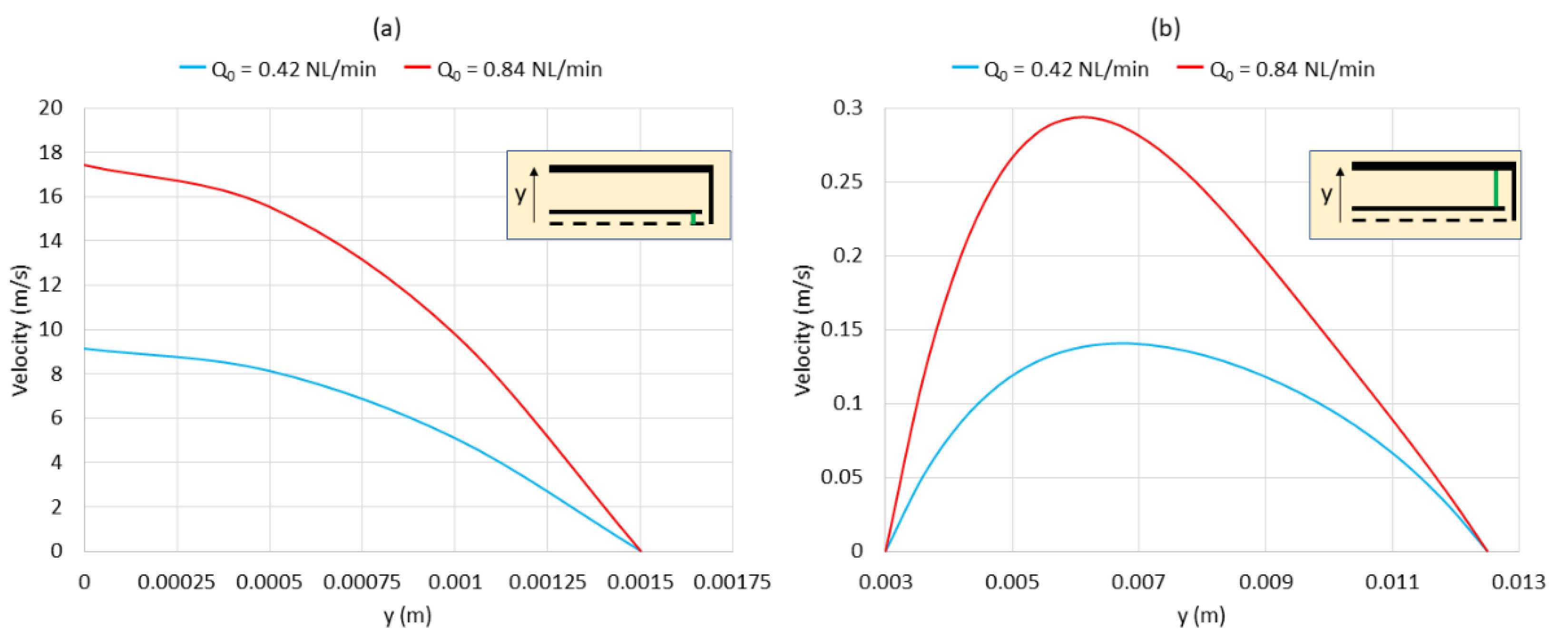
4.5. Effect of Residence Time
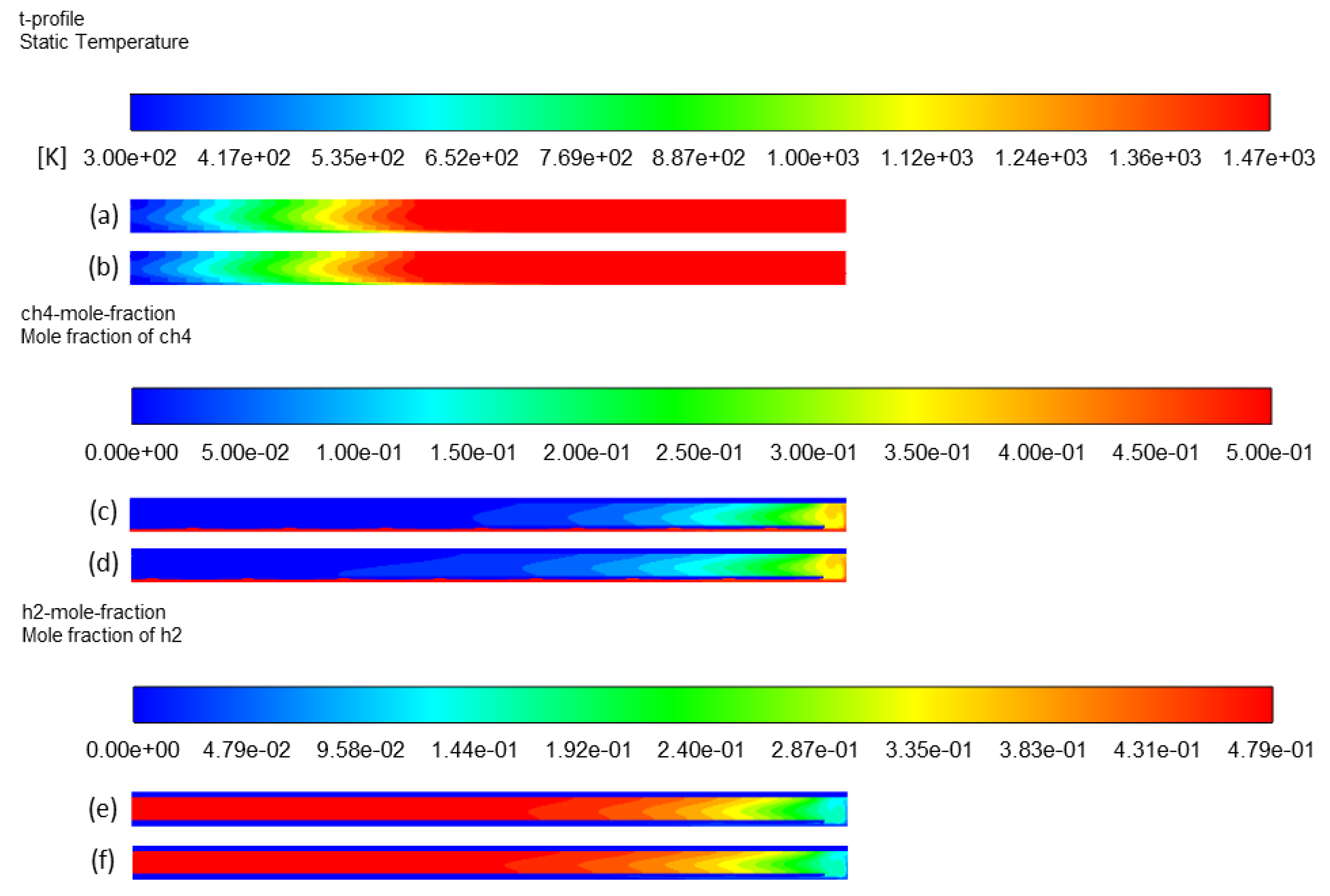
4.6. Effect of Methane Molar Fraction
4.7. Effect of Heating Temperature
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kothari, R.; Buddhi, D.; Sawhney, R.L. Comparison of Environmental and Economic Aspects of Various Hydrogen Production Methods. Renew. Sustain. Energy Rev. 2008, 12, 553–563. [Google Scholar] [CrossRef]
- Abánades, A. The Challenge of Hydrogen Production for the Transition to a CO2-Free Economy. Agron. Res. 2012, 10, 11–16. [Google Scholar]
- Abbas, H.F.; Wan Daud, W.M.A. Hydrogen Production by Methane Decomposition: A Review. Int. J. Hydrogen Energy 2010, 35, 1160–1190. [Google Scholar] [CrossRef]
- Abánades, A.; Ruiz, E.; Ferruelo, E.M.; Hernández, F.; Cabanillas, A.; Martínez-Val, J.M.; Rubio, J.A.; López, C.; Gavela, R.; Barrera, G.; et al. Experimental Analysis of Direct Thermal Methane Cracking. Int. J. Hydrogen Energy 2011, 36, 12877–12886. [Google Scholar] [CrossRef]
- Lemus, R.G.; Martínez Duart, J.M. Updated Hydrogen Production Costs and Parities for Conventional and Renewable Technologies. Int. J. Hydrogen Energy 2010, 35, 3929–3936. [Google Scholar] [CrossRef]
- Msheik, M.; Rodat, S.; Abanades, S. Methane Cracking for Hydrogen Production: A Review of Catalytic and Molten Media Pyrolysis. Energies 2021, 14, 3107. [Google Scholar] [CrossRef]
- Dahl, J. Intrinsic Kinetics for Rapid Decomposition of Methane in an Aerosol Flow Reactor. Int. J. Hydrogen Energy 2002, 27, 377–386. [Google Scholar] [CrossRef]
- Dahl, J.K.; Buechler, K.J.; Weimer, A.W.; Lewandowski, A.; Bingham, C. Solar-Thermal Dissociation of Methane in a Fluid-Wall Aerosol Flow Reactor. Int. J. Hydrogen Energy 2004, 29, 725–736. [Google Scholar] [CrossRef]
- Dahl, J.K.; Buechler, K.J.; Finley, R.; Stanislaus, T.; Weimer, A.W.; Lewandowski, A.; Bingham, C.; Smeets, A.; Schneider, A. Rapid Solar-Thermal Dissociation of Natural Gas in an Aerosol Flow Reactor. Energy 2004, 29, 715–725. [Google Scholar] [CrossRef]
- Dahl, J.K.; Tamburini, J.; Weimer, A.W.; Lewandowski, A.; Pitts, R.; Bingham, C. Solar-Thermal Processing of Methane to Produce Hydrogen and Syngas. Energy Fuels 2001, 15, 1227–1232. [Google Scholar] [CrossRef]
- Rodat, S.; Abanades, S.; Grivei, E.; Patrianakos, G.; Zygogianni, A.; Konstandopoulos, A.G.; Flamant, G. Characterisation of Carbon Blacks Produced by Solar Thermal Dissociation of Methane. Carbon N. Y. 2011, 49, 3084–3091. [Google Scholar] [CrossRef]
- Abanades, S.; Kimura, H.; Otsuka, H. A Drop-Tube Particle-Entrained Flow Solar Reactor Applied to Thermal Methane Splitting for Hydrogen Production. Fuel 2015, 153, 56–66. [Google Scholar] [CrossRef]
- Rodat, S.; Abanades, S.; Flamant, G. Experimental Evaluation of Indirect Heating Tubular Reactors for Solar Methane Pyrolysis. Int. J. Chem. React. Eng. 2010, 8, A25. [Google Scholar] [CrossRef]
- Berndt, F.M.; Perez-Lopez, O.W. Catalytic Decomposition of Methane over Ni/SiO2: Influence of Cu Addition. React. Kinet. Mech. Catal. 2017, 120, 181–193. [Google Scholar] [CrossRef]
- Rahman, M.S.; Croiset, E.; Hudgins, R.R. Catalytic Decomposition of Methane for Hydrogen Production. Top. Catal. 2006, 37, 137–145. [Google Scholar] [CrossRef]
- Lázaro, M.J.; Pinilla, J.L.; Suelves, I.; Moliner, R. Study of the Deactivation Mechanism of Carbon Blacks Used in Methane Decomposition. Int. J. Hydrogen Energy 2008, 33, 4104–4111. [Google Scholar] [CrossRef]
- Msheik, M.; Rodat, S.; Abanades, S. Experimental Comparison of Solar Methane Pyrolysis in Gas-Phase and Molten-Tin Bubbling Tubular Reactors. Energy 2022, 260, 124943. [Google Scholar] [CrossRef]
- Zaghloul, N.; Kodama, S.; Sekiguchi, H. Hydrogen Production by Methane Pyrolysis in a Molten-Metal Bubble Column. Chem. Eng. Technol. 2021, 44, 1986–1993. [Google Scholar] [CrossRef]
- Geißler, T.; Abánades, A.; Heinzel, A.; Mehravaran, K.; Müller, G.; Rathnam, R.K.; Rubbia, C.; Salmieri, D.; Stoppel, L.; Stückrad, S.; et al. Hydrogen Production via Methane Pyrolysis in a Liquid Metal Bubble Column Reactor with a Packed Bed. Chem. Eng. J. 2016, 299, 192–200. [Google Scholar] [CrossRef]
- Wang, K.; Li, W.S.; Zhou, X.P. Hydrogen Generation by Direct Decomposition of Hydrocarbons over Molten Magnesium. J. Mol. Catal. A Chem. 2008, 283, 153–157. [Google Scholar] [CrossRef]
- Zeng, J.; Tarazkar, M.; Pennebaker, T.; Gordon, M.J.; Metiu, H.; McFarland, E.W. Catalytic Methane Pyrolysis with Liquid and Vapor Phase Tellurium. ACS Catal. 2020, 10, 8223–8230. [Google Scholar] [CrossRef]
- Palmer, C.; Tarazkar, M.; Kristoffersen, H.H.; Gelinas, J.; Gordon, M.J.; McFarland, E.W.; Metiu, H. Methane Pyrolysis with a Molten Cu-Bi Alloy Catalyst. ACS Catal. 2019, 9, 8337–8345. [Google Scholar] [CrossRef]
- Upham, D.C.; Agarwal, V.; Khechfe, A.; Snodgrass, Z.R.; Gordon, M.J.; Metiu, H.; McFarland, E.W. Catalytic Molten Metals for the Direct Conversion of Methane to Hydrogen and Separable Carbon. Science 2017, 358, 917–921. [Google Scholar] [CrossRef] [PubMed]
- Kang, D.; Rahimi, N.; Gordon, M.J.; Metiu, H.; McFarland, E.W. Catalytic Methane Pyrolysis in Molten MnCl2-KCl. Appl. Catal. B Environ. 2019, 254, 659–666. [Google Scholar] [CrossRef]
- Kang, D.; Palmer, C.; Mannini, D.; Rahimi, N.; Gordon, M.J.; Metiu, H.; McFarland, E.W. Catalytic Methane Pyrolysis in Molten Alkali Chloride Salts Containing Iron. ACS Catal. 2020, 10, 7032–7042. [Google Scholar] [CrossRef]
- Parkinson, B.; Patzschke, C.F.; Nikolis, D.; Raman, S.; Dankworth, D.C.; Hellgardt, K. Methane Pyrolysis in Monovalent Alkali Halide Salts: Kinetics and Pyrolytic Carbon Properties. Int. J. Hydrogen Energy 2021, 46, 6225–6238. [Google Scholar] [CrossRef]
- Rahimi, N.; Kang, D.; Gelinas, J.; Menon, A.; Gordon, M.J.; Metiu, H.; McFarland, E.W. Solid Carbon Production and Recovery from High Temperature Methane Pyrolysis in Bubble Columns Containing Molten Metals and Molten Salts. Carbon N. Y. 2019, 151, 181–191. [Google Scholar] [CrossRef]
- Patzschke, C.F.; Parkinson, B.; Willis, J.J.; Nandi, P.; Love, A.M.; Raman, S.; Hellgardt, K. Co-Mn Catalysts for H2 Production via Methane Pyrolysis in Molten Salts. Chem. Eng. J. 2021, 414, 128730. [Google Scholar] [CrossRef]
- Sánchez-Bastardo, N.; Schlögl, R.; Ruland, H. Methane Pyrolysis for CO2-Free H2 Production: A Green Process to Overcome Renewable Energies Unsteadiness. Chem.-Ing.-Tech. 2020, 92, 1596–1609. [Google Scholar] [CrossRef]
- Rodat, S.; Abanades, S.; Sans, J.-L.; Flamant, G. A Pilot-Scale Solar Reactor for the Production of Hydrogen and Carbon Black from Methane Splitting. Int. J. Hydrogen Energy 2010, 35, 7748–7758. [Google Scholar] [CrossRef]
- Maag, G.; Zanganeh, G.; Steinfeld, A. Solar Thermal Cracking of Methane in a Particle-Flow Reactor for the Co-Production of Hydrogen and Carbon. Int. J. Hydrogen Energy 2009, 34, 7676–7685. [Google Scholar] [CrossRef]
- Rodat, S.; Abanades, S.; Sans, J.-L.; Flamant, G. Hydrogen Production from Solar Thermal Dissociation of Natural Gas: Development of a 10 kW Solar Chemical Reactor Prototype. Sol. Energy 2009, 83, 1599–1610. [Google Scholar] [CrossRef]
- Guil-Lopez, R.; Botas, J.A.; Fierro, J.L.G.; Serrano, D.P. Comparison of Metal and Carbon Catalysts for Hydrogen Production by Methane Decomposition. Appl. Catal. A Gen. 2011, 396, 40–51. [Google Scholar] [CrossRef]
- Utrilla, R.; Pinilla, J.L.; Suelves, I.; Lázaro, M.J.; Moliner, R. Catalytic Decomposition of Methane for the Simultaneous Co-Production of CO2-Free Hydrogen and Carbon Nanofibre Based Polymers. Fuel 2011, 90, 430–432. [Google Scholar] [CrossRef]
- Muradov, N.; Smith, F.; T-Raissi, A. Catalytic Activity of Carbons for Methane Decomposition Reaction. Catal. Today 2005, 102–103, 225–233. [Google Scholar] [CrossRef]
- Botas, J.A.; Serrano, D.P.; Guil-López, R.; Pizarro, P.; Gómez, G. Methane Catalytic Decomposition over Ordered Mesoporous Carbons: A Promising Route for Hydrogen Production. Int. J. Hydrogen Energy 2010, 35, 9788–9794. [Google Scholar] [CrossRef]
- Serrano, D.P.; Botas, J.A.; Guil-Lopez, R. H2 Production from Methane Pyrolysis over Commercial Carbon Catalysts: Kinetic and Deactivation Study. Int. J. Hydrogen Energy 2009, 34, 4488–4494. [Google Scholar] [CrossRef]
- Pinilla, J.L.; Torres, D.; Lázaro, M.J.; Suelves, I.; Moliner, R.; Cañadas, I.; Rodríguez, J.; Vidal, A.; Martínez, D. Metallic and Carbonaceous-Based Catalysts Performance in the Solar Catalytic Decomposition of Methane for Hydrogen and Carbon Production. Int. J. Hydrogen Energy 2012, 37, 9645–9655. [Google Scholar] [CrossRef]
- Rodat, S.; Abanades, S. A Hybrid Windowless Dual Tube Solar Reactor for Continuous Volumetric Natural Gas Dissociation. Front. Energy Res. 2020, 8, 206. [Google Scholar] [CrossRef]
- Rodat, S.; Abanades, S.; Boujjat, H.; Chuayboon, S. On the Path toward Day and Night Continuous Solar High Temperature Thermochemical Processes: A Review. Renew. Sustain. Energy Rev. 2020, 132, 110061. [Google Scholar] [CrossRef]
- Abanades, S.; Flamant, G. Experimental Study and Modeling of a High-Temperature Solar Chemical Reactor for Hydrogen Production from Methane Cracking. Int. J. Hydrogen Energy 2007, 32, 1508–1515. [Google Scholar] [CrossRef]
- ANSYS, Inc. ANSYS Fluent Theory Guide; ANSYS Inc.: Canonsburg, PA, USA, 2013. [Google Scholar]
- Keipi, T.; Tolvanen, K.E.S.; Tolvanen, H.; Konttinen, J. Thermo-Catalytic Decomposition of Methane: The Effect of Reaction Parameters on Process Design and the Utilization Possibilities of the Produced Carbon. Energy Convers. Manag. 2016, 126, 923–934. [Google Scholar] [CrossRef]
- Abanades, S.; Flamant, G. Hydrogen Production from Solar Thermal Dissociation of Methane in a High-Temperature Fluid-Wall Chemical Reactor. Chem. Eng. Process. Process Intensif. 2008, 47, 490–498. [Google Scholar] [CrossRef]
- Rodat, S.; Abanades, S.; Flamant, G. Co-Production of Hydrogen and Carbon Black from Solar Thermal Methane Splitting in a Tubular Reactor Prototype. Sol. Energy 2011, 85, 645–652. [Google Scholar] [CrossRef]
- Yeheskel, J.; Epstein, M. Thermolysis of Methane in a Solar Reactor for Mass-Production of Hydrogen and Carbon Nano-Materials. Carbon N. Y. 2011, 49, 4695–4703. [Google Scholar] [CrossRef]
- Abanades, S.; Tescari, S.; Rodat, S.; Flamant, G. Natural Gas Pyrolysis in Double-Walled Reactor Tubes Using Thermal Plasma or Concentrated Solar Radiation as External Heating Source. J. Nat. Gas Chem. 2009, 18, 1–8. [Google Scholar] [CrossRef]





| Telectric (K) | Tsolar (K) | Q0 (NL/min) | y0,CH4 | Mesh Size (mm) | Parameter Effect | |
|---|---|---|---|---|---|---|
| Case 1 (ref) | 1473 | 1473 | 0.42 | 0.5 | 0.6 | Reference case: hybridization (coupled heating) |
| Case 2 | 1473 | Insulated | 0.42 | 0.5 | 0.6 | Electric heating only |
| Case 3 | 1473 | 1473 | 0.84 | 0.5 | 0.6 | Residence time |
| Case 4 | 1473 | 1473 | 0.42 | 0.3 | 0.6 | Methane dilution |
| Case 5 | 1573 | 1573 | 0.42 | 0.5 | 0.6 | Heating temperature |
| Telectric (K) | Tsolar (K) | Q0 (NL/min) | y0,CH4 | Mesh Size (mm) | τe (s) | τs (s) | τtotal (s) | CH4 conversion, XCH4 (%) | H2 yield, YH2 (%) | |
|---|---|---|---|---|---|---|---|---|---|---|
| Case 1 (ref) | 1473 | 1473 | 0.42 | 0.5 | 0.6 | 0.94 | 0.56 | 1.5 | 91 | 92 |
| Case 2 | 1473 | Insulated | 0.42 | 0.5 | 0.6 | 0.94 | 0 | 0.94 | 75 | 74 |
| Case 3 | 1473 | 1473 | 0.84 | 0.5 | 0.6 | 0.47 | 0.28 | 0.75 | 67 | 73 |
| Case 4 | 1473 | 1473 | 0.42 | 0.3 | 0.6 | 0.94 | 0.56 | 1.5 | 95 | 94 |
| Case 5 | 1573 | 1573 | 0.42 | 0.5 | 0.6 | 0.88 | 0.52 | 1.4 | 100 | 100 |
| Telectric (K) | Tsolar (K) | Q0 (NL/min) | y0,CH4 | Mesh Size (mm) | τe (s) | τs (s) | τtotal (s) | XCH4 (%) | YH2 (%) | |
|---|---|---|---|---|---|---|---|---|---|---|
| Mesh 1 | 1473 | 1473 | 0.42 | 0.5 | 0.2 | 0.94 | 0.56 | 1.5 | 91 | 90 |
| Mesh 2 | 1473 | 1473 | 0.42 | 0.5 | 0.6 | 0.94 | 0.56 | 1.5 | 91 | 92 |
| Mesh 3 | 1473 | 1473 | 0.42 | 0.5 | 1 | 0.94 | 0.56 | 1.5 | 91 | 90 |
| Mesh 4 | 1473 | 1473 | 0.42 | 0.5 | 2 | 0.94 | 0.56 | 1.5 | 90 | 88 |
| Mesh 5 | 1473 | 1473 | 0.42 | 0.5 | 3 | 0.94 | 0.56 | 1.5 | 90 | 86 |
| Mesh 6 | 1473 | 1473 | 0.42 | 0.5 | 4 | 0.94 | 0.56 | 1.5 | Divergence | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Msheik, M.; Rodat, S.; Abanades, S. CFD Simulation of a Hybrid Solar/Electric Reactor for Hydrogen and Carbon Production from Methane Cracking. Fluids 2023, 8, 18. https://doi.org/10.3390/fluids8010018
Msheik M, Rodat S, Abanades S. CFD Simulation of a Hybrid Solar/Electric Reactor for Hydrogen and Carbon Production from Methane Cracking. Fluids. 2023; 8(1):18. https://doi.org/10.3390/fluids8010018
Chicago/Turabian StyleMsheik, Malek, Sylvain Rodat, and Stéphane Abanades. 2023. "CFD Simulation of a Hybrid Solar/Electric Reactor for Hydrogen and Carbon Production from Methane Cracking" Fluids 8, no. 1: 18. https://doi.org/10.3390/fluids8010018
APA StyleMsheik, M., Rodat, S., & Abanades, S. (2023). CFD Simulation of a Hybrid Solar/Electric Reactor for Hydrogen and Carbon Production from Methane Cracking. Fluids, 8(1), 18. https://doi.org/10.3390/fluids8010018






