Nucleus-Acoustic Solitary Waves in Warm Degenerate Magneto-Rotating Quantum Plasmas
Abstract
1. Introduction
- The works [1,2,3,4] neglected the presence of the magnetic field in various space plasmas. The pressure exerted due to the presence of the magnetic field in the degenerate quantum system is given bywhere , , , and are the magnitude of the charge of the plasma species j, mass of j, velocity of plasma fluid species j, and the magnetic field acting on the plasma system, respectively.
- The investigations made in [30,31,32] neglected the effects of the rotational magnetic field in CDPS, for which, the applications of these works are limited to the non-rotational astrophysical objects (viz. white dwarfs). Neutron stars, pulsars, magnetars, etc., are highly dense quantum systems as well as rotational plasma systems. Therefore, in order to completely understand the characteristics of the said CDPS, one must consider the rotation of the plasma system at an angle around the direction axis of a constant magnetic field. A Coriolis force was used by the authors for describing the effect of the rotational ionized plasma system [27,28,29]. The Coriolis force effect can be introduced as [27,28,29]where and are the number density of the plasma species j, and angular velocity of the rotational plasma system. It may be noted here that the higher order terms, viz. , which describe the strong rotation, may be neglected in case of slow rotation.
- The degenerate pressure () exerted by the highly dense plasma species is given by the Chandrasekhar limit [11,12,13,14], which was introduced earlier in this section. The works [1,2,3,4,10,26,27,28,29] considered the Chandrasekhar limit for describing the degenerate pressure, which is only valid for CDPS (zero temperature). Astrophysical objects such as a hot white dwarf [33,34,35,36] have degenerate plasma species of finite temperature [37,38,39]. Therefore, the effect of the finite temperature of the degenerate plasma species must be considered to overcome the limitations of the previous works. The Chandrasekhar equation of state at a finite temperature can be introduced as [9,39]where , , and . , , , and represent the Boltzmann constant, Fermi energy of plasma species j, plasma species temperature, and relativity parameter [9,39,40], respectively. At a value of and , the equation describes a warm non-relativistic degenerate plasma state [9,39,40]. On the other hand, to describe an ultra-relativistic degenerate plasma state, and should be considered in the Chandrasekhar equation of state [9,39,40]. It is clear from the above equation that, at zero temperature, viz. , the equation reduces to Chandrasekhar’s cold degenerate plasma limit.
- The authors [6,10,28,29] considered a two-component plasma system containing cold degenerate electrons and an inertial light nucleus. A heavy nucleus such as [18] or [19] or [19] is found at the core of highly dense astrophysical plasmas. The heavy nucleus in such a degenerate plasma system can play a vital role in modifying the characteristics of the wave mode generated in the system. Due to their massive size compared to the light nucleus , and low number density, the heavy nucleus may be considered immobile. The presence of a heavy nucleus gives rise to the neutrality condition for a warm degenerate quantum plasma as .
2. Warm Degenerate Magnetized Plasma Model
3. Derivation of KdV Equation
4. Discussion
- The presence of non-relativistic or ultra-relativistic electron species in the WDMRQP system supports the existence and propagation of compressional NASWs.
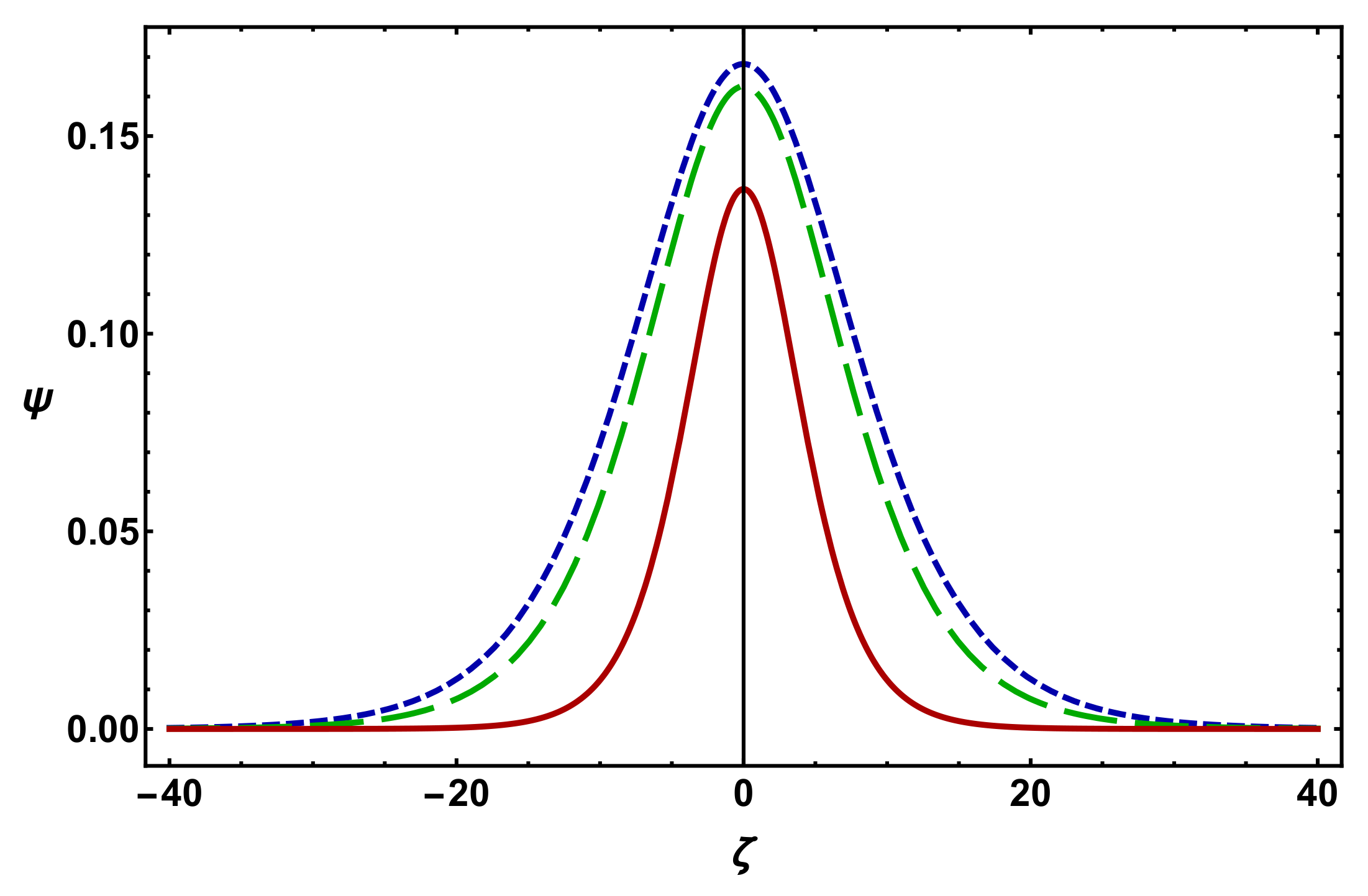
- The amplitude of the NASW potential decreases as the temperature is increased in the degenerate non-relativistic plasma medium as shown in Figure 3. The potential height and width of NASWs is maximum for the cold degenerate system (i.e., ) in the presence of non-relativistic electron species.
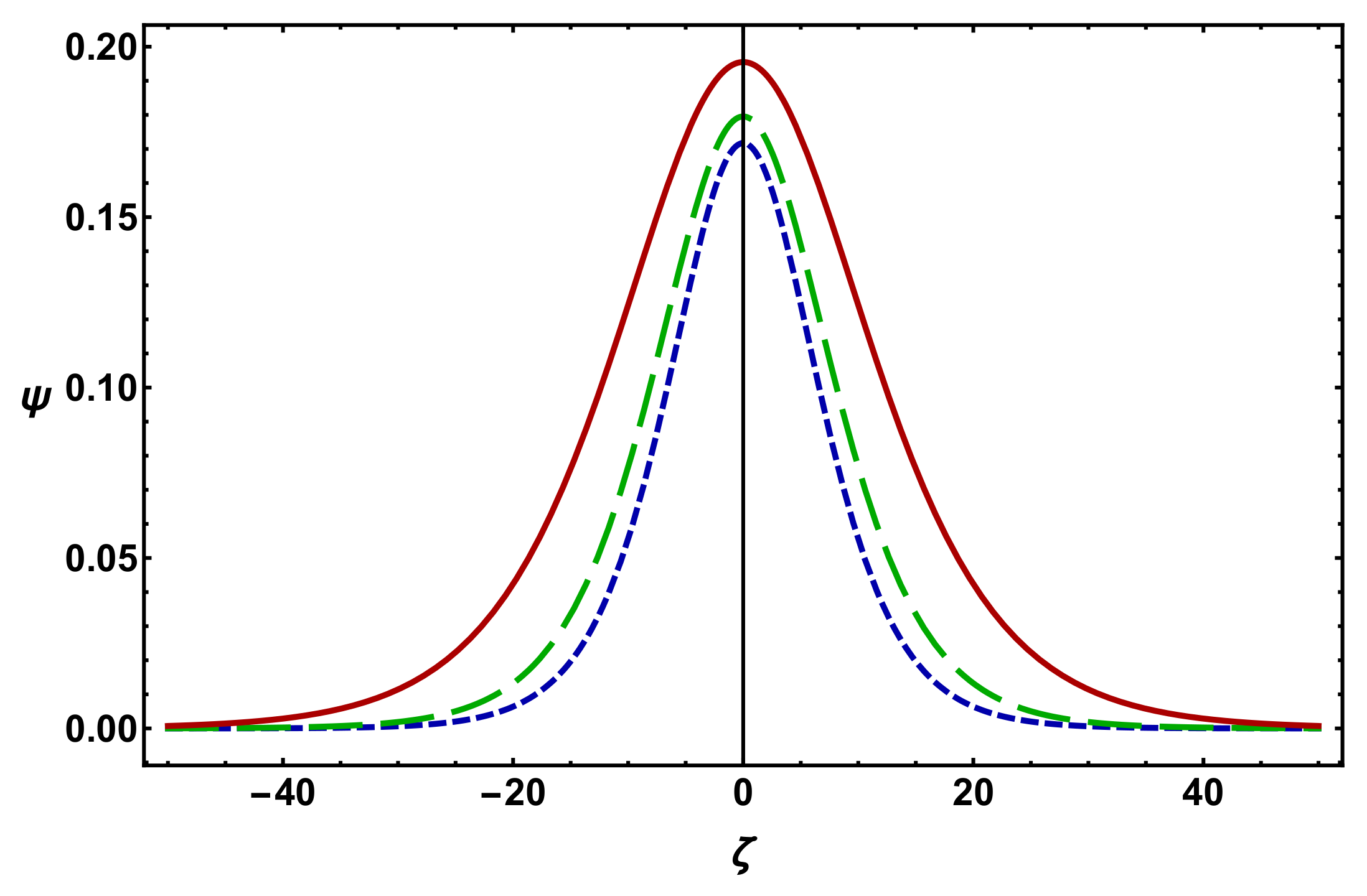
- As depicted in Figure 4, due to the presence of ultra-relativistic electrons, the potential height becomes maximum as the temperature of the system is increased. Hence, it can be said that the strength of the NASWs increases in the warm degenerate ultra-relativistic magneto-rotating plasma system. Both the amplitude and width of the NASW potential decrease for the cold degenerate ultra-relativistic system. It is evident from Figure 3 and Figure 4 that the temperature effect on the strength of NAWs is different in the case of the non-relativistic (Figure 3) and ultra-relativistic plasma system (Figure 4). The amplitude and width of the NAWs increase (decrease) with the increase in temperature of the ultra-relativistic (non-relativistic) electrons in the three-dimensional magneto-rotating degenerate plasma system.
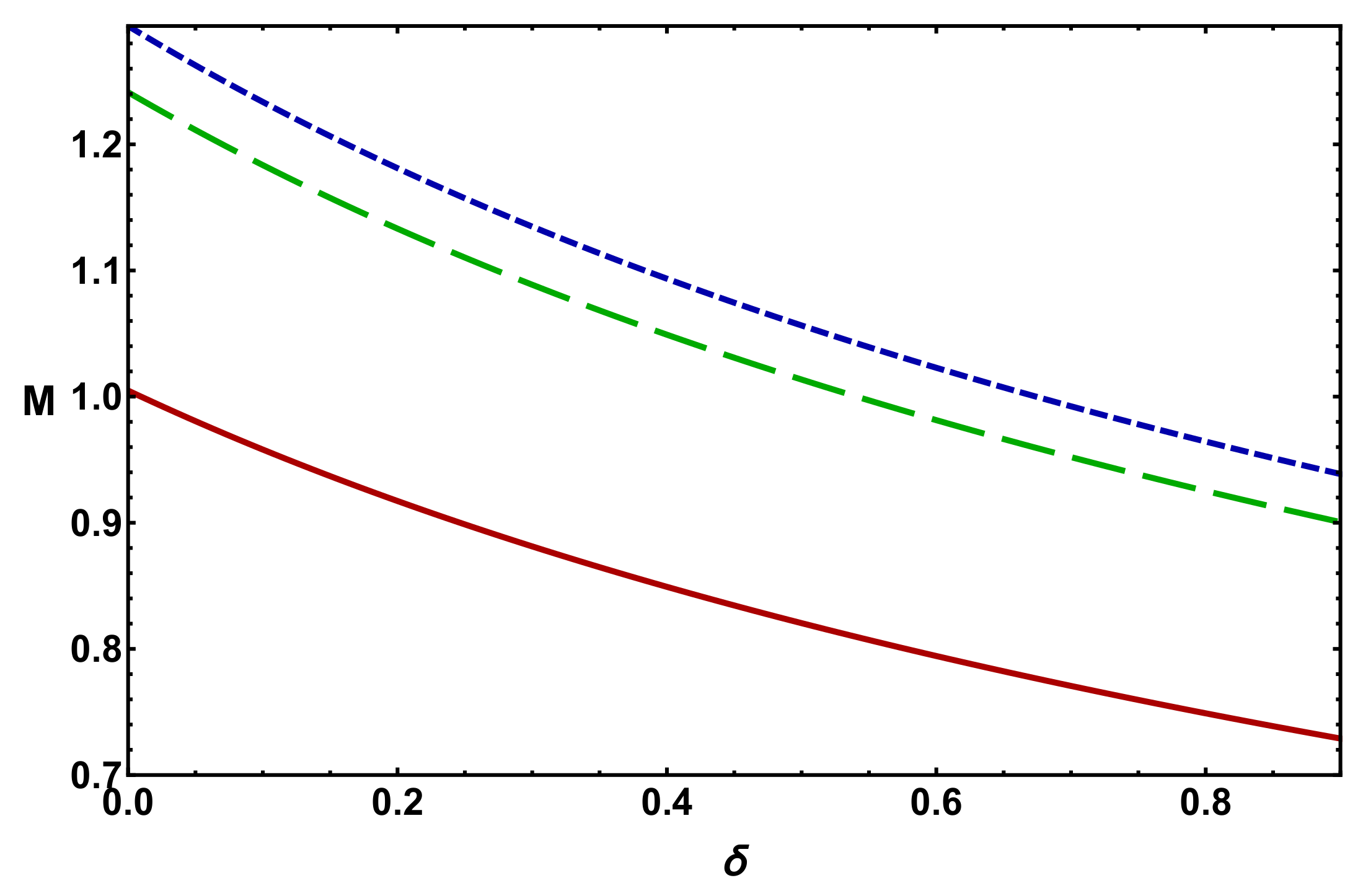
- The rotational frequency of the plasma system does not affect the amplitude of the NASWs. However, the width of the NA wave potential decreases with an increasing rotational speed of the plasma system as shown in Figure 5.
- The width of the NASWs is observed to increase with the increase in the inclination angle of the rotation of the warm degenerate plasma system. As the rotation angle increases, the height of the positive potential decreases (Figure 6).
- The presence of a static heavy nucleus not only supports the existence of a positive NASW potential but also modifies the basic features of NASWs as shown in Figure 7. The amplitude and width of the NASW potential are decreased with the increase in the heavy nucleus number density and charge state. On the other hand, the amplitude and width of the NASWs increase with the decrease in the light nucleus number density and charge state.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Sample Availability
Abbreviations
| KdV | Korteweg–de Vries |
| NAWs | Nucleus-acoustic waves |
| NASWs | Nucleus-acoustic solitary waves |
| WDMRQP | Warm degenerate magneto-rotating quantum plasma |
References
- Mamun, A.A.; Shukla, P.K. Cylindrical and spherical dust ion-acoustic solitary waves. Phys. Plasmas 2002, 9, 1468–1470. [Google Scholar] [CrossRef]
- Mamun, A.A.; Amina, M.; Schlickeiser, R. Nucleus-acoustic shock structures in a strongly coupled self-gravitating degenerate quantum plasma. Phys. Plasmas 2016, 26, 094503. [Google Scholar] [CrossRef]
- Mamun, A.A.; Amina, M.; Schlickeiser, R. Heavy nucleus-acoustic spherical solitons in self-gravitating super-dense plasmas. Phys. Plasmas 2017, 24, 042307. [Google Scholar] [CrossRef]
- Mamun, A.A. Degenerate pressure driven modified nucleus-acoustic waves in degenerate plasmas. Phys. Plasmas 2018, 25, 02402. [Google Scholar] [CrossRef]
- Jannat, S.; Mamun, A.A. Nucleus-acoustic solitary waves in white dwarfs. Chin. J. Phys. 2018, 56, 3046–3052. [Google Scholar] [CrossRef]
- Chowdhury, N.A.; Hasan, M.M.; Mannan, A.; Mamun, A.A. Nucleus-acoustic envelope solitons and their modulational instability in a degenerate quantum plasma system. Vaccum 2018, 147, 31–37. [Google Scholar] [CrossRef]
- Sultana, S.; Islam, S.; Mamun, A.A.; Schlickeiser, R. Modulated heavy nucleus-acoustic waves and associated rogue waves in a degenerate relativistic quantum plasma system. Phys. Plasmas 2018, 25, 012113. [Google Scholar] [CrossRef]
- Zaman, D.M.S.; Amina, M.; Dip, P.R.; Mamun, A.A. Nucleus-acoustic solitary waves in self-gravitating degenerate quantum plasmas. Chin. Phys. B 2018, 27, 040402. [Google Scholar] [CrossRef]
- Mamun, A.A.; Akter, J. Planar and non-planar nucleus-acoustic solitary waves in warm degenerate multi-nucleus plasmas. J. Plasma Phys. 2021, 87, 905870109. [Google Scholar] [CrossRef]
- Sahu, B.; Sinha, A.; Roychoudhury, R. Ion-acoustic waves in dense magneto-rotating quantum plasma. Phys. Plasmas 2019, 26, 072119. [Google Scholar] [CrossRef]
- Chandrasekhar, S. The density of white dwarf stars. Philos. Mag. 1931, 11, 70. [Google Scholar] [CrossRef]
- Chandrasekhar, S. The maximum mass of ideal white dwarfs. Astrophys. J. 1931, 74, 81–82. [Google Scholar] [CrossRef]
- Chandrasekhar, S. The pressure in the interior of a star. Mon. Not. R. Astron. Soc. 1936, 96, 644–647. [Google Scholar] [CrossRef]
- Fowler, R.H. On dense matter. Mon. Not. R. Astron. Soc. 1926, 87, 114–122. [Google Scholar] [CrossRef]
- Koester, D. White dwarfs: Recent developments. Astron. Astrophys. Rev. 2002, 11, 33–36. [Google Scholar] [CrossRef]
- Koester, D.; Chanmugam, G. Physics of white dwarf stars. Rep. Prog. Phys. 1990, 53, 837–916. [Google Scholar] [CrossRef]
- Horn, H.M.V. Dense astrophysical plasmas. Science 1991, 252, 384–389. [Google Scholar] [CrossRef]
- Vanderburg, A.; Johnson, J.A.; Bieryla, A.; Irwin, J.; Lewis, J.A.; Kipping, D.; Brown, W.R.; Angus, R.; Schaefer, L.; Latham, D.W.; et al. A disintegrating minor planet transiting a white dwarf. Nature 2015, 526, 546–549. [Google Scholar] [CrossRef]
- Witze, A. Space-station science ramps up. Nature 2014, 510, 196. [Google Scholar] [CrossRef]
- Chavanis, P.H. White dwarf stars in D ddimensions. Phys. Rev. D 2007, 76, 023004. [Google Scholar] [CrossRef]
- Tonks, L.; Langmuir, R. Oscillations in ionized gases. Phys. Rev. 1929, 33, 195–210. [Google Scholar] [CrossRef]
- Zaman, D.M.S.; Amina, M.; Dip, P.R.; Mamun, A.A. Planar and non-planar nucleus-acoustic shock structures in self-gravitating degenerate quantum plasma systems. Eur. Phys. J. Plus 2017, 132, 457. [Google Scholar] [CrossRef]
- Sultana, S.; Schlickeiser, R. Arbitray amplitude nucleus-acoustic solitons in multi-ion quantum plasmas with relativistically degenerate electrons. Phys. Plasmas 2018, 25, 022110. [Google Scholar] [CrossRef]
- Shapiro, L.; Teukolsky, S.A. Black Holes, White Dwarfs, and Neutron Stars: The Physics of Compact Objects; Wiley: New York, NY, USA, 1983. [Google Scholar]
- Lai, D. Oscillations in ionized gases. Rev. Mod. Phys. 2001, 73, 629. [Google Scholar] [CrossRef]
- Singh, K.; Sethi, P.; Saini, N.S. Nonlinear excitations in a degenerate relativistic magneto-rotating quantum plasma. Phys. Plasmas 2019, 26, 092104. [Google Scholar] [CrossRef]
- Saini, S.; Kaur, M.; Singh, K. Heavy nucleus-acoustic periodic waves in a degenerate relativistic magneto-rotating quantum plasma. Waves Random Complex Media 2020, 32, 743–754. [Google Scholar] [CrossRef]
- Hussain, S.; Ur-Rehman, H.; Mahmood, S. Obliquely propagating solitary wave structures in nonextensive magneto-rotating plasmas. Astrophys. Space Sci. 2014, 350, 185–190. [Google Scholar] [CrossRef]
- Saini, N.S.; Kaur, R. Ion-acoustic solitary, breathers, and freak waves inadegenerate quantum plasma. Waves Random Complex Media 2021, 1–22. [Google Scholar] [CrossRef]
- Mahmood, S.; Akhtar, N. Ion acoustic solitary waves with adiabatic ions in magnetized electron-positron-ion plasmas. Eur. Phys. J. D 2008, 49, 217–222. [Google Scholar] [CrossRef]
- Abdelsalam, U.M.; Moslem, W.M.; Shukla, P.K. Ion acoustic solitary waves in a dense pair-ion plasma containing degenerate electrons and positrons. Phys. Lett. A 2008, 372, 4057–4061. [Google Scholar] [CrossRef]
- Soltani, H.; Mohsenpour, T.; Sohbatzadeh, F. Obliquely propagating quantum solitary waves in quantum-magnetized plasma with ultra-relativistic degenerate electrons and positrons. Contrib. Plasma Phys. 2019, 49, e201900038. [Google Scholar] [CrossRef]
- Dufour, P.; Fontaine, G.; Liebert, J.; Schmidt, G.D.; Behara, N. Hot DQ white dwarfs: Something different. Astrpohys. J. 2008, 683, 978–989. [Google Scholar] [CrossRef]
- Dufour, P.; Béland, S.; Fontaine, G.; Chayer, P.; Bergeron, P. Taking advantage of the COS time-tag capability: Observation of pulsating Hot DQ white dwarfs and discovery of a new one. Astrophys. J. Lett. 2011, 733, L19. [Google Scholar] [CrossRef]
- Werner, K.; Rauch, T. Analysis of HST/COS spectra of the bare C-O stellar core H1504+65 and a high-velocity twin in the Galactic halo. Astron. Astrophys. 2015, 584, A19. [Google Scholar] [CrossRef]
- Werner, K.; Rauch, T.; Reindl, N. Spectral analysis of the extremely hot DA white dwarf PG 0948+534. Mon. Not. R. Astron. Soc. 2019, 483, 5291–5300. [Google Scholar] [CrossRef]
- Manfredi, G. How to model quantum plasmas. Fields Inst. Commun. 2005, 46, 263–287. [Google Scholar]
- Vavrukh, M.V.; Smerechynskyi, S.V. A finite temperature Chandrasekhar model: Determining the parameters and calculation of the characteristics of degenerate dwarfs. Astron. Rep. 2012, 56, 363–378. [Google Scholar] [CrossRef]
- Hossain, G.M.; Mandal, S. Revisiting equation of state for white dwarfs within finite temperature quantum field theory. arXiv 2019, 252, 384–389. [Google Scholar]
- Salpeter, E.E. Energy and pressure of a zero-temperature plasma. Astrophys. J. 1961, 134, 669–682. [Google Scholar] [CrossRef]
- Washimi, H.; Taniuti, T. Propagation of ion-acoustic solitary waves of small amplitude. Phys. Rev. Lett. 1966, 17, 996–997. [Google Scholar] [CrossRef]
- Ourabah, K.; Tribeche, M. Quantum ion-acoustic solitary waves: The effect of exchange correlation. Phys. Rev. E 2013, 88, 045101. [Google Scholar] [CrossRef]
- Malone, F.D.; Blunt, N.S.; Brown, E.W.; Lee, D.K.K.; Spencer, J.S.; Foulkes, W.M.C.; Shepherd, J.J. Accurate exchange-correlation energies for the warm dense electron gas. Phys. Rev. Lett. 2016, 117, 115701. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Akter, J.; Mamun, A.A. Nucleus-Acoustic Solitary Waves in Warm Degenerate Magneto-Rotating Quantum Plasmas. Fluids 2022, 7, 305. https://doi.org/10.3390/fluids7090305
Akter J, Mamun AA. Nucleus-Acoustic Solitary Waves in Warm Degenerate Magneto-Rotating Quantum Plasmas. Fluids. 2022; 7(9):305. https://doi.org/10.3390/fluids7090305
Chicago/Turabian StyleAkter, Jhorna, and A A Mamun. 2022. "Nucleus-Acoustic Solitary Waves in Warm Degenerate Magneto-Rotating Quantum Plasmas" Fluids 7, no. 9: 305. https://doi.org/10.3390/fluids7090305
APA StyleAkter, J., & Mamun, A. A. (2022). Nucleus-Acoustic Solitary Waves in Warm Degenerate Magneto-Rotating Quantum Plasmas. Fluids, 7(9), 305. https://doi.org/10.3390/fluids7090305






