Stability Analysis of Concrete Block Anchor on Steep-Slope Floating Breakwater
Abstract
:1. Introduction
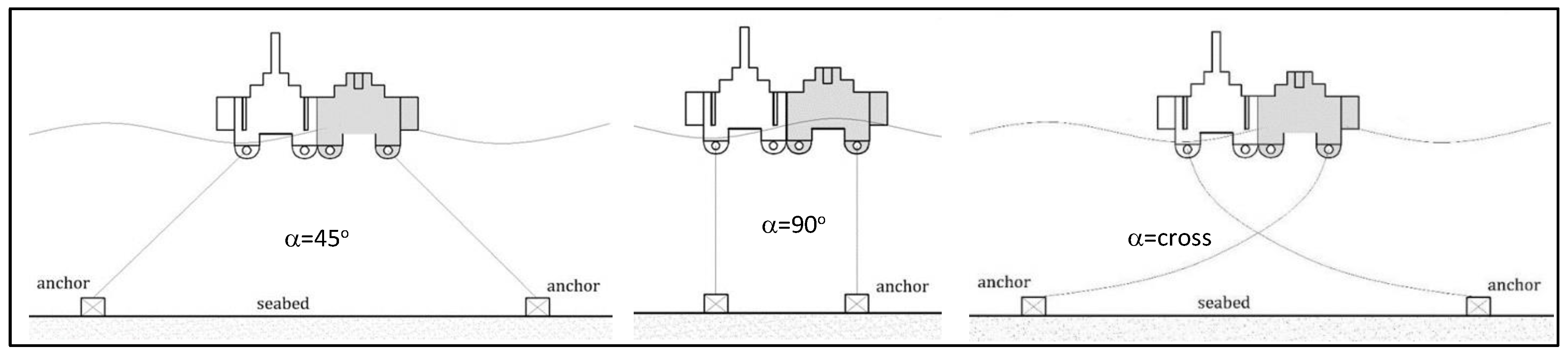
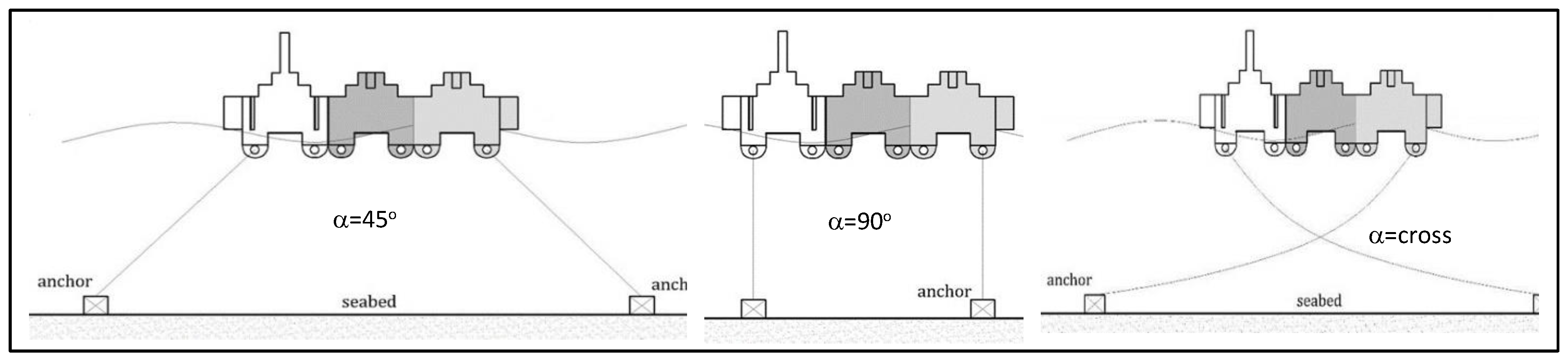
1.1. Mooring System
1.2. Concrete Block Anchor
2. Materials and Methods
2.1. Dimensional Analysis
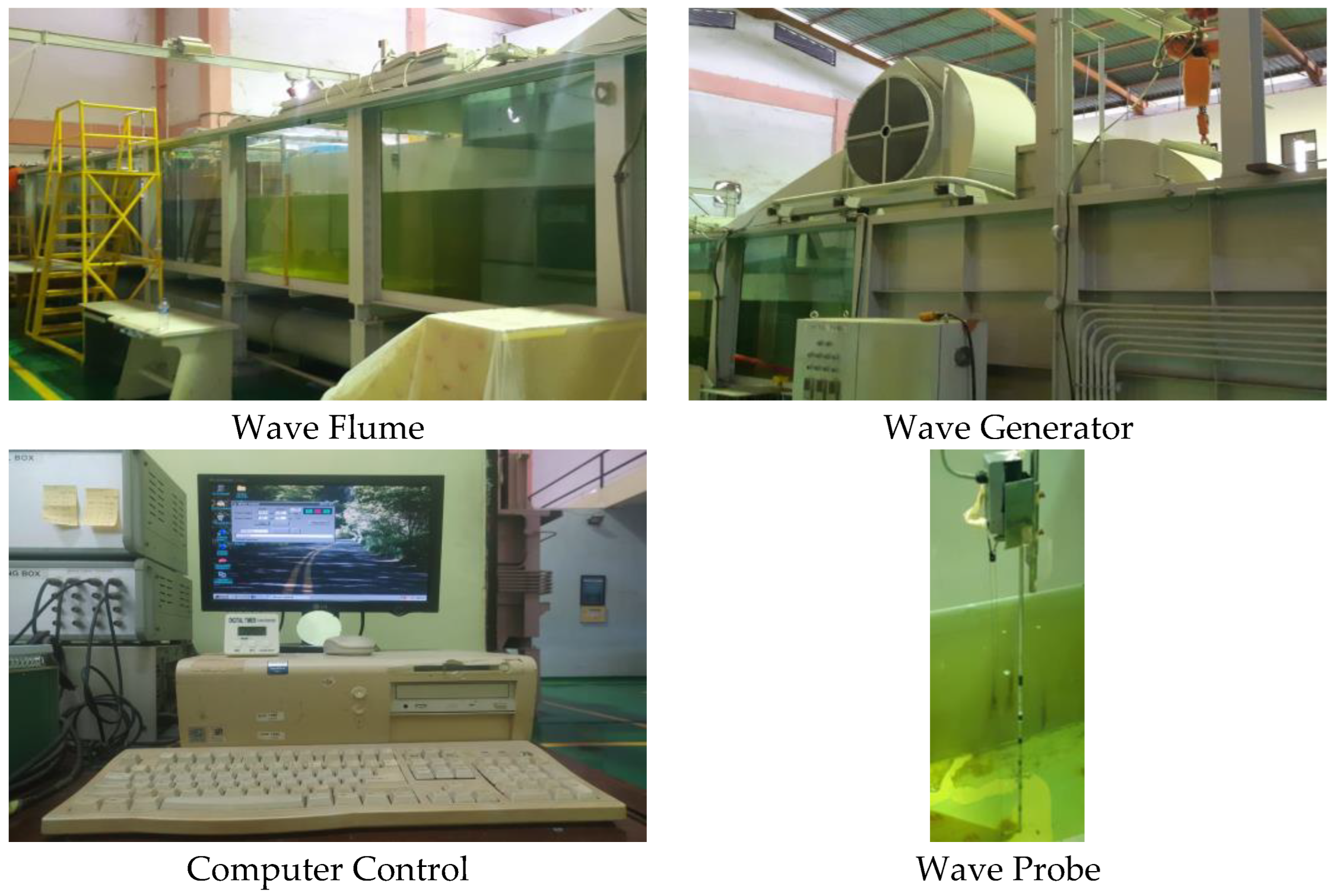
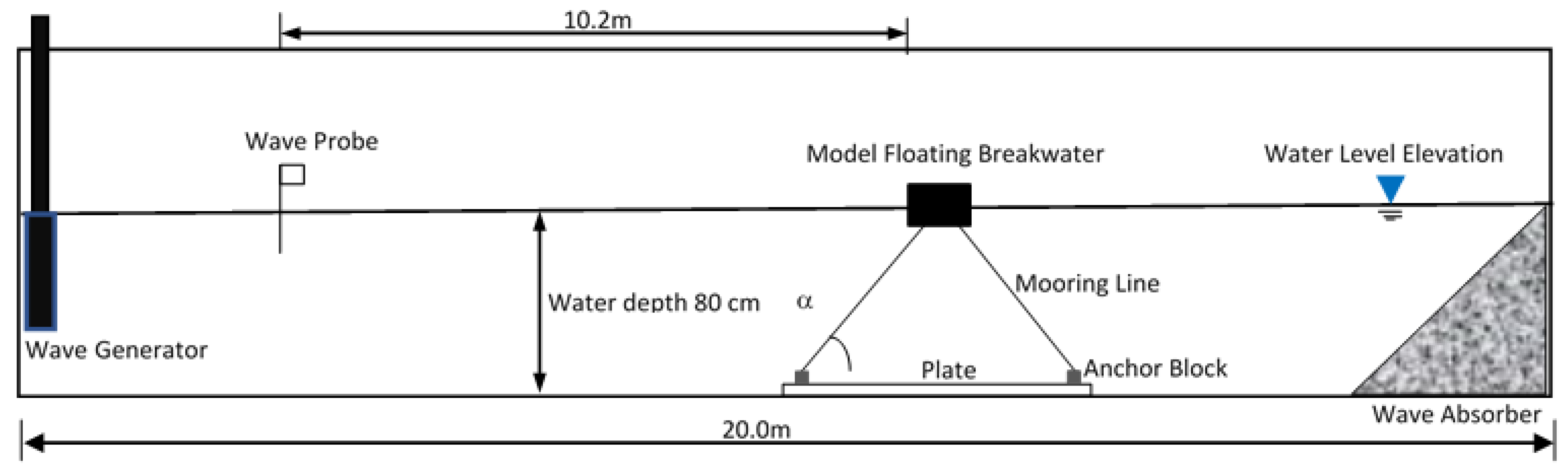
2.2. Experiment Setup
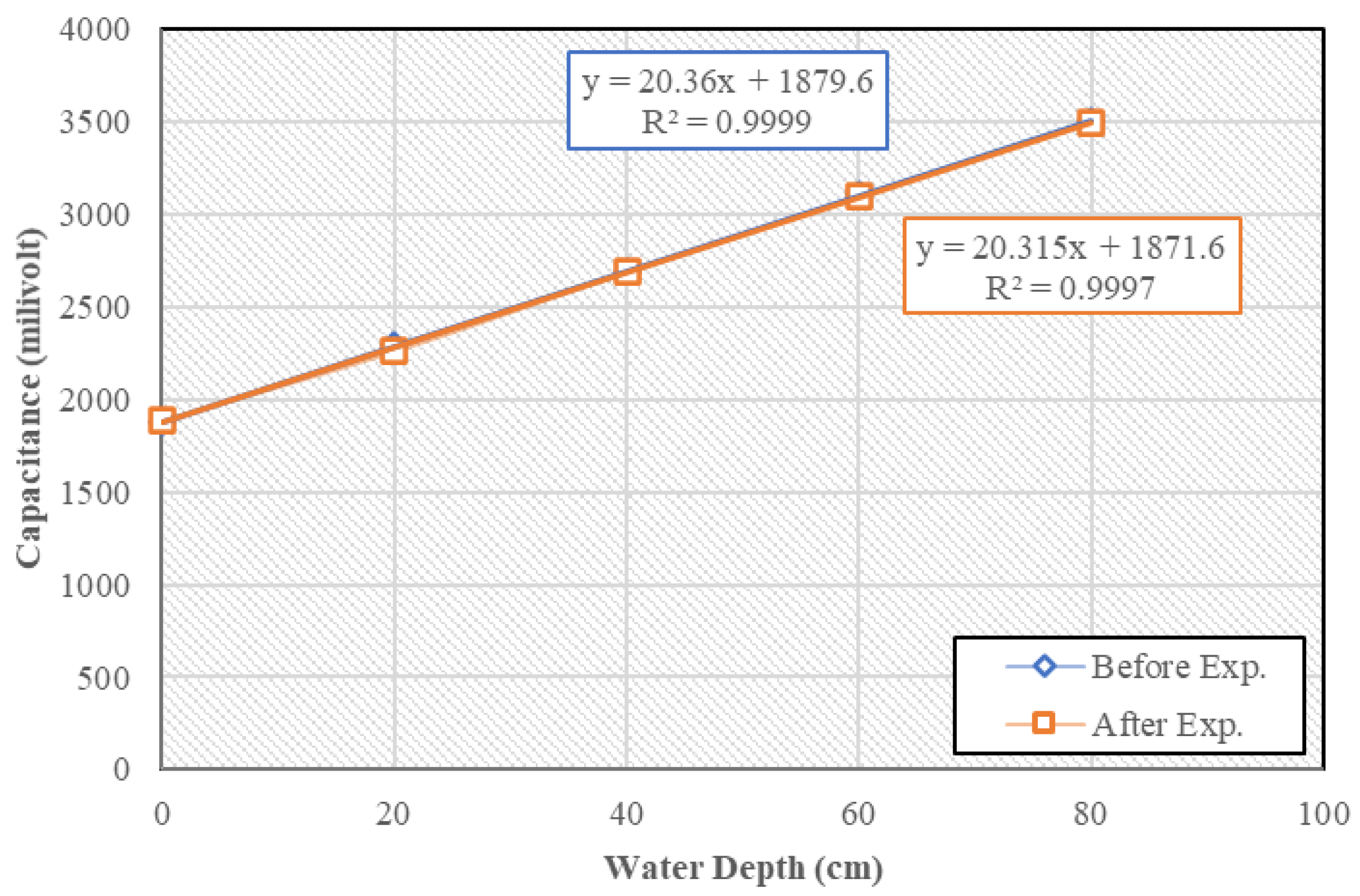
2.3. Wave Probe Calibration
2.4. Data Analysis
3. Result and Discussion
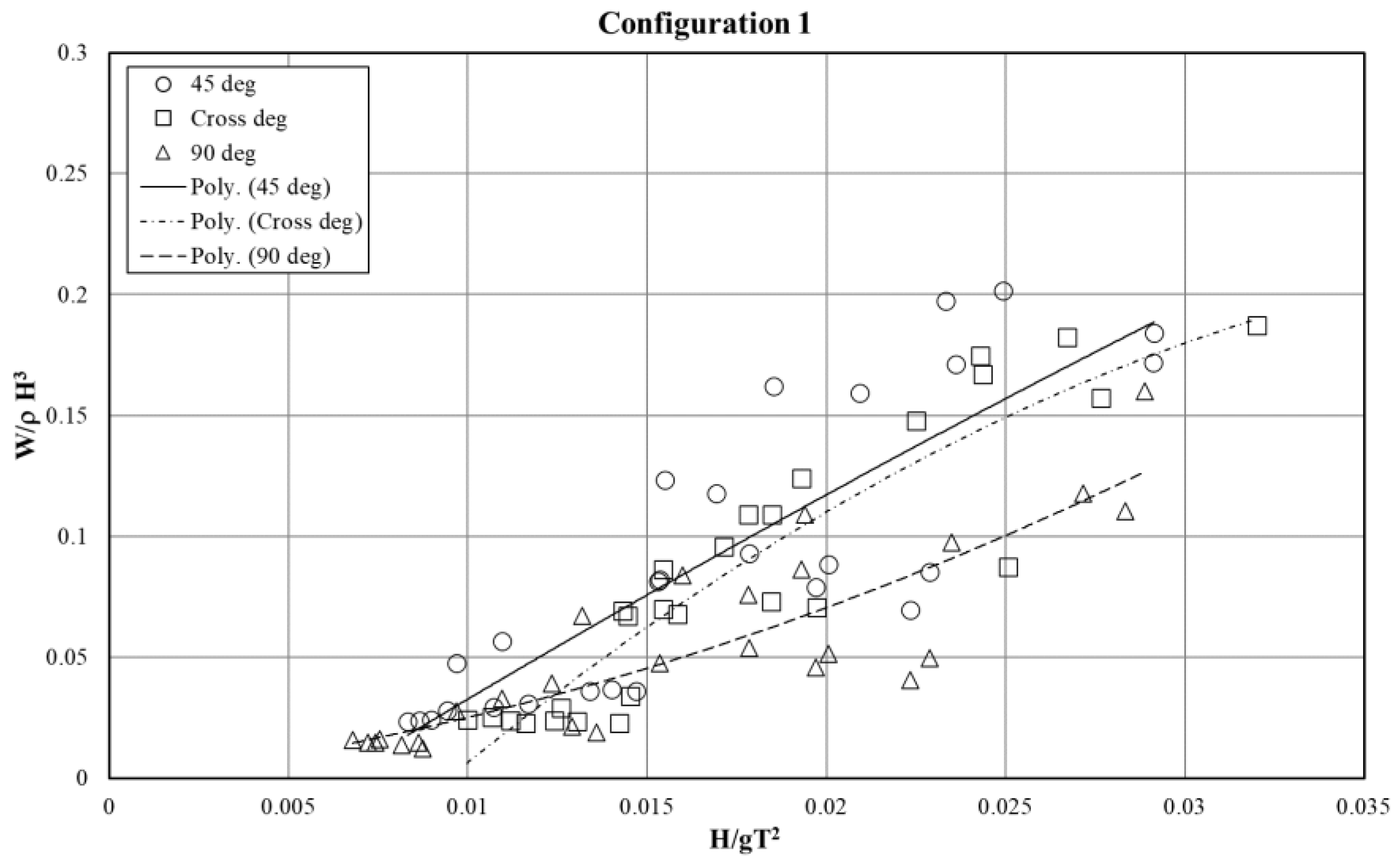
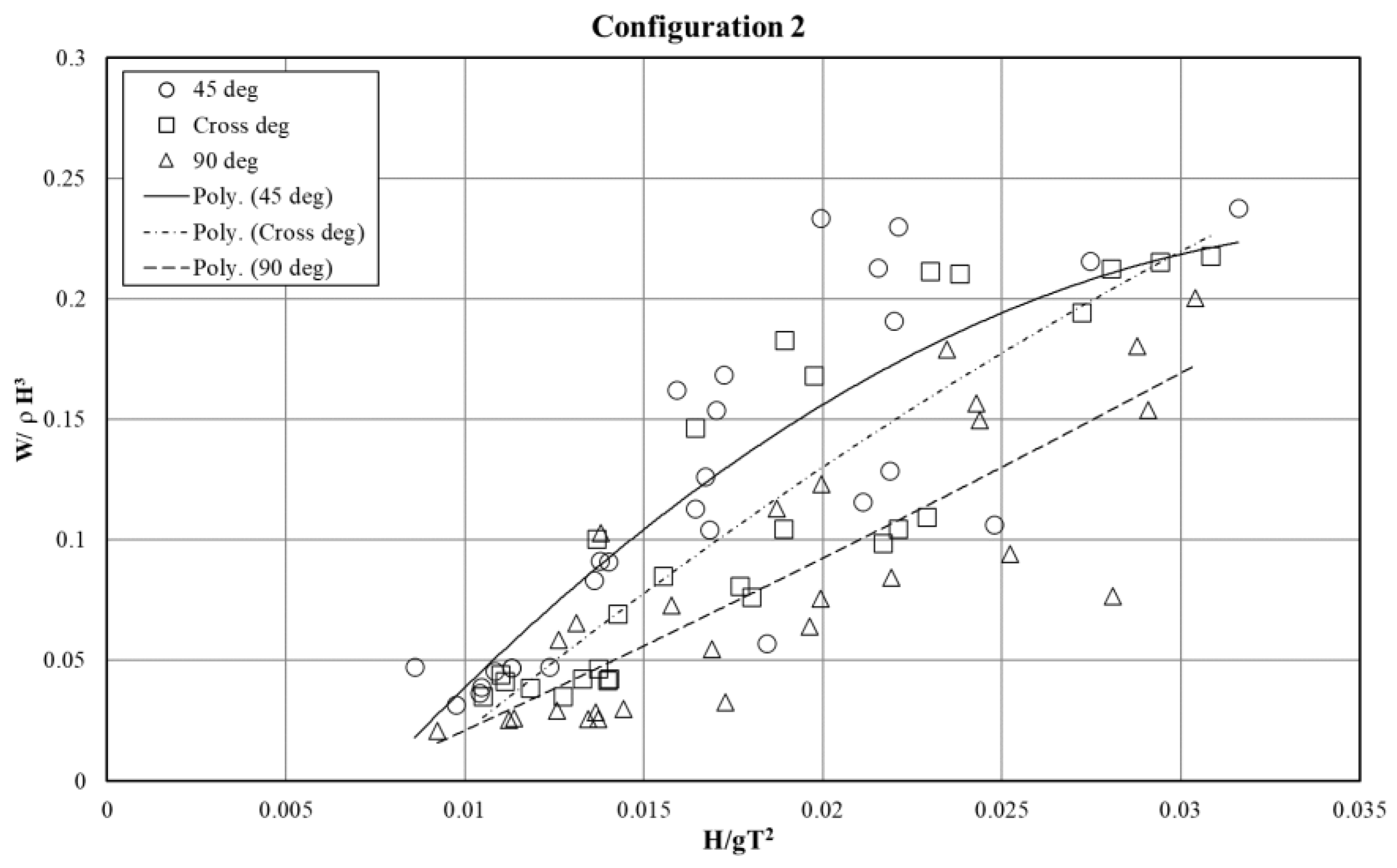
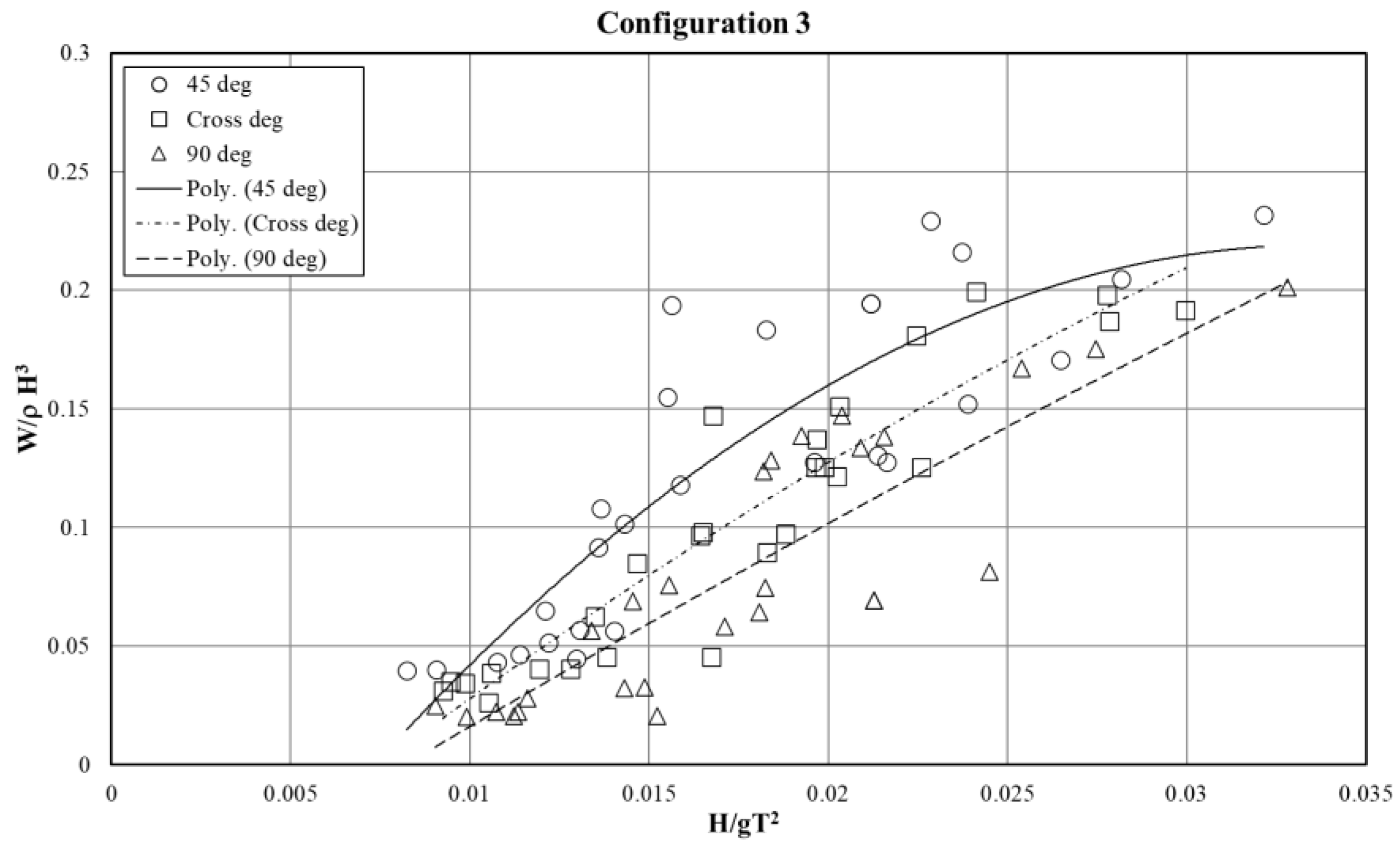
3.1. Effect of Anchor Stability Parameter (W/ρ H3) on Wave Steepness (H/gT2)
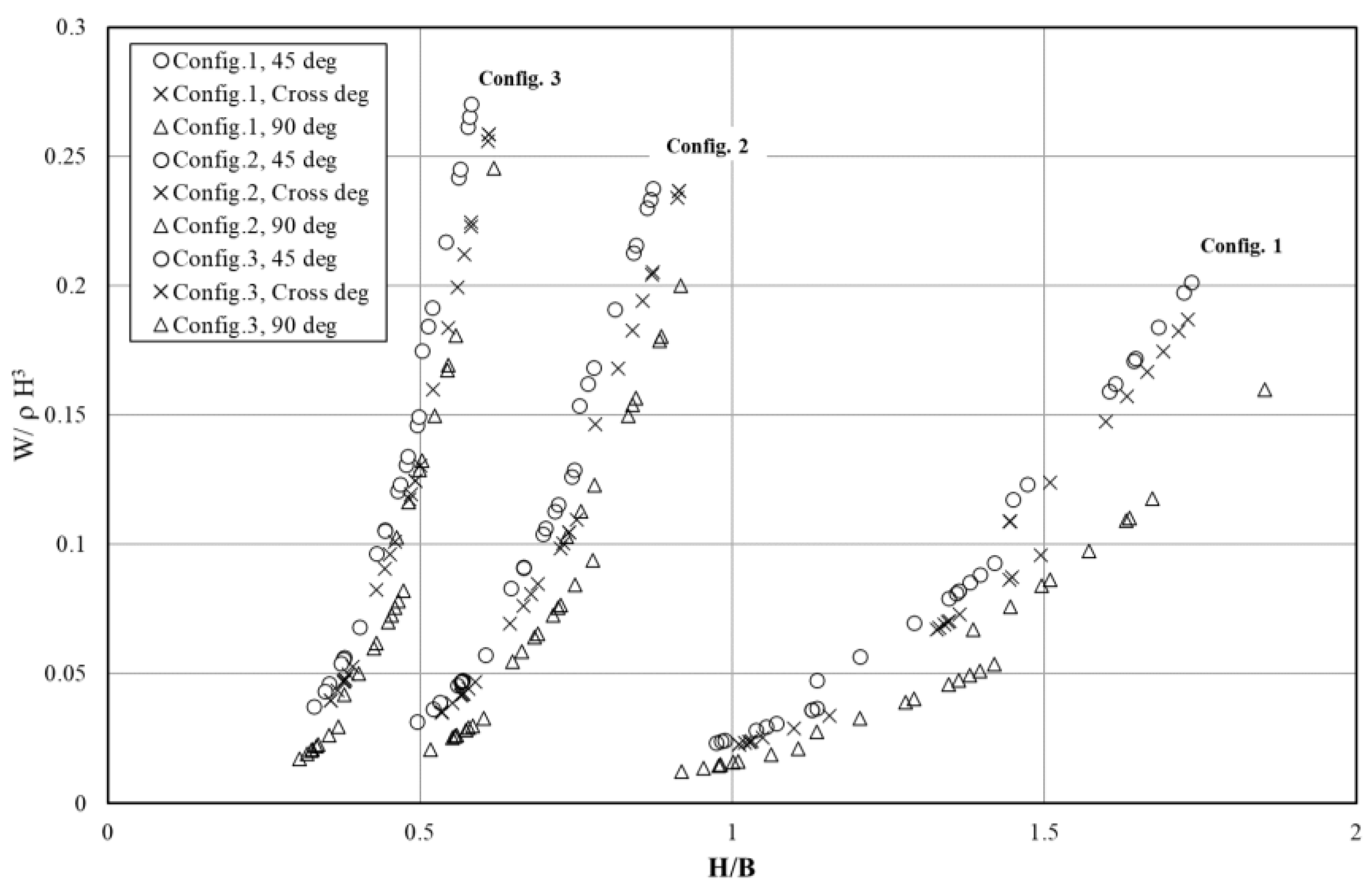
3.2. Effect of Anchor Stability Parameter (W/ρ H3) on H/B
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- National Disaster Management Agency (BNPB). The Fate of Indonesia’s Coasts in the Middle of Abrasion Threats. 2019. (In Indonesian). Available online: https://foto.kompas.com/photo/read/2019/11/19/1574158801a47/1/nasibpesisir-pantai-indonesia-di-tengah-ancaman-abrasi (accessed on 11 November 2019).
- Armono, H.D.; Bromo, B.H.; Sholihin; Sujantoko. Numerical Study of Bamboo Breakwater for Wave Reduction. Fluids 2022, 7, 14. [Google Scholar] [CrossRef]
- Armono, H.D.; Winarto, A.; Sujantoko; Ketut Suastika, I.A. laboratory study on wave transmission over hexagonal artificial reef. IOP Conf. Ser. Earth Environ. Sci 2021, 799, 012011. [Google Scholar] [CrossRef]
- Hegde, A.V.; Rao, S.; Kumar, K. Run-up, run-down and reflection characteristics of semicircular breakwater for varying seaside perforations. ISH J. Hydraul. Eng. 2012, 18, 145–151. [Google Scholar] [CrossRef]
- Venkateswarlu, V.; Karmakar, D. Numerical investigation on the wave dissipating performance due to multiple porous structures. ISH J. Hydraul. Eng. 2019, 27, 202–219. [Google Scholar] [CrossRef]
- Zhao, E.; Mu, L.; Hu, Z.; Wang, X.; Sun, J.; Zhang, Z. Physical and Numerical Investigations on Wave Run-Up and Dissipation under Breakwater with Fence Revetment. J. Mar. Sci. Eng. 2021, 9, 1355. [Google Scholar] [CrossRef]
- Lee, I.-G.; Kim, D.-H. Load Resistance Factor for Vertical Caisson Breakwater in Korea. J. Mar. Sci. Eng. 2022, 10, 468. [Google Scholar] [CrossRef]
- Mares-Nasarre, P.; Argente, G.; Gómez-Martín, M.E.; Medina, J.R. Armor Damage of Overtopped Mound Breakwaters in Depth-Limited Breaking Wave Conditions. J. Mar. Sci. Eng. 2021, 9, 952. [Google Scholar] [CrossRef]
- Cardenas-Rojas, D.; Mendoza, E.; Escudero, M.; Verduzco-Zapata, M. Assessment of the Performance of an Artificial Reef Made of Modular Elements through Small Scale Experiments. J. Mar. Sci. Eng. 2021, 9, 130. [Google Scholar] [CrossRef]
- Konispoliatis, D.N. Performance of an Array of Oscillating Water Column Devices in Front of a Fixed Vertical Breakwater. J. Mar. Sci. Eng. 2020, 8, 912. [Google Scholar] [CrossRef]
- Konispoliatis, D.N.; Mavrakos, S.A. Hydrodynamic Efficiency of a Wave Energy Converter in Front of an Orthogonal Breakwater. J. Mar. Sci. Eng. 2021, 9, 94. [Google Scholar] [CrossRef]
- McCartney, B.L. Floating breakwater design. J. Waterw. Port Coast. Ocean. Eng. 1985, 111, 304–318. [Google Scholar] [CrossRef]
- Hales, L.Z. Floating Breakwater: State of the Art Literature Review; Technical Report No. 81-1; U.S. Army Coastal Engineering Research Center, Fort Belvoir: Fairfax County, VA, USA, 1981. [Google Scholar] [CrossRef] [Green Version]
- Kelly, H. Discussion about Floating Breakwaters; Naval Facilities Engineering Command: Norfolk, VA, USA, 1999. [Google Scholar]
- Tsinker, G.P. Marine Structures Engineering: Specialized Application; An International Thomson Publishing Company: New York, NY, USA, 1995. [Google Scholar]
- Fousert, M.W. Floating Breakwater: A Theoretical Study of a Dynamic Wave Attenuating System. Master’s Thesis, Delft University of Technology, Delft, The Netherland, 2006. [Google Scholar]
- Murali, K.; Mani, J.S. Performance of cage floating breakwater. J. Waterw. Port Coast. Ocean. Eng. 1997, 123, 172–179. [Google Scholar] [CrossRef]
- Dai, J.; Wang, C.M.; Utsunomiya, T.; Duan, W. Review of recent research and development on floating breakwaters. Ocean. Eng. 2018, 158, 132–151. [Google Scholar] [CrossRef]
- Sujantoko, S.; Djatmiko, E.B.; Wardhana, W.; Armono, H.D.; Wahyudi, W. Dynamic behavior analysis of porous saw floating breakwater under regular waves. In Proceedings of the 7th International Seminar on Ocean and Coastal Engineering, Environmental and Natural Disaster Management, ISOCEEN, Surabaya, Indonesia, 6–7 November 2019; pp. 236–241. [Google Scholar] [CrossRef]
- Sujantoko, S.; Djatmiko, E.B.; Wardhana, W.; Armono, H.D.; Sholihin; Ali, M.F. Experimental study on the effect of mooring angle on mooring tension of porous saw type floating breakwater. In Proceedings of the 7th International Seminar on Ocean and Coastal Engineering, Environmental and Natural Disaster Management, ISOCEEN, Surabaya, Indonesia, 6–7 November 2019; pp. 94–99. [CrossRef]
- Sujantoko, S.; Wardhana, W.; Djatmiko, E.B.; Armono, H.D.; Putro, W.D.; Haryono, R.A. Study on wave characteristics of floating breakwaters for piling and tethered type. J. Hydraul. Eng. 2021, 12, 39–52. (In Indonesian) [Google Scholar] [CrossRef]
- Sujantoko, S.; Djatmiko, E.B.; Wardhana, W. Experimental Investigation of the Performance of Porous-Slope Floating Breakwater. Int. J. Eng. Appl. 2022, 10, 149–157. [Google Scholar] [CrossRef]
- Nece, R.E.; Richey, E.P. Wave Transmission Tests of Floating Breakwater for Oak Harbor; Water Resources Series Tech. Report 32; Harris Hydraulics Laboratory, University of Washington: Seattle, DC, USA, 1972. [Google Scholar]
- Adee, B.H.; Richey, E.P.; Christensen, D.R. Floating Breakwater Field Assessment Program, Friday Harbor, Washington; Technical Report 76-17; U.S. Army, Corps of Engineers, Coastal Engineering Research Center, Fort Belvoir: Fairfax County, VA, USA, 1976. [Google Scholar] [CrossRef]
- Jones, D.B. An Assessment of Transportable Breakwaters with Reference to the Container Offloading and Transfer System (COTS); Technical Note No. N-1529; Civil Engineering Laboratory, Naval Construction Battalion Center: Gulfport, MS, USA, 1978. [Google Scholar]
- PIANC. Floating Breakwater: A Practical Guide for Design and Construction; Report of Working Group No. 13 of the Permanent Committee II, Supplement to Buletin 85; PIANC: Brussel, Belgium, 1994. [Google Scholar]
- Sawaragi, T. Coastal Engineering: Waves, Beaches, Wave-Structure Interactions; Elsevier Science: Armsterdam, The Netherlands, 1995. [Google Scholar]
- Loukogeorgaki, E.; Angelides, D.C. Stiffness of mooring lines and performance of floating breakwater in three dimensions. Appl. Ocean. Res. 2005, 27, 187–208. [Google Scholar] [CrossRef]
- Kim, C.G.; Kim, H.-S.; Kim, T.-H.; Baik, C.-I. Monitoring of Fish Reef Installed in Koje Coastal Waters. Ocean. Polar Res. 2001, 23, 305–310. [Google Scholar]
- Cox, R.; Beach, D. Floating breakwater performance—Wave transmission and reflection, energy dissipation, motions, and restraining forces. In Proceedings of the First International Conference on the Application of Physical Modeling to Port and Coastal Protection, Porto, Putugal, 8–10 May 2006; pp. 371–381. [Google Scholar]
- Le Tirant, P. Anchoring of Floating Structures; Technip: Paris, France, 1990. [Google Scholar]
- Hughes, S.A. Physical Models and Laboratory Techniques in Coastal Engineering; World Scientific: Singapore, 1993. [Google Scholar]
- Escudero, M.; Hernández-Fontes, J.V.; Hernández, I.D.; Mendoza, E. Virtual Level Analysis Applied to Wave Flume Experiments: The Case of Waves-Cubipod Homogeneous Low-Crested Structure Interaction. J. Mar. Sci. Eng. 2021, 9, 230. [Google Scholar] [CrossRef]
- Amaechi, C.V.; Wang, F.; Ye, J. Experimental Study on Motion Characterisation of CALM Buoy Hose System under Water Waves. J. Mar. Sci. Eng. 2022, 10, 204. [Google Scholar] [CrossRef]
- Djatmiko, E.B. Perilaku Dan Operabilitas Bangunan Laut di Atas Gelombang Acak; ITS Press: Surabaya, Indonesia, 2012. [Google Scholar]



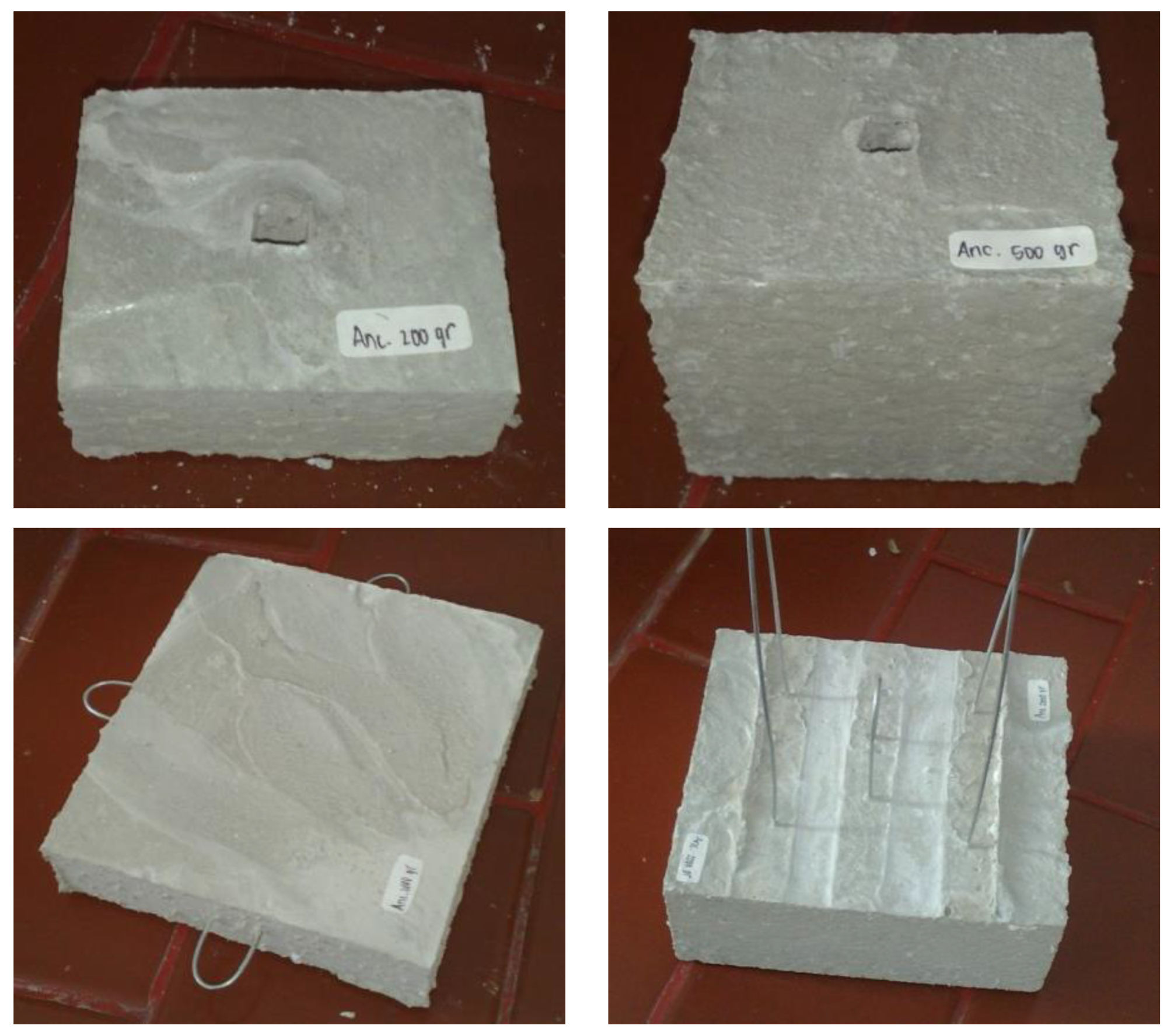

| Symbol | Parameter | Dimension |
|---|---|---|
| H | Significant wave height (m) | L |
| T | Wave period (sec) | T |
| h | Water depth (m) | L |
| α | The angle of the mooring (deg) | - |
| B | Floating breakwater width (m) | L |
| ρ | Water density (kg/m3) | ML−3 |
| g | Earth’s gravitational acceleration (m/det2) | LT−2 |
| W | Anchor weight (kg) | M |
| Density of Concrete | Weight | Volume | Length | Width | Height |
|---|---|---|---|---|---|
| (gram/cm3) | (gram) | (cm3) | (cm) | (cm) | (cm) |
| 2083.3 | 200 | 96.0015 | 6.7 | 5.7 | 2.5 |
| 500 | 240.0038 | 6.7 | 5.7 | 6.3 | |
| 1000 | 480.0077 | 14 | 12 | 2.9 | |
| 2000 | 960.0154 | 14 | 12 | 5.7 |
| Floating Breakwater | α = 45 deg | α = Cross | α = 90 deg |
|---|---|---|---|
| Config 1 | 0.21 | 0.17 | 0.13 |
| Config 2 | 0.23 | 0.21 | 0.18 |
| Config 3 | 0.24 | 0.20 | 0.17 |
| Floating Breakwater | α = 45 deg | α = Cross | α = 90 deg |
|---|---|---|---|
| Config 1 | 0.202 | 0.187 | 0.160 |
| Config 2 | 0.238 | 0.200 | 0.207 |
| Config 3 | 0.271 | 0.259 | 0.246 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sujantoko; Armono, H.D.; Djatmiko, E.B.; Putra, R.D. Stability Analysis of Concrete Block Anchor on Steep-Slope Floating Breakwater. Fluids 2022, 7, 259. https://doi.org/10.3390/fluids7080259
Sujantoko, Armono HD, Djatmiko EB, Putra RD. Stability Analysis of Concrete Block Anchor on Steep-Slope Floating Breakwater. Fluids. 2022; 7(8):259. https://doi.org/10.3390/fluids7080259
Chicago/Turabian StyleSujantoko, Haryo Dwito Armono, Eko Budi Djatmiko, and Risandi Dwirama Putra. 2022. "Stability Analysis of Concrete Block Anchor on Steep-Slope Floating Breakwater" Fluids 7, no. 8: 259. https://doi.org/10.3390/fluids7080259
APA StyleSujantoko, Armono, H. D., Djatmiko, E. B., & Putra, R. D. (2022). Stability Analysis of Concrete Block Anchor on Steep-Slope Floating Breakwater. Fluids, 7(8), 259. https://doi.org/10.3390/fluids7080259








