Experimental Investigation on the Impact of Dam-Break Induced Surges on a Vertical Wall
Abstract
:1. Introduction
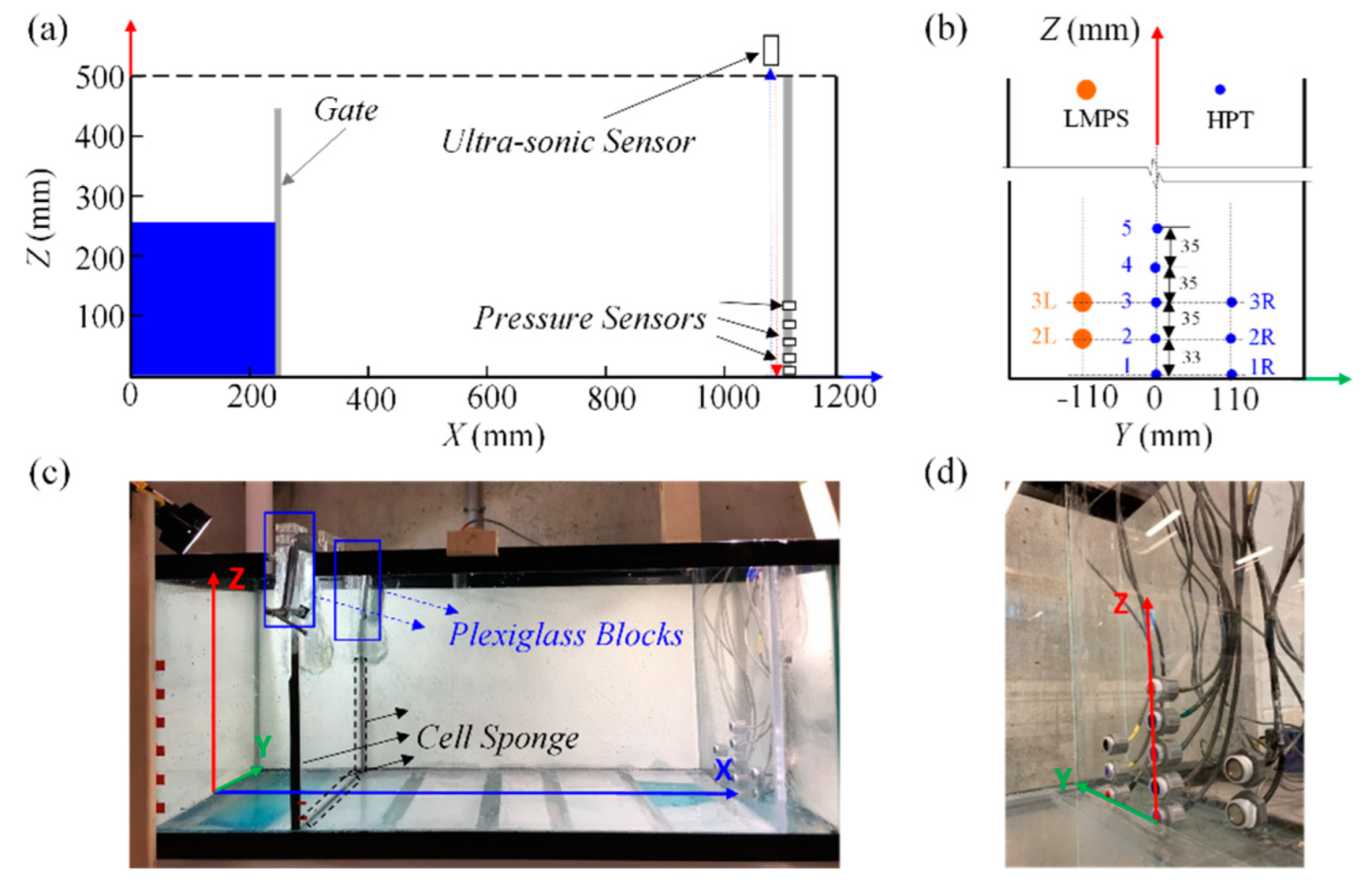
2. Experimental Setup
2.1. Tank Apparatus
2.2. Pressure Transducers
2.3. Ultrasonic Wave Sensor
2.4. Data Acquisition System
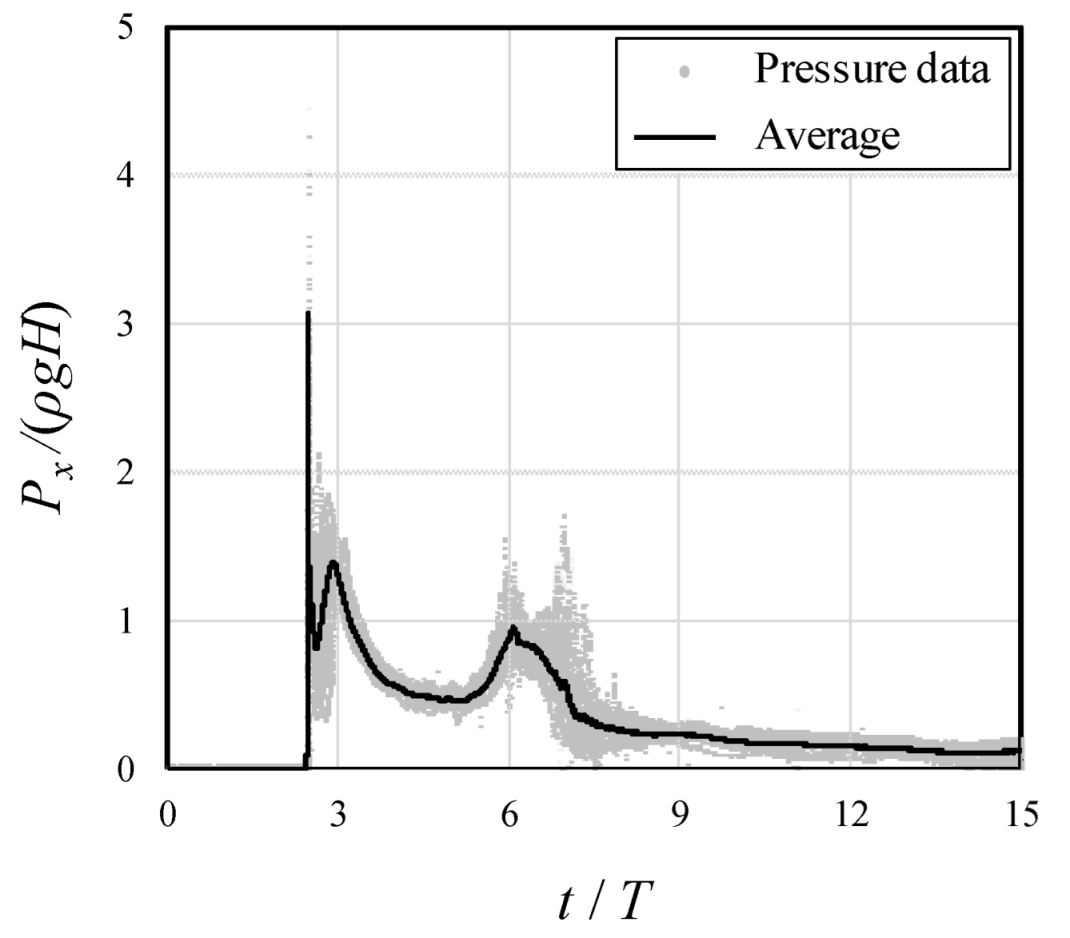
3. Pressure Data Analysis
4. Results
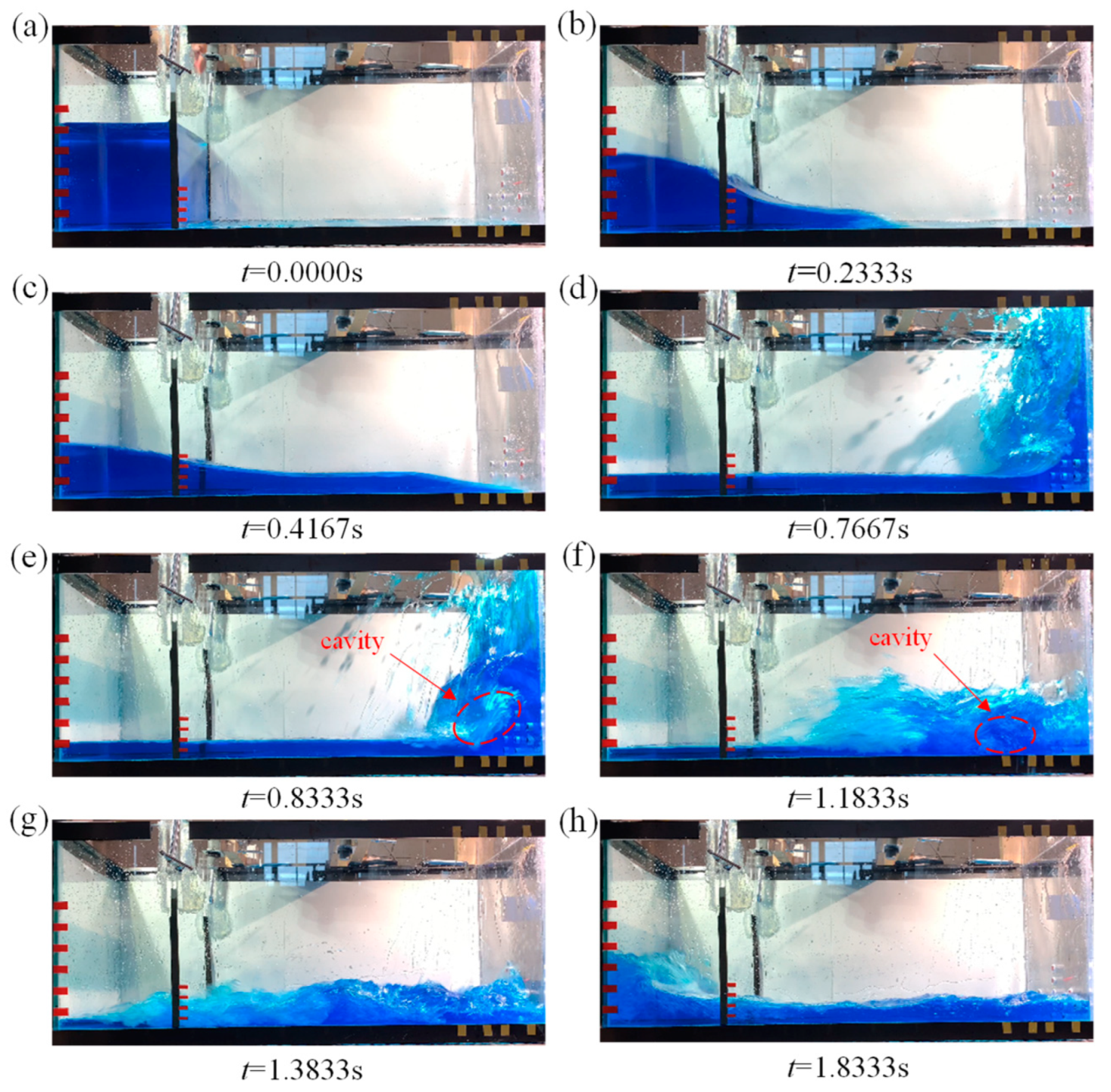
4.1. Free Surface Profiles
4.2. Time-History of the Water Surface Elevation
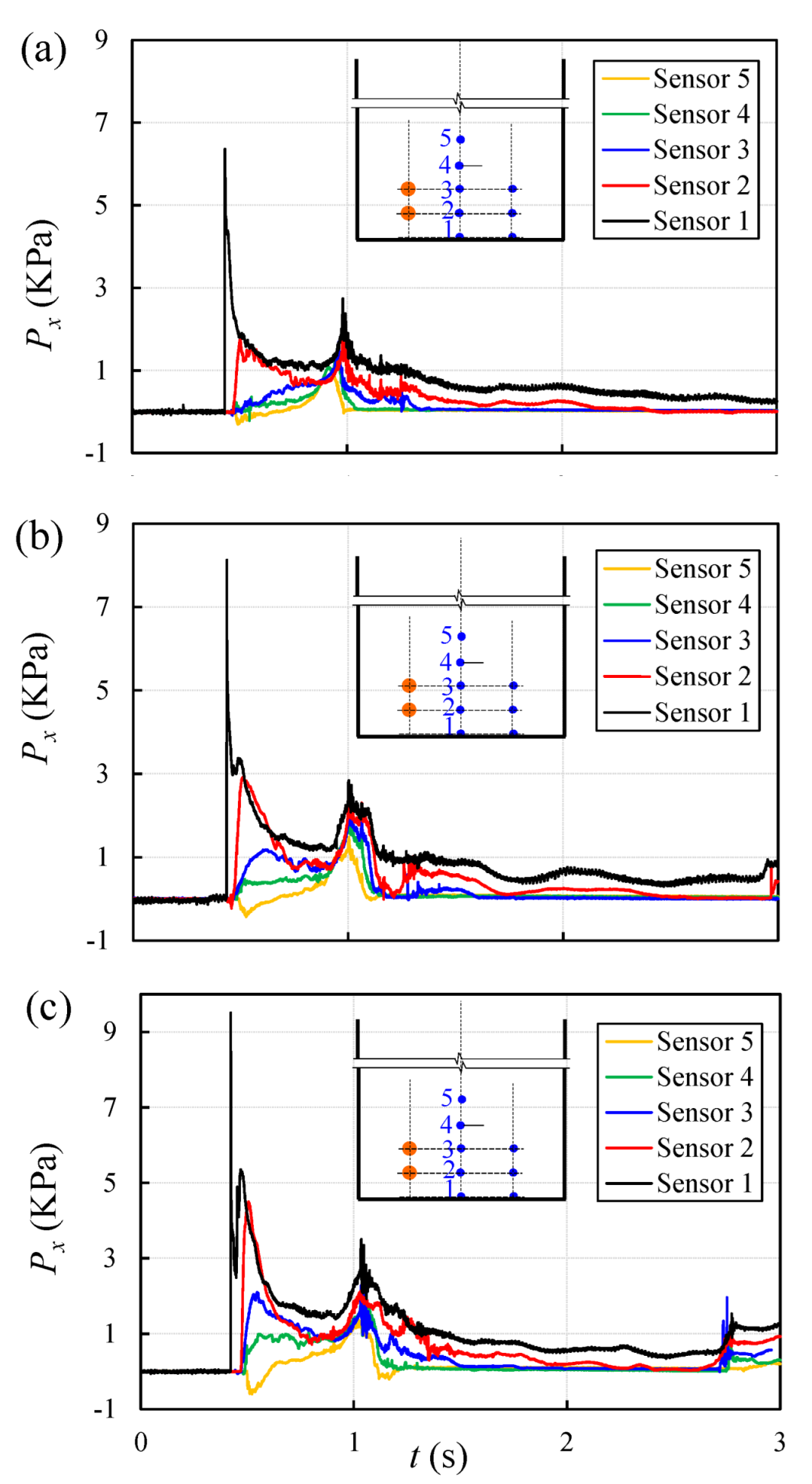
4.3. Dynamic Pressure
4.4. Vertical Distribution of Dynamic Pressure along the Wall
5. Discussion
6. Conclusions
- Detailed analysis of time-history of the water surface profiles revealed four stages of surge motion during impact with a vertical wall. The four stages of wave–wall impact were (1) impact, (2) runup, (3) fallback, and (4) breaking. The recorded time-histories of the surge depth indicated that the runup depth and impact duration increased when the impoundment depth increased.
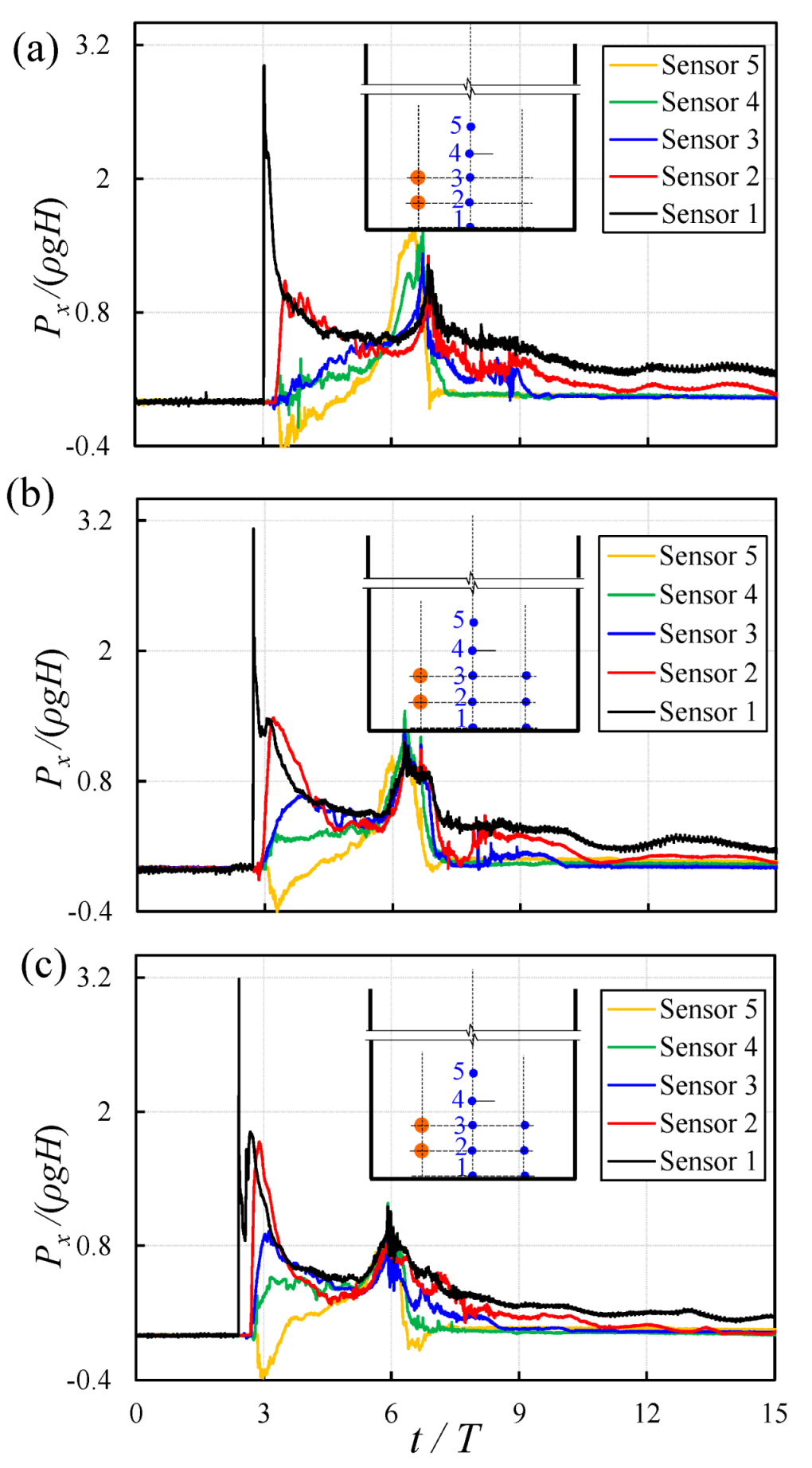
- Peak horizontal dynamic pressures were extracted from time-history for different water elevations to study the pressure distribution of the surge during the impact. It was found that the horizontal dynamic pressure at the bed was three times higher than the initial hydrostatic pressure at the reservoir location. Negative pressures generated by the suction effect generated during the fast runup were observed all the way up at the highest transducer level (i.e., z = 140 mm).
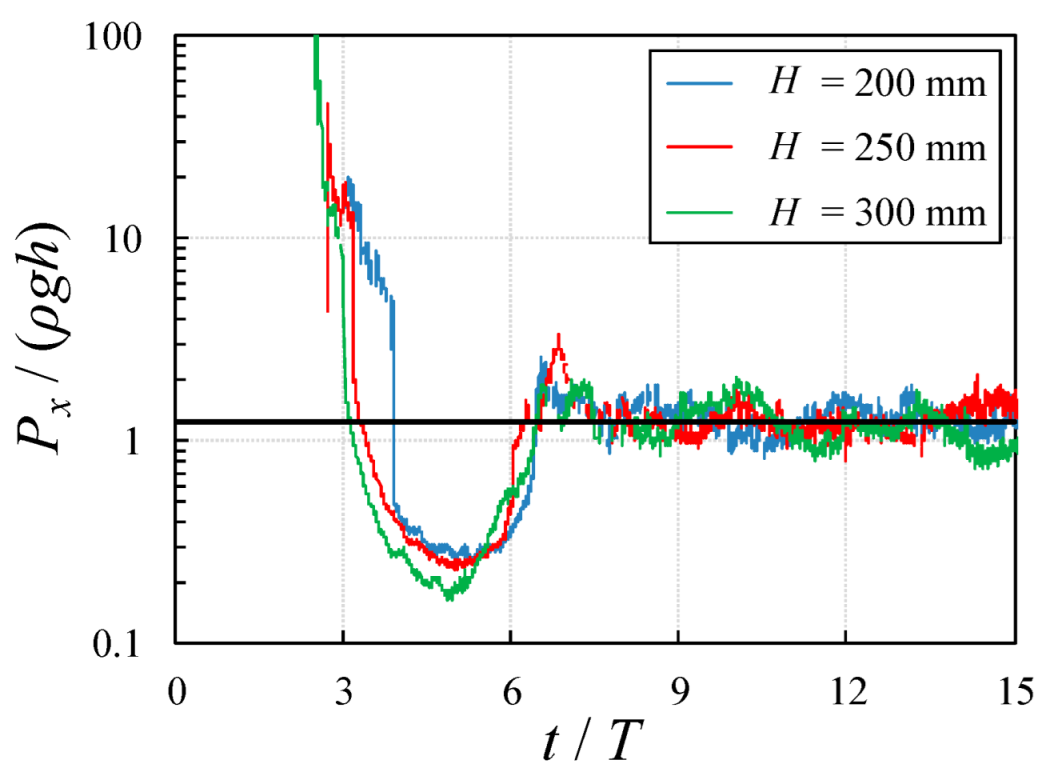
- The time lag between the horizontal dynamic pressure and surge height showed an inverse relationship between dynamic pressure and surge height curves during the impact. It was found that the horizontal dynamic pressure reached its maximum value when the wave runup height was at its minimum level. After the surge started falling off the wall and breaking at its base, on the bed, the magnitude of the dynamic pressure was close to that of the hydrostatic pressure. Additionally, a detailed analysis of the dynamic to static pressure ratio at transducer 1 showed that the normalized pressure was independent of impoundment depth.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Mikami, T.; Shibayama, T.; Esteban, M.; Takabatake, T.; Nakamura, R.; Nishida, Y.; Achiari, H.; Rusli, A.; Marzuki, A.G.; Marzuki, M.F.H.; et al. Field Survey of the 2018 Sulawesi Tsunami: Inundation and Run-up Heights and Damage to Coastal Communities. Pure Appl. Geophys. 2019, 176, 3291–3304. [Google Scholar] [CrossRef]
- Nistor, I.; Palermo, D.; Cornett, A.; Al-Faesly, T. Experimental and Numerical Modeling of Tsunami Loading on Structures. Coast. Eng. Proc. 2011, 1. [Google Scholar] [CrossRef] [Green Version]
- Palermo, D.; Nistor, I.; Saatcioglu, M.; Ghobarah, A. Impact and damage to structures during the 27 February 2010 Chile tsunami. Can. J. Civ. Eng. 2013, 40, 750–758. [Google Scholar] [CrossRef]
- Shibayama, A.; Kihara, N.; Miyagawa, Y.; Takabatake, D.; Kaida, H. Large Scale Experiments on Response of RC Tide Wall to Tsunami Debris Impact. In Proceedings of the Twenty-fifth International Ocean and Polar Engineering Conference, Kona, HI, USA, 21–26 June 2015. [Google Scholar]
- AAl-Faesly, T.; Palermo, D.; Nistor, I.; Cornett, A. Experimental modeling of extreme hydrodynamic forces on structural models. Int. J. Prot. Struct. 2012, 3, 477–505. [Google Scholar] [CrossRef]
- Nistor, I.; Palermo, D.; Nouri, Y.; Murty, T.; Saatcioglu, M. Tsunami-Induced Forces on Structures. In Handbook of Coastal and Ocean Engineering; Kim, Y.C., Ed.; World Scientific: Singapore, 2009; pp. 261–286. [Google Scholar]
- Wuthrich, D.; Pfister, M.; Nistor, I.; Schleiss, A.J. Experimental Study of Tsunami-Like Waves Generated with a Vertical Release Technique on Dry and Wet Beds. J. Waterw. Port Coast. Ocean. Eng. 2018, 144, 04018006. [Google Scholar] [CrossRef]
- Wuthrich, D.; Pfister, M.; Nistor, I.; Schleiss, A.J. Effect of building overtopping on induced loads during extreme hydrodynamic events. J. Hydraul. Res. 2019, 58, 289–304. [Google Scholar] [CrossRef]
- Nouri, Y.; Nistor, I.; Palermo, D.; Cornett, A. Experimental Investigation of Tsunami Impact on Free Standing Structures. Coast. Eng. J. 2010, 52, 43–70. [Google Scholar] [CrossRef]
- St-Germain, P.; Nistor, I.; Townsend, R.; Shibayama, T. Smoothed-Particle Hydrodynamics Numerical Modeling of Structures Impacted by Tsunami Bores. J. Waterw. Port Coast. Ocean. Eng. 2014, 140, 66–81. [Google Scholar] [CrossRef]
- Stolle, J.; Ghodoosipour, B.; Derschum, C.; Nistor, I.; Petriu, E.; Goseberg, N. Swing gate generated dam-break waves. J. Hydraul. Res. 2019, 57, 675–687. [Google Scholar] [CrossRef]
- Lobovsky, L.; Botia-Vera, E.; Castellana, F.; Mas-Soler, J.; Souto-Iglesias, A. Experimental investigation of dynamic pressure loads during dam break. J. Fluids Struct. 2014, 48, 407–434. [Google Scholar] [CrossRef] [Green Version]
- Häfen, H.V.; Stolle, J.; Goseberg, N.; Nistor, I. Lift and Swing Gate Modelling For Dam-break Generation with a Particle-Based Method. In Proceedings of the 7th IAHR International Symposium on Hydrolic Strcutures, Aachen, Germany, 15–18 May 2018. [Google Scholar]
- Rossetto, T.; Allsop, W.; Charvet, I.; Robinson, D.I. Physical modelling of tsunami using a new pneumatic wave generator. Coast. Eng. 2011, 58, 517–527. [Google Scholar] [CrossRef]
- Nistor, I.; Goseberg, N.; Stolle, J.; Mikami, T.; Shibayama, T.; Nakamura, R.; Matsuba, S. Experimental Investigations of Debris Dynamics over a Horizontal Plane. J. Waterw. Port Coast. Ocean. Eng. 2017, 143, 04016022. [Google Scholar] [CrossRef]
- Stolle, J.; Takabatake, T.; Hamano, G.; Ishii, H.; Iimura, K.; Shibayama, T.; Nistor, I.; Goseberg, N.; Petriu, E. Debris transport over a sloped surface in tsunami-like flow conditions. Coast. Eng. J. 2019, 61, 241–255. [Google Scholar] [CrossRef]
- Chanson, H. Tsunami Surges on Dry Coastal Plains: Application of Dam Break Wave Equations. Coast. Eng. J. 2006, 48, 355–370. [Google Scholar] [CrossRef] [Green Version]
- St-Germain, P.; Nistor, I.; Townsend, R. Numerical modeling of tsunami-induced hydrodynamic forces on onshore structures using SPH. In Proceedings of the 33rd International Coference on Coastal Engineering, Santander, Sapin, 1–6 July 2012; ASCE: Reston, VA, USA, 2012. [Google Scholar]
- Kamra, M.M.; Mohd, N.; Liu, C.; Sueyoshi, M.; Hu, C.H. Numerical and experimental investigation of three-dimensionality in the dam-break flow against a vertical wall. J. Hydrodyn. 2018, 30, 682–693. [Google Scholar] [CrossRef]
- Wuthrich, D.; Pfister, M.; Schleiss, A.J. Effect of bed roughness on tsunami-like waves and induced loads on buildings. Coast. Eng. 2019, 152, 103508. [Google Scholar] [CrossRef]
- Ritter, A. Die Fortpflanzung der Wasserwellen (The propagation of water waves). Z. Ver. Dtsch. Ing. 1892, 36, 947–954. [Google Scholar]
- Dressler, R.F. Hydraulic Resistance Effect Upon the Dam-Break Functions. J. Res. Natl. Bur. Stand. 1952, 49, 217–225. [Google Scholar] [CrossRef]
- Dressler, R.F. Comparison of theories and experiments for the hydraulic dam-break wave. Int. Assoc. Sci. Hydrol. 1954, 3, 319–328. [Google Scholar]
- Chanson, H. Application of the method of characteristics to the dam break wave problem. J. Hydraul. Res. 2009, 47, 41–49. [Google Scholar] [CrossRef] [Green Version]
- Martin, J.C.; Moyce, W.J. An Experimental Study of the Collapse of Liquid Columns on a Rigid Horizontal Plane. Philos. Trans. R. Soc. Lond. Ser. Math. Phys. Sci. 1952, 244, 312–324. [Google Scholar]
- Hu, C.H.; Sueyoshi, M. Numerical Simulation and Experiment on Dam Break Problem. J. Mar. Sci. Appl. 2010, 9, 109–114. [Google Scholar] [CrossRef]
- Hernández-Fontes, J.V.; Vitola, M.A.; Silva, M.C.; Esperança, P.d.T.T.; Sphaier, S.H. On the Generation of Isolated Green Water Events Using Wet Dam-Break. J. Offshore Mech. Arct. Eng. 2018, 140, 051101. [Google Scholar] [CrossRef]
- Hernández-Fontes, J.V.; Esperança, P.d.T.T.; Graniel, J.F.B.; Sphaier, S.H.; Silva, R. Green Water on A Fixed Structure Due to Incident Bores: Guidelines and Database for Model Validations Regarding Flow Evolution. Water 2019, 11, 2584. [Google Scholar] [CrossRef] [Green Version]
- Hernández-Fontes, J.V.; Vitola, M.A.; Esperança, P.T.T.; Sphaier, S.H.; Silva, R. Patterns and vertical loads in water shipping in systematic wet dam-break experiments. Ocean. Eng. 2020, 197, 106891. [Google Scholar] [CrossRef]
- Hernández-Fontes, J.V.; Esperança, P.T.T.; Silva, R.; Mendoza, E.; Sphaier, S.H. Violent water-structure interaction: Overtopping features and vertical loads on a fixed structure due to broken incident flows. Mar. Struct. 2020, 74, 102816. [Google Scholar] [CrossRef]
- Soares-Frazao, S.; Zech, Y. Experimental study of dam-break flow against an isolated obstacle. J. Hydraul. Res. 2007, 45, 27–36. [Google Scholar] [CrossRef]
- Soares-Frazao, S.; Canelas, R.; Cao, Z.X.; Cea, L.; Chaudhry, H.M.; Moran, A.D.; El Kadi, K.; Ferreira, R.; Cadorniga, I.F.; Gonzalez-Ramirez, N.; et al. Dam-break flows over mobile beds: Experiments and benchmark tests for numerical models. J. Hydraul. Res. 2012, 50, 364–375. [Google Scholar] [CrossRef]
- Kleefsman, K.M.T.; Fekken, G.; Veldman, A.E.P.; Iwanowski, B.; Buchner, B. A Volume-of-Fluid based simulation method for wave impact problems. J. Comput. Phys. 2005, 206, 363–393. [Google Scholar] [CrossRef] [Green Version]
- Wüthrich, D.; Pfister, M.; Schleiss, A. Example of wave impact on a residential house. In Sustainable Hydraulics in the Era of Globle Change. In Proceedings of the 4th IAHR European Congress, Liege, Belgium, 27–29 July 2016; CRC Press: Boca Raton, FL, USA, 2016. [Google Scholar]
- Karunya, R.; Rebeca Roldan, G.; Hocine, O.; Stefan, S.; Matthias, K.; Koen Van, D.; Julien De, R.; Tom, V.; Koen, T. Loading of Vertical Walls by Overtopping Bores using Pressure and Force Sensors—A Large Scale Model Study. In Proceedings of the Coastal Engineering Proceedings, Santander, Spain, 1–6 July 2012; ASCE: Reston, VA, USA, 2012. [Google Scholar]
- Wuthrich, D.; Pfister, M.; Nistor, I.; Schleiss, A.J. Experimental study on forces exerted on buildings with openings due to extreme hydrodynamic events. Coast. Eng. 2018, 140, 72–86. [Google Scholar] [CrossRef]
- Lee, T.H.; Zhou, Z.Q.; Cao, Y.S. Numerical simulations of hydraulic jumps in water sloshing and water impact. J. Fluids Eng. 2002, 124, 215–226. [Google Scholar] [CrossRef]
- Kocaman, S.; Ozmen-Cagatay, H. The effect of lateral channel contraction on dam break flows: Laboratory experiment. J. Hydrol. 2012, 432, 145–153. [Google Scholar] [CrossRef]
- Liu, S.; Nistor, I.; Mohammadian, M. Evaluation of the Solid Boundary Treatment Methods in SPH. Int. J. Ocean. Coast. Eng. 2018, 1, 1840002. [Google Scholar] [CrossRef]
- Lauber, G.; Hager, W.H. Experiments to dambreak wave: Horizontal channel. J. Hydraul. Res. 1998, 36, 291–307. [Google Scholar] [CrossRef]
- Kim, S.-Y.; Kim, K.-H.; Kim, Y. Comparative study on pressure sensors for sloshing experiment. Ocean. Eng. 2015, 94, 199–212. [Google Scholar] [CrossRef] [Green Version]
- Okada, T.; Sugano, T.; Ishikawa, T.; Ohgi, T.; Takai, S.; Hamabe, C. Structural Design Method of Buildings for Tsunami Resistance (SMBTR); The Building Center of Japan: Tokyo, Japan, 2005. [Google Scholar]
- Hernández, I.D.; Hernández-Fontes, J.V.; Vitola, M.A.; Silva, M.C.; Esperança, P.T.T. Water elevation measurements using binary image analysis for 2D hydrodynamic experiments. Ocean. Eng. 2018, 157, 325–338. [Google Scholar] [CrossRef]
- Kocaman, S.; Güzel, H.; Evangelista, S.; Ozmen-Cagatay, H.; Viccione, G. Experimental and Numerical Analysis of a Dam-Break Flow through Different Contraction Geometries of the Channel. Water 2020, 12, 1124. [Google Scholar] [CrossRef] [Green Version]





| Test No. | H (mm) | Number of Repetitions |
|---|---|---|
| 1 | 200 | 10 |
| 2 | 250 | 10 |
| 3 | 300 | 40 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, S.; Nistor, I.; Mohammadian, A.; Azimi, A.H. Experimental Investigation on the Impact of Dam-Break Induced Surges on a Vertical Wall. Fluids 2022, 7, 258. https://doi.org/10.3390/fluids7080258
Liu S, Nistor I, Mohammadian A, Azimi AH. Experimental Investigation on the Impact of Dam-Break Induced Surges on a Vertical Wall. Fluids. 2022; 7(8):258. https://doi.org/10.3390/fluids7080258
Chicago/Turabian StyleLiu, Shilong, Ioan Nistor, Abdolmajid Mohammadian, and Amir H. Azimi. 2022. "Experimental Investigation on the Impact of Dam-Break Induced Surges on a Vertical Wall" Fluids 7, no. 8: 258. https://doi.org/10.3390/fluids7080258
APA StyleLiu, S., Nistor, I., Mohammadian, A., & Azimi, A. H. (2022). Experimental Investigation on the Impact of Dam-Break Induced Surges on a Vertical Wall. Fluids, 7(8), 258. https://doi.org/10.3390/fluids7080258









