Design Parameters on Impingement Steam Jet Heat Transfer of Continuous Liquid Food Sterilization
Abstract
:1. Introduction
2. Materials and Methods
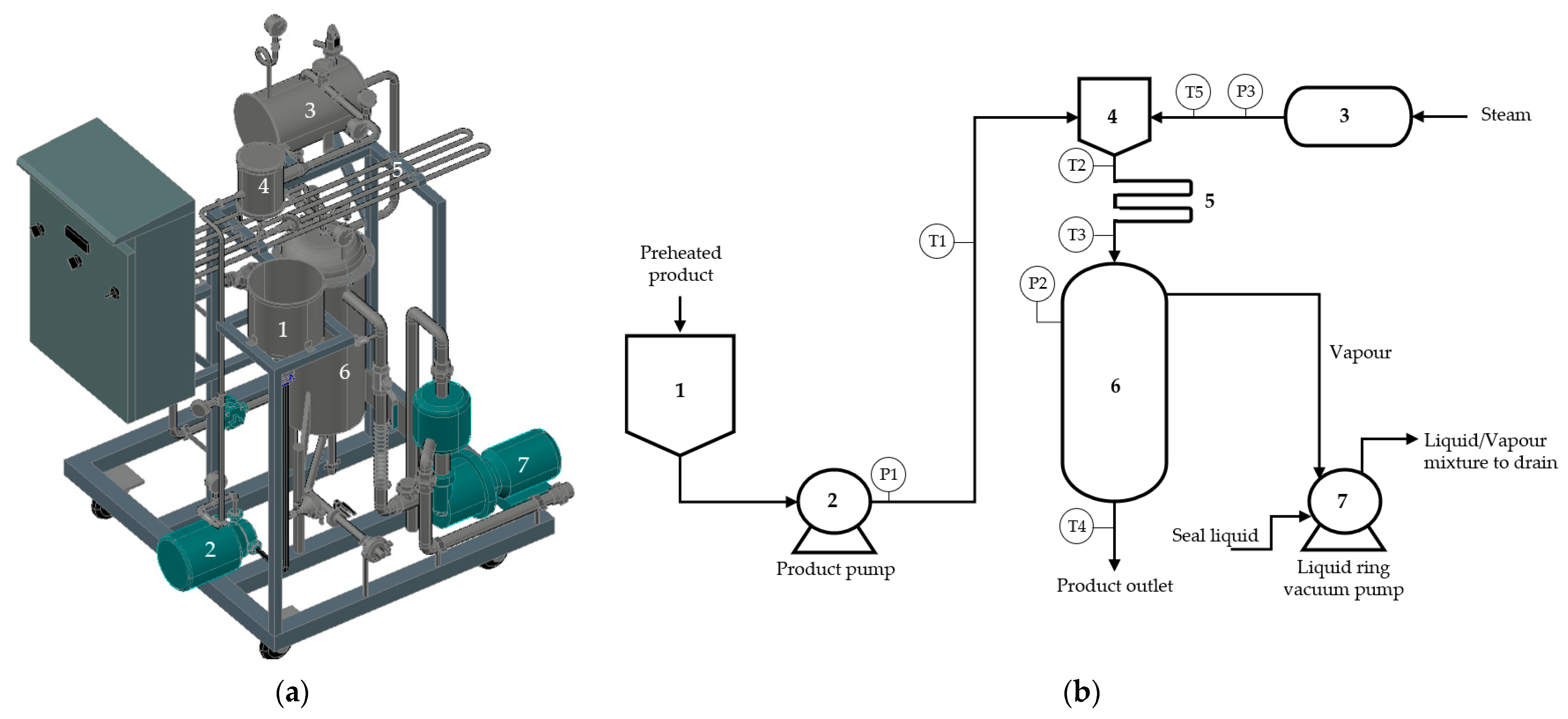
2.1. Experimental Setup
2.2. Measurement Procedure
2.2.1. The Nusselt Number
2.2.2. Water Temperature
3. Results
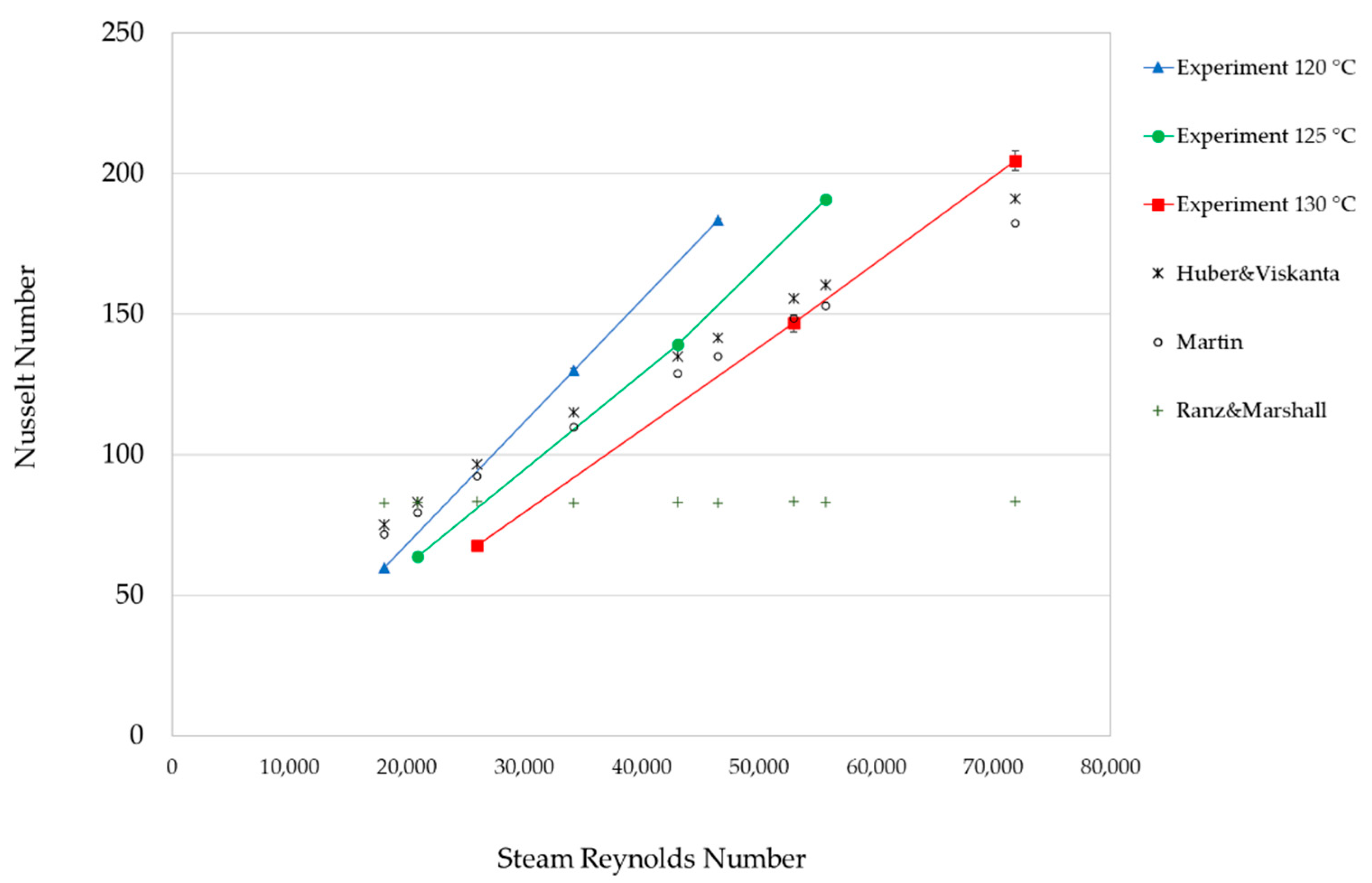
3.1. The Nusselt Number
3.1.1. Effect of Reynolds Number on Nusselt Number
3.1.2. Effect of Jet-to-Target Distance (H/d) on Nusselt Number
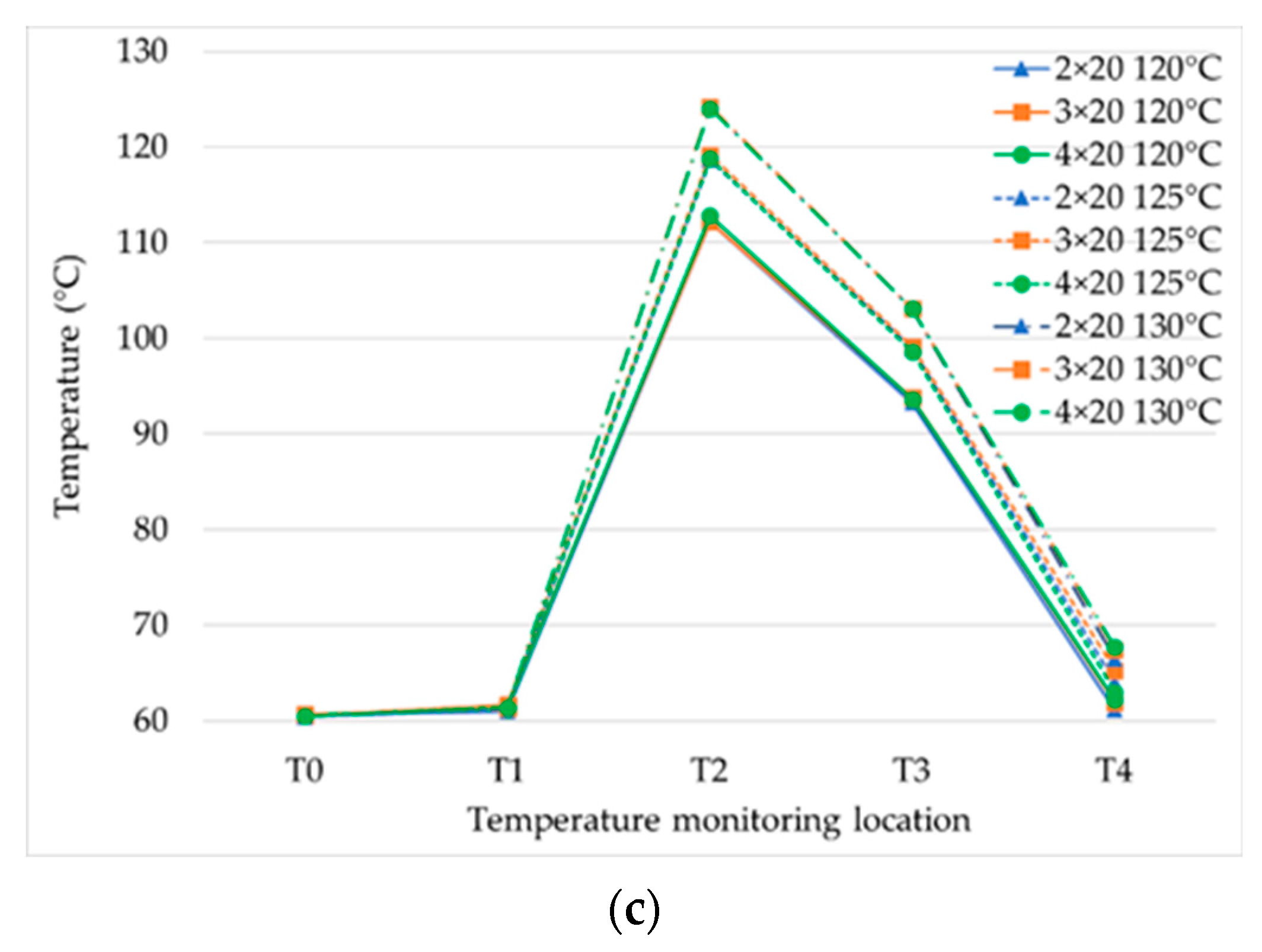
3.2. Water Temperature
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Parameters: | |
| A | target surface (m2) |
| Cp | specific heat (J/kg·°C) |
| d | nozzle diameter (m) |
| h | local heat transfer coefficient (W/m2·°C) |
| H | jet-to-target distance (m) |
| k | thermal conductivity (W/m·°C) |
| m | product flow rate (kg/h) |
| Nu | Nusselt number |
| Pr | Prandtl number |
| qw | heat flux supplied to the target surface (W/m2) |
| Re | Reynolds number |
| t | Jet-to-jet distance (m) |
| T | product temperature (°C) |
| Tj | jet temperature (°C) |
| Tw | target surface temperature (°C) |
| ρ | density (kg/m3) |
| μ | dynamic viscosity (kg/m·s) |
References
- Gut, J.A.; Pinto, J.M. Optimal Design of Continuous Sterilization Processes with Plate Heat Exchangers. Comput. Aided Chem. Eng. 2005, 20, 191–924. [Google Scholar]
- Doran, P.M. Bioprocess Engineering Principles, 2nd ed.; Elsevier: Amsterdam, The Netherlands, 2013; pp. 761–852. ISBN 978-0-12-220851-5. [Google Scholar]
- Jelen, P. Experience with Direct and Indirect UHT Processing of Milk—A Canadian Viewpoint. J. Food Prot. 1982, 45, 878–883. [Google Scholar] [CrossRef] [PubMed]
- Lewis, M.; Heppell, N. Continuous Thermal Processing of Foods Pasteurization and UHT Sterilization; Aspen Publishers, Inc.: Gaithersburg, MD, USA, 2000; pp. 57–103. ISBN 0-8342-1259-5. [Google Scholar]
- Emond, S. Continuous Heat Processing. In Thermal Technologies in Food Processing; Richardson, P., Ed.; Woodhead Publishing Limited: Cambridge, UK, 2001; pp. 29–48. ISBN 1-85573-558-X. [Google Scholar]
- Casoli, P.; Copelli, G. Modelling and Simulation of Direct Steam Injection for Tomato Concentrate Sterilization. In Proceedings of the International Conference on Modeling and Applied Simulation, Vienna, Austria, 19–21 September 2012. [Google Scholar]
- Shidara, H. Steriling Effect of Steam Injection UHT. In Proceedings of the Asian Pacific Confederation of Chemical Engineering Congress Program and Abstracts, The Society of Chemical Engineers, Tokyo, Japan, 17–21 October 2004. [Google Scholar]
- Geers, L.F.G. Multiple Impinging Jet Arrays: An Experimental Study on Flow and Heat Transfer. Ph.D. Thesis, Technical University Delft, Delft, The Netherlands, 9 February 2004. [Google Scholar]
- Wae-hayee, M.; Tekasakul, P.; Nuntadusit, C. Influence of Nozzle Arrangement on Flow and Heat Transfer Characteristics of Arrays of Circular Impinging Jets. Songklanakarin J. Sci. Technol. 2013, 35, 203–212. [Google Scholar]
- Penumadu, P.S.; Rao, A.G. Numerical Investigations of Heat Transfer and Pressure Drop Characteristics in Multiple Jet Impingement System. Appl. Therm. Eng. 2016, 110, 1511–1524. [Google Scholar] [CrossRef]
- Specht, E. Impinging Jet Drying. In Modern Drying Technology, 1st ed.; Tsotsas, E., Mujumdar, A.S., Eds.; Wiley-VCH GmbH: Weinheim, Germany, 2014; pp. 1–25. ISBN 978-3-527-31560-4. [Google Scholar]
- Wen, X.; Li, Z.; Zhou, W.; Liu, Y. Interaction of Dual Sweeping Impinging Jets at Different Reynolds Numbers. Phys. Fluids 2018, 30, 105105. [Google Scholar] [CrossRef] [Green Version]
- Molana, M.; Banooni, S. Investigation of Heat Transfer Processes Involved Liquid Impingement Jets: A Review. Braz. J. Chem. Eng. 2013, 30, 413–435. [Google Scholar] [CrossRef] [Green Version]
- Xu, Q.; Liu, W.; Li, W.; Yao, T.; Chu, X.; Guo, L. Experimental Investigation on Interfacial Behavior and Its Associated Pressure Oscillation in Steam Jet Condensation in Subcooled Water Flow. Int. J. Heat Mass Transf. 2019, 145, 118779. [Google Scholar] [CrossRef]
- Yamane, Y.; Ichikawa, Y.; Yamamoto, M.; Honami, S. Effect of Injection Parameters on Jet Array Impingement Heat Transfer. Int. J. Gas Turbine Propuls. Power Syst. 2012, 4, 27–34. [Google Scholar] [CrossRef]
- Rahimi-Gorji, M.; Debbaut, C.; Ghorbaniasl, G.; Cosyns, S.; Willaert, W.; Ceelen, W. Optimization of Intraperitoneal Aerosolized Drug Delivery Using Computational Fluid Dynamics (CFD) Modeling. Sci. Rep. 2022, 12, 6305. [Google Scholar] [CrossRef] [PubMed]
- Huber, A.M.; Viskanta, R. Effect of jet-jet spacing on convective heat transfer to confined, impinging arrays of axisymmetric air jets. Int. J. Heat Mass Transf. 1994, 37, 2859–2869. [Google Scholar] [CrossRef]
- Martin, H. Heat and Mass Transfer between Impinging Gas Jets and Solid Surfaces. Adv. Heat Transf. 1977, 13, 1–60. [Google Scholar]
- Attalla, M.A.M. Experimental Investigation of Heat Transfer Characteristics from Arrays of Free Impinging Circular Jets and Hole Channels. Master’s Thesis, Otto von Guericke University Magdeburg, Magdeburg, Germany, 21 December 2005. [Google Scholar]
- Garimella, S.V.; Schroeder, V.P. Local Heat Transfer Distributions in Confined Multiple Air Jet Impingement. J. Electron. Packag. 2001, 123, 165–172. [Google Scholar] [CrossRef] [Green Version]
- Sangsom, W.; Inprasit, C. Design and Development of Innovative Steam Injection for High-Temperature Short-Time Liquid Foods. Processes 2022, 10, 161. [Google Scholar] [CrossRef]
- Singh, R.P.; Heldman, D.R. Introduction to Food Engineering, 2nd ed.; Academic Press, Inc.: Cambridge, MA, USA, 1993; pp. 95–112. ISBN 0-12-646381-6. [Google Scholar]
- Ostermann, R.A. Direct Steam Injection Heating of Liquid Food Products. Master’s Thesis, Oklahoma State University, Stillwater, OK, USA, December 2005. [Google Scholar]
- O’Donovan, T.S. Fluid Flow and Heat Transfer of an Impinging Air Jet. Ph.D. Thesis, University of Dublin, Dublin, Ireland, March 2005. [Google Scholar]
- Gao, L. Effect of Jet Hole Arrays Arrangement on Impingement Heat Transfer. Master’s Thesis, Louisiana State University and Agricultural and Mechanical College, Baton Rouge, LA, USA, May 2003. [Google Scholar]
- Ekkad, S.V.; Gao, L.; Hebert, R.T. Effect of Jet-to-Jet Spacing in Impingement Arrays on Heat Transfer. In Proceedings of the IMECE2002 ASME International Mechanical Engineering Congress & Exposition, New Orleans, LA, USA, 17–22 November 2002. [Google Scholar]



| Fluid Impingement | Pressure Bar | Density (ρ) kg/m3 | Dynamic Viscosity (μ) kg/m·s |
|---|---|---|---|
| Water at 60 °C | 1.72 ± 0.04 | 983 | 4.66 × 10−4 |
| Steam at 120 °C | 0.66 ± 0.06 | 1.22 | 1.30 × 10−5 |
| Steam at 125 °C | 1.09 ± 0.05 | 1.30 | 1.31 × 10−5 |
| Steam at 130 °C | 1.58 ± 0.03 | 1.50 | 1.33 × 10−5 |
| Steam Temperature = 120 °C | Steam Temperature = 125 °C | Steam Temperature = 130 °C | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Number of Steam Nozzles | 6 | 9 | 20 | 6 | 9 | 20 | 6 | 9 | 20 |
| Re = 46,543 | Re = 34,228 | Re = 18,098 | Re = 55,721 | Re = 43,123 | Re = 20,932 | Re = 71,865 | Re = 52,984 | Re = 26,009 | |
| 2 water nozzles Re = 9476 | 183.38 ± 0.58 c | 129.95 ± 0.66 b | 59.67 ± 0.19 a | 190.68 ± 0.92 b | 139.11 ± 1.13 b | 63.67 ± 1.25 a | 204.54 ± 3.35 b | 146.73 ± 3.05 b | 67.68 ± 0.54 a |
| 3 water nozzles Re = 6317 | 175.94 ± 0.38 a | 135.34 ± 1.35 c | 60.74 ± 0.82 a | 183.79 ± 0.31 a | 139.26 ± 1.92 b | 63.65 ± 0.45 a | 199.94 ± 0.82 a | 139.88 ± 0.22 a | 68.05 ± 0.50 a |
| 4 water nozzles Re = 4738 | 179.67 ± 0.99 b | 123.66 ± 0.45 a | 60.30 ± 0.50 a | 190.54 ± 0.57 b | 130.82 ± 0.85 a | 63.50 ± 0.76 a | 200.14 ± 1.12 a | 141.83 ± 0.97 a | 68.16 ± 0.76 a |
| The Correlations | c1 | m | R2 |
|---|---|---|---|
| Experiment at 120 °C | 0.0005 | 1.1936 | 0.9993 |
| Experiment at 125 °C | 0.0010 | 1.1119 | 0.9987 |
| Experiment at 130 °C | 0.0011 | 1.0880 | 1.0000 |
| Huber and Viskanta | 0.1026 | 0.6731 | 0.9999 |
| Martin | 0.9770 | 0.6733 | 0.9998 |
| The Correlations | c2 | n | R2 |
|---|---|---|---|
| Experiment at 120 °C | 188.03 | −0.033 | 0.6448 |
| Huber and Viskanta | 171.57 | −0.174 | 0.9952 |
| Martin | 178.13 | −0.276 | 0.8940 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sangsom, W.; Inprasit, C. Design Parameters on Impingement Steam Jet Heat Transfer of Continuous Liquid Food Sterilization. Fluids 2022, 7, 185. https://doi.org/10.3390/fluids7060185
Sangsom W, Inprasit C. Design Parameters on Impingement Steam Jet Heat Transfer of Continuous Liquid Food Sterilization. Fluids. 2022; 7(6):185. https://doi.org/10.3390/fluids7060185
Chicago/Turabian StyleSangsom, Wilasinee, and Chouw Inprasit. 2022. "Design Parameters on Impingement Steam Jet Heat Transfer of Continuous Liquid Food Sterilization" Fluids 7, no. 6: 185. https://doi.org/10.3390/fluids7060185
APA StyleSangsom, W., & Inprasit, C. (2022). Design Parameters on Impingement Steam Jet Heat Transfer of Continuous Liquid Food Sterilization. Fluids, 7(6), 185. https://doi.org/10.3390/fluids7060185





