Simulations of Graphene Oxide Dispersions as Discotic Nematic Liquid Crystals in Couette Flow Using Ericksen-Leslie (EL) Theory
Abstract
:1. Introduction
2. Methods
2.1. EL Theory
2.2. Simplified Equations of Couette Flow
2.3. Numerical Setup
3. Results and Discussions
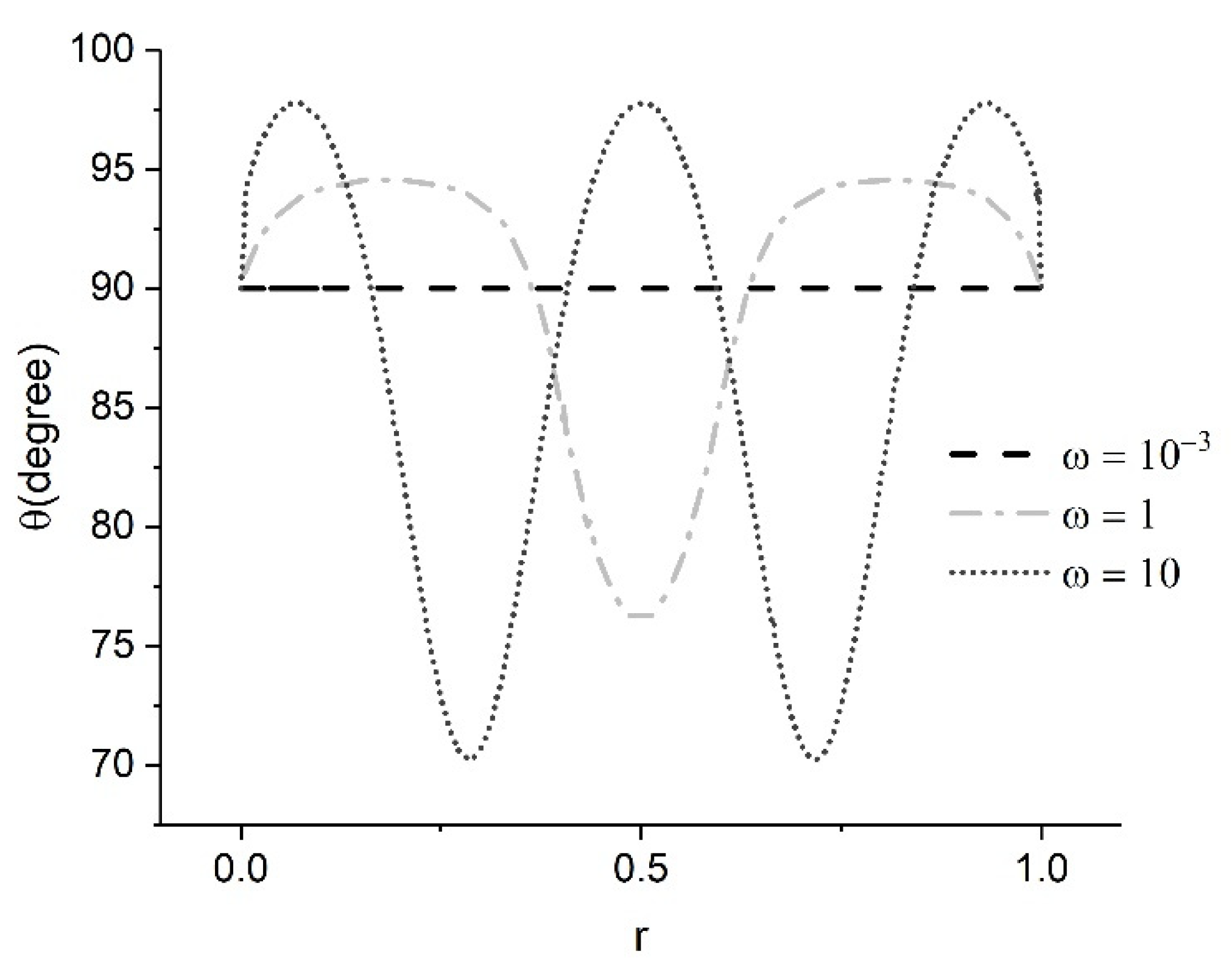
3.1. Orientation Profile of GO
3.2. Multi-Stability and Multiplicity of Solutions
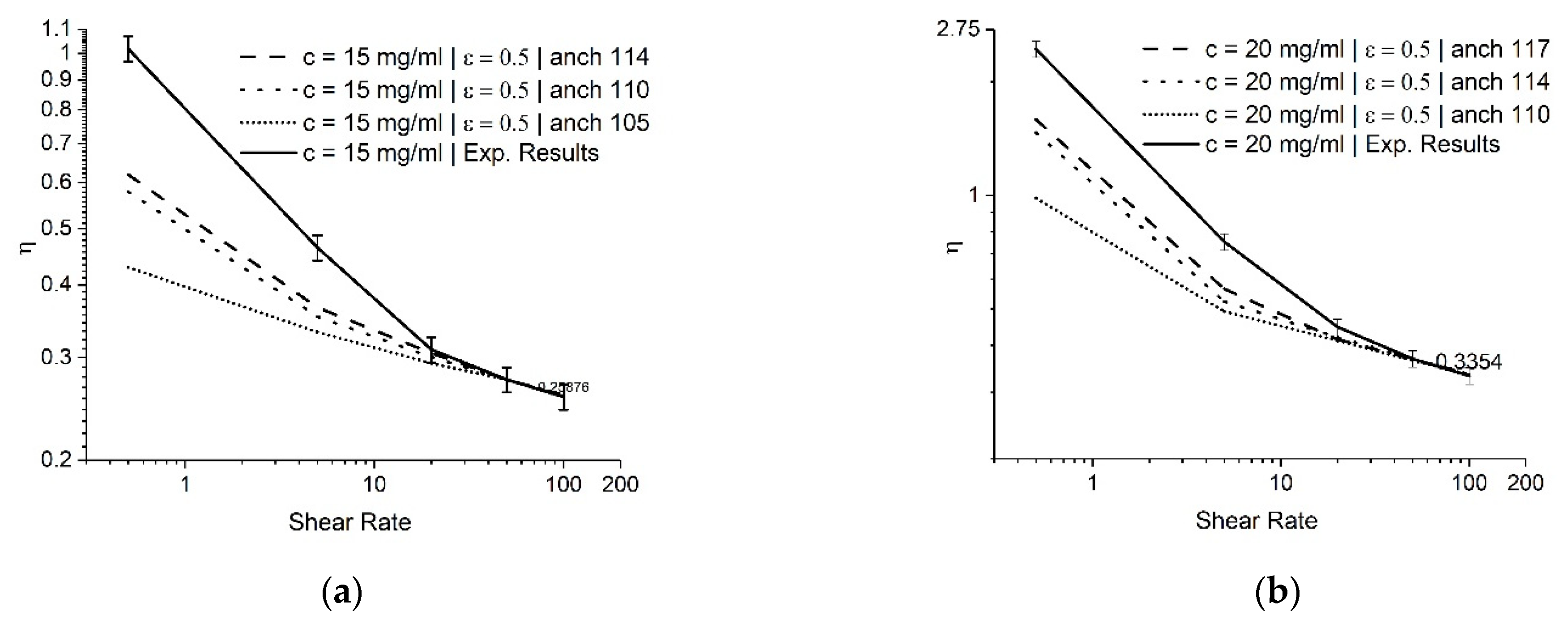
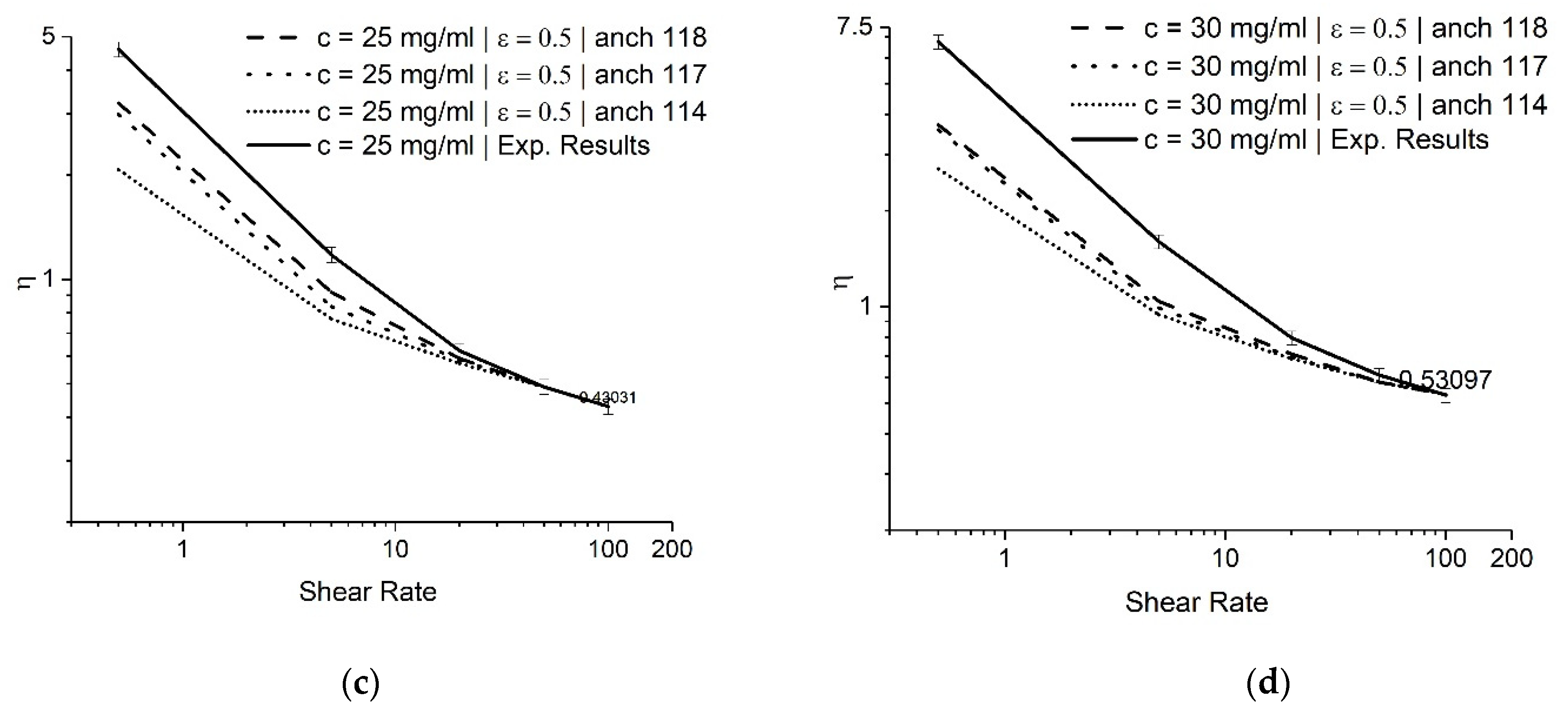
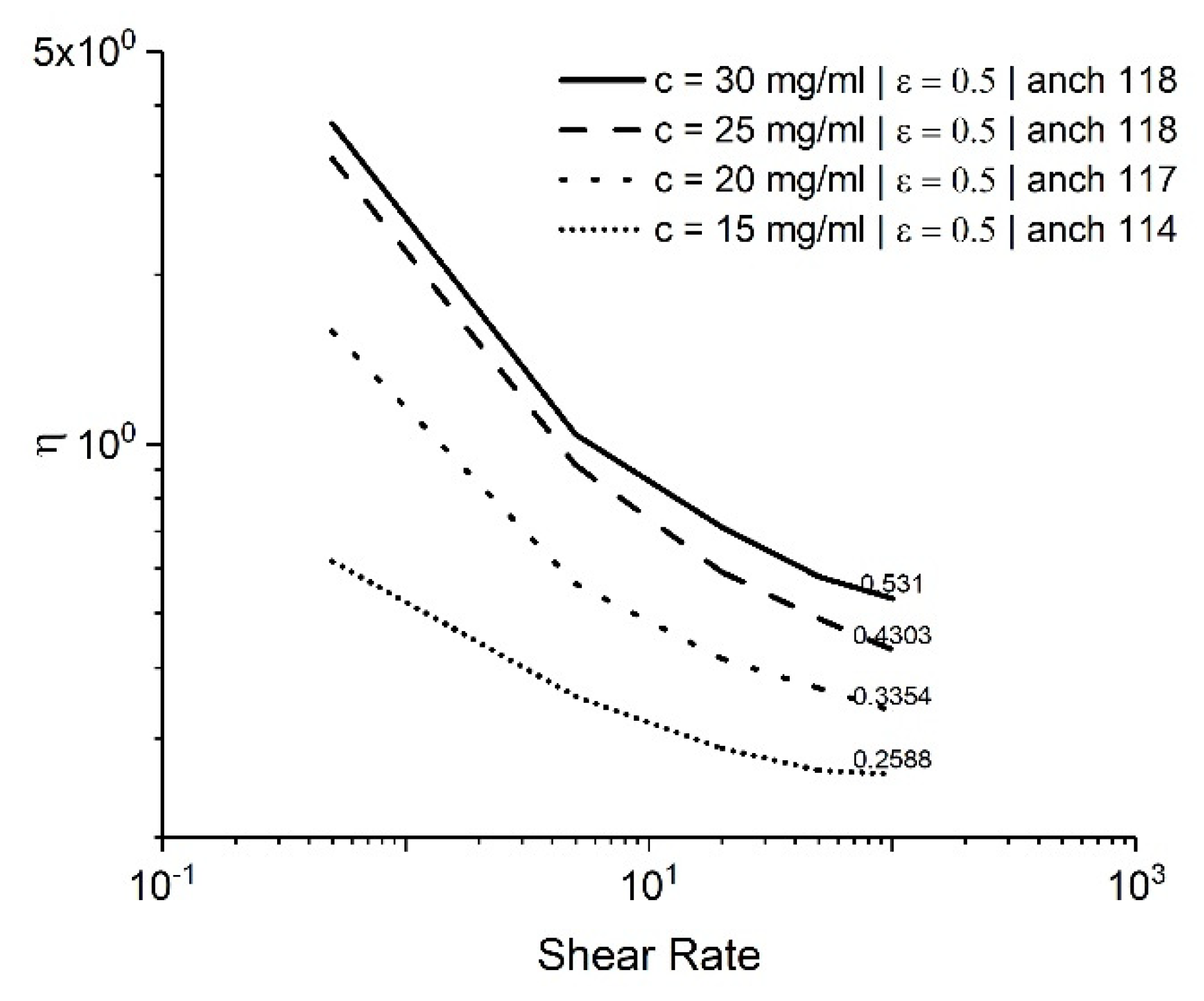
3.3. Viscosity Response of Graphene Oxide
3.4. Sensitivity Analysis of Frank’s Elasticity Coefficients
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Care, C.M.; Cleaver, D.J. Computer simulation of liquid crystals. Rep. Prog. Phys. 2005, 68, 2665–2700. [Google Scholar] [CrossRef]
- De Gennes, P.; Prost, J. The Physics of Liquid Crystals, 3rd ed.; Oxford University Press: Oxford, UK, 1996; ISBN 019851882X. [Google Scholar]
- Larson, R.G. The Structure and Rheology of Complex Fluids; Oxford University Press: Oxford, UK, 1999; ISBN 9780195121971. [Google Scholar]
- Chandrasekhar, S. Liquid Crystals; Cambridge University Press: Cambridge, UK, 1992; ISBN 9780521427418. [Google Scholar]
- Axenov, K.V.; Laschat, S. Thermotropic Ionic Liquid Crystals. Materials 2011, 4, 206–259. [Google Scholar] [CrossRef] [PubMed]
- Ferreira, G.A. Geometric features in lyotropic liquid crystalline phase transitions observed in aqueous surfactant systems. J. Dispers. Sci. Technol. 2021. [Google Scholar] [CrossRef]
- Nikzad, A.; Akbari, A.; Grecov, D. Rheological properties of discotic nematic liquid crystals: Graphene oxide dispersions study. Liq. Cryst. 2021, 48, 1685–1698. [Google Scholar] [CrossRef]
- Akbari, A.; Sheath, P.; Martin, S.T.; Shinde, D.B.; Shaibani, M.; Banerjee, P.C.; Tkacz, R.; Bhattacharyya, D.; Majumder, M. Large-area graphene-based nanofiltration membranes by shear alignment of discotic nematic liquid crystals of graphene oxide. Nat. Commun. 2016, 7, 10891. [Google Scholar] [CrossRef]
- Liu, B.; Wang, C.; Muhuo, Y. The effect of liquid crystalline graphene oxide compared with non-liquid crystalline graphene oxide on the rheological properties of polyacrylonitrile solution. High Temp. Mater. Process. 2021, 40, 428–438. [Google Scholar] [CrossRef]
- Mahalingam, D.K.; Wang, S.; Nunes, S.P. Graphene Oxide Liquid Crystal Membranes in Protic Ionic Liquid for Nanofiltration. ACS Appl. Nano Mater. 2018, 1, 4661–4670. [Google Scholar] [CrossRef] [Green Version]
- Shim, Y.H.; Ahn, H.; Lee, S.; Kim, S.O.; Kim, S.Y. Universal Alignment of Graphene Oxide in Suspensions and Fibers. ACS Nano 2021, 15, 13453–13462. [Google Scholar] [CrossRef]
- Kulyk, B.; Freitas, M.A.; Santos, N.F.; Mohseni, F.; Carvalho, A.F.; Yasakau, K.; Fernandes, A.J.S.; Bernardes, A.; Figueiredo, B.; Silva, R.; et al. A critical review on the production and application of graphene and graphene-based materials in anti-corrosion coatings. Crit. Rev. Solid State Mater. Sci. 2021. [Google Scholar] [CrossRef]
- Xu, Z.; Gao, C. Aqueous Liquid Crystals of Graphene Oxide. ACS Nano 2011, 5, 2908–2915. [Google Scholar] [CrossRef]
- Shao, Y.; Iliut, M.; Dierking, I.; Vijayaraghavan, A. Hybrid molecular/mineral lyotropic liquid crystal system of CTAB and graphene oxide in water. Carbon N. Y. 2021, 173, 105–114. [Google Scholar] [CrossRef]
- Abedin, M.J.; Gamot, T.D.; Martin, S.T.; Ali, M.; Hassan, K.I.; Mirshekarloo, M.S.; Tabor, R.F.; Green, M.J.; Majumder, M. Graphene Oxide Liquid Crystal Domains: Quantification and Role in Tailoring Viscoelastic Behavior. ACS Nano 2019, 13, 8957–8969. [Google Scholar] [CrossRef] [PubMed]
- Nam, K.-H.; Kim, U.J.; Jeon, M.H.; Lee, T.-R.; Yu, J.; You, N.-H.; Kim, Y.-K.; Suk, J.W.; Ku, B.-C. Green, fast, and scalable production of reduced graphene oxide via Taylor vortex flow. Chem. Eng. J. 2020, 391, 123482. [Google Scholar] [CrossRef]
- Alamer, M.; Lim, A.R.; Joo, Y.L. Continuous Synthesis of Structurally Uniform Graphene Oxide Materials in a Model Taylor–Couette Flow Reactor. Ind. Eng. Chem. Res. 2018, 58, 1167–1176. [Google Scholar] [CrossRef]
- Berman, D.; Erdemir, A.; Sumant, A.V. Reduced wear and friction enabled by graphene layers on sliding steel surfaces in dry nitrogen. Carbon N. Y. 2013, 59, 167–175. [Google Scholar] [CrossRef]
- Li, P.F.; Zhou, H.; Cheng, X. Investigation of a hydrothermal reduced graphene oxide nano coating on Ti substrate and its nano-tribological behavior. Surf. Coat. Technol. 2014, 254, 298–304. [Google Scholar] [CrossRef]
- Berman, D.; Erdemir, A.; Sumant, A.V. Few layer graphene to reduce wear and friction on sliding steel surfaces. Carbon N. Y. 2013, 54, 454–459. [Google Scholar] [CrossRef]
- Sarno, M.; Senatore, A.; Cirillo, C.; Petrone, V.; Ciambelli, P. Oil lubricant tribological behaviour improvement through dispersion of few layer graphene oxide. J. Nanosci. Nanotechnol. 2014, 14, 4960–4968. [Google Scholar] [CrossRef]
- Liu, Y.; Wang, X.; Pan, G.; Luo, J. A comparative study between graphene oxide and diamond nanoparticles as water-based lubricating additives. Sci. China Technol. Sci. 2013, 56, 152–157. [Google Scholar] [CrossRef]
- Su, F.; Chen, G.; Huang, P. Lubricating performances of graphene oxide and onion-like carbon as water-based lubricant additives for smooth and sand-blasted steel discs. Friction 2018, 8, 47–57. [Google Scholar] [CrossRef] [Green Version]
- Kinoshita, H.; Nishina, Y.; Alias, A.A.; Fujii, M. Tribological properties of monolayer graphene oxide sheets as water-based lubricant additives. Carbon N. Y. 2014, 66, 720–723. [Google Scholar] [CrossRef]
- Elomaa, O.; Singh, V.K.; Iyer, A.; Hakala, T.J.; Koskinen, J. Graphene oxide in water lubrication on diamond-like carbon vs. stainless steel high-load contacts. Diam. Relat. Mater. 2015, 52, 43–48. [Google Scholar] [CrossRef]
- Ericksen, J.L. Anisotropic fluids. Arch. Ration. Mech. Anal. 1959, 4, 231–237. [Google Scholar] [CrossRef]
- Frank, F.C. Liquid Crystals: On the theory of liquid crystals. Discuss. Faraday Soc. 1958, 25, 19–28. [Google Scholar] [CrossRef]
- Grecov, D.; Rey, A.D. Theoretical and Computational Rheology for Discotic Nematic Liquid Crystals. Mol. Cryst. Liq. Cryst. 2003, 391, 57–94. [Google Scholar] [CrossRef]
- Noroozi, N.; Grecov, D.; Shafiei-Sabet, S. Estimation of viscosity coefficients and rheological functions of nanocrystalline cellulose aqueous suspensions. Liq. Cryst. 2014, 41, 56–66. [Google Scholar] [CrossRef]
- Park, M.; Lee, H.S. Rotational motions of repulsive graphene oxide domains in aqueous dispersion during slow shear flow. J. Rheol. (N. Y.) 2019, 64, 29–41. [Google Scholar] [CrossRef]
- De Andrade Lima, L.R.P.; Rey, A.D. Poiseuille flow of Leslie-Ericksen discotic liquid crystal: Solution multiplicity, multistability, and non-Newtonian rheology. J. Non-Newton. Fluid Mech. 2003, 110, 103–142. [Google Scholar] [CrossRef]
- Atkin, R.J. Poiseuille flow of liquid crystals of the nematic type. Arch. Ration. Mech. Anal. 1970, 38, 224–240. [Google Scholar] [CrossRef]
- Ho, A.S.K.; Rey, A.D. Orienting properties of discotic nematic liquid crystals in Jeffrey-Hamel flows. Rheol. Acta 1991, 30, 77–88. [Google Scholar] [CrossRef]
- Chono, S.; Tsuji, T.; Denn, M.M. Spatial development of director orientation of tumbling nematic liquid crystals in pressure-driven channel flow. J. Nonnewton. Fluid Mech. 1998, 79, 515–527. [Google Scholar] [CrossRef]
- Cruz, P.A.; Tomé, M.F.; Stewart, I.W.; McKee, S. A numerical method for solving the dynamic three-dimensional Ericksen–Leslie equations for nematic liquid crystals subject to a strong magnetic field. J. Non-Newton. Fluid Mech. 2010, 165, 143–157. [Google Scholar] [CrossRef]
- Anderson, T.G.; Mema, E.; Kondic, L.; Cummings, L.J. Transitions in Poiseuille flow of nematic liquid crystal. Int. J. Non-Linear Mech. 2015, 75, 15–21. [Google Scholar] [CrossRef] [Green Version]
- Carou, J.Q.; Duffy, B.R.; Mottram, N.J.; Wilson, S.K. Shear-driven and pressure-driven flow of a nematic liquid crystal in a slowly varying channel. Phys. Fluids 2006, 18, 027105. [Google Scholar] [CrossRef] [Green Version]
- Choi, G.M.; Park, M.; Jeong, S.Y.; Lee, H.S. Orientation effect on the rheology of graphene oxide dispersions in isotropic phase, ordered isotropic biphase, and discotic phase. J. Rheol. (N. Y.) 2021, 65, 791–806. [Google Scholar] [CrossRef]
- Ericksen, J.L. Conservation laws for liquid crystals conservation laws for liquid crystals. Cit. Trans. Soc. Rheol. J. Chem. Phys. I Interfacial Free Energy J. Chem. Phys. J. Rheol. 1961, 5, 1205–1959. [Google Scholar] [CrossRef]
- Leslie, F.M. Some constitutive equations for liquid crystals. Arch. Ration. Mech. Anal. 1968, 28, 265–283. [Google Scholar] [CrossRef]
- Atkin, R.J.; Leslie, F.M. Couette flow of nematic liquid crystals. Q. J. Mech. Appl. Math. 1970, 23, 3–24. [Google Scholar] [CrossRef]
- Noroozi, N.; Grecov, D. Flow modelling and rheological characterization of nematic liquid crystals between concentric cylinders. Liq. Cryst. 2013, 40, 871–883. [Google Scholar] [CrossRef]
- Miesowicz, M. The three coefficients of viscosity of anisotropic liquids. Nature 1946, 158, 27. [Google Scholar] [CrossRef]
- Rey, A.D.; Tsuji, T. Recent advances in theoretical liquid crystal rheology. Macromol. Theory Simul. 1998, 7, 623–639. [Google Scholar] [CrossRef]
- de Kort, G.W.; Leoné, N.; Stellamanns, E.; Auhl, D.; Wilsens, C.H.R.M.; Rastogi, S. Effect of Shear Rate on the Orientation and Relaxation of a Vanillic Acid Based Liquid Crystalline Polymer. Polymers 2018, 10, 935. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dietrich, C.F.; Collings, P.J.; Sottmann, T.; Rudquist, P.; Giesselmann, F. Extremely small twist elastic constants in lyotropic nematic liquid crystals. Proc. Natl. Acad. Sci. USA 2020, 117, 27238–27244. [Google Scholar] [CrossRef] [PubMed]
- Del Giudice, F.; Cunning, B.V.; Ruoff, R.S.; Shen, A.Q. Filling the gap between transient and steady shear rheology of aqueous graphene oxide dispersions. Rheol. Acta 2018, 57, 293–306. [Google Scholar] [CrossRef]
- Del Giudice, F.; Shen, A.Q. Shear rheology of graphene oxide dispersions. Curr. Opin. Chem. Eng. 2017, 16, 23–30. [Google Scholar] [CrossRef]
- Adnan, A.M.; Lü, C.; Luo, X.; Wang, J. Impact of Graphene Oxide on Zero Shear Viscosity, Fatigue Life and Low-Temperature Properties of Asphalt Binder. Materials 2021, 14, 3073. [Google Scholar] [CrossRef]
- Tesfai, W.; Singh, P.; Shatilla, Y.; Iqbal, M.Z.; Abdala, A.A. Rheology and microstructure of dilute graphene oxide suspension. JNR J. Nanoparticle Res. 2013, 15, 1989. [Google Scholar] [CrossRef]
- Straley, J.P. Frank elastic constants of the hard-rod liquid crystal. Phys. Rev. A 1973, 8, 2181. [Google Scholar] [CrossRef]
- Bradshaw, M.J.; Raynes, E.P.; Bunning, J.D.; Faber, T.E. The Frank constants of some nematic liquid crystals. J. Phys. 1985, 46, 1513–1520. [Google Scholar] [CrossRef]
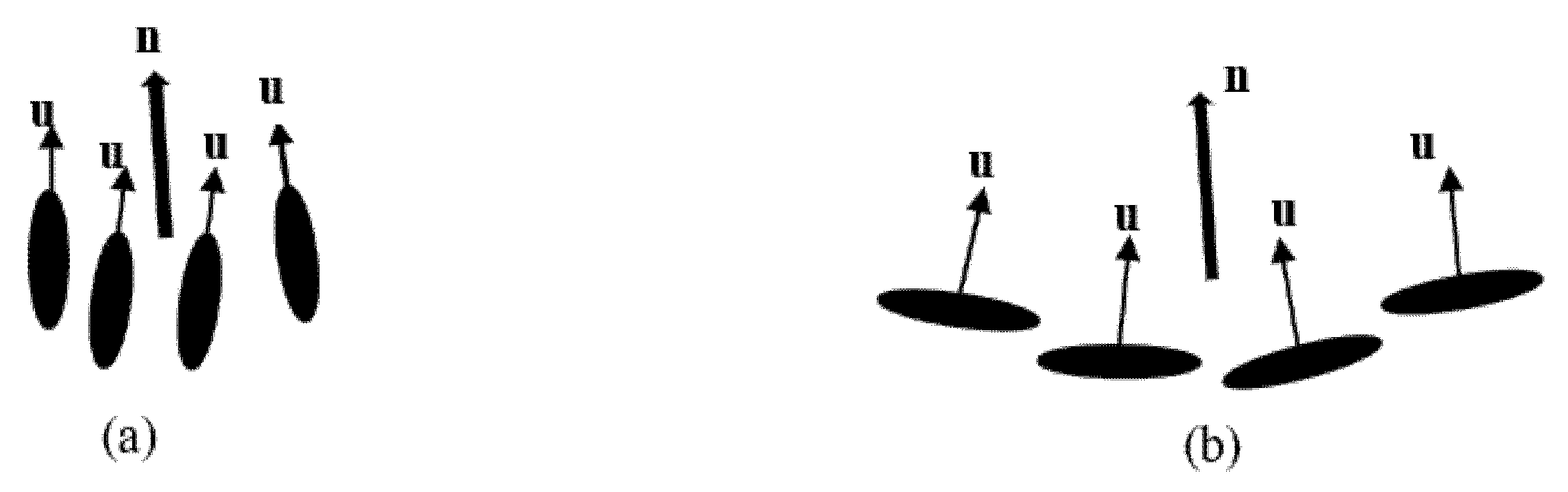


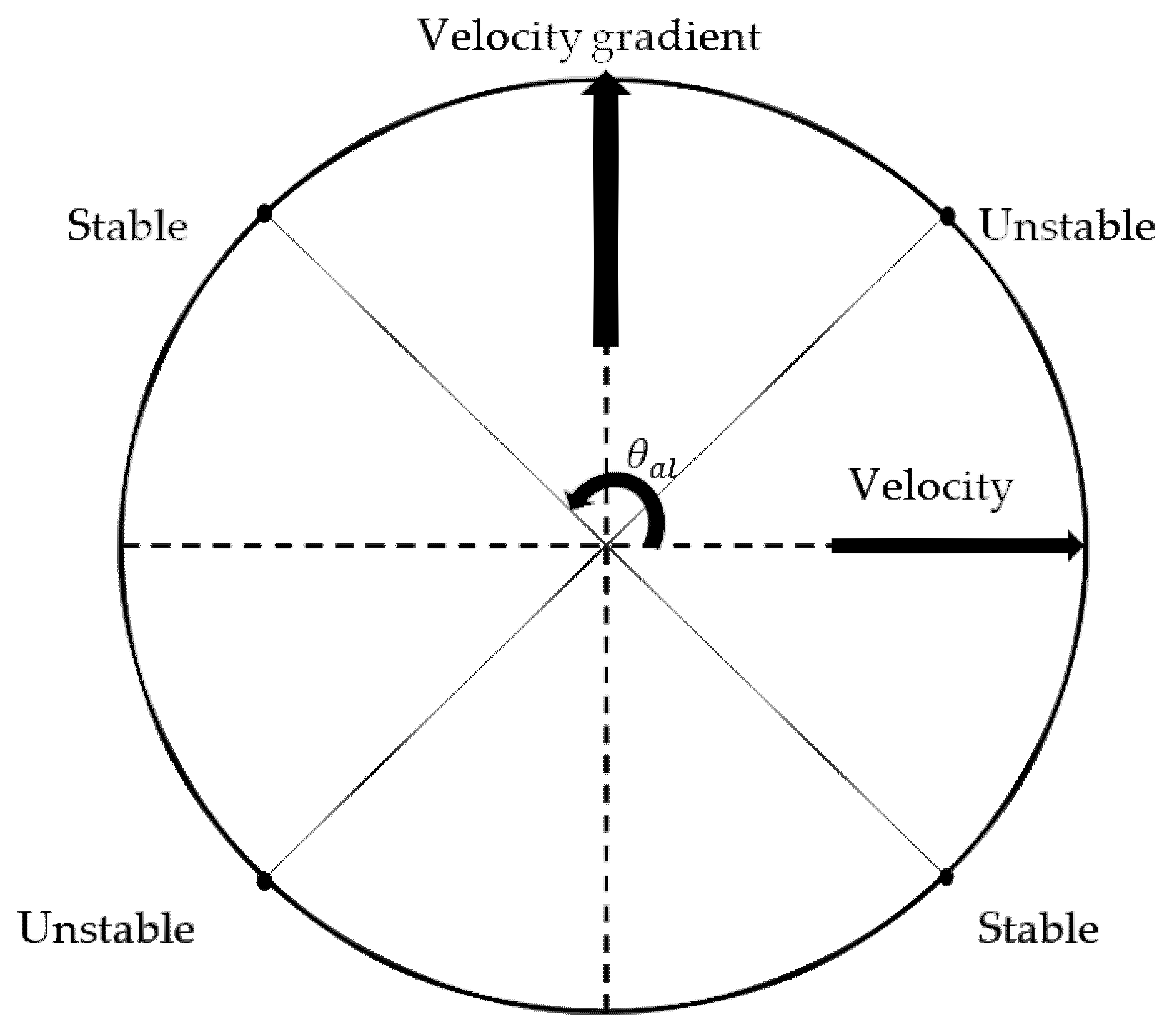

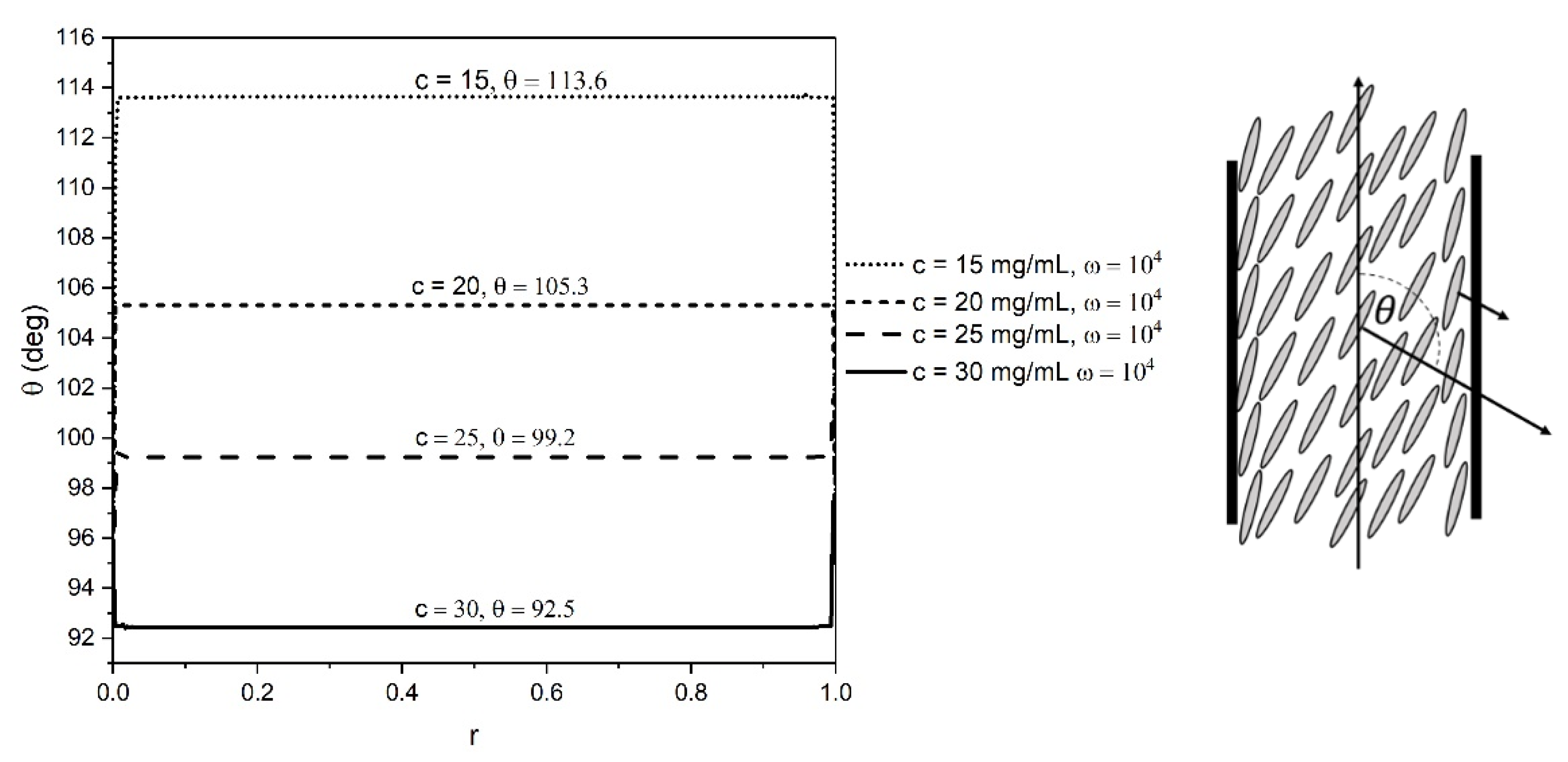
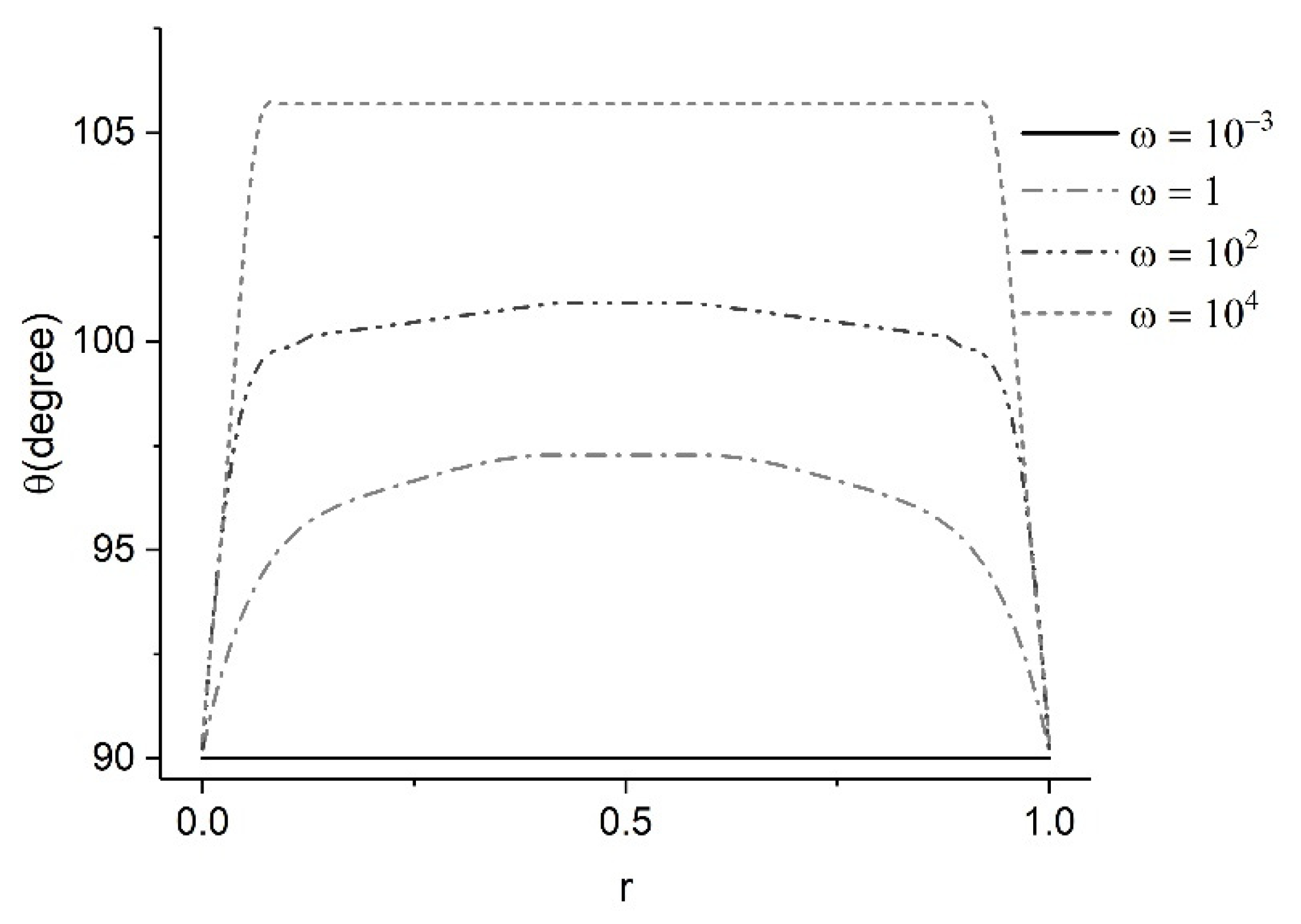


| Concentration (mg/mL) | ||||||
|---|---|---|---|---|---|---|
| 15 | −2.28 | 0.47 | 2.54 | 0.77 | −0.14 | 2.89 |
| 20 | −4.71 | 0.38 | 5.04 | 1.25 | −0.14 | 5.28 |
| 25 | −7.88 | 0.23 | 8.42 | 1.9 | −0.14 | 8.5 |
| 30 | −11.79 | 0.023 | 12.21 | 2.59 | −0.1437 | 12.09 |
| Concentration (mg/mL) | Simplified Theory (deg) | Numerical Results from the EL (deg) | EL Error (%) |
|---|---|---|---|
| 15 | 113.3 | 113.6 | 0.26% |
| 20 | 105.3 | 105.3 | 0.00% |
| 25 | 99.3 | 99.2 | −0.10% |
| 30 | 92.4 | 92.5 | 0.11% |
| Concentration (mg/mL) | Difference | ||
|---|---|---|---|
| 15 | 1.3737 | 1.3741 | 0.03% |
| 20 | 2.5599 | 2.5603 | 0.01% |
| 25 | 4.1659 | 4.1659 | 0.00% |
| 30 | 5.9493 | 5.9497 | 0.01% |
| Concentration (mg/mL) | Difference | ||
|---|---|---|---|
| 15 | 0.036416 | 0.036535 | 0.32% |
| 20 | 0.161637 | 0.161637 | 0.00% |
| 25 | 0.338584 | 0.336735 | −0.55% |
| 30 | 0.516128 | 0.5140956 | 0.90% |
| Concentration (mg/mL) | ||
|---|---|---|
| 15 | 0.2568 ± 0.0885 | 0.2588 |
| 20 | 0.3319 ± 0.0221 | 0.3354 |
| 25 | 0.4303 ± 0.0177 | 0.4303 |
| 30 | 0.5288 ± 0.0044 | 0.5310 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nikzad, A.; Bhatia, S.; Grecov, D. Simulations of Graphene Oxide Dispersions as Discotic Nematic Liquid Crystals in Couette Flow Using Ericksen-Leslie (EL) Theory. Fluids 2022, 7, 103. https://doi.org/10.3390/fluids7030103
Nikzad A, Bhatia S, Grecov D. Simulations of Graphene Oxide Dispersions as Discotic Nematic Liquid Crystals in Couette Flow Using Ericksen-Leslie (EL) Theory. Fluids. 2022; 7(3):103. https://doi.org/10.3390/fluids7030103
Chicago/Turabian StyleNikzad, Arash, Somesh Bhatia, and Dana Grecov. 2022. "Simulations of Graphene Oxide Dispersions as Discotic Nematic Liquid Crystals in Couette Flow Using Ericksen-Leslie (EL) Theory" Fluids 7, no. 3: 103. https://doi.org/10.3390/fluids7030103
APA StyleNikzad, A., Bhatia, S., & Grecov, D. (2022). Simulations of Graphene Oxide Dispersions as Discotic Nematic Liquid Crystals in Couette Flow Using Ericksen-Leslie (EL) Theory. Fluids, 7(3), 103. https://doi.org/10.3390/fluids7030103








