Computational Analysis of Actuation Techniques Impact on the Flow Control around the Ahmed Body
Abstract
:1. Introduction
2. Numerical Model
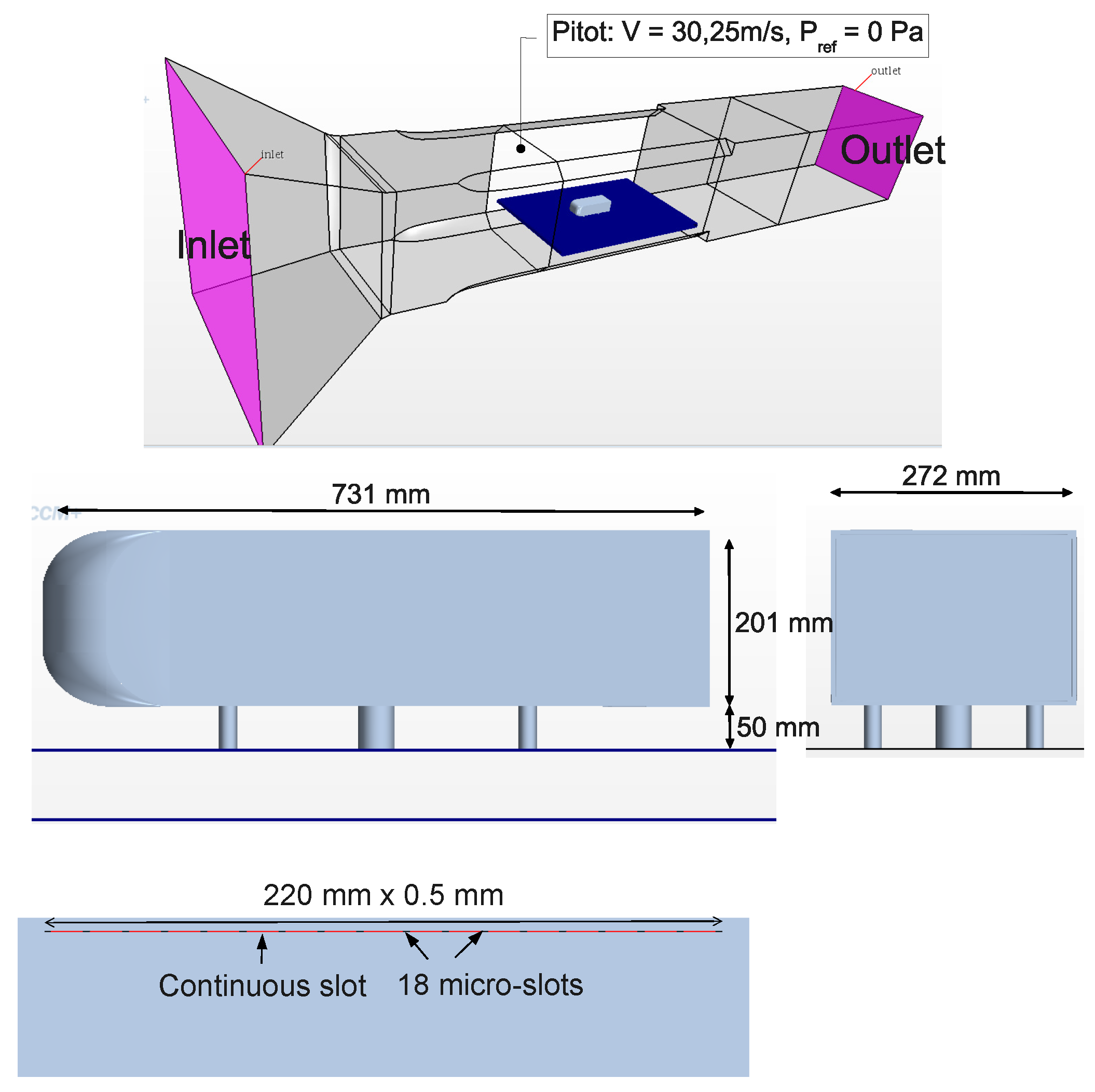
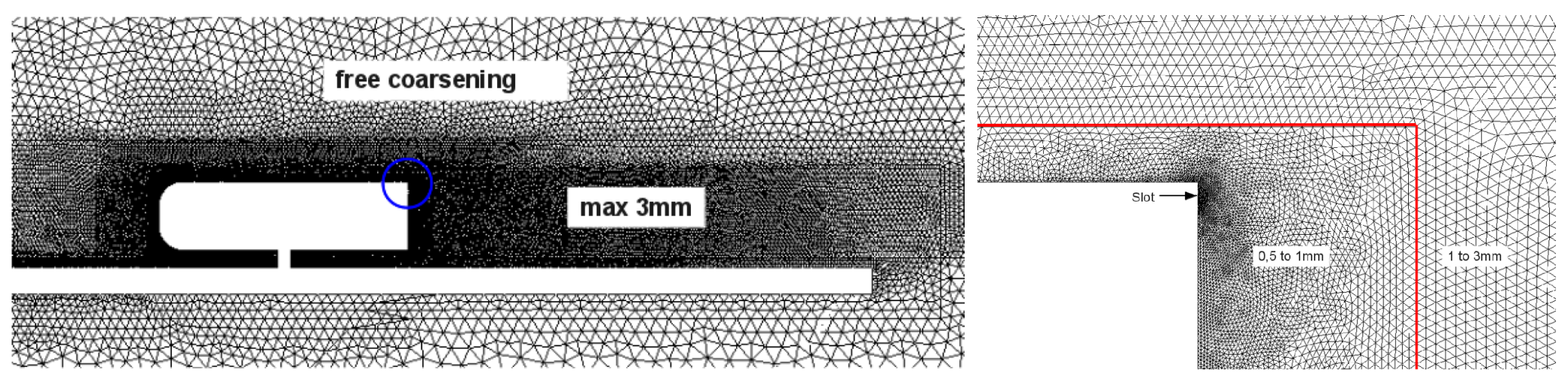
2.1. Computational Set-Up
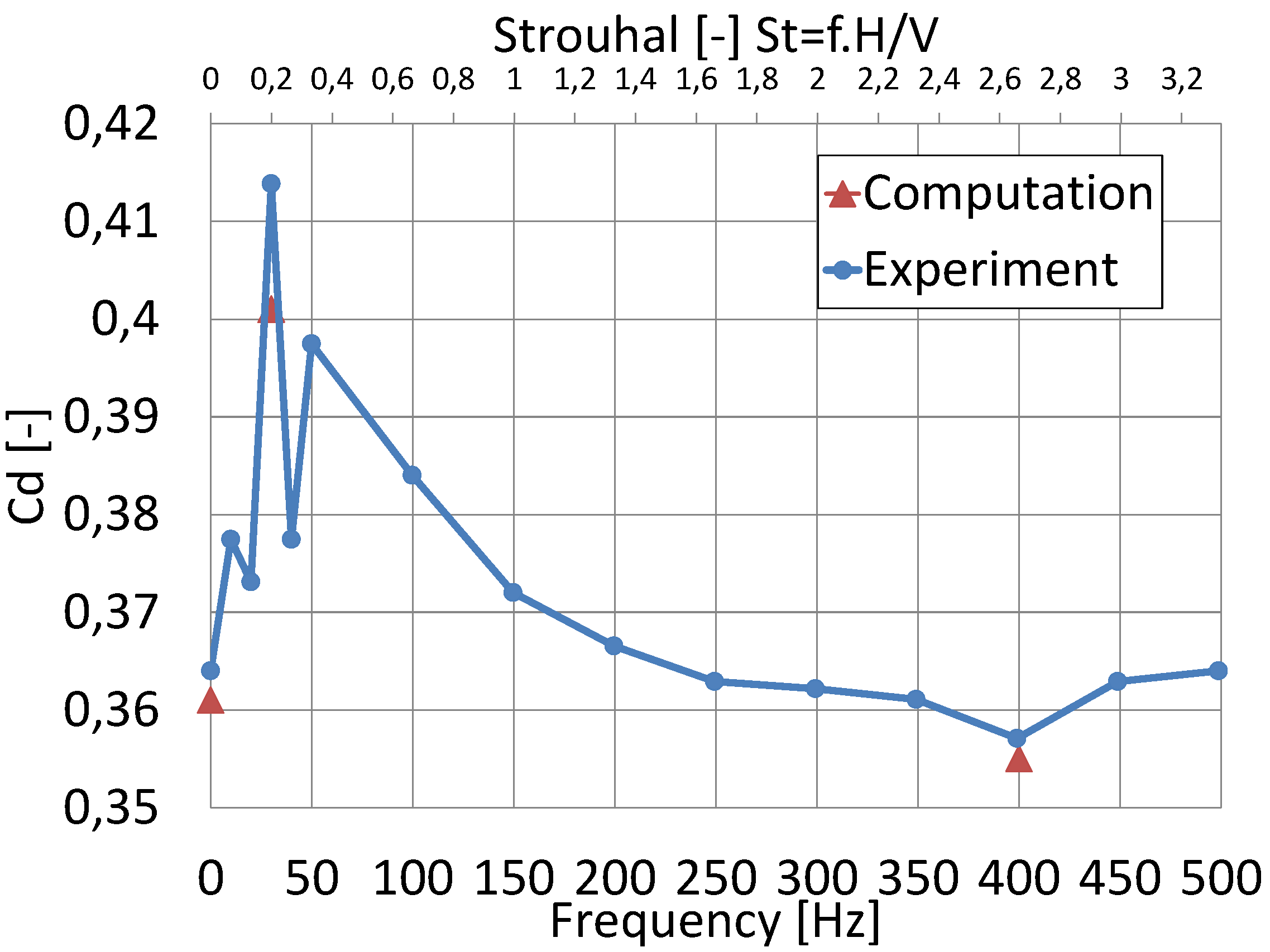
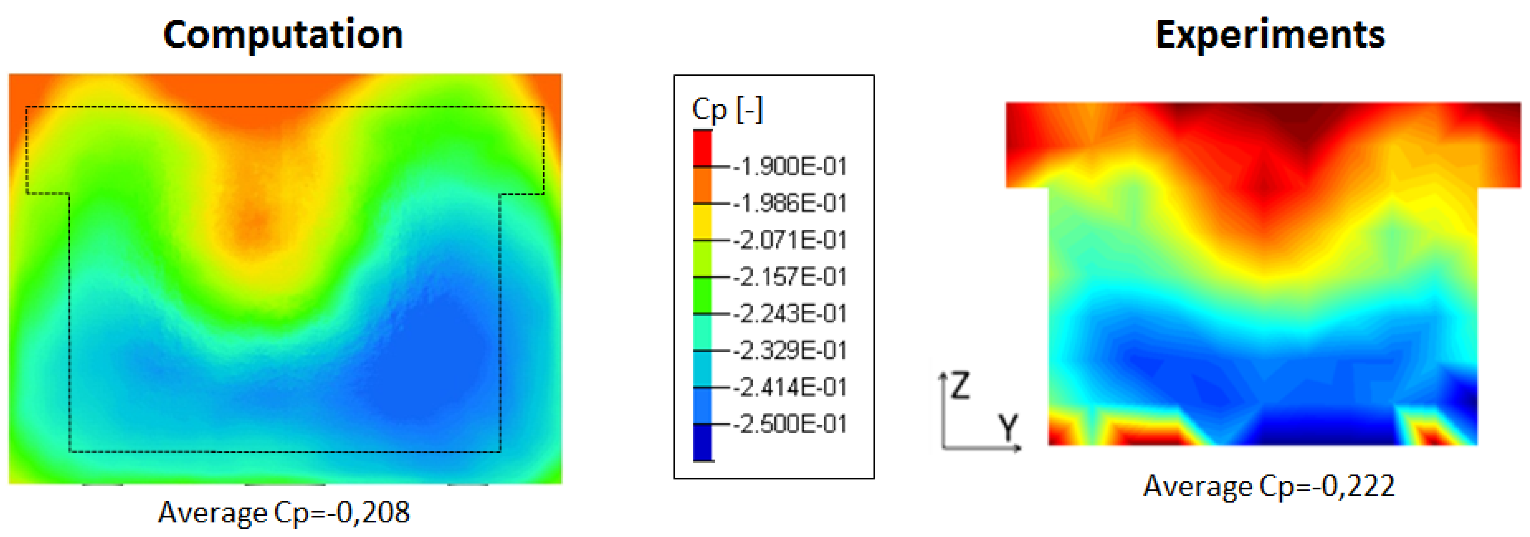
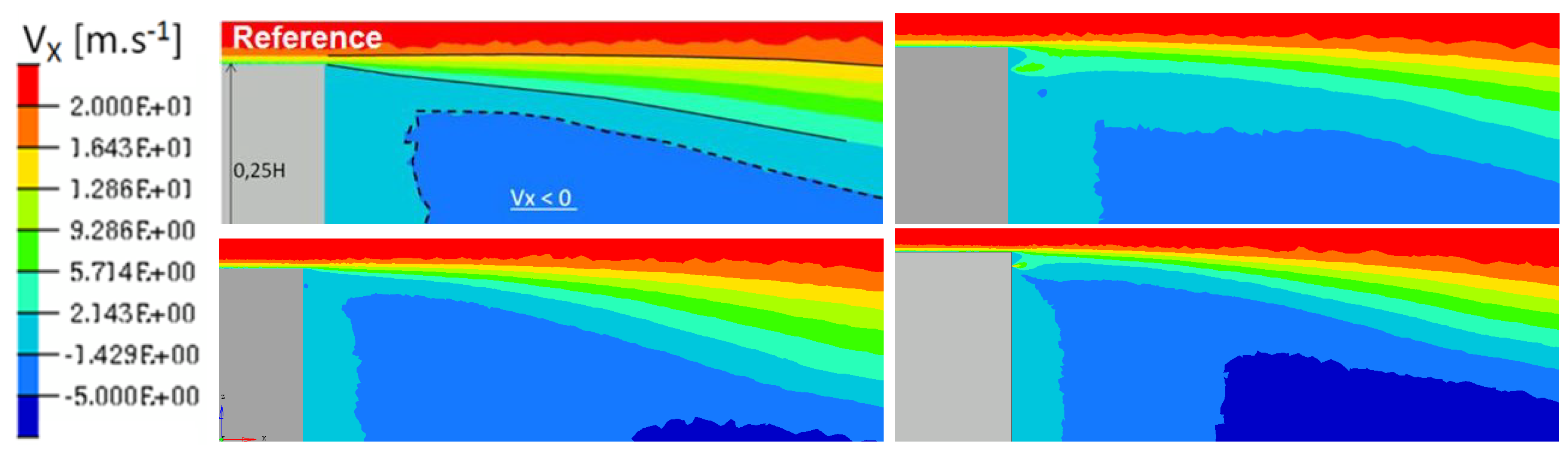
2.2. Numerical Results Validation with Experiments
3. Control Strategy Analysis
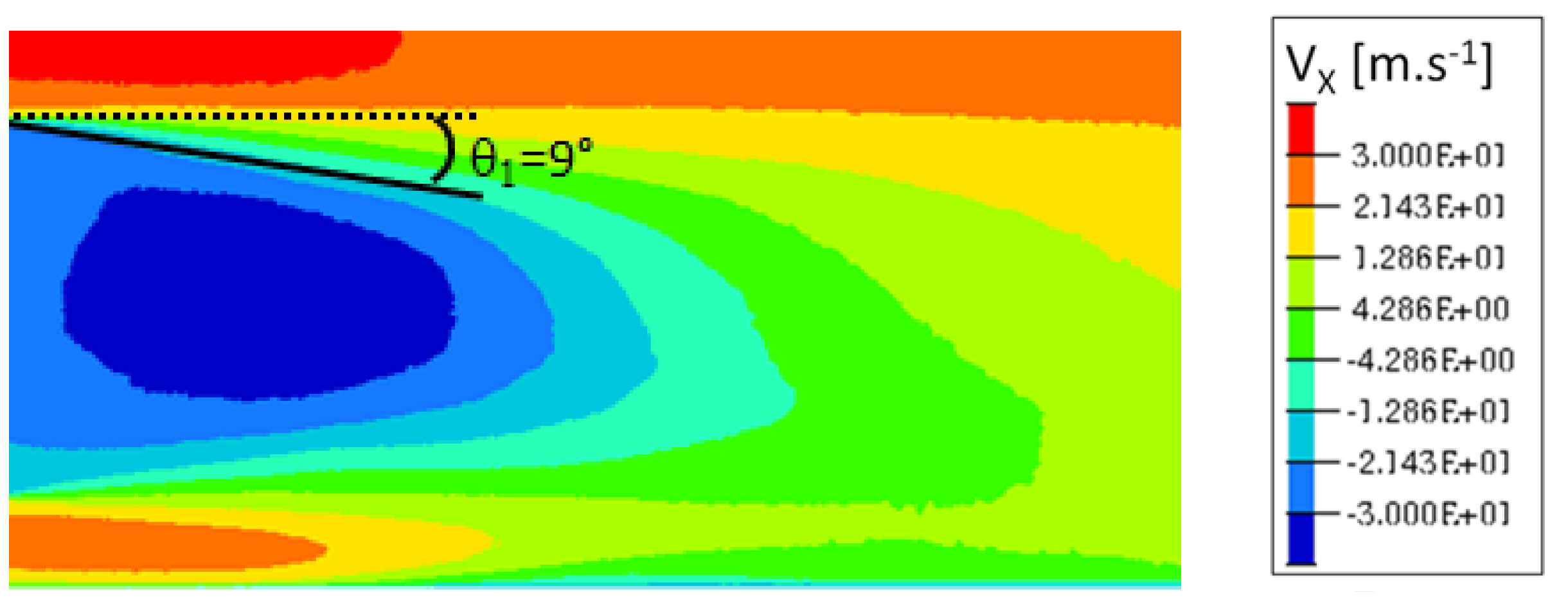
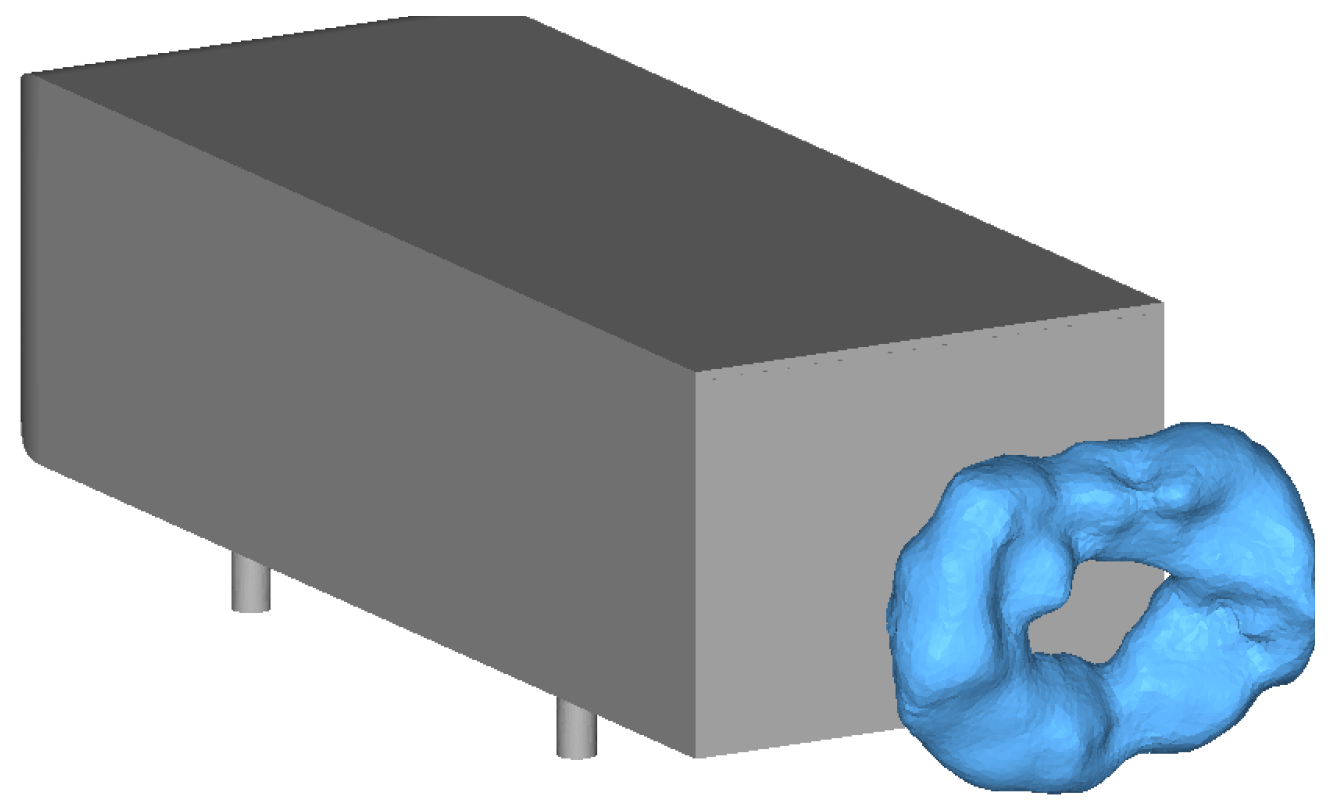
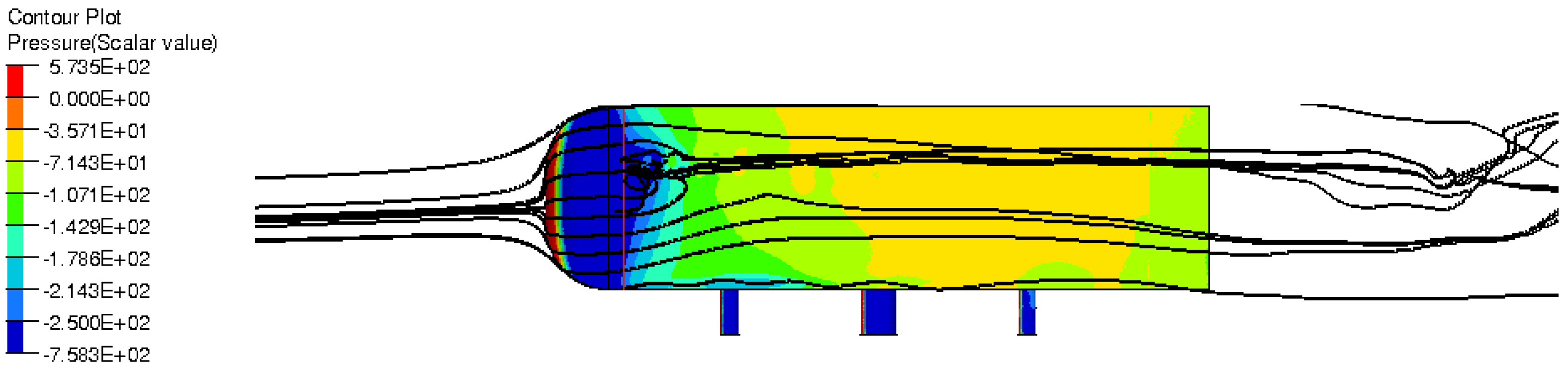
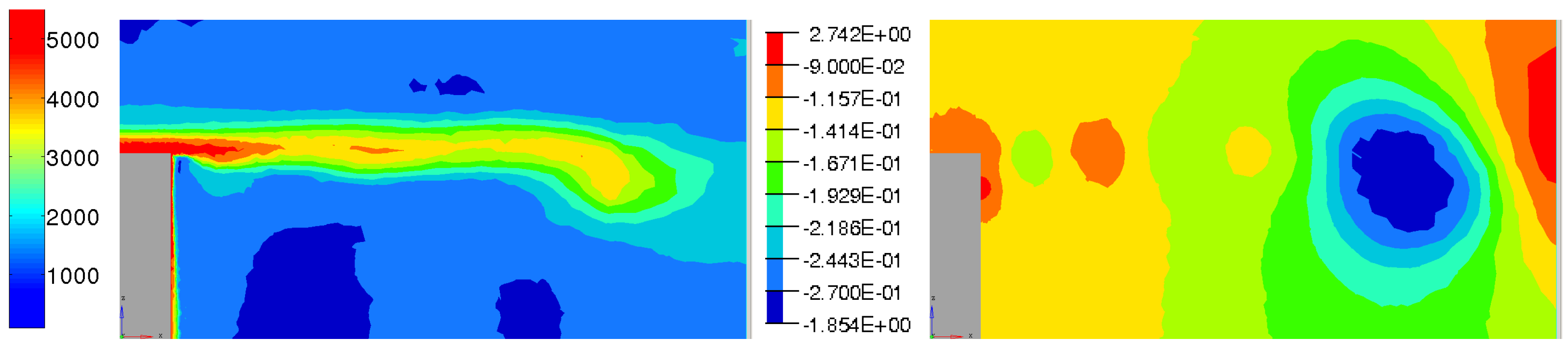
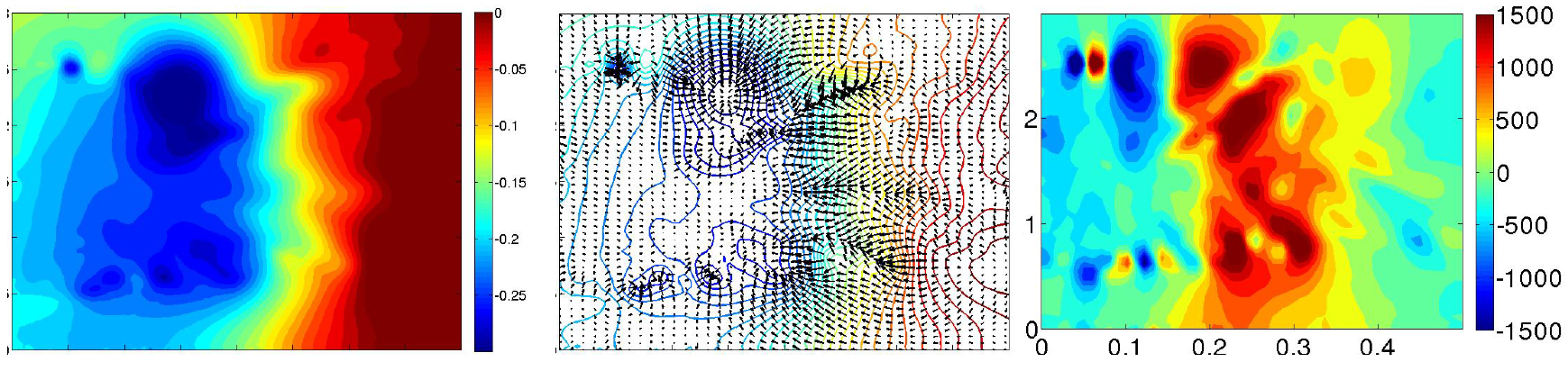
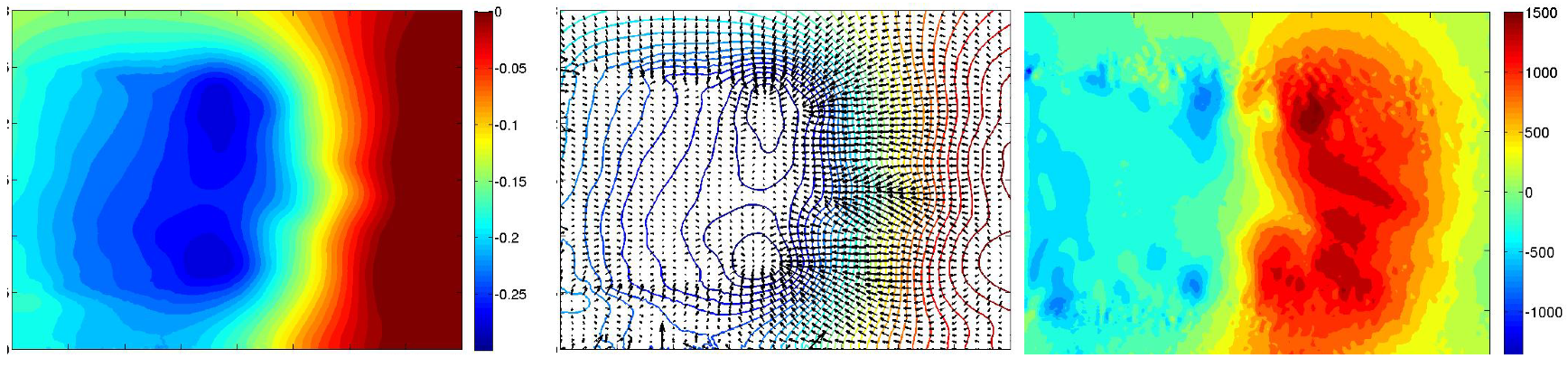
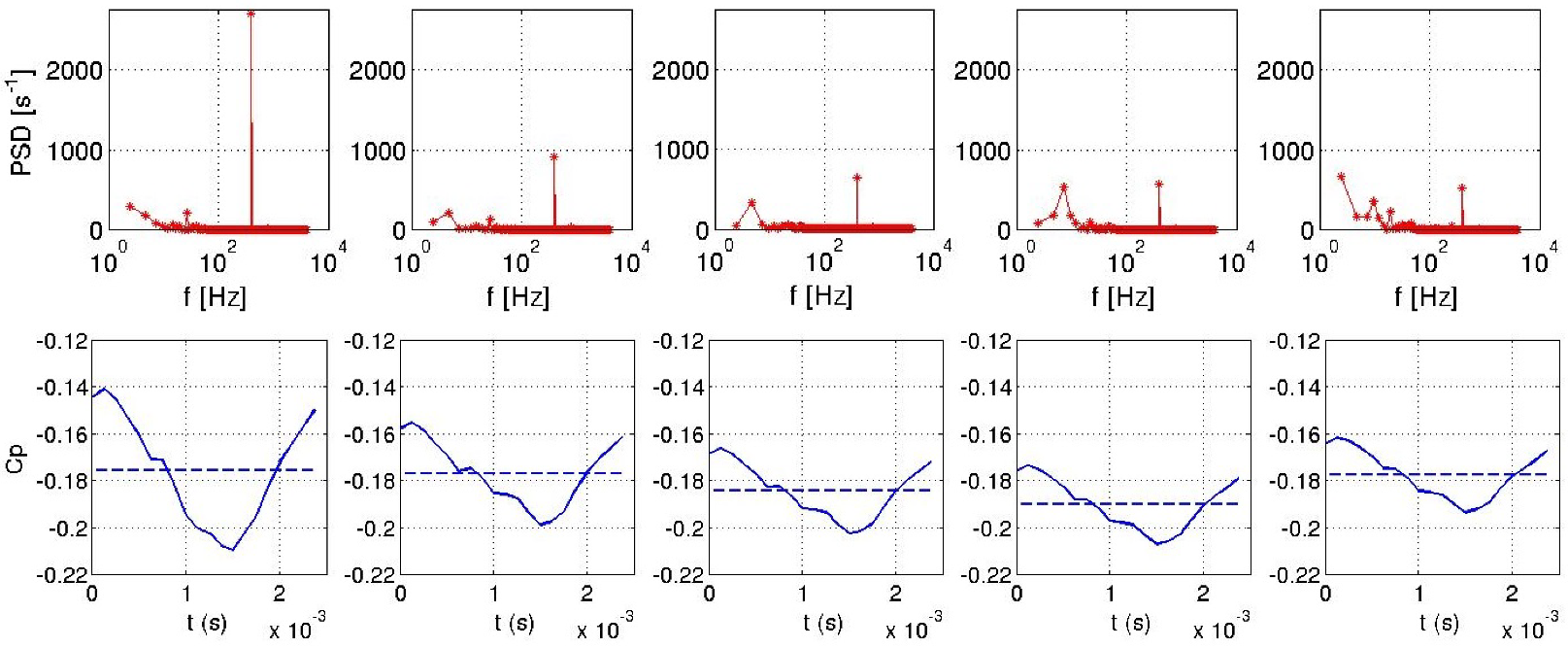
3.1. Description of the No-Controled Flow Topology
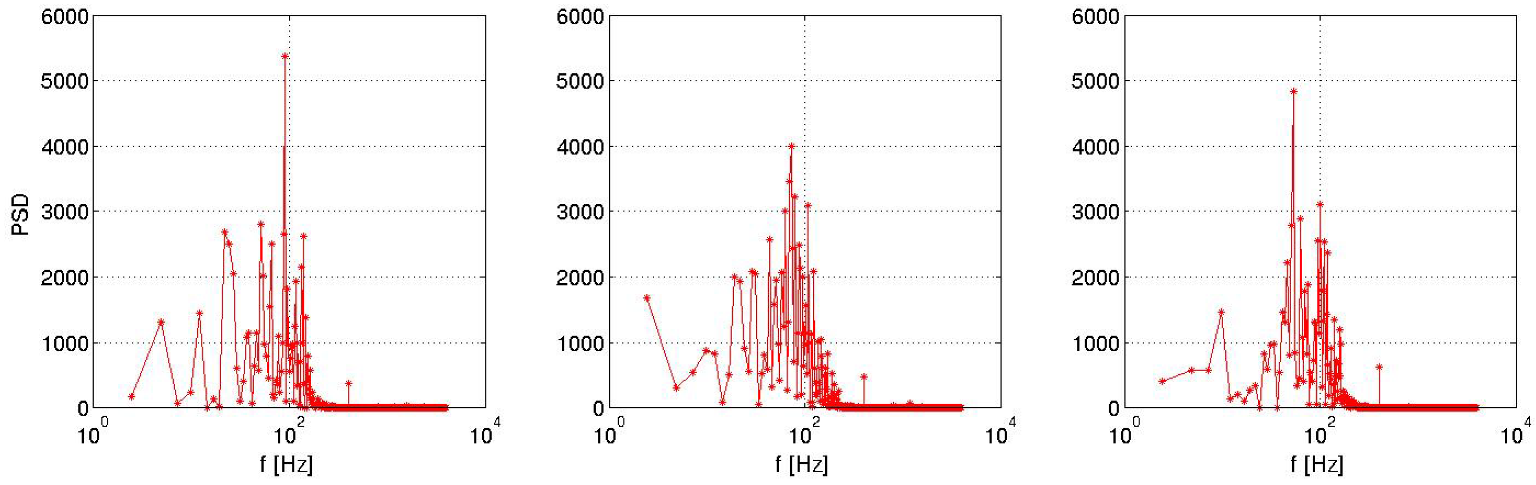
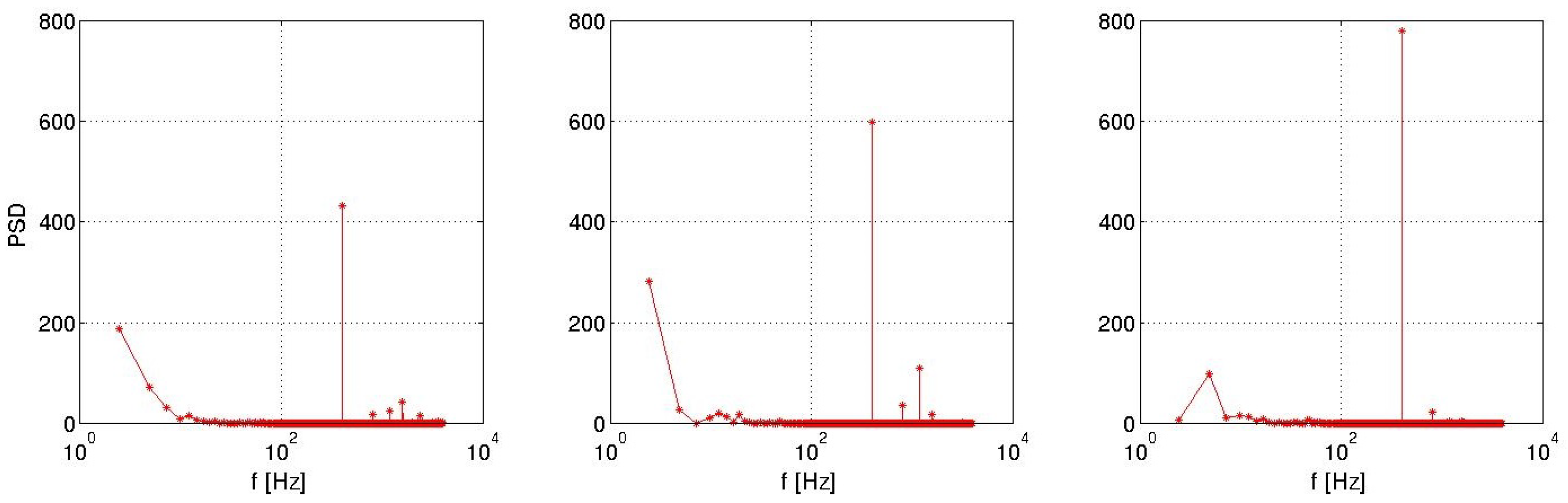
3.2. Design of Experiment and Sensitivity Analysis for Flow Control
- Jet angles: 90 degrees to 30 degrees from rear back wall;
- Velocity magnitude: 30 m/s to 150 m/s;
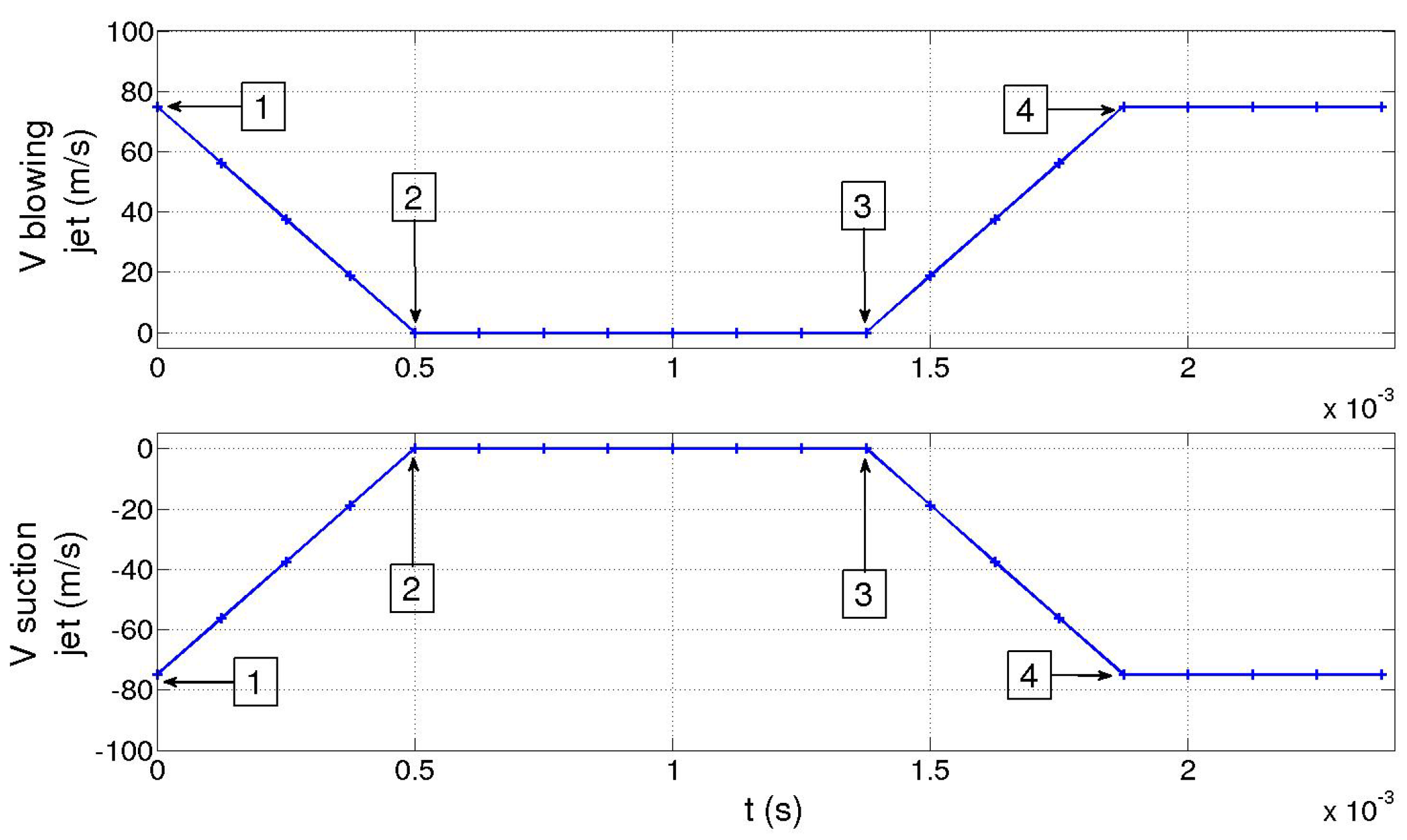
- Signal type: constant jet vs pulsed suction jet vs pulsed blowing jet vs synthetic jet;
- Actuator frequency: 30 to 750 Hz.
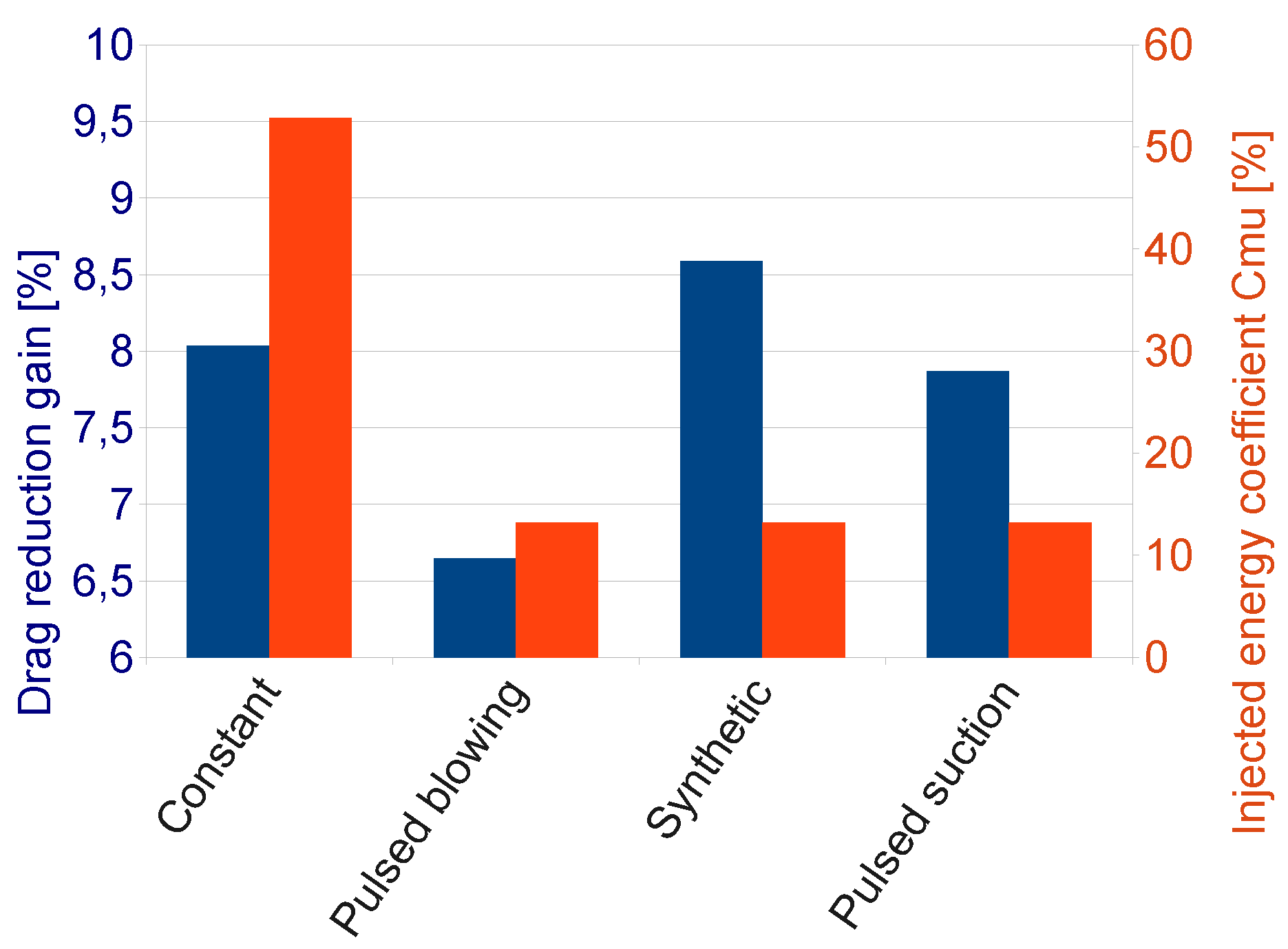
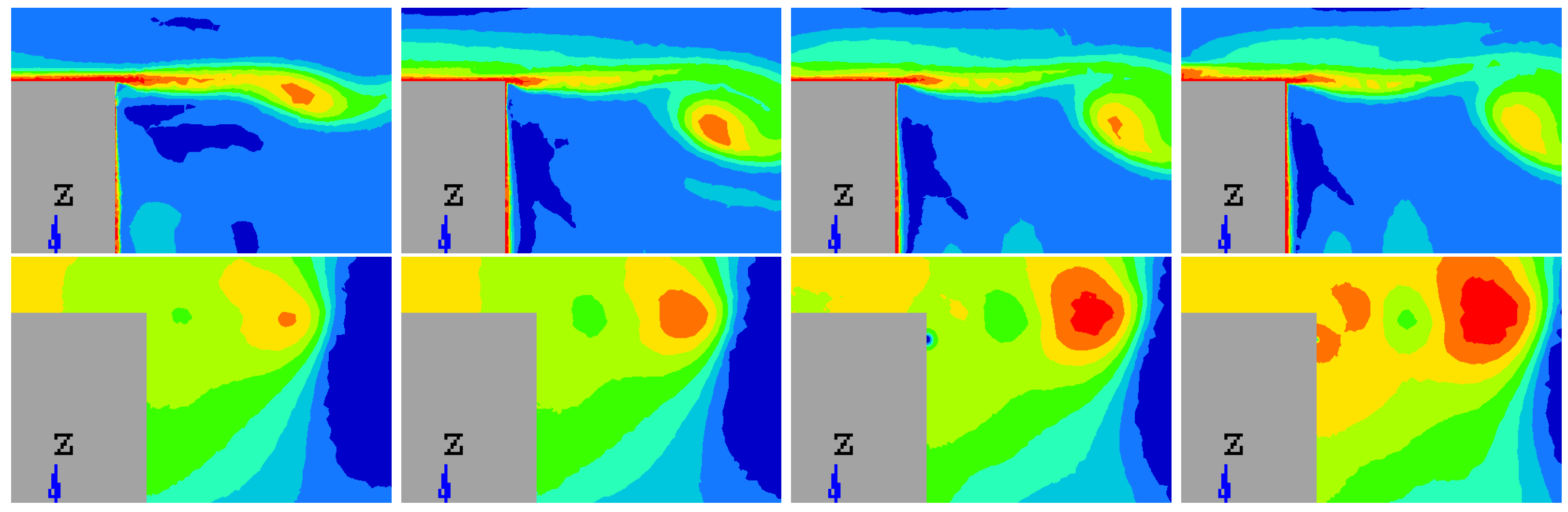
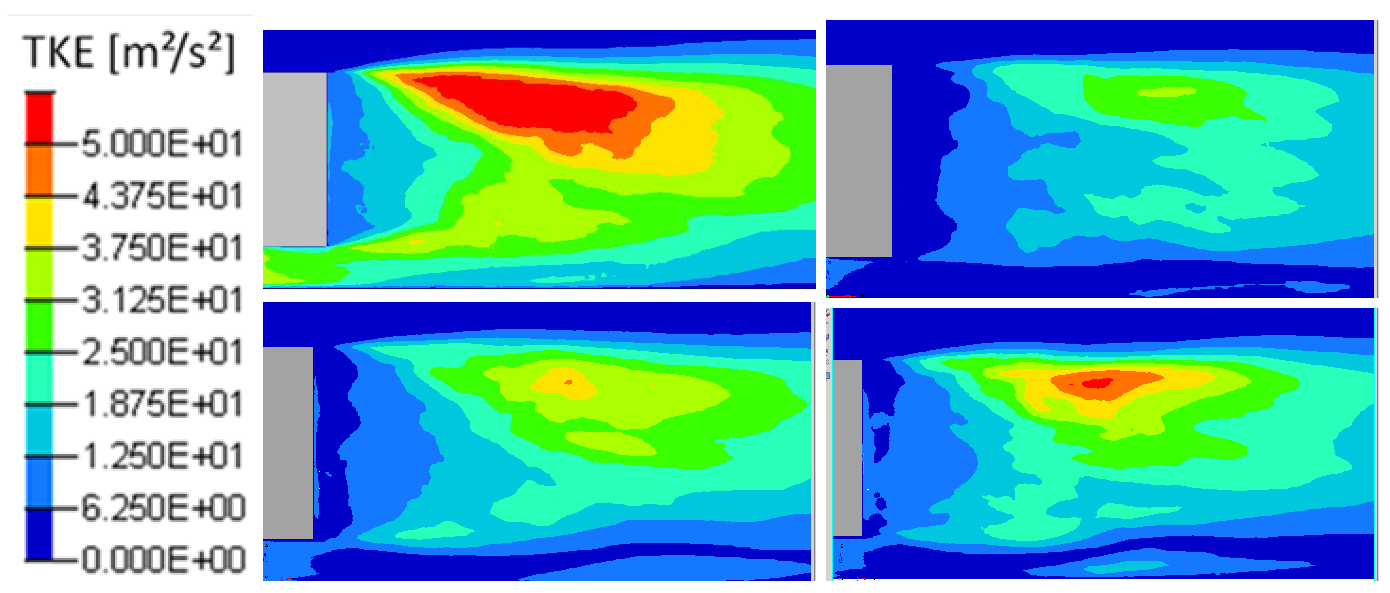
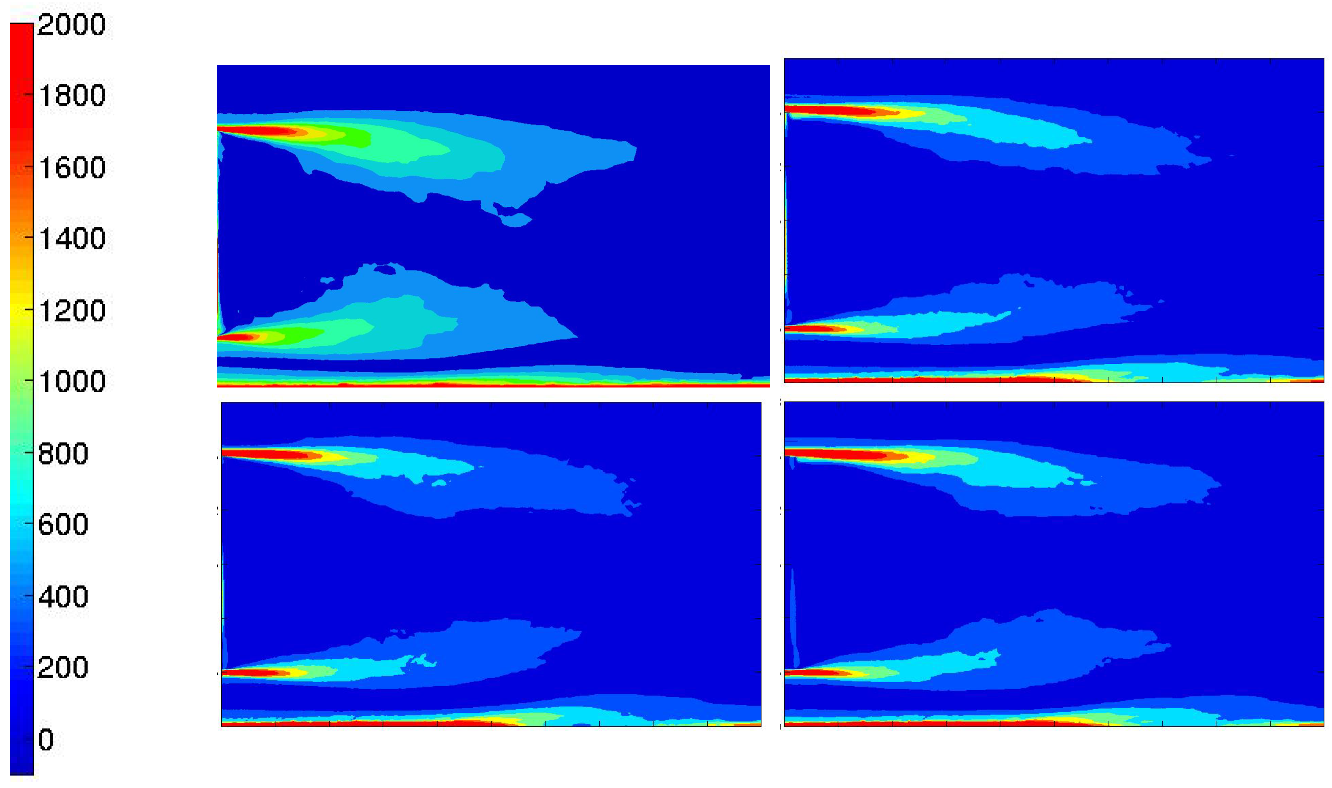
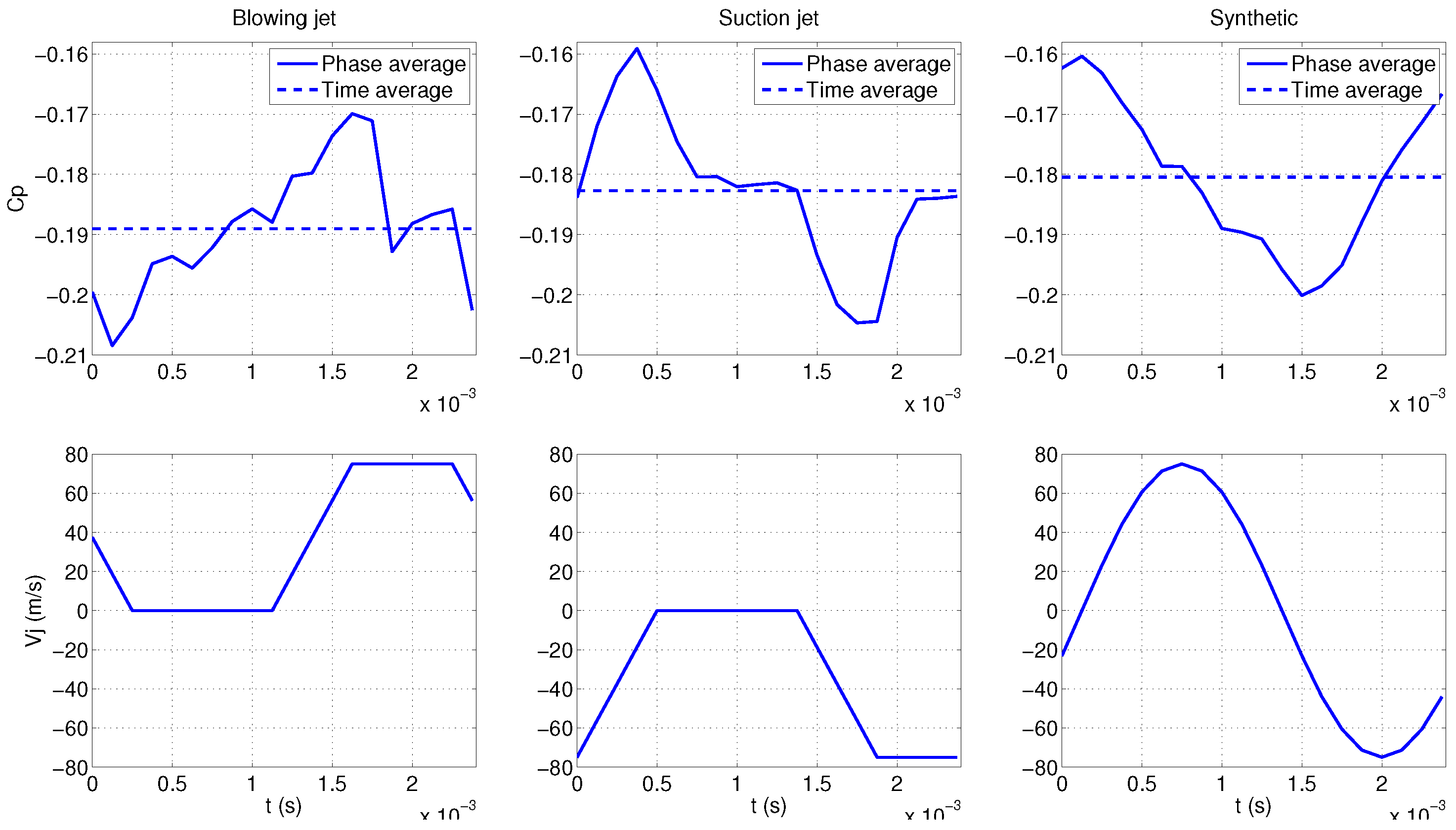
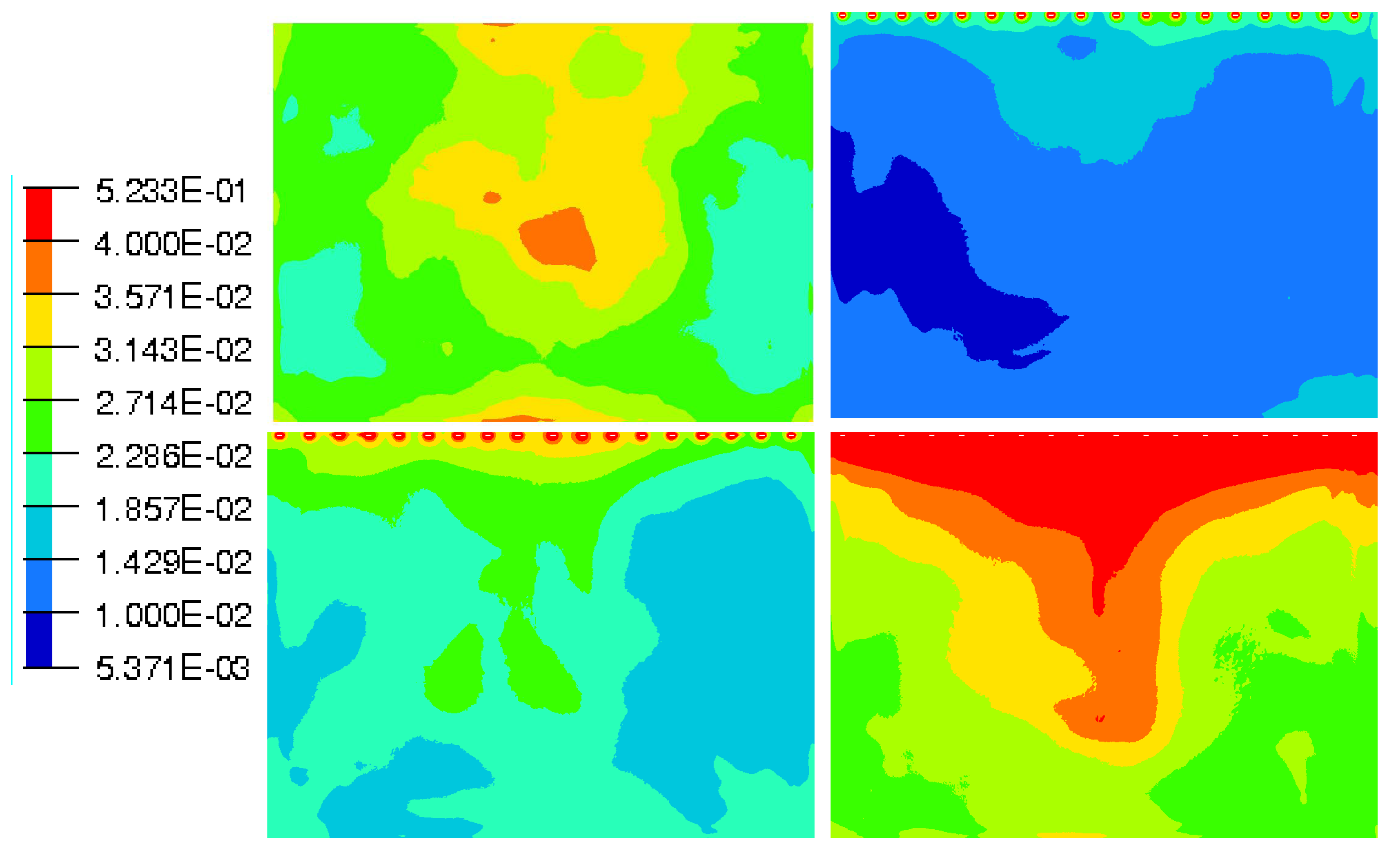
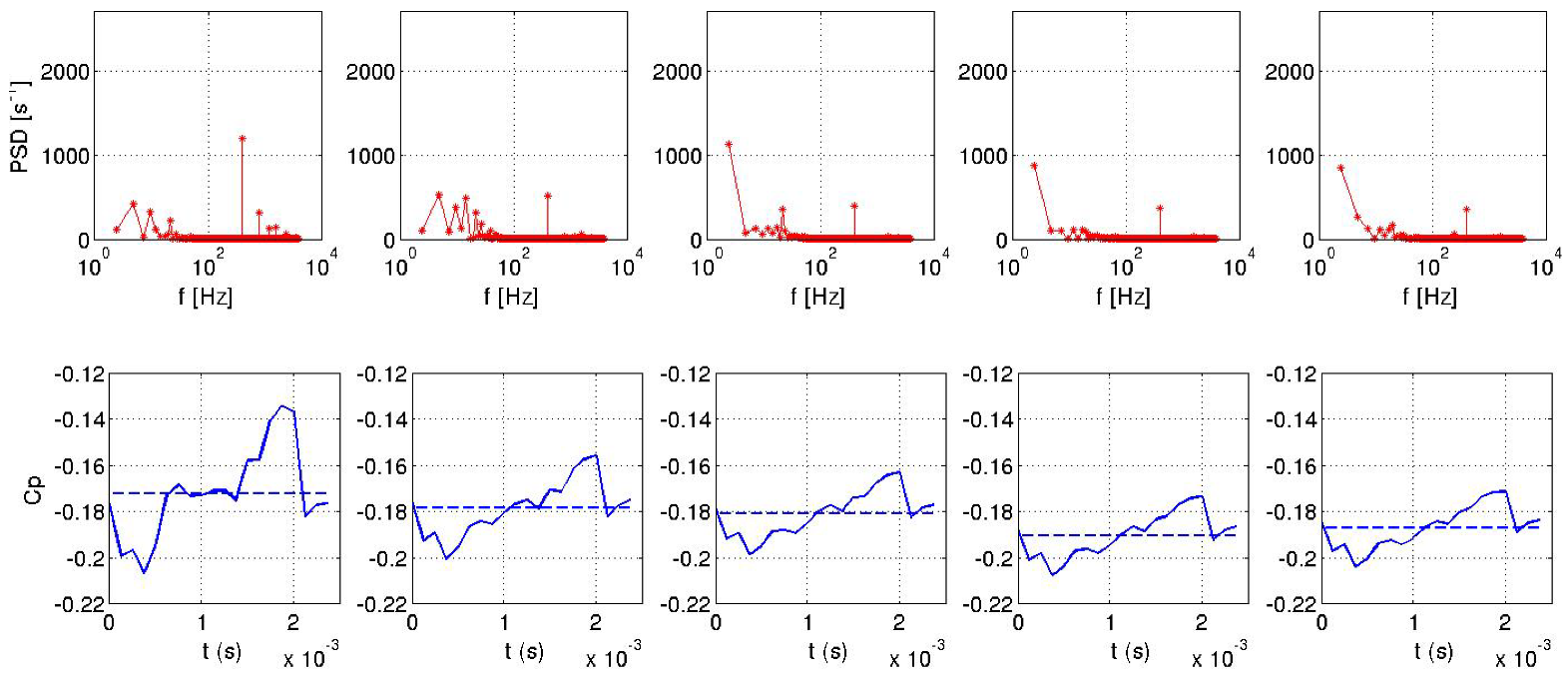
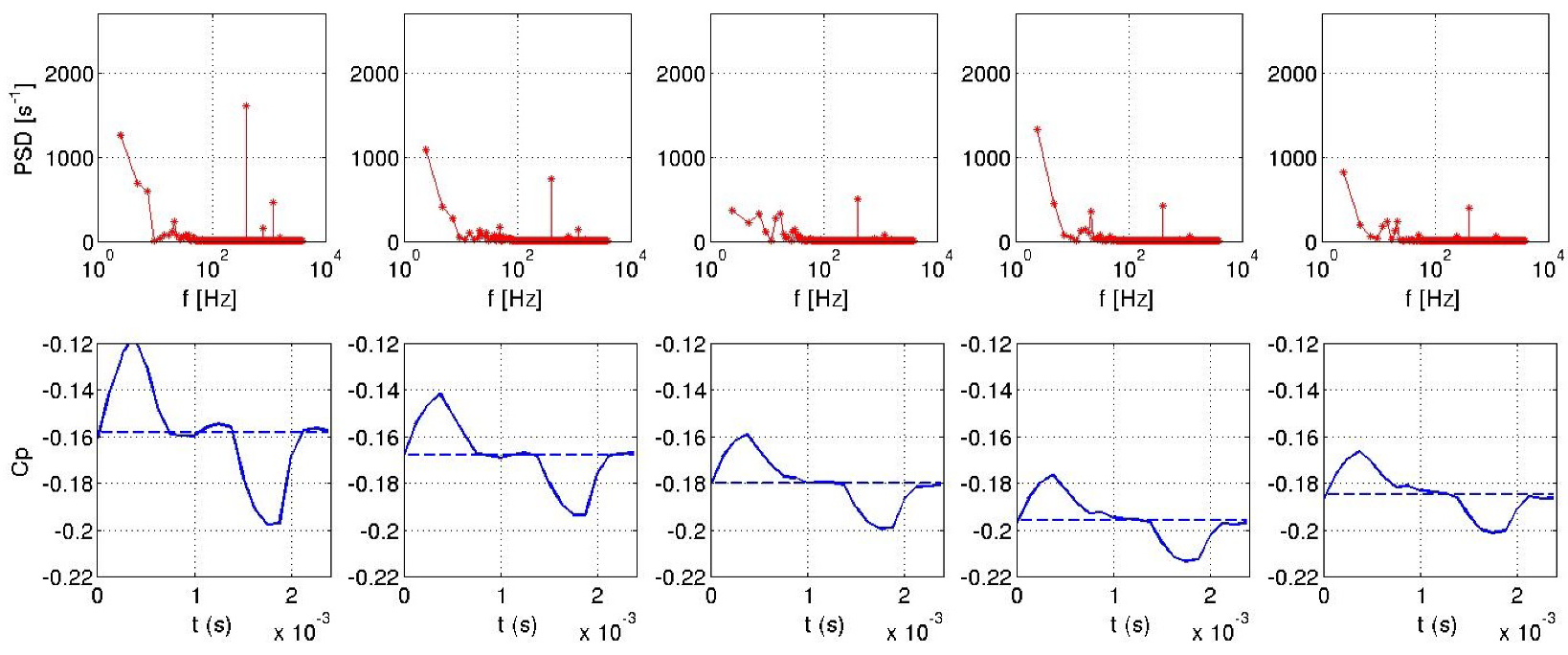
3.3. Actuation Type Influence on the Flow Topology and Behavior
- Time averaged maincharacteristics
- Shearlayer
- Recirculation bubble
- Rear pressure
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Barnard, R. Road Vehicle Aerodynamic Design: An Introduction; Longman: Harlow, UK, 1996. [Google Scholar]
- Fiedler, H.E.; Fernholz, H.H. On management and control of turbulent shear flows. Prog. Aerosp. Sci. 1990, 27, 305–387. [Google Scholar] [CrossRef]
- Roshko, A.; Koening, K. Interaction effects on the drag of bluff bodies in tandem. In Aerodynamic Drag Mechanisms of Bluff Bodies and Road Vehicles; Springer: Boston, MA, USA, 1978; pp. 253–286. [Google Scholar] [CrossRef]
- Bruneau, C.H.; Gillieron, P.; Mortazavi, I. Passive control around the two-dimensional square back Ahmed body using porous devices. J. Fluids Eng. 2008, 130, 061101. [Google Scholar] [CrossRef]
- Khalighi, B.; Chen, K.H.; Iccarino, I.G. Unsteady Aerodynamic Flow Investigation Around a Simplified Square-Back Road Vehicle With Drag Reduction Devices. J. Fluids Eng. 2012, 134, 061101. [Google Scholar] [CrossRef]
- Aider, J.L.; Joseph, P.; Ruiz, T.; Gilotte, P.; Eulalie, Y.; Edouard, C.; Amandolese, X. Active flow control using pulsed micro- jets on a full-scale production car. Int. J. Flow Control 2014, 6, 1–20. [Google Scholar] [CrossRef]
- Mohseni, K.; Mittal, R. Synthetic Jets: Fundamentals and Applications; CRC Press: Boca Raton, FL, USA, 2014. [Google Scholar]
- Schmidt, H.; Woszidlo, R.; Nayeri, C.; Paschereit, C. Separation control with fluidic oscillators in water. Exp. Fluids 2017, 58, 135. [Google Scholar] [CrossRef]
- Karimi, S.; Zargar, A.; Mani, M.; Hemmati, A. The Effect of Single Dielectric Barrier Discharge Actuators in Reducing Drag on an Ahmed Body. Fluids 2020, 5, 244. [Google Scholar] [CrossRef]
- Ahmed, S.R.; Ramm, G.; Faltin, G. Some salient features of time-averaged ground vehicle wake. SAE Tech. Pap. Ser. 1984, 93, 840222–840402. Available online: https://www.jstor.org/stable/44434262 (accessed on 10 December 2021).
- Minguez, M.; Brun, C.; Pasquetti, R.; Serre, E. Experimental and high-order LES analysis of the flow in near-wall region of a square cylinder. Int. J. Heat Fluid Flow 2011, 32, 558–566. [Google Scholar] [CrossRef]
- Roumeas, M.; Gilliéron, P.; Kourta, A. Separated Flows Around the Rear Window of a Simplified Car Geometry. J. Fluids Eng. 2008, 130, 021101. [Google Scholar] [CrossRef]
- Bonnavion, G.; Cadot, O. Unstable wake dynamics of rectangular flat-backed bluff bodies with inclination and ground proximity. J. Fluid Mech. 2018, 854, 196–232. [Google Scholar] [CrossRef]
- Podvin, B.; Pellerin, S.; Fraigneau, Y.; Bonnavion, G.; Cadot, O. Low-order modelling of the wake dynamics of an Ahmed body. J. Fluid Mech. 2021, 927, R6. [Google Scholar] [CrossRef]
- Roumeas, M.; Gilliéron, P.; Kourta, A. Analysis and control of the near-wake flow over a square-back geometry. Comput. Fluids 2009, 38, 60–70. [Google Scholar] [CrossRef]
- Castelain, T.; Michard, M.; Szmigiel, M.; Chacaton, D.; Juvé, D. Identification of flow classes in the wake of a simplified truck model depending on the underbody velocity. J. Wind Eng. Ind. Aerodyn. 2018, 175, 352–363. [Google Scholar] [CrossRef]
- Bruneau, C.H.; Khadra, K.; Mortazavi, I. Flow analysis of square-back simplified vehicles in platoon. Int. J. Heat Fluid Flow 2017, 66, 43–59. [Google Scholar] [CrossRef]
- Zancanaro, M.; Mrosek, M.; Stabile, G.; Othmer, C.; Rozza, G. Hybrid Neural Network Reduced Order Modelling for Turbulent Flows with Geometric Parameters. Fluids 2021, 6, 296. [Google Scholar] [CrossRef]
- Eulalie, Y.; Gilotte, P.; Mortazavi, I. Numerical study of flow control strategies for a simplified square back ground vehicle. Fluid Dyn. Res. 2017, 49, 035502. [Google Scholar] [CrossRef]
- Cooper, K.R. The effect of front-edge rounding and rear-edge shapping on the aerodynamic drag of bluff vehicles in ground proximity. SAE Pap. 1985, 94, 727–757. Available online: https://www.sae.org/publications/technical-papers/content/850288/ (accessed on 10 December 2021).
- Bruneau, C.H.; Creusé, E.; Depeyras, D.; Gillieron, P.; Mortazavi, I. Active procedures to control the flow past the Ahmed body with a 25 degree rear window. Int. J. Aerodyn. 2011, 1, 299–317. [Google Scholar] [CrossRef]
- Schmidt, H.; Woszidlo, R.; Nayeri, C.; Paschereit, C. The effect of flow control on the wake dynamics of a rectangular bluff body in ground proximity. Exp. Fluids 2018, 59, 107. [Google Scholar] [CrossRef]
- Basso, M.; Cravero, C.; Marsano, D. Aerodynamic Effect of the Gurney Flap on the Front Wing of a F1 Car and Flow Interactions with Car Components. Energies 2021, 14, 2059. [Google Scholar] [CrossRef]
- Fernandez-Gamiz, U.; Gomez-Mármol, M.; Chacón-Rebollo, T. Computational Modeling of Gurney Flaps and Microtabs by POD Method. Energies 2018, 11, 2091. [Google Scholar] [CrossRef] [Green Version]
- Maulenkul, S.; Yerzhanov, K.; Kabidollayev, A.; Kamalov, B.; Batay, S.; Zhao, Y.; Wei, D. An Arbitrary Hybrid Turbulence Modeling Approach for Efficient and Accurate Automotive Aerodynamic Analysis and Design Optimization. Fluids 2021, 6, 407. [Google Scholar] [CrossRef]
- Krajnović, S. Large Eddy Simulation Exploration of Passive Flow Control Around an Ahmed Body. J. Fluids Eng. 2014, 136, 121103. [Google Scholar] [CrossRef] [Green Version]
- Eulalie, Y. Etude Aérodynamique et Contrôle de la Traînée sur un corps de Ahmed Culot Droit. Ph.D. Thesis, Université de Bordeaux, Talence, France, 2014. [Google Scholar]
- Kolmogorov, A.N. The local structure of turbulence in incompressible viscous fluid for very large Reynolds numbers. R. Soc. Lond. Proc. Ser. A 1991, 434, 9–13. [Google Scholar] [CrossRef]
- Krajnovic, S.; Davidson, L. Large Eddy Simulation of the Flow around Simplified Car Model; SAE Paper No. 2004-01-0227; SAE International: Detroit, MI, USA, 2004. [Google Scholar]
- Krajnovic, S.; Minelli, G.; Basara, B. Partially-Averaged Navier-Stokes Simulations of Bluff Body Flows. In Proceedings of the ICCFD8 International Conference on Computational Fluid Dynamics, Chengdu, China, 14–16 July 2014. [Google Scholar]
- Mirzaei, M.; Krajnović, S.; Basara, B. Partially-Averaged Navier–Stokes simulations of flows around two different Ahmed bodies. Comput. Fluids 2015, 117, 273–286. [Google Scholar] [CrossRef]
- Ekman, P.; Wieser, D.; Virdung, T.; Karlsson, M. Assessment of hybrid RANS-LES methods for accurate automotive aerodynamic simulations. J. Wind Eng. Ind. Aerodyn. 2020, 206, 104301. [Google Scholar] [CrossRef]
- Guilmineau, E.; Deng, G.; Leroyer, A.; Queutey, P.; Visonneau, M.; Wackers, J. Assessment of hybrid RANS-LES formulations for flow simulation around the Ahmed body. Comput. Fluids 2018, 176, 302–319. [Google Scholar] [CrossRef]
- Delassaux, F.; Mortazavi, I.; Itam, E.; Herbert, V.; Ribes, C. Sensitivity analysis of hybrid methods for the flow around the ahmed body with application to passive control with rounded edges. Comput. Fluids 2021, 214, 104757. [Google Scholar] [CrossRef]
- Corson, D.; Jaiman, R.; Shakib, F. Industrial application of RANS modelling: Capabilities and needs. Int. J. Comput. Fluid Dyn. 2009, 23, 337–347. [Google Scholar] [CrossRef]
- Germano, M.; Piomelli, U.; Moin, P.; Cabot, W.H. A Dynamic Subgrid-Scale Eddy Viscosity Model. Phys. Fluids A Fluid Dyn. 1991, 3, 1760–1769. [Google Scholar] [CrossRef] [Green Version]
- Cintolesi, C.; Mémin, E. Stochastic Modelling of Turbulent Flows for Numerical Simulations. Fluids 2020, 5, 108. [Google Scholar] [CrossRef]
- Pope, S.B. Turbulent Flows; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar] [CrossRef]
- Bruneau, C.H.; Creuse, E.; Depeyras, D.; Gilleron, P.; Mortazav, I. Coupling active and passive techniques to control the flow past the square back Ahmed body. Comput. Fluids 2010, 39, 1875–1892. [Google Scholar] [CrossRef]











| Ref | Blowing Pulsed Jet | Suction Pulsed Jet | Synthetic Jet | |
|---|---|---|---|---|
| Cd | 0.364 | 0.337 | 0.333 | 0.330 |
| Drag reduction [%] | - | −6.74 | −7.867 | −8.61 |
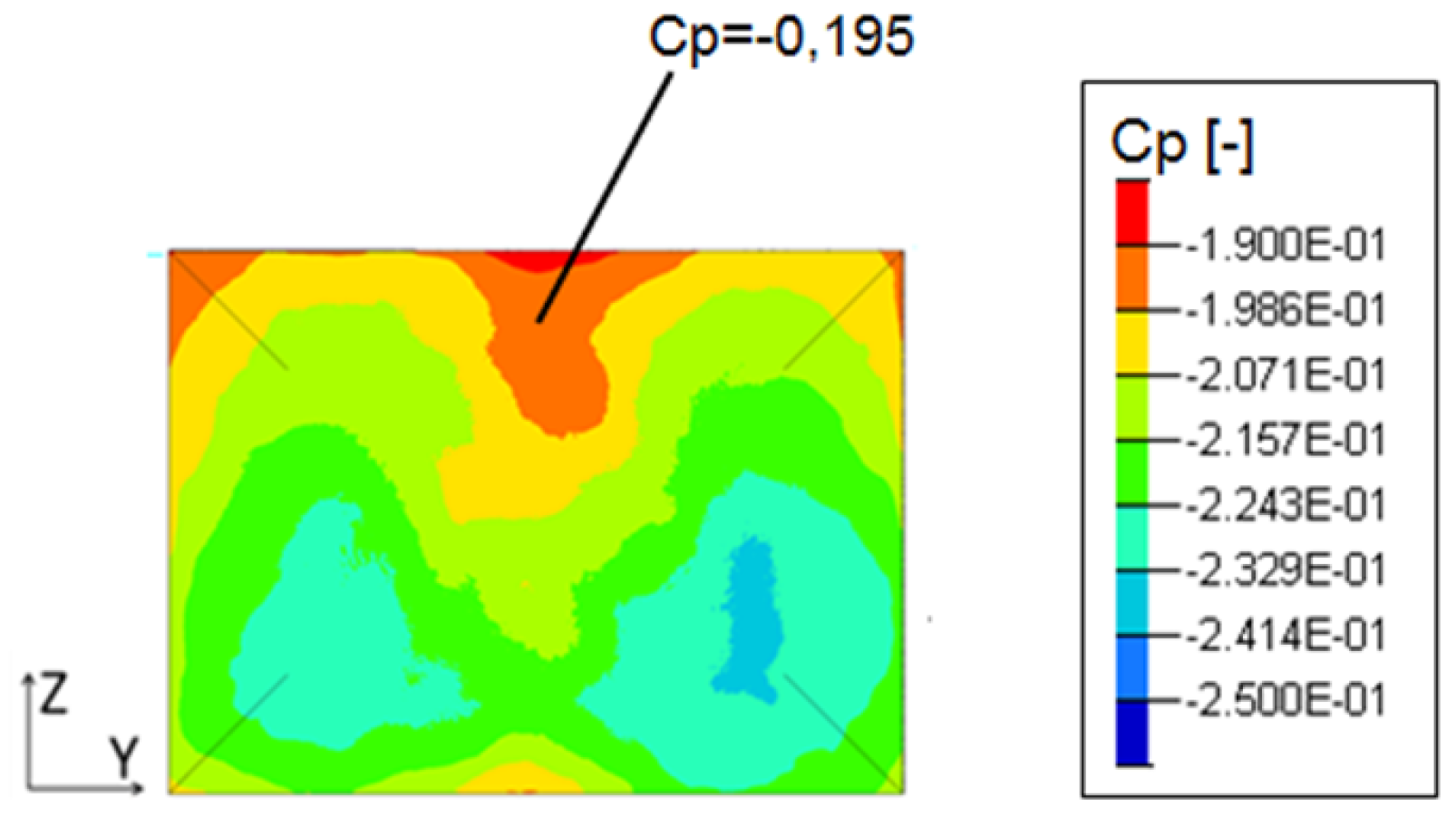
| Cp | −0.201 | −0.186 | −0.1825 | −0.1826 |
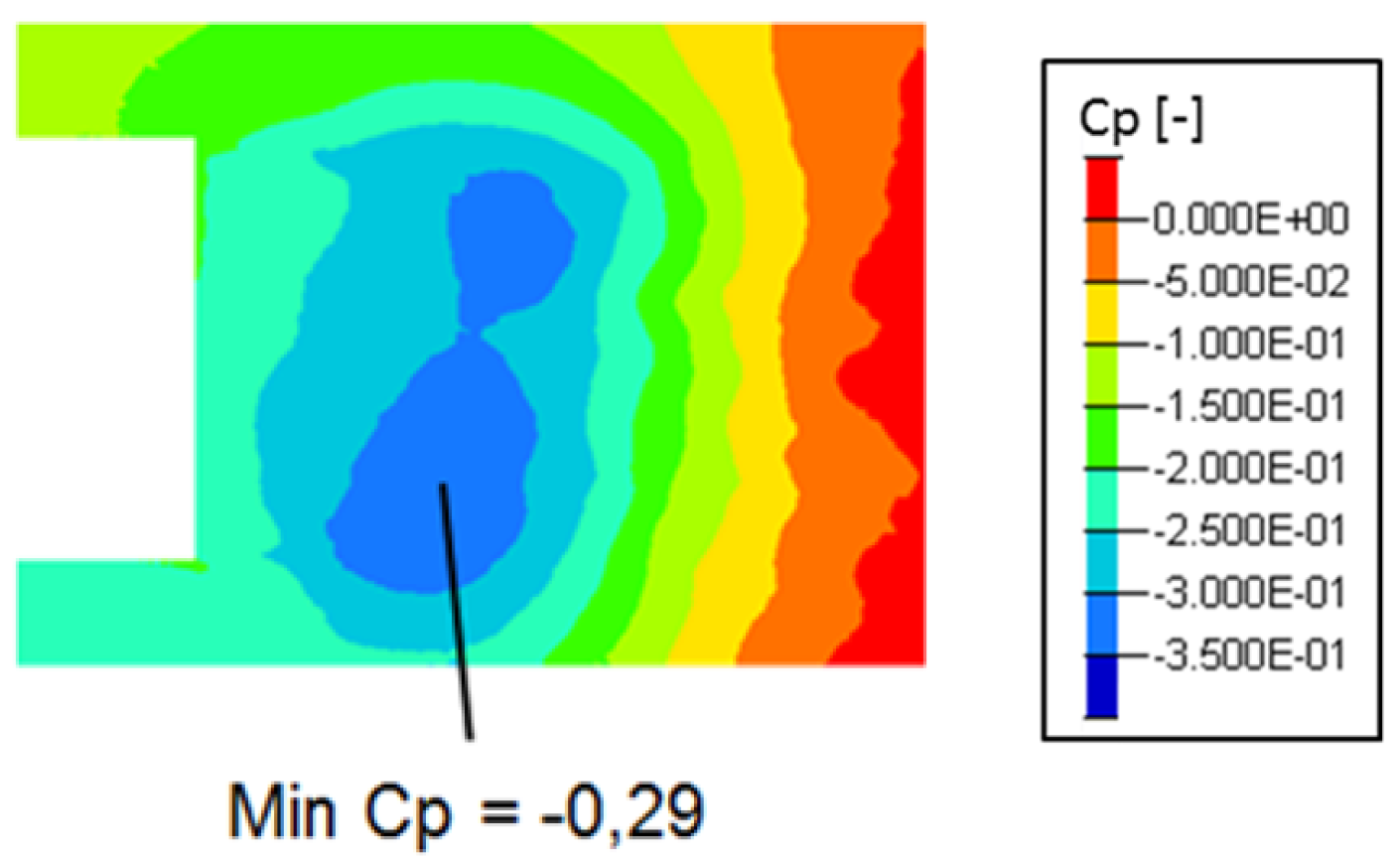
| Upper recirculation | −0.29 | −0.267 | −0.318 | −0.2731 |
| min pressure | (at 0.78H) | (at 0.76H) | (at 0.74H) | (at 0.91H) |
| Lower recirculation | −0.29 | −0.270 | −0.282 | −0.2753 |
| min pressure | (at 0.6H) | (at 0.78H) | (at 0.69H) | (at 0.88H) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Edwige, S.; Gilotte, P.; Mortazavi, I. Computational Analysis of Actuation Techniques Impact on the Flow Control around the Ahmed Body. Fluids 2022, 7, 52. https://doi.org/10.3390/fluids7020052
Edwige S, Gilotte P, Mortazavi I. Computational Analysis of Actuation Techniques Impact on the Flow Control around the Ahmed Body. Fluids. 2022; 7(2):52. https://doi.org/10.3390/fluids7020052
Chicago/Turabian StyleEdwige, Stephie, Philippe Gilotte, and Iraj Mortazavi. 2022. "Computational Analysis of Actuation Techniques Impact on the Flow Control around the Ahmed Body" Fluids 7, no. 2: 52. https://doi.org/10.3390/fluids7020052
APA StyleEdwige, S., Gilotte, P., & Mortazavi, I. (2022). Computational Analysis of Actuation Techniques Impact on the Flow Control around the Ahmed Body. Fluids, 7(2), 52. https://doi.org/10.3390/fluids7020052






