Computational Fluid Dynamics Model for Analysis of the Turbulent Limits of Hydrogen Combustion
Abstract
1. Introduction
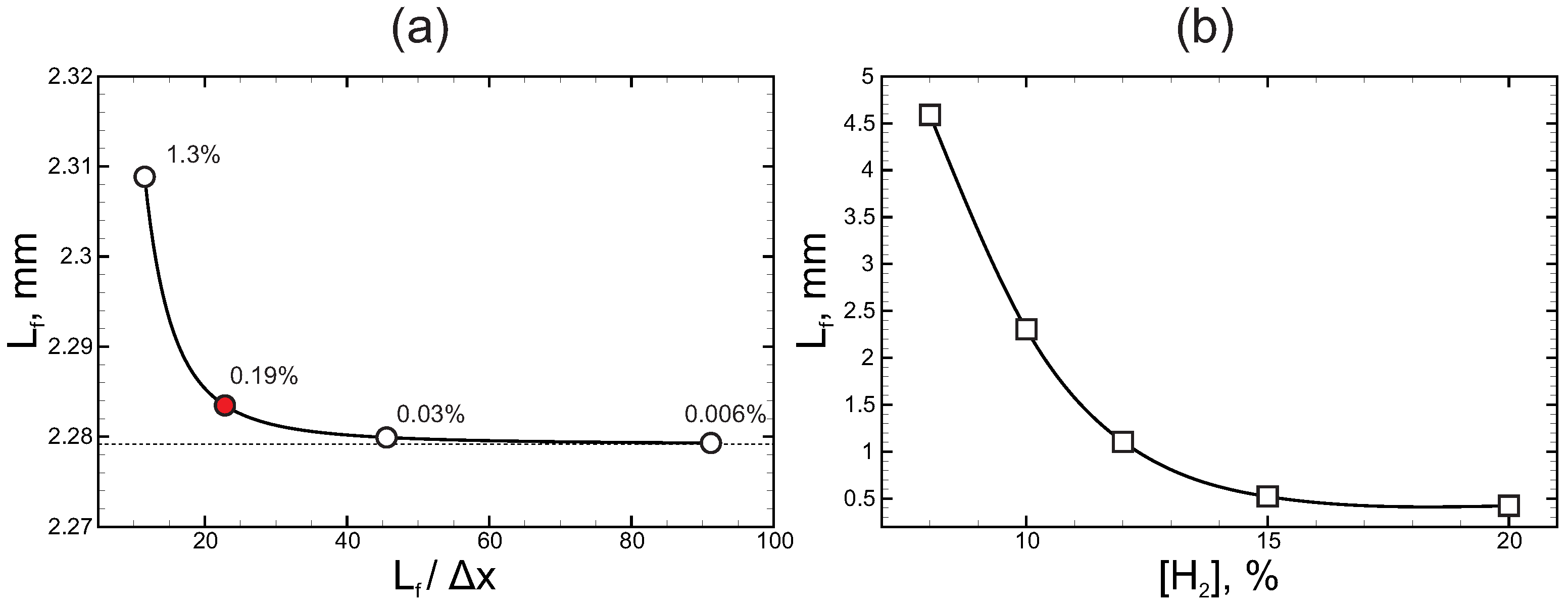
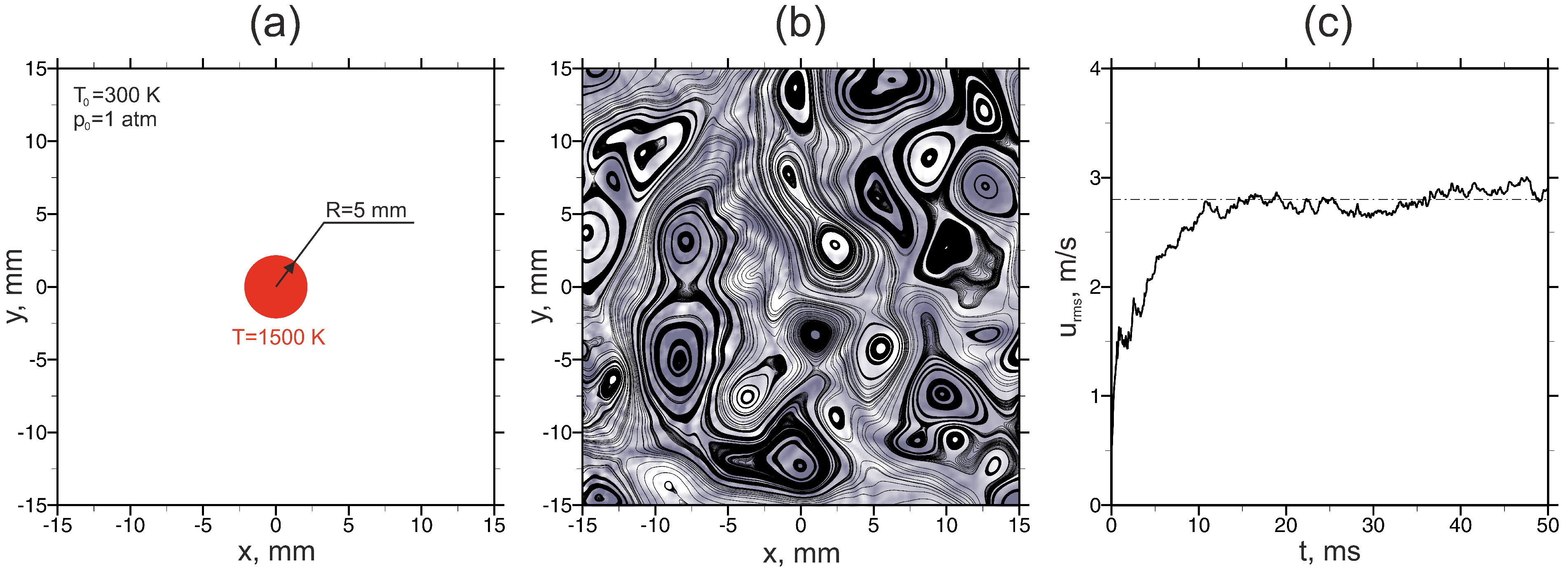
2. Problem Setup
3. Results and Discussion
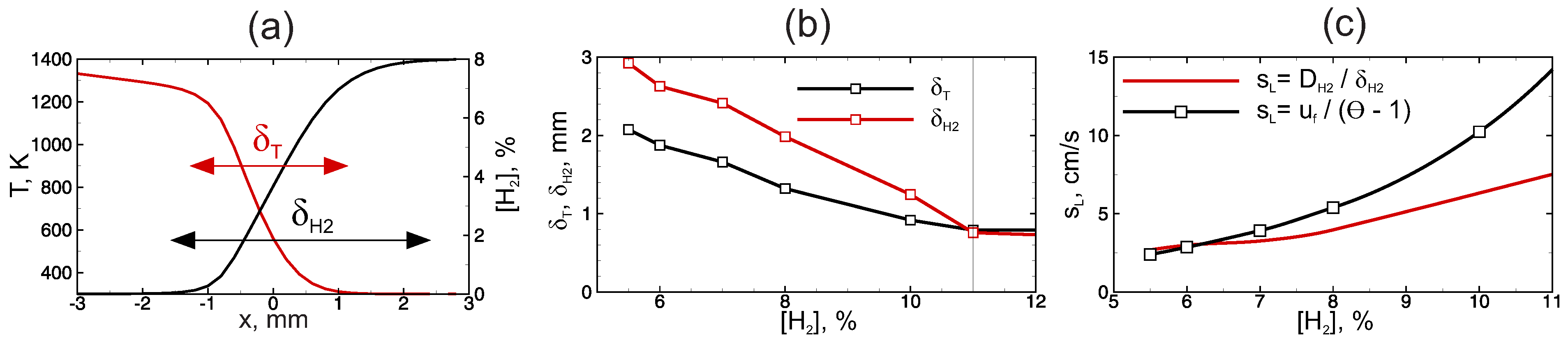
3.1. Flame Structure in Lean Hydrogen–Air Mixtures
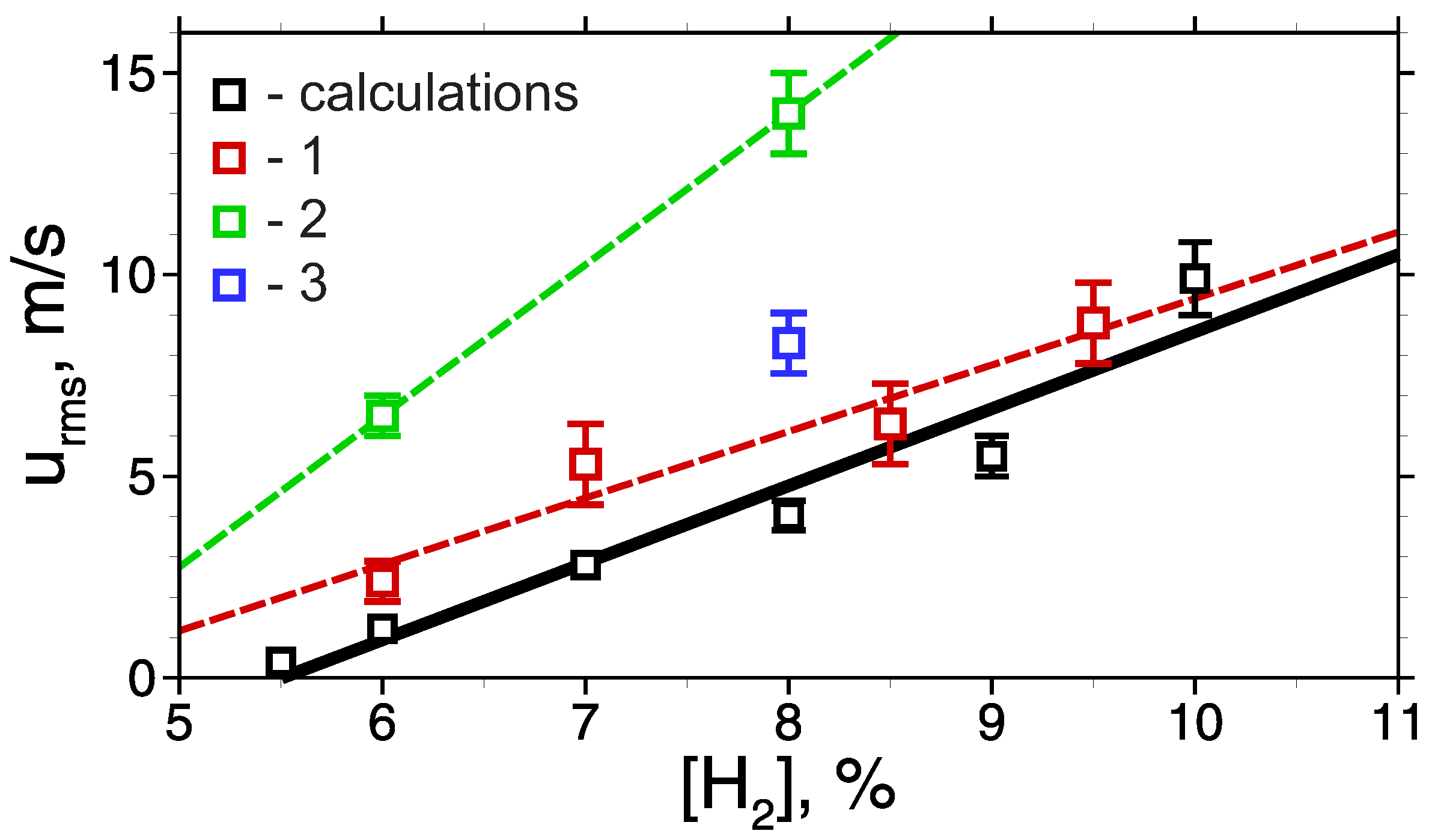
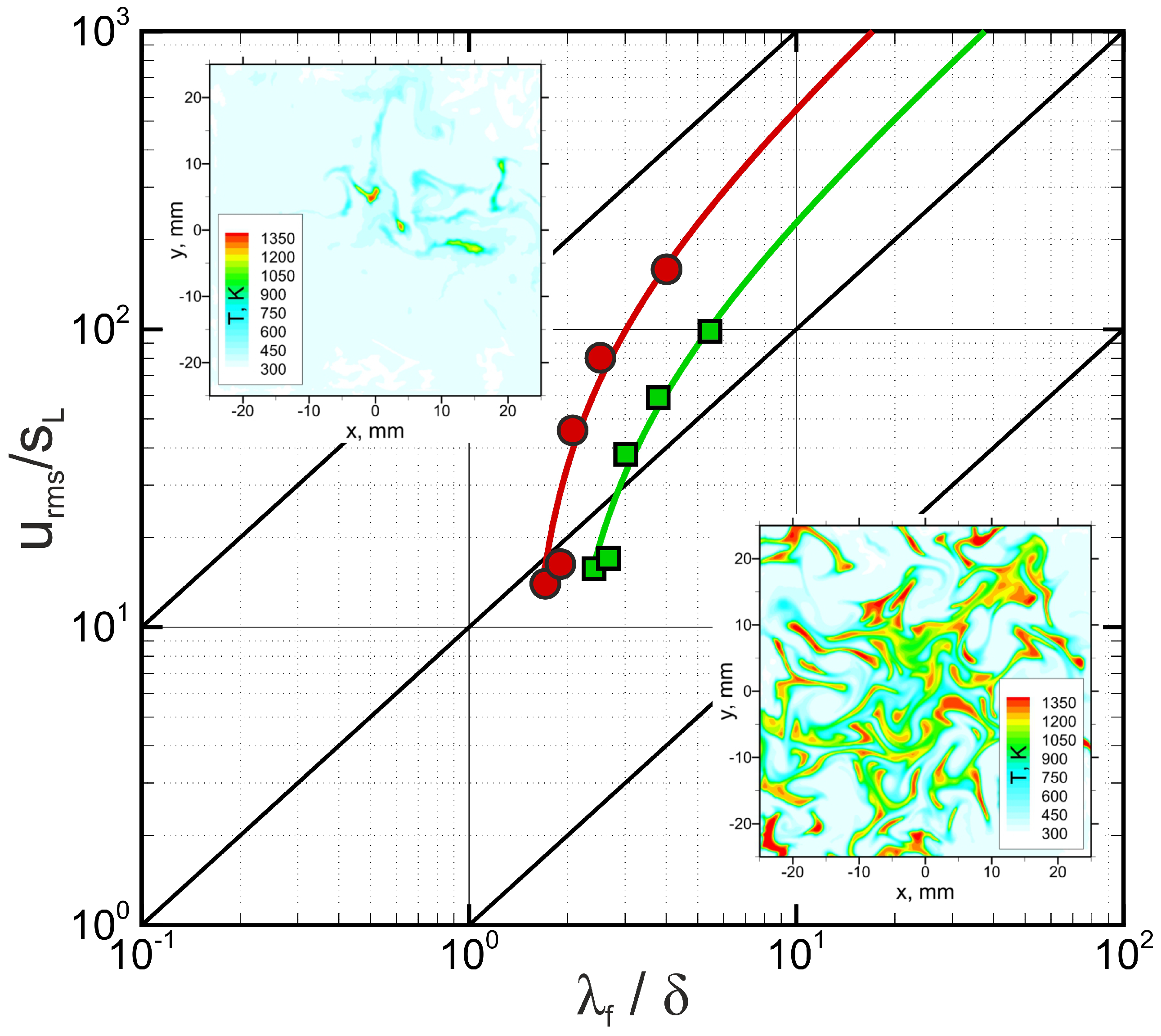
3.2. Modes of Flame–Turbulence Interaction and Definition of the Turbulent Combustion Limit
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| JANAF | Joint Army, Navy, and Air Force |
References
- Coward, H.F.; Jones, G.W. Limits of Flammability of Gases and Vapors; Technical Report Bulletin 503; U.S. Bureau of Mines: Washington, DC, USA, 1952.
- Gelfand, B.E.; Silnikov, M.V.; Medvedev, S.P.; Khomik, S.V. Thermo-Gas Dynamics of Hydrogen Combustion and Explosion, 1st ed.; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Udagawa, Y.; Yamaguchi, M.; Abe, H.; Sekimura, N.; Fuketa, T. Ab initio study on plane defects in zirconium–hydrogen solid solution and zirconium hydride. Acta Mater. 2010, 58, 3927–3938. [Google Scholar] [CrossRef]
- Petukhov, V.; Naboko, I.; Fortov, V. Explosion hazard of hydrogen—Air mixtures in the large volumes. Int. J. Hydrogen Energy 2009, 34, 5924–5931. [Google Scholar] [CrossRef]
- Eichert, H.; Fischer, M. Combustion-related safety aspects of hydrogen in energy applications. Int. J. Hydrogen Energy 1986, 11, 117–124. [Google Scholar] [CrossRef]
- Kumar, R. Flammability Limits of Hydrogen-Oxygen-Diluent Mixtures. J. Fire Sci. 1985, 3, 245–262. [Google Scholar] [CrossRef]
- Medvedev, S.P.; Gel’fand, B.E.; Polenov, A.N.; Khomik, S.V. Flammability limits for hydrogen-air mixtures in the presence of ultrafine droplets of water (fog). Combust. Explos. Shock Waves 2002, 38, 381–386. [Google Scholar] [CrossRef]
- Project SAFEKINEX. Report on the Experimentally Determined Explosion Limits, Explosion Pressures and Rates of Explosion Pressure Rise; Technical Report Contractual Deliverable No. 8; Federal Institute for Materials Research and Testing (BAM): Berlin, Germany, 2002. [Google Scholar]
- Leblanc, L.; Manoubi, M.; Dennis, K.; Liang, Z.; Radulescu, M.I. Dynamics of unconfined spherical flames. Phys. Fluids 2012, 25, 091106. [Google Scholar] [CrossRef]
- Ronney, P.D. Near-limit flame structures at low Lewis number. Combust. Flame 1990, 82, 1–14. [Google Scholar] [CrossRef]
- Yakovenko, I.; Kiverin, A.; Melnikova, K. Ultra-Lean Gaseous Flames in Terrestrial Gravity Conditions. Fluids 2021, 6, 21. [Google Scholar] [CrossRef]
- Kiverin, A.D.; Yakovenko, I.S.; Melnikova, K.S. On the structure and stability of ultra-lean flames. J. Phys. Conf. Ser. 2019, 1147, 012048. [Google Scholar] [CrossRef]
- Yang, S.; Saha, A.; Liang, W.; Wu, F.; Law, C.K. Extreme role of preferential diffusion in turbulent flame propagation. Combust. Flame 2018, 188, 498–504. [Google Scholar] [CrossRef]
- Karpov, V.; Severin, E. Effects of molecular-transport coefficients on the rate of turbulent combustion. Combust. Explos. Shock Waves 1980, 16, 41–46. [Google Scholar] [CrossRef]
- Abdel-Gayed, R.; Bradley, D. Criteria for turbulent propagation limits of premixed flames. Combust. Flame 1985, 62, 61–68. [Google Scholar] [CrossRef]
- Chomiak, J.; Jarosiński, J. Flame quenching by turbulence. Combust. Flame 1982, 48, 241–249. [Google Scholar] [CrossRef]
- Bradley, D.; Shehata, M.; Lawes, M.; Ahmed, P. Flame extinctions: Critical stretch rates and sizes. Combust. Flame 2020, 212, 459–468. [Google Scholar] [CrossRef]
- Bilger, R.; Pope, S.; Bray, K.; Driscoll, J. Paradigms in turbulent combustion research. Proc. Combust. Inst. 2005, 30, 21–42. [Google Scholar] [CrossRef]
- Hawkes, E.R.; Sankaran, R.; Sutherland, J.C.; Chen, J.H. Direct numerical simulation of turbulent combustion: Fundamental insights towards predictive models. J. Phys. Conf. Ser. 2005, 16, 65–79. [Google Scholar] [CrossRef]
- Trisjono, P.; Pitsch, H. Systematic Analysis Strategies for the Development of Combustion Models from DNS: A Review. Flow Turbul. Combust. 2015, 95, 231–259. [Google Scholar] [CrossRef]
- Williams, F.A. Combustion Theory, 2nd ed.; CRC Press: Boca Raton, FL, USA, 1985. [Google Scholar]
- Cant, R.; Pope, S.; Bray, K. Modelling of flamelet surface-to-volume ratio in turbulent premixed combustion. Symp. Int. Combust. 1991, 23, 809–815. [Google Scholar] [CrossRef]
- Bechtold, J.K.; Matalon, M. The dependence of the Markstein length on stoichiometry. Combust. Flame 2001, 127, 1906–1913. [Google Scholar] [CrossRef]
- Giannakopoulos, G.K.; Gatzoulis, A.; Frouzakis, C.E.; Matalon, M.; Tomboulides, A.G. Consistent definitions of “Flame Displacement Speed” and “Markstein Length” for premixed flame propagation. Combust. Flame 2015, 162, 1249–1264. [Google Scholar] [CrossRef]
- Dave, H.L.; Chaudhuri, S. Evolution of local flame displacement speeds in turbulence. J. Fluid Mech. 2020, 884, A46. [Google Scholar] [CrossRef]
- Lipatnikov, A.; Nishiki, S.; Hasegawa, T. Closure Relations for Fluxes of Flame Surface Density and Scalar Dissipation Rate in Turbulent Premixed Flames. Fluids 2019, 4, 43. [Google Scholar] [CrossRef]
- Pope, S.B. PDF methods for turbulent reactive flows. Prog. Energy Combust. Sci. 1985, 11, 119–192. [Google Scholar] [CrossRef]
- Kuznetsov, V.R.; Sabelnikov, V.A. Turbulence and Combustion, 1st ed.; Hemisphere Publishing Corporation: New York, NY, USA, 1990. [Google Scholar]
- Haworth, D.C. Progress in probability density function methods for turbulent reacting flows. Prog. Energy Combust. Sci. 2010, 36, 168–259. [Google Scholar] [CrossRef]
- Zimont, V.L. Conceptual Limitations of the Probability Density Function Method for Modeling Turbulent Premixed Combustion and Closed-Form Description of Chemical Reactions’ Effects. Fluids 2021, 6, 142. [Google Scholar] [CrossRef]
- Sabelnikov, V.A.; Lipatnikov, A.N. A new mathematical framework for describing thin-reaction-zone regime of turbulent reacting flows at low damköhler number. Fluids 2020, 5, 109. [Google Scholar] [CrossRef]
- Betev, A.; Kiverin, A.; Medvedev, S.; Yakovenko, I. Numerical Simulation of Turbulent Hydrogen Combustion Regimes Near the Lean Limit. Russ. J. Phys. Chem. B 2020, 14, 940–945. [Google Scholar] [CrossRef]
- Uranakara, H.A.; Chaudhuri, S.; Lakshmisha, K. On the extinction of igniting kernels in near-isotropic turbulence. Proc. Combust. Inst. 2017, 36, 1793–1800. [Google Scholar] [CrossRef]
- Volodin, V.V.; Golub, V.V.; Kiverin, A.D.; Melnikova, K.S.; Mikushkin, A.Y.; Yakovenko, I.S. Large-scale Dynamics of Ultra-lean Hydrogen-air Flame Kernels in Terrestrial Gravity Conditions. Combust. Sci. Technol. 2020, 193, 225–234. [Google Scholar] [CrossRef]
- Yakovenko, I.S.; Ivanov, M.F.; Kiverin, A.D.; Melnikova, K.S. Large-scale flame structures in ultra-lean hydrogen-air mixtures. Int. J. Hydrogen Energy 2018, 43, 1894–1901. [Google Scholar] [CrossRef]
- Ronney, P.D.; Whaling, K.N.; Abbud-Madrid, A.; Gatto, J.L.; Pisowicz, V.L. Stationary premixed flames in spherical and cylindrical geometries. AIAA J. 1994, 32, 569–577. [Google Scholar] [CrossRef]
- Kuo, K.K.; Acharya, R. Fundamentals of Turbulent and Multiphase Combustion, 1st ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2012. [Google Scholar]
- Poinsot, T.; Veynante, D. Theoretical Numerical Combustion, 2nd ed.; R.T. Edwards, Inc.: Philadelphia, PA, USA, 2005. [Google Scholar]
- Bykov, V.; Kiverin, A.; Koksharov, A.; Yakovenko, I. Analysis of transient combustion with the use of contemporary CFD techniques. Comput. Fluids 2019, 194, 104310. [Google Scholar] [CrossRef]
- Chase, M.W. NIST-JANAF Themochemical Tables, 4th ed.; American Institute of Physics: Woodbury, NY, USA, 1998; Volume 9. [Google Scholar]
- Hirschfelder, J.O.; Curtiss, C.F.; Bird, R.B. The Molecular Theory of Gases and Liquids, Revised ed.; Wiley-Interscience: Hoboken, NJ, USA, 1964. [Google Scholar]
- Kee, R.J.; Coltrin, M.E.; Glarborg, P. Chemically Reacting Flow: Theory and Practice, 1st ed.; Wiley-Interscience: Hoboken, NJ, USA, 2003. [Google Scholar]
- Coffee, T.; Heimerl, J. Transport algorithms for premixed, laminar steady-state flames. Combust. Flame 1981, 43, 273–289. [Google Scholar] [CrossRef]
- Kéromnès, A.; Metcalfe, W.K.; Heufer, K.A.; Donohoe, N.; Das, A.K.; Sung, C.J.; Herzler, J.; Naumann, C.; Griebel, P.; Mathieu, O.; et al. An experimental and detailed chemical kinetic modeling study of hydrogen and syngas mixture oxidation at elevated pressures. Combust. Flame 2013, 160, 995–1011. [Google Scholar] [CrossRef]
- McGrattan, K.; McDermott, R.; Hostikka, S.; Floyd, J.; Vanella, M. Fire Dynamics Simulator Technical Reference Guide Volume 1: Mathematical Model; Technical Report NIST Special Publication 1018-1; U.S. Department of Commerce, National Institute of Standards and Technology: Gaithersburg, MD, USA, 2019. [CrossRef]
- Carroll, P.L.; Blanquart, G. A proposed modification to Lundgren’s physical space velocity forcing method for isotropic turbulence. Phys. Fluids 2013, 25, 105114. [Google Scholar] [CrossRef]
- Lu, Z.; Yang, Y. Modeling pressure effects on the turbulent burning velocity for lean hydrogen/air premixed combustion. Proc. Combust. Inst. 2021, 38, 2901–2908. [Google Scholar] [CrossRef]
- Wang, Z.; Abraham, J. Effects of Karlovitz Number on Flame Surface Wrinkling in Turbulent Lean Premixed Methane-Air Flames. Combust. Sci. Technol. 2018, 190, 363–392. [Google Scholar] [CrossRef]
- Basevich, V.Y.; Belyaev, A.A.; Frolov, S.M.; Frolov, F.S. Direct Numerical Simulation of Turbulent Combustion of Hydrogen—Air Mixtures of Various Compositions in a Two-Dimensional Approximation. Russ. J. Phys. Chem. B 2019, 13, 75–85. [Google Scholar] [CrossRef]
- Haugen, N.E.L.; Brandenburg, A.; Dobler, W. Simulations of nonhelical hydromagnetic turbulence. Phys. Rev. E Stat. Phys. Plasmas Fluids Relat. Interdiscip. Top. 2004, 70, 14. [Google Scholar] [CrossRef]
- Semenov, E.S. Measurement of turbulence characteristics in a closed volume with artificial turbulence. Combust. Explos. Shock Waves 1965, 1, 57–62. [Google Scholar] [CrossRef]
- Karpov, V.P.; Semenov, E.S.; Sokolik, A.S. Turbulent combustion in closed volume. Dokl. USSR 1959, 128, 1220–1223. [Google Scholar]
- Kusharin, A.Y.; Agafonov, G.L.; Popov, O.E.; Gelfand, B.E. Detonability of H2/CO/CO2/Air Mixtures. Combust. Sci. Technol. 1998, 135, 85–98. [Google Scholar] [CrossRef]
- Konnov, A. Refinement of the kinetic mechanism of hydrogen combustion. Khimicheskaya Fiz. 2004, 23, 5–19. [Google Scholar]
- Boivin, P. Reduced-Kinetic Mechanisms for Hydrogen and Syngas Combustion Including Autoignition. Ph.D. Thesis, Universidad Carlos III de Madrid, Leganés, Spain, 2011. [Google Scholar]
- Starik, A.; Titova, N. Kinetics of detonation initiation in the supersonic flow of the H2 + O2 (air) mixture in O2 molecule excitation by resonance laser radiation. Kinet. Catal. 2003, 44, 28–39. [Google Scholar] [CrossRef]
- Al-Khishali, K.; Bradley, D.; Hall, S. Turbulent combustion of near-limit hydrogen-air mixtures. Combust. Flame 1983, 54, 61–70. [Google Scholar] [CrossRef]
- Breitung, W.; Chan, C.; Dorofeev, S.; Eder, A.; Gelfand, B.; Heitch, M.; Klein, R.; Malliakos, A.; Shepherd, J.; Studer, A.; et al. Flame Acceleration and Deflagration-to-Detonation Transition in Nuclear Safety, State-of-the-Art Report by a Group of Experts; Technical Report; Technical Report No. NEA/CSNI; OECD Nuclear Energy Agency: Le Seine Saint-Germain, France, 2000. [Google Scholar]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yakovenko, I.; Kiverin, A.; Melnikova, K. Computational Fluid Dynamics Model for Analysis of the Turbulent Limits of Hydrogen Combustion. Fluids 2022, 7, 343. https://doi.org/10.3390/fluids7110343
Yakovenko I, Kiverin A, Melnikova K. Computational Fluid Dynamics Model for Analysis of the Turbulent Limits of Hydrogen Combustion. Fluids. 2022; 7(11):343. https://doi.org/10.3390/fluids7110343
Chicago/Turabian StyleYakovenko, Ivan, Alexey Kiverin, and Ksenia Melnikova. 2022. "Computational Fluid Dynamics Model for Analysis of the Turbulent Limits of Hydrogen Combustion" Fluids 7, no. 11: 343. https://doi.org/10.3390/fluids7110343
APA StyleYakovenko, I., Kiverin, A., & Melnikova, K. (2022). Computational Fluid Dynamics Model for Analysis of the Turbulent Limits of Hydrogen Combustion. Fluids, 7(11), 343. https://doi.org/10.3390/fluids7110343