An Elementary Model for a Self-Accelerating Outward Propagating Flame Subject to the Rayleigh–Taylor Instability: Transition to Detonation
Abstract
1. Introduction
2. Modelling
3. Numerical Experiments
4. Concluding Remarks
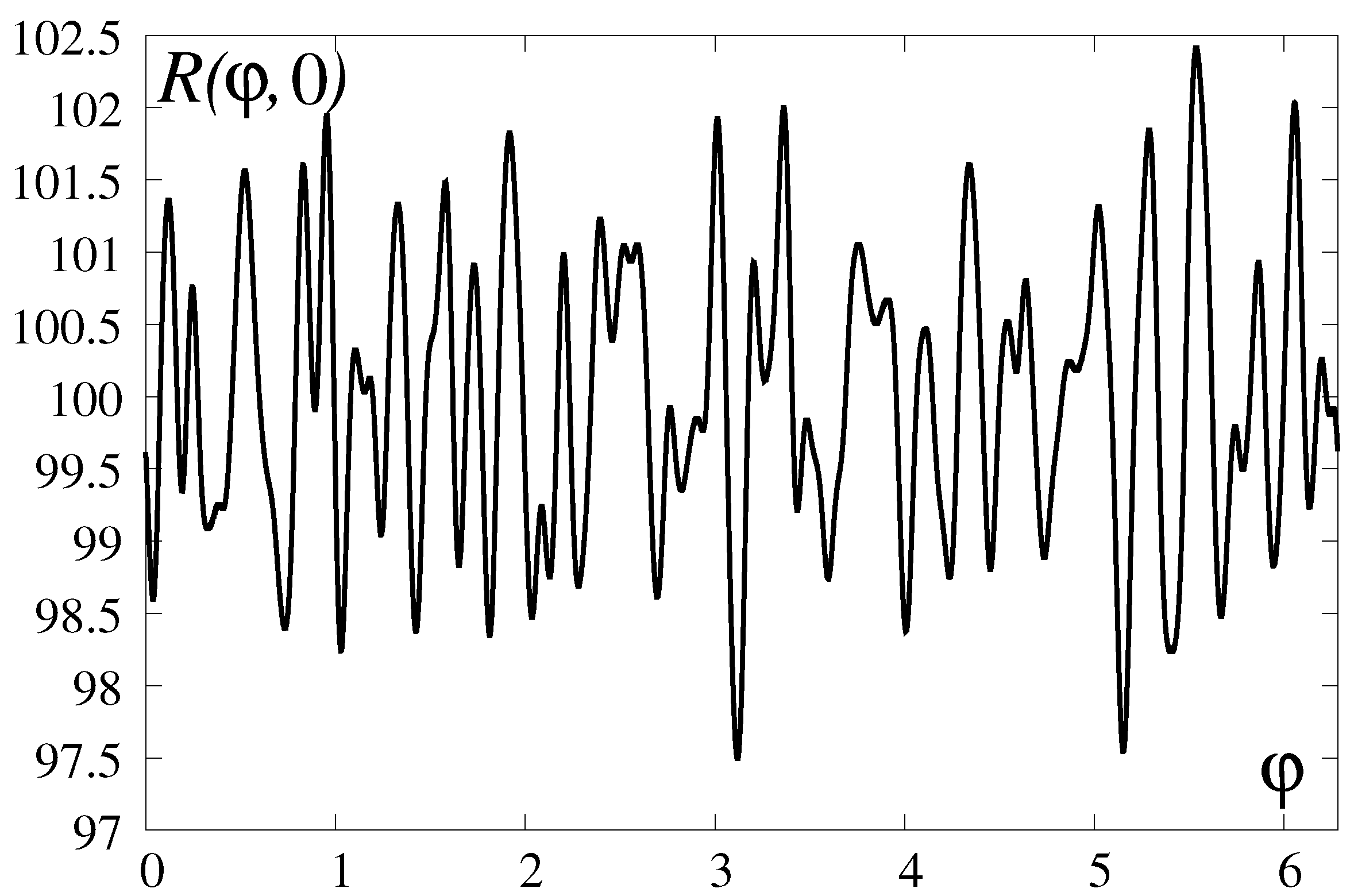
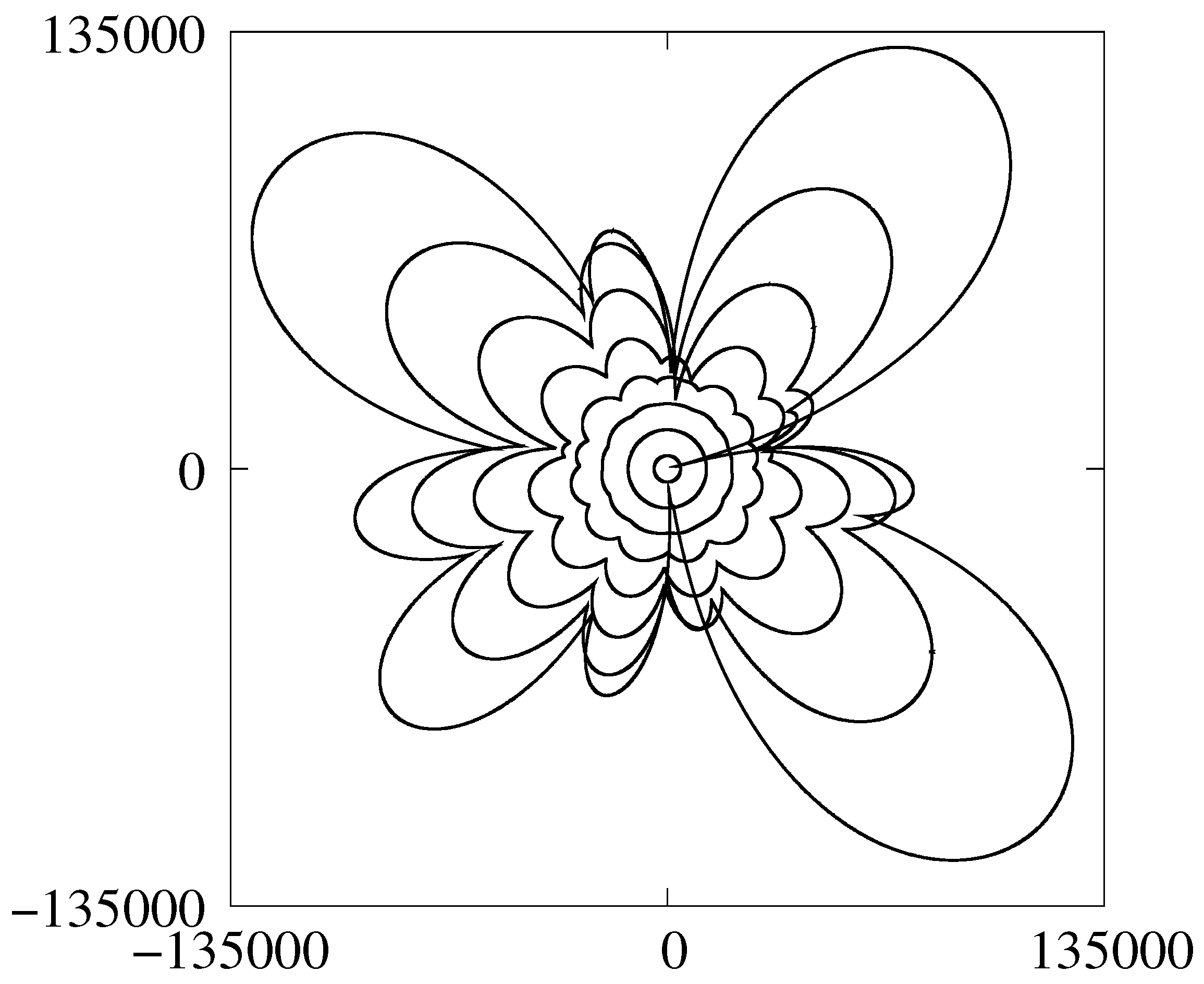
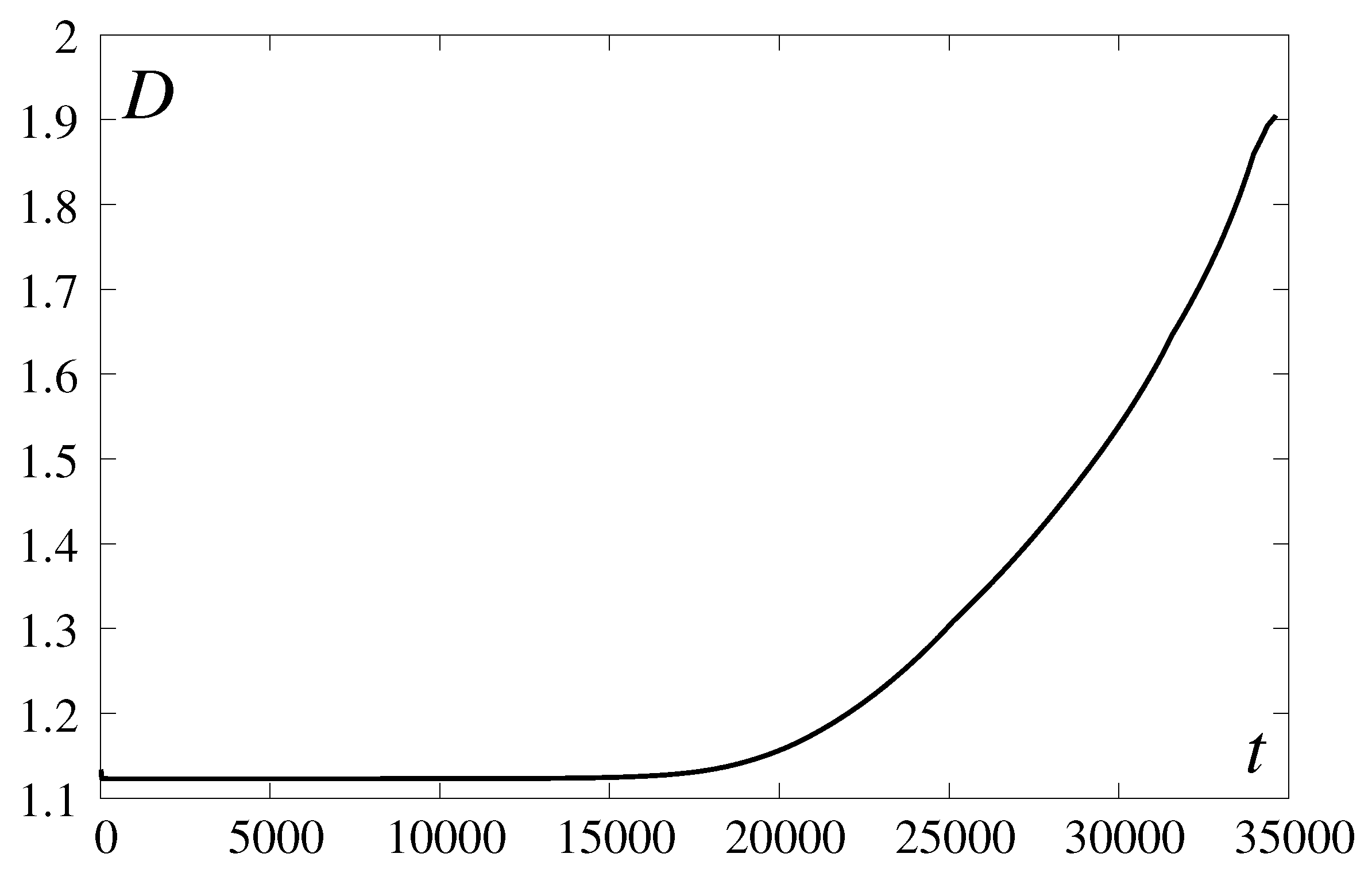
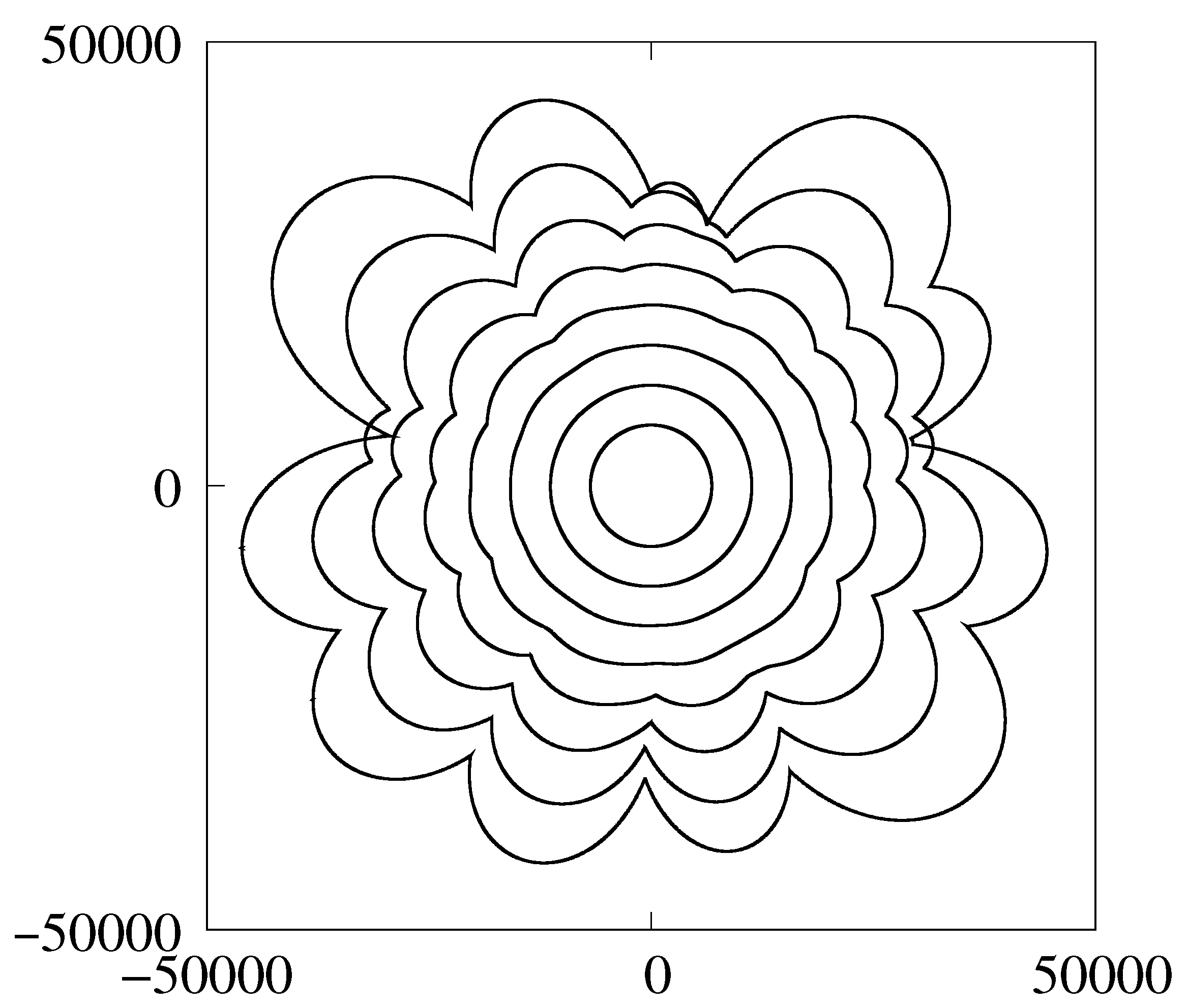
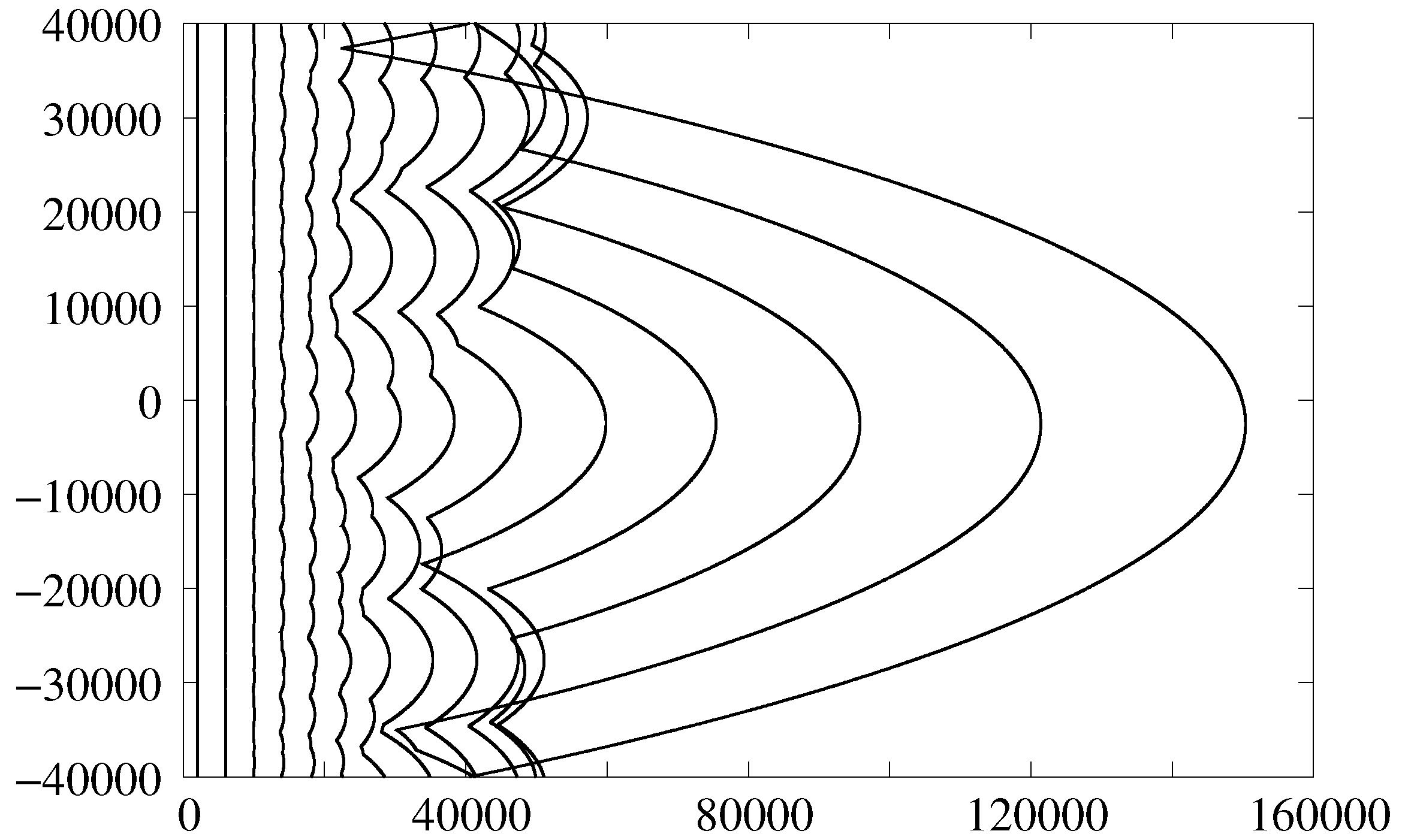
- The proposed weakly nonlinear models are certainly unable to capture the full morphology of the RT-mushrooming [7]. Yet, the models proved adequate enough to imitate the buoyancy-induced corrugations, the inverse cascade, self-acceleration of the front, and occurrence of the deflagrability threshold—the precursor of DDT.
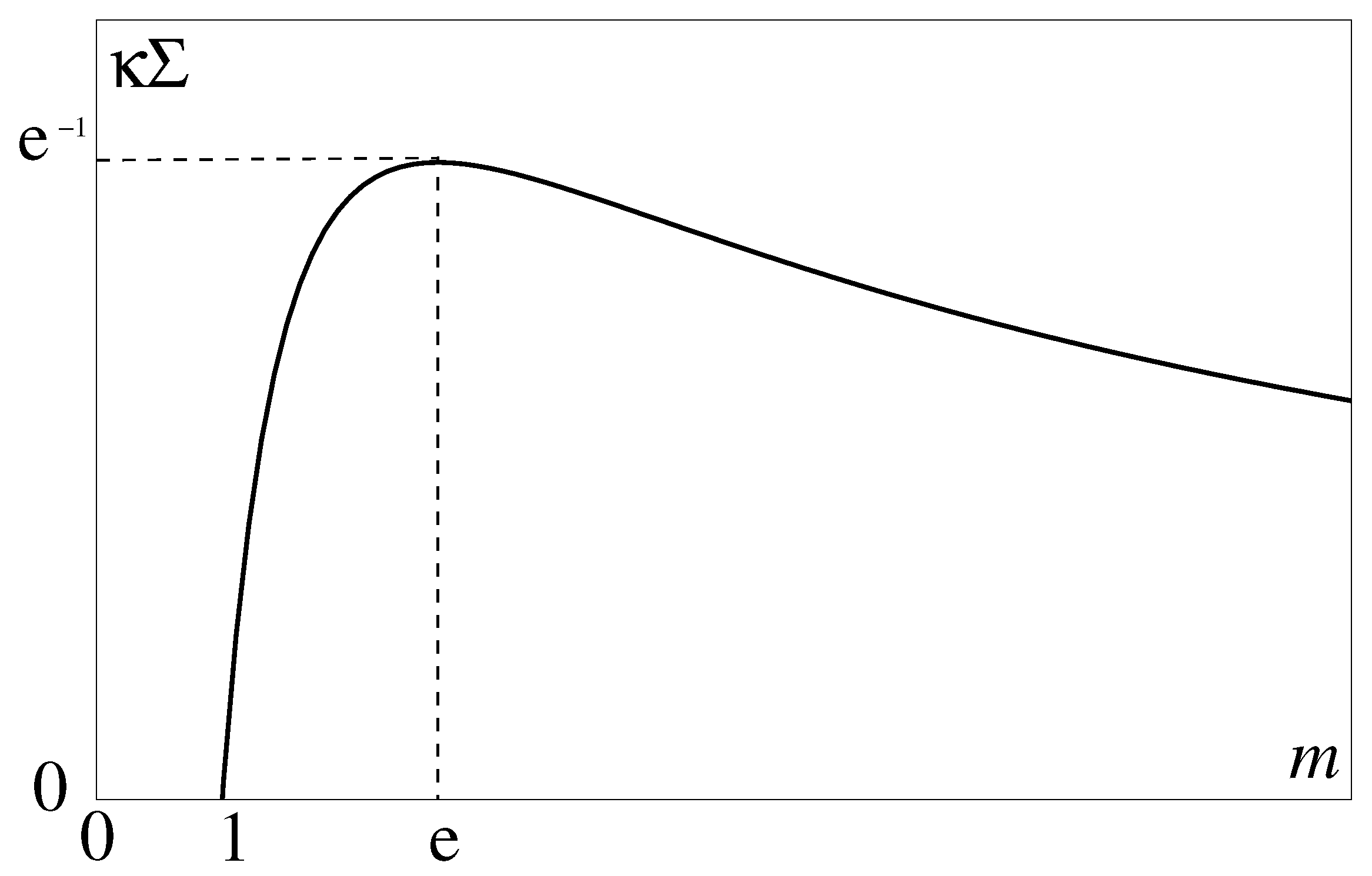
- Due to the constancy of the buoyancy factor kept at , the spatio-temporal scales and are likely to be grossly underestimated. Accounting for G vanishing at is expected to yield much larger numbers.
- Our preliminary exploration of the problem for one-step nuclear reaction kinetics and the equation of state for the degenerate electron gas shows that in this case the structure of the compressibility parameter is much more involved. This, however, does not affect the form of Equation (16) and the associated dynamical picture.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Röpke, F.K. Combustion in thermonuclear supernovae explosions. In Handbook of Supernovae; Springer: Cham, Switzerland, 2017. [Google Scholar]
- Oran, E.S. Astrophysical combustion. Proc. Combust. Inst. 2005, 30, 1823–1840. [Google Scholar] [CrossRef]
- Deshaies, B.; Joulin, G. Flame-speed sensitivity to temperature changes and the deflagration-to-detonation transition. Combust. Flame 1989, 77, 201–212. [Google Scholar] [CrossRef]
- Timmes, F.X.; Woosley, S.E. The conductive propagation of nuclear flames, I. Degenerate C+O and O+Ne+Mg white dwarfs. Astrophys. J. 1992, 396, 649–667. [Google Scholar] [CrossRef]
- Bychkov, V.V.; Liberman, M.A. Thermal instability and pulsations of the flame front in white dwarfs. Astrophys. J. 1995, 451, 711–716. [Google Scholar] [CrossRef]
- Poludnenko, A.Y.; Chambers, J.; Ahmed, K.; Gamezo, V.N.; Taylor, B.D. A unified mechanism for unconfined deflagration-to-detonation transition in terrestrial chemical systems and type Ia supernovae. Science 2019, 366, eaau 7365. [Google Scholar] [CrossRef] [PubMed]
- Vladimirova, N.; Rosner, R. Model flames in the Boussinesq limit: The effect of feedback. Phys. Rev. E 2003, 67, 0066305. [Google Scholar] [CrossRef] [PubMed]
- Koksharov, A.; Bykov, V.; Kagan, L.; Sivashinsky, G. Deflagration-to-detonation transition in an unconfined space. Combust. Flame 2018, 195, 163–169. [Google Scholar] [CrossRef]
- Gordon, P.V.; Kagan, L.; Sivashinsky, G. Parametric transition from deflagration to detonation revisited: Planar geometry. Combust. Flame 2020, 211, 465–476. [Google Scholar] [CrossRef]
- Koksharov, A.; Kagan, L.; Sivashinsky, G. Deflagration-to-detonation transition in an unconfined space: Expanding hydrogen-oxygen flames. Proc. Combust. Inst. 2020, 38. to appear. [Google Scholar] [CrossRef]
- Zeldovich, Y.B.; Barenblatt, G.I.; Librovich, V.B.; Makhviladze, G.M. The Mathematical Theory of Combustion and Explosions; Plenum: New York, NY, USA, 1985. [Google Scholar]
- Sivashinsky, G.I. Nonlinear analysis of hydrodynamic instability in laminar flames. Part 1. Derivation of basic equations. Acta Astronaut. 1977, 4, 1177–1206. [Google Scholar] [CrossRef]
- Michelson, D.M.; Sivashinsky, G.I. Nonlinear analysis of hydrodynamic instability in laminar flames. Part 2. Numerical experiments. Acta Astronaut. 1977, 4, 1207–1221. [Google Scholar] [CrossRef]
- Filyand, L.; Sivashinsky, G.I.; Frankel, M.L. On self-acceleration of outward propagating wrinkled flames. Phys. D Nonlinear Phenom. 1994, 72, 110–118. [Google Scholar] [CrossRef]
- Kagan, L.; Sivashinsky, G. Modelling of outward propagating flames subjected to Darrieus-Landau and Rayleigh-Taylor instabilities: Transition to detonation. Combust. Theory Model. 2020. submitted. [Google Scholar]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kagan, L.; Sivashinsky, G. An Elementary Model for a Self-Accelerating Outward Propagating Flame Subject to the Rayleigh–Taylor Instability: Transition to Detonation. Fluids 2020, 5, 196. https://doi.org/10.3390/fluids5040196
Kagan L, Sivashinsky G. An Elementary Model for a Self-Accelerating Outward Propagating Flame Subject to the Rayleigh–Taylor Instability: Transition to Detonation. Fluids. 2020; 5(4):196. https://doi.org/10.3390/fluids5040196
Chicago/Turabian StyleKagan, Leonid, and Gregory Sivashinsky. 2020. "An Elementary Model for a Self-Accelerating Outward Propagating Flame Subject to the Rayleigh–Taylor Instability: Transition to Detonation" Fluids 5, no. 4: 196. https://doi.org/10.3390/fluids5040196
APA StyleKagan, L., & Sivashinsky, G. (2020). An Elementary Model for a Self-Accelerating Outward Propagating Flame Subject to the Rayleigh–Taylor Instability: Transition to Detonation. Fluids, 5(4), 196. https://doi.org/10.3390/fluids5040196




