Numerical Simulation of Breathing Mode Oscillation on Bubble Detachment
Abstract
1. Introduction
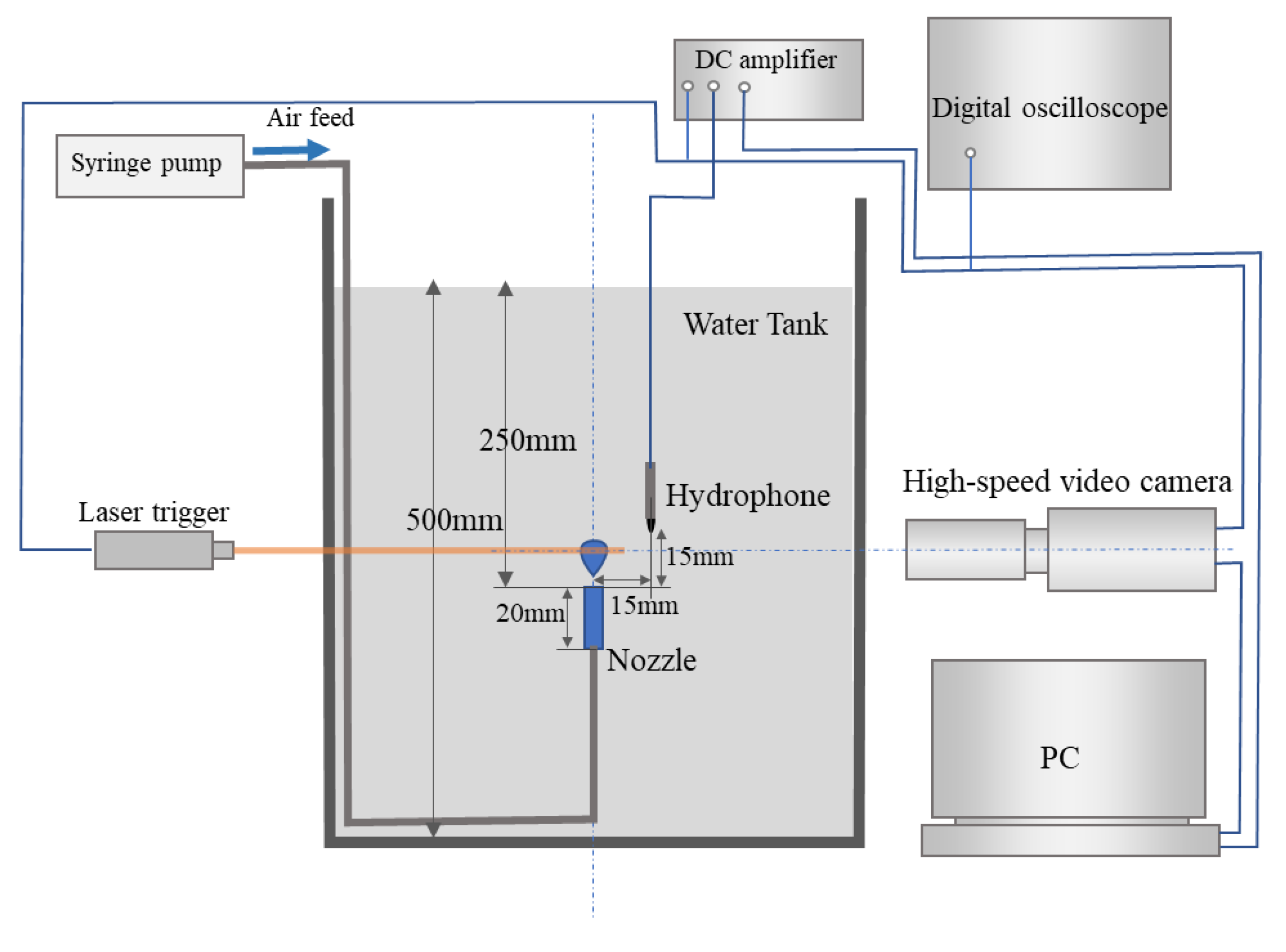
2. Experiment
2.1. Experimental Apparatus
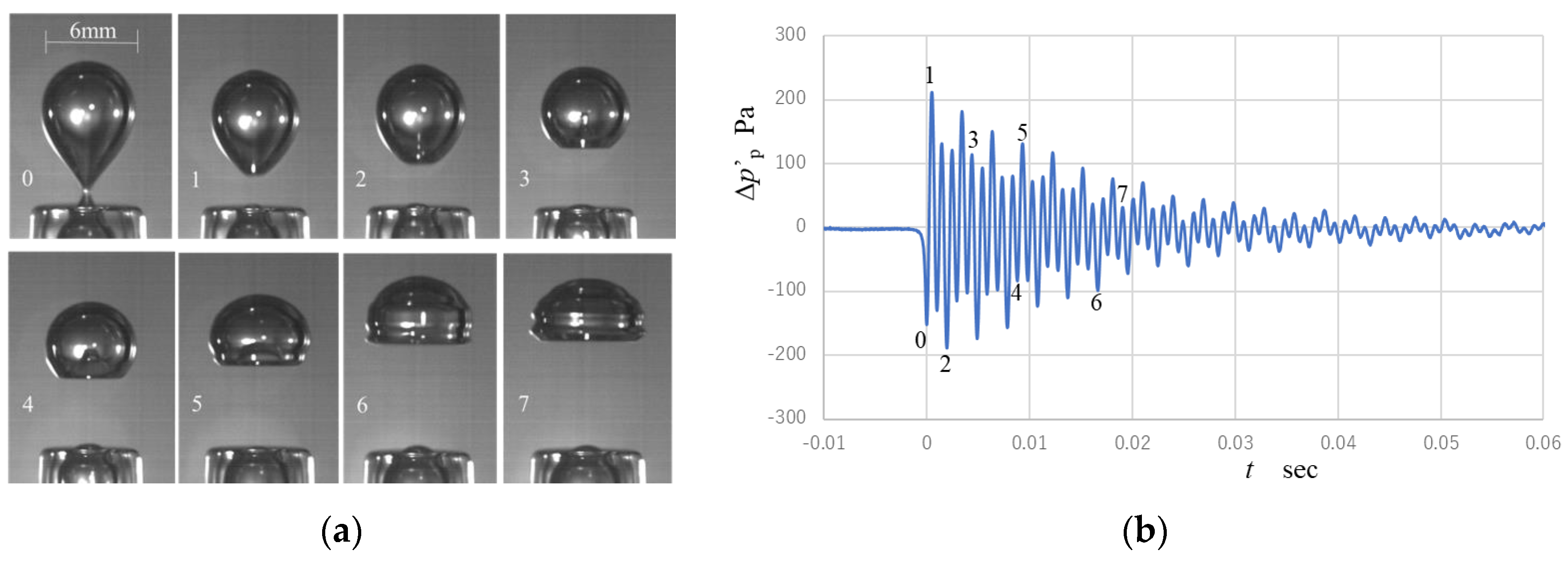
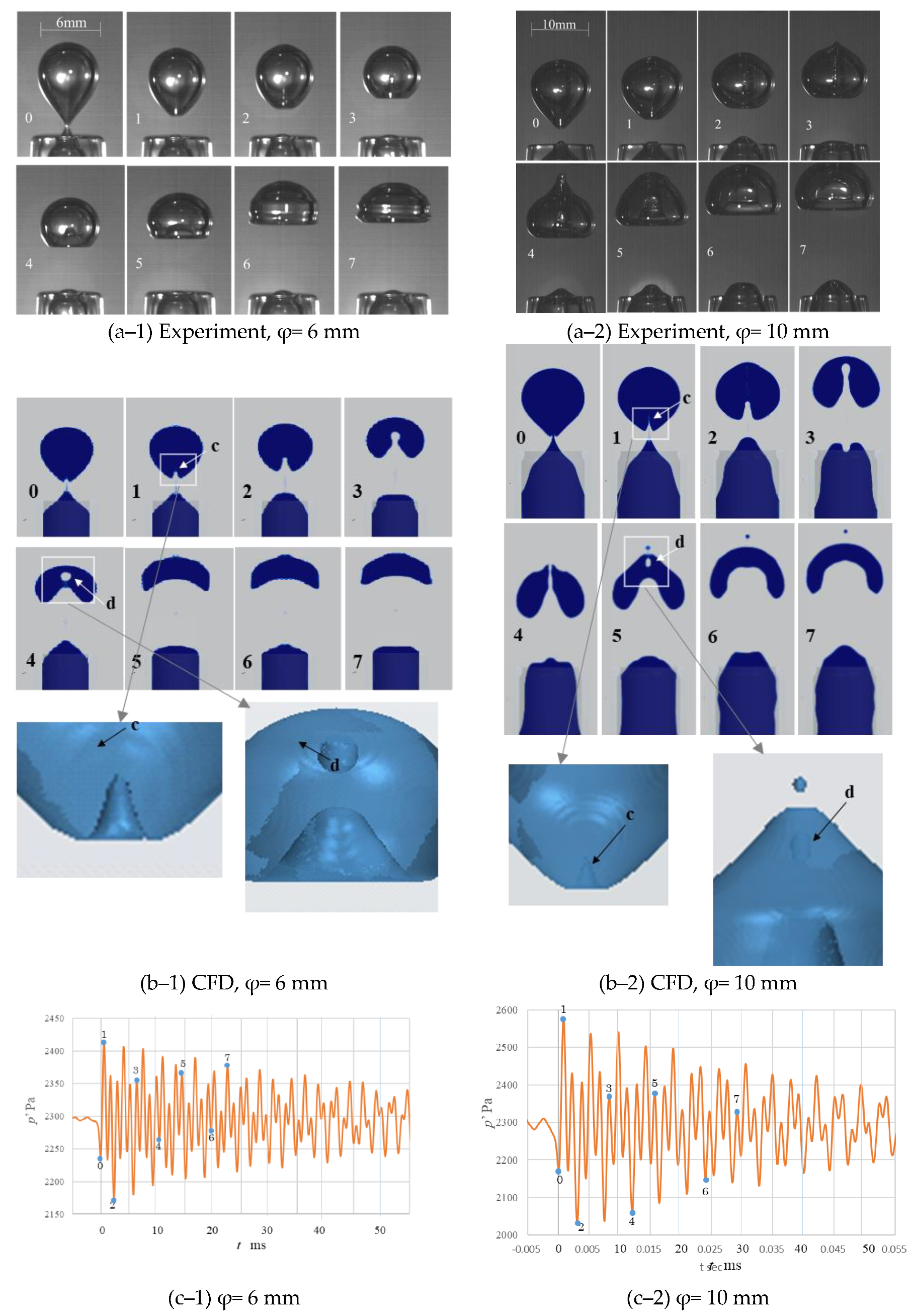
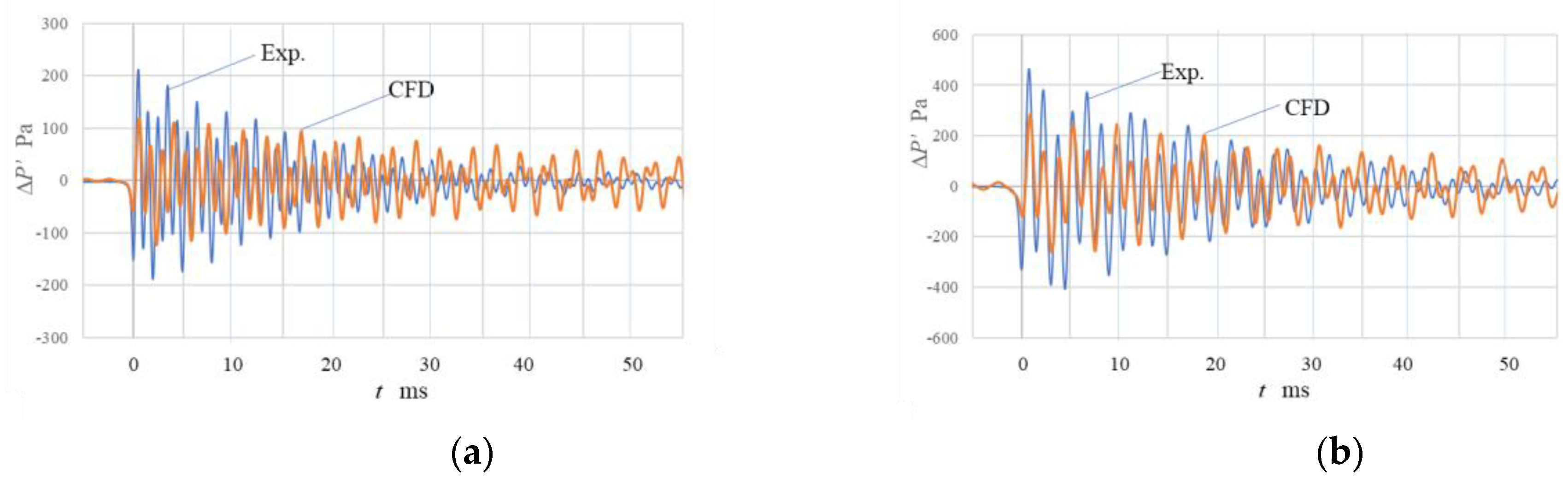
2.2. Overview of Experimental Results
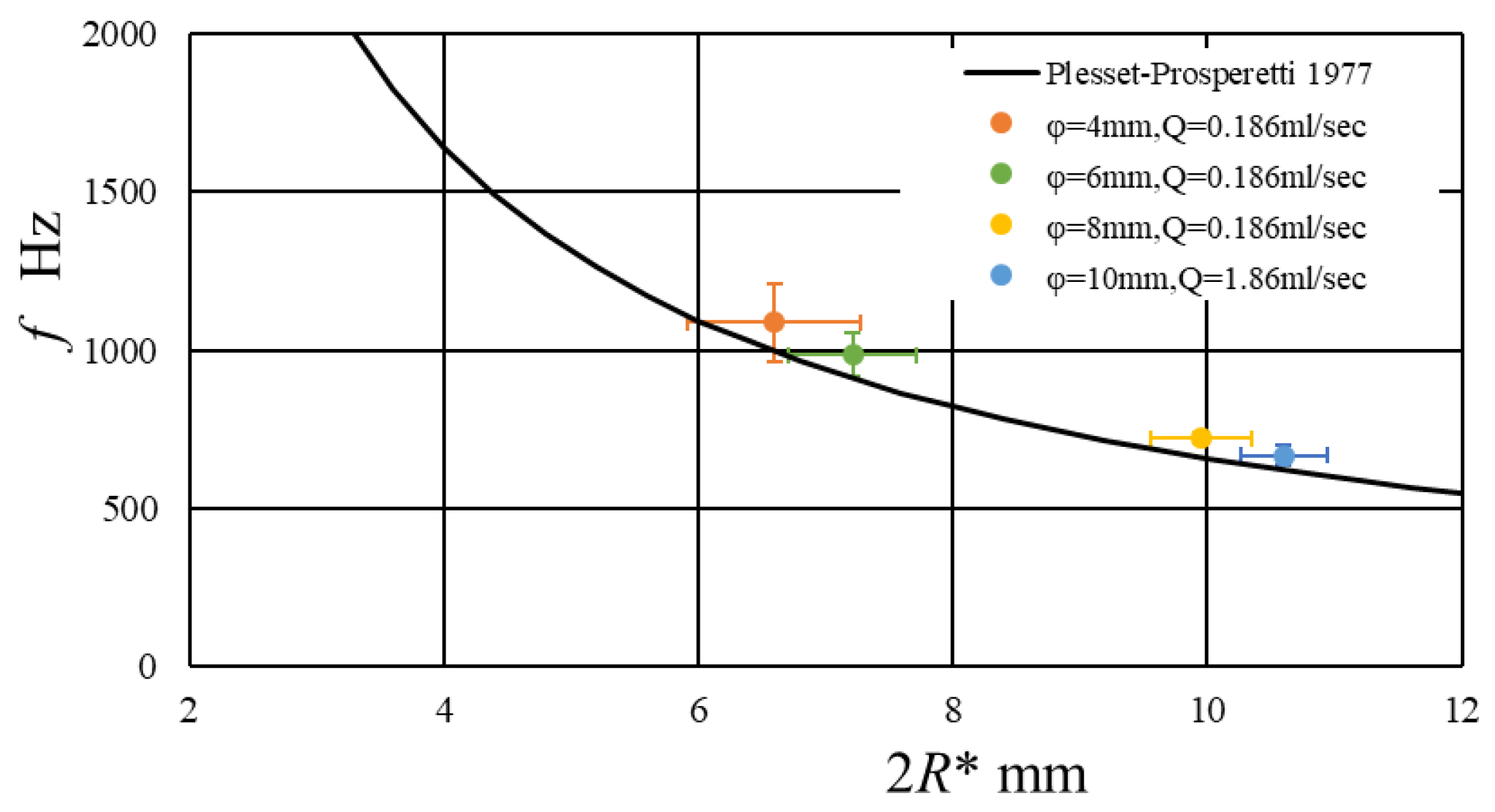
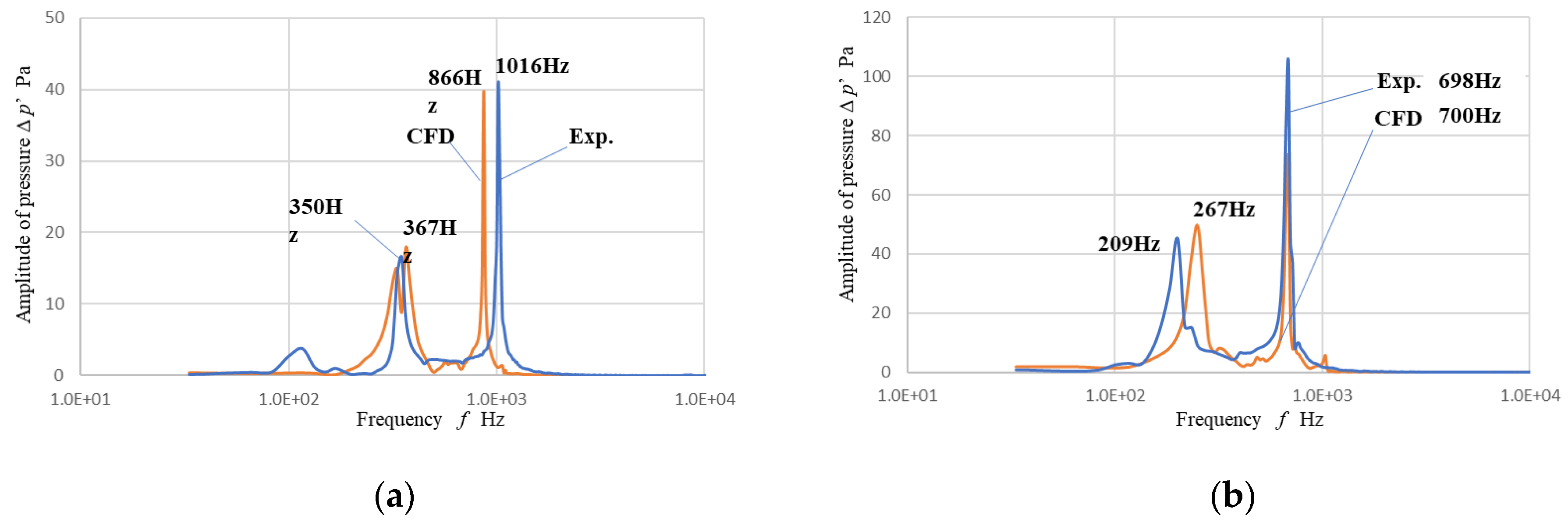
3. Vibrational Motion in the Breathing Mode
4. Computational Fluid Dynamics Simulation
4.1. Governing Equation and Scheme
4.2. Computational Parameters and Mesh Validation
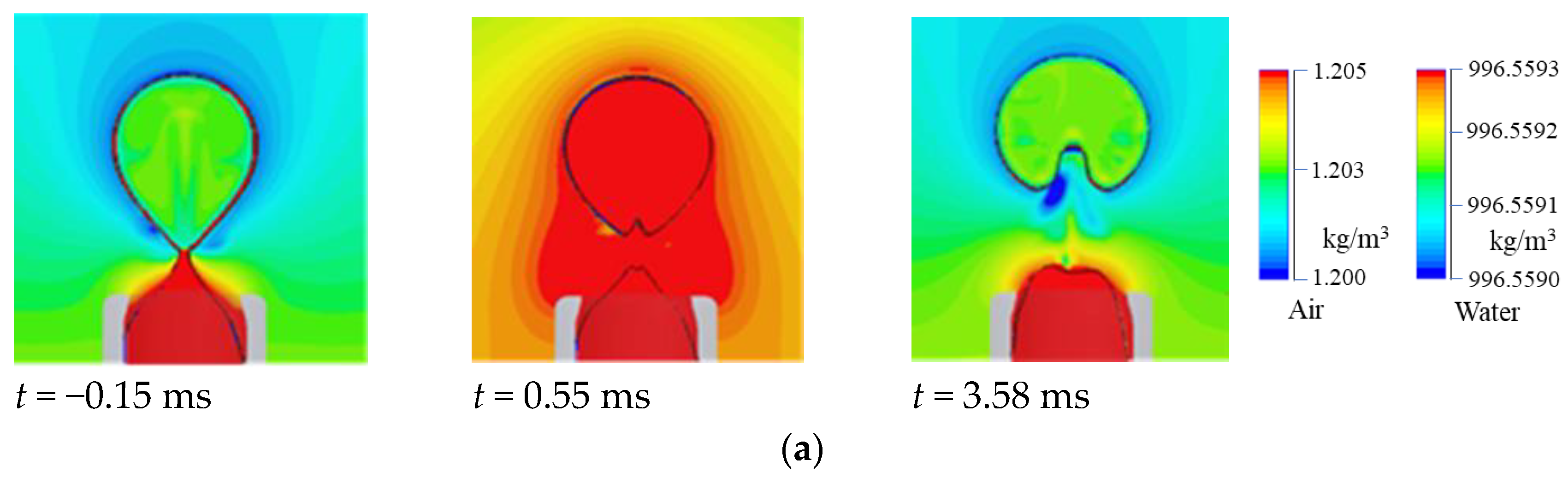
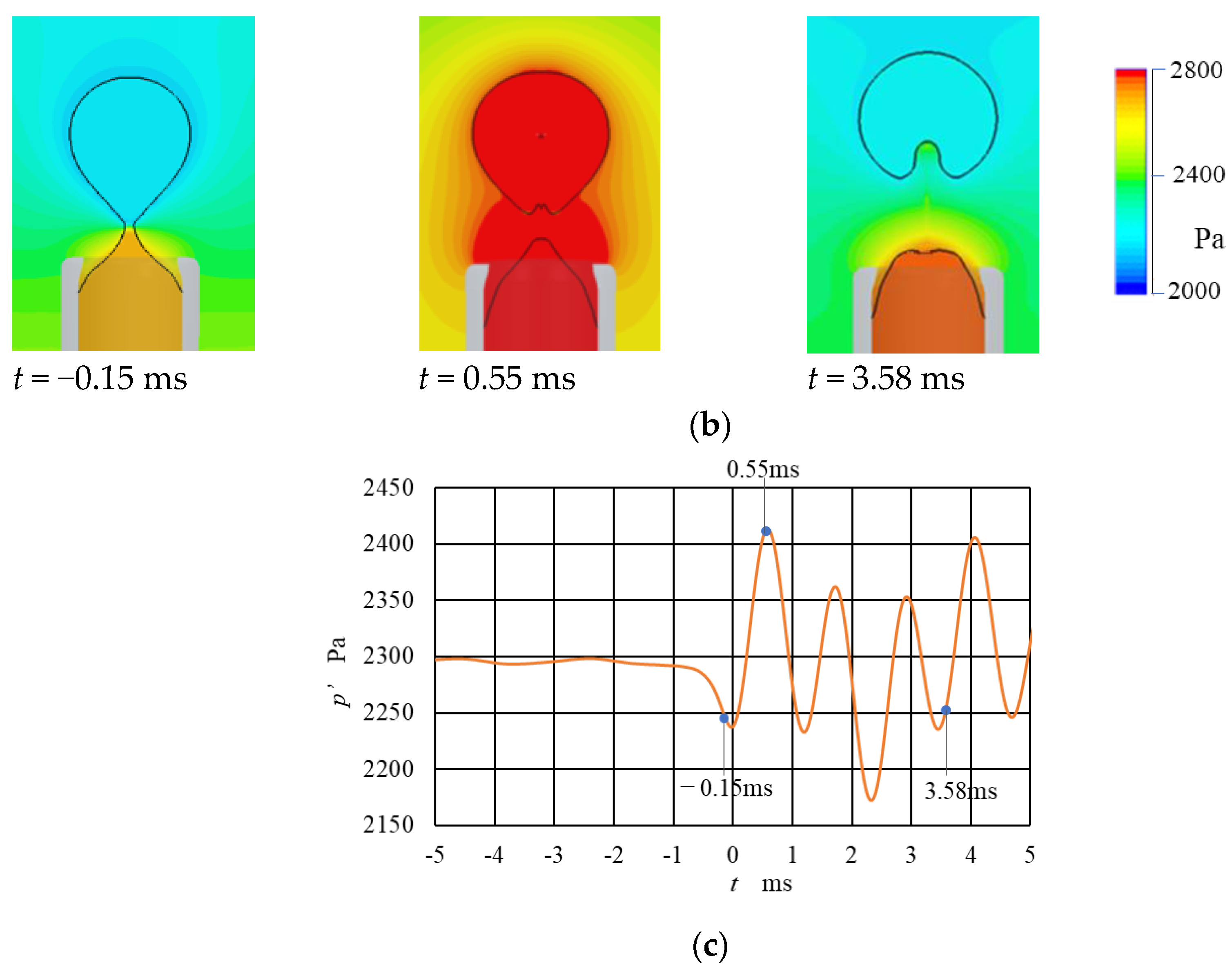
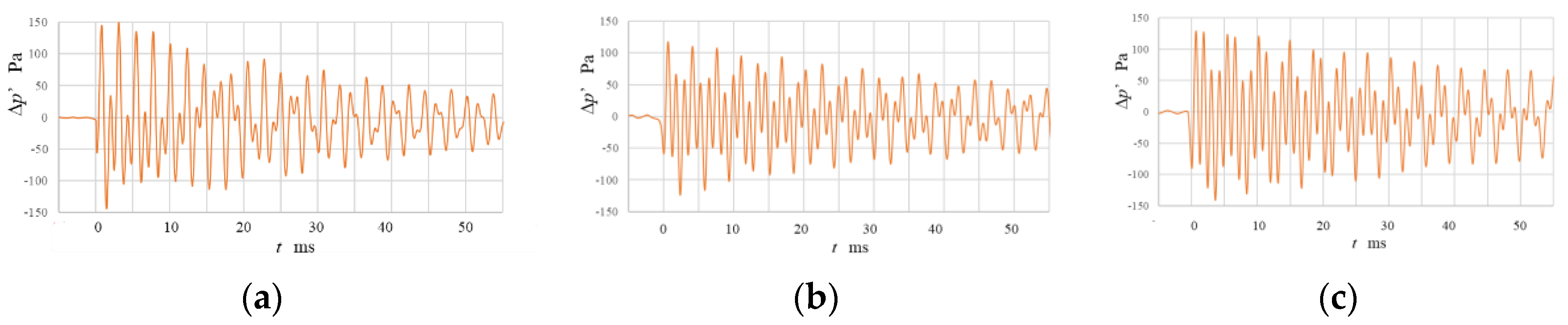
4.3. Overview of Density and Pressure Change
4.4. Deformation and Pressure Diagram for Long-Term
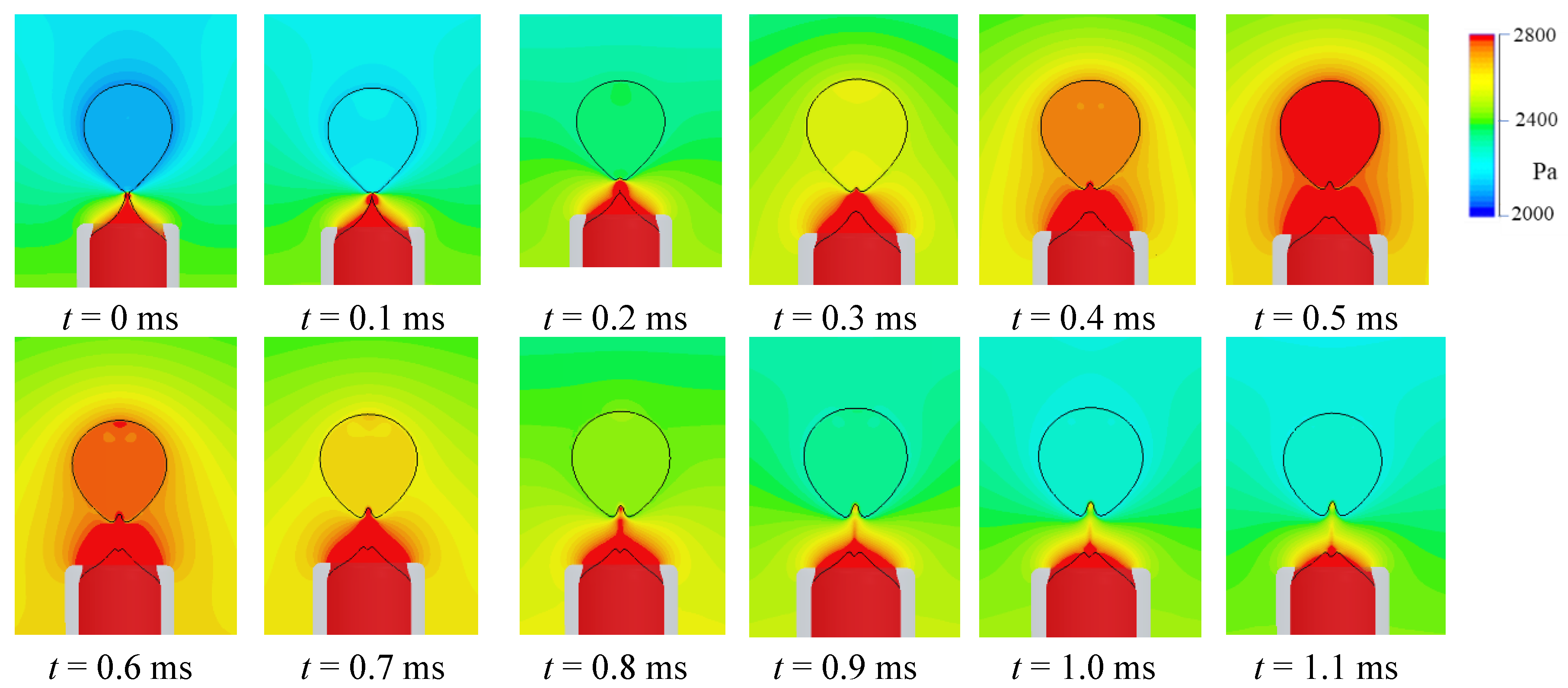
4.5. Pressure Distribution for One Cycle
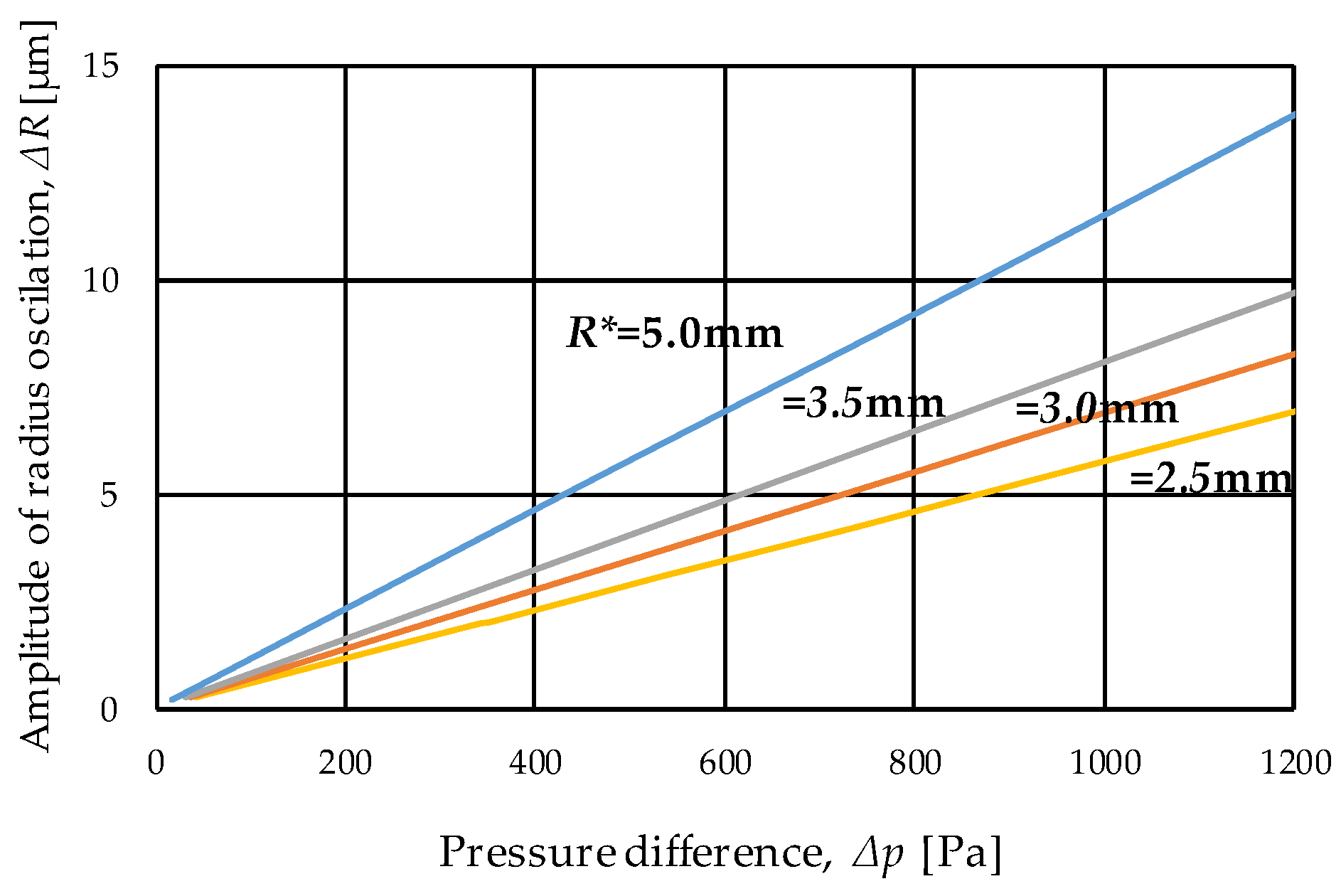
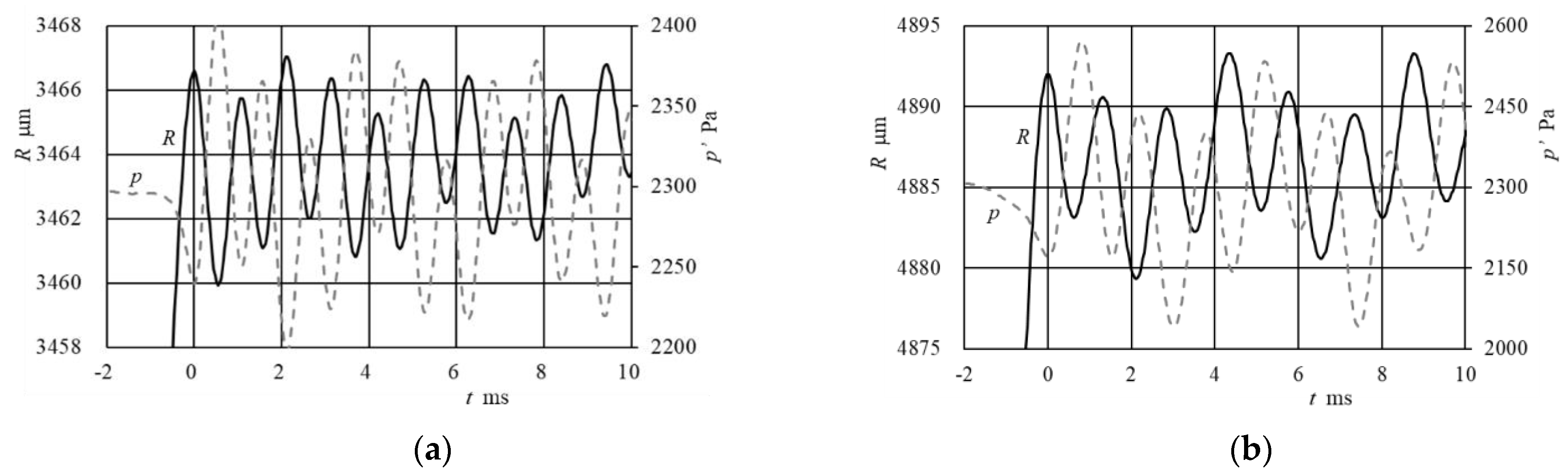
4.6. Radial Variation in the Breathing Mode
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| Eötvös number | |
| L | length of the nozzle |
| Q | air flow rate |
| equilibrium radius of the bubble | |
| φ | inner diameter of the nozzle |
| Reynolds number | |
| distance between the bubble center and hydrophone | |
| frequency | |
| gravitational acceleration | |
| ambient pressure | |
| pressure drop near the bubble | |
| pressure drop measured by the hydrophone | |
| air density | |
| water density | |
| rising velocity of the bubble | |
| γ | polytropic exponent |
| viscosity coefficient of water. | |
| σ | surface tension of water |
| κ | specific heat ratio of air |
References
- Watanabe, Y. Analytical study of acoustic mechanism of “suikinkutsu”. Jpn. J. Appl. Phys. Part 1 Regul. Pap. Short Notes Rev. Pap. 2004, 43, 6429–6443. [Google Scholar] [CrossRef]
- Oku, T.; Hirahara, H.; Akimoto, T. Visualization of deformation and sound emission from bubble in water using VOF method. In Proceedings 18th International Symposium on Flow Visualization; ETH: Zurich, Switzerland, 2018; pp. 1–9. [Google Scholar]
- Rayleigh, L., VIII. On the pressure developed in a liquid during the collapse of a spherical cavity. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1917, 34, 94–98. [Google Scholar] [CrossRef]
- Minnaert, M. On musical air-bubbles and the sounds of running water. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1933, 16, 235–248. [Google Scholar] [CrossRef]
- Plesset, M.S.; Prosperetti, A. Bubble dynamics and cavitation. Annu. Rev. Fluid Mech. 1977, 9, 145–185. [Google Scholar] [CrossRef]
- Strasberg, M. Gas Bubbles as Sources of Sound in Liquids. J. Acoust. Soc. Am. 1956, 28, 20–26. [Google Scholar] [CrossRef]
- Longuet-Higgins, M.S. Monopole emission of sound by asymmetric bubble oscillations. Part 1. Normal modes. J. Fluid Mech. 1989, 201, 525–541. [Google Scholar] [CrossRef]
- Longuet-Higgins, M.S. Monopole emission of sound by asymmetric bubble oscillations. Part 2. An initial-value problem. J. Fluid Mech. 1989, 201, 543–565. [Google Scholar] [CrossRef]
- Longuet-Higgins, M.S.; Lunde, K. The release of air bubbles from an underwater nozzle. J. Fluid Mech. 1991, 230, 365–390. [Google Scholar] [CrossRef]
- Oguz, H.N.; Prosperetti, A. Dynamics of Bubble Growth and Detachment from a Needle. J. Fluid Mech. 1993, 257, 111–145. [Google Scholar] [CrossRef]
- Oğuz, H.N.; Prosperetti, A. The natural frequency of oscillation of gas bubbles in tubes. J. Acoust. Soc. Am. 1998, 103, 3301–3308. [Google Scholar] [CrossRef]
- Leighton, T.G. The Freely-oscillating Bubble. In The Acoustic Bubble; Academic Press: London, UK, 1994; pp. 129–286. [Google Scholar]
- Devaud, M.; Hocquet, T.; Bacri, J.; Leroy, V.; Devaud, M.; Hocquet, T.; Bacri, J.; Leroy, V. The Minnaert bubble: A new approach. To cite this version: HAL Id: Hal-00145867. Available online: https://hal.archives-ouvertes.fr/hal-00145867 (accessed on 14 May 2007).
- Manasseh, R.; Riboux, G.; Bui, A.; Risso, F. Sound emission on bubble coalescence: Imaging, acoustic and numerical experiments. In Proceedings of the 16th Australian Fluid Mechanics Conference, 16AFMC, Crown Plaza, Gold Coast, Australia, 2–7 December 2007; pp. 167–173. [Google Scholar]
- Czerski, H.; Deane, G.B. Contributions to the acoustic excitation of bubbles released from a nozzle. J. Acoust. Soc. Am. 2010, 128, 2625–2634. [Google Scholar] [CrossRef] [PubMed]
- Phillips, S.; Agarwal, A.; Jordan, P. The Sound Produced by a Dripping Tap is Driven by Resonant Oscillations of an Entrapped Air Bubble. Sci. Rep. 2018, 8, 1–12. [Google Scholar] [CrossRef] [PubMed]
- di Bari, S.; Robinson, A.J. Experimental study of gas injected bubble growth from submerged orifices. Exp. Therm. Fluid Sci. 2013, 44, 124–137. [Google Scholar] [CrossRef]
- Van Sint Annaland, M.; Deen, N.G.; Kuipers, J.A.M. Numerical simulation of gas bubbles behaviour using a three-dimensional volume of fluid method. Chem. Eng. Sci. 2005, 60, 2999–3011. [Google Scholar] [CrossRef]
- Quan, S.; Hua, J. Numerical studies of bubble necking in viscous liquids. Phys. Rev. E Stat. Nonlinear Soft Matter Phys. 2008, 77, 1–11. [Google Scholar] [CrossRef]
- Hanafizadeh, P.; Eshraghi, J.; Kosari, E.; Ahmed, W.H. The effect of gas properties on bubble formation, growth, and detachment. Part. Sci. Technol. 2015, 33, 645–651. [Google Scholar] [CrossRef]
- Ambrose, S.; Hargreaves, D.M.; Lowndes, I.S. Numerical modeling of oscillating Taylor bubbles. Eng. Appl. Comput. Fluid Mech. 2016, 10, 578–598. [Google Scholar] [CrossRef]
- Xi, W.Q. Numerical simulation of violent bubble motion. Phys. Fluids 2004, 16, 1610–1619. [Google Scholar] [CrossRef]
- Bui, A.; Manasseh, R. A CFD Study of the Bubble Deformation during Detachment. In Proceedings of the Fifth International Conference on CFD in the Process Industries, CSIRO, Melbourne, Australia, 13–15 December 2006; pp. 1–6. [Google Scholar]
- Liu, J.; Chu, N.; Qin, S.; Wu, D. Acoustic analysis on jet-bubble formation based on 3D numerical simulations. In Proceedings of the INTER-NOISE 2016—45th International Congress and Exposition on Noise Control Engineering: Towards a Quieter Future, Hamburg, Germany, 21–24 August 2016; pp. 5103–5111. [Google Scholar]
- Liu, J.; Chu, N.; Qin, S.; Wu, D. Numerical simulations of bubble formation and acoustic characteristics from a submerged orifice: The effects of nozzle wall configurations. Chem. Eng. Res. Des. 2017, 123, 130–140. [Google Scholar] [CrossRef]
- Clift, R.; Grace, J.R.; Weber, M.E. Bubbles, Drops and Particles; Academic Press: Cambridge, MA, USA, 1978; Volume 61, ISBN 012176950X. [Google Scholar]
- Prosperetti, A. Bubble phenomena in sound fields: Part one. Ultrasonics 1984, 22, 69–77. [Google Scholar] [CrossRef]
- Vokurka, K. On Rayleigh’s model of a freely oscillating bubble. I. Basic relations. Czechoslov. J. Phys. 1985, 35, 28–40. [Google Scholar] [CrossRef]
- Muzaferija, S.; Peric, M. Computation of free-surface flows using interface-tracking and interface-capturing methods. In Nonlinear Water Waves Interaction; WIT Press: Alpha House, UK, 1999. [Google Scholar]
- Islam, M.T.; Ganesan, P.; Sahu, J.N.; Hamad, F.A. Numerical study to investigate the effect of inlet gas velocity and Reynolds number on bubble formation in a viscous liquid. Therm. Sci. 2015, 19, 2127–2138. [Google Scholar] [CrossRef][Green Version]
- Manasseh, R.; Riboux, G.; Risso, F. Sound generation on bubble coalescence following detachment. Int. J. Multiph. Flow 2008, 34, 938–949. [Google Scholar] [CrossRef]
- Leighton, T.G.; White, P.R.; Morfey, C.L.; Clarke, J.W.L.; Heald, G.J.; Dumbrell, H.A.; Holland, K.R. The effect of reverberation on the damping of bubbles. J. Acoust. Soc. Am. 2002, 112, 1366–1376. [Google Scholar] [CrossRef] [PubMed]
- Wu, W.B.; Liu, Y.L.; Zhang, A.M. Numerical investigation of 3D bubble growth and detachment. Ocean Eng. 2017, 138, 86–104. [Google Scholar] [CrossRef]


| φ mm | mm | m/s | Re | Eo |
|---|---|---|---|---|
| 4 | 3.2 | 0.24 | 7.7 × 102 | 1.39 |
| 6 | 3.5 | 0.19 | 6.6 × 102 | 1.66 |
| 8 | 4.8 | 0.17 | 8.1 × 102 | 3.13 |
| 10 | 5.0 | 0.17 | 8.5 × 102 | 3.39 |
| φ mm | R* mm | Images | d mm | Δp′p Pa | Δpp Pa | ΔR by Equation (4) μm |
|---|---|---|---|---|---|---|
| 4 | 3.2 |  | 18 | −86 | −480 | ±3.2 |
| 6 | 3.5 |  | 17 | −152 | −740 | ±5.7 |
| 8 | 4.8 |  | 16 | −270 | −900 | ±9.9 |
| 10 | 5.0 |  | 16 | −329 | −1050 | ±11.9 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Oku, T.; Hirahara, H.; Akimoto, T.; Tsuchida, D. Numerical Simulation of Breathing Mode Oscillation on Bubble Detachment. Fluids 2020, 5, 96. https://doi.org/10.3390/fluids5020096
Oku T, Hirahara H, Akimoto T, Tsuchida D. Numerical Simulation of Breathing Mode Oscillation on Bubble Detachment. Fluids. 2020; 5(2):96. https://doi.org/10.3390/fluids5020096
Chicago/Turabian StyleOku, Takao, Hiroyuki Hirahara, Tomohiro Akimoto, and Daiki Tsuchida. 2020. "Numerical Simulation of Breathing Mode Oscillation on Bubble Detachment" Fluids 5, no. 2: 96. https://doi.org/10.3390/fluids5020096
APA StyleOku, T., Hirahara, H., Akimoto, T., & Tsuchida, D. (2020). Numerical Simulation of Breathing Mode Oscillation on Bubble Detachment. Fluids, 5(2), 96. https://doi.org/10.3390/fluids5020096




