A 2D reference solution is hereafter considered, obtained with EUROPLEXUS software, a fast-transient dynamics code for fluids and structures, resolving the Euler equations introduced in
Section 3 for a transverse pressure wave propagating through a
array of rods. Thanks to this detailed local solution, it is possible to compute the wavelet coefficients of the reference pressure field and thus fully assess the accuracy of the filtering process on the computation of the relevant quantity of interest in our context, i.e., the resulting force acting on the structure, directly related to the accuracy of the reconstruction of the pressure gradient.
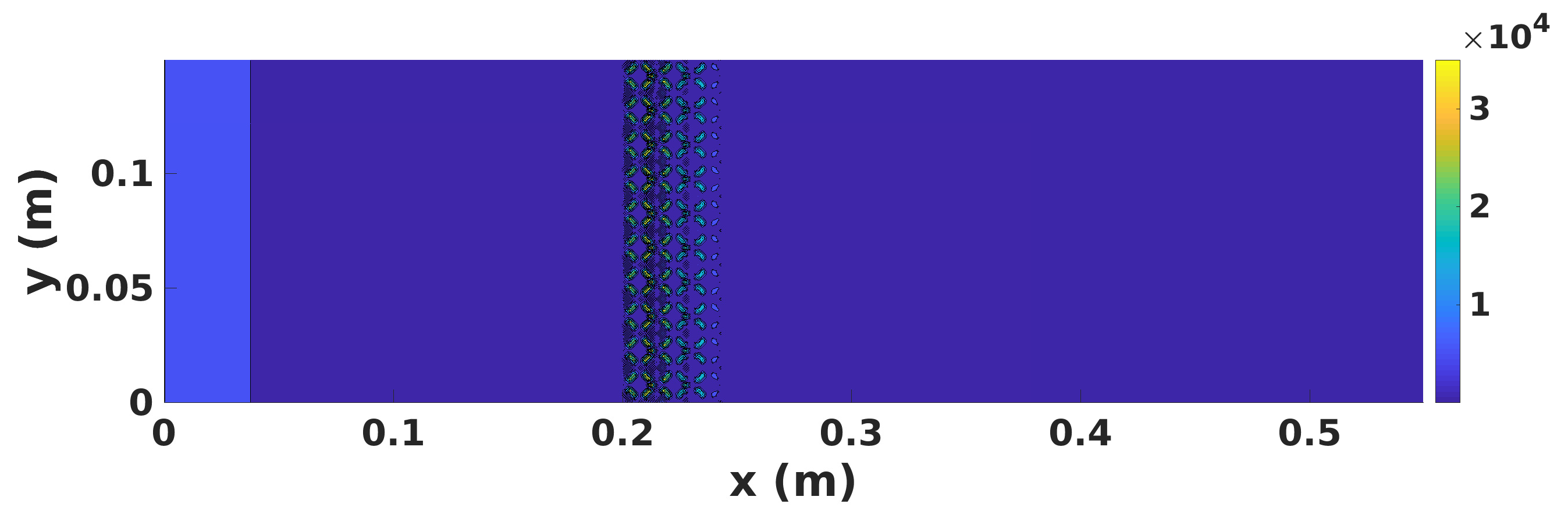
The simulation is designed as shown in
Figure 3: a 2D transverse pressure wave propagates through a rod bundle composed of
rods. As stated in the introduction, this test case can be seen as a simplified, yet representative, version of the actual pressure loading that would impact PWR fuel assemblies during a depressurization transient (known as loss of cooling accident, or LOCA).
As for the solid medium, the rods can here be considered as rigid bodies, whose centers are kinematically blocked, so that the sum of the reaction forces to the central blockages directly provides the force applied by the fluid to the microstructure.
The mesh size is set to
, where
is the rods’ radius. For the sake of convergence, finer mesh sizes of
,
and
are also computed. The spatial discretization of the equations is implemented through a finite element method for the solid medium (with 3-noded triangle elements), and a cell-centered finite volume scheme for the fluid (quadrangle elements, order 2 in time and space, HLL Riemann solver). An Euler explicit scheme is then used for the time discretization. The resulting pressure field is displayed in
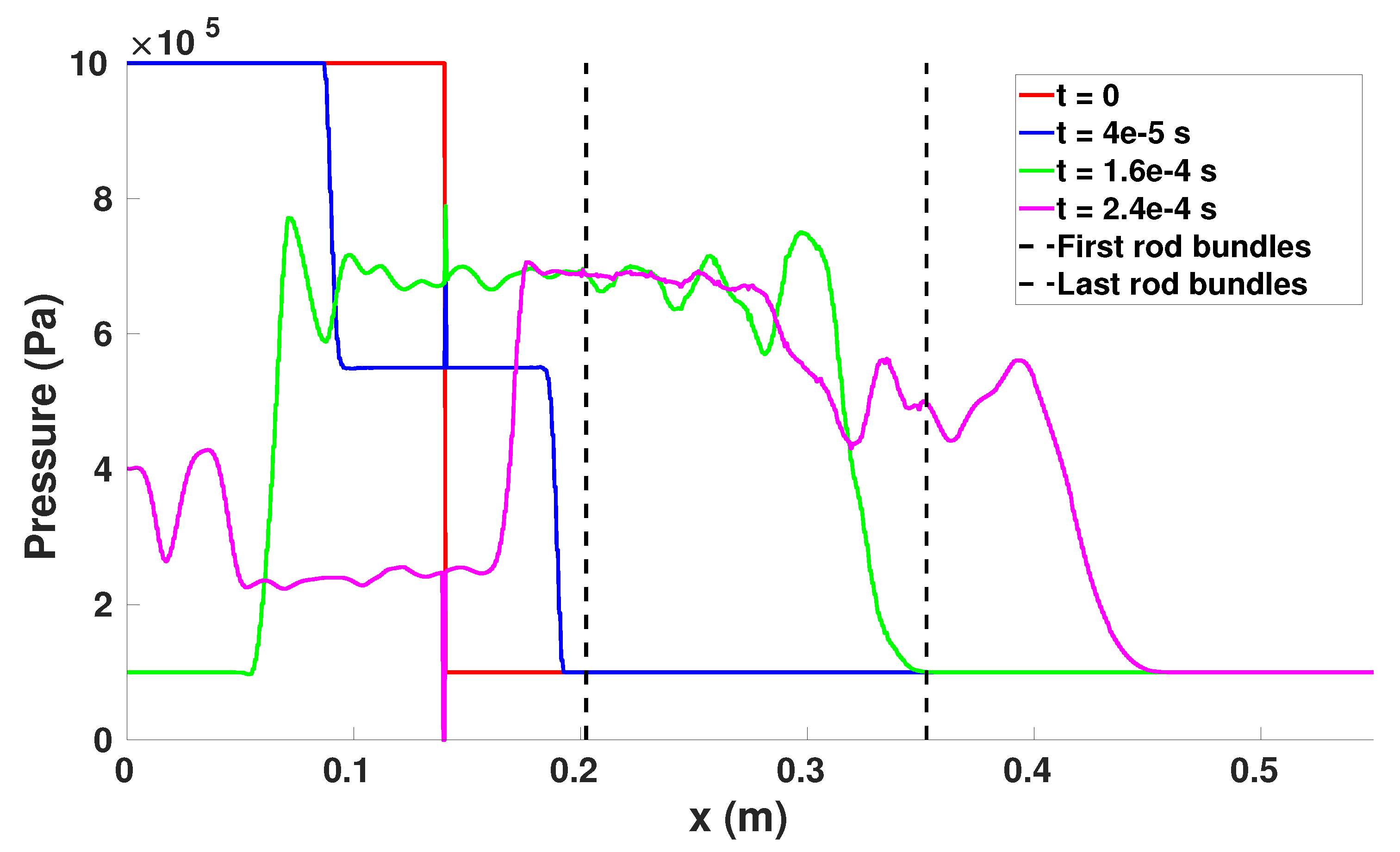
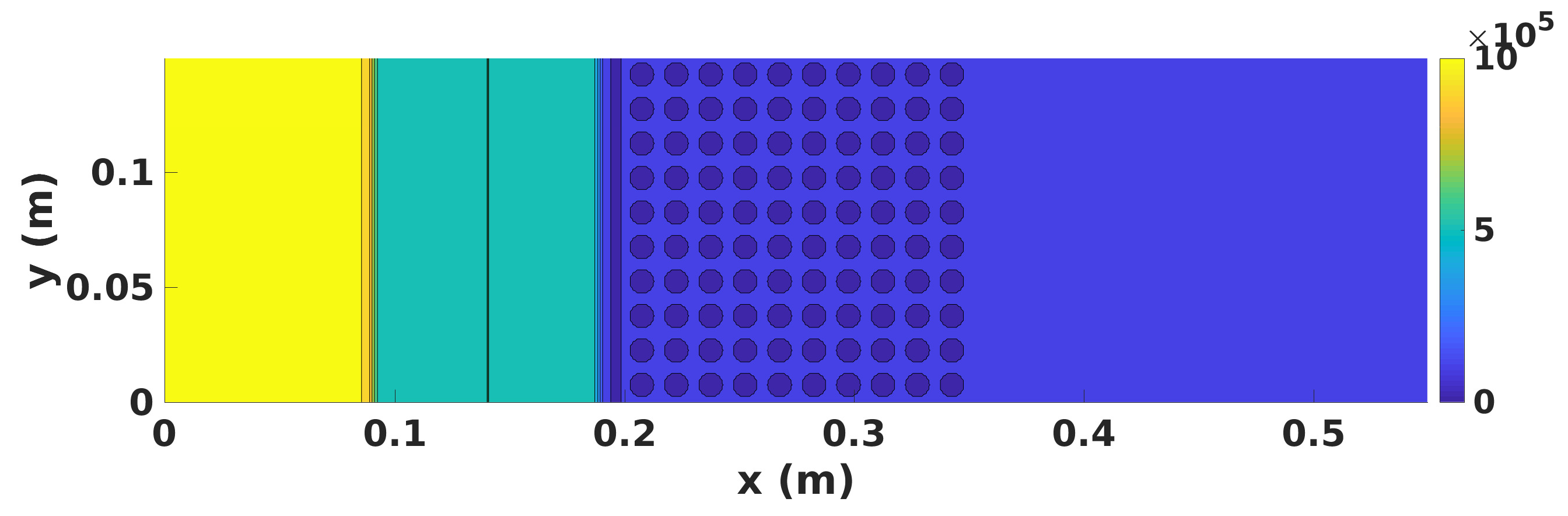
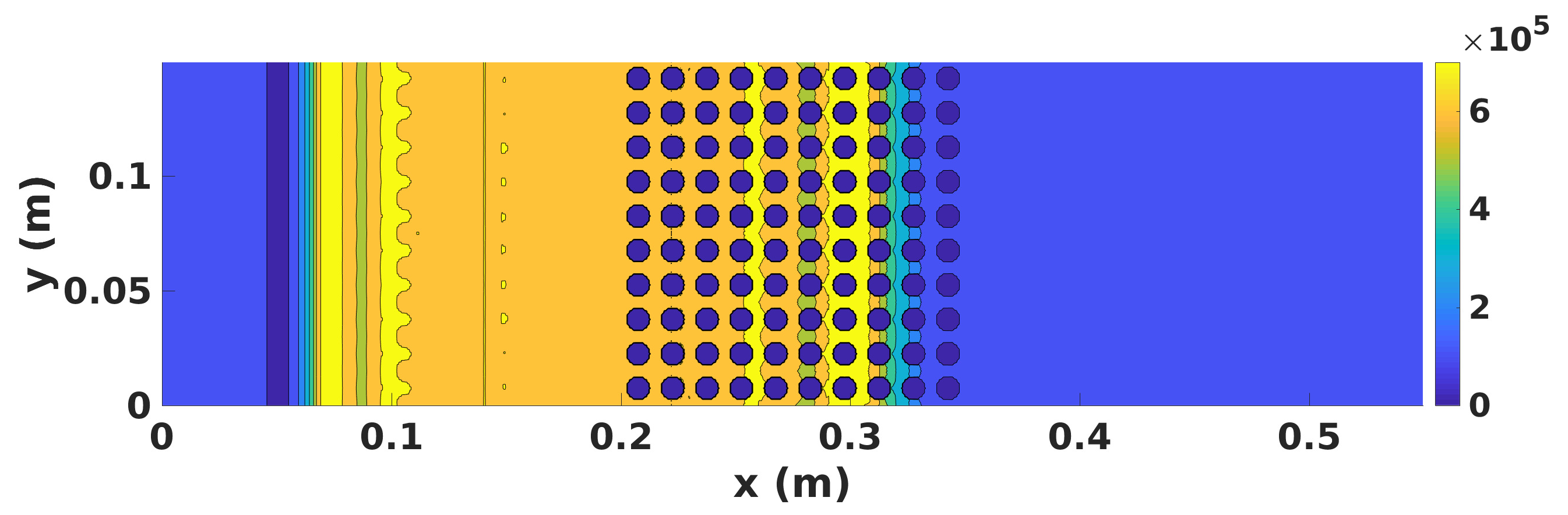
Figure 5,
Figure 6,
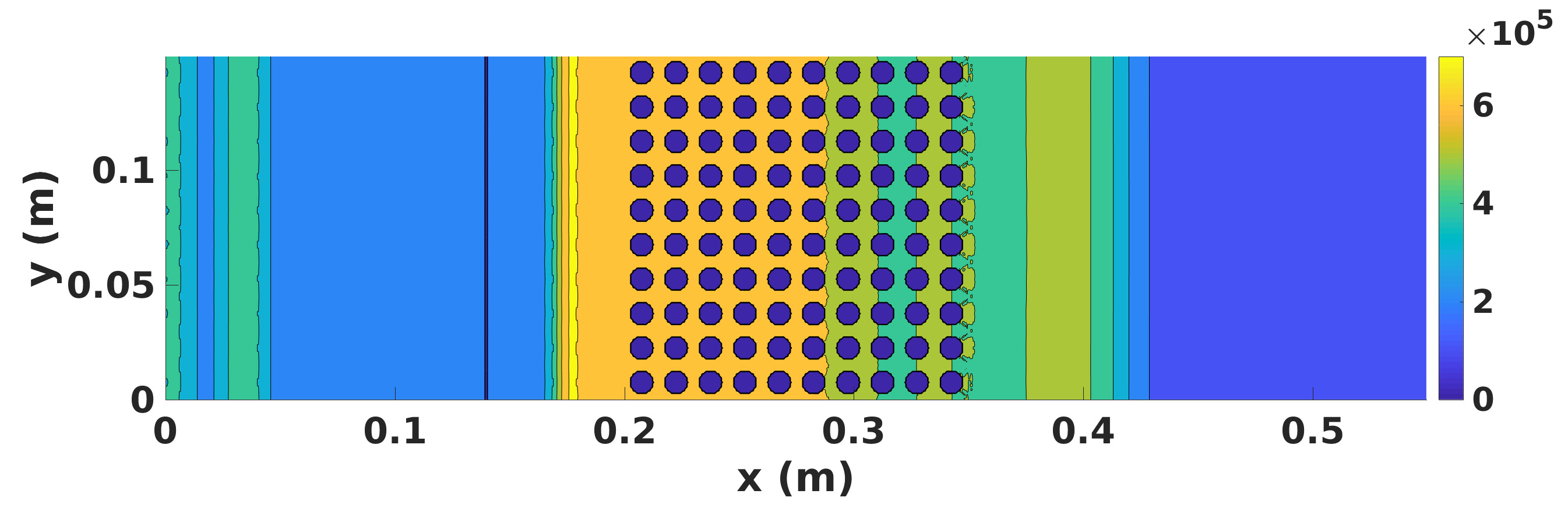
Figure 7 and
Figure 8. It can be noted that, during the postprocessing, the pressure is by default set to zero on the nodes located outside the fluid domain.
As in a classical shock tube situation, the simulation shows two waves propagating in opposite directions from the initial discontinuity (
Figure 5 at
, and
Figure 6). The left one then bounces back on the left vertical boundary (defined with absorbing conditions) and heads back towards the solid medium (
Figure 5,
). This left boundary condition, not very familiar in shock tube computations or experiments, does not here affect the propagation of the pressure wave within the solid medium. Indeed, the simulation stops before the reflected wave hits back the solid medium. The same is true for the wave bouncing back on the right vertical boundary.
5.1. A Priori Accuracy Results
The reference solution being computed at the microscopic scale, the following wavelet postprocessing uses an interpolation of the pressure field on a 2D regular cartesian grid, whose mesh size equals
, i.e., half the value of the reference computation grid. It is recalled that the pressure is by default set to zero on the nodes located outside the fluid domain. Given the microstructure geometry and reference pressure field (
Figure 7), wavelengths around
and
are expected to convey a significant part of the pressure field information. In order to confront this estimation and determine both the relevant cutoff scale and the required number of wavelet coefficients, two accuracy criteria are hereafter introduced:
- •
first, a criterion based on wavelets ability to preserve a signal
-energy:
which allows studying the impact of the number of wavelet coefficients
on the
-energy recovery:
- •
second, a physics-driven criterion based on the force applied to the solid medium microstructure:
which shall be compared to its approximation:
where
is an approximation of the microscopic pressure field, obtained by computing the reconstruction formula (9) with
scales on the range
.
Starting with the
-energy criterion,
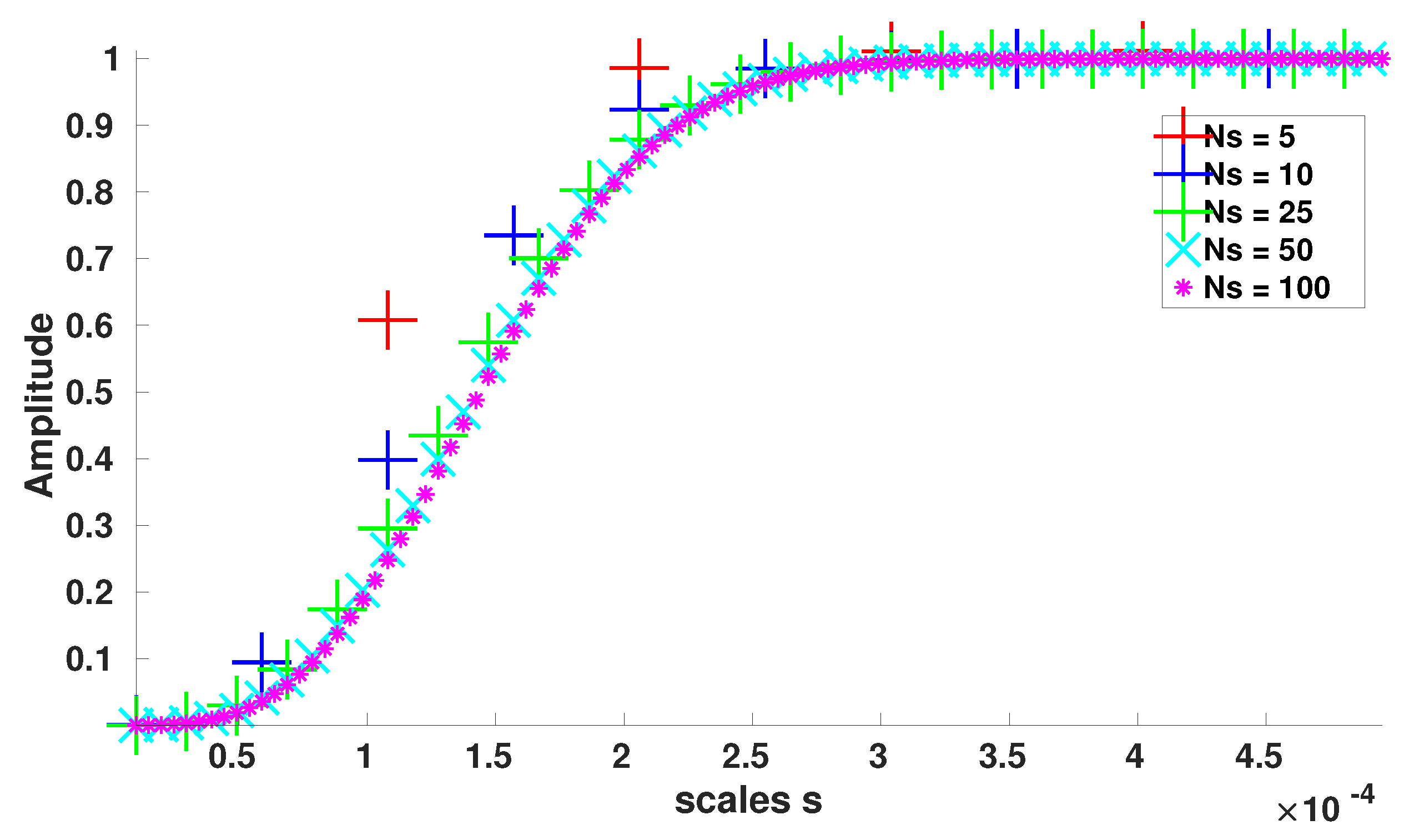
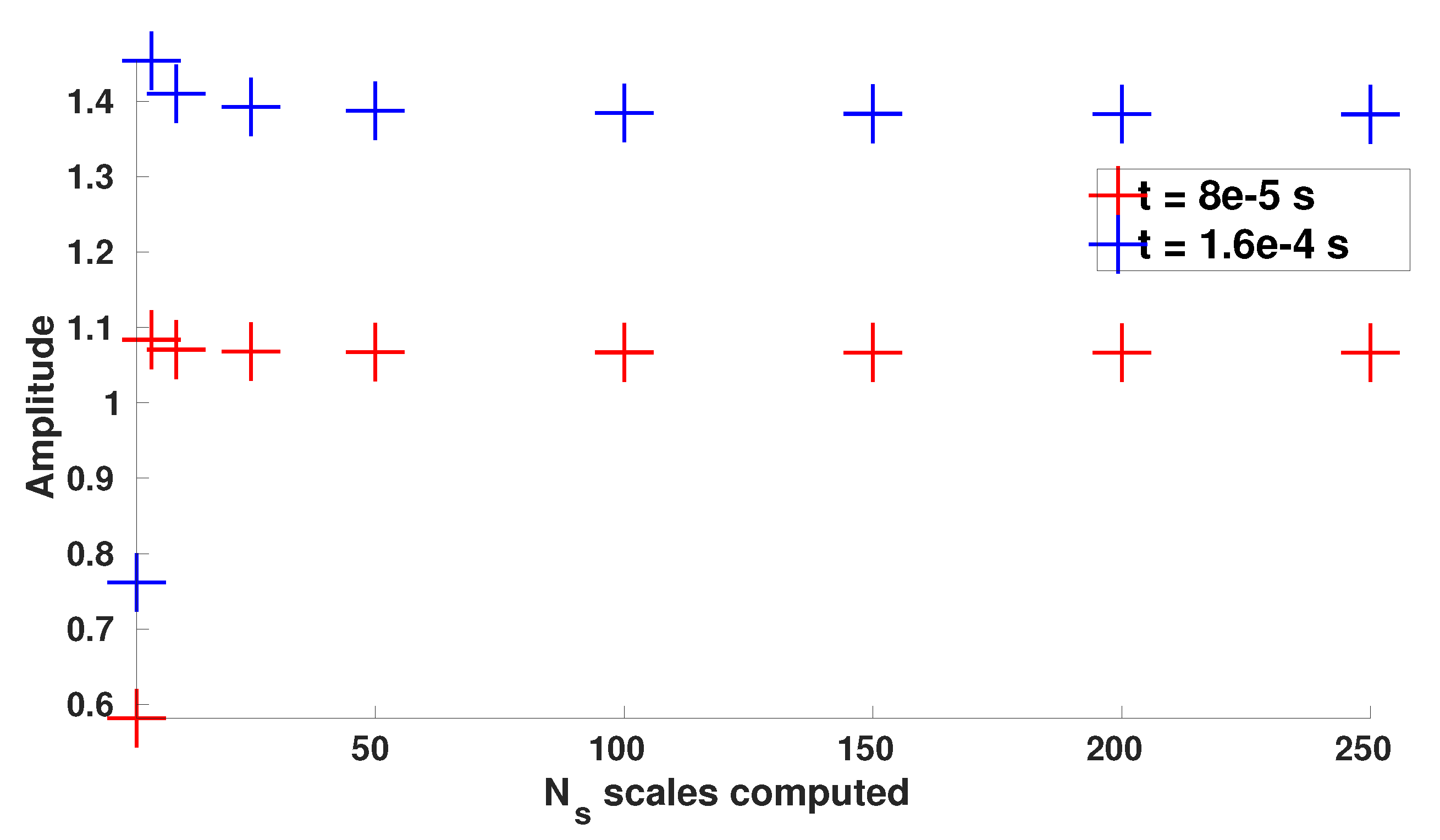
Figure 9 and
Figure 10 display, for two time instants for which the pressure discontinuity is located at different locations within the array of rods, the percentage of the reference pressure field
-energy that can be recovered with an increasing number of wavelet coefficients
, on the scale range
.
Thus, it appears that for the two different time instants, the scale range , which theoretically corresponds to wavelengths starting from up to , conveys around of the pressure field -energy. Thus, regardless of the location of the pressure discontinuity within the array of rods, the most energetic scales seem to be invariant and constrained by the microstructure geometry.
Nevertheless, it could seem surprising to detect “wavelengths” smaller than the grid mesh size
. However, it is here important to highlight the fact that the scale parameter does not only drive the wavelets bandwidth (and thus the “observable” wavelengths), but also the amplitude of the wavelet coefficients, as can be seen in Equation (6). Thus, by decreasing the scale parameter, it is possible to increase the value of the homogenized density and pressure variables in the vicinity of the solid medium microstructure and thus better represent the fact that the fluid shall not penetrate the solid medium. Therefore, the smallest scales detected in
Figure 9 and
Figure 10 are not connected to actual and physical wavelengths. Conversely, it can be outlined that wavelet coefficients
with
, which gather around
of the pressure
-energy, are connected to (actual) wavelengths wider than the grid mesh size
.
Finally, it can be noted that a decrease in the number of computed coefficients does not have a significant impact on the -energy recovery. Indeed, the asymptotic value still reaches around , even with only five wavelet coefficients.
Let us now turn towards the second criterion in order to check whether similar conclusions are reached.
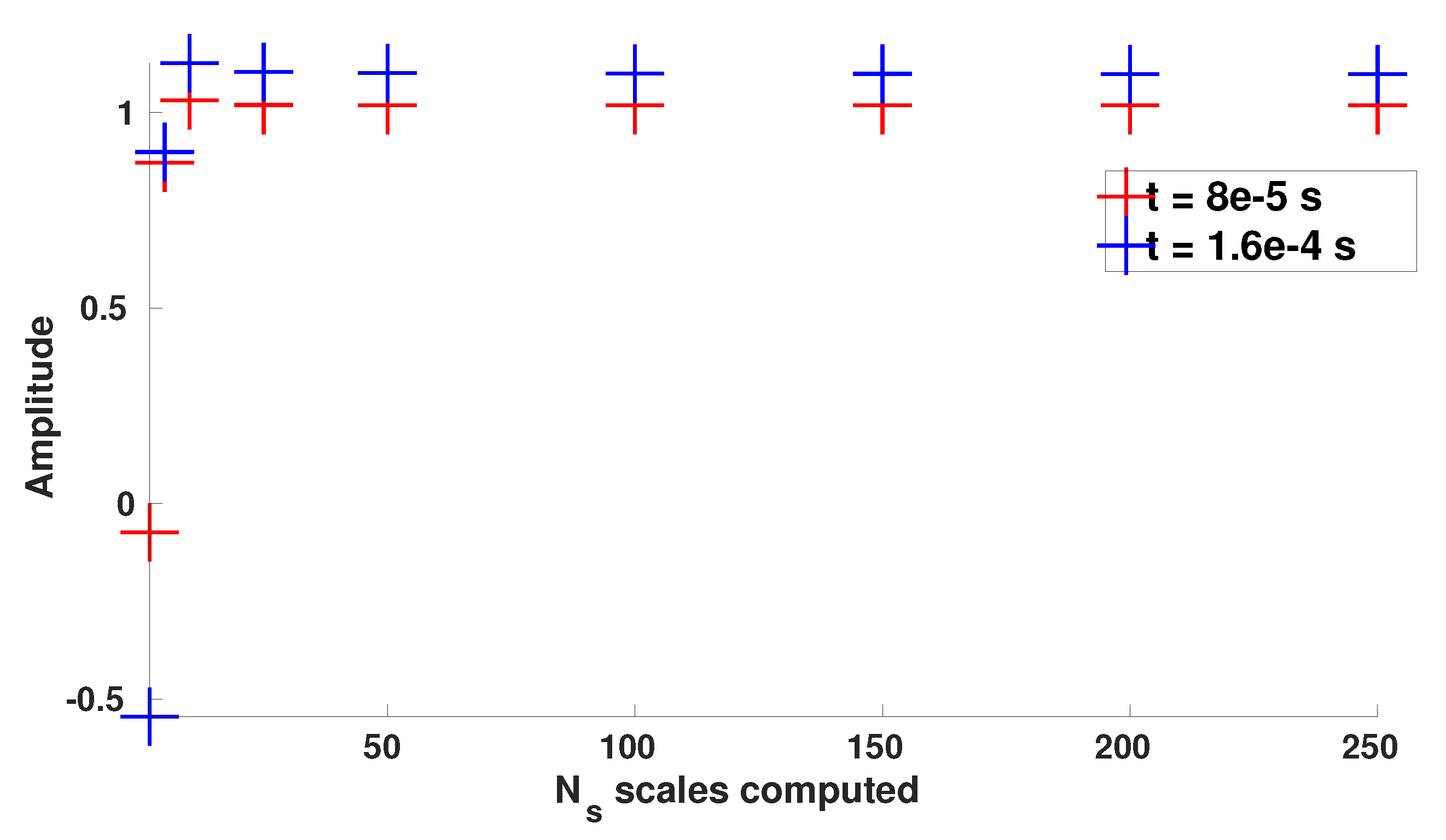
Figure 11,
Figure 12 and
Figure 13 show the evolution of the force ratio, evaluated on the whole microstructure, with the number of computed scales
, and for three different scale ranges:
,
, and
. It is recalled that the force ratio is defined by:
where
and
are defined in Equations (
16) and (
17), and
is a unit horizontal vector, heading from left to right.
Conversely to the
-energy criterion, the scale range
seems here unsuited to properly reconstruct the force applied to the solid medium microstructure, as an almost
overestimation can be witnessed for the time instant
. Furthermore, an
overestimation is still visible for the time instant
. This significant difference between the two time instants can be explained by the following fact: as the initial pressure wave has almost exited the array of rods for
(see
Figure 7), wavelengths around
(driven by the rods radius), which are not taken into account in the scale range
, are much more present within the solid medium microstructure than for the time instant
.
Thus, the wider scale range
allows to better reconstruct the force for both time instants, with, for instance, an overestimation around
for
. Additionally,
Figure 13 proves that the smallest scales could even be neglected without losing accuracy, thus leading to the range
, which contains wavelengths
. This is a very interesting result in the perspective of building a filtered solver for the fluid equations, since it will allow a coarser and thus more efficient discretization at macroscale while preserving the quantity of interest at microscale for the interaction with the structure.
Finally, it can be noticed that
wavelet coefficients would already allow reaching a good accuracy
on the force applied to the solid medium microstructure. The results and recommendations for the most efficient filtering obtained with this physics-driven criterion are thus summarized in
Table 6.
For the sake of completeness,
Figure 14 and
Figure 15 display the reference and reconstructed pressure fields along the medium horizontal axis, while
Figure 16 and
Figure 17 display the absolute error between the 2D reference and reconstructed pressure fields. The absolute error is logically located in the vicinity of the rods, where the reference pressure variations are maximal, but it remains small compared to the reference pressure range (less than 10% for maximum values). Furthermore, the good results obtained in terms of forces acting on the structure indicate that the pressure gradient is well preserved.
5.2. Short Summary of the Main Results
To summarize the results regarding the implementation of the CWT filtering technique to a representative unsteady pressure field, it can be emphasized that the use of continuous wavelet transform, with the isotropic Mexican hat as the analyzing wavelet, allowed, on the one hand, selecting the most relevant wavelengths within the reference field and, on the other hand, reconstructing, with a small number of homogenized coefficients and a very good accuracy, the force applied to the solid medium microstructure.
These results demonstrate the promising expected properties of the proposed approximation approach for the construction of a homogenized representation of the fluid problem in the section of fuel assembly crossed by pressure waves. Moreover, it is important to quote that the results were obtained above in the most selective case of a stiff shock propagating through the rod bundle. In a situation closer to the addressed physics where the pressure signal coming from a partially diffracted rarefaction wave is much smoother, the scale selection is likely to be even more efficient, and with it, the computational performance of an associated macroscale solver for the filtered fluid equations.