Energy Transport by Kelvin-Helmholtz Instability at the Magnetopause
Abstract
:1. Introduction
2. Numerical Method
3. Simulation Results
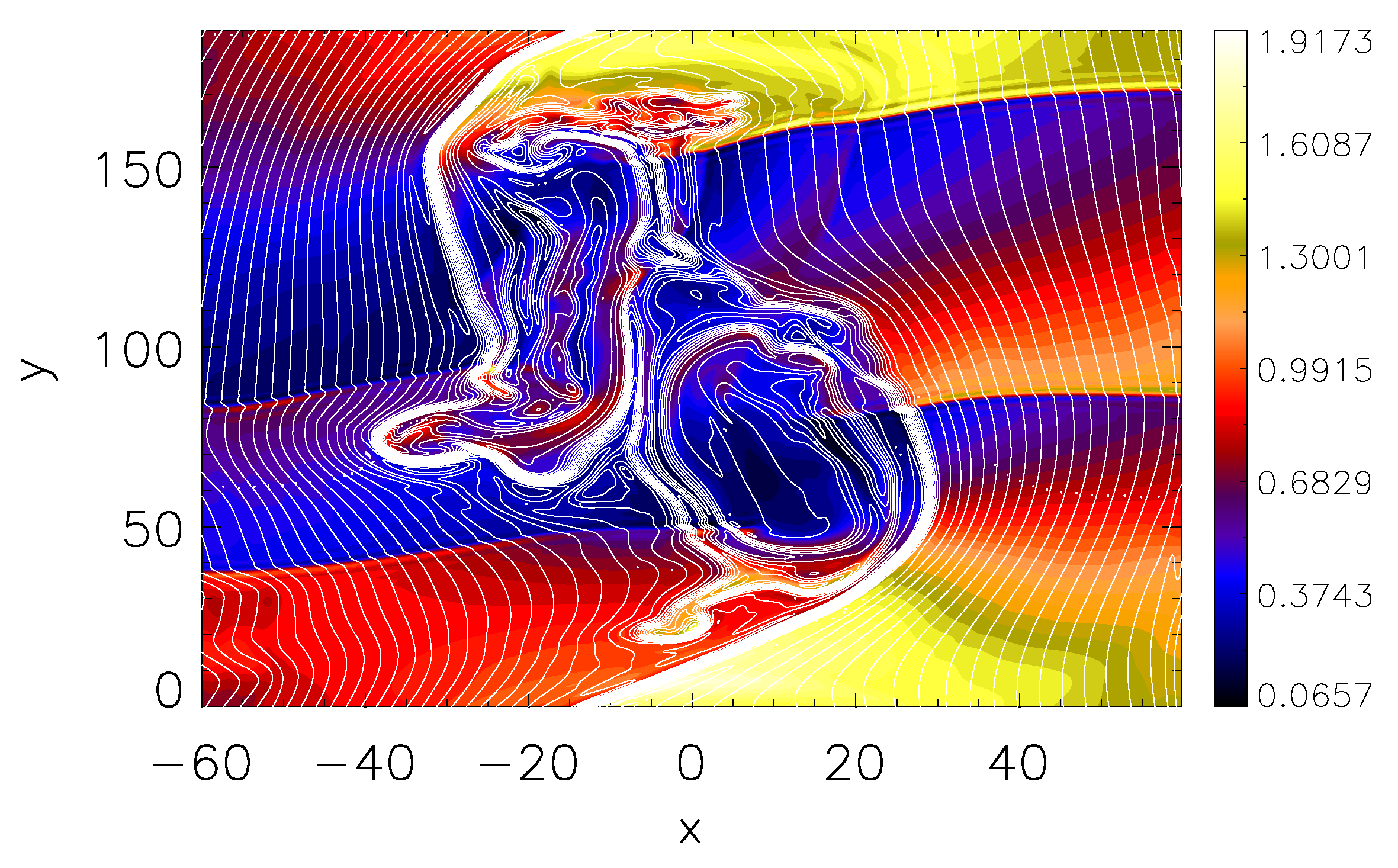
3.1. Plasma Configuration
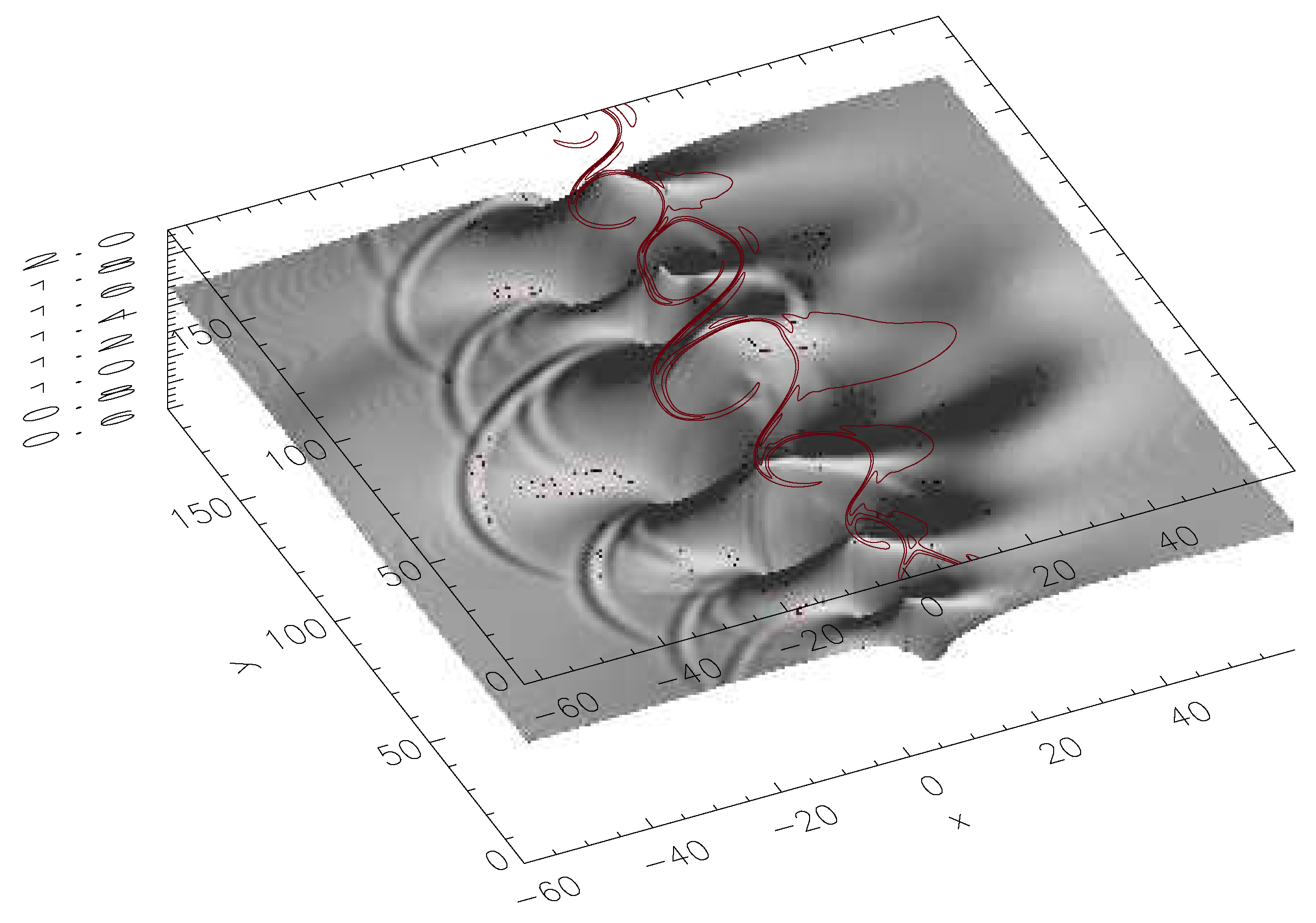
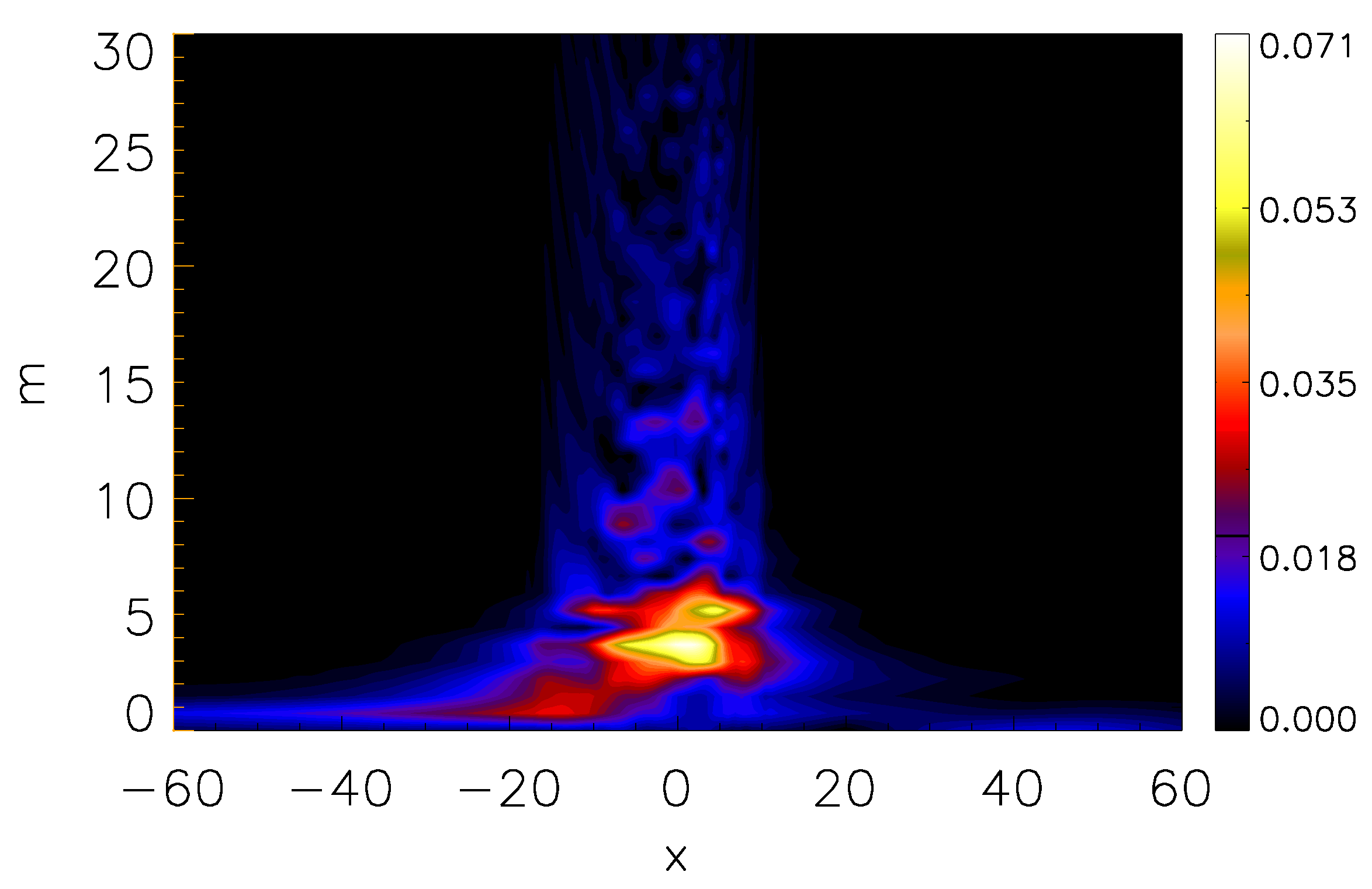
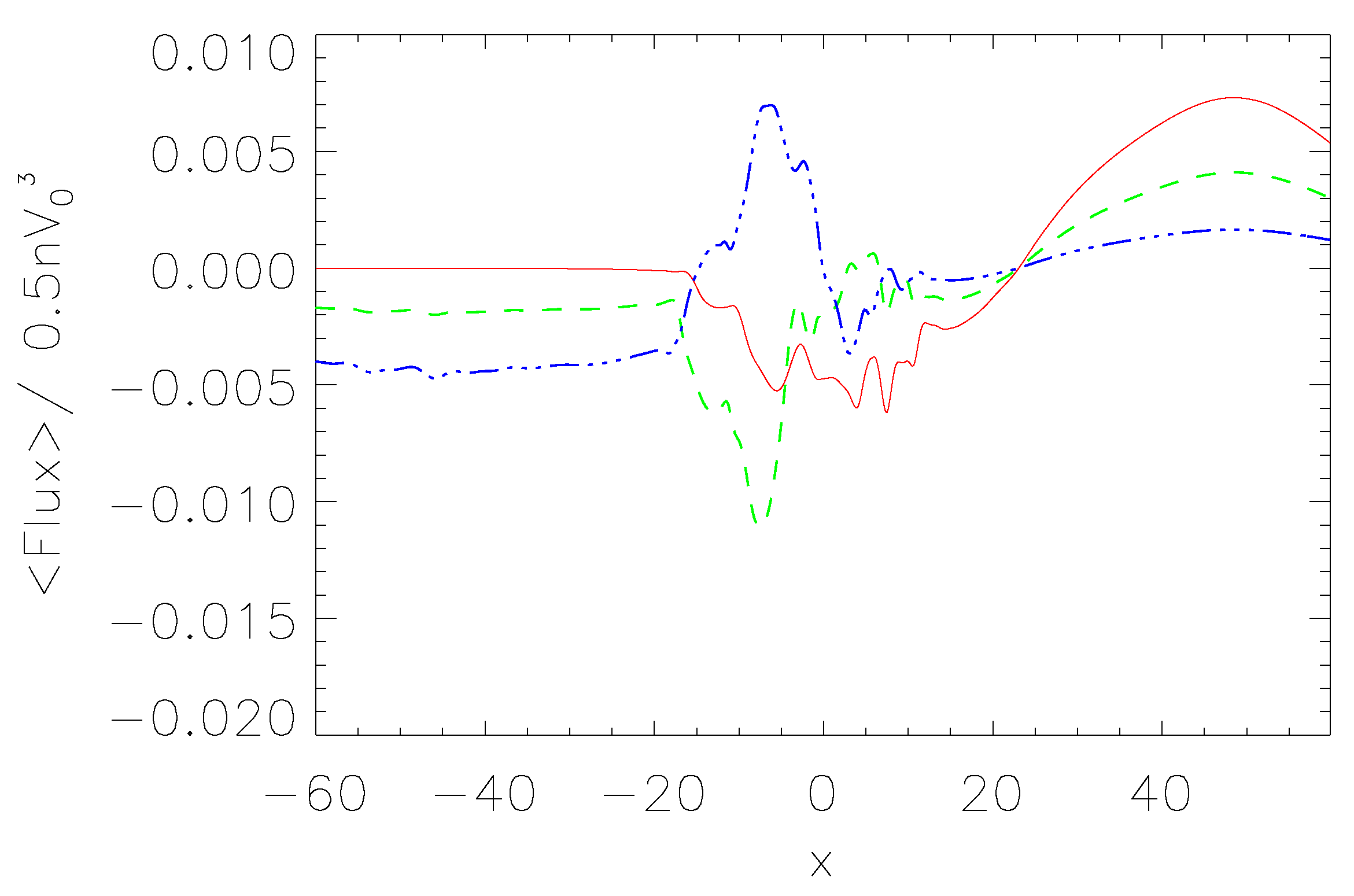
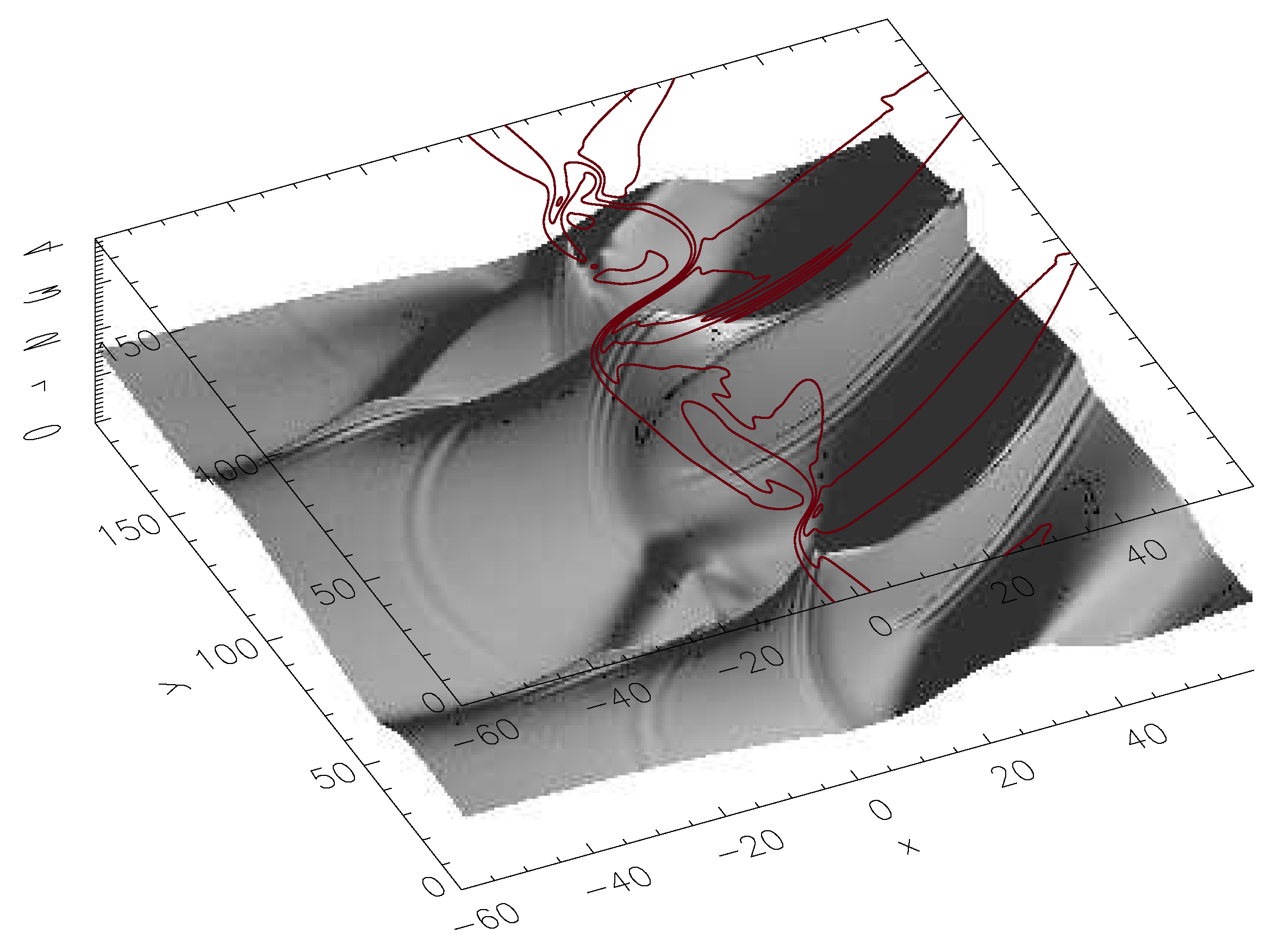
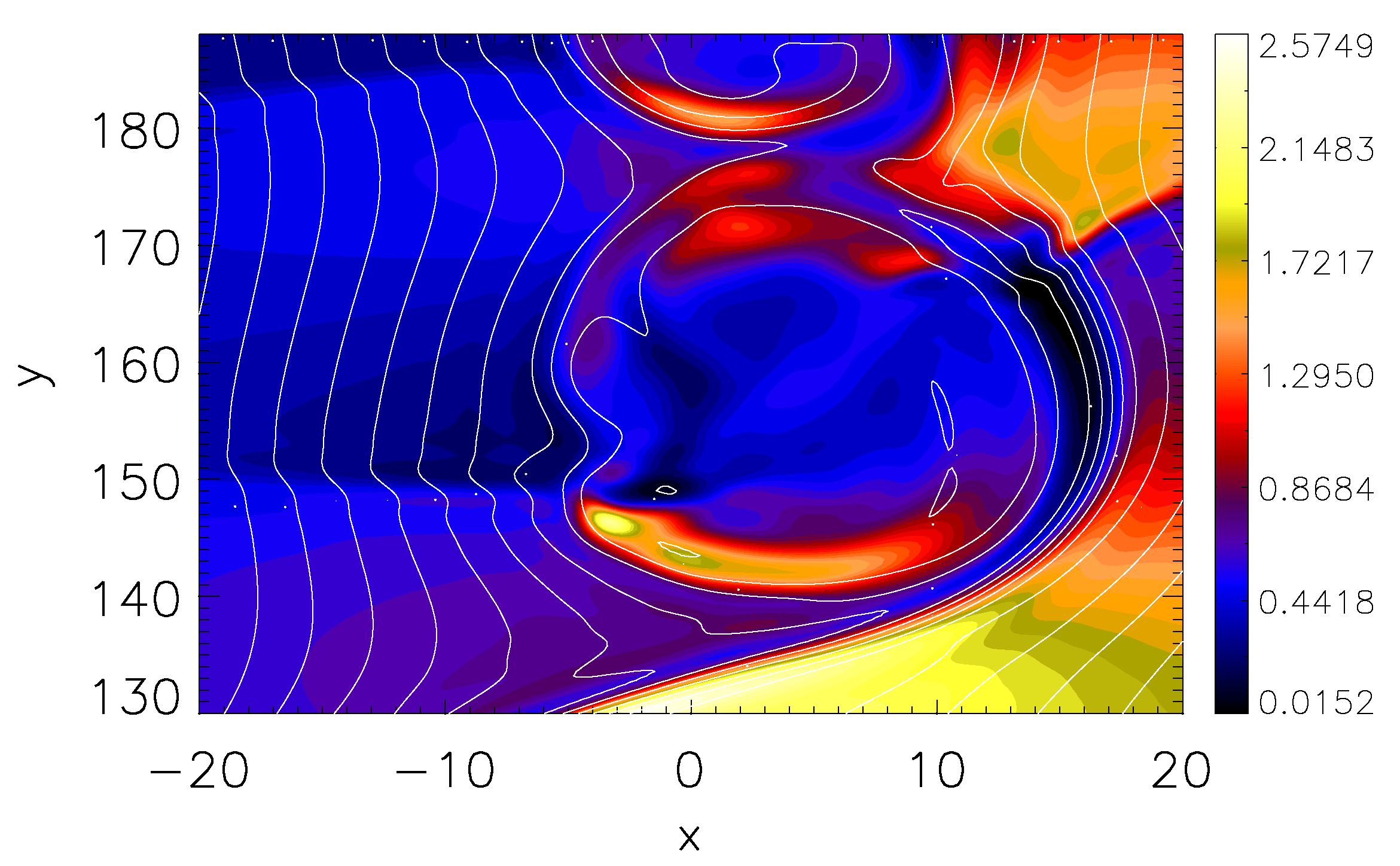
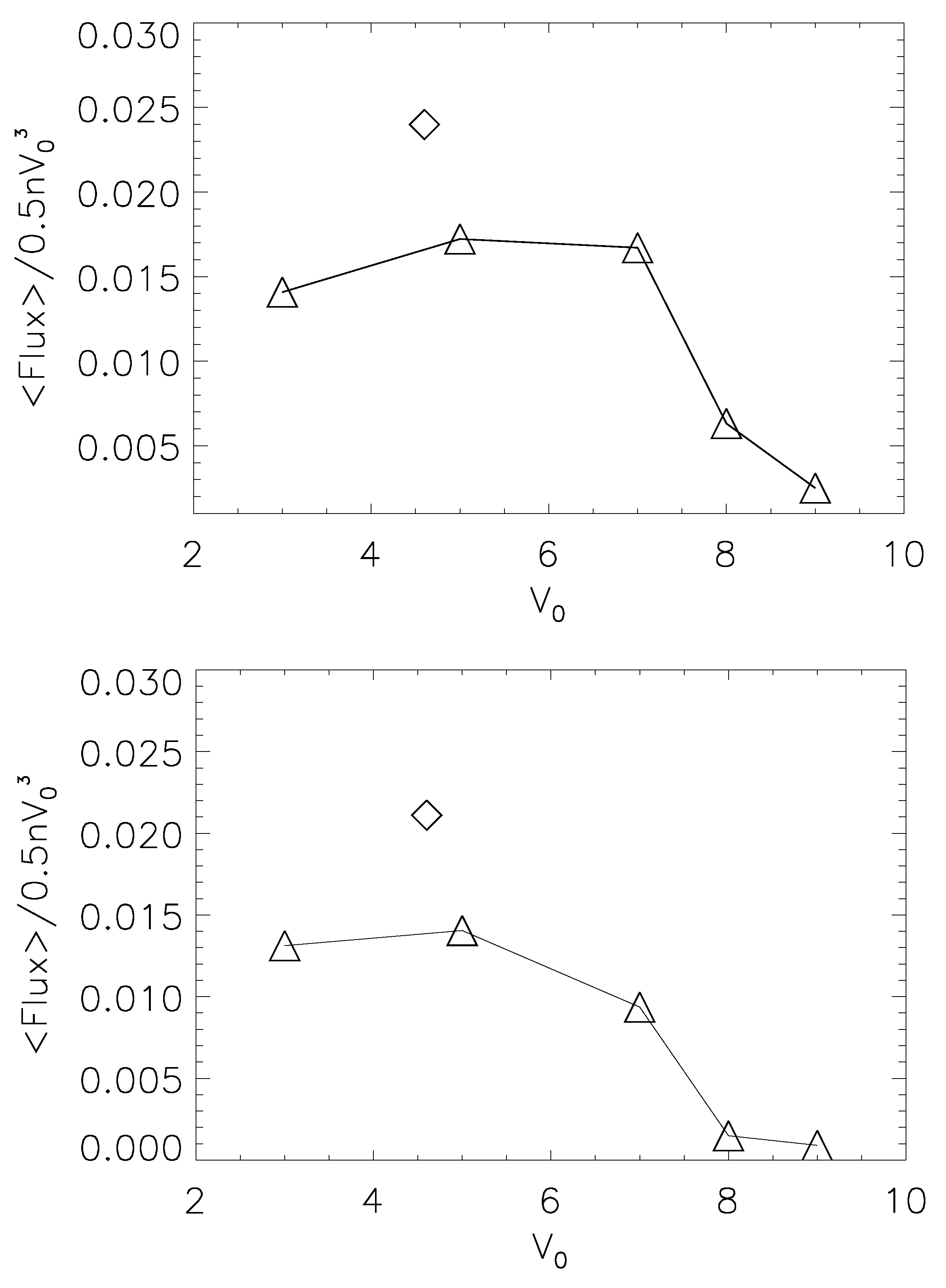
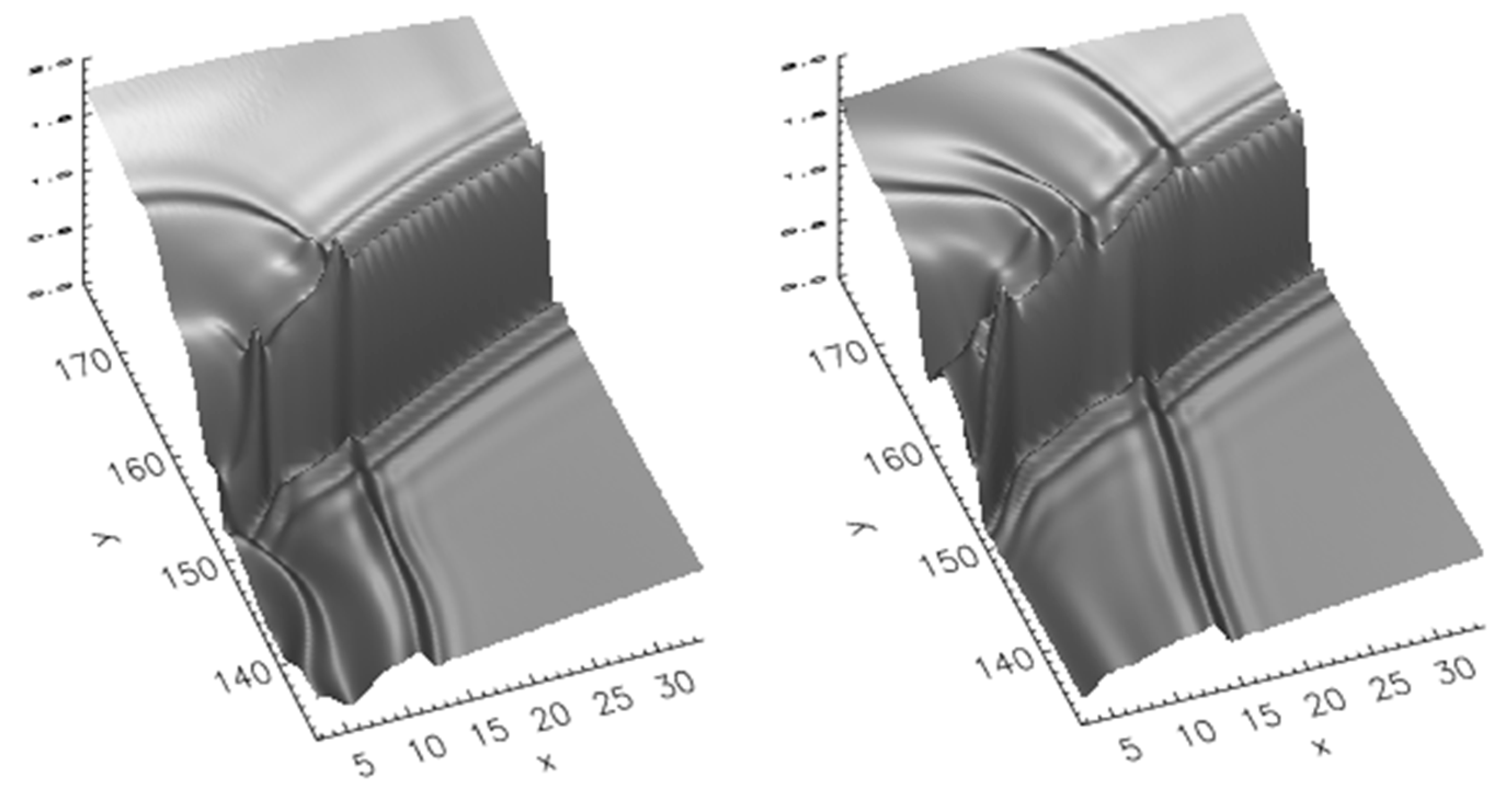
3.2. Energy Flux by Kelvin Helmholtz (KH) Instability
4. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Song, P.; Elphic, R.C.; Russell, C.T. ISEE 1 & 2 observations of the oscillating magnetopause. Geophys. Res. Lett. 1988, 15, 744–747. [Google Scholar]
- Yagodkina, O.I.; Vorobjev, V.G. Daytime high latitude pulsations associated with solar wind dynamic pressure impulses and flux transfer events. J. Geophys. Res. 1997, 102, 57–67. [Google Scholar] [CrossRef]
- Lee, S.H.; Zhang, H.; Zong, Q.G.; Wang, Y.; Otto, A.; Réme, H.; Glassmeier, K.H. Asymmetric ionospheric outflow observed at the dayside magnetopause. J. Geophys. Res. Space Phys. 2015, 120, 3564–3573. [Google Scholar] [CrossRef] [Green Version]
- Ofman, L.; Thompson, B.J. Observation of Kelvin Helmholtz instability in the solar corona. Astrophys. J. Lett. 2011, 734, L11. [Google Scholar] [CrossRef]
- Rogers, B.N.; Dorland, W.; Kotschenreuther, M. Generation and Stability of Zonal Flows in Ion-Temperature- Gradient Mode Turbulence. Phys. Rev. Lett. 2000, 85, 5336. [Google Scholar] [CrossRef] [PubMed]
- Palermo, F.; Garbet, X.; Ghizzo, A.; Cartier-Michaud, T.; Ghendrih, P.; Grandgirard, V.; Sarazin, Y. Shear flow instabilities induced by trapped ion modes in collisionless temperature gradient turbulence. Phys. Plasmas 2015, 22, 042304. [Google Scholar] [CrossRef]
- Ghizzo, A.; Palermo, F. Shear-flow trapped-ion-mode interaction revisited. I. Influence of low-frequency zonal flow on ion-temperature-gradient driven turbulence. Phys. Plasmas 2015, 22, 082303. [Google Scholar] [CrossRef] [Green Version]
- Palermo, F.; Garbet, X.; Ghizzo, A. Bicoherence analysis of streamer dynamics induced by trapped ion modes. Europ. Phys. J. D 2015, 69, 8. [Google Scholar] [CrossRef]
- Axford, W.I.; Hincs, C.O. A unifying theory of high-latitude. Geophysical phenomena and geomagnetic storms. Can. J. Phys. 1961, 39, 1433–1464. [Google Scholar] [CrossRef]
- Lemaire, J.; Roth, M. Penetration of the solar wind plasma elements into the magnetosphere. J. Atmos. Terr. Phys. 1978, 40, 331–335. [Google Scholar] [CrossRef]
- Miura, A.; Pritchett, P. Nonlocal stability analysis of the MHD Kelvin-Helmholtz instability in a compressible plasma. J. Geophys. Res. 1982, 87, 7431–7444. [Google Scholar] [CrossRef]
- Miura, A. Anomalous transport by magnetohydrodynamic Kelvin-Helmholtz instabilities in the solar wind-magnetosphere interaction. J. Geophys. Res. 1984, 89, 801–848. [Google Scholar] [CrossRef]
- Miura, A. Simulation of Kelvin-Helmholtz Instability at the Magnetospheric Boundary. J. Geophys. Res. 1987, 97, 3195–3206. [Google Scholar] [CrossRef]
- Hasegawa, H.; Fujimoto, M.; Phan, T.D.; Réme, H.; Balogh, A.; Dunlop, M.W.; Hashimoto, C.; TanDokoro, R. Transport of solar wind into Earth’s magnetosphere through rolled-up Kelvin-Helmholtz vortices. Nature 2004, 430, 755. [Google Scholar] [CrossRef]
- Nishino, M.N.; Hasegawa, H.; Fujimoto, M.; Saito, Y.; Mukai, T.; Dandouras, I.; Reme, H.; Retino, A.; Nakamura, R.; Lucek, E.; et al. A case study of Kelvin–Helmholtz vortices on both flanks of the Earth’s magnetotail. Planet. Space Sci. 2011, 59, 502–509. [Google Scholar] [CrossRef]
- Otto, A.; Fairfield, D.H. Kelvin-Helmholtz instability at the magnetotail boundary: MHD simulation and comparison with Geotail observations. J. Geophys. Res. 2000, 105, 21175–21190. [Google Scholar] [CrossRef]
- Palermo, F.; Califano, F.; Pegoraro, F.; Le Contel, O. Possible magnetospheric Kelvin-Helmholtz vortex signatures near the post-noon magnetopause. Mem. Soc. Astron. Ital. Suppl. 2010, 14, 189. [Google Scholar]
- Faganello, M.; Califano, F.; Pegoraro, F.; Retino, A. Kelvin-Helmholtz vortices and doublemid-latitude reconnection at the Earth’smagnetopause: Comparison between observations and simulations. Eur. Phys. J. 2014, 107, 19001. [Google Scholar]
- Matsumoto, Y.; Hoshino, M. Onset of turbulence induced by a Kelvin-Helmholtz vortex. Geophys. Res. Lett. 2004, 31, L02807. [Google Scholar] [CrossRef]
- Faganello, M.; Califano, F.; Pegoraro, F. Competing mechanisms of plasma transport in inhomogeneous configurations with velocity shear: The solar-wind interaction with Earth’s magnetosphere. Phys. Rev. Lett. 2008, 100, 015001. [Google Scholar] [CrossRef]
- Fadanelli, S.; Faganello, M.; Califano, F.; Cerri, S.S.; Pegoraro, F.; Lavraud, B. North-South Asymmetric Kelvin-Helmholtz Instability and Induced Reconnection at the Earth’s Magnetospheric Flanks. J. Geophys. Res. 2018, 123, 9340–9356. [Google Scholar] [CrossRef]
- Leroy, M.H.J.; Keppens, R. On the influence of environmental parameters on mixing and reconnection caused by the Kelvin-Helmholtz instability at the magnetopause. Phys. Plasmas 2017, 24, 012906. [Google Scholar] [CrossRef]
- Ma, X.; Delamere, P.; Antonius Otto, A.; Burkholder, B. Plasma Transport Driven by the Three-Dimensional Kelvin-Helmholtz Instability. J. Geophys. Res. 2017, 122, 10382–10395. [Google Scholar] [CrossRef]
- Leroy, M.H.J.; Ripperda, B.; Keppens, R. Particle Orbits at the Magnetopause: Kelvin-Helmholtz Induced Trapping. J. Geophys. Res. 2019, 124, 6715–6729. [Google Scholar] [CrossRef]
- Blumen, W. Shear layer instability of an inviscid compressible fluid. J. Fluid Mech. 1970, 40, 769–781. [Google Scholar] [CrossRef]
- Drazin, P.G.; Reid, W.H. Hydrodynamic Stability; Cambridge University Press: Cambridge, UK, 1977. [Google Scholar]
- Pu, Z.Y.; Kivelson, M.G. Kelvin-Helmholtz Instability at the Magnetopause’ Energy Flux Into the Magnetosphere. J. Geoph. Res. 1983, 88, 853–861. [Google Scholar] [CrossRef]
- Fairfield, D.H.; Otto, A.; Mukai, T.; Kokubun, S.; Lepping, R.P.; Steinberg, J.T.; Lazarus, A.J.; Yamamoto, T. Geotail observations of the Kelvin-Helmholtz instability at the equatorial magnetotail boundary for parallel northward fields. J. Geophys. Res. 2000, 105, 21159–21173. [Google Scholar] [CrossRef]
- Spreiter, J.R.; Summers, A.L.; Alksne, A.Y. Hydromagnetic flow around the magnetosphere. Planet. Space Sci. 1966, 14, 223–253. [Google Scholar] [CrossRef] [Green Version]
- Chen, S.H.; Kivelson, M.G.; Gosling, J.T.; Walker, R.J.; Lazarus, A.J. Anomalous aspects of magnetosheath flow and of the shape and oscillations of the magnetopause during an interval of strongly northward interplanetary magnetic field. J. Geophys. Res. 1993, 98, 5727–5742. [Google Scholar] [CrossRef] [Green Version]
- Lai, S.H.; Lyu, L.H. A simulation and theoretical study of energy transport in the event of MHD Kelvin-Helmholtz instability. J. Geophys. Res. 2010, 115, A10215. [Google Scholar] [CrossRef]
- Kobayashi, Y.; Kato, M.; Nakamura, K.T.A.; Nakamura, T.K.M.; Fujimoto, M. The structure of Kelvin-Helmholtz vortices with super-sonic flow. Adv. Space Res. 2008, 41, 1325–1330. [Google Scholar] [CrossRef]
- Miura, A. Kelvin-Helmholtz instability for supersonic shear flow at the magnetospheric boundary. Geophys. Res. Lett. 1990, 17, 749–752. [Google Scholar] [CrossRef]
- Miura, A. Kelvin-Helmholtz instability at the magnetospheric boundary: Dependence on the magnetosheath sonic Mach number. J. Geophys. Res. 1992, 97, 10655–10675. [Google Scholar] [CrossRef]
- Miura, A. Nonlinear evolution of the magnetohydrodynamic Kelvin-Helmholtz instability. Phys. Rev. Lett. 1982, 49, 779. [Google Scholar] [CrossRef]
- Palermo, F.; Faganello, M.; Califano, F.; Pegoraro, F.; le Contel, O. Compressible Kelvin-Helmholtz instability in supermagnetosonic regimes. J. Geoph. Res. 2011, 116, A04223. [Google Scholar] [CrossRef]
- Palermo, F.; Faganello, M.; Califano, F.; Pegoraro, F.; Le Contel, O. The Role of the magnetosonic mach number on the evolution of Kelvin Helmholtz vortices. Europ. Conf. Lab. Astroph. 2012, 58, 91–94. [Google Scholar] [CrossRef]
- Palermo, F.; Faganello, M.; Califano, F.; Pegoraro, F.; Le Contel, O. Kelvin-Helmholtz vortices and secondary instabilities in super-magnetosonic regimes. Ann. Geophys. 2011, 29, 1169–1178. [Google Scholar] [CrossRef] [Green Version]
- Valentini, F.; Trávníček, P.; Califano, F.; Hellinger, P.; Mangeney, A. A hybrid-Vlasov model based on the current advance method for the simulation of collisionless magnetized plasma. J. Comp. Phys. 2007, 225, 753–770. [Google Scholar] [CrossRef]
- Faganello, M.; Califano, F.; Pegoraro, F. Being on time in magnetic reconnection. New J. Phys. 2009, 11, 063008. [Google Scholar] [CrossRef]
- Lele, S.K. Compact finite difference schemes with spectral-like resolution. J. Comput. Phys. 1992, 103, 16–42. [Google Scholar] [CrossRef]



© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Palermo, F. Energy Transport by Kelvin-Helmholtz Instability at the Magnetopause. Fluids 2019, 4, 189. https://doi.org/10.3390/fluids4040189
Palermo F. Energy Transport by Kelvin-Helmholtz Instability at the Magnetopause. Fluids. 2019; 4(4):189. https://doi.org/10.3390/fluids4040189
Chicago/Turabian StylePalermo, Francesco. 2019. "Energy Transport by Kelvin-Helmholtz Instability at the Magnetopause" Fluids 4, no. 4: 189. https://doi.org/10.3390/fluids4040189
APA StylePalermo, F. (2019). Energy Transport by Kelvin-Helmholtz Instability at the Magnetopause. Fluids, 4(4), 189. https://doi.org/10.3390/fluids4040189




