A Theoretical Investigation of Flow Topologies in Bubble- and Droplet-Affected Flows
Abstract
1. Introduction
- to demonstrate that the topology distribution in the interior phase is independent of the dynamic viscosity ratio and the boundary conditions applied in the exterior phase;
- to check upon the existence of a narrow band of intermediate nodal topologies;
- to derive explicit mathematical expressions for all topology borders in both phases;
- to calculate the universal topology volume fractions in the interior phase; and
- to provide an analytical reference solution for the purpose of numerical topology code validation.
2. Mathematical Description
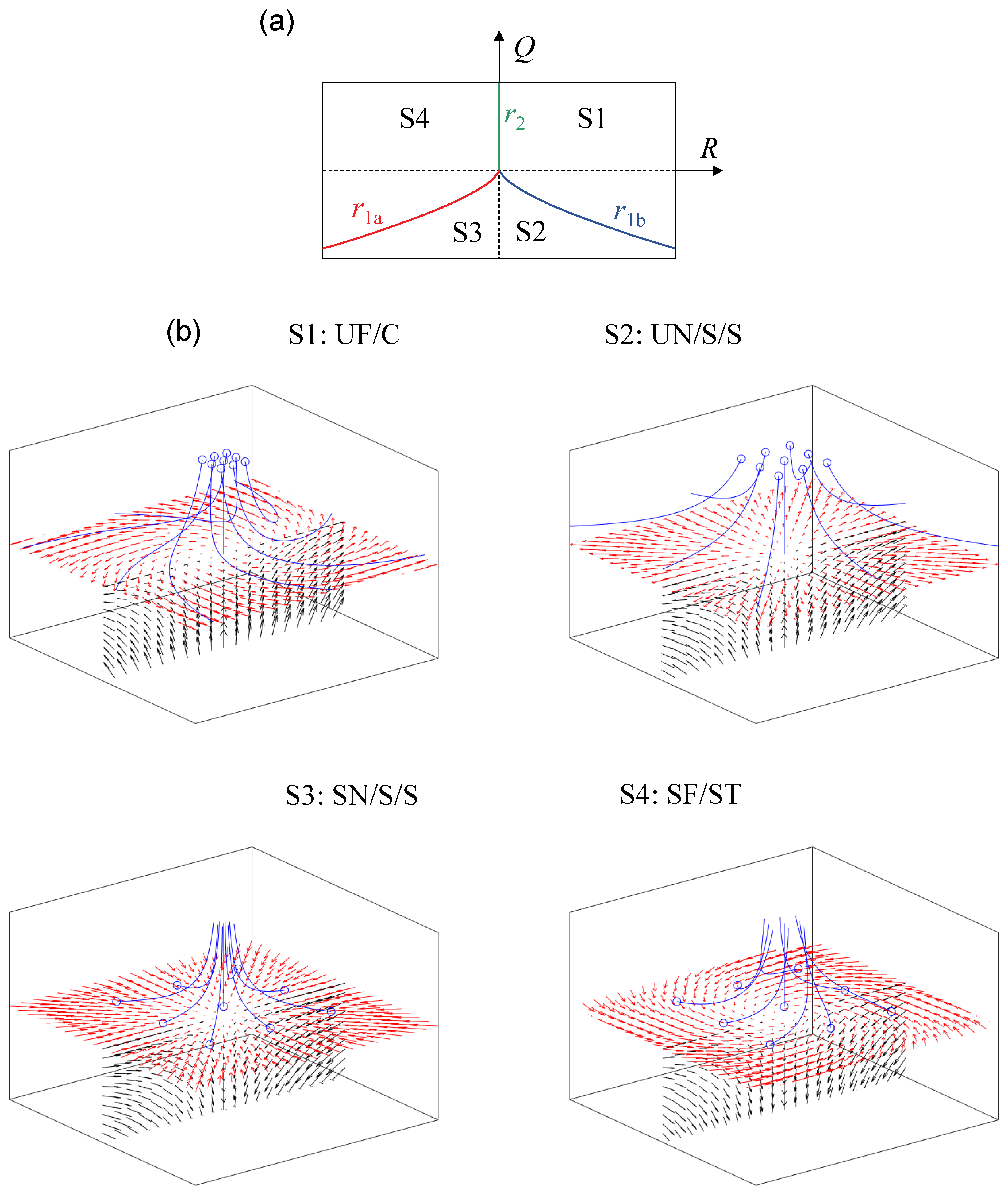
2.1. Invariants of the Velocity Gradient Tensor and Flow Topologies
2.2. Stream Function Ansatz
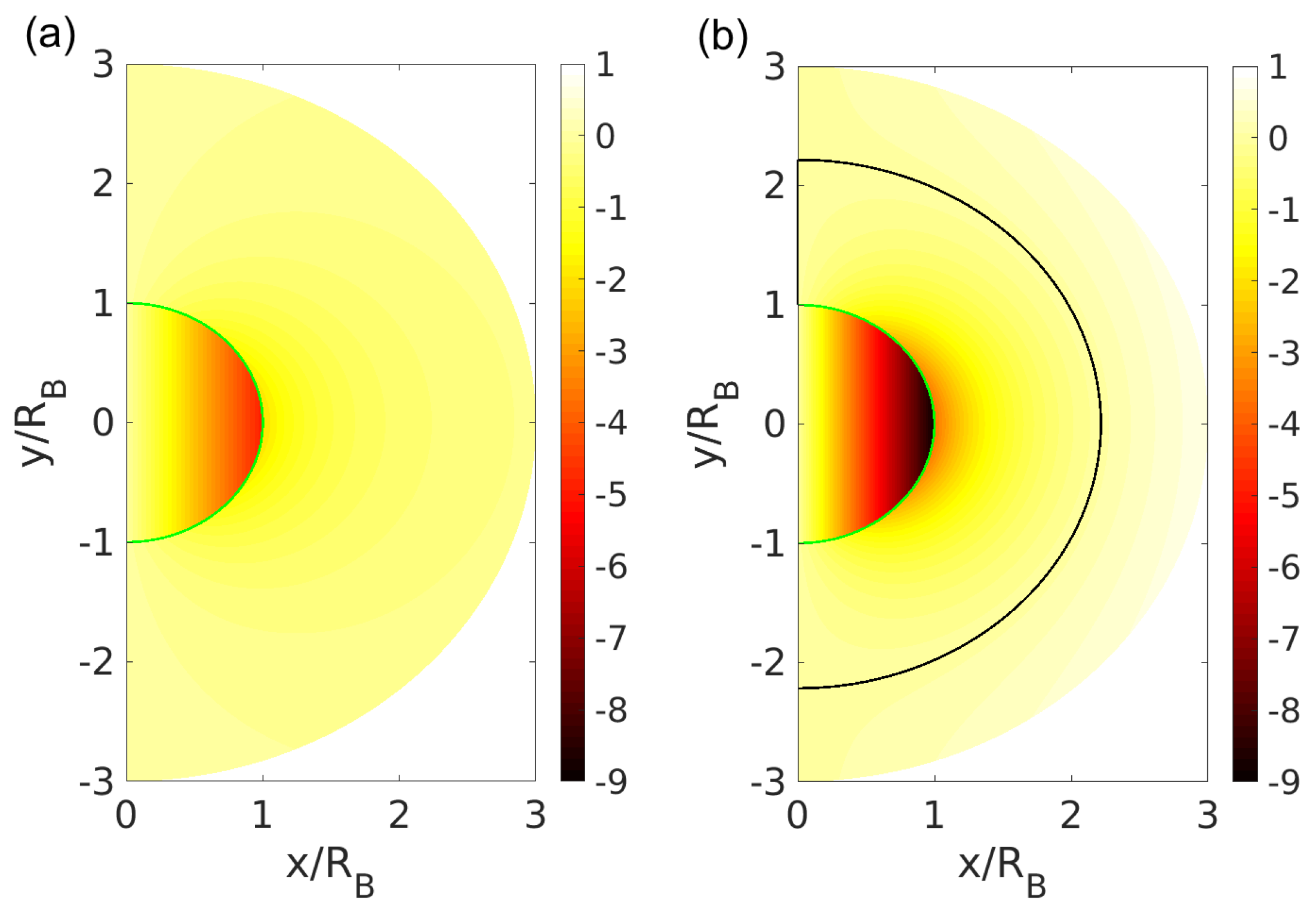
2.3. Vorticity Field
2.4. Far-Field Boundary Conditions
2.5. Near-Field Boundary Conditions
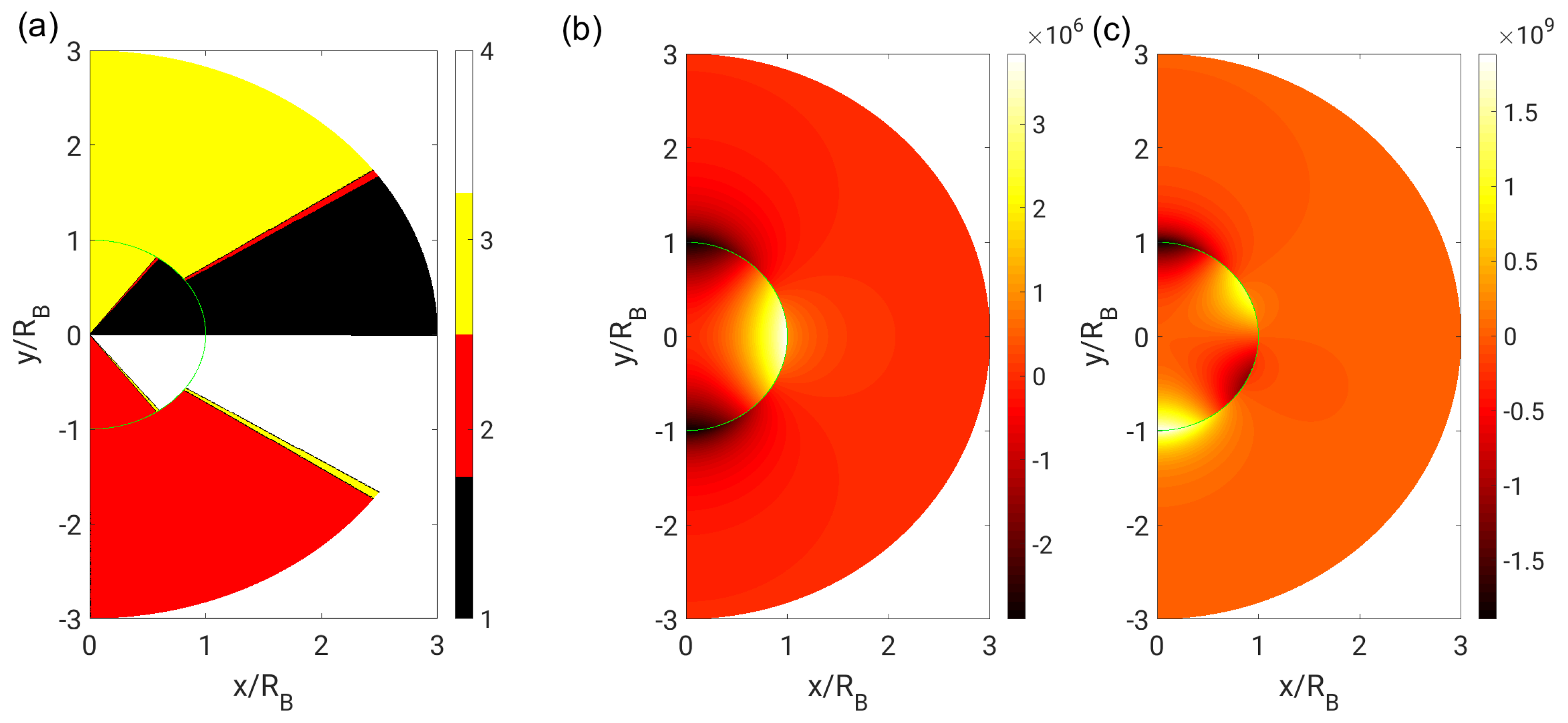
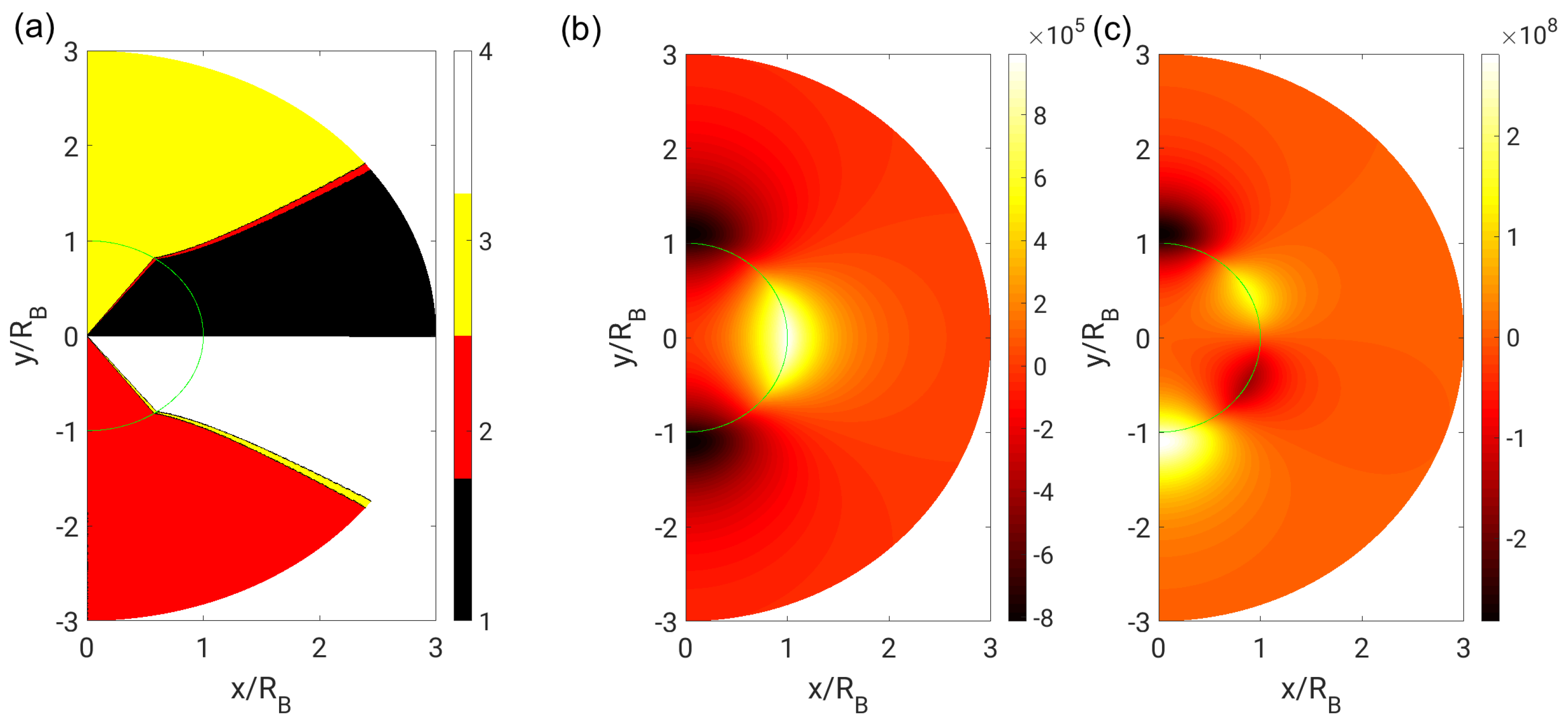
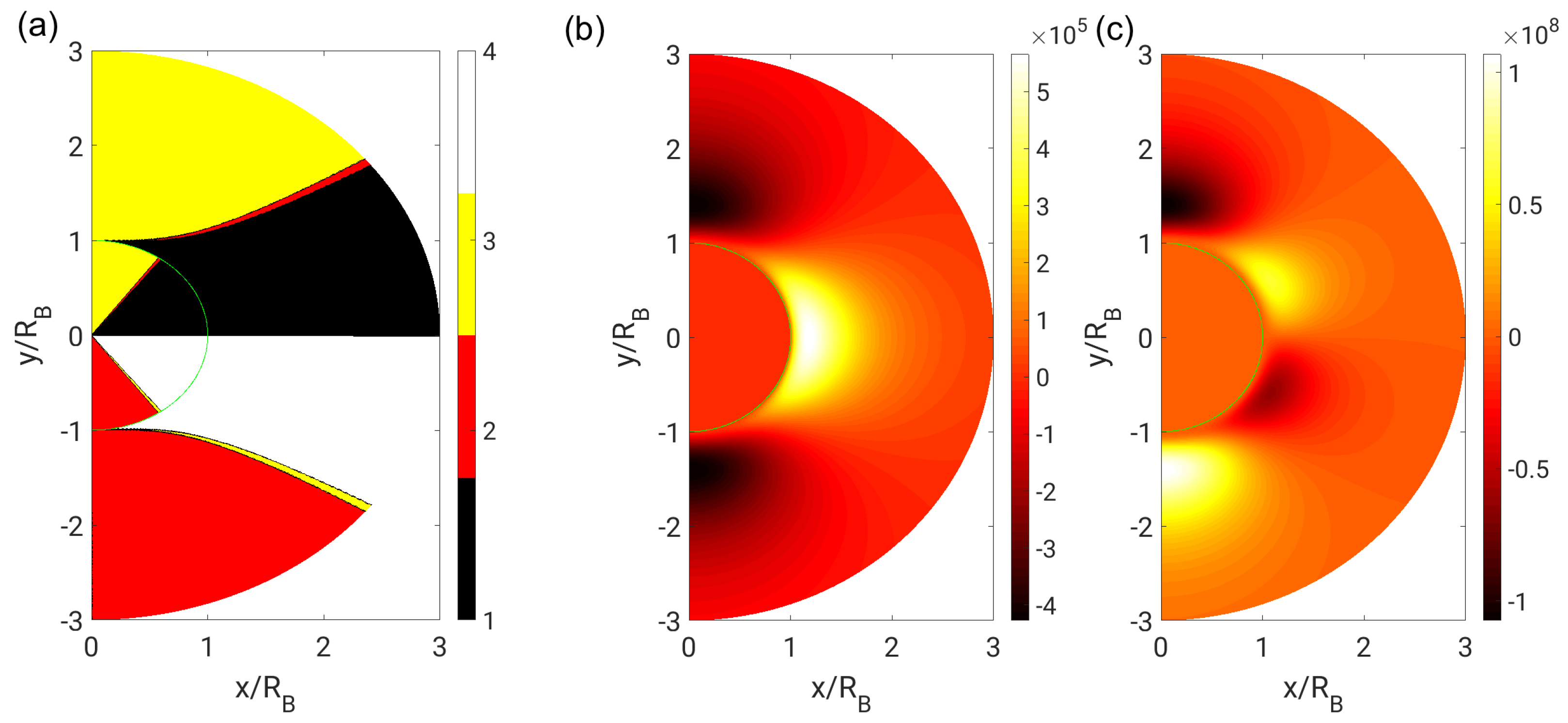
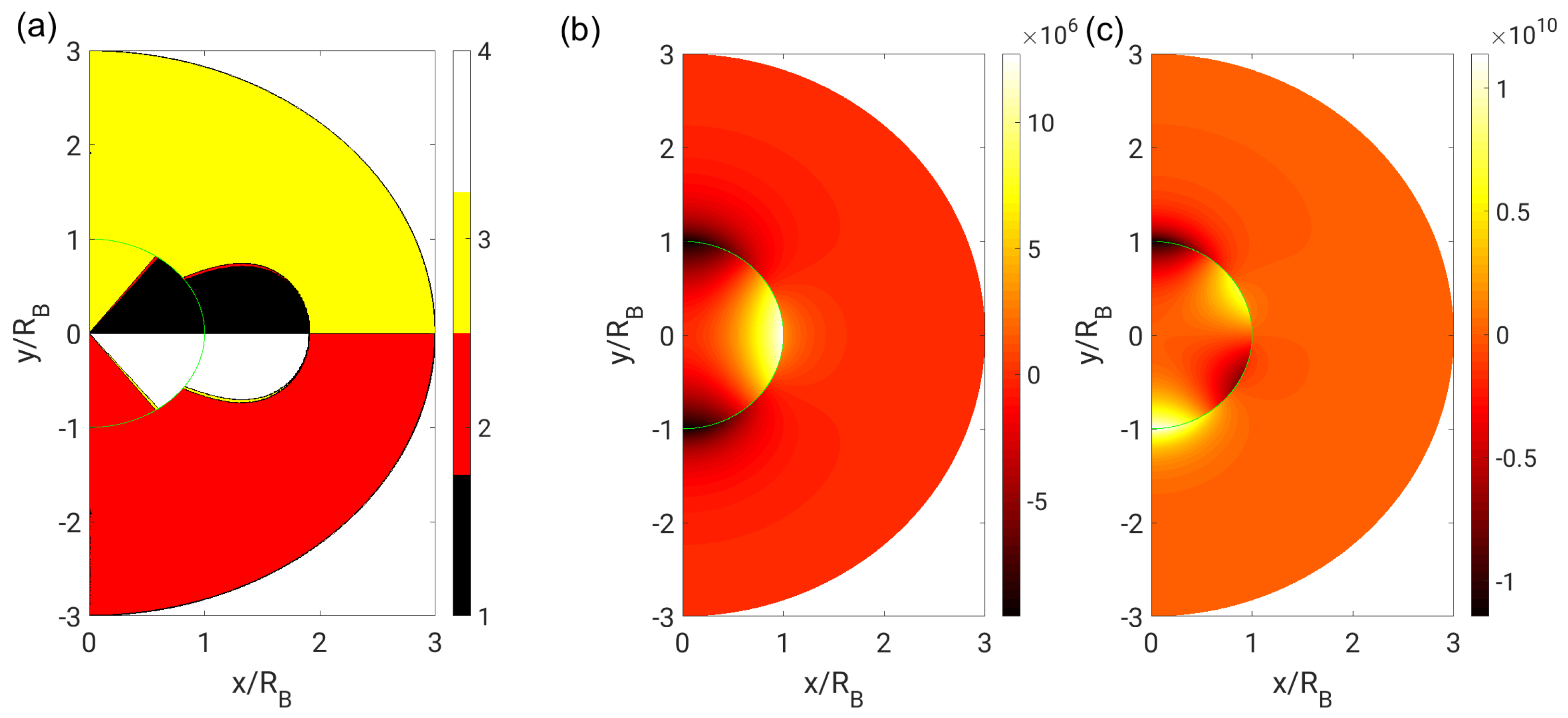
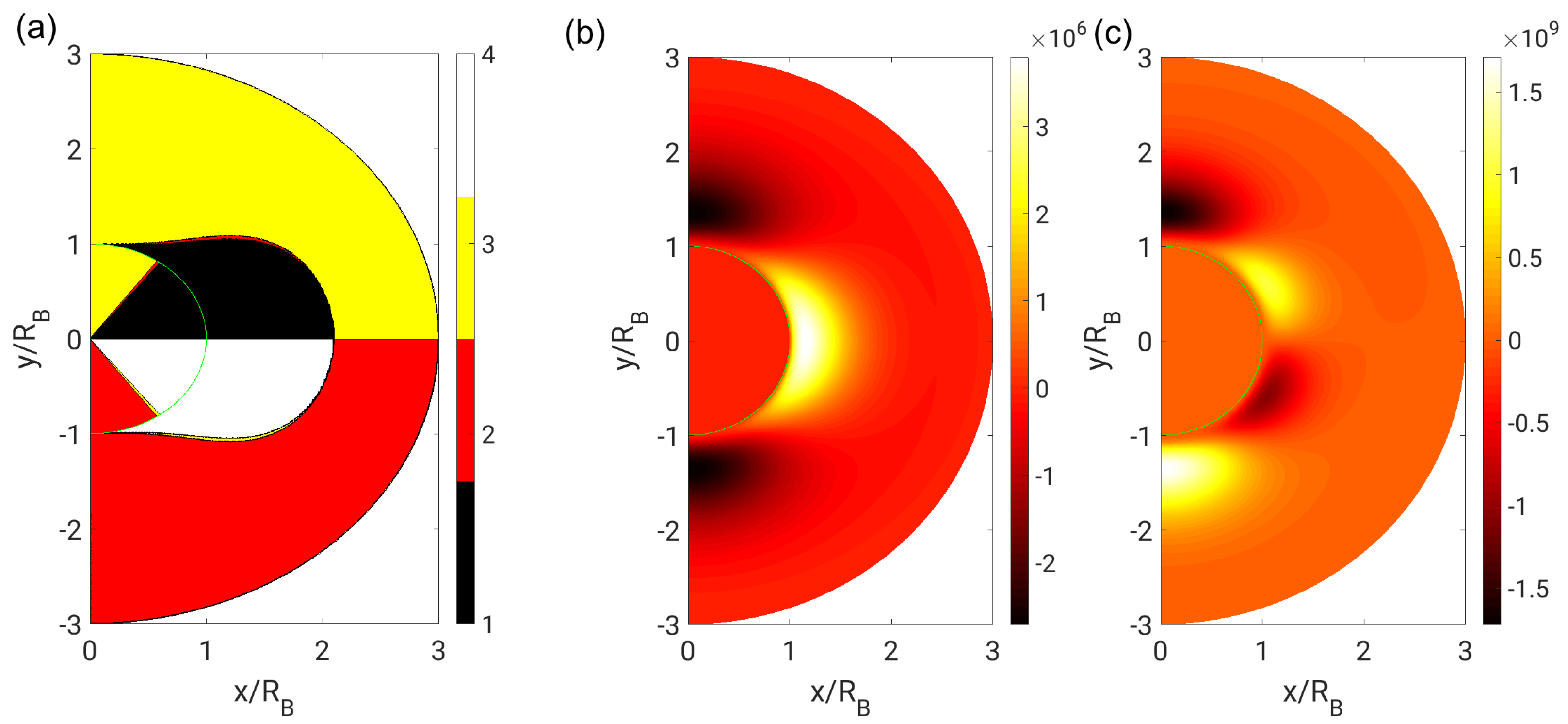
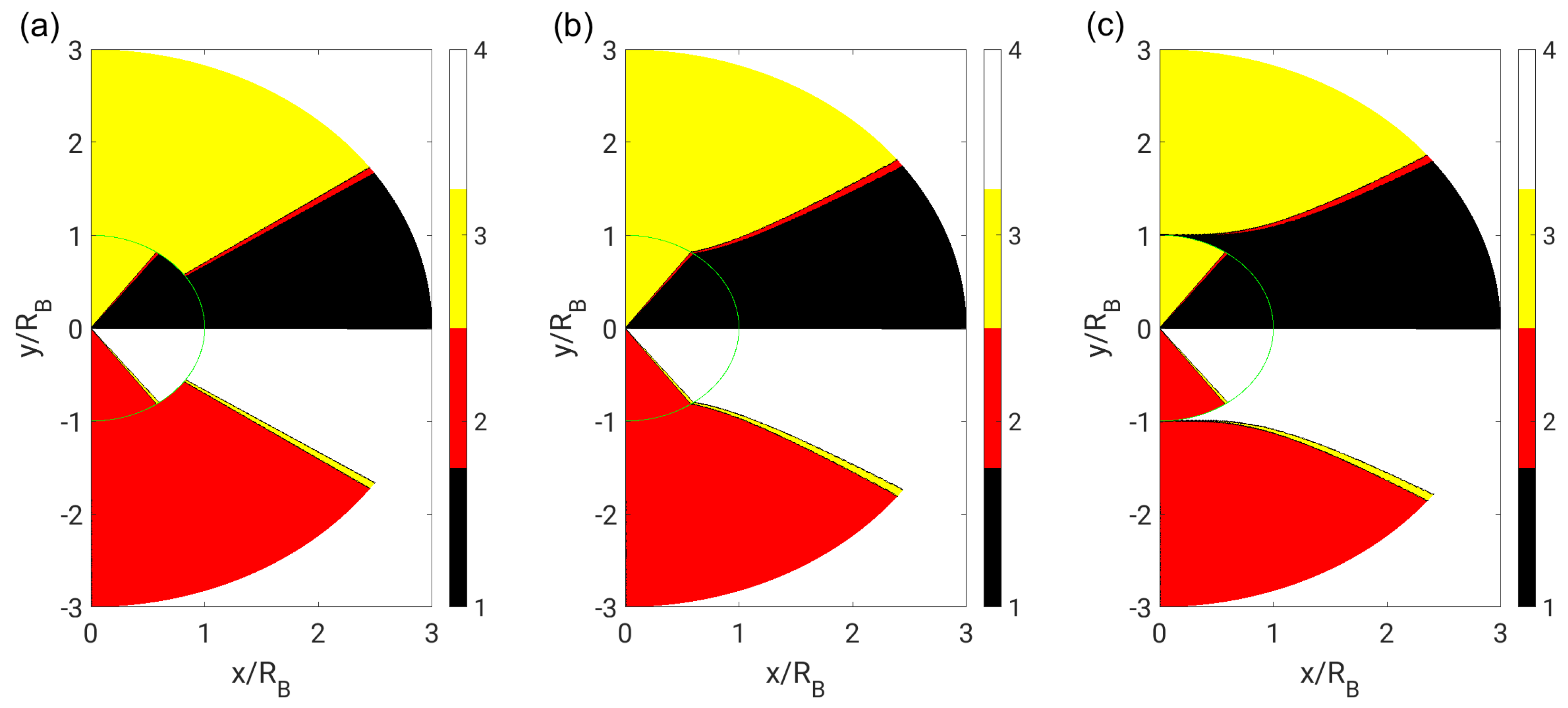
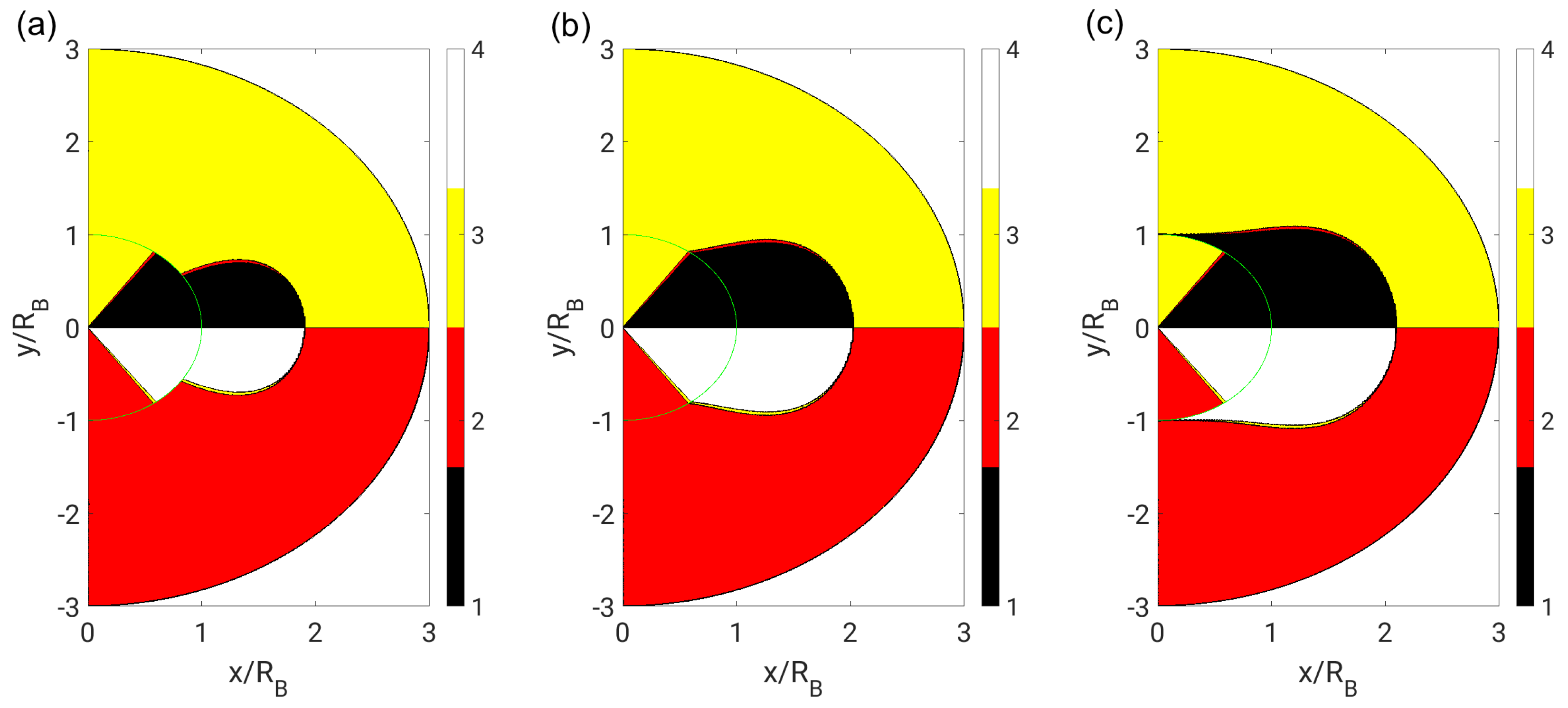
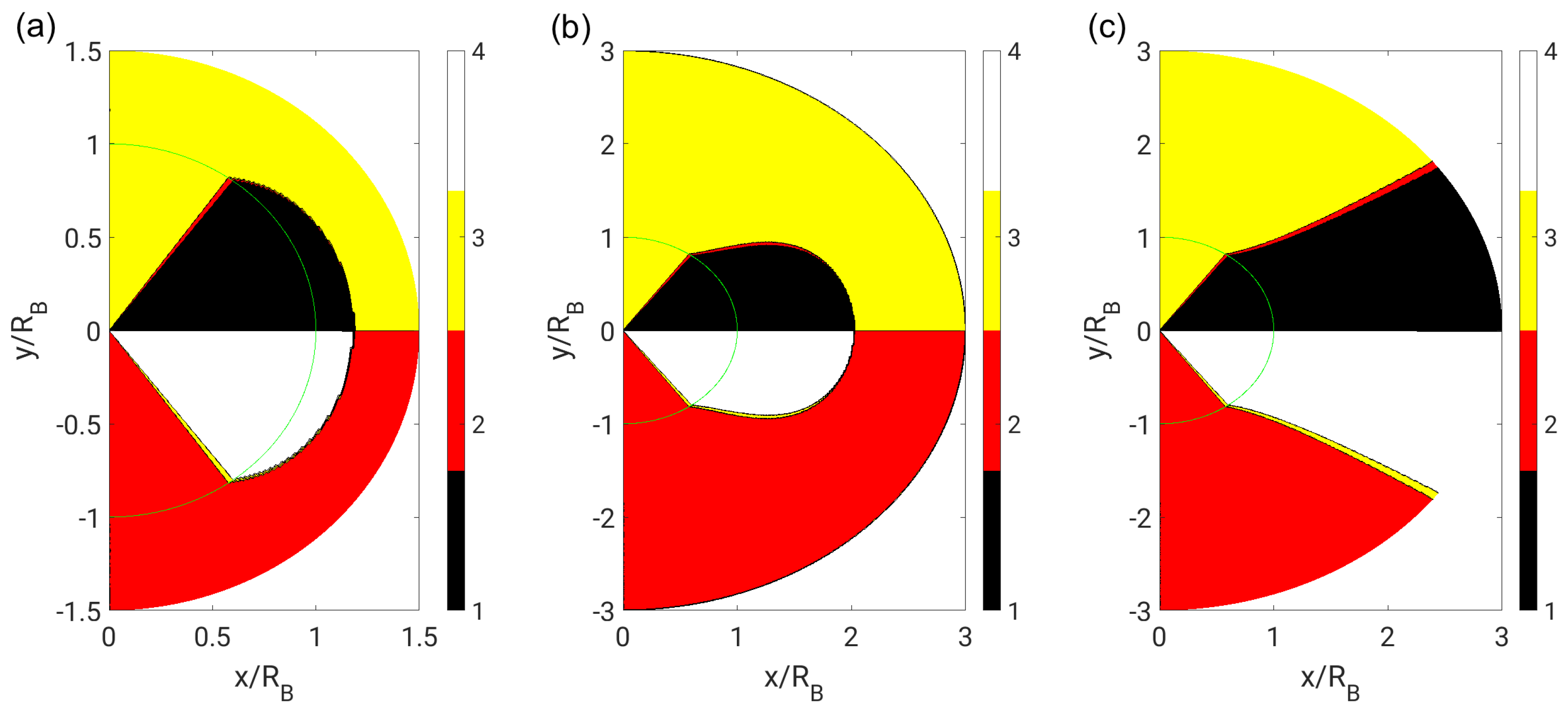
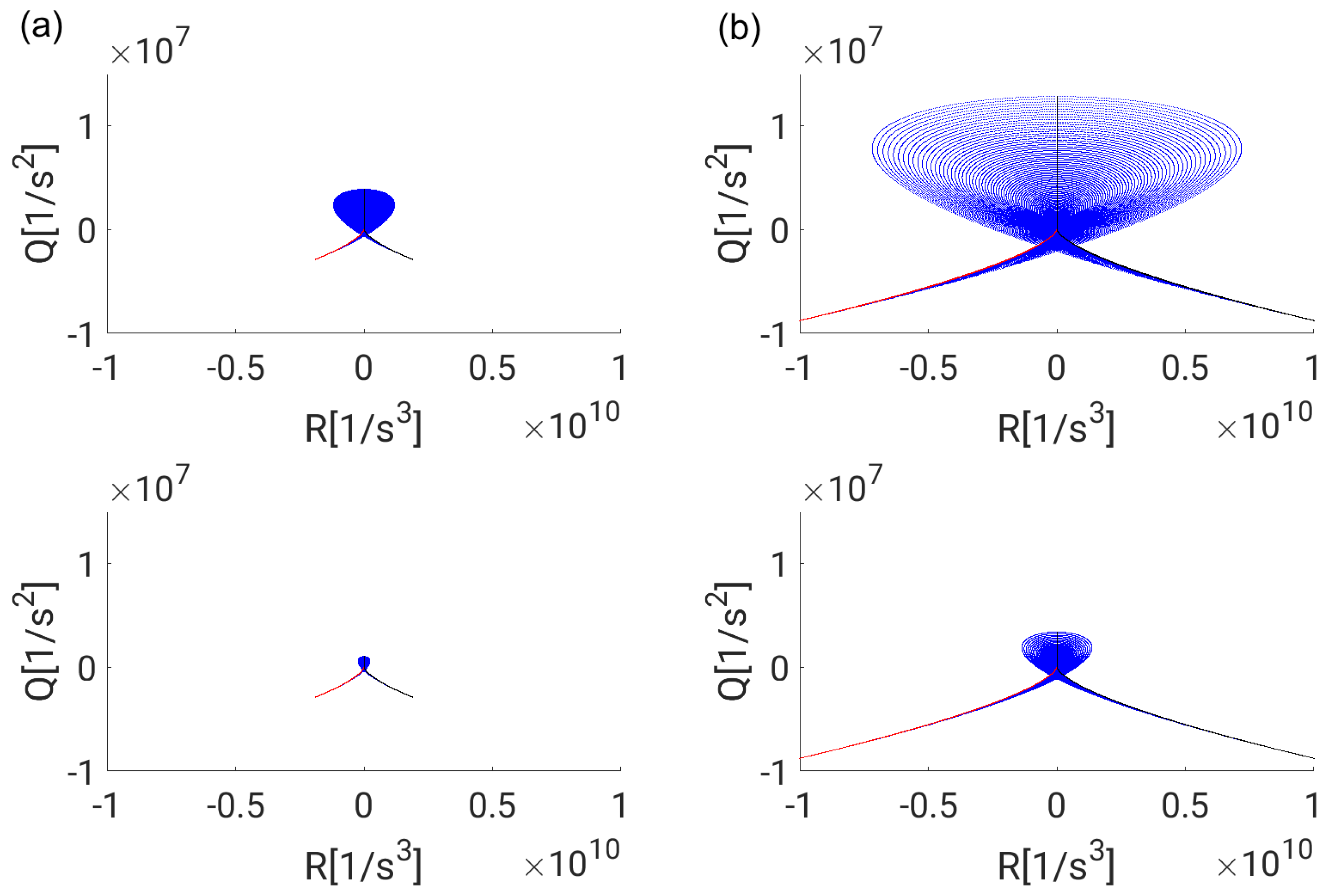
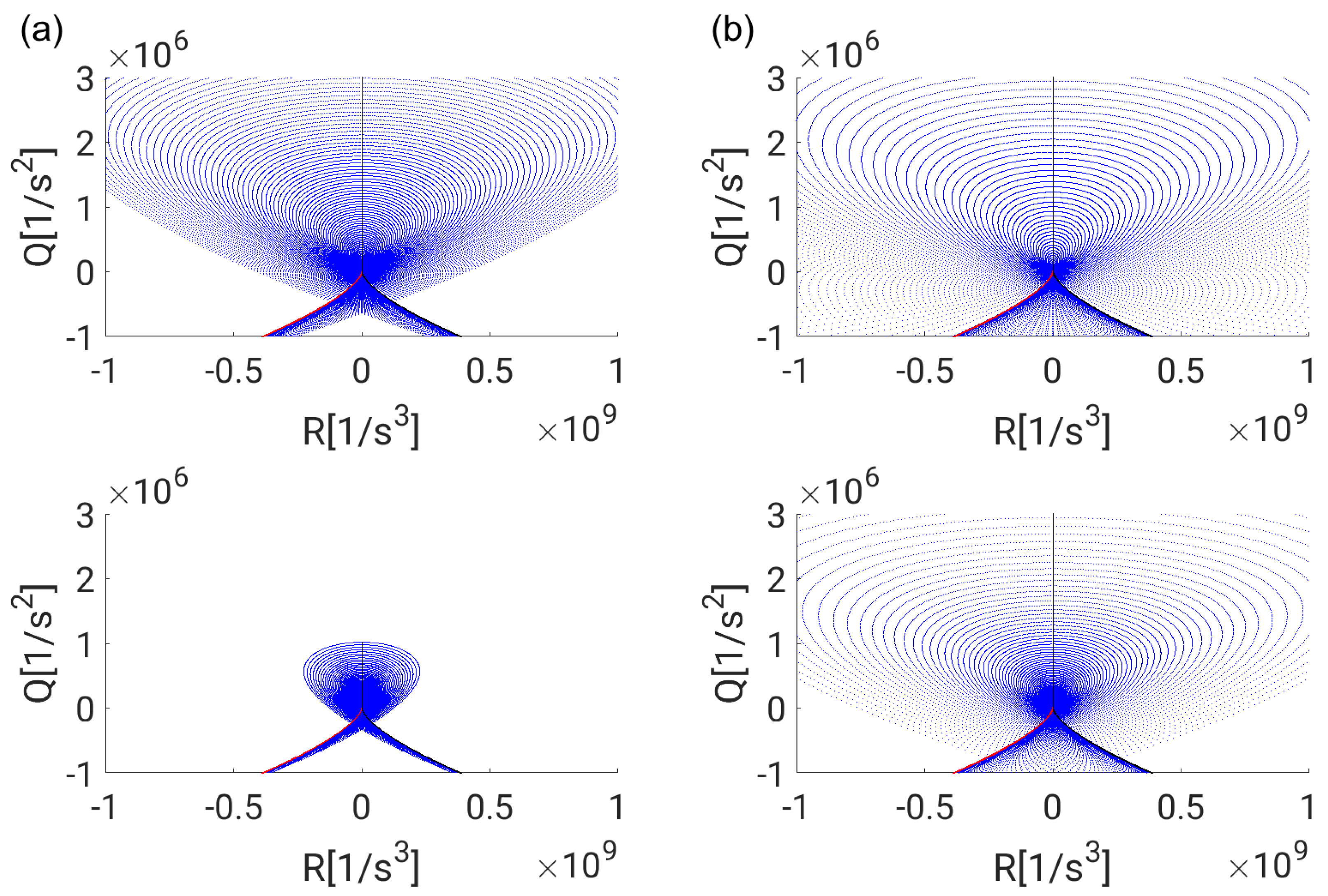
3. Results and Discussion
3.1. Vorticity Field
3.2. Invariants and Flow Topologies
3.3. Phase-Space Projection
4. Concluding Remarks
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| A | velocity gradient tensor |
| D | discriminant |
| unit normal | |
| p | pressure |
| P | first invariant of the velocity gradient tensor |
| Q | second invariant of the velocity gradient tensor |
| r | radial coordinate |
| bubble radius | |
| wall or symmetry line distance | |
| R | third invariant of the velocity gradient tensor |
| Reynolds number | |
| u | velocity component |
| velocity vector | |
| V | volume |
| abbreviation | |
| circumferential coordinate | |
| eigenvalue | |
| dynamic viscosity | |
| dynamic viscosity ratio | |
| azimuthal coordinate | |
| stream function | |
| vorticity component | |
| vorticity vector |
References
- Sadhal, S.; Ayyaswamy, P.; Chung, J. Transport Phenomena with Drops and Bubbles; Springer Science and Business Media: New York, NY, USA, 2012. [Google Scholar]
- Satapathy, R.; Smith, W. The motion of single immiscible drops through a liquid. J. Fluid Mech. 1961, 10, 561–570. [Google Scholar] [CrossRef]
- Chong, M.; Perry, A.; Cantwell, B. A general classification of three-dimensional flow fields. Phys. Fluids A Fluid Dyn. 1990, 2, 765–777. [Google Scholar] [CrossRef]
- Perry, A.; Chong, M. A description of eddying motions and flow patterns using critical-point concepts. Ann. Rev. Fluid Mech. 1987, 19, 125–155. [Google Scholar] [CrossRef]
- Chong, M.; Soria, J.; Perry, A.; Chacin, J.; Cantwell, B.; Na, Y. Turbulence structures of wall-bounded shear flows found using DNS data. J. Fluid Mech. 1998, 357, 225–247. [Google Scholar] [CrossRef]
- Elsinga, G.; Marusic, I. Universal aspects of small-scale motions in turbulence. J. Fluid Mech. 2010, 662, 514–539. [Google Scholar] [CrossRef]
- Wacks, D.; Chakraborty, N.; Klein, M.; Arias, P.; Im, H. Flow topologies in different regimes of premixed turbulent combustion: A direct numerical simulation analysis. Phys. Rev. Fluids 2016, 1, 083401. [Google Scholar] [CrossRef]
- Dopazo, C.; Martín, J.; Hierro, J. Local geometry of isoscalar surfaces. Phys. Rev. E 2007, 76, 056316. [Google Scholar] [CrossRef] [PubMed]
- Hasslberger, J.; Klein, M.; Chakraborty, N. Local flow topology analysis applied to bubble-induced turbulence. In Proceedings of the 12th International ERCOFTAC Symposium on Engineering Turbulence Modelling and Measurements, Montpellier, France, 26–28 September 2018. [Google Scholar]
- Hasslberger, J.; Klein, M.; Chakraborty, N. Flow topologies in bubble-induced turbulence: a direct numerical simulation analysis. J. Fluid Mech. 2018, 857, 270–290. [Google Scholar] [CrossRef]
- Hasslberger, J.; Ketterl, S.; Klein, M.; Chakraborty, N. Flow topologies in primary atomization of liquid jets: A direct numerical simulation analysis. J. Fluid Mech. 2019, 859, 819–838. [Google Scholar] [CrossRef]



© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hasslberger, J.; Marten, S.; Klein, M. A Theoretical Investigation of Flow Topologies in Bubble- and Droplet-Affected Flows. Fluids 2019, 4, 117. https://doi.org/10.3390/fluids4030117
Hasslberger J, Marten S, Klein M. A Theoretical Investigation of Flow Topologies in Bubble- and Droplet-Affected Flows. Fluids. 2019; 4(3):117. https://doi.org/10.3390/fluids4030117
Chicago/Turabian StyleHasslberger, Josef, Svenja Marten, and Markus Klein. 2019. "A Theoretical Investigation of Flow Topologies in Bubble- and Droplet-Affected Flows" Fluids 4, no. 3: 117. https://doi.org/10.3390/fluids4030117
APA StyleHasslberger, J., Marten, S., & Klein, M. (2019). A Theoretical Investigation of Flow Topologies in Bubble- and Droplet-Affected Flows. Fluids, 4(3), 117. https://doi.org/10.3390/fluids4030117





