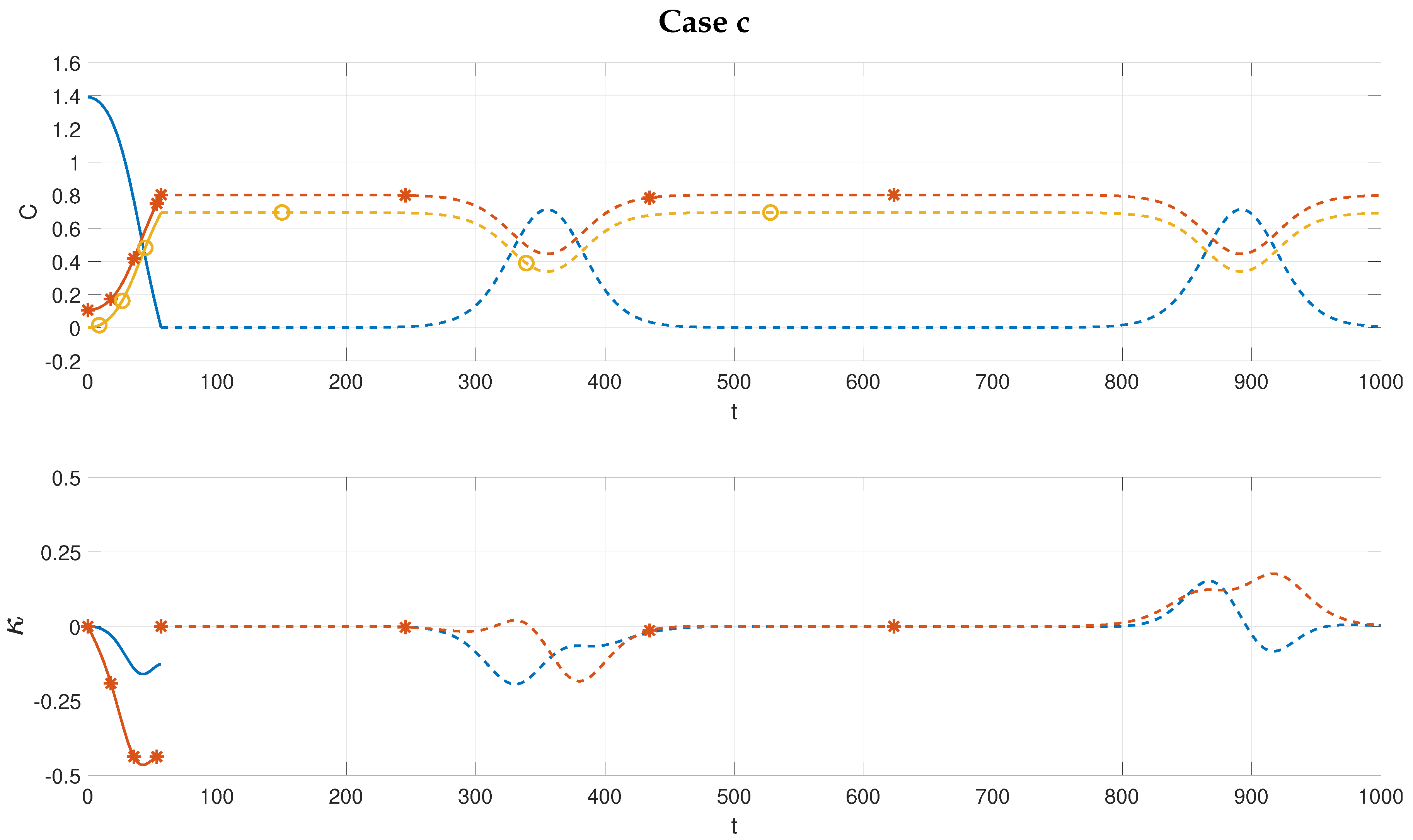On the Generalized Kinetic Equation for Surface Gravity Waves, Blow-Up and Its Restraint

Abstract

This article is concerned with the non-linear interaction of homogeneous random ocean surface waves. Under this umbrella, numerous kinetic equations have been derived to study the evolution of the spectral action density, each employing slightly different assumptions. Using analytical and numerical tools, and providing exact formulas, we demonstrate that the recently derived generalized kinetic equation exhibits blow up in finite time for certain degenerate quartets of waves. This is discussed in light of the assumptions made in the derivation, and this equation is contrasted with other kinetic equations for the spectral action density.

1. Introduction

The dominant non-linear effect on the propagation of surface gravity waves in deep water is due to the resonant and almost resonant four-wave interactions discovered by Phillips [1]. This process of four-wave interaction is succinctly described by the Zakharov equation [2] which is a deterministic model accurate to third order in the wave steepness.

In the continuous case the Zakharov equation is written in terms of complex amplitudes $B(\mathbf{k},t)$ as

$$\frac{\partial B_0}{\partial t}=-i{\int \int \int }_{-\infty }^{\infty }{T}_{0,1,2,3}{B}_1^{*}{B}_2{B}_3{\delta }_{0+1-2-3}{e}^{i{\mathsf{\Delta }}_{0,1}^{2,3}t}d{\mathbf{k}}_{1}d{\mathbf{k}}_{2}d{\mathbf{k}}_3.$$

(1)

Here, and elsewhere, subscripts denote a wavenumber component, so $B_i=B({\mathbf{k}}_i,t)$. The wavenumber resonance condition for quartets of waves is evident in the delta function ${\delta }_{0+1-2-3}=\delta ({\mathbf{k}}_0+{\mathbf{k}}_1-{\mathbf{k}}_2-{\mathbf{k}}_3),$ while the frequency detuning ${\mathsf{\Delta }}_{0,1}^{2,3}={\omega }_0+{\omega }_1-{\omega }_2-{\omega }_3$ marks the departure from exact resonance. The asterisk denotes complex conjugation, while the kernel $T_{0,1,2,3}=T({\mathbf{k}}_0,{\mathbf{k}}_1,{\mathbf{k}}_2,{\mathbf{k}}_3)$ is given in Krasitskii [3], and throughout this article the dispersion relation for deep water ${\omega }^2=g\left|\mathbf{k}\right|$, is used.

To describe averaged properties of a wave field, a statistical theory of wave evolution was developed in the 1950s. This culminated in Hasselmann’s [4] derivation of what is often called the kinetic equation for water waves, or the Hasselmann equation. This equation describes the time evolution of the wave spectral density due to four-wave interactions in exact resonance.

The Hasselmann equation is derived under three basic premises that, in principle, pose limitations on its applicability. These assumptions are (i) spatial homogeneity and weak non-Gaussianity of the wave field, (ii) slow temporal evolution of the spectral density on a time-scale $\mathcal{O}\left({\epsilon }^{-4}\right)$, for $\epsilon$ a typical wave steepness, and (iii) an assumption of quasi-stationarity for the fourth order cumulants.

One of the consequences of assumption (iii) is that near resonant interactions are ruled out in Hasselmann’s equation. In the deterministic description, near resonant interactions are responsible for the side-band instability of a uniform wave train. Stiassnie and Shemer [5] have further highlighted the equal importance of nearly resonant and exactly resonant interactions for the Zakharov equation. It is therefore desirable to include them in any statistical model.

The limitation imposed by quasi-stationarity was first studied in detail by Janssen [6], who derived a more general form of Hasselmann’s equation. Janssen’s equation essentially modifies (iii) by assuming the wave field to be strictly Gaussian at an arbitrary (but finite) initial time $t_0$. This has the immediate consequence that a Dirac delta function appearing in Hasselmann’s equation is smeared out into a time dependent term involving the frequency detuning ${\mathsf{\Delta }}_{0,1}^{2,3}$, thereby including near resonant interactions. Although Janssen’s equation includes near-resonant interactions, which leads to a time evolution on the time scale $\mathcal{O}\left({\epsilon }^{-2}\right)$, it still uses a simplification that is justified under assumption (ii).

A simple physical example serves to demonstrate why it is advantageous to dispense with assumption (ii). Assuming a wave of period $T=10$ s and steepness $\epsilon =0.1$ leads to an evolution time–scale $t_4=T/{\epsilon }^4\approx 27.7$ hours. To justify wave evolution under this paradigm, a sea-state must remain spatially homogeneous on such a time-scale, which is clearly an idealization. On the other hand, evolution on a faster time scale $t_2=T/{\epsilon }^2$, or $16.6$ min in the physical example above, would be a more suitable alternative.

A generalization of Janssen’s approach was presented by Annenkov and Shrira [7]. Their result, called the generalized kinetic equation (GKE) is obtained as an intermediate step in the derivation of the kinetic equation, before assumptions (ii) and (iii) are imposed. This results in an equation valid on a fast time scale of order $\mathcal{O}\left({\epsilon }^{-2}\right)$. The equation was further generalized by Gramstad and Stiassnie [8], who also included higher order terms corresponding to the non-linear Stokes correction in their so-called phase averaged equation (PAE). Recently Gramstad and Babanin implemented the GKE, without Stokes’ correction, in the third generation wave model WAVEWATCH-III [9].

The two equations dealt with in what follows will be Janssen’s kinetic equation (henceforth JKE), and the generalized kinetic equation (GKE) of Annenkov and Shrira. While some recent efforts have been focused on the influence of inhomogeneities on the evolution of wave spectra, see Stuhlmeier and Stiassnie [10], thereby dispensing with assumption (i) along lines first suggested by Crawford et al. [11], it is important to put the homogeneous theory on sound footing. Thus, while JKE and GKE both essentially use the spatial homogeneity and weak non-Gaussianity of the wave field, they are differentiated by assumption (ii) on the slow evolution of the wave field. It will be shown that this seemingly small difference has a significant effect on the qualitative behavior of the solutions.

Our main result is that some solutions of the generalized kinetic equation exhibit blow-up. This means that the components of the spectrum tend to infinity in finite time. This raises a red flag on the GKE, as the blow-up is possible for initial conditions that are physically legitimate. Moreover, based only on the initial conditions we characterize when the blow-up occurs. One possible method to circumvent blow-up, by means of phase-mixing, is also explored in the appendix. On the other hand we show that solutions of the JKE always remain bounded, even in cases when there is blow-up in the GKE. Surprisingly, away from blow-up, periodic and bounded solutions of the GKE are qualitatively similar to those of the JKE.

2. Theoretical Background

Our starting point is the discrete version of Zakharov’s equation, which assumes that the complex amplitudes can be written as

$$B(\mathbf{k},t)=\sum _{j=1}^N{B}_j\left(t\right)\delta ({\mathbf{k}}_j-\mathbf{k}).$$

(2)

Substituting (2) into (1) yields the following system of ordinary differential equations for the discrete complex amplitudes $B_j$

$$\frac{d}{dt}{B}_j=-i\sum _{m,n,p}{T}_{j,m,n,p}{\delta }_{j,m}^{n,p}{e}^{i{\mathsf{\Delta }}_{j,m}^{n,p}t}{B}_m^{*}{B}_n{B}_p,$$

(3)

where ${\delta }_{j,m}^{n,p}$ denotes the Kronecker delta

$${\delta }_{j,m}^{n,p}=\left\{\begin{array}{c}1\mathrm{when}{\mathbf{k}}_j+{\mathbf{k}}_m={\mathbf{k}}_n+{\mathbf{k}}_p,\hfill \\ 0\mathrm{otherwise}.\hfill \end{array}\right.$$

(4)

Randomness is introduced by regarding the complex amplitudes as stochastic processes, where $\langle ·\rangle$ is used to denote an ensemble average. Assuming that $\langle B_j\rangle =0$ the next quantities of interest are the second-order moments, the pair correlators. Homogeneity, from assumption (i) above, imposes the following constraint on the correlators

$$\langle B_i^{*}\left(t\right)B_j\left(t\right)\rangle =C_j\left(t\right){\delta }_i^j.$$

(5)

The set of functions $C_j\left(t\right)$ form the wave-action spectrum and ${\delta }_i^j$ is the Kronecker delta.

The evolution equation for the wave action is found by multiplying (3) by $B_j^{*}$ and adding its complex conjugate. The resulting equation is

$$\frac{d}{dt}{C}_j=2\sum _{m,n,p}{T}_{j,m,n,p}{\delta }_{j,m}^{n,p}Im\left[e^{i{\mathsf{\Delta }}_{j,m}^{n,p}t}\langle B_j^{*}{B}_m^{*}{B}_n{B}_p\rangle \right].$$

(6)

Higher order averages, such as the one appearing on the right hand side of (6), can be decomposed into products of lower order averages as follows

$$\langle B_j^{*}{B}_m^{*}{B}_n{B}_p\rangle =C_j{C}_m\left({\delta }_j^n{\delta }_m^p+{\delta }_j^p{\delta }_m^n\right)+{\kappa }_{j,m,n,p},$$

(7)

where $\kappa$ is called the fourth-order cumulant. It is well known that all higher-order cumulants vanish for a Gaussian distribution, thus $\kappa$ indicates a deviation from Gaussianity.

Substituting (7) into (6), the evolution equation for the wave action becomes

$$\frac{d}{dt}{C}_j=2\sum _{m,n,p}{T}_{j,m,n,p}{\delta }_{j,m}^{n,p}Im\left[e^{i{\mathsf{\Delta }}_{j,m}^{n,p}t}{\kappa }_{j,m,n,p}\right].$$

(8)

In order to find an equation for $\kappa$ we differentiate the fourth order average as follows

$$\frac{d}{dt}\langle B_j^{*}{B}_m^{*}{B}_n{B}_p\rangle =\langle \frac{d{B}_j^{*}}{dt}{B}_m^{*}{B}_n{B}_p\rangle +\langle B_j^{*}\frac{d{B}_m^{*}}{dt}{B}_n{B}_p\rangle +\langle B_j^{*}{B}_m^{*}\frac{B_n}{dt}{B}_p\rangle +\langle B_j^{*}{B}_m^{*}{B}_n\frac{B_p}{dt}\rangle ,$$

(9)

and use (3) in the right hand side of (9) to yield

$$\begin{array}{cc}\hfill \frac{d}{dt}\langle B_j^{*}{B}_m^{*}{B}_n{B}_p\rangle & =i\sum _{u,v,w}{T}_{j,u,v,w}{\delta }_{j,u}^{v,w}{e}^{-i{\mathsf{\Delta }}_{j,u}^{v,w}t}\langle B_u{B}_v^{*}{B}_w^{*}{B}_m^{*}{B}_n{B}_p\rangle \hfill \\ & +i\sum _{u,v,w}{T}_{m,u,v,w}{\delta }_{m,u}^{v,w}{e}^{-i{\mathsf{\Delta }}_{m,u}^{v,w}t}\langle B_j^{*}{B}_u{B}_v^{*}{B}_w^{*}{B}_n{B}_p\rangle \hfill \\ & -i\sum _{u,v,w}{T}_{n,u,v,w}{\delta }_{n,u}^{v,w}{e}^{i{\mathsf{\Delta }}_{n,u}^{v,w}t}\langle B_j^{*}{B}_m^{*}{B}_u^{*}{B}_v{B}_w{B}_p\rangle \hfill \\ & -i\sum _{u,v,w}{T}_{p,u,v,w}{\delta }_{p,u}^{v,w}{e}^{i{\mathsf{\Delta }}_{p,u}^{v,w}t}\langle B_j^{*}{B}_m^{*}{B}_n{B}_u^{*}{B}_v{B}_w\rangle .\hfill \end{array}$$

(10)

To close what would otherwise be an infinite hierarchy of equations we invoke the weak non-Gaussianity assumption. Akin to (7), one may decompose the sixth order averages in (10) into products of lower order averages and cumulants, as detailed in Lvov et al [12]. The terms are then ordered with respect to the small parameter $\epsilon$; $C_j=\mathcal{O}\left({\epsilon }^2\right)$ and ${\kappa }_{j,m,n,p}=o\left({\epsilon }^4\right)$, as in Gramstad and Stiassnie [8]. Therefore, up to order $\mathcal{O}\left({\epsilon }^4\right),$ the decomposition of the averages is

$$\langle B_j^{*}{B}_m^{*}{B}_n^{*}{B}_p{B}_u{B}_v\rangle =C_j{C}_m{C}_n\left({\delta }_j^p({\delta }_m^u{\delta }_n^v+{\delta }_m^v{\delta }_n^u)+{\delta }_j^u({\delta }_m^v{\delta }_n^p+{\delta }_m^p{\delta }_n^v)+{\delta }_j^v({\delta }_m^u{\delta }_n^p+{\delta }_m^p{\delta }_n^u)\right).$$

(11)

Differentiating (7) with respect to t and using Equation (10) together with (11) for the sixth-order terms we reach the following equation for $\kappa$, valid up to order $\mathcal{O}\left({\epsilon }^4\right)$

$$\frac{d}{dt}{\kappa }_{j,m,n,p}=2i{T}_{j,m,n,p}{\delta }_{j,m}^{n,p}{e}^{-i{\mathsf{\Delta }}_{j,m}^{n,p}t}\left[C_n{C}_p(C_j+C_m)-C_j{C}_m(C_n+C_p)\right].$$

(12)

Equations (8) and (12) form the discrete version of the generalized kinetic equation first derived by Annenkov and Shrira [7] for a continuous wavenumber spectrum. Later, Gramstad and Stiassnie [8] derived their phase-averaged equation, by extending (11) to include terms up to $\mathcal{O}\left({\epsilon }^6\right).$ Our focus shall remain on the GKE, rather than the higher order extension.

The GKE contains both Janssen’s and Hasselmann’s kinetic equations. To derive Janssen’s result we set ${\kappa }_{j,m,n,p}\left(0\right)=0$ and integrate Equation (12) from 0 to $t,$ yielding

$${\kappa }_{j,m,n,p}=2i{T}_{j,m,n,p}{\int }_0^t{e}^{-i{\mathsf{\Delta }}_{j,m}^{n,p}s}\left[C_n{C}_p(C_j+C_m)-C_j{C}_n(C_m+C_p)\right]ds.$$

(13)

Now we use assumption (ii). We assume that $\left[C_n{C}_p(C_j+C_m)-C_j{C}_n(C_m+C_p)\right]$ varies slowly in time and we extract it out of the integral to obtain

$${\kappa }_{j,m,n,p}=-2T_{j,m,n,p}\left[C_n{C}_p(C_j+C_m)-C_j{C}_n(C_m+C_p)\right]\frac{\left(e^{-i{\mathsf{\Delta }}_{j,m}^{n,p}t}-1\right)}{{\mathsf{\Delta }}_{j,m}^{n,p}}.$$

(14)

Substituting (14) into (8) yields a single equation for C:

$$\frac{d}{dt}{C}_j=4\sum _{m,n,p}{T}_{j,m,n,p}^2{\delta }_{j,m}^{n,p}\left[C_n{C}_p(C_j+C_m)-C_j{C}_n(C_m+C_p)\right]\frac{sin\left({\mathsf{\Delta }}_{j,m}^{n,p}t\right)}{{\mathsf{\Delta }}_{j,m}^{n,p}}.$$

(15)

Equation (15) is the discrete version of Janssen’s kinetic equation (denoted JKE), originally derived in continuous form by Janssen [6].

To elucidate the difference between the JKE (15) and the GKE (8) and (12), it is instructive to consider simple cases with few waves. The fundamental interaction in deep water is between quartets of waves, and the simplest such case consists of three distinct wavenumbers forming a degenerate quartet.

3. Formulation for a Degenerate Quartet

A degenerate quartet of waves consists of a discrete wave spectrum of the form

$$B(\mathbf{k},t)=B_a\left(t\right)\delta ({\mathbf{k}}_a-\mathbf{k})+B_b\left(t\right)\delta ({\mathbf{k}}_b-\mathbf{k})+B_c\left(t\right)\delta ({\mathbf{k}}_c-\mathbf{k}),$$

(16)

where the wave-vectors ${\mathbf{k}}_a$, ${\mathbf{k}}_b$ and ${\mathbf{k}}_c$ satisfy the relation

$$2{\mathbf{k}}_a={\mathbf{k}}_b+{\mathbf{k}}_c.$$

(17)

The JKE and GKE thus describe the time evolution of the wave action $C_j=\langle |B_j{|}^2\rangle$, for $j=a,b,c$. For the GKE (8) and (12), taking all sums over only the three indices $a,b$ and c yields, with the Kronecker delta function (17), the system of coupled ordinary differential equations

$$\begin{array}{cc}\hfill \frac{d}{dt}{C}_a& =4T_{a,a,b,c}Im\left[e^{i{\mathsf{\Delta }}_{a,a}^{b,c}t}{\kappa }_{a,a,b,c}\right],\hfill \end{array}$$

(18)

$$\begin{array}{cc}\hfill \frac{d}{dt}{C}_b& =-2T_{a,a,b,c}Im\left[e^{i{\mathsf{\Delta }}_{a,a}^{b,c}t}{\kappa }_{a,a,b,c}\right],\hfill \end{array}$$

(19)

$$\begin{array}{cc}\hfill \frac{d}{dt}{C}_c& =-2T_{a,a,b,c}Im\left[e^{i{\mathsf{\Delta }}_{a,a}^{b,c}t}{\kappa }_{a,a,b,c}\right],\hfill \end{array}$$

(20)

$$\begin{array}{cc}\hfill \frac{d}{dt}{\kappa }_{a,a,b,c}& =2i{T}_{a,a,b,c}{e}^{-i{\mathsf{\Delta }}_{a,a}^{b,c}t}\left[2C_a{C}_b{C}_c-C_a^2\left(C_b+C_c\right)\right].\hfill \end{array}$$

(21)

Likewise, the JKE (15) reduces to the following system of equations for the discrete wave action spectrum (16)

$$\begin{array}{cc}\hfill \frac{d}{dt}{C}_a& =16T_{a,a,b,c}^2\left[C_a{C}_b{C}_c-\frac{1}{2}{C}_a^2(C_b+C_c)\right]\frac{sin\left({\mathsf{\Delta }}_{a,a}^{b,c}t\right)}{{\mathsf{\Delta }}_{a,a}^{b,c}},\hfill \end{array}$$

(22)

$$\begin{array}{cc}\hfill \frac{d}{dt}{C}_b& =-8T_{a,a,b,c}^2\left[C_a{C}_b{C}_c-\frac{1}{2}{C}_a^2(C_b+C_c)\right]\frac{sin\left({\mathsf{\Delta }}_{a,a}^{b,c}t\right)}{{\mathsf{\Delta }}_{a,a}^{b,c}},\hfill \end{array}$$

(23)

$$\begin{array}{cc}\hfill \frac{d}{dt}{C}_c& =-8T_{a,a,b,c}^2\left[C_a{C}_b{C}_c-\frac{1}{2}{C}_a^2(C_b+C_c)\right]\frac{sin\left({\mathsf{\Delta }}_{a,a}^{b,c}t\right)}{{\mathsf{\Delta }}_{a,a}^{b,c}}.\hfill \end{array}$$

(24)

4. Analytic Solution for the GKE

In this section we study the initial value problem associated with the system of equations (18)–(21). We begin by defining an auxiliary real function $Z\left(t\right)$ as the solution to the initial value problem

$$\frac{dZ}{dt}=Im\left[e^{i\mathsf{\Delta }t}\kappa \right],$$

(25)

with $Z\left(0\right)=0$. All subsequent calculations are based on the degenerate quartet, so we will omit subscripts and denote $\mathsf{\Delta }={\mathsf{\Delta }}_{a,a}^{b,c}$, $\kappa ={\kappa }_{a,a,b,c}$ and $T=T_{a,a,b,c}$ where there is no risk of confusion.

In terms of $Z,$ Equation (18)–(20) simplify to

$$\frac{d}{dt}{C}_a=-2\frac{d}{dt}{C}_b=-2\frac{d}{dt}{C}_b=4T\frac{d}{dt}Z.$$

(26)

Denoting the initial values by $C_a\left(0\right)={\rho }_a$, $C_b\left(0\right)={\rho }_b$ and $C_c\left(0\right)={\rho }_c$ and integrating in time we find an expression for $C_j$ in terms of Z:

$$\begin{array}{cc}\hfill C_a\left(t\right)& ={\rho }_a+4TZ,\hfill \end{array}$$

(27)

$$\begin{array}{cc}\hfill C_b\left(t\right)& ={\rho }_b-2TZ,\hfill \end{array}$$

(28)

$$\begin{array}{cc}\hfill C_c\left(t\right)& ={\rho }_c-2TZ.\hfill \end{array}$$

(29)

Our goal is to find an equation that determines $Z\left(t\right)$. To this end we define a further auxiliary real function

$$W=Re\left[e^{i\mathsf{\Delta }t}\kappa \right].$$

(30)

Differentiating in time and using (21) yields

$$\frac{d}{dt}W=-\mathsf{\Delta }Im\left[e^{i\mathsf{\Delta }t}\kappa \right]+Re\left[4iT\left[C_a{C}_b{C}_c-\frac{1}{2}{C}_a^2\left(C_b+C_c\right)\right]\right]=-\mathsf{\Delta }\frac{d}{dt}Z,$$

(31)

which becomes, after integration,

$$W=Re\left[{\kappa }_0\right]-\mathsf{\Delta }Z,$$

(32)

where $\kappa \left(0\right)={\kappa }_0$. Taking the time derivative of (25) yields

$$\frac{d^{2}Z}{d{t}^2}=\mathsf{\Delta }W+4T\left[C_a{C}_b{C}_c-\frac{1}{2}{C}_a^2(C_b+C_c)\right].$$

(33)

Now we use Equations (27)–(29) to express the right hand side of (33) in terms of Z. The resulting equation has the form

$$\frac{d^{2}Z}{d{t}^2}=P_3\left(Z\right)=b_0+b_{1}Z+b_2{Z}^2+b_3{Z}^3,$$

(34)

where the coefficients of $P_3$ are:

$$\begin{array}{cc}\hfill b_0& =\mathsf{\Delta }Re\left[{\kappa }_0\right]+4T\left[{\rho }_a{\rho }_b{\rho }_c-\frac{1}{2}{\rho }_a^2({\rho }_b+{\rho }_c)\right],\hfill \end{array}$$

(35)

$$\begin{array}{cc}\hfill b_1& =-{\mathsf{\Delta }}^2+4T^2\left[4{\rho }_b{\rho }_c-6{\rho }_a{\rho }_b-6{\rho }_a{\rho }_c+2{\rho }_a^2\right],\hfill \end{array}$$

(36)

$$\begin{array}{cc}\hfill b_2& =4T^3\left[20{\rho }_a-16{\rho }_b-16{\rho }_c\right],\hfill \end{array}$$

(37)

$$\begin{array}{cc}\hfill b_3& =192T^4.\hfill \end{array}$$

(38)

We multiply both sides of (34) by $dZ/dt$ and integrate

$${\left(\frac{dZ}{dt}\right)}^2=2{\int }_0^t{P}_3\left(Z\right)\frac{d}{dt}Zdt=2{\int }_0^Z{P}_3\left(z\right)dz,$$

(39)

obtaining a non-linear first order differential equation for Z

$${\left(\frac{dZ}{dt}\right)}^2=P_4\left(Z\right)=c_0+c_{1}Z+c_2{Z}^2+c_3{Z}^3+c_4{Z}^4.$$

(40)

The coefficients of $P_4$ are:

$$\begin{array}{cc}\hfill c_0& ={\left(Im\left[{\kappa }_0\right]\right)}^2,\hfill \end{array}$$

(41)

$$\begin{array}{cc}\hfill c_1& =2b_0,\hfill \end{array}$$

(42)

$$\begin{array}{cc}\hfill c_2& =b_1,\hfill \end{array}$$

(43)

$$\begin{array}{cc}\hfill c_3& =\frac{2}{3}{b}_2,\hfill \end{array}$$

(44)

$$\begin{array}{cc}\hfill c_4& =\frac{1}{2}{b}_3.\hfill \end{array}$$

(45)

4.1. Analytical Solution for Z

We assume that ${\kappa }_0$ is real, so that 0 is always a root of $P_4$ (see (41)) and we are able to factor $P_4$ as

$$P_4\left(Z\right)=Z{Q}_3\left(Z\right),$$

(46)

where $Q_3\left(Z\right)$ is a cubic polynomial of the form

$$Q_3\left(Z\right)=c_1+c_{2}Z+c_3{Z}^2+c_4{Z}^3$$

(47)

with coefficients given by (42)–(45) (note that the JKE is derived assuming ${\kappa }_0=0$ (see (13)), and for a consistent comparison this initial condition is used in all subsequent examples).

It is known that the nature of the roots of a third order polynomial is determined by the sign of the following discriminant

$$D=18c_4{c}_3{c}_2{c}_1-4c_3^3{c}_1+c_3^2{c}_2^2-4c_4{c}_2^3-27c_4^2{c}_1^2.$$

(48)

If $D>0$ then all non-zero roots of $P_4$ are real and distinct. If $D=0$ then $P_4$ has a multiple non-zero real root. If $D<0$ then $P_4$ has two real roots and two complex conjugate roots. We refer to [13] for a detailed exposition about the discriminant of a cubic polynomial, and formula (48). Knowing the roots of $P_3$ allows us to find exact formulas for the solution of (40).

4.1.1. Case $D>0$

In all cases of interest, when $D>0$, the roots of $P_4$ can be written from largest to smallest as $Z_4>Z_3=0>Z_2>Z_1$. Therefore the solution of (40) can be found by inverting the integral

$$t={\int }_0^Z\frac{dz}{\sqrt{P_4\left(z\right)}}=-{\int }_Z^0\frac{dz}{\sqrt{P_4\left(z\right)}}={\int }_{Z_2}^Z\frac{dz}{\sqrt{P_4\left(z\right)}}-{\int }_{Z_2}^0\frac{dz}{\sqrt{P_4\left(z\right)}},$$

(49)

by applying formulas $\left(254.00\right)$ of Byrd and Friedman [14]. Let

$$K^2=\frac{Z_2(Z_4-Z_1)}{Z_1(Z_4-Z_2)},$$

(50)

be the modulus of the Jacobian elliptic functions sn, cn and dn. Also let

$$\begin{array}{ccc}\hfill \gamma & =& \frac{2}{\sqrt{-(Z_4-Z_2)Z_1}},\hfill \end{array}$$

(51)

$$\begin{array}{ccc}\hfill sin\left(\varphi \right)& =& \sqrt{\frac{Z_1(Z-Z_3)}{Z_3(Z-Z_1)}}.\hfill \end{array}$$

(52)

Then

$${sn}^{-1}(sin\left(\varphi \right),K)={sn}^{-1}(1,K)+c_4^{1/2}\frac{t}{\gamma }=u,$$

(53)

where ${sn}^{-1}$ is the inverse of sn. In term of u the inverse of (49) is

$$Z=\frac{Z_2{Z}_1({sn}^2(u,K)-1)}{Z_2{sn}^2(u,K)-Z_1},$$

(54)

We point out that the denominator of (54) never vanishes because $Z_1/Z_2>1$ whereas $|{sn}^2(u,K)|\le 1$. Finally we compute the value of $sn(u,K)$ by means of addition formulas for elliptic functions. The resulting expression is

$$sn(u,K)=\frac{cn(c_4^{1/2}t/\gamma ,K)dn(c_4^{1/2}t/\gamma ,K)}{1-K^2{sn}^2(c_4^{1/2}t/\gamma ,K)}.$$

(55)

The solution of (40), given by (54), is a bounded and periodic function of time with period

$$T=\frac{2\gamma {sn}^{-1}(1,K)}{c_4^{1/2}}=\frac{2\gamma }{c_4^{1/2}}\mathcal{K}\left(K\right),$$

(56)

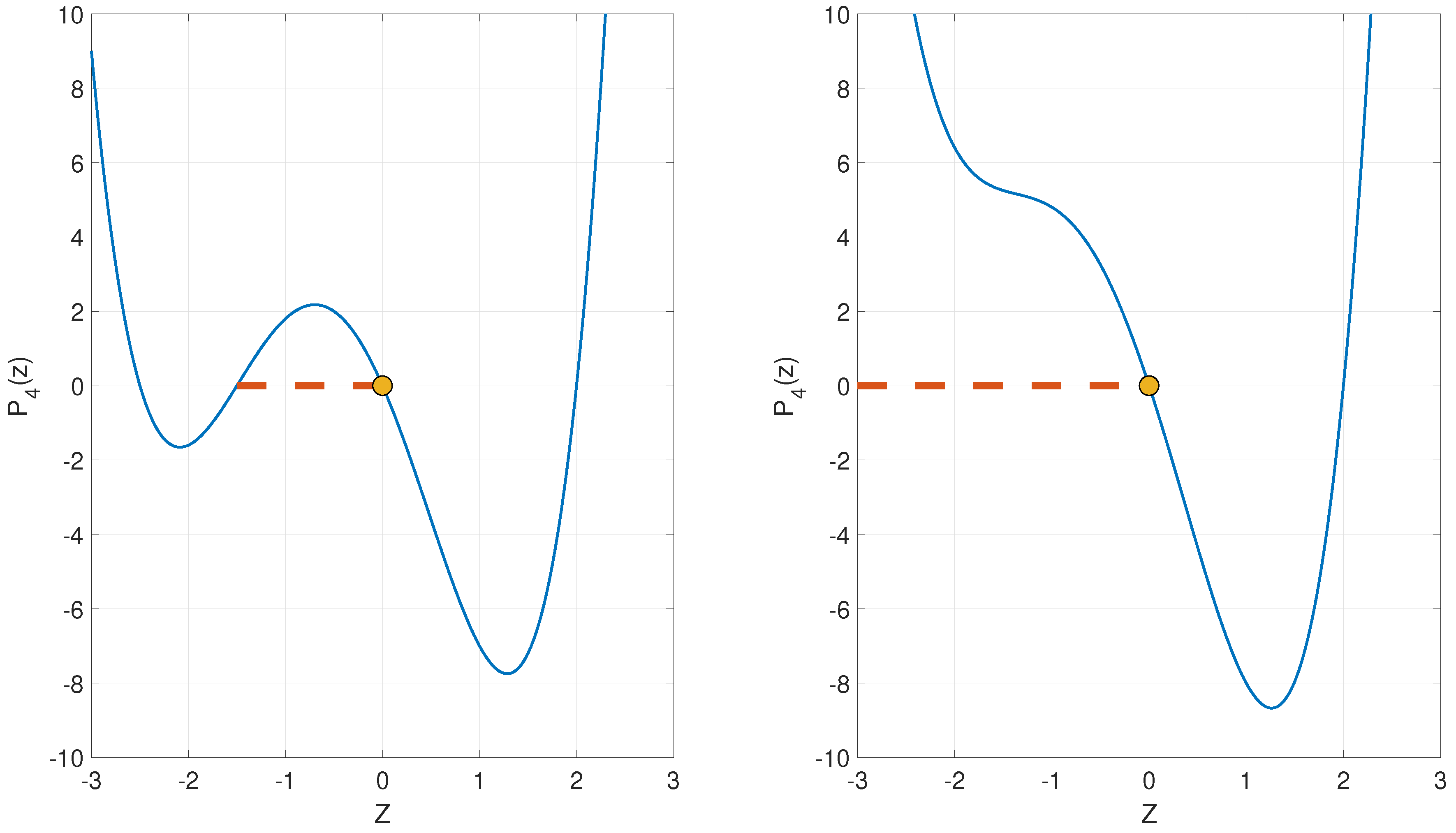
where $\mathcal{K}$ is the complete elliptic integral of the first kind. The qualitative behavior of the solution is shown in the left panel of Figure 1. The function $Z\left(t\right)$ oscillates back and forth between the roots $Z_2$ and $Z_3$ of the polynomial, starting from the initial value $Z_3=0$.

4.1.2. Case $D\le 0$

In this case let $Z_3$, $Z_3^{*}$, $Z_1=0<Z_2$ be the roots of $P_4$. Notice that $D<0$ if and only if $Im\left[Z_3\right]\ne 0$ and $D=0$ if and only if $Im\left[Z_3\right]=0$. In either case the solution of (40) is found by inverting the integral

$$t={\int }_0^Z\frac{dz}{\sqrt{P_4\left(z\right)}}.$$

(57)

The value of (57) is found by applying formulas $\left(260.00\right)$ of Byrd and Friedman [14]. Let

$$K^2=\frac{{\left(|Z_3|+|Z_2+Z_3|\right)}^2-Z_2^2}{4|Z_3\left|\right|Z_2+Z_3|},$$

(58)

be the elliptic modulus and define the parameters

$$\begin{array}{ccc}\hfill \gamma & =& \frac{1}{\sqrt{|Z_3\left|\right|Z_2+Z_3|}},\hfill \end{array}$$

(59)

$$\begin{array}{ccc}\hfill cos\left(\varphi \right)& =& \frac{\left(\right|Z_3|-|Z_2+Z_3\left|\right)Z-Z_2\left|Z_3\right|}{\left(\right|Z_3|+|Z_2+Z_3\left|\right)Z-Z_2\left|Z_3\right|}.\hfill \end{array}$$

(60)

Then

$${cn}^{-1}(cos\left(\varphi \right),K)=-c_4^{1/2}\frac{t}{\gamma }=u.$$

(61)

We invert this relation to find Z as a function of t

$$Z=\frac{Z_2\left|Z_3\right|(1-cn(u,K))}{\left(\right|Z_3|-|Z_2+Z_3\left|\right)-\left(\right|Z_3|+|Z_2+Z_3\left|\right)cn(u,K)}.$$

(62)

In this case we expect the solution to blow up when the denominator of (62) vanishes. The critical time $T_{\infty }$ when this happens is

$$T_{\infty }=\frac{\gamma }{c_4^{1/2}}{cn}^{-1}\left(\frac{|Z_3|-|Z_2+Z_3|}{|Z_3|+|Z_2+Z_3|},K\right).$$

(63)

In the right panel of Figure 1 we show a schematic representation of the blow-up in terms of the roots of the quartic polynomial. The function $Z\left(t\right),$ initially at the root $Z_1=0,$ tends towards $-\infty$ as t goes from 0 to $T_{\infty }$.

5. Analytic Solution of JKE

Our analysis for the JKE follows the steps of the previous section. First we reduce the three coupled Equations (22)–(24) to a single equation for a new auxiliary function. To this end, let $z\left(t\right)$ be the solution of the initial value problem

$$\frac{dz}{dt}=4T\left[C_a{C}_b{C}_c-\frac{1}{2}{C}_a^2(C_b+C_c)\right]\frac{sin\left(\mathsf{\Delta }t\right)}{\mathsf{\Delta }},$$

(64)

with $z\left(0\right)=0$. The relationship between z and C is given by (27)–(29), as in Section 4. This is substituted into the right-hand side of (64) to obtain the following equation for z

$$\frac{dz}{dt}=P_3\left(z\right)\frac{sin\left(\mathsf{\Delta }t\right)}{\mathsf{\Delta }},$$

(65)

where $P_3\left(z\right)$ is a third order polynomial

$$P_3\left(z\right)=d_0+d_{1}z+d_2{z}^2+d_3{z}^3,$$

(66)

with coefficients

$$\begin{array}{cc}\hfill d_0& =4T\left[{\rho }_a{\rho }_b{\rho }_c-\frac{1}{2}{\rho }_a^2({\rho }_b+{\rho }_c)\right],\hfill \end{array}$$

(67)

$$\begin{array}{cc}\hfill d_1& =4T^2\left[4{\rho }_b{\rho }_c-6{\rho }_a{\rho }_b-6{\rho }_a{\rho }_c+2{\rho }_a^2\right],\hfill \end{array}$$

(68)

$$\begin{array}{cc}\hfill d_2& =4T^3\left[20{\rho }_a-16{\rho }_b-16{\rho }_c\right],\hfill \end{array}$$

(69)

$$\begin{array}{cc}\hfill d_3& =192T^4.\hfill \end{array}$$

(70)

Let ${\lambda }_1<{\lambda }_2<{\lambda }_3$ be the roots of $P_3$. Notice that $z\left(t\right)={\lambda }_i$, for $i=1,2,3$, satisfies Equation (65). Moreover if ${\lambda }_2<0<{\lambda }_3$, then for all time t, ${\lambda }_2<z\left(t\right)<{\lambda }_3$. The subsequent analysis will be restricted to those cases encountered for physically relevant initial conditions—namely where $P_3$ has two negative roots and one positive root.

We integrate Equation (65) to obtain

$${\left(\frac{z-{\lambda }_1}{{\lambda }_1}\right)}^{{\gamma }_1}{\left(\frac{z-{\lambda }_2}{{\lambda }_2}\right)}^{{\gamma }_2}{\left(\frac{{\lambda }_3-z}{{\lambda }_3}\right)}^{{\gamma }_3}=exp\left[\frac{d_3}{{\mathsf{\Delta }}^2}\left(1-cos\left(\mathsf{\Delta }t\right)\right)\right],$$

(71)

with exponents

$$\begin{array}{cc}\hfill {\gamma }_1& =\frac{1}{({\lambda }_1-{\lambda }_2)({\lambda }_1-{\lambda }_3)}>0,\hfill \end{array}$$

(72)

$$\begin{array}{cc}\hfill {\gamma }_2& =\frac{1}{({\lambda }_2-{\lambda }_1)({\lambda }_2-{\lambda }_3)}<0,\hfill \end{array}$$

(73)

$$\begin{array}{cc}\hfill {\gamma }_3& =\frac{1}{({\lambda }_3-{\lambda }_1)({\lambda }_3-{\lambda }_2)}>0.\hfill \end{array}$$

(74)

Equation (71) defines $z\left(t\right)$ implicitly. Nevertheless it shows that $z\left(t\right)$ has period $T=2\pi /\mathsf{\Delta }$. In case of exact resonance we take the limit as $\mathsf{\Delta }\to 0,$ obtaining

$${\left(\frac{z-{\lambda }_1}{{\lambda }_1}\right)}^{{\gamma }_1}{\left(\frac{z-{\lambda }_2}{{\lambda }_2}\right)}^{{\gamma }_2}{\left(\frac{{\lambda }_3-z}{{\lambda }_3}\right)}^{{\gamma }_3}=exp\left[\frac{d_3}{2}{t}^2\right].$$

(75)

This shows that in exact resonance conditions the solution is no longer periodic, but instead converges to the root ${\lambda }_2$.

In the examples presented below, rather than solving Equation (71) for $z\left(t\right)$ we integrate the system of Equations (22)–(24) directly using MATLAB’s ode15s solver with absolute and relative tolerances set to ${10}^{-14}$.

6. List of Examples

In the following examples we consider three waves of the form ${\mathbf{k}}_a=k_0(1,0)$, ${\mathbf{k}}_b=k_0(1+p,q)$ and ${\mathbf{k}}_c=k_0(1-p,-q)$ and, following Stuhlmeier and Stiassnie [10], we assume that the initial complex amplitudes are independent, Gaussian random variables, written as

$$B_j\left(0\right)={\beta }_j{e}^{i{\varphi }_j},$$

(76)

where the real amplitudes ${\beta }_j$ are Rayleigh distributed and the phases ${\varphi }_j$ are uniformly distributed over the interval $[0,2\pi )$.

Under these assumptions we have

$$C_j\left(0\right)=\langle |B_j\left(0\right){|}^2\rangle =\langle {\beta }_j^2\rangle =\frac{4}{\pi }{\langle {\beta }_j\rangle }^2,$$

(77)

and we relate the average value of ${\beta }_j$ to the wave amplitude by

$$\langle {\beta }_j\rangle =\frac{\pi {\epsilon }_j}{|{\mathbf{k}}_j|}\sqrt{\frac{\pi g}{2{\omega }_j}},\mathrm{so}\mathrm{that}{C}_j=2g{\pi }^2\frac{{\epsilon }_j^2}{|{\mathbf{k}}_j{|}^2{\omega }_j},$$

(78)

where ${\epsilon }_j$ are the wave slopes. Finally we set ${\kappa }_{a,a,b,c}\left(0\right)=0,$ in order to have consistent initial conditions with which to compare GKE and JKE. In all calculations we take $k_0=1$ m${}^{-1}$, $g=9.81$ m s${}^{-2}$ and the period of the wave ${\mathbf{k}}_a$ is $T_a=2\pi /\sqrt{g{k}_0}\approx 2.01$ s.

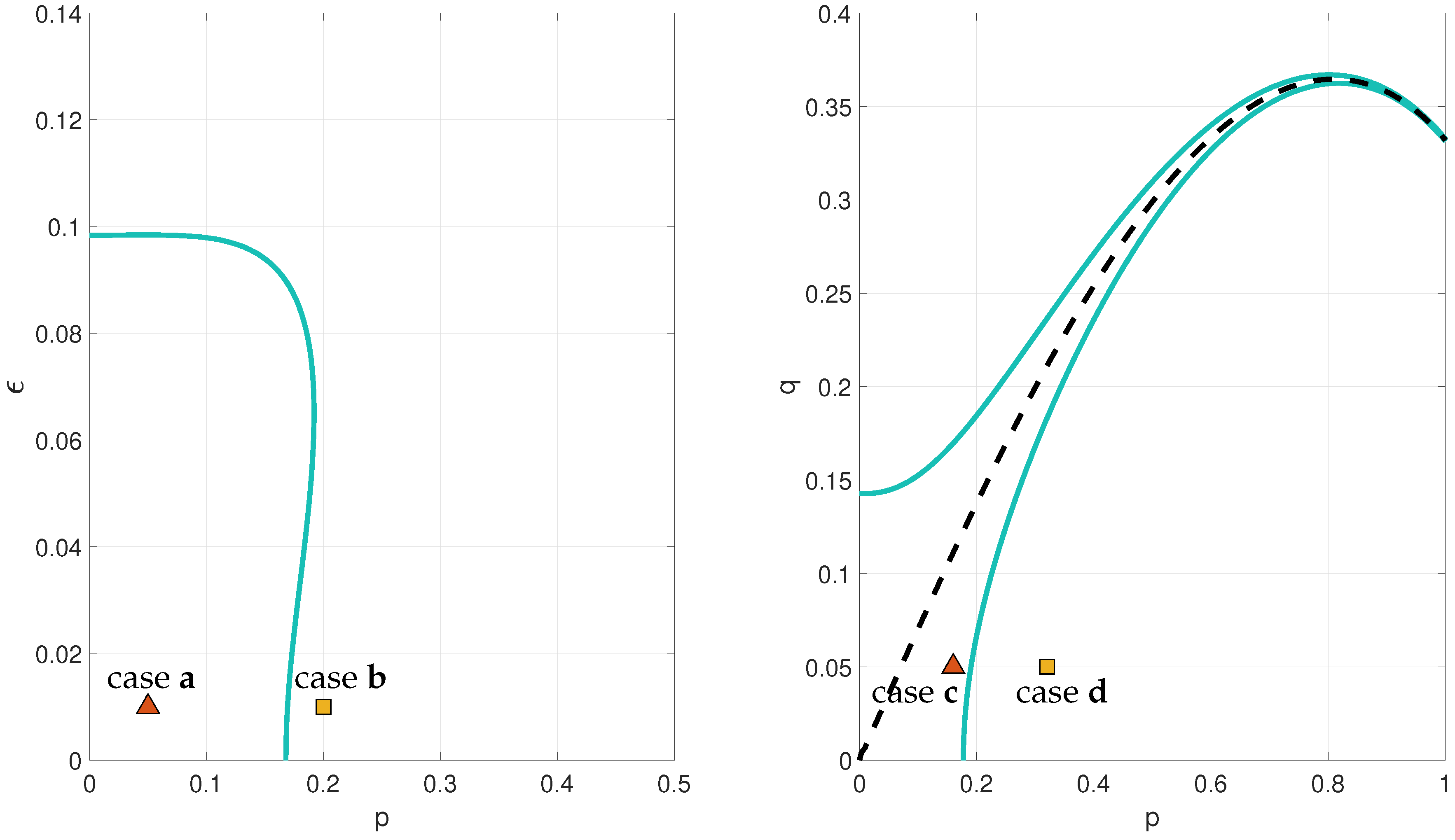
We shall consider four particular initial conditions below. In cases a and b we initially assume that the waves are collinear ($q=0$) and fix the steepness ${\epsilon }_a=0.15$ of the wave ${\mathbf{k}}_a$. Then we take $ϵ={\epsilon }_b={\epsilon }_c$ to be the steepness of the waves ${\mathbf{k}}_b$ and ${\mathbf{k}}_c$. In cases c and d the wave slopes are fixed ${\epsilon }_a=0.15$, ${\epsilon }_b=0.05$ and ${\epsilon }_c=0$ and we explore the nature of the solutions in the $(p,q)$-plane. Notice that $q\ne 0$ implies a two-dimensional disturbance. We recall that while solutions to the JKE are periodic, the behavior of the GKE is determined by the initial conditions via the discriminant D given in (48). To visualize how wave slope and wavenumber impact the solutions of the GKE, the sign of D is plotted in Figure 2. It establishes when the solutions remain bounded $(D>0)$ or tend to infinity in finite time $(D\le 0)$.

The four cases a–d marked in Figure 2 are studied numerically, and the relevant parameters for these cases are summarized in

Table 1. Degenerate quartets studied numerically for time-evolution.

	Wave Numbers		Steepness			Nature of Solution		Detuning
case	$\mathit{p}$	$\mathit{q}$	${\mathit{\epsilon }}_{\mathit{a}}$	${\mathit{\epsilon }}_{\mathit{b}}$	${\mathit{\epsilon }}_{\mathit{c}}$	GKE	JKE	${\mathsf{\Delta }}_{\mathit{a},\mathit{a}}^{\mathit{b},\mathit{c}}$ (s${}^{-\mathbf{1}}$)
a	$0.05$	0	$0.15$	$0.01$	$0.01$	blow-up	periodic	$0.002$
b	$0.2$	0	$0.15$	$0.01$	$0.01$	periodic	periodic	$0.0317$
c	$0.16$	$0.05$	$0.15$	$0.05$	0	blow-up	periodic	$0.0161$
d	$0.32$	$0.05$	$0.15$	$0.05$	0	periodic	periodic	$0.0781$

.

7. Comparison between GKE and JKE—Blow Up

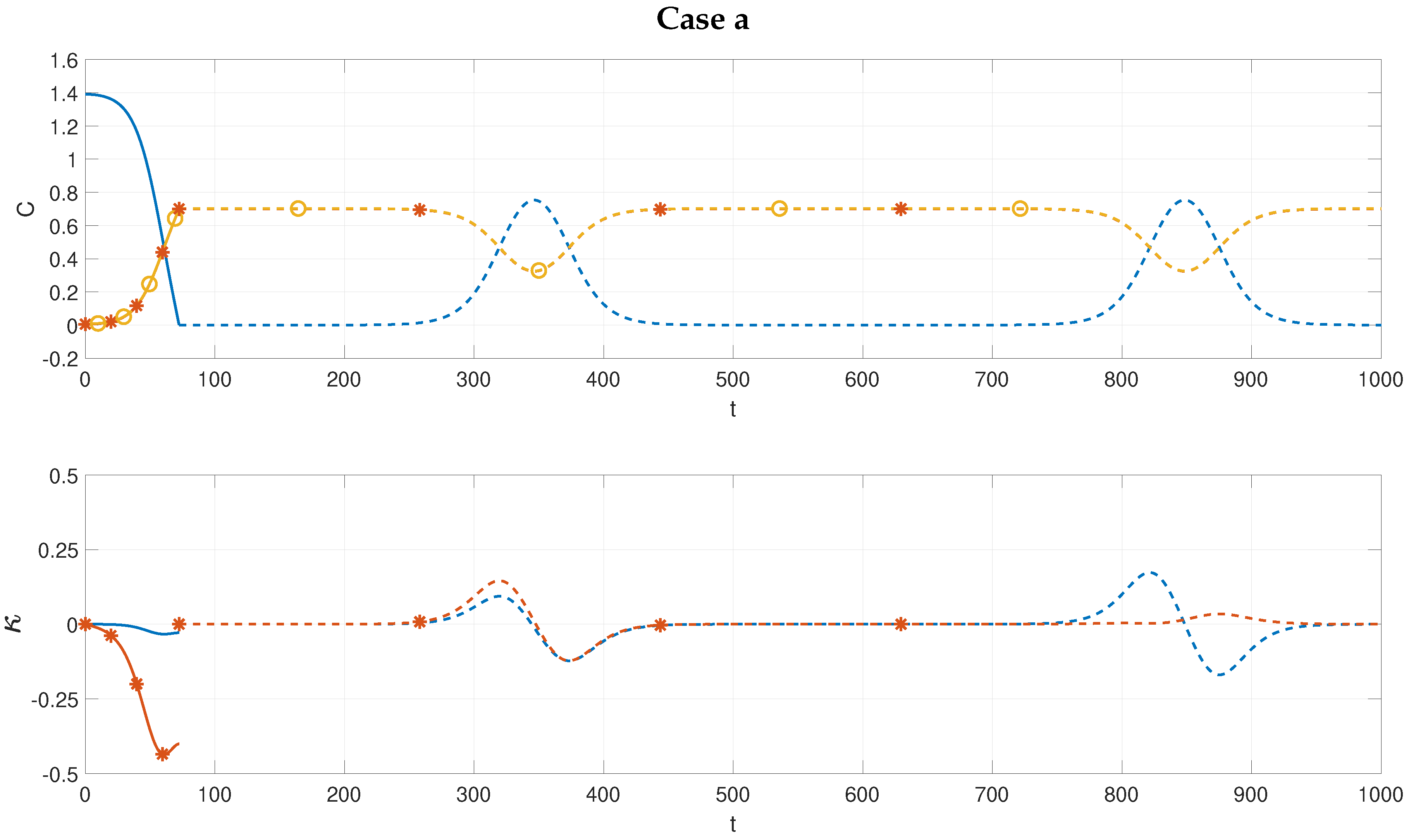
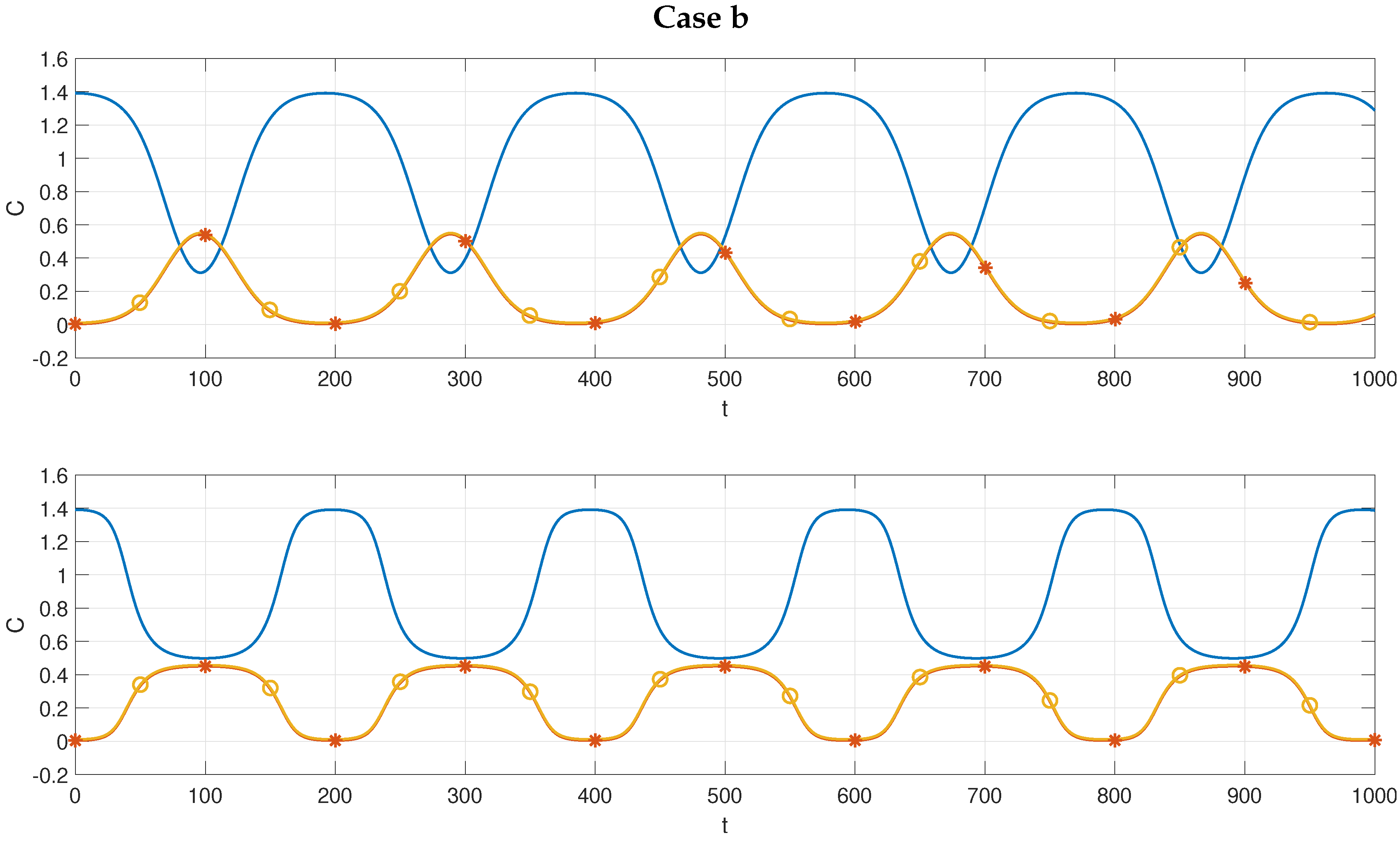
We compare the solutions of the GKE with those of the JKE for cases a and c (see Table 1), shown as triangles in Figure 2. Figure 3 shows the time evolution of case a. In the upper panel we see the solution of the GKE that tends to infinity as time approaches $T_{\infty }=100.02$ s, see (63). The solution becomes physically meaningless as $C_b$ and $C_c$ become negative, prior to the critical time. On the other hand the solution of the JKE, on the lower panel of Figure 3 remains bounded, and is periodic with period $T=3207.19$ s. Adopting the notation $t_n=(2\pi /{\omega }_a)/{\epsilon }_a^n$ for the time scales we see that the blow-up occurs at $\mathcal{O}\left(t_2\right)$. The corresponding evolution for the JKE looks initially similar, but settles down to near equipartition of wave action, before eventually exhibiting periodicity on a time scale $\mathcal{O}\left(t_4\right).$

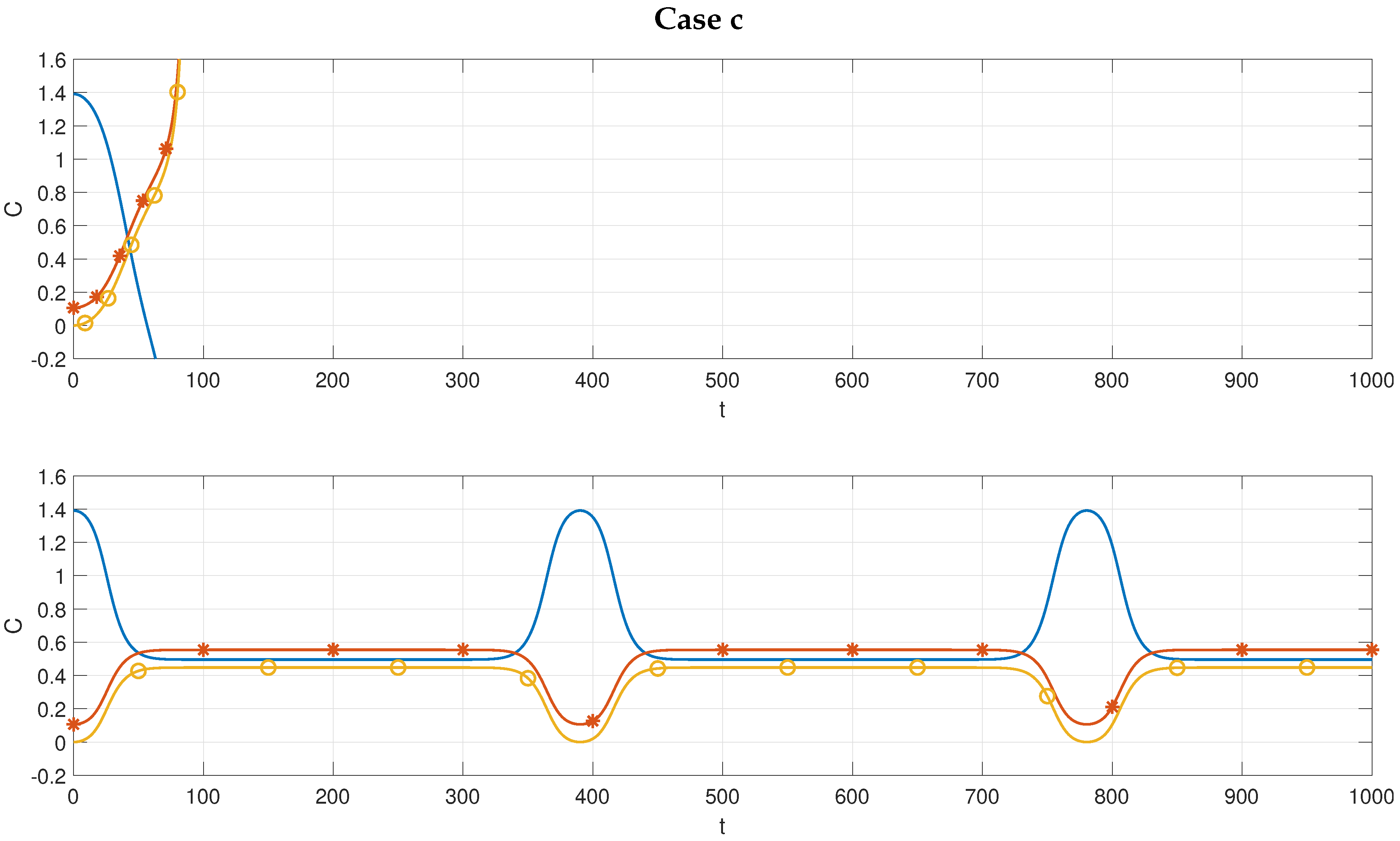
The time evolution for case c is shown in Figure 4. The upper panel shows blow-up for the solution of the GKE. The critical time is $T_{\infty }=89.80$ s. This non-physical behavior is similar to case a (above) and occurs on the same time scale $\mathcal{O}\left(t_2\right)$.

On the lower panel we have the solution of the JKE. The system also reaches a state where the energy among the three wavenumbers is similar, at a time of $\mathcal{O}\left(t_2\right)$, and is periodic and bounded with a period $T=390.19$ s which is of order $\mathcal{O}\left(t_3\right)$.

According to Hasselmann’s kinetic equation the time evolution of the wave action depends only on exact resonant waves. With the settings of cases c and d it is possible to have triads that are in exact resonance. The dashed line on the right panel of Figure 2 shows such triads. According to the GKE the time evolution of exact resonant triads blows up.

8. Comparison between GKE and JKE—Bounded Solutions

Having studied cases with $D<0,$ it is instructive to compare the solutions of the GKE with those of the JKE for the cases b and d where both are periodic and bounded. These cases are depicted by squares $(p=0.2,ϵ=0.01)$ and $(p=0.32,q=0.05)$ in Figure 2.

Figure 5 shows the time evolution for case b. The upper panel shows the solution of the GKE with period $T=192.51$ s, see (56), while the lower panel shows the solution of the JKE with a period of $T=198.07$ s. In both cases the period of the solution is $\mathcal{O}\left(t_2\right)$ and the qualitative behavior of both solutions is rather similar.

In Figure 5 one can see behavior similar to the stochastic Fermi-Pasta-Ulam recurrence (FPU recurrence), which is usually related to the long time behavior of the deterministic Benjamin-Feir instability. Details of the FPU recurrence can be found in Stiassnie and Kroszynski [15]. For stochastic recurrence derived from the Alber equation see Stiassnie, Regev and Agnon [16].

Case d, depicted in Figure 6, shows considerable similarity between the solutions of the GKE and solutions of the JKE. The periods are $T=94.22$ s for the GKE and $T=80.42$ s for JKE, both of $\mathcal{O}\left(t_2\right)$.

9. Discussion and Concluding Remarks

9.1. Time Scales

In Hasselmann’s kinetic equation it is assumed that the wave field evolves on the slow time scale $\mathcal{O}\left(t_4\right),$ and only exactly resonant quartets are taken into account. In all of the examples presented here the degenerated quartet of waves is in near resonance, as indicated by the value of the detuning ${\mathsf{\Delta }}_{a,a}^{b,c}$ in Table 1. According to the exactly resonant kinetic theory there should be no temporal evolution for such initial data, which in turn is consistent with the assumption that the wave field can be described entirely on the slow $t_4$ scale.

As pointed out by Janssen, the solutions of the JKE (15) capture changes that happen on the much faster time-scale $t_2$. Indeed, all of our simulations show an initial monotonic evolution on this $t_2$ scale. Nevertheless the period $T=2\pi /\mathsf{\Delta }$, with $\mathsf{\Delta }$ given in Table 1, is of $\mathcal{O}\left(t_4\right)$ for case a, $\mathcal{O}\left(t_3\right)$ for case c and $\mathcal{O}\left(t_2\right)$ for cases b and d.

9.2. Assumption (i), Weak Non-Gaussianity

Both the GKE and the JKE are derived using the ordering assumption, in Equation (7), that $C_j=\mathcal{O}\left({\epsilon }^2\right)$ and $\kappa =o\left({\epsilon }^4\right)$, where $\epsilon$ is a typical wave steepness. In order to verify this assumption we compute the following ratio

$$\delta =\frac{\underset{0\le t\le 1000}{max}\left|{\kappa }_{a,a,b,c}\right|}{C_a^2\left(0\right)},$$

(79)

in all the examples presented here.

The results are summarized in

Table 2. Values of $\delta =\underset{0\le t\le 1000}{max}\left|{\kappa }_{a,a,b,c}\left(t\right)\right|/C_a^2\left(0\right)$ for computed solutions to the GKE and JKE in the four cases a–d.

	GKE	JKE
case a	blow up	$0.2516$
case b	$0.2268$	$0.2478$
case c	blow up	$0.2097$
case d	$0.1142$	$0.1460$

. Note that ${\epsilon }_a=0.15$ and that for all cases $\delta =\mathcal{O}\left({\epsilon }_a\right)$. Hence the computed results support the ordering of the cumulants in terms of $\epsilon .$

9.3. Assumption (ii), Slow Temporal Evolution

The key difference between the JKE and GKE is assumption (ii), that $C_a{C}_b{C}_c-C_a^2(C_b+C_c)/2$ varies slowly compared with $exp\left(i{\mathsf{\Delta }}_{a,a}^{b,c}t\right)$. In fact, our numerical simulations with the JKE show that in cases a and c the period of $C_a{C}_b{C}_c-C_a^2(C_b+C_c)/2$ is of the order of $\mathcal{O}\left(t_4\right)$ and $\mathcal{O}\left(t_3\right)$ respectively, although there are some rapid changes on the scale $\mathcal{O}\left(t_2\right)$.

In cases b and d $C_a{C}_b{C}_c-C_a^2(C_b+C_c)/2$ has variations on the $\mathcal{O}\left(t_2\right)$ scale, which is also the time scale of their period. Note that the solution of the JKE has the same period as the oscillatory term $e^{i{\mathsf{\Delta }}_{a,a}^{b,c}t}$, see formula (71). This contradicts assumption (ii) for the JKE. Nevertheless, the surprising agreement between the GKE and the JKE in cases b and d suggests that the approximation may be valid, but it is not clear at this stage how it could be justified.

9.4. Numerical Computations

As mentioned in Section 5, Equation (71) was not used to compute solutions of the JKE because it is practically simpler to integrate the JKE numerically. However, for case c, (71) was used as a consistency check.

We used the numerical solution for $C_a$, $C_b$ and $C_c$ and through relations (27)–(29) obtained $z\left(t\right)$ as

$$z\left(t\right)=\frac{C_a-2C_b-2C_c}{12T_{a,a,b,c}\left({\rho }_a-2{\rho }_b-2{\rho }_c\right)},$$

(80)

which was used to compute both sides of (71). We subsequently measured the relative error at each time step, and found it always to be below $2.91\times {10}^{-6}$.

For the GKE the exact formulas (54) and (62) can be used directly to obtain the time evolution of the system. However, for completeness’ sake, we also considered a direct numerical integration of (18)–(21) using MATLAB ode15s as before. For the bounded cases b and d we obtained a relative error between the exact and the numerical solutions below $2.8\times {10}^{-8}$ and $6.1\times {10}^{-10}$ at each time step from 0 to 1000 s, respectively. For the unbounded cases a and c we measured the difference at each time step between 0 and $t_f=T_{\infty }-1$ s, which exhibited a relative error of $1.9\times {10}^{-8}$ for case a and $2.8\times {10}^{-9}$ for case c.

We also ran numerical simulations with the GKE including the Stokes correction term of Gramstad and Stiassnie, see [8]. Note that the GKE with the Stokes corrections still blows-up, with a blow-up time similar to that shown in Figure 3 and Figure 4.

9.5. Conclusions

At this stage blow-up in the GKE seems unavoidable, although it has hitherto not been reported in studies applying the GKE to wave-fields with many modes. For degenerate quartets and given initial data, the roots of the polynomial $P_4$ determine the nature of the solutions of the GKE, but there is no clear physical distinction, in terms of wave slope or wave number, that would enable one to distinguish bounded cases from those which blow-up. It seems further study is warranted before a clear successor to Hasselmann’s kinetic equation, which incorporates near-resonant interactions, can be identified.