Improving Accuracy in α-Models of Turbulence through Approximate Deconvolution
Abstract
:1. Introduction
2. Mathematical Context
3. Numerical Scheme for the -Models of Turbulence
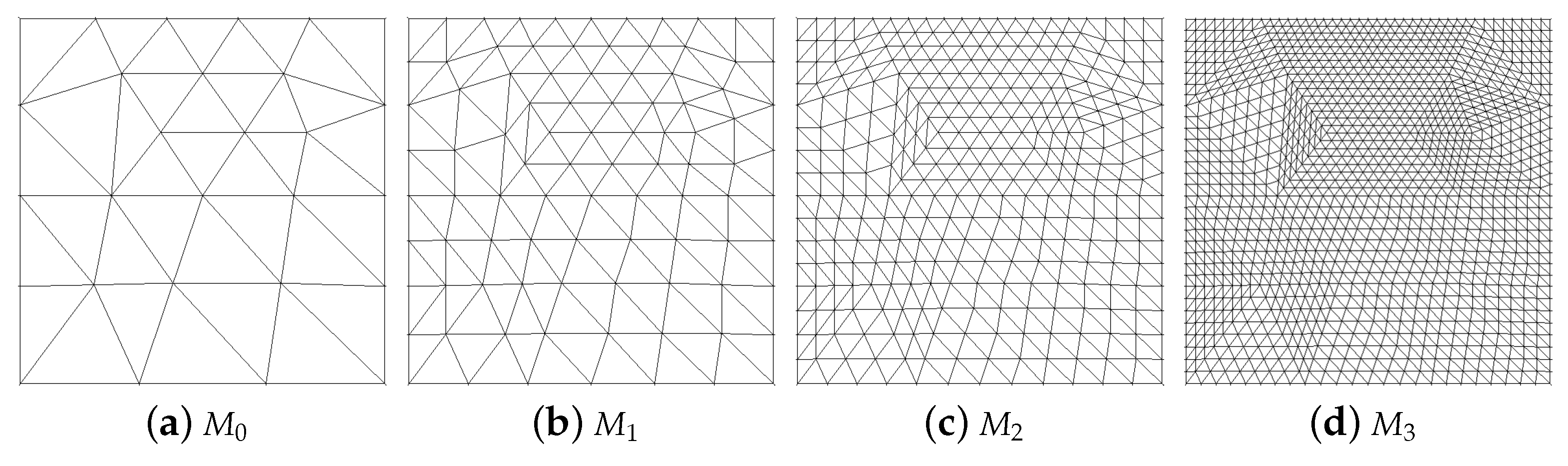
4. A Numerical Experiment
5. Conclusions
Acknowledgments
Conflicts of Interest
References
- Kolmogorov, A.V. The Local Structure of Turbulence in Incompressible Viscous Fluids for Very Large Reynolds Number. Dokl. Akad. Nauk SSSR 1941, 30, 209–303. [Google Scholar] [CrossRef]
- Cheskidov, A.; Holm, D.; Olson, E.; Titi, E. On a Leray-alpha model of turbulence. Proc. Ser. A Math. Phys. Eng. Sci. 2005, 461, 629–649. [Google Scholar] [CrossRef]
- Ilyin, A.; Lunasin, E.; Titi, E. A modified-Leray-α subgrid scale model of turbulence. Nonlinearity 2006, 19, 879–897. [Google Scholar] [CrossRef]
- Layton, W.; Lewandowski, R. A simple and stable scale similarity model for large scale eddy simulation: Energy balance and existence of weak solutions. Appl. Math. Lett. 2003, 16, 1205–1209. [Google Scholar] [CrossRef]
- Dunca, A. On an energy inequality for the approximate deconvolution models. Nonlinear Anal. Real World Appl. 2016, 32, 294–300. [Google Scholar] [CrossRef]
- Germano, M. Differential filters for the large eddy numerical simulation of turbulent flows. Phys. Fluids 1986, 29, 1755–1757. [Google Scholar] [CrossRef]
- Leray, J. Essay sur les mouvements plans d’une liquide visqueux que limitent des parois. J. Math. Pures Appl. Paris Ser. IX 1934, 13, 331–418. [Google Scholar]
- Leray, J. Sur les mouvements d’une liquide visqueux emplissant l’espace. ACTA Math. 1934, 63, 193–248. [Google Scholar] [CrossRef]
- Chepyzhov, V.V.; Titi, E.S.; Vishik, M.I. On the convergence of solutions of the Leray-α model to the trajectory attractor of the 3D Navier-Stokes system. J. Discrete Contin. Dyn. Syst.-Ser. A 2007, 17, 33–52. [Google Scholar]
- Vishik, M.I.; Titi, E.S.; Chepyzhov, V.V. Trajectory attractor approximations of the 3D Navier-Stokes system by the Leray-α model. Russ. Math. Dokl. 2005, 71, 91–95. [Google Scholar]
- Layton, W.; Lewandowski, R. A high accuracy Leray-deconvolution model of turbulence and its limiting behavior. Anal. Appl. (Singap.) 2008, 6, 23–49. [Google Scholar] [CrossRef]
- Foias, C.; Holm, D.; Titi, E. The three dimensional viscous Camassa-Holm equations, and their relation to the Navier-Stokes equations and turbulence theory. J. Dyn. Differ. Equ. 2002, 14, 1–35. [Google Scholar] [CrossRef]
- Stolz, S.; Adams, N. An approximate deconvolution procedure for large eddy-simulation. Phys. Fluids 1999, 11, 1699–1701. [Google Scholar] [CrossRef]
- Adams, N.; Stolz, S. Deconvolution methods for subgrid-scale approximation in large-eddy simulation. In Modern Simulation Strategies for Turbulent Flow; Geurts, B., Ed.; R.T. Edwards: Philadelphia, PA, USA, 2001; pp. 21–41. [Google Scholar]
- Foias, C.; Holm, D.; Titi, E. The Navier-Stokes-alpha model of fluid turbulence. Phys. D 2001, 152–153, 505–519. [Google Scholar] [CrossRef]
- Layton, W.; Lewandowski, R. On a well-posed turbulence model. Discrete Contin. Dyn. Syst. Ser. B 2006, 6, 111–128. [Google Scholar]
- Cao, Y.; Lunasin, E.M.; Titi, E.S. Global well-posedness of the three-dimensional viscous and inviscid simplified Bardina turbulence models. Commun. Math. Sci. 2006, 4, 823–848. [Google Scholar] [CrossRef]
- Hernandez, M.M.; Rebholz, L.; Tone, C.; Tone, F. On the Stability of the Crank-Nicolson-Adams-Bashforth Scheme for the 2D Leray-alpha model. Numer. Methods Partial Differ. Equ. 2016, 32, 1155–1183. [Google Scholar] [CrossRef]
- Miles, W.; Rebholz, L. An enhanced physics based scheme for the NS-alpha turbulence model. Numer. Methods Partial Differ. Equ. 2010, 26, 1530–1555. [Google Scholar]
- Kaya, S.; Manica, C.C. Convergence Analysis of the Finite Element Method for a Fundamental Model in Turbulence. Math. Models Methods Appl. Sci. 2012, 22. [Google Scholar] [CrossRef]
- Dunca, A. Estimates of the discrete van Cittert deconvolution error in approximate deconvolution models of turbulence. 2017; submitted. [Google Scholar]
- Layton, W.; Manica, C.; Neda, M.; Rebholz, L. Numerical Analysis and Computational Testing of a high-order Leray-deconvolution turbulence model. Numer. Methods Partial Differ. Equ. 2008, 24, 555–582. [Google Scholar] [CrossRef]
- Stolz, S.; Adams, N.A.; Kleiser, L. An approximate deconvolution model for large-eddy simulation with application to incompressible wall-bounded flows. Phys. Fluids 2001, 13, 997–1015. [Google Scholar] [CrossRef]
- Dunca, A.; Epshteyn, Y. On the Stolz-Adams deconvolution model for the large-eddy simulation of turbulent flows. SIAM J. Math. Anal. 2006, 37, 1890–1902. [Google Scholar] [CrossRef]
- Stanculescu, I. Existence theory of abstract approximate deconvolution models of turbulence. Ann. Dell’Univ. Ferrara Sez. VII Sci. Mat. 2008, 54, 145–168. [Google Scholar] [CrossRef]
- Kaya, S.; Manica, C.; Rebholz, L. On Crank-Nicolson Adams-Bashforth timestepping for approximate deconvolution models in two dimensions. Appl. Math. Comput. 2014, 246, 23–38. [Google Scholar] [CrossRef]
- Galvin, K.; Rebholz, L.; Trenchea, C. Efficient, unconditionally stable, and optimally accurate FE algorithms for approximate deconvolution models. SIAM J. Numer. Anal. 2014, 52, 678–707. [Google Scholar] [CrossRef]
- Rebholz, L. Well-posedness of a reduced order approximate deconvolution turbulence model. J. Math. Anal. Appl. 2013, 405, 738–741. [Google Scholar] [CrossRef]
- Dunca, A. Numerical analysis and testing of a stable and convergent finite element scheme for approximate deconvolution turbulence models. Comput. Math. Appl. 2017. [Google Scholar] [CrossRef]
- Layton, W.; Rebholz, L. Approximate Deconvolution Models of Turbulence: Analysis, Phenomenology and Numerical Analysis; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Cuff, V.; Dunca, A.; Manica, C.; Rebholz, L. The reduced order NS-α model for incompressible flow: Theory, numerical analysis and benchmark testing. ESAIM: Math. Model. Numer. Anal. (M2AN) 2015, 49, 641–662. [Google Scholar] [CrossRef]
- Rebholz, L.; Kim, T.Y.; Byon, Y. On an accurate α model for coarse mesh turbulent channel flow simulation. Appl. Math. Model. 2017, 43, 139–154. [Google Scholar] [CrossRef]
- Rebholz, L.; Zerfas, C.; Zhao, K. Global in time analysis and sensitivity analysis for the reduced NS-α model of incompressible flow. J. Math. Fluid Mech. 2017, 19, 445–467. [Google Scholar] [CrossRef]
- Dunca, A. Estimates of the modeling error of the α- models of turbulence in two and three space dimensions. 2017; submitted. [Google Scholar]
- Layton, W. The interior error of van Cittert deconvolution of differential filters is optimal. Appl. Math. E-Notes 2012, 12, 88–93. [Google Scholar]
- Layton, W. Introduction to the Numerical Analysis of Incompressible Viscous Flows, Viscous Flows; SIAM Publications: Philadelphia, PA, USA, 2008; 213p, ISBN 978-0-898716-57-3. [Google Scholar]
- Brenner, S.; Scott, L. The Mathematical Theory of Finite Element Methods, 3rd ed.; Springer: New York, NY, USA, 2008. [Google Scholar]
- Heywood, J.; Rannacher, R. Finite element approximation of the nonstationary Navier-Stokes problem. Part IV. Error analysis for the second order time discretization. SIAM J. Numer. Anal. 1990, 2, 353–384. [Google Scholar] [CrossRef]
- Guermond, J.L.; Quartapelle, L. On the approximation of the unsteady Navier-Stokes equations by finite element projection methods. Numer. Math. 1998, 80, 207–238. [Google Scholar] [CrossRef]
- Hecht, F. New development in FreeFem++. J. Numer. Math. 2012, 20, 251–265. [Google Scholar] [CrossRef]
- Dunca, A.; Neda, M. Numerical Analysis of a Nonlinear Time Relaxation Model of Fluids. J. Math. Anal. Appl. 2014, 420, 1095–1115. [Google Scholar] [CrossRef]
| Level | Nr Iter | h | Error | Error | |||
|---|---|---|---|---|---|---|---|
| 8 | 1/80 | 0.198493 | 0.00349919 | rate | 0.00635556 | rate | |
| 16 | 1/160 | 0.0992465 | 0.000966758 | 1.85 | 0.0017491 | 1.86 | |
| 32 | 1/320 | 0.0496232 | 0.000267232 | 1.85 | 0.000472424 | 1.88 | |
| 64 | 1/640 | 0.0248116 | 1.97 | 0.000118784 | 1.99 | ||
| 128 | 1/1280 | 0.0124058 | 2.00 | 2.01 | |||
| Level | Nr Iter | h | Error | Error | |||
|---|---|---|---|---|---|---|---|
| 8 | 1/80 | 0.198493 | 0.00321879 | rate | 0.00599466 | rate | |
| 16 | 1/160 | 0.0992465 | 0.000735394 | 2.12 | 0.00131779 | 2.18 | |
| 32 | 1/320 | 0.0496232 | 0.000174567 | 2.07 | 0.000291998 | 2.17 | |
| 64 | 1/640 | 0.0248116 | 2.02 | 2.08 | |||
| 128 | 1/1280 | 0.0124058 | 2.00 | 2.04 | |||
| Level | Nr Iter | h | Error | Error | |||
|---|---|---|---|---|---|---|---|
| 8 | 1/80 | 0.198493 | 0.0059773 | rate | 0.00995501 | rate | |
| 16 | 1/160 | 0.0992465 | 0.00259845 | 1.20 | 0.00434837 | 1.19 | |
| 32 | 1/320 | 0.0496232 | 0.000900568 | 1.52 | 0.00149832 | 1.53 | |
| 64 | 1/640 | 0.0248116 | 0.000257406 | 1.80 | 0.000427468 | 1.80 | |
| 128 | 1/1280 | 0.0124058 | 1.92 | 0.000112259 | 1.92 | ||
| Level | Nr Iter | h | Error | Error | |||
|---|---|---|---|---|---|---|---|
| 8 | 1/80 | 0.198493 | 0.00453629 | rate | 0.00788281 | rate | |
| 16 | 1/160 | 0.0992465 | 0.00111931 | 2.01 | 0.00198618 | 1.98 | |
| 32 | 1/320 | 0.0496232 | 0.000209968 | 2.41 | 0.000373953 | 2.40 | |
| 64 | 1/640 | 0.0248116 | 2.24 | 2.28 | |||
| 128 | 1/1280 | 0.0124058 | 2.04 | 2.08 | |||
© 2017 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dunca, A.A. Improving Accuracy in α-Models of Turbulence through Approximate Deconvolution. Fluids 2017, 2, 58. https://doi.org/10.3390/fluids2040058
Dunca AA. Improving Accuracy in α-Models of Turbulence through Approximate Deconvolution. Fluids. 2017; 2(4):58. https://doi.org/10.3390/fluids2040058
Chicago/Turabian StyleDunca, Argus A. 2017. "Improving Accuracy in α-Models of Turbulence through Approximate Deconvolution" Fluids 2, no. 4: 58. https://doi.org/10.3390/fluids2040058
APA StyleDunca, A. A. (2017). Improving Accuracy in α-Models of Turbulence through Approximate Deconvolution. Fluids, 2(4), 58. https://doi.org/10.3390/fluids2040058




