Low-Frequency Acoustic Emissions During Granular Discharge in Inclined Silos
Abstract
1. Introduction
2. Experimental Setup
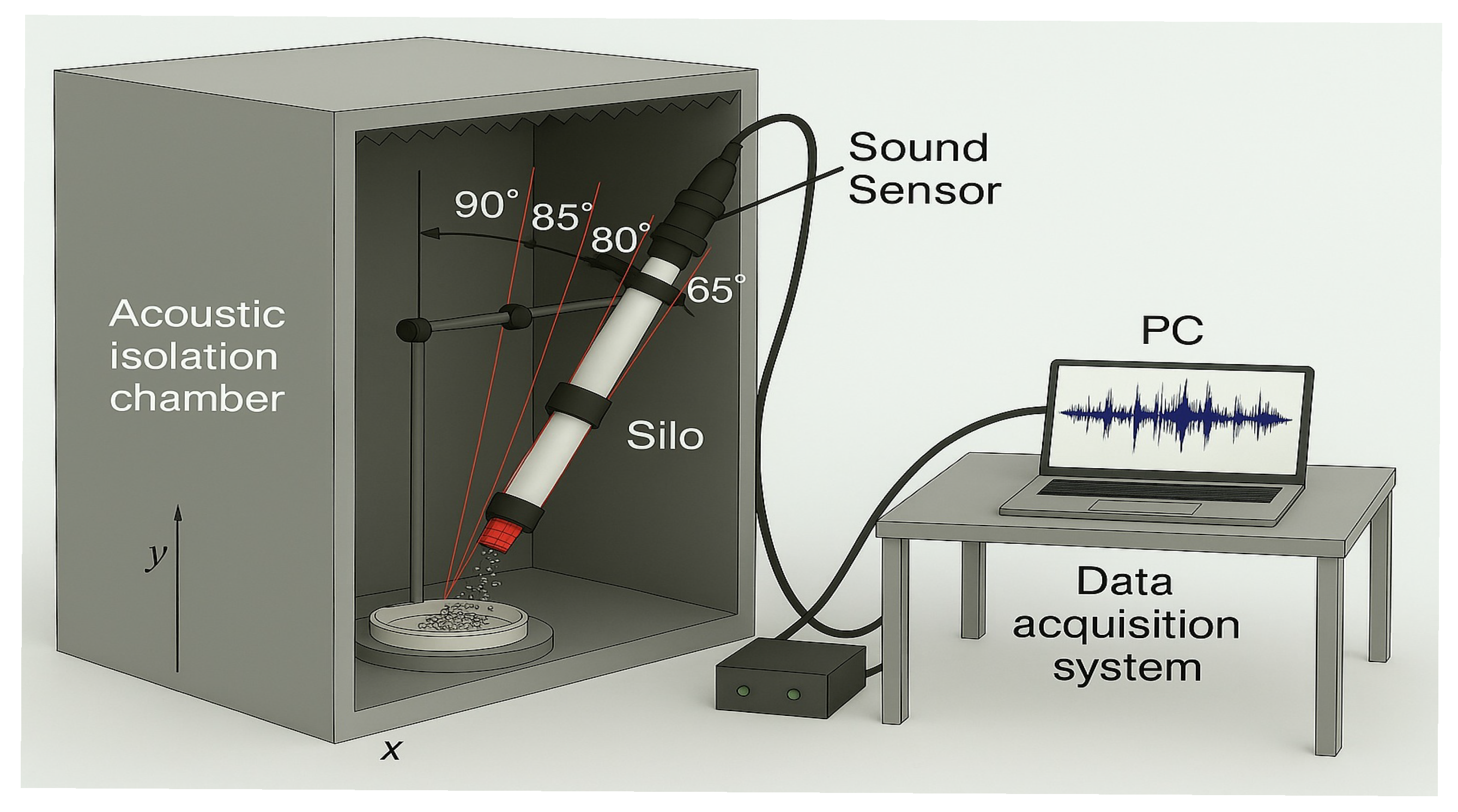
2.1. Characteristics of the Experiment
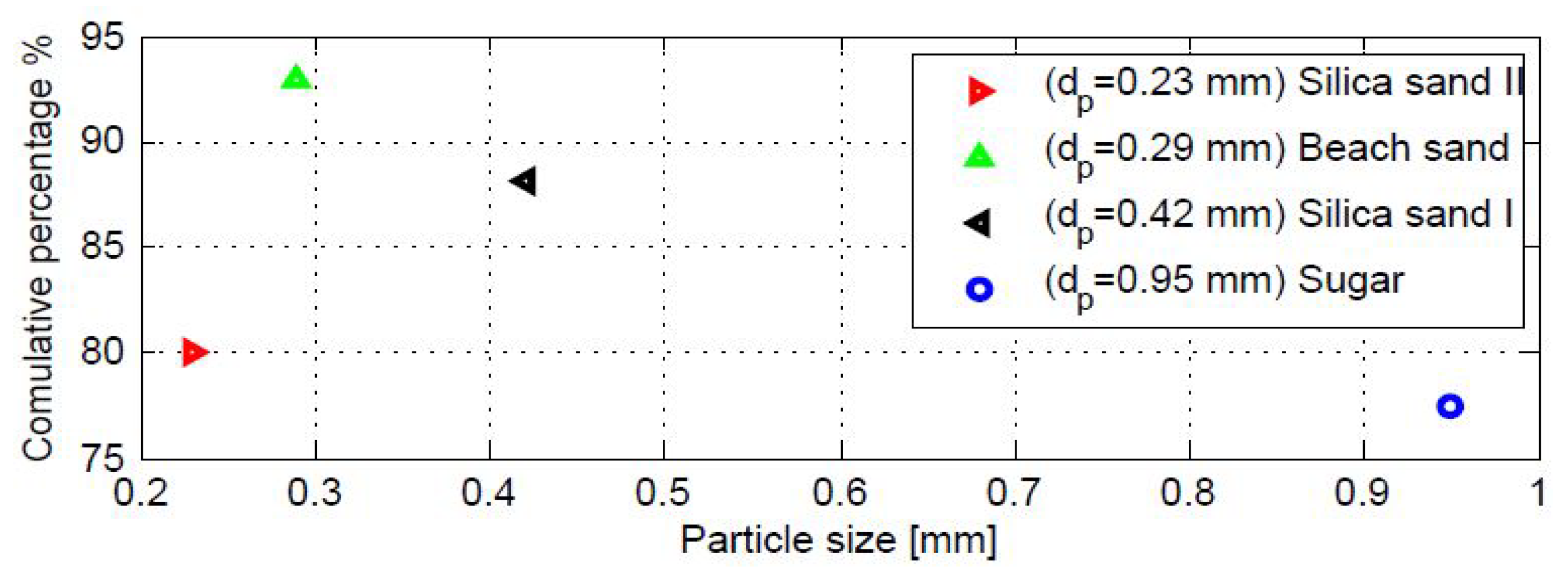
2.2. Granular Materials
3. Acoustic Contributions
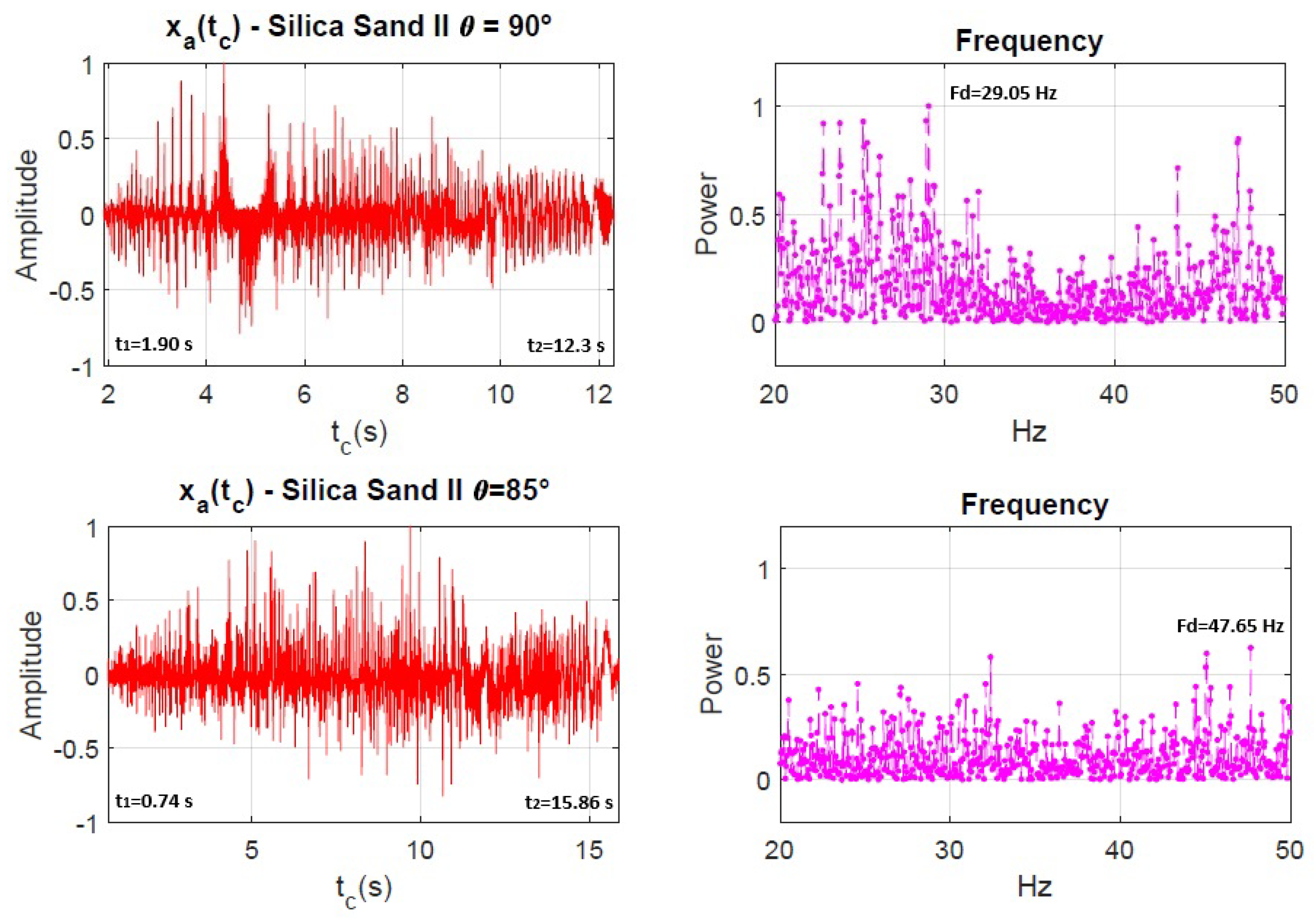
3.1. Time Analysis
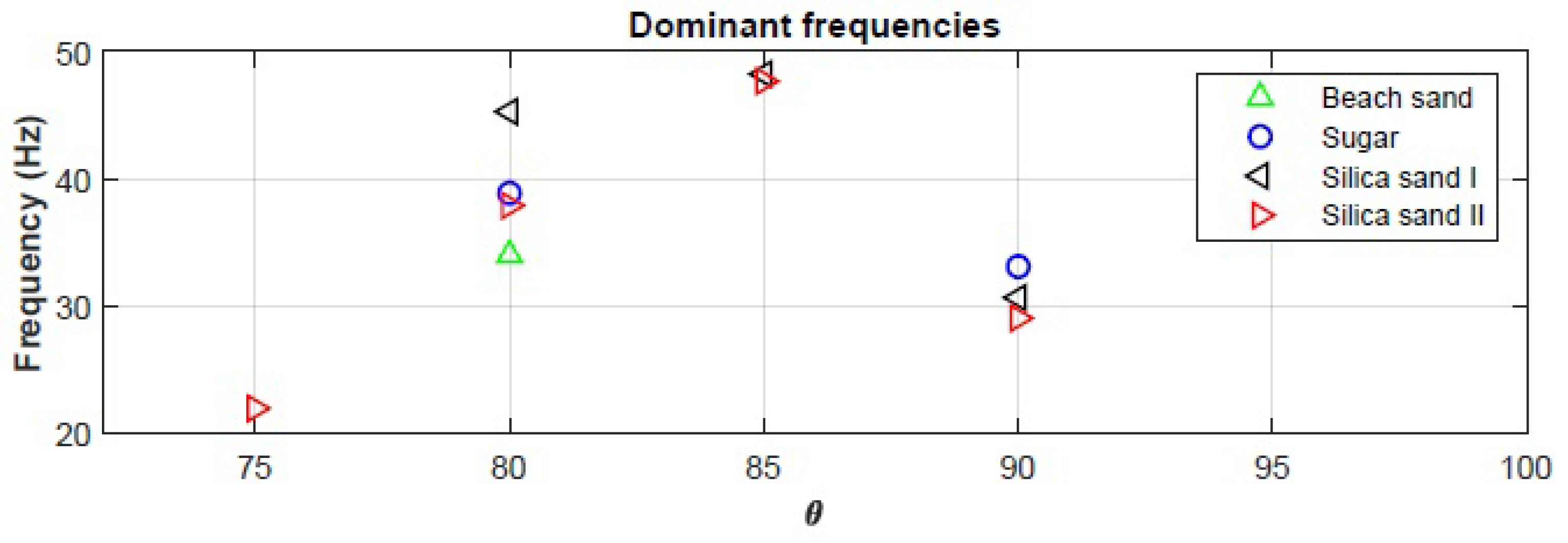
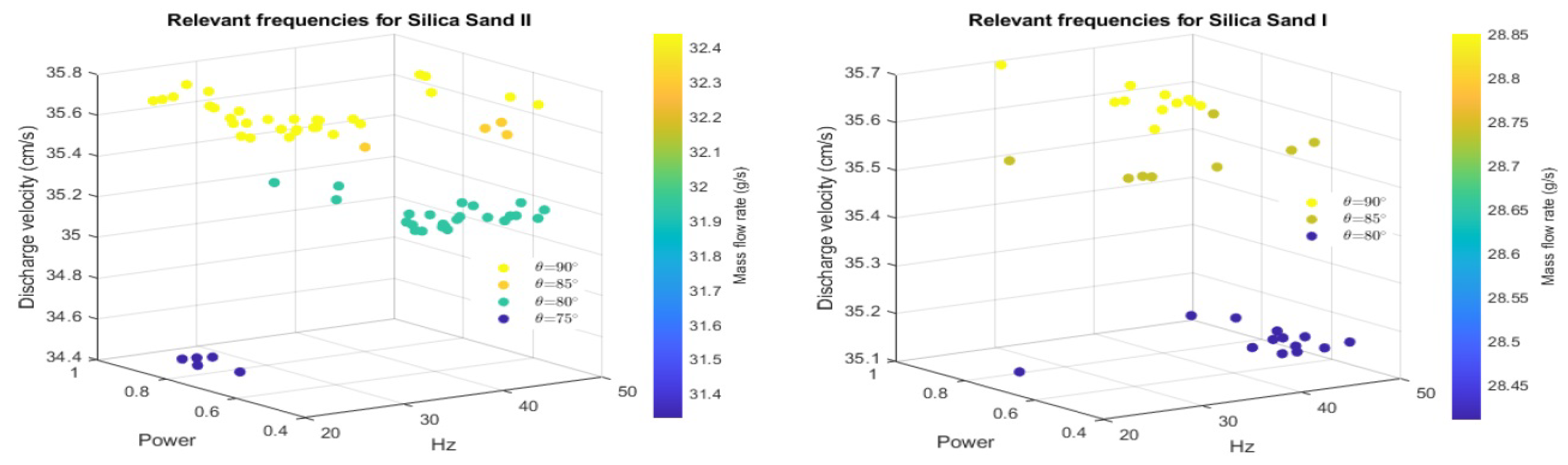
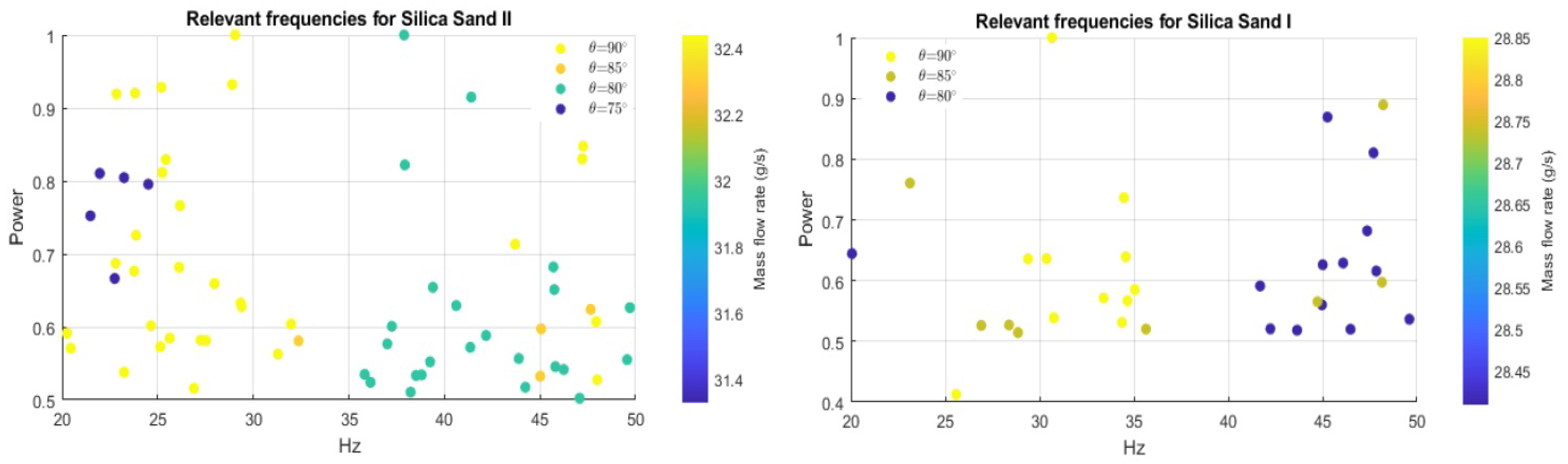
3.2. Frequency Domain
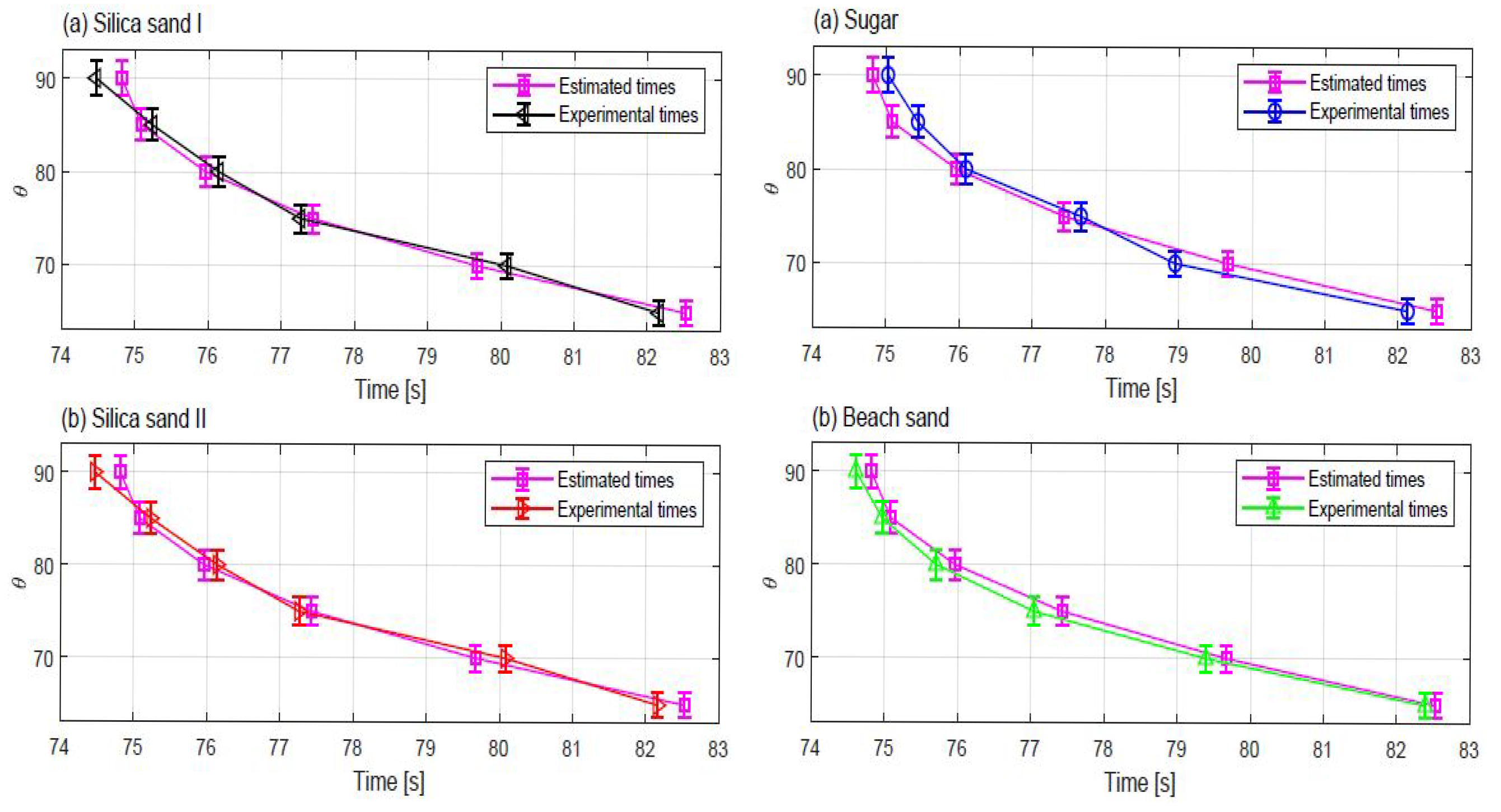
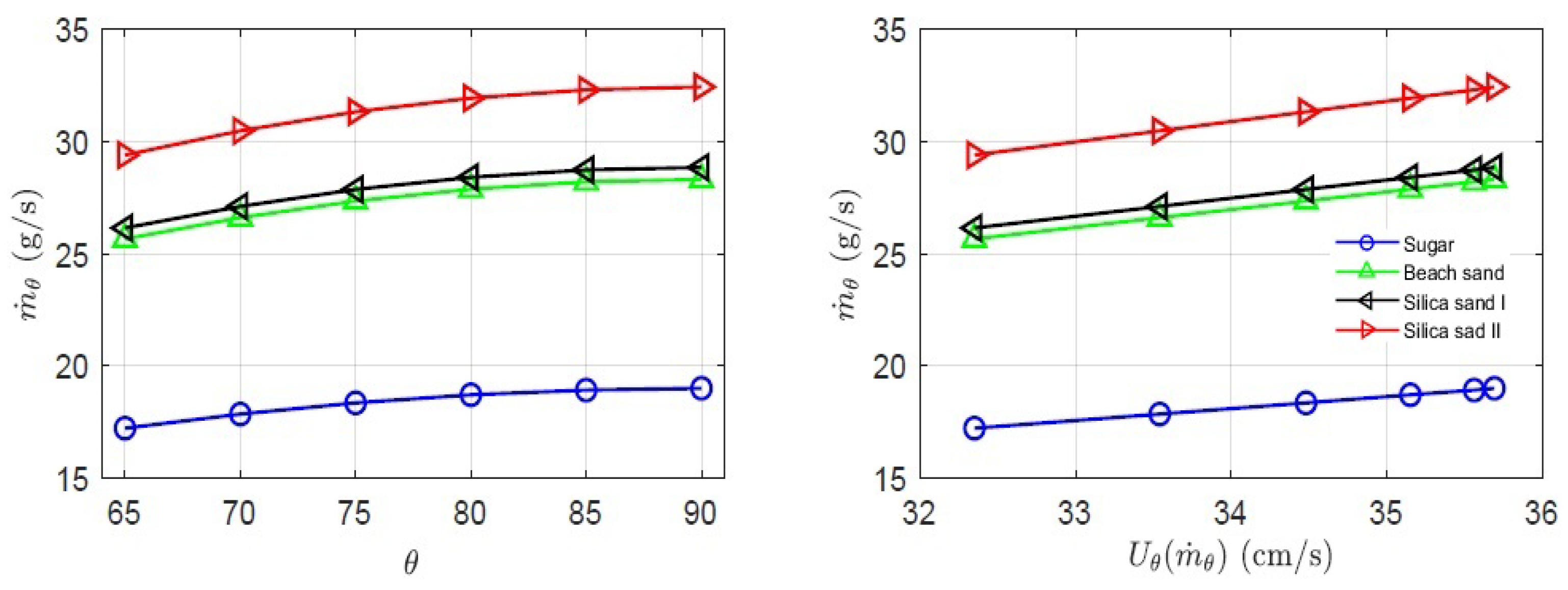
4. Critical Height
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Time Signals and Spectra

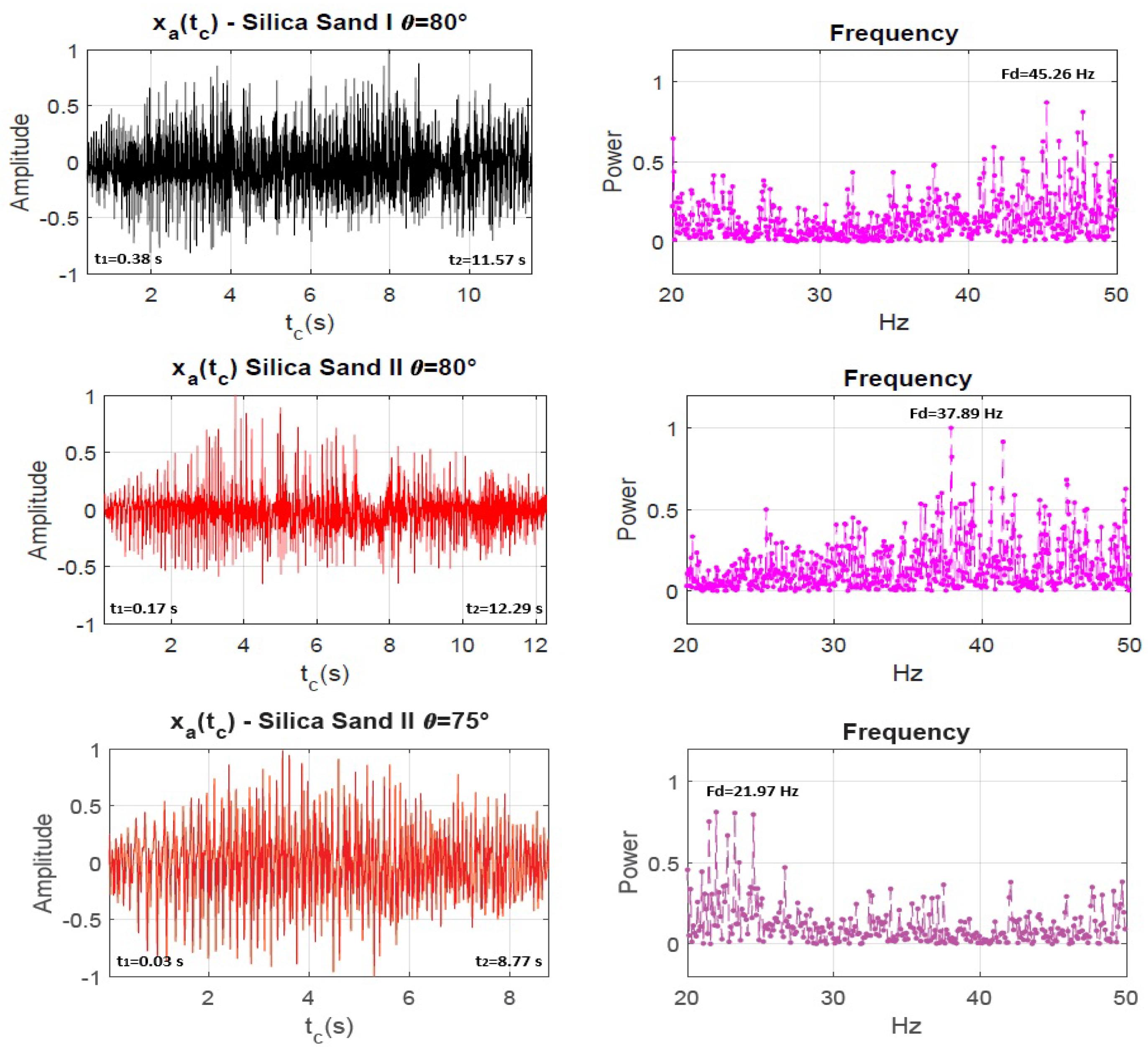
Appendix B. Some Notations
| Granular Material | (s) | (s) | (s) | (cm) | (cm) |
|---|---|---|---|---|---|
| 75.02 | 1.80 | 11.4 | 43.42 | 37.71 | |
| 74.46 | 5.60 | 11.8 | 41.16 | 37.48 | |
| 75.01 | 1.90 | 12.3 | 43.36 | 37.18 | |
| 75.23 | 0.26 | 13.58 | 44.34 | 36.45 | |
| 75.35 | 0.74 | 15.86 | 44.06 | 35.10 | |
| * | 75.70 | 49.1 | 53.8 | 15.73 | 12.98 |
| 76.09 | 1.02 | 6.02 | 43.90 | 40.97 | |
| 76.14 | 0.38 | 11.57 | 44.27 | 37.72 | |
| 76.15 | 0.17 | 12.29 | 44.40 | 37.30 | |
| 77.62 | 0.03 | 8.77 | 44.48 | 39.46 |
Appendix C. Estimated Times
References
- Schulze, D. Powders and Bulk Solids: Behavior, Characterization, Storage and Flow; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Bonneau, L.; Catelin-Jullien, T.; Andreotti, B. Friction-induced amplification of acoustic waves in a low Mach number granular flow. Phys. Rev. E 2010, 82, 011309. [Google Scholar] [CrossRef] [PubMed]
- Buick, J.M.; Chavez-Sagarnaga, J.; Zhong, Z.; Ooi, J.Y.; Pankaj; Campbell, D.M.; Greated, C.A. Investigation of silo Honking: Slip-Stick excitation and wall vibration. J. Eng. Mech. 2005, 131, 299–307. [Google Scholar] [CrossRef]
- Dhoriyani, M.L.; Jonnalagadda, K.K.; Kikatla, R.K.; Kesava Rao, K. Silo music: Sound emission during the flow of granular materials through tubes. Powder Technol. 2006, 167, 55–71. [Google Scholar] [CrossRef]
- Muite, B.K.; Quinn, S.F.; Sundaresan, S.; Kesava Rao, K. Silo music and silo quake: Granular flow-induced vibration. Powder Technol. 2004, 145, 190–202. [Google Scholar] [CrossRef]
- Niedostatkiewicz, M.; Tejchman, J. Experimental and theoretical studies of resonance effects during confined flow in silos. TASK Q. 2003, 7, 595–610. [Google Scholar]
- Warburton, K.; Porte, E.; Vriend, N. Slip-stick excitation and travelling waves excite silo honking. EPJ Web Conf. 2017, 140, 10009. [Google Scholar] [CrossRef]
- Wilde, K.; Rucka, M.; Tejchman, J. Silo music—Mechanism of dynamic flow and structure interaction. Powder Technol. 2008, 186, 113–129. [Google Scholar] [CrossRef]
- Wilde, K.; Tejchman, J.; Rucka, M.; Niedostatkiewicz, M. Experimental and theoretical investigations of silo music. Powder Technol. 2010, 198, 38–48. [Google Scholar] [CrossRef]
- Hernández-Juárez, J.R.; Serrano, D.A.; López-Villa, A.; Medina, A. Acoustic radiation patterns of the silo music phenomenon. EPJ Web Conf. 2021, 249, 03018. [Google Scholar] [CrossRef]
- Zhang, D.; Liu, L.; Yuan, J.; Zhang, D. Fluctuation of particles during funnel flow discharge from flat-bottomed silos. AIChE J. 2021, 68, e17414. [Google Scholar] [CrossRef]
- Zhang, D.; Lu, Y.; Lv, C.; Liu, L.; Yuan, J. Flow behavior of granular material during funnel and mixed flow discharges: A comparative analysis. Powder Technol. 2022, 396, 127–138. [Google Scholar] [CrossRef]
- Zhang, D.; Lu, Y.; Yuan, J. Why the presence of insert above the outlet can enhance silo discharge: A tentative answer. Powder Technol. 2023, 421, 118384. [Google Scholar] [CrossRef]
- Kamrin, K. Non-locality in Granular Flow: Phenomenology and Modeling Approaches. Front. Phys. 2019, 7, 116. [Google Scholar] [CrossRef]
- Grudzien, K.; Chaniecki, Z.; Romanowski, A.; Niedostatkiewicz, M.; Sankowski, D. ECT Image analysis methods for shear zone measurements during silo discharging process. Chin. J. Chem. Eng. 2012, 20, 337–345. [Google Scholar] [CrossRef]
- Grudzien, K.; Romanowski, A.; Chaniecki, Z.; Niedostatkiewicz, M.; Sankowski, D. Description of the silo flow and bulk solid pulsation detection using ECT. Flow Meas. Instrum. 2010, 21, 198–206. [Google Scholar] [CrossRef]
- Andreotti, B. Sonic sands. Rep. Prog. Phys. 2012, 75, 24. [Google Scholar] [CrossRef]
- Rao, K.K.; Nott, P.R. An Introduction to Granular Flow; Cambridge University Press: Cambridge, UK, 2008. [Google Scholar]
- Aguirre, M.A.; Grande, J.G.; Calvo, A.; Pugnaloni, L.A.; Géminard, J.-C. Granular flow through an aperture: Pressure and flow rate are independent. Phys. Rev. E Stat. Nonlin. Soft. Matter. Phys. 2011, 83, 061305. [Google Scholar] [CrossRef]
- Choi, J.; Kudrolli, A.; Bazant, M.Z. Velocity profile of granular flows inside silos and hoppers. J. Phys. Condens. Matter. 2005, 17, S2533–S2548. [Google Scholar] [CrossRef]
- Maiti, R.; Das, G.; Das, P.K. Granular drainage from a quasi-2D rectangular silo through two orifices symmetrically and asymmetrically placed at the bottom. Phys. Fluids 2017, 29, 103303. [Google Scholar] [CrossRef]
- Mankoc, C.; Janda, A.; Arévalo, R.; Pastor, J.M.; Zuriguel, I.; Garcimartín, A.; Maza, D. The flow rate of granular materials through an orifice. Granul. Matter. 2007, 9, 407–414. [Google Scholar] [CrossRef]
- Medina, A.; Serrano, D.A.; Gutiérrez, G.J. On the mass flow rate from silo with lateral exit holes. Rev. Mex. Fis. 2013, 59, 287–291. [Google Scholar]
- Medina, A.; Cabrera, D.; López-Villa, A.; Pliego, M. Discharge rates of dry granular material from bins with lateral exit holes. Powder Technol. 2014, 253, 470–475. [Google Scholar] [CrossRef]
- Serrano, D.A.; Medina, A.; Chavarria, G.R.; Pliego, M.; Klapp, J. Mass flow rate of granular material flowing from tilted bins. Powder Technol. 2015, 286, 438–443. [Google Scholar] [CrossRef]
- Serrano, D.A.; Ruiz-Chavarria, G.; Pliego, M.; Vargas, C.A. On the validity of the Hagen and Beverloo formulas for grains discharge through thin sidewalls of bins. Rev. Mex. Fis. 2019, 65, 139–147. [Google Scholar] [CrossRef]
- Serrano, D.A.; Sanchez-Silva, F.; Klapp, J.; Medina, A. The Hagen-Beverloo law for outflow of granular solids from holes on side walls. Rev. Mex. Fis. 2015, 61, 207–210. [Google Scholar]
- Beverloo, W.A.; Leniger, H.A.; van de Velde, J. The flow of granular solids through orifices. Chem. Eng. Sci. 1961, 15, 260–269. [Google Scholar] [CrossRef]
- Tighe, B.P.; Sperl, M. Pressure and motion of dry sand: Translation of Hagen’s paper from 1852. Granul. Matter 2007, 9, 141–144. [Google Scholar] [CrossRef]
- Madrid, M.A.; Darias, J.R.; Pugnaloni, L.A. A differential equation for the flow rate during silo discharge: Beyond the Beverloo rule. EPJ Web Conf. 2017, 140, 03041. [Google Scholar] [CrossRef]
- Darias, J.R.; Madrid, M.A.; Pugnaloni, L.A. Differential equation for the flow rate of discharging silos based on energy balance. Phys. Rev. E 2020, 101, 052905. [Google Scholar] [CrossRef]
- Sperl, M. Experiments on corn pressure in silo cells—Translation and comment of Janssen’s paper from 1895. Granul. Matter. 2006, 8, 59–65. [Google Scholar] [CrossRef]
- Walters, J.K. A theoretical analysis of stresses in silos with vertical walls. Chem. Eng. Sci. 1973, 28, 13–21. [Google Scholar] [CrossRef]
- Nedderman, R.M. Statics and Kinematics of Granular Materials; Cambridge University Press: Cambridge, UK, 1992. [Google Scholar]
- Boutreux, T.; Raphaël, E.; de Gennes, P.G. Propagation of a pressure step in a granular material: The role of wall friction. Phys. Rev. E 1997, 55, 5759–5773. [Google Scholar] [CrossRef]
- de Gennes, P.G. Granular matter: A tentative view. Rev. Mod. Phys. 1999, 71, S374–S382. [Google Scholar] [CrossRef]
- Andreotti, B.; Forterre, Y.; Pouliquen, O. Granular Media: Between Fluid and Solid; Cambridge University Press: Cambridge, UK, 2013. [Google Scholar]
- Wang, X.; Lianga, C.; Guoa, X.; Chen, Y.; Liua, D.; Ma, J.; Chen, X.; An, H. Experimental study on the dynamic characteristics of wall normal stresses during silo discharge. Powder Technol. 2020, 363, 509–518. [Google Scholar] [CrossRef]
- Dyck, G.; Rogers, A.; Paliwal, J. A Review of Analytical Methods for Calculating Static Pressures in Bulk Solids Storage Structures. KONA Powder Part J. 2024, 41, 108–122. [Google Scholar] [CrossRef]
- Balmer, R.T. The Operation of Sand Clocks and Their Medieval Development. Tech. and Cult. 1978, 19, 615–632. [Google Scholar] [CrossRef]
- Sack, A.; Pöschel, T. Weight of an hourglass–Theory and experiment in quantitative comparison. Am. J. Phys. 2017, 85, 98–107. [Google Scholar] [CrossRef]
- Pongó, T.; Stiga, V.; Török, J.; Lévay, S.; Szabo, B.; Stannarius, R.; Cruz Hidalgo, R.; Börzsönyi. Flow in an hourglass: Particle friction and stiffness matter. New J. Phys. 2021, 23, 023001. [Google Scholar] [CrossRef]
- Yang, X.; Liu, Z.; Liu, L. DEM investigation on the dynamic characteristics of wall normal stress and particle flow during silo discharge. Powder Technol. 2024; in press. [Google Scholar] [CrossRef]
- Breard, E.C.P.; Dufek, J.; Fullard, L.; Carrara, A. The basal friction coefficient of granular flows with and without excess pore pressure: Implications for pyroclastic density currents, water-rich debris flows, and rock and submarine avalanches. JGR Solid Earth 2020, 125, 22. [Google Scholar] [CrossRef]
- Duran, J. Sands, Powders, and Grains; Springer: New York, NY, USA, 1999. [Google Scholar]
- Goldhirsch, I.; Goldenberg, C. Stress in Dense Granular Materials. In The Physics of Granular Media; WILEY-VCH Verlag GmbH and Co. KGaA: Weinheim, Germany, 2004. [Google Scholar] [CrossRef]
- Wieghardt, K. Experiments in granular flow. Annu. Rev. Fluid Mech. 1975, 7, 89–114. [Google Scholar] [CrossRef]
- Lumay, G.; Boschini, F.; Traina, K.; Bontempi, S.; Remy, J.-C.; Cloots, R.; Vandewalle, N. Measuring the flowing properties of powders and grains. Powder Technol. 2012, 224, 19–27. [Google Scholar] [CrossRef]
- Harcarik, T.; Bocko, J.; Maslakova, K. Frequency Analysis of Acoustic Signal using the Fast Fourier Transformation in MATLAB. Procedia Eng. 2012, 48, 199–204. [Google Scholar] [CrossRef]
- Hernández-Juárez, J.; López-Villa, A.; Serrano, D.A.; Medina, A. Measurement of the sound pressure level during the discharge process of a tilted silo. In Proceedings of the 2017 CHILEAN Conference on Electrical, Electronics Engineering, Information and Communication Technologies (CHILECON), Pucon, Chile, 18–20 October 2017; pp. 1–6. [Google Scholar]
- Zhou, Y.; Lagrée, P.-Y.; Popinet, S.; Ruyer, P.; Aussillous, P. Experiments on, and discrete and continuum simulations of, the discharge of granular media from silos with a lateral orifice. J. Fluid Mech. 2017, 289, 459–485. [Google Scholar] [CrossRef]
- Serrano, D.A.; Sánchez-Silva, F.; Klapp, J.; Tamayo, P.; Medina, A.; Domínguez, G. Trajectories of Water and Sand Jets. In Recent Advances in Fluid Dynamics with Environmental Applications; Environmental Science and Engineering; Springer: Berlin/Heidelberg, Germany, 2016. [Google Scholar] [CrossRef]
- Sheldon, H.G.; Douglas, J.D. Granular discharge and clogging for tilted hoppers. Granul. Matter. 2010, 12, 579–585. [Google Scholar] [CrossRef]
- Howard, I. Vibration signal processing using MATLAB. Acoust. Aust. 1995, 23, 9–13. [Google Scholar]

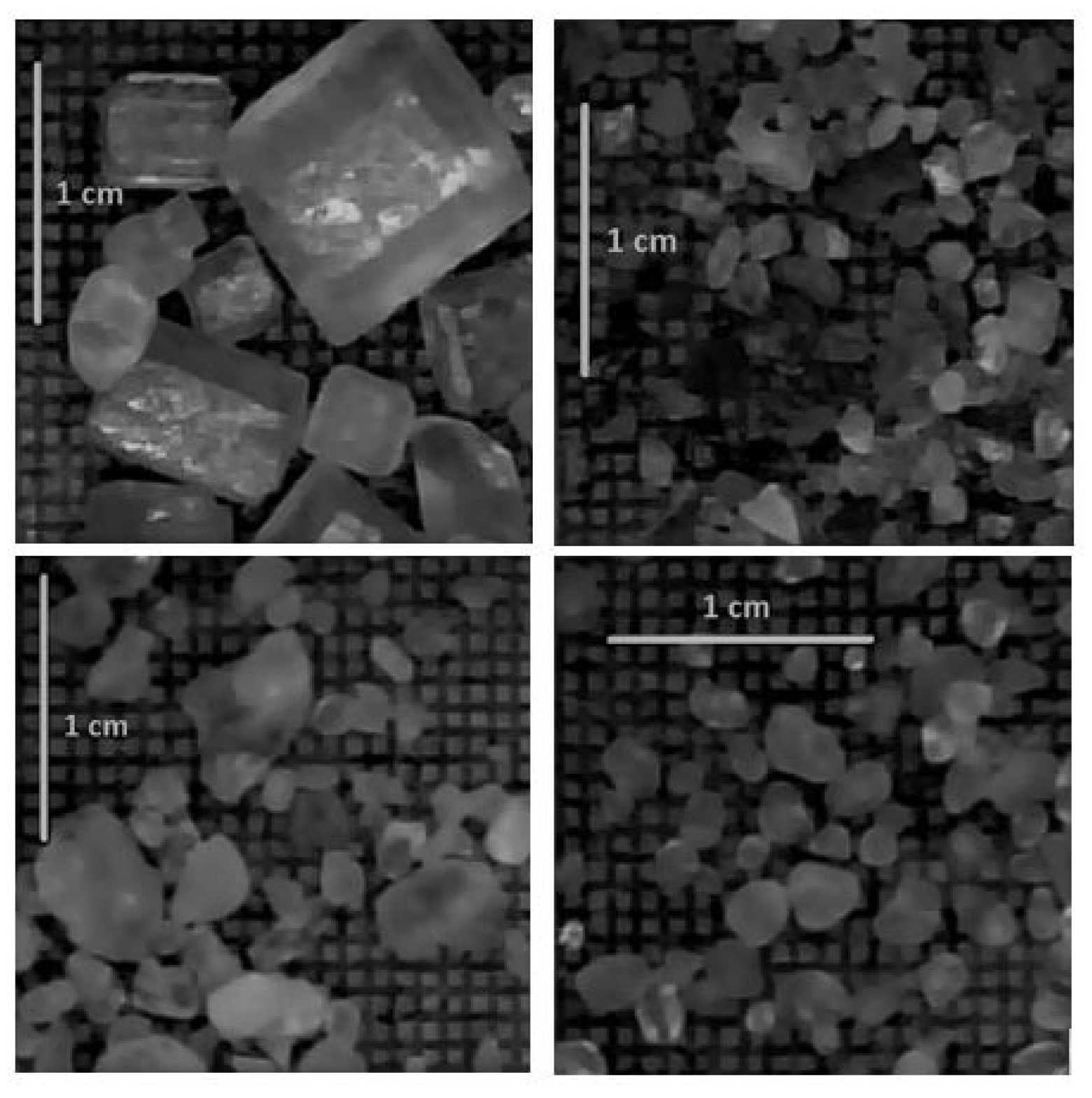


| Material | Length | External | Wall | Surface | Natural Frequency |
|---|---|---|---|---|---|
| Glass | 46.5 cm | 4.5 cm | 0.9 cm | smooth | 175.8 Hz |
| Granular Material | [] | |||
|---|---|---|---|---|
| Beach Sand | 0.29 | 1.58 ± 0.01 | ± 0.5 | 0.65 ± 0.01 |
| Sugar | 0.95 | 1.06 ± 0.01 | ± 0.5 | 0.62 ± 0.01 |
| Silica Sand I | 0.42 | 1.61 ± 0.01 | ± 0.5 | 0.65 ± 0.01 |
| Silica Sand II | 0.23 | 1.81 ± 0.01 | ± 0.5 | 0.53 ± 0.01 |
| Granular Material | (s) | (cm) |
|---|---|---|
| 9.6 | 5.71 | |
| Silica sand | 6.2 | 3.68 |
| Silica sand | 10.4 | 6.18 |
| Silica sand | 13.32 | 7.89 |
| Silica sand | 15.12 | 8.96 |
| * | 4.7 | 2.75 |
| 5.01 | 2.93 | |
| Silica sand | 11.19 | 6.55 |
| Silica sand | 12.12 | 7.1 |
| Silica sand | 8.74 | 5.02 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hernández-Juárez, J.R.; López-Villa, A.; Medina, A.; Serrano Huerta, D.A. Low-Frequency Acoustic Emissions During Granular Discharge in Inclined Silos. Fluids 2025, 10, 138. https://doi.org/10.3390/fluids10050138
Hernández-Juárez JR, López-Villa A, Medina A, Serrano Huerta DA. Low-Frequency Acoustic Emissions During Granular Discharge in Inclined Silos. Fluids. 2025; 10(5):138. https://doi.org/10.3390/fluids10050138
Chicago/Turabian StyleHernández-Juárez, Josué Roberto, Abel López-Villa, Abraham Medina, and Daniel Armando Serrano Huerta. 2025. "Low-Frequency Acoustic Emissions During Granular Discharge in Inclined Silos" Fluids 10, no. 5: 138. https://doi.org/10.3390/fluids10050138
APA StyleHernández-Juárez, J. R., López-Villa, A., Medina, A., & Serrano Huerta, D. A. (2025). Low-Frequency Acoustic Emissions During Granular Discharge in Inclined Silos. Fluids, 10(5), 138. https://doi.org/10.3390/fluids10050138





