A Multifractal Vision of 5-Fluorouracil Release from Chitosan-Based Matrix
Abstract
1. Introduction
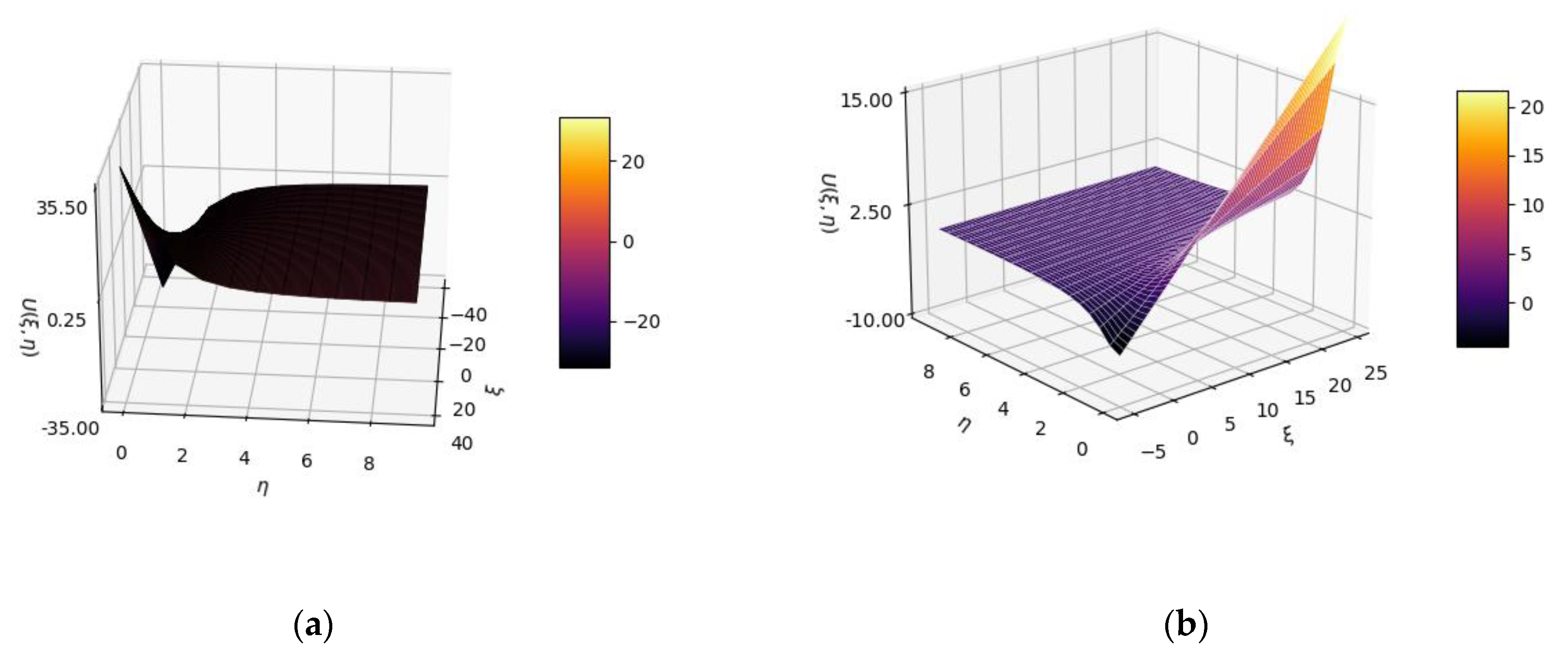
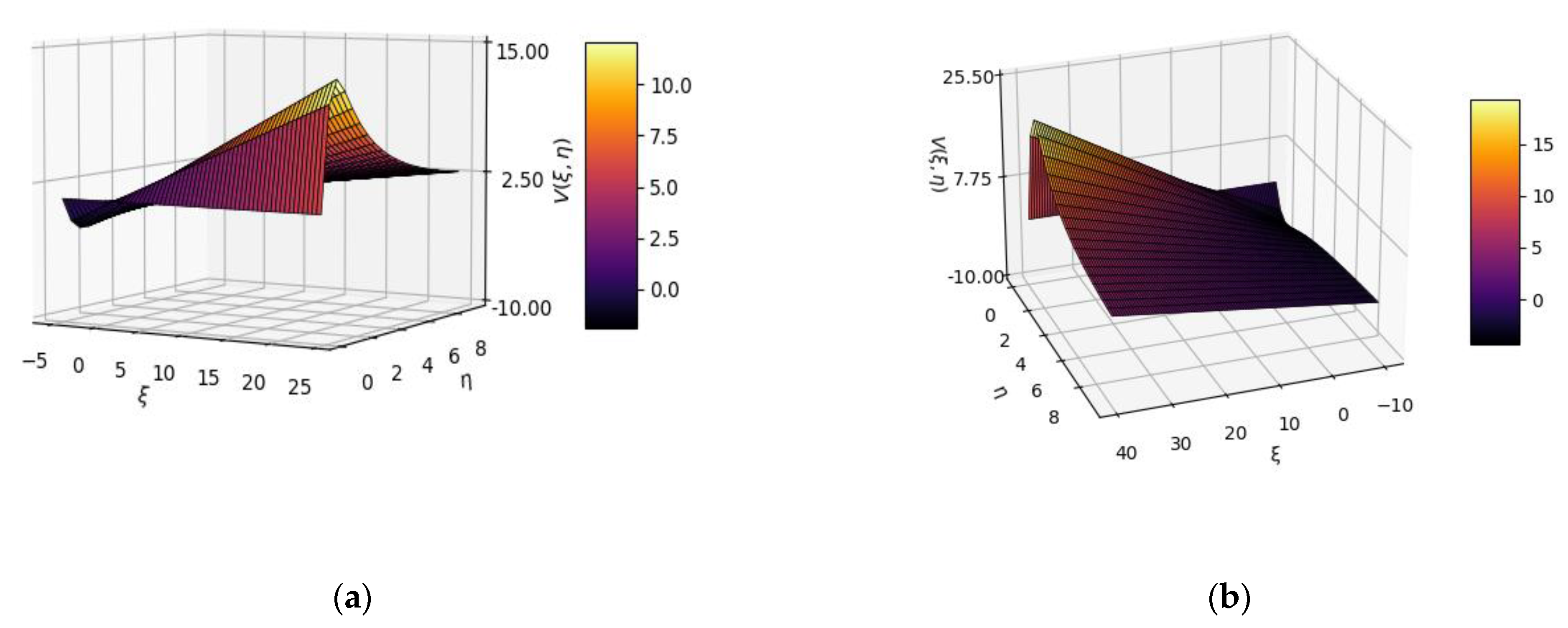
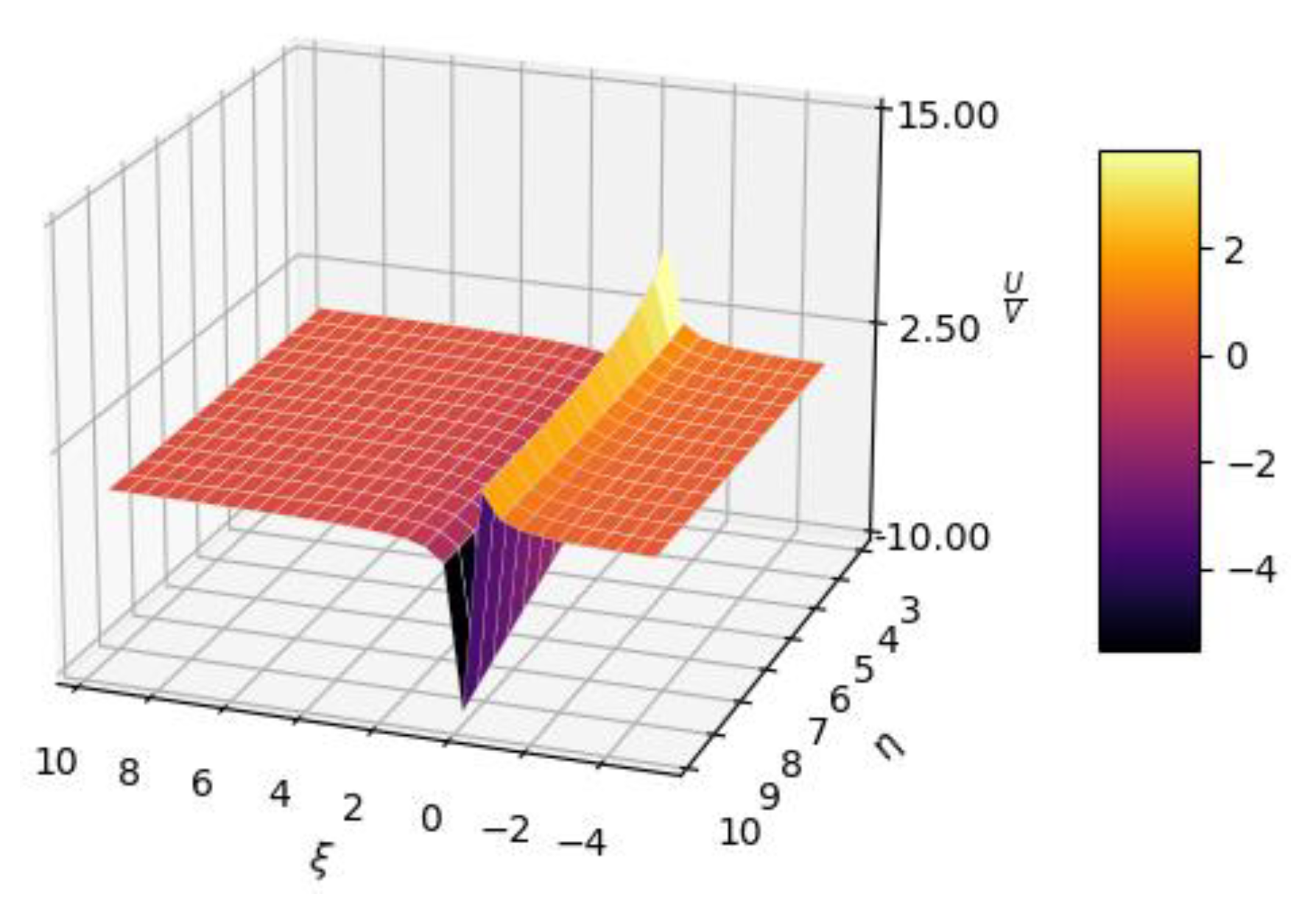
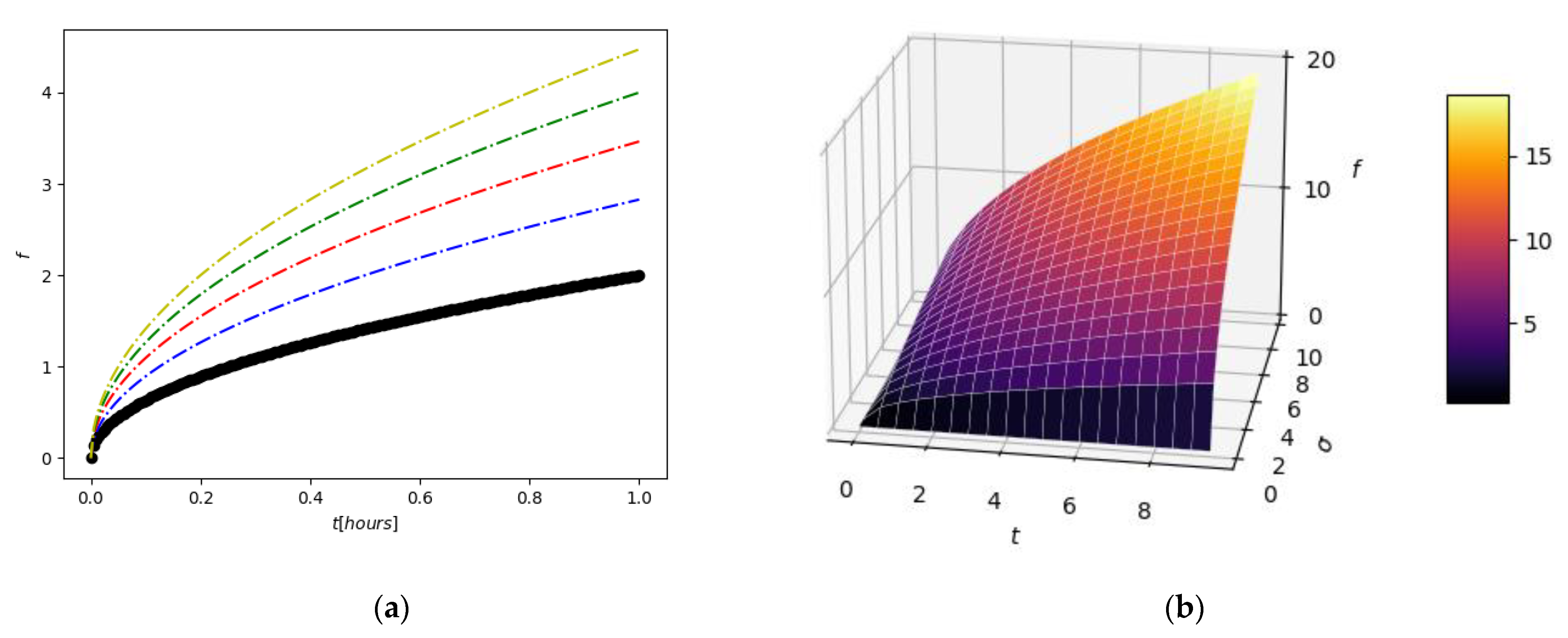
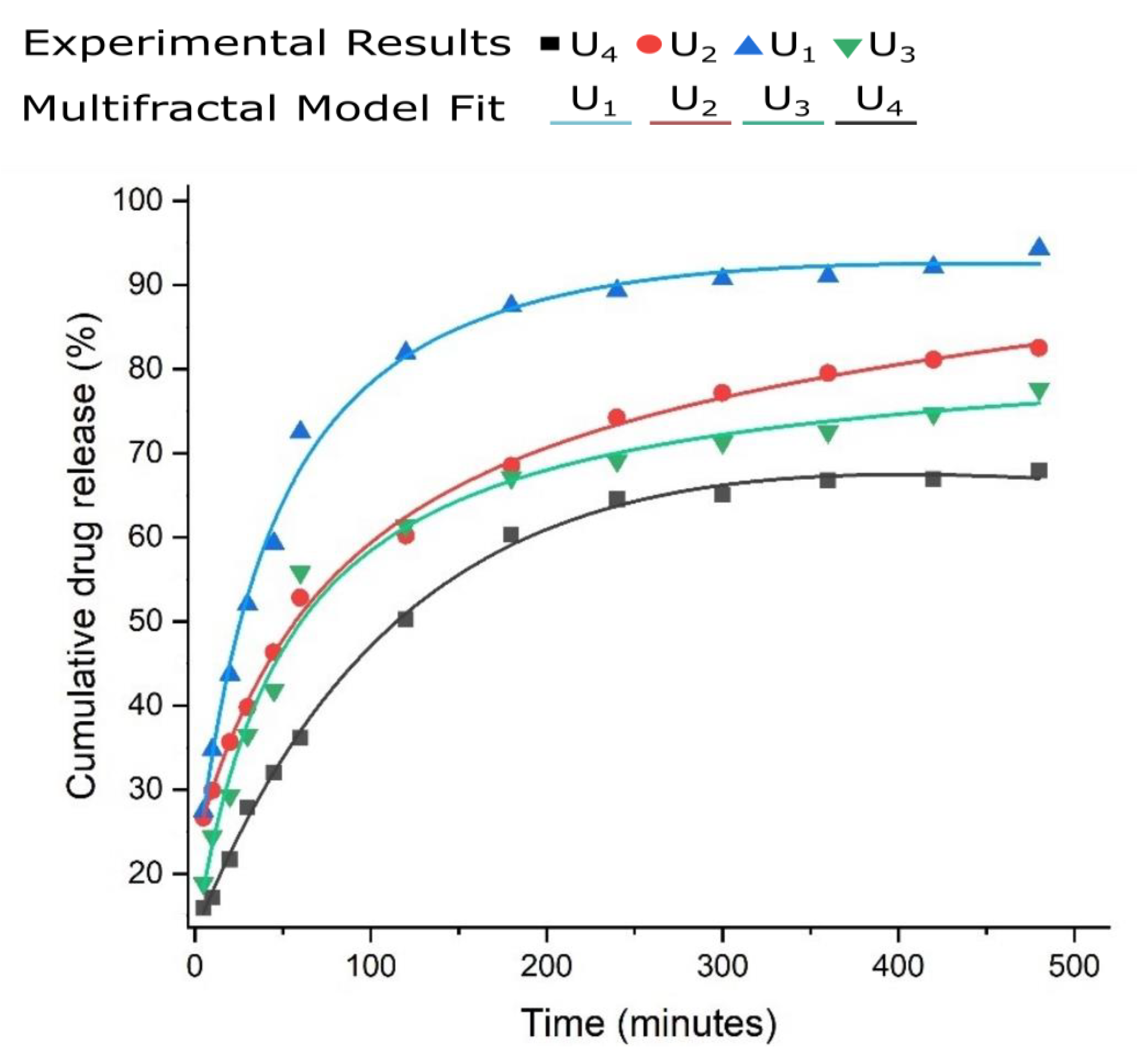
2. Results and Discussion
Theoretical Model
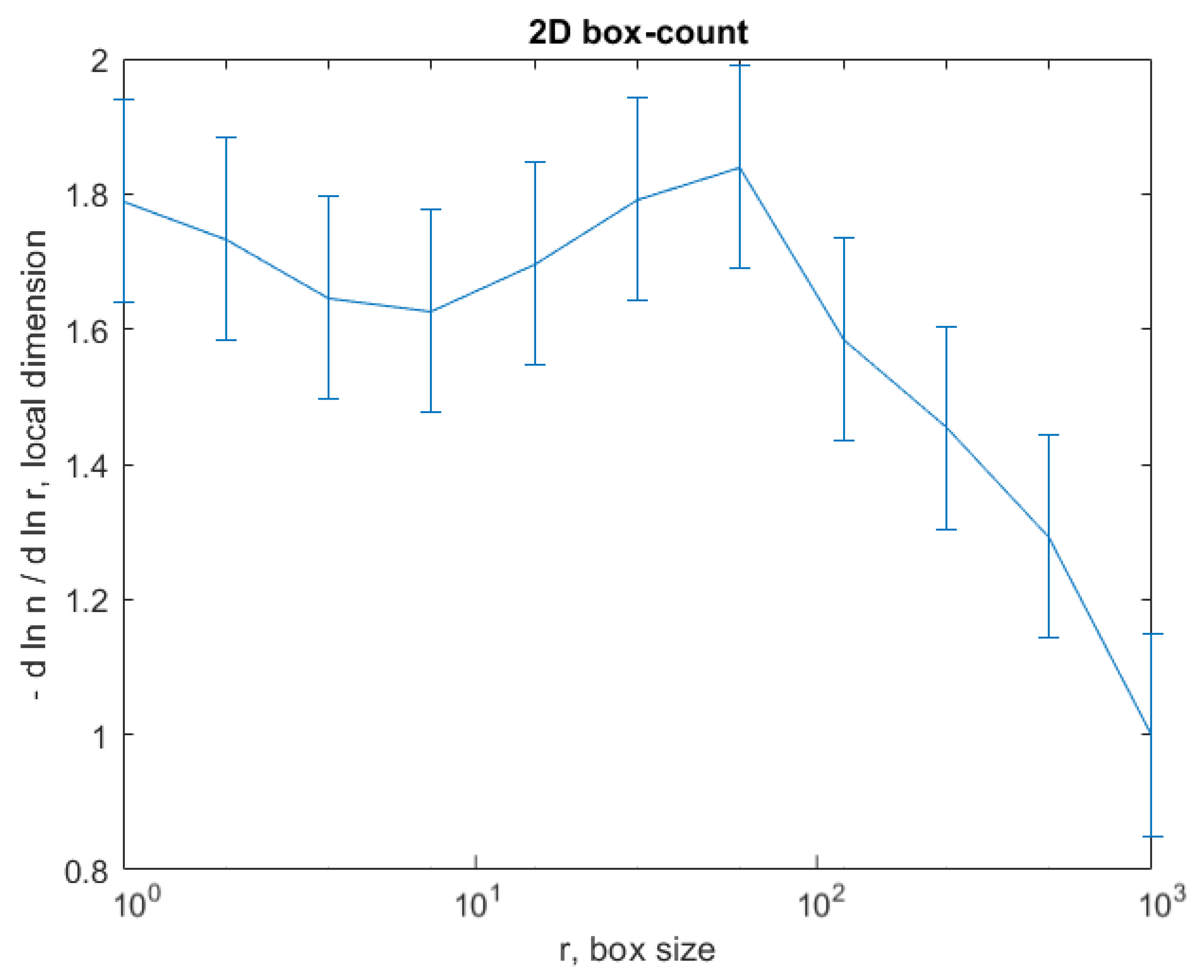
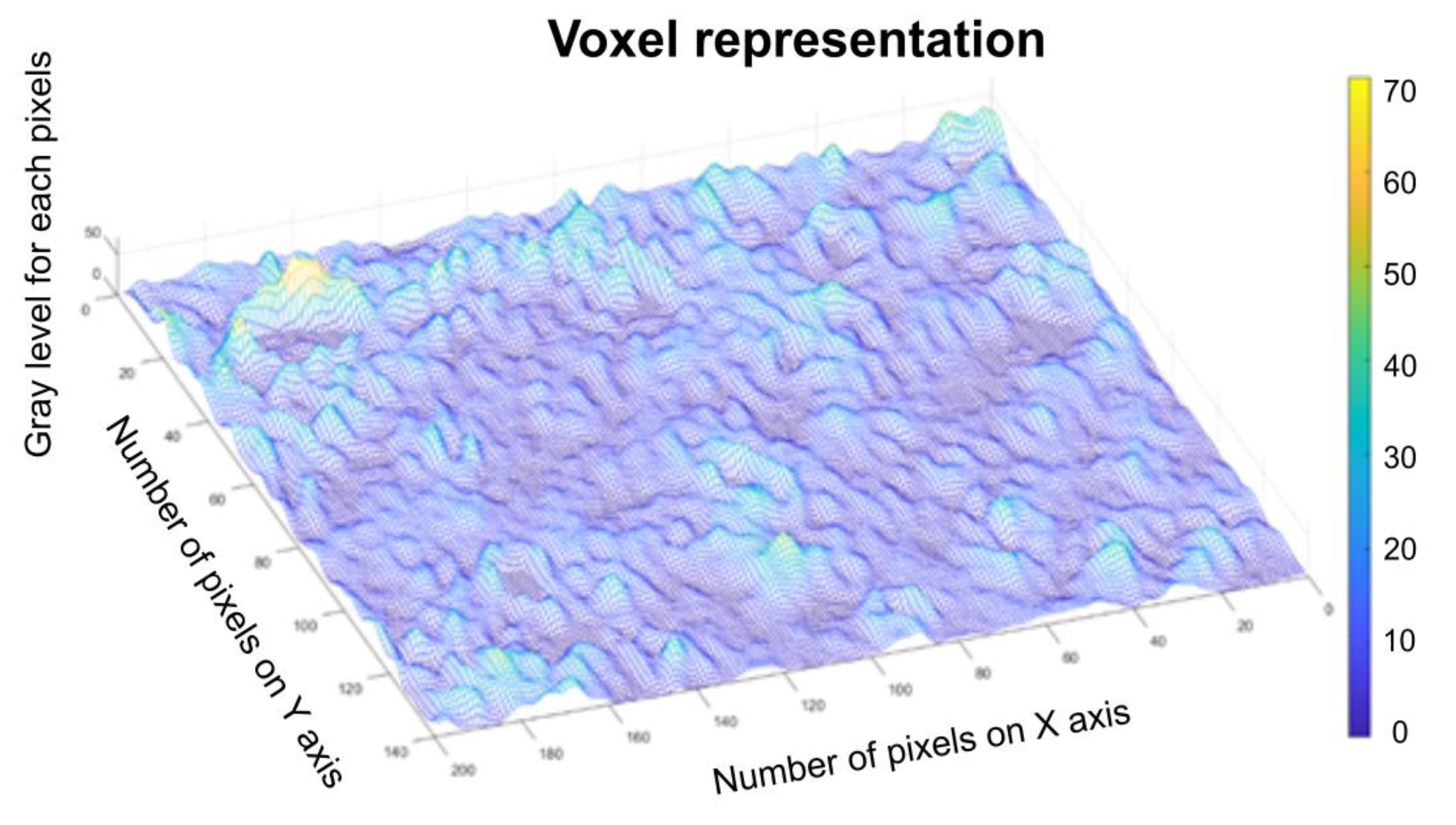
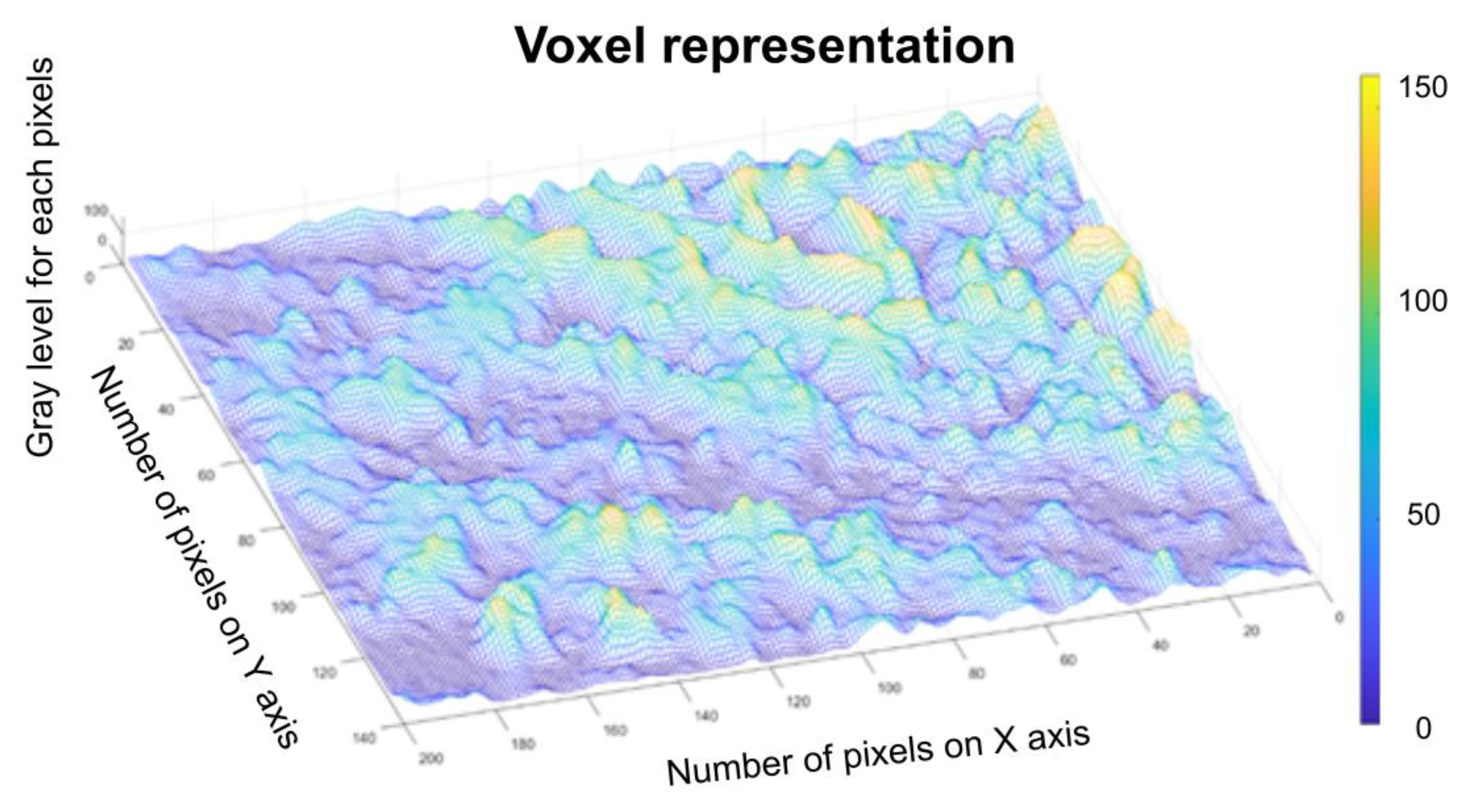
3. Evaluation of Polarized Optical Microscopy Pictures by Fractal Analysis
4. Conclusions
5. Materials and Methods
5.1. Materials
5.2. Synthesis of the Hydrogel Formulation Preparations
5.3. Methods
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Prausnitz, M.R. Langer, R. Transdermal drug delivery. Nat. Biotechnol. 2008, 26, 1261–1268. [Google Scholar] [CrossRef] [PubMed]
- Patel, A.; Cholkar, K.; Agrahari, V.; Mitra, A.K. Ocular drug delivery systems: An overview. World J. Pharmacol. 2013, 2, 47–64. [Google Scholar] [CrossRef]
- Jacob, J.; Haponiuk, J.T.; Thomas, S.; Gopi, S. Biopolymer based nanomaterials in drug delivery systems: A review. Mater. Today Chem. 2018, 9, 43–55. [Google Scholar] [CrossRef]
- Xiong, S.; Marin, L.; Duan, L.; Cheng, X. Fluorescent chitosan hydrogel for highly and selectively sensing of p-nitrophenol and 2, 4, 6-trinitrophenol. Carbohydr. Polym. 2019, 225, 115253. [Google Scholar] [CrossRef] [PubMed]
- Pereira, L.M. Fractal Pharmacokinetics. Comput. Math. Methods Med. 2010, 11, 161–184. [Google Scholar]
- Kosmidis, K.; Argyrakis, P.; Macheras, P. Fractal kinetics in drug release from finite fractal matrices. J. Chem. Phys. 2003, 119, 6373–6377. [Google Scholar] [CrossRef]
- Le Mehaute, A.; Crepy, G. Introduction to transfer and motion in fractal media: The geometry of kinetics. Solid State Ionics 1983, 9-10, 17–30. [Google Scholar] [CrossRef]
- Barkai, E.; Metzler, R.; Klafter, J. From continuous time random walk to the fractional Fokker-Planck equation. Phys. Rev. E 2000, 61, 132–138. [Google Scholar] [CrossRef]
- Zhang, L.; He, G.; Yu, Y.; Zhang, Y.; Li, X.; Wang, S. Design of Biocompatible Chitosan/Polyaniline/Laponite Hydrogel with Photothermal Conversion Capability. Biomolecules 2022, 12, 1089. [Google Scholar] [CrossRef] [PubMed]
- Jiang, W.; Zhao, P.; Song, W.; Wang, M.; Yu, D.-G. Electrospun Zein/Polyoxyethylene Core-Sheath Ultrathin Fibers and Their Antibacterial Food Packaging Applications. Biomolecules 2022, 12, 1110. [Google Scholar] [CrossRef] [PubMed]
- Liu, H.; Wang, H.; Lu, X.; Murugadoss, V.; Huang, M.; Yang, H.; Wan, F.; Yu, D.-G.; Guo, Z. Electrospun structural nanohybrids combining three composites for fast helicide delivery. Adv. Compos. Hybrid Mater. 2022, 5, 1017–1029. [Google Scholar] [CrossRef]
- Higaki, K.; Yamashita, S.; Amidon, G.L. Time-dependent oral absorption models. J. Pharmacokinet. Pharmacodyn. 2001, 28, 109–128. [Google Scholar] [CrossRef] [PubMed]
- Karalis, V.; Tsantili-Kakoulidou, A.; Macheras, P. Quantitative structure–pharmacokinetic relationships for disposition parameters of cephalosporins. Eur. J. Pharm. Sci. 2003, 20, 115–123. [Google Scholar] [CrossRef]
- Chechetkin, V.R.; Lutovinov, V.S.; Turygin, A.Y. Multifractal structure of fully developed hydrodynamic turbulence. I. Kolmogorov’s third hypothesis revisited. J. Stat. Phys. 1990, 61, 573–588. [Google Scholar] [CrossRef]
- Basu, A.; Chakrabarti, B.K. Hydrodynamic descriptions for surface roughness in fracture front propagation. Philos Trans A Math Phys Eng Sci. 2019, 377, 20170387. [Google Scholar] [CrossRef]
- Kwak, K.; Yang, S. Developing a Multi-Dimensional Hydrodynamics Code with Astrochemical Reactions. IAU Gen. Assem. 2015, 29, 2250754. [Google Scholar]
- Iancu, R.; Irimiciuc, S.A.; Agop, M.; Frasila, M.; Paun, M.-A.; Paun, V.-A.; Paun, V.-P.; Stratulat, S. 5-fluorouracil release from chitosan-based matrix. Experimental and theoretical aspects. Mater. Plast. 2020, 57, 180–188. [Google Scholar] [CrossRef]
- O’Shaughnessy, B.; Procaccia, I. Analytical solutions for diffusion on fractal objects. Phys. Rev. Lett. 1985, 54, 455–458. [Google Scholar] [CrossRef]
- Richard, R. 1D Diffusion PDE. Introduction to Partial Differential Equations. 2019. Available online: http://personal.ph.surrey.ac.uk/~phs1rs/teaching/l3_pdes.pdf (accessed on 15 June 2022).
- Polowsky, P.J.; Tansman, G.; Kindstedt, P.S.; Hughes, J.M. Characterization and identification of surface crystals on smear-ripened cheese by polarized light microscopy. J. Dairy Sci. 2018, 101, 7714–7723. [Google Scholar] [CrossRef]
- Karperien, A.L.; Jelinek, H.F. Box-Counting Fractal Analysis: A Primer for the Clinician. In The Fractal Geometry of the Brain; Di Ieva, A., Ed.; Second Chapter; Springer Series in Computational Neuroscience; Springer Science + Business Media: New York, NY, USA, 2016. [Google Scholar]
- Karperien, A.; Jelinek, H.F.; Miloševic, N.T. Reviewing Lacunarity Analysis and Classification of Microglia in Neuroscience. In Proceedings of the 8th European Conference on Mathematical and Theoretical Biology, Kraków, Poland, 28 June–2 July 2011; pp. 1–6. [Google Scholar]
- Lungu, R.; Paun, M.-A.; Peptanariu, D.; Ailincai, D.; Marin, L.; Nichita, M.-V.; Paun, V.-A.; Paun, V.-P. Biocompatible Chitosan-Based Hydrogels for Bioabsorbable Wound Dressings. Gels 2022, 8, 107. [Google Scholar] [CrossRef]
- Nichita, M.V.; Paun, M.A.; Paun, V.A.; Paun, V.P. Fractal analysis of brain glial cells. Fractals dimension and lacunarity. Univ. Politeh. Buchar. Sci. Bull. Ser. A Appl. Math. Phys. 2019, 81, 273–284. [Google Scholar]
- Bordescu, D.; Paun, M.A.; Paun, V.A.; Paun, V.P. Fractal analysis of Neuroimagistic. Lacunarity degree, a precious indicator in the detection of Alzheimer’s disease. Univ. Politeh. Buchar. Sci. Bull. Ser. A Appl. Math. Phys. 2018, 80, 309–320. [Google Scholar]
- Postolache, P.; Borsos, Z.; Paun, V.A.; Paun, V.P. New Way in Fractal Analysis Of Pulmonary Medical Images. Univ. Politeh. Buchar. Sci. Bull. Ser. A Appl. Math. Phys. 2018, 80, 313–322. [Google Scholar]
- Scott, D.W. Statistics: A Concise Mathematical Introduction for Students, Scientists, and Engineers; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2020. [Google Scholar]
- Available online: http://imagesci.fch.vut.cz/includes/harfa_download.inc.php (accessed on 15 June 2022).
- Wang, X.; Ahmed, B.N.; Alvarez, S.G.; Tuttolomondo, M.V.; Hélary, C.; Desimone, M.F.; Coradin, T. Sol-gel encapsulation of biomolecules and cells for medicinal applications. Curr. Top. Med. Chem. 2015, 15, 223–244. [Google Scholar] [CrossRef] [PubMed]


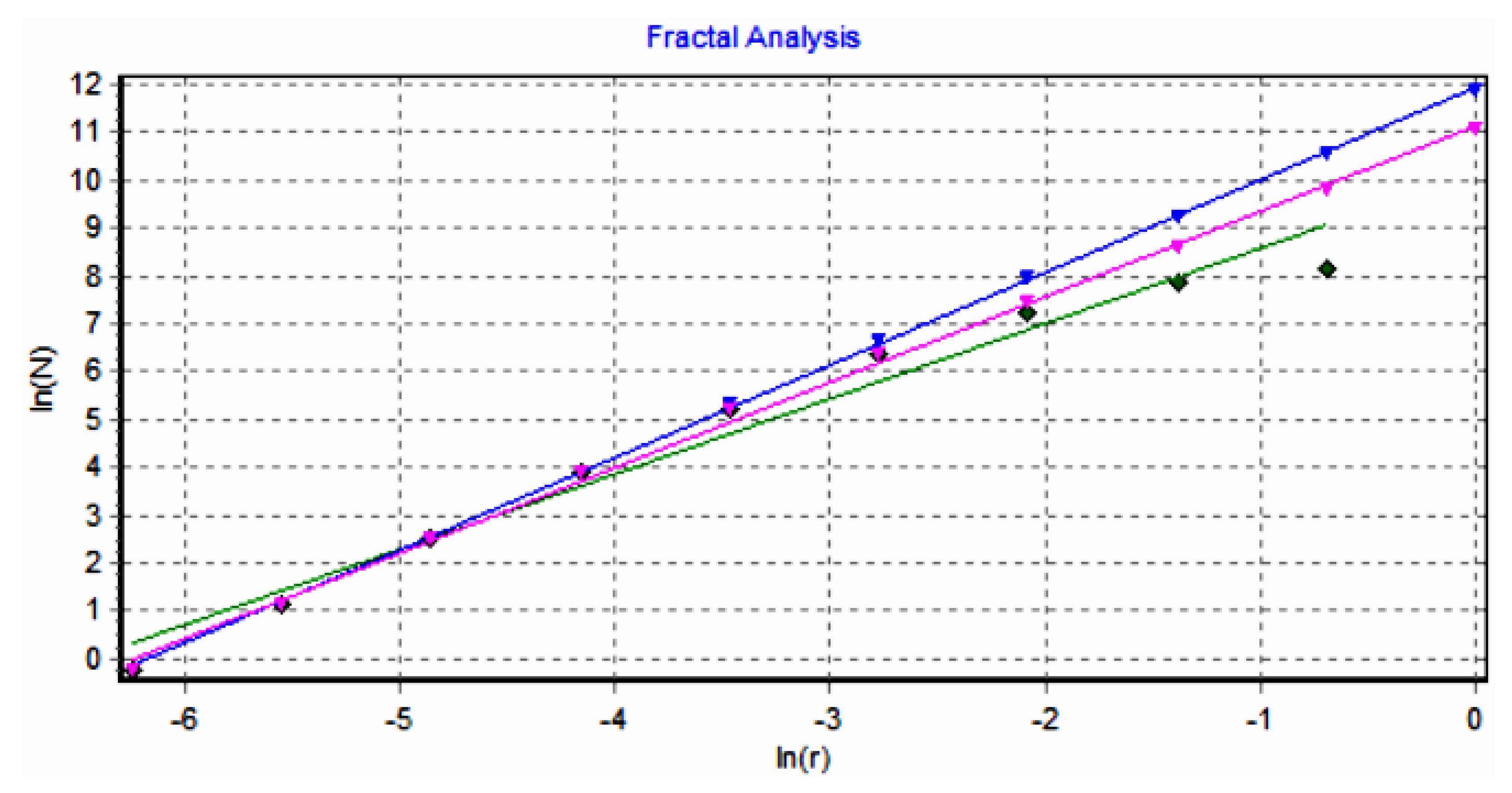
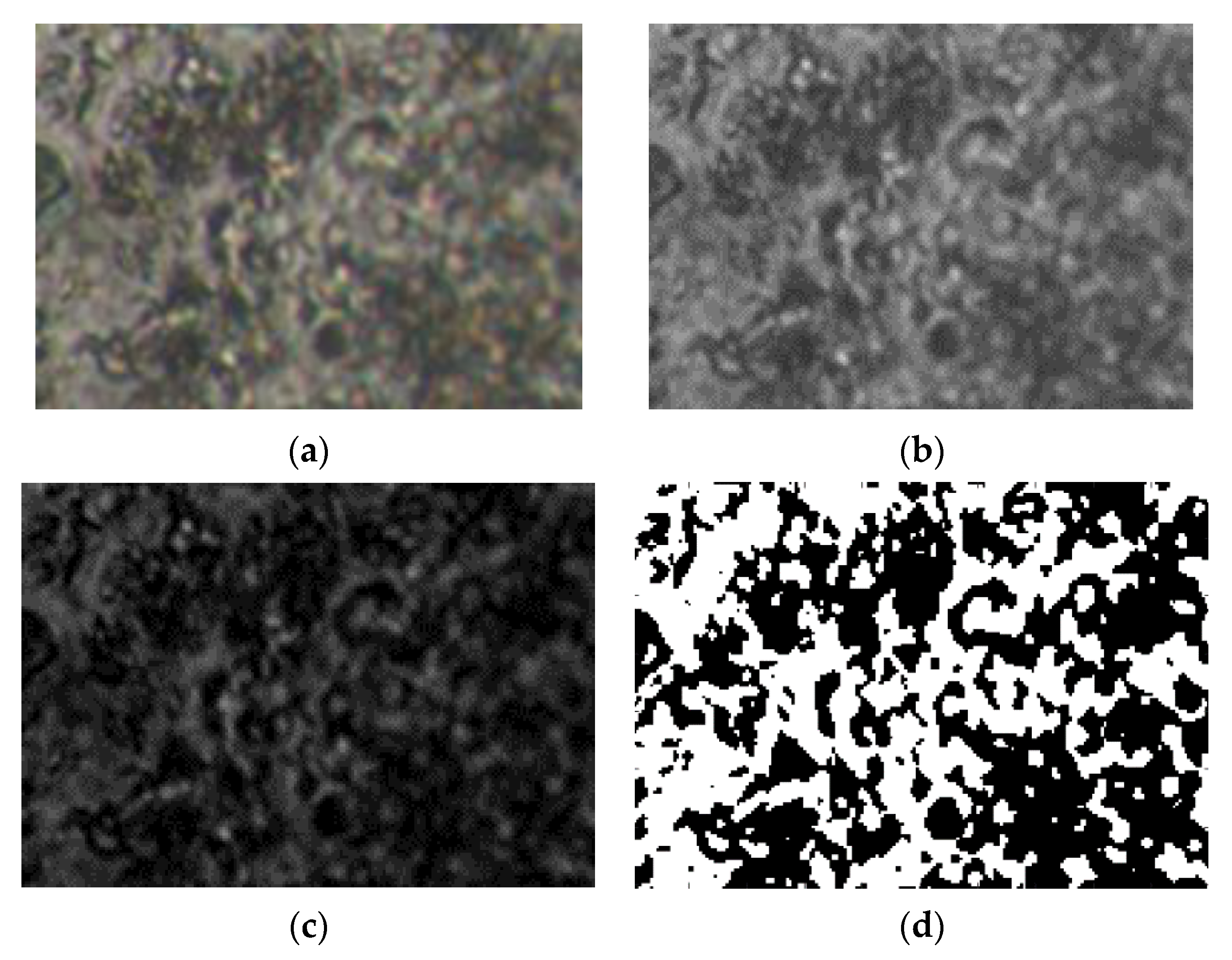

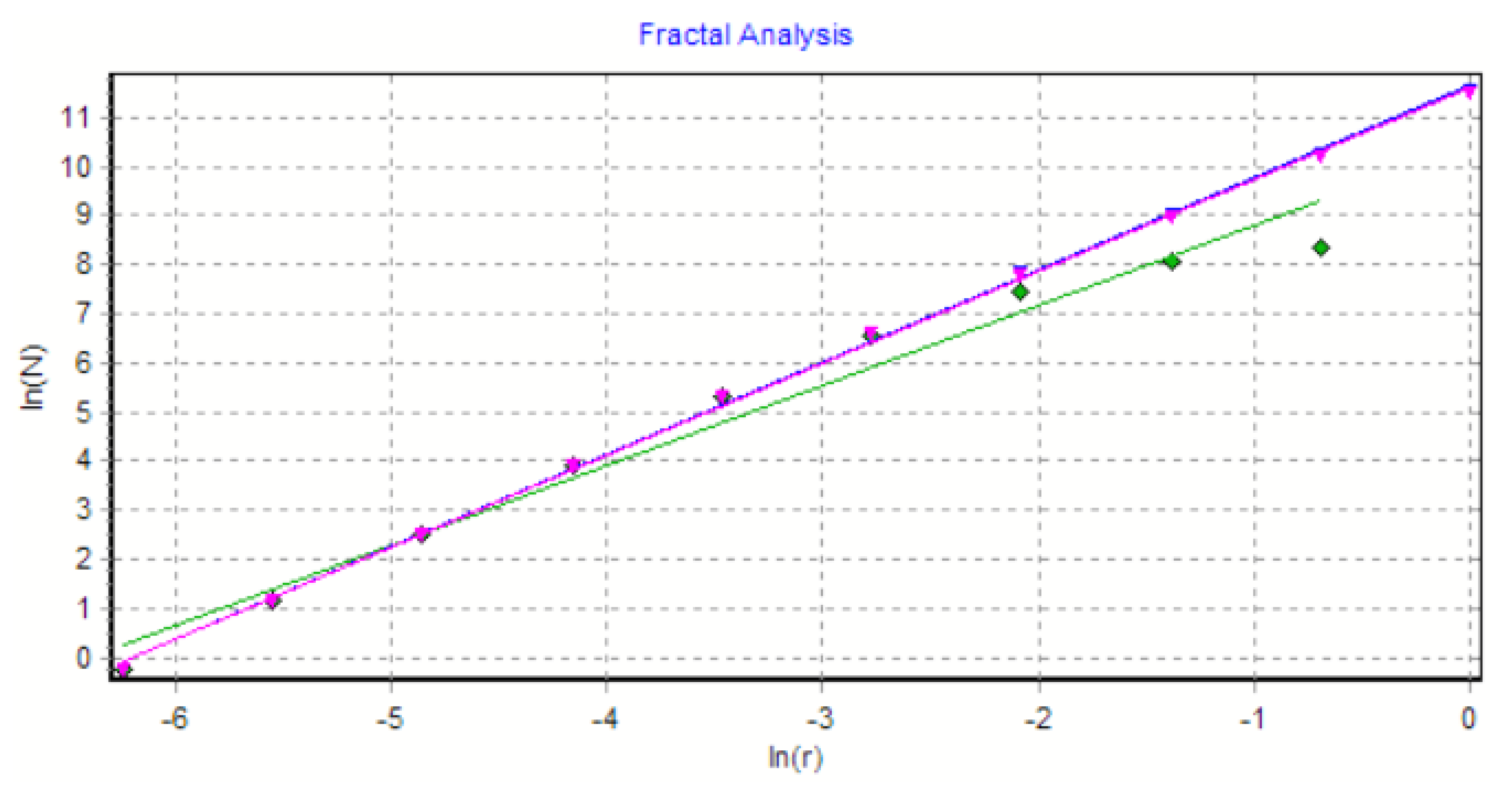
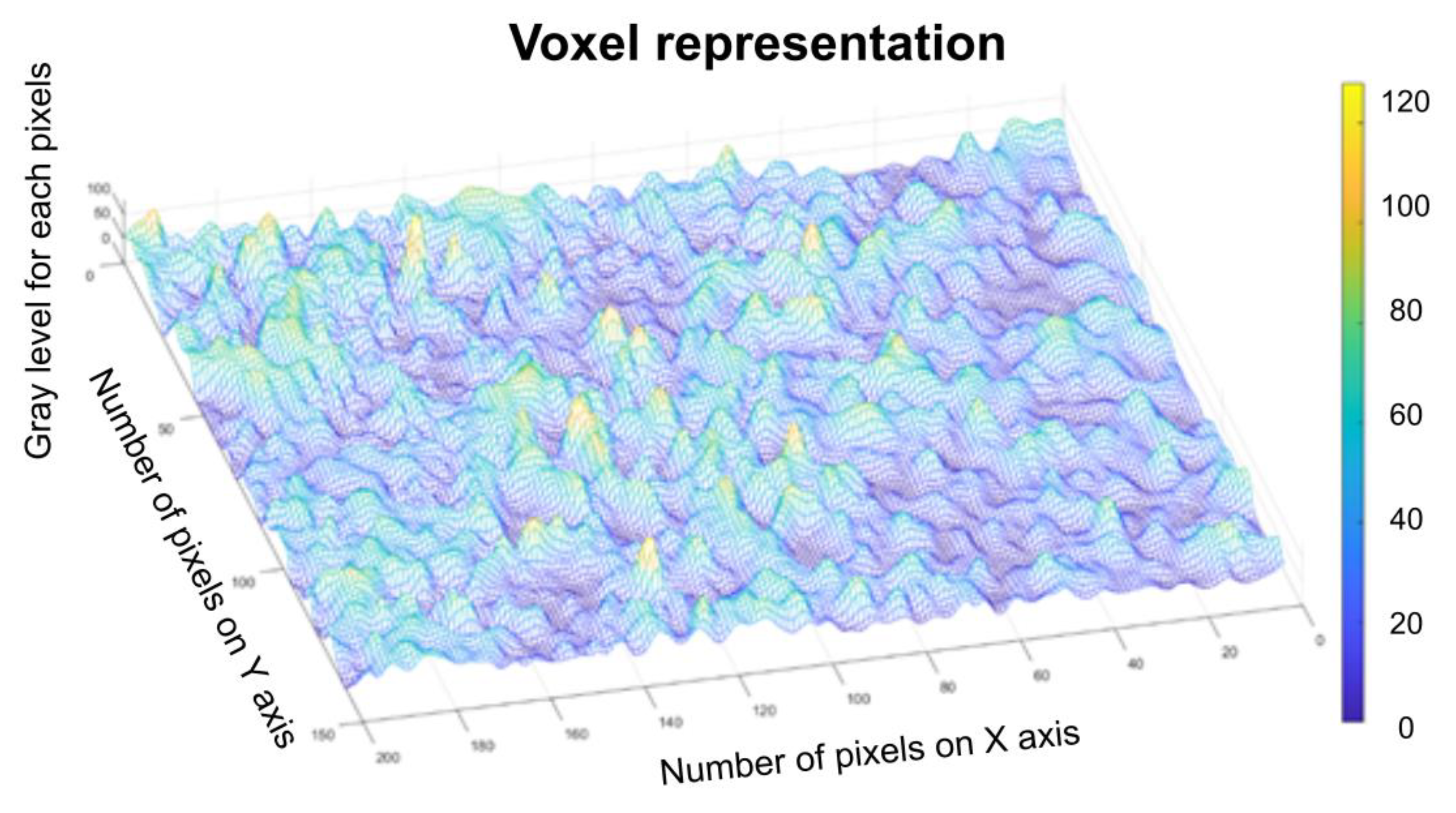
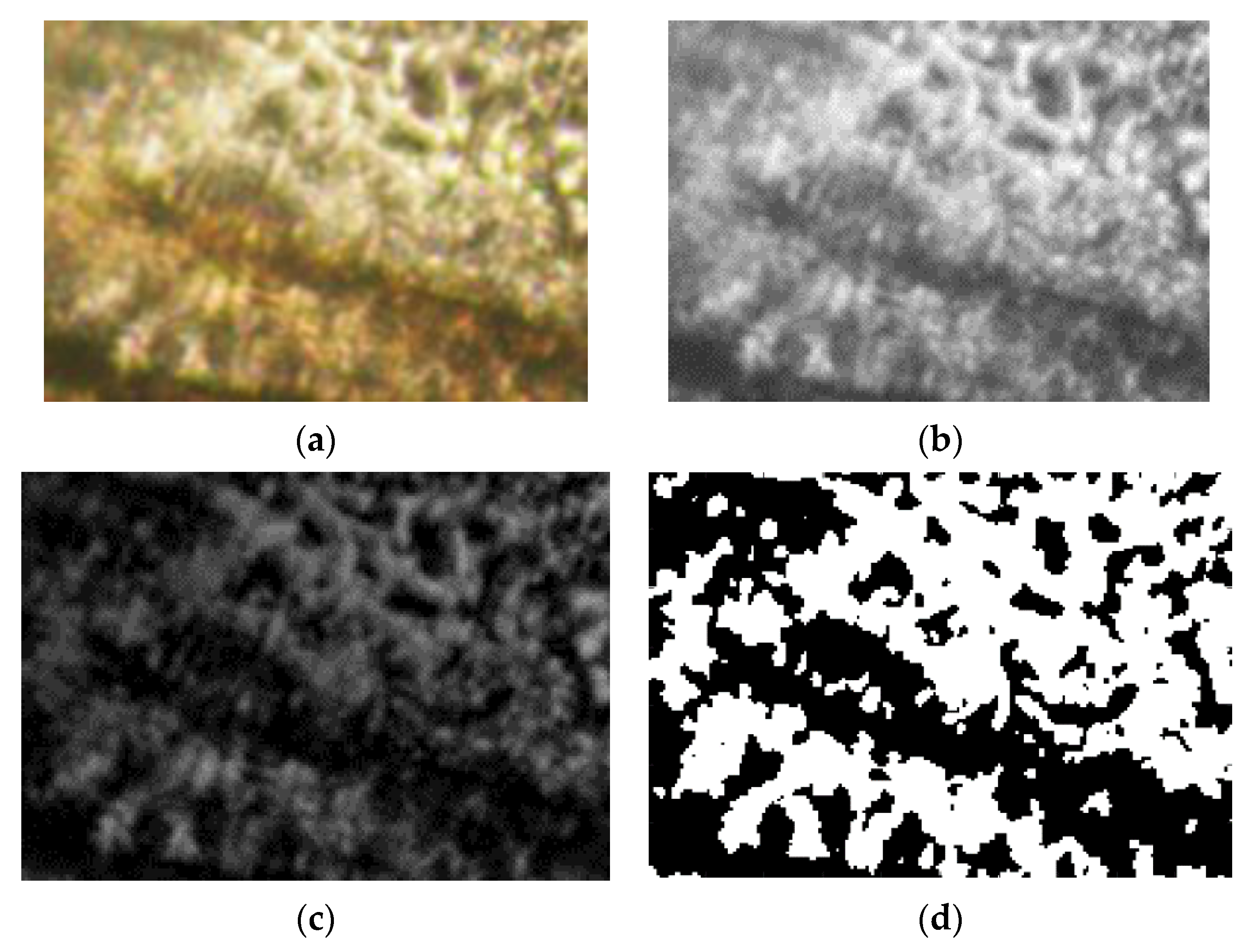


| Name | Fractal Dimension | Standard Deviation | Lacunarity |
|---|---|---|---|
| Image U1 | 1.7602 | ±0.2026 | 0.0215 |
| Name | Fractal Dimension | Standard Deviation | Lacunarity |
|---|---|---|---|
| Image U2 | 1.7523 | ±0.1949 | 0.0363 |
| Name | Fractal Dimension | Standard Deviation | Lacunarity |
|---|---|---|---|
| Image U4 | 1.7352 | ±0.1831 | 0.0385 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Paun, M.-A.; Paun, V.-A.; Paun, V.-P. A Multifractal Vision of 5-Fluorouracil Release from Chitosan-Based Matrix. Gels 2022, 8, 661. https://doi.org/10.3390/gels8100661
Paun M-A, Paun V-A, Paun V-P. A Multifractal Vision of 5-Fluorouracil Release from Chitosan-Based Matrix. Gels. 2022; 8(10):661. https://doi.org/10.3390/gels8100661
Chicago/Turabian StylePaun, Maria-Alexandra, Vladimir-Alexandru Paun, and Viorel-Puiu Paun. 2022. "A Multifractal Vision of 5-Fluorouracil Release from Chitosan-Based Matrix" Gels 8, no. 10: 661. https://doi.org/10.3390/gels8100661
APA StylePaun, M.-A., Paun, V.-A., & Paun, V.-P. (2022). A Multifractal Vision of 5-Fluorouracil Release from Chitosan-Based Matrix. Gels, 8(10), 661. https://doi.org/10.3390/gels8100661








