Transport Characteristics of Branched-Preformed Particle Gel in Porous Media: Influence of Elastic Modulus, Matching Coefficient, and Injection Rate
Abstract
1. Introduction
2. Results and Discussion
2.1. Swelling Ratio of B-PPG
2.2. Particle Size Distribution and D50 Determination of B-PPGs
2.3. FTIR Characterization of B-PPG
2.4. Factors Affecting the Transport Characteristics of B-PPG
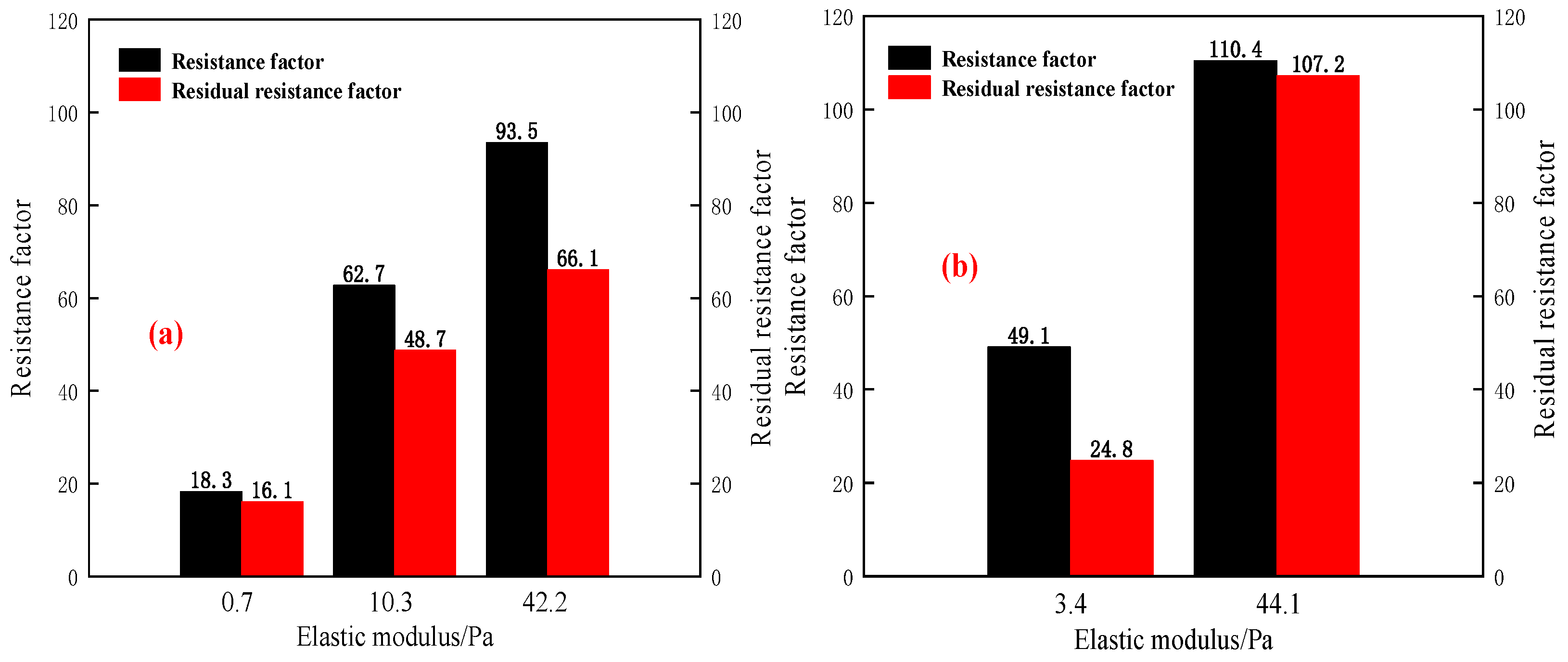
2.4.1. Effect of Elastic Modulus of B-PPG
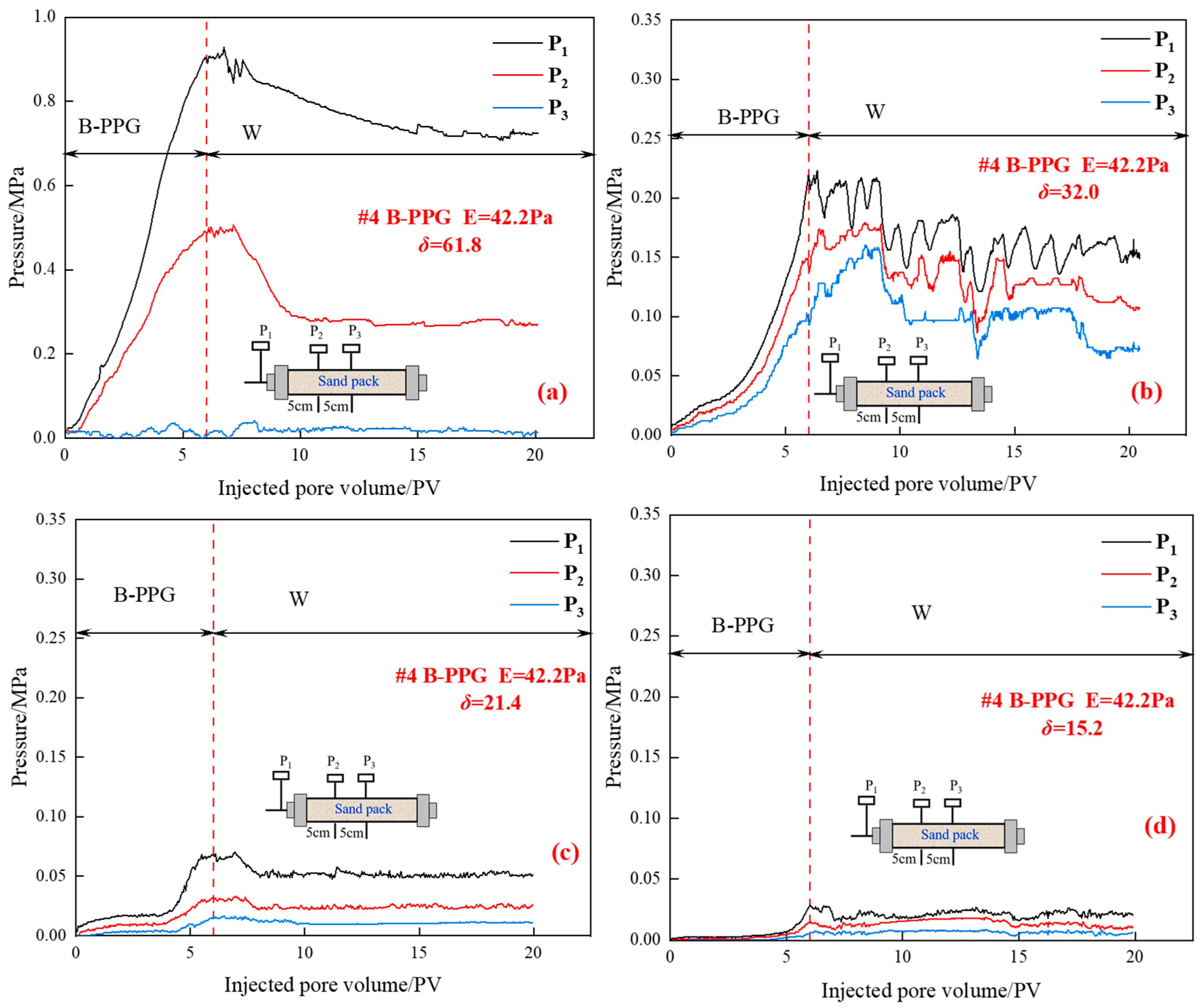
2.4.2. Effect of Matching Coefficient
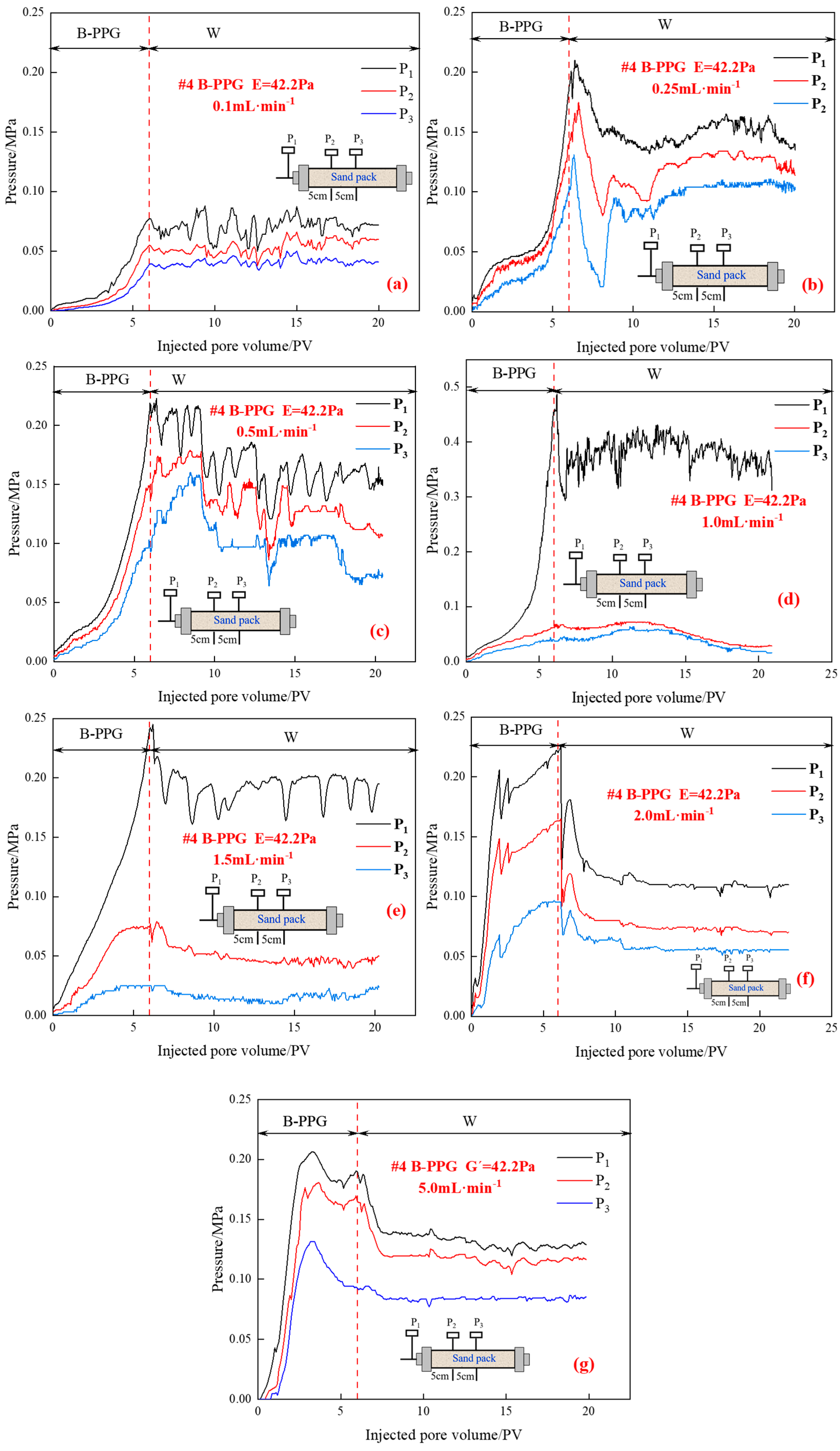
2.4.3. Effect of Injection Rate
3. Conclusions
4. Materials and Methods
4.1. Experimental Materials
4.2. Experimental Methods
4.2.1. Measurement of Swelling Ratio
4.2.2. Measurement of Particle Size Distribution Characteristics
4.2.3. Fourier Transform Infrared Characterization
4.2.4. Evaluation of Transport Characteristics of B-PPG
4.2.5. Calculation Method of Matching Coefficient Between B-PPG and Core Pore Throat
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Liu, H.; Pei, X.; Luo, K.; Sun, F.; Zheng, L.; Yang, Q. Current Status and Trend of Separated Layer Water Flooding in China. Pet. Explor. Dev. 2013, 40, 785–790. [Google Scholar] [CrossRef]
- Vledder, P.; Gonzalez, I.E.; Fonseca, J.C.C.; Wells, T.; Ligthelm, D.J. Low Salinity Water Flooding: Proof of Wettability Alteration on a Field Wide Scale, Paper SPE–129564-MS. In Proceedings of the SPE Improved Oil Recovery Symposium, Tulsa, OK, USA, 24–28 April 2010. [Google Scholar] [CrossRef]
- Mandal, A. Chemical Flood Enhanced Oil Recovery: A review. Int. J. Oil Gas Coal Technol. 2015, 9, 241. [Google Scholar] [CrossRef]
- Song, Z.; Li, Z.; Lai, F.; Liu, G.; Gan, H. Derivation of Water Flooding Characteristic Curve for High Water-Cut Oilfields. Pet. Explor. Dev. 2013, 40, 216–223. [Google Scholar] [CrossRef]
- Ma, J.; Morozov, I. AVO Modeling of Pressure-Saturation Effects in Weyburn CO2 Sequestration. Lead. Edge 2010, 29, 178–183. [Google Scholar] [CrossRef]
- Katende, A.; Farad, S. A Critical Review of Low Salinity Water Flooding: Mechanism, Laboratory and Field Application. J. Mol. Liq. 2019, 278, 627–649. [Google Scholar] [CrossRef]
- Sheng, J.J.; Bernd, L.; Nasser, A. Status of Polymer-Flooding Technology. J. Can. Pet. Technol. 2015, 54, 116–126. [Google Scholar] [CrossRef]
- Liu, S.; Zhang, D.L.; Yan, W.; Puerto, M.; Hirasaki, G.J.; Miller, C.A. Favorable Attributes of Alkaline-Surfactant-Polymer Flooding. SPE J. 2008, 13, 5–16. [Google Scholar] [CrossRef]
- Zerpa, L.E.; Queipo, N.V.; Pintos, S.; Salager, J.-L. An Optimization Methodology of Alkaline–Surfactant–Polymer Flooding Processes Using Field Scale Numerical Simulation and Multiple Surrogates. J. Pet. Sci. Eng. 2005, 47, 197–208. [Google Scholar] [CrossRef]
- Delamaide, E.; Zaitoun, A.; Renard, G.; Tabary, R. Pelican Lake Field: First Successful Application of Polymer Flooding in a Heavy-Oil Reservoir. SPE Reserv. Eval. Eng. 2014, 17, 340–354. [Google Scholar] [CrossRef]
- Flaaten, A.K.; Nguyen, Q.P.; Pope, G.A.; Zhang, J. A Systematic Laboratory Approach to Low-Cost, High-Performance Chemical Flooding. SPE Reserv. Eval. Eng. 2009, 12, 713–723. [Google Scholar] [CrossRef]
- Zhang, H.; Dong, M.; Zhao, S. Which One Is More Important in Chemical Flooding for Enhanced Court Heavy Oil Recovery, Lowering Interfacial Tension or Reducing Water Mobility? Energy Fuels 2010, 24, 1829–1836. [Google Scholar] [CrossRef]
- Zhou, K.; Wu, D.; An, Z.; Liu, S. Selective Penetration and Profile Control Performance of Preformed Particle Gels for Heterogeneous Oil Reservoirs. Gels 2022, 8, 601. [Google Scholar] [CrossRef] [PubMed]
- Raffa, P.; Broekhuis, A.A.; Picchioni, F. Polymeric Surfactants for Enhanced Oil Recovery: A review. J. Pet. Sci. Eng. 2016, 145, 723–733. [Google Scholar] [CrossRef]
- Sakthivel, S.; Gardas, R.L.; Sangwai, J.S. Effect of Alkyl Ammonium Ionic Liquids on the Interfacial Tension of the Crude Oil–Water System and Their Use for the Enhanced Oil Recovery Using Ionic Liquid-Polymer Flooding. Energy Fuels 2016, 30, 2514–2523. [Google Scholar] [CrossRef]
- Yelemessova, G.; Gussenov, I.; Ayazbayeva, A.; Shakhvorostov, A.; Orazzhanova, L.; Klivenko, A.; Kudaibergenov, S. Preparation and Characterization of Preformed Polyelectrolyte and Polyampholyte Gel Particles for Plugging of High-Permeability Porous Media. Gels 2024, 10, 562. [Google Scholar] [CrossRef]
- Gudiña, E.J.; Pereira, J.F.; Costa, R.; Coutinho, J.A.; Teixeira, J.A.; Rodrigues, L.R. Biosurfactant-Producing and Oil-Degrading Bacillus Subtilis Strains Enhance Oil Recovery in Laboratory Sand-Pack Columns. J. Hazard. Mater. 2013, 261, 106–113. [Google Scholar] [CrossRef] [PubMed]
- You, Q.; Wang, H.; Zhang, Y.; Liu, Y.; Fang, J.; Dai, C. Experimental Study on Spontaneous Imbibition of Recycled Fracturing Flow-Back Fluid to Enhance Oil Recovery in Low Permeability Sandstone Reservoirs. J. Pet. Sci. Eng. 2018, 166, 375–380. [Google Scholar] [CrossRef]
- Zhou, X.; Yuan, Q.; Rui, Z.; Wang, H.; Feng, J.; Zhang, L.; Zeng, F. Feasibility Study of CO2 Huff ‘n’ Puff Process to Enhance Heavy Oil Recovery via Long Core Experiments. Appl. Energy 2019, 236, 526–539. [Google Scholar] [CrossRef]
- Chu, Y.; Fan, C.; Zhang, Q.; Zan, C.; Ma, D.; Jiang, H.; Wang, Y.; Wei, F. The Oxidation of Heavy Oil to Enhance Oil Recovery: The Numerical Model and the Criteria to Describe the Low and High Temperature Oxidation. Chem. Eng. J. 2014, 248, 422–429. [Google Scholar] [CrossRef]
- Bao, M.; Kong, X.; Jiang, G.; Wang, X.; Li, X. Laboratory Study ON Activating Indigenous Microorganisms TO Enhance Oil Recovery IN Shengli Oilfield. J. Pet. Sci. Eng. 2009, 66, 42–46. [Google Scholar] [CrossRef]
- Gou, S.; Yin, T.; Ye, Z.; Jiang, W.; Yang, C.; Ma, Y.; Feng, M.; Xia, Q. High-Temperature Resistance Water-Soluble Copolymer Derived from Acrylamide, DMDAAC, and Functionalized Sul-fonamide for Potential Application in Enhance Oil Recovery. J. Appl. Polym. Sci. 2014, 131, 40727. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, Y.; Xue, F.; Wang, Y.; Ren, B.; Zhang, L.; Ren, S. CO2 Foam Flooding for Improved Oil Recovery: Reservoir Simulation Models and Influencing Factors. J. Pet. Sci. Eng. 2015, 133, 838–850. [Google Scholar] [CrossRef]
- Zhou, W.; Xin, C.; Chen, S.; Yu, Q.; Wang, K. Polymer-Enhanced Foam Flooding for Improving Heavy Oil Recovery in Thin Reservoirs. Energy Fuels 2020, 34, 4116–4128. [Google Scholar] [CrossRef]
- Li, S.; Wang, Q.; Zhang, K.; Li, Z. Monitoring of CO2 and CO2 Oil-Based Foam Flooding Processes In Fractured Low-Permeability Cores Using Nuclear Magnetic Resonance (Nmr). Fuel 2020, 263, 153–159. [Google Scholar] [CrossRef]
- Xu, X.; Saeedi, A.; Liu, K. Laboratory Studies on CO2 Foam Flooding Enhanced by a Novel Amphiphilic Ter-Polymer. J. Pet. Sci. Eng. 2016, 138, 153–159. [Google Scholar] [CrossRef]
- Moosavi, S.R.; Wood, D.A.; Ahmadi, M.A.; Choubineh, A. ANN-Based Prediction of Laboratory-Scale Performance of CO2-Foam Flooding for Improving Oil Recovery. Nat. Resour. Res. 2019, 28, 1619–1637. [Google Scholar] [CrossRef]
- Zhang, H.; Bai, B. Preformed particle gel transport through open fractures and its effect on water flow. SPE J. 2011, 16, 388–400. [Google Scholar] [CrossRef]
- Imqam, A.; Bai, B.; Al Ramadan, M.; Wei, M.; Delshad, M.; Sepehrnoori, K. Preformed-Particle-Gel Extrusion Through Open Conduits During Conformance-Control Treatments. SPE J. 2015, 20, 1083–1093. [Google Scholar] [CrossRef]
- Ma, W.; Li, Y.; Liu, P.; Liu, Z.; Song, T. Progress of Research into Preformed Particle Gels for Profile Control and Water Shutoff Techniques. Gels 2024, 10, 372. [Google Scholar] [CrossRef]
- Almakimi, A.; Ben Ali, A.; Hussein, I.A.; Bai, B. Evaluation of Novel Preformed Particle Gel System for Conformance Control in Mature Oil Reservoirs. Gels 2024, 10, 70. [Google Scholar] [CrossRef]
- Chen, X.; Feng, Q.; Liu, W.; Sepehrnoori, K. Modeling Preformed Particle Gel Surfactant Combined Flooding for Enhanced Oil Recovery After Polymer Flooding. Fuel 2017, 194, 42–49. [Google Scholar] [CrossRef]
- Gong, H.; Zhang, H.; Xu, L.; Li, K.; Yu, L.; Li, Y.; Dong, M. Further Enhanced Oil Recovery by Branched-Preformed Particle Gel/Hpam/Surfactant Mixed Solutions After Polymer Flooding in Parallel-Sandpack Models. RSC Adv. 2017, 7, 39564–39575. [Google Scholar] [CrossRef]
- Xu, L.; Qiu, Z.; Gong, H.; Zhu, C.; Sang, Q.; Li, Y.; Dong, M. Synergy OF Microbial Polysaccharides and Branched-Preformed Particle Gel on Thickening and Enhanced Oil Recovery. Chem. Eng. Sci. 2019, 208, 115138. [Google Scholar] [CrossRef]
- He, H.; Fu, J.; Hou, B.; Yuan, F.; Guo, L.; Li, Z.; You, Q. Investigation of Injection Strategy of Branched-Preformed Particle Gel/Polymer/Surfactant for Enhanced Oil Recovery after Polymer Flooding in Heterogeneous Reservoirs. Energies 2018, 11, 1950. [Google Scholar] [CrossRef]
- Dai, C.; Liu, Y.; Zou, C.; You, Q.; Yang, S.; Zhao, M.; Zhao, G.; Wu, Y.; Sun, Y. Investigation on matching relationship between dispersed particle gel (DPG) and reservoir pore-throats for in-depth profile control. Fuel 2017, 207, 109–120. [Google Scholar] [CrossRef]
- Huang, B.; Zhang, W.; Xu, R.; Shi, Z.; Fu, C.; Wang, Y.; Song, K. A Study on the Matching Relationship of Polymer Molecular Weight and Reservoir Permeability in ASP Flooding for Duanxi Reservoirs in Daqing Oil Field. Energies 2017, 10, 951. [Google Scholar] [CrossRef]
- Zhang, X.; Zhang, Y.; Liu, H.; Li, S.; Liu, L. Dynamic Sweep Experiments on a Heterogeneous Phase Composite System Based on Branched-Preformed Particle Gel in High Water-Cut Reservoirs after Polymer Flooding. Gels 2023, 9, 364. [Google Scholar] [CrossRef]
- He, H.; Tian, Y.; Zhang, L.; Li, H.; Guo, Y.; Liu, Y.; Liu, Y. Insights into the Injectivity and Propagation Behavior of Preformed Particle Gel (PPG) in a Low–Medium-Permeability Reservoir. Gels 2024, 10, 475. [Google Scholar] [CrossRef]
- Zhao, Z. Study on the Flow Law of B-PPG in Porous Media. Master’s Thesis, China University of Petroleum (East China), Dongying, China, 2016. [Google Scholar]
- Li, J.; Jiang, Z.; Wang, Y.; Zheng, J.; Huang, G. Stability, Seepage and Displacement Characteristics of Heterogeneous Branched-Preformed Particle Gels for Enhanced Oil Recovery. RSC Adv. 2018, 8, 4881–4889. [Google Scholar] [CrossRef]
- Liu, Y.G.; Ding, M.C.; Han, Y.G.; Wang, Y.F.; Zou, J.; Zhao, P. Migration and Profile Control Properties Of B-Ppg in Oil Reservoirs. Pet. Drill. Prod. Technol. 2018, 40, 393–399. [Google Scholar]
- Gong, H.; Zhang, H.; Xu, L.; Li, K.; Yu, L.; San, Q.; Li, Y.; Dong, M. The Synergistic Effect of Branched-Preformed Particle Gel and Hydrolyzed Polyacrylamide on Further-Enhanced Oil Recovery after Polymer Flooding. Energy Fuels 2017, 31, 7904–7910. [Google Scholar] [CrossRef]
- Liu, W.; He, H. Factors of Affecting Flow Behavior of Heterogeneous Oil Displacement System in Porous Media. J. Xi’an Pet. Univ. Nat. Sci. Ed. 2022, 37, 32–38. [Google Scholar]
- Tang, K.; Gao, Y.; Zhao, Y.; Xie, H.; Du, J.; Yuan, F.; Zhu, D. Conformance Control and Improved Oil Recovery Mechanism of Branched Preformed Particle Gel in Fractured Reservoirs Utilizing Nuclear Magnetic Resonance Technology. Energy Fuels 2025, 39, 1570–1578. [Google Scholar] [CrossRef]
- Li, Z.; Wang, Y.; He, H.; Yuan, F.; Liu, H.; Chen, Y. Insights into the Effects of Salinity on the Transport Behavior of Polymer-Enhanced Branched-Preformed Particle Gel Suspension in Porous Media. Energy Fuels 2021, 35, 1104–1112. [Google Scholar] [CrossRef]
- Farasat, A.; Sefti, M.V.; Sadeghnejad, S.; Saghafi, H.R. Mechanical entrapment analysis of enhanced preformed particle gels (PPGs) in mature reservoirs. J. Pet. Sci. Eng. 2017, 157, 441–450. [Google Scholar] [CrossRef]
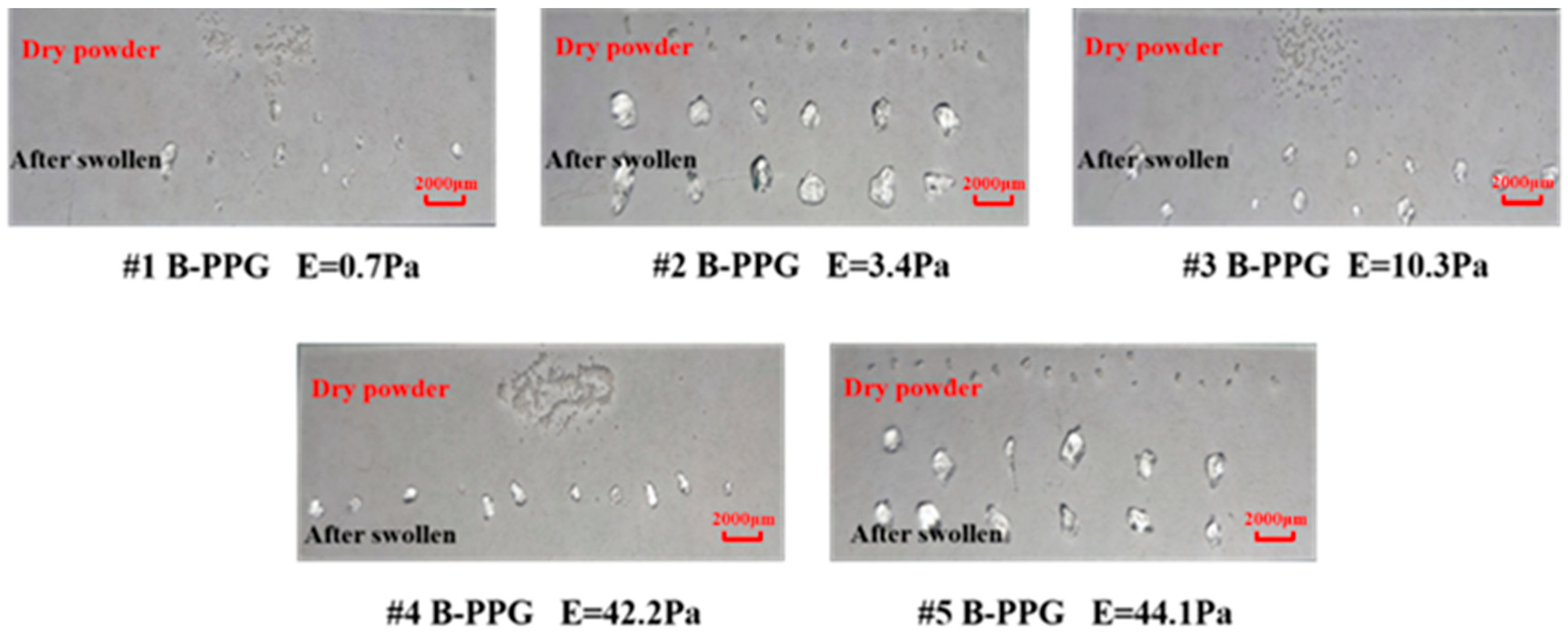

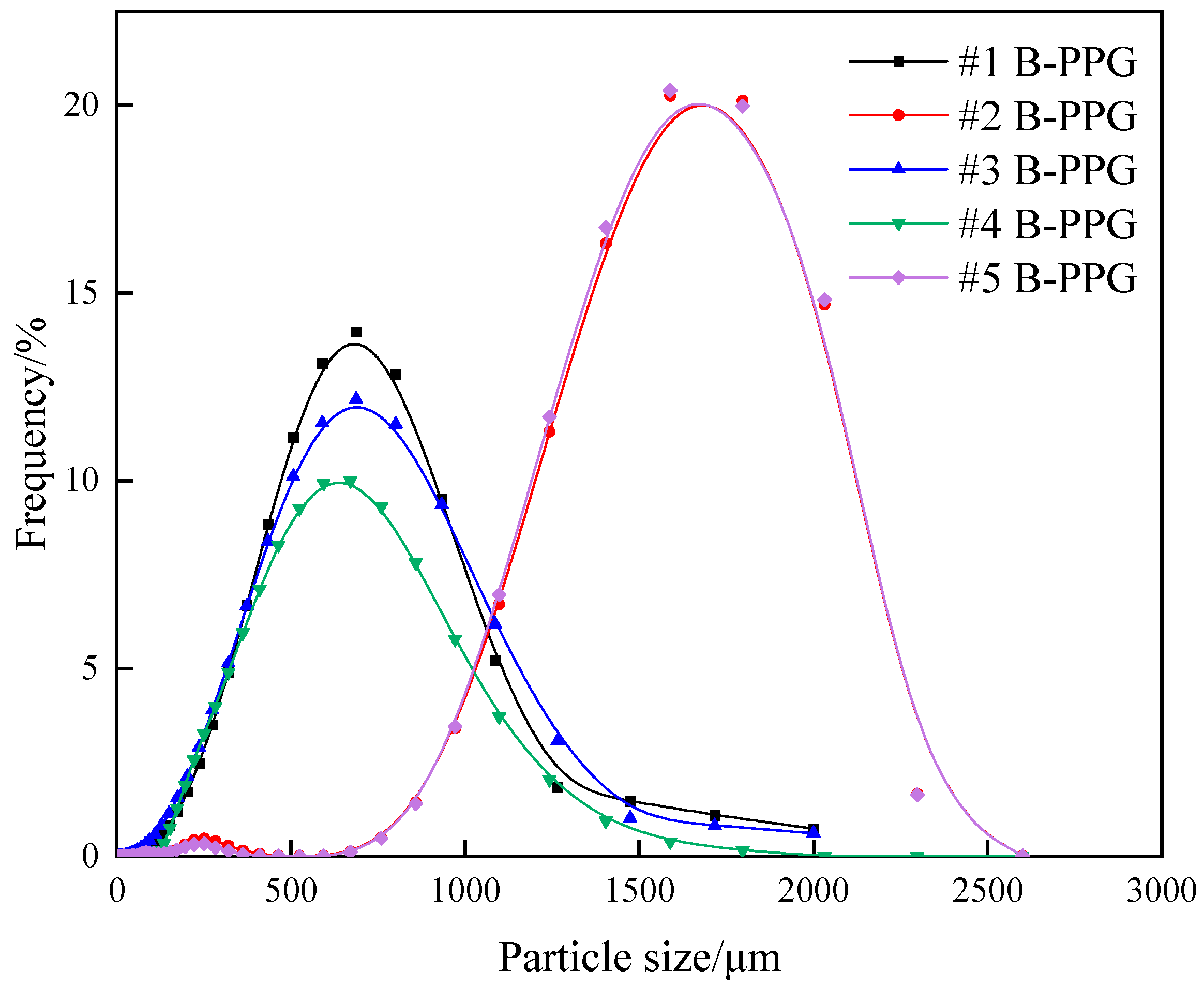
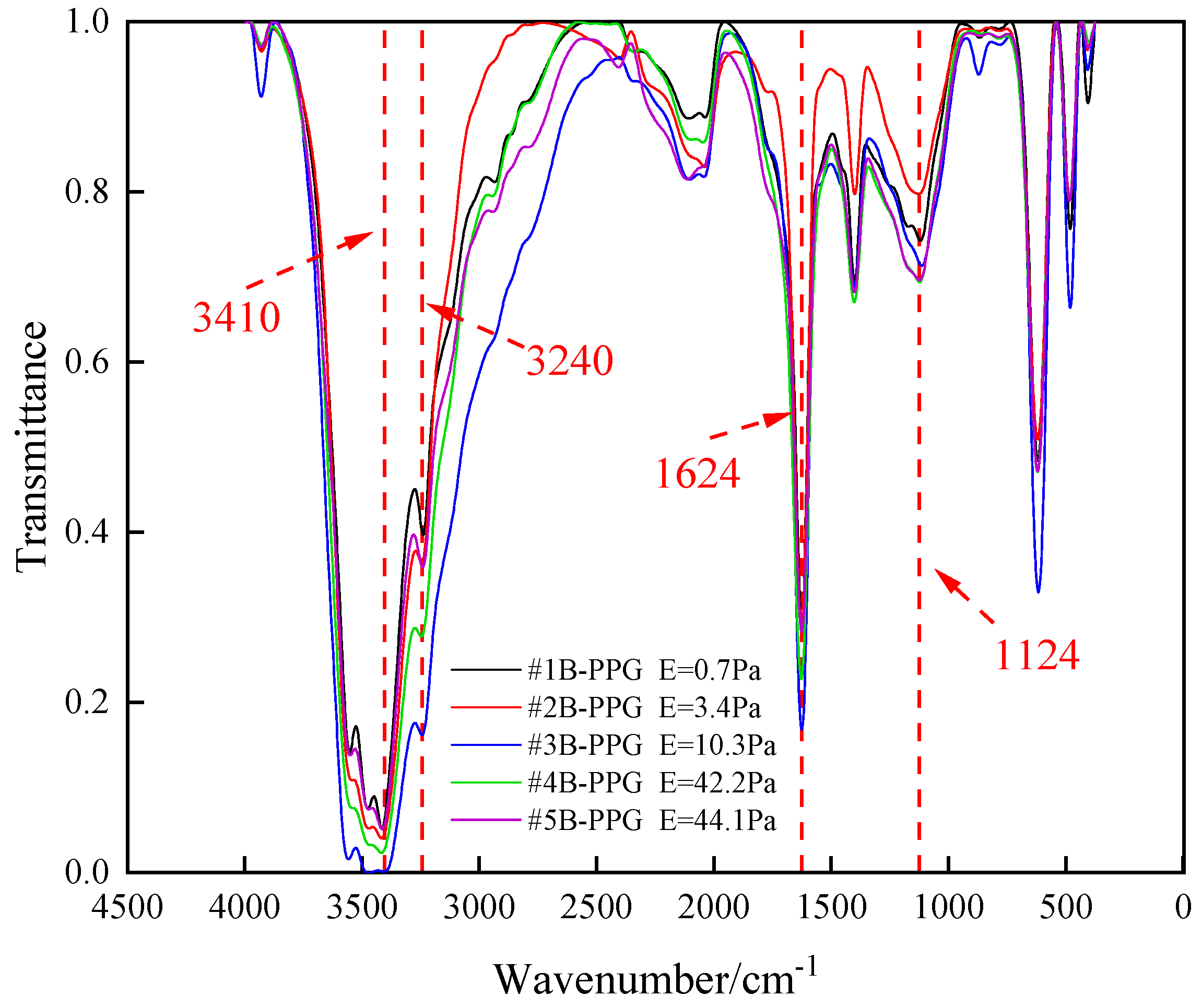



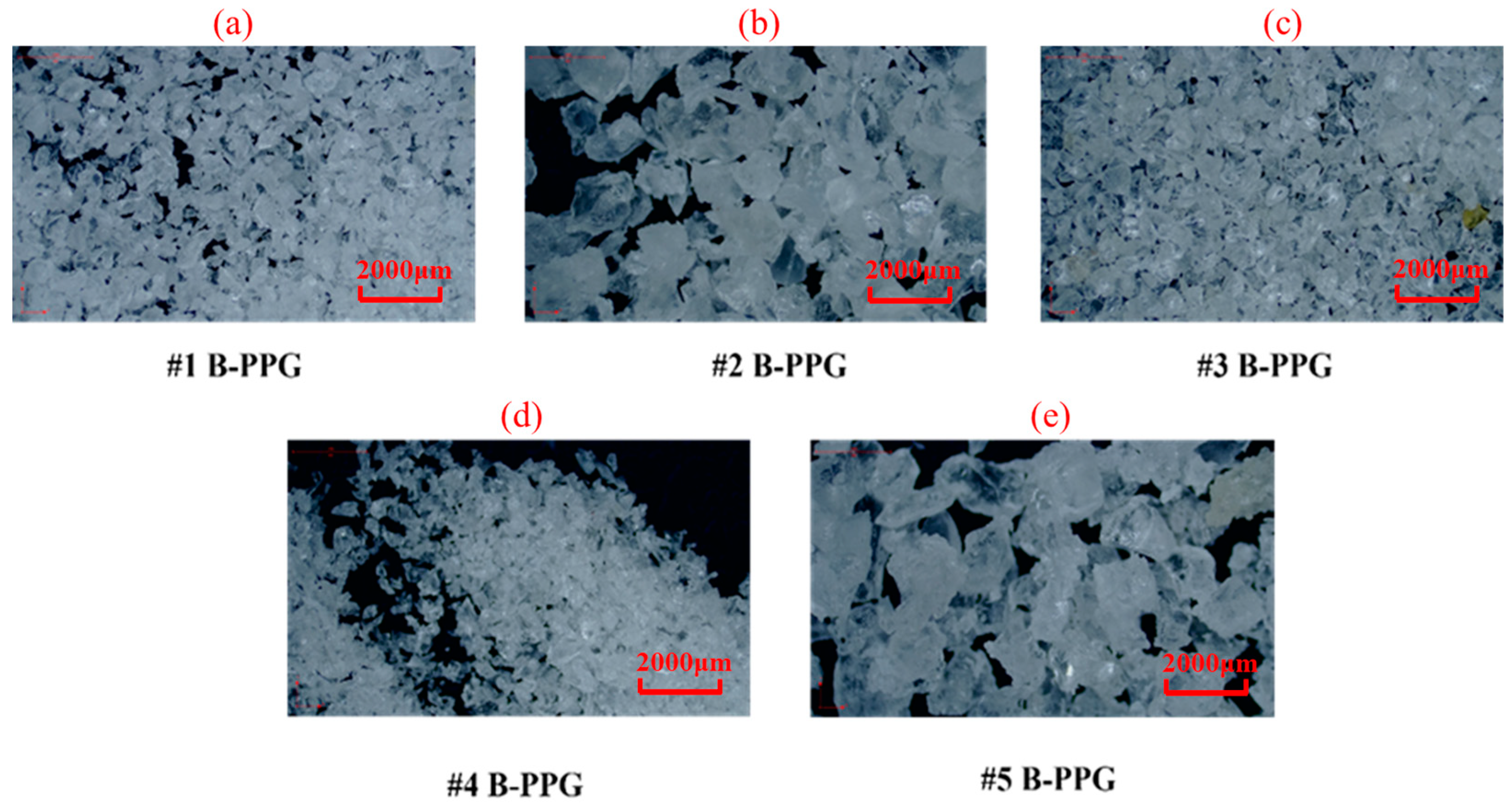

| B-PPG | Elastic Modulus/Pa | Particle Size | ||
|---|---|---|---|---|
| D10/μm | D50/μm | D90/μm | ||
| #1 | 0.7 | 259 | 550 | 889 |
| #2 | 3.4 | 992 | 1465 | 1898 |
| #3 | 10.3 | 228 | 542 | 942 |
| #4 | 42.2 | 247 | 525 | 917 |
| #5 | 44.1 | 1013 | 1466 | 1856 |
| No. | B-PPG | Elastic Modulus /Pa | Particle Size D50/μm | Injection Rate /mL·min−1 | Permeability /μm2 | Porosity /% |
|---|---|---|---|---|---|---|
| 1 | #1 | 0.7 | 550 | 0.5 | 1.20 | 30.8 |
| 2 | #2 | 3.4 | 1465 | 1.20 | 31.1 | |
| 3 | #3 | 10.3 | 542 | 1.00 | 30.6 | |
| 4 | #4 | 42.2 | 525 | 1.18 | 31.6 | |
| 5 | #5 | 44.1 | 1466 | 1.08 | 30.4 |
| No. | Elastic Modulus /Pa | D50 /μm | Permeability /μm2 | ∆Pwa /MPa | ∆PB-PPG /MPa | ∆Pwb /MPa | Fr | Frr |
|---|---|---|---|---|---|---|---|---|
| 1 | 0.7 | 550 | 1.20 | 0.0023 | 0.042 | 0.037 | 18.3 | 16.1 |
| 2 | 3.4 | 1465 | 1.20 | 0.0023 | 0.113 | 0.057 | 49.1 | 24.8 |
| 3 | 10.3 | 542 | 1.00 | 0.0030 | 0.188 | 0.146 | 62.7 | 48.7 |
| 4 | 42.2 | 525 | 1.18 | 0.0024 | 0.215 | 0.152 | 93.5 | 66.1 |
| 5 | 44.1 | 1466 | 1.08 | 0.0025 | 0.276 | 0.268 | 110.4 | 107.2 |
| No. | Elastic Modulus /Pa | D50 /μm | Injection Rate /mL·min−1 | Permeability /μm2 | Porosity /% | Dp /μm | Matching Coefficient δ |
|---|---|---|---|---|---|---|---|
| 6 | 42.2 Pa | 525 | 0.5 | 0.30 | 30.2 | 8.5 | 61.8 |
| 7 | 1.18 | 31.6 | 16.4 | 32.0 | |||
| 8 | 2.70 | 32.4 | 24.5 | 21.4 | |||
| 9 | 5.30 | 32.1 | 34.5 | 15.2 |
| No. | D50 /μm | Permeability /μm2 | Dp /μm | δ | ∆Pwa /MPa | ∆PB-PPG /MPa | ∆Pwb /MPa | Fr | Frr |
|---|---|---|---|---|---|---|---|---|---|
| 6 | 525 | 0.30 | 8.5 | 61.8 | 0.0090 | 0.910 | 0.724 | 101.1 | 80.4 |
| 7 | 1.18 | 16.4 | 32.0 | 0.0024 | 0.215 | 0.152 | 93.5 | 66.1 | |
| 8 | 2.70 | 24.5 | 21.4 | 0.0010 | 0.068 | 0.051 | 68.0 | 51.0 | |
| 9 | 5.30 | 34.5 | 15.2 | 0.0005 | 0.029 | 0.020 | 58.0 | 40.0 |
| No. | D50 /μm | Elastic Modulus /Pa | Injection Rate /mL·min−1 | Permeability /μm2 | Porosity /% |
|---|---|---|---|---|---|
| 10 | 525 | 42.2 | 0.25 | 0.95 | 31.2 |
| 11 | 0.50 | 1.18 | 31.6 | ||
| 12 | 1.00 | 0.92 | 30.8 | ||
| 13 | 1.50 | 1.07 | 32.1 | ||
| 14 | 2.00 | 0.95 | 31.0 |
| No. | Injection Rate /mL·min−1 | Permeability /μm2 | ∆Pwa /MPa | ∆PB-PPG /MPa | ∆Pwb /MPa | Fr | Frr |
|---|---|---|---|---|---|---|---|
| 10 | 0.10 | 1.08 | 0.00051 | 0.077 | 0.072 | 151.0 | 141.2 |
| 11 | 0.25 | 0.95 | 0.00145 | 0.184 | 0.137 | 126.9 | 94.5 |
| 12 | 0.50 | 1.18 | 0.00240 | 0.215 | 0.152 | 93.5 | 66.1 |
| 13 | 1.00 | 0.92 | 0.00590 | 0.450 | 0.358 | 76.3 | 60.7 |
| 14 | 1.50 | 1.07 | 0.00750 | 0.241 | 0.186 | 32.1 | 24.8 |
| 15 | 2.00 | 0.95 | 0.01150 | 0.220 | 0.108 | 19.1 | 9.4 |
| 16 | 5.00 | 1.01 | 0.02700 | 0.190 | 0.129 | 7.0 | 4.8 |
| No. | #1 | #2 | #3 | #4 | #5 |
|---|---|---|---|---|---|
| Elastic modulus/Pa | 0.7 | 3.4 | 10.3 | 42.2 | 44.1 |
| Type of Ions | Na+ | Ca2+ | Mg2+ | Cl− |
|---|---|---|---|---|
| Concentration of ions/(mg·L−1) | 7466.15 | 428 | 255.7 | 13,040.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, R.; He, H.; Tian, Y.; Xiong, Z.; Ke, W.; Pei, H.; Zhang, P. Transport Characteristics of Branched-Preformed Particle Gel in Porous Media: Influence of Elastic Modulus, Matching Coefficient, and Injection Rate. Gels 2025, 11, 315. https://doi.org/10.3390/gels11050315
Chen R, He H, Tian Y, Xiong Z, Ke W, Pei H, Zhang P. Transport Characteristics of Branched-Preformed Particle Gel in Porous Media: Influence of Elastic Modulus, Matching Coefficient, and Injection Rate. Gels. 2025; 11(5):315. https://doi.org/10.3390/gels11050315
Chicago/Turabian StyleChen, Ruping, Hong He, Yuhang Tian, Zixiang Xiong, Wenli Ke, Haihua Pei, and Peng Zhang. 2025. "Transport Characteristics of Branched-Preformed Particle Gel in Porous Media: Influence of Elastic Modulus, Matching Coefficient, and Injection Rate" Gels 11, no. 5: 315. https://doi.org/10.3390/gels11050315
APA StyleChen, R., He, H., Tian, Y., Xiong, Z., Ke, W., Pei, H., & Zhang, P. (2025). Transport Characteristics of Branched-Preformed Particle Gel in Porous Media: Influence of Elastic Modulus, Matching Coefficient, and Injection Rate. Gels, 11(5), 315. https://doi.org/10.3390/gels11050315






