Analysis of Pressure Distribution in Transfemoral Prosthetic Socket for Prefabrication Evaluation via the Finite Element Method
Abstract
1. Introduction
2. Materials and Methods
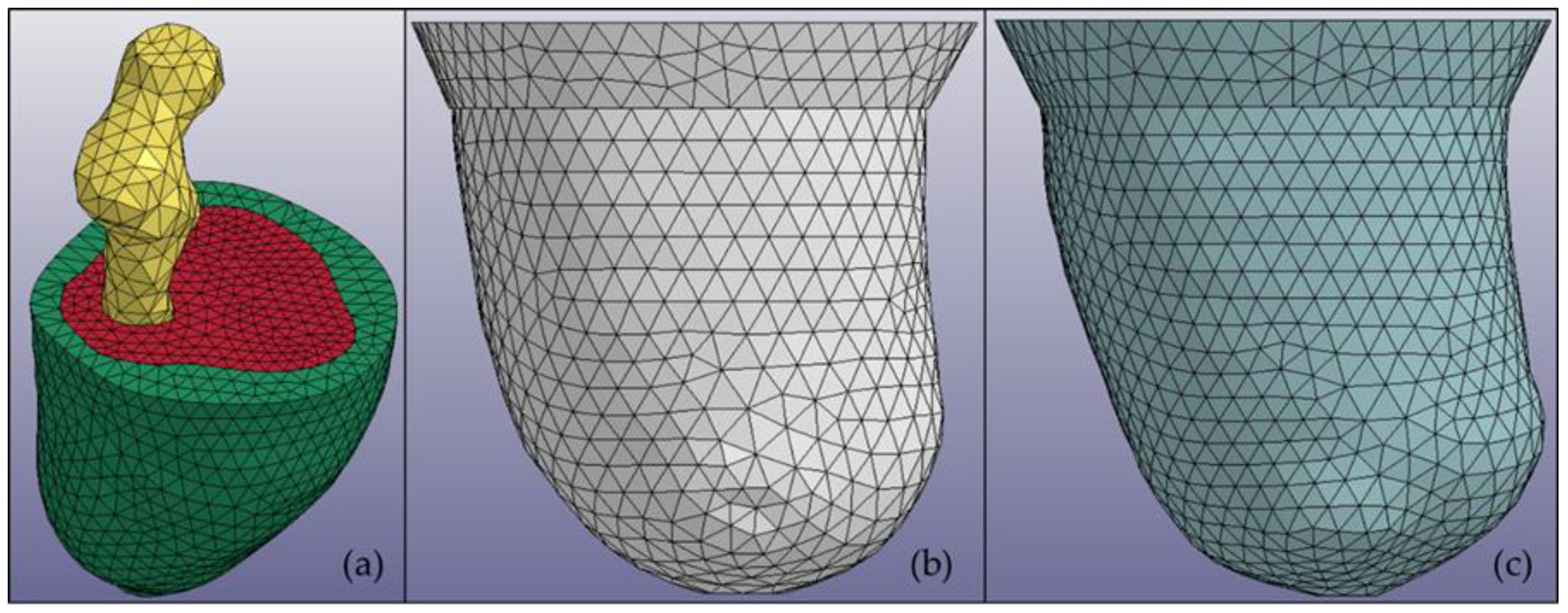
2.1. Residuum and Socket Model Construction
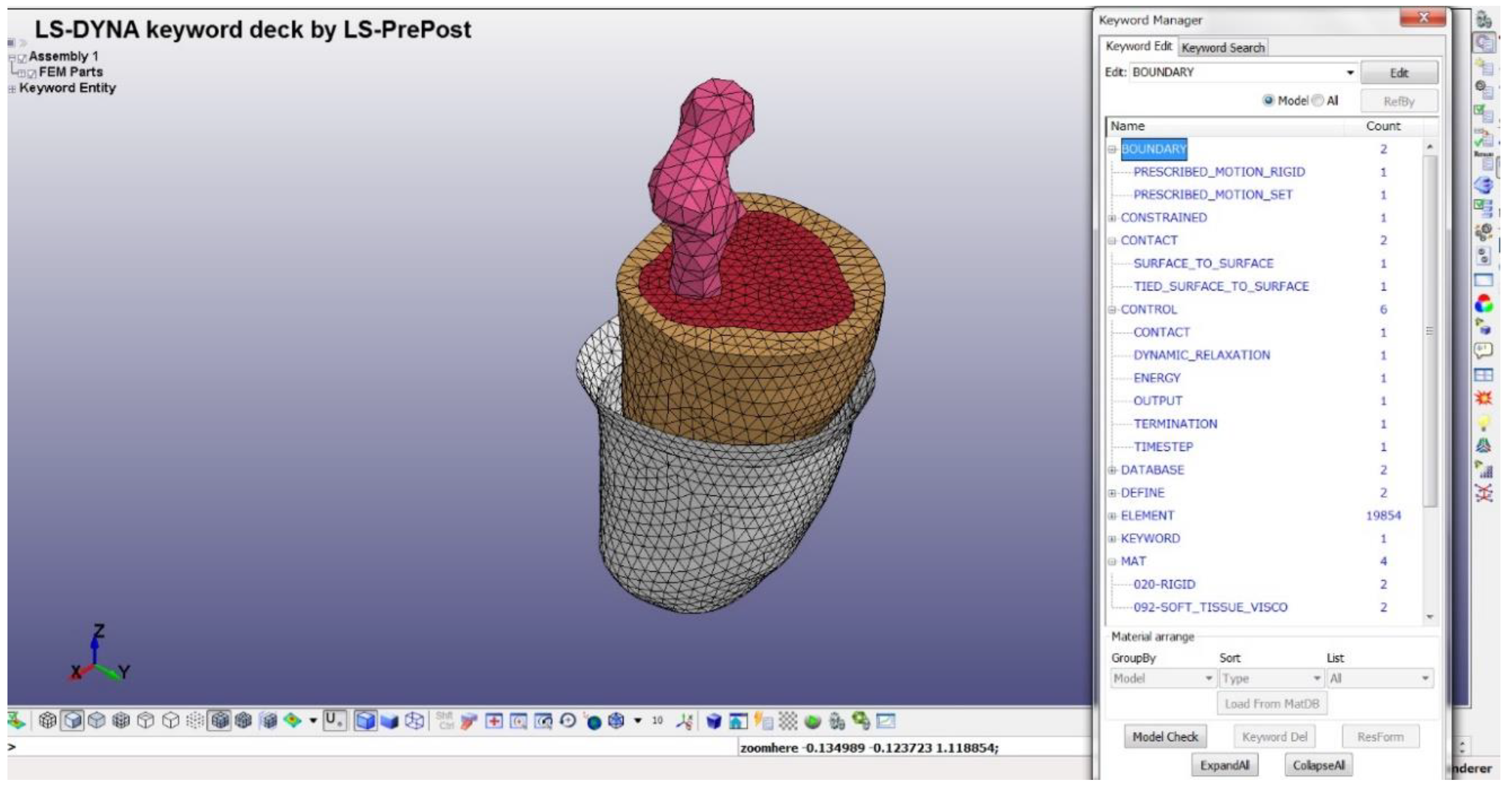
2.2. Predetermined Environment for Simulation
2.3. Experiment Analysis
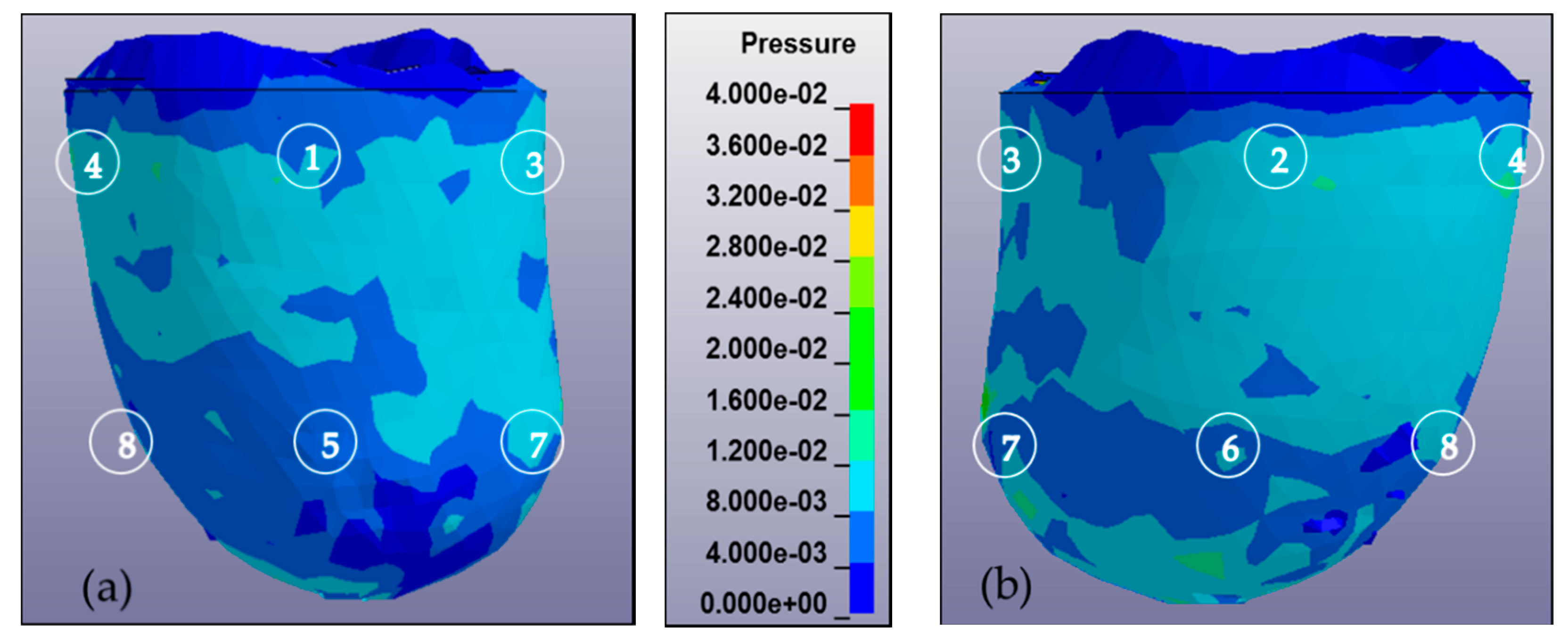
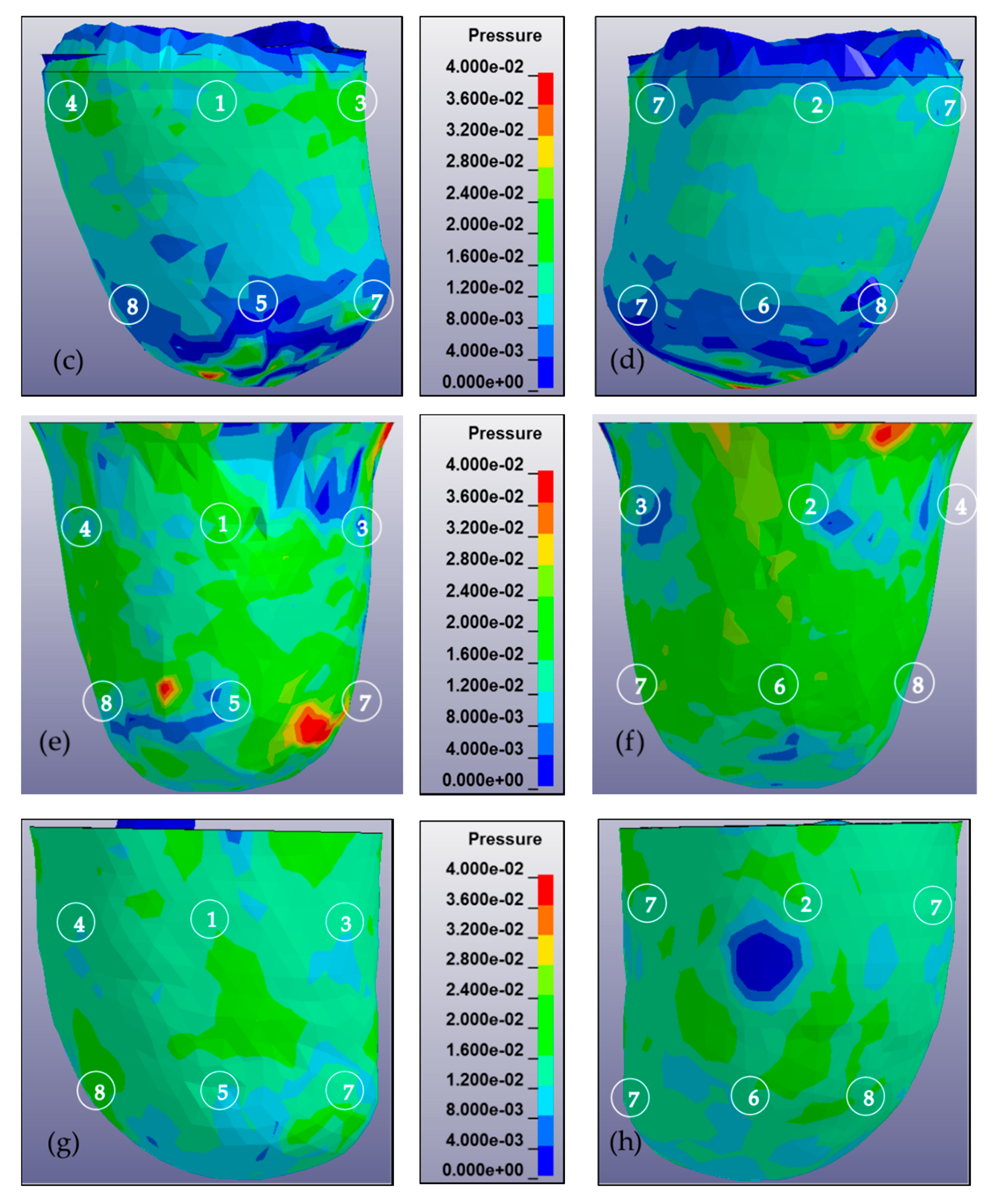
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Zhang, M.; Lord, M.; Turner-Smith, A.R.; Robert, V.C. Development of a non-linear finite element modelling of the below-knee prosthetic socket interface. Med. Eng. Phys. 1995, 17, 559–566. [Google Scholar] [CrossRef]
- Sugimoto, A. Characteristic of Stump Shape and Stress during Gait Cycle for Transfemoral Prosthesis 345 Socket. Master’s Thesis, Shibaura Institute of Technology, Tokyo, Japan, 2013. [Google Scholar]
- Mak, A.F.; Zhang, M.; Boone, D.A. State-of-the-art research in lower-limb prosthetic biomechanics socket interface. J. Rehabil. Res. Dev. 2001, 38, 161174. [Google Scholar]
- Childress, D.S.; Silver-Thorn, M.B.; Steege, J.W. A review of prosthetic interface stress investigations. J. Rehabil. Res. Dev. 1996, 33, 253266. [Google Scholar]
- Sanders, J.E.; Zachariah, S.G. Interface mechanics in lower-limb external prosthetics: A review of finite element models. IEEE Trans. Rehabil. Eng. 1996, 4, 288302. [Google Scholar]
- Zhang, M.; Mak, A.F.; Roberts, V.C. Finite element modeling of a residual lower-limb in a prosthetic socket: A survey of the development in the first decade. Med. Eng. Phys. 1998, 20, 360273. [Google Scholar] [CrossRef]
- Sengeh, D.M.; Moerman, K.M.; Petron, A.; Herr, H. Multi-material 3-D viscoelastic model of a transtibial residuum from in-vivo indentation and MRI data. J. Mech. Behav. Biomed. Mater. 2016, 59, 379–392. [Google Scholar] [CrossRef] [PubMed]
- Portnoy, S.; Yarnitzky, G.; Yizhar, Z.; Kristal, A.; Oppenheim, U.; Siev-Ner, I.; Gefen, A. Real-time patient-specific finite element analysis of internal stresses in the soft tissues of a residual limb: A new tool for prosthetic fitting. Ann. Biomed. Eng. 2007, 35, 120–135. [Google Scholar] [CrossRef] [PubMed]
- Colombo, G.; Rizzi, C.; Regazzoni, D.; Vitali, A. 3D interactive environment for the design of medical devices. Int. J. Interact. Des. Manuf. (IJIDeM) 2018, 12, 699–715. [Google Scholar] [CrossRef]
- Schuch, C.M. Report From: International Workshop on Above-Knee Fitting and Alignment Techniques. Clin. Prosthet. Orthot. 1988, 12, 81–98. [Google Scholar]
- Jamaludin, M.S.; Hanafusa, A.; Yamamoto, S.I.; Agarie, Y.; Otsuka, H.; Onishi, K. Evaluation of the effect of the geometrical changes in prosthetic socket towards transfemoral residuum via finite element method. In Proceedings of the IEEE–EMBS Conference on Biomedical Engineering and Science (IECBES), Sarawak, Malaysia, 3–6 December 2018. [Google Scholar]
- Portnoy, S.; Siev-Ner, I.; Shabshin, N.; Kristal, A.; Yizhar, Z.; Gefen, A. Patient-specific analyses of deep tissue loads post transtibial amputation in residual limbs of multiple prosthetic users. J. Biomech. 2009, 42, 2686–2693. [Google Scholar] [CrossRef] [PubMed]
- Meulenbelt, H.E.J.; Dijkstra, P.U.; Jonkman, M.F.; Geertzen, J.H.B. Skin problems in lower limb amputees: A systematic review. Disabil. Rehabil. 2006, 28, 603–608. [Google Scholar] [CrossRef] [PubMed]
- Reynolds, D.P.; Lord, M. Interface load analysis for computer-aided design of below-knee prosthetic sockets. Med. Boil. Eng. 1992, 30, 419–426. [Google Scholar] [CrossRef] [PubMed]
- Silver-Thorn, M.B.; Childress, D.S. Parametric analysis using the finite element method to investigate prosthetic interface stresses for persons with trans-tibial amputation. J. Rehabil. Res. Dev. 1996, 33, 227–238. [Google Scholar] [PubMed]
- Weiss, J.A. A Constitutive Model and Finite Element Representation for Transversely Isotropic Soft Tissues. Ph.D. Thesis, University of Utah, Salt Lake City, UT, USA, 1994. [Google Scholar]
- Untaroiu, C.; Darvish, K.; Crandall, J.; Deng, B.; Wang, J. Development and validation of a finite element model of the lower limb. In Proceedings of the ASME 2004 International Mechanical Engineering Congress and Exposition Transportation: Transportation and Environment, Anaheim, CA, USA, 13–19 November 2004; pp. 53–62. [Google Scholar]
- Untaroiu, C.; Darvish, K.; Crandall, J.; Deng, B.; Wang, J. Characterization of the Lower Limb Soft Tissues in Pedestrian Finite Element Models. In Proceedings of the 19th International technical conference on the Enhanced Safety of Vehicles, Washington, DC, USA, 6–9 June 2005; pp. 124–131. [Google Scholar]
- Winson, A.B.D.C.L.; Ming, Z.; Bill, C. Finite element analysis to determine effect of mono limb flexibility on structural strength and interaction. J. Rehabil. Res. Dev. 2004, 41, 775–786. [Google Scholar]
- Ramrez, J.F.; Vlez, J.A. Incidence of the boundary condition between bone and soft tissue in a finite element model of a transfemoral amputee. Prosthet. Orthot. Int. 2012, 36, 405–414. [Google Scholar] [CrossRef] [PubMed]
- NETTA Corporation RETS Division Sensor Group. PFS Series. PD 3-32 User’s Manual; NETTA Corporation: Osaka, Japan, 2015. [Google Scholar]
- Sanders, J.E.; Fatone, S. Residual limb volume change: Systematic review of measurement and management. J. Rehabil. Res. Dev. 2011, 48, 949–986. [Google Scholar] [CrossRef] [PubMed]
- Ellankavi, R.; Okan, A.; Beate, D.; Sook-Yee, C.; Leonardo, G.; Günter, S.; Fritz, S.; Oliver, R. An Efficient Modelling-Simulation-Analysis Workflow to Investigate Stump-Socket Interaction Using Patient-Specific, Three-Dimensional, Continuum-Mechanical, Finite Element Residual Limb Models. Front. Bioeng. Biotechnol. 2018, 6, 126. [Google Scholar]
- Jamaludin, M.S.; Hanafusa, A. Accuracy Evaluation of 3D Reconstruction of Transfemoral Residual Limb Model Using Basic Spline Interpolation. IFMBE Proc. 2017, 68, 675–680. [Google Scholar]




| Part | Density (kg/m3) | C1 (kPa) | C2 (kPa) | S1 | S2 | T1 (ms) | T2 (ms) | K (MPa) |
|---|---|---|---|---|---|---|---|---|
| Skin | 906 | 0.186 | 0.178 | 0.968 | 0.864 | 10.43 | 84.1 | 20 |
| Fat | 906 | 0.19 | 0.18 | 1 | 0.9 | 10 | 84 | 20 |
| Muscle | 1051 | 0.12 | 0.25 | 1.2 | 0.8 | 23 | 63 | 20 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jamaludin, M.S.; Hanafusa, A.; Shinichirou, Y.; Agarie, Y.; Otsuka, H.; Ohnishi, K. Analysis of Pressure Distribution in Transfemoral Prosthetic Socket for Prefabrication Evaluation via the Finite Element Method. Bioengineering 2019, 6, 98. https://doi.org/10.3390/bioengineering6040098
Jamaludin MS, Hanafusa A, Shinichirou Y, Agarie Y, Otsuka H, Ohnishi K. Analysis of Pressure Distribution in Transfemoral Prosthetic Socket for Prefabrication Evaluation via the Finite Element Method. Bioengineering. 2019; 6(4):98. https://doi.org/10.3390/bioengineering6040098
Chicago/Turabian StyleJamaludin, Mohd Syahmi, Akihiko Hanafusa, Yamamoto Shinichirou, Yukio Agarie, Hiroshi Otsuka, and Kengo Ohnishi. 2019. "Analysis of Pressure Distribution in Transfemoral Prosthetic Socket for Prefabrication Evaluation via the Finite Element Method" Bioengineering 6, no. 4: 98. https://doi.org/10.3390/bioengineering6040098
APA StyleJamaludin, M. S., Hanafusa, A., Shinichirou, Y., Agarie, Y., Otsuka, H., & Ohnishi, K. (2019). Analysis of Pressure Distribution in Transfemoral Prosthetic Socket for Prefabrication Evaluation via the Finite Element Method. Bioengineering, 6(4), 98. https://doi.org/10.3390/bioengineering6040098





