Anatomical Features and Material Properties of Human Surrogate Head Models Affect Spatial and Temporal Brain Motion under Blunt Impact
Abstract
1. Introduction
2. Methods
2.1. Characterizing Brain Surrogate Stiffness (Exp. SBM)
2.2. Changing the Surrogate Skull Stiffness (Exp. SSS)
2.3. Creating a CSF Layer (Exp. CSF)
2.4. Creating Different Surrogate Head Sizes (Exp. SHS)
2.5. Creating Vasculature in SH (Exp. VASC)
2.6. Altering the Surrogate Neck Stiffness (Exp. NS)
2.7. Surrogate Skull Mechanical Properties
2.8. Head Impact Testing Parameters
2.9. Motion Tracking and Strain Calculations
2.10. Relating Surrogate Brain Deformation to Injury Risk
2.11. Statistical Analysis
3. Results
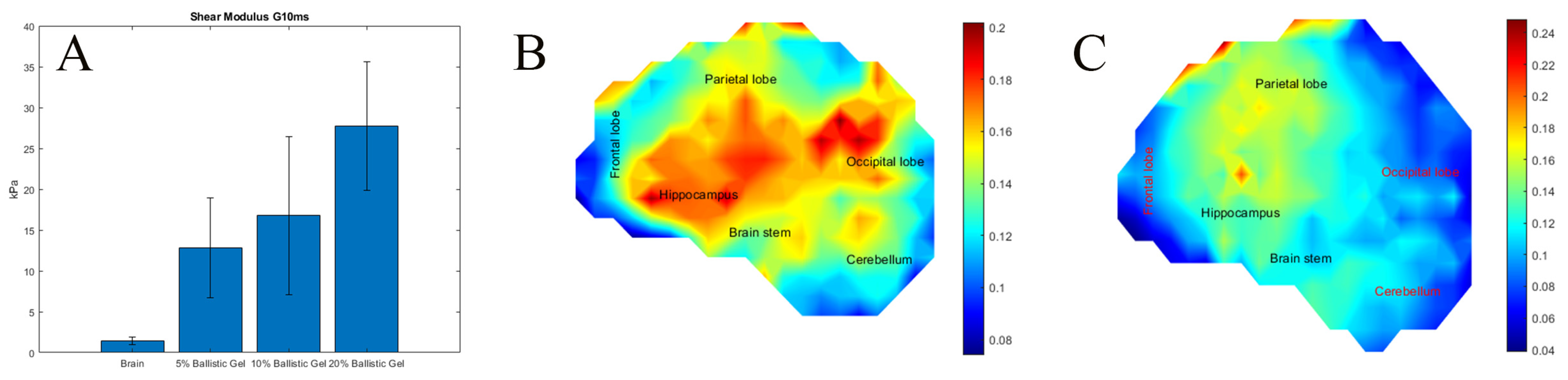
3.1. The Effects of Surrogate Brain Stiffness on the Development of Strain
3.2. The Effects of Skull Stiffness on the Spatial Development of Strain (Exp. SSS)
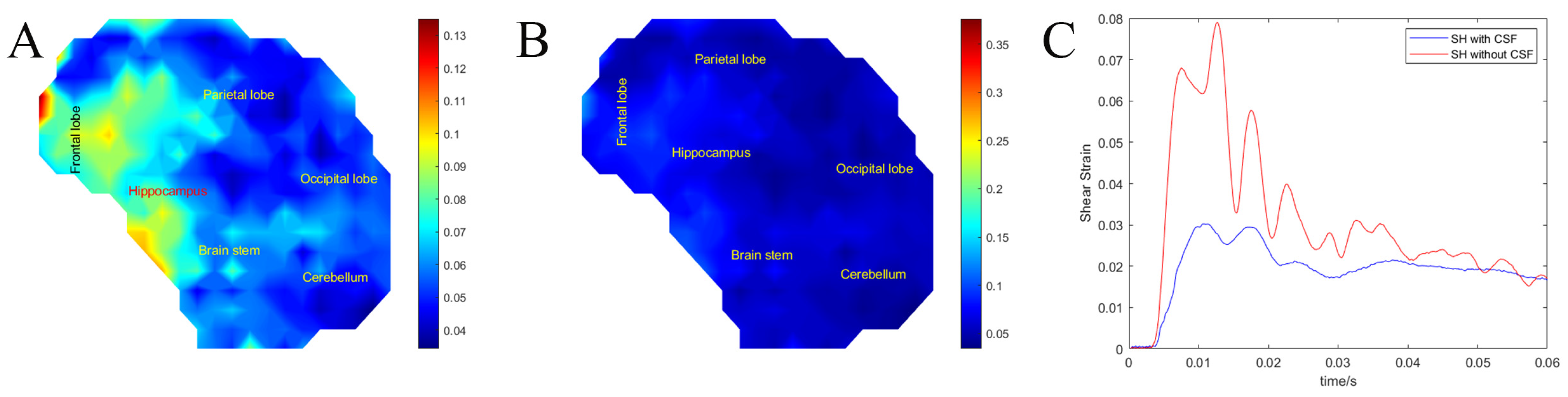
3.3. Effects of the CSF Layer on Brain Motion (Exp. CSF)
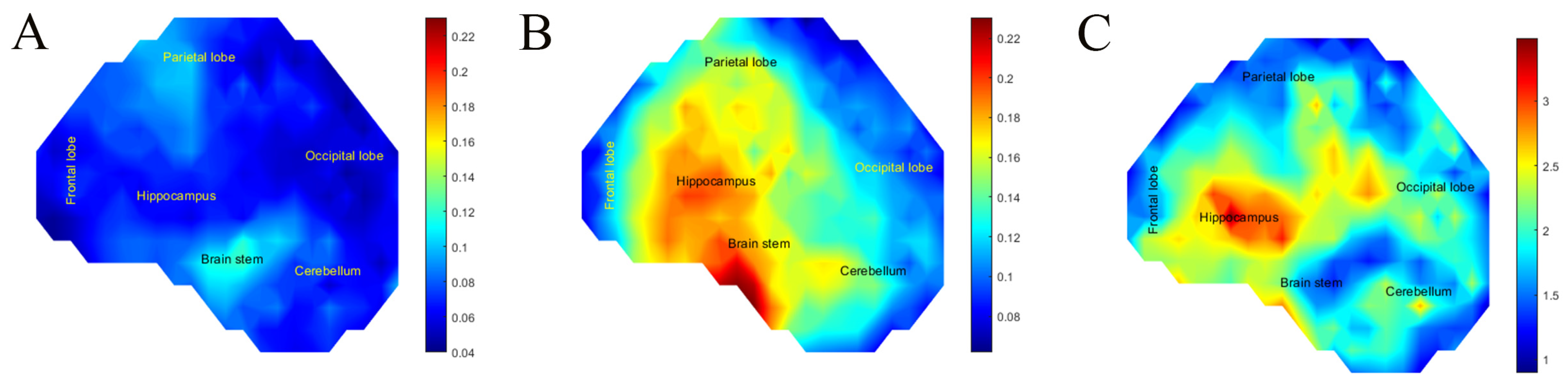
3.4. Surrogate Head Size (Exp. SHS)
3.5. Vasculature (Exp. VASC)
3.6. Neck Stiffness (Exp. NS)
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Dewan, M.C.; Rattani, A.; Gupta, S.; Baticulon, R.E.; Hung, Y.-C.; Punchak, M.; Agrawal, A.; Adeleye, A.O.; Shrime, M.G.; Rubiano, A.M.; et al. Estimating the global incidence of traumatic brain injury. J. Neurosurg. JNS 2019, 130, 1080–1097. [Google Scholar] [CrossRef] [PubMed]
- Surveillance Report of Traumatic Brain Injury-Related Emergency Department Visits, Hospitalizations, and Deaths; The National Center for Injury Prevention and Control (NCIPC), Centers for Disease Control and Prevention (CDC), U.S. Department of Health and Human Services: Atlanta, Georgia, 2014.
- Courtney, A.; Courtney, M. The Complexity of Biomechanics Causing Primary Blast-Induced Traumatic Brain Injury: A Review of Potential Mechanisms. Front. Neurol. 2015, 6, 221. [Google Scholar] [CrossRef] [PubMed]
- Meaney, D.F.; Morrison, B.; Dale Bass, C. The mechanics of traumatic brain injury: A review of what we know and what we need to know for reducing its societal burden. J. Biomech. Eng. 2014, 136, 021008. [Google Scholar] [CrossRef] [PubMed]
- Coronado, V.G.; Xu, L.; Basavaraju, S.V.; McGuire, L.C.; Wald, M.M.; Faul, M.D.; Guzman, B.R.; Hemphill, J.D.; Centers for Disease Control and Prevention (CDC). Surveillance for traumatic brain injury-related deaths--United States, 1997–2007. MMWR Surveill. Summ. 2011, 60, 1–32. [Google Scholar] [PubMed]
- Wright, D.W.; Kellermann, A.; McGuire, L.C.; Chen, B.; Popovic, T. CDC grand rounds: Reducing severe traumatic brain injury in the United States. MMWR Morb. Mortal. Wkly. Rep. 2013, 62, 549–552. [Google Scholar]
- Gurkoff, G.; Shahlaie, K.; Lyeth, B.; Berman, R. Voltage-gated calcium channel antagonists and traumatic brain injury. Pharmaceuticals 2013, 6, 788–812. [Google Scholar] [CrossRef] [PubMed]
- Ma, V.Y.; Chan, L.; Carruthers, K.J. Incidence, prevalence, costs, and impact on disability of common conditions requiring rehabilitation in the United States: Stroke, spinal cord injury, traumatic brain injury, multiple sclerosis, osteoarthritis, rheumatoid arthritis, limb loss, and back pain. Arch. Phys. Med. Rehabil. 2014, 95, 986–995.e1. [Google Scholar] [CrossRef] [PubMed]
- Thurman, D.J.; Alverson, C.; Browne, D.; Dunn, K.A.; Guerrero, J.; Johnson, R.; Johnson, V.; Langlois, J.; Pilkey, D.; Sniezek, J.E.; et al. Traumatic Brain Injury in the United States: A Report to Congress; Centers for Disease Control and Prevention Atlanta GA USA(Control, N.C.f.I.P.a.), Ed.; National Center for Injury Prevention and Control; Division of Unintentional Injury Prevention: Atlanta, GA, USA, 1999.
- Cernak, I.; Stein, D.G.; Elder, G.A.; Ahlers, S.; Curley, K.; DePalma, R.G.; Duda, J.; Ikonomovic, M.; Iverson, G.L.; Kobeissy, F.; et al. Preclinical modelling of militarily relevant traumatic brain injuries: Challenges and recommendations for future directions. Brain Inj. 2017, 31, 1168–1176. [Google Scholar] [CrossRef] [PubMed]
- Hoge, C.W.; McGurk, D.; Thomas, J.L.; Cox, A.L.; Engel, C.C.; Castro, C.A. Mild traumatic brain injury in U.S. Soldiers returning from Iraq. N. Engl. J. Med. 2008, 358, 453–463. [Google Scholar] [CrossRef]
- Hoge, C.W.; Goldberg, H.M.; Castro, C.A. Care of War Veterans with Mild Traumatic Brain Injury—Flawed Perspectives. N. Engl. J. Med. 2009, 360, 1588–1591. [Google Scholar] [CrossRef]
- Ng, H.K.; Mahaliyana, R.D.; Poon, W.S. The pathological spectrum of diffuse axonal injury in blunt head trauma: Assessment with axon and myelin stains. Clin. Neurol. Neurosurg. 1994, 96, 24–31. [Google Scholar] [CrossRef]
- Adams, J.H.; Graham, D.I.; Doyle, D.; Lawrence, A.E.; Mclellan, D.R. Diffuse axonal injury in head injuries caused by a fall. Lancet 1984, 2, 1420–1422. [Google Scholar] [CrossRef] [PubMed]
- Gennarelli, T.A. Mechanisms of brain injury. J. Emerg. Med. 1993, 11 (Supp 1), 5–11. [Google Scholar] [PubMed]
- Grady, M.S.; McLaughlin, M.R.; Christman, C.W.; Valadka, A.B.; Fligner, C.L.; Povlishock, J.T. The use of antibodies targeted against the neurofilament subunits for the detection of diffuse axonal injury in humans. J. Neuropathol. Exp. Neurol. 1993, 52, 143–152. [Google Scholar] [CrossRef]
- Smith, D.H.; Meaney, D.F. Axonal Damage in Traumatic Brain Injury. Neuroscientist 2000, 6, 483–495. [Google Scholar] [CrossRef]
- Thibault, L.E.; Gennarelli, T.A.; Margulies, S.S.; Marcus, J.; Eppinger, R. The Strain Dependent Pathophysiological Consequences of Inertial Loading on Central Nervous System Tissue. In Proceedings of the International Conference on the Biomechanics of Impact, Lyon, France, 12–14 September 1990; pp. 191–202. [Google Scholar]
- Walter Reed Army Medical Center (Center, W.R.A.M.) Traumatic Brain Injury Program. 2006. Available online: http://www.wramc.amedd.army.mil/Patients/healthcare/aasc/speech/Pages/brain.aspx (accessed on 12 April 2007).
- Holbourn, A.H.S. Mechanics of Head Injury. Lancet 1943, 2, 438–441. [Google Scholar] [CrossRef]
- Holbourn, A.H.S. The Mechanics of Brain Injuries. Br. Med. Bull. 1945, 3, 147–149. [Google Scholar] [CrossRef]
- Strich, S.J. Shearing of Nerve Fibres as a Cause of Brain Damage Due to Head Injury. Lancet 1961, 278, 443–448. [Google Scholar] [CrossRef]
- Martin, R.M.; Wright, M.J.; Lutkenhoff, E.S.; Ellingson, B.M.; Van Horn, J.D.; Tubi, M.; Alger, J.R.; McArthur, D.L.; Vespa, P.M. Traumatic hemorrhagic brain injury: Impact of location and resorption on cognitive outcome. J. Neurosurg. 2017, 126, 796–804. [Google Scholar] [CrossRef]
- Mychasiuk, R.; Hehar, H.; Candy, S.; Ma, I.; Esser, M.J. The direction of the acceleration and rotational forces associated with mild traumatic brain injury in rodents effect behavioural and molecular outcomes. J. Neurosci. Methods 2016, 257, 168–178. [Google Scholar] [CrossRef]
- Post, A.; Hoshizaki, T.B.; Gilchrist, M.D.; Brien, S.; Cusimano, M.; Marshall, S. Traumatic brain injuries: The influence of the direction of impact. Neurosurgery 2015, 76, 81–91. [Google Scholar] [CrossRef] [PubMed]
- Hanna, M.; Ali, A.S.; Klienberger, M.; Pfister, B.J. A method for evaluating brain deformation under sagittal blunt impacts using a half-skull human-scale surrogate. J. Biomech. Eng. 2023, 145, 061001. [Google Scholar] [CrossRef] [PubMed]
- Petrone, N.; Candiotto, G.; Marzella, E.; Uriati, F.; Carraro, G.; Bäckström, M.; Koptyug, A. Feasibility of using a novel instrumented human head surrogate to measure helmet, head and brain kinematics and intracranial pressure during multidirectional impact tests. J. Sci. Med. Sport 2019, 22, S78–S84. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Domel, A.G.; Cecchi, N.J.; Rice, E.; Callan, A.A.; Raymond, S.J.; Zhou, Z.; Zhan, X.; Li, Y.; Zeineh, M.M.; et al. Time Window of Head Impact Kinematics Measurement for Calculation of Brain Strain and Strain Rate in American Football. Ann. Biomed. Eng. 2021, 49, 2791–2804. [Google Scholar] [CrossRef] [PubMed]
- Cloots, R.J.H.; van Dommelen, J.A.W.; Kleiven, S.; Geers, M.G.D. Multi-scale mechanics of traumatic brain injury: Predicting axonal strains from head loads. Biomech. Model. Mechanobiol. 2013, 12, 137–150. [Google Scholar] [CrossRef] [PubMed]
- Takhounts, E.G.; Ridella, S.A.; Hasija, V.; Tannous, R.E.; Campbell, J.Q.; Malone, D.; Danelson, K.; Stitzel, J.; Rowson, S.; Duma, S. Investigation of Traumatic Brain Injuries Using the Next Generation of Simulated Injury Monitor (SIMon) Finite Element Head Model; SAE Technical Paper; SAE International: Warrendale, PA, USA, 2008. [Google Scholar]
- Mao, H.; Zhang, L.; Jiang, B.; Genthikatti, V.V.; Jin, X.; Zhu, F.; Makwana, R.; Gill, A.; Jandir, G.; Singh, A. Development of a finite element human head model partially validated with thirty five experimental cases. J. Biomech. Eng. 2013, 135, 111002. [Google Scholar] [CrossRef] [PubMed]
- Takhounts, E.G.; Eppinger, R.H.; Campbell, J.Q.; Tannous, R.E.; Power, E.D.; Shook, L.S. On the Development of the SIMon Finite Element Head Model. Stapp Car Crash J. 2003, 47, 107–133. [Google Scholar] [PubMed]
- Carlsen, R.W.; Daphalapurkar, N.P. The importance of structural anisotropy in computational models of traumatic brain injury. Front. Neurol. 2015, 6, 28. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Madhukar, A.; Ostoja-Starzewski, M. Finite Element Methods in Human Head Impact Simulations: A Review. Ann. Biomed. Eng. 2019, 47, 1832–1854. [Google Scholar] [CrossRef]
- Hardy, W.N.; Mason, M.J.; Foster, C.D.; Shah, C.S.; Kopacz, J.M.; Yang, K.H.; King, A.I.; Bishop, J.; Bey, M.; Anderst, W.; et al. A Study of the Response of the Human Cadaver Head to Impact. Stapp Car Crash J. 2007, 51, 17–80. [Google Scholar]
- Yang, B.; Tse, K.-M.; Chen, N.; Tan, L.-B.; Zheng, Q.-Q.; Yang, H.-M.; Hu, M.; Pan, G.; Lee, H.-P. Development of a Finite Element Head Model for the Study of Impact Head Injury. BioMed Res. Int. 2014, 2014, 408278. [Google Scholar] [CrossRef] [PubMed]
- Freitas, C.J.; Mathis, J.T.; Scott, N.; Bigger, R.P.; Mackiewicz, J. Dynamic response due to behind helmet blunt trauma measured with a human head surrogate. Int. J. Med Sci. 2014, 11, 409–425. [Google Scholar] [CrossRef]
- Singh, A.; Ganpule, S.G.; Khan, M.K.; Iqbal, M.A. Measurement of brain simulant strains in head surrogate under impact loading. Biomech. Model. Mechanobiol. 2021, 20, 2319–2334. [Google Scholar] [CrossRef] [PubMed]
- Knutsen, A.K.; Vidhate, S.; McIlvain, G.; Luster, J.; Galindo, E.J.; Johnson, C.L.; Pham, D.L.; Butman, J.A.; Mejia-Alvarez, R.; Tartis, M.; et al. Characterization of material properties and deformation in the ANGUS phantom during mild head impacts using MRI. J. Mech. Behav. Biomed. Mater. 2023, 138, 105586. [Google Scholar] [CrossRef]
- Raymond, D.E.; Bir, C.A. A biomechanical evaluation of skull-brain surrogates to blunt high-rate impacts to postmortem human subjects. J. Forensic Sci. 2015, 60, 370–373. [Google Scholar] [CrossRef]
- Ono, K.; Kikuchi, A.; Nakamura, M.; Kobayashi, H.; Nakamura, N. Human Head Tolerance to Sagittal Impact Reliable Estimation Deduced from Experimental Head Injury Using Subhuman Primates and Human Cadaver Skulls; SAE International: Warrendale, PA, USA, 1980. [Google Scholar]
- Budday, S.; Ovaert, T.C.; Holzapfel, G.A.; Steinmann, P.; Kuhl, E. Fifty shades of brain: A review on the mechanical testing and modeling of brain tissue. Arch. Comput. Methods Eng. 2020, 27, 1187–1230. [Google Scholar] [CrossRef]
- Bayly, P.V.; Cohen, T.S.; Leister, E.P.; Ajo, D.; Leuthardt, E.C.; Genin, G.M. Deformation of the human brain induced by mild acceleration. J. Neurotrauma 2005, 22, 845–856. [Google Scholar] [CrossRef] [PubMed]
- Meaney, D.F.; Thibault, L.E. Using physical models to determine cortical strains in the head during dynamic loading. In Proceedings of the Images of the Twenty-First Century, Annual International Engineering in Medicine and Biology Society, Seattle, WA, USA, 9–12 November 1989. [Google Scholar]
- Margulies, S.S.; Thibault, L.E.; Gennarelli, T.A. Physical model simulations of brain injury in the primate. J. Biomech. 1990, 23, 823–836. [Google Scholar] [CrossRef]
- Salzar, R.S.; Treichler, D.; Wardlaw, A.; Weiss, G.; Goeller, J. Experimental Investigation of Cavitation as a Possible Damage Mechanism in Blast-Induced Traumatic Brain Injury in Post-Mortem Human Subject Heads. J. Neurotrauma 2017, 34, 1589–1602. [Google Scholar] [CrossRef]
- Ganpule, S.; Alai, A.; Plougonven, E.; Chandra, N. Mechanics of blast loading on the head models in the study of traumatic brain injury using experimental and computational approaches. Biomech. Model. Mechanobiol. 2013, 12, 511–531. [Google Scholar] [CrossRef]
- Carr, D.; Lindstrom, A.-C.; Jareborg, A.; Champion, S.; Waddell, N.; Miller, D.; Teagle, M.; Horsfall, I.; Kieser, J. Development of a skull/brain model for military wound ballistics studies. Int. J. Leg. Med. 2015, 129, 505–510. [Google Scholar] [CrossRef] [PubMed]
- Davidsson, J.; Risling, M. Characterization of Pressure Distribution in Penetrating Traumatic Brain Injuries. Front. Neurol. 2015, 6, 51. [Google Scholar] [CrossRef] [PubMed]
- Carr, D.J.; Stevenson, T.; Mahoney, P.F. The use of gelatine in wound ballistics research. Int. J. Leg. Med. 2018, 132, 1659–1664. [Google Scholar] [CrossRef] [PubMed]
- Mahoney, P.; Carr, D.; Arm, R.; Gibb, I.; Hunt, N.; Delaney, R.J. Ballistic impacts on an anatomically correct synthetic skull with a surrogate skin/soft tissue layer. Int. J. Leg. Med. 2018, 132, 519–530. [Google Scholar] [CrossRef] [PubMed]
- Wermer, A.; Kerwin, J.; Welsh, K.; Mejia-Alvarez, R.; Tartis, M.; Willis, A. Materials Characterization of Cranial Simulants for Blast-Induced Traumatic Brain Injury. Mil. Med. 2020, 185, 205–213. [Google Scholar] [CrossRef] [PubMed]
- Moy, P.; Weerasooriya, T.; Juliano, T.F.; VanLandingham, M.R.; Chen, W. Dynamic Response of an Alternative Tissue Simulant, Physically Associating Gels (PAG). In Proceedings of the Society for Experimental Mechanics Conference, St. Louis, MO, USA, 4–7 June 2006. [Google Scholar]
- Pasumarthy, R.K.A.; Tippur, H.V. Mechanical and optical characterization of a tissue surrogate polymer gel. Polym. Test. 2016, 55, 219–229. [Google Scholar] [CrossRef]
- Finan, J.D.; Sundaresh, S.N.; Elkin, B.S.; McKhann, G.M., 2nd; Morrison, B., 3rd. Regional mechanical properties of human brain tissue for computational models of traumatic brain injury. Acta Biomater. 2017, 55, 333–339. [Google Scholar] [CrossRef] [PubMed]
- Elkin, B.S.; Ilankova, A.; Morrison, B., III. Dynamic, Regional Mechanical Properties of the Porcine Brain: Indentation in the Coronal Plane. J. Biomech. Eng. 2011, 133, 071009. [Google Scholar] [CrossRef] [PubMed]
- Bloomfield, I.G.; Johnston, I.H.; Bilston, L.E. Effects of Proteins, Blood Cells and Glucose on the Viscosity of Cerebrospinal Fluid. Pediatr. Neurosurg. 1998, 28, 246–251. [Google Scholar] [CrossRef]
- Sakka, L.; Coll, G.; Chazal, J. Anatomy and physiology of cerebrospinal fluid. Eur. Ann. Otorhinolaryngol. Head Neck Dis. 2011, 128, 309–316. [Google Scholar] [CrossRef]
- Thomas, J.H. Fluid dynamics of cerebrospinal fluid flow in perivascular spaces. J. R. Soc. Interface 2019, 16, 20190572. [Google Scholar] [CrossRef] [PubMed]
- Wu, J.Z.; Pan, C.S.; Wimer, B.M.; Rosen, C.L. An improved finite element modeling of the cerebrospinal fluid layer in the head impact analysis. Bio-Med. Mater. Eng. 2017, 28, 187–199. [Google Scholar] [CrossRef] [PubMed]
- Young, J.W. Head and Face Anthropometry of Adult U.S. Civilians; Federal Aviation Administration Washington DC Office of Aviation Medicine: Washington, DC, USA, 1993.
- Yu, R.; Lui, F. Neuroanatomy, Brain Arteries. In StatPearls; StatPearls Publishing Copyright © 2022; StatPearls Publishing LLC.: Treasure Island, FL, USA, 2022. [Google Scholar]
- Gent, A.N. On the Relation between Indentation Hardness and Young’s Modulus. Rubber Chem. Technol. 1958, 31, 896–906. [Google Scholar] [CrossRef]
- Qi, H.J.; Joyce, K.; Boyce, M.C. Durometer hardness and the stress-strain behavior of elastomeric materials. Rubber Chem. Technol. 2003, 76, 419. [Google Scholar] [CrossRef]
- Larson, K. Can You Estimate Modulus from Durometer Hardness for Silicones? Yes, but Only Roughly … and You Must Choose Your Modulus Carefully! 2017. Available online: https://www.researchgate.net/publication/336239577_Can_You_Estimate_Modulus_From_Durometer_Hardness_for_Silicones_Yes_but_only_roughly_and_you_must_choose_your_modulus_carefully (accessed on 12 April 2024).
- Ebrahimi, A.P. Mechanical properties of normal and diseased cerebrovascular system. J. Vasc. Interv. Neurol. 2009, 2, 155–162. [Google Scholar] [PubMed]
- Spittle, E.K.; Miller, D.J.; Shipley, B.W., Jr.; Kaleps, I. Hybrid II and Hybrid III Dummy Neck Properties for Computer Modeling; Armstrong Lab Wright-Patterson AFB OH: Dayton, Ohio, 1992. [Google Scholar]
- McGill, S.M.; Jones, K.; Bennett, G.; Bishop, P.J. Passive stiffness of the human neck in flexion, extension, and lateral bending. Clin. Biomech. 1994, 9, 193–198. [Google Scholar] [CrossRef] [PubMed]
- Tierney, R.T.; Sitler, M.R.; Swanik, C.B.; Swanik, K.A.; Higgins, M.; Torg, J. Gender differences in head-neck segment dynamic stabilization during head acceleration. Med. Sci. Sports Exerc. 2005, 37, 272–279. [Google Scholar] [CrossRef]
- Kendall, M.J.; Siviour, C.R. Strain rate dependence in plasticized and un-plasticized PVC. EPJ Web Conf. 2012, 26, 02009. [Google Scholar] [CrossRef]
- Kendall, M.J.; Siviour, C.R. Rate dependence of poly(vinyl chloride), the effects of plasticizer and time–temperature superposition. Proc. R. Soc. A Math. Phys. Eng. Sci. 2014, 470, 20140012. [Google Scholar] [CrossRef]
- Königshofer, M.; Stoiber, M.; Unger, E.; Grasl, C.; Moscato, F. Mechanical and Dimensional Investigation of Additive Manufactured Multimaterial Parts. Front. Phys. 2021, 9, 635736. [Google Scholar] [CrossRef]
- Pugalendhi, A.; Ranganathan, R.; Ganesan, S. Impact of process parameters on mechanical behaviour in multi-material jetting. Mater. Today Proc. 2021, 46, 9139–9144. [Google Scholar] [CrossRef]
- Bellini, A.; Güçeri, S. Mechanical characterization of parts fabricated using fused deposition modeling. Rapid Prototyp. J. 2003, 9, 252–264. [Google Scholar] [CrossRef]
- Rodríguez, J.F.; Thomas, J.P.; Renaud, J.E. Mechanical behavior of acrylonitrile butadiene styrene (ABS) fused deposition materials. Experimental investigation. Rapid Prototyp. J. 2001, 7, 148–158. [Google Scholar] [CrossRef]
- Yoganandan, N.; Pintar, F.A.; Sances, A., Jr.; Walsh, P.R.; Ewing, C.L.; Thomas, D.J.; Snyder, R.G. Biomechanics of skull fracture. J. Neurotrauma 1995, 12, 659–668. [Google Scholar] [CrossRef] [PubMed]
- Zhai, X.; Nauman, E.A.; Moryl, D.; Lycke, R.; Chen, W.W. The effects of loading-direction and strain-rate on the mechanical behaviors of human frontal skull bone. J. Mech. Behav. Biomed. Mater. 2020, 103, 103597. [Google Scholar] [CrossRef] [PubMed]
- McElhaney, J.H.; Fogle, J.L.; Melvin, J.W.; Haynes, R.R.; Roberts, V.L.; Alem, N.M. Mechanical properties on cranial bone. J. Biomech. 1970, 3, 495–511. [Google Scholar] [CrossRef] [PubMed]
- Motherway, J.A.; Verschueren, P.; Van der Perre, G.; Vander Sloten, J.; Gilchrist, M.D. The mechanical properties of cranial bone: The effect of loading rate and cranial sampling position. J. Biomech. 2009, 42, 2129–2135. [Google Scholar] [CrossRef] [PubMed]
- Alexander, S.L.; Gunnarsson, C.A.; Rafaels, K.; Weerasooriya, T. Multiscale response of the human skull to quasi-static compression. J. Mech. Behav. Biomed. Mater. 2020, 102, 103492. [Google Scholar] [CrossRef] [PubMed]
- Sahoo, D.; Deck, C.; Willinger, R. Brain injury tolerance limit based on computation of axonal strain. Accid. Anal. Prev. 2016, 92, 53–70. [Google Scholar] [CrossRef]
- Sanchez, E.J.; Gabler, L.F.; McGhee, J.S.; Olszko, A.V.; Chancey, V.C.; Crandall, J.R.; Panzer, M.B. Evaluation of Head and Brain Injury Risk Functions Using Sub-Injurious Human Volunteer Data. J. Neurotrauma 2017, 34, 2410–2424. [Google Scholar] [CrossRef]
- Pervin, F.; Chen, W.W. Mechanically Similar Gel Simulants for Brain Tissues; Springer: New York, NY, USA, 2011; pp. 9–13. [Google Scholar] [CrossRef]
- Ahmadzadeh, S.M.H.; Hukins, D.W.L. Feasibility of using mixtures of silicone elastomers and silicone oils to model the mechanical behaviour of biological tissues. Proc. Inst. Mech. Eng. Part H J. Eng. Med. 2014, 228, 730–734. [Google Scholar] [CrossRef] [PubMed]
- Chanda, A.; Callaway, C.; Clifton, C.; Unnikrishnan, V. Biofidelic human brain tissue surrogates. Mech. Adv. Mater. Struct. 2018, 25, 1335–1341. [Google Scholar] [CrossRef]
- Singh, D.; Boakye-Yiadom, S.; Cronin, D.S. Comparison of porcine brain mechanical properties to potential tissue simulant materials in quasi-static and sinusoidal compression. J. Biomech. 2019, 92, 84–91. [Google Scholar] [CrossRef] [PubMed]
- Kleiven, S.; von Holst, H. Consequences of head size following trauma to the human head. J. Biomech. 2002, 35, 153–160. [Google Scholar] [CrossRef] [PubMed]
- Jones, D.A.; Urban, J.E.; Lillie, E.M.; Stitzel, J.D. Skull Thickness Morphing for an Age and Sex Specific FE Model of the Skull. Biomed. Sci. Instrum. 2015, 51, 173–180. [Google Scholar] [PubMed]
- Sahoo, D.; Deck, C.; Yoganandan, N.; Willinger, R. Influence of head mass on temporo-parietal skull impact using finite element modeling. Med. Biol. Eng. Comput. 2015, 53, 869–878. [Google Scholar] [CrossRef]
- Shaoo, D.; Deck, C.; Yoganandan, N.; Willinger, R. Influence of stiffness and shape of contact surface on skull fractures and biomechanical metrics of the human head of different population underlateral impacts. Accid. Anal. Prev. 2015, 80, 97–105. [Google Scholar] [CrossRef] [PubMed]
- Lillie, E.M.; Urban, J.E.; Lynch, S.K.; Weaver, A.A.; Stitzel, J.D. Evaluation of Skull Cortical Thickness Changes With Age and Sex From Computed Tomography Scans. J. Bone Miner. Res. 2016, 31, 299–307. [Google Scholar] [CrossRef] [PubMed]
- Zhang, L.; Bae, J.; Hardy, W.N.; Monson, K.L.; Manley, G.T.; Goldsmith, W.; Yang, K.H.; King, A.I. Computational Study of the Contribution of the Vasculature on the Dynamic Response of the Brain; The Stapp Association: Danvers, MA, USA, 2002. [Google Scholar]
- Zhao, W.; Ji, S. Incorporation of vasculature in a head injury model lowers local mechanical strains in dynamic impact. J. Biomech. 2020, 104, 109732. [Google Scholar] [CrossRef] [PubMed]
- Subramaniam, D.R.; Unnikrishnan, G.; Sundaramurthy, A.; Rubio, J.E.; Kote, V.B.; Reifman, J. The importance of modeling the human cerebral vasculature in blunt trauma. Biomed. Eng. Online 2021, 20, 11. [Google Scholar] [CrossRef]
- Subramaniam, D.R.; Unnikrishnan, G.; Sundaramurthy, A.; Rubio, J.E.; Kote, V.B.; Reifman, J. Cerebral Vasculature Influences Blast-Induced Biomechanical Responses of Human Brain Tissue. Front. Bioeng. Biotechnol. 2021, 9, 744808. [Google Scholar] [CrossRef] [PubMed]
- Zhao, W.; Ji, S. Cerebral vascular strains in dynamic head impact using an upgraded model with brain material property heterogeneity. J. Mech. Behav. Biomed. Mater. 2022, 126, 104967. [Google Scholar] [CrossRef] [PubMed]
- Mansell, J.; Tierney, R.T.; Sitler, M.R.; Swanik, K.A.; Stearne, D. Resistance training and head-neck segment dynamic stabilization in male and female collegiate soccer players. J. Athl. Train. 2005, 40, 310–319. [Google Scholar] [PubMed]
- Kimpara, H.; Nakahira, Y.; Iwamoto, M.; Miki, K.; Ichihara, K.; Kawano, S.; Taguchi, T. Investigation of anteroposterior head-neck responses during severe frontal impacts using a brain-spinal cord complex FE model. Stapp Car Crash J. 2006, 50, 509–544. [Google Scholar] [PubMed]
- Rousseau, P.; Hoshizaki, T.B.; Gilchrist, M.D.; Post, A. Estimating the influence of neckform compliance on brain tissue strain during a Helmeted impact. Stapp Car Crash J. 2010, 54, 37–48. [Google Scholar] [PubMed]
- Schmidt, J.D.; Guskiewicz, K.M.; Blackburn, J.T.; Mihalik, J.P.; Siegmund, G.P.; Marshall, S.W. The influence of cervical muscle characteristics on head impact biomechanics in football. Am. J. Sports Med. 2014, 42, 2056–2066. [Google Scholar] [CrossRef] [PubMed]
- Fanton, M.; Kuo, C.; Sganga, J.; Hernandez, F.; Camarillo, D.B. Dependency of Head Impact Rotation on Head-Neck Positioning and Soft Tissue Forces. IEEE Trans. Biomed. Eng. 2019, 66, 988–999. [Google Scholar] [CrossRef] [PubMed]
- Atsumi, N.; Nakahira, Y.; Tanaka, E.; Iwamoto, M. Human Brain Modeling with Its Anatomical Structure and Realistic Material Properties for Brain Injury Prediction. Ann. Biomed. Eng. 2018, 46, 736–748. [Google Scholar] [CrossRef] [PubMed]
- Levadnyi, I.; Awrejcewicz, J.; Zhang, Y.; Goethel, M.F.; Gu, Y. Finite Element Analysis of Impact for Helmeted and Non-helmeted Head. J. Med. Biol. Eng. 2018, 38, 587–595. [Google Scholar] [CrossRef]
- Cargill, R.S., 2nd; Thibault, L.E. Acute alterations in [Ca2+]i in NG108-15 cells subjected to high strain rate deformation and chemical hypoxia: An in vitro model for neural trauma. J. Neurotrauma 1996, 13, 395–407. [Google Scholar] [CrossRef]
- Geddes, D.M.; Cargill, R.S., 2nd; LaPlaca, M.C. Mechanical stretch to neurons results in a strain rate and magnitude-dependent increase in plasma membrane permeability. J. Neurotrauma 2003, 20, 1039–1049. [Google Scholar] [CrossRef] [PubMed]
- Cullen, D.K.; Simon, C.M.; LaPlaca, M.C. Strain rate-dependent induction of reactive astrogliosis and cell death in three-dimensional neuronal-astrocytic co-cultures. Brain Res. 2007, 1158, 103–115. [Google Scholar] [CrossRef] [PubMed]
- Skotak, M.; Wang, F.; Chandra, N. An in vitro injury model for SH-SY5Y neuroblastoma cells: Effect of strain and strain rate. J. Neurosci. Methods 2012, 205, 159–168. [Google Scholar] [CrossRef] [PubMed]
- Ahmadzadeh, H.; Smith, D.H.; Shenoy, V.B. Viscoelasticity of tau proteins leads to strain rate-dependent breaking of microtubules during axonal stretch injury: Predictions from a mathematical model. Biophys. J. 2014, 106, 1123–1133. [Google Scholar] [CrossRef]
- Meaney, D.F.; Smith, D.H.; Shreiber, D.I.; Bain, A.C.; Miller, R.T.; Ross, D.T.; Gennarelli, T.A. Biomechanical analysis of experimental diffuse axonal injury. J. Neurotrauma 1995, 12, 689–694. [Google Scholar] [CrossRef]
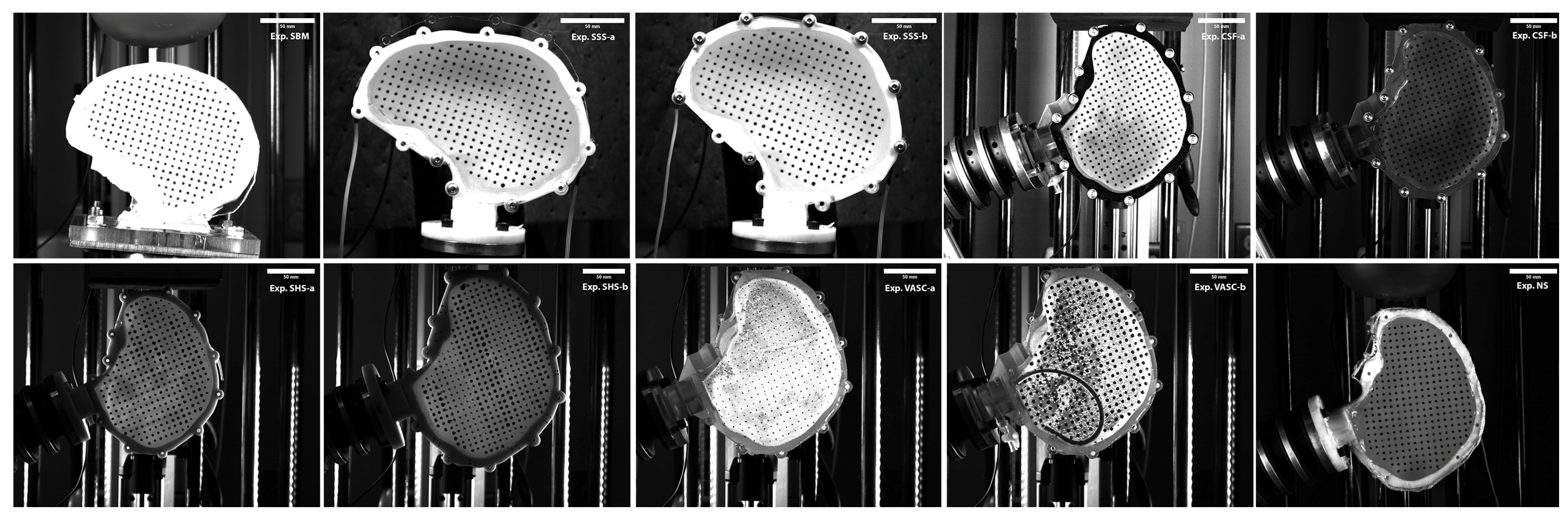






| Acronym | Experiment | SH Size Percentile Male | Surrogate Skull Material | Ballistic Gel Used | Impact Orientation | Impactor |
|---|---|---|---|---|---|---|
| SBM | Surrogate brain material properties | 50th | PVC | 5%, 10%, and 20% | Crown | Head-form |
| SSS | Surrogate skull stiffness | 50th | ABS | 20% | Crown | Head-form |
| CSF | CSF layer | 50th | VeroClear | 20% | Frontal | Flat |
| SHS | Surrogate head size | 10th and 90th | ABS | 20% | Frontal | Flat |
| VASC | Effect of Vasculature | 50th | VeroClear | 20% | Frontal | Flat |
| NS | Effect of neck stiffness | 50th | ABS | 20% | Frontal | Head-form |
| Injury Parameter | Surrogate Brain Material (SBM) | Surrogate Skull Stiffness (SSS) | Cerebrospinal Fluid (CSF) |
|---|---|---|---|
| Maximum shear strain | 1.24 | 1.92 | 2.69 |
| Shear strain rate/s−1 | 1.56 | 1.29 | 6.83 |
| Shear strain impulse/ms | none | 2.33 | 2.27 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hanna, M.; Ali, A.; Bhatambarekar, P.; Modi, K.; Lee, C.; Morrison, B., III; Klienberger, M.; Pfister, B.J. Anatomical Features and Material Properties of Human Surrogate Head Models Affect Spatial and Temporal Brain Motion under Blunt Impact. Bioengineering 2024, 11, 650. https://doi.org/10.3390/bioengineering11070650
Hanna M, Ali A, Bhatambarekar P, Modi K, Lee C, Morrison B III, Klienberger M, Pfister BJ. Anatomical Features and Material Properties of Human Surrogate Head Models Affect Spatial and Temporal Brain Motion under Blunt Impact. Bioengineering. 2024; 11(7):650. https://doi.org/10.3390/bioengineering11070650
Chicago/Turabian StyleHanna, Michael, Abdus Ali, Prasad Bhatambarekar, Karan Modi, Changhee Lee, Barclay Morrison, III, Michael Klienberger, and Bryan J. Pfister. 2024. "Anatomical Features and Material Properties of Human Surrogate Head Models Affect Spatial and Temporal Brain Motion under Blunt Impact" Bioengineering 11, no. 7: 650. https://doi.org/10.3390/bioengineering11070650
APA StyleHanna, M., Ali, A., Bhatambarekar, P., Modi, K., Lee, C., Morrison, B., III, Klienberger, M., & Pfister, B. J. (2024). Anatomical Features and Material Properties of Human Surrogate Head Models Affect Spatial and Temporal Brain Motion under Blunt Impact. Bioengineering, 11(7), 650. https://doi.org/10.3390/bioengineering11070650





