Finite Element Modeling of Residual Hearing after Cochlear Implant Surgery in Chinchillas
Abstract
1. Introduction
1.1. Cochlear Electrodes Importance and Trauma
1.2. Effect of CI Surgery on Residual Hearing
1.3. Effect of Insertion Angle on CI Effectiveness and Residual Hearing
1.4. Advantages of the FE Method over In Vivo Testing
1.5. Prior FE Models
1.6. Chinchilla as an Animal Model
1.7. Focus of the Study
2. Materials and Methods
2.1. Data Source and Segmentation
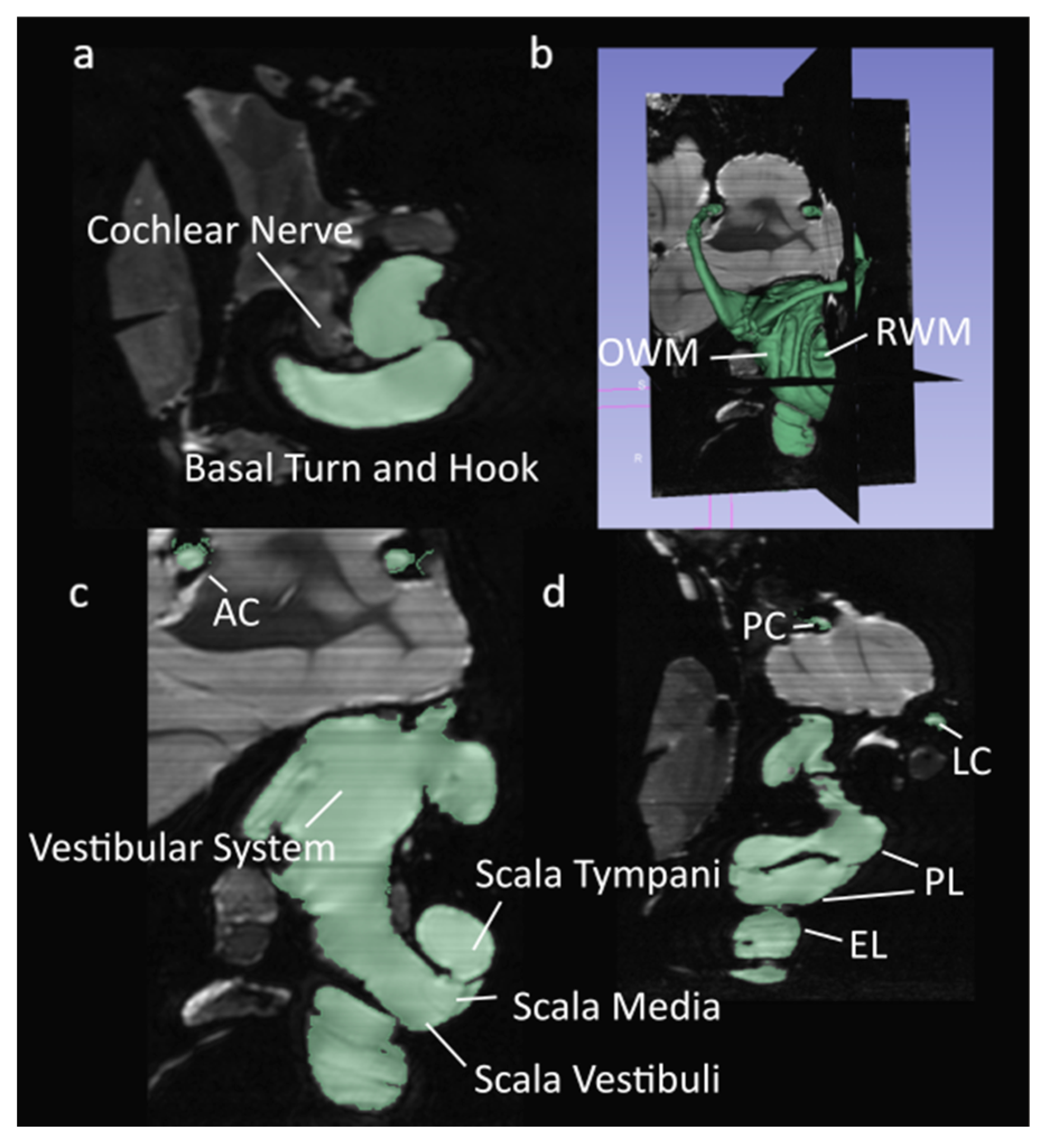
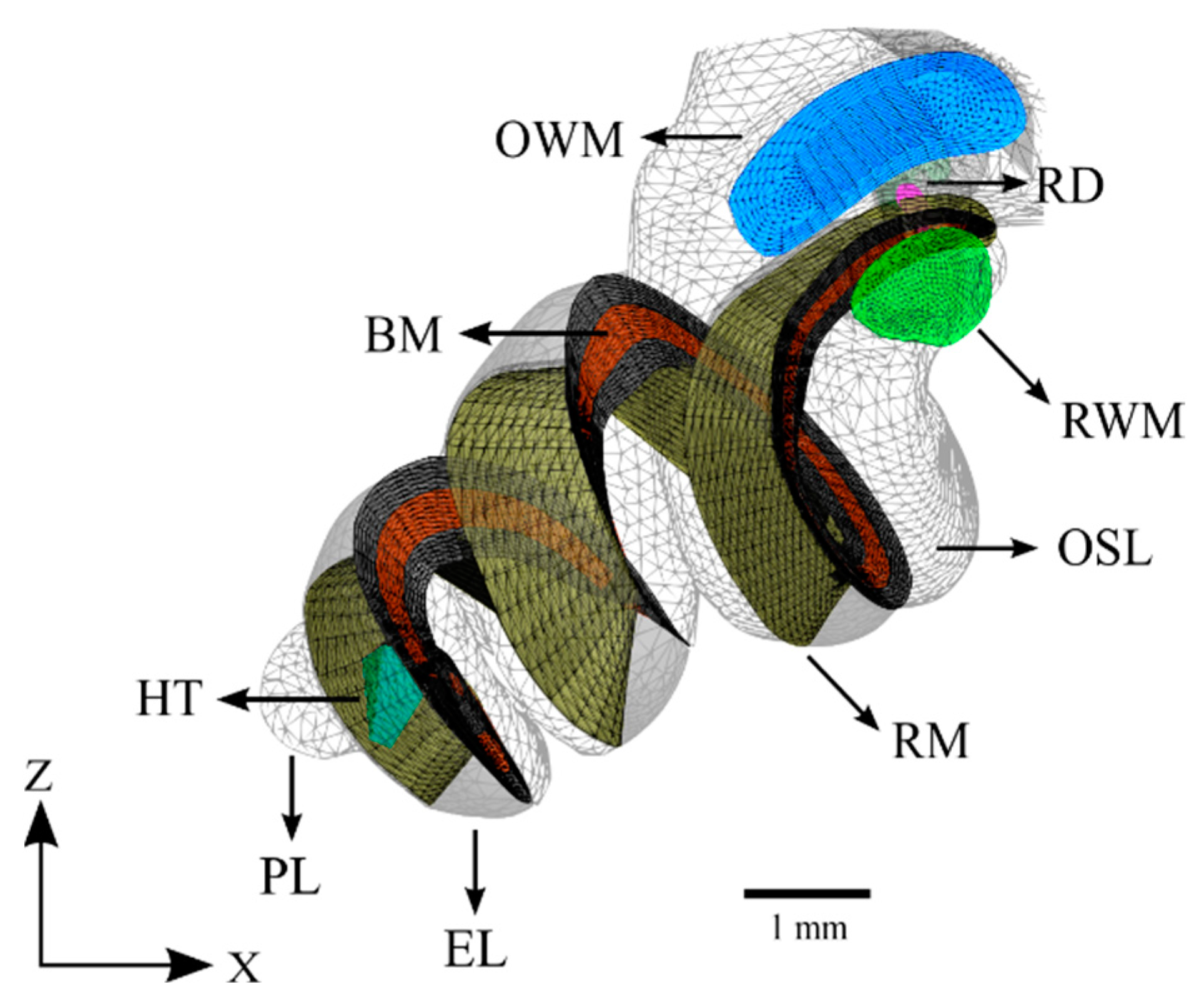
2.2. Geometry
2.3. Meshing
2.4. Material Properties
| Structure | Parameter | Source |
|---|---|---|
| Basilar Membrane | ||
| Density () | 1 × | [46] |
| Elastic Modulus (Pa) | ||
| β Damping Coefficient | ||
| Reissner’s Membrane | ||
| Density | 1 × | [46] |
| Elastic Modulus | ||
| β Damping Coefficient | ||
| Cupulae | ||
| Density | 1 × | [41] |
| Elastic Modulus | 2.8 | |
| Maculae | ||
| Gel Layer: | [34] | |
| Density | 1 × | |
| Elastic Modulus | 10 | |
| Otoconial Layer: | ||
| Density | 2.71 × | |
| Elastic Modulus | 500 | |
| Bone | ||
| Density | 1.2 × | [42,49] |
| Elastic Modulus | 13.4 × | |
| β Damping Coefficient | 0.45 | |
| Membranous Labyrinth | ||
| Density | 1 × | [41] |
| Elastic Modulus | 1.3 × | |
| β Damping Coefficient | 0.14 | |
| Lymphatic Fluids | ||
| Density | 1 × | [41,48] |
| Elastic Modulus | 2.6 × | |
| β Damping Coefficient | 1.5 × | |
| Viscosity (Pa·s) | 1 × | |
| Speed of Sound (m/s) | 1498 | |
| Oval Window Membrane | ||
| Density | 1 × | [45] |
| Elastic Modulus | 3.5 × | |
| β Damping Coefficient | 5 × | |
| Round Window Membrane | ||
| Density | 1.5 × | [44,45] |
| Elastic Modulus | 3.5 × | |
| β Damping Coefficient Cochlear Implant | 5 × | |
| Density | 3.4 × | [20,47] |
| Elastic Modulus | 4 × | |
| β Damping Coefficient | 7.7 × |
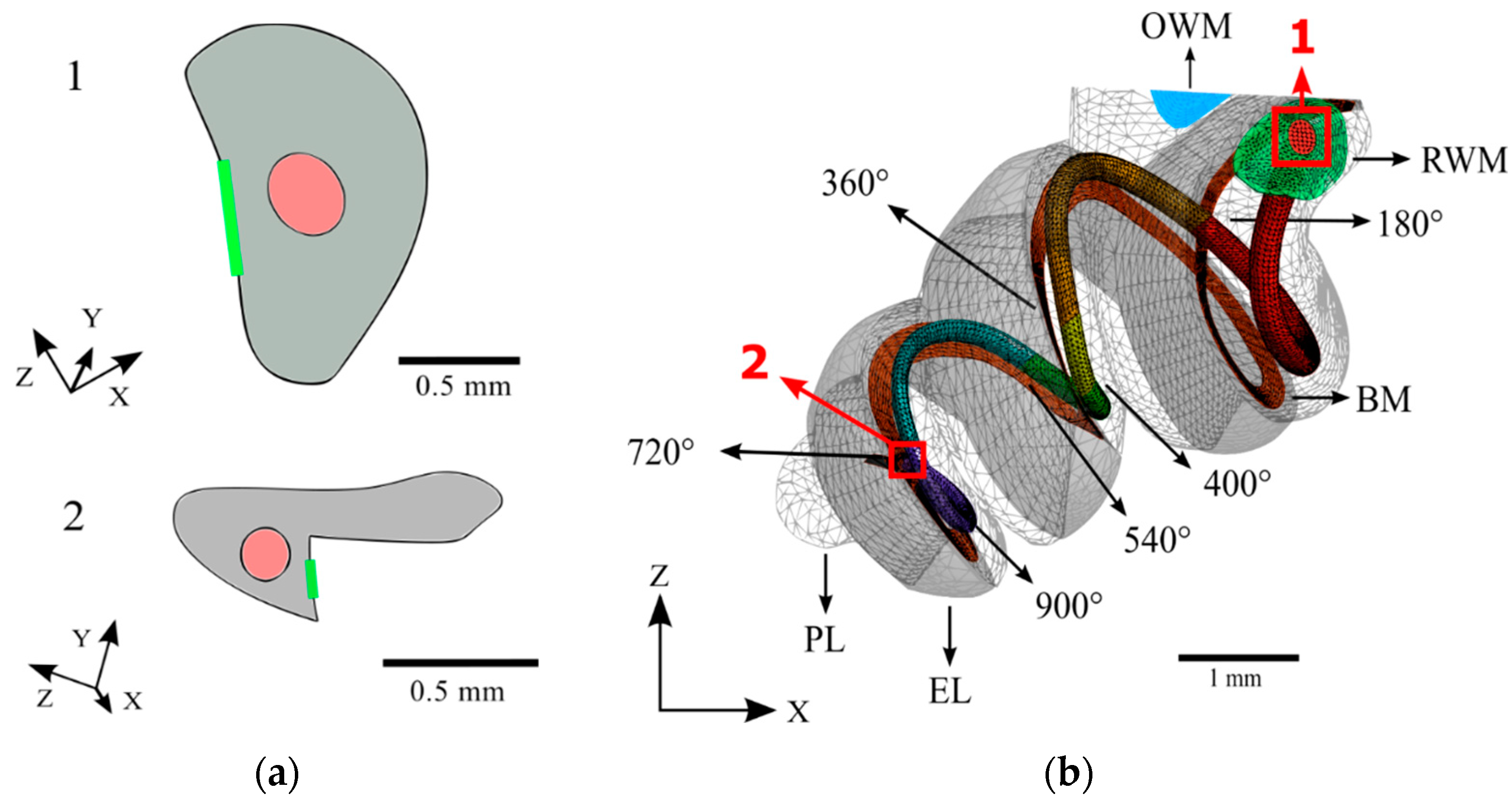
2.5. Boundary Conditions
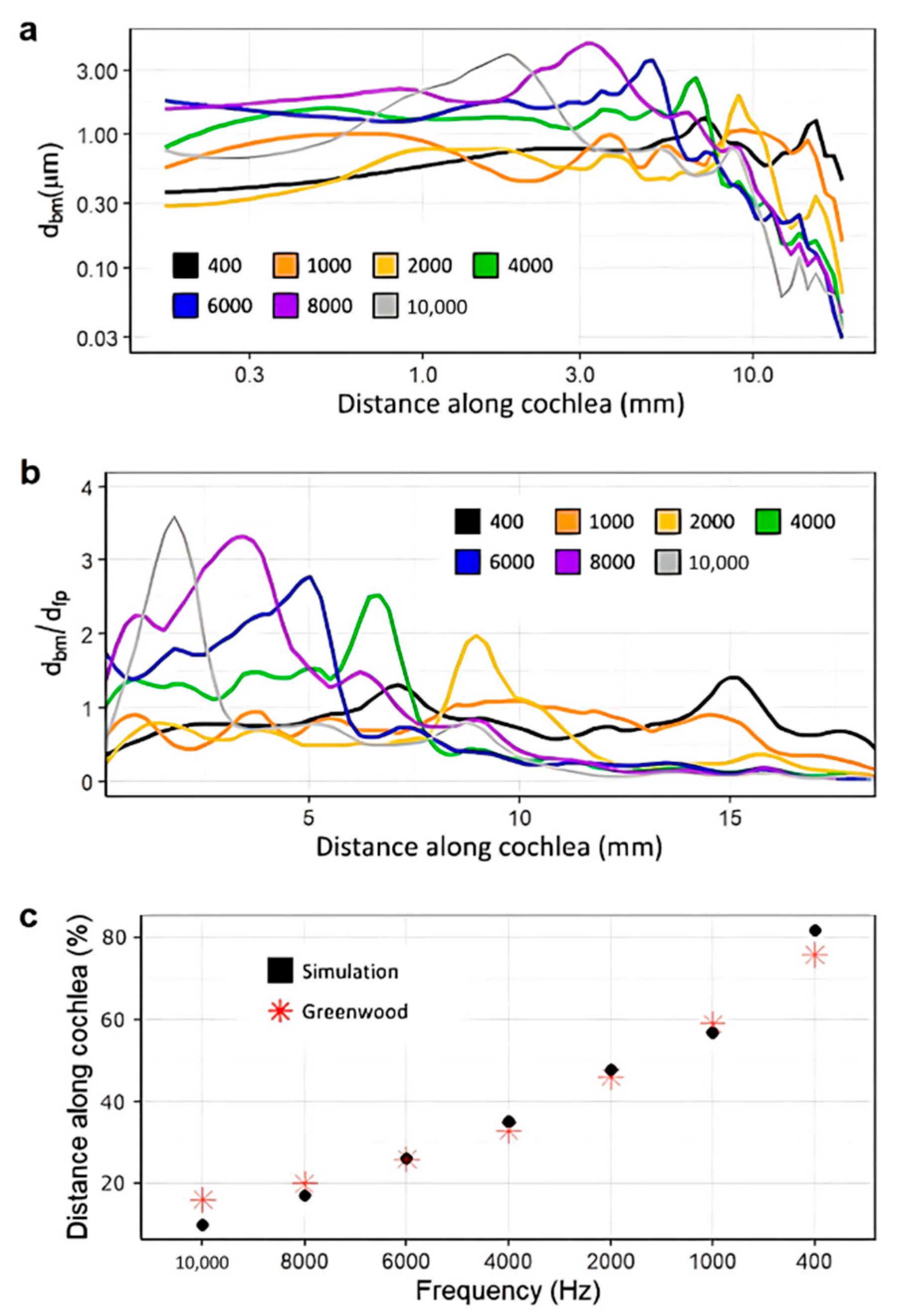
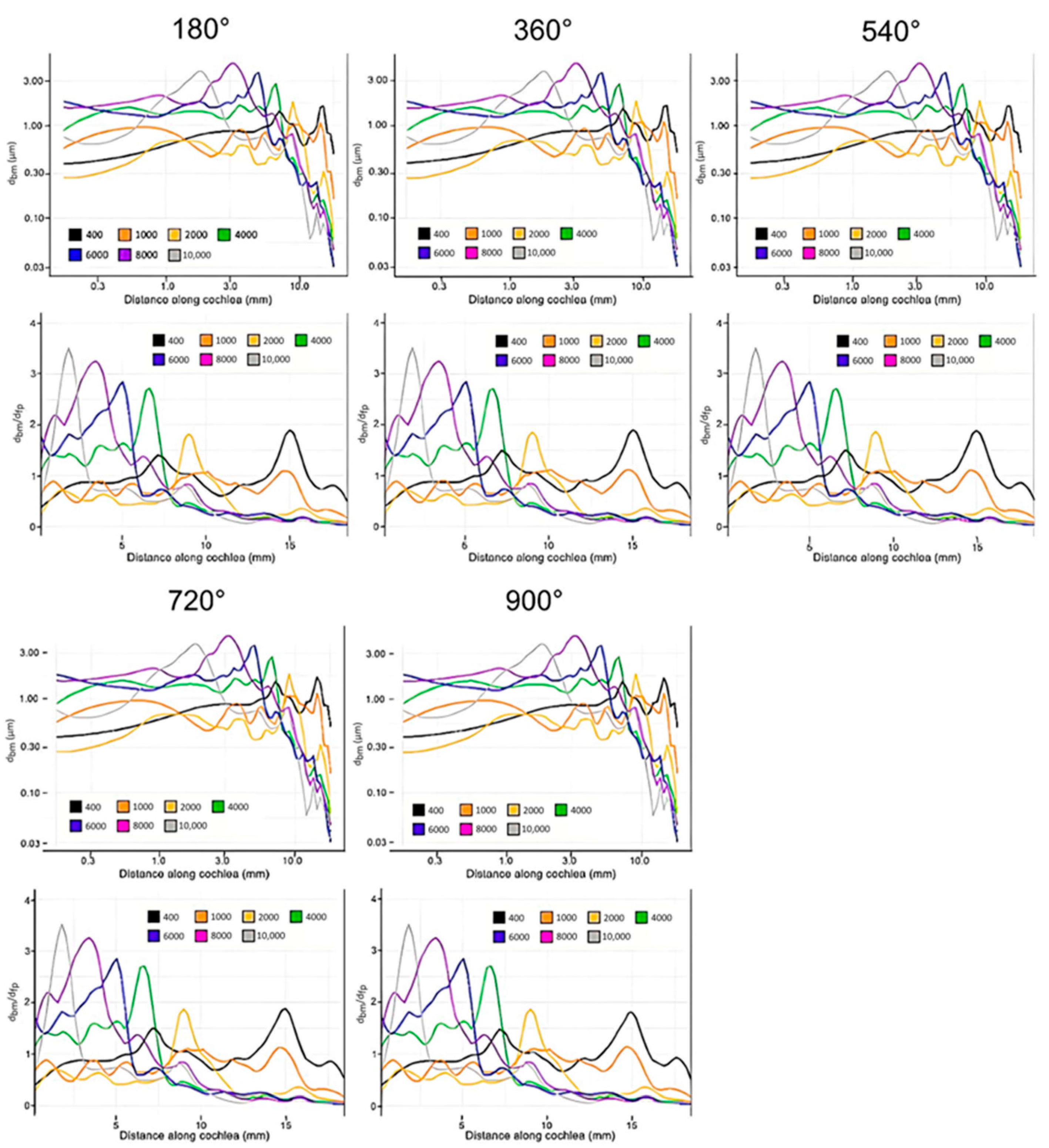
3. Results
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- World Health Organization Deafness and Hearing Loss. Available online: https://www.who.int/news-room/fact-sheets/detail/deafness-and-hearing-loss (accessed on 1 January 2022).
- Olze, H.; Szczepek, A.J.; Haupt, H.; Förster, U.; Zirke, N.; Gräbel, S.; Mazurek, B. Cochlear Implantation Has a Positive Influence on Quality of Life, Tinnitus, and Psychological Comorbidity. Laryngoscope 2011, 121, 2220–2227. [Google Scholar] [CrossRef]
- Sousa, A.F.D.; Couto, M.I.V.; Martinho-Carvalho, A.C. Quality of Life and Cochlear Implant: Results in Adults with Postlingual Hearing Loss. Braz. J. Otorhinolaryngol. 2018, 84, 494–499. [Google Scholar] [CrossRef]
- Hofmann, M.; Meloche, M.; Zwolan, T.A. Health Related Quality of Life in Adolescent Cochlear Implant Users. Cochlear Implant. Int. 2020, 21, 198–205. [Google Scholar] [CrossRef]
- Issing, C.; Baumann, U.; Pantel, J.; Stöver, T. Cochlear Implant Therapy Improves the Quality of Life in Older Patients—A Prospective Evaluation Study. Otol. Neurotol. 2020, 41, 1214–1221. [Google Scholar] [CrossRef]
- McRackan, T.R.; Hand, B.N.; Velozo, C.A.; Dubno, J.R. Association of Demographic and Hearing-Related Factors with Cochlear Implant–Related Quality of Life. JAMA Otolaryngol. Head Neck Surg. 2019, 145, 422–430. [Google Scholar] [CrossRef]
- O’Connell, B.P.; Cakir, A.; Hunter, J.B.; Francis, D.O.; Noble, J.H.; Labadie, R.F.; Zuniga, G.; Dawant, B.M.; Rivas, A.; Wanna, G.B. Electrode Location and Angular Insertion Depth Are Predictors of Audiologic Outcomes in Cochlear Implantation. Otol. Neurotol. Off. Publ. Am. Otol. Soc. Am. Neurotol. Soc. Eur. Acad. Otol. Neurotol. 2016, 37, 1016–1023. [Google Scholar] [CrossRef]
- Dhanasingh, A. The Rationale for FLEX (Cochlear Implant) Electrode with Varying Array Lengths. World J. Otorhinolaryngol. Head Neck Surg. 2021, 7, 45–53. [Google Scholar] [CrossRef]
- Wanna, G.B.; Noble, J.H.; Gifford, R.H.; Dietrich, M.S.; Sweeney, A.D.; Zhang, D.; Dawant, B.M.; Rivas, A.; Labadie, R.F. Impact of Intrascalar Electrode Location, Electrode Type, and Angular Insertion Depth on Residual Hearing in Cochlear Implant Patients. Otol. Neurotol. 2015, 36, 1343–1348. [Google Scholar] [CrossRef]
- Bruce, I.A.; Todt, I. Hearing Preservation Cochlear Implant Surgery. Adv. Hear. Rehabil. 2018, 81, 66–73. [Google Scholar] [CrossRef]
- Ketterer, M.C.; Aschendorff, A.; Arndt, S.; Hassepass, F.; Wesarg, T.; Laszig, R.; Beck, R. The Influence of Cochlear Morphology on the Final Electrode Array Position. Eur. Arch. Oto-Rhino-Laryngol. 2017, 275, 385–394. [Google Scholar] [CrossRef]
- Roy, A.T.; Penninger, R.T.; Pearl, M.S.; Wuerfel, W.; Jiradejvong, P.; Carver, C.; Buechner, A.; Limb, C.J. Deeper Cochlear Implant Electrode Insertion Angle Improves Detection of Musical Sound Quality Deterioration Related to Bass Frequency Removal. Otol. Neurotol. 2016, 37, 146–151. [Google Scholar] [CrossRef] [PubMed]
- Vibert, R.; Häusler, M.; Kompis, M.; Vischer, D. Vestibular Function in Patients with Cochlear Implantation. Acta Oto-Laryngol. 2001, 121, 29–34. [Google Scholar] [CrossRef] [PubMed]
- Ferdowsian, H.M.; Beck, N. Ethical and scientific considerations regarding animal testing and research. PLoS ONE 2011, 6, e24059. [Google Scholar] [CrossRef] [PubMed]
- Bottini, A.A.; Hartung, T. Food for thought… on the economics of animal testing. ALTEX 2009, 26, 3–16. [Google Scholar] [CrossRef] [PubMed]
- Doke, S.K.; Dhawale, S.C. Alternatives to animal testing: A review. Saudi Pharm. J. 2015, 23, 223–229. [Google Scholar] [CrossRef]
- Liebsch, M.; Grune, B.; Seiler, A.; Butzke, D.; Oelgeschläger, M.; Pirow, R.; Adler, S.; Riebeling, C.; Luch, A. Alternatives to animal testing: Current status and future perspectives. Arch. Toxicol. 2011, 85, 841–858. [Google Scholar] [CrossRef]
- Akhtar, A. The flaws and human harms of animal experimentation. Camb. Q. Healthc. Ethics 2015, 24, 407–419. [Google Scholar] [CrossRef]
- Areias, B.; Parente, M.P.L.; Gentil, F.; Natal Jorge, R.M. Finite element modelling of the surgical procedure for placement of a straight electrode array: Mechanical and clinical consequences. J. Biomech. 2021, 129, 110812. [Google Scholar] [CrossRef]
- Lim, J.; Kim, Y.; Kim, N. Mechanical Effects of Cochlear Implants on Residual Hearing Loss: A Finite Element Analysis. IEEE Trans. Biomed. Eng. 2020, 67, 3253–3261. [Google Scholar] [CrossRef]
- Ren, L.J.; Yu, Y.; Zhang, Y.H.; Liu, X.D.; Sun, Z.J.; Yao, W.J.; Zhang, T.Y.; Wang, C.; Li, C.L. Three-dimensional finite element analysis on cochlear implantation electrode insertion. Biomech. Model. Mechanobiol. 2022, 22, 467–478. [Google Scholar] [CrossRef]
- Vrettakos, P.A.; Dear, S.P.; Saunders, J.C. Middle Ear Structure in the Chinchilla: A Quantitative Study. Am. J. Otolaryngol. 1988, 9, 58–67. [Google Scholar] [CrossRef]
- Rosowski, J.J.; Ravicz, M.E.; Songer, J.E. Structures That Contribute to Middle-Ear Admittance in Chinchilla. J. Comp. Physiol. A 2006, 192, 1287–1311. [Google Scholar] [CrossRef] [PubMed]
- Trevino, M.; Lobarinas, E.; Maulden, A.C.; Heinz, M.G. The Chinchilla Animal Model for Hearing Science and Noise-Induced Hearing Loss. J. Acoust. Soc. Am. 2019, 146, 3710–3732. [Google Scholar] [CrossRef] [PubMed]
- Fedorov, A.; Beichel, R.; Kalpathy-Cramer, J.; Finet, J.; Fillion-Robin, J.-C.; Pujol, S.; Bauer, C.; Jennings, D.; Fennessy, F.; Sonka, M.; et al. 3D Slicer as an Image Computing Platform for the Quantitative Imaging Network. Magn. Reson. Imaging 2012, 30, 1323–1341. [Google Scholar] [CrossRef] [PubMed]
- Desai, S.S.; Zeh, C.; Lysakowski, A. Comparative Morphology of Rodent Vestibular Periphery. I. Saccular and Utricular Maculae. J. Neurophysiol. 2005, 93, 251–266. [Google Scholar] [CrossRef]
- Lo, W.W.; Daniels, D.L.; Chakeres, D.W.; Linthicum, F.H.; Ulmer, J.L.; Mark, L.P.; Swartz, J.D. The Endolymphatic Duct and Sac. AJNR Am. J. Neuroradiol. 1997, 18, 881–887. [Google Scholar] [PubMed]
- Simon, F.; Guichard, J.-P.; Kania, R.; Franc, J.; Herman, P.; Hautefort, C. Saccular Measurements in Routine MRI Can Predict Hydrops in Menière’s Disease. Eur. Arch. Oto-Rhino-Laryngol. 2017, 274, 4113–4120. [Google Scholar] [CrossRef]
- Goldberg, J.M.; Baird, R.A.; Fernández, C. Morphophysiological Studies of the Mammalian Vestibular Labyrinth. Prog. Clin. Biol. Res. 1985, 176, 231–245. [Google Scholar]
- Rabbitt, R.D.; Breneman, K.D.; King, C.; Yamauchi, A.M.; Boyle, R.; Highstein, S.M. Dynamic Displacement of Normal and Detached Semicircular Canal Cupula. J. Assoc. Res. Otolaryngol. 2009, 10, 497–509. [Google Scholar] [CrossRef]
- Wu, X.; Yu, S.; Liu, W.; Shen, S. Numerical Modeling and Verification by Nystagmus Slow-Phase Velocity of the Function of Semicircular Canals. Biomech. Model. Mechanobiol. 2020, 19, 2343–2356. [Google Scholar] [CrossRef]
- Iversen, M.M.; Rabbitt, R.D. Wave Mechanics of the Vestibular Semicircular Canals. Biophys. J. 2017, 113, 1133–1149. [Google Scholar] [CrossRef] [PubMed]
- Wu, X.; Yu, S.; Shen, S.; Liu, W. Exploring the Biomechanical Responses of Human Cupula by Numerical Analysis of Temperature Experiments. Sci. Rep. 2021, 11, 8208. [Google Scholar] [CrossRef] [PubMed]
- Kondrachuk, A.V. Finite element modeling of the 3D otolith structure. J. Vestib. Res. 2001, 11, 13–32. [Google Scholar] [CrossRef] [PubMed]
- Rabbitt, R.D.; Damiano, E.R.; Grant, J.W. Biomechanics of the Semicircular Canals and Otolith Organs. Vestib. Syst. 2004, 19, 153–201. [Google Scholar] [CrossRef]
- Takano, S.; Iguchi, H.; Sakamoto, H.; Yamane, H.; Anniko, M. Blockage Pattern of Longitudinal Flow in Meniere’s Disease. Acta Oto-Laryngol. 2013, 133, 692–698. [Google Scholar] [CrossRef] [PubMed]
- Yamane, H.; Sunami, K.; Iguchi, H.; Sakamoto, H.; Imoto, T.; Rask-Andersen, H. Assessment of Meniere’s Disease from a Radiological Aspect—Saccular Otoconia as a Cause of Meniere’s Disease? Acta Oto-Laryngol. 2012, 132, 1054–1060. [Google Scholar] [CrossRef]
- Lim, D.J. Functional structure of the organ of Corti: A review. Hear. Res. 1986, 22, 117–146. [Google Scholar] [CrossRef]
- Bohne, B.A.; Carr, C.D. Location of Structurally Similar Areas in Chinchilla Cochleas of Different Lengths. J. Acoust. Soc. Am. 1979, 66, 411–414. [Google Scholar] [CrossRef]
- Santi, P.; Mancini, P. Cochlear Anatomy and Central Auditory Pathways. Otolaryngol. Head Neck Surg. 2005, 3, 2803-26. [Google Scholar]
- Liang, J.; Ke, Z.; Welch, P.V.; Gan, R.Z.; Dai, C. A Comprehensive Finite Element Model for Studying Cochlear-Vestibular Interaction. Comput. Methods Biomech. Biomed. Eng. 2021, 25, 204–214. [Google Scholar] [CrossRef]
- Gan, R.Z.; Reeves, B.P.; Wang, X. Modeling of Sound Transmission from Ear Canal to Cochlea. Ann. Biomed. Eng. 2007, 35, 2180–2195. [Google Scholar] [CrossRef] [PubMed]
- Wang, M.; Gao, J.; Wang, X. High-Quality Mesh Generation for Human Hip Based on Ideal Element Size: Methods and Evaluation. Comput. Assist. Surg. 2017, 22, 212–220. [Google Scholar] [CrossRef] [PubMed]
- Zhang, X.; Gan, R.Z. Dynamic Properties of Human Round Window Membrane in Auditory Frequencies. Med. Eng. Phys. 2013, 35, 310–318. [Google Scholar] [CrossRef] [PubMed]
- Gan, R.Z.; Nakmali, D.; Zhang, X. Dynamic Properties of Round Window Membrane in Guinea Pig Otitis Media Model Measured with Electromagnetic Stimulation. Hear. Res. 2013, 301, 125–136. [Google Scholar] [CrossRef]
- Naidu, R.C.; Mountain, D.C. Longitudinal Coupling in the Basilar Membrane. J. Assoc. Res. Otolaryngol. 2001, 2, 257–267. [Google Scholar] [CrossRef]
- Rotaru, I.; Bujoreanu, C.; Bele, A.; Cazacu, M.; Olaru, D. Experimental Testing on Free Vibration Behaviour for Silicone Rubbers Proposed within Lumbar Disc Prosthesis. Mater. Sci. Eng. C 2014, 42, 192–198. [Google Scholar] [CrossRef]
- Shen, S.; Liu, Y.; Sun, X.; Zhao, W.; Su, Y.; Yu, S.; Liu, W. A Biomechanical Model of the Inner Ear: Numerical Simulation of the Caloric Test. Sci. World J. 2013, 2013, 160205. [Google Scholar] [CrossRef]
- Wang, X.; Gan, R.Z. 3D Finite Element Model of the Chinchilla Ear for Characterizing Middle Ear Functions. Biomech. Model. Mechanobiol. 2016, 15, 1263–1277. [Google Scholar] [CrossRef]
- Ulfendahl, M. Mechanical Responses of the Mammalian Cochlea. Prog. Neurobiol. 1997, 53, 331–380. [Google Scholar] [CrossRef]
- Zhang, X.; Gan, R.Z. A Comprehensive Model of Human Ear for Analysis of Implantable Hearing Devices. IEEE Trans. Biomed. Eng. 2011, 58, 3024–3027. [Google Scholar] [CrossRef]
- Dhanasingh, A.; Jolly, C. An Overview of Cochlear Implant Electrode Array Designs. Hear. Res. 2017, 356, 93–103. [Google Scholar] [CrossRef] [PubMed]
- Landsberger, D.M.; Svrakic, M.; Roland, J.T.; Svirsky, M. The Relationship between Insertion Angles, Default Frequency Allocations, and Spiral Ganglion Place Pitch in Cochlear Implants. Ear Hear. 2015, 36, e207–e213. [Google Scholar] [CrossRef] [PubMed]
- Greenwood, D.D. A Cochlear Frequency-Position Function for Several Species—29 Years Later. J. Acoust. Soc. Am. 1990, 87, 2592–2605. [Google Scholar] [CrossRef] [PubMed]
- Prodanovic, S.; Gracewski, S.M.; Nam, J.-H. Power Dissipation in the Cochlea Can Enhance Frequency Selectivity. Biophys. J. 2019, 116, 1362–1375. [Google Scholar] [CrossRef] [PubMed]
- Gautschi-Mills, K.; Khoza-Shangase, K.; Pillay, D. Preservation of Residual Hearing after Cochlear Implant Surgery: An Exploration of Residual Hearing Function in a Group of Recipients at Cochlear Implant Units. Braz. J. Otorhinolaryngol. 2019, 85, 310–318. [Google Scholar] [CrossRef] [PubMed]
- Iso-Mustajärvi, M.; Sipari, S.; Löppönen, H.; Dietz, A. Preservation of Residual Hearing after Cochlear Implant Surgery with Slim Modiolar Electrode. Eur. Arch. Oto-Rhino-Laryngol. 2019, 277, 367–375. [Google Scholar] [CrossRef]


| EL | Endolymph | UM | Utricular Macula |
| PL | Perilymph | SM | Saccular Macula |
| BM | Basilar Membrane | SCC | Semicircular Canal |
| RM | Reissner’s Membrane | AC | Anterior Semicircular Canal |
| OSL | Osseous Spiral Lamina | PC | Posterior Semicircular Canal |
| HT | Helicotrema | LC | Lateral Semicircular Canal |
| RD | Reuniting Duct | CAC | Cupula of the AC |
| ML | Membranous Labyrinth | CPC | Cupula of the PC |
| RWM | Round Window Membrane | CLC | Cupula of the LC |
| OWM | Oval Window Membrane | CI | Cochlear Implant |
| U | Utricle | CIE | Cochlear Implant Electrode |
| S | Saccule | CIS | Cochlear Implant Surgery |
| SCC | Semicircular Canal |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Castle, N.; Liang, J.; Smith, M.; Petersen, B.; Matson, C.; Eldridge, T.; Zhang, K.; Lee, C.-H.; Liu, Y.; Dai, C. Finite Element Modeling of Residual Hearing after Cochlear Implant Surgery in Chinchillas. Bioengineering 2023, 10, 539. https://doi.org/10.3390/bioengineering10050539
Castle N, Liang J, Smith M, Petersen B, Matson C, Eldridge T, Zhang K, Lee C-H, Liu Y, Dai C. Finite Element Modeling of Residual Hearing after Cochlear Implant Surgery in Chinchillas. Bioengineering. 2023; 10(5):539. https://doi.org/10.3390/bioengineering10050539
Chicago/Turabian StyleCastle, Nicholas, Junfeng Liang, Matthew Smith, Brett Petersen, Cayman Matson, Tara Eldridge, Ke Zhang, Chung-Hao Lee, Yingtao Liu, and Chenkai Dai. 2023. "Finite Element Modeling of Residual Hearing after Cochlear Implant Surgery in Chinchillas" Bioengineering 10, no. 5: 539. https://doi.org/10.3390/bioengineering10050539
APA StyleCastle, N., Liang, J., Smith, M., Petersen, B., Matson, C., Eldridge, T., Zhang, K., Lee, C.-H., Liu, Y., & Dai, C. (2023). Finite Element Modeling of Residual Hearing after Cochlear Implant Surgery in Chinchillas. Bioengineering, 10(5), 539. https://doi.org/10.3390/bioengineering10050539








