Straightforward Magnetic Resonance Temperature Measurements Combined with High Frame Rate and Magnetic Susceptibility Correction
Abstract
1. Introduction
2. Materials and Methods
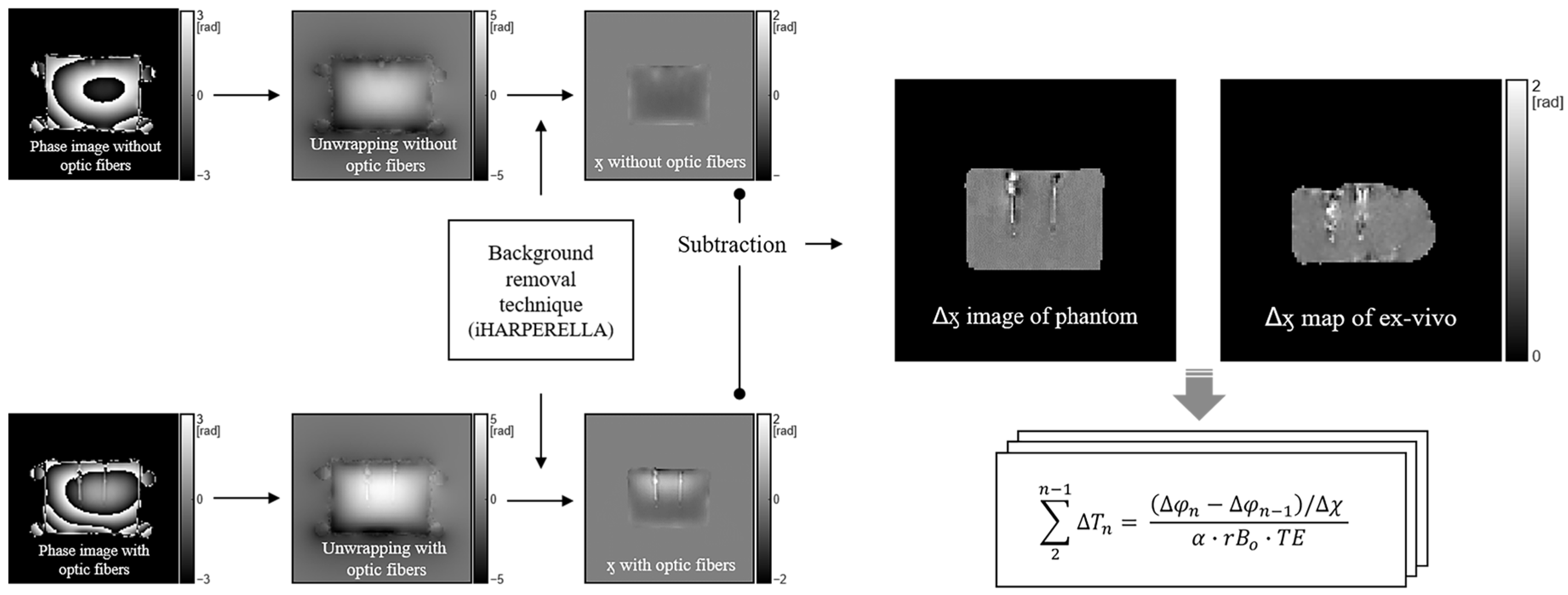
2.1. Calculation of the Susceptibility-Corrected Value of PRFS
2.2. Phantom and Ex Vivo Experiment for Temperature Reading of PRFS
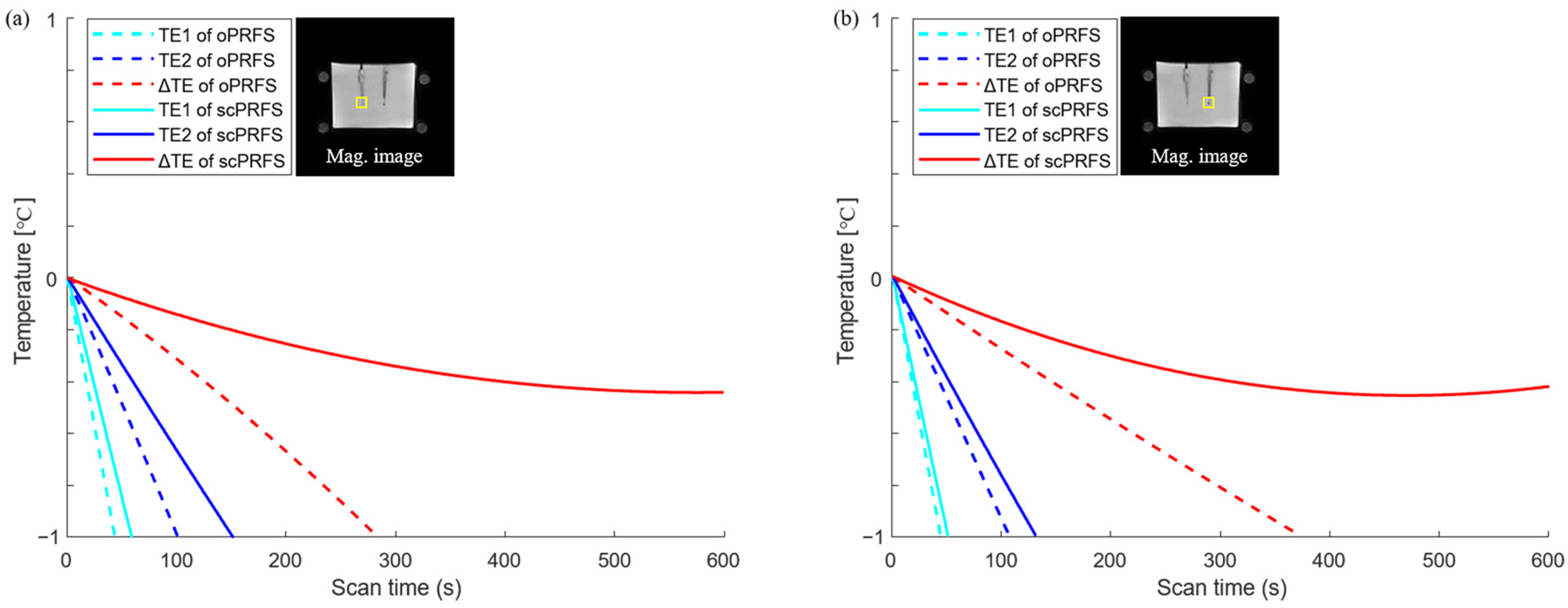
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Winter, L.; Oberacker, E.; Paul, K.; Ji, Y.; Oezerdem, C.; Ghadjar, P.; Thieme, A.; Budach, V.; Wust, P.; Niendorf, T. Magnetic Resonance Thermometry: Methodology, Pitfalls and Practical Solutions. Int. J. Hyperth. 2016, 32, 63–75. [Google Scholar] [CrossRef] [PubMed]
- Bing, C.; Staruch, R.M.; Tillander, M.; Köhler, M.O.; Mougenot, C.; Ylihautala, M.; Laetsch, T.W.; Chopra, R. Drift Correction for Accurate PRF-Shift MR Thermometry During Mild Hyperthermia Treatments with MR-HIFU. Int. J. Hyperth. 2016, 32, 673–687. [Google Scholar] [CrossRef]
- Zhu, M.; Sun, Z.; Ng, C.K. Image-Guided Thermal Ablation with MR-Based Thermometry. Quant. Imaging Med. Surg. 2017, 7, 356–368. [Google Scholar] [CrossRef] [PubMed]
- Falk, M.H.; Issels, R.D. Hyperthermia in Oncology. Int. J. Hyperth. 2001, 17, 1–18. [Google Scholar] [CrossRef] [PubMed]
- Wust, P.; Hildebrandt, B.; Sreenivasa, G.; Rau, B.; Gellermann, J.; Riess, H.; Felix, R.; Schlag, P.M. Hyperthermia in Combined Treatment of Cancer. Lancet Oncol. 2002, 3, 487–497. [Google Scholar] [CrossRef] [PubMed]
- Behrouzkia, Z.; Joveini, Z.; Keshavarzi, B.; Eyvazzadeh, N.; Aghdam, R.Z. Hyperthermia: How Can It Be Used? Oman Med. J. 2016, 31, 89–97. [Google Scholar] [CrossRef]
- Van Rhoon, G.C.; Wust, P. Introduction: Non-invasive Thermometry for Thermotherapy. Int. J. Hyperth. 2005, 21, 489–495. [Google Scholar] [CrossRef]
- Kok, H.P.; Cressman, E.N.K.; Ceelen, W.; Brace, C.L.; Ivkov, R.; Grüll, H.; Ter Haar, G.; Wust, P.; Crezee, J. Heating Technology for Malignant Tumors: A Review. Int. J. Hyperth. 2020, 37, 711–741. [Google Scholar] [CrossRef]
- Lyon, P.C.; Mannaris, C.; Gray, M.; Carlisle, R.; Gleeson, F.V.; Cranston, D.; Wu, F.; Coussios, C.C. Large-Volume Hyperthermia for Safe and Cost-Effective Targeted Drug Delivery Using a Clinical Ultrasound-Guided Focused Ultrasound Device. Ultrasound Med. Biol. 2021, 47, 982–997. [Google Scholar] [CrossRef]
- Hergt, R.; Andra, W.; d’Ambly, C.G.; Hilger, I.; Kaiser, W.A.; Richter, U.; Schmidt, H.-G. Physical Limits of Hyperthermia Using Magnetite Fine Particles. IEEE Trans. Magn. 1998, 34, 3745–3754. [Google Scholar] [CrossRef]
- Rieke, V.; Butts-Pauly, K. MR Thermometry. J. Magn. Reson. Imaging 2008, 27, 376–390. [Google Scholar] [CrossRef]
- Yuan, J.; Mei, C.S.; Panych, L.P.; McDannold, N.J.; Madore, B. Towards Fast and Accurate Temperature Mapping with Proton Resonance Frequency-Based MR Thermometry. Quant. Imaging Med. Surg. 2012, 2, 21–32. [Google Scholar] [CrossRef]
- Busch, J.; Vannesjo, S.J.; Barmet, C.; Pruessmann, K.P.; Kozerke, S. Analysis of temperature dependence of background phase errors in phase-contrast cardiovascular magnetic resonance. J. Cardiovasc. Magn. Reson. 2014, 16, 97. [Google Scholar] [CrossRef] [PubMed]
- El-Sharkawy, A.M.; Schär, M.; Bottomley, P.A.; Atalar, E. Monitoring and Correcting Spatio-temporal Variations of the MR Scanner’s Static Magnetic Field. Magn. Reson. Mater. Phys. Biol. Med. 2006, 19, 223–236. [Google Scholar] [CrossRef]
- Hernandez, D.; Kim, K.S.; Michel, E.; Lee, S.Y. Correction of B0 drift effects in magnetic resonance thermometry using magnetic field monitoring technique. Concepts Magn. Reson. Part B Magn. Reson. Eng. 2016, 46, 81–89. [Google Scholar] [CrossRef]
- Sprinkhuizen, S.M.; Konings, M.K.; van der Bom, M.J.; Viergever, M.A.; Bakker, C.J.; Bartels, L.W. Temperature-Induced Tissue Susceptibility Changes Lead to Significant Temperature Errors in PRFS-Based MR Thermometry During Thermal Interventions. Magn. Reson. Med. 2010, 64, 1360–1372. [Google Scholar] [CrossRef] [PubMed]
- Boss, A.; Graf, H.; Müller-Bierl, B.; Clasen, S.; Schmidt, D.; Pereira, P.L.; Schick, F. Magnetic Susceptibility Effects on the Accuracy of MR Temperature Monitoring by the Proton Resonance Frequency Method. J. Magn. Reson. Imaging 2005, 22, 813–820. [Google Scholar] [CrossRef]
- De Landro, M.; Giraudeau, C.; Verde, J.; Ambarki, K.; Korganbayev, S.; Wolf, A.; Odéen, H.; Saccomandi, P. Characterization of Susceptibility Artifacts in Magnetic Resonance Thermometry Images During Laser Interstitial Thermal Therapy: Dimension Analysis and Temperature Error Estimation. Phys. Med. Biol. 2023, 68, 085022. [Google Scholar] [CrossRef]
- Winter, L.; Oberacker, E.; Özerdem, C.; Ji, Y.; von Knobelsdorff-Brenkenhoff, F.; Weidemann, G.; Ittermann, B.; Seifert, F.; Niendorf, T. On the RF Heating of Coronary Stents at 7.0 Tesla MRI. Magn. Reson. Med. 2015, 74, 999–1010. [Google Scholar] [CrossRef]
- Santoro, D.; Winter, L.; Müller, A.; Vogt, J.; Renz, W.; Özerdem, C.; Grässl, A.; Tkachenko, V.; Schulz-Menger, J.; Niendorf, T. Detailing Radio Frequency Heating Induced by Coronary Stents: A 7.0 Tesla Magnetic Resonance Study. PLoS ONE 2012, 7, e49963. [Google Scholar] [CrossRef][Green Version]
- Kickhefel, A.; Weiss, C.; Roland, J.; Gross, P.; Schick, F.; Salomir, R. Correction of Susceptibility-Induced GRE Phase Shift for Accurate PRFS Thermometry Proximal to Cryoablation Iceball. Magn. Reson. Mater. Phys. Biol. Med. 2012, 25, 23–31. [Google Scholar] [CrossRef] [PubMed]
- Hensen, B.; Hellms, S.; Werlein, C.; Jonigk, D.; Gronski, P.A.; Bruesch, I.; Rumpel, R.; Wittauer, E.M.; Vondran, F.W.R.; Parker, D.L.; et al. Correction of Heat-Induced Susceptibility Changes in Respiratory-Triggered 2D-PRF-Based Thermometry for Monitoring of Magnetic Resonance-Guided Hepatic Microwave Ablation in a Human-like In Vivo Porcine Model. Int. J. Hyperth. 2022, 39, 1387–1396. [Google Scholar] [CrossRef] [PubMed]
- Boehm, C.; Goeger-Neff, M.; Mulder, H.T.; Zilles, B.; Lindner, L.H.; van Rhoon, G.C.; Karampinos, D.C.; Wu, M. Susceptibility Artifact Correction in MR Thermometry for Monitoring of Mild Radiofrequency Hyperthermia Using Total Field Inversion. Magn. Reson. Med. 2022, 88, 120–132. [Google Scholar] [CrossRef] [PubMed]
- Wu, M.; Mulder, H.T.; Baron, P.; Coello, E.; Menzel, M.I.; van Rhoon, G.C.; Haase, A. Correction of Motion-Induced Susceptibility Artifacts and B0 Drift During Proton Resonance Frequency Shift-Based MR Thermometry in the Pelvis with Background Field Removal Methods. Magn. Reson. Med. 2020, 84, 2495–2511. [Google Scholar] [CrossRef] [PubMed]
- Dadakova, T.; Gellermann, J.; Voigt, O.; Korvink, J.G.; Pavlina, J.M.; Hennig, J.; Bock, M. Fast PRF-Based MR Thermometry Using Double-Echo EPI: In Vivo Comparison in a Clinical Hyperthermia Setting. Magn. Reson. Mater. Phys. Biol. Med. 2015, 28, 305–314. [Google Scholar] [CrossRef]
- Yamato, M.; Kimura, T. Magnetic Processing of Diamagnetic Materials. Polymers 2020, 12, 1491. [Google Scholar] [CrossRef]
- Schena, E.; Tosi, D.; Saccomandi, P.; Lewis, E.; Kim, T. Fiber optic sensors for temperature monitoring during thermal treatments: An overview. Sensors 2016, 16, 1144. [Google Scholar] [CrossRef]
- Li, W.; Avram, A.V.; Wu, B.; Xiao, X.; Liu, C. Integrated Laplacian-Based Phase Unwrapping and Background Phase Removal for Quantitative Susceptibility Mapping. NMR Biomed. 2014, 27, 219–227. [Google Scholar] [CrossRef]
- Haacke, E.M.; Liu, S.; Buch, S.; Zheng, W.; Wu, D.; Ye, Y. Quantitative Susceptibility Mapping: Current Status and Future Directions. Magn. Reson. Imaging 2015, 33, 1–25. [Google Scholar] [CrossRef]
- Oh, S.; Ryu, Y.C.; Carluccio, G.; Sica, C.T.; Collins, C.M. Measurement of SAR-Induced Temperature Increase in a Phantom and In Vivo with Comparison to Numerical Simulation. Magn. Reson. Med. 2014, 71, 1923–1931. [Google Scholar] [CrossRef]
- Oh, S.; Webb, A.G.; Neuberger, T.; Park, B.; Collins, C.M. Experimental and Numerical Assessment of MRI-Induced Temperature Change and SAR Distributions in Phantoms and In Vivo. Magn. Reson. Med. 2010, 63, 218–223. [Google Scholar] [CrossRef] [PubMed]
- Smith, S.M. Fast Robust Automated Brain Extraction. Hum. Brain Mapp. 2002, 17, 143–155. [Google Scholar] [CrossRef] [PubMed]
- Robinson, S.; Jovicich, J. B0 Mapping with Multi-channel RF Coils at High Field. Magn. Reson. Med. 2011, 66, 976–988. [Google Scholar] [CrossRef]
- Robinson, S.; Grabner, G.; Witoszynskyj, S.; Trattnig, S. Combining Phase Images from Multi-channel RF Coils Using 3D Phase Offset Maps Derived from a Dual-Echo Scan. Magn. Reson. Med. 2011, 65, 1638–1648. [Google Scholar] [CrossRef] [PubMed]
- Ferrer, C.J.; Bartels, L.W.; van der Velden, T.A.; Grüll, H.; Heijman, E.; Moonen, C.T.W.; Bos, C. Field Drift Correction of Proton Resonance Frequency Shift Temperature Mapping with Multichannel Fast Alternating Nonselective Free Induction Decay Readouts. Magn. Reson. Med. 2020, 83, 962–973. [Google Scholar] [CrossRef]
- Jenkinson, M.; Smith, S. A Global Optimisation Method for Robust Affine Registration of Brain Images. Med. Image Anal. 2001, 5, 143–156. [Google Scholar] [CrossRef]
- Jenkinson, M.; Bannister, P.; Brady, M.; Smith, S. Improved Optimization for the Robust and Accurate Linear Registration and Motion Correction of Brain Images. Neuroimage 2002, 17, 825–841. [Google Scholar] [CrossRef]
- McDannold, N. Quantitative MRI-Based Temperature Mapping Based on the Proton Resonant Frequency Shift: Review of Validation Studies. Int. J. Hyperth. 2005, 21, 533–546. [Google Scholar] [CrossRef]
- Liu, C.; Wei, H.; Gong, N.J.; Cronin, M.; Dibb, R.; Decker, K. Quantitative Susceptibility Mapping: Contrast Mechanisms and Clinical Applications. Tomography 2015, 1, 3–17. [Google Scholar] [CrossRef]
- Wang, Y.; Liu, T. Quantitative Susceptibility Mapping (QSM): Decoding MRI Data for a Tissue Magnetic Biomarker. Magn. Reson. Med. 2015, 73, 82–101. [Google Scholar] [CrossRef]
- Haacke, E.M.; Xu, Y.; Cheng, Y.C.N.; Reichenbach, J.R. Susceptibility Weighted Imaging (SWI). Magn. Reson. Med. 2004, 52, 612–618. [Google Scholar] [CrossRef] [PubMed]
- Peters, R.T.; Hinks, R.S.; Henkelman, R.M. Ex vivo tissue-type independence in proton-resonance frequency shift MR thermometry. Magn. Reson. Med. 1998, 40, 454–459. [Google Scholar] [CrossRef] [PubMed]
- Odéen, H.; Parker, D.L. Magnetic Resonance Thermometry and Its Biological Applications–Physical Principles and Practical Considerations. Prog. Nucl. Magn. Reson. Spectrosc. 2019, 110, 34–61. [Google Scholar] [CrossRef] [PubMed]
- Bauer, T.; Steinmann, W.-D.; Laing, D.; Tamme, R. Thermal Energy Storage Materials and Systems. Annu. Rev. Heat Transf. 2012, 15, 131–177. [Google Scholar] [CrossRef]
- Lewis, M.J. Physical Properties of Foods and Food Processing Systems; Elsevier: Amsterdam, The Netherlands, 1990; pp. 246–251. [Google Scholar]
- Zhang, Z. Rock Fracture and Blasting: Theory and applications; Butterworth-Heinemann: Oxford, UK, 2016; pp. 111–113. [Google Scholar]
- Peters, R.D.; Henkelman, R.M. Proton-Resonance Frequency Shift MR Thermometry Is Affected by Changes in the Electrical Conductivity of Tissue. Magn. Reson. Med. 2000, 43, 62–71. [Google Scholar] [CrossRef]
- Stoy, R.D.; Foster, K.R.; Schwan, H.P. Dielectric Properties of Mammalian Tissues from 0.1 to 100 MHz; a Summary of Recent Data. Phys. Med. Biol. 1982, 27, 501–513. [Google Scholar] [CrossRef]
- Stafford, R.J.; Fuentes, D.; Elliott, A.A.; Weinberg, J.S.; Ahrar, K. Laser-Induced Thermal Therapy for Tumor Ablation. Crit. Rev. Biomed. Eng. 2010, 38, 79–100. [Google Scholar] [CrossRef]
- Ashraf, O.; Patel, N.V.; Hanft, S.; Danish, S.F. Laser-Induced Thermal Therapy in Neuro-oncology: A Review. World Neurosurg. 2018, 112, 166–177. [Google Scholar] [CrossRef]
- Thies, M.; Oelze, M.L. Combined Therapy Planning, Real-Time Monitoring, and Low Intensity Focused Ultrasound Treatment Using a Diagnostic Imaging Array. IEEE Trans. Med. Imaging 2022, 41, 1410–1419. [Google Scholar] [CrossRef]
- Rappaport, C. Treating Cardiac Disease with Catheter-Based Tissue Heating. IEEE Microw. Mag. 2002, 3, 57–64. [Google Scholar] [CrossRef]
- Paul, A.; Narasimhan, A.; Kahlen, F.J.; Das, S.K. Temperature Evolution in Tissues Embedded with Large Blood Vessels During Photo-thermal Heating. J. Therm. Biol. 2014, 41, 77–87. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Michaelis, T.; Frahm, J. Towards MRI Temperature Mapping in Real Time—The Proton Resonance Frequency Method with Undersampled Radial MRI and Nonlinear Inverse Reconstruction. Quant. Imaging Med. Surg. 2017, 7, 251–258. [Google Scholar] [CrossRef] [PubMed]
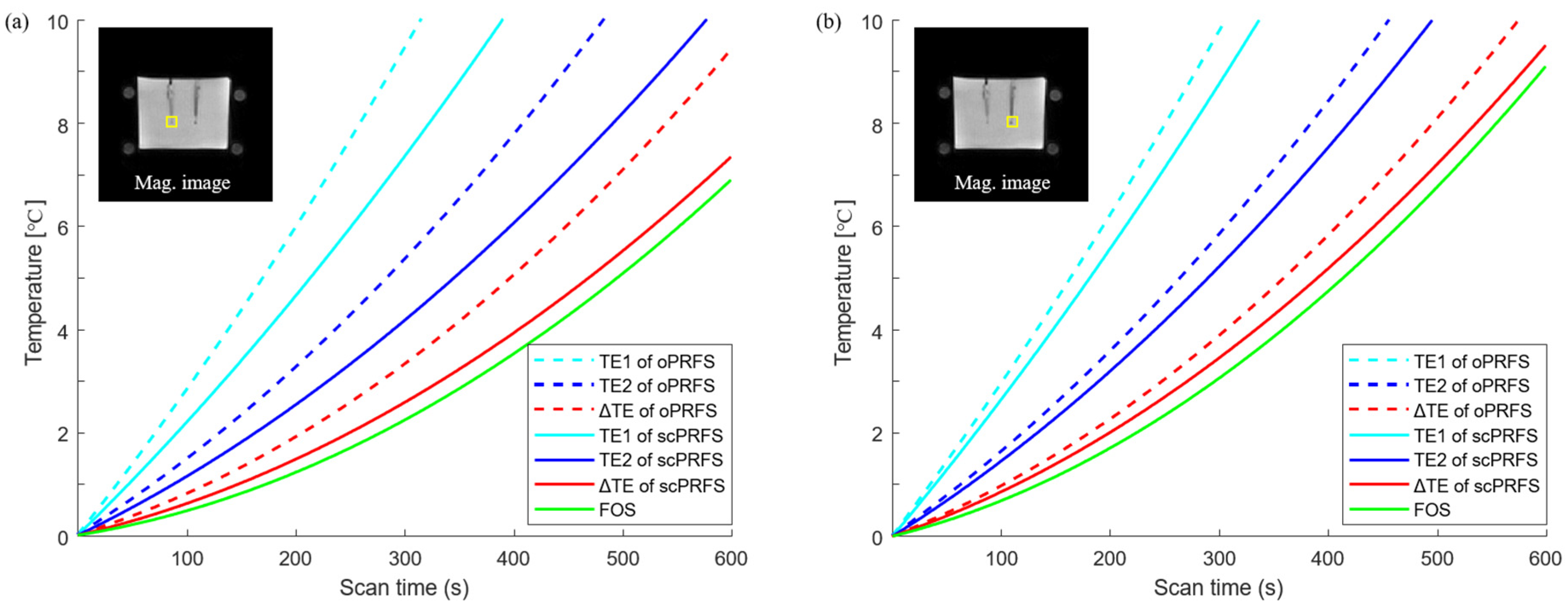

| Phantom | Individual Region #1 | Individual Region #2 | ||
| oPRFS (°C) | scPRFS (°C) | oPRFS (°C) | scPRFS (°C) | |
| TE1 | 21.73 | 17.08 | 22.15 | 20.10 |
| TE2 | 13.50 | 10.61 | 14.48 | 13.11 |
| ΔTE | 9.38 | 7.38 | 10.65 | 9.61 |
| Optic fiber [°C] | 6.88 | 9.09 | ||
| Ex vivo | Center region | Outer region | ||
| oPRFS (°C) | scPRFS (°C) | oPRFS (°C) | scPRFS (°C) | |
| TE1 | 5.02 | 5.73 | 3.22 | 2.58 |
| TE2 | 4.41 | 5.06 | 4.04 | 3.00 |
| ΔTE | 4.10 | 4.73 | 4.45 | 3.20 |
| Optic fiber (°C) | 4.76 | 3.02 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, S.; Kim, D.; Oh, S. Straightforward Magnetic Resonance Temperature Measurements Combined with High Frame Rate and Magnetic Susceptibility Correction. Bioengineering 2023, 10, 1299. https://doi.org/10.3390/bioengineering10111299
Kim S, Kim D, Oh S. Straightforward Magnetic Resonance Temperature Measurements Combined with High Frame Rate and Magnetic Susceptibility Correction. Bioengineering. 2023; 10(11):1299. https://doi.org/10.3390/bioengineering10111299
Chicago/Turabian StyleKim, Sangwoo, Donghyuk Kim, and Sukhoon Oh. 2023. "Straightforward Magnetic Resonance Temperature Measurements Combined with High Frame Rate and Magnetic Susceptibility Correction" Bioengineering 10, no. 11: 1299. https://doi.org/10.3390/bioengineering10111299
APA StyleKim, S., Kim, D., & Oh, S. (2023). Straightforward Magnetic Resonance Temperature Measurements Combined with High Frame Rate and Magnetic Susceptibility Correction. Bioengineering, 10(11), 1299. https://doi.org/10.3390/bioengineering10111299








