Hydrodynamic Modeling of Nokoué Lake in Benin
Abstract
:1. Introduction
2. Materials and Methods
2.1. Study Area
2.2. SMS Model Presentation
2.3. Model Building
2.3.1. Discretization of the Physical Space
2.3.2. Boundary and Initial Conditions
The Condition at the Upstream Limit: the Lake Tributaries Flows
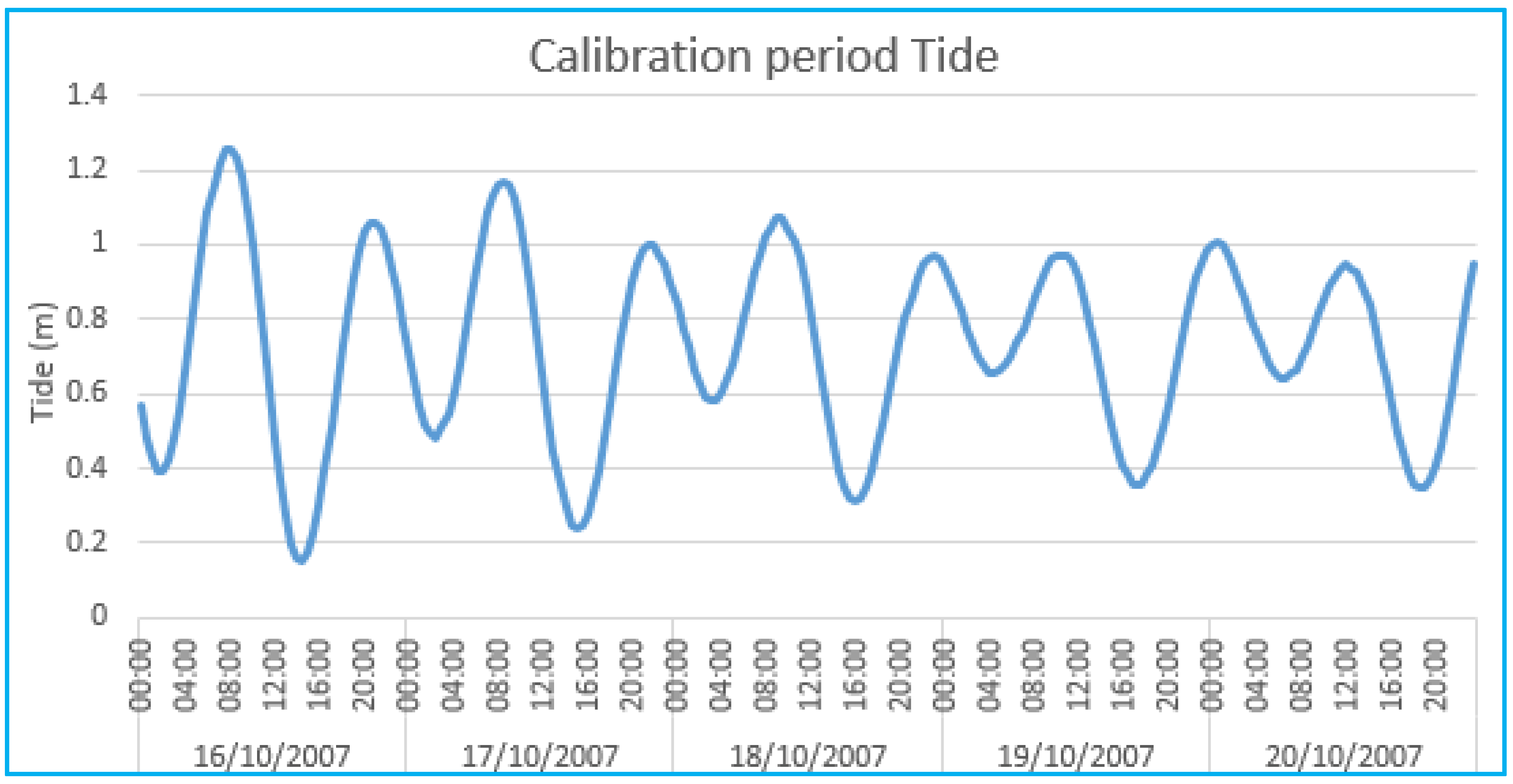
The Boundary Condition at the Downstream: The Tide
Condition at Upper Limit: Wind, Precipitation, and Evaporation
2.4. Calibration Parameters
- -
- The Manning roughness coefficient of the bottom (n), which translated the bottom friction;
- -
- The turbulent exchange coefficient (Eij), which reflects the turbulence and dispersion phenomena due to non-uniformity of velocity along the water level.
2.5. Model Performance Evaluation
2.5.1. The Root Mean Square Error (RMSE)
2.5.2. Coefficient of Determination R2
2.5.3. Correlation Coefficient, r
2.5.4. Nash-Sutcliffe Model Efficiency Coefficient (NSE)
3. Results and Discussion
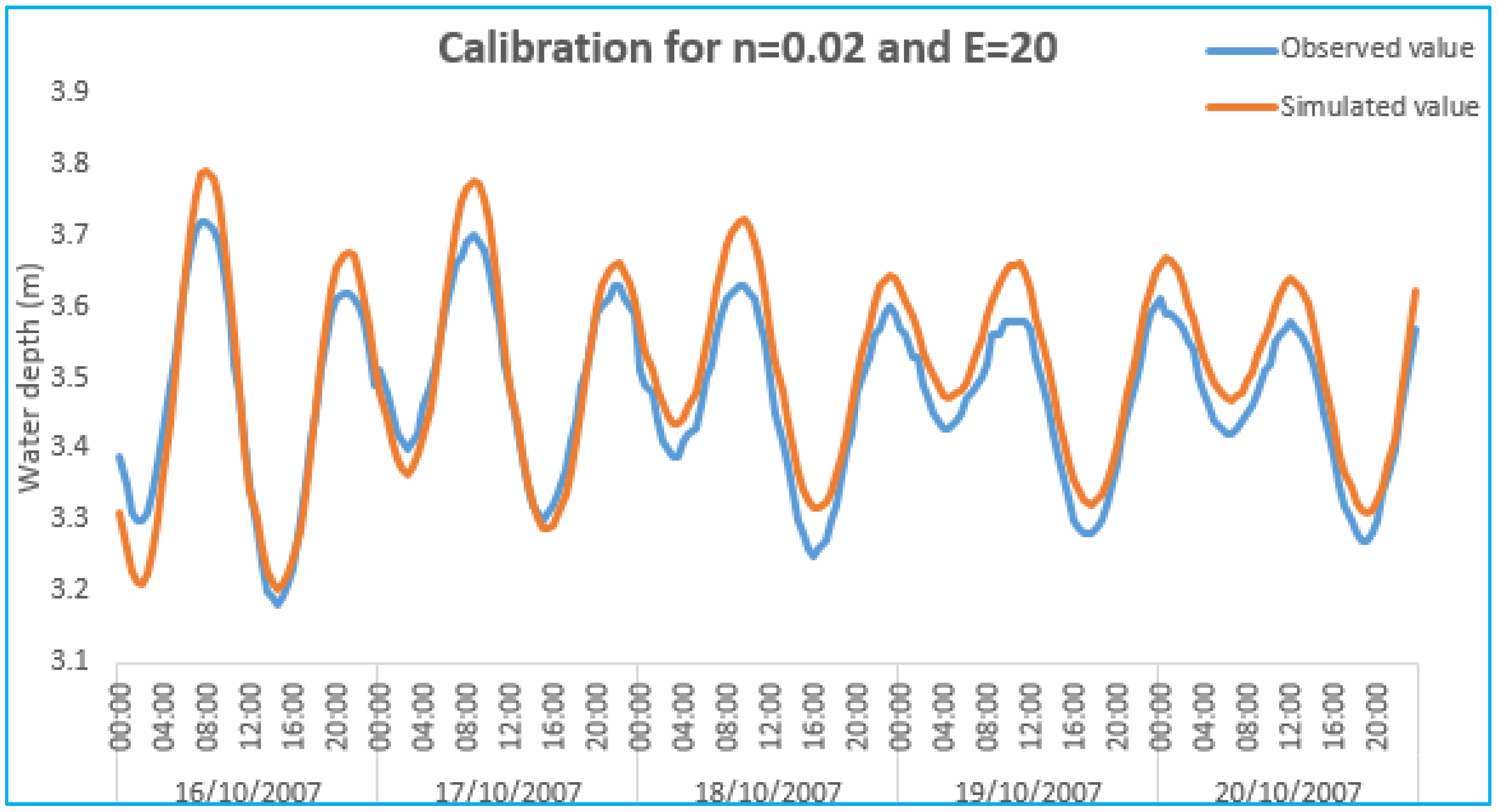
3.1. Model Calibration
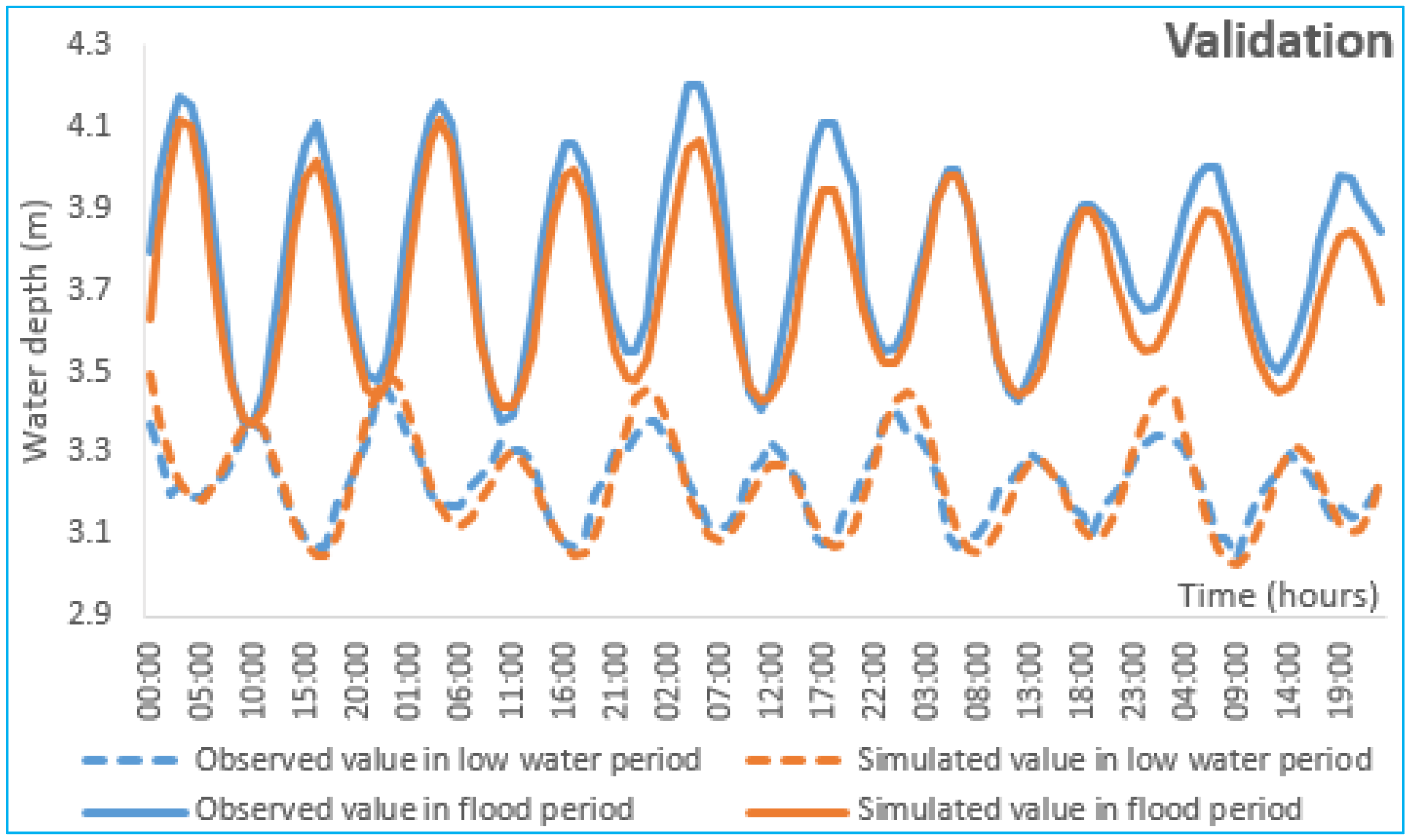
3.2. Validation
3.3. Hydrodynamic Functioning of the Lake
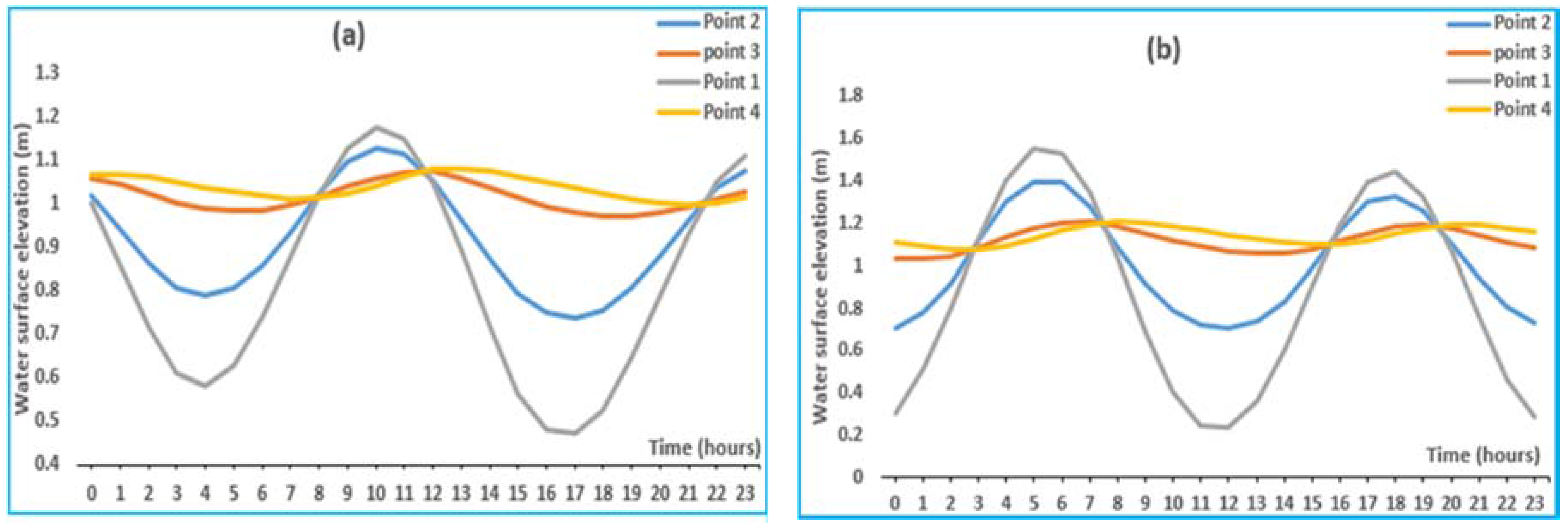
3.3.1. Variation of the Water Surface Level
3.3.2. Flow and Water Exchange Rates
3.3.3. Velocity Field on the Lake Complex
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Casini, M.; Mocenni, C.; Paoletti, S.; Pranzo, M. Model-based decision support for integrated management and control of coastal lagoons. In Proceedings of the European Control Conference, Kos, Greece, 2–5 July 2007; p. 8.
- Arfi, R.; Dufour, P.; Mayer, D. Phytoplancton et pollution: Premières études en baie de Biétry (Côte d’Ivoire). Traitement mathématique des données. Oceanol. Acta 1981, 4, 319–329. [Google Scholar]
- Zabi, G.S. Les peuplements benthiques lagunaires liés à la pollution en zone urbaine d’Abidjan (Côte d’Ivoire). In Proceedings of the Actes du Symposium International sur les lagunes côtières, Scor/Labo/Unesco, Bordeaux, France, 8–14 September 1981; pp. 441–455.
- Guiral, D.; Lanusse, A. Contribution à l’étude hydro-dynamique de la baie de Biétri, lagune Ebrié, Côte d’Ivoire. Doc. Sci. Cent. Rech. Océano. Abidj. 1984, 15, 1–18. [Google Scholar]
- Arfi, R.; Guiral, D. Un écosystème estuarien eutrophe: La baie de Biétri. In Environnement et Ressources Aquatiques de Côte d’Ivoire. T.II. Les milieux lagunaires; Durand, J.R., Dufour, P., Guiral, D., Zabi, S., Eds.; ORSTOM: Paris, France, 1994; pp. 59–90. [Google Scholar]
- French Research Institute for Exploitation of the Sea (IFREMER). Réseau de Suivi Lagunaire du Languedoc-Roussillon; Bilan des Résultats 2002; IFREMER: Issy-les-Moulineaux, France, 2003; p. 36.
- Moussa, M. Modélisation Dynamique des Milieux Lacustres et Marins, et Dispersion de Polluants. Cours de Mécanique des Fluides Environnementale; ENIT: Tunisie, 2013; 136p. [Google Scholar]
- Derolez, V.; Fiandrino, A.; Munaron, N. Bilan Sur Les Principales Pressions Pesant Sur Les Lagunes Méditerranéennes et Leur Lien Avec l’état DCE; IFREMER: Issy-les-Moulineaux, France, 2014; p. 46. [Google Scholar]
- Capo, S. Hydrodynamique et Dynamique Sédimentaire en Milieu Tropical de Mangrove, Observations et Modélisation de L’estuaire du Konkouré, République de Guinée. Ph.D. Thesis, de l’Université de Bordeaux en Géologie Marine, Océanographie, France, 2006; p. 260. [Google Scholar]
- Rezgui, A.; Ben Maiz, N.; Moussa, M. Modélisation du fonctionnement hydrodynamique et écologique du Lac Nord de Tunis. Revue des Sciences de l’Eau 2008, 21, 349–361. [Google Scholar] [CrossRef]
- Monde, S.; Coulibaly, A.S.; Wognin, V.A.I.; Brenon, I.; Aka, K. Hydrological modelling of a two-outlet tropical lagoon (Ebrié lagoon, Ivory Coast) during an exceptional flood of the Comoé river. J. Environ. Hydrol. 2011, 19, 13. [Google Scholar]
- Wango, T.E.; Moussa, M.; N’Guessan, Y.A.; Monde, S. Hydrodynamique du complexe lagunaire Grand-Lahou, Ebrié et Aby (Côte d’Ivoire): Impacts des forçages fluviaux et de la marée. Bull. Inst. Sci. Rabat Sect. Sci. Terre 2013, 35, 27–38. [Google Scholar]
- Hilmi, K.; Makaoui, A.; Idrissi, M.; Abdellaoui, B.; El Ouehabi, Z. Circulation marine de la lagune de nador (maroc) par modelisation hydrodynamique. Eur. Sci. J. 2015, 11, 32. [Google Scholar]
- Albaret, J.J. Les peuplements des estuaires et des lagunes. In Les Poissons Des Eaux Continentales Africaines: Diversité, Écologie, Utilisation par L’homme; Lévêque, C., Paugy, D., Eds.; Éditions de l’IRD: Paris, France, 1999; pp. 325–349. [Google Scholar]
- Dehotin, U.A.; Laleye, P.A.; Dauta, A.; Moreau, J. Facteurs écologiques et diversité piscicole d’une lagune Ouest Africaine: Le lac Nokoué au Bénin. J. Afrotrop. Zool. 2007, 49–55. [Google Scholar]
- Surface Water Modelling System (SMS). Users Guide to RMA2; US Army Corps of Engineer-Waterways Experiment Station: Vicksburg, MS, USA, 2009; p. 296. [Google Scholar]
- Mama, D. Méthodologie et Résultats du Diagnostic de L’eutrophisation du lac Nokoué (Bénin); Ecole Doctorale Science Technologie et Santé, Université de Limoge: Limoge, France, 2010; p. 157. [Google Scholar]
- Cheng, R.T.; Burau, J.R.; Gartner, J.W. Interfacing data analysis and numerical modeling for tidal hydrodynamic phenomena. In Tidal Hydrodynamics; Parker, B.B., Ed.; Wiley: New York, NY, USA, 1991; pp. 201–219. [Google Scholar]
- Fernandes, E.H.L.; Dyer, K.R.; Niencheski, L.F.H. Calibration and validation of the TELEMAC-2D Model to the Patos Lagoon (Brazil). J. Coast. Res. 2001, 34, 470–488. [Google Scholar]
- Wango, T.D.; Moussa, M.; Adopo, K.L.; Mondé, S. Calage du modèle hydrodynamique à 2D du complexe lagunaire de Côte d’Ivoire. Geo-Eco-Trop 2011, 35, 23–32. [Google Scholar]
- Nash, J.E.; Sutcliffe, J.V. River flow forecasting through conceptual models. A discussion of principles. J. Hydrol. 1970, 10, 282–290. [Google Scholar] [CrossRef]
- Cheng, R.T.; Casulli, V.; Gartner, J.W. Tidal, residual, intertidal mudflat (TRIM) model and its applications to San Francisco Bay, California. Estuar. Coast. Shelf Sci. 1993, 36, 235–280. [Google Scholar] [CrossRef]
- Lawrence, G.A.; Ashley, K.I.; Yonemitsu, N.; Ellis, J.R. Natural dispersion in a small lake. Limnology and natural dispersion in a small lake. Limnol. Oceanogr. 1995, 40, 1519–1526. [Google Scholar] [CrossRef]
- List, E.J.; Gartrell, G.; Winant, C.D. Diffusion and dispersion in coastal waters. ASCE J. Hydraul. SCE J. Hydraul. Eng. 1990, 116, 1158–1179. [Google Scholar] [CrossRef]
- Hsu, M.H.; Kuo, A.Y.; Kuo, J.T.; Liu, W.C. Procedure to calibrate and verify numerical models of estuarine hydrodynamics. J. Hydraul. Eng. 1999, 125, 166–182. [Google Scholar] [CrossRef]
- Dias, J.M.; Valentim, J.M. Numerical modeling of Tagus estuary tidal dynamics. In Proceedings of the 11th International Coastal Symposium, Szczecin, Poland, 9–13 May 2011; pp. 1495–1499.
- Olympiades de physiques. Des ondes à la surface de l’eau:une histoire qui fait des vagues. Available online: http://odpf.org/images/archives_docs/18eme/memoires/gr-23/memoire.pdf (accessed on 1 May 2016).
- Pugh, D.T. Tides, Surges and Mean Sea-Level: A Handbook for Engineers and Scientists; John Wiley & Sons: Chichester, UK, 1987; p. 472. [Google Scholar]
- Neves, F.J. Dynamics and Hydrology of the Tagus Estuary: Results from In Situ Observations. Ph.D. Thesis, Universidade de Lisboa, Lisboa, Portugal, 2010; p. 210. [Google Scholar]


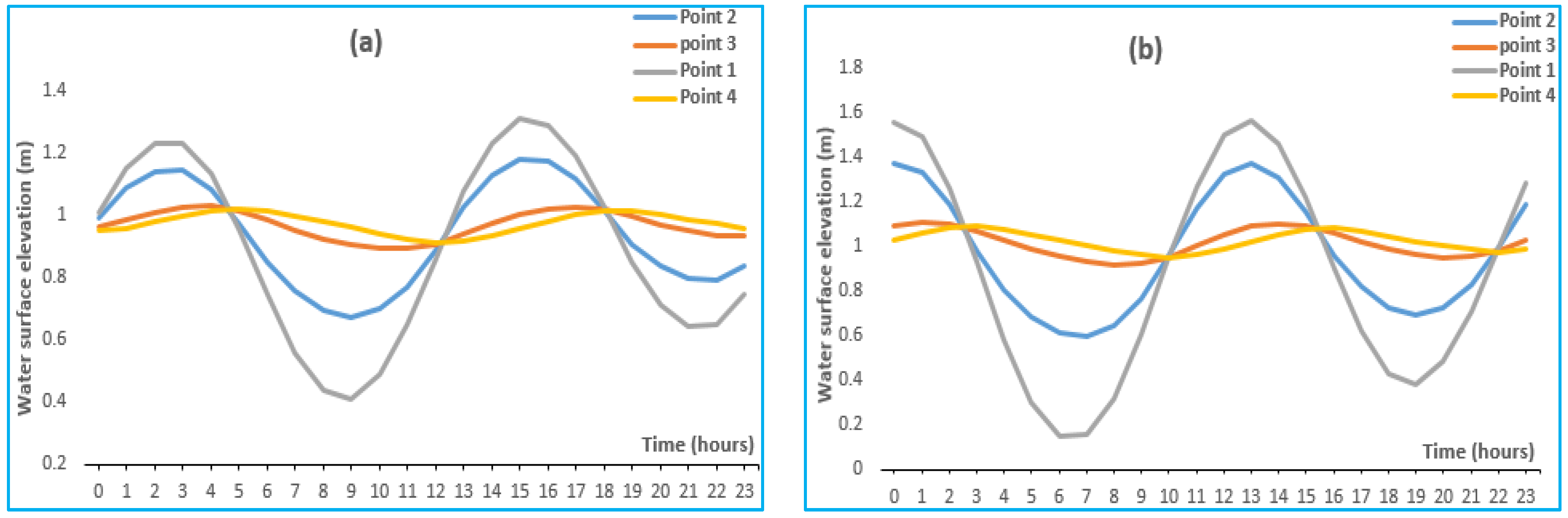
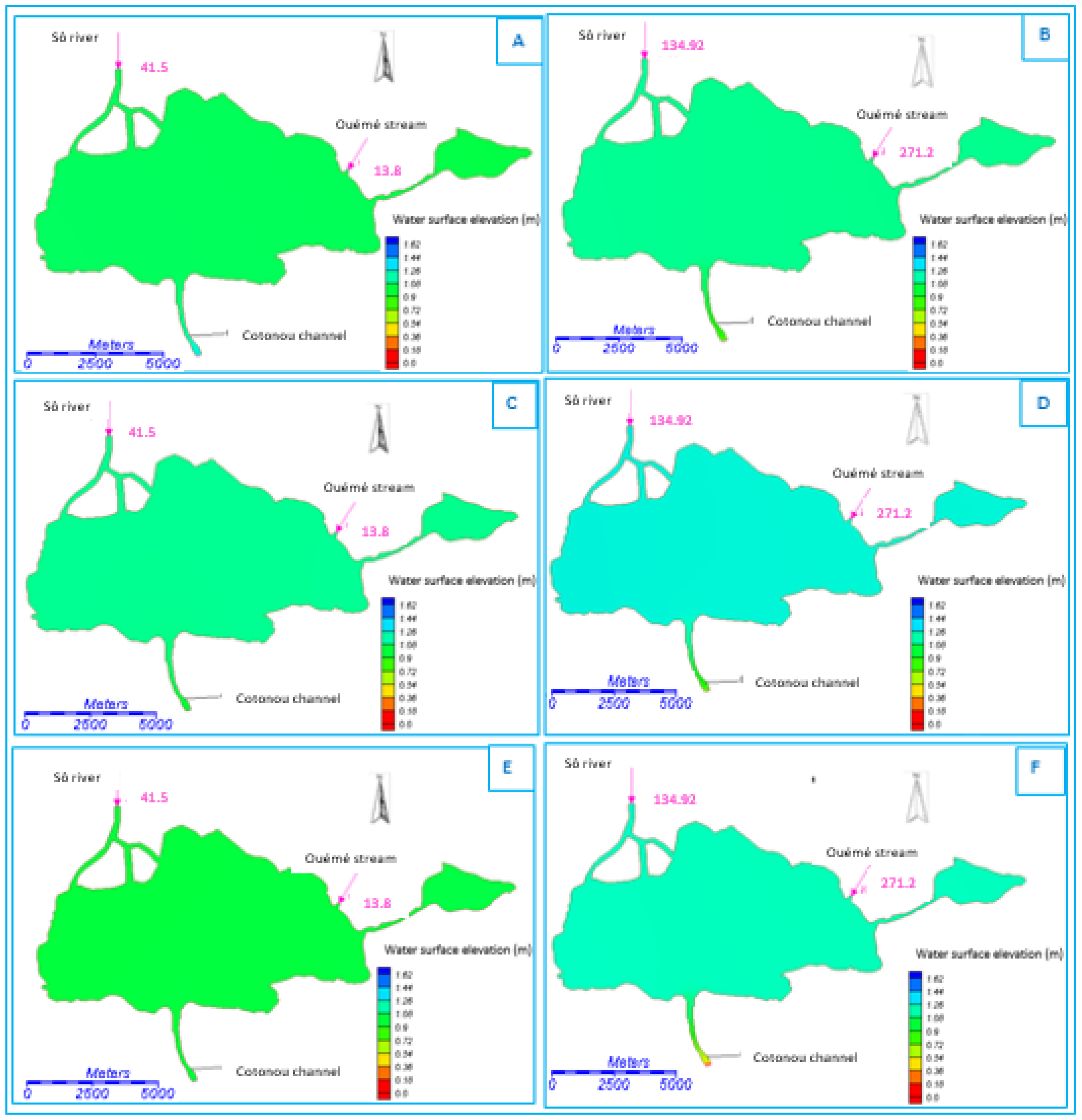
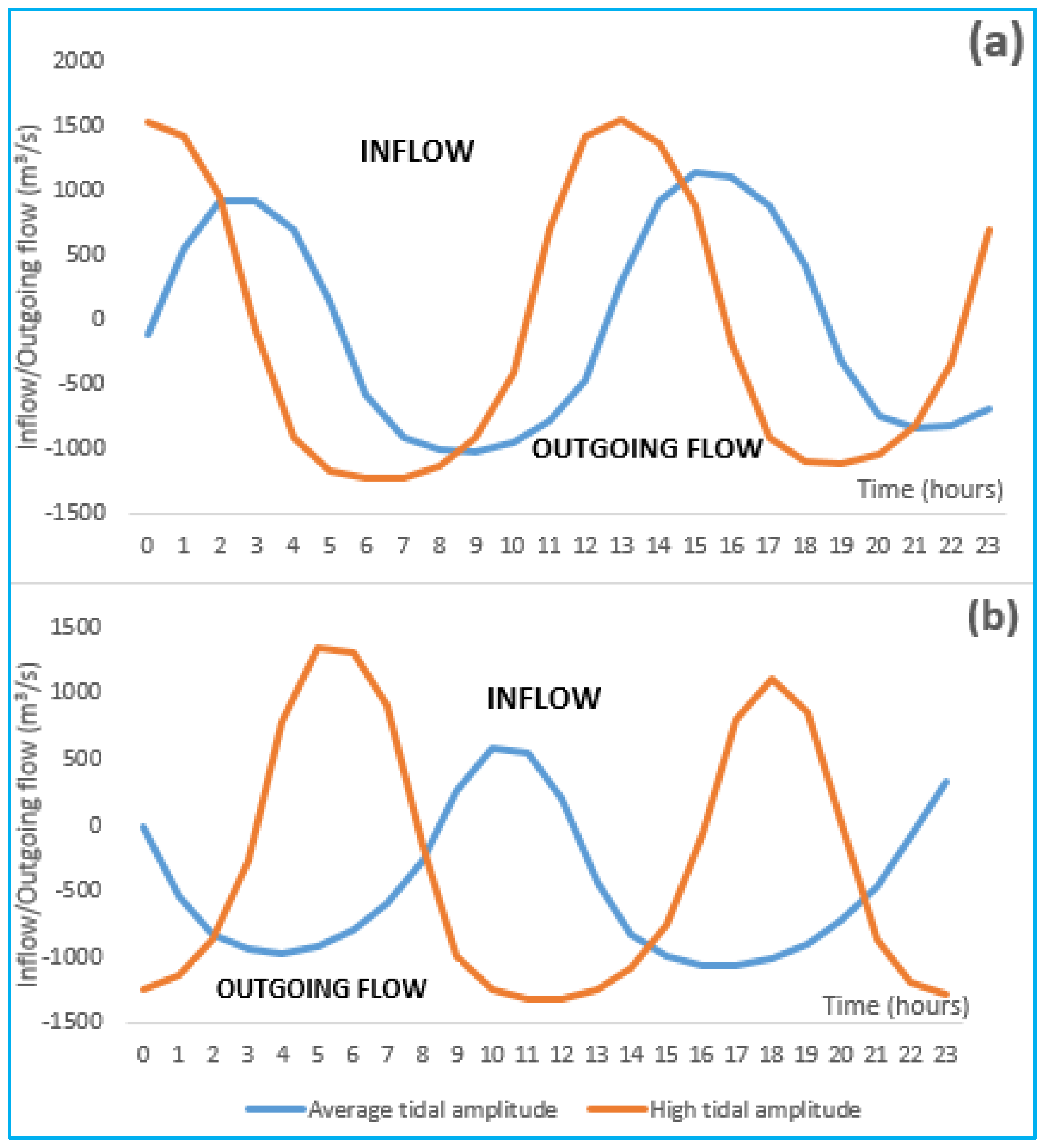

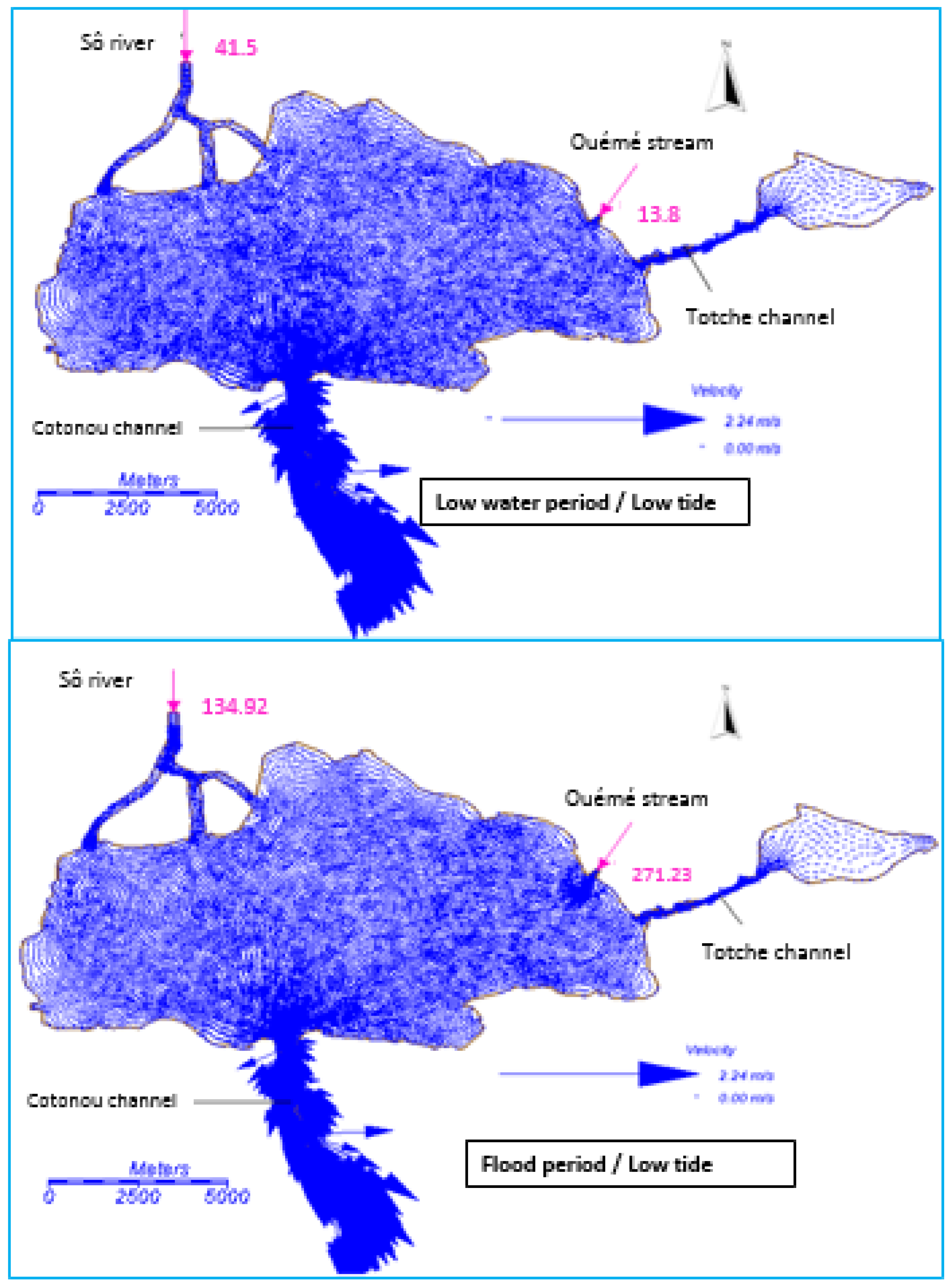
| Lake Entrance | Calibration Period | Validation Period | Simulation Period | |||
|---|---|---|---|---|---|---|
| 16 to 20 October 2007 | Flood period (16 to 20 October 2008) | Low water period (19 to 23 December 2008) | Flood period (15 September to 15 October 2014) | Low water period (1 to 31 January 2015) | ||
| Average flow estimated (m3/s) | Sô | 90.11 | 124.1 | 38.24 | 134.92 | 41.5 |
| Ouémé | 150.8 | 243.5 | 5 | 271.23 | 13.8 | |
| Period | Tidal Amplitude | Day |
|---|---|---|
| Flood Period | High tidal amplitude | 8 October 2014 |
| average tidal amplitude | 1 October 2014 | |
| Low water period | High tidal amplitude | 20 January 2015 |
| average tidal amplitude | 9 January 2015 |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zandagba, J.; Moussa, M.; Obada, E.; Afouda, A. Hydrodynamic Modeling of Nokoué Lake in Benin. Hydrology 2016, 3, 44. https://doi.org/10.3390/hydrology3040044
Zandagba J, Moussa M, Obada E, Afouda A. Hydrodynamic Modeling of Nokoué Lake in Benin. Hydrology. 2016; 3(4):44. https://doi.org/10.3390/hydrology3040044
Chicago/Turabian StyleZandagba, Josué, Mahmoud Moussa, Ezéchiel Obada, and Abel Afouda. 2016. "Hydrodynamic Modeling of Nokoué Lake in Benin" Hydrology 3, no. 4: 44. https://doi.org/10.3390/hydrology3040044
APA StyleZandagba, J., Moussa, M., Obada, E., & Afouda, A. (2016). Hydrodynamic Modeling of Nokoué Lake in Benin. Hydrology, 3(4), 44. https://doi.org/10.3390/hydrology3040044





