Scour Reduction around Bridge Pier Using the Airfoil-Shaped Collar
Abstract
1. Introduction
- (a)
- Experiments are carried out to study the reduction of scour around the bridge pier with and without an airfoil-shaped collar, which is placed at four locations under clear water conditions.
- (b)
- This paper estimated the percentage of scour reduction and efficiency of airfoil-shaped collars.
- (c)
- Experimental results are also validated with numerically simulated results using FLOW-3D.
- (d)
- Morphological changes, scour depth contours and streamlines are plotted with and without the airfoil-shaped collar.
2. Dimensional Analysis
- (a)
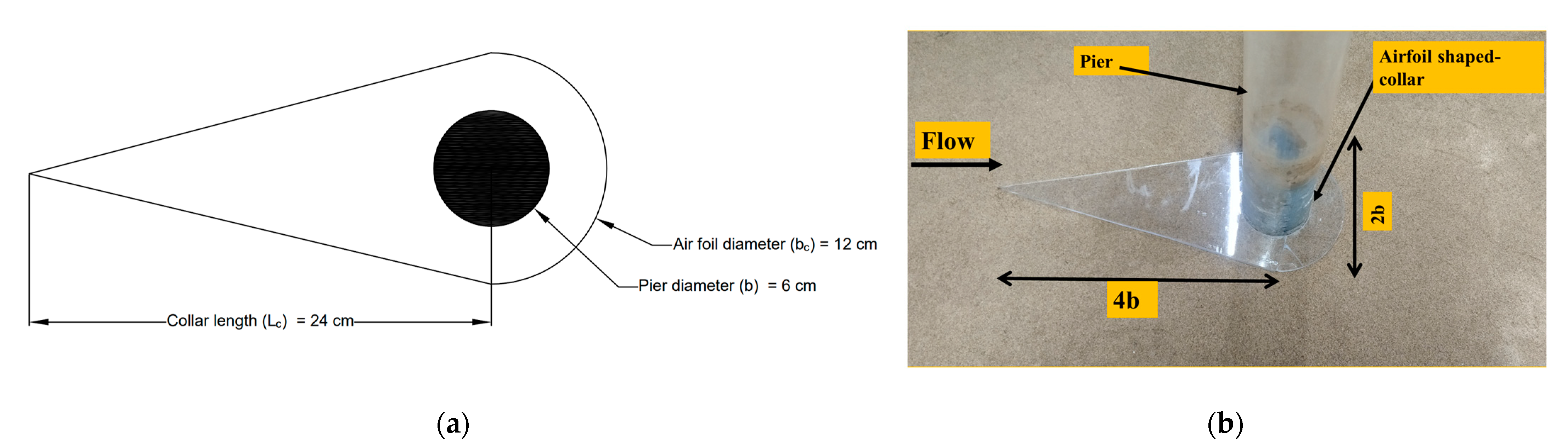
- Flow geometry: width of channel (), diameter of pier (), diameter of airfoil-shaped collar (), thickness of collar (), length of airfoil-shaped collar ), elevation of collar from the sediment bed (z).
- (b)
- Flow properties: water depth (), approach flow velocity (), acceleration due to gravity ().
- (c)
- Fluid properties: density of fluid (), kinematic viscosity ().
- (d)
- Bed properties: median particle size of sediment bed (), density of sediment bed (), standard deviation ().
- (e)
- Time: equilibrium time ().
3. Experimental Setup and Materials
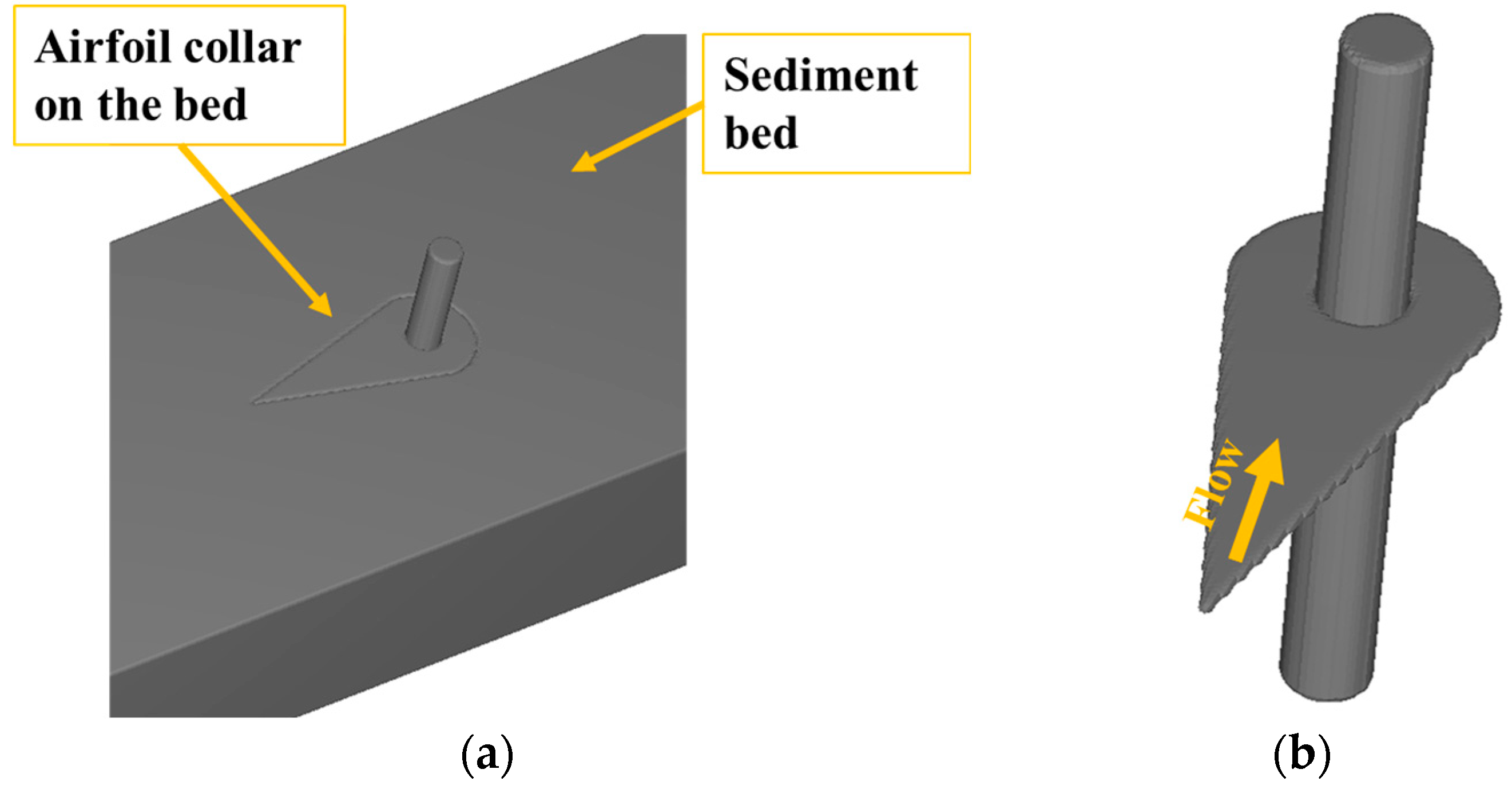
3.1. Description of Airfoil-Shaped Collar
3.2. Hydraulic Conditions for Experiments
4. Results and Discussion
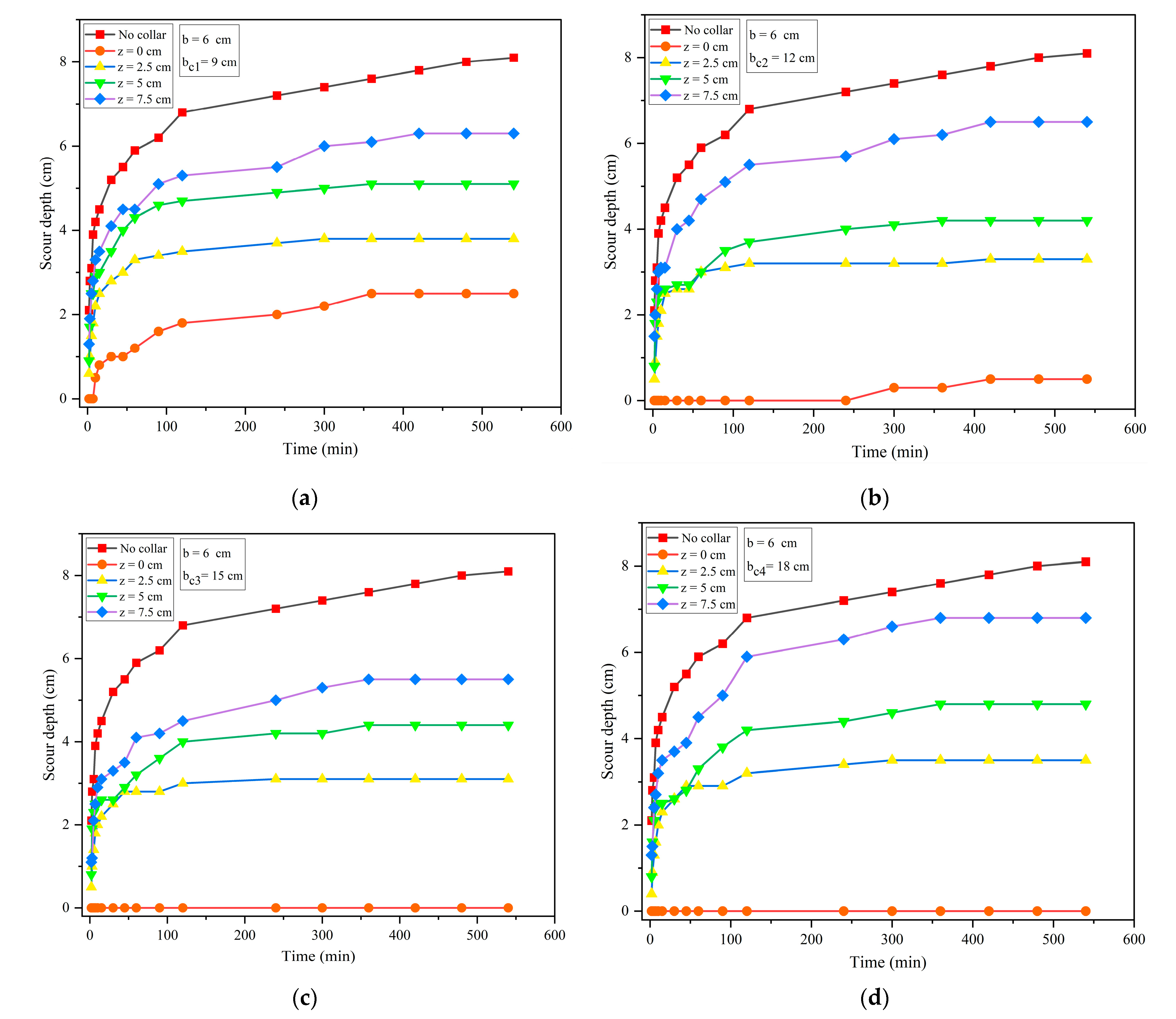
4.1. Temporal Variation of Scour Depth with and without Airfoil-Shaped Collars
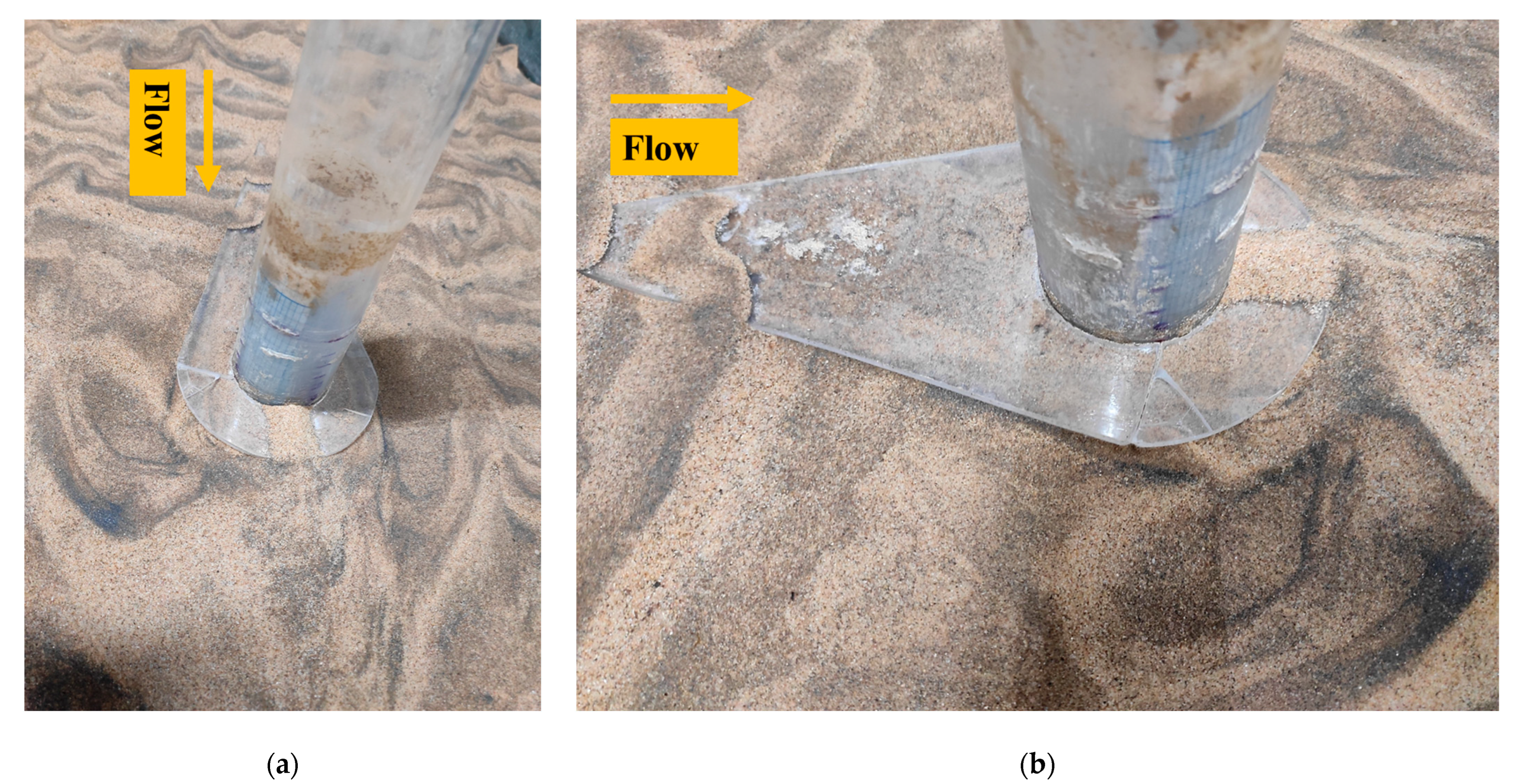
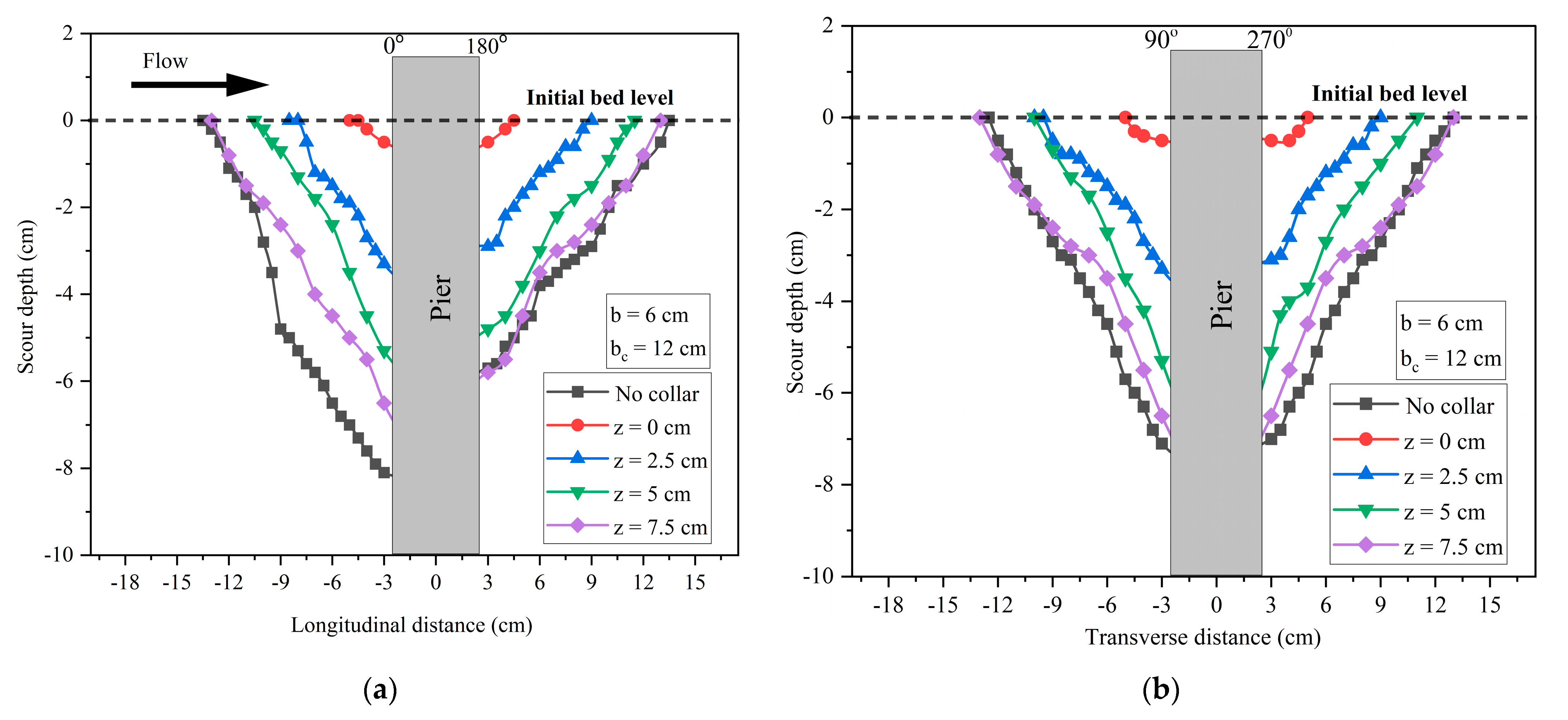
4.2. Scour Hole Profile with Airfoil-Shaped Collar
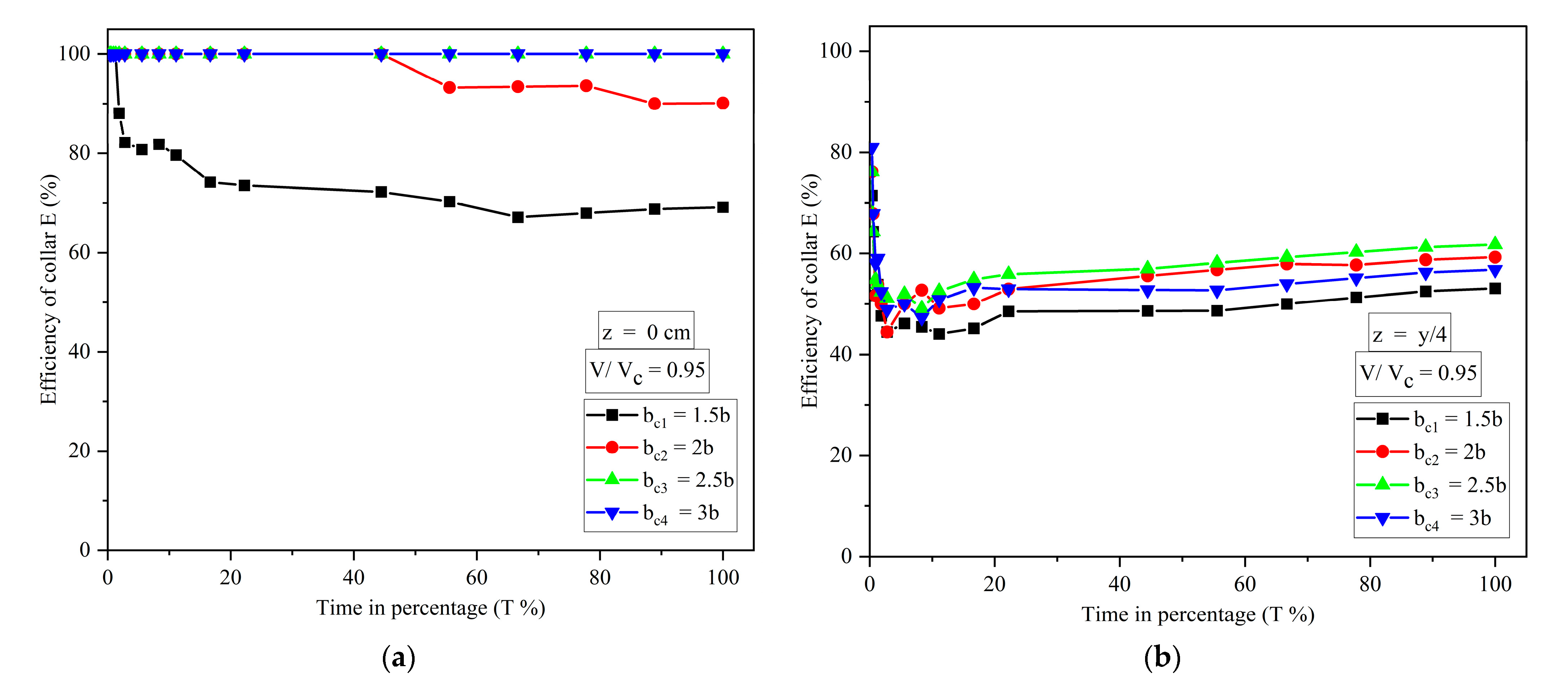
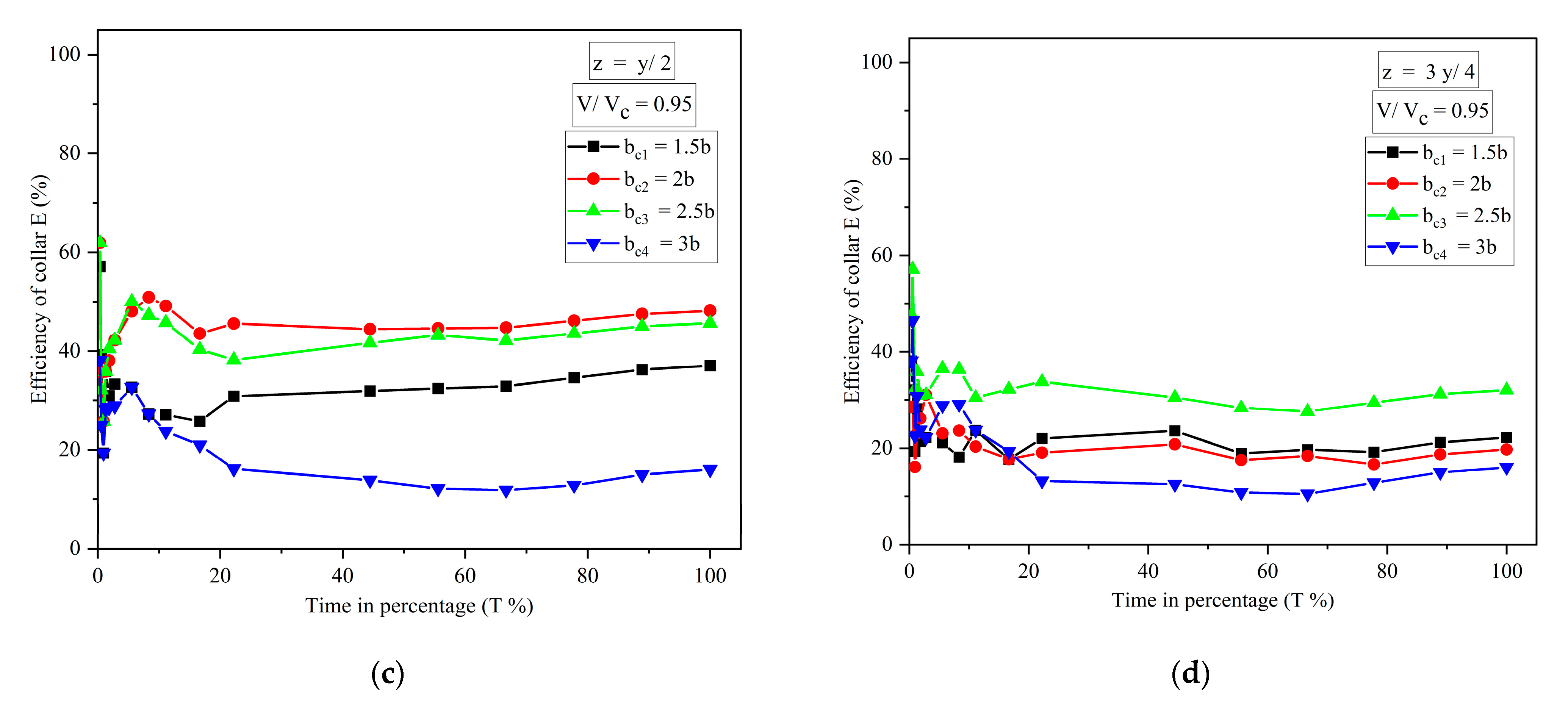
4.3. Efficiency of the Airfoil-Shaped Collar
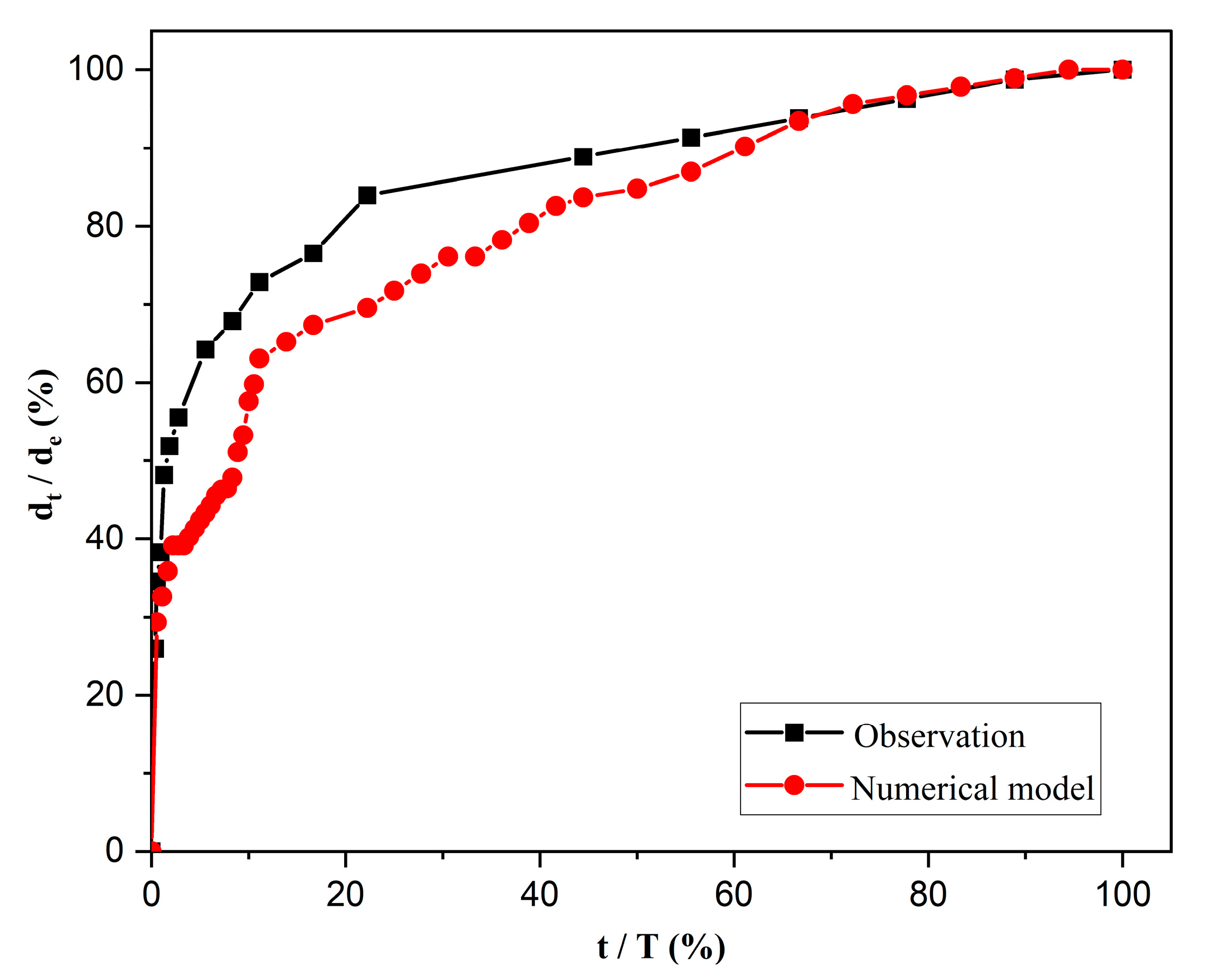
5. Numerical Simulation
5.1. Simulation Results
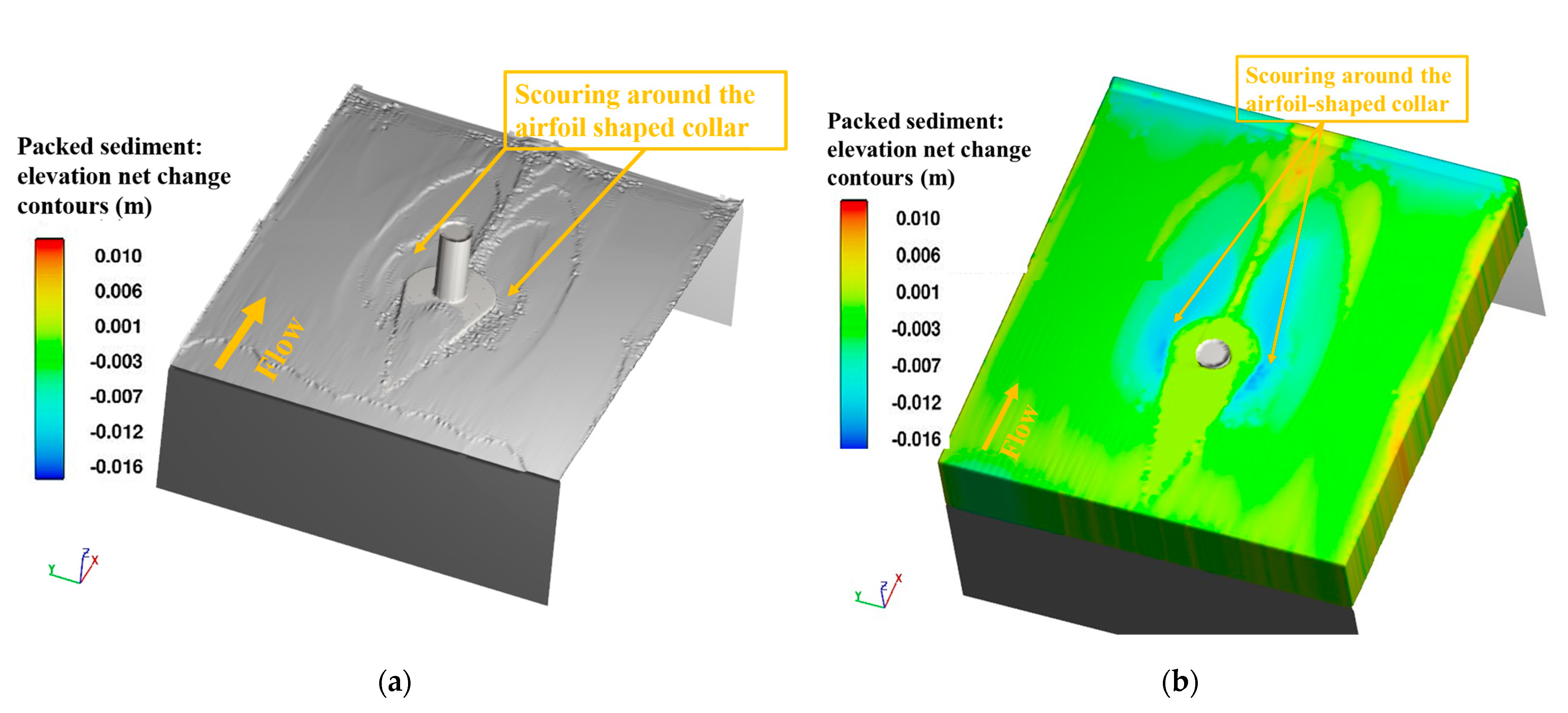
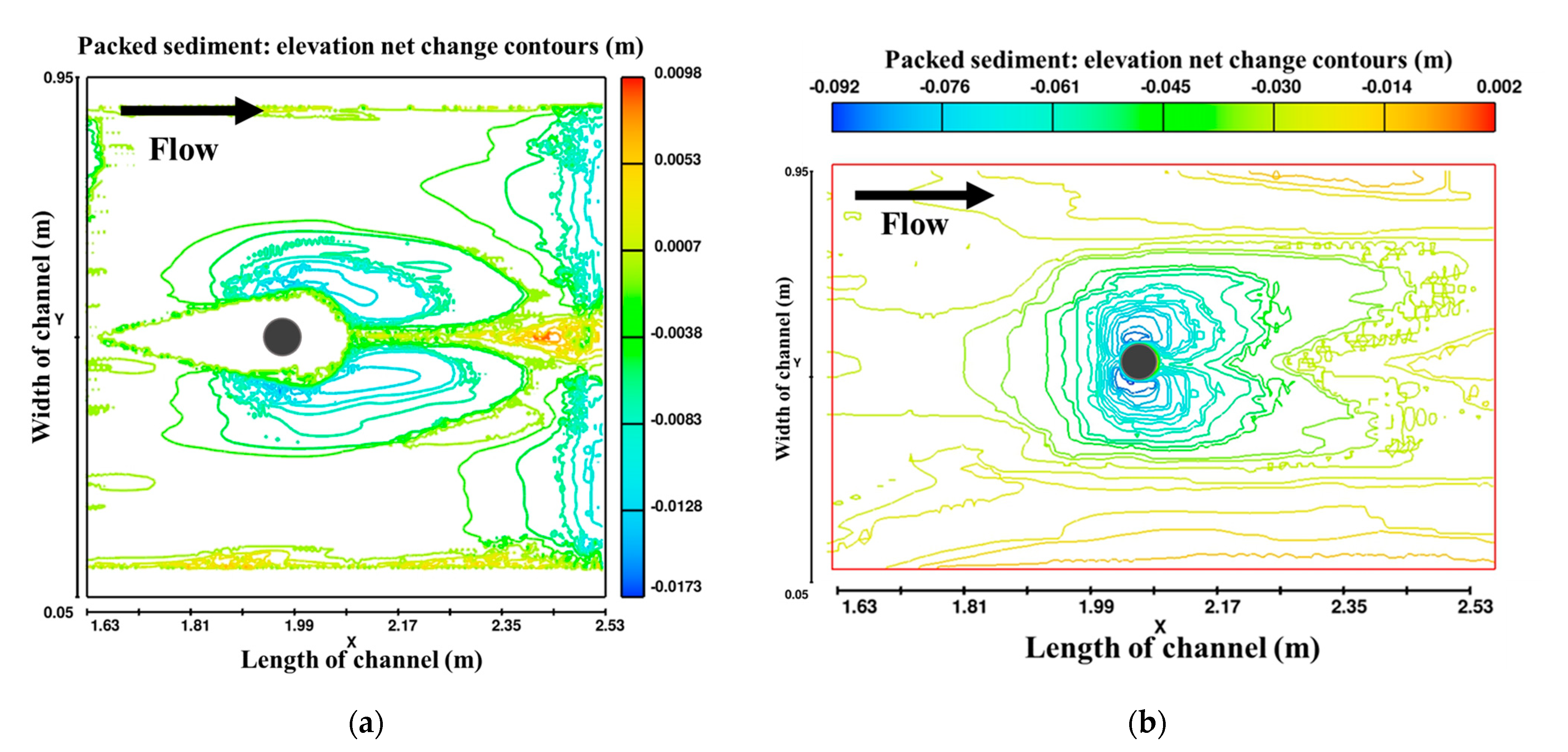
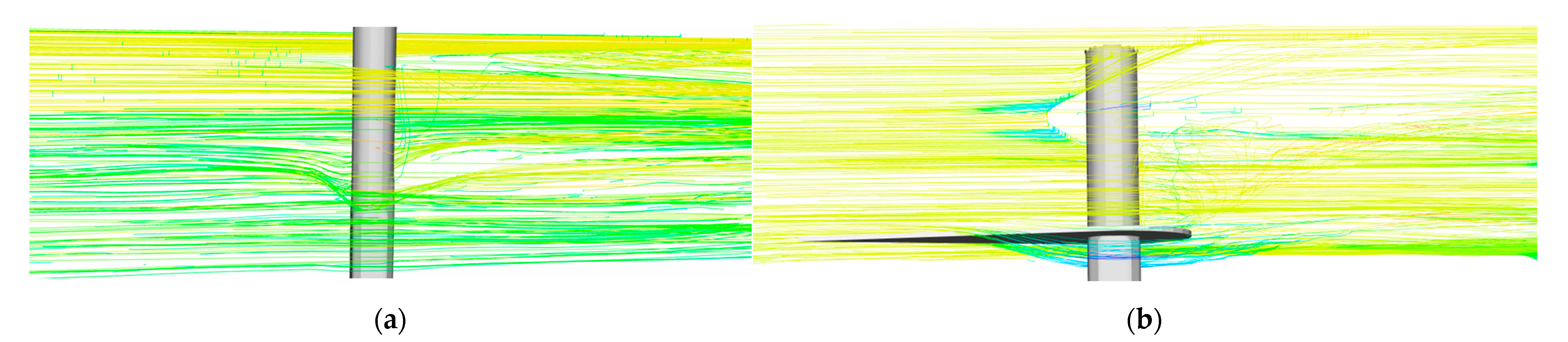
5.2. Morphological Changes, Scour Depth Contour and Streamlines Pattern
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Melville, B.W.; Chiew, Y. Time Scale For Local Scour At Bridge Piers. J. Hydraul. Eng. 1999, 125, 59–65. [Google Scholar] [CrossRef]
- Kothyari, U.C.; Kumar, A. Temporal Variation of Scour around Circular Compound Piers. J. Hydraul. Eng. 2012, 138, 945–957. [Google Scholar] [CrossRef]
- Negm, A.M.; Moustafa, G.M.; Abdalla, Y.M.; Fathy, A.A.; Affairs, S. Optimal Shape of Collar To Minimize Local Scour Around Bridge Piers. In Proceedings of the Thirteenth International Water Technology Conferenc, IWTC13 2009, Hurghada, Egypt, 12–15 March 2009; pp. 1–13. [Google Scholar]
- Lagasse, P.F.; Clopper, P.E.; Zevenbergen, L.W.; Girard, L.G. Countermeasures to Protect Bridge Piers from Scour; NCHRP: Washington, DC, USA, 2007; ISBN 9780309099097. [Google Scholar]
- Deng, L.; Wang, W.; Yu, Y. State-of-the-Art Review on the Causes and Mechanisms of Bridge Collapse. J. Perform. Constr. Facil. 2016, 30, 04015005. [Google Scholar] [CrossRef]
- Gupta, L.K.; Pandey, M.; Raj, P.A.; Shukla, A.K. Fine Sediment Intrusion and Its Consequences for River Ecosystems: A Review. J. Hazard. Toxic Radioact. Waste 2023, 27, 04022036. [Google Scholar] [CrossRef]
- Khaple, S.; Hanmaiahgari, P.R.; Gaudio, R.; Dey, S. Interference of an Upstream Pier on Local Scour at Downstream Piers. Acta Geophys. 2017, 65, 29–46. [Google Scholar] [CrossRef]
- Pandey, M.; Pu, J.H.; Pourshahbaz, H.; Khan, M.A. Reduction of Scour around Circular Piers Using Collars. J. Flood Risk Manag. 2022, 15, e12812. [Google Scholar] [CrossRef]
- Wang, S.; Wei, K.; Shen, Z.; Xiang, Q. Experimental Investigation of Local Scour Protection for Cylindrical Bridge Piers Using Anti-Scour Collars. Water 2019, 11, 1515. [Google Scholar] [CrossRef]
- Wardhana, K.; Hadipriono, F.C. Analysis of Recent Bridge Failures in the United States. J. Perform. Constr. Facil. 2003, 17, 144–150. [Google Scholar] [CrossRef]
- Hunt, B.E. NCHRP Synthesis 396: Monitoring Scour Critical Bridges—A Synthesis of Highway Practice; NCHRP: Washington, WA, USA, 2009; ISBN 9780309098342. [Google Scholar]
- Shahriar, A.R.; Ortiz, A.C.; Montoya, B.M.; Gabr, M.A. Bridge Pier Scour: An Overview of Factors Affecting the Phenomenon and Comparative Evaluation of Selected Models. Transp. Geotech. 2021, 28, 100549. [Google Scholar] [CrossRef]
- Prendergast, L.J.; Gavin, K. A Review of Bridge Scour Monitoring Techniques. J. Rock Mech. Geotech. Eng. 2014, 6, 138–149. [Google Scholar] [CrossRef]
- Mashahir, A.R.Z.M.N. and M.B. Reduction of Local Scour in the Vicinity of Bridge Pier Groups Using Collars and Riprap. XI Jorn. Españolas Presas 2006, 132, 154–162. [Google Scholar] [CrossRef]
- Dey, S.; Bose, S.K.; Sastry, G.L.N. Clear Water Scour at Circular Piers: A Model. J. Hydraul. Eng. 1995, 121, 869–876. [Google Scholar] [CrossRef]
- Zhang, H.; Nakagawa, H.; Mizutani, H. Bed Morphology and Grain Size Characteristics around a Spur Dyke. Int. J. Sediment Res. 2012, 27, 141–157. [Google Scholar] [CrossRef]
- Valela, C.; Nistor, I.; Rennie, C.D.; Lara, J.L.; Maza, M. Hybrid Modeling for Design of a Novel Bridge Pier Collar for Reducing Scour. J. Hydraul. Eng. 2021, 147, 04021012. [Google Scholar] [CrossRef]
- Christopher, V.; Loan, N.; Colin, R. Reduction of Bridge Pier Scour through the Use of a Novel Collar Design. In Proceedings of the Canadian Society for Civil Engineering, Fredericton, NB, Canada, 13–16 June 2018; pp. 235–244. [Google Scholar]
- Gazi, A.H.; Purkayastha, S.; Afzal, M.S. The Equilibrium Scour Depth around a Pier under the Action of Collinearwaves and Current. J. Mar. Sci. Eng. 2020, 8, 36. [Google Scholar] [CrossRef]
- Ahmad, N.; Bihs, H.; Afzal, M.S.; Arntsen, Ø.A. Three-Dimensional Numerical Modeling of Local Scour Around a Non-Slender Cylinder Under Varying Wave Conditions. In Proceedings of the 36th IAHR World Congress, Hague, The Netherlands, 28 June–3 July 2015; pp. 1–14. [Google Scholar]
- Ghodsi, H.; Najafzadeh, M.; Khanjani, M.J.; Beheshti, A. Effects of Different Geometric Parameters of Complex Bridge Piers on Maximum Scour Depth: Experimental Study. J. Waterw. Port, Coastal, Ocean Eng. 2021, 147, 1–15. [Google Scholar] [CrossRef]
- Najafzadeh, M.; Barani, G.A. Experimental Study of Local Scour around a Vertical Pier in Cohesive Soils. Sci. Iran. 2014, 21, 241–250. [Google Scholar]
- Kumar, V.; Raju, K.G.R.; Vittal, N. Reduction of Local Scour Around Bridge Piers Using Slots and Collars. J. Hydraul. Eng. 1999, 125, 1302–1305. [Google Scholar] [CrossRef]
- Chiew, Y. Scour Protection at Bridge Piers. J. Hydraul. Eng. 1992, 118, 1260–1269. [Google Scholar] [CrossRef]
- Zarrati, A.R.; Gholami, H.; Mashahir, M.B. Application de Collier Pour Contrôler l’affouillement Autour Des Piles de Pont Rectangulaires. J. Hydraul. Res. 2004, 42, 97–103. [Google Scholar] [CrossRef]
- Chen, S.C.; Tfwala, S.; Wu, T.Y.; Chan, H.C.; Chou, H. Ter A Hooked-Collar for Bridge Piers Protection: Flow Fields and Scour. Water 2018, 10, 1251. [Google Scholar] [CrossRef]
- Khodashenas, S.R.; Shariati, H.; Esmaeeli, K. Comparison between the Circular and Square Collar in Reduction of Local Scouring around Bridge Piers. E3S Web Conf. 2018, 40, 03002. [Google Scholar] [CrossRef]
- Pandey, M.; Azamathulla, H.M.; Chaudhuri, S.; Pu, J.H.; Pourshahbaz, H. Reduction of Time-Dependent Scour around Piers Using Collars. Ocean Eng. 2020, 213, 107692. [Google Scholar] [CrossRef]
- Moncada-M, A.T.; Aguirre-Pe, J.; Bolívar, J.C.; Flores, E.J. Scour Protection of Circular Bridge Piers with Collars and Slots. J. Hydraul. Res. 2009, 47, 119–126. [Google Scholar] [CrossRef]
- Masjedi, A.; Bejestan, M.S.; Esfandi, A. Reduction of Local Scour at a Bridge Pier Fitted with a Collar in a 180 Degree Flume Bend (Case Study: Oblong Pier). J. Hydrodyn. 2010, 22, 669–673. [Google Scholar] [CrossRef]
- Tafarojnoruz, A.; Gaudio, R.; Calomino, F. Evaluation of Flow-Altering Countermeasures against Bridge Pier Scour. J. Hydraul. Eng. 2012, 138, 297–305. [Google Scholar] [CrossRef]
- Salamatian, M.; Zarrati, A.R.; Zokaei, S.A.; Karimaee, M. Study on Scouring around Bridge Piers Protected by Collar Using Low Density Sediment. Int. J. Civ. Eng. 2013, 11, 199–205. [Google Scholar]
- Tabarestani, M.K.; Zarrati, A.R. Local Scour Depth at a Bridge Pier Protected by a Collar in Steady and Unsteady Flow. Proc. Inst. Civ. Eng. Water Manag. 2019, 172, 301–311. [Google Scholar] [CrossRef]
- Kothyari, U.C.; Hager, W.H.; Oliveto, G. Generalized Approach for Clear-Water Scour at Bridge Foundation Elements. J. Hydraul. Eng. 2007, 133, 1229–1240. [Google Scholar] [CrossRef]
- Singh, R.K.; Pandey, M.; Pu, J.H.; Pasupuleti, S.; Villuri, V.G.K. Experimental Study of Clear-Water Contraction Scour. Water Sci. Technol. Water Supply 2020, 20, 943–952. [Google Scholar] [CrossRef]
- Melville, B.W. Pier and Abutment Scour: Integrated Approach. J. Hydraul. Eng. 1997, 123, 125–136. [Google Scholar] [CrossRef]
- Pourshahbaz, H.; Abbasi, S.; Pandey, M.; Pu, J.H.; Taghvaei, P.; Tofangdar, N. Morphology and Hydrodynamics Numerical Simulation around Groynes. ISH J. Hydraul. Eng. 2022, 28, 53–61. [Google Scholar] [CrossRef]
- Giglou, A.N.; Mccorquodale, J.A.; Solari, L. Numerical Study on the Effect of the Spur Dikes on Sedimentation Pattern. Ain Shams Eng. J. 2018, 9, 2057–2066. [Google Scholar] [CrossRef]
- Choufu, L.; Abbasi, S.; Pourshahbaz, H.; Taghvaei, P.; Tfwala, S. Investigation of Flow, Erosion, and Sedimentation Pattern around Varied Groynes under Different Hydraulic and Geometric Conditions: A Numerical Study. Water 2019, 11, 235. [Google Scholar] [CrossRef]
- Gautam, S.; Dutta, D.; Bihs, H.; Afzal, M.S. Three-Dimensional Computational Fluid Dynamics Modelling of Scour around a Single Pile Due to Combined Action of the Waves and Current Using Level-Set Method. Coast. Eng. 2021, 170, 104002. [Google Scholar] [CrossRef]
- Kirkil, G.; Constantinescu, G.; Ettema, R. Detached Eddy Simulation Investigation of Turbulence at a Circular Pier with Scour Hole. J. Hydraul. Eng. 2009, 135, 888–901. [Google Scholar] [CrossRef]
- Zhiyin, Y. Large-Eddy Simulation: Past, Present and the Future. Chin. J. Aeronaut. 2015, 28, 11–24. [Google Scholar] [CrossRef]
- van Rijn, L.C. Mathematical Modelling of Morphological Processes in the Case of Suspended Sediment Transport; Waterloopkundig Laboratorium: Delft, The Netherlands, 1987. [Google Scholar]
- Flow Science, I. Flow-3d User Manual; V11.2.; Flow Science: Santa Fe, NM, USA, 2016. [Google Scholar]

| Medium Size of Particles, d50 (mm) | Flow Depth, y (cm) | Flow Intensity | Reynolds Number | Froude Number |
|---|---|---|---|---|
| 0.32 | 10 | 0.95 | 14,976 | 0.252 |
| Experimental Runs | Medium Size of Particles, d50 (mm) | Diameter of Pier, b (cm) | Width of the Channel, B (m) | Equilibrium Scour around the Pier, de (cm) |
|---|---|---|---|---|
| ER 1 | 0.32 | 6 | 0.8 | 8.1 |
| Experimental Runs with the Collar | Medium Size of Particles, d50 (mm) | Diameter of Pier, b (cm) | Diameter of Collar, bc (cm) | Length of Collar, Lc | Collar Locations, z (cm) | Equilibrium Scour around the Pier with Collar, dce (cm) |
|---|---|---|---|---|---|---|
| ERC1 | 0.32 | 6 | 9 | 18 | 0 | 2.5 |
| ERC2 | 0.32 | 6 | 9 | 18 | 2.5 | 3.8 |
| ERC3 | 0.32 | 6 | 9 | 18 | 5 | 5.1 |
| ERC4 | 0.32 | 6 | 9 | 18 | 7.5 | 6.3 |
| ERC5 | 0.32 | 6 | 12 | 24 | 0 | 0.8 |
| ERC6 | 0.32 | 6 | 12 | 24 | 2.5 | 3.3 |
| ERC7 | 0.32 | 6 | 12 | 24 | 5 | 4.2 |
| ERC8 | 0.32 | 6 | 12 | 24 | 7.5 | 6.5 |
| ERC9 | 0.32 | 6 | 15 | 30 | 0 | 0 |
| ERC10 | 0.32 | 6 | 15 | 30 | 2.5 | 3.1 |
| ERC11 | 0.32 | 6 | 15 | 30 | 5 | 4.4 |
| ERC12 | 0.32 | 6 | 15 | 30 | 7.5 | 5.5 |
| ERC13 | 0.32 | 6 | 18 | 36 | 0 | 0 |
| ERC14 | 0.32 | 6 | 18 | 36 | 2.5 | 3.5 |
| ERC15 | 0.32 | 6 | 18 | 36 | 5 | 4.8 |
| ERC16 | 0.32 | 6 | 18 | 36 | 7.5 | 5.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gupta, L.K.; Pandey, M.; Raj, P.A.; Pu, J.H. Scour Reduction around Bridge Pier Using the Airfoil-Shaped Collar. Hydrology 2023, 10, 77. https://doi.org/10.3390/hydrology10040077
Gupta LK, Pandey M, Raj PA, Pu JH. Scour Reduction around Bridge Pier Using the Airfoil-Shaped Collar. Hydrology. 2023; 10(4):77. https://doi.org/10.3390/hydrology10040077
Chicago/Turabian StyleGupta, Lav Kumar, Manish Pandey, P. Anand Raj, and Jaan H. Pu. 2023. "Scour Reduction around Bridge Pier Using the Airfoil-Shaped Collar" Hydrology 10, no. 4: 77. https://doi.org/10.3390/hydrology10040077
APA StyleGupta, L. K., Pandey, M., Raj, P. A., & Pu, J. H. (2023). Scour Reduction around Bridge Pier Using the Airfoil-Shaped Collar. Hydrology, 10(4), 77. https://doi.org/10.3390/hydrology10040077










