Modeling and Adoption of Technological Solutions in Order to Enhance the Effectiveness of Measures to Limit Water Inflows into Oil Wells under Conditions of Uncertainty

Abstract

This article is devoted to the construction and statistical analysis of models that express the relationship between performance indicators and a large number of geological and technological factors. The volume of additionally produced oil, the volume of limited water, the duration of the effect and profit per well, taking into account the cost of the polymer, are taken as performance indicators. The key goal of the article is to develop a method and models for making technological choices to enhance the effectiveness of measures to limit water inflows in production wells under conditions of uncertainty. The methodological basis of the study was the provisions and principles of mathematical statistics, the theory of fuzzy sets, the theory of decision-making under conditions of uncertainty based on materials generated by statistical processing of data on physical and geological conditions, and the results of waterproofing work, obtaining, and analyzing information. The scientific novelty of the study lies in the construction of technological solutions based on modeling the performance indicators of waterproofing works with an assessment of the significance of each factor and the reliability of the models and decision-making under conditions of uncertainty, expressed by multi-criteria and multi-factoriality. The practical significance follows from a solution that satisfies the conditions for achieving the maximum of all indicators of the efficiency of the process of limiting water inflows, both technological and economic. An algorithm was developed and implemented for evaluating optimal technological solutions according to four criteria based on information about the geological and physical conditions of the field and the experience of implementing geological and technical measures to limit water inflows, including the analysis of factors, their weighted contribution, model building, statistical evaluation of reliability indicators, decision-making taking into account uncertainty.

1. Introduction

1.1. Statement of the Research Problem

Production wells often experience complete flooding, while a significant portion of the oil reserves still remain undeveloped. These features are inherent in different geological conditions. The most reliable geological and technical measure in the noted circumstances is the restriction of water inflows, which requires the use of modern technologies and chemicals. In turn, for the effectiveness of these works, a large length of the radius of the waterproofing shield is required [1,2,3].

One of the technologies that improve the efficiency of bottom-hole zone treatments is the correct use of sediment-gel-forming compositions, which requires a deep analysis of geological conditions and experience in applying various technological solutions, analysis of performance indicators and the influence of various factors on them, building models and predictive estimates for them, and making decisions [4,5]. Here, when making decisions, it is essential to consider the potential of using methods that take into account uncertainty, which requires the collection and processing of data, analysis of the information received, and the reasonable application of methods that allow making decisions under conditions of uncertainty. An analysis of the material accumulated to date shows the need to apply modern methods in this case, which allow substantiating and clarifying the criteria necessary for making a decision and evaluating the effectiveness of decisions both from technological and economic perspectives. For this, it is necessary to study the influence of physical, geological, and technological factors on the performance indicators of solutions [6,7]. Based on this, this article proposes a calculation scheme for constructing models of decision efficiency indicators depending on a number of technological, geological, and physical factors. A preliminary analysis of the literature made it possible to focus on the tasks that need to be solved and thereby justify the use of mathematical and statistical methods for solving the tasks that determine the whole problem of making technological decisions under conditions of uncertainty [8,9].

1.2. Literature Review

One of the primary challenges encountered by oil companies worldwide is the problem of excessive water production. While this issue is often associated with aging wells, it can also manifest in newly developed production wells [1]. This creates numerous economical, technical, and technological difficulties for oil companies. Firstly, excess water adversely impacts the efficiency of production wells and reduces their operational lifespan. Increasing demands on the lifting capacity arise because the water present in the wellbore amplifies the weight of the liquid column [1]. This increases operating costs and results in a lower drawdown. For example, as noted in [1], for gas-lift wells, the quantity of injected gas to raise fluid from the wellbore to the surface is greater when excess water production is present compared to when it is not. Water production also increases the occurrence of scale, corrosion, and degradation in field structures, from the wellbore to surface structures [2]. An additional major concern is related to the costs of separating, treating, and disposing of produced water, which creates a significant financial strain on oil companies. For example, in Alberta, the disposal of produced water costs about $1 billion a year [1]. Getting rid of such production helps to reduce the costs of operators and increase the profitability of their activities [3]. Therefore, measures to limit water inflows are necessary. Finally, with sufficient information about the characteristics of the reservoir and the distinctive problems of the field, unnecessary water production at the wellbore design stage can be avoided [1]. In this case, a question arises. Is it always bad to have water inflows? Naturally, the unambiguously correct answer is “no”. Water production stands as one of the primary factors in oil recovery as it assists in controlling the formation, mobilizing oil, and displacing it from homogeneous rocks. Known as required or good water production, this water is typically linked to oil production from the concluding phases of waterflooding operations or active aquifers. In addition, maintaining profitability of the production well is achieved through the production of water at a low oil-to-water ratio (WOR) [1]. Efforts to decrease this type of water production lead to a reduction in oil production [4]. Conversely, the elimination and reduction of unwanted water production is imperative to enhance the efficiency and financial performance of production wells. On the contrary, unwanted water production must be eliminated and reduced in order to increase the productivity and profitability of production wells [1]. Shut-off operations concentrate on the removal of undesired water supply, also referred to as “bad water”. This kind of production gives rise to additional issues beyond those already discussed, including diminished oil production and reduced sweep efficiency of wall rocks. Simply put, this means a loss of finances. The most problematic issues with water production are unwashed areas and oil pockets remaining due to the result of poor-quality work, as agreed. This case is well known in waterflooding, where water is injected into a well to displace oil towards the production well and maintain reservoir pressure. However, the water can enter an open fracture or a highly permeable reservoir. Unwanted water production can occur if a production well is linked to an open fracture or a reservoir with high permeability. Distinguishing between these two forms of water production is crucial for preserving well productivity [1,4]. One way to determine the form of excess water production in a particular well is to study the dynamics of water cut in neighboring wells. Poor water production if adjacent wells provide significantly lower water cut [3]. Following the discussion of the issues linked to undesired water release, it is essential to determine the causes responsible for this type of water production so that the water shut-off operation can be successfully performed. The goal of waterflooding is to move oil within the source rock towards the producing wells and maintain reservoir pressure. Fractures that are open and reservoirs characterized by high permeability typically diminish the effectiveness of waterflooding, leading to suboptimal outcomes. As noted earlier, the fluid demonstrates a tendency to follow the path of least resistance, and as a result, the injected water is directed into open fractures and high permeability formations rather than displacing the oil within the reservoir rock. In certain instances, the injection well forms a connection with the production well via open fractures or structures, which are often referred to as “thief zones” [1]. If exposed features are interconnected with an aquifer, they can also lead to excess water. In addition, fractures and exposed features can lead to undesirable water production if connections with aquifers are present [5]. Other sources of excessive water production during isolation include gas hydrate reservoirs [6]. Furthermore, water obtained at a low oil-to-water ratio (WOR) maintains the profitability of the production well [1]. Efforts to decrease this form of water loss result in a reduction in oil production [4,7]. Conversely, eliminating and reducing unwanted water production is essential to enhance the profitability and efficiency of production wells. Shut-down operations are primarily centered around the elimination of undesirable water buildup, often referred to as “bad water”.

This form of production gives rise to additional issues beyond those previously mentioned, including decreased oil production and inefficient sweeping of the wall rocks. In other words, this will ultimately lead to a decrease in performance indicators. A widespread case is when water is pumped through an injection well to push oil towards the production well and keep pressure in the formation. Water escapes into an open fracture or high-permeability formation. The whole point, in this case, is the resistance within the pathways that take place in the layers. The path of least resistance prevails, as it attracts the injected water, while the oil in the underlying formation remains insufficiently swept, resulting in an ineffective oil sweep or a poor match [1]. In cases where a production well is linked to an open fracture or a highly permeable formation, there is a potential for unwanted water production to take place. It holds significant importance to differentiate between these two forms of water production to promptly make the right technological decisions.

The development of measures to limit water inflows usually begins with the collection of geological and technological data from the locations of this geological and technical measure.

In any water shut-off operation, the critical element is a precise analysis of the conditions under which the issue occurs. Understanding the water entry point, the characterization of reservoir rocks, and possessing information about reservoir and bottom-hole pressures, well designs, and related factors are essential. In fact, as noted in [1], all accessible information regarding the well, such as logs, drilling reports, and production history of technological operations is seen as significant. The explanation for this is that each well will have its distinct data package that characterizes the full geological conditions and workflow. Accurate investigation leads to successful decision-making to limit water inflows, increase oil production and save costs for technological operations [8]. The authors of the work performed note the importance of logging in production wells in determining water cut as an important step in planning measures to limit water inflows.

A group of works is devoted to the analysis of the advancements of fields characterized by a high water cut, the dynamics of oil and water production at these fields, in which it is noted that wells are closed when their water cut hits 98% [3]. This enables effective control of the water-to-oil ratio, leading to increased production and cost savings in the development process [9]. During the exploitation of the field under consideration, the water cut increases gradually, the rates of water production remain low, partially as a result of high-water cut wells being closed. As the authors note, over 1100 high-water cut wells were ceased in this field for one year, and the cumulative count of wells closed due to high water cut exceeded more than 1900 after five years. According to the development of another oil field, it was observed that high-water cut wells located on the periphery of the reservoirs have a detrimental impact on oil production from the upper reservoir. Following the shutdown of one of the high-water-cut wells, the oil production rate of two upper-layer wells experienced a more than two-fold increase. After the successful completion of the investigation, nine additional high-water cut wells were ceased. High economic indicators were attained, and oil production was increased under conditions of continuous water injection and fluid generation [10]. It is noted that various technologies of cyclic waterflooding are constantly being developed in the field [9]. Currently, this method is employed in the majority of injection wells, establishing it as the primary approach for managing the water cut within the field [9].

A number of works are devoted to polymer flooding. At present, polymer flooding stands as the most commonly employed chemical flooding technology. The fundamental process in polymer flooding involves the addition of polymers to the injected water with the formation of a highly viscous displacement fluid [11]. Waterproofing work can be carried out using various chemical compositions and treatments. These compositions lead to better blocking of water inflow zones. The concept is to effectively block the routes of least resistance for water by decreasing their permeability, thus preventing water ingress into the well through those pathways. To clarify, the goal is to block open high permeability channels [12]. The results of the application of the compositions can be achieved after a certain time, which depends on the reservoir’s nature and the characteristics of the chemicals, that were injected. Chemical water shut-offs differ from mechanical methods as they address the issue of undesired water production rather than concealing it beneath or behind a packer, plug, or tubing patch. Chemicals introduced into the reservoir can infiltrate its water-bearing zones, reducing permeability and ultimately achieving complete closure. Additionally, these chemicals possess the capability to migrate between layers and features, facilitating their access to remote areas and enabling the closure. An alternative application of chemical injection involves increasing the viscosity of the injected fluid, leading to improved sweep efficiency and, ultimately, a reduction in unwanted water production. The effectiveness of chemical injection procedures relies on the extent of knowledge about reservoirs, their petrophysical characteristics, and the rheological characteristics of the compositions used [13,14]. For instance, the efficiency of water sealing agents (polymers, sediment-gelling compositions) strongly depends on the properties of the formation and must be compatible with other factors to achieve effective water sealing [15].

Polymer flooding is one of the most common waterproofing methods. Polymer flooding is a commonly employed technique in the oil industry, where polymers are dissolved in injection water and subsequently delivered through dedicated injection wells. In this approach, there are typically two types of polymers: biopolymers and synthetic polymers. Biopolymers offer advantages over synthetic polymers in that they remain unaffected by water salinity and are resilient to mechanical failures. Nonetheless, they come at a higher cost compared to synthetic polymers. Two types of well-known biopolymers include xanthan and scleroglucan. Due to their lower cost, widespread availability, and compatibility with brackish water, synthetic polymers are more commonly used. Polyacrylamide (PAA) and hydrolyzed polyacrylamide (HPAA) represent two kinds of synthetic polymers. Polymers can also contribute to reducing permeability [4]. Ultimately, the appropriate polymer is chosen for chemical injection based on reservoir characteristics and economic considerations [16]. The work [17] provides a general overview of polymer systems used for waterproofing works, as well as their properties and chemical compositions. Additional chemical water sealing techniques, including resins, solids, and foams, have also been proven effective in enhancing sealing precision and expanding coverage. More details about these methods are also noted in [4].

Among the widely recognized mechanical approaches for wellbore waterproofing, it is worth highlighting the installation of packers and plugs. They are commonly used by oil operators to shut off excess water supply [18,19]. This equipment is known for its economy and reliability in achieving waterproofing. These solutions can be installed using coiled tubing, which can be threaded through the wellbore.

Thus, from the above brief review, it follows that in connection with the entry of the largest oil fields in the world into the stage of high water cut and a decrease in the oil recovery factor, with an extremely complex distribution of residual oil, the main method necessary for further enhanced oil recovery is a more accurate selection and evaluation of the effectiveness of measures in reservoirs with high water cut. The development (improvement) of methods and approaches to decision-making in order to enhance the technological and economic efficiency of oil displacement, oil recovery factor, and reduce reservoir water cut are of great importance for increasing oil production, as follows from the review, is an urgent problem. The use of various applied methods for constructing models of the effectiveness of measures, including those that take into account the uncertainty of the conditions for modeling and decision-making, will ultimately achieve water inflow limitation and enhanced oil recovery. Depending on the specific situation with a high water cut reservoir, a practical choice of oil production technologies based on the use of polymeric and sediment-gelling compositions is promising.

2. Materials and Methods

The present research is aimed at developing and improving the scientific foundations of decision-making under conditions of uncertainty. Preliminary studies substantiate the need to consider the possibility of using modern methods of data processing, information analysis, and decision-making, identifying the reasons for the insufficiency of previous studies, and filling in varying degrees of gaps in the studies of the problem under consideration. For analysis, data were collected and subjected to statistical processing. The information obtained was subjected to statistical analysis with the construction of multiple correlation dependencies, the degrees of importance of each factor were justified, and the reliability of each of these dependencies was assessed.

The research system further provides for the decision-making stage, which is one of the most responsible. Decision-making is carried out using fuzzy logic.

As a result, the problem on which it was necessary to focus attention was established, which determined the purpose of this article. The desire to achieve this goal was a complex process that had its own logical sequence of tasks to be solved, the corresponding stages and levels. Methodologically, this study comes down to considering them as part of an integral system with its elements located at different levels.

The methodological basis of the study was the provisions and principles of mathematical statistics, the theory of fuzzy sets, the theory of decision-making under conditions of uncertainty based on materials generated by statistical processing of data on geological and physical conditions and the results of waterproofing work, obtaining and analyzing information.

Technological methods in the operation of wells include the determination of the optimal parameters of technical and technological factors that ensure, under the considered geological and physical conditions, the maximum values of the timeframes of the effect, additionally produced oil, the volume of limited water, and profit. Proceeding from this, according to its formulation, the article solves a multi-criteria decision-making problem. This determines the focus of the research topics presented in the article. Methodological approaches and the sequence of application of the theory of fuzzy sets and methods of mathematical statistics make it possible to make decisions under conditions of uncertainty due to multi-criteria and multi-factoriality.

The applied methods for solving problems under conditions of uncertainty, being a tool for conducting scientific research, still allow modeling at the modern level, assessing the contribution of each factor, assessing the reliability of forecast calculations, and thereby substantiating the decisions made in scientific terms.

To build dependencies of a selected number of criteria that affect the efficiency of geological and technological activities, their processing is carried out in accordance with the correlation analysis. At the same time, statistical data processing was carried out in two directions through the use of a linear regression program. To do this, the input and output variables were first presented in a logarithmic form. Subsequently, after running the linear regression program, equations were constructed in the form of a polynomial, then, expressions in a multiplicative form were obtained by potentiation. Based on the same initial information collected for one of the fields in Kazakhstan, the obtained models were identified. We did this by comparing the tables calculated according to preliminary models of the type:

$$\mathit{Y}={\mathit{a}}_{\mathbf{0}}+{\displaystyle \sum }_{\mathit{i}=\mathbf{1}}^{\mathbf{12}}{\mathit{a}}_{\mathit{i}}{\mathit{x}}_{\mathit{i}}$$

(1)

and the actual data were refined and identified under reservoir conditions. A detailed description of each parameter of the equation is given in

Table 1. Values of geological, physical, and technical factors of wells of one of the fields in Kazakhstan.

No.	Permeability, µm2	Compartmentalization	Reservoir Pressure, MPa	Bottomhole Pressure, MPa	Oil viscosity in Reservoir Conditions, mPa × s	Current Oil Recovery Factor	Well Flow Rate before Treatment		Water Cut, %	Filter Length, m	1 m Thick Filter, kg	% CCD Polymer Flooding
							Oil, t/day	Water, m3/day
	x1	x2	x3	x4	x5	x6	x7	x8	x9	x10	x11	x12
1	0.728	5.8	36	38	0.997	31.4	1.3	24.9	94.99	8	4.4	19.2
2	1.34	5.8	19.6	22.1	0.997	31.4	6.1	129.1	95.47	19.5	3.8	16.5
3	15.6	5.8	26.8	28.8	0.997	31.4	3.4	140.6	97.61	26	7.8	34.2
4	5.67	5.8	25.7	29.2	1.22	31.4	3.6	116.2	96.97	14	14	61.4
5	2.98	2.2	22.6	24.6	0.97	31.4	5.4	183.7	97.14	18	16.6	72.6
6	14.4	2.2	20.4	22.4	0.997	31.4	6.7	232.6	97.19	16	11.3	49.5
7	4.6	2.2	28	30	0.8	31.4	4.1	53.6	92.87	31	2.7	11.7
8	3.91	5.8	27.4	29.4	0.997	31.4	3	139	97.86	15	20.5	90
9	9.35	5.8	27.4	29.4	0.997	31.4	7.7	279.9	97.33	19	10.3	45.2
10	45.6	2.5	21.3	23.3	1.02	31.4	3.4	46.7	93.16	13	3.1	13.7

.

As a result of such processing, marked models were obtained for each performance indicator.

• Dependences of the values of the efficiency indicator and geological and technological factors were built according to their actual values. For this, the logarithms of the input and output variables were found, and utilizing the linear regression program, linear multiple equations of type (1) were constructed in the form of the following polynomials (polynomials) [17,18,19,20,21].

For effect duration:

$$\begin{array}{c}{\mathit{Y}}_{\mathbf{1}}^{*} \\ = \mathbf{2.088} + \mathbf{0.0279}{\mathit{X}}_{\mathbf{1}}\\ + \mathbf{0.2117}{\mathit{X}}_{\mathbf{2}} + \mathbf{0.8552}{\mathit{X}}_{\mathbf{3}} - \mathbf{0.8354}{\mathit{X}}_{\mathbf{4}} + \mathbf{0.1911}{\mathit{X}}_{\mathbf{5}} + \mathbf{0.2134}{\mathit{X}}_{\mathbf{6}}\\ - \mathbf{0.1116}{\mathit{X}}_{\mathbf{7}} - \mathbf{0.0122}{\mathit{X}}_{\mathbf{8}}-\mathbf{1.0794}{\mathit{X}}_{\mathbf{9}} - \mathbf{0.6955}{\mathit{X}}_{\mathbf{10}}\\ - \mathbf{0.0266}{\mathit{X}}_{\mathbf{11}} + \mathbf{0.5022}{\mathit{X}}_{\mathbf{12}}\end{array}$$

(2)

additional oil production:

$$\begin{array}{c}{\mathit{Y}}_{\mathbf{2}}^{*}\\ = \mathbf{4.011} - \mathbf{0.0329}{\mathit{X}}_{\mathbf{1}}\\ + \mathbf{0.0199}{\mathit{X}}_{\mathbf{2}} + \mathbf{0.2676}{\mathit{X}}_{\mathbf{3}}-\mathbf{0.3559}{\mathit{X}}_{\mathbf{4}} + \mathbf{0.2869}{\mathit{X}}_{\mathbf{5}} - \mathbf{0.0187}{\mathit{X}}_{\mathbf{6}}\\ - \mathbf{0.1458}{\mathit{X}}_{\mathbf{7}} + \mathbf{0.1664}{\mathit{X}}_{\mathbf{8}} - \mathbf{1.2373}{\mathit{X}}_{\mathbf{9}} - \mathbf{0.2664}{\mathit{X}}_{\mathbf{10}} - \mathbf{0.0446}{\mathit{X}}_{\mathbf{11}} + \mathbf{0.15}{\mathit{X}}_{\mathbf{12}}\end{array}$$

(3)

limited water volume:

$$\begin{array}{c}{\mathit{Y}}_{\mathbf{3}}^{*}\\ = \mathbf{2.673} - \mathbf{0.2207}{\mathit{X}}_{\mathbf{1}}\\ - \mathbf{0.1253}{\mathit{X}}_{\mathbf{2}} - \mathbf{0.8418}{\mathit{X}}_{\mathbf{3}} + \mathbf{0.9415}{\mathit{X}}_{\mathbf{4}} - \mathbf{0.6897}{\mathit{X}}_{\mathbf{5}} - \mathbf{0.1753}{\mathit{X}}_{\mathbf{6}}\\ + \mathbf{0.2407}{\mathit{X}}_{\mathbf{7}} - \mathbf{0.4635}{\mathit{X}}_{\mathbf{8}} + \mathbf{0.3228}{\mathit{X}}_{\mathbf{9}} + \mathbf{1.0051}{\mathit{X}}_{\mathbf{10}}\\ - \mathbf{0.0456}{\mathit{X}}_{\mathbf{11}} - \mathbf{0.5124}{\mathit{X}}_{\mathbf{12}}\end{array}$$

(4)

well profit taking into account the cost of the polymer:

$$\begin{array}{c}{\mathit{Y}}_{\mathbf{4}}^{*}\\ = -\mathbf{0.75} \\ - \mathbf{0.0078}{\mathit{X}}_{\mathbf{1}}-\mathbf{0.2062}{\mathit{X}}_{\mathbf{2}} -\mathbf{1.1539}{\mathit{X}}_{\mathbf{3}} + \mathbf{1.6104}{\mathit{X}}_{\mathbf{4}} - \mathbf{0.9984}{\mathit{X}}_{\mathbf{5}} + \mathbf{0.0487}{\mathit{X}}_{\mathbf{6}}\\ + \mathbf{0.017}{\mathit{X}}_{\mathbf{7}} + \mathbf{0.0217}{\mathit{X}}_{\mathbf{8}} + \mathbf{1.1505}{\mathit{X}}_{\mathbf{9}} + \mathbf{1.0366}{\mathit{X}}_{\mathbf{10}} - \mathbf{0.1685}{\mathit{X}}_{\mathbf{11}} + \mathbf{0.0943}{\mathit{X}}_{\mathbf{12}}\end{array}$$

(5)

where ${\mathit{X}}_{\mathit{i}}=\mathit{l}\mathit{o}\mathit{g} {\mathit{x}}_{\mathit{i}}$, ${\mathit{Y}}_{\mathit{i}}^{*}=\mathit{l}\mathit{o}\mathit{g} {\mathit{Y}}_{\mathit{i}}$.

This is done by applying a linear regression program. As for the * sign, we decided to distinguish the values of the output variable in the model in the form of a polynomial from the models in the multiplicative form.

2.

By carrying out the operations of potentiation of Expressions (2)–(5), the required dependences were obtained in a multiplicative form with the subsequent refinement of the parameters: For the duration of the effect, the following dependence was obtained:

$${\mathit{Y}}_{\mathbf{1}}=\mathbf{122.504} \frac{{\mathit{x}}_{\mathbf{1}}^{\mathbf{0.0279}}{\mathit{x}}_{\mathbf{2}}^{\mathbf{0.2117}}{\mathit{x}}_{\mathbf{3}}^{\mathbf{0.8552}}{\mathit{x}}_{\mathbf{5}}^{\mathbf{0.1911}}{\mathit{x}}_{\mathbf{6}}^{\mathbf{0.2134}}{\mathit{x}}_{\mathbf{12}}^{\mathbf{0.5022}}}{{\mathit{x}}_{\mathbf{4}}^{\mathbf{0.8354}}{\mathit{x}}_{\mathbf{7}}^{\mathbf{0.1116}}{\mathit{x}}_{\mathbf{8}}^{\mathbf{0.0122}}{\mathit{x}}_{\mathbf{9}}^{\mathbf{1.0794}}{\mathit{x}}_{\mathbf{10}}^{\mathbf{0.6955}}{\mathit{x}}_{\mathbf{11}}^{\mathbf{0.0266}}}$$

(6)

additional oil production:

$${\mathit{Y}}_{\mathbf{2}}=\mathbf{10,258.863} \frac{{\mathit{x}}_{\mathbf{2}}^{\mathbf{0.0199}}{\mathit{x}}_{\mathbf{3}}^{\mathbf{0.2676}}{\mathit{x}}_{\mathbf{5}}^{\mathbf{0.2869}}{\mathit{x}}_{\mathbf{8}}^{\mathbf{0.1664}}{\mathit{x}}_{\mathbf{12}}^{\mathbf{0.15}}}{{\mathit{x}}_{\mathbf{1}}^{\mathbf{0.0329}}{\mathit{x}}_{\mathbf{4}}^{\mathbf{0.3559}}{\mathit{x}}_{\mathbf{6}}^{\mathbf{0.0187}}{\mathit{x}}_{\mathbf{7}}^{\mathbf{0.1458}}{\mathit{x}}_{\mathbf{9}}^{\mathbf{1.2373}}{\mathit{x}}_{\mathbf{10}}^{\mathbf{0.2664}}{\mathit{x}}_{\mathbf{11}}^{\mathbf{0.0446}}}$$

(7)

limited water volume:

$${\mathit{Y}}_{\mathbf{3}}=\mathbf{471.068} \frac{{\mathit{x}}_{\mathbf{4}}^{\mathbf{0.9415}}{\mathit{x}}_{\mathbf{7}}^{\mathbf{0.2407}}{\mathit{x}}_{\mathbf{9}}^{\mathbf{0.3228}}{\mathit{x}}_{\mathbf{10}}^{\mathbf{1.0051}}}{{\mathit{x}}_{\mathbf{1}}^{\mathbf{0.2207}}{\mathit{x}}_{\mathbf{2}}^{\mathbf{0.1253}}{\mathit{x}}_{\mathbf{3}}^{\mathbf{0.8418}}{\mathit{x}}_{\mathbf{5}}^{\mathbf{0.6897}}{\mathit{x}}_{\mathbf{6}}^{\mathbf{0.1753}}{\mathit{x}}_{\mathbf{8}}^{\mathbf{0.4635}}{\mathit{x}}_{\mathbf{11}}^{\mathbf{0.0456}}{\mathit{x}}_{\mathbf{12}}^{\mathbf{0.5124}}}$$

(8)

well profit taking into account the cost of the polymer:

$${\mathit{Y}}_{\mathbf{4}}=\mathbf{0.1779} \frac{{\mathit{x}}_{\mathbf{4}}^{\mathbf{1.6104}}{\mathit{x}}_{\mathbf{6}}^{\mathbf{0.0487}}{\mathit{x}}_{\mathbf{7}}^{\mathbf{0.017}}{\mathit{x}}_{\mathbf{8}}^{\mathbf{0.0217}}{\mathit{x}}_{\mathbf{9}}^{\mathbf{1.1505}}{\mathit{x}}_{\mathbf{10}}^{\mathbf{1.0366}}{\mathit{x}}_{\mathbf{12}}^{\mathbf{0.0943}}}{{\mathit{x}}_{\mathbf{1}}^{\mathbf{0.0078}}{\mathit{x}}_{\mathbf{2}}^{\mathbf{0.2062}}{\mathit{x}}_{\mathbf{3}}^{\mathbf{1.1539}}{\mathit{x}}_{\mathbf{5}}^{\mathbf{0.9984}}{\mathit{x}}_{\mathbf{11}}^{\mathbf{0.1685}}}$$

(9)

Estimates of the contributions of these factors in regression models (6)–(9) are found using the following expression [22]:

$${\mathsf{\alpha }}_{⚜}=\frac{100\%\left|a_i{x}_i^{*}\right|}{{{\displaystyle \sum }}_{j=1}^2\left|a_j{x}_j^{*}\right|}$$

(10)

where $x_j^{*}$ is the maximum value of the j-th variable in the entire sample.

Quantification of the degree of conformity is determined by the measure of identity using the following formula, the values of which should vary from zero to one:

$$\mathrm{I}=\frac{1}{1+\frac{{{\displaystyle \sum }}_{i=1}^N{\left(Y_{\mathrm{pac}ч i} - Y\right)}^2}{{{\displaystyle \sum }}_{i=1}^N{\left(Y_{\mathrm{pac}ч i} - \overline{Y}\right)}^2}},$$

(11)

The results of the calculations and estimation of errors showed sufficient reliability of the constructed models.

3. Results and Discussions

3.1. Analysis of the Factors Influencing the Efficiency of Isolation of Water Inflows in Production Wells by Sediment-Gelling Compositions

To increase the efficiency of waterproofing works, it is necessary to comprehensively study the impact of geological-physical, technical, and technological factors on the process of limiting water inflows.

For this purpose, the results of CCD treatments with a polymer solution at one of the oil-producing enterprises were collected, processed, and analyzed. Equations were constructed that express the dependences of performance indicators. Based on the analysis performed, the following were selected as geological, physical, and technical factors characterizing the bottomhole zone (BZ) and the well: permeability (x₁), formation compartmentalization (x₂), reservoir x₃, and bottomhole x₄ pressures, oil viscosity in reservoir conditions x₅, current oil recovery factor (ORF) x₆, average oil production x₇, water x₈ 3 months before well treatment with polymer, water cut x₉, well filter length x₁₀ (Table 1). The following were selected as criteria characterizing the effect of isolation of water inflows during the treatment of the wellbore with a polymer-based solution: the duration of the isolation effect Y₁, the amount of additionally produced oil Y₂, the volume of limited water Y₃, the profit from the well, taking into account the cost of the polymer Y₄ (

Table 2. Values of technological indicators for wells of field X.

No.	Y1	Y2	Y3	Y4
	Effect Duration, Months	Average Values of Additional Oil Produced and Water Restrictions during the Effect Time		Profit from the Well, Taking into Account the Cost of the Polymer, Thousand Tenge
		Oil, t	Water, m3
1.	8	37.1	48	257.1
2.	4	32.3	75	714.9
3.	6	30.6	27	1141.2
4.	9	34.5	11	542.2
5.	7	29.2	45	646.3
6.	6	28.3	21.8	626.3
7.	2	18.8	241	1216.1
8.	14	39.5	22	574.2
9.	7	33.1	18	875.2
10.	5	24.7	35	568

). When analyzing and refining at some stage of the models, it is more convenient to use expressions in a multiplicative form.

As shown in earlier works [15,16,17,18,19,20], for effective and long-term limitation of water inflow by polymer solutions, it is necessary to create a polymer screen in the CCD in the area of action of formation shear rates (0.1–1 s⁻¹) or shear rates of the corresponding order. This area for each well, depending on reservoir and technical conditions, will be located in the PZ at a different distance from the wellbore. Therefore, when assessing the influence of various factors on the efficacy of water inflow isolation using a polymer solution, along with geological, physical, and technical factors, certain technological parameters were also taken into account. These parameters include the quantity of injected polymer per 1 m of filter x₁₁ and the percentage of wellbore zone area filled with the polymer solution in the region of formation shear rates x₁₂.

Analysis of the data shows that almost all wells, the wellbore of which was treated with a polymer solution, have limited water inflow and production of additionally produced oil. At the same time, the volume of restricted water, the amount of additional oil produced, and the duration of the effect for each of the treated wells differ significantly.

To find out the reasons for the different efficiency of CCD treatment with a polymer solution, we first analyzed the influence of each of the above geological-physical, technical, and technological factors on the duration of the isolation effect, the amount of additional oil produced, the volume of limited water and the well profit, taking into account the cost of the polymer. To do this, field data were analyzed by considering the impact of individual factors on the efficiency of isolation work, as well as the multiple, simultaneous influence of all the factors under consideration.

In this case, statistical analysis was carried out in order to build models expressing the relationship between the factors noted in Table 1 and Table 2 output variables, i.e., indicators of the effectiveness of isolation work—the duration of the effect, the value of the amount of additional oil produced, the volume of water inflow limitation during the time of the effect and the profit from the well.

Upon implementing the linear regression program, multiple correlation equations were obtained. It should be noted that in scientific research using regression analysis, a comparative assessment of the degree of importance (significance) of input (independent) variables is considered necessary according to the degree of their influence on the output. In articles [20,21,22,23], in order to work out the decision-making on shutting down production wells for water, based on the random search method, an approach is proposed for estimating weight coefficients. The same factors were considered as variables, taking into account pressure, well water cut, and residual oil saturation. The purpose of using this algorithm is to improve the accuracy of the well selection decision. Since the weight of a factor or the importance of a feature is part of the calculation, this will definitely affect the final result.

The work [21] presents a method for calculating the estimates of the significance of the factors of linear regression models. In [22], the assessment of the significance of factors in the regression model is carried out by calculating the contributions of independent factors. The object of analysis in this paper is the factors that characterize the geological and physical conditions and the technological process, which have a joint effect on the performance indicators of waterproofing works (output variables). The computation results of the weight contributions are shown in

Table 3. Results of calculations of weight contributions.

Model	Weight Contribution
	α⚜	α⚜	α⚜	α⚜	α⚜	α⚜	α⚜	α⚜	α⚜	α⚜⚜	α⚜⚜	α⚜⚜
$Y_1$	1	3.7	21.9	21.6	2.1	5.3	2.1	0.3	21.5	10.4	0.3	9.8
$Y_2$	2	0.6	11.4	15.3	5.2	0.8	4.6	6.8	41	6.6	1	4.9
$Y_3$	7	1.9	18.6	21	6.5	3.8	3.9	9.8	5.5	12.9	0.52	8.6
$Y_4$	0.2	2.79	22.64	31.89	8.32	0.93	0.2	0.41	17.58	11.87	1.70	1.41

α_⚜, α_⚜⚜—factors in regression models

. Calculations were performed using Equation (10).

As can be seen from Table 3, such factors as reservoir pressure, bottomhole pressure, oil viscosity in reservoir conditions, water cut, and filter length make the greatest contribution to the values of the effectiveness of measures to limit water inflows.

After regression equations were obtained, the degree of compliance of the calculated values with the actual ones was established. This is also confirmed by the results of calculations of the degrees of identity, the values of which are given in

Table 4. The values of the regression equation coefficients, the contribution of each factor to the isolation efficiency criteria, and the overall assessment of the contribution of each factor to the isolation effect (Negative values mean that as this parameter increases, the output parameter decreases, and, conversely, as it decreases, the output parameter increases (i.e., feedback).

Factors	Regression Equation Coefficients				The Contribution of Each Factor to the Corresponding Isolation Effect Criterion, %				Overall Assessment of Each Factor Contribution to the Isolation Effect, %
	Y1	Y2	Y3	Y4	α1	α2	α3	α4	α
a0	2.088	4.011	2.673	2.673	-	-	-	-	-
X1	0.0279	−0.0329	−0.2207	0.0078	1	2	7	0.2	1.294
X2	0.2117	0.0199	−0.1253	−0.2062	3.7	0.6	1.9	2.79	1.852
X3	0.8552	0.2676	−0.8418	−1.1539	21.9	11.4	18.6	22.64	18.007
X4	−0.8354	−0.3559	0.9415	1.6104	21.6	15.3	21	31.89	21.69
X5	0.1911	0.2869	−0.6897	−0.9984	2.1	5.2	6.5	8.32	4.93
X6	0.2134	−0.0187	−0.1753	0.0487	5.3	0.8	3.8	0.93	1.967
X7	−0.1116	−0.1458	0.2407	0.017	2.1	4.6	3.9	0.2	1.657
X8	−0.0122	0.1664	−0.4635	0.0217	0.3	6.8	9.8	0.41	1.692
X9	−1.0794	−1.2373	+0.3228	1.1505	21.5	41	5.5	17.58	17.086
X10	−0.6955	−0.2664	1.0051	1.0366	10.4	6.6	12.9	11.87	10.125
X11	−0.0266	−0.0446	−0.0456	−0.1685	0.3	1	0.52	1.70	0.718
X12	0.5022	0.15	−0.5124	0.0943	9.8	4.9	8.6	1.41	4.912
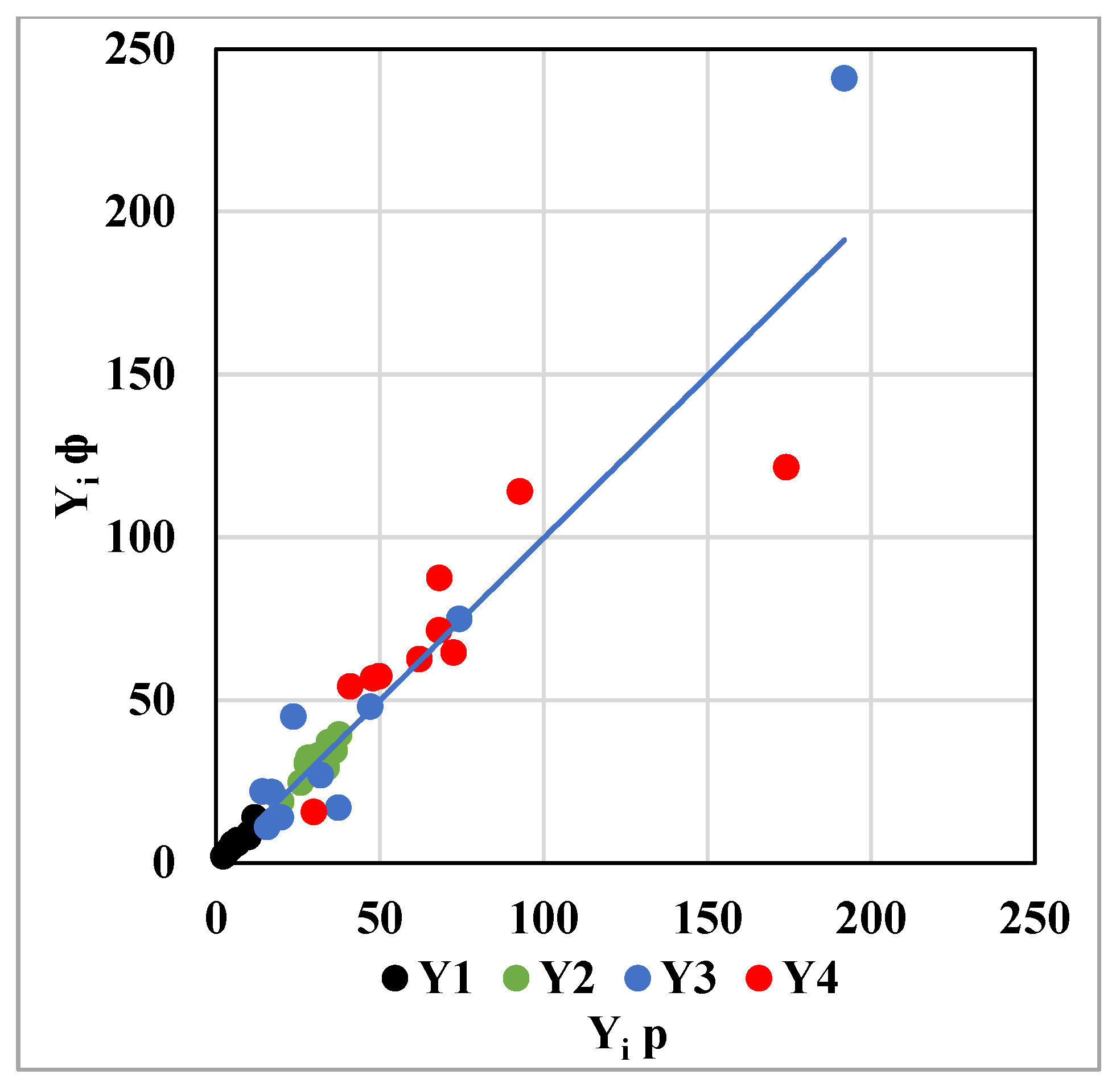
Degree of identity	0.881895	0.761143	0.884733	0.779909	-	-	-		-

.

Figure 1 shows a graph and points showing their relative location on the coordinate plane, which allows us to visually assess the coincidence of the actual and calculated values of the output parameters (efficiency indicators of insulation work). Reliability is evaluated using the degree of identity, the values of which are between zero and one. The given values are within acceptable limits, i.e., close to one. At the same time, Figure 1 shows a graph of the correspondence between the calculated and actual values for all four models. The accumulation of points near the bisector on the coordinate plane also indicates the reliability of the results. We also calculated the errors, both absolute and relative. The maximum error was about 20% in one case, and the average did not exceed 10%.

The resulting equations made it possible to establish the degree of influence of each factor, within the limits of its change considered in this case, on the duration of the isolation effect, the amount of additionally produced oil, and the volume of limited water (Table 3).

As can be seen from Table 3, depending on the criterion characterizing the effectiveness of limiting water inflows with polymer solutions, certain geological-physical, technical, or technological factors come to the fore. The change in the significance of one or another factor is directly related to the physicochemical processes occurring in the CCD. For example, the greatest influence on the duration of the Y₁ isolation effect is exerted by factors affecting the longevity of the polymer screen in the CCD and the effectiveness of water inflow isolation: reservoir and bottomhole pressures—21.9 and 21.6%, respectively, water cut 21.5%, filter length 10.4%, current oil recovery factor—5.3%, etc. The amount of oil produced Y₂ is most affected by the factors that determine its significance: water cut is in the first place—41%, then in terms of the degree of influence, bottomhole and reservoir pressures follow—respectively 15.3 and 11.4%, well water flow rate—6.8%, filter length—6.6%, etc. The volume of limited water Y₃ is most affected primarily by bottomhole and reservoir pressures—21 and 18.6%, respectively, then the filter length—12.9%, then the well water flow rate—9.8%, etc.

Bottomhole and reservoir pressures take the first place in terms of the degree of influence on well profit, taking into account the cost of polymer Y₄—respectively 31.89 and 22.64%, followed by water cut—17.58%, filter length—11.87%, oil viscosity in reservoir conditions—8.32%, etc. To assess the overall impact of each factor on the effectiveness of water inflow isolation using polymer solutions, we will use the approach previously applied in [23]. In this case, the overall score α is the mean proportional to the partial scores [23]:

$$\alpha =\sqrt[\mathbf{4}]{{\alpha }_{\mathbf{1}}{\alpha }_{\mathbf{2}}{\alpha }_{\mathbf{3}}{\alpha }_{\mathbf{4}}},$$

(12)

where $\mathsf{\alpha }$₁, $\mathsf{\alpha }$₂, $\mathsf{\alpha }$₃,$\mathsf{\alpha }$₄—the contribution of each factor to the corresponding isolation effect criterion Y1, Y₂, Y₃.

As can be seen from Table 4, the first place (in%) in terms of the degree of influence on the overall effect of water isolation by a polymer solution, in this case, is taken by such a factor as the current recovery factor—11.11, then the well flow rate for oil—9.95, then the bottomhole and reservoir pressures—9.6 and 9.55, well water flow rate—9.23, amount of polymer pumped per 1 m of filter—9.08, filling with CCD polymer—6.26, filter length—6.06, water cut—3.96, permeability—3.6, oil viscosity in reservoir conditions—3.38 and formation compartmentalization—1.84.

Thus, a comprehensive analysis of the geological, physical, technical, and technological factors that characterize the process of isolating water inflows with polymer solutions showed that when choosing wells for work to limit water inflows, in order to achieve a high effect, it is necessary, first of all, to take into account, in terms of their significance, such geological, physical, and technical factors as the current oil recovery factor, well oil production rate, bottomhole and reservoir pressure, well water flow rate. Orientation mainly on such a factor as water cut, which is considered a priority when choosing a well for work to limit water inflow, can lead to a decrease in their efficiency. The analysis also showed that the correctly chosen amount of the polymer solution injected into the wellbore zone significantly increases the magnitude and duration of the water inflow isolation effect.

3.2. Making Decisions on the Choice of Technology for Waterproofing Works

Based on the formulation and the need to solve our problem, the challenge lies in this case in decision-making under conditions of uncertainty. This is justified by multi-criteria and multi-factoriality. As follows from the analysis above, in this case, it is necessary to make a decision that would satisfy all four criteria. Namely, it (the decision) should reflect such a set of technological options that, for given geological conditions, will achieve the maximum duration of the effect, additional oil production, volume of limited water, profit from the well, taking into account the cost of the polymer. To do this, first, calculations were carried out on the basis of the obtained models. The best options were determined using the four criteria noted (representing goals and restrictions) using the theory of fuzzy sets proposed by Zadeh. According to the provisions of this theory, the membership functions of sets of goals and restrictions were evaluated based on the desire to achieve the maximum of each criterion, in connection with which the value of the membership function, close to one, was assigned to the maximum value of each of them. The expression for defining membership functions looks like this:

$${\mu }_i=\frac{1}{1+9e^{-a{Y}_i}}$$

(13)

The values of the parameter a of Expression (13) for each of the criteria are determined, the corresponding expressions are given in

Table 5. Indicators of the effectiveness of measures to limit water inflows and the corresponding membership functions.

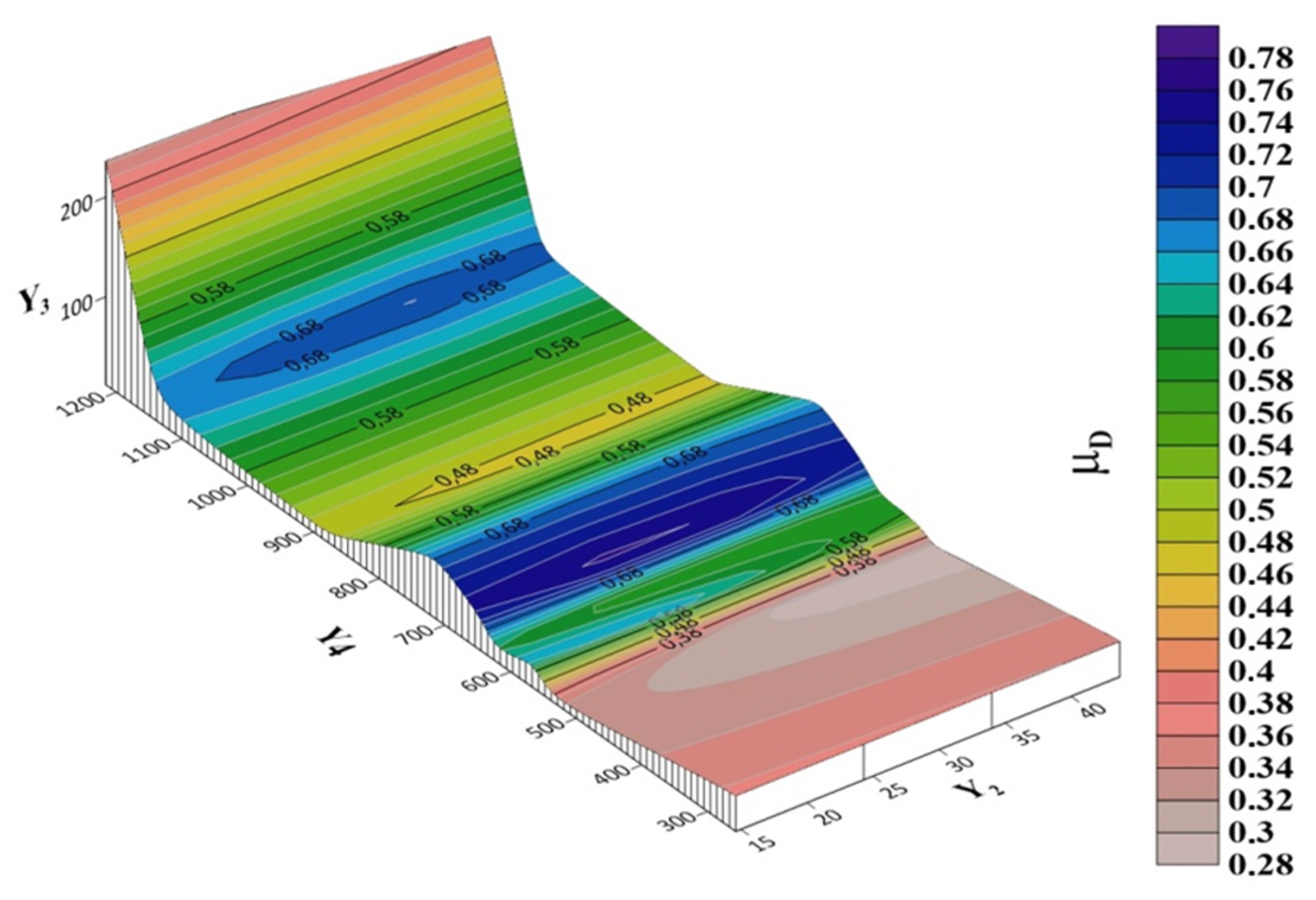
N	Y1	Y2	Y3	Y4	${\mathsf{\mu }}_{\mathbf{1}}\mathbf{=}\frac{\mathbf{1}}{\mathbf{1}\mathbf{+}\mathbf{9}{\mathit{e}}^{\mathbf{-}\mathbf{0.74}{\mathit{y}}_{\mathbf{1}}}}$	${\mathsf{\mu }}_{\mathbf{2}}\mathbf{=}\frac{\mathbf{1}}{\mathbf{1}\mathbf{+}\mathbf{9}{\mathit{e}}^{\mathbf{-}\mathbf{0.12}{\mathit{y}}_{\mathbf{2}}}}$	${\mathsf{\mu }}_{\mathbf{3}}\mathbf{=}\frac{\mathbf{1}}{\mathbf{1}\mathbf{+}\mathbf{9}{\mathit{e}}^{\mathbf{-}\mathbf{0.11}{\mathit{y}}_{\mathbf{3}}}}$	${\mathsf{\mu }}_{\mathbf{4}}\mathbf{=}\frac{\mathbf{1}}{\mathbf{1}\mathbf{+}\mathbf{9}{\mathit{e}}^{\mathbf{-}\mathbf{0.006}{\mathit{y}}_{\mathbf{4}}}}$	$\begin{array}{c}{\mathsf{\mu }}_{\mathit{D}}\\ =\mathbf{min}\left({\mathsf{\mu }}_{\mathbf{1}},{\mathsf{\mu }}_{\mathbf{2}},{\mathsf{\mu }}_{\mathbf{3}},{\mathsf{\mu }}_{\mathbf{4}}\right)\end{array}$
1	8	37.1	48	257.1	0.975722	0.899162	0.963551	0.361174	0.361
2	4	32.3	75	714.9	0.678784	0.834876	0.998261	0.911067	0.679
3	6	30.6	27	1141.2	0.902111	0.805284	0.706975	0.993467	0.707
4	9	34.5	11	542.2	0.988225	0.867683	0.280246	0.774495	0.28
5	7	29.2	45	646.3	0.950605	0.778022	0.94944	0.869054	0.778
6	6	28.3	21,8	626.3	0.902111	0.759108	0.571493	0.853967	0.571
7	2	18.8	241	1216.1	0.326399	0.506227	1	0.995923	0.326
8	14	39.5	22	574.2	0.9997	0.922128	0.577067	0.807891	0.577
9	7	33.1	18	875.2	0.950605	0.847503	0.463752	0.965813	0.464
10	5	24.7	35	568	0.815258	0.673109	0.857261	0.801728	0.673

, and membership functions are calculated using them.

To make a decision, membership functions of the solution set are found. Each of the membership functions in the table is a “membership function of a set of goals (or constraints)”. The set of solutions, according to the theory of fuzzy sets, is a set that is the intersection of these sets. The intersection of fuzzy sets is called some fuzzy set, the membership function, which for this case looks like this:

$${\mu }_D=\min ({\mu }_{1,}{\mu }_{2,}{\mu }_{3,}{\mu }_4)$$

(14)

The table in the last column shows the values of the membership functions of the decision set, each row of which represents the smallest value among the criteria membership sets. The optimal solution will be (highlighted in red) the line corresponding to the largest value of the membership function of the set of solutions. Figure 2 shows a geometric representation of the surface of change in the membership function of a set of solutions depending on three (additional oil production, volume of restricted water, profit per well, considering the cost of the polymer) indicators of the effectiveness of water control. The dark blue color on this surface corresponds to the optimal solution or close to it.

Thus, the largest value of the membership function of the set of solutions in the set of calculated data corresponds to the best solution, which corresponds to the fifth row of Table 1.

4. Conclusions

The article presents the calculation scheme and the results of building and evaluating models using the methods of mathematical statistics, relevant criteria, and approaches, and field data. Reliability assessment using the degree of identity showed that its values are within acceptable limits, i.e., close to one. The accumulation of points near the bisector on the coordinate plane on the graph of the correspondence between the calculated and actual values of the efficiency indicators also indicates the reliability of the results. The performed analysis showed that different factors affect the efficiency of water inflow isolation in different ways: With a change in one group of physical, geological, technical, and technological factors characterizing the wellbore well and processing technology, the values of the indicators selected as criteria for the effectiveness of isolation of water inflows with polymer solutions increase, the other—decrease, and the third—an increase or decrease in values is selective.

To determine the quantitative effect of these factors on the efficiency of isolation of water inflows by polymer solutions, a program of correlation analysis was applied, as a result of which mathematical models were derived that express the dependence of the duration of the isolation effect, the amount of additionally produced oil, the volume of limited water and the profit from technological measures to limit water inflows on geological, physical, technical, and technological factors. Summing up the results of the research, we can formulate the following conclusions.

• The performed analysis showed that with a change in one group of geological-physical, technical, and technological factors that characterize the bottomhole zone, the well, and the treatment technology, the values of the indicators selected as criteria for the effectiveness of isolation of water inflows by polymer solutions, increase, the other—decrease, and the third—the increase or decrease in values is selective. For example, an increase in the permeability and compartmentalization of the reservoir, reservoir pressure, oil viscosity in reservoir conditions, the current oil recovery factor, and coverage of the CCD with a polymer solution leads to an increase and an increase in bottomhole pressure, well flow rates for oil and water, water cut, filter length and amount of polymer per 1 m of filter leads to a decrease in the duration of the water inflow isolation effect.
• With an increase in reservoir compartmentalization, reservoir pressure, oil viscosity in reservoir conditions, well water flow rate and coverage of the CCD with a polymer solution, it leads to an increase, and an increase in reservoir permeability, bottom hole pressure, current oil recovery factor, well oil production rate, water cut, filter length and polymer amount per 1 m of filter—to a decrease in additional oil production. In the same way, it is possible to evaluate the influence of factors on other indicators of the effectiveness of waterproofing works.
• As a result of the performed analysis of changes in the efficiency indicators of the water inflow limitation technology, estimates were given for the parameters of the studied dependencies—the duration of the effect, additional oil production, volume of limited water, well profits taking into account the cost of the polymer by considering them as functions of geological and physical conditions and technological measures. Dependences of the noted indicators on the characteristics of geological and physical conditions and technological measures are constructed.
• A methodology has been developed in which, using the methods of mathematical statistics and fuzzy logic, an algorithm for evaluating optimal technological solutions according to four criteria is implemented based on information about the geological and physical conditions of the field and the experience of implementing geological and technical measures to limit water inflows, including the analysis of factors, their weighted contribution, building models, statistical evaluation of reliability indicators, decision-making taking into account uncertainty.