TiO2 Assisted Photodegradation for Low Substrate Concentrations and Transition Metal Electron Scavengers
Abstract
1. Introduction
2. Materials and Methods
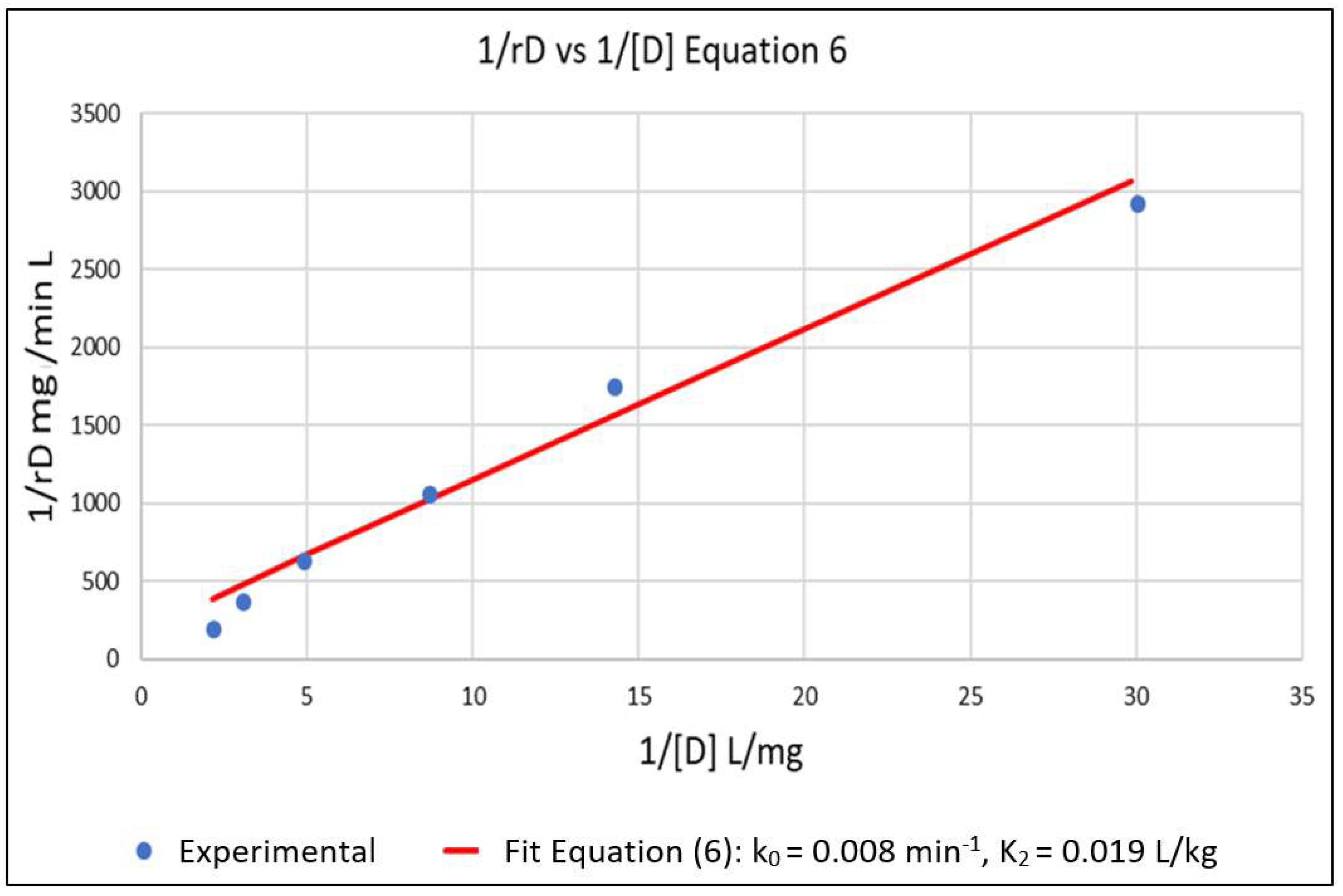
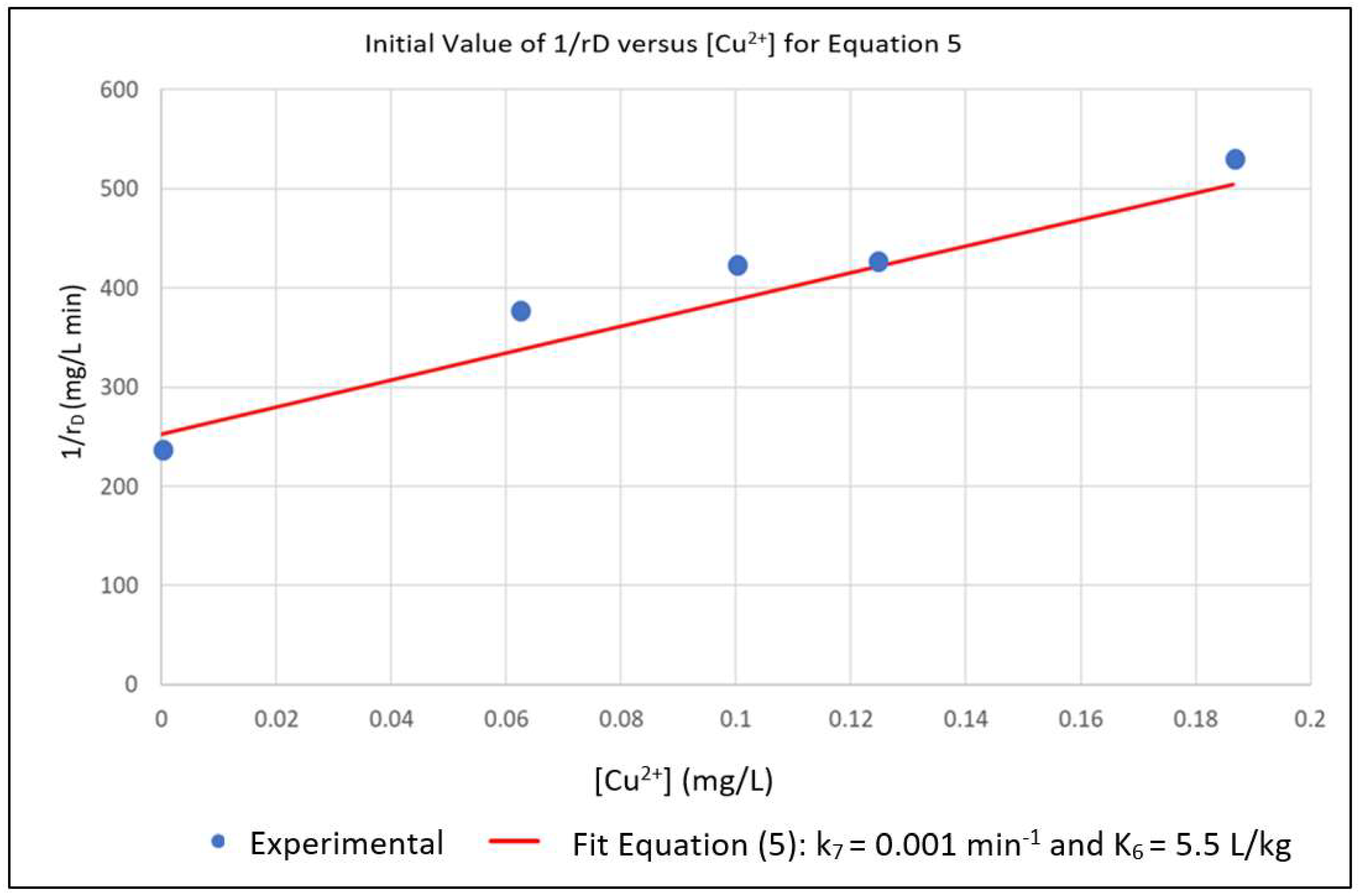
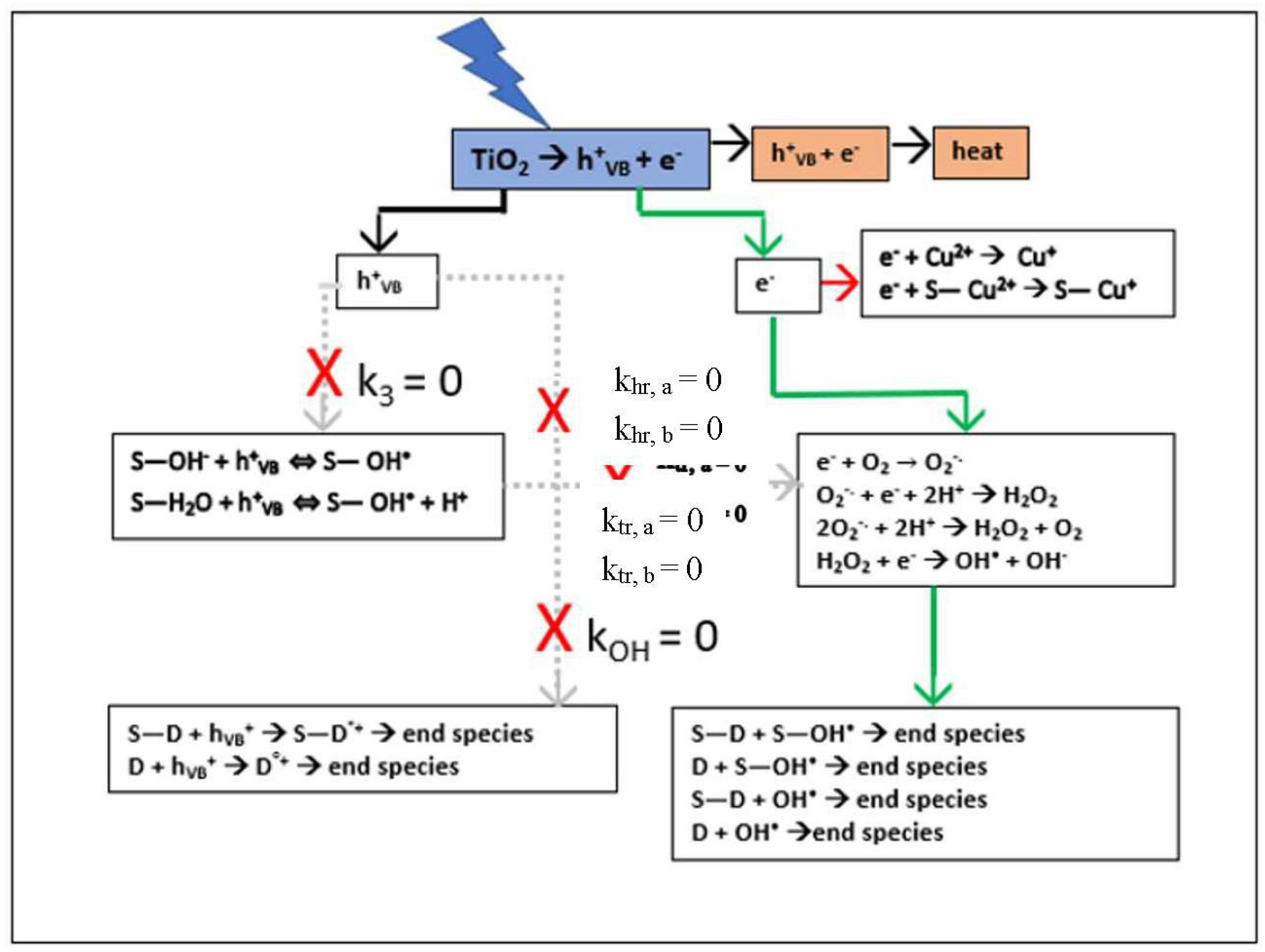
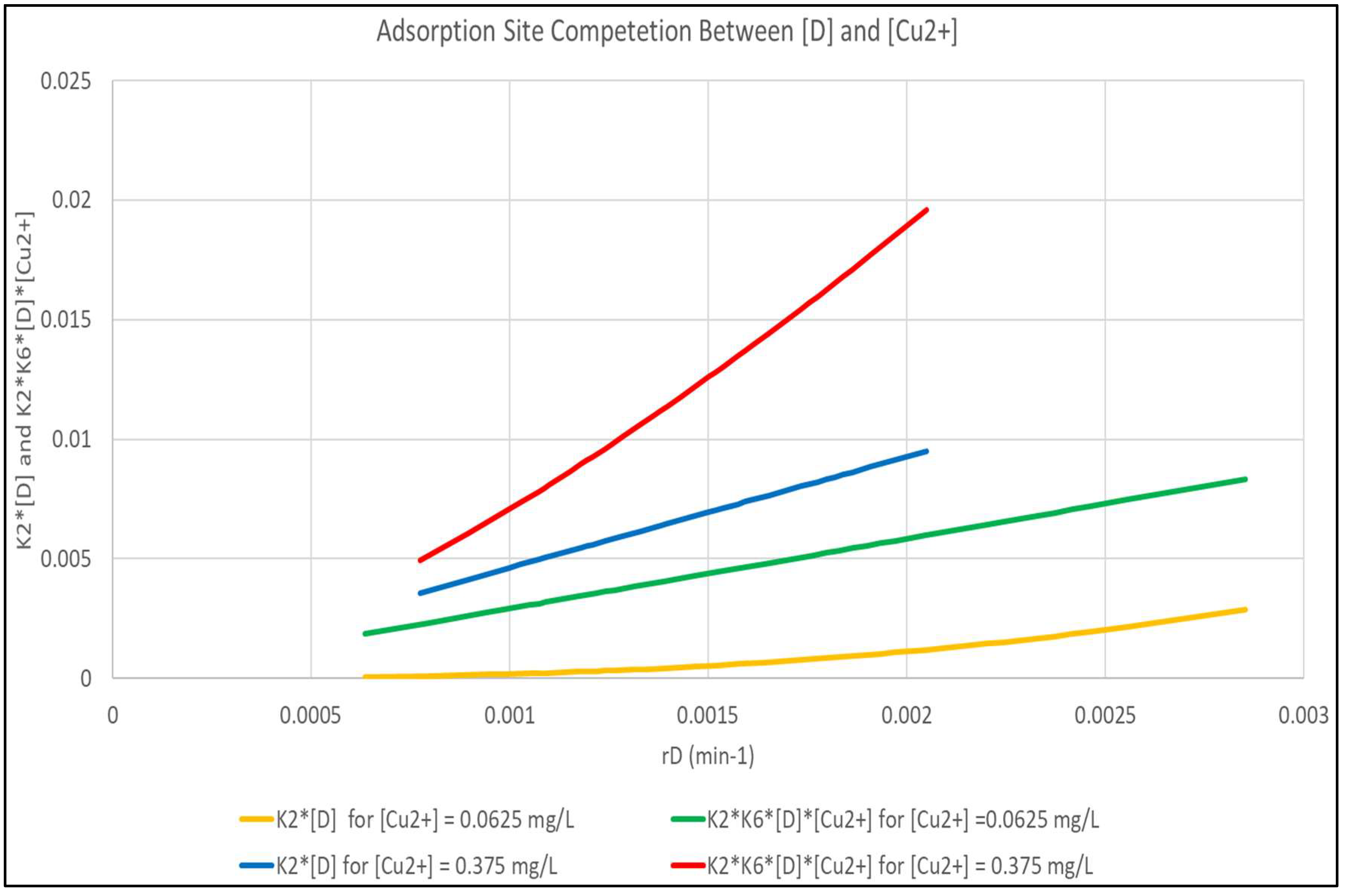
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A
References
- Ibhadon, A.O.; Fitzpatrick, P. Heterogeneous Photocatalysis: Recent Advances and Applications. Catalysts 2013, 3, 189–218. [Google Scholar] [CrossRef]
- Salimi, M.; Esrafili, A.; Gholami, M.; Jonidi Jafari, A.; Rezaei Kalantary, R.; Farzadkia, M.; Kermani, M.; Sobhi, H.R. Contaminants of emerging concern: A review of new approach in AOP technologies. Environ. Monit. Assess. 2017, 189, 414. [Google Scholar] [CrossRef] [PubMed]
- Lazar, M.; Varghese, S.; Nair, S. Photocatalytic Water Treatment by Titanium Dioxide: Recent Updates. Catalysts 2012, 2, 572–601. [Google Scholar] [CrossRef]
- Mills, A.; LeHunte, S. An overview of semiconductor photocatalysis. J. Photochem. Photobiol. A 1997, 108, 1–35. [Google Scholar] [CrossRef]
- Sunada, K.; Watanabe, T.; Hashimoto, K. Studies on photo-killing of bacteria on TiO2 thin film. J. Photochem. Photobiol. A 2003, 156, 227–233. [Google Scholar] [CrossRef]
- Paleologou, A.; Marakas, H.; Xekoukoulotakis, N.P.; Moya, A.; Vergara, Y.; Kalogerakis, N.; Gikas, P.; Mantzavinos, D. Disinfection of water and wastewater by TiO2 photocatalysis, sonolysis and UV-C irradiation. Catal. Today 2007, 129, 136–142. [Google Scholar] [CrossRef]
- Khairy, M.; Zakaria, W. Effect of metal-doping of TiO2 nanoparticles on their photocatalytic activities toward removal of organic dyes. Egypt. J. Pet. 2014, 23, 419–426. [Google Scholar] [CrossRef]
- Chan, P.Y.; El-Din, M.G.; Bolton, J.R. A solar-driven UV/Chlorine advanced oxidation process. Water Res. 2012, 46, 5672–5682. [Google Scholar] [CrossRef] [PubMed]
- Oller, I.; Malato, S.; Sánchez-Pérez, J. Combination of Advanced Oxidation Processes and biological treatments for wastewater decontamination—A review. Sci. Total Environ. 2011, 409, 4141–4166. [Google Scholar] [CrossRef] [PubMed]
- Sharma, V.K.; Triantis, T.M.; Antoniou, M.G.; He, X.; Pelaez, M.; Han, C.; Song, W.; O’Shea, K.E.; Armah, A.; Kaloudis, T.; et al. Destruction of microcystins by conventional and advanced oxidation processes: A review. Sep. Purif. Technol. 2012, 91, 3–17. [Google Scholar] [CrossRef]
- Cortez, P.; Teixeira, P.; Oliveira, R.; Mota, M. Evaluation of Fenton and ozone-based advanced oxidation processes as mature landfill leachate pre-treatments. Environ. Manag. 2011, 92, 749–755. [Google Scholar] [CrossRef] [PubMed]
- Blake, D. Bibliography of Work on the Heterogeneous Photocatalytic Removal of Hazardous Compounds from Water and Air; National Renewable Energy Laboratory: Denver, CO, USA, 2001; pp. 1–158. [Google Scholar]
- Hisanaga, T.; Harada, K.; Tanaka, K. Photocatalytic degradation of organochlorine compounds in suspended TiO2. J. Photochem. Photobiol. A Chem. 1990, 56, 113–118. [Google Scholar] [CrossRef]
- Ohtani, B.; Ueda, Y.; Nishimoto, S.; Kagiya, T.; Hachisuka, H. Photocatalytic oxidative decomposition of fluoroalkenes by titanium dioxide. J. Chem. Soc. Perkin Trans. 2 1990, 11. [Google Scholar] [CrossRef]
- Minero, C.; Aliberti, C.; Pelizzetti, E.; Terzian, R.; Serpone, N. Kinetic studies in heterogeneous photocatalysis. 6. AM1 simulated sunlight photodegradation over titania in aqueous media: A first case of fluorinated aromatics and identification of intermediates. Langmuir 1991, 7, 928–936. [Google Scholar] [CrossRef]
- Matthews, R. Photocatalytic oxidation of chlorobenzene in aqueous suspensions of titanium dioxide. J. Catal. 1986, 20, 569–578. [Google Scholar] [CrossRef]
- Pruden, A.L.; Ollis, D.F. Photoassisted heterogeneous catalysis: The degradation of trichloroethylene in water. J. Catal. 1983, 82, 404–417. [Google Scholar] [CrossRef]
- Ollis, D.F.; Hsiao, C.Y.; Budiman, L.; Lee, C.L. Heterogeneous photoassisted catalysis: Conversions of perchloroethylene, dichloroethane, chloroacetic acids, and chlorobenzenes. J. Catal. 1984, 88, 89–96. [Google Scholar] [CrossRef]
- Bahnemann, D.W.; Moenig, J.; Chapman, R. Efficient photocatalysis of the irreversible one-electron and two-electron reduction of halothane on platinized colloidal titanium dioxide in aqueous suspension. J. Phys. Chem. 1987, 91, 3782–3788. [Google Scholar] [CrossRef]
- Serphone, N.; Pelizzetti, E. (Eds.) Photocatalysis—Fundamentals and Applications; Wiley Interscience: New York, NY, USA, 1989. [Google Scholar]
- Fox, M.A. Organic heterogeneous photocatalysis: Chemical conversions sensitized by irradiated semiconductors. Account. Chem. Res. 1983, 16, 314–321. [Google Scholar] [CrossRef]
- Hoffmann, M.R.; Martin, S.T.; Choi, W.; Bahnemann, D.W. Environmental Applications of Semiconductor Photocatalysis. Chem. Rev. 1995, 95, 69–96. [Google Scholar] [CrossRef]
- Nosaka, Y.; Marye, A.F. Kinetics for Electron Transfer from Laser-Pulse Irradiated Colloidal Semiconductors to Adsorbed Methylviologen: Dependence of the Quantum Yield on Incident Pulse Width. J. Phys. Chem. 1988, 92, 1893–1897. [Google Scholar] [CrossRef]
- Al-Sayyed, G.; D’Oliveira, J.-C.; Pichat, P. Semiconductor-Sensitized Photodegradation of 4-Chlorophenol in Water. J. Photochem. Photobiol. A Chem. 1991, 58, 99–114. [Google Scholar] [CrossRef]
- Sclafani, A.; Palmisano, L.; Schiavello, M. Influence of the preparation methods of titanium dioxide on the photocatalytic degradation of phenol in aqueous dispersion. J. Phys. Chem. 1990, 94, 829–832. [Google Scholar] [CrossRef]
- Qu, P.; Zhao, J.; Shen, T.; Hidaka, H. TiO2-assisted photodegradation of dyes: A study of two competitive primary processes in the degradation of RB in an aqueous TiO2 colloidal solution. J. Mol. Catal. A Chem. 1998, 129, 257–268. [Google Scholar] [CrossRef]
- Fox, M.; Dulay, M. Hetrogeneous Photocatalysis. Chem. Rev. 1993, 93, 341–357. [Google Scholar] [CrossRef]
- Brezova, V.; Stasko, A.; Lapcik, L., Jr. Electron paramagnetic resonance study of photogenerated radicals in titanium dioxide powder and its aqueous suspensions. J. Photochem. Photobiol. A Chem. 1991, 59, 115–121. [Google Scholar] [CrossRef]
- Howe, R.F.; Gratzel, M. Electron-Paramagnetic-Res Study of Hydrated Anatase under UV Irradiation. J. Phys. Chem. 1987, 91, 3906–3909. [Google Scholar] [CrossRef]
- Soria, J.; Conesa, J.C.; Auguliaro, V.; Palmisano, L.; Schiavello, M.; Sclafani, A. Dinitrogen photoreduction to ammonia over titanium dioxide powders doped with ferric ions. J. Phys. Chem. 1991, 95, 274–282. [Google Scholar] [CrossRef]
- Tanaka, K.; White, J.M. Characterization of Species Adsorbed on Oxidized and Reduced Anatase. J. Phys. Chem. 1982, 86, 4708–4714. [Google Scholar] [CrossRef]
- Peral, J.; Casado, J.; Doménech, J. Light-Induced Oxidation of Phenol over ZnO Powder. J. Photochem. Photobiol. A Chem. 1988, 44, 209–217. [Google Scholar] [CrossRef]
- Weir, A.; Westerhoff, P.; Fabricus, L.; Hristovski, K.; von Goetz, N. Titanium dioxide nanoparticles in food and personal care products. Environ. Sci. Technol. 2012, 46, 2242–2250. [Google Scholar] [CrossRef] [PubMed]
- Aarthi, T.; Madras, G. Photocatalytic Degradation of Rhodamine Dyes with Nano-TiO2. Ind. Eng. Chem. Res. 2007, 46, 7–14. [Google Scholar] [CrossRef]
- Turchi, C.S.; Ollis, D.F. Photocatalytic degradation of organic water contaminants: Mechanisms involving hydroxyl radical attack. J. Catal. 1990, 122, 178–192. [Google Scholar] [CrossRef]
- Nagaveni, K.; Sivalingam, J.; Hegde, M.S.; Giridhar, M. Photocatalytic Degradation of Organic Compounds over Combustion-Synthesized Nano-TiO2. Environ. Sci. Technol. 2004, 38, 1600–1604. [Google Scholar] [CrossRef] [PubMed]
- Chen, C.; Li, X.; Ma, W.; Zhao, J. Effect of Transition Metal Ions on the TiO2-Assisted Photodegradation of Dyes under Visible Irradiation: A Probe for the Interfacial Electron Transfer Process and Reaction Mechanism. J. Phys. Chem. B 2002, 106, 318–324. [Google Scholar] [CrossRef]
- Yu, L.; Achari, G.; Langford, C.H. Photocatalytic degradation of 2,4-D with a LED based photoreactor. In Proceedings of the 12th International Environmental Specialty Conference, Edmonton, Canada, 12–16 March 2012. [Google Scholar]
- Available online: http://www.comm-tec.com/Prods/mfgs/TurnerDesigns/application_notes_pdf/TD-700%20FAQ.pdf (accessed on 20 October 2017).


© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alsaud, H.A.; Abibat, A.E.; Painter, R.; Sharpe, L.; Hargrove, S.K. TiO2 Assisted Photodegradation for Low Substrate Concentrations and Transition Metal Electron Scavengers. ChemEngineering 2018, 2, 33. https://doi.org/10.3390/chemengineering2030033
Alsaud HA, Abibat AE, Painter R, Sharpe L, Hargrove SK. TiO2 Assisted Photodegradation for Low Substrate Concentrations and Transition Metal Electron Scavengers. ChemEngineering. 2018; 2(3):33. https://doi.org/10.3390/chemengineering2030033
Chicago/Turabian StyleAlsaud, Hassan A., Ahmed E. Abibat, Roger Painter, Lonnie Sharpe, and Samuel Keith Hargrove. 2018. "TiO2 Assisted Photodegradation for Low Substrate Concentrations and Transition Metal Electron Scavengers" ChemEngineering 2, no. 3: 33. https://doi.org/10.3390/chemengineering2030033
APA StyleAlsaud, H. A., Abibat, A. E., Painter, R., Sharpe, L., & Hargrove, S. K. (2018). TiO2 Assisted Photodegradation for Low Substrate Concentrations and Transition Metal Electron Scavengers. ChemEngineering, 2(3), 33. https://doi.org/10.3390/chemengineering2030033




