Dissolution of Trihexyltetradecylphosphonium Chloride in Supercritical CO2
Abstract
:1. Introduction
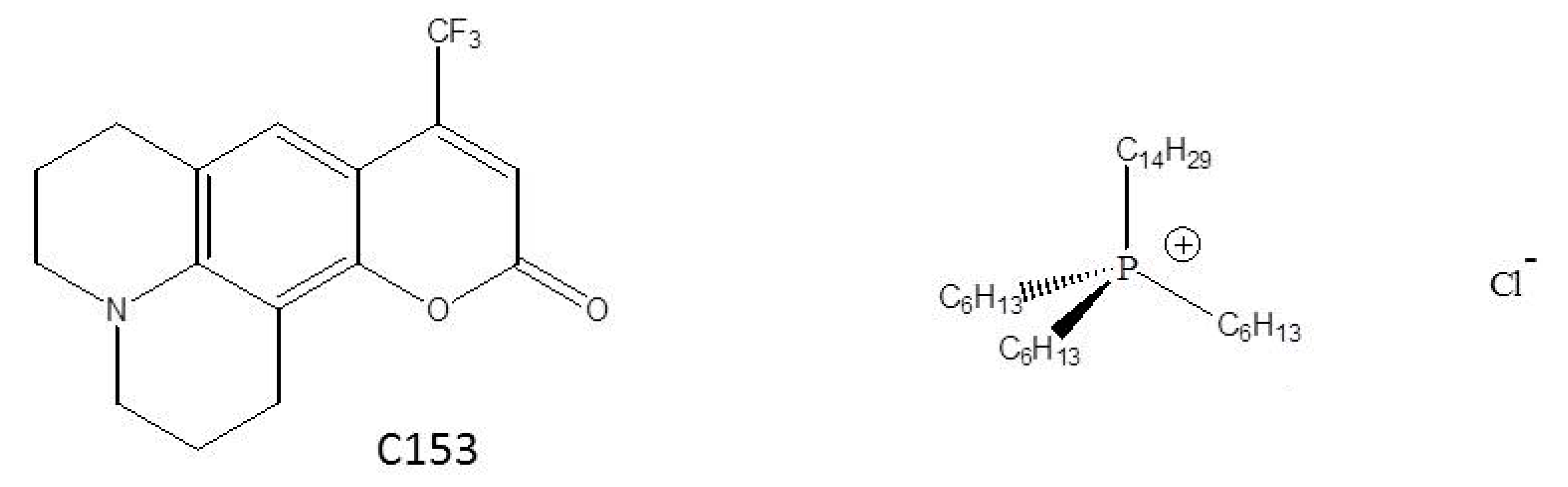
2. Materials and Methods
3. Results and Discussion
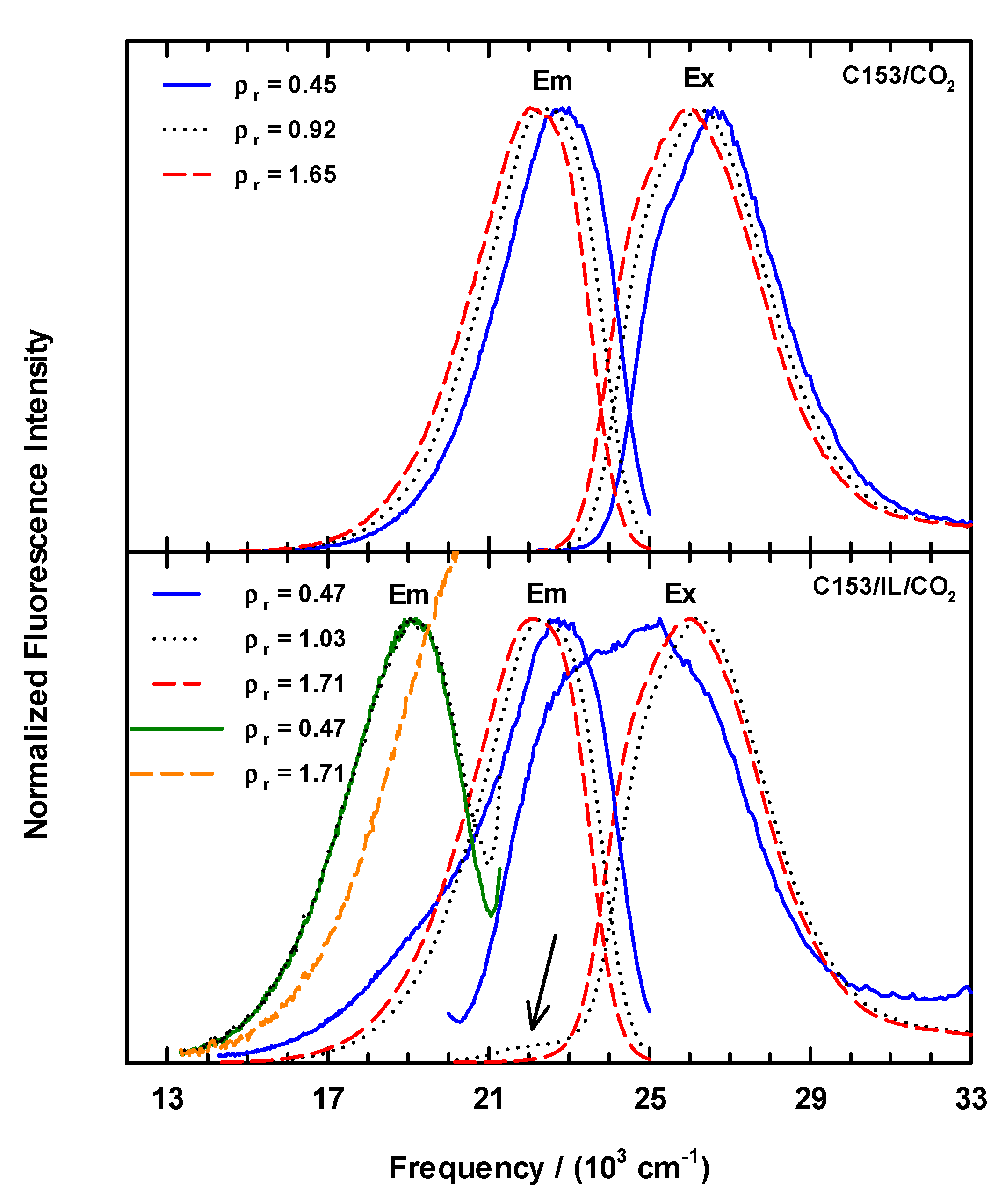
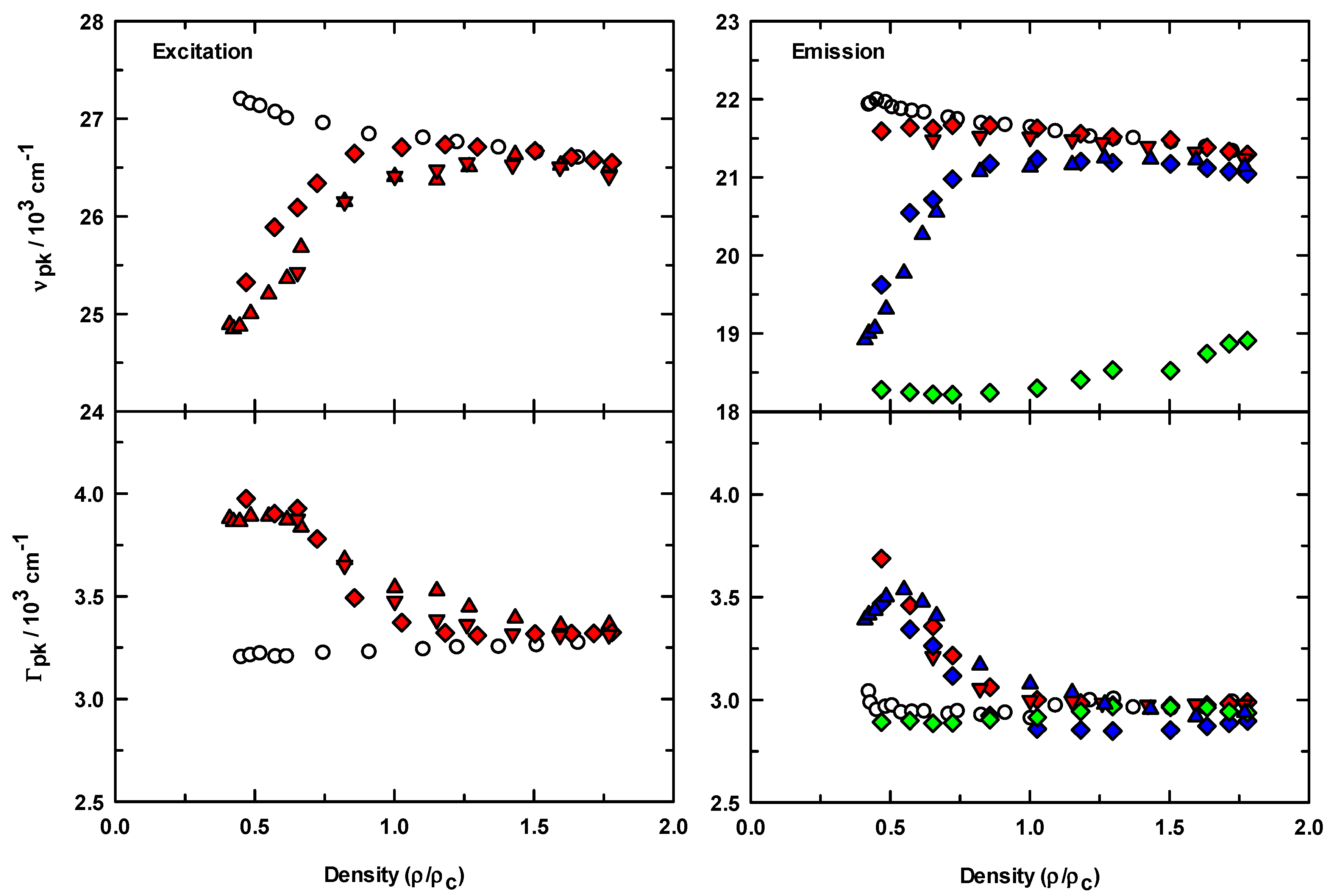
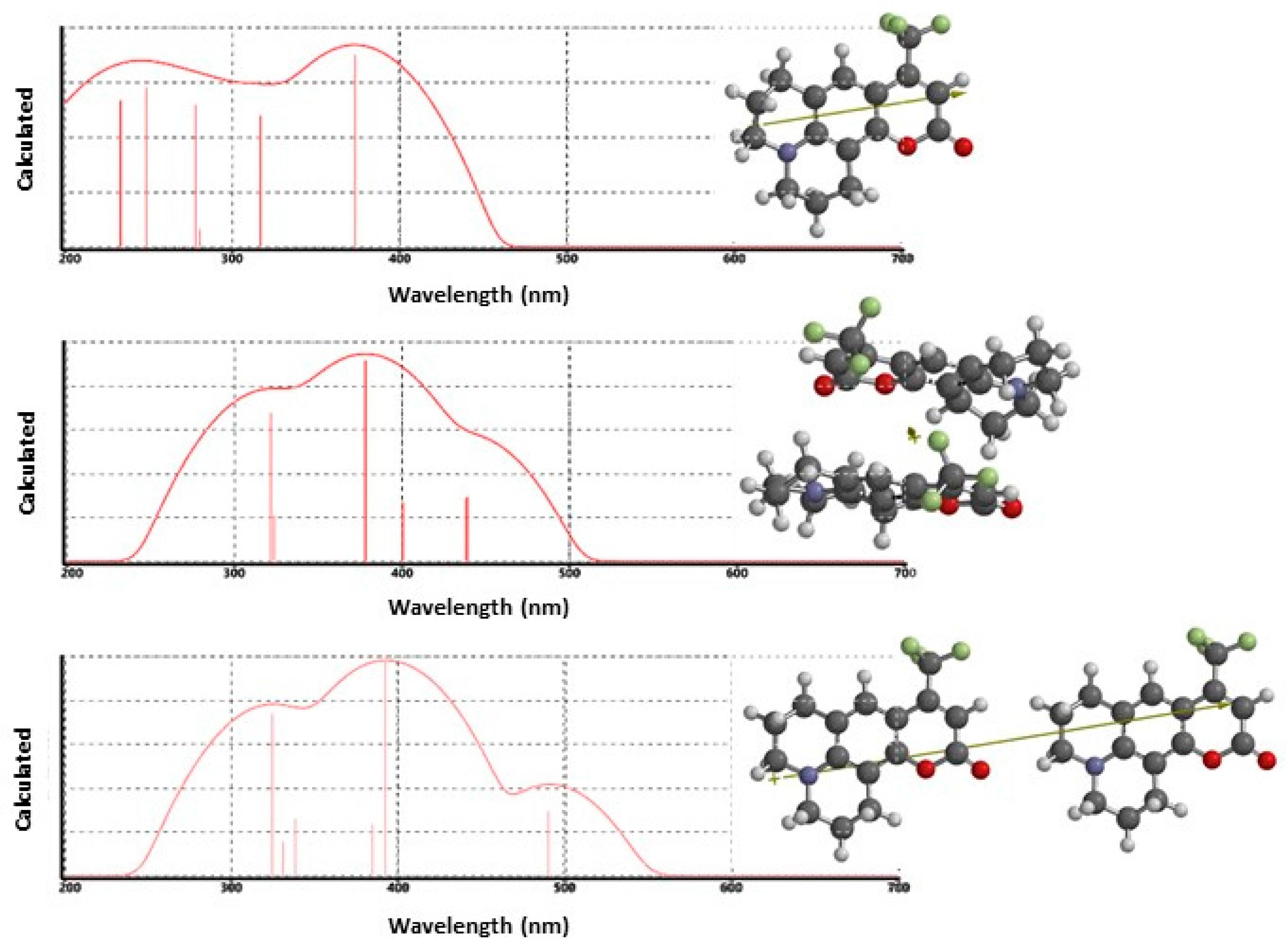
3.1. Steady-State Spectroscopy
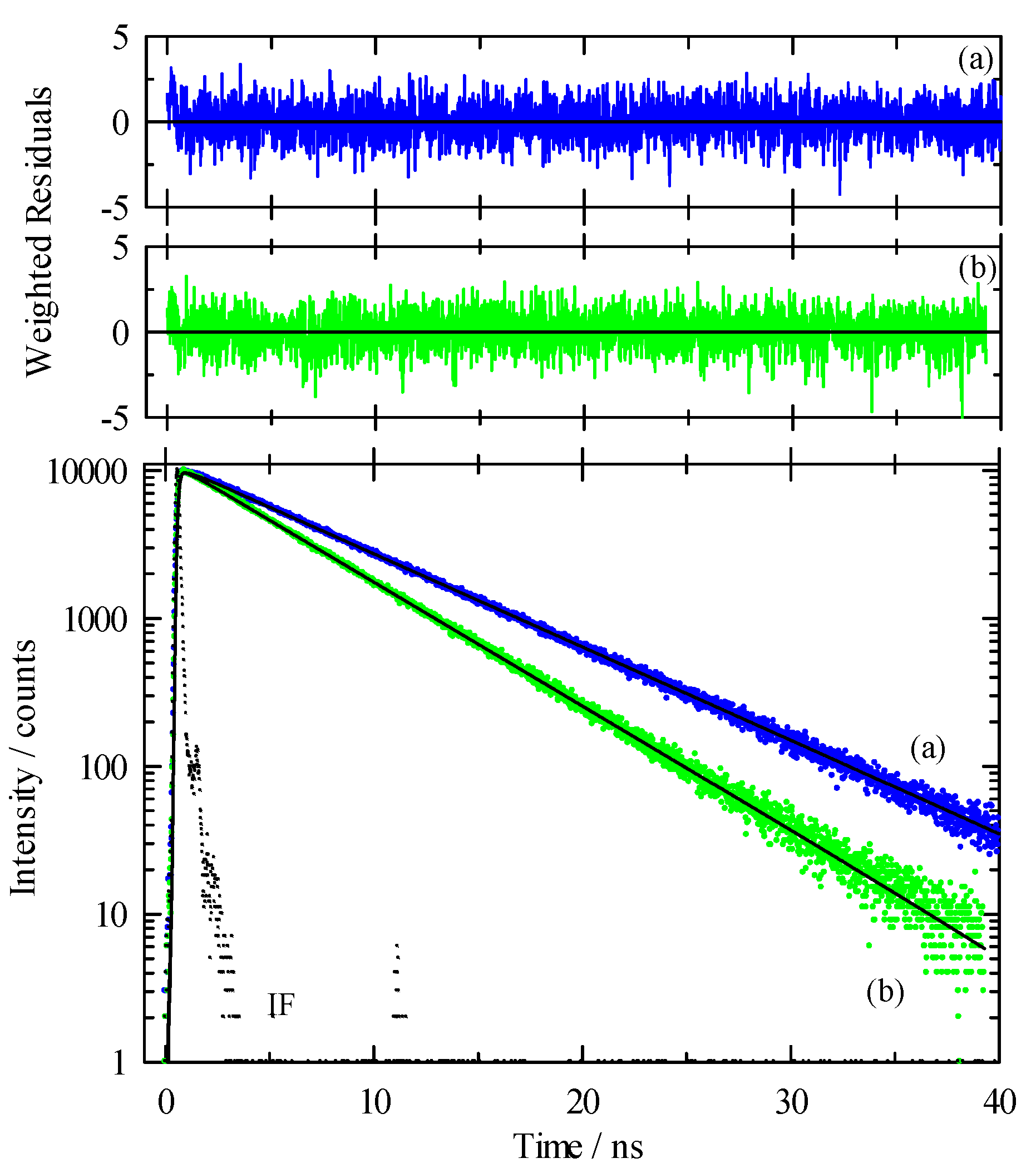
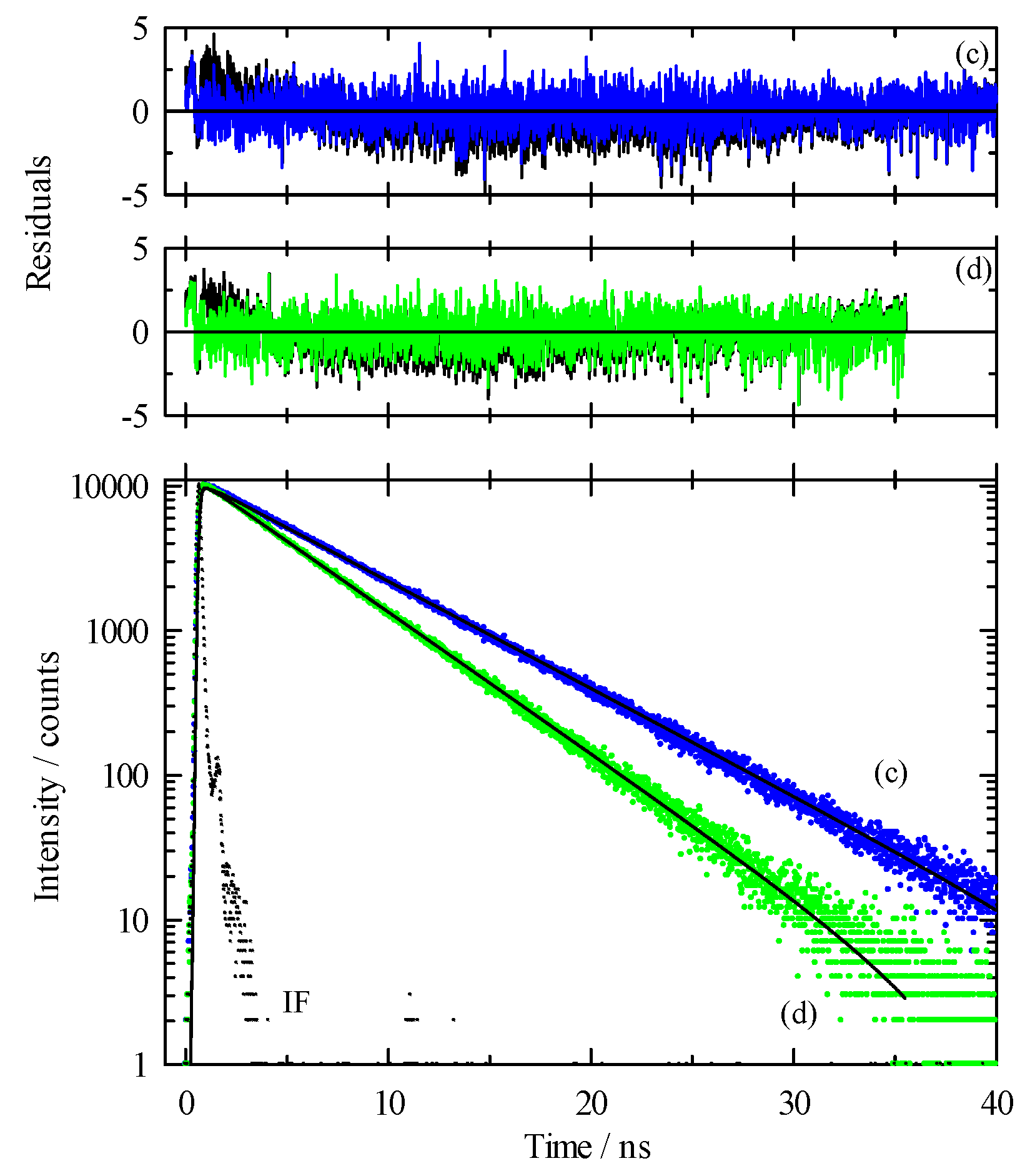
3.2. Time-Resolved Spectroscopy
4. Conclusions
Supplementary Materials
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Pollet, P.; Davey, E.A.; Urena-Benavides, E.E.; Eckert, C.A.; Liotta, C.L. Solvents for sustainable chemical processes. Green Chem. 2014, 16, 1034–1055. [Google Scholar] [CrossRef]
- Huang, Z.; Kawi, S.; Chiew, Y.C. Solubility of cholesterol and its esters in supercritical carbon dioxide with and without cosolvents. J. Supercrit. Fluids 2004, 30, 25–39. [Google Scholar] [CrossRef]
- Gao, L.; Jiang, T.; Zhao, G.; Mu, T.; Wu, W.; Hou, Z.; Han, B. Transesterification between isoamyl acetate and ethanol in supercritical CO2, ionic liquid, and their mixture. J. Supercrit. Fluids 2004, 29, 107–111. [Google Scholar] [CrossRef]
- Shariati, A.; Peters, C.J. High-pressure phase behavior of systems with ionic liquids: II. The binary system carbon dioxide+1-ethyl-3-methylimidazolium hexafluorophosphate. J. Supercrit. Fluids 2004, 29, 43–48. [Google Scholar] [CrossRef]
- Shariati, A.; Peters, C.J. High-pressure phase behavior of systems with ionic liquids: Part III. The binary system carbon dioxide+1-hexyl-3-methylimidazolium hexafluorophosphate. J. Supercrit. Fluids 2004, 30, 139–144. [Google Scholar] [CrossRef]
- Shariati, A.; Peters, C.J. High-pressure phase behavior of systems with ionic liquids: Measurements and modeling of the binary system fluoroform+1-ethyl-3-methylimidazolium hexafluorophosphate. J. Supercrit. Fluids 2003, 25, 109–117. [Google Scholar] [CrossRef]
- Batista, M.L.S.; Neves, C.M.S.S.; Carvalho, P.J.; Gani, R.; Coutinho, J.A.P. Chameleonic behavior of ionic liquids and its impact on the estimation of solubility parameters. J. Phys. Chem. B 2011, 115, 12879–12888. [Google Scholar] [CrossRef] [PubMed]
- Jodry, J.J.; Mikami, K. New chiral imidazolium ionic liquids: 3D-network of hydrogen bonding. Tetrahedron Lett. 2004, 45, 4429–4431. [Google Scholar] [CrossRef]
- Li, Z.; Xiao, Y.; Xue, W.; Yang, Q.; Zhong, C. Ionic liquid/metal–organic framework composites for H2S removal from natural gas: A computational exploration. J. Phys. Chem. C 2015, 119, 3674–3683. [Google Scholar] [CrossRef]
- Ahmed, O.U.; Mjalli, F.S.; Gujarathi, A.M.; Al-Wahaibi, T.; Al-Wahaibi, Y.; AlNashef, I.M. Feasibility of phosphonium-based ionic liquids as solvents for extractive desulfurization of liquid fuels. Fluid Phase Equilib. 2015, 401, 102–109. [Google Scholar] [CrossRef]
- Zhou, Y.; Dyck, J.; Graham, T.W.; Luo, H.; Leonard, D.N.; Qu, J. Ionic liquids composed of phosphonium cations and organophosphate, carboxylate, and sulfonate anions as lubricant antiwear additives. Langmuir 2014, 30, 13301–13311. [Google Scholar] [CrossRef] [PubMed]
- Zakrewsky, M.; Lovejoy, K.S.; Kern, T.L.; Miller, T.E.; Le, V.; Nagy, A.; Goumas, A.M.; Iyer, R.S.; Del Sesto, R.E.; Koppisch, A.T.; et al. Ionic liquids as a class of materials for transdermal delivery and pathogen neutralization. Proc. Natl. Acad. Sci. USA 2014, 111, 13313–13318. [Google Scholar] [CrossRef] [PubMed]
- Cieszynska, A.; Wisniewski, M. Extraction of palladium(Ⅱ) from chloride solutions with cyphos® IL 101/toluene mixtures as novel extractant. Sep. Purif. Technol. 2010, 73, 202–207. [Google Scholar] [CrossRef]
- Kawano, R.; Matsui, H.; Matsuyama, C.; Sato, A.; Susan, M.A.B.H.; Tanabe, N.; Watanabe, M. High performance dye-sensitized solar cells using ionic liquids as their electrolytes. J. Photochem. Photobiol. A 2004, 164, 87–92. [Google Scholar] [CrossRef]
- Sato, T.; Masuda, G.; Takagi, K. Electrochemical properties of novel ionic liquids for electric double layer capacitor applications. Electrochim. Acta 2004, 49, 3603–3611. [Google Scholar] [CrossRef]
- Zhao, G.; Jiang, T.; Han, B.; Li, Z.; Zhang, J.; Liu, Z.; He, J.; Wu, W. Electrochemical reduction of supercritical carbon dioxide in ionic liquid 1-N-butyl-3-methylimidazolium hexafluorophosphate. J. Supercrit. Fluids 2004, 32, 287–291. [Google Scholar] [CrossRef]
- Gui, J.; Cong, X.; Liu, D.; Zhang, X.; Hu, Z.; Sun, Z. Novel brønsted acidic ionic liquid as efficient and reusable catalyst system for esterification. Catal. Commun. 2004, 5, 473–477. [Google Scholar] [CrossRef]
- Ionic Liquids in Synthesis; Wasserscheid, P.; Welton, T. (Eds.) Wiley-VCH: Weinheim, Germany, 2003. [Google Scholar]
- Fortunato, R.; González-Muñoz, M.J.; Kubasiewicz, M.; Luque, S.; Alvarez, J.R.; Afonso, C.A.M.; Coelhoso, I.M.; Crespo, J.G. Liquid membranes using ionic liquids: The influence of water on solute transport. J. Membr. Sci. 2005, 249, 153–162. [Google Scholar] [CrossRef]
- Scovazzo, P.; Kieft, J.; Finan, D.A.; Koval, C.; DuBois, D.; Noble, R. Gas separations using non-hexafluorophosphate [PF6]− anion supported ionic liquid membranes. J. Membr. Sci. 2004, 238, 57–63. [Google Scholar] [CrossRef]
- Gruttadauria, M.; Riela, S.; Lo Meo, P.; D’Anna, F.; Noto, R. Supported ionic liquid asymmetric catalysis. A new method for chiral catalysts recycling. The case of proline-catalyzed aldol reaction. Tetrahedron Lett. 2004, 45, 6113–6116. [Google Scholar] [CrossRef]
- Ito, N.; Arzhantsev, S.; Heitz, M.; Maroncelli, M. Solvation dynamics and rotation of coumarin 153 in alkylphosphonium ionic liquids. J. Phys. Chem. B 2004, 108, 5771–5777. [Google Scholar] [CrossRef]
- Kroon, M.C.; Peters, C.J. Supercritical fluids in ionic liquids. In Ionic Liquids Further Uncoiled; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2014; pp. 39–57. [Google Scholar]
- Biswas, R.; Lewis, J.E.; Maroncelli, M. Electronic spectral shifts, reorganization energies, and local density augmentation of coumarin 153 in supercritical solvents. Chem. Phys. Lett. 1999, 310, 485–494. [Google Scholar] [CrossRef]
- Iwai, Y.; Nagano, H.; Lee, G.S.; Uno, M.; Arai, Y. Measurement of entrainer effects of water and ethanol on solubility of caffeine in supercritical carbon dioxide by FT-IR spectroscopy. J. Supercrit. Fluids 2006, 38, 312–318. [Google Scholar] [CrossRef]
- Li, A.; Tian, Z.; Yan, T.; Jiang, D.-E.; Dai, S. Anion-functionalized task-specific ionic liquids: Molecular origin of change in viscosity upon CO2 capture. J. Phys. Chem. B 2014, 118, 14880–14887. [Google Scholar] [CrossRef] [PubMed]
- Bates, E.D.; Mayton, R.D.; Ntai, I.; Davis, J.H., Jr. CO2 capture by a task-specific ionic liquid. J. Am. Chem. Soc. 2002, 124, 926–927. [Google Scholar] [CrossRef] [PubMed]
- Vesna, N.-V.; Ana, S.; José, M.S.S.E.; Henrique, J.R.G.; Luis, P.N.R.; da Manuel Nunes, P. Multiphase equilibrium in mixtures of [C4mim][PF6] with supercritical carbon dioxide, water, and ethanol: Applications in catalysis. In Ionic Liquids III A: Fundamentals, Progress, Challenges, and Opportunities; American Chemical Society: Washington, DC, USA, 2005; Volume 901, pp. 301–310. [Google Scholar]
- Shariati, A.; Peters, C.J. High-pressure phase equilibria of systems with ionic liquids. J. Supercrit. Fluids 2005, 34, 171–176. [Google Scholar] [CrossRef]
- Kumełan, J.; Tuma, D.; Maurer, G. Simultaneous solubility of carbon dioxide and hydrogen in the ionic liquid [hmim][Tf2N]: Experimental results and correlation. Fluid Phase Equilib. 2011, 311, 9–16. [Google Scholar] [CrossRef]
- Jang, S.; Cho, D.-W.; Im, T.; Kim, H. High-pressure phase behavior of CO2 + 1-butyl-3-methylimidazolium chloride system. Fluid Phase Equilib. 2010, 299, 216–221. [Google Scholar] [CrossRef]
- Gutkowski, K.I.; Shariati, A.; Peters, C.J. High-pressure phase behavior of the binary ionic liquid system 1-octyl-3-methylimidazolium tetrafluoroborate + carbon dioxide. J. Supercrit. Fluids 2006, 39, 187–191. [Google Scholar] [CrossRef]
- Kroon, M.C.; Florusse, L.J.; Kühne, E.; Witkamp, G.-J.; Peters, C.J. Achievement of a homogeneous phase in ternary ionic liquid/carbon dioxide/organic systems. Ind. Eng. Chem. Res. 2010, 49, 3474–3478. [Google Scholar] [CrossRef]
- Mena, M.; Shirai, K.; Tecante, A.; Bárzana, E.; Gimeno, M. Enzymatic syntheses of linear and hyperbranched poly-l-lactide using compressed r134a–ionic liquid media. J. Supercrit. Fluids 2015, 103, 77–82. [Google Scholar] [CrossRef]
- Timko, M.T.; Nicholson, B.F.; Steinfeld, J.I.; Smith, K.A.; Tester, J.W. Partition coefficients of organic solutes between supercritical carbon dioxide and water: Experimental measurements and empirical correlations. J. Chem. Eng. Data 2004, 49, 768–778. [Google Scholar] [CrossRef]
- Andanson, J.-M.; Jutz, F.; Baiker, A. Investigation of binary and ternary systems of ionic liquids with water and/or supercritical CO2 by in situ attenuated total reflection infrared spectroscopy. J. Phys. Chem. B 2010, 114, 2111–2117. [Google Scholar] [CrossRef] [PubMed]
- Tian, Q.; Li, R.; Sun, H.; Xue, Z.; Mu, T. Theoretical and experimental study on the interaction between 1-butyl-3-methylimidazolium acetate and CO2. J. Mol. Liq. 2015, 208, 259–268. [Google Scholar] [CrossRef]
- Bhargava, B.L.; Balasubramanian, S. Insights into the structure and dynamics of a room-temperature ionic liquid: Ab initio molecular dynamics simulation studies of 1-N-butyl-3-methylimidazolium hexafluorophosphate ([Bmim][PF6]) and the [Bmim][PF6]−CO2 mixture. J. Phys. Chem. B 2007, 111, 4477–4487. [Google Scholar] [CrossRef] [PubMed]
- Kanakubo, M.; Makino, T.; Umecky, T.; Sakurai, M. Effect of partial pressure on CO2 solubility in ionic liquid mixtures of 1-butyl-3-methylimidazolium acetate and 1-butyl-3-methylimidazolium bis(trifluoromethanesulfonyl)amide. Fluid Phase Equilib. 2016, 420, 74–82. [Google Scholar] [CrossRef]
- Koller, T.M.; Heller, A.; Rausch, M.H.; Wasserscheid, P.; Economou, I.G.; Fröba, A.P. Mutual and self-diffusivities in binary mixtures of [Emim][B(CN)4] with dissolved gases by using dynamic light scattering and molecular dynamics simulations. J. Phys. Chem. B 2015, 119, 8583–8592. [Google Scholar] [CrossRef] [PubMed]
- Lu, J.; Liotta, C.L.; Eckert, C.A. Spectroscopically probing microscopic solvent properties of room-temperature ionic liquids with the addition of carbon dioxide. J. Phys. Chem. A 2003, 107, 3995–4000. [Google Scholar] [CrossRef]
- Kumelan, J.; Tuma, D.; Maurer, G. Partial molar volumes of selected gases in some ionic liquids. Fluid Phase Equilib. 2009, 275, 132–144. [Google Scholar] [CrossRef]
- Kim, J.E.; Lim, J.S.; Kang, J.W. Measurement and correlation of solubility of carbon dioxide in 1-alkyl-3-methylimidazolium hexafluorophosphate ionic liquids. Fluid Phase Equilib. 2011, 306, 251–255. [Google Scholar] [CrossRef]
- Blanchard, L.A.; Gu, Z.; Brennecke, J.F. High-pressure phase behavior of ionic liquid/CO2 systems. J. Phys. Chem. B 2001, 105, 2437–2444. [Google Scholar] [CrossRef]
- Afzal, W.; Liu, X.; Prausnitz, J.M. High solubilities of carbon dioxide in tetraalkyl phosphonium-based ionic liquids and the effect of diluents on viscosity and solubility. J. Chem. Eng. Data 2014, 59, 954–960. [Google Scholar] [CrossRef]
- Carvalho, P.J.; Álvarez, V.H.; Marrucho, I.M.; Aznar, M.; Coutinho, J.A.P. High carbon dioxide solubilities in trihexyltetradecylphosphonium-based ionic liquids. J. Supercrit. Fluids 2010, 52, 258–265. [Google Scholar] [CrossRef]
- Hutchings, J.W.; Fuller, K.L.; Heitz, M.P.; Hoffmann, M.M. Surprisingly high solubility of the ionic liquid trihexyltetradecylphosphonium chloride in dense carbon dioxide. Green Chem. 2005, 7, 475–478. [Google Scholar] [CrossRef]
- Lewis, J.E.; Maroncelli, M. On the (uninteresting) dependence of the absorption and emission transition moments of coumarin 153 on solvent. Chem. Phys. Lett. 1998, 282, 197–203. [Google Scholar] [CrossRef]
- Maroncelli, M.; Fleming, G.R. Picosecond solvation dynamics of coumarin 153: The importance of molecular aspects of solvation. J. Chem. Phys. 1987, 86, 6221–6239. [Google Scholar] [CrossRef]
- Zhang, X.-X.; Breffke, J.; Ernsting, N.P.; Maroncelli, M. Observations of probe dependence of the solvation dynamics in ionic liquids. Phys. Chem. Chem. Phys. 2015, 17, 12949–12956. [Google Scholar] [CrossRef] [PubMed]
- Becker, R.S.; Chakravorti, S.; Gartner, C.A.; de Graca Miguel, M. Photosensitizers: Comprehensive photophysics/photochemistry and theory of coumarins, chromones, their homologues and thione analogues. J. Chem. Soc. Faraday Trans. 1993, 89, 1007–1019. [Google Scholar] [CrossRef]
- Kaliya, O.L. The photochemistry of coumarins. Rus. Chem. Rev. 1992, 61, 683. [Google Scholar]
- Reynolds, L.; Gardecki, J.A.; Frankland, S.J.V.; Horng, M.L.; Maroncelli, M. Dipole solvation in nondipolar solvents: Experimental studies of reorganization energies and solvation dynamics. J. Phys. Chem. 1996, 100, 10337–10354. [Google Scholar] [CrossRef]
- Horng, M.L.; Gardecki, J.A.; Papazyan, A.; Maroncelli, M. Subpicosecond measurements of polar solvation dynamics: Coumarin 153 revisited. J. Phys. Chem. 1995, 99, 17311–17337. [Google Scholar] [CrossRef]
- Bradaric, C.J.; Downard, A.; Kennedy, C.; Robertson, A.J.; Zhou, Y. Industrial preparation of phosphonium ionic liquids. Green Chem. 2003, 5, 143–152. [Google Scholar] [CrossRef]
- McAtee, Z.P.; Heitz, M.P. Density, viscosity and excess properties in the trihexyltetradecylphosphonium chloride ionic liquid/methanol cosolvent system. J. Chem. Thermodyn. 2016, 93, 34–44. [Google Scholar] [CrossRef]
- Lemmon, E.W.; McLinden, M.O.; Friend, D.G. Thermophysical properties of fluid systems. In NIST Chemistry Webbook; Linstrom, P.J., Mallard, W.G., Eds.; Nist Standard Reference Database Number 69; National Institute of Standards and Technology: Gaithersburg, MD, USA, 2001. Available online: http://webbook.nist.gov (accessed on 18 April 2017).
- Span, R.; Wagner, W. A new equation of state for carbon dioxide covering the fluid region from the triple-point temperature to 1100 K at pressures up to 800 MPa. J. Phys. Chem. Ref. Data 1996, 25, 1509–1596. [Google Scholar] [CrossRef]
- Barra, K.M.; Sabatini, R.P.; McAtee, Z.P.; Heitz, M.P. Solvation and rotation dynamics in the trihexyl(tetradecyl)phosphonium chloride ionic liquid/methanol cosolvent system. J. Phys. Chem. B 2014, 118, 12979–12992. [Google Scholar] [CrossRef] [PubMed]
- O’Connor, D.V.; Phillips, D. Time-Correlated Single Photon Counting; Academic Press: Cambridge, MA, USA, 1984. [Google Scholar]
- Becker, W. Advanced Time-Correlated Single Photon Counting Techniques; Springer: Berlin, Germany, 2005. [Google Scholar]
- Lakowicz, J.R. Principles of Fluorescence Spectroscopy; Springer: New York, NY, USA, 2006. [Google Scholar]
- Spartan’16, version 16; Parallel Suite; Wavefunction Inc.: Irvine, CA, USA, 2016.
- Shao, Y.; Molnar, L.F.; Jung, Y.; Kussmann, J.; Ochsenfeld, C.; Brown, S.T.; Gilbert, A.T.B.; Slipchenko, L.V.; Levchenko, S.V.; O’Neill, D.P.; et al. Advances in methods and algorithms in a modern quantum chemistry program package. Phys. Chem. Chem. Phys. 2006, 8, 3172–3191. [Google Scholar] [CrossRef] [PubMed]
- Shao, Y.; Gan, Z.; Epifanovsky, E.; Gilbert, A.T.B.; Wormit, M.; Kussmann, J.; Lange, A.W.; Behn, A.; Deng, J.; Feng, X.; et al. Advances in molecular quantum chemistry contained in the Q-Chem 4 program package. Mol. Phys. 2015, 113, 184–215. [Google Scholar] [CrossRef]
- Arzhantsev, S.; Ito, N.; Heitz, M.; Maroncelli, M. Solvation dynamics of coumarin 153 in several classes of ionic liquids: Cation dependence of the ultrafast component. Chem. Phys. Lett. 2003, 381, 278–286. [Google Scholar] [CrossRef]
- Hoffmann, M.M.; Heitz, M.P.; Carr, J.B.; Tubbs, J.D. Surfactants in green solvent systems—Current and future research directions. J. Dispers. Sci. Technol. 2003, 24, 155–171. [Google Scholar] [CrossRef]
- Heitz, M.P.; Carlier, C.; deGrazia, J.; Harrison, K.L.; Johnston, K.P.; Randolph, T.W.; Bright, F.V. Water core within perfluoropolyether-based microemulsions formed in supercritical carbon dioxide. J. Phys. Chem. B 1997, 101, 6707–6714. [Google Scholar] [CrossRef]
- Johnston, K.P.; Harrison, K.L.; Clarke, M.J.; Howdle, S.M.; Heitz, M.P.; Bright, F.V.; Carlier, C.; Randolph, T.W. Water-in-carbon dioxide microemulsions: An environment for hydrophiles including proteins. Science 1996, 271, 624–626. [Google Scholar] [CrossRef]
- Holmes, J.D.; Ziegler, K.J.; Audriani, M.; Lee, C.T., Jr.; Bhargave, P.A.; Steytler, D.C.; Johnston, K.P. Buffering the aqueous phase ph in water-in- CO2 microemulsions. J. Phys. Chem. B 1999, 103, 5703–5711. [Google Scholar] [CrossRef]
- Lim, K.T.; Hwang, H.S.; Lee, M.S.; Lee, G.D.; Hong, S.S.; Johnston, K.P. Formation of TiO2 nanoparticles in water-in-CO2 microemulsions. Chem. Commun. 2002, 1528–1529. [Google Scholar] [CrossRef]
- Lim, K.T.; Hwang, H.S.; Ryoo, W.; Johnston, K.P. Synthesis of TiO2 nanoparticles utilizing hydrated reverse micelles in CO2. Langmuir 2004, 20, 2466–2471. [Google Scholar] [CrossRef] [PubMed]
- Verma, P.; Pal, H. Aggregation studies of dipolar coumarin-153 dye in polar solvents: A photophysical study. J. Phys. Chem. A 2014, 118, 6950–6964. [Google Scholar] [CrossRef] [PubMed]
- Sen, T.; Bhattacharyya, S.; Mandal, S.; Patra, A. Spectroscopic investigations on the h-type aggregation of coumarin 153 dye molecules: Role of au nanoparticles and γ-cyclodextrin. J. Fluoresc. 2012, 22, 303–310. [Google Scholar] [CrossRef] [PubMed]
- Verma, P.; Pal, H. Intriguing h-aggregate and h-dimer formation of coumarin-481 dye in aqueous solution as evidenced from photophysical studies. J. Phys. Chem. A 2012, 116, 4473–4484. [Google Scholar] [CrossRef] [PubMed]
- Verma, P.; Pal, H. Unusual h-type aggregation of coumarin-481 dye in polar organic solvents. J. Phys. Chem. A 2013, 117, 12409–12418. [Google Scholar] [CrossRef] [PubMed]
- Lee, D.; Greenman, L.; Sarovar, M.; Whaley, K.B. Ab initio calculation of molecular aggregation effects: A coumarin-343 case study. J. Phys. Chem. A 2013, 117, 11072–11085. [Google Scholar] [CrossRef] [PubMed]
- Liu, X.; Cole, J.M.; Low, K.S. Molecular origins of dye aggregation and complex formation effects in coumarin 343. J. Phys. Chem. C 2013, 117, 14723–14730. [Google Scholar] [CrossRef]
- Kasha, M.; Rawls, H.R.; El-Bayoumi, M.A. The exciton model in molecular spectroscopy. Pure Appl. Chem. 1965, 11, 371–392. [Google Scholar] [CrossRef]
- Kasha, M. Energy transfer mechanisms and the molecular exciton model for molecular aggregates. Radiat. Res. 1963, 20, 55–70. [Google Scholar] [CrossRef] [PubMed]
- Minami, K.; Aizawa, T.; Kanakubo, M.; Hiejima, Y.; Nanjo, H.; Smith, J.; Richard, L. Local density augmentation of excited 1-(dimethylamino)naphthalene in supercritical water. J. Supercrit. Fluids 2006, 39, 206–210. [Google Scholar] [CrossRef]
- Ruckenstein, E.; Shulgin, I.L. Why density augmentation occurs in dilute supercritical solutions. Chem. Phys. Lett. 2000, 330, 551–557. [Google Scholar] [CrossRef]
- Ingrosso, F.; Ladanyi, B.M.; Mennucci, B.; Scalmani, G. Solvation of coumarin 153 in supercritical fluoroform. J. Phys. Chem. B 2006, 110, 4953–4962. [Google Scholar] [CrossRef] [PubMed]
- Heitz, M.P.; Maroncelli, M. Rotation of aromatic solutes in supercritical CO2: Are rotation times anomalously slow in the near critical regime? J. Phys. Chem. A 1997, 101, 5852–5868. [Google Scholar] [CrossRef]
- Cigáň, M.; Donovalová, J.; Szöcs, V.; Gašpar, J.; Jakusová, K.; Gáplovský, A. 7-(dimethylamino)coumarin-3-carbaldehyde and its phenylsemicarbazone: Tict excited state modulation, fluorescent h-aggregates, and preferential solvation. J. Phys. Chem. A 2013, 117, 4870–4883. [Google Scholar] [CrossRef] [PubMed]
- Birks, J.B. Photophysics of Aromatic Molecules; Wiley-Interscience: Hoboken, NJ, USA, 1970. [Google Scholar]
- Jin, H.; Baker, G.A.; Arzhantsev, S.; Dong, J.; Maroncelli, M. Solvation and rotational dynamics of coumarin 153 in ionic liquids: Comparisons to conventional solvents. J. Phys. Chem. B 2007, 111, 7291–7302. [Google Scholar] [CrossRef] [PubMed]

© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Heitz, M.P.; Fuller, K.L.; Ordiway, K.A. Dissolution of Trihexyltetradecylphosphonium Chloride in Supercritical CO2. ChemEngineering 2017, 1, 12. https://doi.org/10.3390/chemengineering1020012
Heitz MP, Fuller KL, Ordiway KA. Dissolution of Trihexyltetradecylphosphonium Chloride in Supercritical CO2. ChemEngineering. 2017; 1(2):12. https://doi.org/10.3390/chemengineering1020012
Chicago/Turabian StyleHeitz, Mark P., Kristina L. Fuller, and Kaitlin A. Ordiway. 2017. "Dissolution of Trihexyltetradecylphosphonium Chloride in Supercritical CO2" ChemEngineering 1, no. 2: 12. https://doi.org/10.3390/chemengineering1020012
APA StyleHeitz, M. P., Fuller, K. L., & Ordiway, K. A. (2017). Dissolution of Trihexyltetradecylphosphonium Chloride in Supercritical CO2. ChemEngineering, 1(2), 12. https://doi.org/10.3390/chemengineering1020012





