Lanthanide-Based Single-Molecule Magnets Derived from Schiff Base Ligands of Salicylaldehyde Derivatives
Abstract
1. Introduction
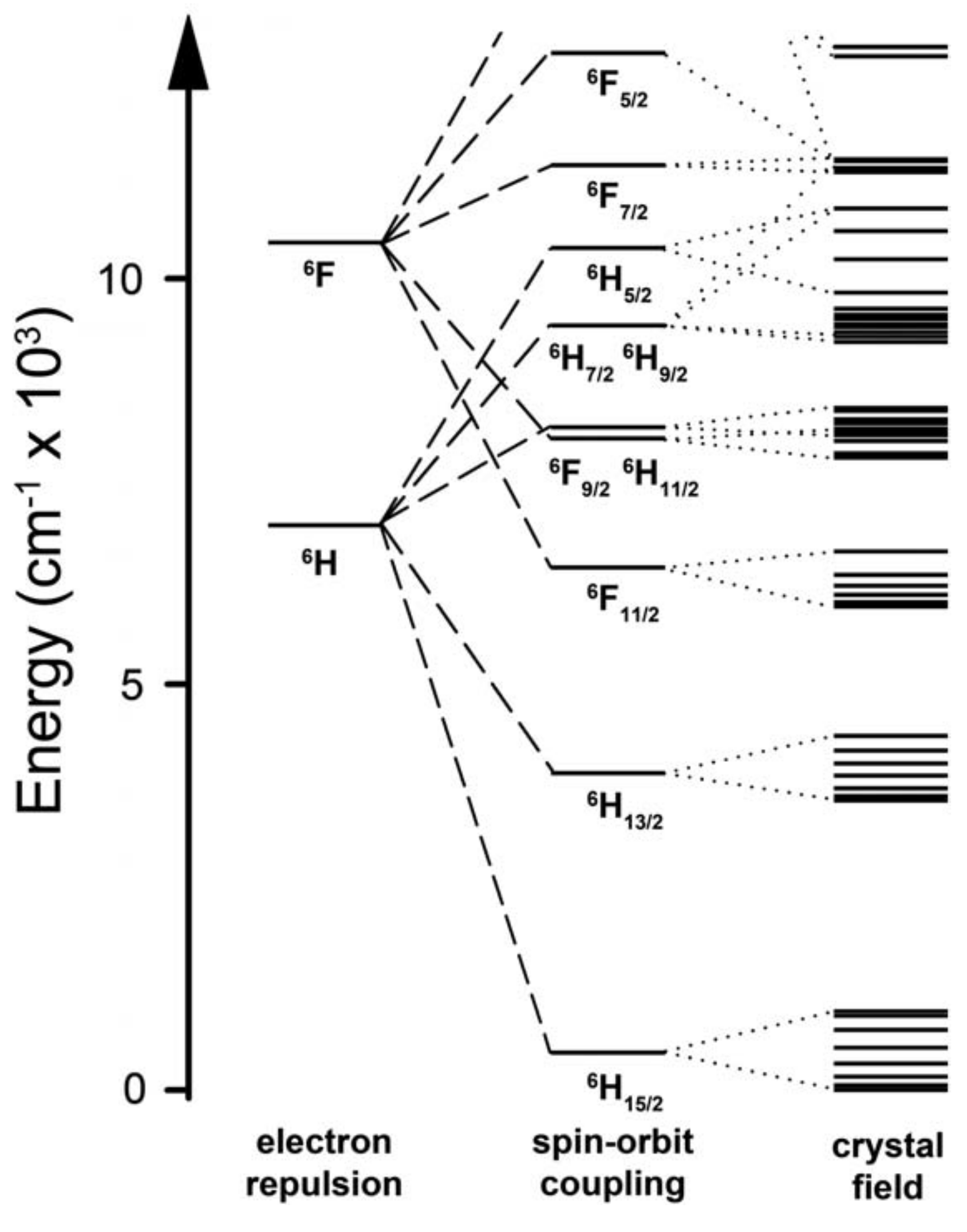
1.1. General Introduction to Single-Molecule Magnets (SMMs)
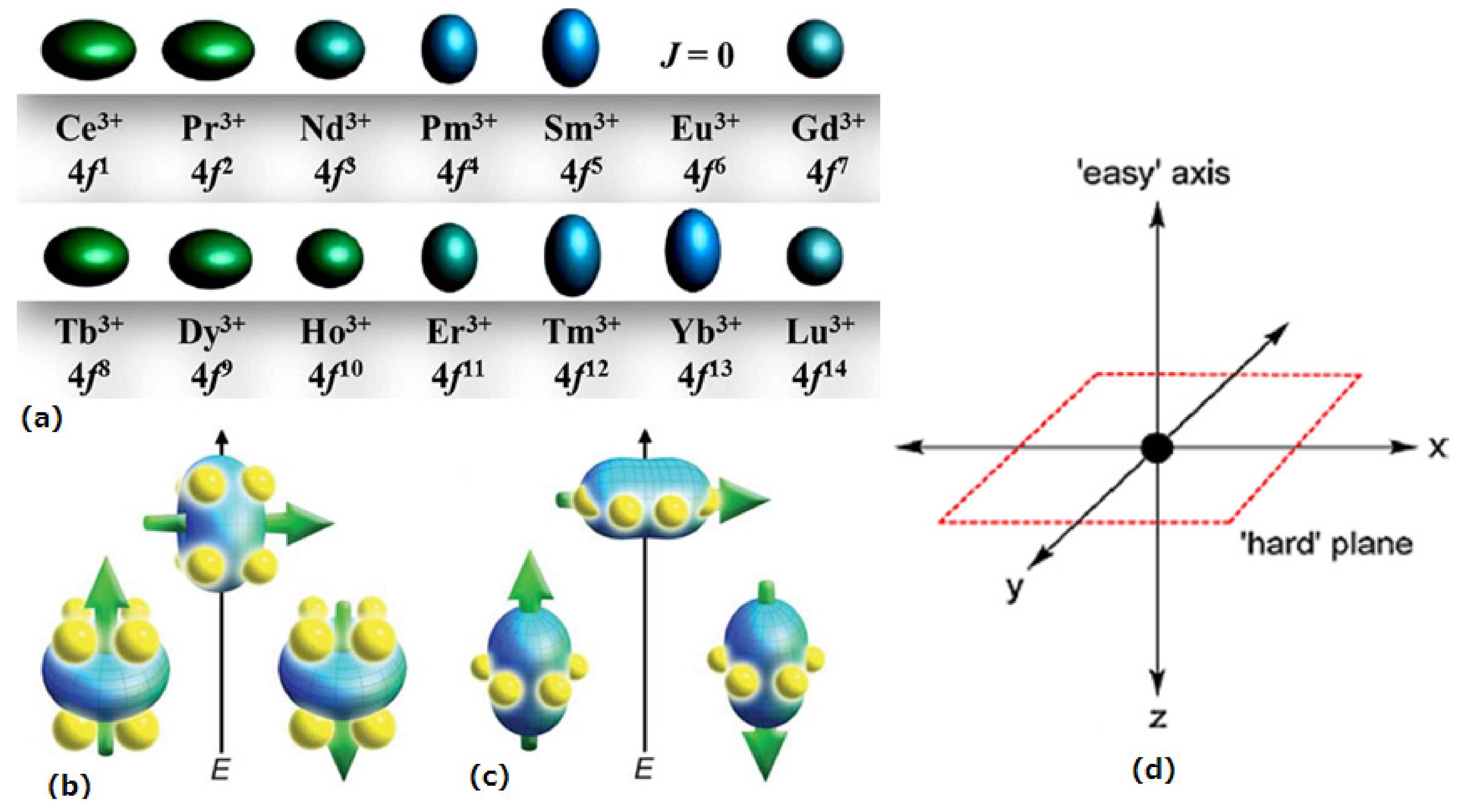
1.2. The Anisotropy of Lanthanide Ions-Oblate/Prolate Model

1.3. Phthalocyanine Double-Decker SIM and Dy(III) Triangular SIM/SMM

1.4. The Current Progresses in Ln(III)-Based SMMs
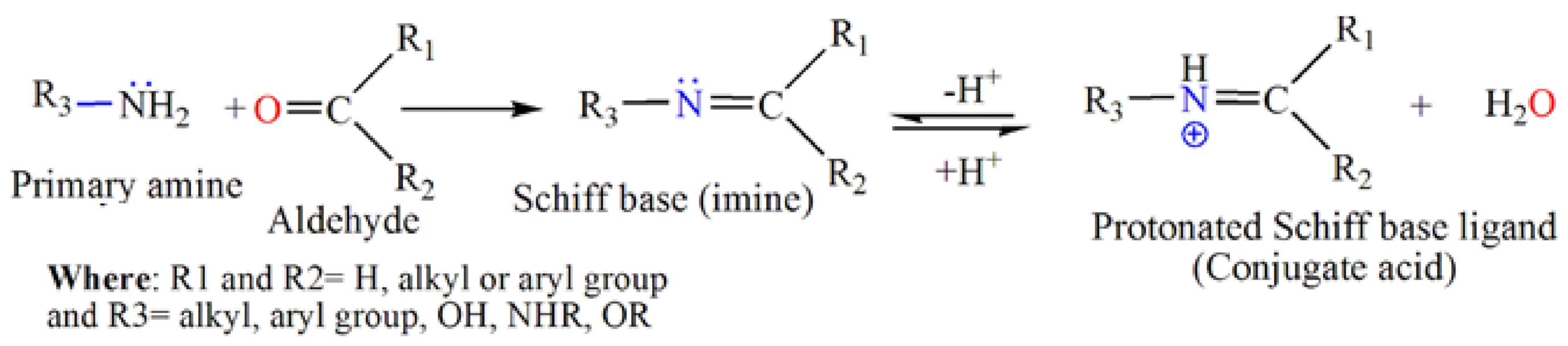
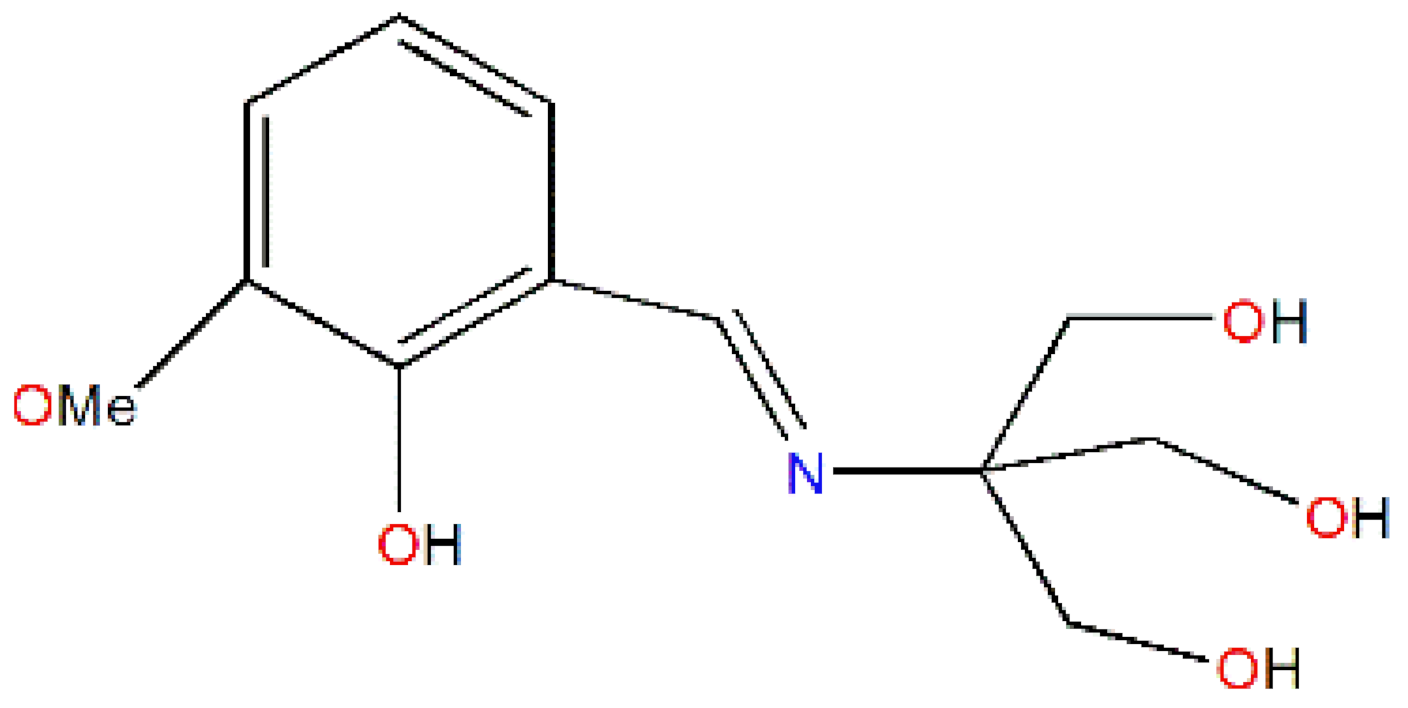
2. Schiff Base Ligands
3. Designing Schiff Bases in Ln(III)-Based SMM Systems
4. Ln(III)-Based Schiff Base SMMs of Different Nuclearities
4.1. Mononuclear Schiff Base Ln(III)-Complexes of SMMs
4.2. Dinuclear and Trinuclear Schiff Base Ln(III) SMM Complexes
4.3. Tetranuclear Schiff Base Ln(III)-Complexes of SMMs
4.4. Hexanuclear Schiff Base Ln(III)-Complexes of SMMs
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Ritter, S.K. Single-molecule magnets evolve. Chem. Eng. News 2004, 82, 29–32. [Google Scholar] [CrossRef]
- Kahn, O. Molecular Magnetism; VCH Pubilishers: New York, NY, USA, 1993; ISBN 1560815663. [Google Scholar]
- Caneschi, A.; Gatteschi, D.; Sessoli, R.; Barra, A.L.; Brunel, L.C.; Guillot, M. Alternating Current Susceptibility, High Field Magnetization, and Millimeter Band EPR Evidence for a Ground S = 10 State in [Mn12O12(CH3COO)16(H2O)4]·2CH3COOH·4H2O. J. Am. Chem. Soc. 1991, 113, 5873–5874. [Google Scholar] [CrossRef]
- Bagai, R.; Christou, G. The Drosophila of single-molecule magnetism: [Mn12O12(O2CR)16(H2O)4]. Chem. Soc. Rev. 2009, 38, 1011–1026. [Google Scholar] [CrossRef] [PubMed]
- Bar, A.-K.; Kalita, P.; Singh, M.-K.; Rajaraman, G.; Chandrasekhar, V. Low-coordinate mononuclear lanthanide complexes as molecular nanomagnets. Coord. Chem. Rev. 2018, 367, 163–216. [Google Scholar] [CrossRef]
- Woodruff, D.N.; Winpenny, R.E.P.; Layfield, R.A. Lanthanide Single-Molecule Magnets. Chem. Rev. 2013, 113, 5110–5148. [Google Scholar] [CrossRef]
- Liu, J.L.; Chen, Y.C.; Tong, M.L. Symmetry strategies for high performance lanthanide-based single-molecule magnets. Chem. Soc. Rev. 2018, 47, 2431–2453. [Google Scholar] [CrossRef]
- Guo, Y.-N.; Xu, G.-F.; Guo, Y.; Tang, J. Relaxation dynamics of dysprosium(III) single molecule magnets. Dalton Trans. 2011, 40, 9953–9963. [Google Scholar] [CrossRef]
- Liddle, S.T.; Slageren, J. van Improving f-element single molecule magnets. Chem. Soc. Rev. 2015, 6655–6669. [Google Scholar] [CrossRef]
- Pedersen, K.S.; Schau-Magnussen, M.; Bendix, J.; Weihe, H.; Palii, A.V.; Klokishner, S.I.; Ostrovsky, S.; Reu, O.S.; Mutka, H.; Tregenna-Piggott, P.L.W. Enhancing the blocking temperature in single-molecule magnets by incorporating 3d–5d exchange interactions. Chem. Eur. J. 2010, 16, 13458–13464. [Google Scholar] [CrossRef]
- Pedersen, K.S.; Dreiser, J.; Nehrkorn, J.; Gysler, M.; Schau-Magnussen, M.; Schnegg, A.; Holldack, K.; Bittl, R.; Piligkos, S.; Weihe, H.; et al. A linear single-molecule magnet based on [RuIII(CN)6]3−. Chem. Commun. 2011, 47, 6918–6920. [Google Scholar] [CrossRef]
- Sorace, L.; Benelli, C.; Gatteschi, D. Lanthanides in molecular magnetism: Old tools in a new field. Chem. Soc. Rev. 2011, 40, 3092–3104. [Google Scholar] [CrossRef] [PubMed]
- Rinehart, J.D.; Long, J.R. Exploiting single-ion anisotropy in the design of f-element single-molecule magnets. Chem. Sci. 2011, 2, 2078–2085. [Google Scholar] [CrossRef]
- Baldoví, J.J.; Coronado, E.; Gaita-ariço, A.; Gamer, C.; Gimønez-marquøs, M.; Mínguez, G. A SIM-MOF: Three-Dimensional Organisation of Single-Ion Magnets with Anion-Exchange Capabilities. Chem. Eur. J. 2014, 20, 10695–10702. [Google Scholar] [CrossRef] [PubMed]
- Feltham, H.L.C.; Brooker, S. Review of purely 4f and mixed-metal nd–4f single-molecule magnets containing only one lanthanide ion. Coord. Chem. Rev. 2014, 276, 1–33. [Google Scholar] [CrossRef]
- Boulon, M.; Cucinotta, G.; Luzon, J.; Innocenti, C.D.; Perfetti, M.; Bernot, K.; Calvez, G.; Caneschi, A.; Sessoli, R. Magnetic Anisotropy and Spin-Parity Effect Along the Series of Lanthanide Complexes with DOTA. Angew. Chem. Int. Ed. 2013, 52, 350–354. [Google Scholar] [CrossRef] [PubMed]
- Liu, K.; Zhang, X.; Meng, X.; Shi, W.; Cheng, P.; Powell, A.K. Constraining the coordination geometries of lanthanide centers and magnetic building blocks in frameworks: A new strategy for molecular nanomagnets. Chem. Soc. Rev. 2016, 45, 2423–2439. [Google Scholar] [CrossRef]
- Ungur, L.; Chibotaru, L.F. Strategies toward High-Temperature Lanthanide-Based Single-Molecule Magnets. Inorg. Chem. 2016, 55, 10043–10056. [Google Scholar] [CrossRef]
- Katoh, K.; Komeda, T.; Yamashita, M. The Frontier of Molecular Spintronics Based on Multiple-Decker Phthalocyaninato TbIII Single-Molecule Magnets. Chem. Rec. 2016, 16, 987–1016. [Google Scholar] [CrossRef]
- Zhang, P.; Zhang, L.; Tang, J. Lanthanide single molecule magnets: Progress and perspective. Dalton Trans. 2015, 44, 3923–3929. [Google Scholar] [CrossRef]
- Gregson, M.; Chilton, N.F.; Ariciu, A.M.; Tuna, F.; Crowe, I.F.; Lewis, W.; Blake, A.J.; Collison, D.; McInnes, E.J.L.; Winpenny, R.E.P.; et al. A monometallic lanthanide bis(methanediide) single molecule magnet with a large energy barrier and complex spin relaxation behaviour. Chem. Sci. 2016, 7, 155–165. [Google Scholar] [CrossRef]
- Singh, M.K.; Rajaraman, G. Acquiring a record barrier height for magnetization reversal in lanthanide encapsulated fullerene molecules using DFT and: Ab initio calculations. Chem. Commun. 2016, 52, 14047–14050. [Google Scholar] [CrossRef] [PubMed]
- Guo, F.; Day, B.M.; Chen, Y.; Tong, M.; Mansikkam, A.; Layfield, R.A. A Dysprosium Metallocene Single-Molecule Magnet Functioning at the Axial Limit. Angew. Chem. Int. Ed. 2017, 56, 11445–11449. [Google Scholar] [CrossRef] [PubMed]
- Ishikawa, N.; Sugita, M.; Okubo, T.; Tanaka, N.; Iino, T.; Kaizu, Y. Determination of ligand-field parameters and f-electronic structures of double-decker bis(phthalocyaninato)lanthanide complexes. Inorg. Chem. 2003, 42, 2440–2446. [Google Scholar] [CrossRef] [PubMed]
- Ishikawa, N.; Sugita, M.; Ishikawa, T.; Koshihara, S.Y.; Kaizu, Y. Mononuclear lanthanide complexes with a long magnetization relaxation time at high temperatures: A new category of magnets at the single-molecular level. J. Phys. Chem. B 2004, 108, 11265–11271. [Google Scholar] [CrossRef]
- Ishikawa, N.; Sugita, M.; Ishikawa, T.; Koshihara, S.; Kaizu, Y. Lanthanide Double-Decker Complexes Functioning as Magnets at the Single-Molecular Level. J. Am. Chem. Soc. 2003, 125, 8694–8695. [Google Scholar] [CrossRef]
- Ishikawa, N.; Sugita, M.; Tanaka, N.; Ishikawa, T.; Koshihara, S.; Kaizu, Y. Upward Temperature Shift of the Intrinsic Phase Lag of the Magnetization of Bis(phthalocyaninato) terbium by Ligand Oxidation Creating an S = 1/2 Spin. Inorg. Chem. 2004, 43, 5498–5500. [Google Scholar] [CrossRef]
- Hewitt, I.J.; Tang, J.; Madhu, N.T.; Anson, C.E.; Lan, Y.; Luzon, J.; Etienne, M.; Sessoli, R.; Powell, A.K. Coupling Dy3 triangles enhances their slow magnetic relaxation. Angew. Chem. Int. Ed. 2010, 49, 6352–6356. [Google Scholar] [CrossRef]
- Tang, J.; Hewitt, I.; Madhu, N.T.; Chastanet, G.; Wernsdorfer, W.; Anson, C.E.; Benelli, C.; Sessoli, R.; Powell, A.K. Dysprosium Triangles Showing Single-Molecule Magnet Behavior of Thermally Excited Spin States. Angew. Chem. Int. Ed. 2006, 45, 1729–1733. [Google Scholar] [CrossRef]
- Ungur, L.; Chibotaru, L.F. Magnetic anisotropy in the excited states of low symmetry lanthanide complexes. Phys. Chem. Chem. Phys. 2011, 13, 20086–20090. [Google Scholar] [CrossRef]
- Rinehart, J.D.; Fang, M.; Evans, W.J.; Long, J.R.; Rinehart, D.; Fang, M.; Evans, W.J.; Long, R. A N23- Radical-Bridged Terbium Complex Exhibiting Magnetic Hysteresis at 14 K. J. Am. Chem. Soc. 2011, 133, 14236–14239. [Google Scholar] [CrossRef]
- Liu, J.; Chen, Y.; Liu, J.; Vieru, V.; Ungur, L.; Jia, J.; Tong, M. A Stable Pentagonal Bipyramidal Dy(III) Single-Ion Magnet with a Record Magnetization Reversal Barrier over 1000 K. J. Am. Chem. Soc. 2016, 138, 5441–5450. [Google Scholar] [CrossRef]
- McClain, K.R.; Gould, C.A.; Chakarawet, K.; Teat, S.J.; Groshens, T.J.; Long, J.R.; Harvey, B.G. High-temperature magnetic blocking and magneto-structural correlations in a series of dysprosium(III) metallocenium single-molecule magnets. Chem. Sci. 2018, 9, 8492–8503. [Google Scholar] [CrossRef] [PubMed]
- Carolina, R.G.; Ballesteros, B.; de la Torre, G.; Clemente-Juan, J.M.; Coronado, E.; Torres, T. Influence of Peripheral Substitution on the Magnetic Behavior of Single-Ion Magnets Based on Homo- and Heteroleptic TbIII Bis(phthalocyaninate). Chem. Eur. J. 2013, 19, 1457–1465. [Google Scholar] [CrossRef]
- Schiff, H. Mittheilungen aus dem Universitätslaboratorium in Pisa: Eine neue Reihe organischer Basen. Justus Liebigs Annalen der Chemie 1864, 131, 118–119. [Google Scholar] [CrossRef]
- Lin, P.-H.; Sun, W.-B.; Yu, M.-F.; Li, G.-M.; Yan, P.-F.; Murugesu, M. An unsymmetrical coordination environment leading to two slow relaxation modes in a Dy2 single-molecule magnet. Chem. Commun. 2011, 47, 10993–10995. [Google Scholar] [CrossRef] [PubMed]
- Long, J.; Habib, F.; Lin, P.H.; Korobkov, I.; Enright, G.; Ungur, L.; Wernsdorfer, W.; Chibotaru, L.F.; Murugesu, M. Single-molecule magnet behavior for an antiferromagnetically superexchange-coupled dinuclear dysprosium(III) complex. J. Am. Chem. Soc. 2011, 133, 5319–5328. [Google Scholar] [CrossRef] [PubMed]
- Lin, P.H.; Burchell, T.J.; Clérac, R.; Murugesu, M. Dinuclear dysprosium(III) single-molecule magnets with a large anisotropic barrier. Angew. Chem. Int. Ed. 2008, 47, 8848–8851. [Google Scholar] [CrossRef] [PubMed]
- Guo, Y.; Xu, G.; Wernsdorfer, W.; Ungur, L.; Guo, Y.; Tang, J.; Zhang, H.-J.; Chibotaru, L.F.; Powell, A. Strong Axiality and Ising Exchange Interaction Suppress Zero-Field Tunneling of Magnetization of an Asymmetric Dy2 Single-Molecule Magnet. J. Am. Chem. Soc. 2011, 133, 11948–11951. [Google Scholar] [CrossRef] [PubMed]
- Dhar, D.N.; Taploo, C.L. Schiff-bases and their applications. J. Sci. Ind. Res. 1982, 41, 501–506. [Google Scholar]
- Pearson, R.G. Hard and Soft Acids and Bases, HSAB, Part I Fundamental principles. J. Chem. Educ. 1968, 45, 581–587. [Google Scholar] [CrossRef]
- Pearson, R. Hard and Soft Acids and Bases. J. Am. Chem. Soc. 1963, 85, 3533–3539. [Google Scholar] [CrossRef]
- Anwar, M.U.; Thompson, L.K.; Dawe, L.N.; Habib, F.; Murugesu, M. Predictable self-assembled [2x2] Ln(III)4 square grids (Ln = Dy,Tb)—SMM behaviour in a new lanthanide cluster motif. Chem. Commun. 2012, 48, 4576–4578. [Google Scholar] [CrossRef] [PubMed]
- Guo, Y.; Xu, G.; Gamez, P.; Zhao, L.; Lin, S.; Deng, R.; Tang, J.; Zang, H.-J. Two-Step Relaxation in a Linear Tetranuclear Dysprosium(III) Aggregate Showing Single-Molecule Magnet Behavior. J. Am. Chem. Soc. 2010, 132, 8538–8539. [Google Scholar] [CrossRef]
- Tian, H.; Zhao, L.; Guo, Y.; Guo, Y.; Liu, Z. Quadruple-CO32− bridged octanuclear dysprosium(III) compound showing single-molecule magnet behaviour. Chem. Commun. 2012, 6346, 708–710. [Google Scholar] [CrossRef] [PubMed]
- Guo, Y.; Chen, X.; Xue, S.; Tang, J. Molecular Assembly and Magnetic Dynamics of Two Novel Dy6 and Dy8 Aggregates. Inorg. Chem. 2012, 51, 4035–4042. [Google Scholar] [CrossRef]
- Habib, F.; Long, J.; Lin, P.-H.; Korobkov, I.; Ungur, L.; Wernsdorfer, W.; Chibotaru, L.F.; Murugesu, M. Supramolecular architectures for controlling slow magnetic relaxation in field-induced single-molecule magnets. Chem. Sci. 2012, 3, 2158–2164. [Google Scholar] [CrossRef]
- Wang, H.; Liu, C.; Liu, T.; Zeng, S.; Cao, W.; Duan, C.; Dou, J.; Jiang, J. Mixed (phthalocyaninato)(Schiff-base) di-dysprosium sandwich complexes. Effect of magnetic coupling on the SMM behavior. Dalton Trans. 2013, 42, 15355–15360. [Google Scholar] [CrossRef]
- Andruh, M. The exceptionally rich coordination chemistry generated by Schiff-base ligands derived from o-vanillin. Dalton Trans. 2015, 44, 16633–16653. [Google Scholar] [CrossRef]
- Wu, G.; Hewitt, I.J.; Mameri, S.; Lan, Y.; Clérac, R.; Anson, C.E.; Qiu, S.; Powell, A.K. Bifunctional Ligand Approach for Constructing 3d−4f Heterometallic Clusters. Inorg. Chem. 2007, 46, 7229–7231. [Google Scholar] [CrossRef]
- Dey, M.; Rao, C.P.; Saarenketo, P.K.; Rissanen, K. Mono-, di- and tri-nuclear Ni(II) complexes of N–, O–donor ligands: Structural diversity and reactivity. Inorg. Chem. Commun. 2002, 5, 924–928. [Google Scholar] [CrossRef]
- Senthil Kumar, K.; Bayeh, Y.; Gebretsadik, T.; Elemo, F.; Gebrezgiabher, M.; Thomas, M.; Ruben, M. Spin-crossover in iron(ii)-Schiff base complexes. Dalton Trans. 2019, 48, 15321–15337. [Google Scholar] [CrossRef] [PubMed]
- Lucaccini, E.; Baldoví, J.J.; Chelazzi, L.; Barra, A.L.; Grepioni, F.; Costes, J.P.; Sorace, L. Electronic Structure and Magnetic Anisotropy in Lanthanoid Single-Ion Magnets with C3 Symmetry: The Ln(trenovan) Series. Inorg. Chem. 2017, 56, 4728–4738. [Google Scholar] [CrossRef] [PubMed]
- Guo, M.; Tang, J. Six-Coordinate Ln(III) Complexes with Various Magnetic Properties. Inorganics 2018, 6, 16. [Google Scholar] [CrossRef]
- Cheng, L.W.; Zhang, C.L.; Wei, J.Y.; Lin, P.H. Mononuclear and trinuclear DyIII SMMs with Schiff-base ligands modified by nitro-groups: First triangular complex with a N–N pathway. Dalton Trans. 2019, 48, 17331–17339. [Google Scholar] [CrossRef] [PubMed]
- Lin, P.H.; Korobkov, I.; Burchell, T.J.; Murugesu, M. Connecting single-ion magnets through ligand dimerisation. Dalton Trans. 2012, 41, 13649–13655. [Google Scholar] [CrossRef] [PubMed]
- Zhao, L.; Wu, J.; Ke, H.; Tang, J. Three dinuclear lanthanide (III) compounds of a relaxation behaviour of the DyIII derivative. CrystEngComm 2013, 15, 5301–5306. [Google Scholar] [CrossRef]
- Zhang, K.; Wang, Y. A dinuclear dysprosium single-molecule magnet constructed by o-vanillin Schiff base ligand: Magnetic properties and solution behaviour. Inorg. Chem. Commun. 2017, 76, 103–107. [Google Scholar] [CrossRef]
- Habib, F.; Brunet, G.; Vieru, V.; Korobkov, I.; Chibotaru, L.F.; Murugesu, M. Significant Enhancement of Energy Barriers in Dinuclear Dysprosium Single-Molecule Magnets Through Electron-Withdrawing Effects. J. Am. Chem. Soc. 2013, 135, 13242–13245. [Google Scholar] [CrossRef]
- Zou, L.F.; Zhao, L.; Guo, Y.N.; Yu, G.M.; Guo, Y.; Tang, J.; Li, Y.H. A dodecanuclear heterometallic dysprosium-cobalt wheel exhibiting single-molecule magnet behaviour. Chem. Commun. 2011, 47, 8659–8661. [Google Scholar] [CrossRef]
- Benelli, C.; Gatteschi, D. Magnetism of Lanthanides in Molecular Materials with Transition-Metal Ions and Organic Radicals. Chem. Rev. 2002, 102, 2369–2387. [Google Scholar] [CrossRef]
- Rogers, M.T. Magnetochemistry; Springer: Berlin/Heidelberg, Germany, 1957; Volume 79, ISBN 9783642707353. [Google Scholar]
- Sun, O.; Chen, P.; Li, H.; Gao, T.; Sun, W.; Li, G.; Yan, P. A series of dinuclear lanthanide(III) complexes constructed from Schiff base and β-diketonate ligands: Synthesis, structure, luminescence and SMM behavior. CrystEngComm 2016, 18, 4627–4635. [Google Scholar] [CrossRef]
- Dou, W.; Yao, J.; Liu, W.; Wang, Y.; Zheng, J.-R.; Wang, D.-Q. Crystal structure and luminescent properties of new rare earth complexes with a flexible Salen-type ligand. Inorg. Chem. Commun. 2007, 10, 105–108. [Google Scholar] [CrossRef]
- Zhang, Y.X.; Cheng, X.Y.; Tang, Y.T.; Zhang, Y.H.; Wang, S.C.; Wei, H.Y.; Wu, Z.L. Two dinuclear lanthanide(III) clusters (Gd2 and Dy2) constructed by bis-(o-vanillin) schiff base ligand exhibiting fascinating magnetic behaviors. Polyhedron 2019, 166, 23–27. [Google Scholar] [CrossRef]
- Sharples, J.W.; Zheng, Y.Z.; Tuna, F.; McInnes, E.J.L.; Collison, D. Lanthanide discs chill well and relax slowly. Chem. Commun. 2011, 47, 7650–7652. [Google Scholar] [CrossRef] [PubMed]
- Li, X.H.; Li, M.; Gao, N.; Wei, H.Y.; Hou, Y.L.; Wu, Z.L. Solvent-driven structures and slow magnetic relaxation behaviors of dinuclear dysprosium clusters. Inorg. Chim. Acta 2020, 500, 119242. [Google Scholar] [CrossRef]
- Liu, S.J.; Yao, S.L.; Cao, C.; Zheng, T.F.; Liu, C.; Wang, Z.X.; Zhao, Q.; Liao, J.S.; Chen, J.L.; Wen, H.R. Two di- and trinuclear Gd(III) clusters derived from monocarboxylates exhibiting significant magnetic entropy changes. Polyhedron 2017, 121, 180–184. [Google Scholar] [CrossRef]
- Cole, K.S.; Cole, R.H. Dispersion and absorption in dielectrics I. Alternating current characteristics. J. Chem. Phys. 1941, 9, 341–351. [Google Scholar] [CrossRef]
- Anastasiadis, N.C.; Granadeiro, C.M.; Mayans, J.; Raptopoulou, C.P.; Bekiari, V.; Cunha-Silva, L.; Psycharis, V.; Escuer, A.; Balula, S.S.; Konidaris, K.F.; et al. Multifunctionality in two families of dinuclear lanthanide(III) complexes with a tridentate schiff-base ligand. Inorg. Chem. 2019, 58, 9581–9585. [Google Scholar] [CrossRef]
- Holmberg, R.J.; Kuo, C.J.; Gabidullin, B.; Wang, C.W.; Clérac, R.; Murugesu, M.; Lin, P.H. A propeller-shaped μ4-carbonate hexanuclear dysprosium complex with a high energetic barrier to magnetisation relaxation. Dalton Trans. 2016, 45, 16769–16773. [Google Scholar] [CrossRef]
- Zhang, L.; Zhang, P.; Zhao, L.; Wu, J.; Guo, M.; Tang, J. Anions influence the relaxation dynamics of mono-3-OH-capped triangular dysprosium aggregates. Inorg. Chem. 2015, 54, 5571–5578. [Google Scholar] [CrossRef]
- Lin, S.Y.; Zhao, L.; Ke, H.; Guo, Y.N.; Tang, J.; Guo, Y.; Dou, J. Steric hindrances create a discrete linear Dy4complex exhibiting SMM behaviour. Dalton Trans. 2012, 41, 3248–3252. [Google Scholar] [CrossRef]
- Chen, G.-J.; Gao, C.-Y.; Tian, J.-L.; Tang, J.; Gu, W.; Liu, X.; Yan, S.-P.; Liao, D.-Z.; Cheng, P. Coordination-perturbed single-molecule magnet behaviour of mononuclear dysprosium complexes. Dalton Trans. 2011, 40, 5579–5583. [Google Scholar] [CrossRef]
- Ke, H.; Gamez, P.; Zhao, L.; Xu, G.F.; Xue, S.; Tang, J. Magnetic properties of dysprosium cubanes dictated by the M–O–M angles of the [Dy4(μ3-OH)4] core. Inorg. Chem. 2010, 49, 7549–7557. [Google Scholar] [CrossRef] [PubMed]
- Gao, H.; Huang, S.; Zhou, X.; Liu, Z.; Cui, J. Magnetic properties and structure of tetranuclear lanthanide complexes based on 8-hydroxylquinoline Schiff base derivative and β-diketone coligand. Dalton Trans. 2018, 47, 3503–3511. [Google Scholar] [CrossRef] [PubMed]
- Gavrilenko, K.S.; Cador, O.; Bernot, K.; Rosa, P.; Sessoli, R.; Golhen, S.; Pavlishchuk, V.V.; Ouahab, L. Delicate Crystal Structure Changes Govern the Magnetic Properties of 1D Coordination Polymers Based on 3d Metal Carboxylates. Chem. Eur. J. 2008, 14, 2034–2043. [Google Scholar] [CrossRef] [PubMed]
- Lacelle, T.; Brunet, G.; Pialat, A.; Holmberg, R.J.; Lan, Y.; Gabidullin, B.; Korobkov, I.; Wernsdorfer, W.; Murugesu, M. Single-molecule magnet behaviour in a tetranuclear DyIII complex formed from a novel tetrazine-centered hydrazone Schiff base ligand. Dalton Trans. 2017, 46, 2471–2478. [Google Scholar] [CrossRef]
- Ke, H.; Xu, G.; Guo, Y.; Gamez, P.; Beavers, C.M.; Teat, S.J.; Tang, J. A linear tetranuclear dysprosium(III) compound showing single-molecule magnet behaviour. Chem. Commun. 2010, 46, 6057–6059. [Google Scholar] [CrossRef]
- Kahn, M.L.; Ballou, R.; Porcher, P.; Kahn, O.; Sutter, J.P. Analytical determination of the {Ln–aminoxyl radical} exchange interaction taking into account both the ligand-field effect and the spin - Orbit coupling of the lanthanide ion (Ln = DyIII and HoIII). Chem. Eur. J. 2002, 8, 525–531. [Google Scholar] [CrossRef]
- Osa, S.; Kido, T.; Matsumoto, N.; Re, N.; Pochaba, A.; Mrozinsk, J. A Tetranuclear 3d–4f Single Molecule Magnet: [CuIILTbIII(hfac)2]2. J. Am. Chem. Soc. 2004, 420–421. [Google Scholar] [CrossRef]
- Dekker, C.; Arts, A.F.M.; Wijn, H.W.d.; Duyneveldt, A.J.v.; Mydosh, J.A. Activated dynamics in a two-dimensional Ising spin glass: Rb2Cu1−xCoxF4. Phys. Rev. B Condens. Matter 1989, 40, 11243–11251. [Google Scholar] [CrossRef]
- Spencer, G.H.; Cross, P.C.; Wiberg, K.B. 5-tetrazine. II. Infrared spectra. J. Chem. Phys. 1961, 35, 1939–1945. [Google Scholar] [CrossRef]
- Sueur, S.; Lagrenee, M.; Abraham, F.; Bremard, C. New syntheses of polyaza derivatives. Crystal structure of pyridazine-3,6-dicarboxylic acid. J. Hetrocycl. Cham. 1987, 24, 1285–1289. [Google Scholar] [CrossRef]
- Lu, J.; Li, X.L.; Zhu, Z.; Liu, S.; Yang, Q.; Tang, J. Linear hexanuclear helical dysprosium single-molecule magnets: The effect of axial substitution on magnetic interactions and relaxation dynamics. Dalton Trans. 2019, 48, 14062–14068. [Google Scholar] [CrossRef]
- Lu, J.; Li, X.-L.; Jin, C.; Yu, Y.; Tang, J. Dysprosium-based linear helicate clusters: Syntheses, structures, and magnetism. New J. Chem. 2020, 994–1000. [Google Scholar] [CrossRef]
- Zhang, L.; Zhang, Y.Q.; Zhang, P.; Zhao, L.; Guo, M.; Tang, J. Single-Molecule Magnet Behavior Enhanced by Synergic Effect of Single-Ion Anisotropy and Magnetic Interactions. Inorg. Chem. 2017, 56, 7882–7889. [Google Scholar] [CrossRef]
- Lu, J.; Zhang, Y.Q.; Li, X.L.; Guo, M.; Wu, J.; Zhao, L.; Tang, J. Influence of Magnetic Interactions and Single-Ion Anisotropy on Magnetic Relaxation within a Family of Tetranuclear Dysprosium Complexes. Inorg. Chem. 2019, 58, 5715–5724. [Google Scholar] [CrossRef]
- Wu, J.; Zhao, L.; Zhang, L.; Li, X.L.; Guo, M.; Powell, A.K.; Tang, J. Macroscopic Hexagonal Tubes of 3d–4 f Metallocycles. Angew. Chem. Int. Ed. 2016, 55, 15574–15578. [Google Scholar] [CrossRef]
- Mukherjee, S.; Lu, J.; Velmurugan, G.; Singh, S.; Rajaraman, G.; Tang, J.; Ghosh, S.K. Influence of Tuned Linker Functionality on Modulation of Magnetic Properties and Relaxation Dynamics in a Family of Six Isotypic Ln2 (Ln = Dy and Gd) Complexes. Inorg. Chem. 2016, 55, 11283–11298. [Google Scholar] [CrossRef] [PubMed]
- Lin, S.Y.; Xu, G.F.; Zhao, L.; Guo, Y.N.; Guo, Y.; Tang, J. Observation of slow magnetic relaxation in triple-stranded lanthanide helicates. Dalton Trans. 2011, 40, 8213–8217. [Google Scholar] [CrossRef] [PubMed]
- Wang, W.M.; Kang, X.M.; Shen, H.Y.; Wu, Z.L.; Gao, H.L.; Cui, J.Z. Modulating single-molecule magnet behavior towards multiple magnetic relaxation processes through structural variation in Dy4 clusters. Inorg. Chem. Front. 2018, 5, 1876–1885. [Google Scholar] [CrossRef]
- Wu, J.; Cador, O.; Li, X.L.; Zhao, L.; Le Guennic, B.; Tang, J. Axial Ligand Field in D4d Coordination Symmetry: Magnetic Relaxation of Dy SMMs Perturbed by Counteranions. Inorg. Chem. 2017, 56, 11211–11219. [Google Scholar] [CrossRef] [PubMed]
- Wu, J.; Li, X.L.; Zhao, L.; Guo, M.; Tang, J. Enhancement of Magnetocaloric Effect through Fixation of Carbon Dioxide: Molecular Assembly from Ln4 to Ln4 Cluster Pairs. Inorg. Chem. 2017, 56, 4104–4111. [Google Scholar] [CrossRef] [PubMed]
- Hou, Y.L.; Liu, L.; Hou, Z.T.; Sheng, C.Y.; Wang, D.T.; Ji, J.; Shi, Y.; Wang, W.M. A novel Dy4III cluster constructed by a multidentate 8-hydroxyquinoline Schiff base ligand: Structure and slow magnetic relaxation behavior. Inorg. Chem. Commun. 2020, 112, 107691. [Google Scholar] [CrossRef]
- Qin, L.; Yu, Y.Z.; Liao, P.Q.; Xue, W.; Zheng, Z.; Chen, X.M.; Zheng, Y.Z. A “Molecular Water Pipe”: A Giant Tubular Cluster {Dy72} Exhibits Fast Proton Transport and Slow Magnetic Relaxation. Adv. Mater. 2016, 28, 10772–10779. [Google Scholar] [CrossRef]
- Wang, W.M.; Qiao, W.Z.; Zhang, H.X.; Wang, S.Y.; Nie, Y.Y.; Chen, H.M.; Liu, Z.; Gao, H.L.; Cui, J.Z.; Zhao, B. Structures and magnetic properties of several phenoxo-O bridged dinuclear lanthanide complexes: Dy derivatives displaying substituent dependent magnetic relaxation behavior. Dalton Trans. 2016, 45, 8182–8191. [Google Scholar] [CrossRef]
- Lu, J.; Montigaud, V.; Cador, O.; Wu, J.; Zhao, L.; Li, X.L.; Guo, M.; Le Guennic, B.; Tang, J. Lanthanide(III) Hexanuclear Circular Helicates: Slow Magnetic Relaxation, Toroidal Arrangement of Magnetic Moments, and Magnetocaloric Effects. Inorg. Chem. 2019, 58, 11903–11911. [Google Scholar] [CrossRef]
- Mukherjee, S.; Chaudhari, A.K.; Xue, S.; Tang, J.; Ghosh, S.K. An asymmetrically connected hexanuclear DyIII6 cluster exhibiting slow magnetic relaxation. Inorg. Chim. Acta 2013, 35, 144–148. [Google Scholar] [CrossRef]
- Tian, H.; Guo, Y.; Zhao, L.; Tang, J.; Liu, Z. Hexanuclear Dysprosium(III) Compound Incorporating Vertex- and Edge-Sharing Dy 3 Triangles Exhibiting Single-Molecule-Magnet Behavior. Inorg. Chem. 2011, 50, 8688–8690. [Google Scholar] [CrossRef] [PubMed]

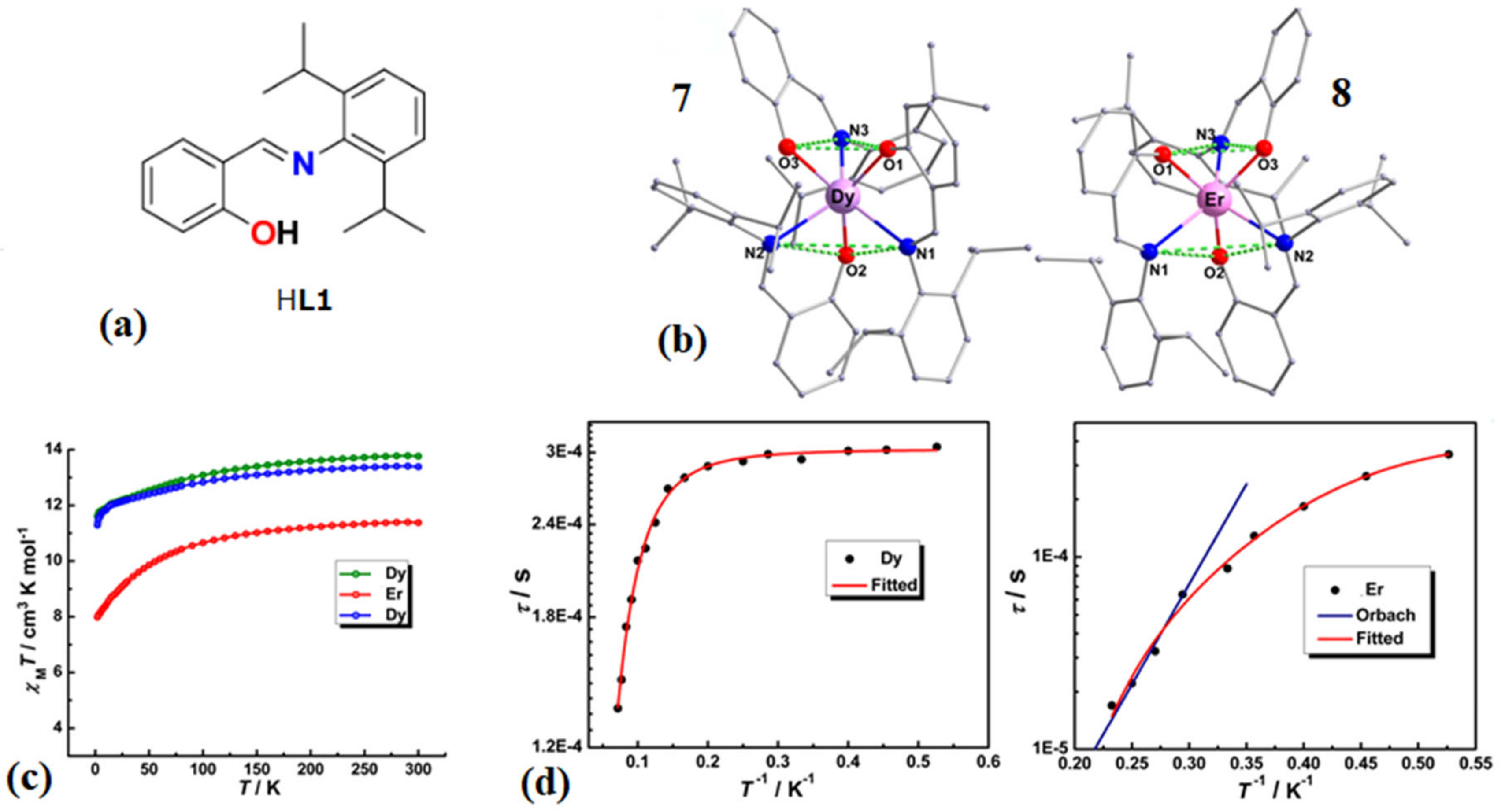
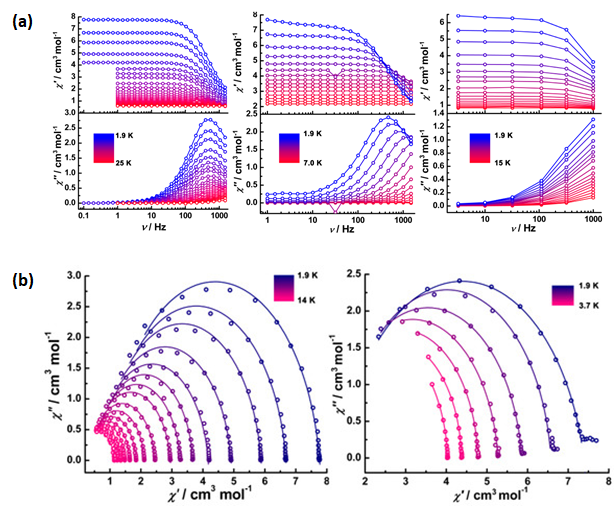
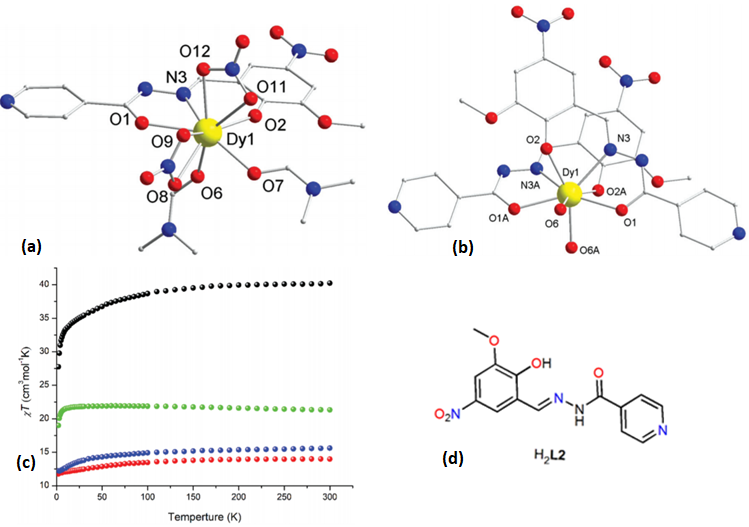
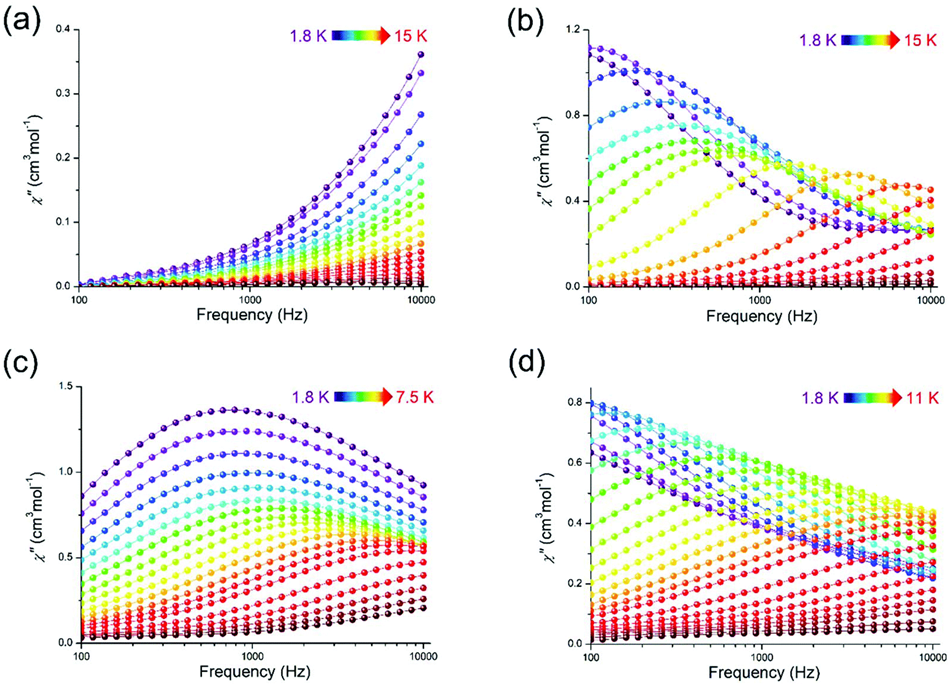
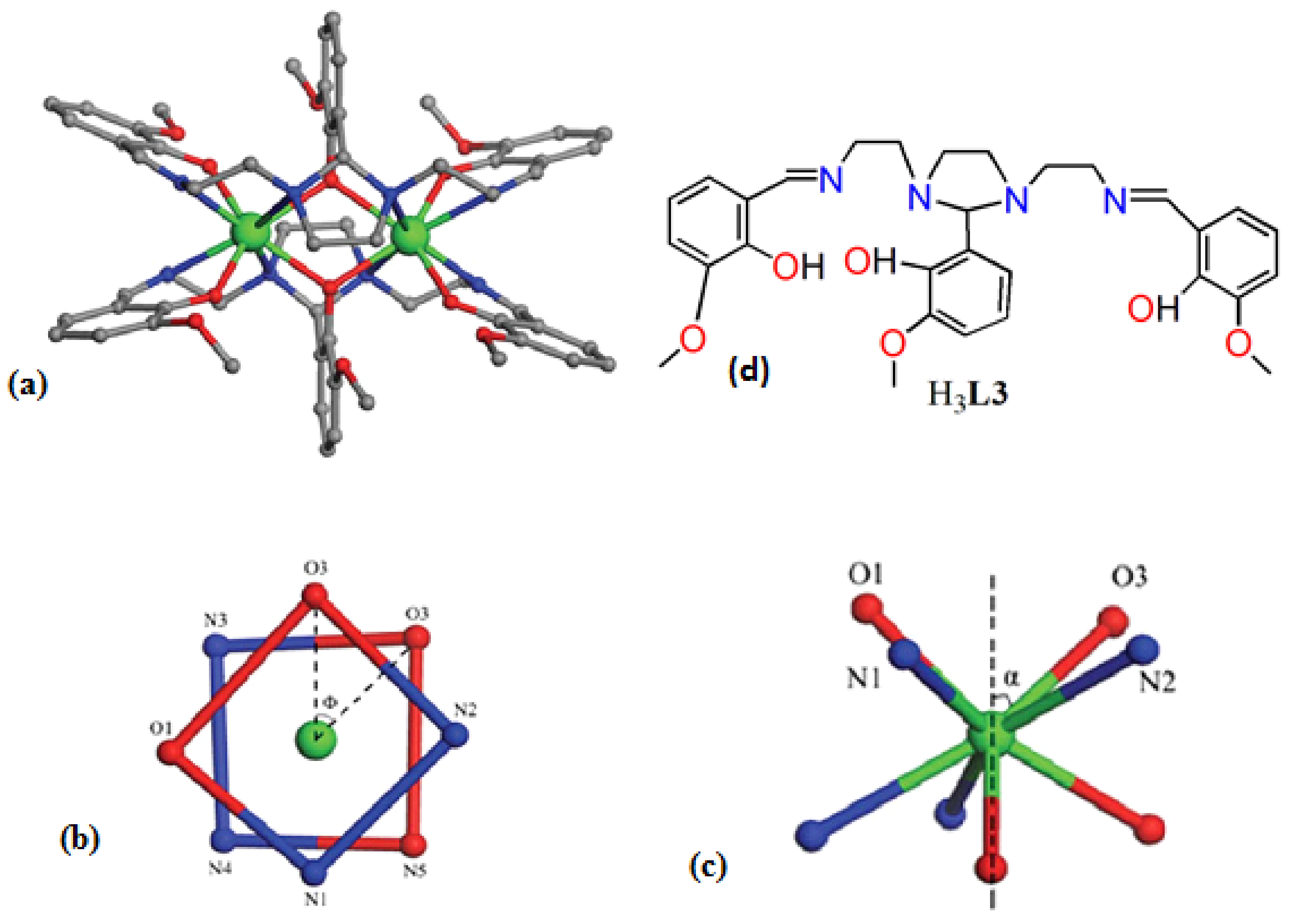
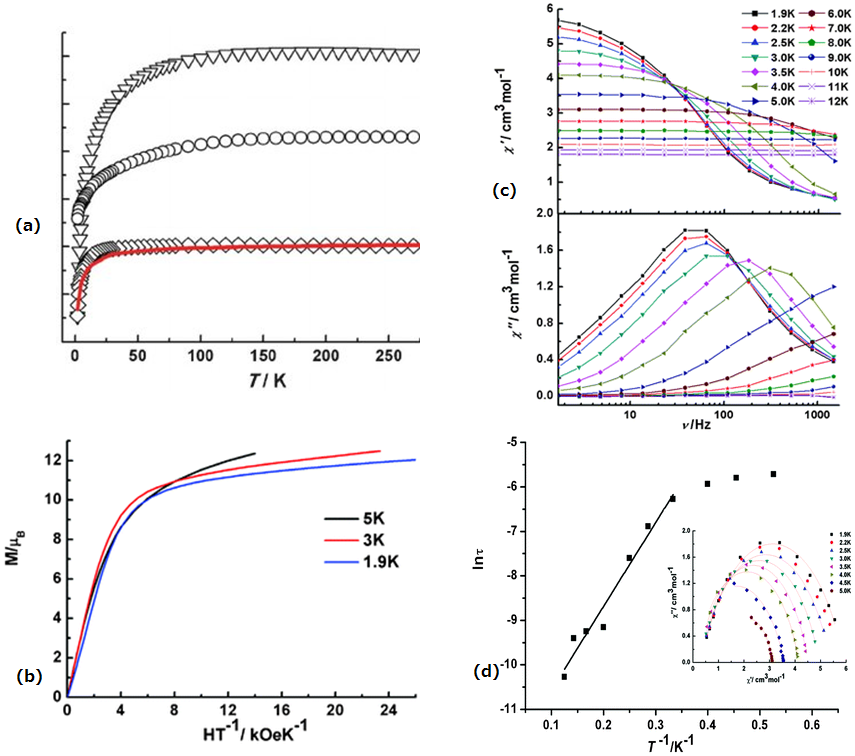
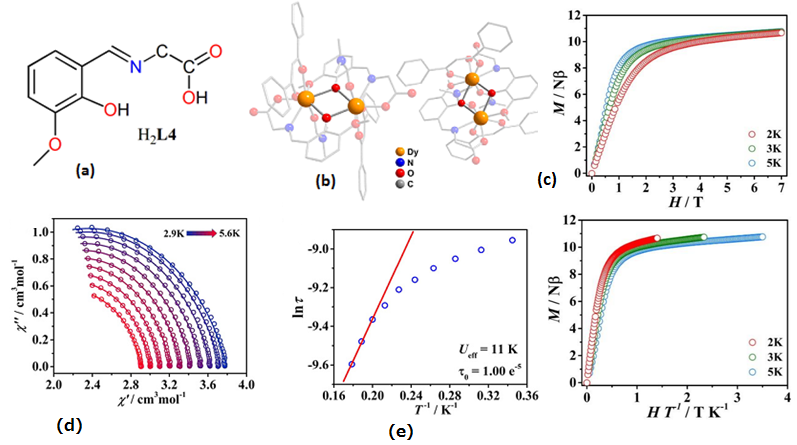


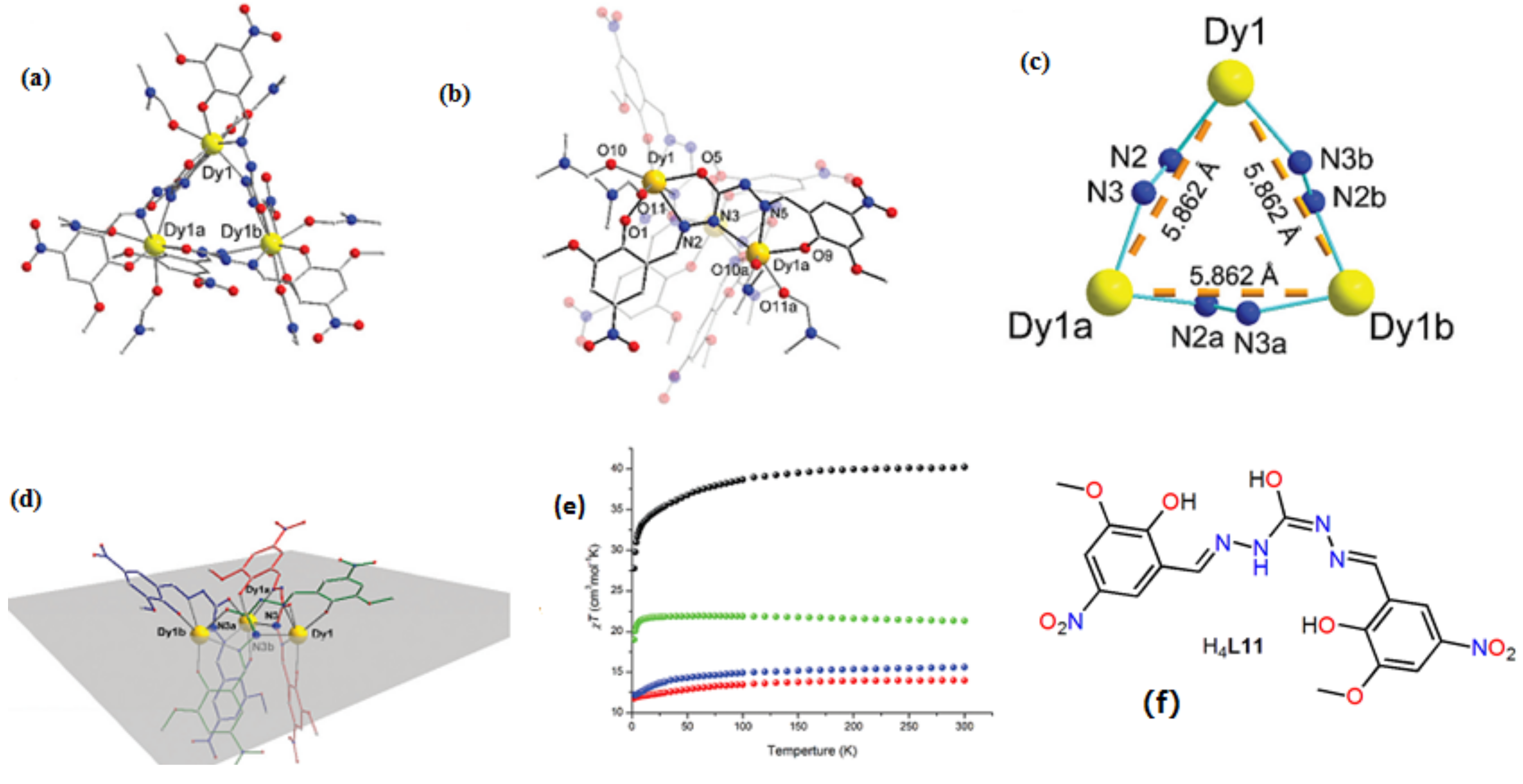
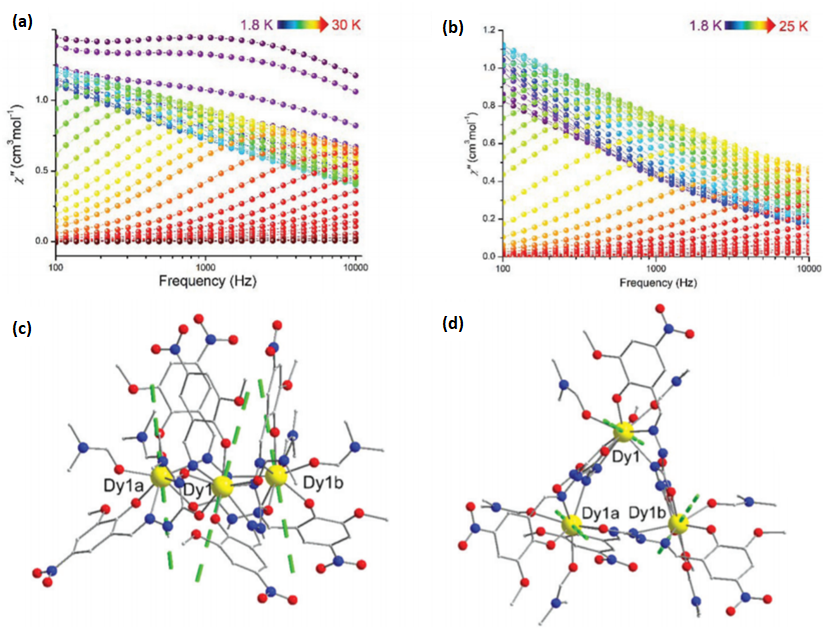
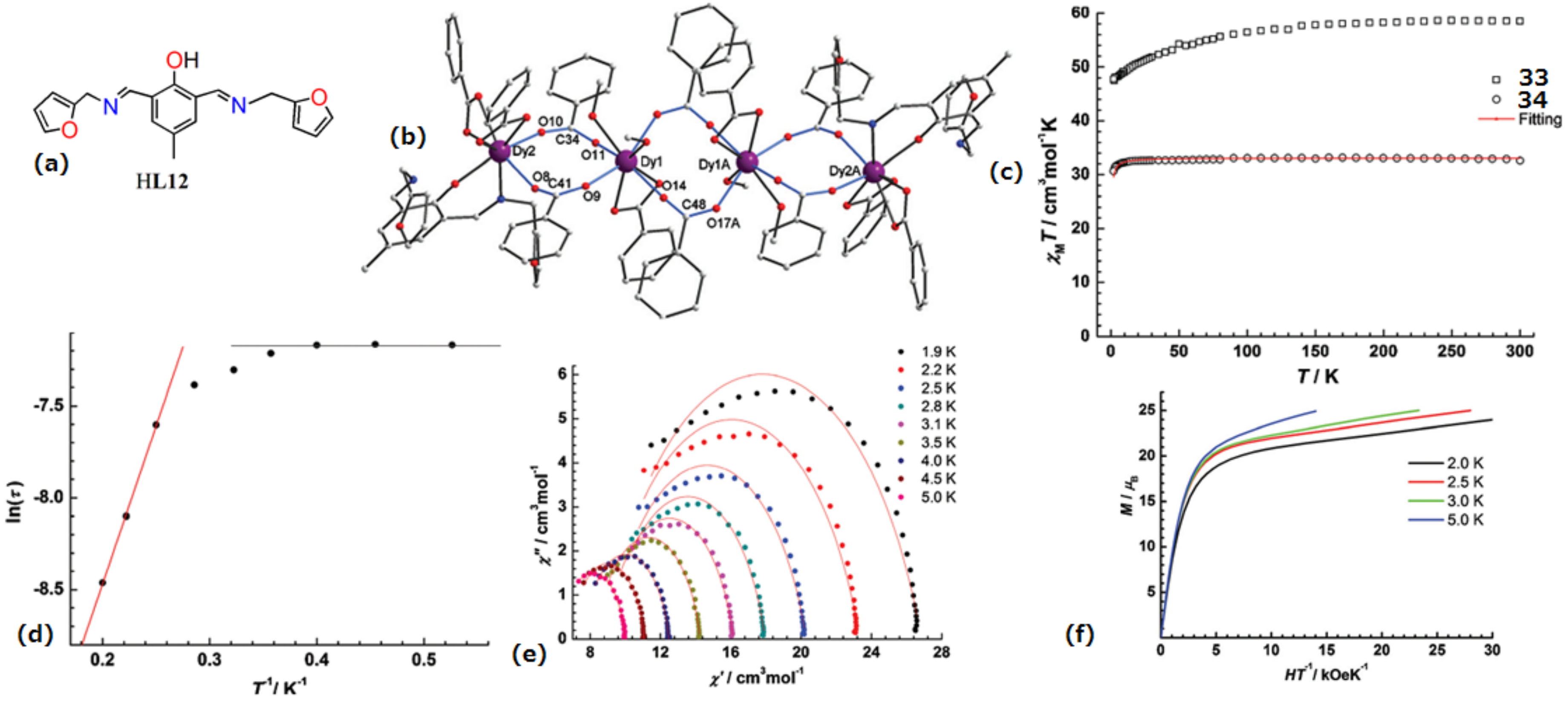
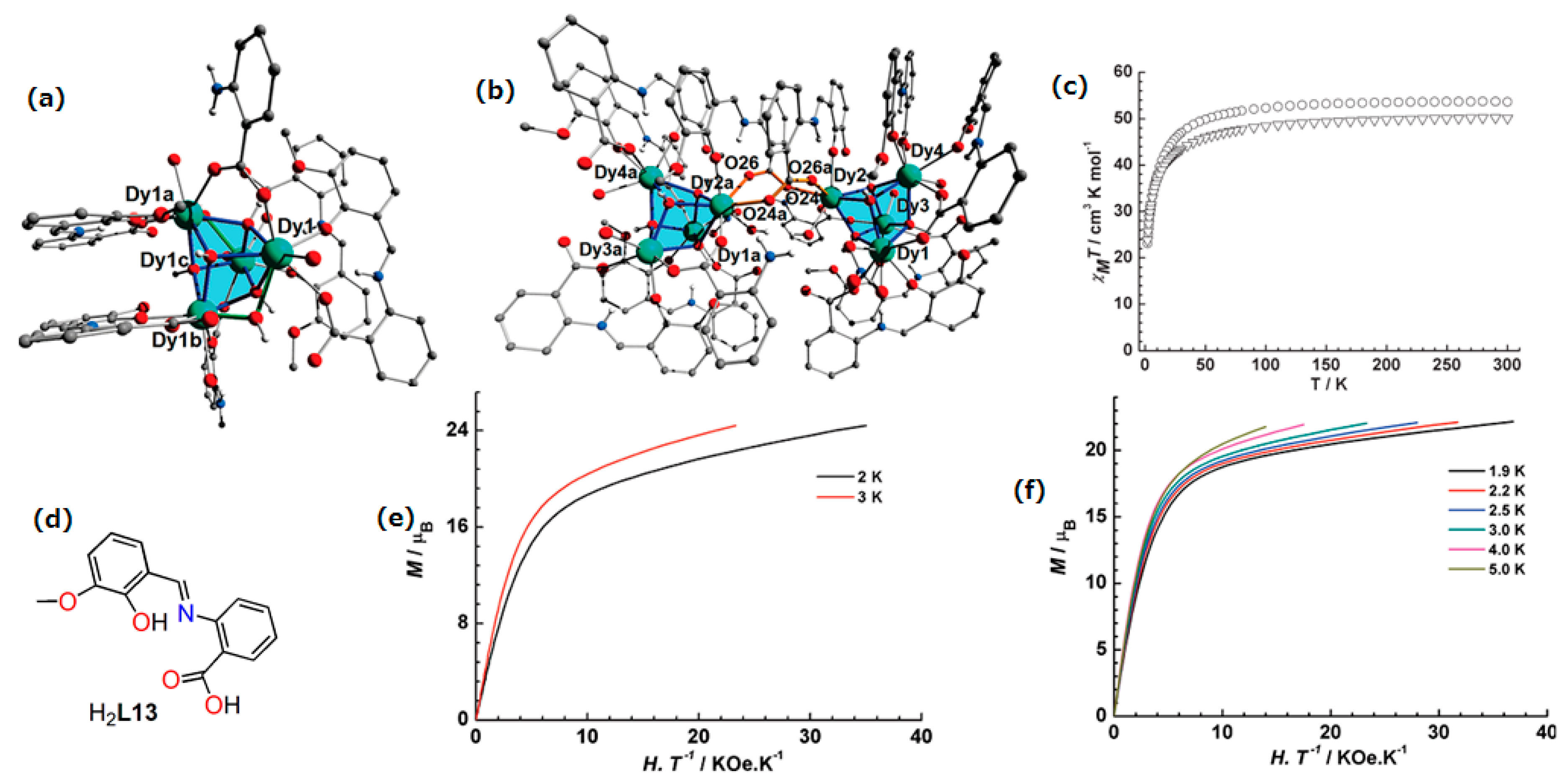
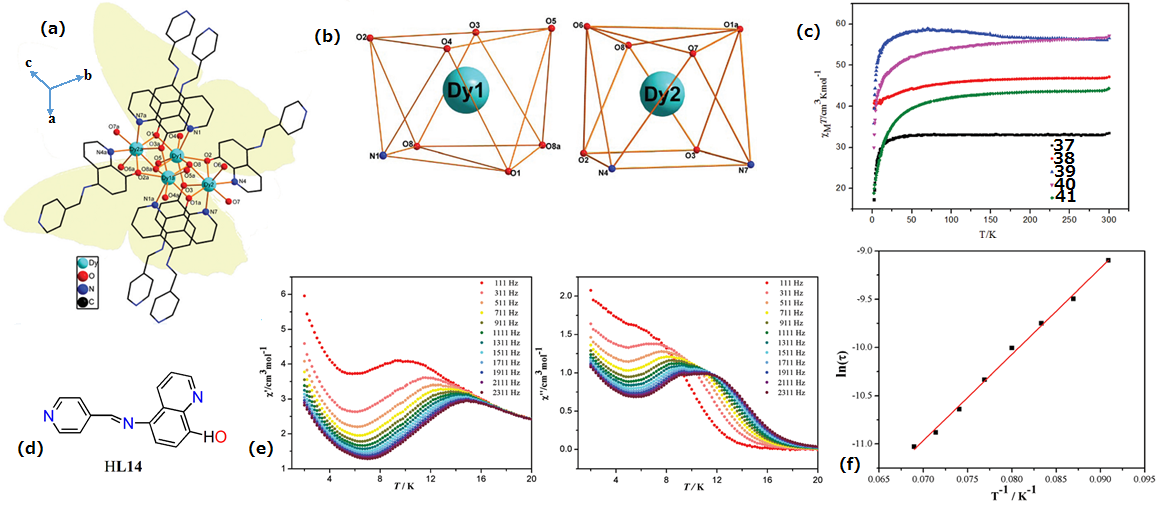
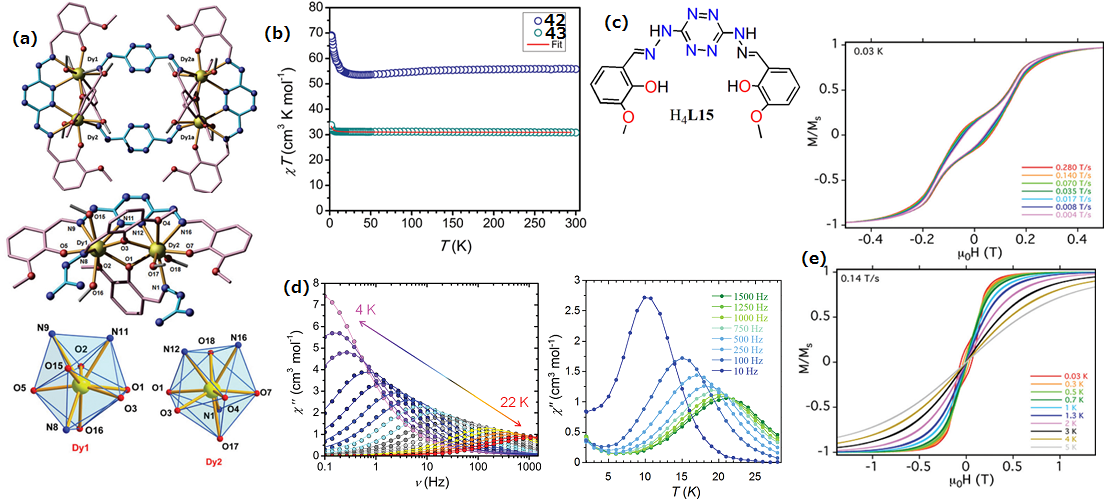
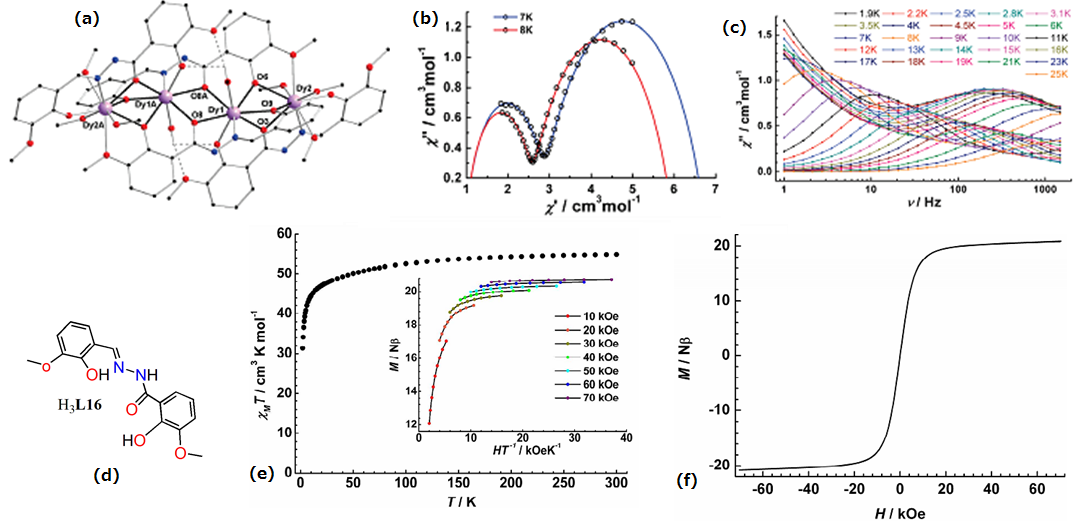

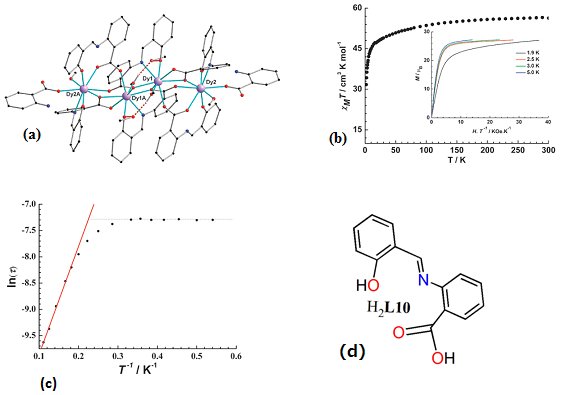
| Complex Formula and Number | Nuclearity | Coordination Environments | Coordination Polyhedra | Energy Barrier (Ueff/K) | Ref. |
|---|---|---|---|---|---|
| [Ln(L1)3](7) (ln = Dy) | Mononuclear | N3O3 | Distorted trigonal-prismatic | 31.4 K | [54] |
| [Ln(L1)3](8) (Ln = Er) | Mononuclear | N3O3 | Distorted trigonal-prismatic | 24 K | [54] |
| [Dy(L2)(NO3)(DMF)2]·DMF(9·DMF) | Mononuclear | N2O7 | Spherical triangular dodecahedron | 34 K | [55] |
| [Dy(L2)2(H2O)2]·NO3·EtOH (10·NO3·EtOH) | Mononuclear | N2O6 | Spherical triangular dodecahedron | 19 K | [55] |
| [Ln2(HL3)2]∙nCH3CN (Ln = Gd) (11) | Dinuclear | N4O4 | Square-antiprismatic | N/A | [57] |
| [Ln2(HL3)2]∙nCH3CN (Ln = Tb (12) | Dinuclear | N4O4 | Square-anti-prismatic | N/A | [57] |
| [Ln2(HL3)2]∙nCH3CN(Ln = Dy (13) | Dinuclear | N4O4 | Square-antiprismatic | 19 K | [57] |
| [Dy2(L4)2(DBM)2(DMF)2]·3CH3OH(14) | Dinuclear | NO7 | Square-antiprismatic | 11 K | [58] |
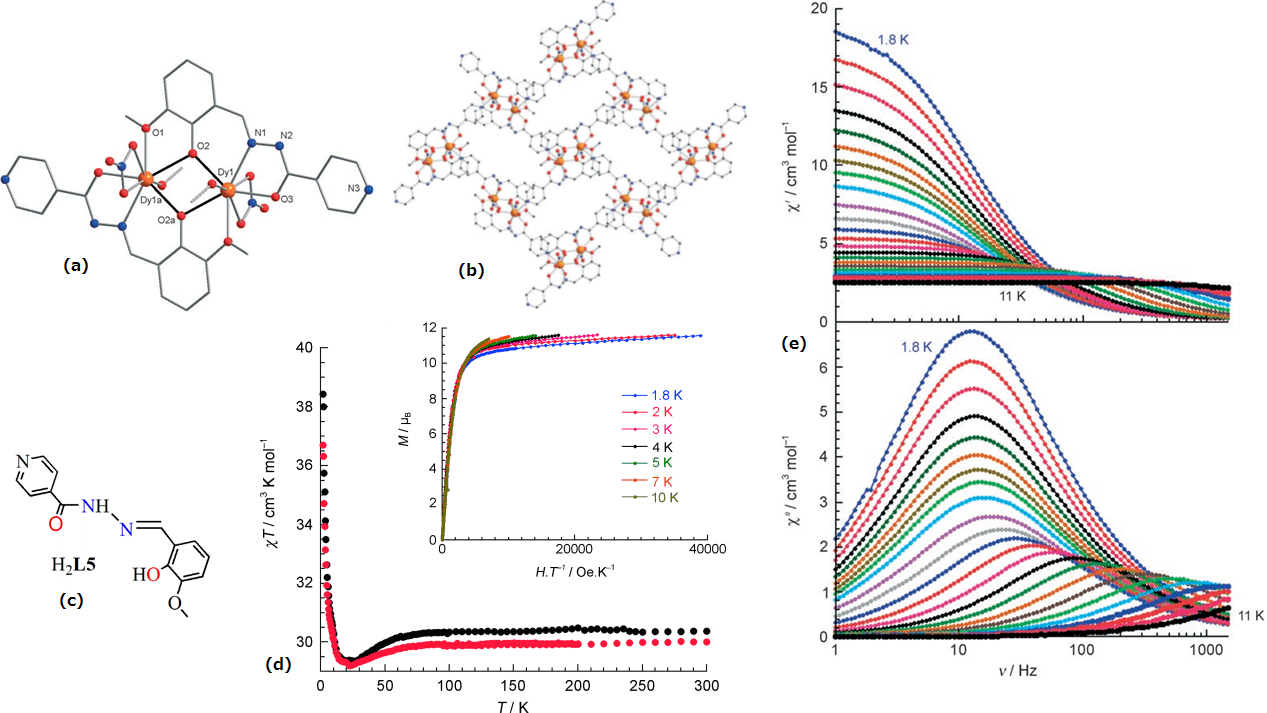
| [Dy2(HL5)2(NO3)2(MeOH)2](15) | Dinuclear | NO7 | Square-antiprismatic | 56 K | [38] |
| [Dy2(HL5)2(NO3)2(MeOH)2]∞(MeCN)∙(2MeCN)(16) | Dinuclear | NO7 | Square-antiprismatic | 71 K | [38] |
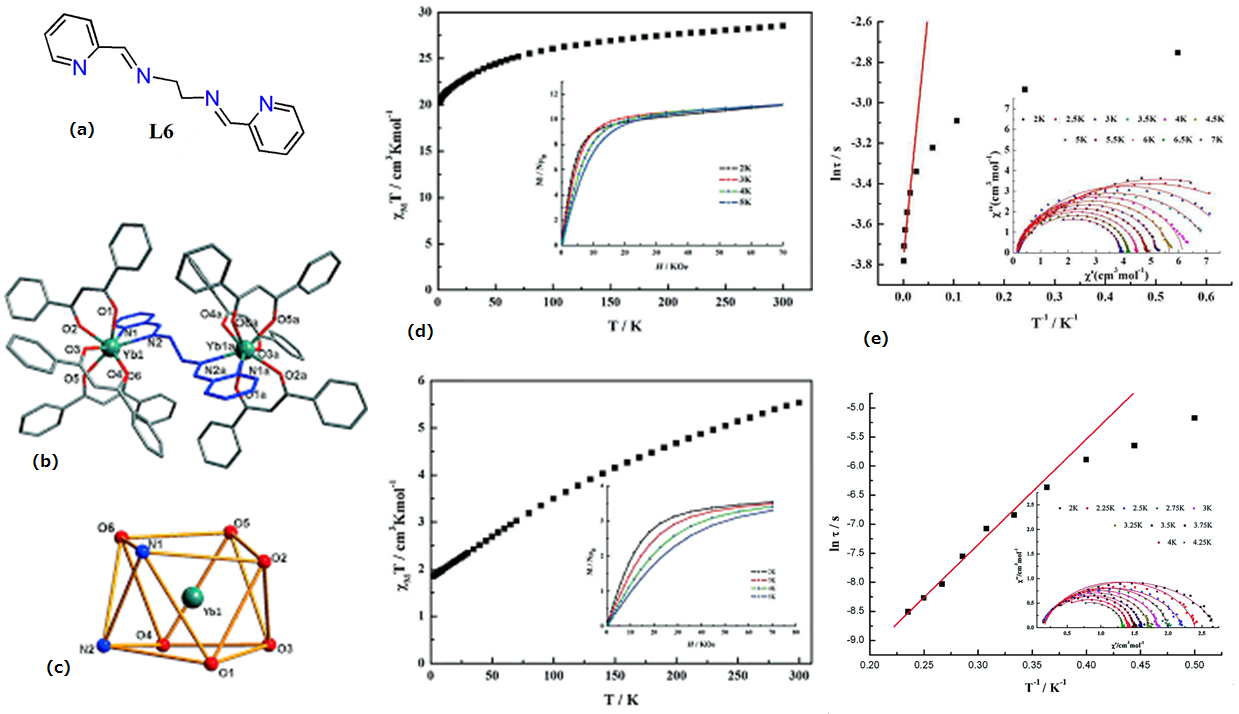
| Ln2(DBM)6(L6)] (Ln = Dy(17) and Ln = Yb(18) | Dinuclear | N2O6 | Distorted square antiprism | 47 (Dy) | [63] |
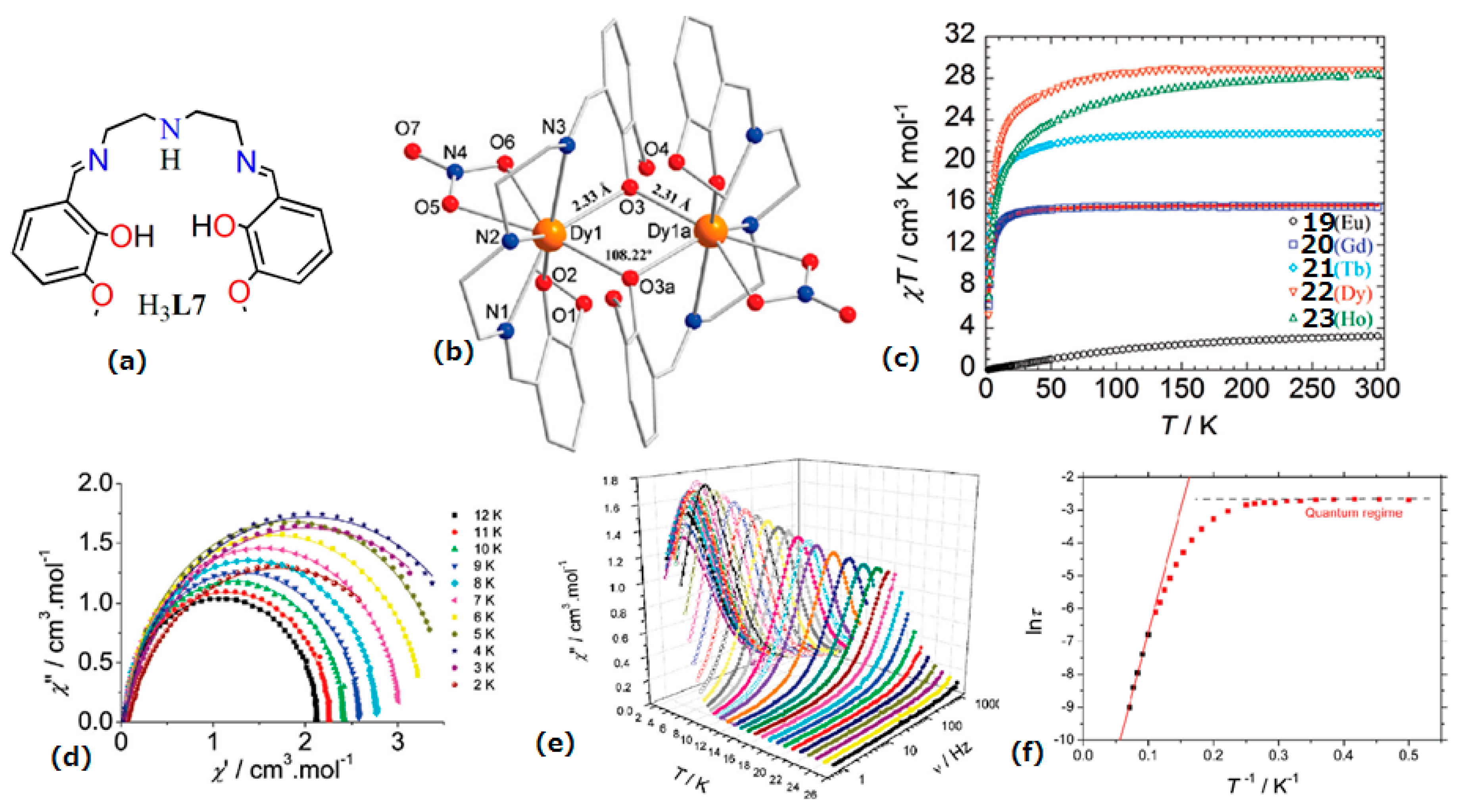
| [Ln(III)2(L7)2(NO3)2] (Ln(III) = Dy(III) (22) | Dinuclear | N3O5 | Square antiprism or/and dodecahedron | 76 K | [37] |
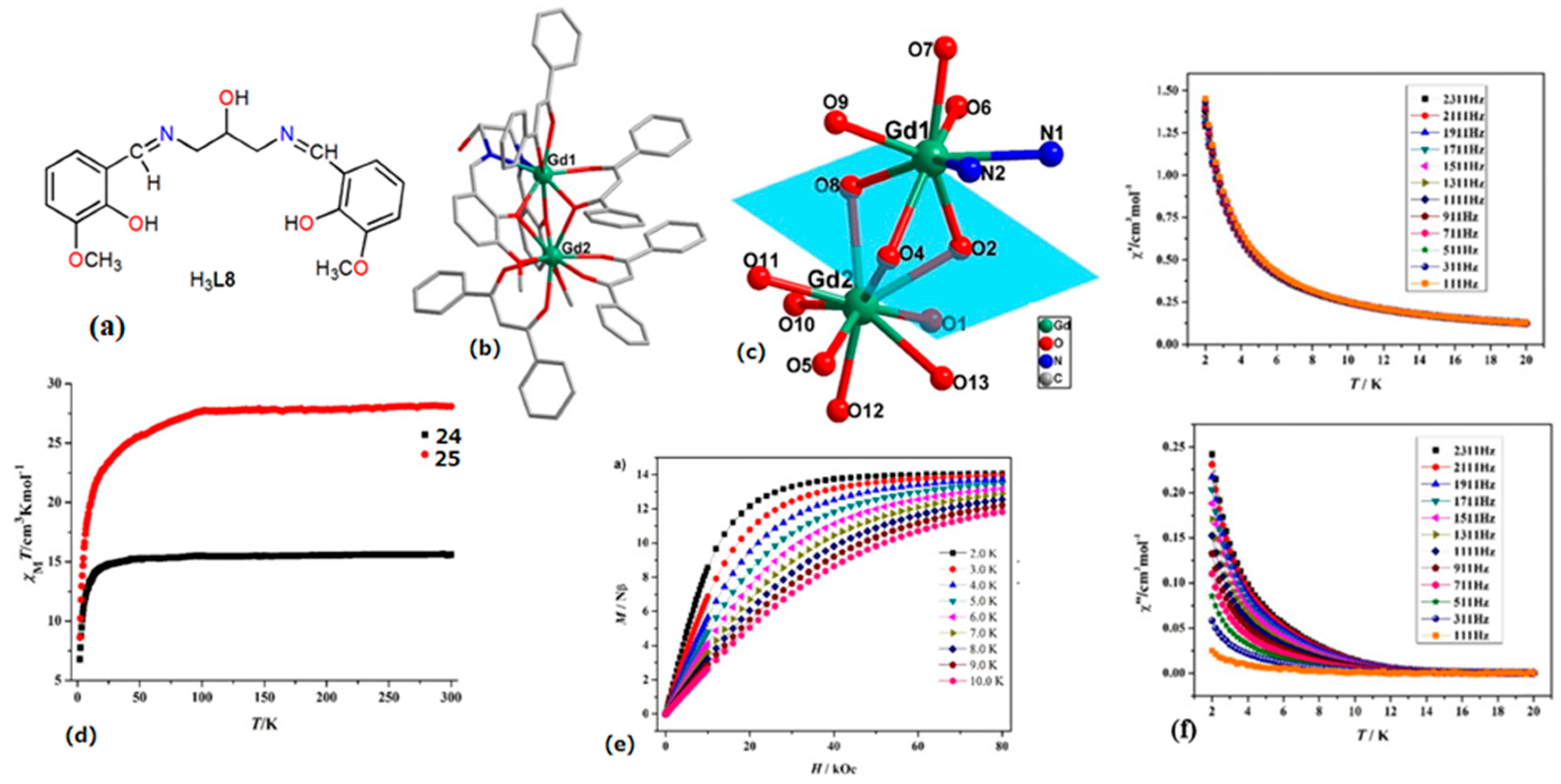
| [Ln2(HL8)(dbm)4]∙2CH3OH (Ln = Dy (25), | Dinuclear | N2O6 | Distorted dodecahedron | 0.73 K | [65] |
| [Dy2(dbm)2(HL9)2(C2H5OH)2] (28) | Dinuclear | N2O6 | Distorted bicapped trigonal-prismatic | 46 ± 3.2 K | [67] |
| [Ln2(NO3)4(HL10)2(MeOH)4]·MeOH, Ln = Gd (29·MeOH) and [Ln2(NO3)2(HL10)4]·6MeCN, Ln = Dy (30·6MeCN) | Dinuclear | N2O7 | Square-antiprismatic | N/A | [70] |
| [Dy3(HL11)3(DMF)6] (31) | Trinulear | N2O4 | Triangular dodecahedron | 80 K | [55] |
| [Dy4(L12)2(C6H5COO)12(MeOH)4](33) and [Gd4(L12)2- (C6H5COO)12(MeOH)4](34) | Tetranuclear | NO7 | Distorted bicapped trigonal-prismatic | 17 K | [73] |
| [Dy4(HL13)4(C6H4NH2COO)2(μ3-OH)4(μ-OH)2(H2O)4]∙4CH3CN3∙12H2O(35) | Tetranuclear | O8 | Tetranuclear cubane for 35 and octanuclear, bis-cubane for 36 | N/A | [75] |
| [Dy4(dbm)4(L14)6(μ3-OH)2]·4CH3CN·2H2O (39) | Tetranuclear | N2O6 | Square-antiprismatic | 89 K | [76] |
| [Ln4(H2L15)4(MeOH)8](NO3)4·aMeOH·bH2O, (Ln = Dy(III) (42) | Tetranuclear | N3O6 | Distorted spherical capped square antiprism | 158 K | [78] |
| [Dy4(HL16)4(MeOH)6]·2MeOH (44) | Tetranuclear | NO7 | Distorted bicapped trigonal-prismatic | 173 K | [44] |
| [Dy4(L10)4(HL10)2(C6H4NH2COO)2(CH3OH)4]·5CH3OH (45) | Tetranuclear | NO7 | Bi-capped trigonal prism or/and a square antiprism | 20 K | [79] |
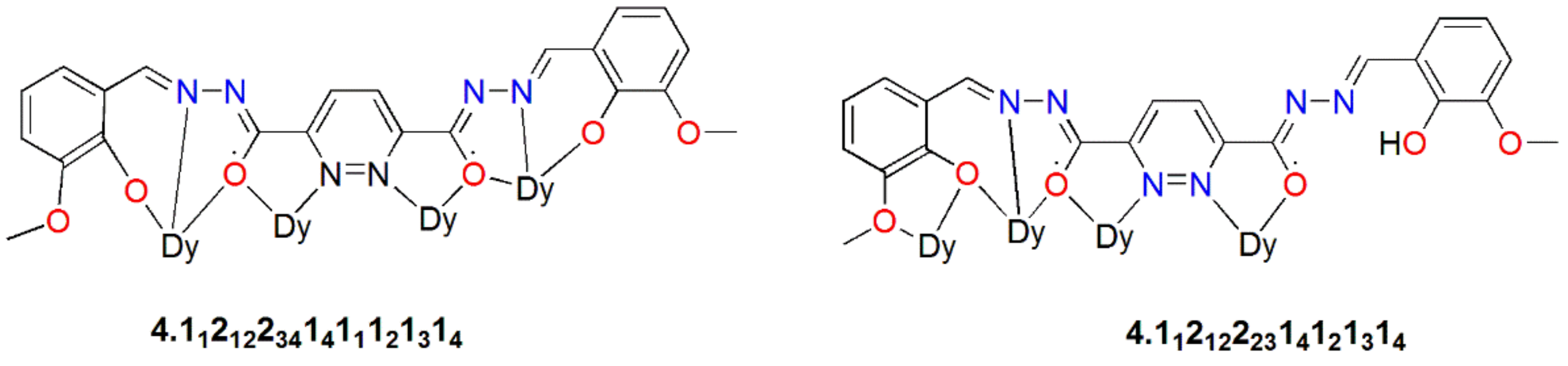
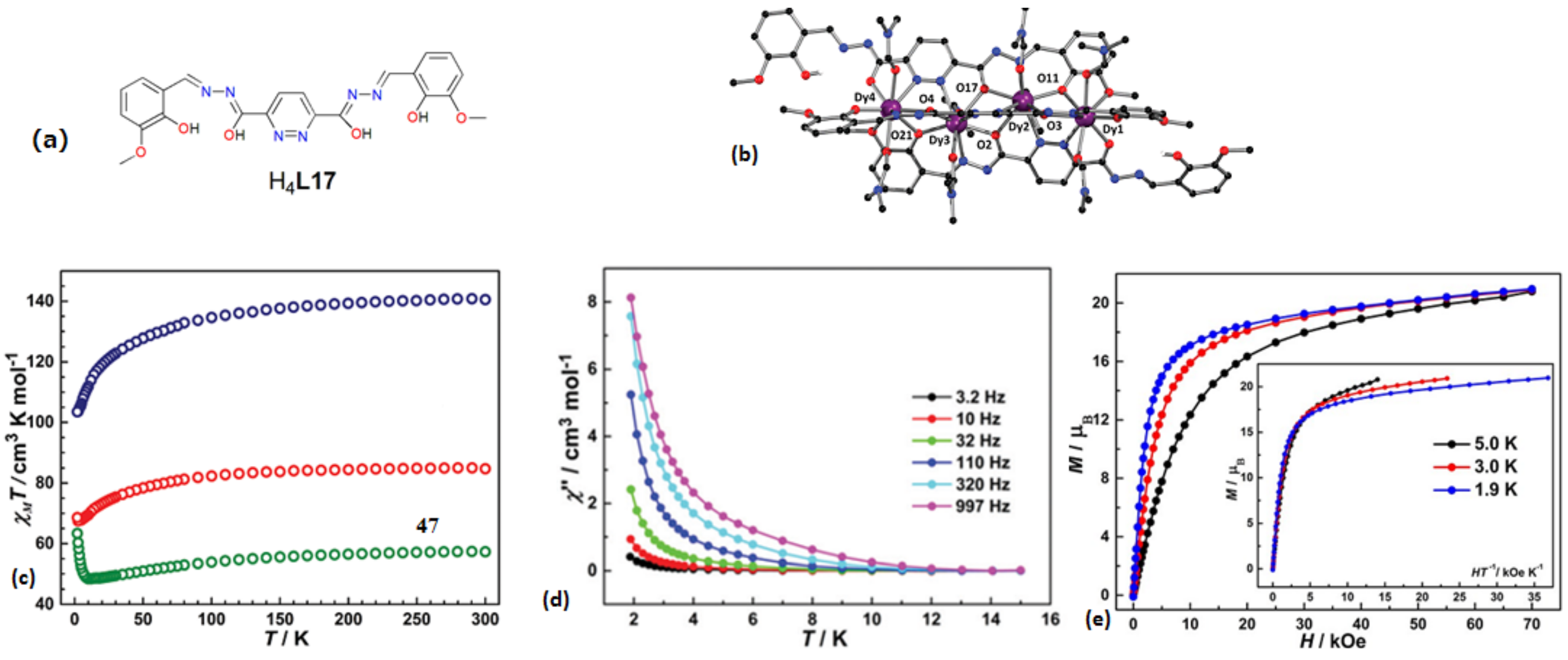
| [Dy4(HL17)2L17(DMF)8]∙2ClO4∙CH2Cl2∙4DMF∙(CH3CH2)∙2H2O(46) | Tetranuclear | O7N2/O6N3 | Distorted spherical tricapped trigonal prism/spherical capped square antiprism | ~4 K | [86] |
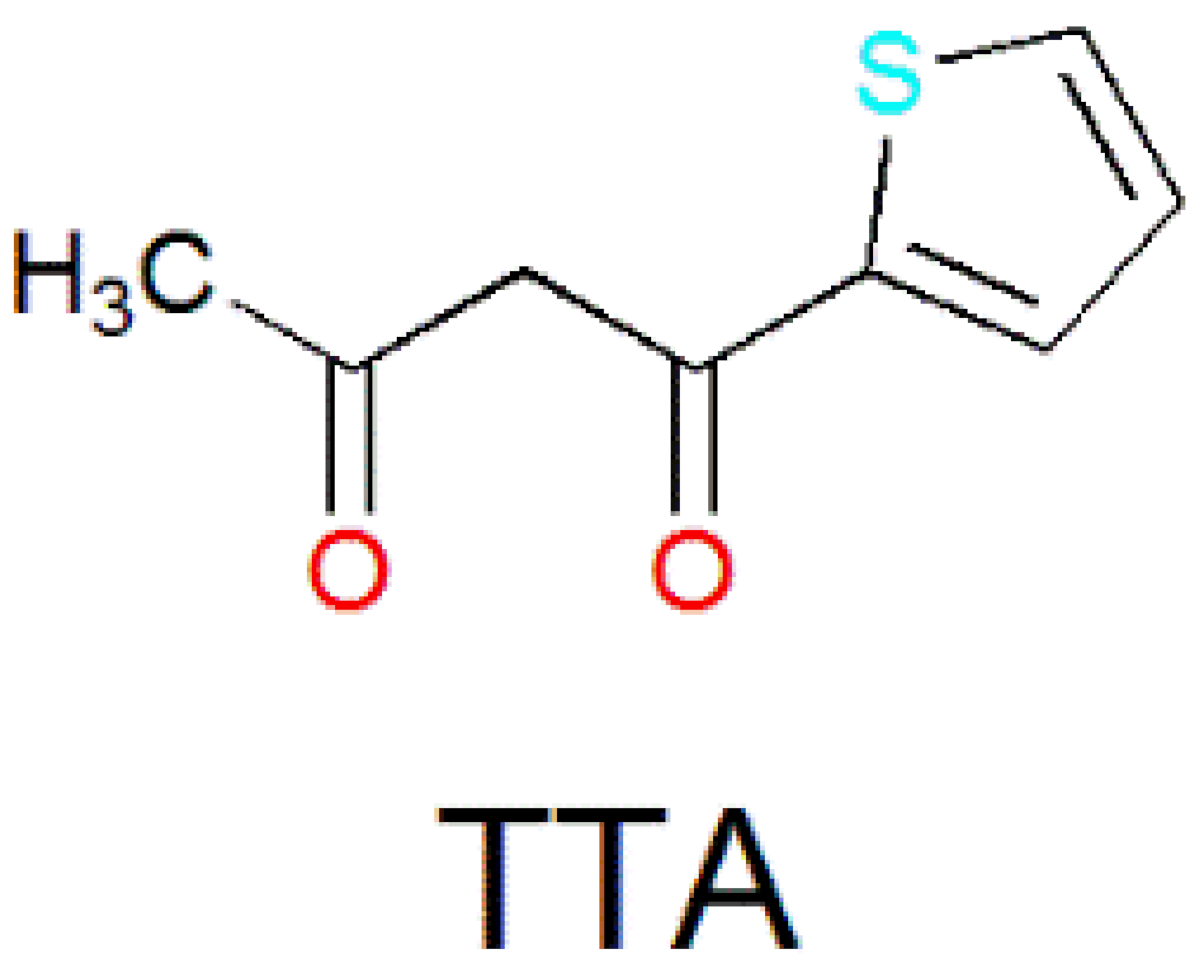
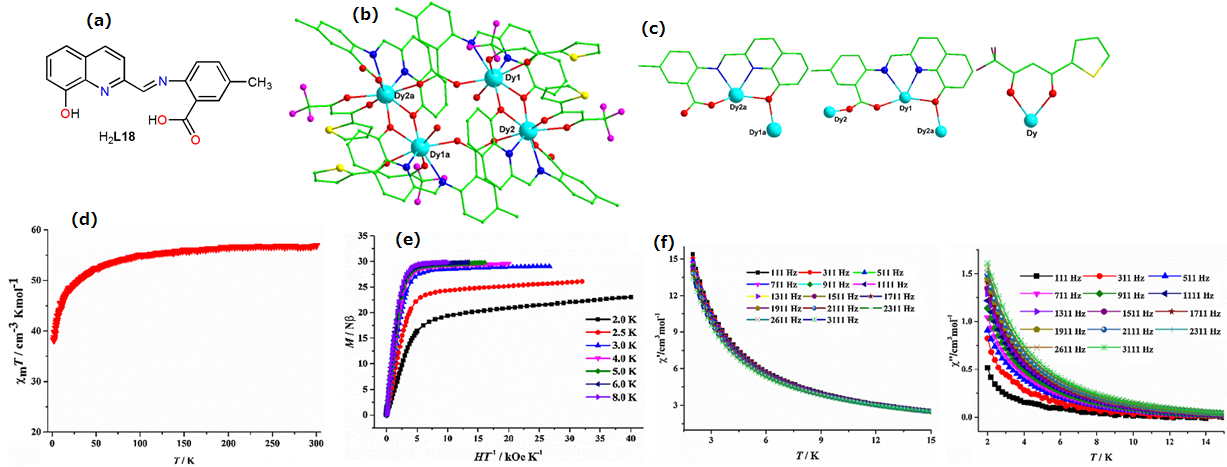
| [Dy4(TTA)4(L18)4(H2O)2]·4CH3OH (47) | Tetranuclear | N2O6 | Distorted square-antiprismatic | 1.5 K | [95] |
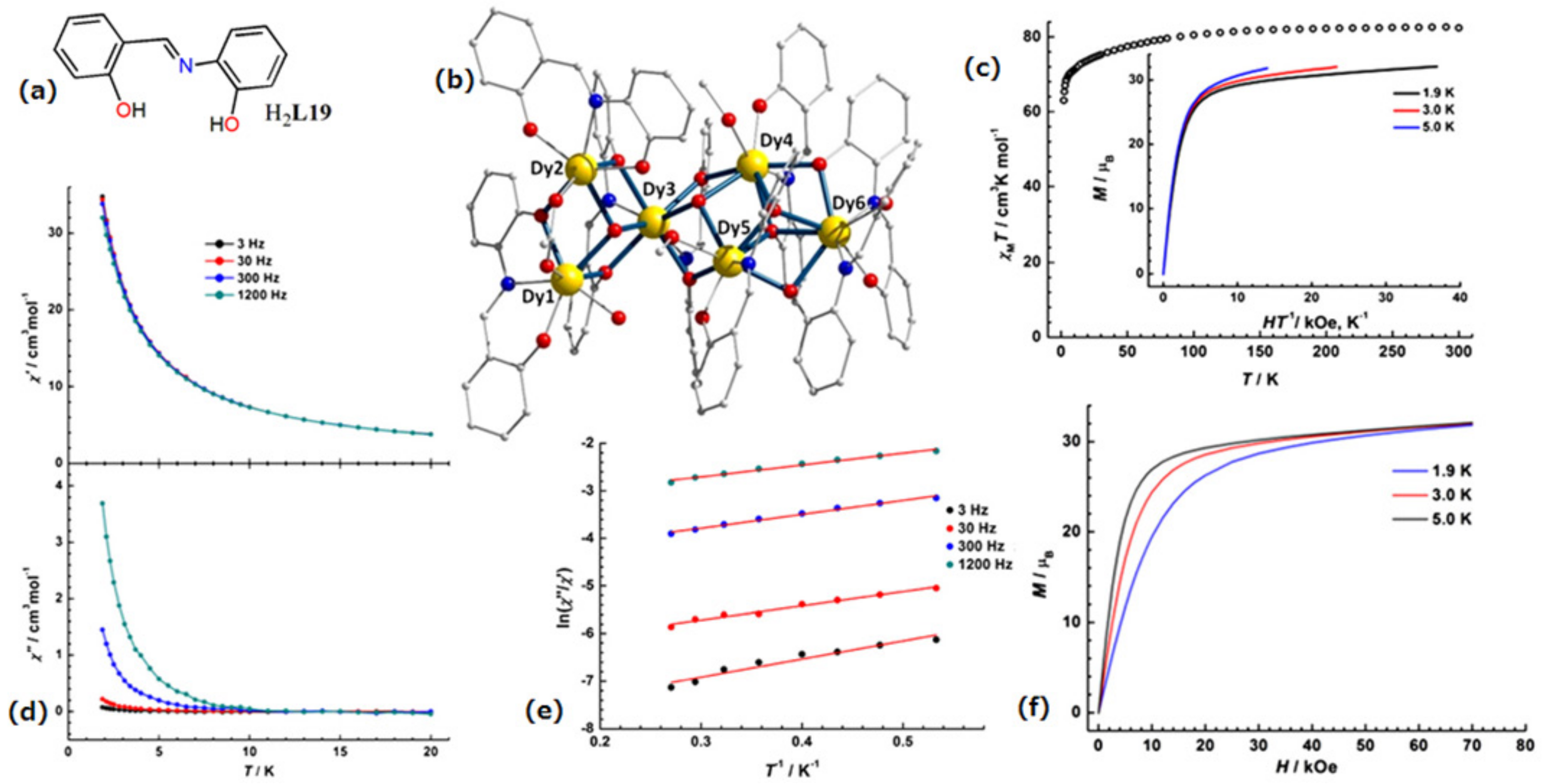
| [Dy6(L19)7(HL19)(MeOH)2(H2O)(OH)2(OAc)] (48) | Hexanuclear | N2O6 | square-antiprismatic | 3.0 K | [99] |
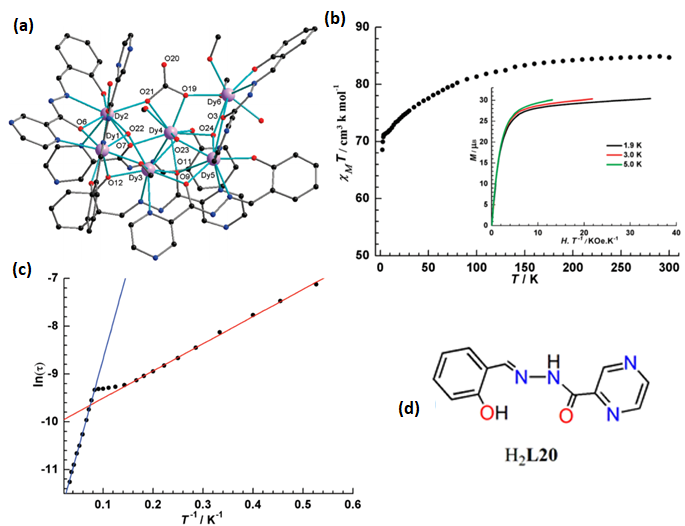
| [Dy6(µ3-OH)3(µ3-CO3)(µ-OMe)(L20)6(MeOH)4-(H2O)2]·3MeOH·2H2O (49) | Hexanuclear | NO7 | square-antiprismatic | 5.6 and 38 K | [100] |
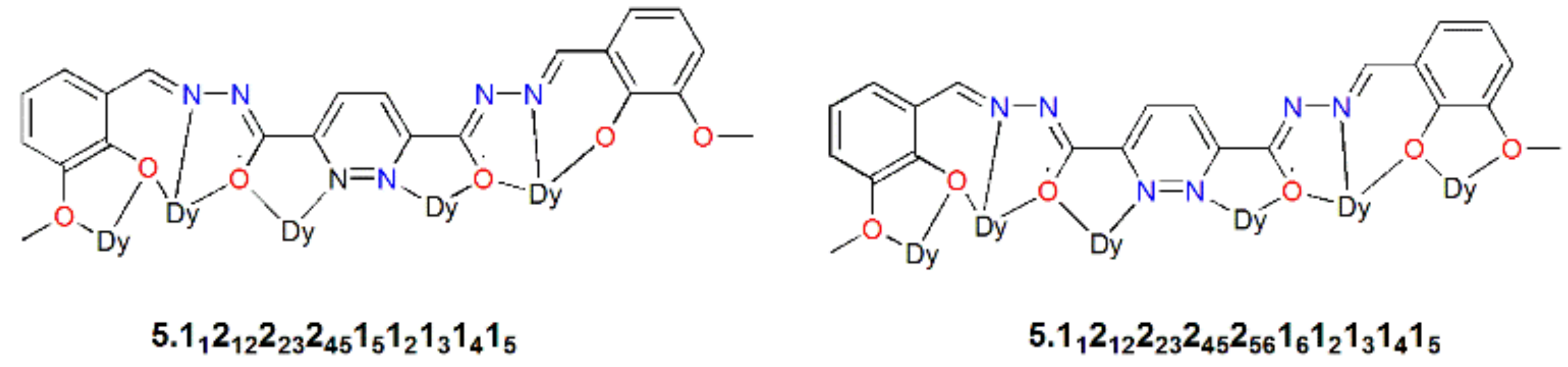
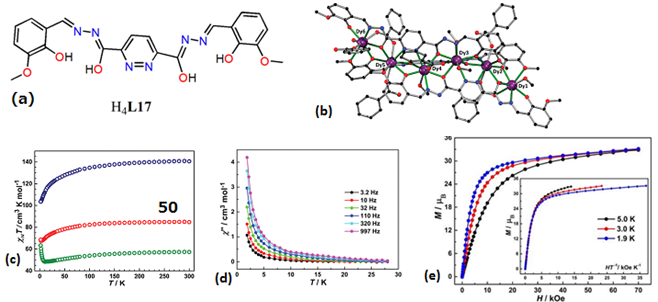
| [Dy6(L17)3(PhCOO)6(CH3OH)6]∙11CH3OH∙H2O (50) | Hexanuclear | N5O3/O7N2/O6N3 | Triple-stranded helical structure | 2 K | [86] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gebrezgiabher, M.; Bayeh, Y.; Gebretsadik, T.; Gebreslassie, G.; Elemo, F.; Thomas, M.; Linert, W. Lanthanide-Based Single-Molecule Magnets Derived from Schiff Base Ligands of Salicylaldehyde Derivatives. Inorganics 2020, 8, 66. https://doi.org/10.3390/inorganics8120066
Gebrezgiabher M, Bayeh Y, Gebretsadik T, Gebreslassie G, Elemo F, Thomas M, Linert W. Lanthanide-Based Single-Molecule Magnets Derived from Schiff Base Ligands of Salicylaldehyde Derivatives. Inorganics. 2020; 8(12):66. https://doi.org/10.3390/inorganics8120066
Chicago/Turabian StyleGebrezgiabher, Mamo, Yosef Bayeh, Tesfay Gebretsadik, Gebrehiwot Gebreslassie, Fikre Elemo, Madhu Thomas, and Wolfgang Linert. 2020. "Lanthanide-Based Single-Molecule Magnets Derived from Schiff Base Ligands of Salicylaldehyde Derivatives" Inorganics 8, no. 12: 66. https://doi.org/10.3390/inorganics8120066
APA StyleGebrezgiabher, M., Bayeh, Y., Gebretsadik, T., Gebreslassie, G., Elemo, F., Thomas, M., & Linert, W. (2020). Lanthanide-Based Single-Molecule Magnets Derived from Schiff Base Ligands of Salicylaldehyde Derivatives. Inorganics, 8(12), 66. https://doi.org/10.3390/inorganics8120066











