The Effect of Ge Doping on α-Ag2S’s Thermoelectric and Mechanical Properties
Abstract
1. Introduction
2. Results
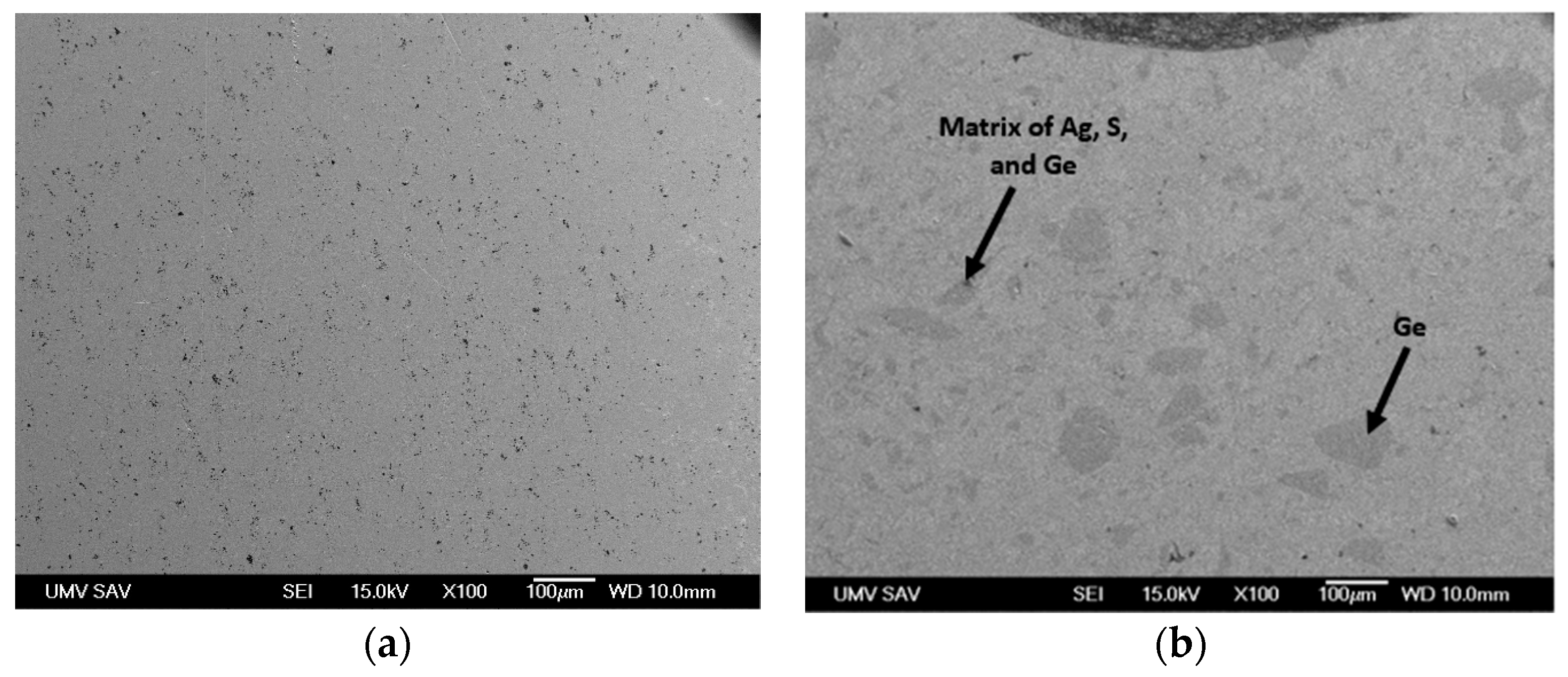
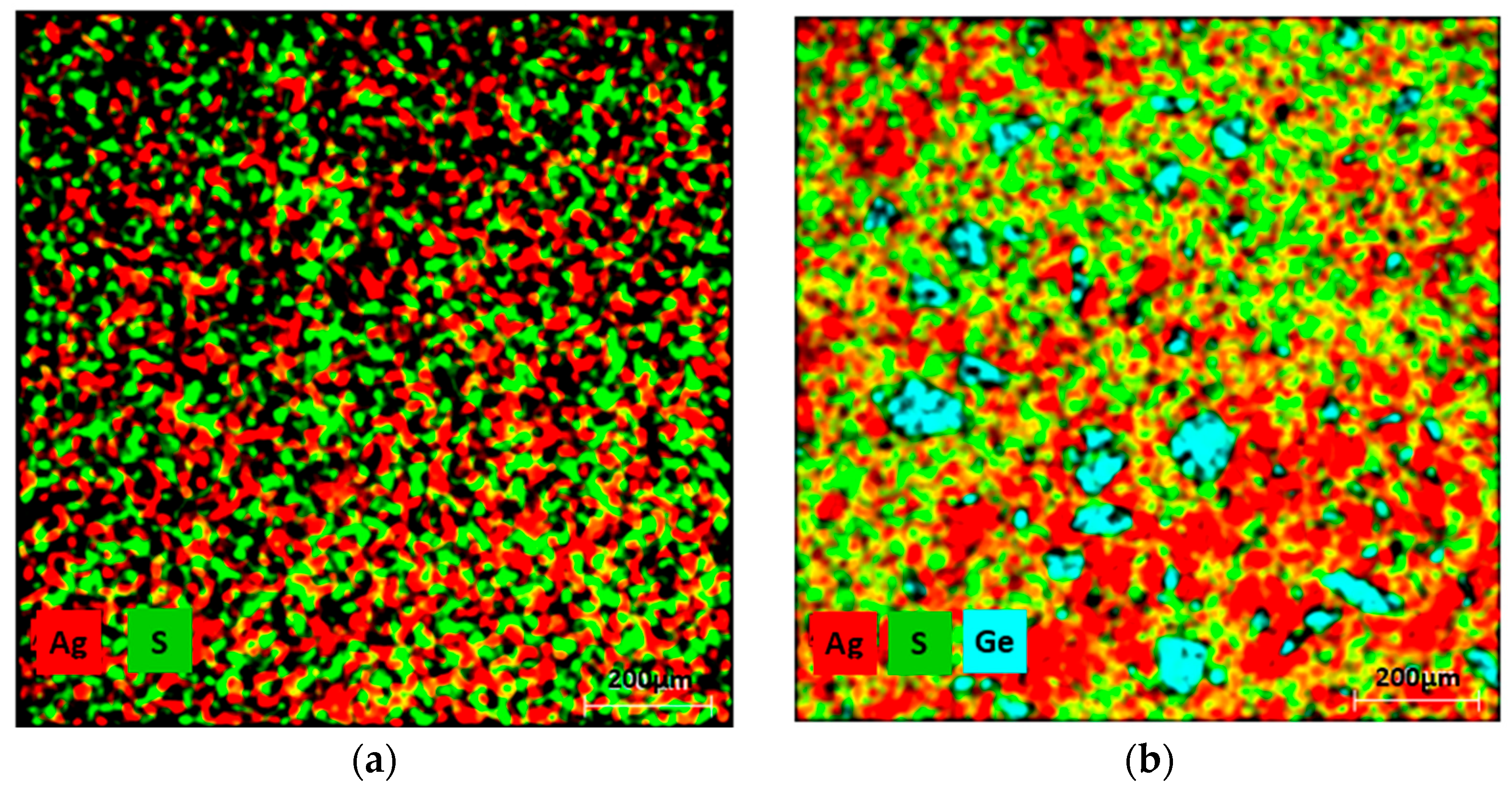
2.1. Chemical Composition and Electron Microscopy Analysis of the Microstructure
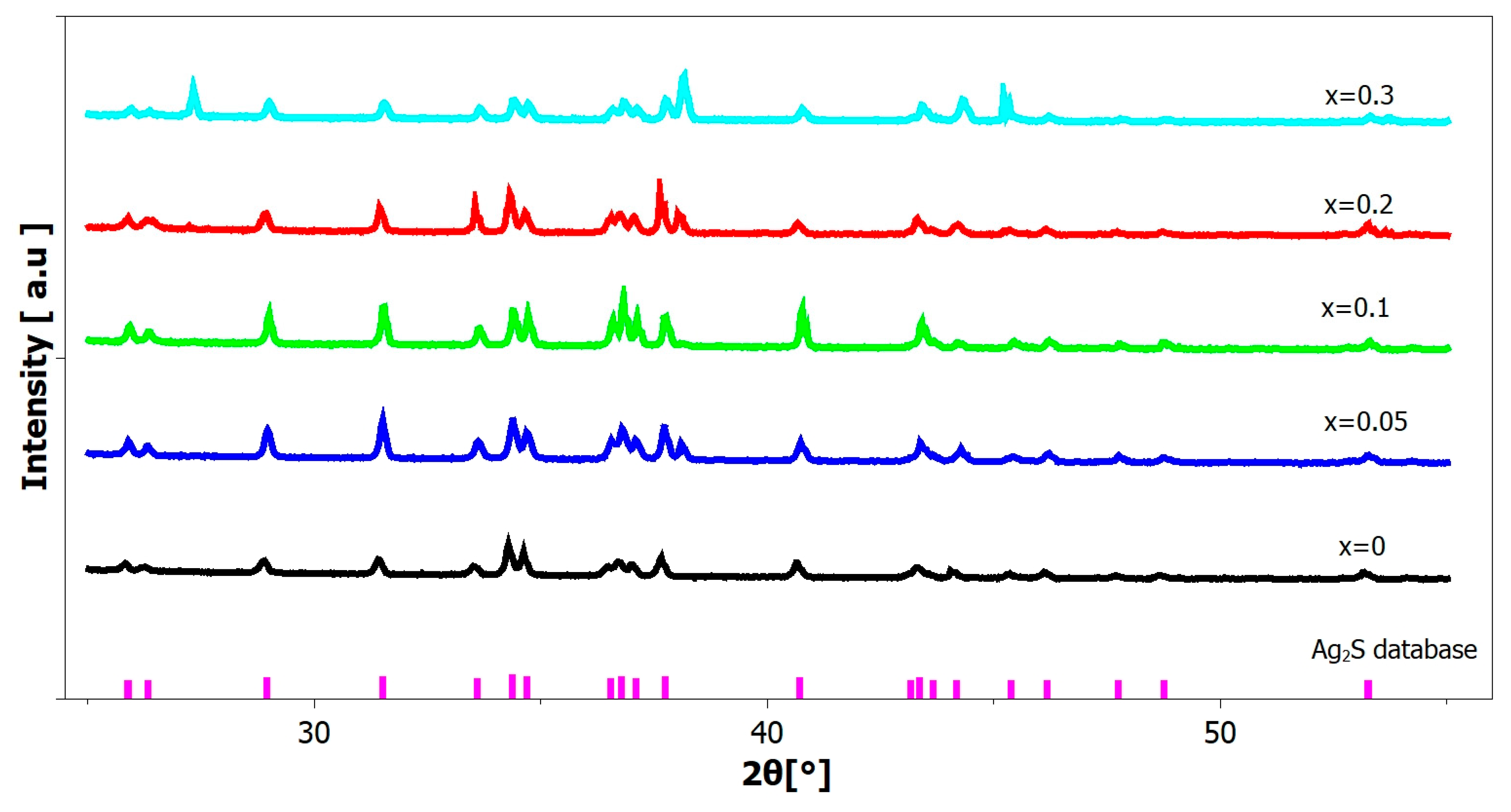
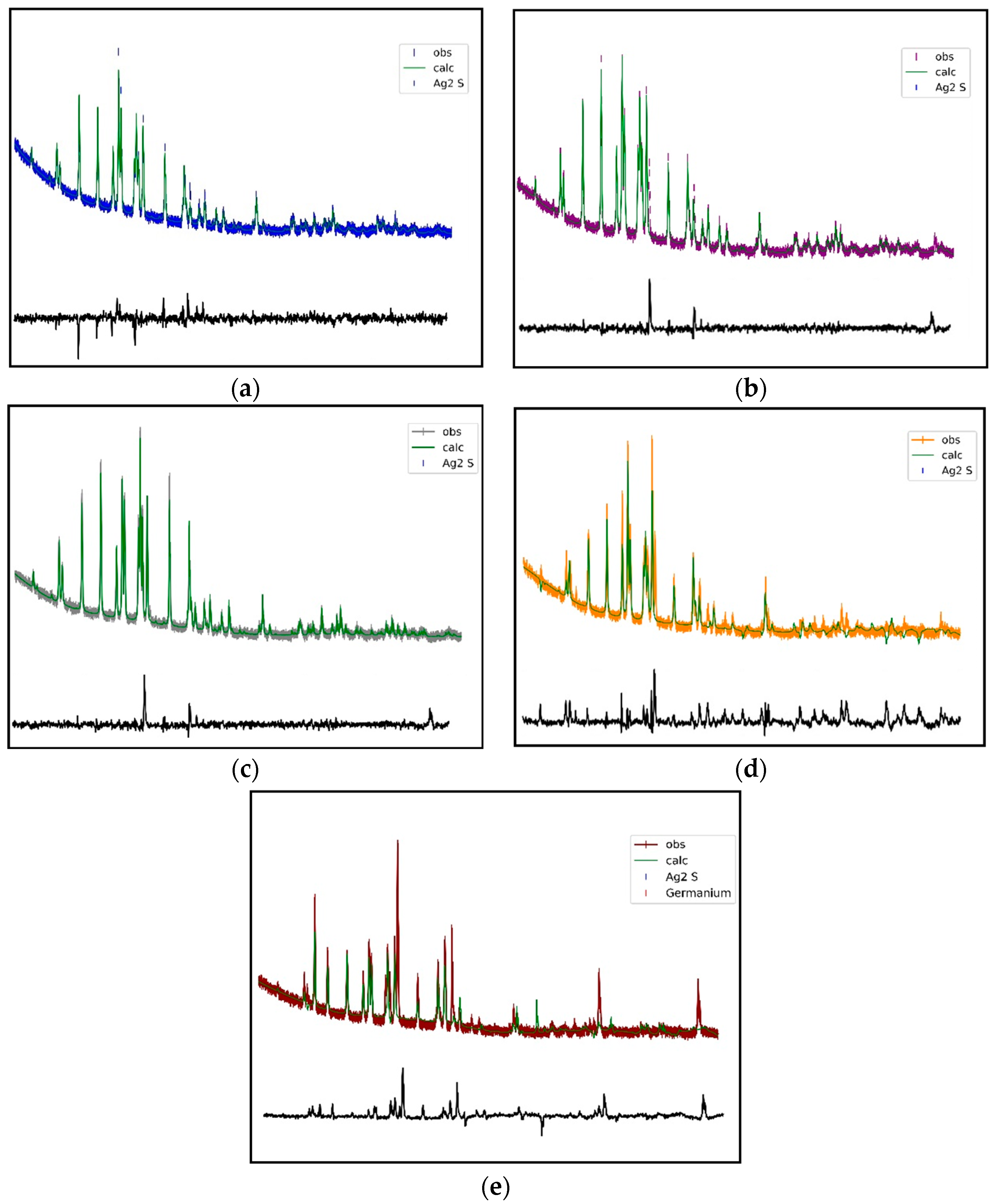
2.2. X-ray Diffraction Analysis of the Microstructure
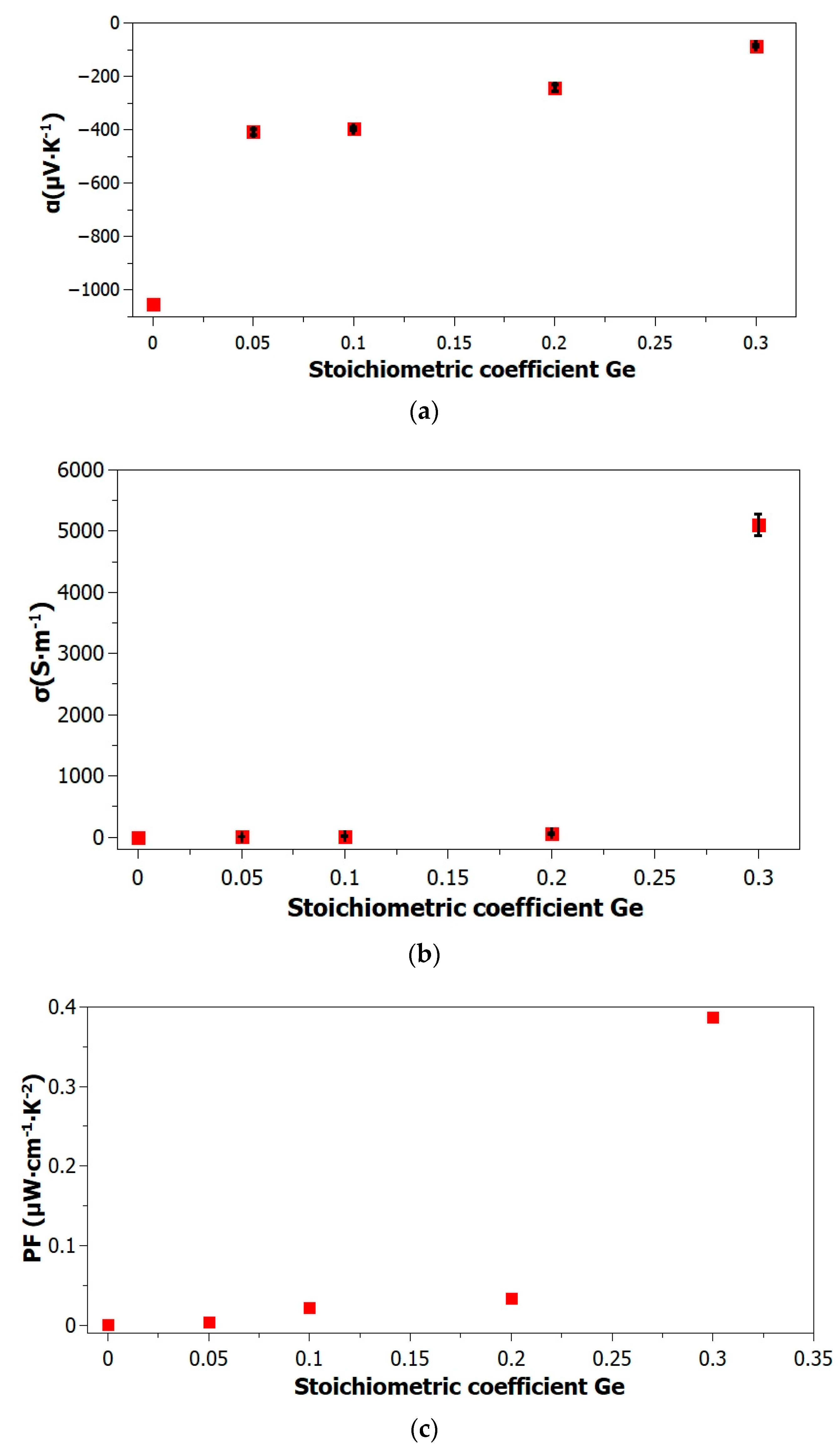
2.3. Thermoelectric Properties
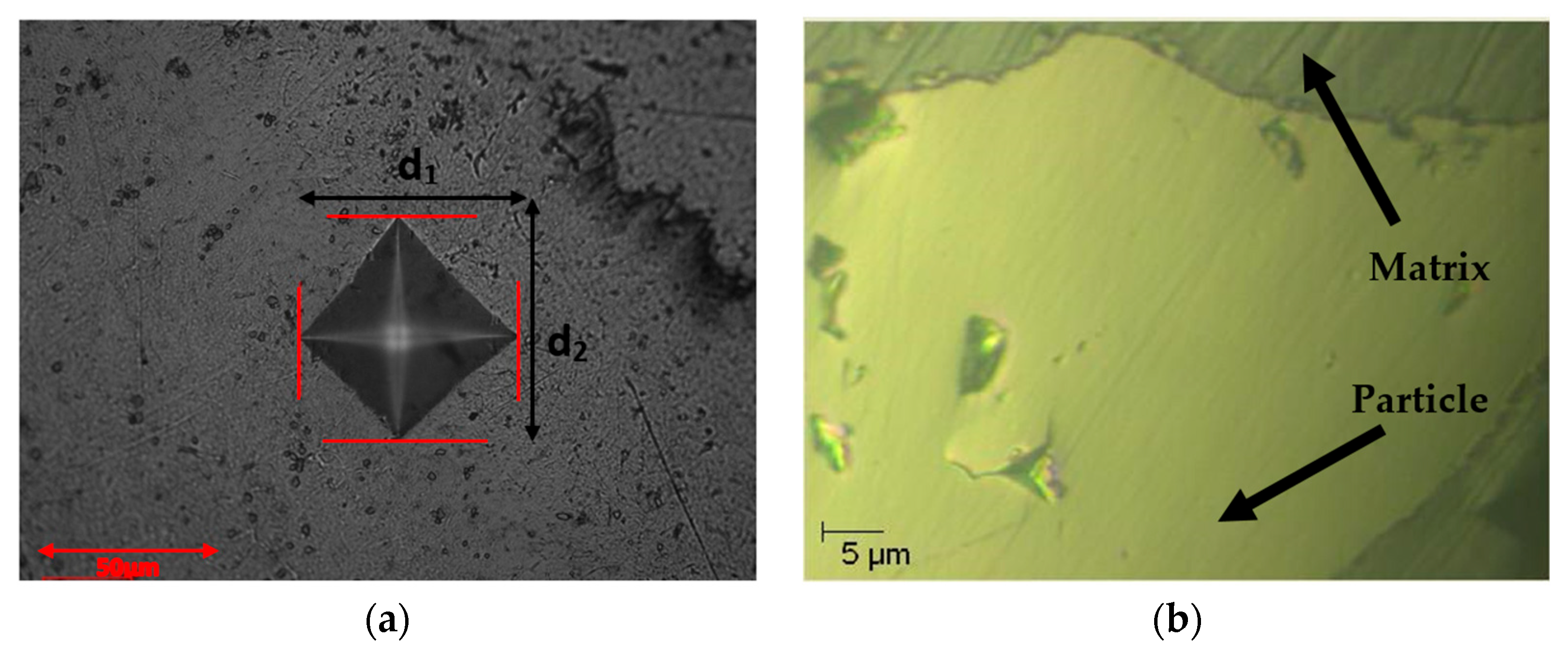
2.4. Mechanical Properties and Hardness
3. Discussion
4. Materials and Methods
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lundstrom, M.; Jeong, C. Near-Equilibrium Transport: Fundamentals and Applications; Lessons from Nanoscience; World Scientific: Singapore; Hackensack, NJ, USA, 2013; Volume 2. [Google Scholar]
- Goldsmid, H.J. Introduction to Thermoelectricity; Springer Series in Materials Science; Springer: Berlin/Heidelberg, Germany, 2016; Volume 121. [Google Scholar] [CrossRef]
- Goldsmid, H.J. The Physics of Thermoelectric Energy Conversion; IOP Publishing: Bristol, UK, 2017. [Google Scholar] [CrossRef]
- Wei, J.; Yang, L.; Ma, Z.; Song, P.; Zhang, M.; Ma, J.; Yang, F.; Wang, X. Review of current high-ZT thermoelectric materials. J. Mater. Sci. 2020, 55, 12642–12704. [Google Scholar] [CrossRef]
- Jayathilaka, W.A.D.M.; Qi, K.; Qin, Y.; Chinnappan, A.; Serrano-García, W.; Baskar, C.; Wang, H.; He, J.; Cui, S.; Thomas, S.W.; et al. Significance of Nanomaterials in Wearables: A Review on Wearable Actuators and Sensors. Adv. Mater. 2019, 31, 1805921. [Google Scholar] [CrossRef] [PubMed]
- He, R.; Schierning, G.; Nielsch, K. Thermoelectric Devices: A Review of Devices, Architectures, and Contact Optimization. Adv. Mater. Technol. 2018, 3, 1700256. [Google Scholar] [CrossRef]
- Yang, S.; Qiu, P.; Chen, L.; Shi, X. Recent Developments in Flexible Thermoelectric Devices. Small Sci. 2021, 1, 2100005. [Google Scholar] [CrossRef]
- Wolf, M.; Hinterding, R.; Feldhoff, A. High Power Factor vs. High zT—A Review of Thermoelectric Materials for High-Temperature Application. Entropy 2019, 21, 1058. [Google Scholar] [CrossRef]
- Sootsman, J.R.; Chung, D.Y.; Kanatzidis, M.G. New and Old Concepts in Thermoelectric Materials. Angew. Chem. Int. Ed. 2009, 48, 8616–8639. [Google Scholar] [CrossRef]
- Mitra, M.; Benton, A.; Akhanda, M.S.; Qi, J.; Zebarjadi, M.; Singh, D.J.; Poon, S.J. Conventional Half-Heusler alloys advance state-of-the-art thermoelectric properties. Mater. Today Phys. 2022, 28, 100900. [Google Scholar] [CrossRef]
- Tian, Z.; Lee, S.; Chen, G. Comprehensive review of heat transfer in thermoelectric materials and devices. Annu. Rev. Heat Transf. 2014, 17, 425–483. [Google Scholar] [CrossRef]
- Riffat, S.B.; Ma, X. Thermoelectrics: A review of present and potential applications. Appl. Therm. Eng. 2003, 23, 913–935. [Google Scholar] [CrossRef]
- Chen, L.; Liu, R.; Shi, X. General principles of thermoelectric technology. In Thermoelectric Materials and Devices; Elsevier: Amsterdam, The Netherlands, 2021; pp. 1–18. [Google Scholar] [CrossRef]
- Dahal, T.; Jie, Q.; Joshi, G.; Chen, S.; Guo, C.; Lan, Y.; Ren, Z. Thermoelectric property enhancement in Yb-doped n-type skutterudites YbxCo4Sb12. Acta Mater. 2014, 75, 316–321. [Google Scholar] [CrossRef]
- Bell, L.E. Cooling, Heating, Generating Power, and Recovering Waste Heat with Thermoelectric Systems. Science 2008, 321, 1457–1461. [Google Scholar] [CrossRef] [PubMed]
- Zuhud, A.M.; Mochammad, F.; Widayat, W. Thermoelectric application in energy conservation. E3S Web Conf. 2018, 73, 01009. [Google Scholar] [CrossRef]
- Liu, Z.; Tian, B.; Li, Y.; Guo, Z.; Zhang, Z.; Luo, Z.; Zhao, L.; Lin, Q.; Lee, C.; Jiang, Z. Evolution of Thermoelectric Generators: From Application to Hybridization. Small 2023, 19, 2304599. [Google Scholar] [CrossRef]
- Lv, H.; Liang, L.; Zhang, Y.; Deng, L.; Chen, Z.; Liu, Z.; Wang, H.; Chen, G. A flexible spring-shaped architecture with optimized thermal design for wearable thermoelectric energy harvesting. Nano Energy 2021, 88, 106260. [Google Scholar] [CrossRef]
- Luan, W. Recent developments of thermoelectric power generation. Chin. Sci. Bull. 2004, 49, 1212. [Google Scholar] [CrossRef]
- Demirbas, A. Global Renewable Energy Projections. Energy Sources Part B Econ. Plan. Policy 2009, 4, 212–224. [Google Scholar] [CrossRef]
- Polozine, A.; Sirotinskaya, S.; Schaeffer, L. History of development of thermoelectric materials for electric power generation and criteria of their quality. Mater. Res. 2014, 17, 1260–1267. [Google Scholar] [CrossRef]
- Cao, T.; Shi, X.-L.; Chen, Z.-G. Advances in the design and assembly of flexible thermoelectric device. Prog. Mater. Sci. 2023, 131, 101003. [Google Scholar] [CrossRef]
- Dhass, A.D.; Babu, L.G.; Pradhan, R.; Murthy, G.V.K.; Sreenivasan, M. Energy Harvesting Through Thermoelectric Generators. In Materials and Technologies for a Green Environment; Harikrishnan, S., Ed.; Bentham Science Publishers: Soest, The Netherlands, 2023; pp. 32–66. [Google Scholar] [CrossRef]
- Zhou, W.-X.; Wu, D.; Xie, G.; Chen, K.-Q.; Zhang, G. α-Ag2S: A Ductile Thermoelectric Material with High ZT. ACS Omega 2020, 5, 5796–5804. [Google Scholar] [CrossRef]
- Zhu, M.; Shi, X.-L.; Wu, H.; Liu, Q.; Chen, Z.-G. Advances in Ag2S-based thermoelectrics for wearable electronics: Progress and perspective. Chem. Eng. J. 2023, 473, 145236. [Google Scholar] [CrossRef]
- Wei, T.; Qiu, P.; Zhao, K.; Shi, X.; Chen, L. Ag2Q-Based (Q = S, Se, Te) Silver Chalcogenide Thermoelectric Materials. Adv. Mater. 2023, 35, 2110236. [Google Scholar] [CrossRef] [PubMed]
- Shi, X.; Chen, H.; Hao, F.; Liu, R.; Wang, T.; Qiu, P.; Burkhardt, U.; Grin, Y.; Chen, L. Room-temperature ductile inorganic semiconductor. Nat. Mater. 2018, 17, 421–426. [Google Scholar] [CrossRef] [PubMed]
- Živković, D.; Sokić, M.; Živković, Ž.; Manasijević, D.; Balanović, L.; Štrbac, N.; Ćosović, V.; Boyanov, B. Thermal study and mechanism of Ag2S oxidation in air. J. Therm. Anal. Calorim. 2013, 111, 1173–1176. [Google Scholar] [CrossRef]
- Gusev, A.I.; Sadovnikov, S.I. Acanthite–argentite transformation in nanocrystalline silver sulfide and the Ag2S/Ag nanoheterostructure. Semiconductors 2016, 50, 682–687. [Google Scholar] [CrossRef]
- Liang, J.; Wang, T.; Qiu, P.; Yang, S.; Ming, C.; Chen, H.; Song, Q.; Zhao, K.; Wei, T.R.; Ren, D.; et al. Flexible thermoelectrics: From silver chalcogenides to full-inorganic devices. Energy Environ. Sci. 2019, 12, 2983–2990. [Google Scholar] [CrossRef]
- Li, L.; Peng, C.; Chen, J.; Ma, Z.; Chen, Y.; Li, S.; Wang, J.; Wang, C. Study the effect of alloying on the phase transition behavior and thermoelectric properties of Ag2S. J. Alloys Compd. 2021, 886, 161241. [Google Scholar] [CrossRef]
- Pal’yanova, G.A.; Chudnenko, K.V.; Zhuravkova, T.V. Thermodynamic properties of solid solutions in the system Ag2S–Ag2Se. Thermochim. Acta 2014, 575, 90–96. [Google Scholar] [CrossRef]
- Wang, H.; Ma, H.; Duan, B.; Geng, H.; Zhou, L.; Li, J.; Zhang, X.; Yang, H.; Li, G.; Zhai, P. High-Pressure Rapid Preparation of High-Performance Binary Silver Sulfide Thermoelectric Materials. ACS Appl. Energy Mater. 2021, 4, 1610–1618. [Google Scholar] [CrossRef]
- Tarachand; Mukherjee, B.; Saxena, M.; Kuo, Y.K.; Okram, G.S.; Dam, S.; Hussain, S.; Lakhani, A.; Deshpande, U.; Shripathi, T. Ag-Nanoinclusion-Induced Enhanced Thermoelectric Properties of Ag2S. ACS Appl. Energy Mater. 2019, 2, 6383–6394. [Google Scholar] [CrossRef]
- Wakhare, S.Y.; Deshpande, M.D. Structural, electronic and optical properties of metalloid element (B, Si, Ge, As, Sb, and Te) doped g-ZnO monolayer: A DFT study. J. Mol. Graph. Model. 2020, 101, 107753. [Google Scholar] [CrossRef]
- Chen, H.; Shao, C.; Huang, S.; Gao, Z.; Huang, H.; Pan, Z.; Zhao, K.; Qiu, P.; Wei, T.R.; Shi, X. High-Entropy Cubic Pseudo-Ternary Ag2 (S, Se, Te) Materials With Excellent Ductility and Thermoelectric Performance. Adv. Energy Mater. 2023, 14, 2303473. [Google Scholar] [CrossRef]
- Singh, J.; Verma, S.S. Global Journal of Researches in Engineering Electrical and Electronics Engineering. Comp. Fig. Merit Some Common Thermocouples High Temp. Range 2013, 11, 1–7. [Google Scholar]
- Chen, L.; Liu, R.; Shi, X. Strategies to optimize thermoelectric performance. In Thermoelectric Materials and Devices; Elsevier: Amsterdam, The Netherlands, 2021; pp. 19–50. [Google Scholar] [CrossRef]
- Giri, K.; Wang, Y.-L.; Chen, T.-H.; Chen, C.-H. Challenges and strategies to optimize the figure of merit: Keeping eyes on thermoelectric metamaterials. Mater. Sci. Semicond. Process. 2022, 150, 106944. [Google Scholar] [CrossRef]
- Tripathi, M.N.; Bhandari, C.M. Material parameters for thermoelectric performance. Pramana J. Phys. 2005, 65, 469–479. [Google Scholar] [CrossRef]
- Dehkordi, A.M.; Zebarjadi, M.; He, J.; Tritt, T.M. Thermoelectric power factor: Enhancement mechanisms and strategies for higher performance thermoelectric materials. Mater. Sci. Eng. R Rep. 2015, 97, 1–22. [Google Scholar] [CrossRef]
- Guttmann, G.M.; Gelbstein, Y. Mechanical Properties of Thermoelectric Materials for Practical Applications. In Bringing Thermoelectricity into Reality; Aranguren, P., Ed.; InTech: London, UK, 2018. [Google Scholar] [CrossRef]
- Toby, B.H.; Von Dreele, R.B. GSAS-II: The genesis of a modern open-source all purpose crystallography software package. J. Appl. Crystallogr. 2013, 46, 544–549. [Google Scholar] [CrossRef]
- Gražulis, S.; Daškevič, A.; Merkys, A.; Chateigner, D.; Lutterotti, L.; Quiros, M.; Serebryanaya, N.R.; Moeck, P.; Downs, R.T.; Le Bail, A. Crystallography Open Database (COD): An open-access collection of crystal structures and platform for world-wide collaboration. Nucleic Acids Res. 2012, 40, D420–D427. [Google Scholar] [CrossRef] [PubMed]
- Chen, H.; Wei, T.R.; Zhao, K.; Qiu, P.; Chen, L.; He, J.; Shi, X. Room-temperature plastic inorganic semiconductors for flexible and deformable electronics. InfoMat 2021, 3, 22–35. [Google Scholar] [CrossRef]
- Gayner, C.; Kar, K.K. Recent advances in thermoelectric materials. Prog. Mater. Sci. 2016, 83, 330–382. [Google Scholar] [CrossRef]
- Qian, L.; Li, M.; Zhou, Z.; Yang, H.; Shi, X. Comparison of nano-indentation hardness to microhardness. Surf. Coat. Technol. 2005, 195, 264–271. [Google Scholar] [CrossRef]
- Oliver, W.C.; Pharr, G.M. An improved technique for determining hardness and elastic modulus using load and displacement sensing indentation experiments. J. Mater. Res. 1992, 7, 1564–1583. [Google Scholar] [CrossRef]
- E33 Committee. Classification for Determination of Single-Number Metrics for Impact Noise; ASTM International: West Conshohocken, PA, USA, 2021. [Google Scholar] [CrossRef]
- E28 Committee. Test Methods of Compression Testing of Metallic Materials at Room Temperature; ASTM International: West Conshohocken, PA, USA, 2022. [Google Scholar] [CrossRef]


| Expected Composition | Ag2S | Ag2Ge0.05S0.95 | Ag2Ge0.1S0.9 | Ag2Ge0.2S0.8 | Ag2Ge0.3S0.7 | |
|---|---|---|---|---|---|---|
| Measured | Ag (at.%) | 2.06 | 2.05 | 1.95 | 1.83 | 1.85 |
| Ge (at.%) | 0 | 0.072 | 0.23 | 0.42 | 0.51 | |
| S (at.%) | 0.94 | 0.88 | 0.82 | 0.75 | 0.64 | |
| a [Å] | b [Å] | c [Å] | β [°] | Rw | |
|---|---|---|---|---|---|
| Ag2S | 4.228 ± 0.001 | 6.928 ± 0.001 | 7.864 ± 0.002 | 99.649 ± 0.004 | 4.57 |
| Ag2Ge0.05S0.95 | 4.225 ± 0.001 | 6.926 ± 0.001 | 7.862 ± 0.002 | 99.662 ± 0.004 | 5.17 |
| Ag2Ge0.1S0.9 | 4.228 ± 0.0002 | 6.929 ± 0.0002 | 7.865 ± 0.001 | 99.652 ± 0.002 | 3.44 |
| Ag2Ge0.2S0.8 | 4.230 ± 0.001 | 6.931 ± 0.001 | 7.867 ± 0.003 | 99.656 ± 0.007 | 5.12 |
| Ag2Ge0.3S0.7 | 4.224 ± 0.002 | 6.924 ± 0.002 | 7.852 ± 0.005 | 99.692 ± 0.013 | 13.09 |
| Ag2S | Ag2Ge0.05S0.95 | Ag2Ge0.1S0.9 | Ag2Ge0.2S0.8 | Ag2Ge0.3S0.7 | |
|---|---|---|---|---|---|
| σ [S·m−1] | 0.102 | 2.3 ± 0.6 | 14.2 ± 6 | 58.2 ± 16.5 | 5106.2 ± 172 |
| α [µV·K−1] | −1051 | −408 ± 10 | −397 ± 5 | −243 ± 12 | −87 ± 4 |
| PF [μW·cm−1·K−2] | 0.0011 | 0.0039 | 0.0224 | 0.0343 | 0.3873 |
| Material | TC [°C] | ZT | PF [μW·cm−1·K−2] |
|---|---|---|---|
| Ag2S | 177 | 6.3 × 10−5 | 0.0011 |
| Ag2S0.9Se0.1 | 147 | 7.6 × 10−4 | 0.013 |
| Ag2S0.7Se0.3 | 109 | 0.075 | 1.08 |
| Ag2S0.5Se0.5 | 82 | 0.26 | 4.84 |
| Ag2S0.4Se0.6 | 350 | 1.08 | 21.45 |
| Ag2S0.8Te0.2 | - | 0.25 | 5.3 |
| Ag2S0.5Se0.45Te0.05 | 57 | 0.44 | 4.96 |
| Ag2S | Ag2Ge0.05S0.95 | Ag2Ge0.1S0.9 | Ag2Ge0.2S0.8 | Ag2Ge0.3S0.7 | |
|---|---|---|---|---|---|
| ρ [g·cm−3] | 7.22 | 7.24 | 7.26 | 7.39 | 7.35 |
| Rp0,2 [MPa] | 122 | 84 | 73 | 91 | 71 |
| HV 0.1 | 36 | 32 | 26 | 26 | 34 |
| HIT [GPa] | 0.5 ± 0.04 | 0.8 ± 0.02 | 0.4 ± 0.05 | 0.4 ± 0.01 | 0.9 ± 0.02 |
| EIT [GPa] | 24 ± 1 | 30 ± 1 | 22 ± 2 | 18 ± 2 | 47 ± 6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hrickova, G.; Mihok, F.; Molcanova, Z.; Ballokova, B.; Mamrilla, W.; Dzunda, R.; Lukacs, P.; Pietrikova, A.; Saksl, K. The Effect of Ge Doping on α-Ag2S’s Thermoelectric and Mechanical Properties. Inorganics 2024, 12, 98. https://doi.org/10.3390/inorganics12040098
Hrickova G, Mihok F, Molcanova Z, Ballokova B, Mamrilla W, Dzunda R, Lukacs P, Pietrikova A, Saksl K. The Effect of Ge Doping on α-Ag2S’s Thermoelectric and Mechanical Properties. Inorganics. 2024; 12(4):98. https://doi.org/10.3390/inorganics12040098
Chicago/Turabian StyleHrickova, Gabriela, Frantisek Mihok, Zuzana Molcanova, Beata Ballokova, Wanda Mamrilla, Robert Dzunda, Peter Lukacs, Alena Pietrikova, and Karel Saksl. 2024. "The Effect of Ge Doping on α-Ag2S’s Thermoelectric and Mechanical Properties" Inorganics 12, no. 4: 98. https://doi.org/10.3390/inorganics12040098
APA StyleHrickova, G., Mihok, F., Molcanova, Z., Ballokova, B., Mamrilla, W., Dzunda, R., Lukacs, P., Pietrikova, A., & Saksl, K. (2024). The Effect of Ge Doping on α-Ag2S’s Thermoelectric and Mechanical Properties. Inorganics, 12(4), 98. https://doi.org/10.3390/inorganics12040098







