URVA and Local Mode Analysis of an Iridium Pincer Complex Efficiently Catalyzing the Hydrogenation of Carbon Dioxide
Abstract
1. Introduction
2. Materials and Methods
3. Results and Discussion
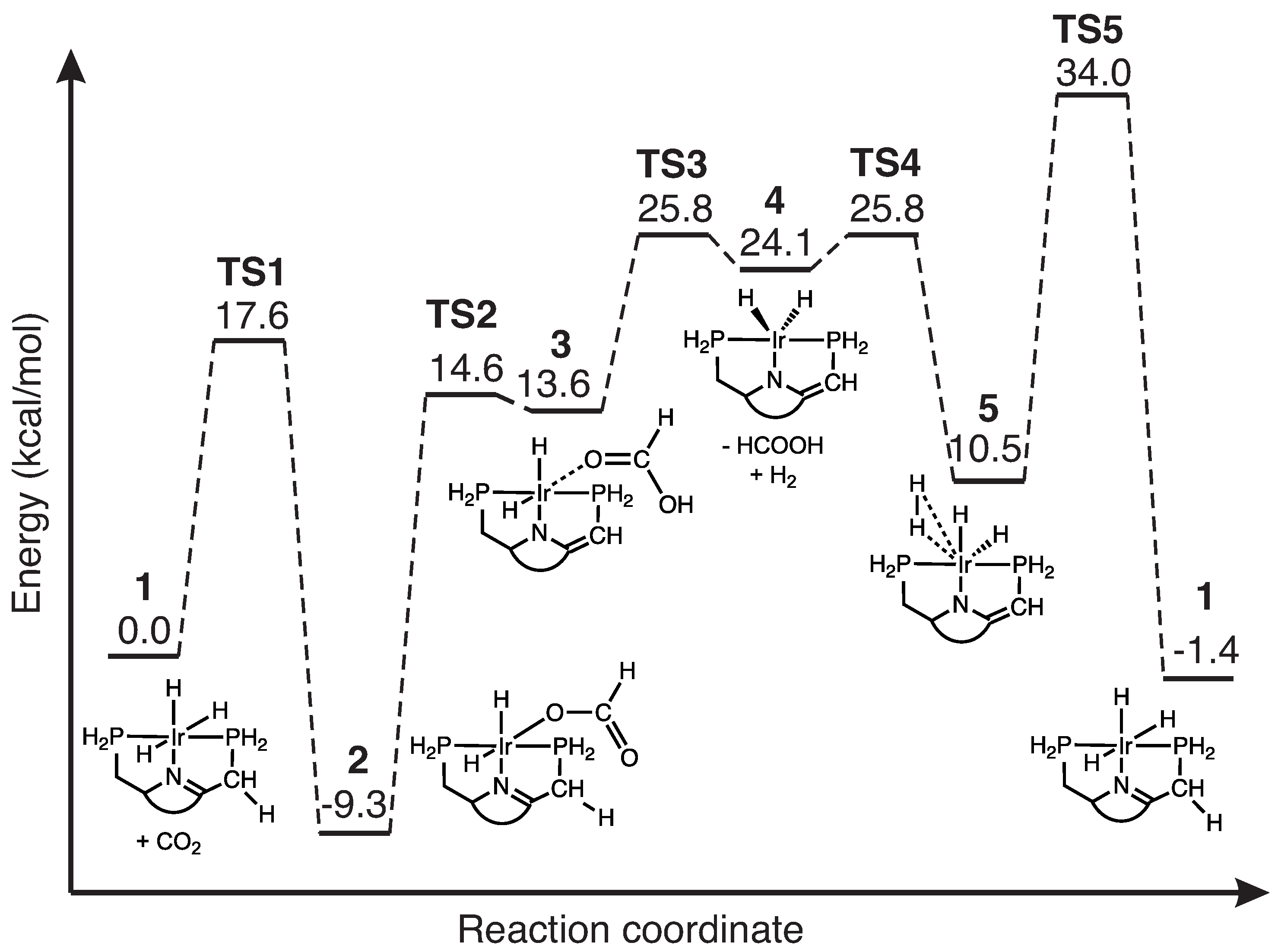
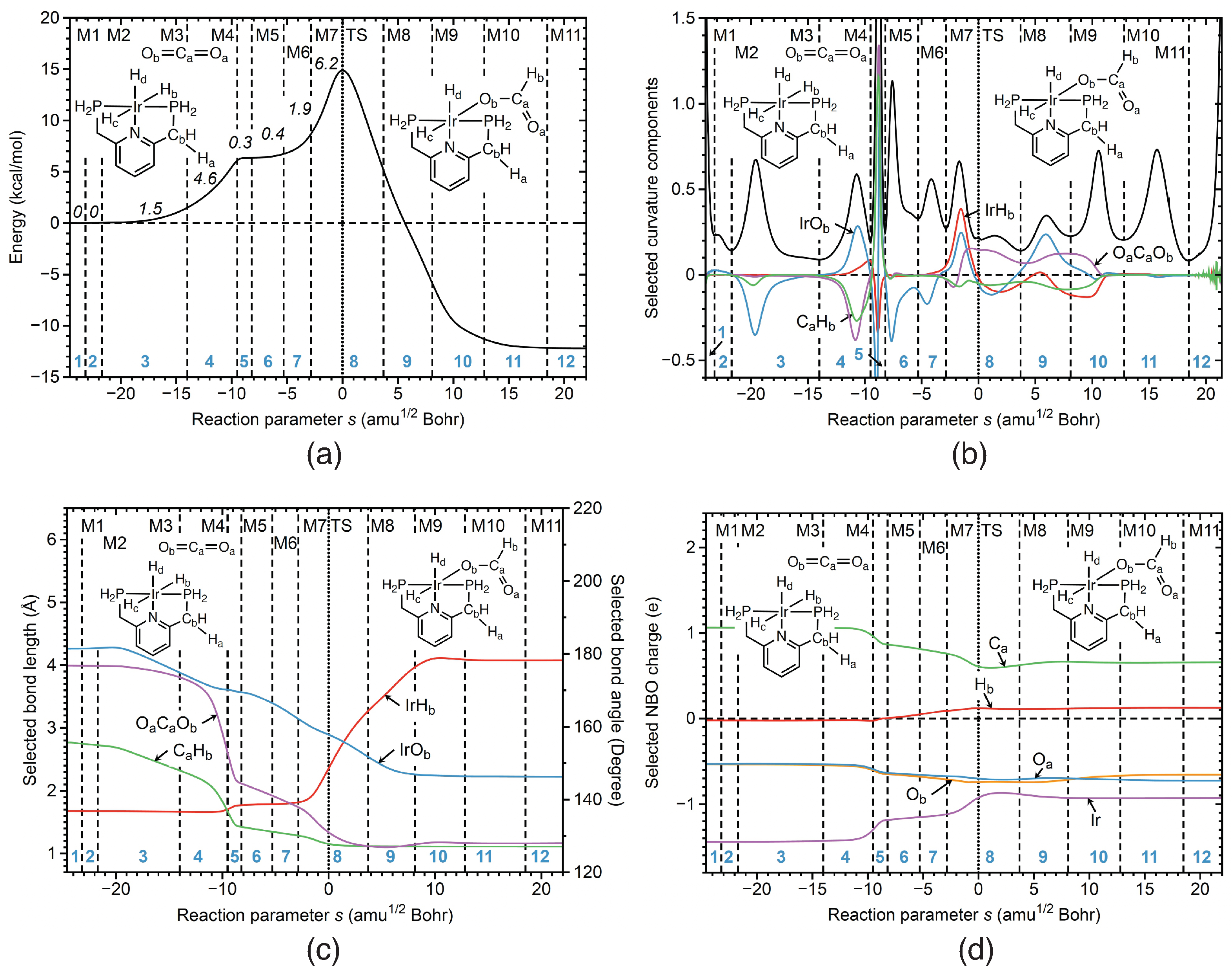
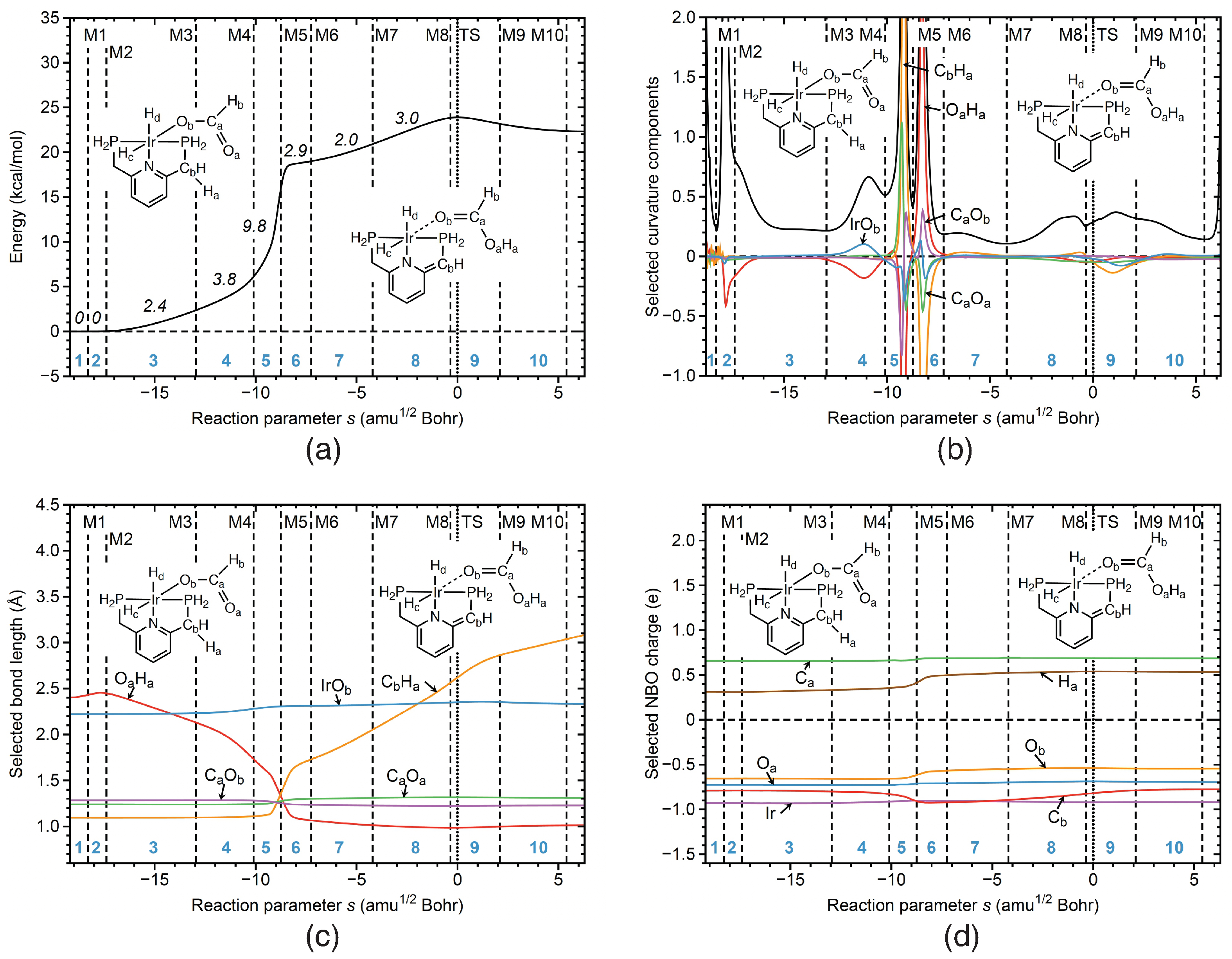
3.1. Energetics
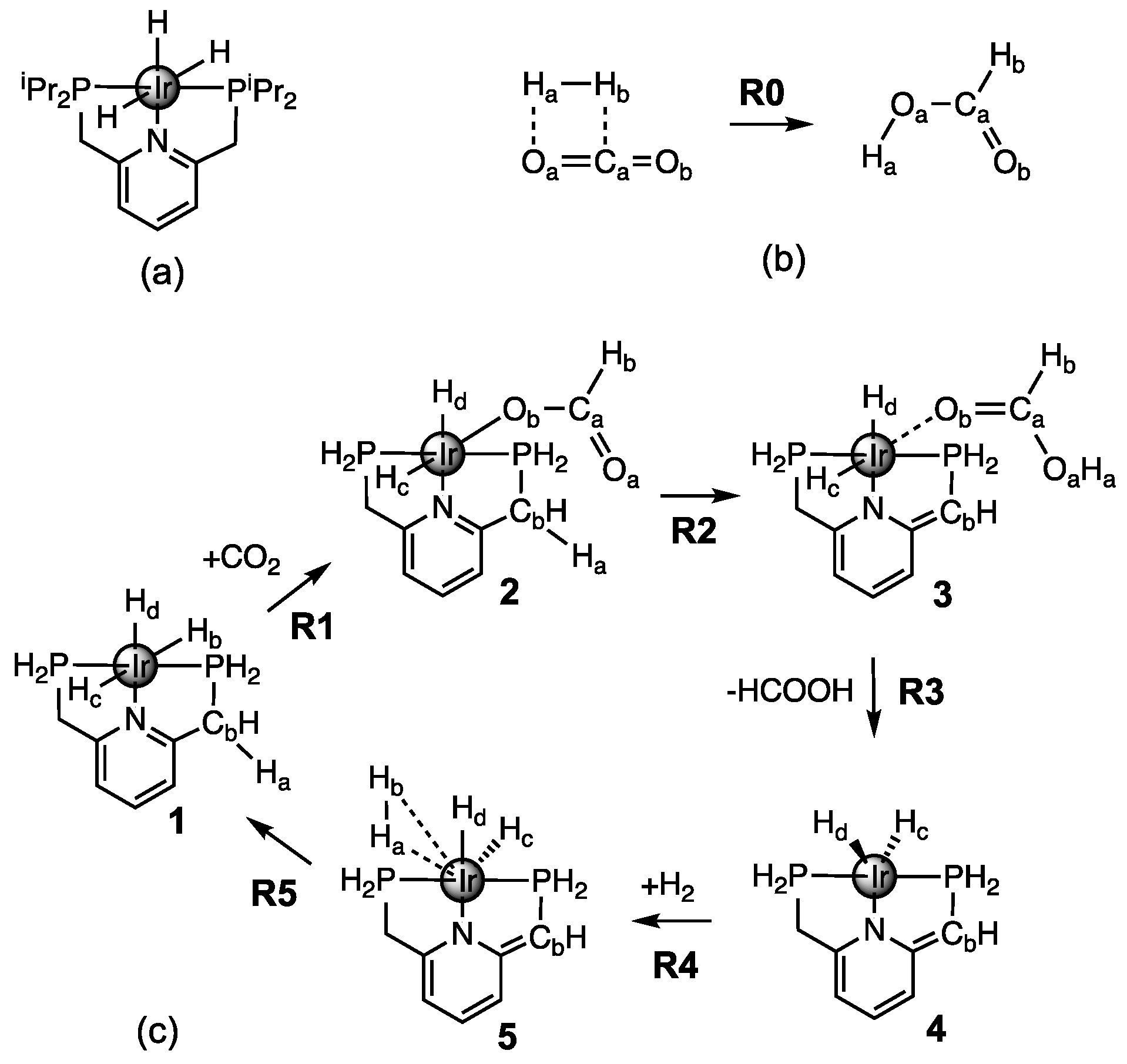
3.2. Reaction Mechanism
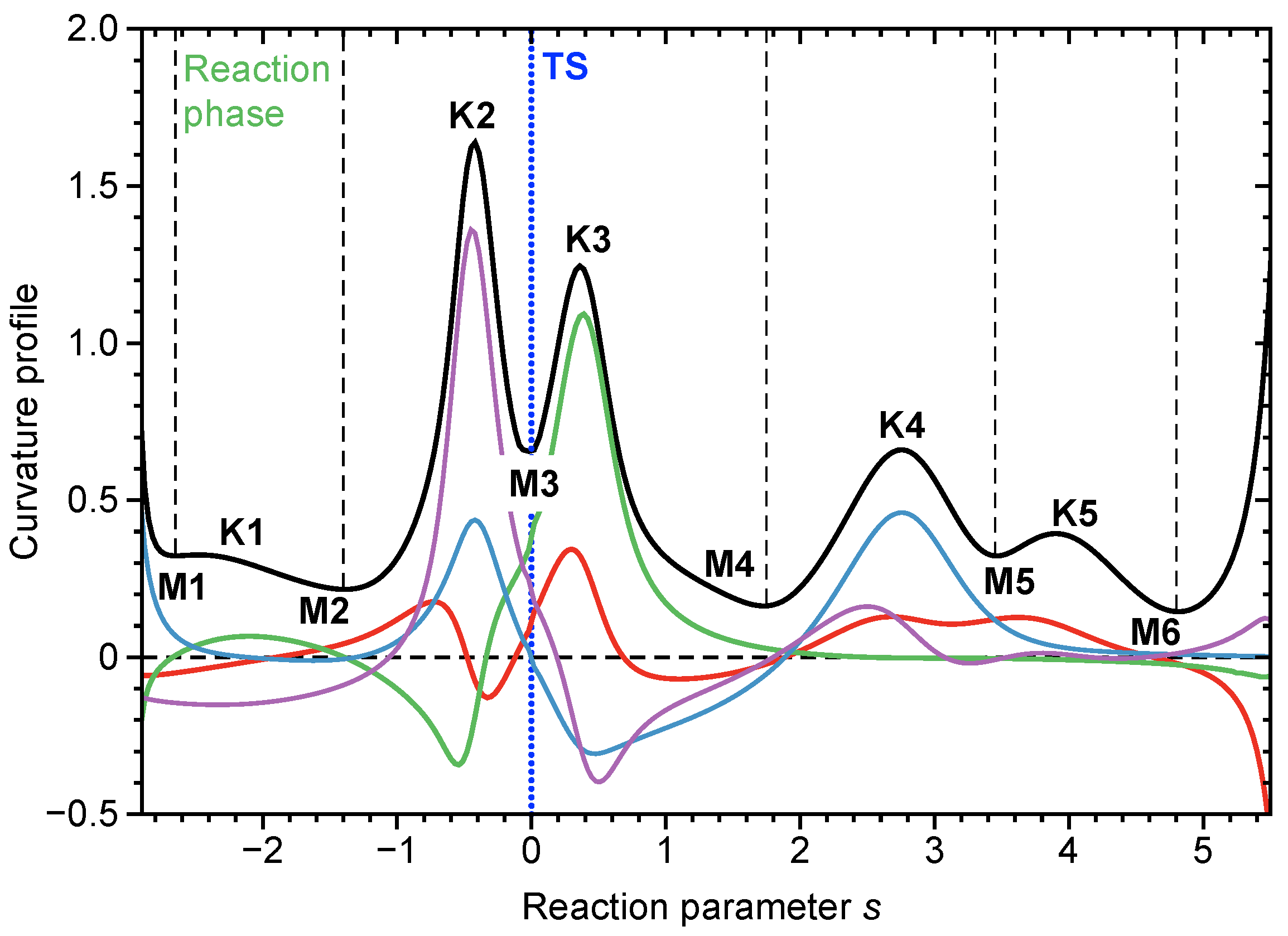
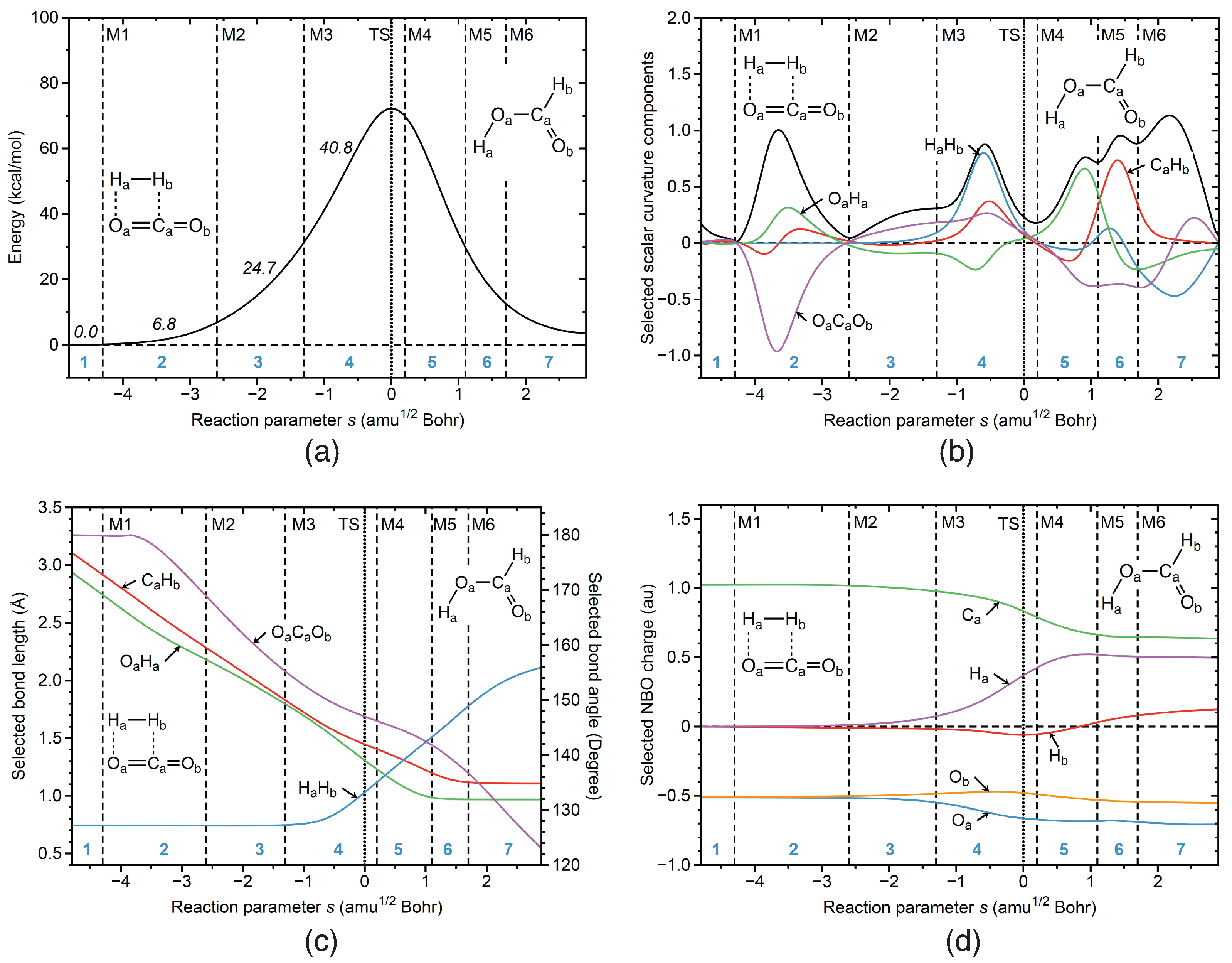
3.3. Chemical Bond Analysis
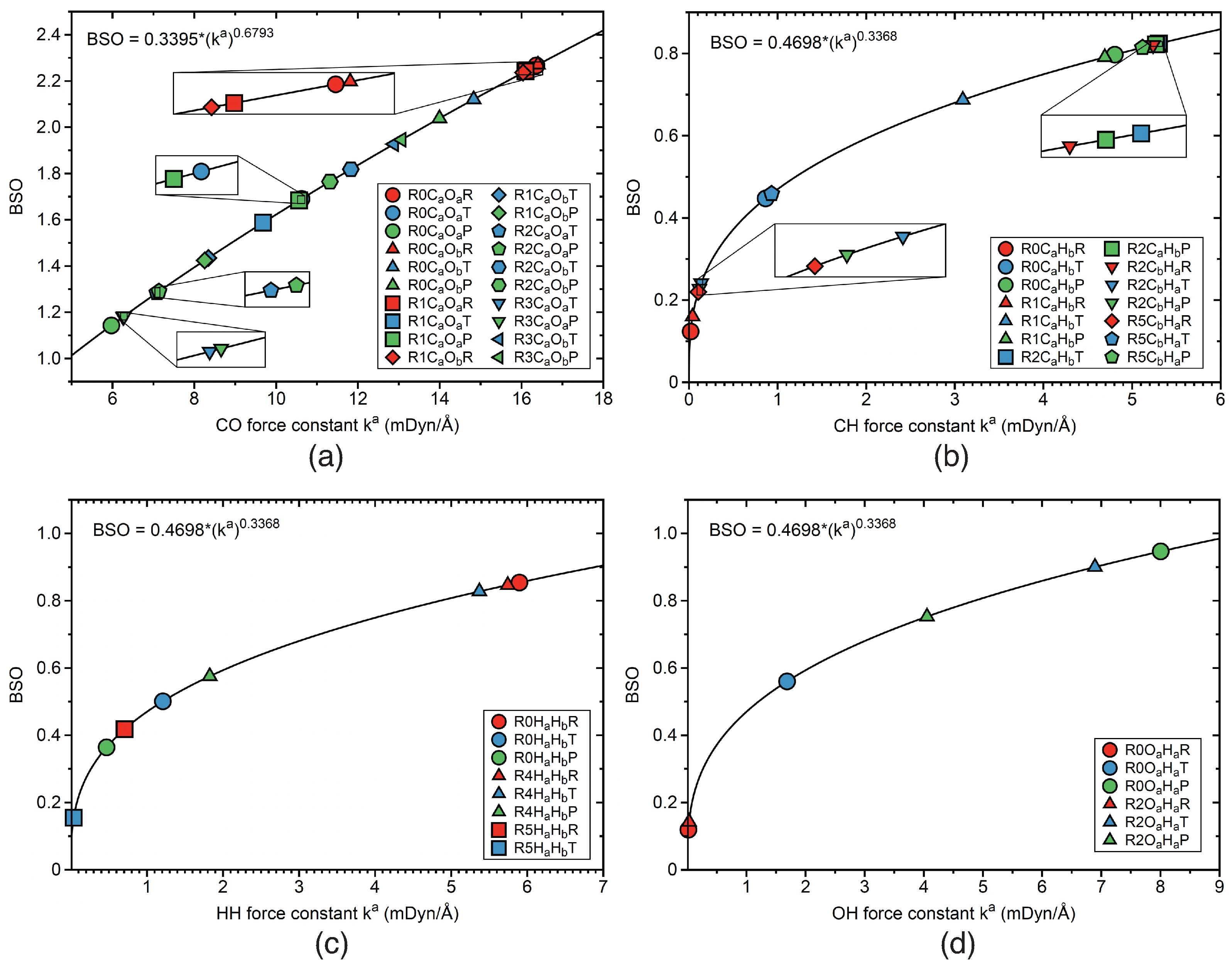
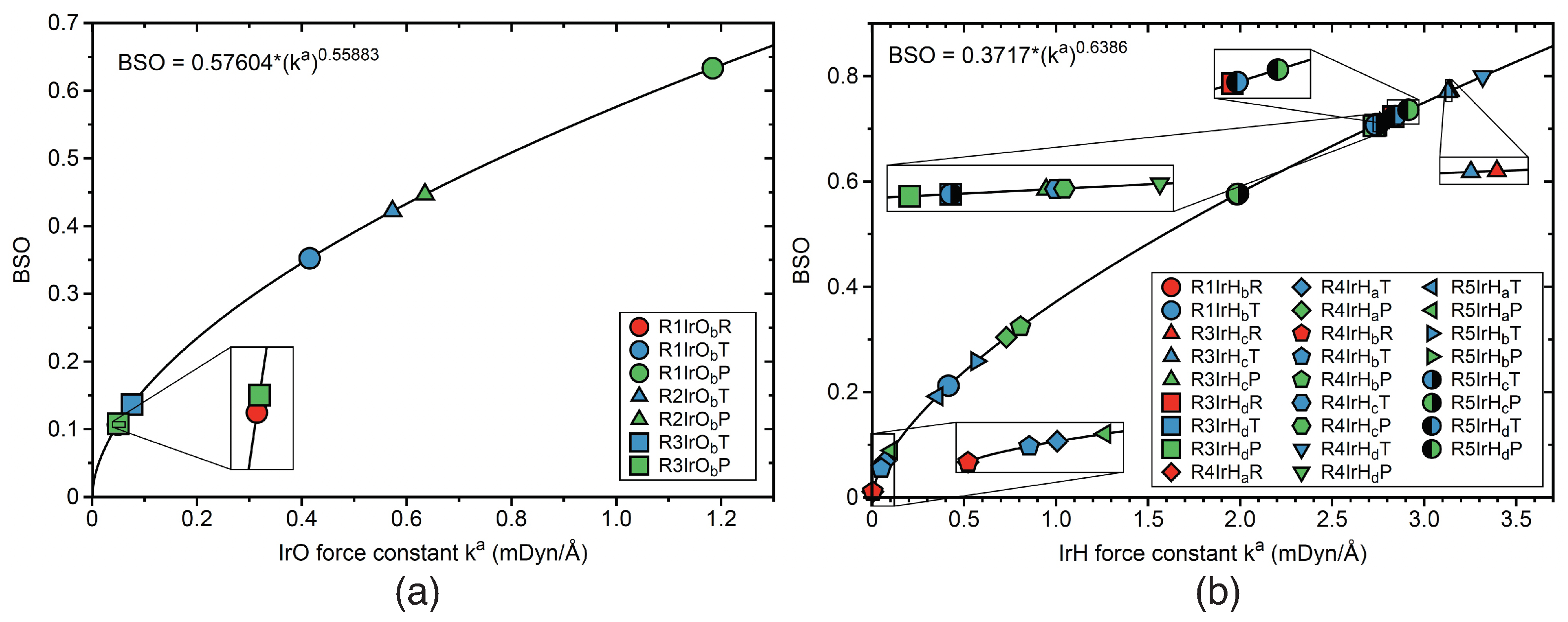
3.4. Comparison of Relative Bond Strength Orders BSO
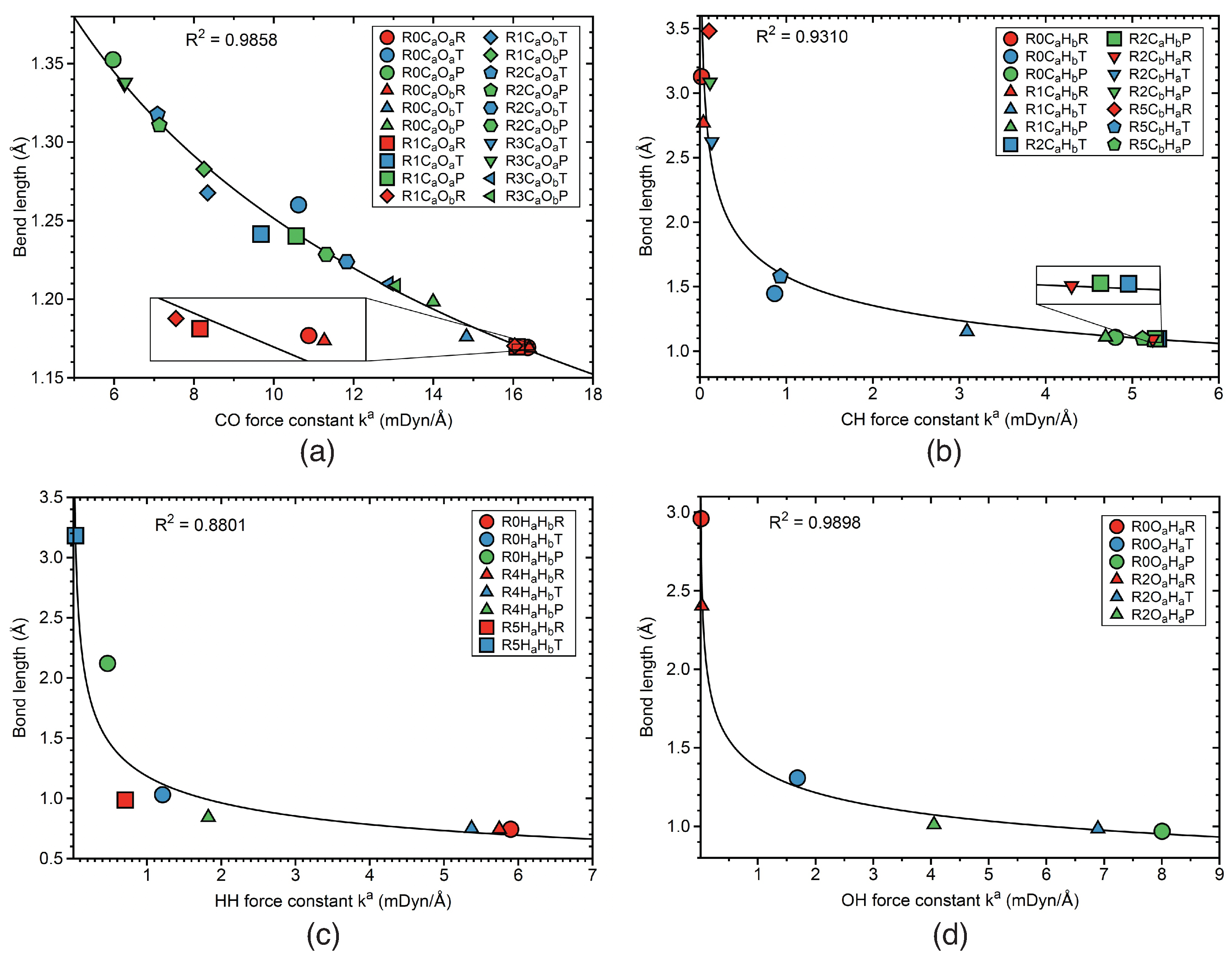
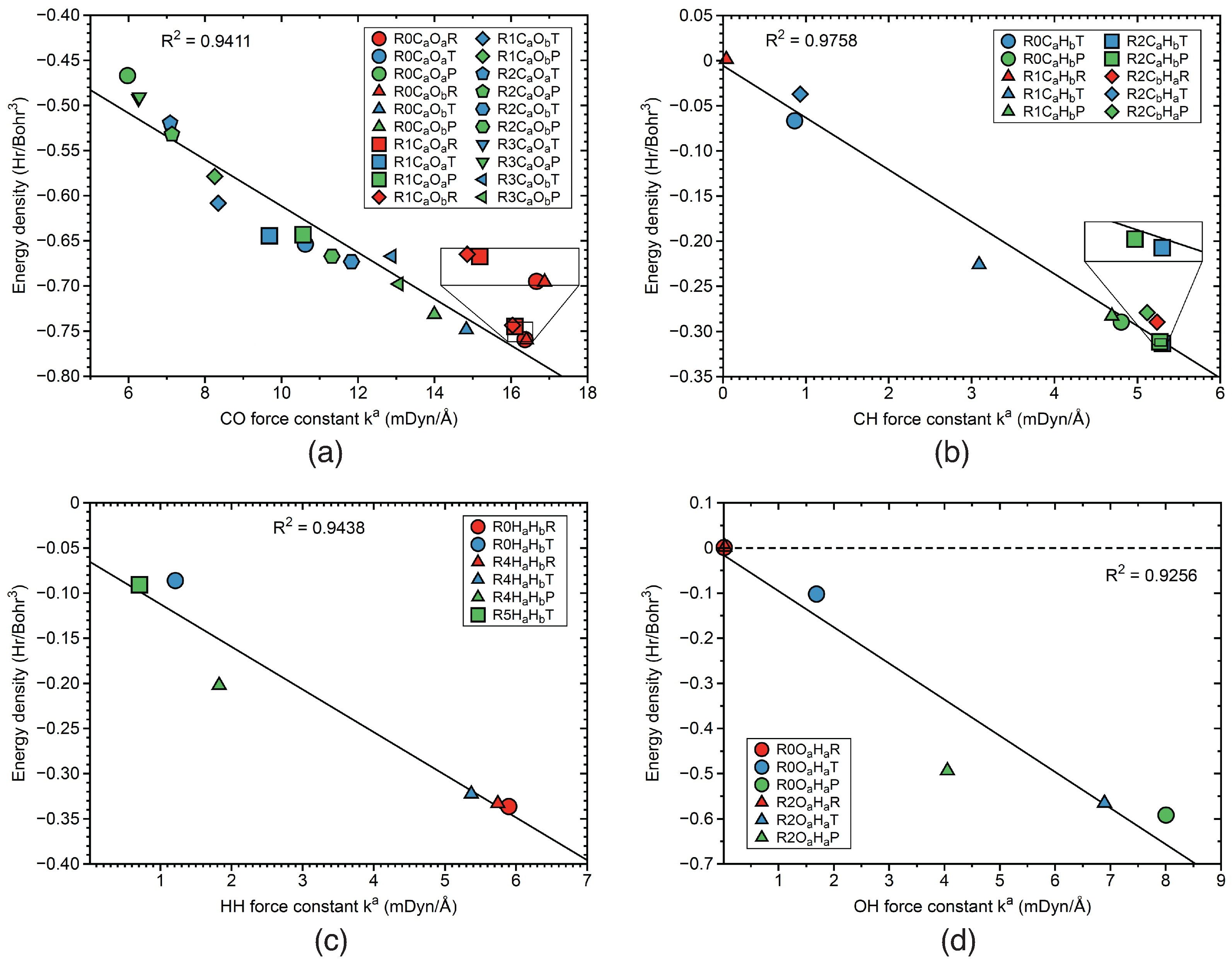
3.5. Correlations between Bond Properties and Local Mode Force Constants
4. Conclusions and Outlook
- According to the URVA analysis, the most energy consuming process of the non-catalyzed reaction R0 is the cleavage of the HH bond of the H reactant, which takes place before TS, and as such contributes to the activation energy with 40.8 kcal/mol.
- In order to avoid direct HH bond cleavage, the catalyst divides the one–step non-catalytic reaction into a multistep catalytic cycle; CO addition to the catalyst, H atom transfer between catalyst and CO ligand, release of HCOOH product, addition of H, reorganization of the coordination sphere of the catalyst to achieve its original form. Each of these decisive steps could be clearly identified in the characteristic curvature profiles for the first time.
- The expensive cleavage of the HH bond in the non-catalyzed reaction, is replaced in the catalyzed reaction by H transfer (reaction R2) requiring the cleavage of an IrH bond with a significantly smaller contribution of 9.8 kcal/mol to the activation energy, which is revealed by both energy and curvature profiles.
- The dissociation of the final product from the catalyst (reaction R3) is characterized by the cleavage of an IrO bond and an intermediate NH hydrogen bond, and according to our URVA analysis, both contribute to the activation energy with a moderate amount of 12.3 kcal/mol.
- As unravelled by the URVA curvature profiles most of the events related to the reorganization of the catalyst to restore its original form (reaction R5) occur after the TS, i.e., they do not contribute to the activation energy.
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| URVA | unified reaction valley approach |
| DFT | density functional theory |
| DLPNO-CCSD(T) | domain–based local pair natural orbital of coupled cluster single and double with perturbative triple excitations |
| PNP | 2,6–bis(di–isopropylphosphinomethyl)pyridine |
| TOF | turnover frequency |
| TON | turnover number |
| LMA | Local Modes Analysis |
| PES | potential energy surface |
| RC | reaction complex |
| QTAIM | quantum theory of atoms-in-molecules |
| IRC | intrinsic reaction coordinate |
| NBO | natural bond orbital |
| BSO | bond strength order |
| Hr | hartree |
| RE | reactant |
| TS | transition state |
| PR | product |
References
- Zhu, S.; Tang, Y.; Qiao, X.; You, W.; Peng, C. Spatial Effects of Participation in Global Value Chains on CO2 Emissions: A Global Spillover Perspective. Emerg. Mark. Financ. Trade 2022, 58, 776–789. [Google Scholar] [CrossRef]
- García, J.; Galán, B. Integrating greenhouse gas capture and C1 biotechnology: A key challenge for circular economy. Microb. Biotechnol. 2022, 15, 228–239. [Google Scholar] [CrossRef]
- Lindsey, R. Climate Change: Atmospheric Carbon Dioxide. Climate Change Report. 2022. Available online: https://www.climate.gov/news-features/understanding-climate/climate-change-atmospheric-carbon-dioxide (accessed on 27 November 2022).
- Betts, R. Met Office: Atmospheric CO2 Now Hitting 50% Higher Than Pre-Industrial Levels. CarbonBrief. 2021. Available online: https://www.carbonbrief.org/met-office-atmospheric-co2-now-hitting-50-higher-than-pre-industrial-levels (accessed on 27 November 2022).
- Heffernan, J.K.; Valgepea, K.; de Souza Pinto Lemgruber, R.; Casini, I.; Plan, M.; Tappel, R.; Simpson, S.D.; Köpke, M.; Nielsen, L.K.; Marcellin, E. Enhancing CO2–Valorization Using Clostridium autoethanogenum for Sustainable Fuel and Chemicals Production. Front. Bioeng. Biotechnol. 2020, 8, 204. [Google Scholar] [CrossRef] [PubMed]
- Gabrielli, P.; Gazzani, M.; Mazzotti, M. The Role of Carbon Capture and Utilization, Carbon Capture and Storage, and Biomass to Enable a Net–Zero–CO2 Emissions Chemical Industry. Ind. Eng. Chem. Res. 2020, 59, 7033–7045. [Google Scholar] [CrossRef]
- Aresta, M. Carbon Dioxide as a Chemical Feedstock; John Wiley & Sons: Hoboken, NJ, USA, 2010. [Google Scholar]
- Sakakura, T.; Choi, J.C.; Yasuda, H. Transformation of Carbon Dioxide. Chem. Rev. 2007, 107, 2365–2387. [Google Scholar] [CrossRef]
- Wang, W.; Wang, S.; Ma, X.; Gong, J. Recent advances in catalytic hydrogenation of carbon dioxide. Chem. Soc. Rev. 2011, 40, 3703–3727. [Google Scholar] [CrossRef]
- Benson, E.E.; Kubiak, C.P.; Sathrum, A.J.; Smieja, J.M. Electrocatalytic and homogeneous approaches to conversion of CO2 to liquid fuels. Chem. Soc. Rev. 2009, 38, 89–99. [Google Scholar] [CrossRef]
- Riduan, S.N.; Zhang, Y. Recent developments in carbon dioxide utilization under mild conditions. Dalton Trans. 2010, 39, 3347–3357. [Google Scholar] [CrossRef]
- Liew, F.E.; Nogle, R.; Abdalla, T.; Rasor, B.J.; Canter, C.; Jensen, R.O.; Wang, L.; Strutz, J.; Chirania, P.; De Tissera, S.; et al. Carbon–negative production of acetone and isopropanol by gas fermentation at industrial pilot scale. Nat. Biotechnol. 2022, 40, 335–344. [Google Scholar] [CrossRef] [PubMed]
- Köpke, M.; Simpson, S.D. Pollution to products: Recycling of above ground carbon by gas fermentation. Curr. Opin. Biotechnol. 2020, 65, 180–189. [Google Scholar] [CrossRef]
- Kato, J.; Takemura, K.; Kato, S.; Fujii, T.; Wada, K.; Iwasaki, Y.; Aoi, Y.; Matsushika, A.; Murakami, K.; Nakashimada, Y. Metabolic engineering of Moorella thermoacetica for thermophilic bioconversion of gaseous substrates to a volatile chemical. AMB Express 2021, 11, 59. [Google Scholar] [CrossRef]
- Garrigues, L.; Maignien, L.; Lombard, E.; Singh, J.; Guillouet, S.E. Isopropanol production from carbon dioxide in Cupriavidus necator in a pressurized bioreactor. New Biotechnol. 2020, 56, 16–20. [Google Scholar] [CrossRef] [PubMed]
- Bontemps, S.; Vendier, L.; Sabo-Etienne, S. Ruthenium–Catalyzed Reduction of Carbon Dioxide to Formaldehyde. J. Am. Chem. Soc. 2014, 136, 4419–4425. [Google Scholar] [CrossRef] [PubMed]
- LeBlanc, F.A.; Piers, W.E.; Parvez, M. Selective Hydrosilation of CO2 to a Bis(silylacetal) Using an Anilido Bipyridyl–Ligated Organoscandium Catalyst. Angew. Chem. Int. Ed. 2014, 53, 789–792. [Google Scholar] [CrossRef] [PubMed]
- Graciani, J.; Mudiyanselage, K.; Xu, F.; Baber, A.E.; Evans, J.; Senanayake, S.D.; Stacchiola, D.J.; Liu, P.; Hrbek, J.; Sanz, J.F.; et al. Highly active copper–ceria and copper–ceria–titania catalysts for methanol synthesis from CO2. Science 2014, 345, 546–550. [Google Scholar] [CrossRef]
- Declercq, R.; Bouhadir, G.; Bourissou, D.; Légaré, M.A.; Courtemanche, M.A.; Nahi, K.S.; Bouchard, N.; Fontaine, F.G.; Maron, L. Hydroboration of Carbon Dioxide Using Ambiphilic Phosphine–Borane Catalysts: On the Role of the Formaldehyde Adduct. ACS Catal. 2015, 5, 2513–2520. [Google Scholar] [CrossRef]
- Rodriguez, J.; Liu, P.; Stacchiola, D.J.; Senanayake, S.D.; White, M.G.; Chen, J.G. Hydrogenation of CO2 to Methanol: Importance of Metal–Oxide and Metal–Carbide Interfaces in the Activation of CO2. ACS Catal. 2015, 5, 6696–6706. [Google Scholar] [CrossRef]
- Jaseer, E.A.; Akhtar, M.N.; Osman, M.; Al-Shammari, A.; Oladipo, H.B.; Garcés, K.; Fernández-Alvarez, F.J.; Al-Khattaf, S.; Oro, L.A. Solvent–free iridium–catalyzed CO2 hydrosilylation: Experiments and kinetic modeling. Cat. Sci. Technol. 2015, 5, 274–279. [Google Scholar] [CrossRef][Green Version]
- Porosoff, M.D.; Yan, B.; Chen, J.G. Catalytic reduction of CO2 by H2 for synthesis of CO, methanol and hydrocarbons: Challenges and opportunities. Energy Environ. Sci. 2016, 9, 62–73. [Google Scholar] [CrossRef]
- Ríos, P.; Rodríguez, A.; López-Serrano, J. Mechanistic Studies on the Selective Reduction of CO2 to the Aldehyde Level by a Bis(phosphino)boryl (PBP)–Supported Nickel Complex. ACS Catal. 2016, 6, 5715–5723. [Google Scholar] [CrossRef]
- Grasemann, M.; Laurenczy, G. Formic acid as a hydrogen source–recent developments and future trends. Energy Environ. Sci. 2012, 5, 8171–8181. [Google Scholar] [CrossRef]
- Wang, W.H.; Himeda, Y.; Muckerman, J.T.; Manbeck, G.F.; Fujita, E. CO2 Hydrogenation to Formate and Methanol as an Alternative to Photo- and Electrochemical CO2 Reduction. Chem. Rev. 2015, 115, 12936–12973. [Google Scholar] [CrossRef]
- Sordakis, K.; Tang, C.; Vogt, L.K.; Junge, H.; Dyson, P.J.; Beller, M.; Laurenczy, G. Homogeneous Catalysis for Sustainable Hydrogen Storage in Formic Acid and Alcohols. Chem. Rev. 2018, 118, 372–433. [Google Scholar] [CrossRef]
- Onishi, N.; Iguchi, M.; Yang, X.; Kanega, R.; Kawanami, H.; Xu, Q.; Himeda, Y. Hydrogen Storage Technology: Development of Effective Catalysts for Hydrogen Storage Technology Using Formic Acid. Adv. Energy Mat. 2019, 9, 1970090. [Google Scholar] [CrossRef]
- Hayashi, H.; Ogo, S.; Fukuzumi, S. Aqueous hydrogenation of carbon dioxide catalysed by water–soluble ruthenium aqua complexes under acidic conditions. Chem. Commun. 2004, 2004, 2714–2715. [Google Scholar] [CrossRef]
- Himeda, Y.; Onozawa-Komatsuzaki, N.; Sugihara, H.; Arakawa, H.; Kasuga, K. Half-Sandwich Complexes with 4,7-Dihydroxy-1,10-phenanthroline: Water-Soluble, Highly Efficient Catalysts for Hydrogenation of Bicarbonate Attributable to the Generation of an Oxyanion on the Catalyst Ligand. Organometallics 2004, 23, 1480–1483. [Google Scholar] [CrossRef]
- Himeda, Y.; Onozawa-Komatsuzaki, N.; Sugihara, H.; Kasuga, K. Simultaneous Tuning of Activity and Water Solubility of Complex Catalysts by Acid–Base Equilibrium of Ligands for Conversion of Carbon Dioxide. Organometallics 2007, 26, 702–712. [Google Scholar] [CrossRef]
- Federsel, C.; Jackstell, R.; Beller, M. State–of–the–Art Catalysts for Hydrogenation of Carbon Dioxide. Angew. Chem. Int. Ed. 2010, 49, 6254–6257. [Google Scholar] [CrossRef]
- Langer, R.; Diskin-Posner, Y.; Leitus, G.; Shimon, L.J.W.; Ben-David, Y.; Milstein, D. Low-Pressure Hydrogenation of Carbon Dioxide Catalyzed by an Iron Pincer Complex Exhibiting Noble Metal Activity. Angew. Chem. Int. Ed. 2011, 50, 9948–9952. [Google Scholar] [CrossRef]
- Maenaka, Y.; Suenobu, T.; Fukuzumi, S. Catalytic interconversion between hydrogen and formic acid at ambient temperature and pressure. Energy Environ. Sci. 2012, 5, 7360–7367. [Google Scholar] [CrossRef]
- Jeletic, M.S.; Mock, M.T.; Appel, A.M.; Linehan, J.C. A Cobalt-Based Catalyst for the Hydrogenation of CO2 under Ambient Conditions. J. Am. Chem. Soc. 2013, 135, 11533–11536. [Google Scholar] [CrossRef]
- Huff, C.A.; Sanford, M.S. Catalytic CO2 Hydrogenation to Formate by a Ruthenium Pincer Complex. ACS Catal. 2013, 3, 2412–2416. [Google Scholar] [CrossRef]
- Filonenko, G.A.; van Putten, R.; Schulpen, E.N.; Hensen, E.J.M.; Pidko, E.A. Highly Efficient Reversible Hydrogenation of Carbon Dioxide to Formates Using a Ruthenium PNP-Pincer Catalyst. ChemCatChem 2014, 6, 1526–1530. [Google Scholar] [CrossRef]
- Kothandaraman, J.; Czaun, M.; Goeppert, A.; Haiges, R.; Jones, J.P.; May, R.B.; Prakash, G.K.S.; Olah, G.A. Amine-Free Reversible Hydrogen Storage in Formate Salts Catalyzed by Ruthenium Pincer Complex without pH Control or Solvent Change. ChemSusChem 2015, 8, 1442–1451. [Google Scholar] [CrossRef] [PubMed]
- Bertini, F.; Gorgas, N.; Stöger, B.; Peruzzini, M.; Veiros, L.F.; Kirchner, K.; Gonsalvi, L. Efficient and Mild Carbon Dioxide Hydrogenation to Formate Catalyzed by Fe(II) Hydrido Carbonyl Complexes Bearing 2,6-(Diaminopyridyl)diphosphine Pincer Ligands. ACS Catal. 2016, 6, 2889–2893. [Google Scholar] [CrossRef]
- Lu, S.M.; Wang, Z.; Li, J.; Xiao, J.; Li, C. Base-free hydrogenation of CO2 to formic acid in water with an iridium complex bearing a N,N’-diimine ligand. Green Chem. 2016, 18, 4553–4558. [Google Scholar] [CrossRef]
- Jantke, D.; Pardatscher, L.; Drees, M.; Cokoja, M.; Herrmann, W.A.; Kühn, F.E. Hydrogen Production and Storage on a Formic Acid/Bicarbonate Platform using Water-Soluble N-Heterocyclic Carbene Complexes of Late Transition Metals. ChemSusChem 2016, 9, 2849–2854. [Google Scholar] [CrossRef] [PubMed]
- Aoki, W.; Wattanavinin, N.; Kusumoto, S.; Nozaki, K. Development of Highly Active Ir–PNP Catalysts for Hydrogenation of Carbon Dioxide with Organic Bases. Bull. Chem. Soc. Jpn. 2016, 89, 113–124. [Google Scholar] [CrossRef]
- Oldenhof, S.; van der Vlugt, J.I.; Reek, J.N.H. Hydrogenation of CO2 to formic acid with iridiumIII(bisMETAMORPhos)(hydride): The role of a dormant fac-IrIII(trihydride) and an active trans-IrIII(dihydride) species. Cat. Sci. Technol. 2016, 6, 404–408. [Google Scholar] [CrossRef]
- Tanaka, R.; Yamashita, M.; Nozaki, K. Catalytic Hydrogenation of Carbon Dioxide Using Ir(III)–Pincer Complexes. J. Am. Chem. Soc. 2009, 131, 14168–14169. [Google Scholar] [CrossRef]
- Tanaka, R.; Yamashita, M.; Chung, L.W.; Morokuma, K.; Nozaki, K. Mechanistic Studies on the Reversible Hydrogenation of Carbon Dioxide Catalyzed by an Ir–PNP Complex. Organometallics 2011, 30, 6742–6750. [Google Scholar] [CrossRef]
- Ahlquist, M.S.G. Iridium catalyzed hydrogenation of CO2 under basic conditions—Mechanistic insight from theory. J. Mol. Catal. A 2010, 324, 3–8. [Google Scholar] [CrossRef]
- Yang, X. Hydrogenation of Carbon Dioxide Catalyzed by PNP Pincer Iridium, Iron, and Cobalt Complexes: A Computational Design of Base Metal Catalysts. ACS Catal. 2011, 1, 849–854. [Google Scholar] [CrossRef]
- Hruszkewycz, D.P.; Wu, J.; Hazari, N.; Incarvito, C.D. Palladium(I)-Bridging Allyl Dimers for the Catalytic Functionalization of CO2. J. Am. Chem. Soc. 2011, 133, 3280–3283. [Google Scholar] [CrossRef]
- Fan, T.; Chen, X.; Lin, Z. Theoretical studies of reactions of carbon dioxide mediated and catalysed by transition metal complexes. Chem. Commun. 2012, 48, 10808–10828. [Google Scholar] [CrossRef]
- Kang, P.; Cheng, C.; Chen, Z.; Schauer, C.K.; Meyer, T.J.; Brookhart, M. Selective Electrocatalytic Reduction of CO2 to Formate by Water–Stable Iridium Dihydride Pincer Complexes. J. Am. Chem. Soc. 2012, 134, 5500–5503. [Google Scholar] [CrossRef]
- Bernskoetter, W.H.; Hazari, N. A Computational Investigation of the Insertion of Carbon Dioxide into Four- and Five-coordinate Iridium Hydrides. Eur. J. Inorg. Chem. 2013, 2013, 4032–4041. [Google Scholar] [CrossRef]
- Fang, S.; Chen, H.; Wei, H. Insight into catalytic reduction of CO2 to methane with silanes using Brookhart’s cationic Ir(III) pincer complex. RSC Adv. 2018, 8, 9232–9242. [Google Scholar] [CrossRef]
- Schmeier, T.J.; Dobereiner, G.E.; Crabtree, R.H.; Hazari, N. Secondary Coordination Sphere Interactions Facilitate the Insertion Step in an Iridium(III) CO2 Reduction Catalyst. J. Am. Chem. Soc. 2011, 133, 9274–9277. [Google Scholar] [CrossRef]
- Onishi, N.; Xu, S.; Manaka, Y.; Suna, Y.; Wang, W.H.; Muckerman, J.T.; Fujita, E.; Himeda, Y. CO2 Hydrogenation Catalyzed by Iridium Complexes with a Proton–Responsive Ligand. Inorg. Chem. 2015, 54, 5114–5123. [Google Scholar] [CrossRef]
- Kanega, R.; Ertem, M.Z.; Onishi, N.; Szalda, D.J.; Fujita, E.; Himeda, Y. CO2 Hydrogenation and Formic Acid Dehydrogenation Using Ir Catalysts with Amide–Based Ligands. Organometallics 2020, 39, 1519–1531. [Google Scholar] [CrossRef]
- Kraka, E.; Zou, W.; Tao, Y.; Freindorf, M. Exploring the Mechanism of Catalysis with the Unified Reaction Valley Approach (URVA)—A Review. Catalysts 2020, 10, 691. [Google Scholar] [CrossRef]
- Marcus, R. On analytical mechanics of chemical reactions. Quantum mechanics of linear collisions. J. Chem. Phys. 1966, 45, 4449–4493. [Google Scholar] [CrossRef]
- Marcus, R. On analytical mechanics of chemical reactions. Classical mechanics of linear collisions. J. Chem. Phys. 1966, 45, 4500–4504. [Google Scholar] [CrossRef]
- Marcus, R. Analytical mechanics of chemical reactions. 3. Natural collision coordinates. J. Chem. Phys. 1968, 49, 2610–2616. [Google Scholar] [CrossRef]
- Hofacker, L. Quantentheorie Chemischer Reaktionen. Z. Naturforsch. A 1963, 18, 60–619. [Google Scholar] [CrossRef][Green Version]
- Kraka, E.; Dunning, T.H., Jr. Characterization of Molecular Potential Energy Surfaces: Critical Points, Reaction Paths and Reaction Valleys. In Advances in Molecular Electronic Structure Theory: The Calculation and Characterization of Molecular Potential Energy Surfaces; Dunning, T.H., Ed.; JAI Press, Inc.: Greenwich, UK, 1990; pp. 129–173. [Google Scholar]
- Dunning, T.H., Jr.; Harding, L.B.; Kraka, E. Calculation and Characterization of Reaction Valleys for Chemical Reactions. In Supercomputer Algorithms for Reactivity, Dynamcis and Kinetics of Small Molecules; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1989; p. 57. [Google Scholar]
- Kühnel, W. Differential Geometry: Curves-Surfaces-Manifolds; American Mathematics Society, AMS: New York, NY, USA, 2005. [Google Scholar]
- Page, M.; McIver, J.W., Jr. On evaluating the Reaction path Hamiltonian. J. Chem. Phys. 1988, 88, 922–935. [Google Scholar] [CrossRef]
- Konkoli, Z.; Kraka, E.; Cremer, D. Unified Reaction Valley Approach Mechanism of the Reaction CH3 + H2→CH4 + H. J. Phys. Chem. A 1997, 101, 1742–1757. [Google Scholar] [CrossRef]
- Zou, W.; Sexton, T.; Kraka, E.; Freindorf, M.; Cremer, D. A New Method for Describing the Mechanism of a Chemical Reaction Based on the Unified Reaction Valley Approach. J. Chem. Theory Comput. 2016, 12, 650–663. [Google Scholar] [CrossRef]
- Cremer, D.; Pople, J.A. General Definition of Ring Puckering Coordinates. J. Am. Chem. Soc. 1975, 97, 1354–1358. [Google Scholar] [CrossRef]
- Miller, W.H.; Handy, N.C.; Adams, J.E. Reaction path Hamiltonian for polyatomic molecules. J. Chem. Phys. 1980, 72, 99–112. [Google Scholar] [CrossRef]
- Kato, S.; Morokuma, K. Potential energy characteristics and energy partitioning in chemical reactions: Ab initio MO study of four-centered elimination reaction CH3CH2F + CH2→CH2 + HF. J. Chem. Phys. 1980, 73, 3900–3914. [Google Scholar] [CrossRef]
- Kraka, E. Reaction Path Hamiltonian and the Unified Reaction Valley Approach. WIREs Comput. Mol. Sci. 2011, 1, 531–556. [Google Scholar] [CrossRef]
- Freindorf, M.; Kraka, E. Mechanistic Details of the Sharpless Epoxidation of Allylic Alcohols—A combined URVA and Local Mode Study. Catalysts 2022, 12, 789. [Google Scholar] [CrossRef]
- Freindorf, M.; Kraka, E. BF3–Catalyzed Diels–Alder Reaction between Butadiene and Methyl Acrylate in Aqueous Solution–An URVA and Local Vibrational Mode Study. Catalysts 2022, 12, 415. [Google Scholar] [CrossRef]
- Freindorf, M.; Tao, Y.; Kraka, E. A Closer Look at the Isomerization of 5–androstene–3,17–dione to 4–androstene–3,17–dione in Ketosteroid Isomerase. J. Comp. Biophys. Chem. 2022, 21, 313–333. [Google Scholar] [CrossRef]
- Kraka, E.; Freindorf, M. Chemical Bonding in Homogenous Catalysis - Seen Through the Eyes of Vibrational Spectroscopy. In Reference Module in Chemistry, Molecular Sciences and Chemical Engineering—Comprehensive Computational Chemistry; Elsevier: Amsterdam, The Netherlands, 2022; pp. 1–27. [Google Scholar] [CrossRef]
- Freindorf, M.; Beiranvand, N.; Delgado, A.A.A.; Tao, Y.; Kraka, E. On the formation of CN bonds in Titan’s atmosphere—A Unified Reaction Valley Approach study. J. Mol. Model. 2021, 27, 320. [Google Scholar] [CrossRef]
- Makoś, M.Z.; Freindorf, M.; Tao, Y.; Kraka, E. Theoretical Insights into [NHC]Au(I) Catalyzed Hydroalkoxylation of Allenes: A Unified Reaction Valley Approach Study. J. Org. Chem. 2021, 86, 5714–5726. [Google Scholar] [CrossRef]
- Nanayakkara, S.; Freindorf, M.; Tao, Y.; Kraka, E. Modeling Hydrogen release from water with Borane and Alane catalysts: A Unified Reaction Valley Approach. J. Phys. Chem. A 2020, 124, 8978–8993. [Google Scholar] [CrossRef]
- Tao, Y.; Zou, W.; Nanayakkara, S.; Kraka, E. PyVibMS: A PyMOL plugin for visualizing vibrations in molecules and solids. J. Mol. Model. 2020, 26, 290. [Google Scholar] [CrossRef]
- Nanayakkara, S.; Kraka, E. A New Way of Studying Chemical Reactions: A Hand-in-hand URVA and QTAIM Approach. Phys. Chem. Chem. Phys. 2019, 21, 15007–15018. [Google Scholar] [CrossRef] [PubMed]
- Freindorf, M.; Tao, Y.; Sethio, D.; Cremer, D.; Kraka, E. New Mechanistic Insights into the Claisen Rearrangement of Chorismate—A Unified Reaction Valley Approach Study. Mol. Phys. 2018, 117, 1172–1192. [Google Scholar] [CrossRef]
- Freindorf, M.; Cremer, D.; Kraka, E. Gold(I)-Assisted Catalysis—A Comprehensive View on the [3,3]-Sigmatropic Rearrangement of Allyl Acetate. Mol. Phys. 2017, 116, 611–630. [Google Scholar] [CrossRef]
- Reis, M.C.; López, C.S.; Kraka, E.; Cremer, D.; Faza, O.N. Rational Design in Catalysis: A Mechanistic Study of β-Hydride Eliminations in Gold(I) and Gold(III) Complexes Based on Features of the Reaction Valley. Inorg. Chem. 2016, 55, 8636–8645. [Google Scholar] [CrossRef] [PubMed]
- Sexton, T.; Kraka, E.; Cremer, D. Extraordinary Mechanism of the Diels-Alder Reaction: Investigation of Stereochemistry, Charge Transfer, Charge Polarization, and Biradicaloid Formation. J. Phys. Chem. A 2016, 120, 1097–1111. [Google Scholar] [CrossRef] [PubMed]
- Cremer, D.; Kraka, E. From Molecular Vibrations to Bonding, Chemical Reactions, and Reaction Mechanism. Curr. Org. Chem. 2010, 14, 1524–1560. [Google Scholar] [CrossRef]
- Kraka, E.; Cremer, D. Computational Analysis of the Mechanism of Chemical Reactions in Terms of Reaction Phases: Hidden Intermediates and Hidden Transition States. Acc. Chem. Res. 2010, 43, 591–601. [Google Scholar] [CrossRef]
- Wilson, E.B. Some Mathematical Methods for the Study of Molecular Vibrations. J. Chem. Phys. 1941, 9, 76–84. [Google Scholar] [CrossRef]
- Konkoli, Z.; Cremer, D. A New Way of Analyzing Vibrational Spectra. I. Derivation of Adiabatic Internal Modes. Int. J. Quantum Chem. 1998, 67, 1–9. [Google Scholar] [CrossRef]
- Konkoli, Z.; Larsson, J.A.; Cremer, D. A New Way of Analyzing Vibrational Spectra. II. Comparison of Internal Mode Frequencies. Int. J. Quantum Chem. 1998, 67, 11–27. [Google Scholar] [CrossRef]
- Konkoli, Z.; Cremer, D. A New Way of Analyzing Vibrational Spectra. III. Characterization of Normal Vibrational Modes in terms of Internal Vibrational Modes. Int. J. Quantum Chem. 1998, 67, 29–40. [Google Scholar] [CrossRef]
- Konkoli, Z.; Larsson, J.A.; Cremer, D. A New Way of Analyzing Vibrational Spectra. IV. Application and Testing of Adiabatic Modes within the Concept of the Characterization of Normal Modes. Int. J. Quantum Chem. 1998, 67, 41–55. [Google Scholar] [CrossRef]
- Cremer, D.; Larsson, J.A.; Kraka, E. New Developments in the Analysis of Vibrational Spectra on the Use of Adiabatic Internal Vibrational Modes. In Theoretical and Computational Chemistry; Parkanyi, C., Ed.; Elsevier: Amsterdam, The Netherlands, 1998; pp. 259–327. [Google Scholar]
- Kraka, E.; Zou, W.; Tao, Y. Decoding Chemical Information from Vibrational Spectroscopy Data: Local Vibrational Mode Theory. WIREs Comput. Mol. Sci. 2020, 10, 1480. [Google Scholar] [CrossRef]
- Kraka, E.; Quintano, M.; Force, H.W.L.; Antonio, J.J.; Freindorf, M. Feature Article: The Local Vibrational Mode Theory and Its Place in the Vibrational Spectroscopy Arena. J. Phys. Chem. A 2022, 126, 8781–8798. [Google Scholar] [CrossRef]
- Wilson, E.; Decius, J.; Cross, P. Molecular Vibrations. The Theory of Infrared and Raman Vibrational Spectra; McGraw-Hill: New York, NY, USA, 1955. [Google Scholar]
- Woodward, L.A. Introduction to the Theory of Molecular Vibrations and Vibrational Spectroscopy; Oxford University Press: Oxford, UK, 1972. [Google Scholar]
- Califano, S. Vibrational States; Wiley: London, UK, 1976. [Google Scholar]
- Zou, W.; Kalescky, R.; Kraka, E.; Cremer, D. Relating Normal Vibrational Modes to Local Vibrational Modes with the Help of an Adiabatic Connection Scheme. J. Chem. Phys. 2012, 137, 084114. [Google Scholar] [CrossRef]
- Zou, W.; Cremer, D. C2 in a Box: Determining its Intrinsic Bond Strength for the X1Σ+g Ground State. Chem. Eur. J. 2016, 22, 4087–4097. [Google Scholar] [CrossRef]
- Zou, W.; Cremer, D. Properties of Local Vibrational Modes: The Infrared Intensity. Theor. Chem. Acc. 2014, 133, 1451–1466. [Google Scholar] [CrossRef]
- Larsson, J.A.; Cremer, D. Theoretical Verification and Extension of the Mckean Relationship between Bond Lengths and Stretching Frequencies. J. Mol. Struct. 1999, 485–486, 385–407. [Google Scholar] [CrossRef]
- Kalescky, R.; Kraka, E.; Cremer, D. Identification of the Strongest Bonds in Chemistry. J. Phys. Chem. A 2013, 117, 8981–8995. [Google Scholar] [CrossRef]
- Setiawan, D.; Sethio, D.; Cremer, D.; Kraka, E. From Strong to Weak NF Bonds: On the Design of a New Class of Fluorinating Agents. Phys. Chem. Chem. Phys. 2018, 20, 23913–23927. [Google Scholar] [CrossRef]
- Humason, A.; Zou, W.; Cremer, D. 11,11-Dimethyl-1,6-methano[10]annulene-An Annulene with an Ultralong CC Bond or a Fluxional Molecule? J. Phys. Chem. A 2014, 119, 1666–1682. [Google Scholar] [CrossRef]
- Kalescky, R.; Kraka, E.; Cremer, D. Are Carbon-Halogen Double and Triple Bonds Possible? Int. J. Quantum Chem. 2014, 114, 1060–1072. [Google Scholar] [CrossRef]
- Kalescky, R.; Zou, W.; Kraka, E.; Cremer, D. Quantitative Assessment of the Multiplicity of Carbon-Halogen Bonds: Carbenium and Halonium Ions with F, Cl, Br, and I. J. Phys. Chem. A 2014, 118, 1948–1963. [Google Scholar] [CrossRef]
- Cremer, D.; Wu, A.; Larsson, J.A.; Kraka, E. Some Thoughts about Bond Energies, Bond Lengths, and Force Constants. J. Mol. Model. 2000, 6, 396–412. [Google Scholar] [CrossRef]
- Kraka, E.; Cremer, D. Weaker Bonds with Shorter Bond Lengths. Rev. Proc. Quim. 2012, 39–42. [Google Scholar] [CrossRef]
- Setiawan, D.; Kraka, E.; Cremer, D. Hidden Bond Anomalies: The Peculiar Case of the Fluorinated Amine Chalcogenides. J. Phys. Chem. A 2015, 119, 9541–9556. [Google Scholar] [CrossRef] [PubMed]
- Kraka, E.; Setiawan, D.; Cremer, D. Re-Evaluation of the Bond Length-Bond Strength Rule: The Stronger Bond Is not Always the Shorter Bond. J. Comput. Chem. 2015, 37, 130–142. [Google Scholar] [CrossRef]
- Kalescky, R.; Zou, W.; Kraka, E.; Cremer, D. Local Vibrational Modes of the Water Dimer—Comparison of Theory and Experiment. Chem. Phys. Lett. 2012, 554, 243–247. [Google Scholar] [CrossRef]
- Kalescky, R.; Kraka, E.; Cremer, D. Local Vibrational Modes of the Formic Acid Dimer—The Strength of the Double H-Bond. Mol. Phys. 2013, 111, 1497–1510. [Google Scholar] [CrossRef]
- Freindorf, M.; Kraka, E.; Cremer, D. A Comprehensive Analysis of Hydrogen Bond Interactions Based on Local Vibrational Modes. Int. J. Quantum Chem. 2012, 112, 3174–3187. [Google Scholar] [CrossRef]
- Kalescky, R.; Zou, W.; Kraka, E.; Cremer, D. Vibrational Properties of the Isotopomers of the Water Dimer Derived from Experiment and Computations. Aust. J. Chem. 2014, 67, 426. [Google Scholar] [CrossRef]
- Tao, Y.; Zou, W.; Jia, J.; Li, W.; Cremer, D. Different Ways of Hydrogen Bonding in Water—Why Does Warm Water Freeze Faster than Cold Water? J. Chem. Theory Comput. 2017, 13, 55–76. [Google Scholar] [CrossRef] [PubMed]
- Tao, Y.; Zou, W.; Kraka, E. Strengthening of Hydrogen Bonding With the Push-Pull Effect. Chem. Phys. Lett. 2017, 685, 251–258. [Google Scholar] [CrossRef]
- Makoś, M.Z.; Freindorf, M.; Sethio, D.; Kraka, E. New Insights into Fe–H2 and Fe–H- Bonding of a [NiFe] Hydrogenase Mimic—A Local Vibrational Mode Study. Theor. Chem. Acc. 2019, 138, 76. [Google Scholar] [CrossRef]
- Lyu, S.; Beiranvand, N.; Freindorf, M.; Kraka, E. Interplay of Ring Puckering and Hydrogen Bonding in Deoxyribonucleosides. J. Phys. Chem. A 2019, 123, 7087–7103. [Google Scholar] [CrossRef] [PubMed]
- Yannacone, S.; Sethio, D.; Kraka, E. Quantitative Assessment of Intramolecular Hydrogen Bonds in Neutral Histidine. Theor. Chem. Acc. 2020, 139, 125. [Google Scholar] [CrossRef]
- Beiranvand, N.; Freindorf, M.; Kraka, E. Hydrogen Bonding in Natural and Unnatural Base Pairs - Explored with Vibrational Spectroscopy. Molecules 2021, 26, 2268. [Google Scholar] [CrossRef]
- Oliveira, V.; Kraka, E.; Cremer, D. The Intrinsic Strength of the Halogen Bond: Electrostatic and Covalent Contributions Described by Coupled Cluster Theory. Phys. Chem. Chem. Phys. 2016, 18, 33031–33046. [Google Scholar] [CrossRef]
- Oliveira, V.; Kraka, E.; Cremer, D. Quantitative Assessment of Halogen Bonding Utilizing Vibrational Spectroscopy. Inorg. Chem. 2016, 56, 488–502. [Google Scholar] [CrossRef]
- Oliveira, V.; Cremer, D. Transition from Metal-Ligand Bonding to Halogen Bonding Involving a Metal as Halogen Acceptor: A Study of Cu, Ag, Au, Pt, and Hg Complexes. Chem. Phys. Lett. 2017, 681, 56–63. [Google Scholar] [CrossRef]
- Yannacone, S.; Oliveira, V.; Verma, N.; Kraka, E. A Continuum from Halogen Bonds to Covalent Bonds: Where Do λ3 Iodanes Fit? Inorganics 2019, 7, 47. [Google Scholar] [CrossRef]
- Oliveira, V.P.; Kraka, E.; Machado, F.B.C. Pushing 3c-4e Bonds to the Limit: A Coupled Cluster Study of Stepwise Fluorination of First-Row Atoms. Inorg. Chem. 2019, 58, 14777–14789. [Google Scholar] [CrossRef] [PubMed]
- Oliveira, V.P.; Marcial, B.L.; Machado, F.B.C.; Kraka, E. Metal-Halogen Bonding Seen through the Eyes of Vibrational Spectroscopy. Materials 2020, 13, 55. [Google Scholar] [CrossRef] [PubMed]
- Oliveira, V.; Kraka, E. Systematic Coupled Cluster Study of Noncovalent Interactions Involving Halogens, Chalcogens, and Pnicogens. J. Phys. Chem. A 2017, 121, 9544–9556. [Google Scholar] [CrossRef] [PubMed]
- Setiawan, D.; Kraka, E.; Cremer, D. Description of Pnicogen Bonding with the help of Vibrational Spectroscopy-The Missing Link Between Theory and Experiment. Chem. Phys. Lett. 2014, 614, 136–142. [Google Scholar] [CrossRef]
- Setiawan, D.; Kraka, E.; Cremer, D. Strength of the Pnicogen Bond in Complexes Involving Group VA Elements N, P, and As. J. Phys. Chem. A 2014, 119, 1642–1656. [Google Scholar] [CrossRef]
- Setiawan, D.; Cremer, D. Super-Pnicogen Bonding in the Radical Anion of the Fluorophosphine Dimer. Chem. Phys. Lett. 2016, 662, 182–187. [Google Scholar] [CrossRef]
- Oliveira, V.; Cremer, D.; Kraka, E. The Many Facets of Chalcogen Bonding: Described by Vibrational Spectroscopy. J. Phys. Chem. A 2017, 121, 6845–6862. [Google Scholar] [CrossRef]
- Sethio, D.; Oliveira, V.; Kraka, E. Quantitative Assessment of Tetrel Bonding Utilizing Vibrational Spectroscopy. Molecules 2018, 23, 2763. [Google Scholar] [CrossRef]
- Li, Y.; Oliveira, V.; Tang, C.; Cremer, D.; Liu, C.; Ma, J. The Peculiar Role of the Au3 Unit in Aum Clusters: σ-Aromaticity of the Au5Zn+ Ion. Inorg. Chem. 2017, 56, 5793–5803. [Google Scholar] [CrossRef]
- Kalescky, R.; Kraka, E.; Cremer, D. New Approach to Tolman’s Electronic Parameter Based on Local Vibrational Modes. Inorg. Chem. 2013, 53, 478–495. [Google Scholar] [CrossRef] [PubMed]
- Setiawan, D.; Kalescky, R.; Kraka, E.; Cremer, D. Direct Measure of Metal-Ligand Bonding Replacing the Tolman Electronic Parameter. Inorg. Chem. 2016, 55, 2332–2344. [Google Scholar] [CrossRef] [PubMed]
- Cremer, D.; Kraka, E. Generalization of the Tolman Electronic Parameter: The Metal-Ligand Electronic Parameter and the Intrinsic Strength of the Metal-Ligand Bond. Dalton Trans. 2017, 46, 8323–8338. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Liu, C.; Oliveira, V.; Cremer, D.; Chen, Z.; Ma, J. Odd-even Effect of the Number of Free Valence Electrons on the Electronic Structure Properties of Gold-thiolate Clusters. Mol. Phys. 2018, 117, 1442–1450. [Google Scholar] [CrossRef]
- Kraka, E.; Freindorf, M. Characterizing the Metal Ligand Bond Strength via Vibrational Spectroscopy: The Metal Ligand Electronic Parameter (MLEP). In Topics in Organometallic Chemistry—New Directions in the Modeling of Organometallic Reactions; Lledós, A., Ujaque, G., Eds.; Springer: Berlin/Heidelberg, Germany, 2020; Volume 67, pp. 1–43. [Google Scholar]
- Zou, W.; Tao, Y.; Freindorf, M.; Cremer, D.; Kraka, E. Local Vibrational Force Constants - from the Assessment of Empirical Force Constants to the Description of Bonding in Large Systems. Chem. Phys. Lett. 2020, 478, 137337. [Google Scholar] [CrossRef]
- Freindorf, M.; Kraka, E. Critical Assessment of the FeC and CO Bond strength in Carboxymyoglobin—A QM/MM Local Vibrational Mode Study. J. Mol. Model. 2020, 26, 281. [Google Scholar] [CrossRef]
- Kraka, E.; Larsson, J.A.; Cremer, D. Generalization of the Badger Rule Based on the Use of Adiabatic Vibrational Modes. In Computational Spectroscopy; Grunenberg, J., Ed.; Wiley: New York, NY, USA, 2010; pp. 105–149. [Google Scholar]
- Mayer, I. Charge, bond brder an valence in the ab initio theory. Chem. Phys. Lett. 1983, 97, 270–274. [Google Scholar] [CrossRef]
- Mayer, I. Bond orders and valences from ab initio wave functions. Int. J. Quantum Chem. 1986, 29, 477–483. [Google Scholar] [CrossRef]
- Mayer, I. Bond order and valence indices: A personal account. J. Comput. Chem. 2007, 28, 204–221. [Google Scholar] [CrossRef]
- Bader, R.F.W. A quantum theory of molecular structure and its applications. Chem. Rev. 1991, 91, 893–928. [Google Scholar] [CrossRef]
- Bader, R.F.W. Atoms in Molecules: A Quantum Theory (International Series of Monographs on Chemistry); Clarendon Press: Oxford, UK, 1994. [Google Scholar]
- Bader, R.F.W. The Quantum Mechanical Basis of Conceptual Chemistry. Monatshefte Chem. 2005, 136, 819–854. [Google Scholar] [CrossRef]
- Popelier, P.L. Atoms in Molecules: An Introduction; Prentice Hall: Hoboken, NJ, USA, 2000. [Google Scholar]
- Cremer, D.; Kraka, E. Chemical Bonds without Bonding Electron Density? Does the Difference Electron-Density Analysis Suffice for a Description of the Chemical Bond? Angew. Chem. Int. Ed. 1984, 23, 627–628. [Google Scholar] [CrossRef]
- Cremer, D.; Kraka, E. A Description of the Chemical Bond in Terms of Local Properties of Electron Density and Energy. Croat. Chem. Acta 1984, 57, 1259–1281. [Google Scholar]
- Kraka, E.; Cremer, D. Chemical Implication of Local Features of the Electron Density Distribution. In Theoretical Models of Chemical Bonding. The Concept of the Chemical Bond; Maksic, Z.B., Ed.; Springer: Berlin/Heidelberg, Germany, 1990; Volume 2, pp. 453–542. [Google Scholar]
- Fukui, K. The Path of Chemical Reactions—The IRC Approach. Acc. Chem. Res. 1981, 14, 363–368. [Google Scholar] [CrossRef]
- Hratchian, H.P.; Kraka, E. Improved Predictor-Corrector Integrators For Evaluating Reaction Path Curvature. J. Chem. Theory Comput. 2013, 9, 1481–1488. [Google Scholar] [CrossRef]
- Becke, A.D. Density-Functional Thermochemistry. III. The Role of Exact Exchange. J. Chem. Phys. 1993, 98, 5648–5652. [Google Scholar] [CrossRef]
- Lee, C.; Yang, W.; Parr, R. Development of the Colle-Salvetti correlation-energy formula into a functional of the electron density. Phys. Rev. B 1988, 37, 785–789. [Google Scholar] [CrossRef]
- Vosko, S.; Wilk, L.; Nusair, M. Accurate spin-dependent electron liquid correlation energies for local spin density calculations: A critical analysis. Can. J. Phys. 1980, 58, 1200–1211. [Google Scholar] [CrossRef]
- Stephens, P.; Devlin, F.; Chabalowski, C.; Frisch, M. Ab Initio Calculation of Vibrational Absorption and Circular Dichroism Spectra Using Density Functional Force Fields. J. Phys. Chem. 1994, 98, 11623–11627. [Google Scholar] [CrossRef]
- McLean, A.D.; Chandler, G.S. Contracted Gaussian basis sets for molecular calculations. I. Second row atoms, Z = 11–18. J. Chem. Phys. 1980, 72, 5639–5648. [Google Scholar] [CrossRef]
- Krishnan, R.; Binkley, J.S.; Seeger, R.; Pople, J.A. Self-consistent molecular orbital methods. XX. A basis set for correlated wave functions. J. Chem. Phys. 1980, 72, 650–654. [Google Scholar] [CrossRef]
- Andrae, D.; Häußermann, U.; Dolg, M.; Stoll, H.; Preuß, H. Energy-adjusted ab initio pseudopotentials for the second and third row transition elements. Theoretica Chim. Acta 1990, 77, 123–141. [Google Scholar] [CrossRef]
- Riplinger, C.; Neese, F. An efficient and near linear scaling pair natural orbital based local coupled cluster method. J. Chem. Phys. 2013, 138, 034106. [Google Scholar] [CrossRef] [PubMed]
- Weigend, F.; Ahlrichs, R. Balanced basis sets of split valence, triple zeta valence and quadruple zeta valence quality for H to Rn: Design and assessment of accuracy. Phys. Chem. Chem. Phys. 2005, 7, 3297–3305. [Google Scholar] [CrossRef] [PubMed]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Mennucci, B.; Petersson, G.A.; et al. Theoretical Study of 5-HTP. Potential New Drug Resulting from the Complexation of 5-HTP with ATP; Gaussian Inc.: Wallingford, CT, USA, 2009. [Google Scholar]
- Neese, F. The ORCA program system. WIREs Comput. Mol. Sci. 2012, 2, 73–78. [Google Scholar] [CrossRef]
- Tao, Y.; Zou, W.; Freindorf, M.; Cremer, D.; Kraka, E. pURVA; Computational and Theoretical Chemistry Group (CATCO), Southern Methodist University: Dallas, TX, USA, 2021. [Google Scholar]
- Zou, W.; Tao, Y.; Freindorf, M.; Makoś, M.Z.; Verma, N.; Cremer, D.; Kraka, E. Local Vibrational Mode Analysis (LModeA); Computational and Theoretical Chemistry Group (CATCO), Southern Methodist University: Dallas, TX, USA, 2022. [Google Scholar]
- Glendening, E.D.; Landis, C.R.; Weinhold, F. NBO 6.0: Natural bond orbital analysis program. J. Comput. Chem. 2013, 34, 1429–1437. [Google Scholar] [CrossRef]
- Reed, A.E.; Curtiss, L.A.; Weinhold, F. Intermolecular Interactions from a Natural Bond Orbital, Donor-Acceptor Viewpoint. Chem. Rev. 1988, 88, 899–926. [Google Scholar] [CrossRef]
- Weinhold, F.; Landis, C.R. Valency and Bonding: A Natural Bond Orbital Donor-Acceptor Perspective; Cambridge University Press: Cambridge, MA, USA, 2003. [Google Scholar]
- Weinhold, F. The Path to Natural Bond Orbitals. Isr. J. Chem. 2021. [Google Scholar] [CrossRef]
- Keith, T.A. AIMALL; TK Gristmill Software: Overland Park, KS, USA, 2017. [Google Scholar]
- Badger, R.M. A Relation between Internuclear Distances and Bond Force Constants. J. Chem. Phys. 1934, 2, 128–131. [Google Scholar] [CrossRef]
- Kraka, E.; Cremer, D. Characterization of CF Bonds with Multiple-Bond Character: Bond Lengths, Stretching Force Constants, and Bond Dissociation Energies. ChemPhysChem 2009, 10, 686–698. [Google Scholar] [CrossRef]
- Kaupp, M.; Danovich, D.; Shaik, S. Chemistry is about energy and its changes: A critique of bond-length/bond-strength correlations. Coord. Chem. Rev. 2017, 344, 355–362. [Google Scholar] [CrossRef]





| Bond | R(Å) | k (mDyn/Å) | BSO 1 | H (Hr/Bohr) | Molecule |
|---|---|---|---|---|---|
| CO | 1.418 | 4.905 | 1.000 | −0.3719 | CHOH |
| 1.207 | 13.607 | 2.000 | −0.6883 | CHO | |
| CH, HH, OH | 1.150 | 1.203 | 0.500 | −0.1951 | FH |
| 0.925 | 9.420 | 1.000 | −0.7275 | HF | |
| IrO | 2.034 | 2.829 | 1.030 | −0.0300 | Ir(CO)OH |
| 1.822 | 5.340 | 1.469 | −0.1035 | Ir(CO)O | |
| IrH | 2.095 | 0.370 | 0.197 | −0.0110 | Ir(CO)H |
| 1.857 | 0.764 | 0.313 | −0.0219 | Ir(CO)H | |
| CO 2 | 1.169 | 16.404 | 2.271 | −0.7595 | CO |
| HH 3 | 0.743 | 5.921 | 0.855 | −0.3360 | H |
| DFT | DLPNO-CCSD(T) | |||||||
|---|---|---|---|---|---|---|---|---|
| Reaction | E | E | H | H | E | E | H | H |
| R0 | 72.2 | 3.6 | 71.8 | 9.2 | 76.3 | 5.1 | 75.9 | 10.7 |
| R1 | 14.9 | −12.2 | 16.2 | −9.3 | 17.6 | −9.3 | 18.9 | −6.3 |
| R2 | 23.9 | 22.3 | 22.8 | 21.7 | 23.9 | 22.9 | 22.7 | 22.3 |
| R3 | 12.3 | 8.7 | 11.3 | 8.0 | 12.2 | 10.5 | 11.3 | 9.7 |
| R4 | 1.2 | −10.3 | 0.6 | −8.5 | 1.7 | −13.6 | 1.2 | −11.7 |
| R5 | 20.9 | −15.3 | 18.6 | −13.8 | 23.5 | −11.9 | 21.3 | −10.4 |
| R (Å) | k (mDyn/Å) | BSO | H (Hr/Bohr) | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Bond | RE | TS | PR | RE | TS | PR | RE | TS | PR | RE | TS | PR |
| R0 | ||||||||||||
| CO | 1.169 | 1.260 | 1.353 | 16.364 | 10.621 | 5.972 | 2.267 | 1.690 | 1.143 | −0.7594 | −0.6537 | −0.4670 |
| CO | 1.169 | 1.176 | 1.198 | 16.402 | 14.830 | 13.994 | 2.271 | 2.120 | 2.039 | −0.7597 | −0.7484 | −0.7315 |
| CH | 3.128 | 1.447 | 1.108 | 0.019 | 0.865 | 4.805 | 0.124 | 0.447 | 0.797 | - | −0.0666 | −0.2896 |
| OH | 2.959 | 1.308 | 0.969 | 0.017 | 1.686 | 8.005 | 0.119 | 0.560 | 0.947 | 0.0010 | −0.1021 | −0.5918 |
| HH | 0.743 | 1.030 | 2.120 | 5.898 | 1.210 | 0.469 | 0.854 | 0.501 | 0.364 | −0.3364 | −0.0861 | - |
| R1 | ||||||||||||
| CO | 1.170 | 1.242 | 1.240 | 16.098 | 9.679 | 10.562 | 2.242 | 1.587 | 1.684 | −0.7449 | −0.6444 | −0.6432 |
| CO | 1.170 | 1.268 | 1.283 | 16.039 | 8.344 | 8.253 | 2.236 | 1.435 | 1.424 | −0.7436 | −0.6083 | −0.5787 |
| CH | 2.769 | 1.153 | 1.111 | 0.041 | 3.090 | 4.692 | 0.160 | 0.687 | 0.791 | 0.0014 | −0.2259 | −0.2828 |
| IrO | 4.262 | 2.893 | 2.222 | 0.049 | 0.415 | 1.184 | 0.107 | 0.352 | 0.633 | - | - | −0.0038 |
| IrH | 1.679 | 2.359 | 4.079 | 1.983 | 0.415 | - | 0.576 | 0.212 | - | −0.0574 | −0.0003 | - |
| R2 | ||||||||||||
| CO | 1.240 | 1.318 | 1.311 | 10.562 | 7.086 | 7.134 | 1.684 | 1.284 | 1.290 | −0.6432 | −0.5196 | −0.5320 |
| CO | 1.283 | 1.224 | 1.229 | 8.253 | 11.827 | 11.316 | 1.424 | 1.818 | 1.765 | −0.5787 | −0.6731 | −0.6671 |
| CH | 1.111 | 1.096 | 1.097 | 4.692 | 5.302 | 5.270 | 0.791 | 0.824 | 0.822 | −0.2828 | −0.3133 | −0.3115 |
| CH | 1.094 | 2.623 | 3.084 | 5.237 | 0.138 | 0.117 | 0.821 | 0.241 | 0.228 | −0.2896 | - | - |
| OH | 2.403 | 0.985 | 1.011 | 0.029 | 6.893 | 4.053 | 0.143 | 0.900 | 0.753 | 0.0020 | −0.5652 | −0.4934 |
| IrO | 2.222 | 2.351 | 2.333 | 1.184 | 0.573 | 0.635 | 0.633 | 0.422 | 0.447 | −0.0038 | 0.0002 | −0.0002 |
| R3 | ||||||||||||
| CO | 1.311 | 1.337 | 1.338 | 7.134 | 6.260 | 6.280 | 1.290 | 1.180 | 1.183 | −0.5320 | −0.4924 | −0.4907 |
| CO | 1.229 | 1.210 | 1.209 | 11.316 | 12.883 | 13.069 | 1.765 | 1.927 | 1.946 | −0.6671 | −0.6978 | −0.6967 |
| IrO | 2.333 | 3.476 | 4.085 | 0.635 | 0.076 | 0.050 | 0.447 | 0.136 | 0.108 | −0.0002 | 0.0004 | - |
| IrH | 1.564 | 1.548 | 1.576 | 3.139 | 3.129 | 2.758 | 0.772 | 0.770 | 0.710 | −0.0981 | −0.1078 | −0.0949 |
| IrH | 1.590 | 1.586 | 1.578 | 2.833 | 2.737 | 2.728 | 0.723 | 0.707 | 0.706 | −0.0874 | −0.0900 | −0.0939 |
| NH | 1.747 | 2.031 | 3.225 | 0.219 | 0.129 | 0.029 | 0.282 | 0.236 | 0.143 | −0.0040 | −0.0007 | - |
| R4 | ||||||||||||
| HH | 0.745 | 0.750 | 0.842 | 5.744 | 5.371 | 1.826 | 0.847 | 0.828 | 0.575 | −0.3330 | −0.3225 | −0.2020 |
| IrH | 4.201 | 3.210 | 1.829 | 0.004 | 0.071 | 0.730 | 0.011 | 0.069 | 0.304 | - | - | - |
| IrH | 3.461 | 2.597 | 1.787 | 0.004 | 0.050 | 0.807 | 0.011 | 0.055 | 0.324 | 0.0004 | 0.0001 | −0.0275 |
| IrH | 1.576 | 1.545 | 1.589 | 2.758 | 3.318 | 2.783 | 0.710 | 0.799 | 0.715 | −0.0949 | −0.1091 | −0.0889 |
| IrH | 1.578 | 1.595 | 1.604 | 2.728 | 2.760 | 2.762 | 0.706 | 0.711 | 0.711 | −0.0939 | −0.0859 | −0.0829 |
| R5 | ||||||||||||
| HH | 0.842 | 0.986 | 3.182 | 1.826 | 0.707 | 0.037 | 0.575 | 0.418 | 0.155 | −0.2020 | −0.0907 | - |
| IrH | 1.829 | 2.335 | 3.588 | 0.730 | 0.355 | 0.107 | 0.304 | 0.192 | 0.089 | - | - | - |
| IrH | 1.787 | 1.909 | 1.679 | 0.807 | 0.567 | 1.987 | 0.324 | 0.259 | 0.576 | −0.0275 | −0.0202 | −0.0581 |
| IrH | 1.589 | 1.591 | 1.679 | 2.783 | 2.737 | 1.988 | 0.715 | 0.707 | 0.576 | −0.0889 | −0.0863 | −0.0581 |
| IrH | 1.604 | 1.592 | 1.585 | 2.762 | 2.842 | 2.914 | 0.711 | 0.724 | 0.736 | −0.0829 | −0.0869 | −0.0894 |
| CH | 3.481 | 1.580 | 1.098 | 0.105 | 0.932 | 5.118 | 0.220 | 0.459 | 0.814 | - | −0.0371 | −0.2792 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Freindorf, M.; Kraka, E. URVA and Local Mode Analysis of an Iridium Pincer Complex Efficiently Catalyzing the Hydrogenation of Carbon Dioxide. Inorganics 2022, 10, 234. https://doi.org/10.3390/inorganics10120234
Freindorf M, Kraka E. URVA and Local Mode Analysis of an Iridium Pincer Complex Efficiently Catalyzing the Hydrogenation of Carbon Dioxide. Inorganics. 2022; 10(12):234. https://doi.org/10.3390/inorganics10120234
Chicago/Turabian StyleFreindorf, Marek, and Elfi Kraka. 2022. "URVA and Local Mode Analysis of an Iridium Pincer Complex Efficiently Catalyzing the Hydrogenation of Carbon Dioxide" Inorganics 10, no. 12: 234. https://doi.org/10.3390/inorganics10120234
APA StyleFreindorf, M., & Kraka, E. (2022). URVA and Local Mode Analysis of an Iridium Pincer Complex Efficiently Catalyzing the Hydrogenation of Carbon Dioxide. Inorganics, 10(12), 234. https://doi.org/10.3390/inorganics10120234







