Performance Comparison of Different Modulation Formats for a 40 Gbps Hybrid Optical CDMA/DWDM System against ISI and FWM
Abstract
:1. Introduction
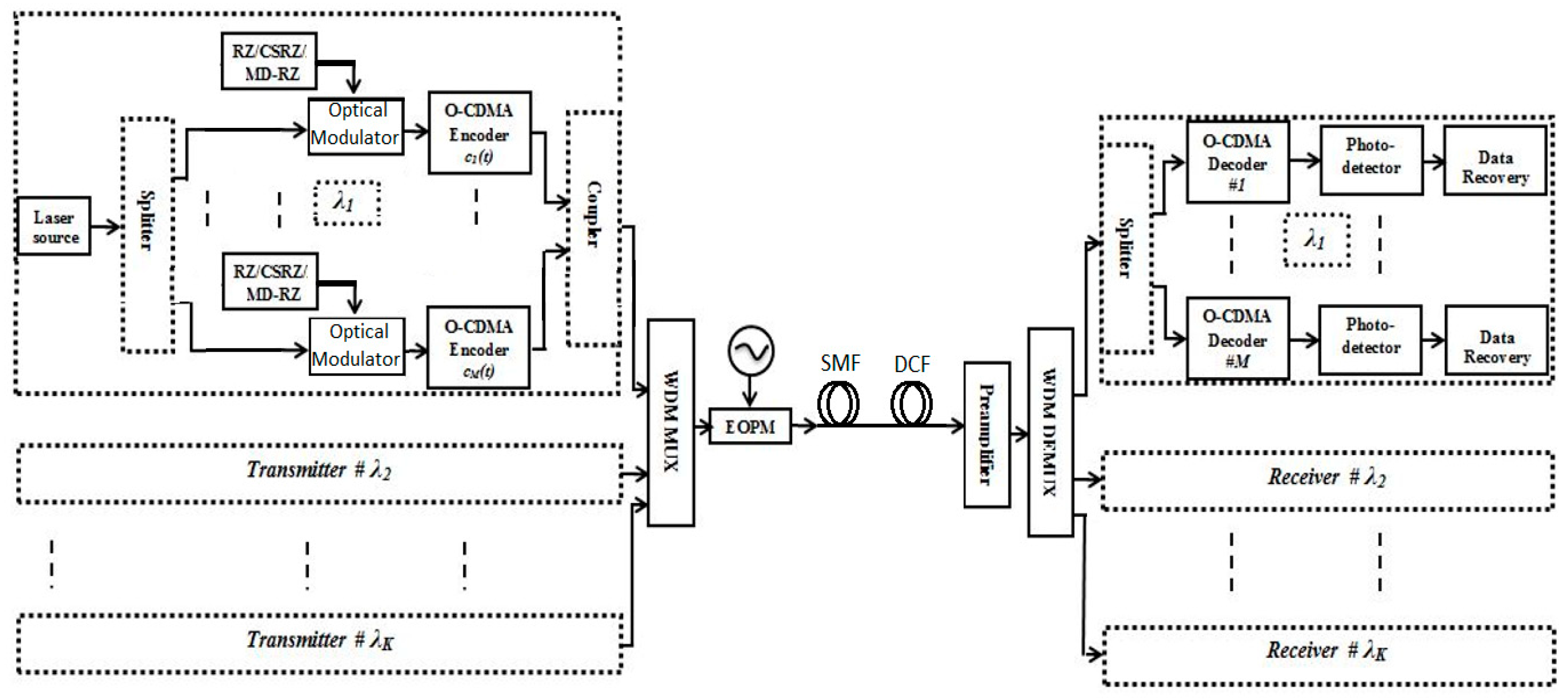
2. Signal Generators and System Description
3. Performance Analysis
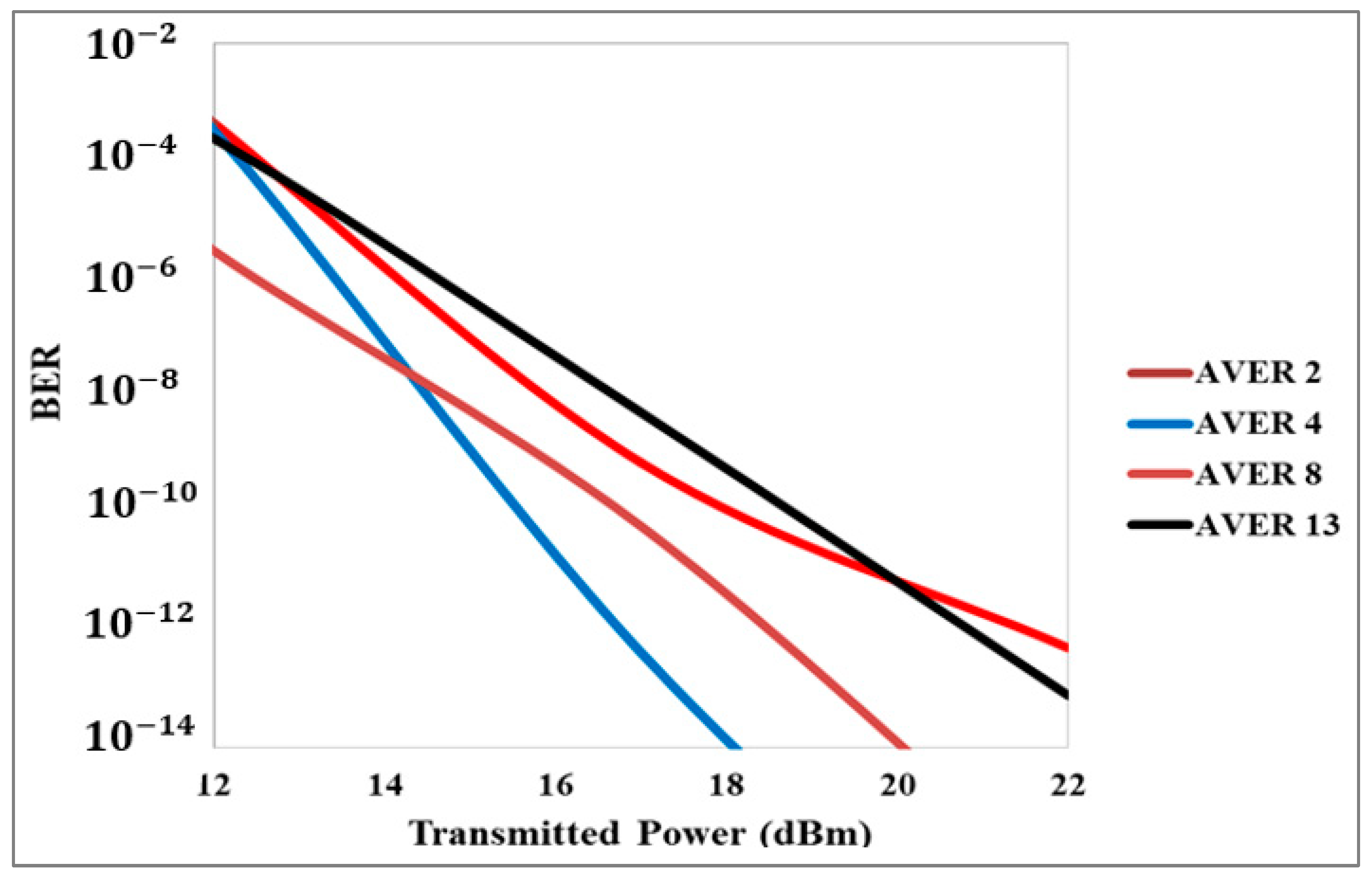
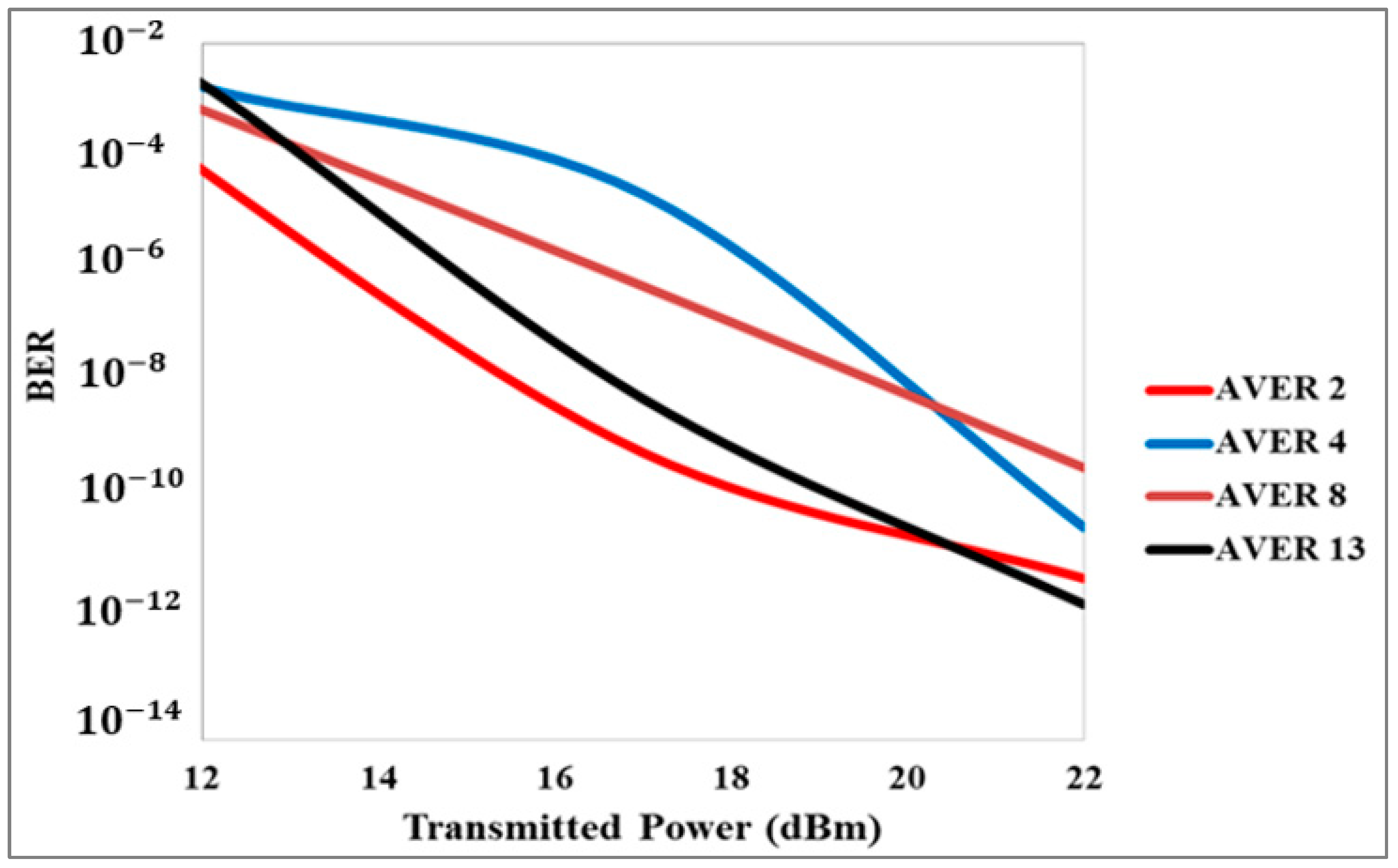
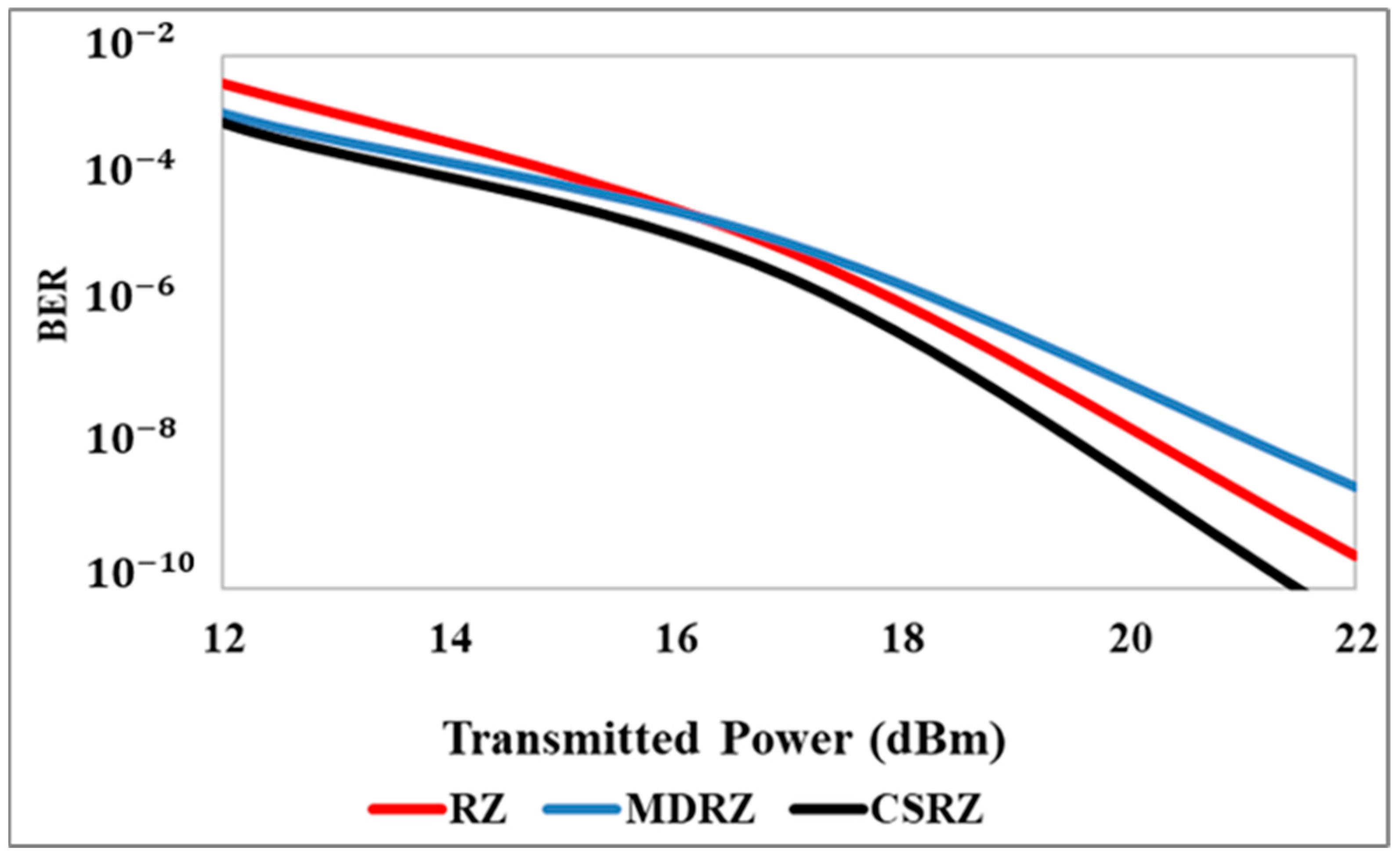
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cisco Annual Internet Report. Available online: https://www.cisco.com/c/en/us/solutions/collateral/executive-perspectives/annual-internet-report/white-paper-c11-741490.pdf (accessed on 14 July 2022).
- Wang, X.; Wada, N.; Miyazaki, T.; Cincotti, G.; Kitayama, K.-I. Field Trial of 3-WDM × 10-OCDMA × 10.71-Gb/s Asynchronous WDM/DPSK-OCDMA Using Hybrid E/D Without FEC and Optical Thresholding. J. Lightwave Technol. 2007, 25, 207–215. [Google Scholar] [CrossRef]
- Kitayama, K.; Wang, X.; Wada, N. OCDMA over WDM PON—A solution path to gigabit-symmetric FTTH-. J. Lightwave Technol. 2006, 24, 1654. [Google Scholar] [CrossRef]
- Chen, H.; Xiao, S.; Zhu, M.; Shi, J.; Bi, M. Hybrid WDMA/OCDM system with the capability of encoding multiple wavelength channels by employing one encoder and one corresponding optical code. Chin. Opt. Lett. 2010, 8, 745–748. [Google Scholar] [CrossRef]
- Huang, J.-F.; Nieh, T.-C.; Chen, K.-S. Structuring waveguide-grating-based wavelength-division multiplexing/optical code division multiple access network codecs over topology of concentric circles. Opt. Eng. 2013, 52, 015006. [Google Scholar] [CrossRef]
- Choi, Y.-K.; Hanawa, M.; Wang, X.; Park, C.-S. Upstream Transmission of WDM/OCDM-PON in a Loop-Back Configuration with Remotely Supplied Short Optical Pulses. J. Opt. Commun. Netw. 2013, 5, 183–189. [Google Scholar] [CrossRef]
- Xu, T.; Jacobsen, G.; Popov, S.; Li, J.; Vanin, E.; Wang, K.; Friberg, A.T.; Zhang, Y. Chromatic dispersion compensation in coherent transmission system using digital filters. Opt. Express 2010, 18, 16243–16257. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dar, A.B.; Jha, R.K. Chromatic dispersion compensation techniques and characterization of fiber Bragg grating for dispersion compensation. Opt. Quantum Electron. 2017, 49, 108. [Google Scholar] [CrossRef]
- Pradhan, S.R.; Sahoo, S.R.; Pradhani, G.R.; Panda, T. Chromatic Dispersion Compensation Using Adaptive Fiber Bragg Grating for High-Speed Optical Communication. ECS Trans. 2022, 107, 7201. [Google Scholar] [CrossRef]
- Ghazi, A.; Aljunid, S.A.; Idrus, S.Z.S.; Rashidi, C.B.M.; Al-dawoodi, A.; Mahmood, B.A.; Rafeeq, R.M. A Systematic review of Multi-Mode Fiber based on Dimensional Code in Optical-CDMA. J. Phys. Conf. Ser. 2021, 1860, 012016. [Google Scholar] [CrossRef]
- Colavolpe, G.; Foggi, T.; Forestieri, E.; Secondini, M. Impact of Phase Noise and Compensation Techniques in Coherent Optical Systems. J. Lightwave Technol. 2011, 29, 2790–2800. [Google Scholar] [CrossRef] [Green Version]
- Jin, C.; Shevchenko, N.A.; Li, Z.; Popov, S.; Chen, Y.; Xu, T. Nonlinear Coherent Optical Systems in the Presence of Equalization Enhanced Phase Noise. J. Lightwave Technol. 2021, 39, 4646–4653. [Google Scholar] [CrossRef]
- Xu, T.; Karanov, B.; Shevchenko, N.; Lavery, D.; Liga, G.; Killey, R.I.; Bayvel, P. Digital nonlinearity compensation in high-capacity optical communication systems considering signal spectral broadening effect. Sci. Rep. 2017, 7, 12986. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Forzati, M.; Berntson, A.; Martensson, J. Asynchronous Phase Modulation for the Suppression of IFWM. J. Lightwave Technol. 2007, 25, 2969–2975. [Google Scholar] [CrossRef]
- Du, J.; Teng, Z.; Shen, N. Semi-analytic modeling of FWM noise in Dispersion-managed DWDM systems with DQPSK/DPSK/OOK channels. Opt. Commun. 2016, 358, 180–189. [Google Scholar] [CrossRef]
- Jiang, L.; Yuan, X.; Cui, Y.; Chen, G.; Zuo, F.; Jiang, C. Optical bistability and four-wave mixing in a hybrid optomechanical system. Phys. Lett. A 2017, 381, 3289–3294. [Google Scholar] [CrossRef]
- Alsowaidi, N.; Eltaif, T.; Mokhtar, M.; Hamida, B.A. Reduction of Four-Wave Mixing in DWDM System Using Electro-Optic Phase Modulator. Int. J. Electr. Comput. Eng. 2018, 8, 2384–2389. [Google Scholar] [CrossRef]
- Lawan, S.; Mohammad, A. Reduction of four wave mixing efficiency in DWDM systems using optimal PMD. Opt. Quantum Electron. 2018, 50, 91. [Google Scholar] [CrossRef]
- Alsowaidi, N.; Eltaif, T.; Mokhtar, M. Suppression of Inter and Intra Channel Four Wave Mixing effect in Optical CDMA over DWDM Hybrid System. Chin. Opt. 2019, 12, 156–166. [Google Scholar] [CrossRef]
- Winzer, P.J.; Essiambre, R.J. Advanced Optical Modulation Formats. Proc. IEEE 2006, 94, 952–985. [Google Scholar] [CrossRef]
- Forzati, M. Phase Modulation Techniques for On-Off Keying Transmission. In Proceedings of the 9th International Conference on Transparent Optical Networks, Rome, Italy, 1–5 July 2007. [Google Scholar]
- Sharan, L.; Shanbhag, A.G.; Chaubey, V.K. Design and simulation of modified duobinary modulated 40 Gbps 32 channel DWDM optical link for improved non-linear performance. Cogent Eng. 2016, 3, 1256562. [Google Scholar] [CrossRef]
- Kassa-Baghdouche, L.; Simohamed, L.M. Performance limitations of an optical RZ-DPSK transmission system affected by frequency chirp, chromatic dispersion and polarization mode dispersion. In Proceedings of the International Workshop on Systems, Signal Processing and their Applications (WOSSPA), Tipaza, Algeria, 9–11 May 2011. [Google Scholar]
- Baghdouche, L.K.; Simohamed, L.M. Interferometer phase error and chromatic dispersion effects on the performances of 40 Gbit/s optical DPSK transmission systems. In Proceedings of the 8th International Workshop on Systems, Signal Processing and their Applications (WoSSPA), Algiers, Algeria, 12–15 May 2013. [Google Scholar]
- Linlin, B.; Jianming, L.; Li, L.; Xuecheng, Z. Comprehensive assessment of new modulation techniques in 40 Gb/s optical communication systems. In Proceedings of the 3rd International Photonics & Optoelectronics Meetings, Wuhan, China, 2–5 November 2010; IOP Publishing: Bristol, UK, 2011; Volume 276, pp. 1–6. [Google Scholar]
- Malhotra, J.; Kumar, M. Performance analysis of NRZ, RZ, CRZ and CSRZ data formats in 10Gb/s optical soliton transmission link under the impact of chirp and TOD. Opt. Int. J. Light Electron Opt. 2010, 121, 800–807. [Google Scholar] [CrossRef]
- Cao, H.; Atai, J.; Yu, Y.; Xiong, B.; Zhou, Y.; Cai, J.; Shu, X. Carrier-suppressed return-to-zero to non-return-to-zero format conversion based on a single fiber Bragg grating with knife-shaped spectra. Appl. Opt. 2014, 53, 5649. [Google Scholar] [CrossRef] [PubMed]
- Cheng, K.; Conradi, J. Reduction of pulse-to-pulse interaction using alternative RZ formats in 40-Gb/s systems. IEEE Photon. Technol. Lett. 2002, 14, 98–100. [Google Scholar] [CrossRef]
- Abbas, H.; Gregory, M.; Austin, M. A New Prime Code for Synchronous Optical Code Division Multiple-Access Networks. J. Comput. Netw. Commun. 2018, 2018, 3192520. [Google Scholar] [CrossRef] [Green Version]
- Yadav, R.; Kaur, G. Design and performance analysis of 1D, 2D and 3D prime sequence code family for optical CDMA network. J. Opt. 2016, 45, 343–356. [Google Scholar] [CrossRef]
- Abd, T.; Aljunid, S.; Fadhil, H.; Junita, M.; Saad, M. Impact of Multi-Diagonal Code on High-Speed Spectral Amplitude Coding Optical Code Division Multiple-Access Networks. Arab. J. Sci. Eng. 2013, 38, 2389–2397. [Google Scholar] [CrossRef]
- Agrawal, G.P. Applications of Nonlinear Fiber Optics, 2nd ed.; Academic Press: Amsterdam, The Netherlands, 2008; pp. 319–321. [Google Scholar]
- Agrawal, G.P. Nonlinear Fiber Optics, 5th ed.; Academic Press: Amsterdam, The Netherlands, 2013; pp. 397–457. [Google Scholar]
- Ramaswami, R.; Sivarajan, K.; Sasaki, G. Optical Networks, 3rd ed.; Elsevier/Morgan Kaufmann: Amsterdam, The Netherlands, 2010; pp. 264–320. [Google Scholar]
- Alsowaidi, N.; Eltaif, T.; Mokhtar, M.R. Performance analysis of a Hybrid Optical CDMA/DWDM System against Inter-Symbol Interference and Four Wave Mixing. Digit. Commun. Netw. 2021, 7, 151–156. [Google Scholar] [CrossRef]
- Gnauck, A.H. Advanced Amplitude- and Phase Coded Formats for 40-Gb/s Fiber Transmission. In Proceedings of the IEEE/LEOS Annual Meeting, Rio Grande, PR, USA, 11–12 November 2004. [Google Scholar]
- Sheetal, A.; Sharma, A.; Kaler, R.S. Impact of optical modulation formats on SPM-limited fiber transmission in 10 and 40 Gb/s optimum dispersion-managed lightwave systems. Optik 2010, 121, 246–252. [Google Scholar] [CrossRef]

| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Number of channels | N = 15 | Dispersion slope for SMF | 0.075 ps/nm2·km |
| Number of users per channel | K = 8 | Cross effective area for SMF | 80 μm2 |
| Channel spacing | 0.2 nm | Length of DCF | 15.075 km |
| Data rate per channel | 40 Gbps | Attenuation for DCF | 0.5 dB/km |
| Data rate per user | 5 Gbps | Dispersion for DCF | −100 ps/nm·km |
| CW DFB Laser launch power | 9.8 dBm | Dispersion slope for DCF | −0.45 ps/nm2·km |
| Total insertion losses | 30.8 dBm | Cross effective area for DCF | 22 μm2 |
| Preamplifier gain | 30 dB | Nonlinear refractive index | 2.6 × 10−20 m2/W |
| Total transmitted power to the fiber | 22 dBm | Max nonlinear phase shift | 3.14 mrad |
| Length of SMF | 90 km | Phase deviation of EOPM | |
| Attenuation for SMF | 0.2 dB/km | Length and weight of the sequence code | F = 24, w = 3 |
| Dispersion for SMF | 16.75 ps/nm·km | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alsowaidi, N.; Eltaif, T.; Mokhtar, M.R. Performance Comparison of Different Modulation Formats for a 40 Gbps Hybrid Optical CDMA/DWDM System against ISI and FWM. Photonics 2022, 9, 555. https://doi.org/10.3390/photonics9080555
Alsowaidi N, Eltaif T, Mokhtar MR. Performance Comparison of Different Modulation Formats for a 40 Gbps Hybrid Optical CDMA/DWDM System against ISI and FWM. Photonics. 2022; 9(8):555. https://doi.org/10.3390/photonics9080555
Chicago/Turabian StyleAlsowaidi, Naif, Tawfig Eltaif, and Mohd Ridzuan Mokhtar. 2022. "Performance Comparison of Different Modulation Formats for a 40 Gbps Hybrid Optical CDMA/DWDM System against ISI and FWM" Photonics 9, no. 8: 555. https://doi.org/10.3390/photonics9080555
APA StyleAlsowaidi, N., Eltaif, T., & Mokhtar, M. R. (2022). Performance Comparison of Different Modulation Formats for a 40 Gbps Hybrid Optical CDMA/DWDM System against ISI and FWM. Photonics, 9(8), 555. https://doi.org/10.3390/photonics9080555





