Ultra-Low-Loss and Broadband All-Silicon Dielectric Waveguides for WR-1 Band (0.75–1.1 THz) Modules
Abstract
:1. Introduction
2. Materials and Method—All-Si Dielectric Waveguides
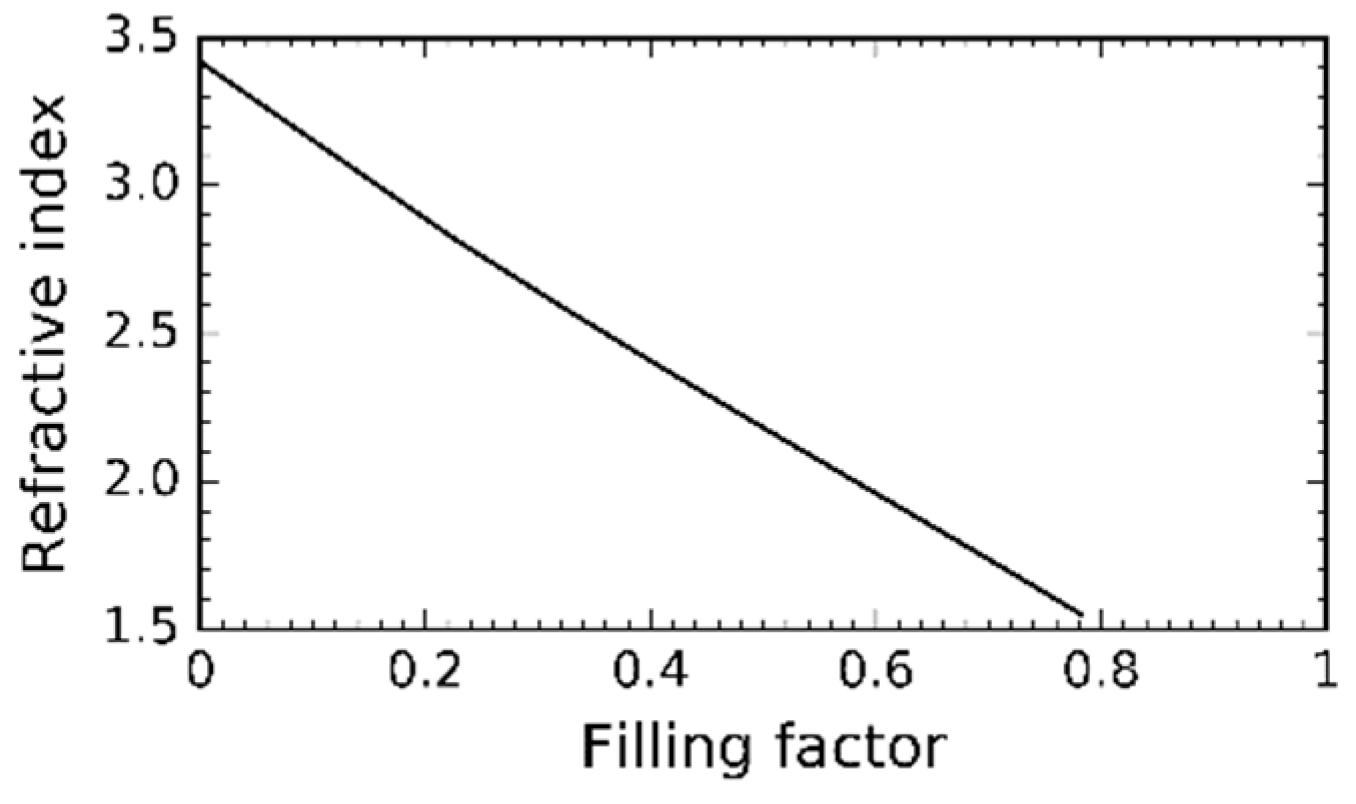
2.1. Design
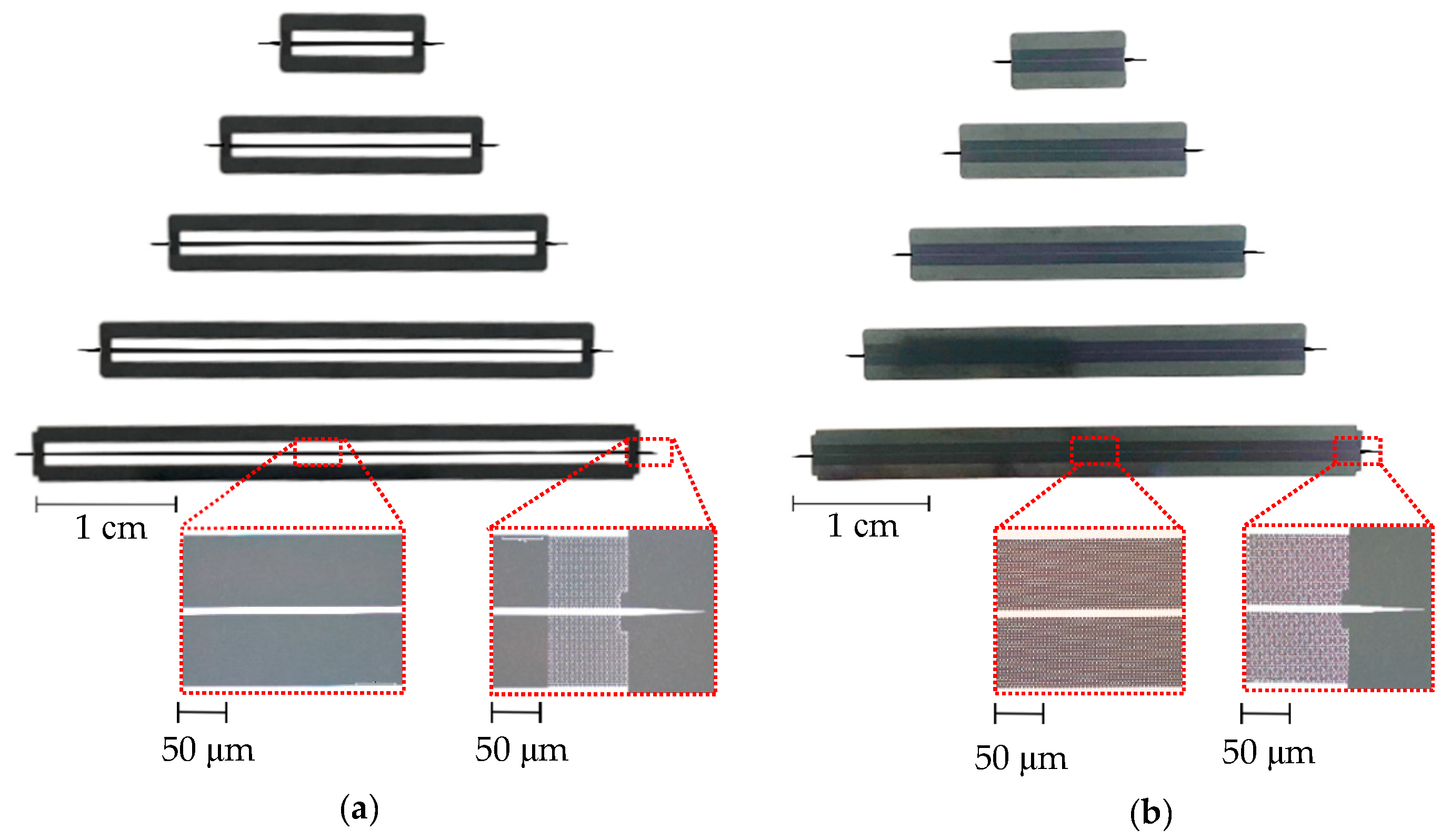
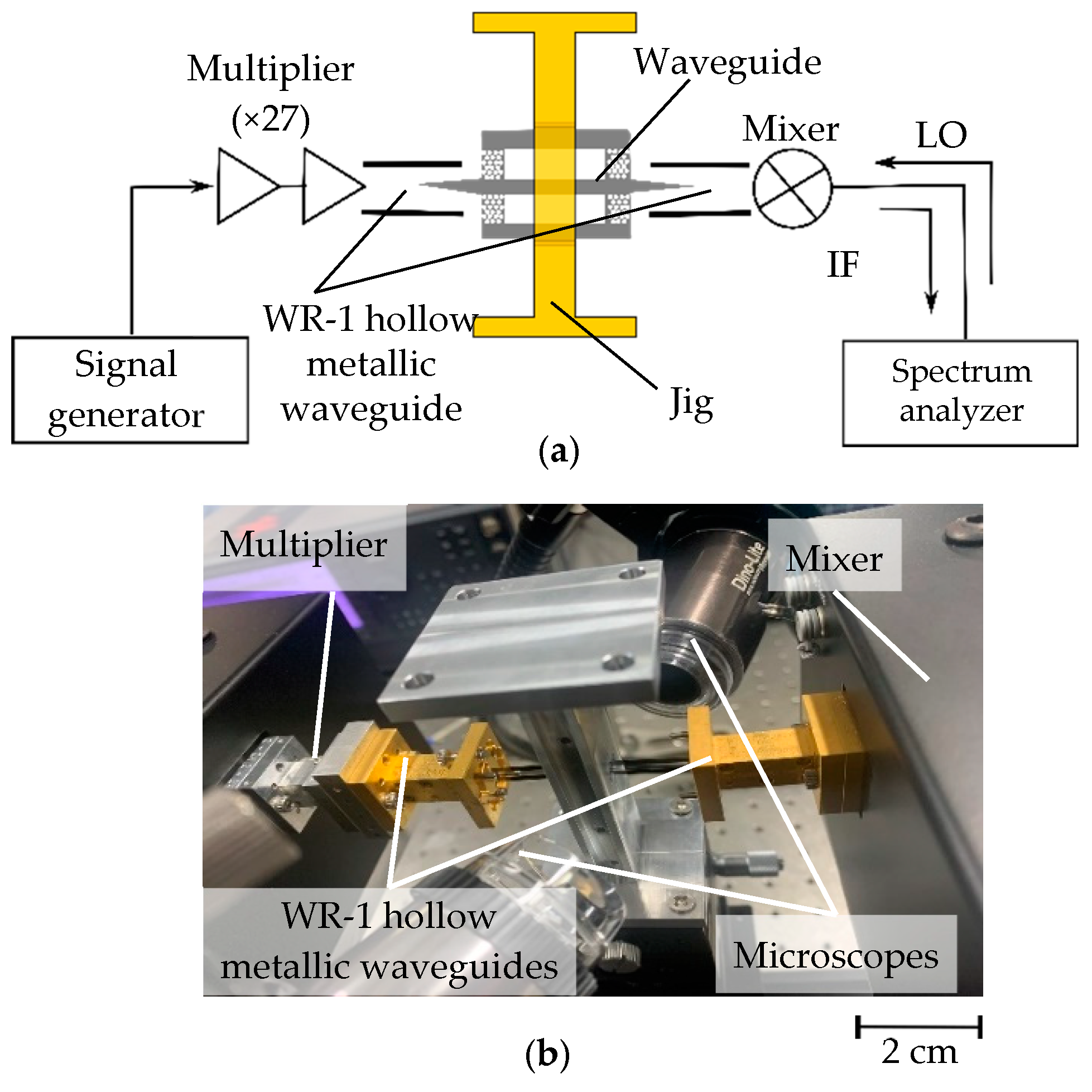
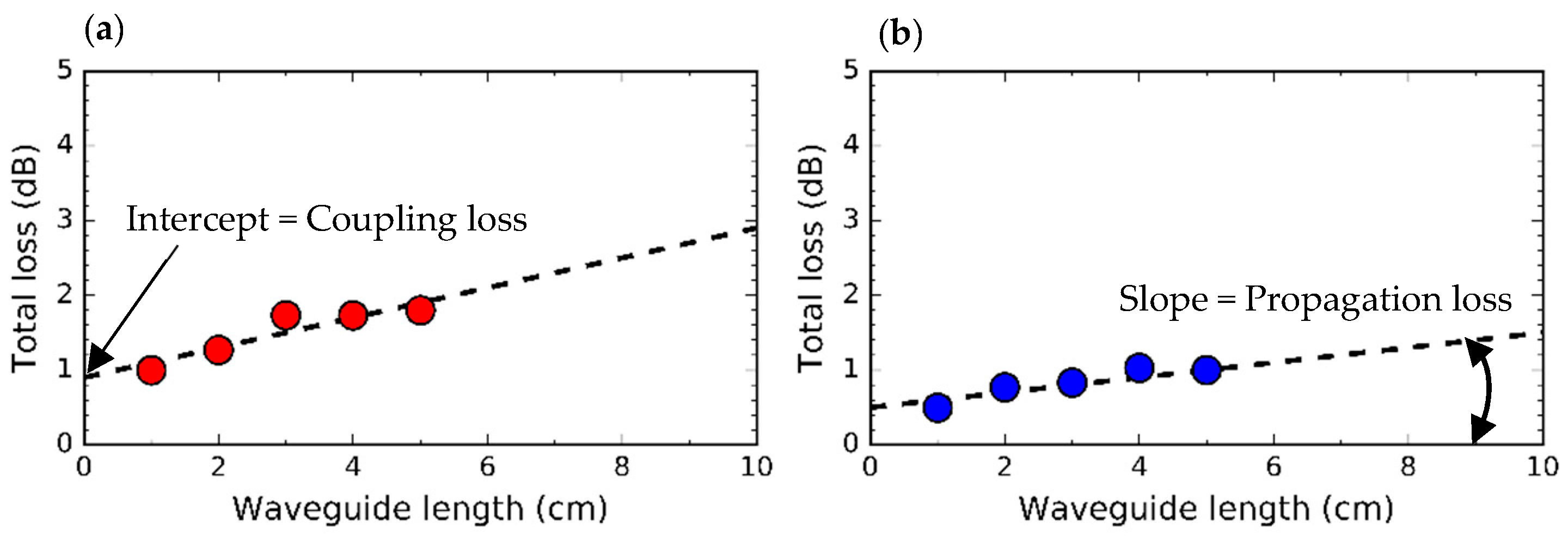
2.2. Fabrication and Experimental Validation
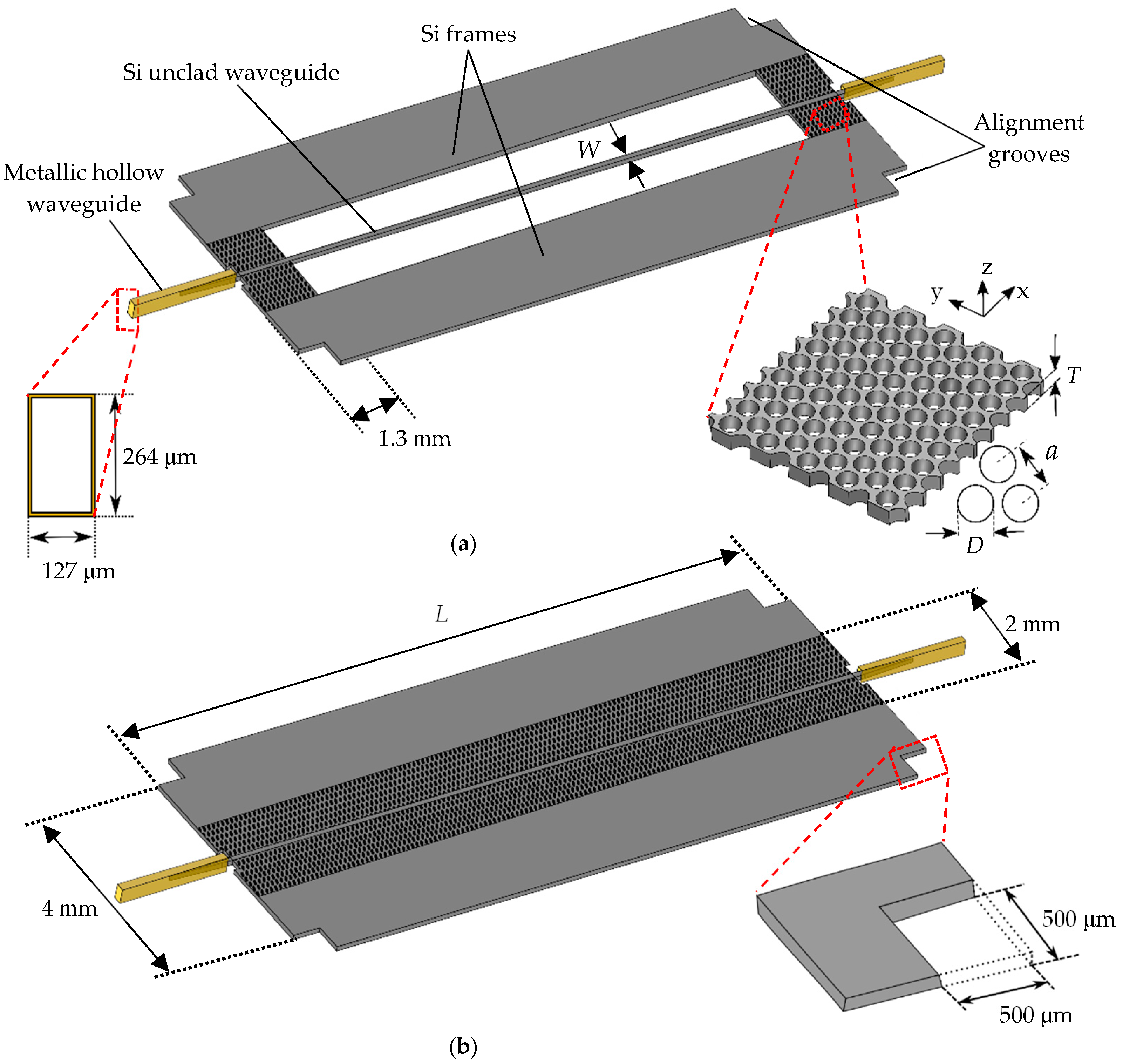
3. All-Silicon Dielectric Waveguide Modules
3.1. Concept
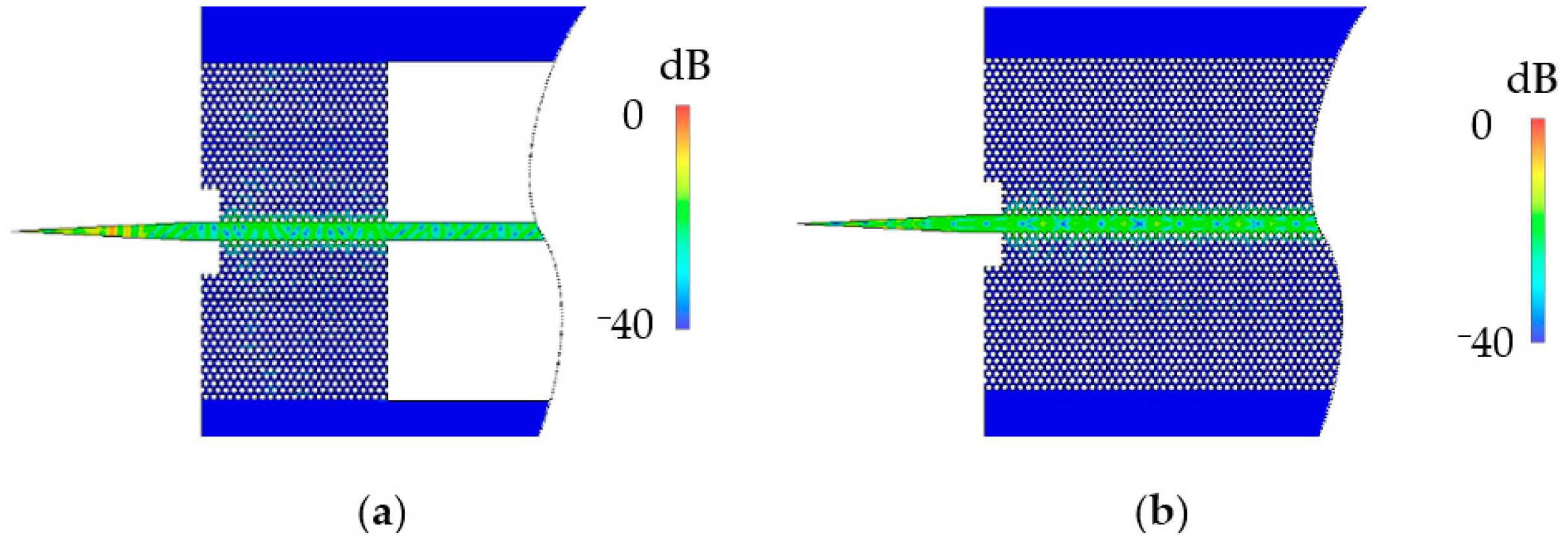
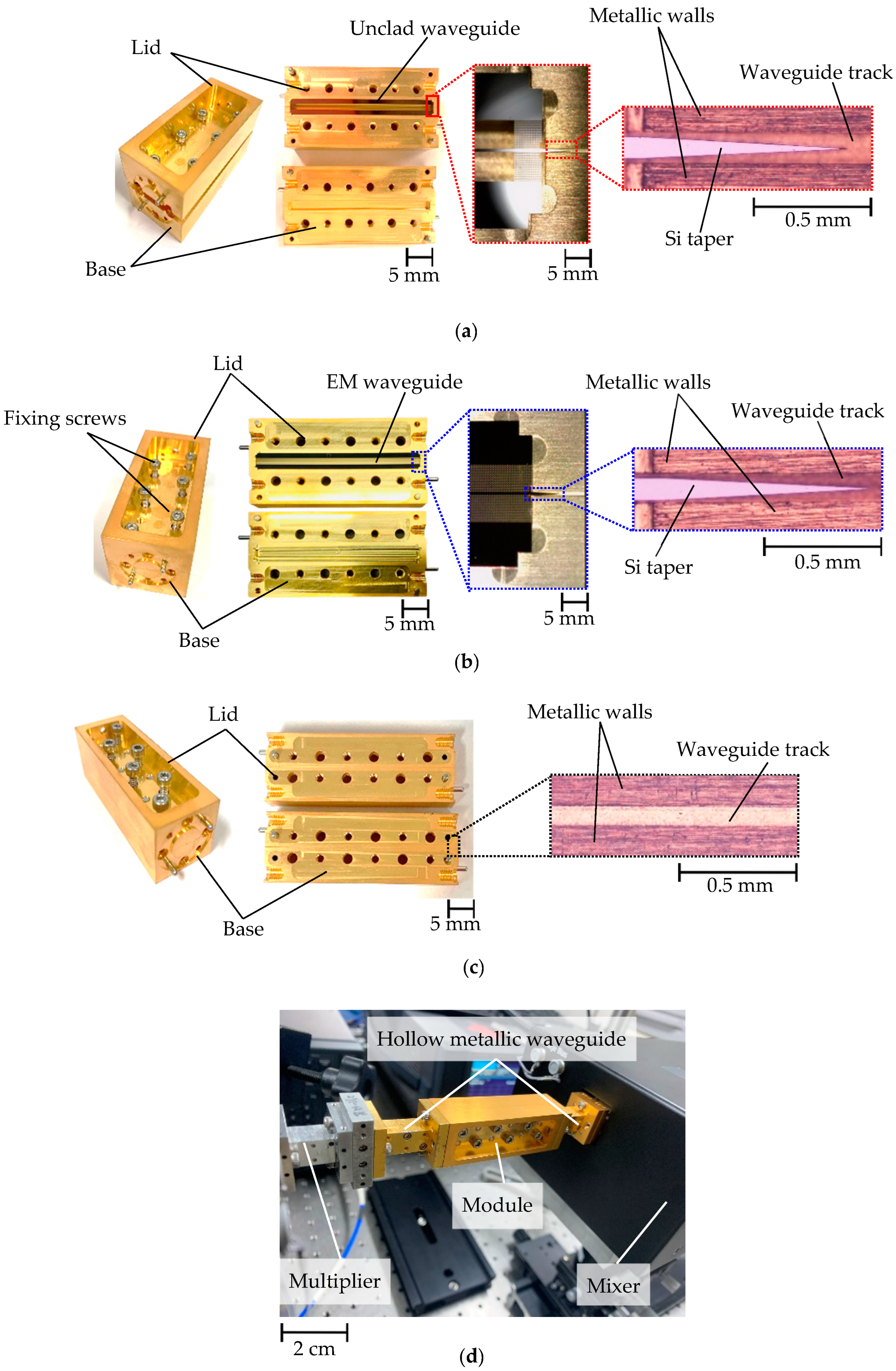
3.2. Unclad Waveguide and Effective-Medium Waveguide Modules
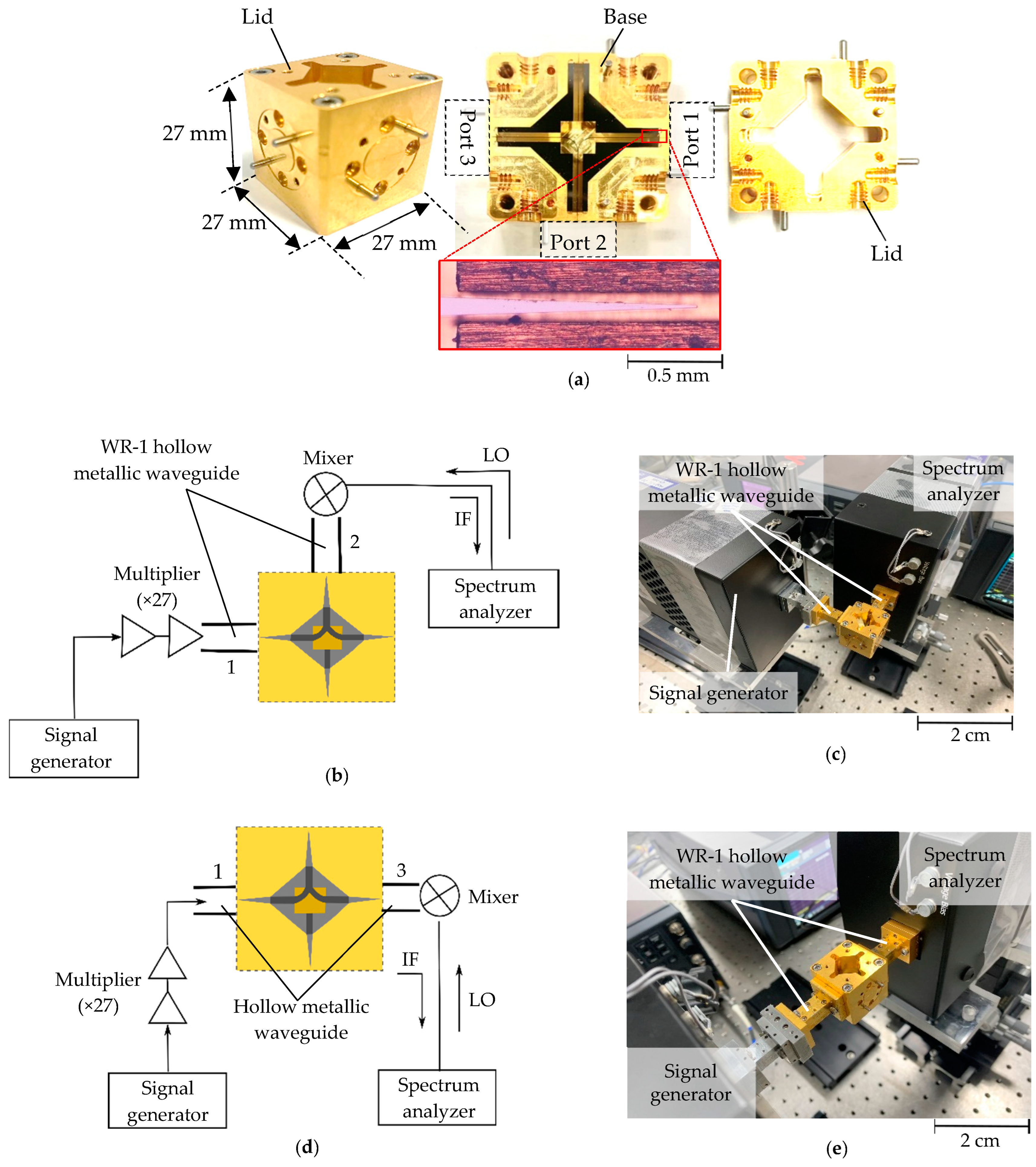
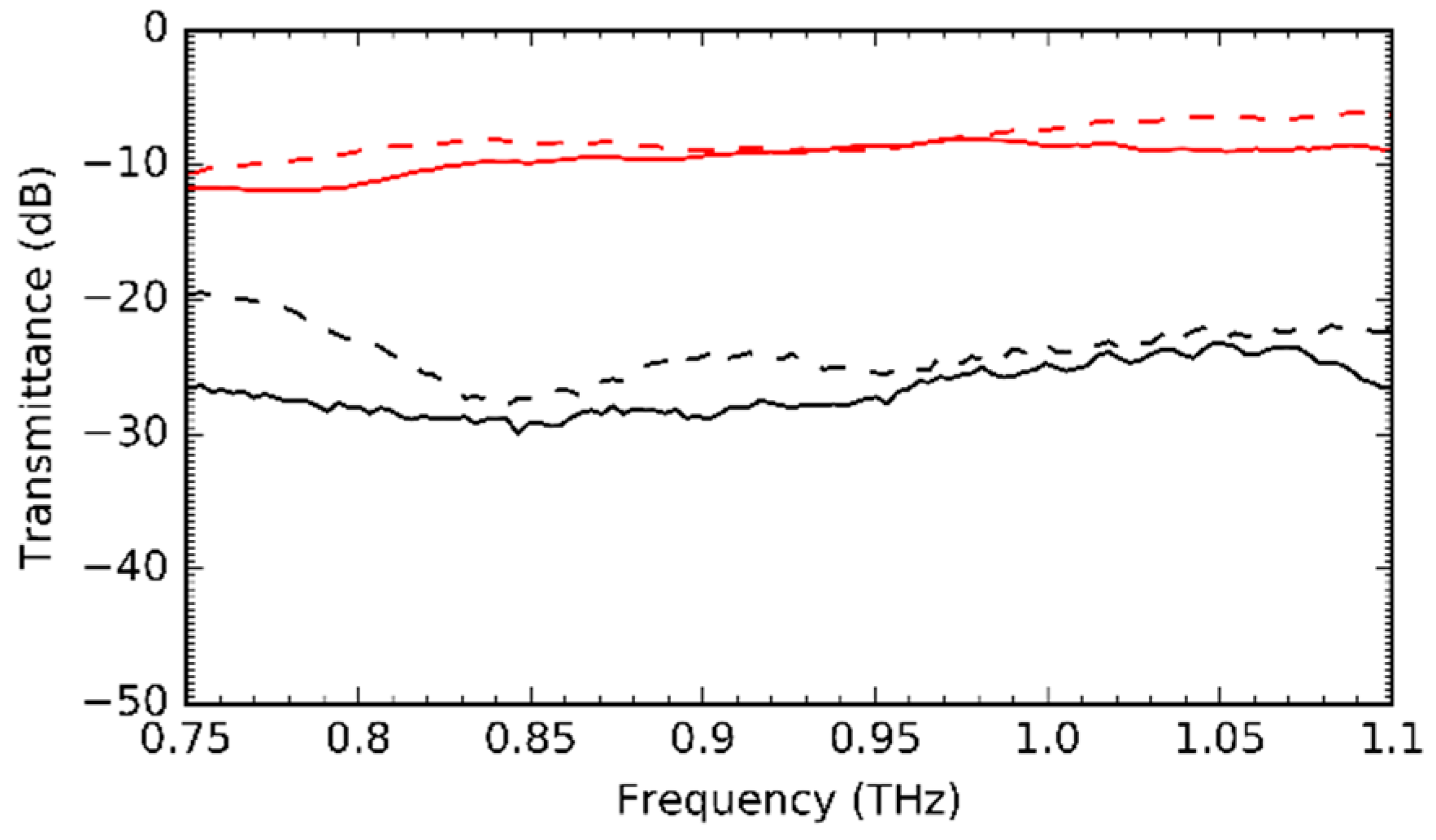
3.3. Y-Junction Module
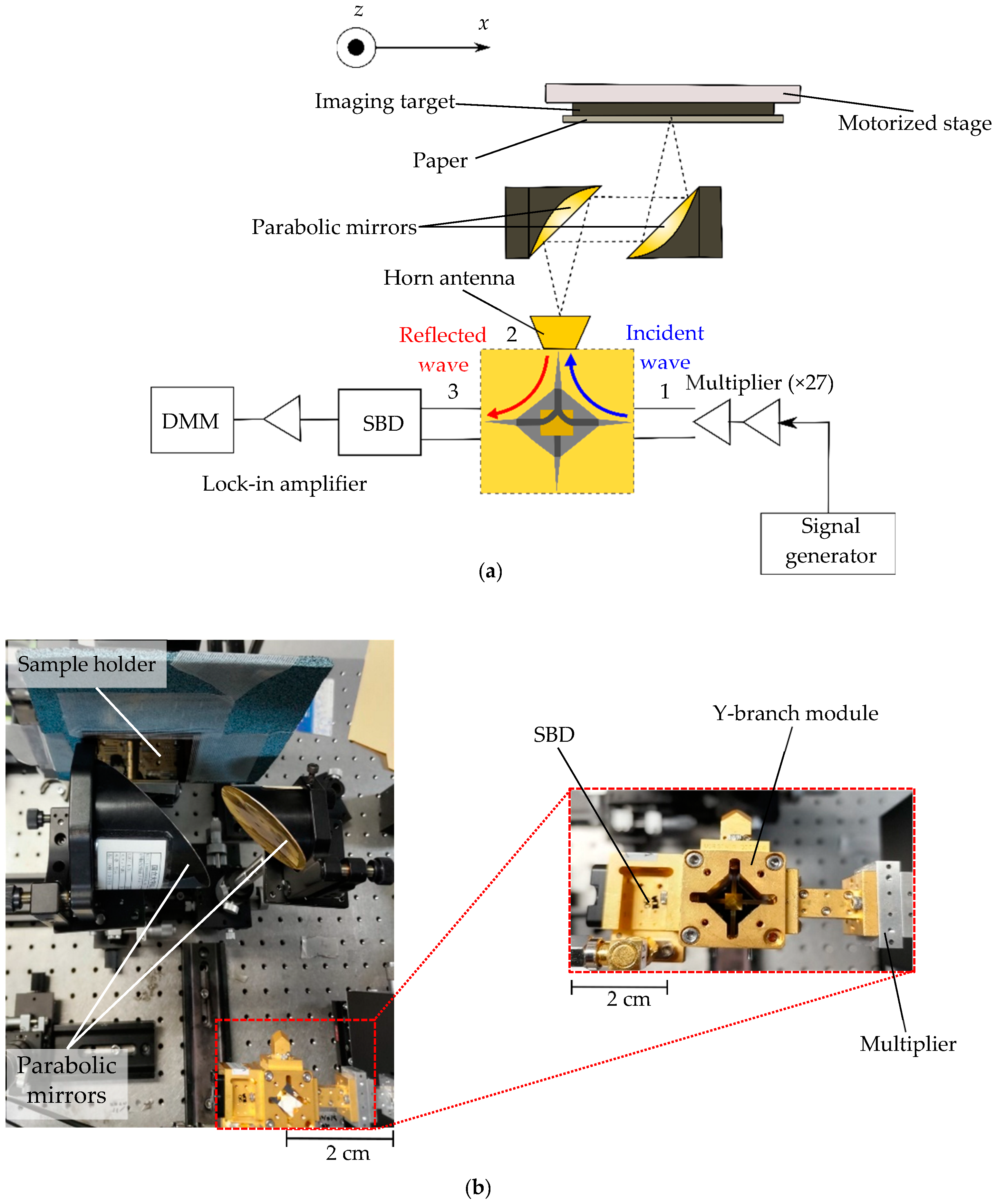
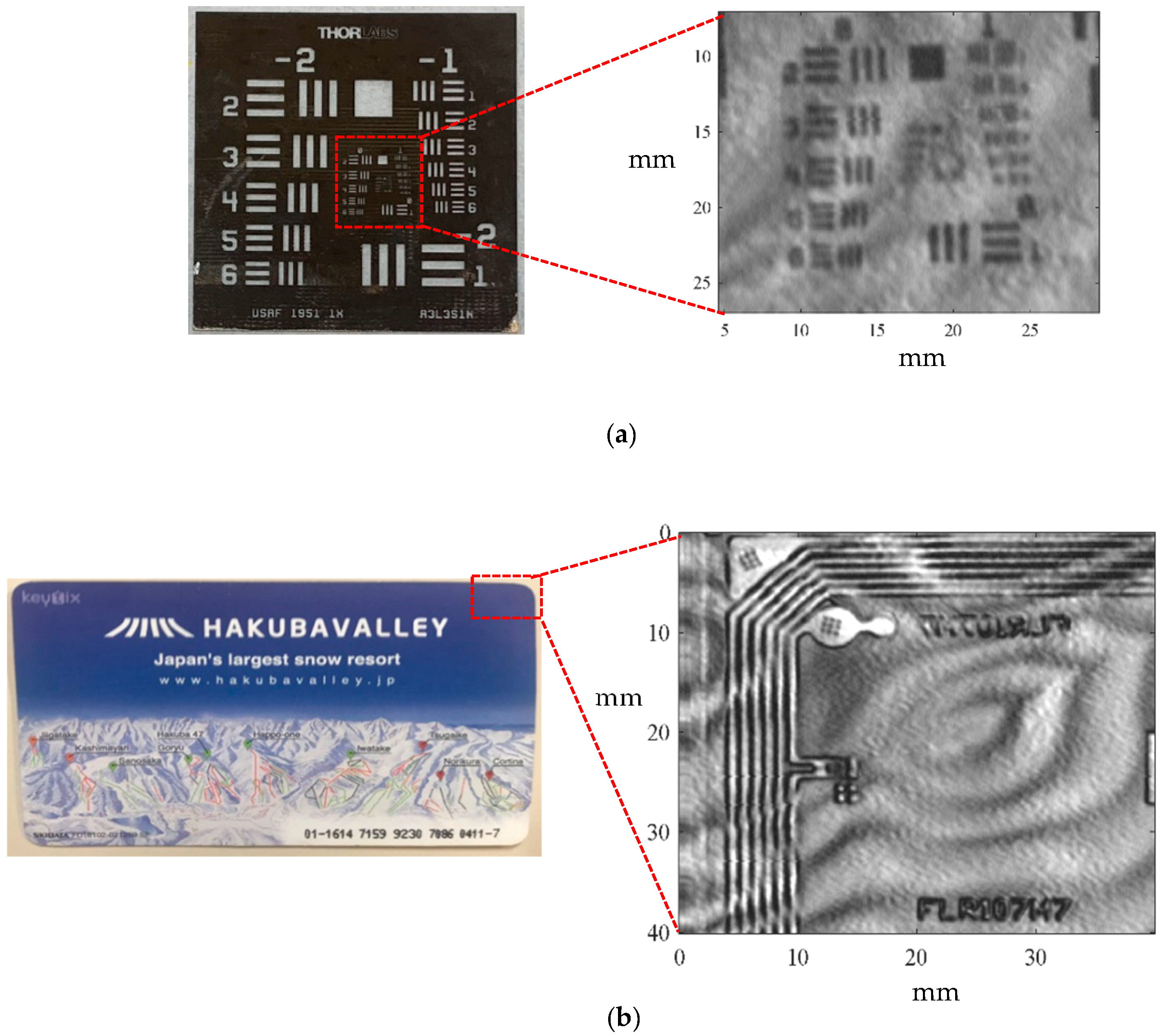
4. Imaging Application
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Koala, R.A.S.D.; Fujita, M.; Nagatsuma, T. Nanophotonics-inspired all-silicon waveguide platforms for terahertz integrated systems. Nanophotonics 2022, 11, 1741–1759. [Google Scholar] [CrossRef]
- Webber, J.; Yamagami, Y.; Ducournau, G.; Szriftgiser, P.; Iyoda, K.; Fujita, M.; Nagatsuma, T.; Singh, R. Terahertz band communications with topological valley photonic crystal waveguide. J. Light. Technol. 2021, 39, 7609–7620. [Google Scholar] [CrossRef]
- Yi, L.; Nishida, Y.; Sagisaka, T.; Kaname, R.; Mizuno, R.; Fujita, M.; Nagatsuma, T. Towards practical terahertz imaging system with compact continuous wave transceiver. J. Light. Technol. 2021, 39, 7850–7861. [Google Scholar] [CrossRef]
- Shiode, T.; Mukai, T.; Kawamura, M.; Nagatsuma, T. Giga-bit wireless communication at 300 GHz using resonant tunneling diode detector. In Proceedings of the Asia-Pacific Microwave Conference Proceedings, APMC, Melbourne, VIC, Australia, 5–8 December 2011. [Google Scholar]
- Shibata, N.; Uemura, Y.; Kawamoto, Y.; Yi, L.; Fujita, M.; Nagatsuma, T. Silicon dielectric diplexer module for 600-GHz-band frequency-division multiplexing wireless communication. IEEE Trans. Terahertz Sci. Technol. 2022, 12, 334–344. [Google Scholar] [CrossRef]
- De Maagt, P.; Bolivar, P.H.; Mann, C. Terahertz science, engineering and systems-from space to earth applications. In Encyclopedia of RF and Microwave Engineering; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2005; Volume 2, pp. 3–10. [Google Scholar]
- Dickinson, J.C.; Goyette, T.M.; Gatesman, A.J.; Joseph, C.S.; Root, Z.G.; Giles, R.H.; Waldman, J.; Nixon, W.E. Terahertz imaging of subjects with concealed weapons. Terahertz Mil. Secur. Appl. IV 2006, 6212, 62120Q. [Google Scholar] [CrossRef] [Green Version]
- Siegel, P. Terahertz technology in biology and medicine. IEEE Trans. Microw. Theory Tech. 2004, 52, 2438–2447. [Google Scholar] [CrossRef]
- Okamoto, K.; Tsuruda, K.; Diebold, S.; Hisatake, S.; Fujita, M.; Nagatsuma, T. Terahertz sensor using photonic crystal cavity and resonant tunneling diodes. J. Infrared Millim. Terahertz Waves 2017, 38, 1085–1097. [Google Scholar] [CrossRef]
- Tsuruda, K.; Okamoto, K.; Diebold, S.; Hisatake, S.; Fujita, M.; Nagatsuma, T. Terahertz sensing based on photonic crystal cavity and resonant tunneling diode. In Proceedings of the 2016 Progress in Electromagnetic Research Symposium (PIERS), Shanghai, China, 8–11 August 2016; pp. 3922–3926. [Google Scholar] [CrossRef]
- Diebold, S.; Nishio, K.; Nishida, Y.; Kim, J.; Tsuruda, K.; Mukai, T.; Fujita, M.; Nagatsuma, T. High-speed error-free wireless data transmission using a terahertz resonant tunnelling diode transmitter and receiver. Electron. Lett. 2016, 52, 1999–2001. [Google Scholar] [CrossRef]
- Song, H.J.; Nagatsuma, T. Handbook of Terahertz Technologies: Devices and Applications, 1st ed.; Pan Stanford: Singapore, 2015. [Google Scholar]
- Yang, S.-H.; Jarrahi, M. Navigating terahertz spectrum via photomixing. Opt. Photonics News 2020, 31, 36–43. [Google Scholar] [CrossRef]
- Schroder, D.; Thomas, R.; Swartz, J. Free carrier absorption in silicon. IEEE J. Solid-State Circuits 1978, 13, 180–187. [Google Scholar] [CrossRef]
- Williams, G.P. Filling the THz gap—High power sources and applications. Rep. Prog. Phys. 2005, 69, 301–326. [Google Scholar] [CrossRef]
- Yu, X.; Sugeta, M.; Yamagami, Y.; Fujita, M.; Nagatsuma, T. Simultaneous low-loss and low-dispersion in a photonic-crystal waveguide for terahertz communications. Appl. Phys. Express 2019, 12, 012005. [Google Scholar] [CrossRef]
- Tsuruda, K.; Fujita, M.; Nagatsuma, T. Extremely low-loss terahertz waveguide based on silicon photonic-crystal slab. Opt. Express 2015, 23, 31977–31990. [Google Scholar] [CrossRef]
- Withayachumnankul, W.; Fujita, M.; Nagatsuma, T. Integrated silicon photonic crystals toward terahertz communications. Adv. Opt. Mater. 2018, 6, 1800401. [Google Scholar] [CrossRef] [Green Version]
- TorresGarcia, A.E.; Escudero, J.M.M.P.; Teniente, J.; Gonzalo, R.; Ederra, I. Silicon integrated subharmonic mixer on a photonic-crystal platform. IEEE Trans. Terahertz Sci. Technol. 2021, 11, 79–89. [Google Scholar] [CrossRef]
- Koala, R.; Headland, D.; Yu, X.; Nishida, Y.; Fujita, M.; Nagatsuma, T. Terahertz RTD chip backside-coupled to photonic-crystal waveguide. In Proceedings of the 2021 46th International Conference on Infrared, Millimeter and Terahertz Waves (IRMMW-THz), Chengdu, China, 29 August–3 September 2021; Volume 2021, pp. 1–2. [Google Scholar] [CrossRef]
- Yu, X.; Hosoda, Y.; Miyamoto, T.; Obata, K.; Kim, J.; Fujita, M.; Nagatsuma, T. Terahertz fibre transmission link using resonant tunnelling diodes integrated with photonic-crystal waveguides. Electron. Lett. 2019, 55, 398–400. [Google Scholar] [CrossRef]
- Gao, W.; Yu, X.; Fujita, M.; Nagatsuma, T.; Fumeaux, C.; Withayachumnankul, W.; Xiongbin, Y. Effective-medium-cladded dielectric waveguides for terahertz waves. Opt. Express 2019, 27, 38721–38734. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Headland, D.; Withayachumnankul, W.; Yu, X.; Fujita, M.; Nagatsuma, T. Unclad microphotonics for terahertz waveguides and systems. J. Light. Technol. 2020, 38, 6853–6862. [Google Scholar] [CrossRef]
- Subashiev, A.; Luryi, S. Modal control in semiconductor optical waveguides with uniaxially patterned layers. J. Light. Technol. 2006, 24, 1513–1522. [Google Scholar] [CrossRef] [Green Version]
- Kawamoto, Y.; Shibata, N.; Uemura, Y.; Iwamatsu, S.; Nishida, Y.; Fujita, M.; Nagatsuma, T. Integrated resonant tunneling diode with rectangular wave guide I/O using photonic crystal interface. In Proceedings of the 2021 46th International Conference on Infrared, Millimeter and Terahertz Waves (IRMMW-THz), Chengdu, China, 29 August–3 September 2021; Volume 2021, pp. 1–2. [Google Scholar] [CrossRef]
- Shibata, N.; Uemura, Y.; Kawamoto, Y.; Yi, L.; Fujita, M.; Nagatsuma, T. 600-GHz-band silicon dielectric waveguide module. In Proceedings of the 2021 46th International Conference on Infrared, Millimeter and Terahertz Waves (IRMMW-THz), Chengdu, China, 29 August–3 September 2021; Volume 2021, pp. 1–2. [Google Scholar] [CrossRef]
- Headland, D.; Fujita, M.; Nagatsuma, T. Bragg-mirror suppression for enhanced bandwidth in terahertz photonic crystal waveguides. IEEE J. Sel. Top. Quantum Electron. 2020, 26, 4900109. [Google Scholar] [CrossRef]
- Koala, R.A.S.D.; Headland, D.; Yamagami, Y.; Masayuki, F.; Nagatsuma, T. Broadband terahertz dielectric rod antenna array with integrated half-Maxwell fisheye lens. In Proceedings of the 2020 International Topical Meeting on Microwave Photonics (MWP), Matsue, Japan, 24–26 November 2020; pp. 54–57. [Google Scholar] [CrossRef]
- Chutinan, A.; Noda, S. Waveguides and waveguide bends in two-dimensional photonic crystal slabs. Phys. Rev. B 2000, 62, 4488–4492. [Google Scholar] [CrossRef]
- Johnson, S.G.; Villeneuve, P.R.; Fan, S.; Joannopoulos, J.D. Linear waveguides in photonic-crystal slabs. Phys. Rev. B 2000, 62, 8212–8222. [Google Scholar] [CrossRef] [Green Version]
- Toccafondo, V.; García-Rupérez, J.; Bañuls, M.-J.; Griol, A.; Castelló, J.G.; Peransi-Llopis, S.; Maquieira, A. Single-strand DNA detection using a planar photonic-crystal-waveguide-based sensor. Opt. Lett. 2010, 35, 3673–3675. [Google Scholar] [CrossRef] [PubMed]
- Quast, H.; Loffler, T. 3D-terahertz-tomography for material inspection and security. In Proceedings of the 2009 34th International Conference on Infrared, Millimeter, and Terahertz Waves, Busan, Korea, 21–25 September 2009; pp. 1–2. [Google Scholar] [CrossRef]
- Withayachumnankul, W.; Yamada, R.; Fujita, M.; Nagatsuma, T. All-dielectric rod antenna array for terahertz communications. APL Photonics 2018, 3, 051707. [Google Scholar] [CrossRef] [Green Version]
- Headland, D.; Klein, A.K.; Fujita, M.; Nagatsuma, T. Dielectric slot-coupled half-Maxwell fisheye lens as octave-bandwidth beam expander for terahertz-range applications. APL Photonics 2021, 6, 096104. [Google Scholar] [CrossRef]
- Lee, W.S.L.; Nirantar, S.; Headland, D.; Bhaskaran, M.; Sriram, S.; Fumeaux, C.; Withayachumnankul, W. Broadband terahertz circular-polarization beam splitter. Adv. Opt. Mater. 2018, 6, 1700852. [Google Scholar] [CrossRef]
- Gao, W.; Lee, W.S.L.; Fumeaux, C.; Withayachumnankul, W. Effective-medium-clad Bragg grating filters. APL Photonics 2021, 6, 076105. [Google Scholar] [CrossRef]
- Sagisaka, T.; Kaname, R.; Kikuchi, M.; Kukutsu, N.; Headland, D.; Yi, L.; Fujita, M.; Nagatsuma, T. Integrated terahertz optics with effective medium for 600-GHz-band imaging. In Proceedings of the 2020 International Topical Meeting on Microwave Photonics (MWP), Matsue, Japan, 24–26 November 2020. [Google Scholar] [CrossRef]






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Koala, R.; Maru, R.; Iyoda, K.; Yi, L.; Fujita, M.; Nagatsuma, T. Ultra-Low-Loss and Broadband All-Silicon Dielectric Waveguides for WR-1 Band (0.75–1.1 THz) Modules. Photonics 2022, 9, 515. https://doi.org/10.3390/photonics9080515
Koala R, Maru R, Iyoda K, Yi L, Fujita M, Nagatsuma T. Ultra-Low-Loss and Broadband All-Silicon Dielectric Waveguides for WR-1 Band (0.75–1.1 THz) Modules. Photonics. 2022; 9(8):515. https://doi.org/10.3390/photonics9080515
Chicago/Turabian StyleKoala, Ratmalgre, Ryoma Maru, Kei Iyoda, Li Yi, Masayuki Fujita, and Tadao Nagatsuma. 2022. "Ultra-Low-Loss and Broadband All-Silicon Dielectric Waveguides for WR-1 Band (0.75–1.1 THz) Modules" Photonics 9, no. 8: 515. https://doi.org/10.3390/photonics9080515
APA StyleKoala, R., Maru, R., Iyoda, K., Yi, L., Fujita, M., & Nagatsuma, T. (2022). Ultra-Low-Loss and Broadband All-Silicon Dielectric Waveguides for WR-1 Band (0.75–1.1 THz) Modules. Photonics, 9(8), 515. https://doi.org/10.3390/photonics9080515




