Influence of Spatio-Temporal Couplings on Focused Optical Vortices
Abstract
:1. Introduction
2. Description of the Method
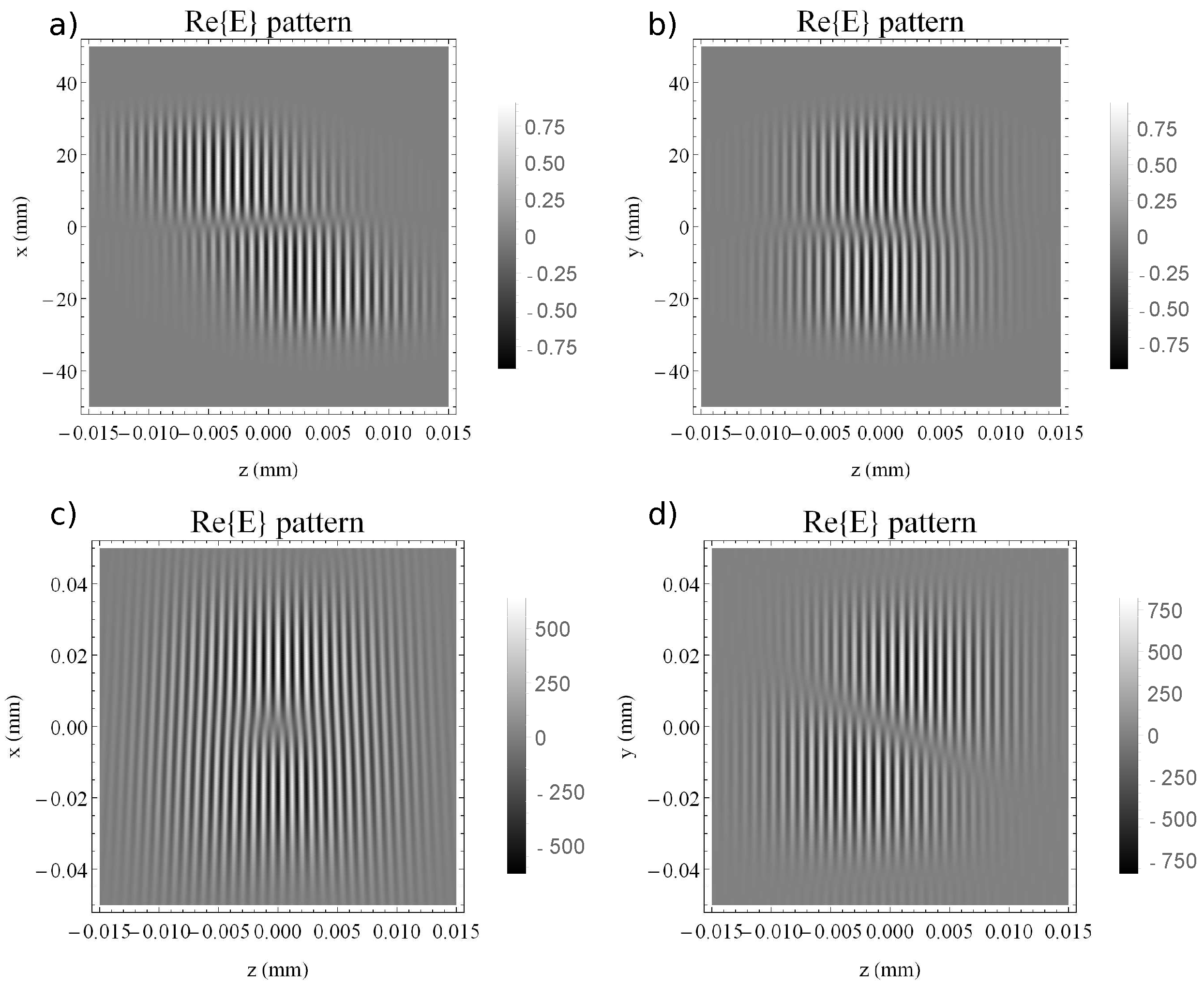
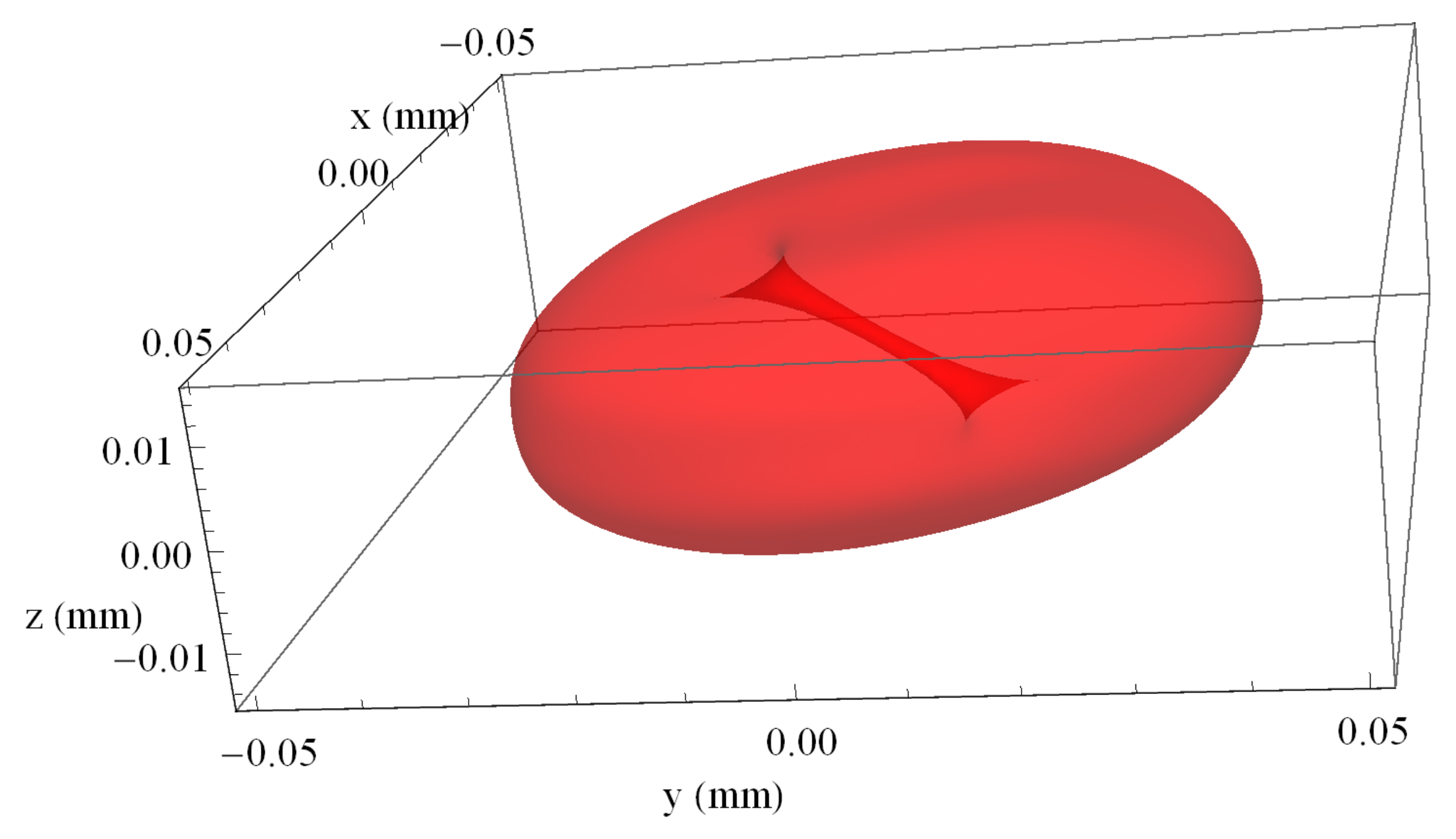
3. Results of the Simulations
3.1. Ultrashort Laser Fields with Helical Phases
3.1.1. Helical Mirror with Matched Step–Wavelength
3.1.2. Helical Mirror with Different Surface Steps
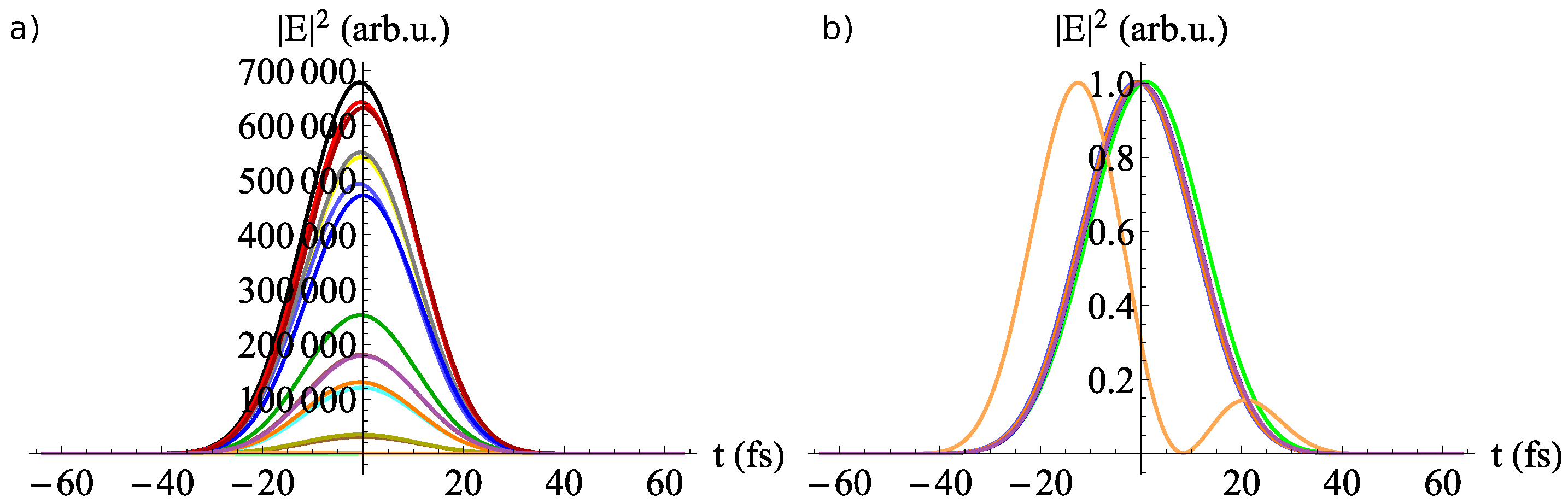
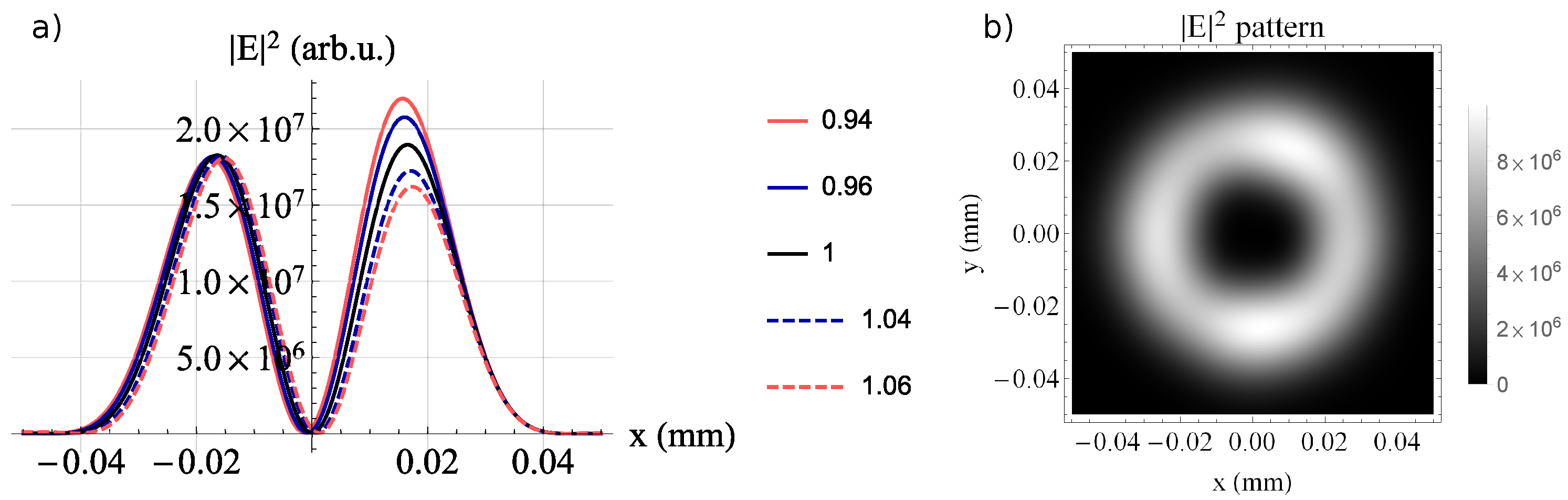
3.1.3. Chirped Laser Pulse and Helical Spatial Phase
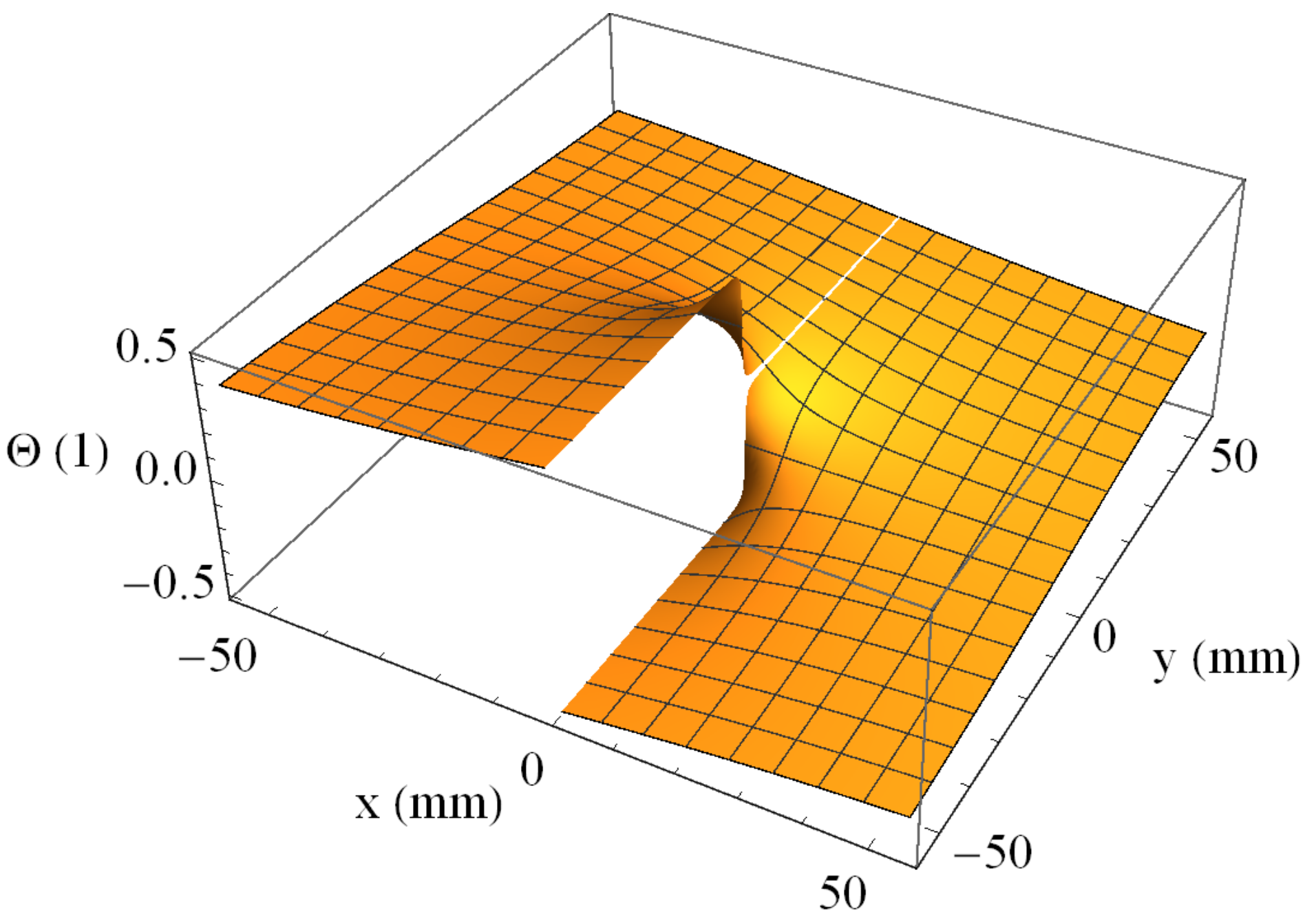
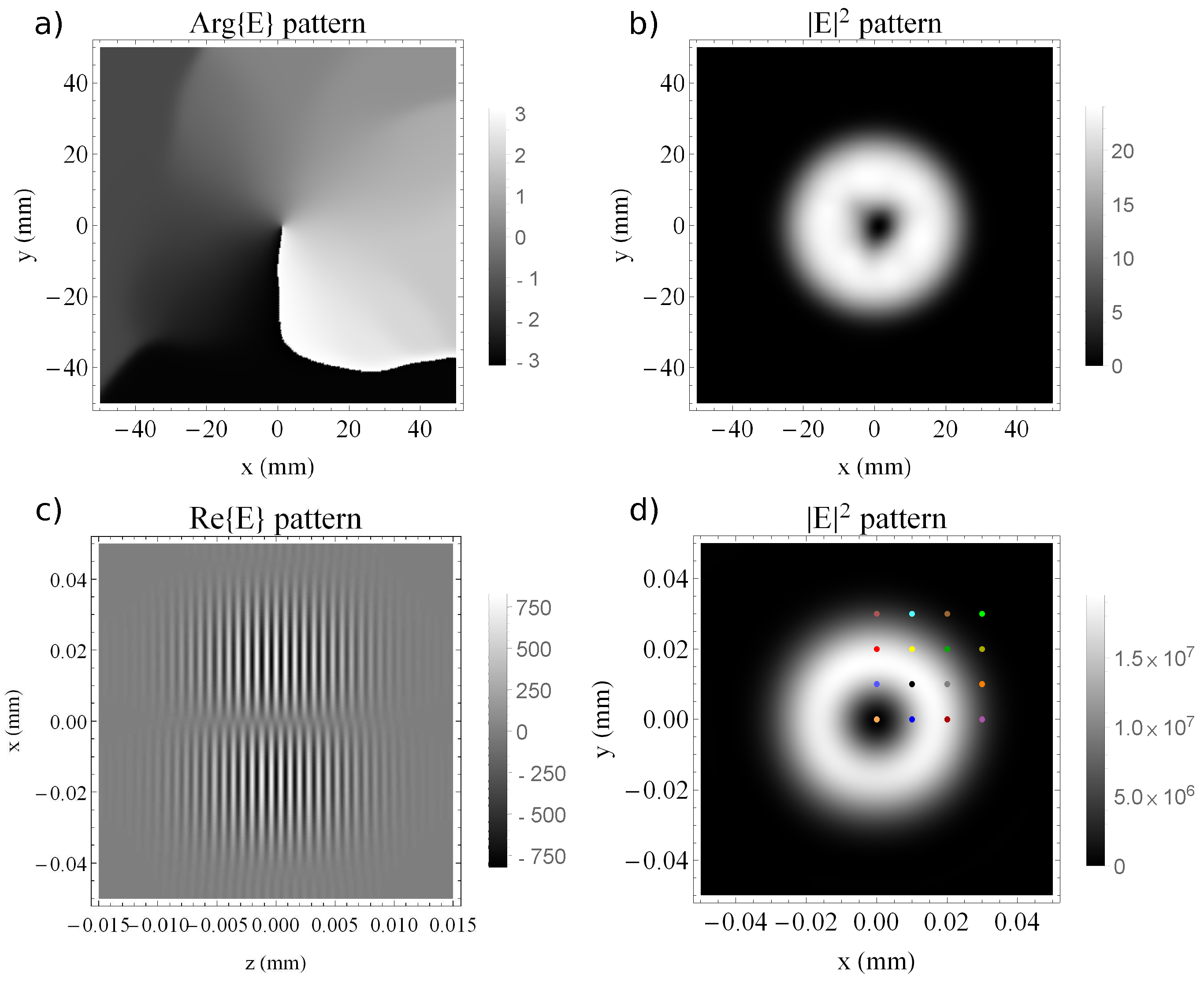
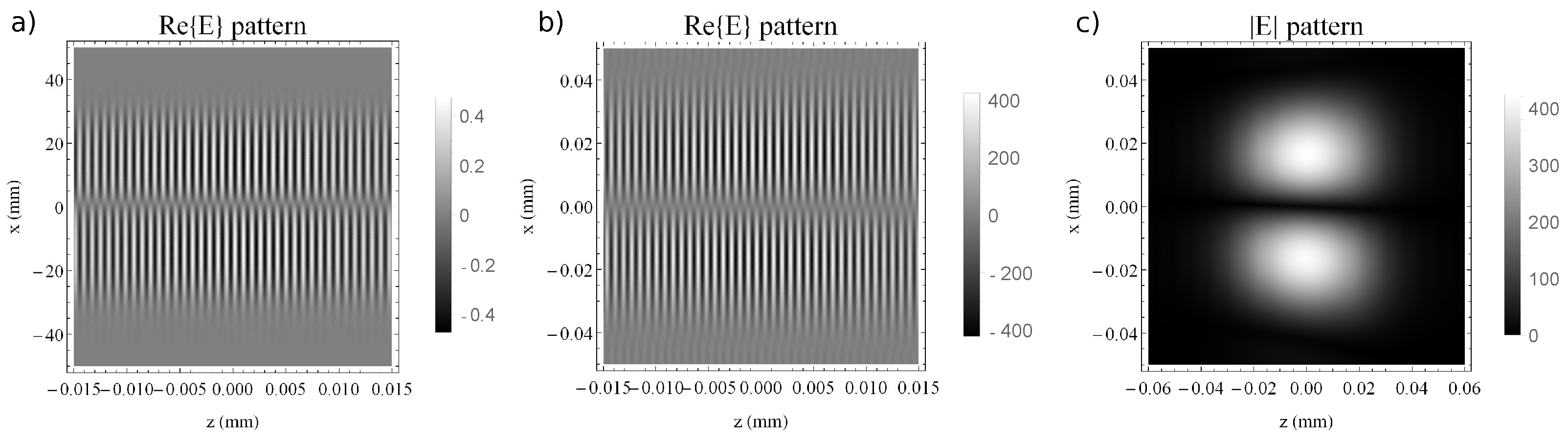
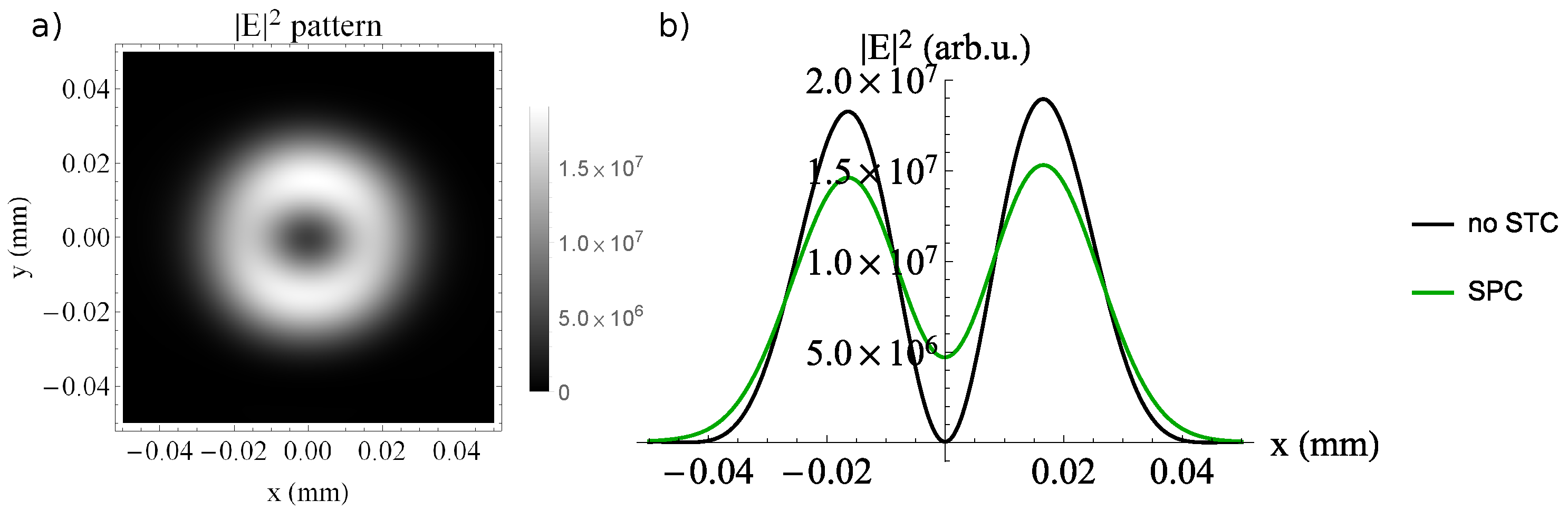
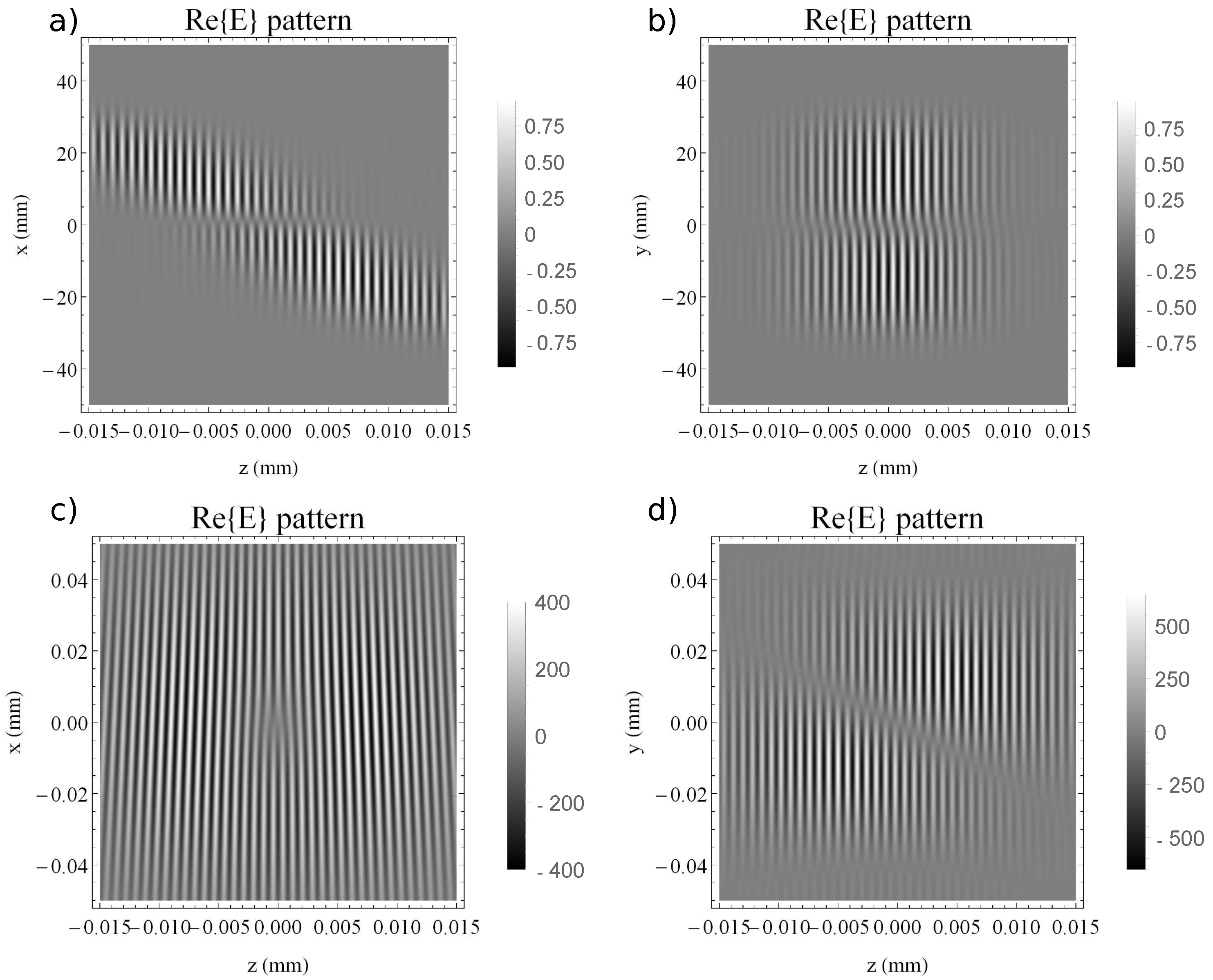
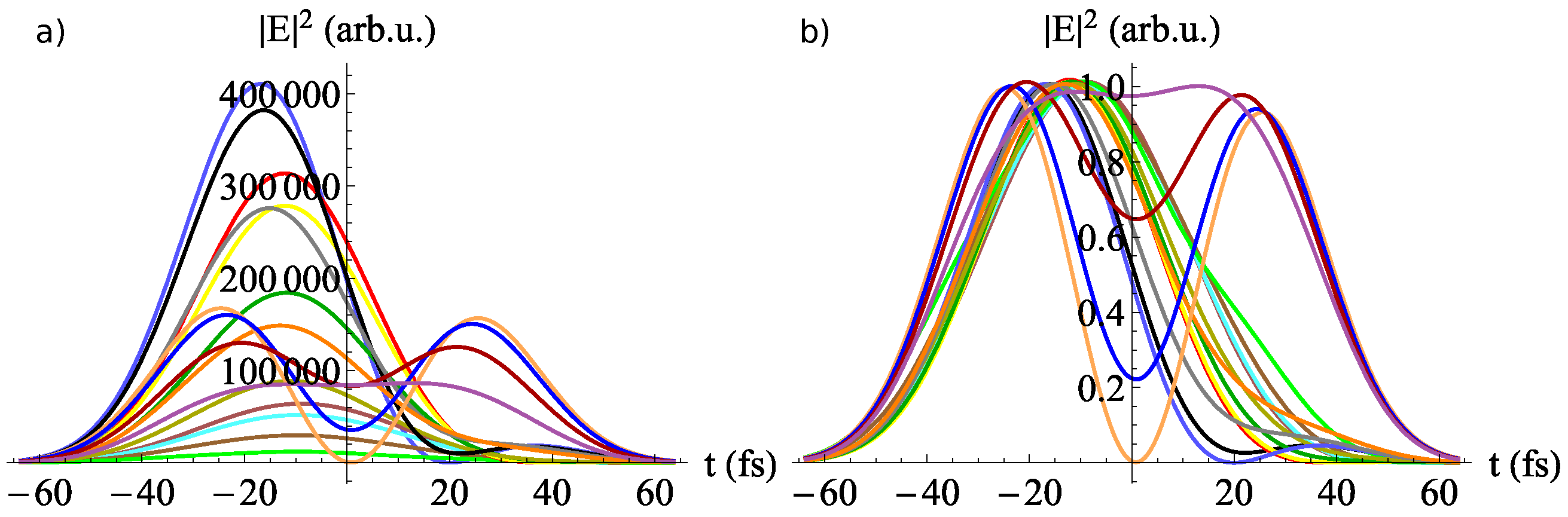
3.2. Laser Fields with Helical Phases and Spatio-Temporal Distortions
3.2.1. Spatial Chirp
3.2.2. Angular Dispersion
3.2.3. Pulse Front Tilt
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| AGC | Angular chirp |
| BPM | Beam propagation method |
| CPA | Chirped pulse amplification |
| ELI-NP | Extreme Light Infrastructure–Nuclear Physics |
| FDTD | Finite-difference time domain |
| FF | Far field |
| FL | Fourier limit |
| HPLS | High-power laser system |
| Laguerre-Gaussian | |
| NF | Near field |
| OAM | Orbital angular momentum |
| OPCPA | Optical parametric chirped pulse amplification |
| OV | Optical vortex |
| PFT | Pulse front tilt |
| PW | Petawatt |
| SPC | Spatial chirp |
| STC | Spatio-temporal couplings |
References
- Strickland, D.; Mourou, G. Compression of amplified chirped optical pulses. Opt. Commun. 1985, 56, 219–221. [Google Scholar] [CrossRef]
- Dubietis, A.; Jonušauskas, G.; Piskarskas, A. Powerful femtosecond pulse generation by chirped and stretched pulse parametric amplification in BBO crystal. Opt. Commun. 1992, 88, 437–440. [Google Scholar] [CrossRef]
- Lureau, F.; Matras, G.; Chalus, O.; Derycke, C.; Morbieu, T.; Radier, C.; Casagrande, O.; Laux, S.; Ricaud, S.; Rey, G.; et al. High-energy hybrid femtosecond laser system demonstrating 2 × 10 PW capability. High Power Laser Sci. Eng. 2020, 8, E43. [Google Scholar] [CrossRef]
- Nye, J.F.; Berry, M.V. Dislocations in wave trains. Proc. R. Soc. London A Math. Phys. Sci. 1974, 336, 165–190. [Google Scholar] [CrossRef]
- Coullet, P.; Gil, L.; Rocca, F. Optical vortices. Opt. Commun. 1989, 73, 403–408. [Google Scholar] [CrossRef]
- Bazhenov, V.; Vasnetsov, M.; Soskin, M. Laser-Beams with Screw Dislocations in Their Wave-Fronts. JETP Lett. 1990, 52, 429–431. [Google Scholar]
- Beijersbergen, M.; Allen, L.; van der Veen, H.; Woerdman, J. Astigmatic laser mode converters and transfer of orbital angular momentum. Opt. Commun. 1993, 96, 123–132. [Google Scholar] [CrossRef]
- Allen, L.; Beijersbergen, M.W.; Spreeuw, R.J.C.; Woerdman, J.P. Orbital angular momentum of light and the transformation of Laguerre-Gaussian laser modes. Phys. Rev. A 1992, 45, 8185–8189. [Google Scholar] [CrossRef]
- Grier, D.G. A revolution in optical manipulation. Nature 2003, 424, 810–816. [Google Scholar] [CrossRef]
- Mair, A.; Vaziri, A.; Weihs, G.; Zeilinger, A. Entanglement of the orbital angular momentum states of photons. Nature 2001, 412, 313–316. [Google Scholar] [CrossRef] [Green Version]
- Molina-Terriza, G.; Torres, J.P.; Torner, L. Twisted photons. Nat. Phys. 2007, 3, 305–310. [Google Scholar] [CrossRef]
- Devlin, R.C.; Ambrosio, A.; Rubin, N.A.; Mueller, J.P.B.; Capasso, F. Arbitrary spin-to–orbital angular momentum conversion of light. Science 2017, 358, 896–901. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Stav, T.; Faerman, A.; Maguid, E.; Oren, D.; Kleiner, V.; Hasman, E.; Segev, M. Quantum entanglement of the spin and orbital angular momentum of photons using metamaterials. Science 2018, 361, 1101–1104. [Google Scholar] [CrossRef] [PubMed]
- Gong, M. Recent Advances on Tunable Vortex Beam Devices for Biomedical Applications. Biomed. J. Sci. Tech. Res. 2018, 9, 5. [Google Scholar] [CrossRef]
- Fuerhapter, S.; Jesacher, A.; Bernet, S.; Ritsch-Marte, M. Spiral phase contrast imaging in microscopy. Opt. Express 2005, 13, 689–694. [Google Scholar] [CrossRef] [Green Version]
- Tamburini, F.; Anzolin, G.; Umbriaco, G.; Bianchini, A.; Barbieri, C. Overcoming the Rayleigh Criterion Limit with Optical Vortices. Phys. Rev. Lett. 2006, 97, 163903. [Google Scholar] [CrossRef] [Green Version]
- Hell, S.W. Microscopy and its focal switch. Nat. Methods 2008, 6, 24–32. [Google Scholar] [CrossRef] [Green Version]
- Barreiro, J.T.; Wei, T.C.; Kwiat, P.G. Beating the channel capacity limit for linear photonic superdense coding. Nat. Phys. 2008, 4, 282–286. [Google Scholar] [CrossRef] [Green Version]
- Lee, J.C.T.; Alexander, S.J.; Kevan, S.D.; Roy, S.; McMorran, B.J. Laguerre–Gauss and Hermite–Gauss soft X-ray states generated using diffractive optics. Nat. Photonics 2019, 13, 205–209. [Google Scholar] [CrossRef] [Green Version]
- De las Heras, A.; Pandey, A.K.; Román, J.S.; Serrano, J.; Baynard, E.; Dovillaire, G.; Pittman, M.; Durfee, C.G.; Plaja, L.; Kazamias, S.; et al. Extreme-ultraviolet vector-vortex beams from high harmonic generation. Optica 2022, 9, 71–79. [Google Scholar] [CrossRef]
- Allen, L.; Padgett, M.; Babiker, M. The Orbital Angular Momentum of Light. In Progress in Optics; Elsevier: Amsterdam, The Netherlands, 1999; pp. 291–372. [Google Scholar] [CrossRef]
- Nuter, R.; Korneev, P.; Dmitriev, E.; Thiele, I.; Tikhonchuk, V.T. Gain of electron orbital angular momentum in a direct laser acceleration process. Phys. Rev. E 2020, 101, 053202. [Google Scholar] [CrossRef] [PubMed]
- Brabetz, C.; Busold, S.; Cowan, T.; Deppert, O.; Jahn, D.; Kester, O.; Roth, M.; Schumacher, D.; Bagnoud, V. Laser-driven ion acceleration with hollow laser beams. Phys. Plasmas 2015, 22, 013105. [Google Scholar] [CrossRef]
- Dennis, M.R.; O’Holleran, K.; Padgett, M.J. Chapter 5 Singular Optics: Optical Vortices and Polarization Singularities. In Progress in Optics; Elsevier: Amsterdam, The Netherlands, 2009; pp. 293–363. [Google Scholar] [CrossRef]
- Pariente, G.; Gallet, V.; Borot, A.; Gobert, O.; Quéré, F. Space–time characterization of ultra-intense femtosecond laser beams. Nat. Photonics 2016, 10, 547–553. [Google Scholar] [CrossRef]
- Dorrer, C. Spatiotemporal Metrology of Broadband Optical Pulses. IEEE J. Sel. Top. Quantum Electron. 2019, 25, 1–16. [Google Scholar] [CrossRef]
- Greynolds, A.W. Propagation Of Generally Astigmatic Gaussian Beams Along Skew Ray Paths. In Proceedings of the Diffraction Phenomena in Optical Engineering Applications; Byrne, D.M., Harvey, J.E., Eds.; International Society for Optics and Photonics, SPIE: Bellingham, WA, USA, 1986; Volume 0560, pp. 33–51. [Google Scholar] [CrossRef]
- Harvey, J.E.; Irvin, R.G.; Pfisterer, R.N. Modeling physical optics phenomena by complex ray tracing. Opt. Eng. 2015, 54, 035105. [Google Scholar] [CrossRef] [Green Version]
- Worku, N.G.; Gross, H. Gaussian pulsed beam decomposition for propagation of ultrashort pulses through optical systems. J. Opt. Soc. Am. A 2020, 37, 98–107. [Google Scholar] [CrossRef]
- Talposi, A.M.; Ursescu, D. Propagation of ultrashort laser fields with spatiotemporal couplings using Gabor’s Gaussian complex decomposition. J. Opt. Soc. Am. A 2022, 39, 267–278. [Google Scholar] [CrossRef]
- Ohland, J.B.; Eisenbarth, U.; Roth, M.; Bagnoud, V. A study on the effects and visibility of low-order aberrations on laser beams with orbital angular momentum. Appl. Phys. B 2019, 125, 202. [Google Scholar] [CrossRef] [Green Version]
- Wolfram Research. NonlinearModelFit, Wolfram Language Function. 2008. Available online: https://reference.wolfram.com (accessed on 30 September 2021).
- Siegman, A. Lasers; University Science Books: Sausalito, CA, USA, 1986; Chapter 17. [Google Scholar]
- Serna, J.; Encinas-Sanz, F.; Nemes, G. Complete spatial characterization of a pulsed doughnut-type beam by use of spherical optics and a cylindrical lens. J. Opt. Soc. Am. A 2001, 18, 1726–1733. [Google Scholar] [CrossRef]
- Zhou, G.; Cai, Y.; Dai, C.Q. Hollow vortex Gaussian beams. Sci. China Phys. Mech. Astron. 2013, 56, 896–903. [Google Scholar] [CrossRef]
- Akturk, S.; Gu, X.; Gabolde, P.; Trebino, R. The general theory of first-order spatio-temporal distortions of Gaussian pulses and beams. Opt. Express 2005, 13, 8642–8661. [Google Scholar] [CrossRef] [PubMed]
- Akturk, S.; Gu, X.; Zeek, E.; Trebino, R. Pulse-front tilt caused by spatial and temporal chirp. Opt. Express 2004, 12, 4399–4410. [Google Scholar] [CrossRef] [PubMed]
- Sukhorukov, A.P.; Yangirova, V.V. Spatio-temporal vortices: Properties, generation and recording. In Proceedings of the Nonlinear Optics Applications; Karpierz, M.A., Boardman, A.D., Stegeman, G.I., Eds.; International Society for Optics and Photonics, SPIE: Bellingham, WA, USA, 2005; Volume 5949, pp. 35–43. [Google Scholar] [CrossRef]
- Hancock, S.W.; Zahedpour, S.; Goffin, A.; Milchberg, H.M. Free-space propagation of spatiotemporal optical vortices. Optica 2019, 6, 1547. [Google Scholar] [CrossRef]
- Chong, A.; Wan, C.; Chen, J.; Zhan, Q. Generation of spatiotemporal optical vortices with controllable transverse orbital angular momentum. Nat. Photonics 2020, 14, 350–354. [Google Scholar] [CrossRef]
- Wang, W.; Jiang, C.; Dong, H.; Lu, X.; Li, J.; Xu, R.; Sun, Y.; Yu, L.; Guo, Z.; Liang, X.; et al. Hollow plasma acceleration driven by a relativistic reflected hollow laser. Phys. Rev. Lett. 2020, 125, 034801. [Google Scholar] [CrossRef]
- Zhang, H.; Zhao, J.; Hu, Y.; Li, Q.; Lu, Y.; Cao, Y.; Zou, D.; Sheng, Z.; Pegoraro, F.; McKenna, P.; et al. Efficient bright γ-ray vortex emission from a laser-illuminated light-fan-in-channel target. High Power Laser Sci. Eng. 2021, 9, 1–24. [Google Scholar] [CrossRef]
- Zhao, J.; Hu, Y.T.; Lu, Y.; Zhang, H.; Hu, L.X.; Zhu, X.L.; Sheng, Z.M.; Turcu, I.C.E.; Pukhov, A.; Shao, F.Q.; et al. All-optical quasi-monoenergetic GeV positron bunch generation by twisted laser fields. Commun. Phys. 2022, 5, 15. [Google Scholar] [CrossRef]
- Hu, L.X.; Yu, T.P.; Sheng, Z.M.; Vieira, J.; Zou, D.B.; Yin, Y.; McKenna, P.; Shao, F.Q. Attosecond electron bunches from a nanofiber driven by Laguerre-Gaussian laser pulses. Sci. Rep. 2018, 8, 7282. [Google Scholar] [CrossRef] [Green Version]
- Hu, L.X.; Yu, T.P.; Li, H.Z.; Yin, Y.; McKenna, P.; Shao, F.Q. Dense relativistic electron mirrors from a Laguerre-Gaussian laser-irradiated micro-droplet. Opt. Lett. 2018, 43, 2615. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Talposi, A.-M.; Iancu, V.; Ursescu, D. Influence of Spatio-Temporal Couplings on Focused Optical Vortices. Photonics 2022, 9, 389. https://doi.org/10.3390/photonics9060389
Talposi A-M, Iancu V, Ursescu D. Influence of Spatio-Temporal Couplings on Focused Optical Vortices. Photonics. 2022; 9(6):389. https://doi.org/10.3390/photonics9060389
Chicago/Turabian StyleTalposi, Anda-Maria, Vicentiu Iancu, and Daniel Ursescu. 2022. "Influence of Spatio-Temporal Couplings on Focused Optical Vortices" Photonics 9, no. 6: 389. https://doi.org/10.3390/photonics9060389
APA StyleTalposi, A.-M., Iancu, V., & Ursescu, D. (2022). Influence of Spatio-Temporal Couplings on Focused Optical Vortices. Photonics, 9(6), 389. https://doi.org/10.3390/photonics9060389





