Design of PCF Supporting 86 OAM Modes with High Mode Quality and Low Nonlinear Coefficient
Abstract
1. Introduction
2. System Structure
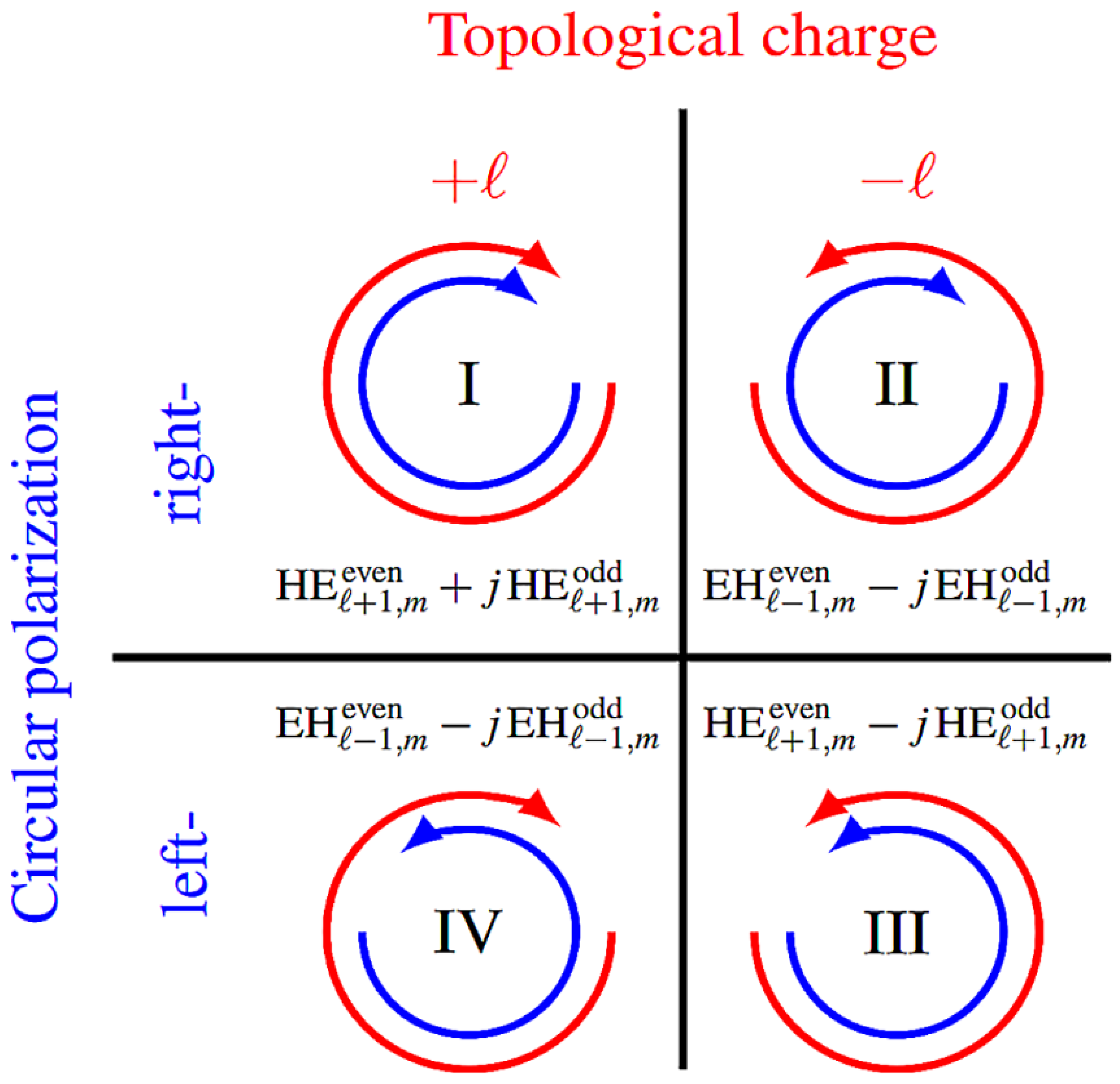
2.1. The Theory of SC-PCF Design
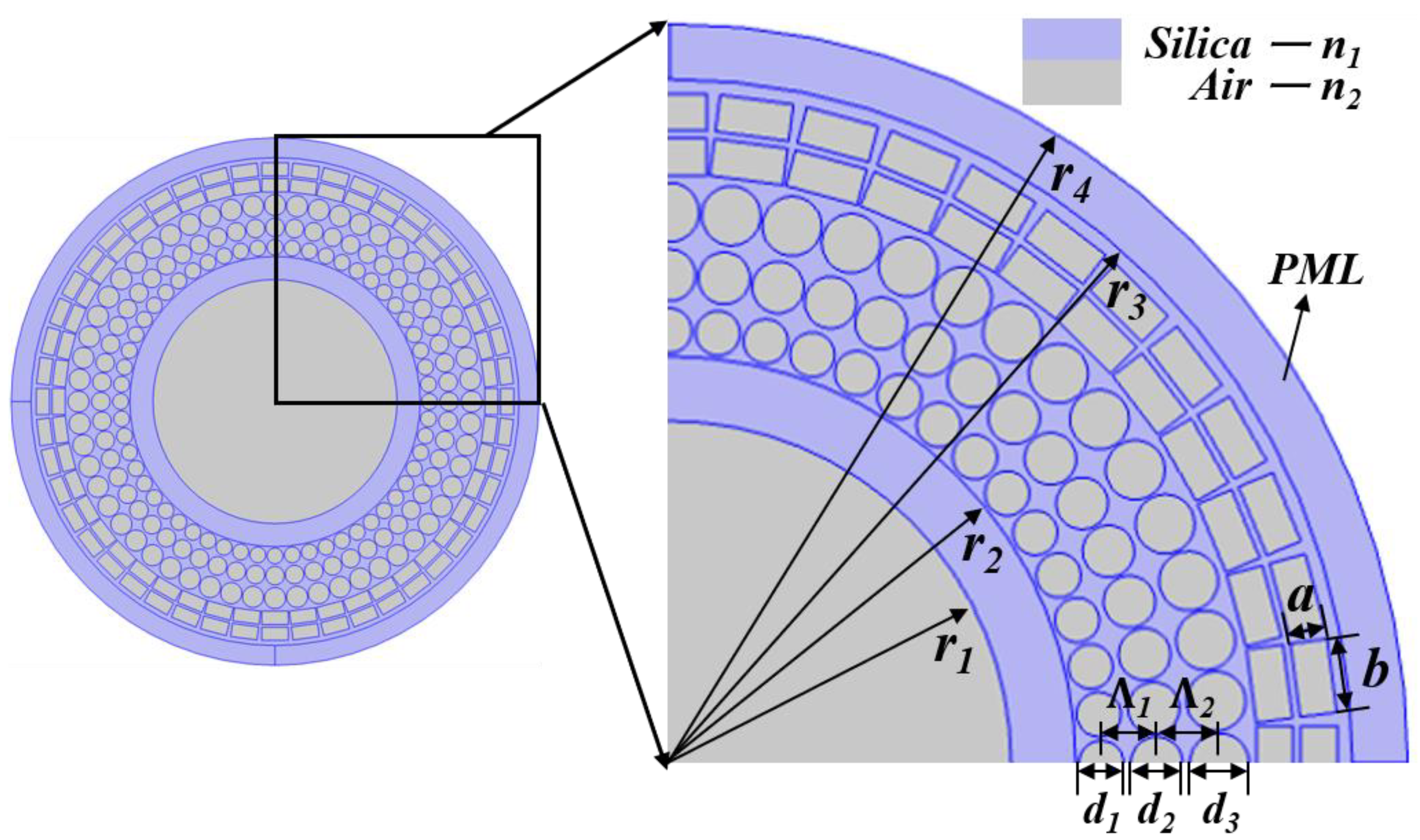
2.2. SC-PCF Structure
3. Simulation Results and Discussion
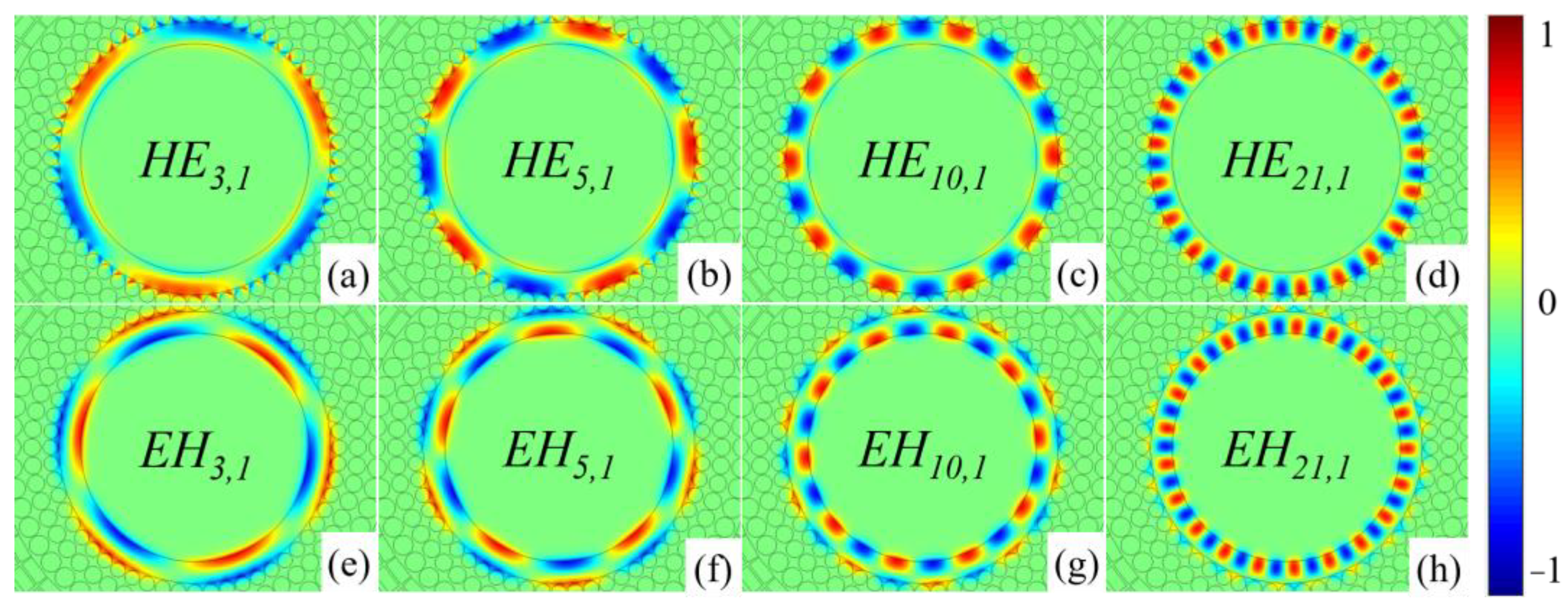
3.1. OAM Mode Analysis
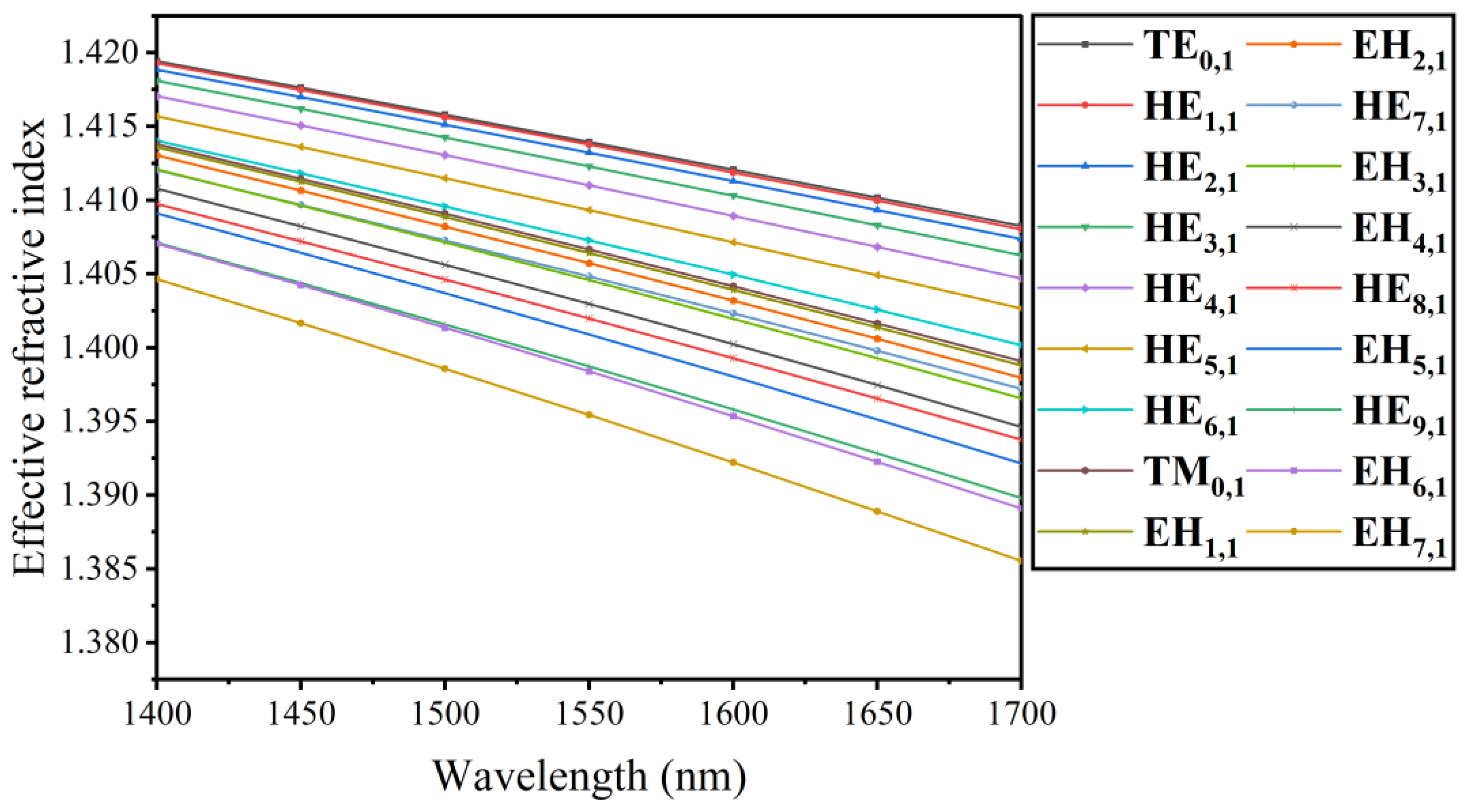
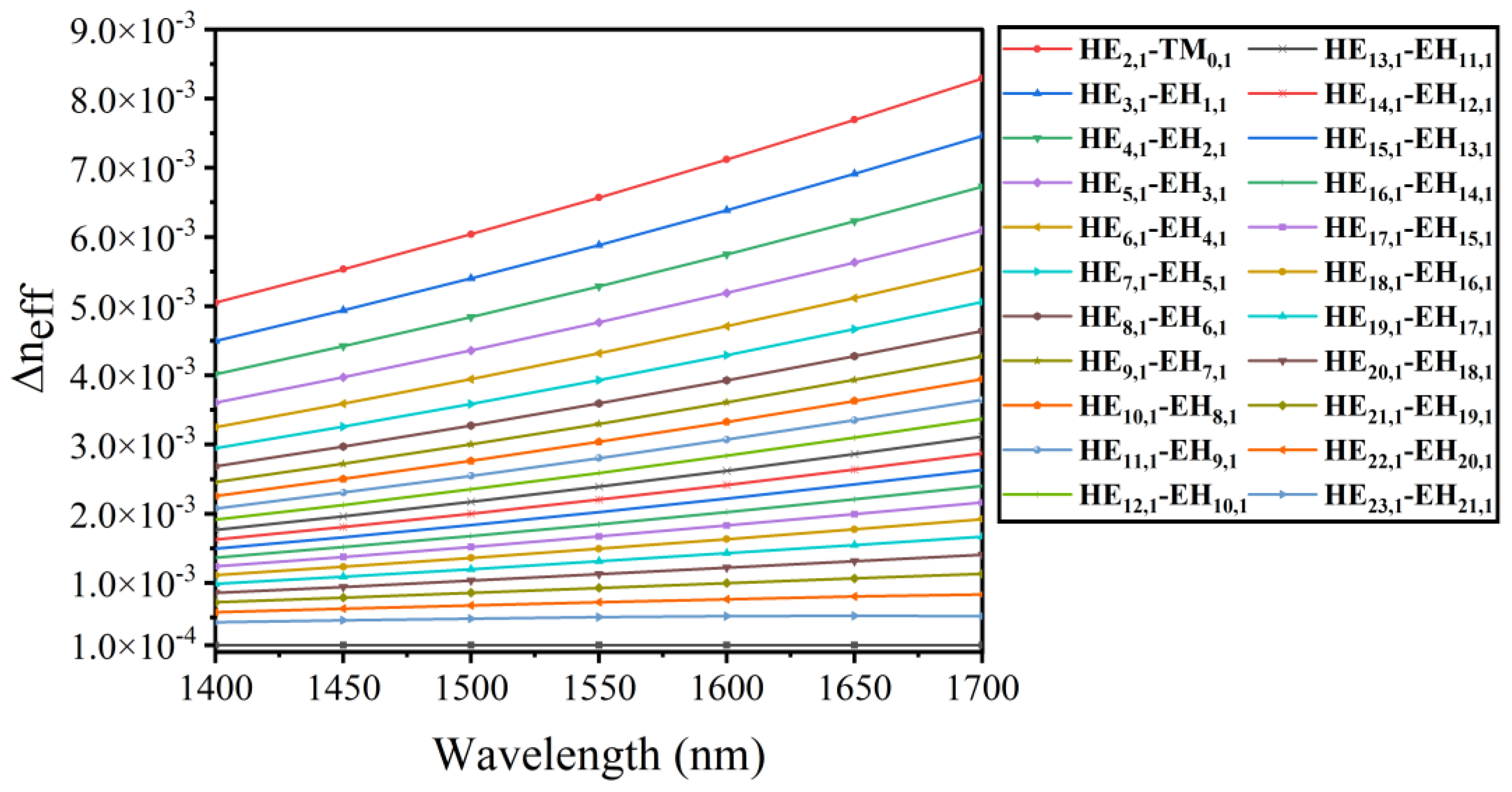
3.2. Effective Refractive Index (ERI) and Its Differences (ERID)
3.3. Dispersion Properties
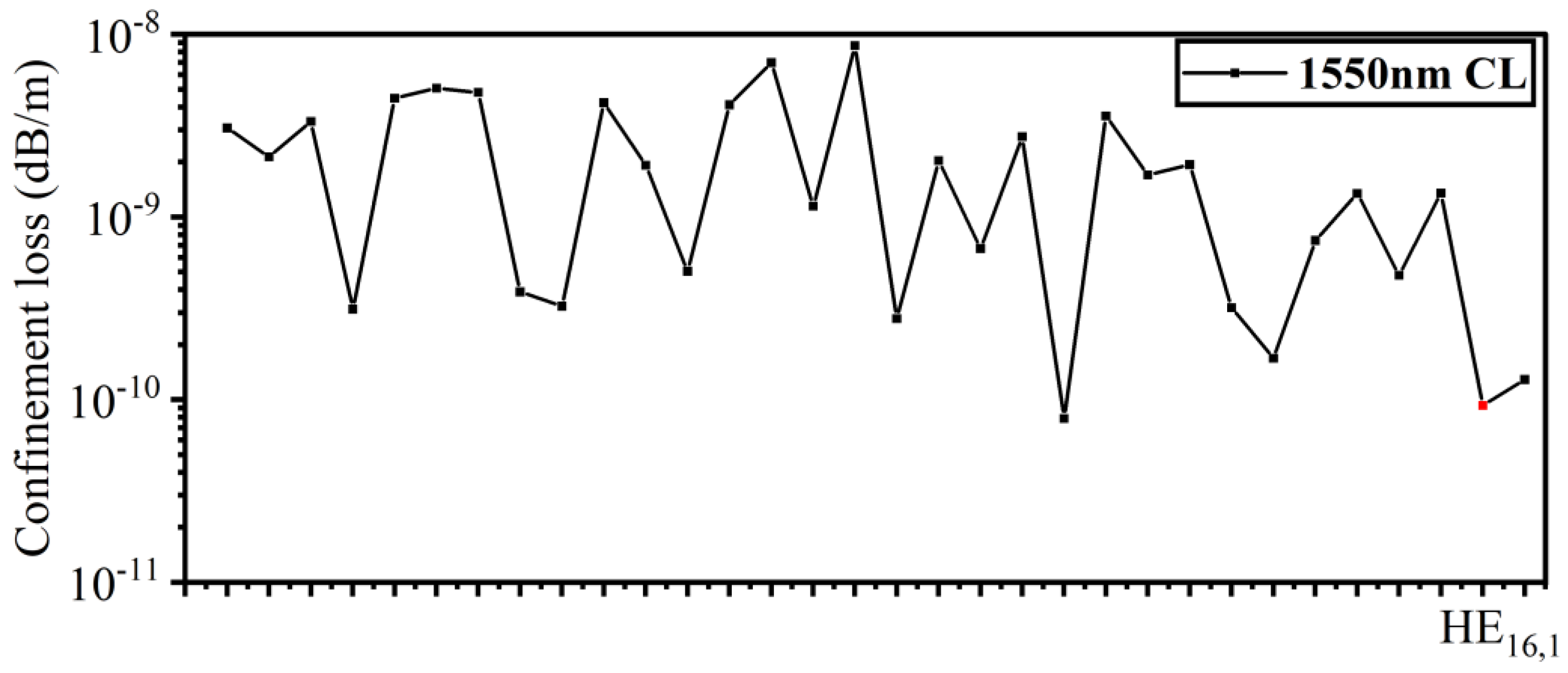
3.4. Confinement Loss (CL)
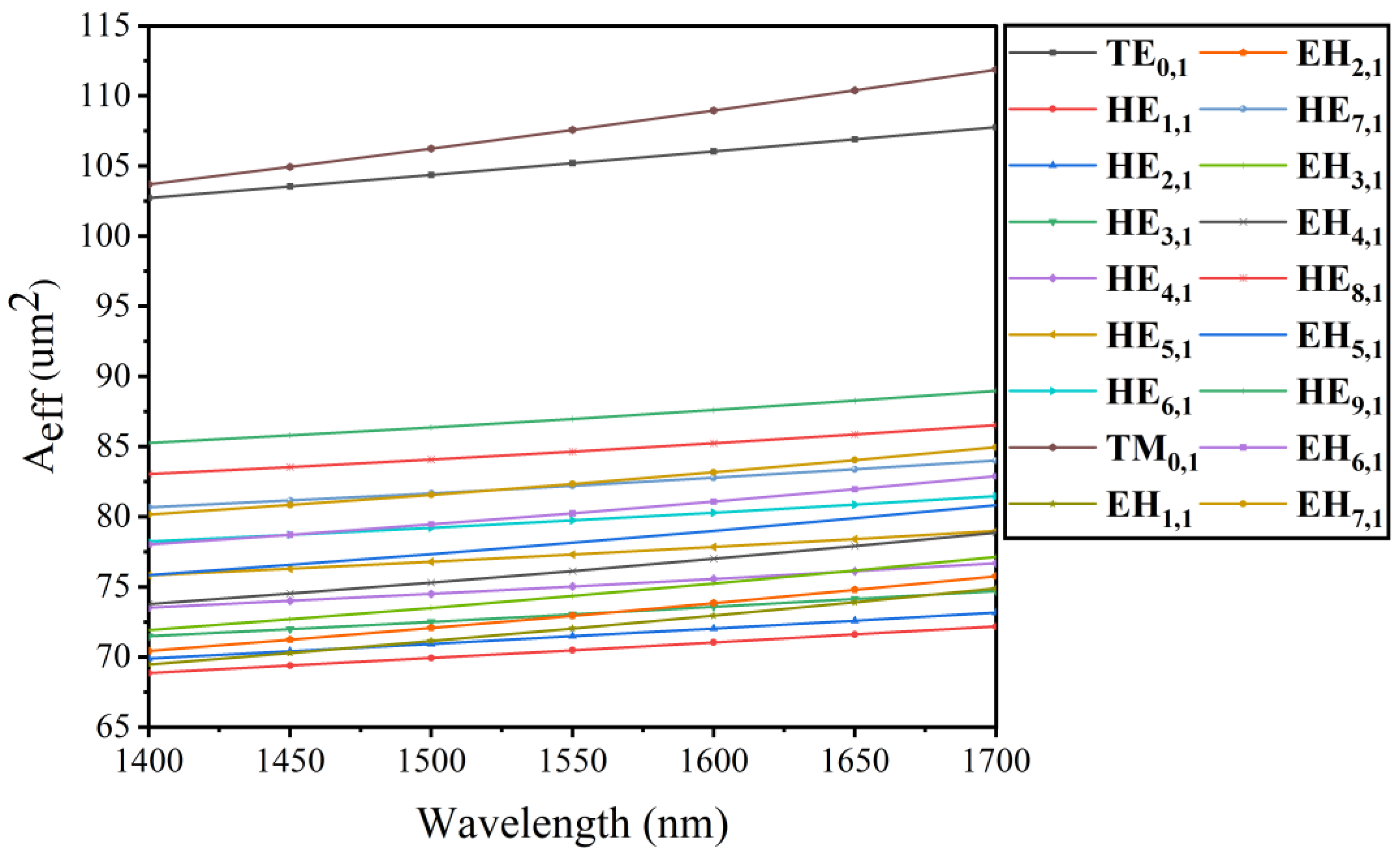
3.5. Effective Mode Field Area (EMFA)
3.6. Numerical Aperture (NA)
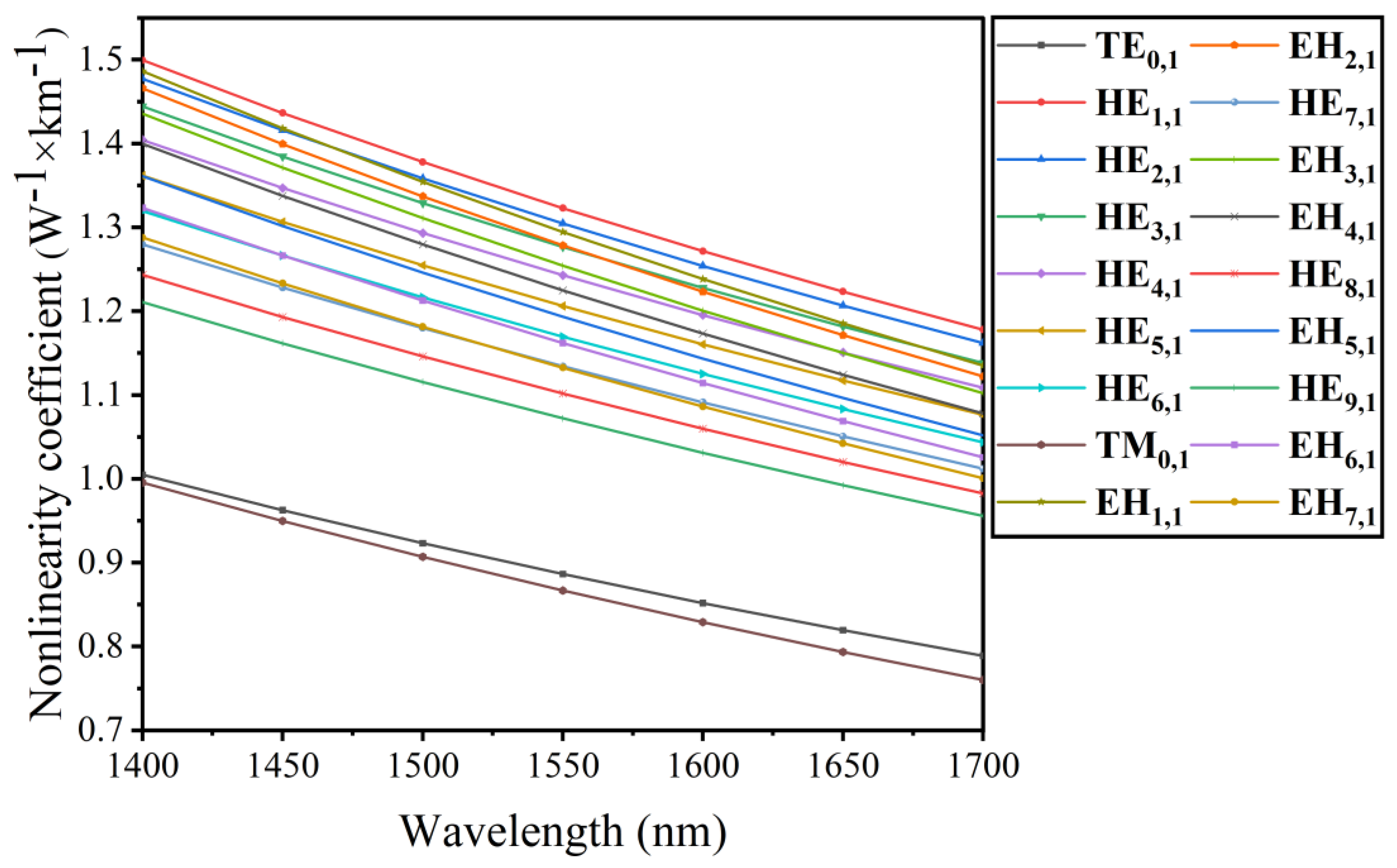
3.7. Nonlinearity Coefficient (NC)
3.8. Mode Purity
3.9. Comparison with Existing Typical PCFs
| Year | Number of OAM Modes | 1550 nm Dispersion (ps/nm/km) | Confinement Loss (dB/m) | Effective Mode Field Area (μm2) | Nonlinear Coefficient (w−1·km−1) | Mode Purity (%) | Bandwidth (nm) | Reference |
|---|---|---|---|---|---|---|---|---|
| 2016 | 26 | flat | <10−9 | - | 356.2 (HE3,1) | - | 1100–2000 | [44] |
| 2018 | 46 | >50 | <10−9 | 54.25 (HE3,1) | <2.58 | - | 1200–2000 | [16] |
| 2018 | 54 | >53.29 | <10−9 | >48 | 1.41–2.1 | - | 1500–1600 | [17] |
| 2020 | 26 | <25 | <10−8 | >35 | 2–3 | - | 800–1800 | [31] |
| 2020 | 38 | >4.75 | >10−10 | - | >1.04 | >85 | 800–2000 | [30] |
| 2020 | 56 | >36.91 | 10−8 | >60 | <4 | <94 | 600–2500 | [45] |
| 2020 | 50 | >46.96 | <10−9 | >80 | 0.6–1.5 | - | 1150–2000 | [18] |
| 2020 | 56 | - | >10−8 | - | - | >80 | 1400–1700 | [46] |
| 2021 | 30 | >3.59 | <10−8 | 30–50 | <4.144 | >90 | 1350–1800 | [37] |
| 2021 | 44 | >50 | >10−10 | 40–65 | <3.36 | - | 1150–1800 | [47] |
| 2021 | 86 | >4.939 | <10−9 | 60–110 | 0.867–1.5 | >96 | 1400–1700 | this paper |
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ellis, A.D.; Jian, Z.; Cotter, D. Approaching the Non-Linear Shannon Limit. J. Lightw. Technol. 2010, 28, 423–433. [Google Scholar] [CrossRef]
- Ellis, A.; Doran, N. Are few-mode fibres a practical solution to the capacity crunch? In Proceedings of the 2013 15th International Conference on Transparent Optical Networks (ICTON), Cartagena, Spain, 23–27 June 2013. [Google Scholar]
- Huang, S.; Lin, W.; Chen, M. Cross-talk analysis of time-division multiplexing of polarization-insensitive fiber-optic Michelson interferometric sensors with a 3 × 3 directional coupler. Appl. Opt. 1997, 36, 921–933. [Google Scholar] [CrossRef] [PubMed]
- Slaveski, F.; Sluss, J., Jr.; Atiquzzaman, M.; Nguyen, H.; Ngo, D. Optical fiber wavelength division multiplexing. IEEE Aerosp. Electron. Syst. Mag. 2003, 18, 3–8. [Google Scholar] [CrossRef]
- Yi, A.; Yan, L.; Luo, B.; Pan, W.; Ye, J. All-Optical Signal Regeneration in Polarization-Division-Multiplexing Systems. IEEE Photonics J. 2011, 3, 703–712. [Google Scholar] [CrossRef]
- Mizuno, T.; Takara, H.; Shibahara, K.; Sano, A.; Miyamoto, Y. Dense Space Division Multiplexed Transmission over Multicore and Multimode Fiber for Long-haul Transport Systems. J. Lightw. Technol. 2016, 34, 1484–1493. [Google Scholar] [CrossRef]
- Richardson, D.J.; Fini, J.M.; Nelson, L.E. Space-division multiplexing in optical fibres. Nat. Photonics 2013, 7, 354–362. [Google Scholar] [CrossRef]
- Yan, Y.; Xie, G.; Lavery, M.P.J.; Huang, H.; Ahmed, N.; Bao, C.; Ren, Y.; Cao, Y.; Li, L.; Zhao, Z.; et al. High-capacity millimetre-wave communications with orbital angular momentum multiplexing. Nat. Commun. 2014, 5, 1–9. [Google Scholar] [CrossRef]
- Huang, H.; Milione, G.; Lavery, M.P.J.; Xie, G.; Ren, Y.; Cao, Y.; Ahmed, N.; Nguyen, T.; Nolan, D.A.; Li, M.; et al. Mode division multiplexing using an orbital angular momentum mode sorter and MIMO-DSP over a graded-index few-mode optical fibre. Sci. Rep. 2015, 5, 1–7. [Google Scholar] [CrossRef]
- Willner, A.E.; Huang, H.; Yan, Y.; Ren, Y.; Ahmed, N.; Xie, G.; Bao, C.; Li, L.; Cao, Y.; Zhao, Z.; et al. Optical communications using orbital angular momentum beams. Adv. Opt. Photonics 2015, 7, 66–106. [Google Scholar] [CrossRef]
- Guo, Z.; Gong, C.; Liu, H.; Li, J.; Wang, Z.; Yang, Y.; Gong, Y. Research advances of orbital angular momentum based optical communication technology. Opto-Electron. Eng. 2020, 47, 190593. [Google Scholar]
- Wang, W.; Hou, L. Present Situation and Future Development in Photonic Crystal Fibers. Laser Optoelectron. Prog. 2008, 45, 43–58. [Google Scholar] [CrossRef]
- Yue, Y.; Zhang, L.; Yan, Y.; Ahmed, N.; Yang, J.; Huang, H.; Ren, Y.; Dolinar, S.; Tur, M.; Willner, A.E. Octave-spanning supercontinuum generation of vortices in an As2S3 ring photonic crystal fiber. Opt. Lett. 2012, 37, 1889–1891. [Google Scholar] [CrossRef]
- Zhang, H.; Zhang, X.; Li, H.; Deng, Y.; Xi, L.; Tang, X.; Zhang, W. The Orbital Angular Momentum Modes Supporting Fibers Based on the Photonic Crystal Fiber Structure. Crystals 2017, 7, 286. [Google Scholar] [CrossRef]
- Tian, W.; Zhang, H.; Zhang, X.; Xi, L.; Zhang, W.; Tang, X. A circular photonic crystal fiber supporting 26 OAM modes. Opt. Fiber Technol. 2016, 30, 184–189. [Google Scholar] [CrossRef]
- Bai, X.; Chen, H.; Yang, H. Design of a circular photonic crystal fiber with square air-holes for orbital angular momentum modes transmission. Opt. Int. J. Light Electron. Opt. 2018, 158, 1266–1274. [Google Scholar] [CrossRef]
- Yang, H. Design and Performance Analysis of a Novel Photonic Crystal Fiber for OAM Mode Transmission; Nanjing University of Posts and Telecommunications: Nanjin, China, 2018; pp. 25–30. [Google Scholar]
- Ke, X.; Wang, S. Design of Photonic Crystal Fiber Capable of Carrying Multiple Orbital Angular Momentum Modes Transmission. Opt. Photonics J. 2020, 10, 49–63. [Google Scholar] [CrossRef][Green Version]
- Hong, S.; Lee, Y.S.; Choi, H.; Quan, C.; Li, Y.; Kim, S.; Oh, K. Hollow silica photonic crystal fiber guiding 101 orbital angular momentum modes without phase distortion in C + L band. J. Lightw. Technol. 2020, 38, 1010–1018. [Google Scholar] [CrossRef]
- Ma, Q.; Luo, A.P.; Hong, W. Numerical Study of Photonic Crystal Fiber Supporting 180 Orbital Angular Momentum Modes with High Mode Quality and Flat Dispersion. J. Lightw. Technol. 2021, 39, 2971–2979. [Google Scholar] [CrossRef]
- Zhao, L.; Zhao, H.; Xu, Z.; Liang, R. A Design of Novel Photonic Crystal Fiber with Low and Flattened Dispersion for Supporting 84 Orbital Angular Momentum Modes. Commun. Theor. Phys. 2021, 73, 085501. [Google Scholar] [CrossRef]
- Ramachandran, S.; Kristensen, P. Optical Vortices in Fiber. Nanophotonics 2013, 2, 455–474. [Google Scholar] [CrossRef]
- Brunet, C.; Vaity, P.; Messaddeq, Y.; LaRochelle, S.; Rusch, L.A. Design, fabrication and validation of an OAM fiber supporting 36 states. Opt. Express 2014, 22, 26117–26127. [Google Scholar] [CrossRef] [PubMed]
- Wei, W.; Zhang, L.; Zhang, Z.; Tang, L.; Ding, L.; Li, Y. Design of Three Vortex Photonic Crystal Fibers. Acta Opt. Sin. 2019, 39, 0906006. [Google Scholar] [CrossRef]
- Zhang, H.; Zhang, X.; Li, H.; Deng, Y.; Zhang, X.; Xi, L.; Tang, X.; Zhang, W. A design strategy of the circular photonic crystal fiber supporting good quality orbital angular momentum mode transmission. Opt. Commun. 2017, 397, 59–66. [Google Scholar] [CrossRef]
- Zhang, X.; Yuan, M.; Chang, M.; Lu, X.; Chen, N.; Nie, F.; He, M.; Qi, N.; Zhuang, S. Characteristics in square air hole structure photonic crystal fiber. Opto-Electron. Eng. 2018, 45, 170633. [Google Scholar]
- Yajima, T.; Yamamoto, J.; Ishii, F.; Hirooka, T.; Yoshida, M.; Nakazawa, M. Low-loss photonic crystal fiber fabricated by a slurry casting method. Opt. Express 2013, 21, 30500–30506. [Google Scholar] [CrossRef]
- Jiang, G.; Fu, Y.; Huang, Y. High birefringence rectangular-hole photonic crystal fiber. Opt. Fiber Technol. 2015, 26, 163–171. [Google Scholar] [CrossRef]
- Hassan, M.M.; Kabir, M.A.; Hossain, M.N.; Biswas, B.; Paul, B.K.; Ahmed, K. Photonic crystal fiber for robust orbital angular momentum transmission: Design and investigation. Opt. Quantum Electron. 2020, 52, 1–14. [Google Scholar] [CrossRef]
- Kabir, M.A.; Hassan, M.M.; Hossain, M.N.; Paul, B.K.; Ahmed, K. Design and performance evaluation of photonic crystal fibers of supporting orbital angular momentum states in optical transmission. Opt. Commun. 2020, 467, 125731. [Google Scholar] [CrossRef]
- Kabir, M.A.; Hassan, M.M.; Ahmed, K.; Rajan, M.S.M.; Aly, A.H.; Hossain, M.N.; Paul, B.K. Novel spider web photonic crystal fiber for robust mode transmission applications with supporting orbital angular momentum transmission property. Opt. Quantum Electron. 2020, 52, 1–17. [Google Scholar] [CrossRef]
- Vienne, G.; Xu, Y.; Jakobsen, C.; Deyerl, H.; Jensen, J.B.; Srensen, T.; Hansen, T.P.; Huang, Y.; Terrel, M.; Lee, R.K.; et al. Ultra-large bandwidth hollow-core guiding in all-silica Bragg fibers with nano-supports. Opt. Express 2004, 12, 3500–3508. [Google Scholar] [CrossRef]
- Al-Zahrani, F.A.; Kabir, M. Ring-Core Photonic Crystal Fiber of Terahertz Orbital Angular Momentum Modes with Excellence Guiding Properties in Optical Fiber Communication. Photonics 2021, 8, 122. [Google Scholar] [CrossRef]
- Kabir, M.A.; Ahmed, K.; Hassan, M.M.; Hossain, M.M.; Paul, B.K. Design a photonic crystal fiber of guiding terahertz orbital angular momentum beams in optical communication. Opt. Commun. 2020, 475, 126192. [Google Scholar] [CrossRef]
- Zhang, L.; Meng, Y. Design and analysis of a photonic crystal fiber supporting stable transmission of 30 OAM modes. Opt. Fiber Technol. 2021, 61, 102423. [Google Scholar] [CrossRef]
- Hassan, M.M.; Kabir, M.A.; Hossain, M.N.; Nguyen, T.K.; Paul, B.K.; Ahmed, K.; Dhasarathan, V. Numerical analysis of circular core shaped photonic crystal fiber for orbital angular momentum with efficient transmission. Appl. Phys. B. 2020, 126, 1–8. [Google Scholar] [CrossRef]
- He, T.; Wu, B. Low confinement loss photonic crystal fibre capable of supporting 54 orbital angular momentum modes. J. Mod. Opt. 2020, 67, 1–7. [Google Scholar] [CrossRef]
- Liu, G.; Zhi, Z.; Wang, R.K. Digital focusing of OCT images based on scalar diffraction theory and information entropy. Biomed. Opt. Express 2012, 3, 2774–2783. [Google Scholar] [CrossRef] [PubMed]
- Zhang, H.; Tao, R.; Zhou, P.; Wang, X.; Xu, X. 1.5-kW Yb-Raman combined nonlinear fiber amplifier at 1120 nm. IEEE Photonics Technol. Lett. 2014, 27, 628–630. [Google Scholar] [CrossRef]
- Marhic, M.E. Fiber Optical Parametric Amplifiers, Oscillators and Related Devices; Cambridge University Press: Cambridge, MA, USA, 2008. [Google Scholar]
- Headley, C.; Agrawal, G. Raman Amplification in Fiber Optical Communication Systems; Academic Press: Cambridge, MA, USA, 2005. [Google Scholar]
- Bian, J.R.; Chan, A.K. Computations of the diffraction effect and the nonlinear effect on spatial solitons in nonlinear planar waveguides. Microw. Opt. Technol. Lett. 1991, 4, 184–191. [Google Scholar] [CrossRef]
- Alfassi, B.; Rotschild, C.; Manela, O.; Segev, M.; Christodoulides, D.N. Boundary force effects exerted on solitons in highly nonlocal nonlinear media. Opt. Lett. 2007, 32, 154–156. [Google Scholar] [CrossRef]
- Zhang, H.; Huang, Y.; Luo, A.; Cui, H.; Luo, Z.; Xu, W. Photonic crystal fiber for supporting 26 orbital angular momentum modes. Opt. Express 2016, 24, 17285–17291. [Google Scholar]
- Israk, M.F.; Razzak, M.A.; Ahmed, K.; Hassan, M.M.; Kabir, M.A.; Hossain, M.N.; Paul, B.K.; Dhasarathan, V. Ring-based coil structure photonic crystal fiber for transmission of Orbital Angular Momentum with large bandwidth: Outline, investigation and analysis. Opt. Commun. 2020, 473, 126003. [Google Scholar] [CrossRef]
- Wang, W.; Wang, N.; Li, K.; Geng, Z.; Jia, H. A novel dual guided modes regions photonic crystal fiber with low crosstalk supporting 56 OAM modes and 4 LP modes. Opt. Fiber Technol. 2020, 57, 102213. [Google Scholar] [CrossRef]
- Wang, W.; Wang, N.; Jia, H. Research on the Dispersion Characteristics of Silica-based Ring-core Photonic Crystal Fiber Used to Transmit Orbital Angular Momentum Modes. Optik 2021, 241, 166935. [Google Scholar] [CrossRef]





| Structure |  | 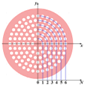 |  |  |  | 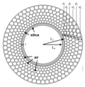 |  |  |
|---|---|---|---|---|---|---|---|---|
| Author | Yue | Tian | Bai | Yang | Ke | Hong | Ma | Zhao |
| Number of OAM Modes | 2 | <26 | 46 | >50 | >50 | 101 | 180 | 84 |
| CL (dB/m) | 0.03 | 0.003 | 10−9 | >10−11 | >10−11 | <10−8 | 10−12~10−7 | >10−13 |
| Reference | [12] | [15] | [16] | [17] | [18] | [19] | [20] | [21] |
| Symbols | Parameters | Symbols | Parameters |
|---|---|---|---|
| r1 | 9.25 μm | d2 | 1.4 μm |
| r2 | 11 μm | d3 | 1.6 μm |
| r3 | 18.5 μm | a | 1 μm |
| r4 | 20 μm | b | 2 μm |
| n1 | 1.444 | Λ | 0.2 μm |
| n2 | 1 | Λ1 | 1.5 μm |
| d1 | 1.2 μm | Λ2 | 1.7 μm |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, Y.; Lian, Y.; Hu, Q.; Xie, L.; Ding, J.; Wang, Y.; Lu, Z. Design of PCF Supporting 86 OAM Modes with High Mode Quality and Low Nonlinear Coefficient. Photonics 2022, 9, 266. https://doi.org/10.3390/photonics9040266
Yu Y, Lian Y, Hu Q, Xie L, Ding J, Wang Y, Lu Z. Design of PCF Supporting 86 OAM Modes with High Mode Quality and Low Nonlinear Coefficient. Photonics. 2022; 9(4):266. https://doi.org/10.3390/photonics9040266
Chicago/Turabian StyleYu, Yang, Yudong Lian, Qi Hu, Luyang Xie, Jie Ding, Yulei Wang, and Zhiwei Lu. 2022. "Design of PCF Supporting 86 OAM Modes with High Mode Quality and Low Nonlinear Coefficient" Photonics 9, no. 4: 266. https://doi.org/10.3390/photonics9040266
APA StyleYu, Y., Lian, Y., Hu, Q., Xie, L., Ding, J., Wang, Y., & Lu, Z. (2022). Design of PCF Supporting 86 OAM Modes with High Mode Quality and Low Nonlinear Coefficient. Photonics, 9(4), 266. https://doi.org/10.3390/photonics9040266







