Flexible Plenoptic X-ray Microscopy
Abstract
:1. Introduction
2. Materials and Methods
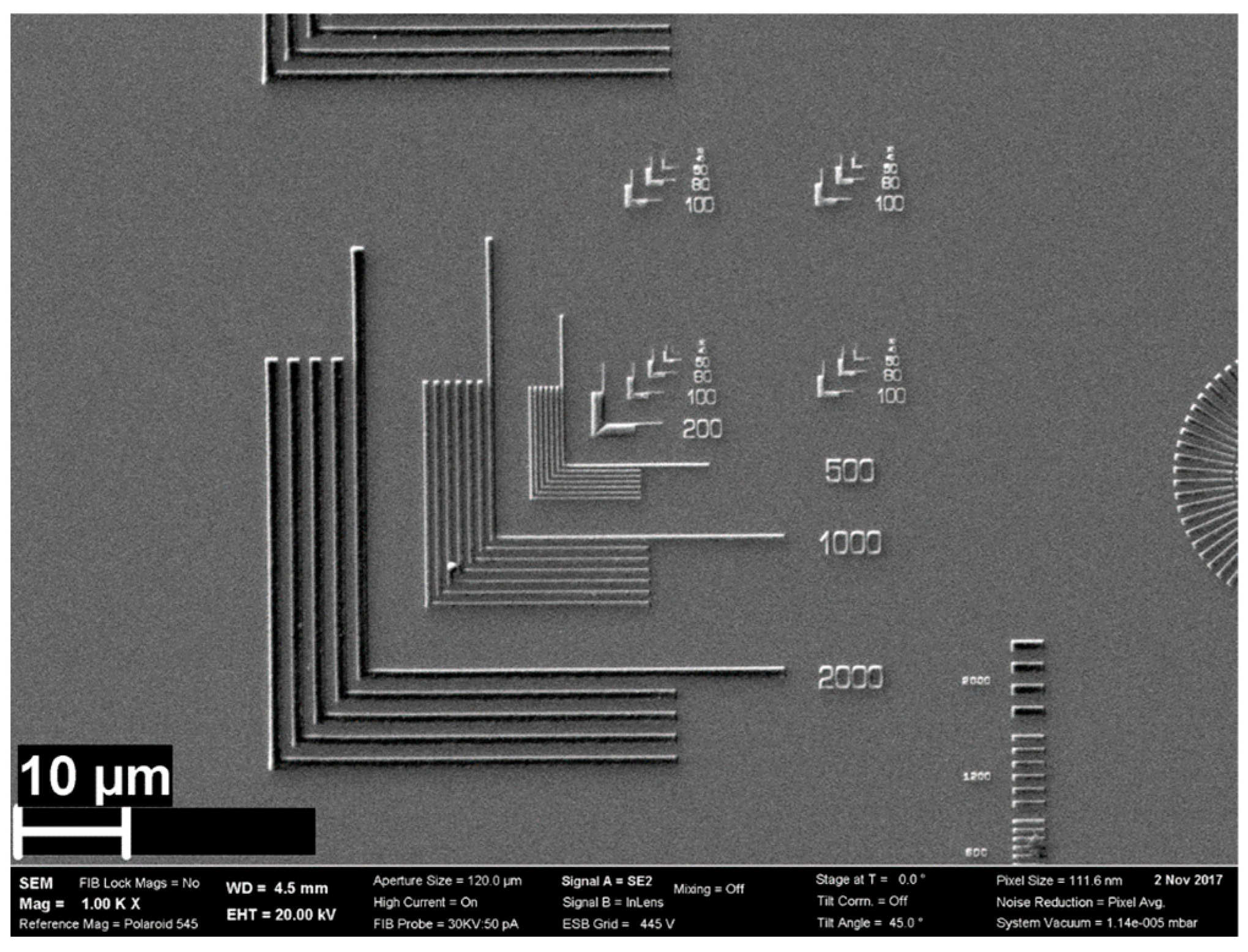
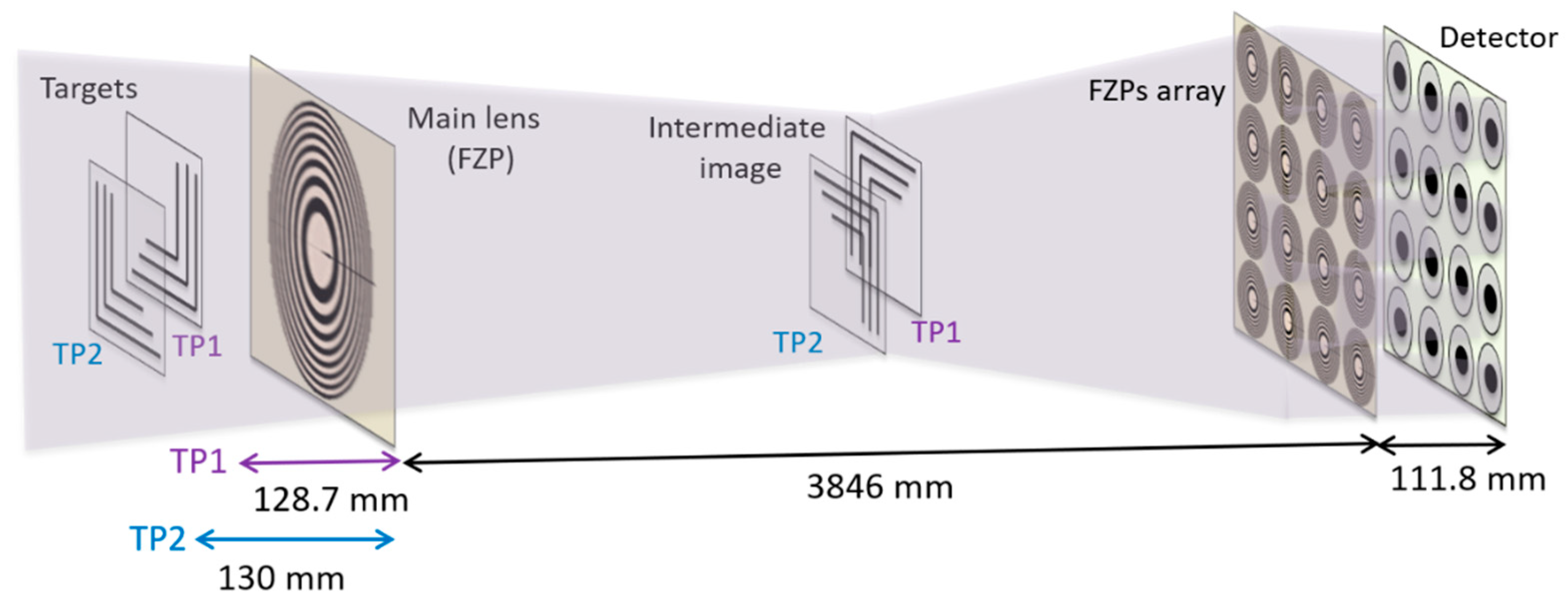
2.1. Experimental Setup
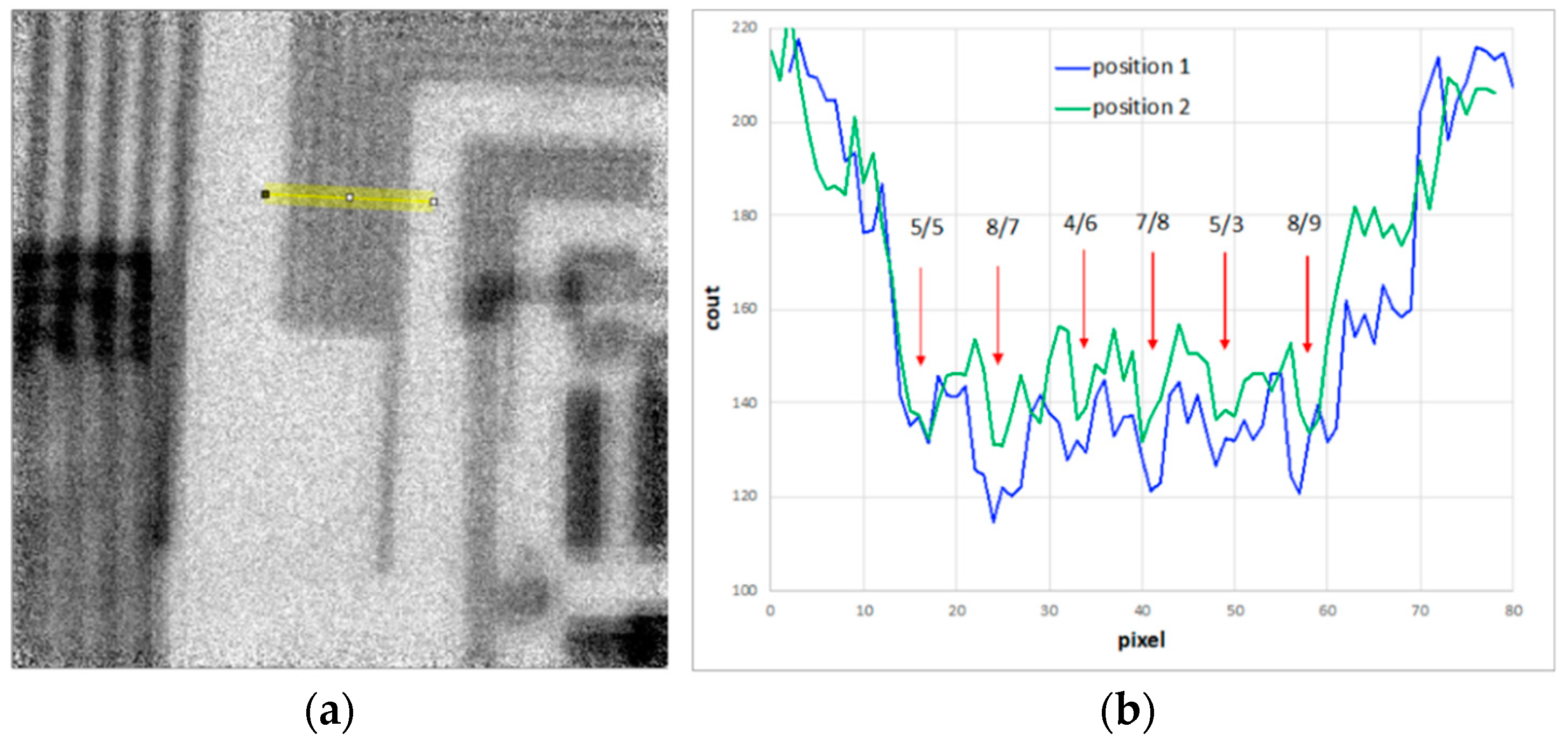
2.2. Data Processing
2.3. Dedicated Software to Generate Synthetic or Refocused Experimental Raw Plenoptic Images
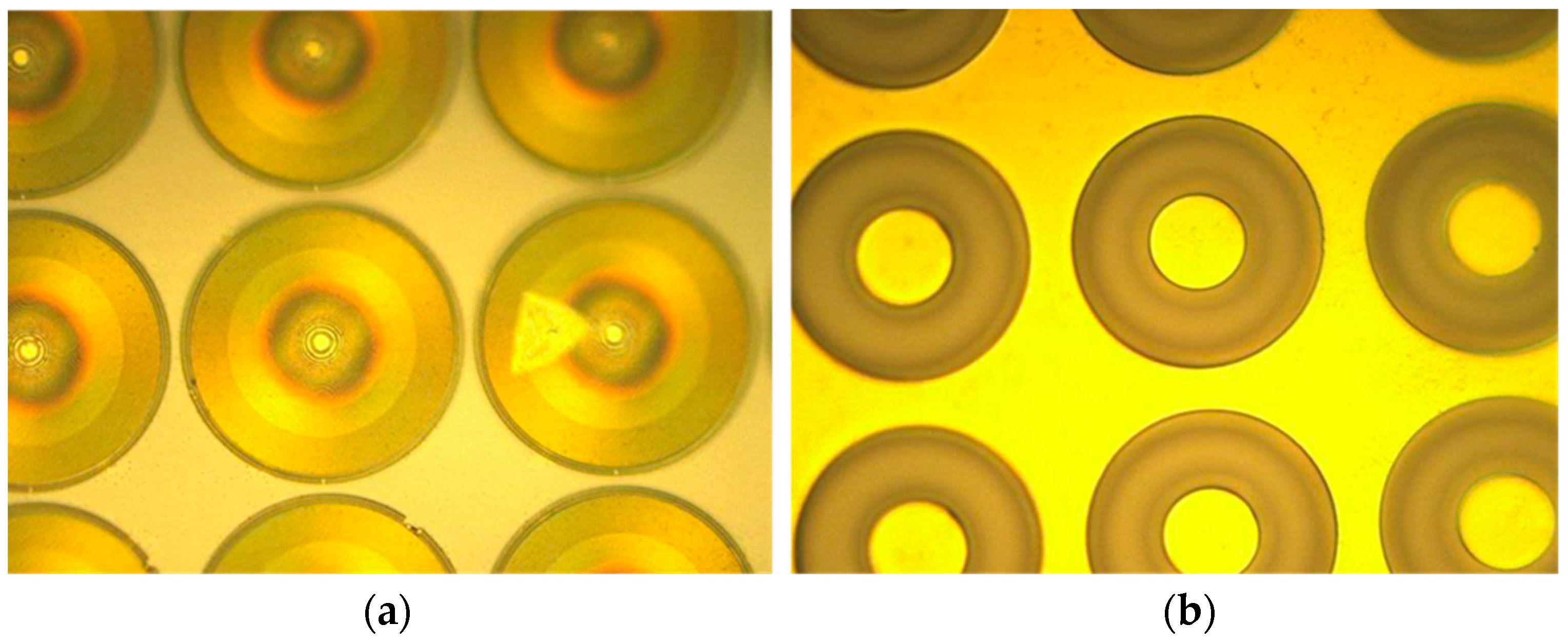
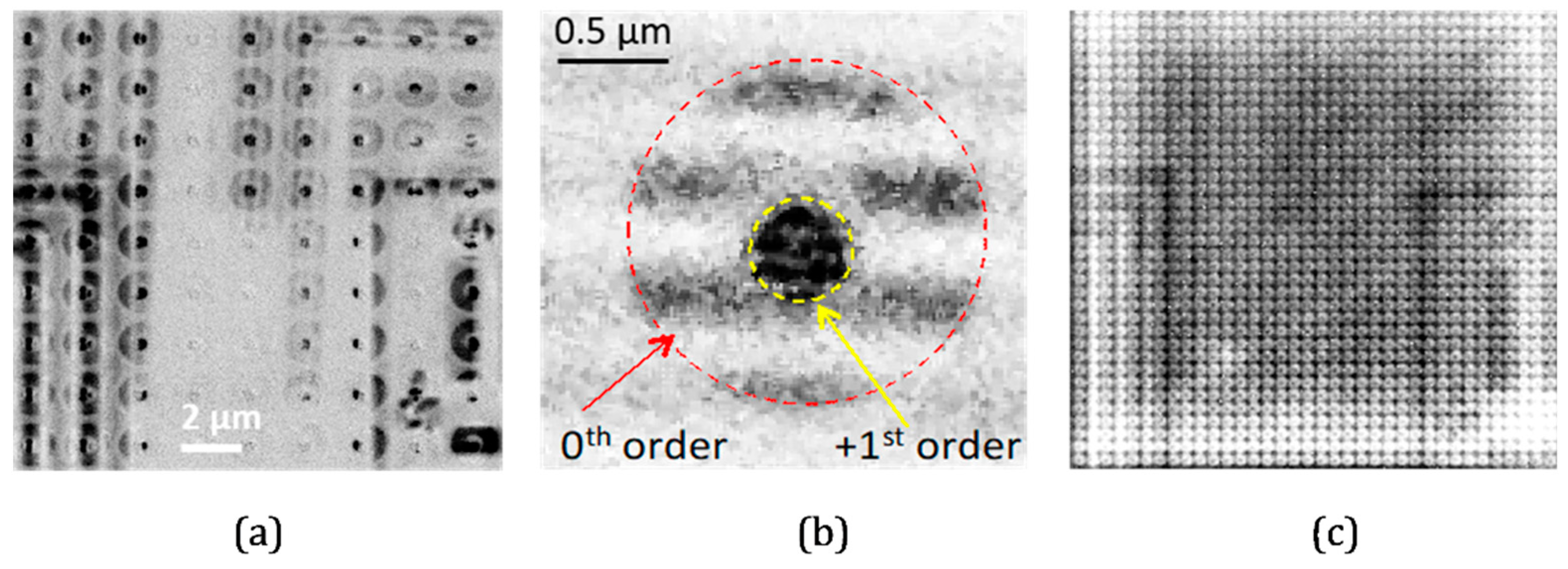
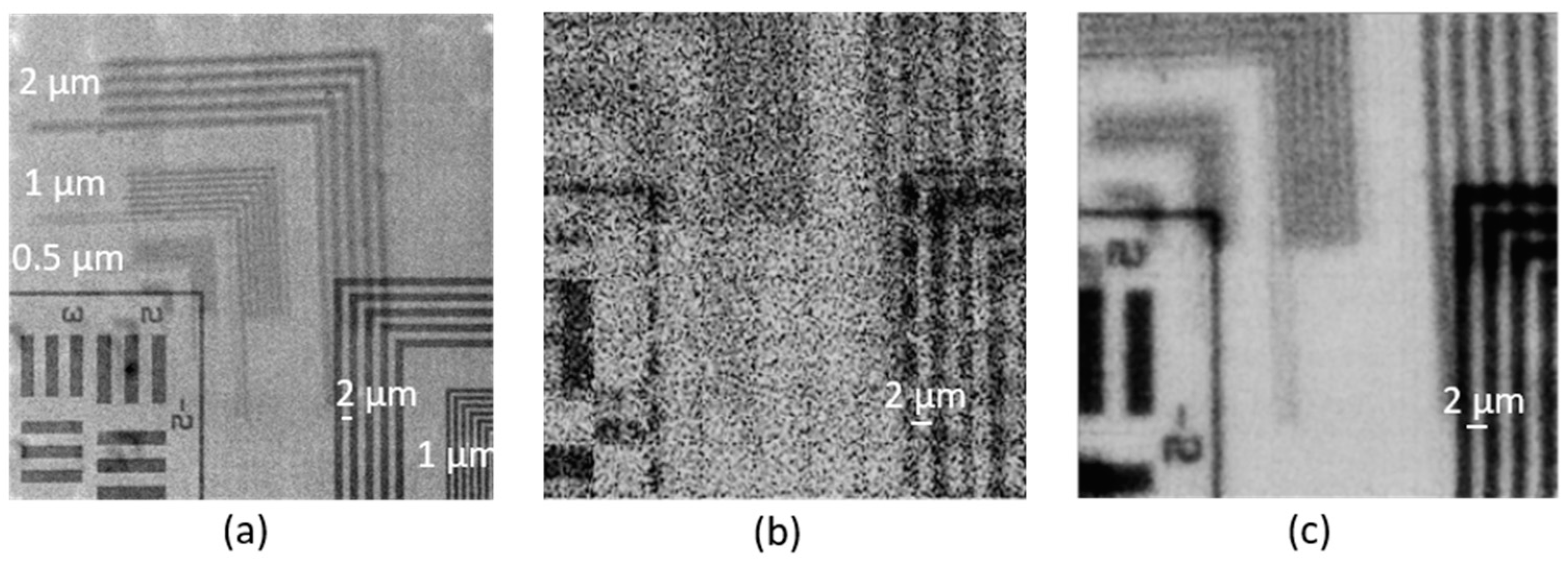
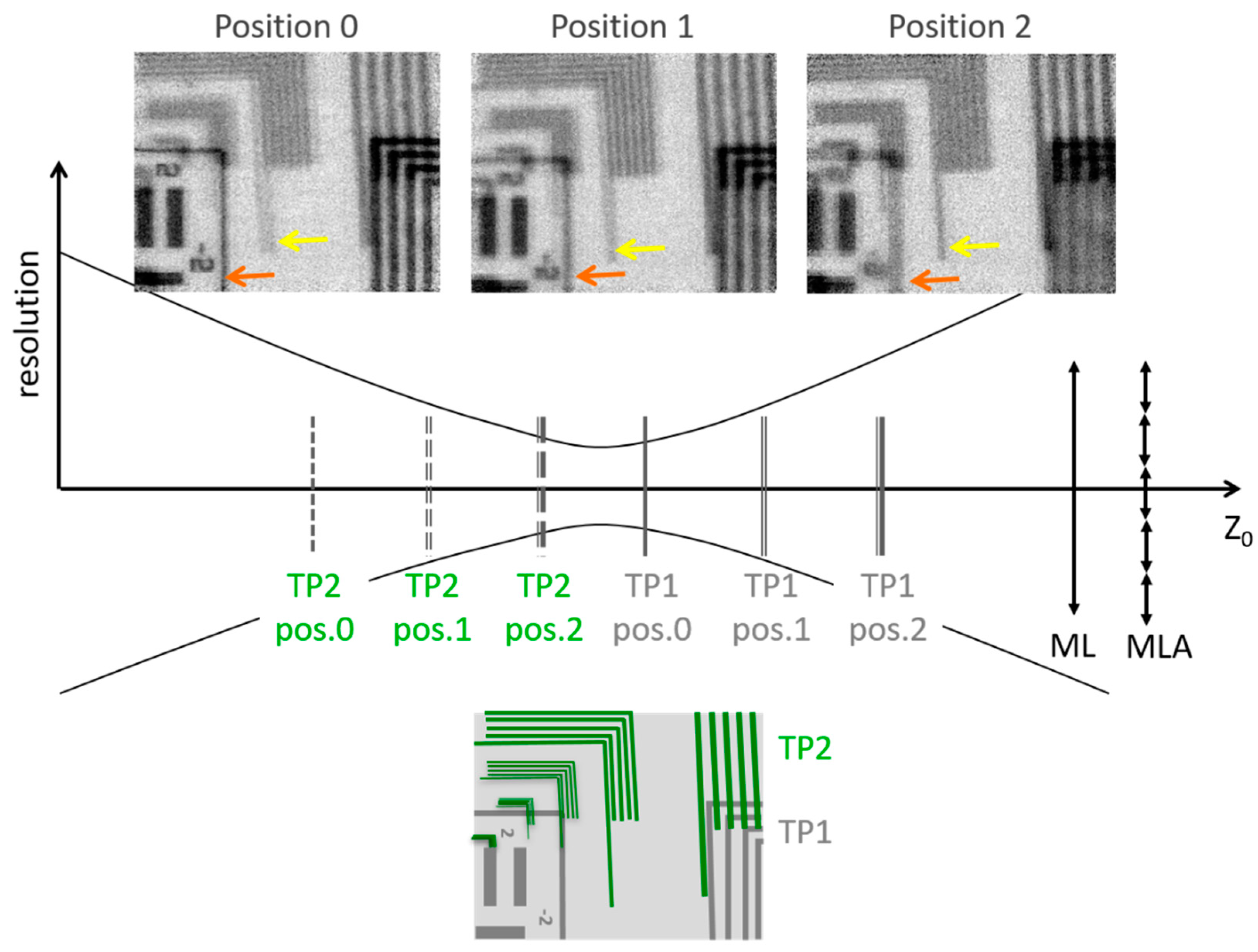
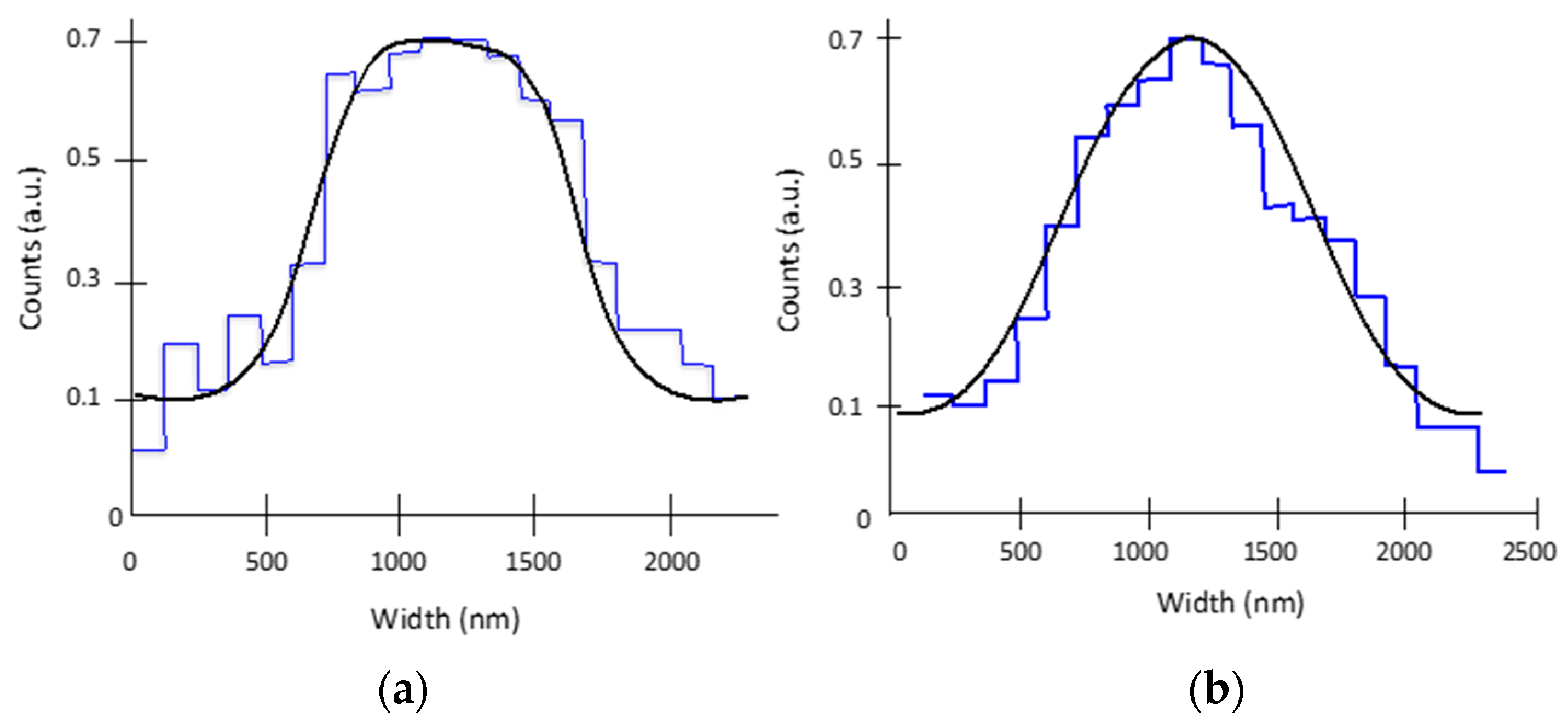
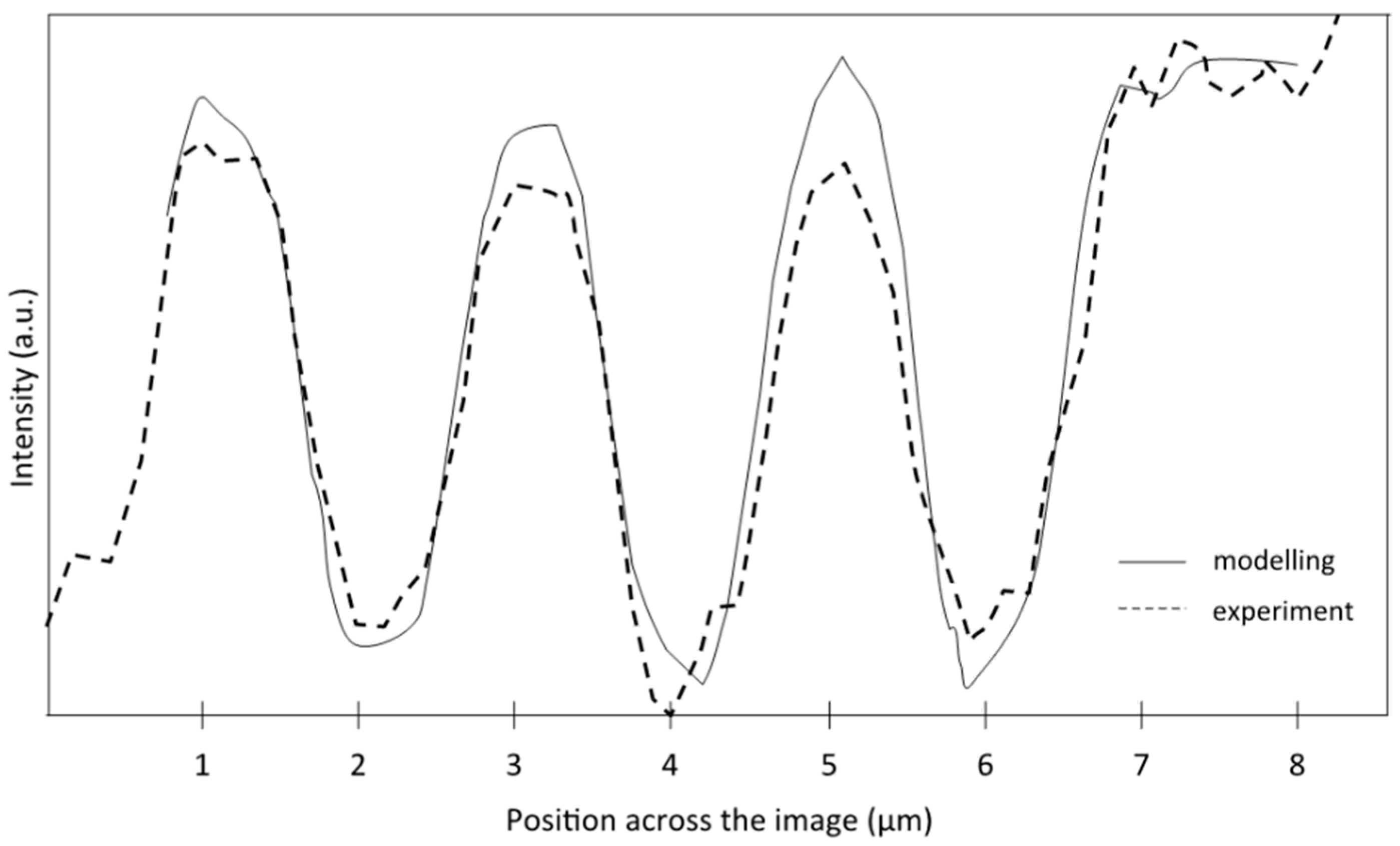
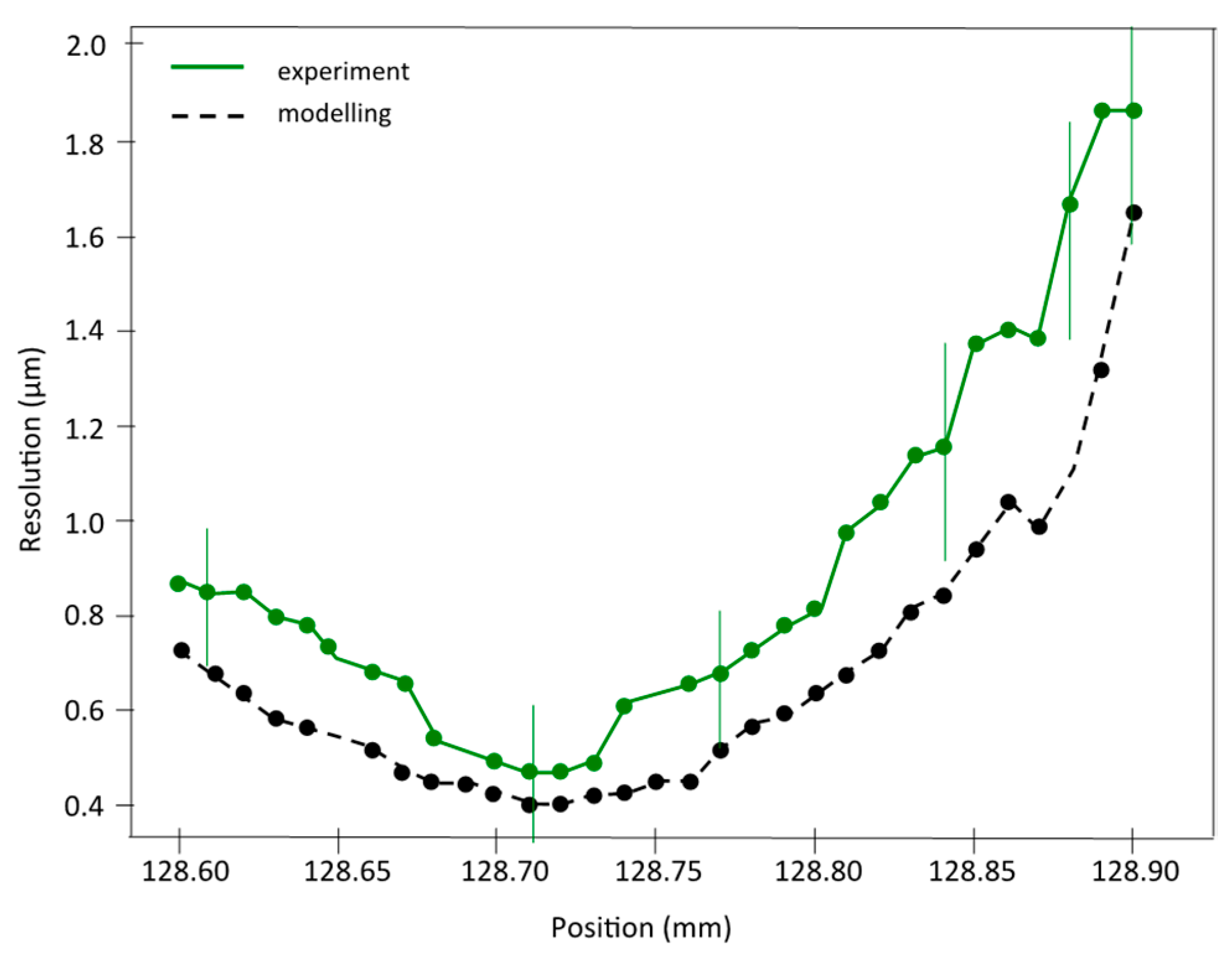
3. Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A

References
- Maire, E.; Buffière, J.Y.; Salvo, L.; Blandin, J.J.; Ludwig, W.; Létang, J.M. On the application of X-ray microtomography in the field of materials science. Adv. Eng. Mater. 2001, 3, 539–546. [Google Scholar] [CrossRef]
- Bukreeva, I.; Asadchikov, V.; Buzmakov, A.; Chukalina, M.; Ingacheva, A.; Korolev, N.A.; Bravin, A.; Mittone, A.; Biella, G.E.M.; Sierra, A.; et al. High resolution 3D visualization of the spinal cord in a post-mortem murine model. Biomed. Opt. Express 2020, 11, 2235. [Google Scholar] [CrossRef] [PubMed]
- Larabell, C.A.; le Gros, M.A. X-ray Tomography Generates 3-D Reconstructions of the Yeast, Saccharomyces cerevisiae, at 60-nm Resolution. Mol. Biol. Cell. 2004, 15, 957–962. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Longo, E.; Sancey, L.; Cedola, A.; Barbier, E.L.; Bravin, A.; Brun, F.; Bukreeva, I.; Fratini, M.; Massimi, L.; Greving, I.; et al. 3D Spatial Distribution of Nanoparticles in Mice Brain Metastases by X-ray Phase-Contrast Tomography. Front. Oncol. 2021, 11, 1828. [Google Scholar] [CrossRef] [PubMed]
- Stampanoni, M.; Groso, A.; Isenegger, A.; Mikuljan, G.; Chen, Q.; Bertrand, A.; Henein, S.; Betemps, R.; Frommherz, U.; Böhler, P.; et al. Trends in synchrotron-based tomographic imaging: The SLS experience. In Developments in X-ray Tomography V; Proceedings SPIE 6318; SPIE Optics + Photonics: San Diego, CA, USA, 2006; Volume 63180M, pp. 193–206. [Google Scholar]
- Flenner, S.; Storm, M.; Kubec, A.; Longo, E.; Doring, F.; Pelt, D.M.; David, C.; Muller, M.; Greving, I. Pushing the temporal resolution in absorption and Zernike phase contrast nanotomography: Enabling fast in situ experiments. J. Synchrotron Radiat. 2020, 27, 1339–1346. [Google Scholar] [CrossRef]
- Duarte, J.; Cassin, R.; Huijts, J.; Iwan, B.; Fortuna, F.; Delbecq, L.; Chapman, H.; Fajardo, M.; Kovacev, M.; Boutu, W.; et al. Computed stereo lensless X-ray imaging. Nat. Photonics 2019, 13, 449–453. [Google Scholar] [CrossRef] [Green Version]
- Hoshino, M.; Uesugi, K.; Pearson, J.; Sonobe, T.; Shirai, M.; Yagi, N. Development of an X-ray real-time stereo imaging technique using synchrotron radiation. J. Synchrotron Radiat. 2011, 18, 569–574. [Google Scholar] [CrossRef] [Green Version]
- Chen, C.C.; Jiang, H.; Rong, L.; Salha, S.; Xu, R.; Mason, T.G.; Miao, J. Three-dimensional imaging of a phase object from a single sample orientation using an optical laser. Phys. Rev. B—Condens. Matter Mater. Phys. 2011, 84, 224104. [Google Scholar] [CrossRef] [Green Version]
- Ng, R.; Levoy, M.; Duval, G.; Horowitz, M.; Hanrahan, P. Light Field Photography with a Hand-Held Plenoptic Camera; Stanford Tech Report CTSR; Stanford University: Stanford, CA, USA, 2005; Volume 2. [Google Scholar]
- Georgiev, T.G.; Lumsdaine, A. Focused plenoptic camera and rendering. J. Electron. Imaging 2010, 19, 021106. [Google Scholar]
- Viganò, N.; Lucka, F.; de la Rochefoucauld, O.; Coban, S.B.; van Liere, R.; Fajardo, M.; Zeitoun, P.; Batenburg, K.J. Emulation of X-ray Light-Field Cameras. J. Imaging 2020, 6, 138. [Google Scholar] [CrossRef]
- Viganò, N.; der Sarkissian, H.; Herzog, C.; de la Rochefoucauld, O.; van Liere, R.; Batenburg, K.J. Tomographic approach for the quantitative scene reconstruction from light field images. Opt. Express 2018, 26, 22574–22602. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sowa, K.M.; Kujda, M.P.; Korecki, P. Plenoptic X-ray microscopy. Appl. Phys. Lett. 2020, 116, 014103. [Google Scholar] [CrossRef]
- Sowa, K.M.; Jany, B.R.; Korecki, P. Multipoint-projection X-ray microscopy. Optica 2018, 5, 577–582. [Google Scholar] [CrossRef]
- Bishop, T.E.; Favaro, P. The light field camera: Extended depth of field, aliasing, and superresolution. IEEE Trans. Pattern Anal. Mach. Intell. 2012, 34, 972–986. [Google Scholar] [CrossRef] [PubMed]
- Ogurreck, M.; Wilde, F.; Herzen, J.; Beckmann, F.; Nazmov, V.; Mohr, J.; Haibel, A.; Müller, M.; Schreyer, A. The nanotomography endstation at the PETRA III imaging beamline. J. Phys. Conf. Ser. 2013, 425, 182002. [Google Scholar] [CrossRef] [Green Version]
- Greving, I.; Wilde, F.; Ogurreck, M.; Herzen, J.; Hammel, J.U.; Hipp, A.; Friedrich, F.; Lottermoser, L.; Dose, T.; Burmester, H.; et al. P05 imaging beamline at PETRA III: First results. In Developments in X-ray Tomography IX; SPIE Optical Engineering + Applications: San Diego, CA, USA, 2014; Volume 9212, p. 92120O. [Google Scholar]
- Jefimovs, K.; Vila-Comamala, J.; Stampanoni, M.; Kaulich, B.; David, C. Beam shaping condenser lenses for full-field transmission X-ray microscopy. J. Synchrotorn Radiat. 2008, 15, 106–108. [Google Scholar] [CrossRef] [Green Version]
- Vartiainen, I.; Holzner, C.; Mohacsi, I.; Karvinen, P.; Diaz, A.; David, C. Artifact characterization and reduction in scanning X-ray Zernike phase contrast microscopy. Opt. Express 2015, 23, 13278–13294. [Google Scholar] [CrossRef] [Green Version]
- Herzog, C.; Granier, X.; Harms, F.; Zeitoun, P.; de la Rochefoucauld, O. Study of contrast variations with depth in focused plenoptic cameras. Opt. Lett. 2019, 44, 4825. [Google Scholar] [CrossRef] [PubMed]
- Herzog, C. Plenoptic Imaging: From Visible Light to X-rays. 2020. Available online: https://tel.archives-ouvertes.fr/tel-03129863 (accessed on 1 January 2021).
- Zhang, B.; Zerubia, J.; Olivo-Marin, J.-C. Gaussian approximations of fluorescence microscope point-spread function models. Appl. Opt. 2007, 46, 1819–1829. [Google Scholar] [CrossRef]
- Döring, F.; Robisch, A.L.; Eberl, C.; Osterhoff, M.; Ruhlandt, A.; Liese, T.; Schlenkrich, F.; Hoffmann, S.; Bartels, M.; Salditt, T.; et al. Sub-5 nm hard x-ray point focusing by a combined Kirkpatrick-Baez mirror and multilayer zone plate. Opt. Express 2013, 21, 19311. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mimura, H.; Yumoto, H.; Matsuyama, S.; Koyama, T.; Tono, K.; Inubushi, Y.; Togashi, T.; Sato, T.; Kim, J.; Fukui, R.; et al. Generation of 1020 Wcm-2 hard X-ray laser pulses with two-stage reflective focusing system. Nat. Commun. 2014, 5, 3539. [Google Scholar] [CrossRef] [PubMed]
- Vila-Comamala, J.; Gorelick, S.; Färm, E.; Kewish, C.M.; Diaz, A.; Barrett, R.; Guzenko, V.A.; Ritala, M.; David, C. Ultra-high resolution zone-doubled diffractive X-ray optics for the multi-keV regime. Opt. Express 2011, 19, 175–184. [Google Scholar] [CrossRef] [PubMed] [Green Version]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Longo, E.; Alj, D.; Batenburg, J.; de La Rochefoucauld, O.; Herzog, C.; Greving, I.; Li, Y.; Lyubomirskiy, M.; Falch, K.V.; Estrela, P.; et al. Flexible Plenoptic X-ray Microscopy. Photonics 2022, 9, 98. https://doi.org/10.3390/photonics9020098
Longo E, Alj D, Batenburg J, de La Rochefoucauld O, Herzog C, Greving I, Li Y, Lyubomirskiy M, Falch KV, Estrela P, et al. Flexible Plenoptic X-ray Microscopy. Photonics. 2022; 9(2):98. https://doi.org/10.3390/photonics9020098
Chicago/Turabian StyleLongo, Elena, Domenico Alj, Joost Batenburg, Ombeline de La Rochefoucauld, Charlotte Herzog, Imke Greving, Ying Li, Mikhail Lyubomirskiy, Ken Vidar Falch, Patricia Estrela, and et al. 2022. "Flexible Plenoptic X-ray Microscopy" Photonics 9, no. 2: 98. https://doi.org/10.3390/photonics9020098
APA StyleLongo, E., Alj, D., Batenburg, J., de La Rochefoucauld, O., Herzog, C., Greving, I., Li, Y., Lyubomirskiy, M., Falch, K. V., Estrela, P., Flenner, S., Viganò, N., Fajardo, M., & Zeitoun, P. (2022). Flexible Plenoptic X-ray Microscopy. Photonics, 9(2), 98. https://doi.org/10.3390/photonics9020098





