Two Polarization Comb Dynamics in VCSELs Subject to Optical Injection
Abstract
:1. Introduction
2. Experimental Polarization Dynamics
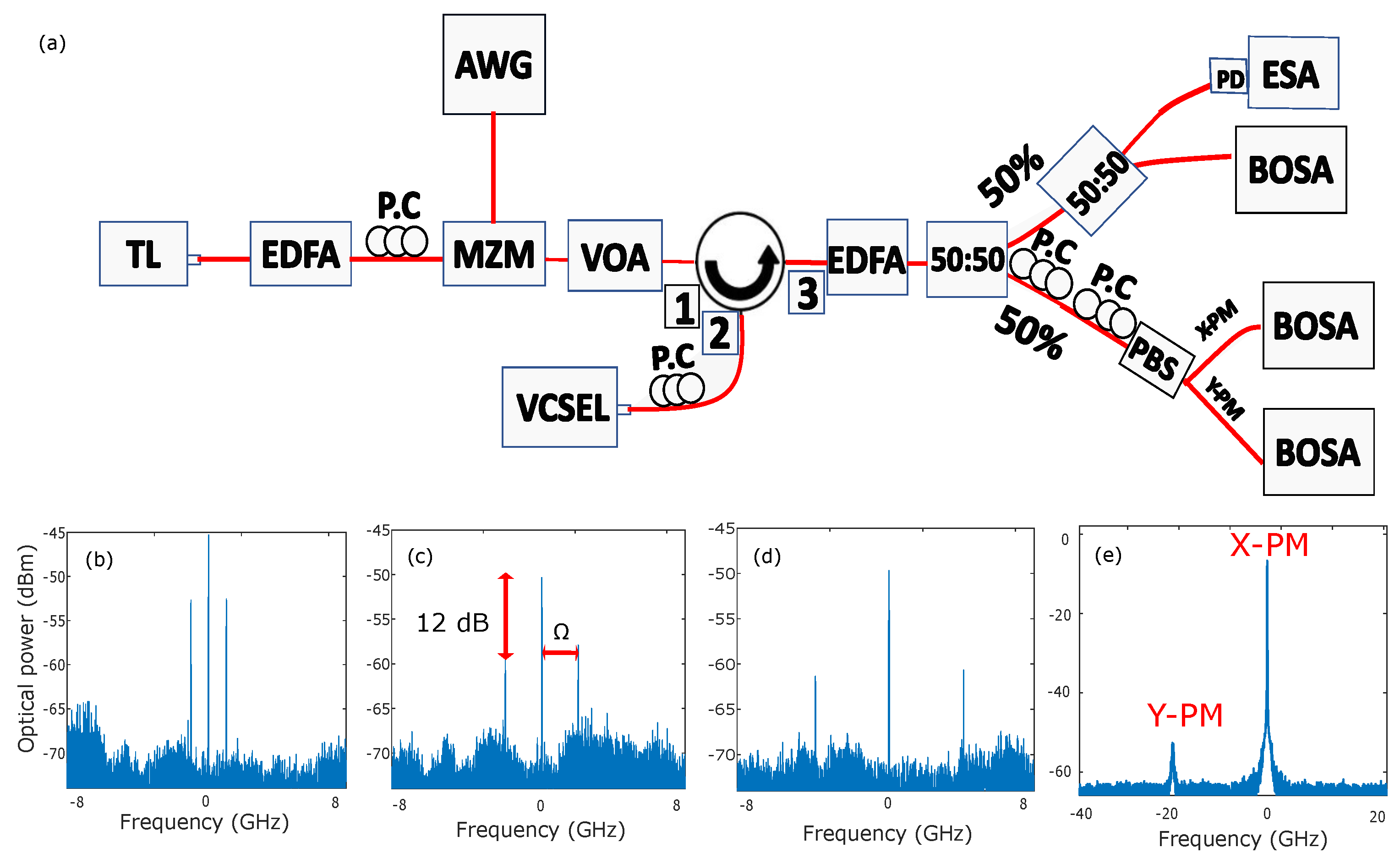
2.1. Experimental Setup
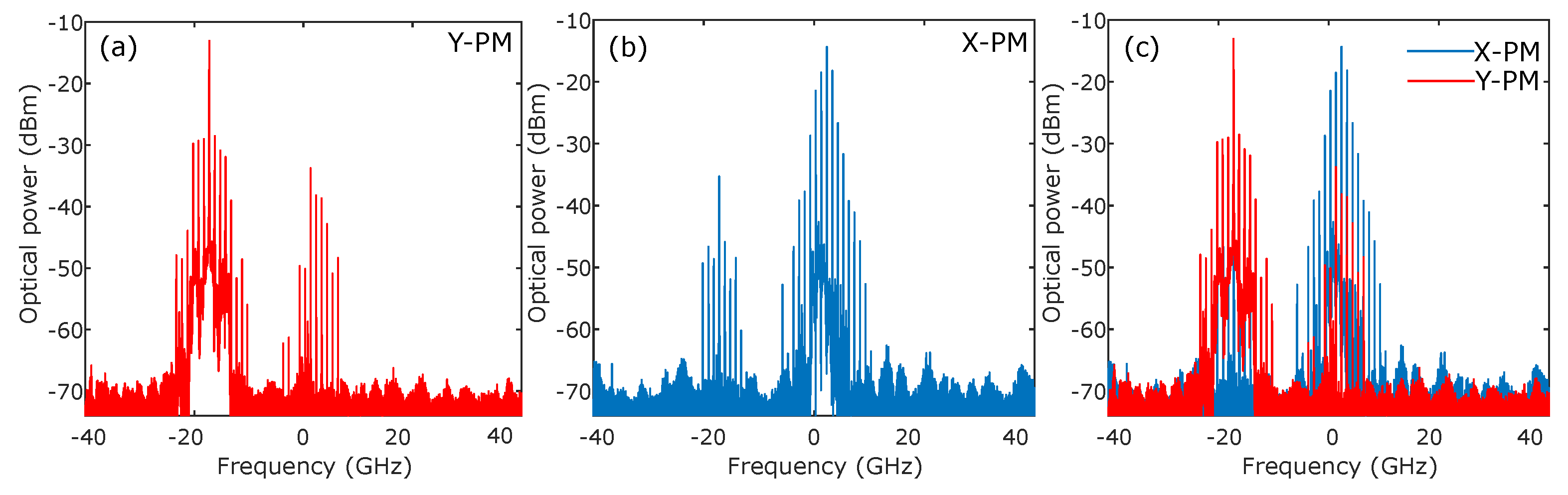
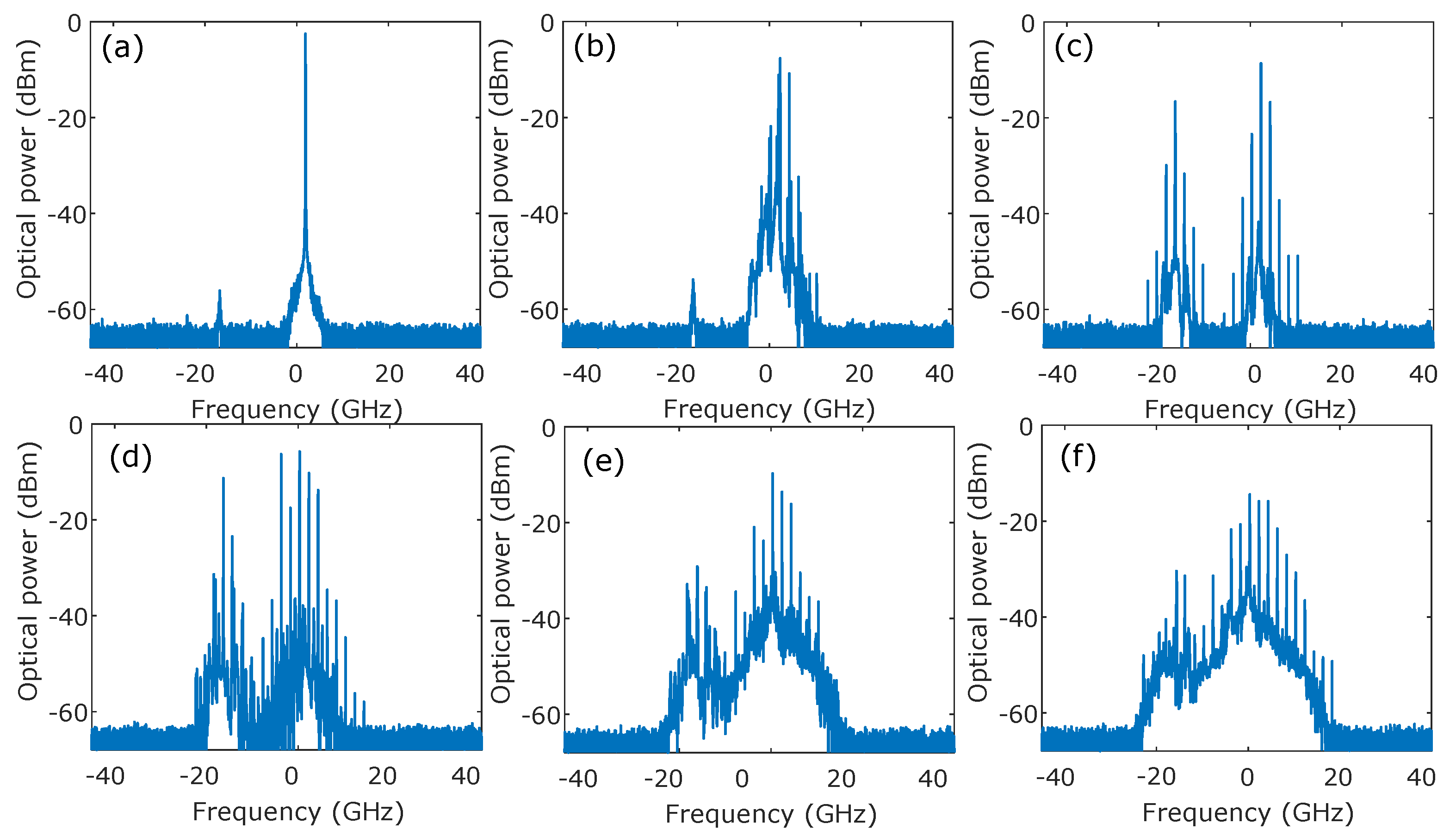
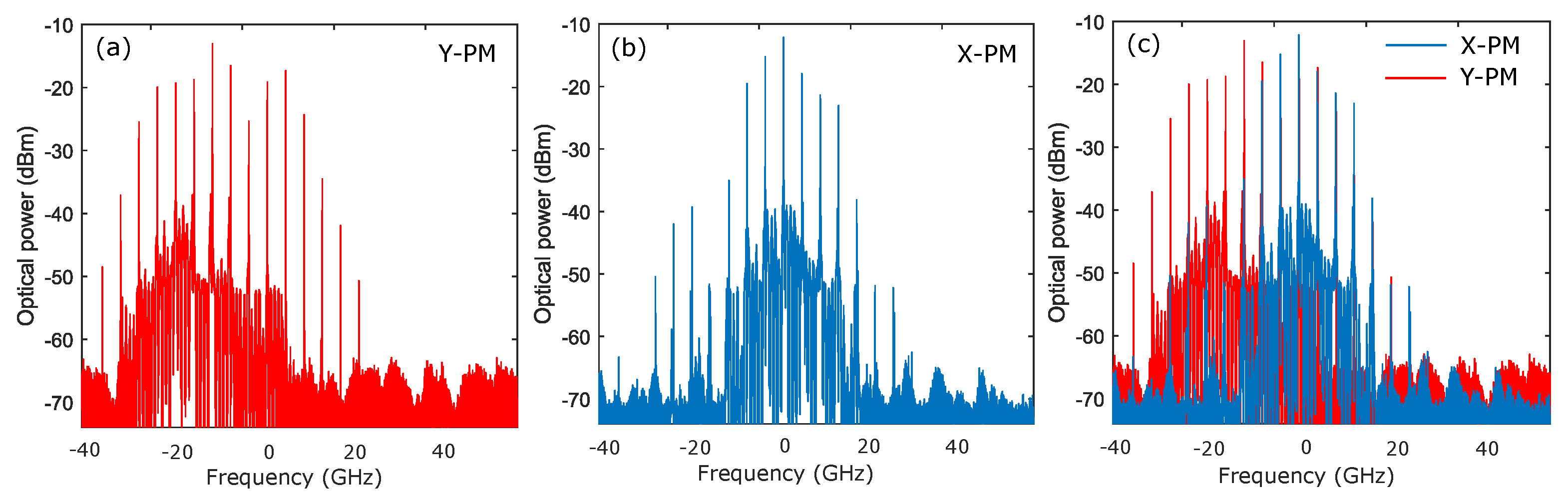
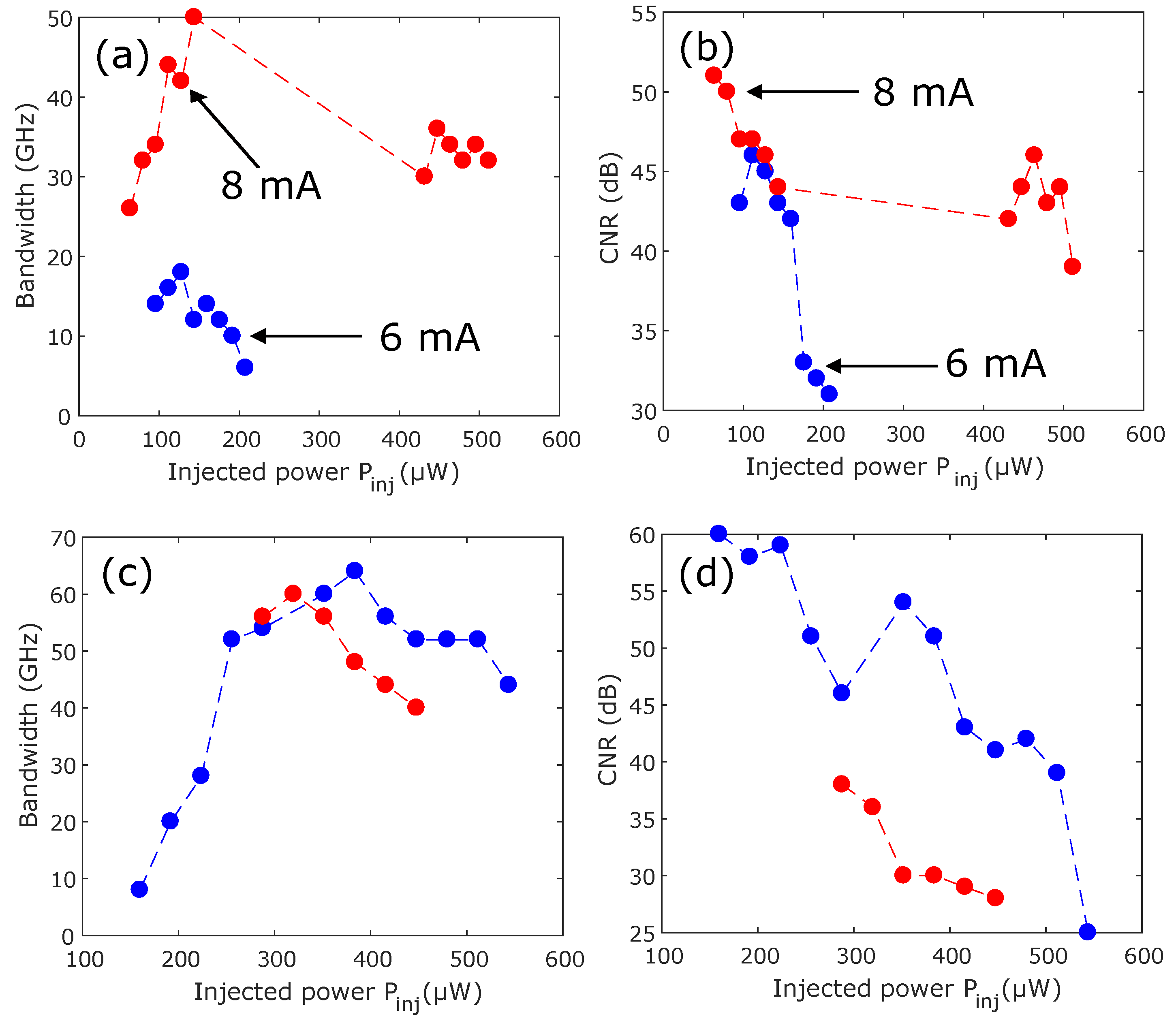
2.2. Impact of the Injected Comb Spacing
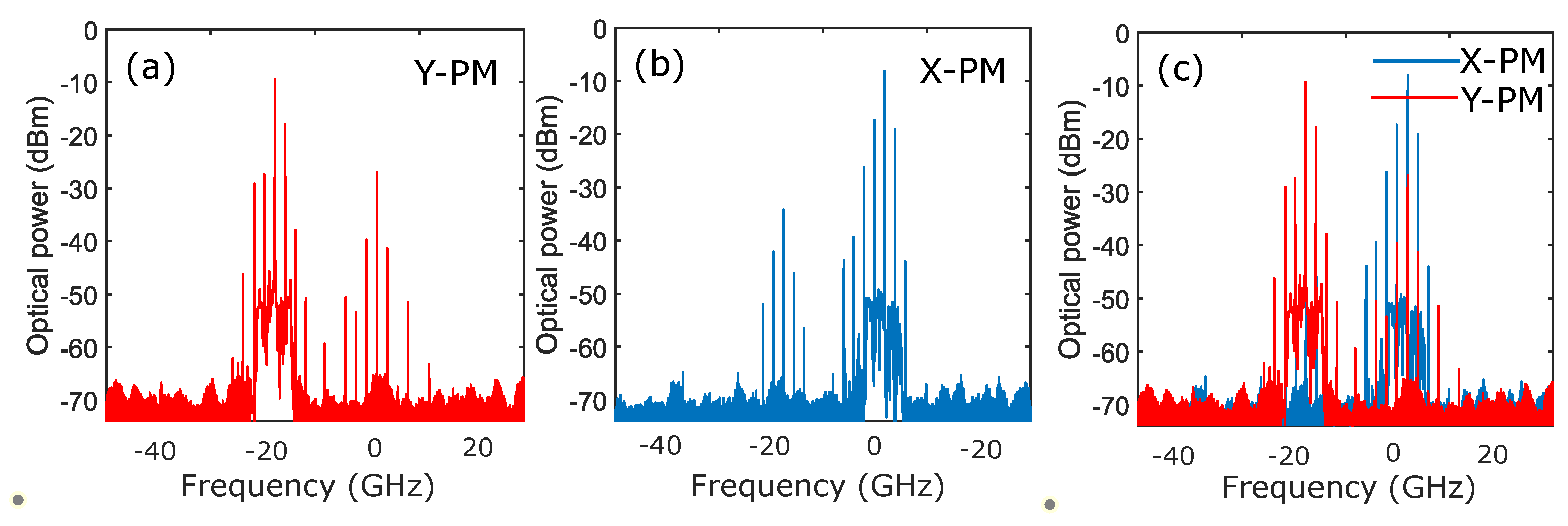
2.3. Influence of the Injection Current
2.4. Tailoring Comb Properties
3. Theoretical Bifurcation Analysis
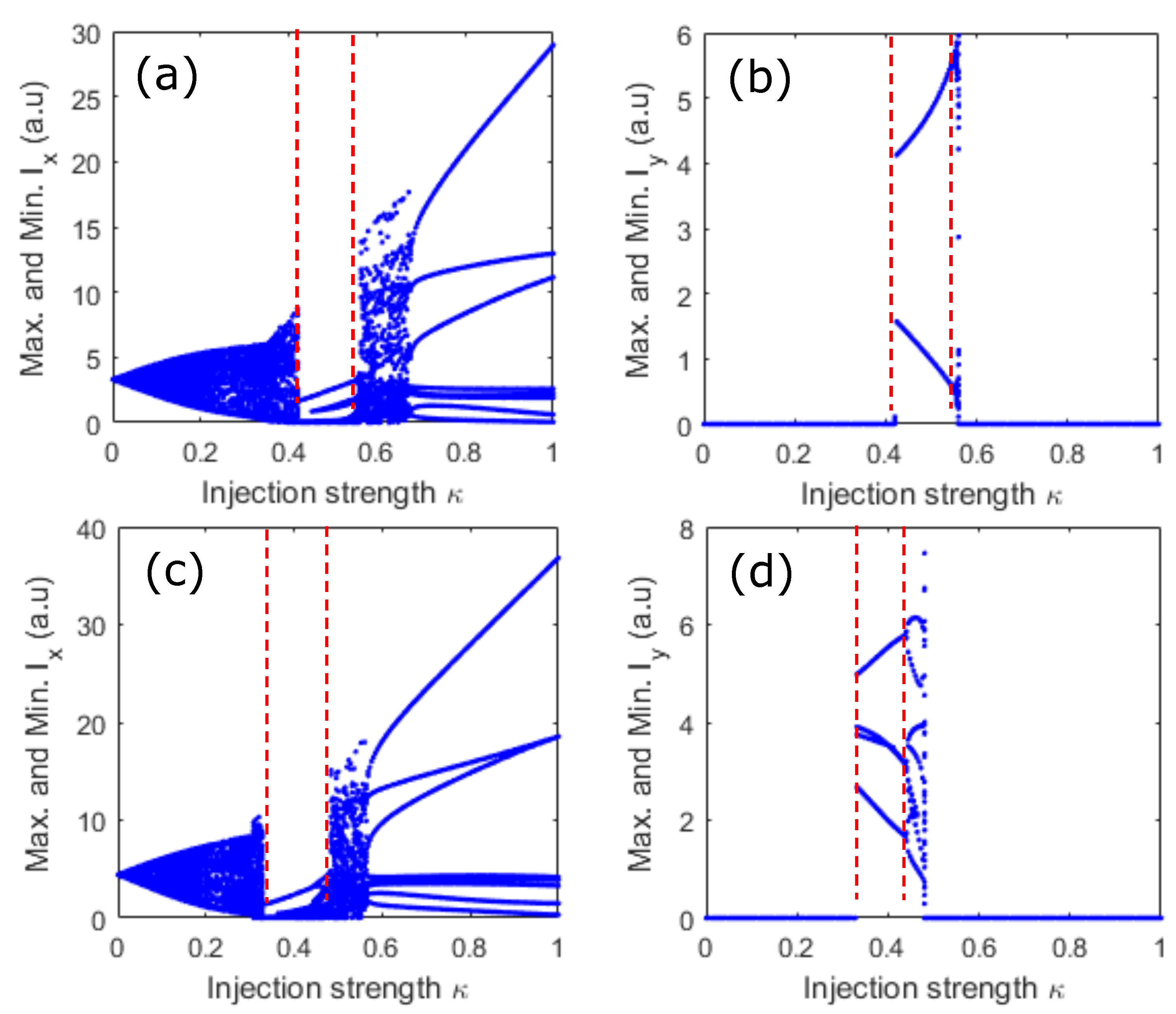
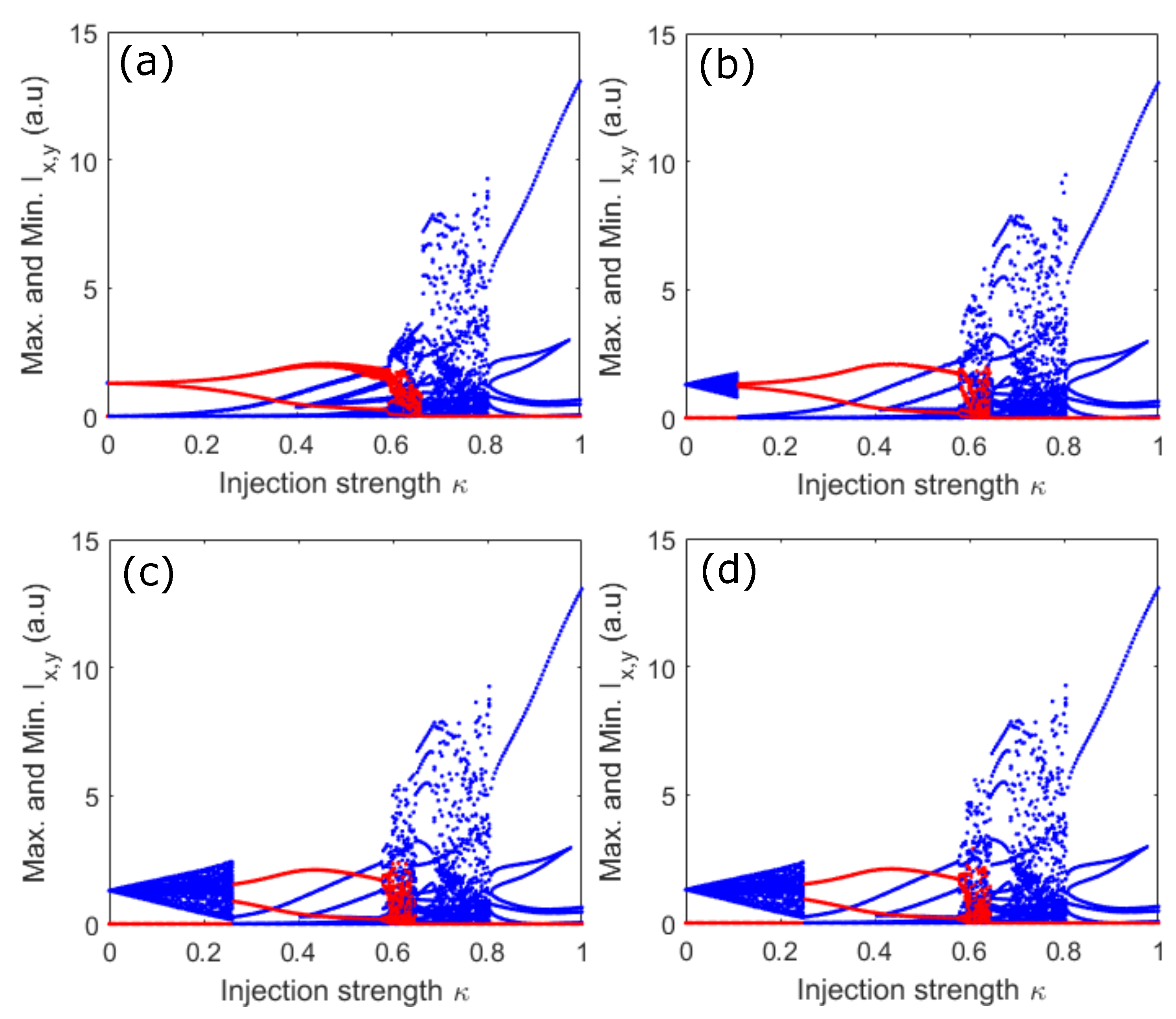
3.1. Y-PM Comb Dynamics
3.2. Influence of Spin-Flip Relaxation Rate
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Sciamanna, M.; Shore, K.A. Physics and applications of laser diode chaos. Nat. Photonics 2015, 9, 151–162. [Google Scholar] [CrossRef] [Green Version]
- Wieczorek, S.; Krauskopf, B.; Simpson, T.B.; Lenstra, D. The dynamical complexity of optically injected semiconductor lasers. Phys. Rep. 2005, 416, 1–128. [Google Scholar] [CrossRef]
- Mogensen, F.; Olesen, H.; Jacobsen, G. Locking conditions and stability properties for a semiconductor laser with external light injection. IEEE J. Quantum Electron. 1985, 21, 784–793. [Google Scholar] [CrossRef]
- Larsson, A. Advances in VCSELs for Communication and Sensing. IEEE J. Sel. Top. Quantum Electron. 2011, 17, 1552–1567. [Google Scholar] [CrossRef]
- Sciamanna, M.; Panajotov, K.; Thienpont, H.; Veretennicoff, I.; Mégret, P.; Blondel, M. Optical feedback induces polarization mode hopping in vertical-cavity surface-emitting lasers. Opt. Lett. 2003, 28, 1543–1545. [Google Scholar] [CrossRef] [PubMed]
- Sciamanna, M.; Panajotov, K. Route to polarization switching induced by optical injection in vertical-cavity surface-emitting lasers. Phys. Rev. A 2006, 73, 023811. [Google Scholar] [CrossRef]
- Altés, J.B.; Gatare, I.; Panajotov, K.; Thienpont, H.; Sciamanna, M. Mapping of the dynamics induced by orthogonal optical injection in vertical-cavity surface-emitting lasers. IEEE J. Quantum Electron. 2006, 42, 198–207. [Google Scholar]
- Choquette, K.D.; Schneider, R.P.; Lear, K.L.; Leibenguth, R.E. Gain-dependent polarization properties of vertical-cavity lasers. IEEE J. Sel. Top. Quantum Electron. 1995, 1, 661–666. [Google Scholar] [CrossRef] [Green Version]
- Hurtado, A.; Quirce, A.; Valle, A.; Pesquera, L.; Adams, M.J. Nonlinear dynamics induced by parallel and orthogonal optical injection in 1550 nm vertical-cavity surface-emitting lasers (VCSELs). Opt. Express 2010, 18, 9423–9428. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gatare, I.; Buesa, J.; Thienpont, H.; Panajotov, K.; Sciamanna, M. Polarization switching bistability and dynamics in vertical-cavity surface-emitting laser under orthogonal optical injection. Opt. Quantum Electron. 2006, 38, 429–443. [Google Scholar] [CrossRef]
- Virte, M.; Panajotov, K.; Thienpont, H.; Sciamanna, M. Deterministic polarization chaos from a laser diode. Nat. Photonics 2013, 7, 60–65. [Google Scholar] [CrossRef] [Green Version]
- Quirce, A.; de Dios, C.; Valle, A.; Acedo, P. VCSEL-based optical frequency combs expansion induced by polarized optical injection. IEEE J. Sel. Top. Quantum Electron. 2018, 25, 1–9. [Google Scholar] [CrossRef]
- Minoshima, K.; Matsumoto, H. High-accuracy measurement of 240-m distance in an optical tunnel by use of a compact femtosecond laser. Appl. Opt. 2000, 39, 5512–5517. [Google Scholar] [CrossRef] [PubMed]
- Link, S.M.; Maas, D.; Waldburger, D.; Keller, U. Dual-comb spectroscopy of water vapor with a free-running semiconductor disk laser. Science 2017, 356, 1164–1168. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Marin-Palomo, P.; Kemal, J.N.; Karpov, M.; Kordts, A.; Pfeifle, J.; Pfeiffer, M.H.; Trocha, P.; Wolf, S.; Brasch, V.; Anderson, M.H.; et al. Microresonator-based solitons for massively parallel coherent optical communications. Nature 2017, 546, 274–279. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hargrove, L.; Fork, R.L.; Pollack, M. Locking of He–Ne laser modes induced by synchronous intracavity modulation. Appl. Phys. Lett. 1964, 5, 4–5. [Google Scholar] [CrossRef]
- Del’Haye, P.; Schliesser, A.; Arcizet, O.; Wilken, T.; Holzwarth, R.; Kippenberg, T.J. Optical frequency comb generation from a monolithic microresonator. Nature 2007, 450, 1214–1217. [Google Scholar] [CrossRef] [Green Version]
- Tilma, B.W.; Mangold, M.; Zaugg, C.A.; Link, S.M.; Waldburger, D.; Klenner, A.; Mayer, A.S.; Gini, E.; Golling, M.; Keller, U. Recent advances in ultrafast semiconductor disk lasers. Light. Sci. Appl. 2015, 4, e310. [Google Scholar] [CrossRef] [Green Version]
- Hugi, A.; Villares, G.; Blaser, S.; Liu, H.; Faist, J. Mid-infrared frequency comb based on a quantum cascade laser. Nature 2012, 492, 229–233. [Google Scholar] [CrossRef]
- Silvestri, C.; Columbo, L.L.; Brambilla, M.; Gioannini, M. Coherent multi-mode dynamics in a quantum cascade laser: Amplitude-and frequency-modulated optical frequency combs. Opt. Express 2020, 28, 23846–23861. [Google Scholar] [CrossRef] [PubMed]
- Weber, C.; Columbo, L.L.; Gioannini, M.; Breuer, S.; Bardella, P. Threshold behavior of optical frequency comb self-generation in an InAs/InGaAs quantum dot laser. Opt. Lett. 2019, 44, 3478–3481. [Google Scholar] [CrossRef] [PubMed]
- Grillot, F.; Duan, J.; Dong, B.; Huang, H. Uncovering recent progress in nanostructured light-emitters for information and communication technologies. Light. Sci. Appl. 2021, 10, 156. [Google Scholar] [CrossRef] [PubMed]
- Villares, G.; Hugi, A.; Blaser, S.; Faist, J. Dual-comb spectroscopy based on quantum-cascade-laser frequency combs. Nat. Commun. 2014, 5, 5192. [Google Scholar] [CrossRef] [PubMed]
- Shortiss, K.; Lingnau, B.; Dubois, F.; Kelleher, B.; Peters, F.H. Harmonic frequency locking and tuning of comb frequency spacing through optical injection. Opt. Express 2019, 27, 36976–36989. [Google Scholar] [CrossRef] [Green Version]
- Lingnau, B.; Shortiss, K.; Dubois, F.; Peters, F.H.; Kelleher, B. Universal generation of devil’s staircases near Hopf bifurcations via modulated forcing of nonlinear systems. Phys. Rev. E 2020, 102, 030201. [Google Scholar] [CrossRef] [PubMed]
- Doumbia, Y.; Malica, T.; Wolfersberger, D.; Panajotov, K.; Sciamanna, M. Nonlinear dynamics of a laser diode with an injection of an optical frequency comb. Opt. Express 2020, 28, 30379–30390. [Google Scholar] [CrossRef]
- Doumbia, Y.; Malica, T.; Wolfersberger, D.; Panajotov, K.; Sciamanna, M. Optical injection dynamics of frequency combs. Opt. Lett. 2020, 45, 435–438. [Google Scholar] [CrossRef]
- Doumbia, Y.; Wolfersberger, D.; Panajotov, K.; Sciamanna, M. Tailoring frequency combs through VCSEL polarization dynamics. Opt. Express 2021, 29, 33976–33991. [Google Scholar] [CrossRef]
- Gatare, I.; Sciamanna, M.; Nizette, M.; Panajotov, K. Bifurcation to polarization switching and locking in vertical-cavity surface-emitting lasers with optical injection. Phys. Rev. A 2007, 76, 031803. [Google Scholar] [CrossRef]
- Gatare, I.; Sciamanna, M.; Buesa, J.; Thienpont, H.; Panajotov, K. Nonlinear dynamics accompanying polarization switching in vertical-cavity surface-emitting lasers with orthogonal optical injection. Appl. Phys. Lett. 2006, 88, 101106. [Google Scholar] [CrossRef]
- Valle, A.; Gatare, I.; Panajotov, K.; Sciamanna, M. Transverse mode switching and locking in vertical-cavity surface-emitting lasers subject to orthogonal optical injection. IEEE J. Quantum Electron. 2007, 43, 322–333. [Google Scholar] [CrossRef] [Green Version]
- Panajotov, K.; Gatare, I.; Valle, A.; Thienpont, H.; Sciamanna, M. Polarization-and transverse-mode dynamics in optically injected and gain-switched vertical-cavity surface-emitting lasers. IEEE J. Quantum Electron. 2009, 45, 1473–1481. [Google Scholar] [CrossRef]
- Gatare, I.; Panajotov, K.; Sciamanna, M. Frequency-induced polarization bistability in vertical-cavity surface-emitting lasers with orthogonal optical injection. Phys. Rev. A 2007, 75, 023804. [Google Scholar] [CrossRef]
- Nizette, M.; Sciamanna, M.; Gatare, I.; Thienpont, H.; Panajotov, K. Dynamics of vertical-cavity surface-emitting lasers with optical injection: A two-mode model approach. JOSA B 2009, 26, 1603–1613. [Google Scholar] [CrossRef]
- Sciamanna, M.; Panajotov, K. Two-mode injection locking in vertical-cavity surface-emitting lasers. Opt. Lett. 2005, 30, 2903–2905. [Google Scholar] [CrossRef] [PubMed]
- Hong, Y.; Spencer, P.S.; Rees, P.; Shore, K.A. Optical injection dynamics of two-mode vertical cavity surface-emitting semiconductor lasers. IEEE J. Quantum Electron. 2002, 38, 274–278. [Google Scholar] [CrossRef]
- Denis-le Coarer, F.; Quirce, A.; Valle, Á.; Pesquera, L.; Sciamanna, M.; Thienpont, H.; Panajotov, K. Polarization dynamics induced by parallel optical injection in a single-mode VCSEL. Opt. Lett. 2017, 42, 2130–2133. [Google Scholar] [CrossRef] [PubMed]
- Quirce, A.; Pérez, P.; Popp, A.; Valle, Á.; Pesquera, L.; Hong, Y.; Thienpont, H.; Panajotov, K. Polarization switching and injection locking in vertical-cavity surface-emitting lasers subject to parallel optical injection. Opt. Lett. 2016, 41, 2664–2667. [Google Scholar] [CrossRef] [Green Version]
- Quirce, A.; Popp, A.; Denis-le Coarer, F.; Pérez, P.; Valle, Á.; Pesquera, L.; Hong, Y.; Thienpont, H.; Panajotov, K.; Sciamanna, M. Analysis of the polarization of single-mode vertical-cavity surface-emitting lasers subject to parallel optical injection. JOSA B 2017, 34, 447–455. [Google Scholar] [CrossRef]
- Denis-le Coarer, F.; Quirce, A.; Pérez, P.; Valle, A.; Pesquera, L.; Sciamanna, M.; Thienpont, H.; Panajotov, K. Injection locking and polarization switching bistability in a 1550 nm VCSEL subject to parallel optical injection. IEEE J. Sel. Top. Quantum Electron. 2017, 23, 1–10. [Google Scholar] [CrossRef]
- Guckenheimer, J.; Holmes, P. Nonlinear Oscillations, Dynamical Systems, and Bifurcations of Vector Fields; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2013; Volume 42. [Google Scholar]
- San Miguel, M.; Feng, Q.; Moloney, J.V. Light-polarization dynamics in surface-emitting semiconductor lasers. Phys. Rev. A 1995, 52, 1728. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Doumbia, Y.; Wolfersberger, D.; Panajotov, K.; Sciamanna, M. Two Polarization Comb Dynamics in VCSELs Subject to Optical Injection. Photonics 2022, 9, 115. https://doi.org/10.3390/photonics9020115
Doumbia Y, Wolfersberger D, Panajotov K, Sciamanna M. Two Polarization Comb Dynamics in VCSELs Subject to Optical Injection. Photonics. 2022; 9(2):115. https://doi.org/10.3390/photonics9020115
Chicago/Turabian StyleDoumbia, Yaya, Delphine Wolfersberger, Krassimir Panajotov, and Marc Sciamanna. 2022. "Two Polarization Comb Dynamics in VCSELs Subject to Optical Injection" Photonics 9, no. 2: 115. https://doi.org/10.3390/photonics9020115
APA StyleDoumbia, Y., Wolfersberger, D., Panajotov, K., & Sciamanna, M. (2022). Two Polarization Comb Dynamics in VCSELs Subject to Optical Injection. Photonics, 9(2), 115. https://doi.org/10.3390/photonics9020115





