Photonic-Assisted Receivers for Instantaneous Microwave Frequency Measurement Based on Discriminators of Resonance Type
Abstract
1. Introduction
2. IFM Based on a Fiber Bragg Grating
2.1. Principles of Operation
2.2. Analysis of the Causes of Measurement Errors and Improvement in Measurement Accuracy
2.3. Discussion of Analysis Results
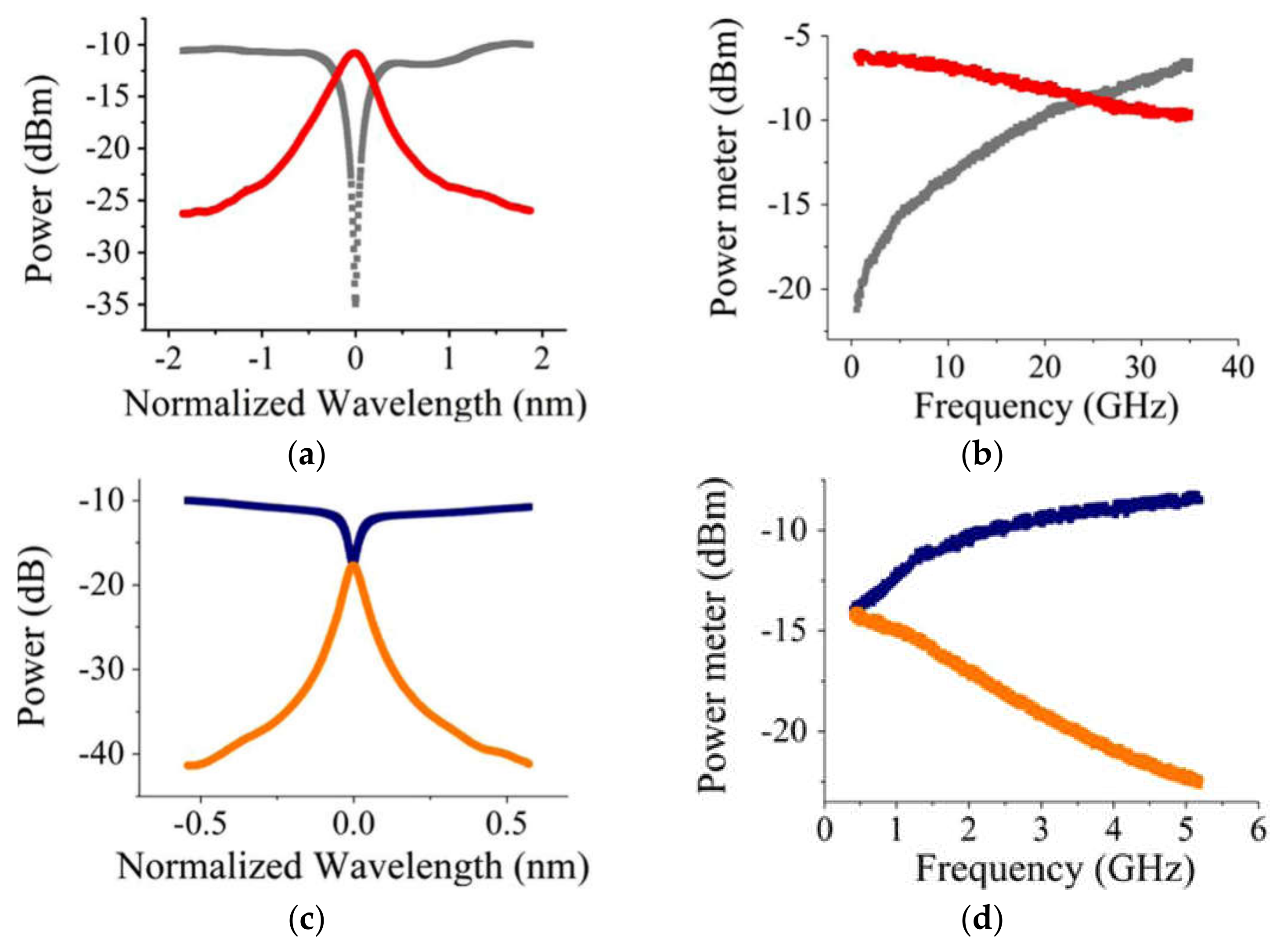
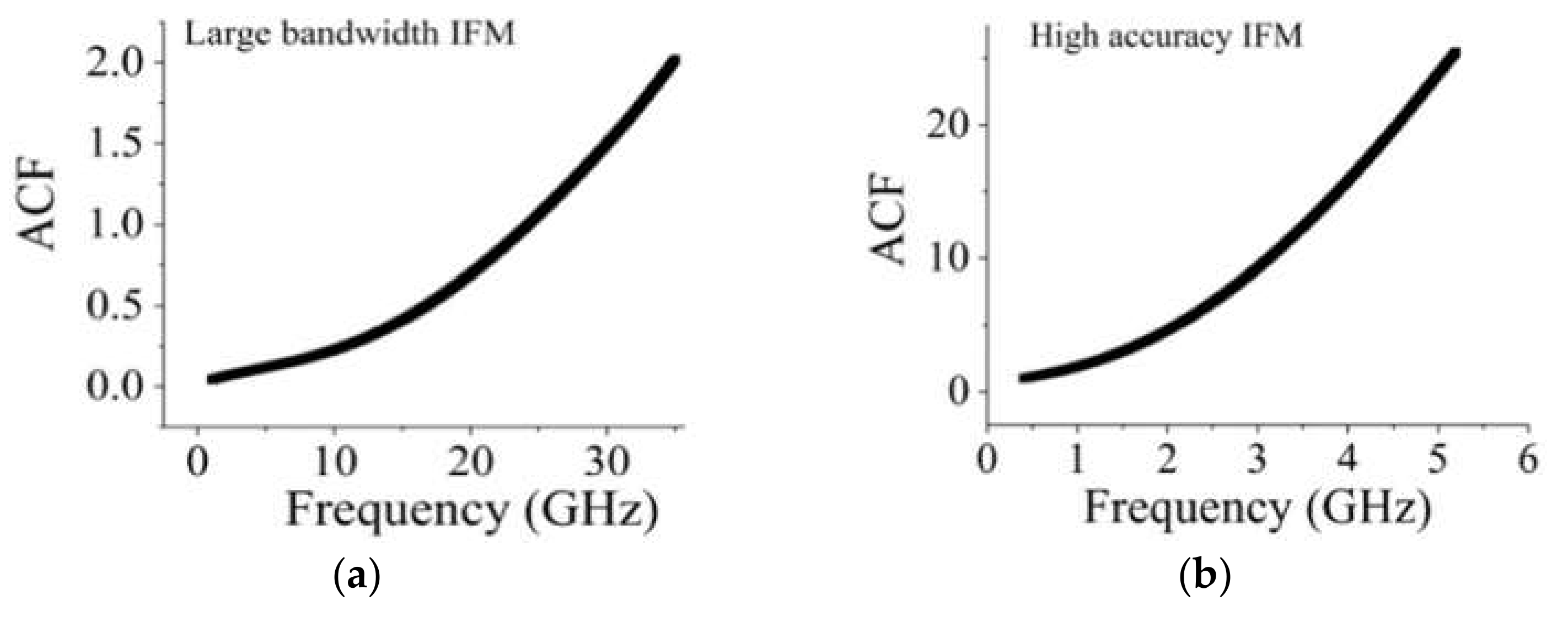
3. IFM Based on Phase-Shifted FBG
3.1. Principles of Operation
3.2. Analysis of the Causes of Measurement Errors and Improvement in Measurement Accuracy
3.3. Discussion of Analysis Results
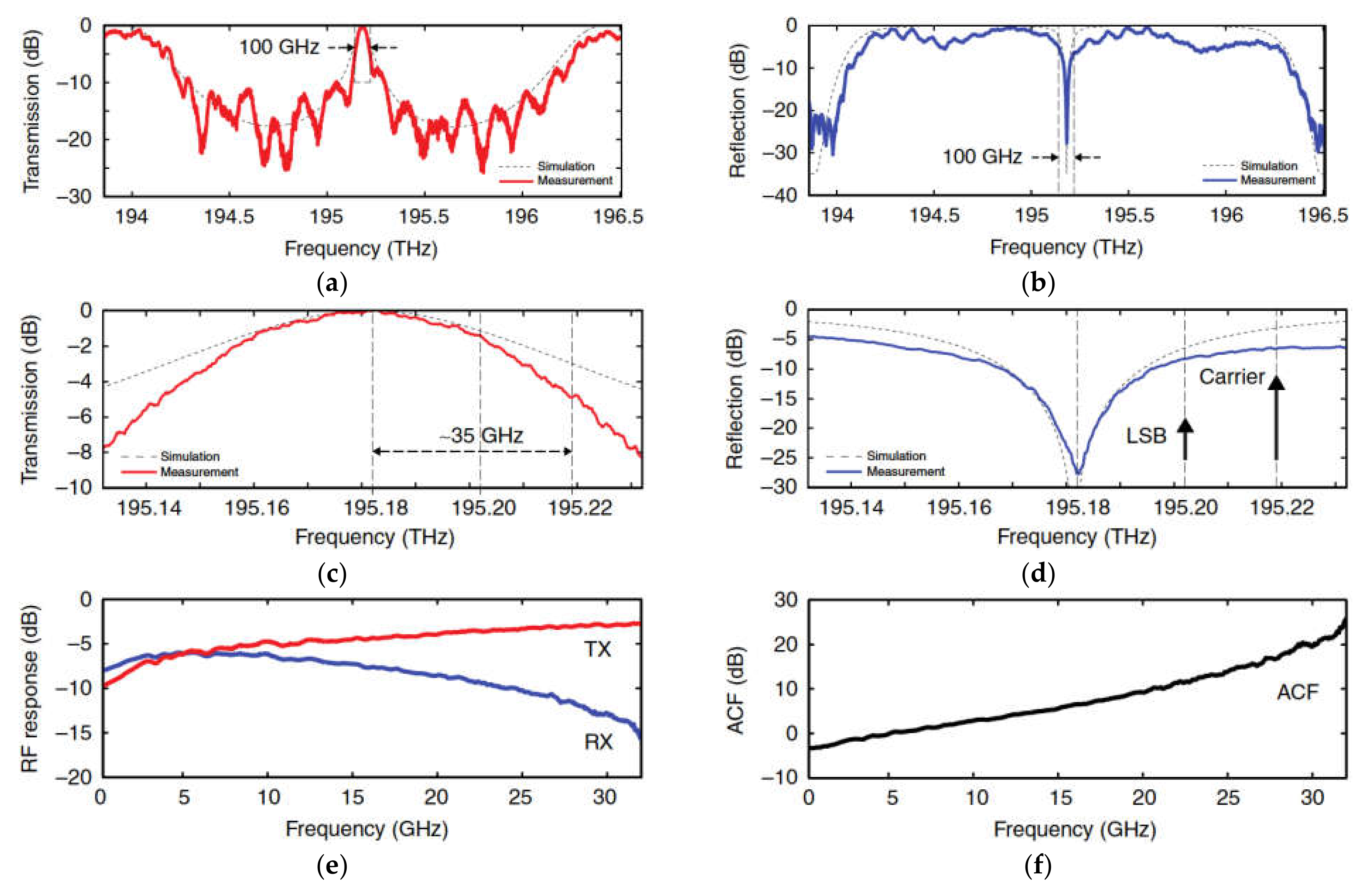
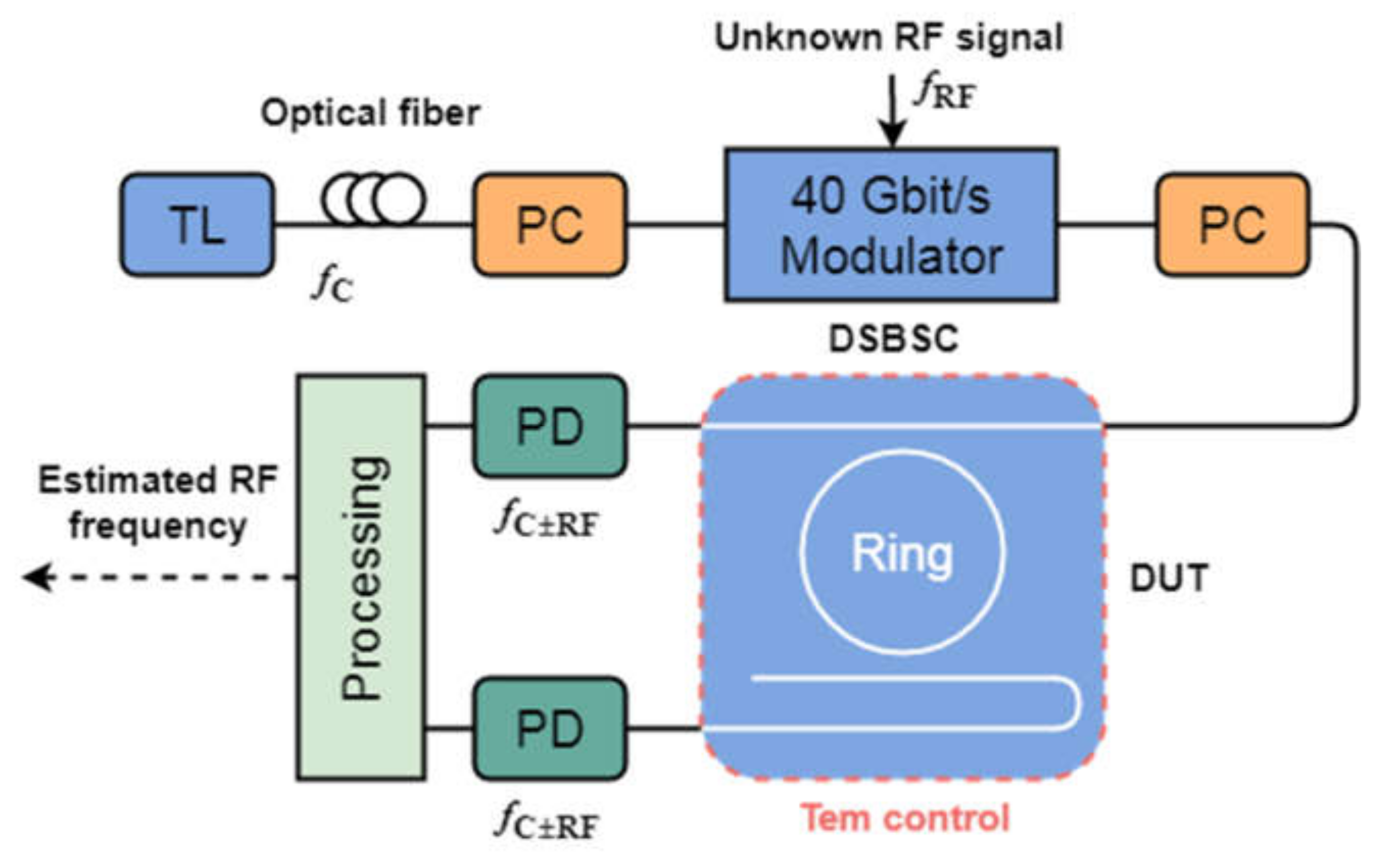
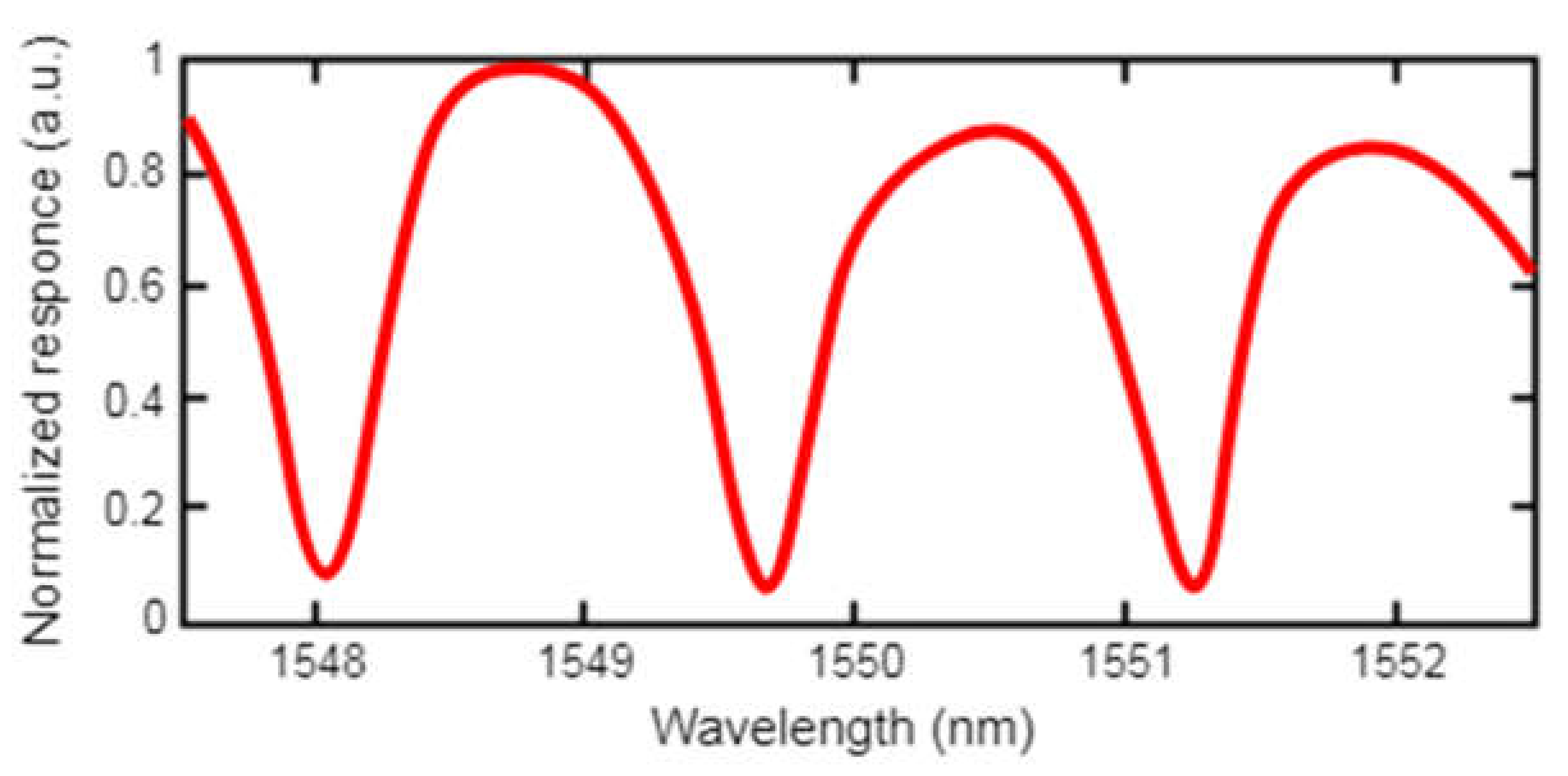
4. IFM Based on an Optical Microring Resonator
4.1. Principles of Operation
4.2. Analysis of the Causes of Measurement Errors and Improvement in Measurement Accuracy
4.3. Discussion of Analysis Results
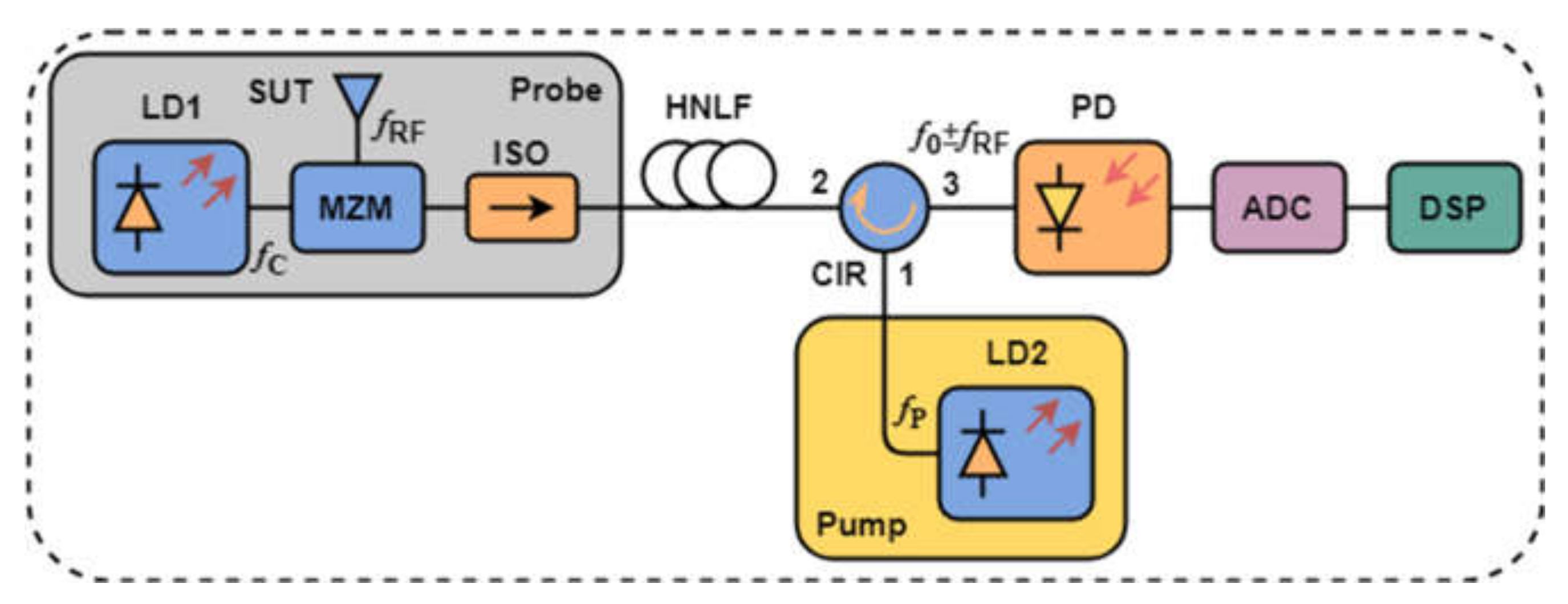
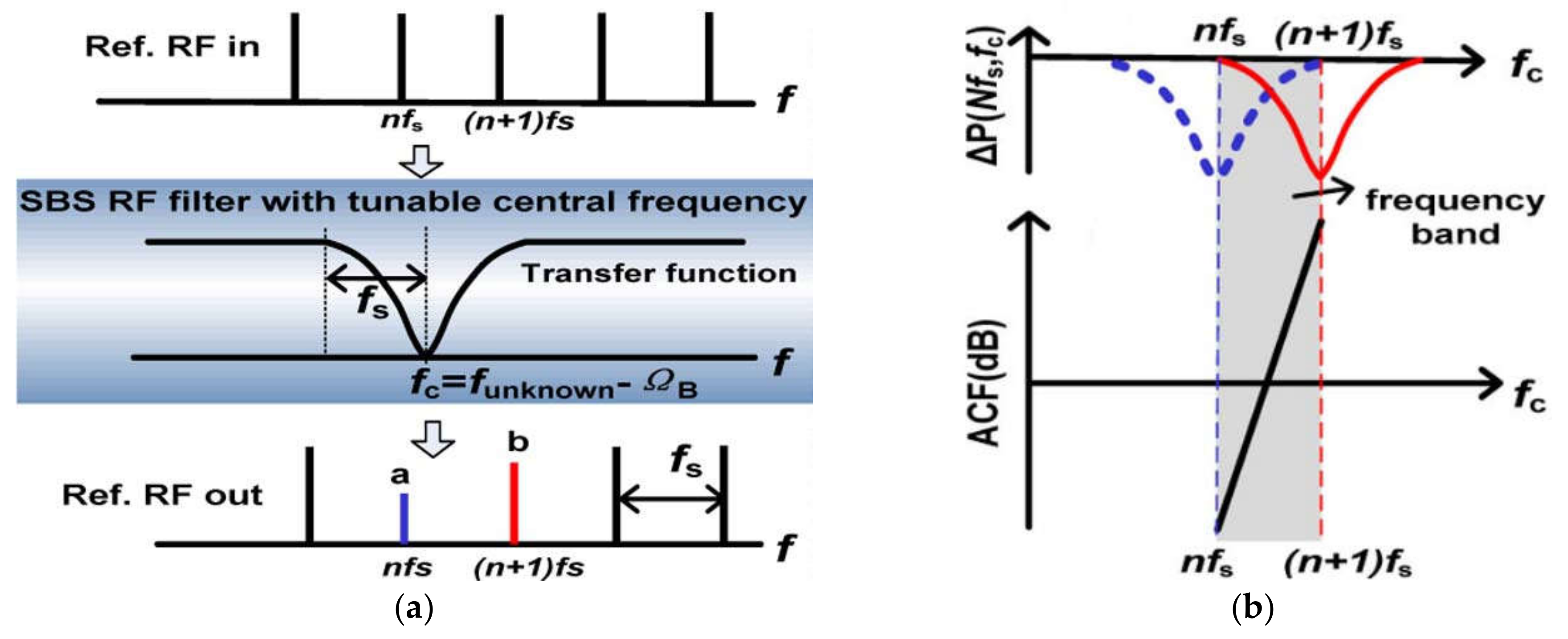
5. IFM Based on Stimulated Brillouin Scattering
5.1. Principles of Operation
5.2. Analysis of the Causes of Measurement Errors and Improvement in Measurement Accuracy
5.3. Discussion of Analysis Results
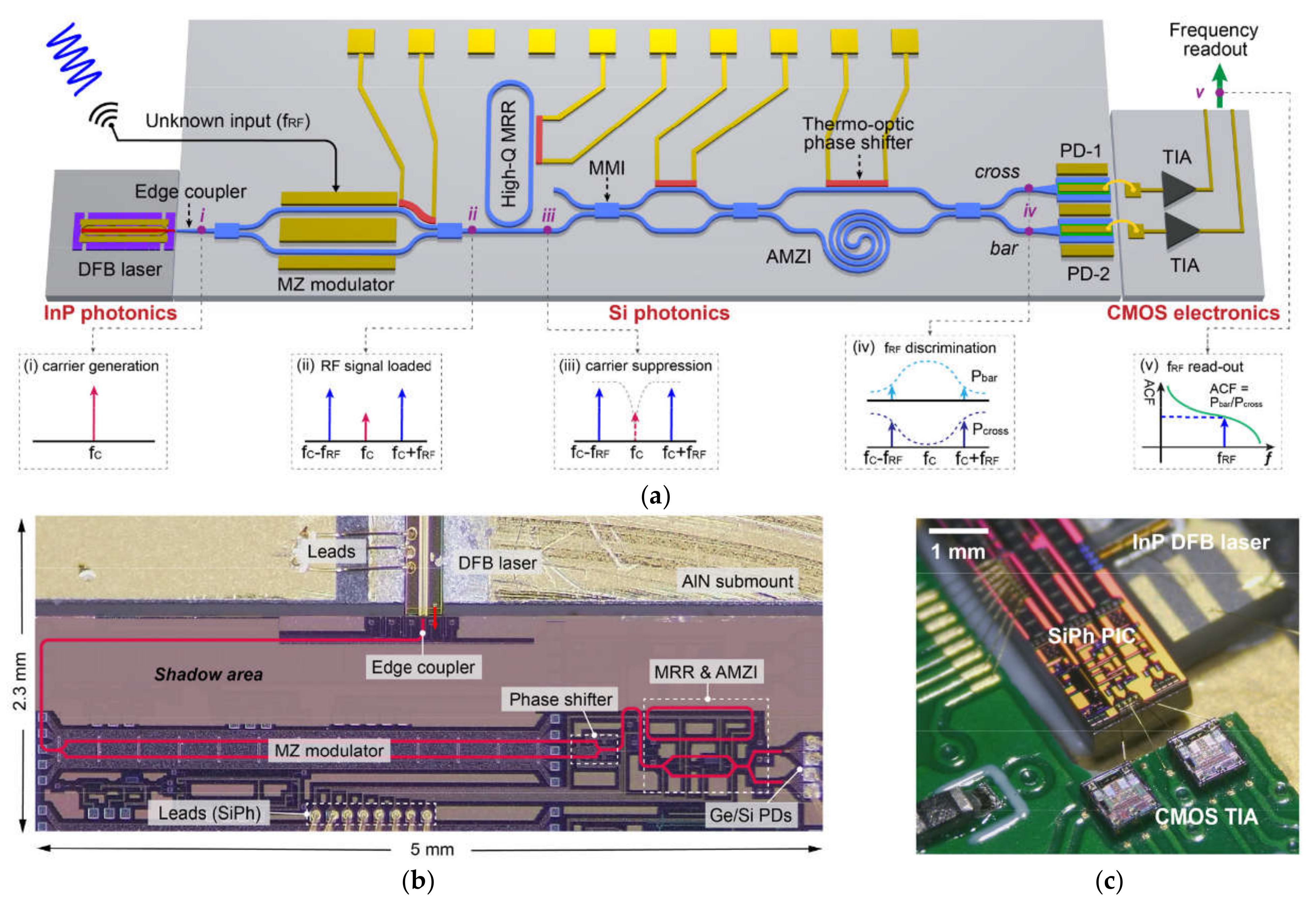
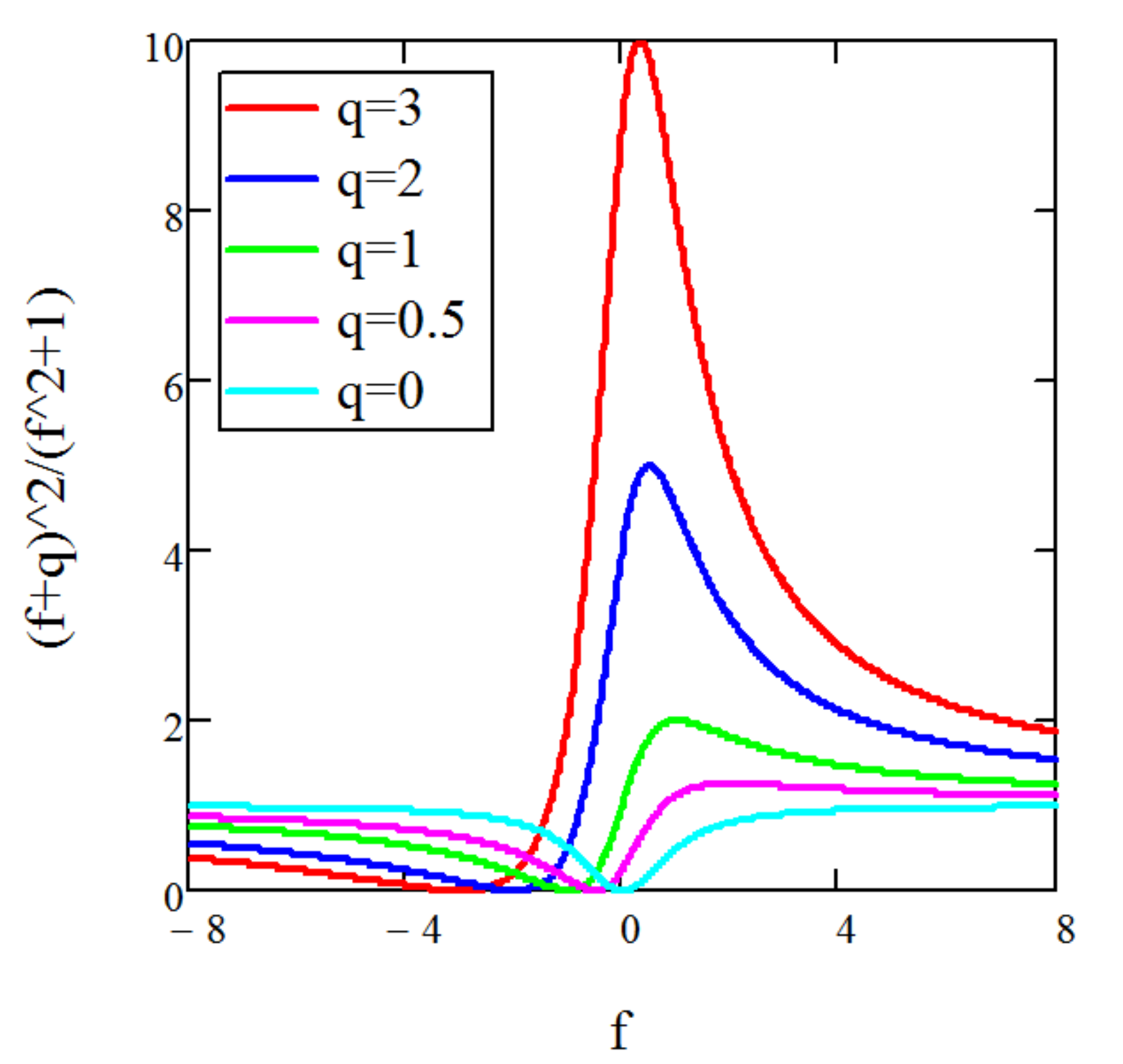
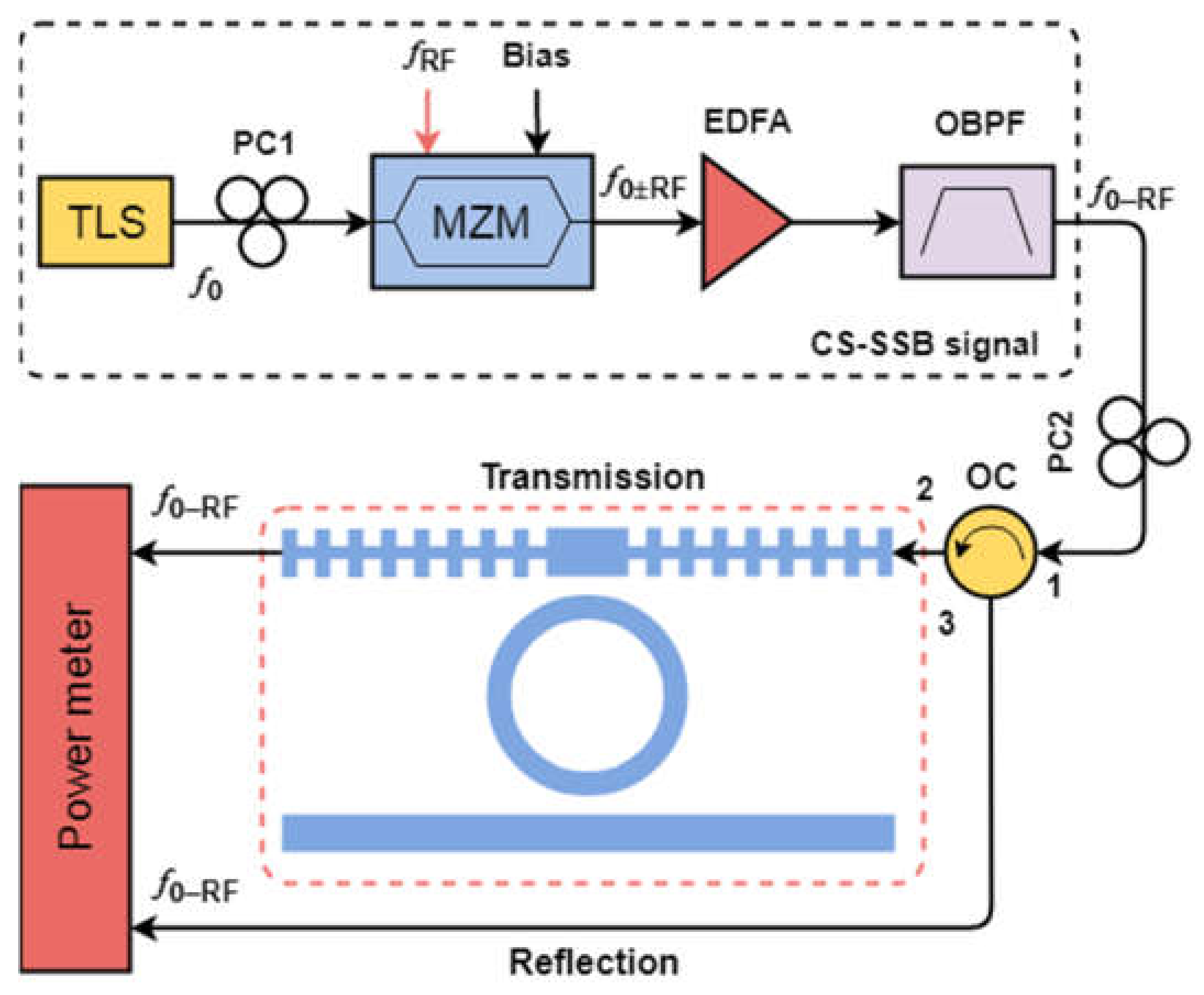
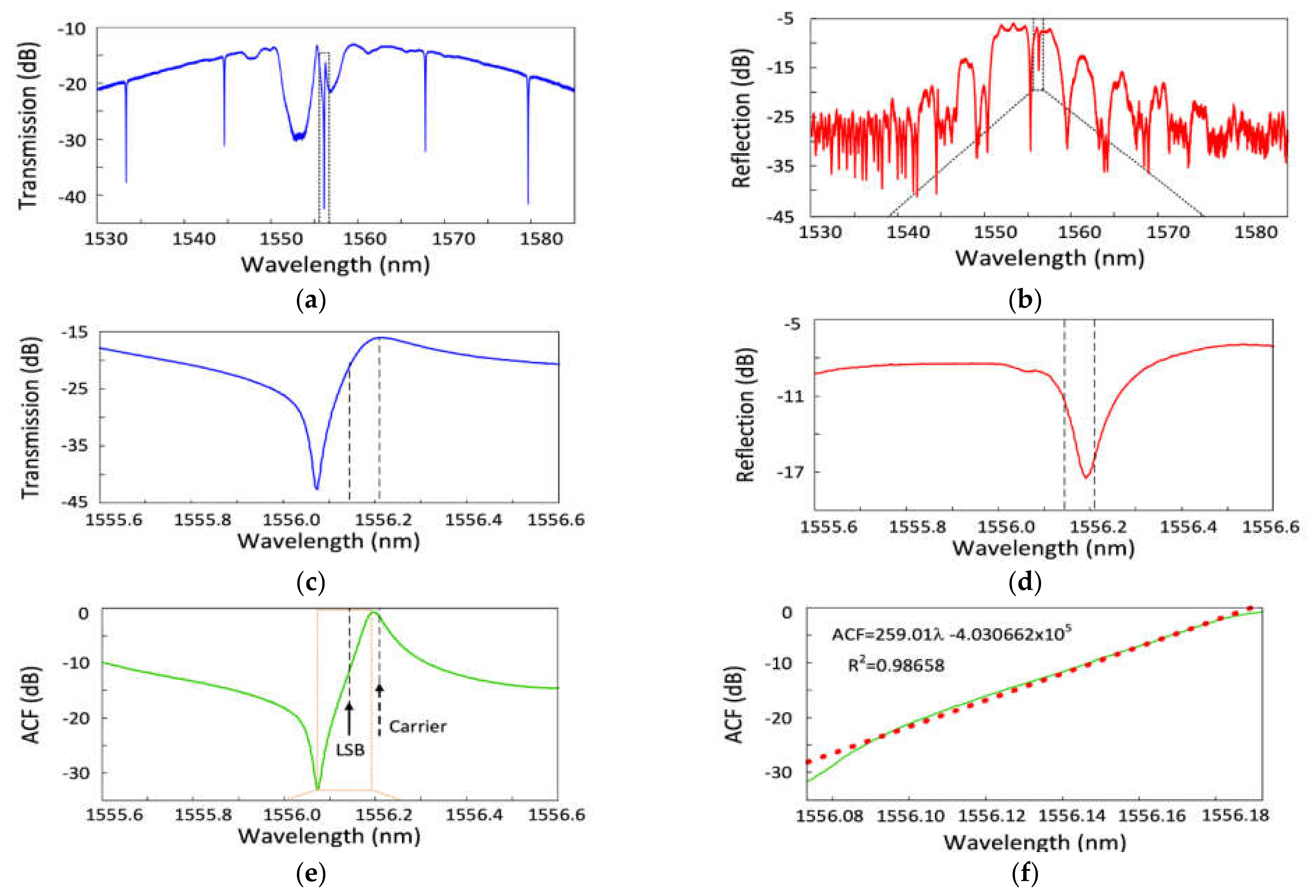
6. IFM Based on Fano Resonance
6.1. Principles of Operation
6.2. Analysis of the Causes of Measurement Errors and Improvement in Measurement Accuracy
6.3. Discussion of Analysis Results
7. Conclusions
- (1)
- The review of works on each type of frequency and amplitude discriminator of resonance type made it possible to analyze the principles of their operation, the main causes of measurement errors and the ways to minimize and/or eliminate these errors. Based on the analysis, a comparative table of metrological characteristics such as frequency range and measurement accuracy was compiled, and further prospects for their development were discussed. That allows the developer to choose ways to solve specific problems during the development of an IFM system.
- (2)
- (3)
- An overview of existing works on IFM systems shows that they also cover the issues of the instantaneous frequency measurement of multiple microwave signals simultaneously [4,37,39,49,84,85], linear frequency-modulated signals [86,87,88], pulse signals [89,90] and other special types of signals [87,88,90] that use frequency and amplitude discriminators of resonance type. Such issues are among the most important areas for research into the construction of a fully functional photonic radar.
- (4)
- Further development of the reviewed methods would make it possible to comprehensively solve the problems in the design and development of an all-photonic radar, which, together with angle of arrival estimation [91,92,93], Doppler frequency shift measurement [94,95], and optical filter design [96,97,98,99] based on the reviewed methods and means, would make it possible to create a prototype of a multifunctional information processing block for an IFM device on a single component base.
Author Contributions
Funding
Conflicts of Interest
References
- East, P. Fifty years of instantaneous frequency measurement. IET Radar Sonar Navig. 2012, 6, 112–122. [Google Scholar] [CrossRef]
- Tsui, J.B.Y. Electronic Warfare Microwave Receiver; Artech House: New York, NY, USA, 1993. [Google Scholar]
- Pan, S.; Yao, J. Photonics-Based Broadband Microwave Measurement. J. Light. Technol. 2016, 35, 3498–3513. [Google Scholar] [CrossRef]
- Long, X.; Zou, W.; Chen, J. Broadband instantaneous frequency measurement based on stimulated Brillouin scattering. Opt. Express 2017, 25, 2206–2214. [Google Scholar] [CrossRef]
- Nguyen, L.V.; Hunter, D.B.; Borg, D.J. Photonic Technique for Radio Frequency Measurement. In Proceedings of the 2005 International Topical Meeting on Microwave Photonics, Seoul, Korea, 14 October 2005; pp. 197–200. [Google Scholar]
- Attygalle, M.; Hunter, D.B. Improved Photonic Technique for Broadband Radio-Frequency Measurement. IEEE Photon Technol. Lett. 2008, 21, 206–208. [Google Scholar] [CrossRef]
- Zou, X.; Pan, S.; Yao, J. Instantaneous Microwave Frequency Measurement With Improved Measurement Range and Resolution Based on Simultaneous Phase Modulation and Intensity Modulation. J. Light. Technol. 2009, 27, 5314–5320. [Google Scholar]
- Li, W.; Zhu, N.H.; Wang, L.X. Reconfigurable Instantaneous Frequency Measurement System Based on Dual-Parallel Mach–Zehnder Modulator. IEEE Photon J. 2012, 4, 427–436. [Google Scholar] [CrossRef]
- Liu, L.; Xue, W. Instantaneous Microwave Frequency Measurement Based on Two Cascaded Photonic Crystal Nanocavities. IEEE Photon J. 2020, 12, 1–9. [Google Scholar] [CrossRef]
- Li, Y.; Pei, L.; Li, J.; Zheng, J.; Wang, Y.; Yuan, J.; Tang, Y. Instantaneous microwave frequency measurement with improved resolution. Opt. Commun. 2015, 354, 140–147. [Google Scholar] [CrossRef]
- Morozov, O.G.; Talipov, A.A.; Nurgazizov, M.R.; Sadeev, T.S.; Fedorov, A.G. Instantaneous frequency measurement using double-frequency probing. Proc. SPIE 2013, 8787, 878708. [Google Scholar] [CrossRef]
- Zou, X.; Lu, B.; Pan, W.; Yan, L.; Stöhr, A.; Yao, J. Photonics for microwave measurements. Laser Photon Rev. 2016, 10, 711–734. [Google Scholar] [CrossRef]
- Vostrikov, E.V.; Litvinov, E.V.; Volkovskii, S.A.; Aleinik, A.S.; Polte, G.A. Application of microwave photonics in fiber optical sensors. Sci. Tech. J. Inf. Technol. Mech. Opt. 2020, 20, 1–23. (In Russian) [Google Scholar] [CrossRef]
- Bui, L.A. Recent advances in microwave photonics instantaneous frequency measurements. Prog. Quantum Electron. 2020, 69, 100237. [Google Scholar] [CrossRef]
- Zhou, J.; Aditya, S.; Lee, K.E.K.; Shum, P.P. Instantaneous microwave frequency measurement using photonic techniques. In Proceedings of the 10th International Conference on Optical Communications and Networks (ICOCN 2011), Guangzhou, China, 5 November–7 December 2011; pp. 1–2. [Google Scholar] [CrossRef]
- Chi, H.; Zou, X.; Yao, J. An Approach to the Measurement of Microwave Frequency Based on Optical Power Monitoring. IEEE Photon Technol. Lett. 2008, 20, 1249–1251. [Google Scholar] [CrossRef]
- Zou, X.; Chi, H.; Yao, J. Microwave Frequency Measurement Based on Optical Power Monitoring Using a Complementary Optical Filter Pair. IEEE Trans. Microw. Theory Tech. 2009, 57, 505–511. [Google Scholar] [CrossRef]
- Lu, B.; Pan, W.; Zou, X.; Luo, B.; Yan, L.; Liu, X.; Xiang, S. Photonic Frequency Measurement and Signal Separation for Pulsed/CW Microwave Signals. IEEE Photon Technol. Lett. 2013, 25, 500–503. [Google Scholar] [CrossRef]
- Fu, S.; Tang, M.; Shum, P. Photonic instantaneous frequency measurement using optical carrier suppression based DC power monitoring. In Proceedings of the IEEE Photonic Society 24th Annual Meeting, Arlington, VA, USA, 9–13 October 2011; pp. 85–86. [Google Scholar] [CrossRef]
- Drummond, M.V.; Monteiro, P.; Nogueira, R.N. Photonic RF instantaneous frequency measurement system by means of a polarization-domain interferometer. Opt. Express 2009, 17, 5433–5438. [Google Scholar] [CrossRef]
- Zou, X.; Pan, W.; Luo, B.; Yan, L. Photonic Instantaneous Frequency Measurement Using a Single Laser Source and Two Quadrature Optical Filters. IEEE Photon Technol. Lett. 2010, 23, 39–41. [Google Scholar] [CrossRef]
- Ghelfi, P.; Laghezza, F.; Scotti, F.; Serafino, G.; Capria, A.; Pinna, S.; Onori, D.; Porzi, C.; Scaffardi, M.; Malacarne, A.; et al. A fully photonics-based coherent radar system. Nature 2014, 507, 341–345. [Google Scholar] [CrossRef]
- Li, Z.; Wang, C.; Li, M.; Chi, H.; Zhang, X.; Yao, J. Instantaneous Microwave Frequency Measurement Using a Special Fiber Bragg Grating. IEEE Microw. Wirel. Compon. Lett. 2010, 21, 52–54. [Google Scholar] [CrossRef]
- Li, Y.; Pei, L.; Li, J.; Wang, Y.; Yuan, J.; Ning, T. Photonic instantaneous frequency measurement of wideband microwave signals. PLoS ONE 2017, 12, e0182231. [Google Scholar] [CrossRef]
- Li, Z.; Yang, B.; Chi, H.; Zhang, X.; Zheng, S.; Jin, X. Photonic instantaneous measurement of microwave frequency using fiber Bragg grating. Opt. Commun. 2010, 283, 396–399. [Google Scholar] [CrossRef]
- Ivanov, A.A.; Morozov, O.G.; Andreev, V.A.; Morozov, G.A.; Kuznetsov, A.A.; Faskhutdinov, L.M. Microwave photonic system for instantaneous frequency measurement based on principles of “frequency-amplitude” conversion in fiber Bragg grating and additional frequency separation. Proc. SPIE 2016, 10342, 103421A. [Google Scholar] [CrossRef]
- Ni, J.; Zhang, X.M.; Zheng, S.L.; Jin, X.F.; Chi, H.; Zhang, X.M. Microwave Frequency Measurement Based on Phase Modulation to Intensity Modulation Conversion using Fiber Bragg Grating. J. Electromagn. Waves Appl. 2011, 25, 755–764. [Google Scholar] [CrossRef]
- Morozov, O.; Talipov, A.A.; Nurgazizov, M.R.; Denisenko, P.E.; Vasilets, A. Instantaneous frequency measurement of microwave signals in optical range using “frequency-amplitude” conversion in the π-phase-shifted fiber-Bragg grating. Proc. SPIE 2014, 9136, 91361B. [Google Scholar] [CrossRef]
- Burla, M.; Wang, X.; Li, M.; Chrostowski, L.; Azaña, J. Wideband dynamic microwave frequency identification system using a low-power ultracompact silicon photonic chip. Nat. Commun. 2016, 7, 13004. [Google Scholar] [CrossRef] [PubMed]
- Hunter, D.B.; Edvell, L.G.; Englund, M.A. Wideband Microwave Photonic Channelised Receiver. In Proceedings of the 2005 International Topical Meeting on Microwave Photonics, Seoul, Korea, 14 October 2005; pp. 249–252. [Google Scholar]
- Sima, C.; Cai, B.; Liu, B.; Gao, Y.; Yu, Y.; Gates, J.C.; Zervas, M.N.; Smith, P.G.R.; Liu, D. Integrated reconfigurable photonic filters based on interferometric fractional Hilbert transforms. Appl. Opt. 2017, 56, 7978–7984. [Google Scholar] [CrossRef]
- Jiang, J.; Shao, H.; Li, X.; Li, Y.; Dai, T.; Wang, G.; Yang, J.; Jiang, X.; Yu, H. Photonic-assisted microwave frequency measurement system based on a silicon ORR. Opt. Commun. 2017, 382, 366–370. [Google Scholar] [CrossRef]
- Tao, Y.; Yang, F.; Tao, Z.; Chang, L.; Shu, H.; Jin, M.; Zhou, Y.; Ge, Z.; Wang, X. Fully on-Chip Microwave Photonics System. 2022. Available online: https://arxiv.org/ftp/arxiv/papers/2202/2202.11495.pdf (accessed on 12 August 2022).
- Zhang, J.; Zhu, C.; Yang, X.; Li, Y.; Zhao, Z.; Li, C. Approach for Microwave Frequency Measurement Based on a Single Photonic Chip Combined with a Phase Modulator and Microring Resonator. Curr. Opt. Photon. 2018, 2, 576–581. [Google Scholar]
- Marpaung, D. On-Chip Photonic-Assisted Instantaneous Microwave Frequency Measurement System. IEEE Photon Technol. Lett. 2013, 25, 837–840. [Google Scholar] [CrossRef]
- Niu, J.; Fu, S.; Xu, K.; Sun, X.; Wu, J.; Lin, J.; Shum, P.P. Instantaneous microwave frequency measurement using a microfiber ring resonator (MRR) based photonic differentiator. In Proceedings of the 2011 International Topical Meeting on Microwave Photonics jointly held with the 2011 Asia-Pacific Microwave Photonics Conference, Singapore, 18–21 October 2011; pp. 226–229. [Google Scholar]
- Jiang, H.; Marpaung, D.; Pagani, M.; Vu, K.; Choi, D.; Madden, S.J.; Yan, L.; Eggleton, B.J. Wide-range, high-precision multiple microwave frequency measurement using a chip-based photonic Brillouin filter. Optica 2016, 3, 30–34. [Google Scholar] [CrossRef]
- Wang, D.; Pan, L.; Wang, Y.; Zhang, Q.; Du, C.; Wang, K.; Dong, W.; Zhang, X. Instantaneous microwave frequency measurement with high-resolution based on stimulated Brillouin scattering. Opt. Laser Technol. 2018, 113, 171–176. [Google Scholar] [CrossRef]
- Zheng, S.; Ge, S.; Zhang, X.; Chi, H.; Jin, X. High-Resolution Multiple Microwave Frequency Measurement Based on Stimulated Brillouin Scattering. IEEE Photonics Technol. Lett. 2012, 24, 1115–1117. [Google Scholar] [CrossRef]
- Li, J.; Xu, P.; Song, K.; Du, K.; Lu, M.; Yu, X.; Yao, Y. A Scheme of Instantaneous Frequency Measurement with High Precision Assisted by Photonics. Int. J. Opt. Photonic Eng. 2021, 6, 38. [Google Scholar]
- Zhu, B.; Zhang, W.; Pan, S.; Yao, J. High-Sensitivity Instantaneous Microwave Frequency Measurement Based on a Silicon Photonic Integrated Fano Resonator. J. Light. Technol. 2018, 37, 2527–2533. [Google Scholar] [CrossRef]
- Shu, X.; Turitsyna, E.; Bennion, I. Broadband fiber Bragg grating with channelized dispersion. Opt. Express 2007, 15, 10733–10738. [Google Scholar] [CrossRef] [PubMed]
- Isa, N.; Altuncu, A. Design of a chirped fiber Bragg grating for use in wideband dispersion compensation. Advances in Computer Science and Engineering: Reports and Monographs. New Trends Comput. Netw. 2005, 114–123. [Google Scholar] [CrossRef]
- Vidal, B. Photonic-based instantaneous microwave frequency measurement with extended range. Opt. Commun. 2011, 284, 3996–3999. [Google Scholar] [CrossRef]
- Zhang, X.; Ni, J.; Cheng, P.; Li, Z. Microwave Frequency Measurement Using Fiber Bragg Grating as V-shape Filter. In Proceedings of the 2010 International Conference on Digital Manufacturing & Automation, Changcha, China, 18–20 December 2010; pp. 921–924. [Google Scholar] [CrossRef]
- Morozov, O.G.; Stepuschenko, O.A.; Sadykov, I.R. Modulation measuring methods in optical refractometric biosensors based on the phase-shift fiber Bragg grating. Vestn. MarSTU 2010, 3, 3–13. [Google Scholar]
- Morozov, O.G.; Talipov, A.A.; Nurgazizov, M.R.; Vasilets, A.A. Instantaneous microwave frequency measurement with monitoring of system temperature. Proc. SPIE 2014, 9156, 91560N. [Google Scholar] [CrossRef]
- Yongkang, G.; Xueming, L.; Leiran, W. Optimized synthesis of fiber Bragg gratings with triangular spectrum for wave-length-interrogation application. Opt. Eng. 2011, 50, 024401. [Google Scholar]
- Ming, L.; Junya, H.; Hongpu, L. Advanced design of a complex fiber Bragg grating for a multichannel asymmetrical triangular filter. J. Opt. Soc. Am. B 2009, 26, 228–234. [Google Scholar]
- Liu, Y.; Lee, S.B.; Choi, S.S. Phase-Shifted Fiber Bragg Grating Transmission Filters Based on the Fabry-Perot Effect. J. Opt. Soc. Korea 1998, 2, 30–33. [Google Scholar] [CrossRef]
- Das, B.; Srivastava, D.; Tiwari, U.K.; Choudhary, B.C. Dynamic strain response of a π-phase-shifted FBG sensor with phase-sensitive detection. OSA Contin. 2018, 1, 1172–1184. [Google Scholar] [CrossRef]
- Falah, A.A.S.; Mokhtar, M.R.; Yusoff, Z.; Ibsen, M. Reconfigurable Phase Shifted Fiber Bragg Grating Using Localized Micro-Strain. IEEE Photon Technol. Lett. 2016, 28, 951–954. [Google Scholar] [CrossRef]
- Loh, W.H.; Cole, M.J.; Zervas, M.N.; Barcelos, S.; Laming, R.I. Complex grating structures with uniform phase masks based on the moving fiber–scanning beam technique. Opt. Lett. 1995, 20, 2051–2053. [Google Scholar] [CrossRef]
- Du, Y.; Chen, T.; Zhang, Y.; Wang, R.; Cao, H.; Li, K. Fabrication of Phase-Shifted Fiber Bragg Grating by Femtosecond Laser Shield Method. IEEE Photon Technol. Lett. 2017, 29, 2143–2146. [Google Scholar] [CrossRef]
- Li, M.; Li, H.; Painchaud, Y. Multi-channel notch filter based on a phase-shift phase-only-sampled fiber Bragg grating. Opt. Express 2008, 16, 19388–19394. [Google Scholar] [CrossRef]
- Li, W.; Li, M.; Yao, J. A Narrow-Passband and Frequency-Tunable Microwave Photonic Filter Based on Phase-Modulation to Intensity-Modulation Conversion Using a Phase-Shifted Fiber Bragg Grating. IEEE Trans. Microw. Theory Tech. 2012, 60, 1287–1296. [Google Scholar] [CrossRef]
- Painchaud, Y.; Aubé, M.; Brochu, G.; Picard, M.-J. Ultra-narrowband notch filtering with highly resonant fiber Bragg gratings. In Proceedings of the Advanced Photonics & Renewable Energy, Karlsruhe, Germany, 21–24 June 2010; p. BTuC3. [Google Scholar] [CrossRef]
- Donzella, V.; Sherwali, A.; Flueckiger, J.; Grist, S.M.; Fard, S.T.; Chrostowski, L. Design and fabrication of SOI micro-ring resonators based on sub-wavelength grating waveguides. Opt. Express 2015, 23, 4791–4803. [Google Scholar] [CrossRef]
- Cheng, X.; Hong, J.; Spring, A.M.; Yokoyama, S. Fabrication of a high-Q factor ring resonator using LSCVD deposited Si_3N_4 film. Opt. Mater. Express 2017, 7, 2182. [Google Scholar] [CrossRef]
- Wang, Z.; Paez, D.; El-Rahman, A.I.A.; Wang, P.; Dow, L.; Cartledge, J.C.; Knights, A.P. Resonance control of a silicon mi-cro-ring resonator modulator under high-speed operation using the intrinsic defect-mediated photocurrent. Opt. Express 2017, 25, 24827–24836. [Google Scholar] [CrossRef] [PubMed]
- Fegadolli, W.S.; Almeida, V.R.; Oliveira, J.E.B. Reconfigurable silicon thermo-optical device based on spectral tuning of ring resonators. Opt. Express 2011, 19, 12727–12739. [Google Scholar] [CrossRef] [PubMed]
- Yariv, A. Critical coupling and its control in optical waveguide-ring resonator systems. IEEE Photon Technol. Lett. 2002, 14, 483–485. [Google Scholar] [CrossRef]
- Geuzebroek, D.H.; Driessen, A. Ring-Resonator-Based Wavelength Filters. In Wavelength Filters in Fibre Optics; Venghaus, H., Ed.; Springer Series in Optical Sciences; Springer: Berlin/Heidelberg, Germany, 2006; Volume 123, pp. 341–379. [Google Scholar]
- Billah, M.R.; Blaicher, M.; Hoose, T.; Dietrich, P.-I.; Marin-Palomo, P.; Lindenmann, N.; Nesic, A.; Hofmann, A.; Troppenz, U.; Moehrle, M.; et al. Hybrid integration of silicon photonics circuits and InP lasers by photonic wire bonding. Optica 2018, 5, 876–883. [Google Scholar] [CrossRef]
- Xiang, C.; Liu, J.; Guo, J.; Chang, L.; Wang, R.N.; Weng, W.; Peters, J.; Xie, W.; Zhang, Z.; Riemensberger, J.; et al. Laser soliton microcombs heterogeneously integrated on silicon. Science 2021, 373, 99–103. [Google Scholar] [CrossRef] [PubMed]
- Thomson, D.; Zilkie, A.; Bowers, J.E.; Komljenovic, T.; Reed, G.T.; Vivien, L.; Marris-Morini, D.; Cassan, E.; Virot, L.; Fédéli, J.-M.; et al. Roadmap on silicon photonics. J. Opt. 2016, 18, 073003. [Google Scholar] [CrossRef]
- Zeng, X.; He, W.; Frosz, M.H.; Geilen, A.; Roth, P.; Wong, G.K.L.; Russell, P.S.; Stiller, B. Stimulated Brillouin scattering in chiral photonic crystal fiber. Photon Res. 2022, 10, 711. [Google Scholar] [CrossRef]
- Gyger, F.; Liu, J.; Yang, F.; He, J.; Raja, A.S.; Wang, R.N.; Bhave, S.A.; Kippenberg, T.J.; Thévenaz, L. Observation of Stimulated Brillouin Scattering in Silicon Nitride Integrated Waveguides. Phys. Rev. Lett. 2020, 124, 013902. [Google Scholar] [CrossRef]
- Merklein, M.; Casas-Bedoya, A.; Marpaung, D.; Buttner, T.F.S.; Pagani, M.; Morrison, B.; Kabakova, I.V.; Eggleton, B.J. Stimulated Brillouin Scattering in Photonic Integrated Circuits: Novel Applications and Devices. IEEE J. Sel. Top. Quantum Electron. 2016, 22, 336–346. [Google Scholar] [CrossRef]
- Reintjes, J.F. Nonlinear Optical Processes. In Encyclopedia of Physical Science and Technology, 3rd ed.; Robert, A., Meyers, R.A., Eds.; Academic Press: Cambridge, MA, USA, 2003; pp. 537–581. [Google Scholar]
- Bashkansky, M.; Reintjes, J. SCATTERING | Stimulated Scattering. In Encyclopedia of Modern Optics; Robert, D., Guenther, R.D., Eds.; Elsevier: Amsterdam, The Netherlands, 2005; pp. 330–340. [Google Scholar]
- Kobyakov, A.; Sauer, M.; Chowdhury, D. Stimulated Brillouin scattering in optical fibers. Adv. Opt. Photon. 2010, 2, 1–59. [Google Scholar] [CrossRef]
- Ma, D.; Zuo, P.; Chen, Y. Time–frequency analysis of microwave signals based on stimulated Brillouin scattering. Opt. Commun. 2022, 516, 128228. [Google Scholar] [CrossRef]
- Debut, A.; Randoux, S.; Zemmouri, J. Linewidth narrowing in Brillouin lasers: Theoretical analysis. Phys. Rev. A 2000, 62, 023803. [Google Scholar] [CrossRef]
- Zhou, W.; Zhao, D.; Shuai, Y.-C.; Yang, H.; Chuwongin, S.; Chadha, A.; Seo, J.-H.; Wang, K.X.; Liu, V.; Ma, Z.; et al. Progress in 2D photonic crystal Fano resonance photonics. Prog. Quantum Electron. 2014, 38, 1–74. [Google Scholar] [CrossRef]
- Limonov, M.F.; Rybin, M.; Poddubny, A.; Kivshar, Y.S. Fano resonances in photonics. Nat. Photon. 2017, 11, 543–554. [Google Scholar] [CrossRef]
- Yu, Y.; Heuck, M.; Hu, H.; Xue, W.; Peucheret, C.; Chen, Y.; Oxenløwe, L.K.; Yvind, K.; Mørk, J. Fano resonance control in a photonic crystal structure and its application to ultrafast switching. Appl. Phys. Lett. 2014, 105, 061117. [Google Scholar] [CrossRef]
- Limonov, M.F. Fano resonance for applications. Adv. Opt. Photon. 2021, 13, 703–771. [Google Scholar] [CrossRef]
- Zhang, W.; Li, W.; Yao, J. Optically tunable Fano resonance in a grating-based Fabry–Perot cavity-coupled microring resonator on a silicon chip. Opt. Lett. 2016, 41, 2474–2477. [Google Scholar] [CrossRef]
- Guo, H.; Xiao, G.; Mrad, N.; Yao, J. Measurement of microwave frequency using a monolithically integrated scannable echelle diffractive grating. IEEE Photon. Tech. Lett. 2009, 34, 45–47. [Google Scholar]
- Fandiño, J.S.; Muñoz, P. Photonics-based microwave frequency measurement using a double-sideband suppressed-carrier modulation and an InP integrated ring-assisted Mach–Zehnder interferometer filter. Opt. Lett. 2013, 38, 4316–4319. [Google Scholar] [CrossRef]
- Marpaung, D.; Yao, J.; Capmany, J. Integrated microwave photonics. Nat. Photonics 2019, 13, 80–90. [Google Scholar] [CrossRef]
- Zhang, W.; Yao, J. Silicon-Based Integrated Microwave Photonics. IEEE J. Quantum Electron. 2015, 52, 1–12. [Google Scholar] [CrossRef]
- Sakhabutdinov, A.J.; Morozov, O.G.; Ivanov, A.A.; Misbakhov, R.S. Multiple frequencies analysis in FBG based instantaneous frequency measurements. In Proceedings of the 2018 Systems of Signals Generating and Processing in the Field of on Board Communications, Moscow, Russia, 14–15 March 2018; pp. 1–5. [Google Scholar]
- Shen, Z.; Jin, C.; He, Q.; Zhang, Z.; Zhao, Y. Photonics-Assisted Non-Scanning High-Accuracy Frequency Measurement Using Low-Speed Components. IEEE Photon J. 2019, 11, 1–8. [Google Scholar] [CrossRef]
- Zhang, B.; Wang, X.; Pan, S. Photonics-Based Instantaneous Multi-Parameter Measurement of a Linear Frequency Modulation Microwave Signal. J. Light. Technol. 2018, 36, 2589–2596. [Google Scholar] [CrossRef]
- Wang, X.; Yang, Y.-L.; Xu, Y.-Q.; Cao, Y.; Zou, W.-W. Power-Independent Microwave Instantaneous Frequency Measurement Based on Combination of Brillouin Gain and Loss Spectra. IEEE Photon J. 2022, 14, 1–7. [Google Scholar] [CrossRef]
- Lu, B.; Pan, W.; Zou, X.; Jiang, H.; Yan, X.; Li, P.; Zou, F.; Yan, L.; Luo, B. Photonic-Assisted Intrapulse Parameters Measurement of Complex Microwave Signals. J. Light. Technol. 2018, 36, 3633–3644. [Google Scholar] [CrossRef]
- Fu, S.; Zhou, J.; Shum, P.P.; Lee, K. Instantaneous Microwave Frequency Measurement Using Programmable Differential Group Delay (DGD) Modules. IEEE Photon J. 2010, 2, 967–973. [Google Scholar] [CrossRef]
- Yao, Y.; Zhao, Y.; Wei, Y.; Zhou, F.; Chen, D.; Zhang, Y.; Xiao, X.; Dong, J.; Yu, S.; Zhang, X. Fully integrated multipurpose microwave frequency identification system on a single chip. In Proceedings of the Conference on Lasers and Electro-Optics, San Jose, CA, USA, 15–20 May 2022; p. SM3N.5. [Google Scholar] [CrossRef]
- Morozov, O.G.; Denisenko, E.P.; Denisenko, P.E.; Lustina, A.A.; Andreev, V.D. Radiophotonic Method for Angle of Arrival Estimation of a Reflected Radar Signal Based on Tandem Amplitude-Phase Modulation. In Proceedings of the 2022 Systems of Signals Generating and Processing in the Field of on Board Communications, Moscow, Russia, 15–17 March 2022; pp. 1–5. [Google Scholar] [CrossRef]
- Lin, T.; Zhang, Z.; Wang, Y.; Zhu, Z.; Zhao, S.; Liu, J.; Jiang, W.; Wu, G. Photonic 2-D angle-of-arrival estimation based on an L-shaped antenna array for an early radar warning receiver. Opt. Express 2020, 28, 38960–38972. [Google Scholar] [CrossRef]
- Tu, Z.; Wen, A.; Xiu, Z.; Zhang, W.; Chen, M. Angle-of-Arrival Estimation of Broadband Microwave Signals Based on Microwave Photonic Filtering. IEEE Photonics J. 2017, 9, 1–8. [Google Scholar] [CrossRef]
- Morozov, O.G.; Ivanov, A.A.; Sakhabutdinov, A.Z.; Denisenko, P.E.; Morozov, G.A.; Denisenko, E.P.; Lustina, A.A.; Andreev, V.D. Radiophotonic module for Doppler frequency shift measurement of a reflected signal for radar type problems solving. Proc. SPIE 2022, 12295, 122950A. [Google Scholar] [CrossRef]
- Zhuo, H.; Wen, A.; Tu, Z. Photonic Doppler frequency shift measurement without ambiguity based on cascade modulation. Opt. Commun. 2020, 470, 125798. [Google Scholar] [CrossRef]
- Fallahi, V.; Seifouri, M. Design of a High-Quality Optical Filter Based on 2D Photonic Crystal Ring Resonator for WDM Systems. J. Opt. Commun. 2018, 41, 355–361. [Google Scholar] [CrossRef]
- Arianfard, H.; Wu, J.; Juodkazis, S.; Moss, D.J. Advanced Multi-Functional Integrated Photonic Filters Based on Coupled Sagnac Loop Reflectors. J. Light. Technol. 2021, 39, 1400–1408. [Google Scholar] [CrossRef]
- Foroughifar, A.; Saghaei, H.; Veisi, E. Design and analysis of a novel four-channel optical filter using ring resonators and line defects in photonic crystal microstructure. Opt. Quantum Electron. 2021, 53, 101. [Google Scholar] [CrossRef]
- Daulay, O.; Botter, R.; Marpaung, D. On-chip programmable microwave photonic filter with an integrated optical carrier processor. OSA Contin. 2020, 3, 2166. [Google Scholar] [CrossRef]
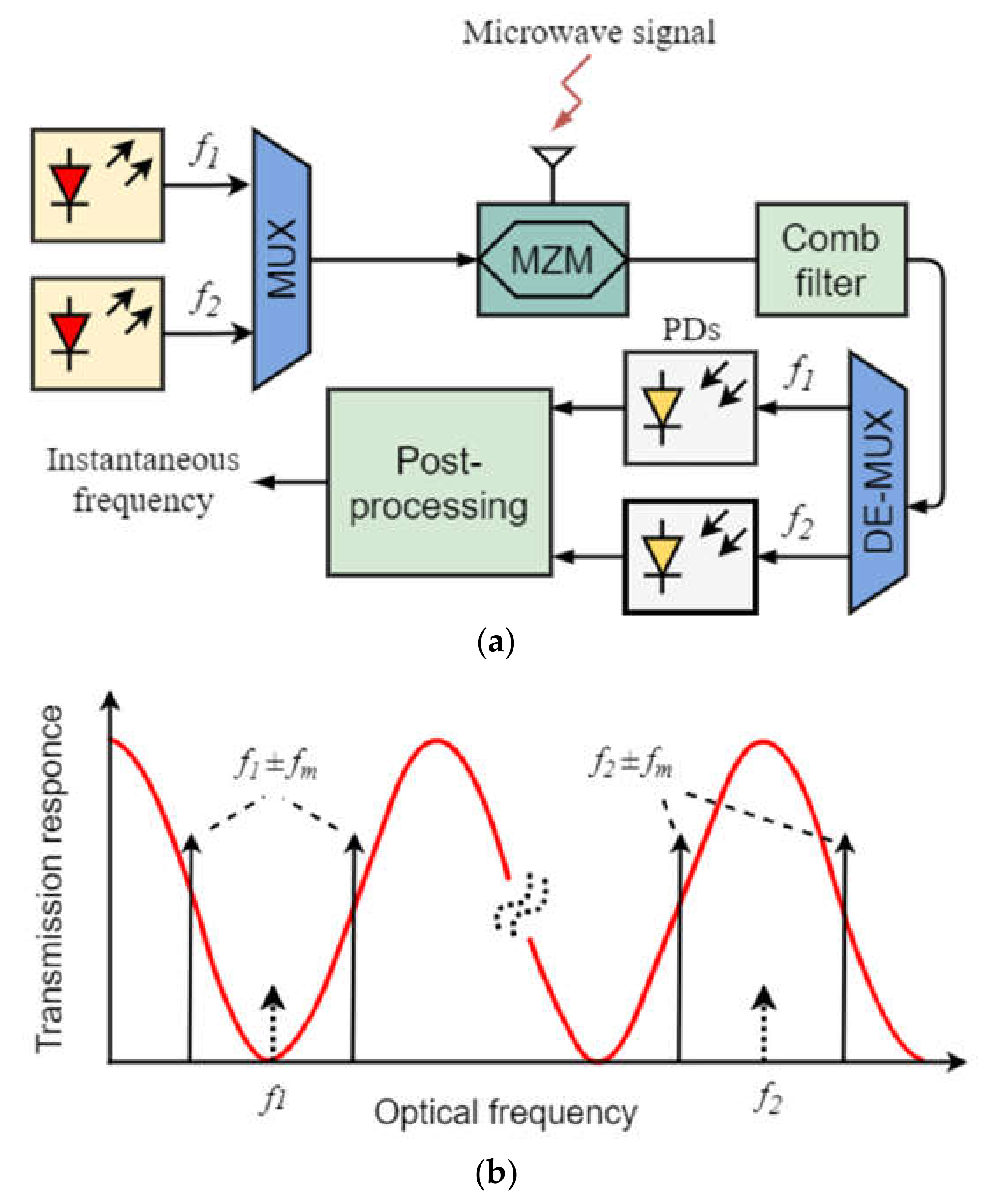
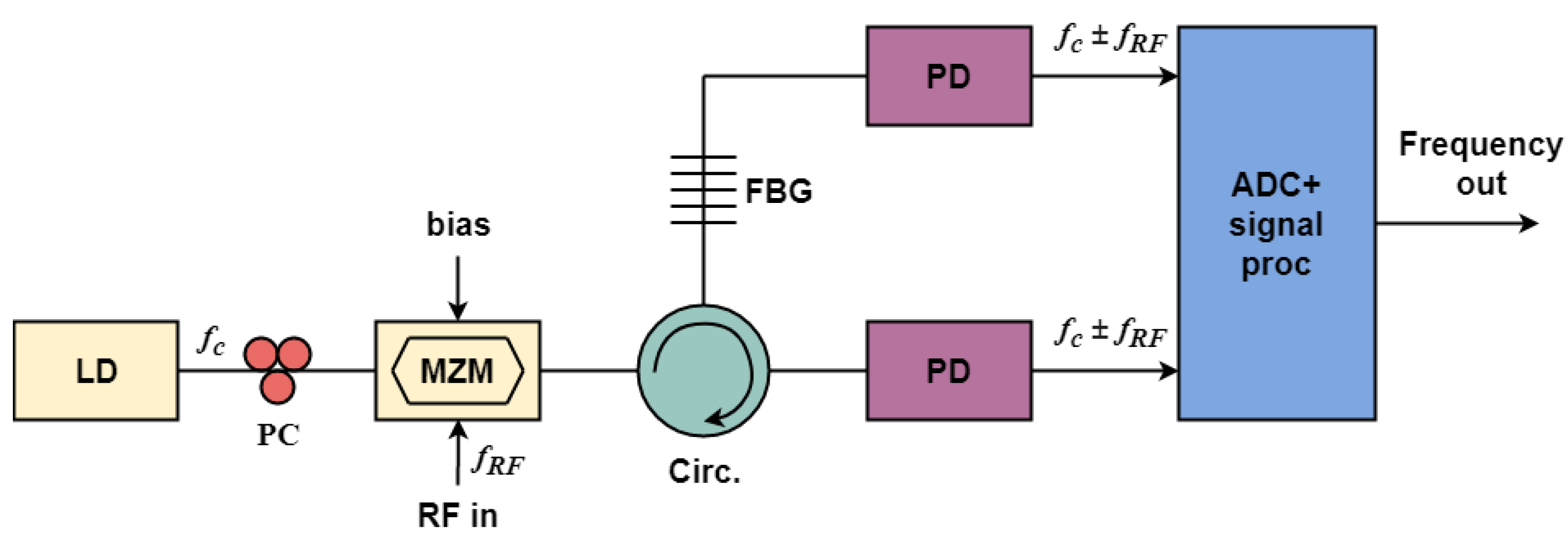
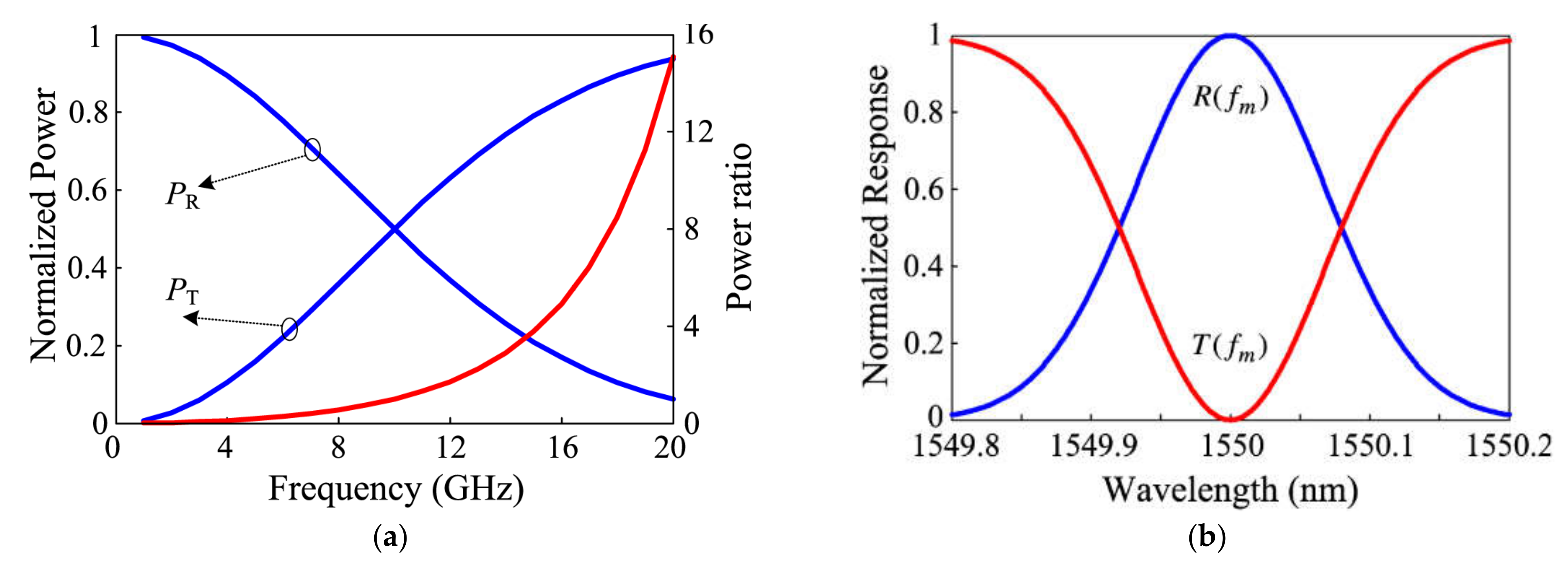

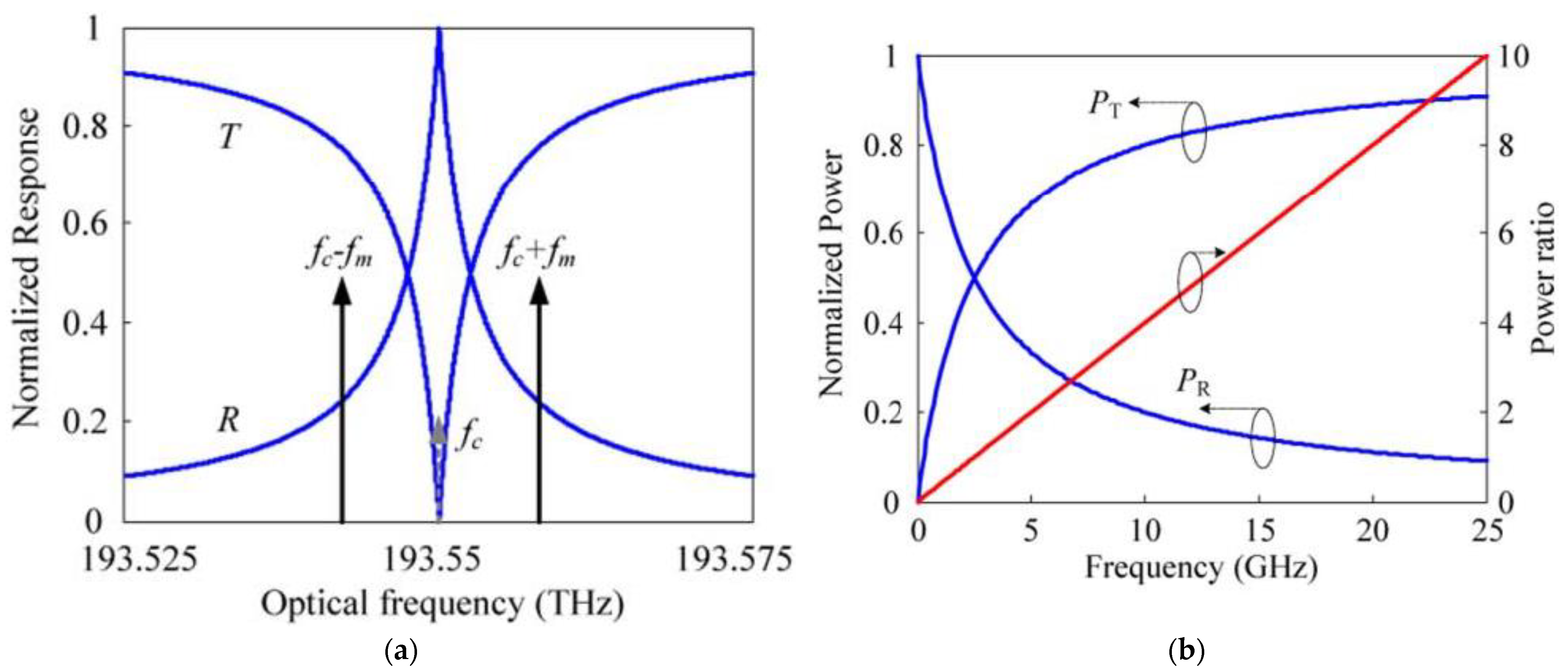

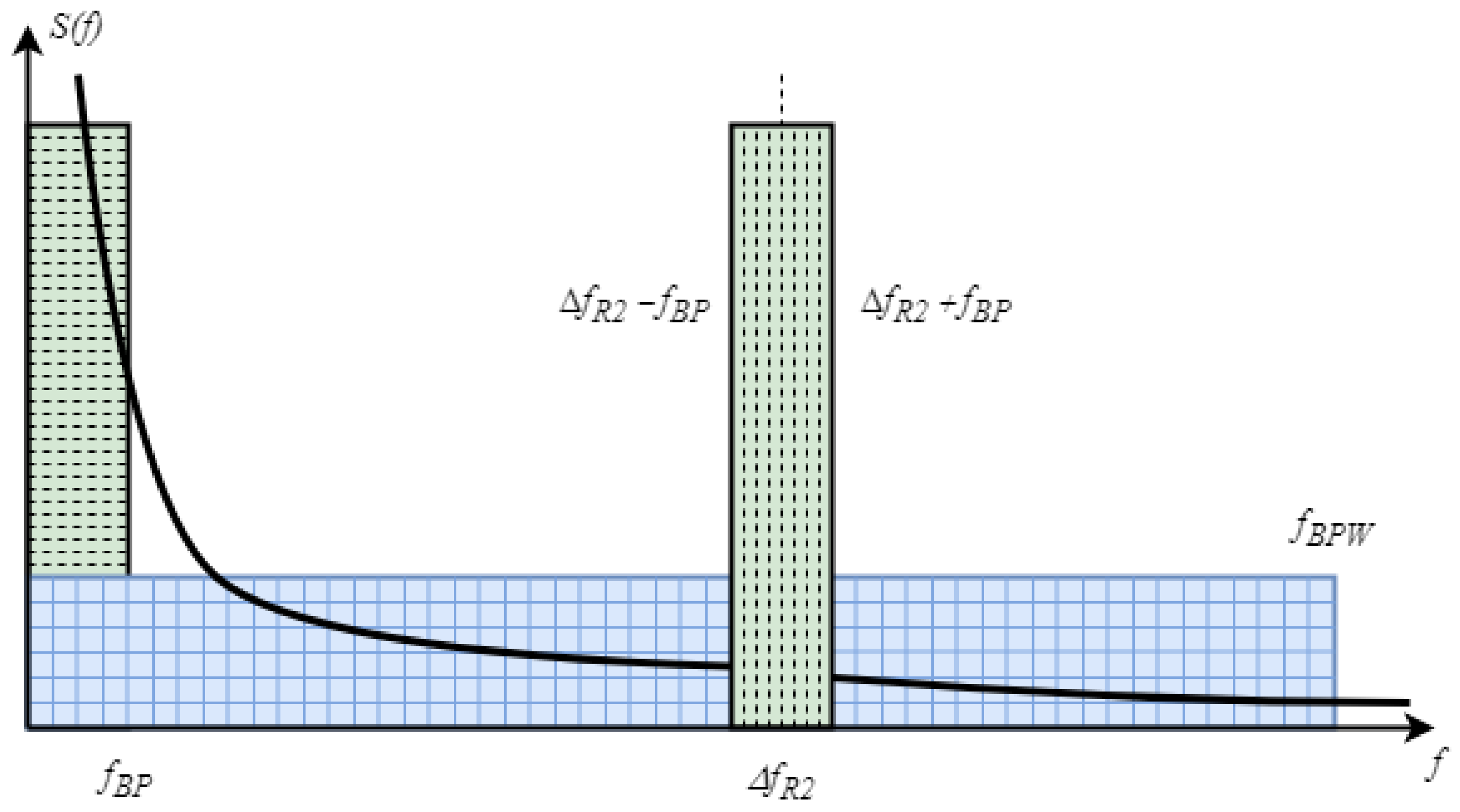
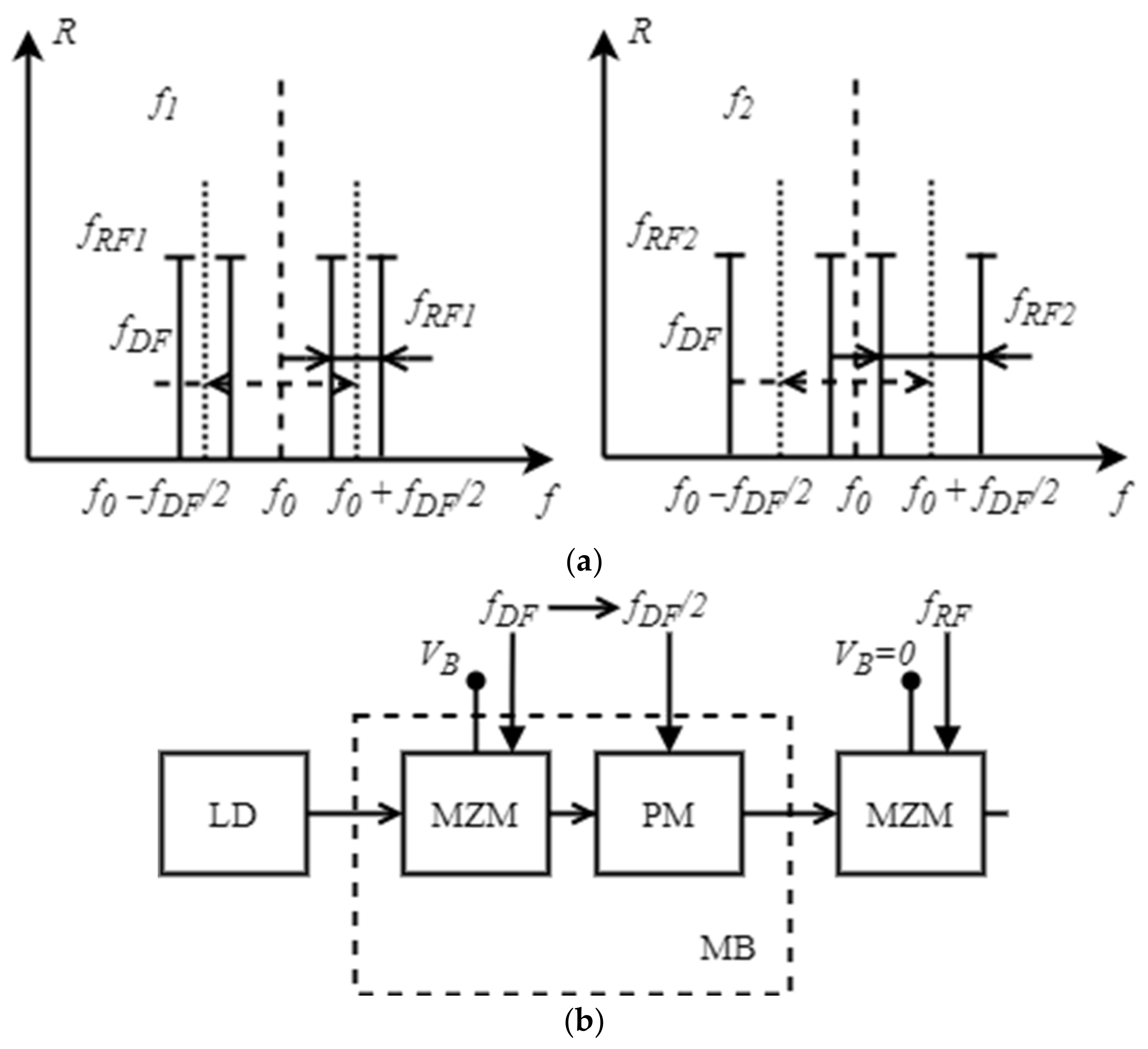
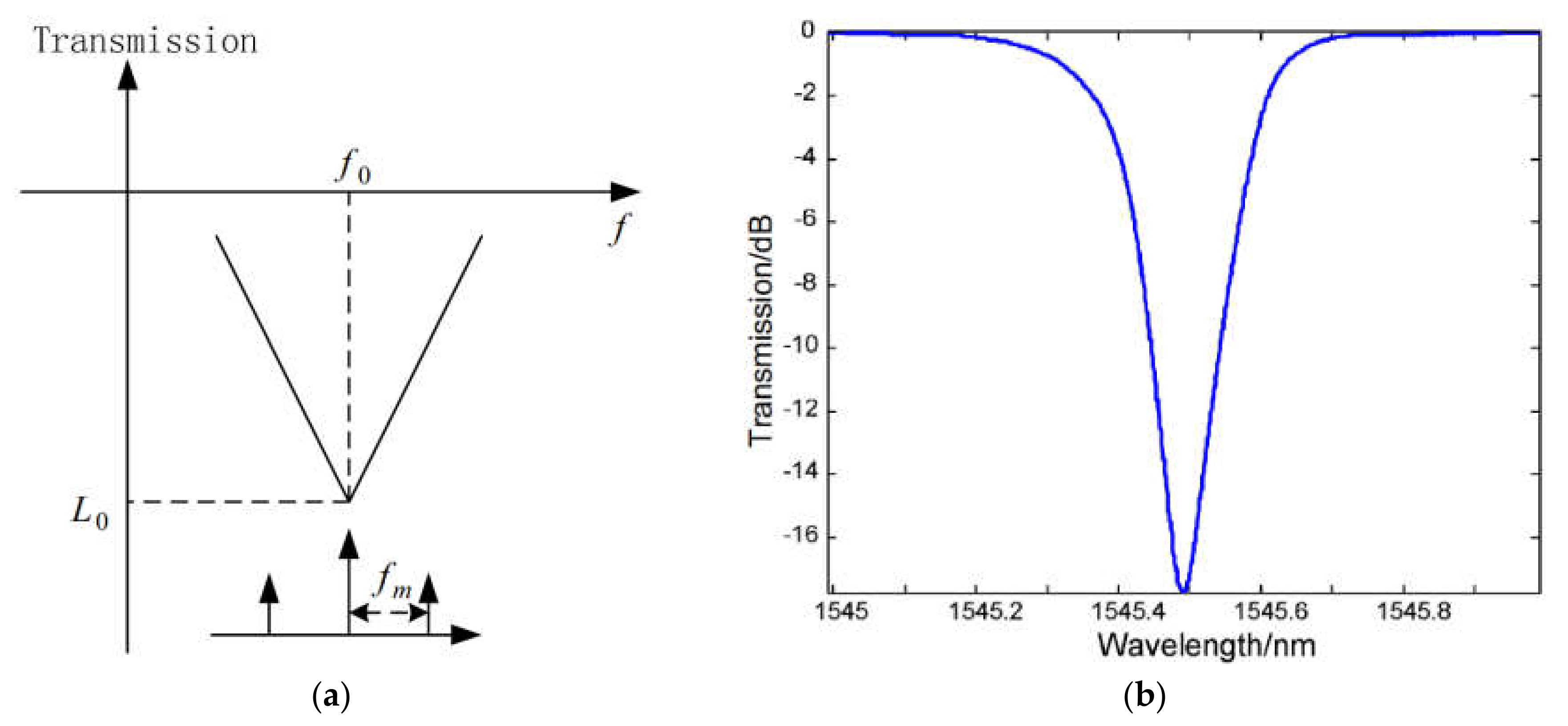
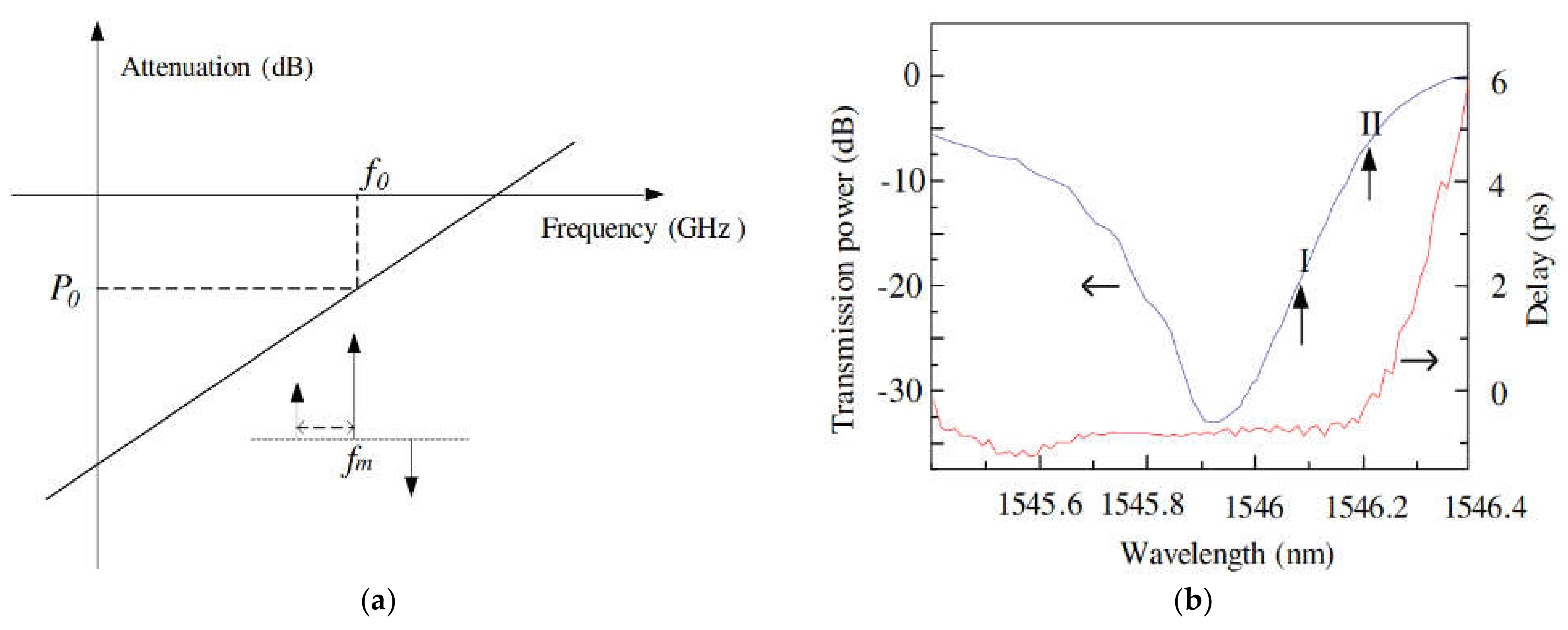
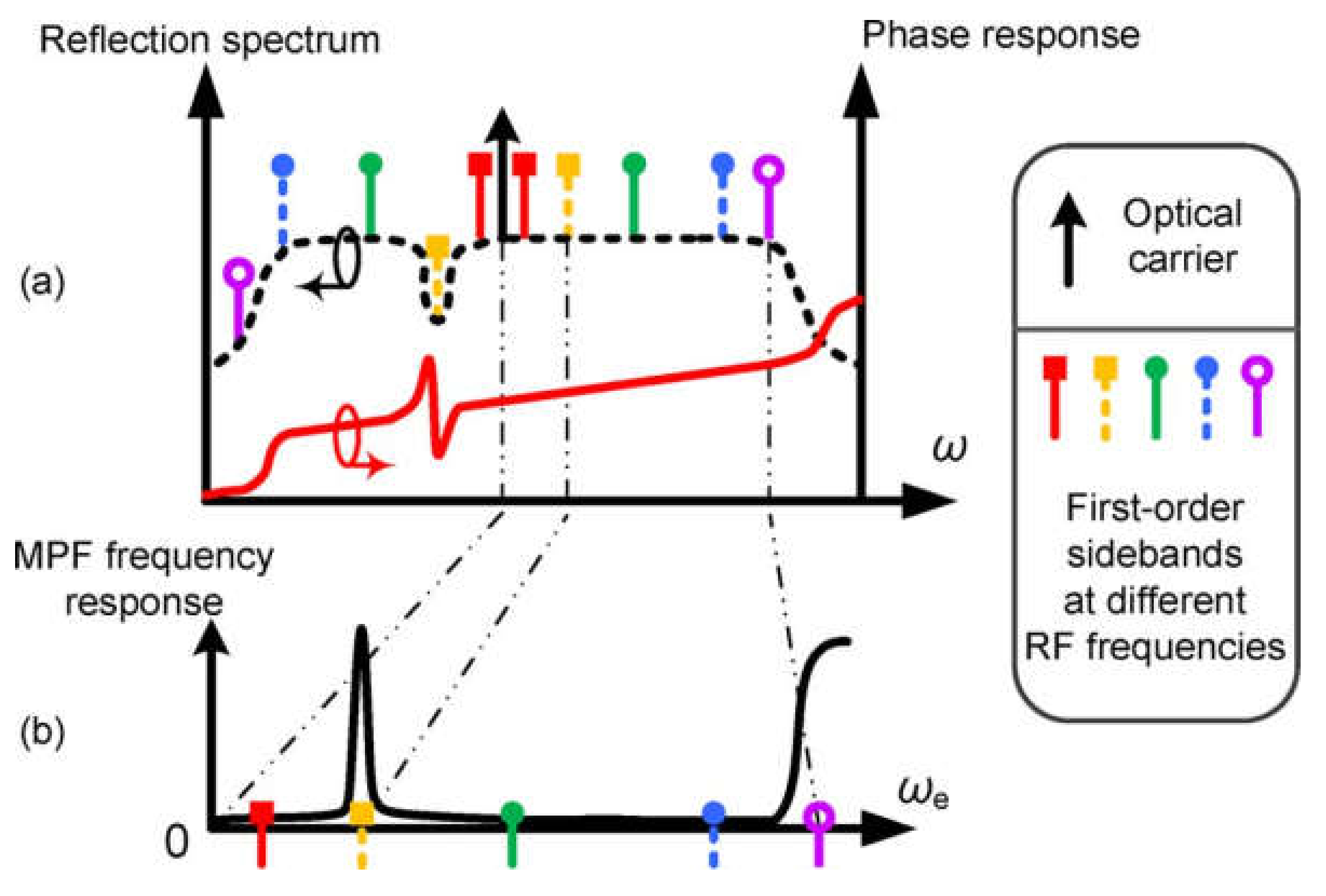

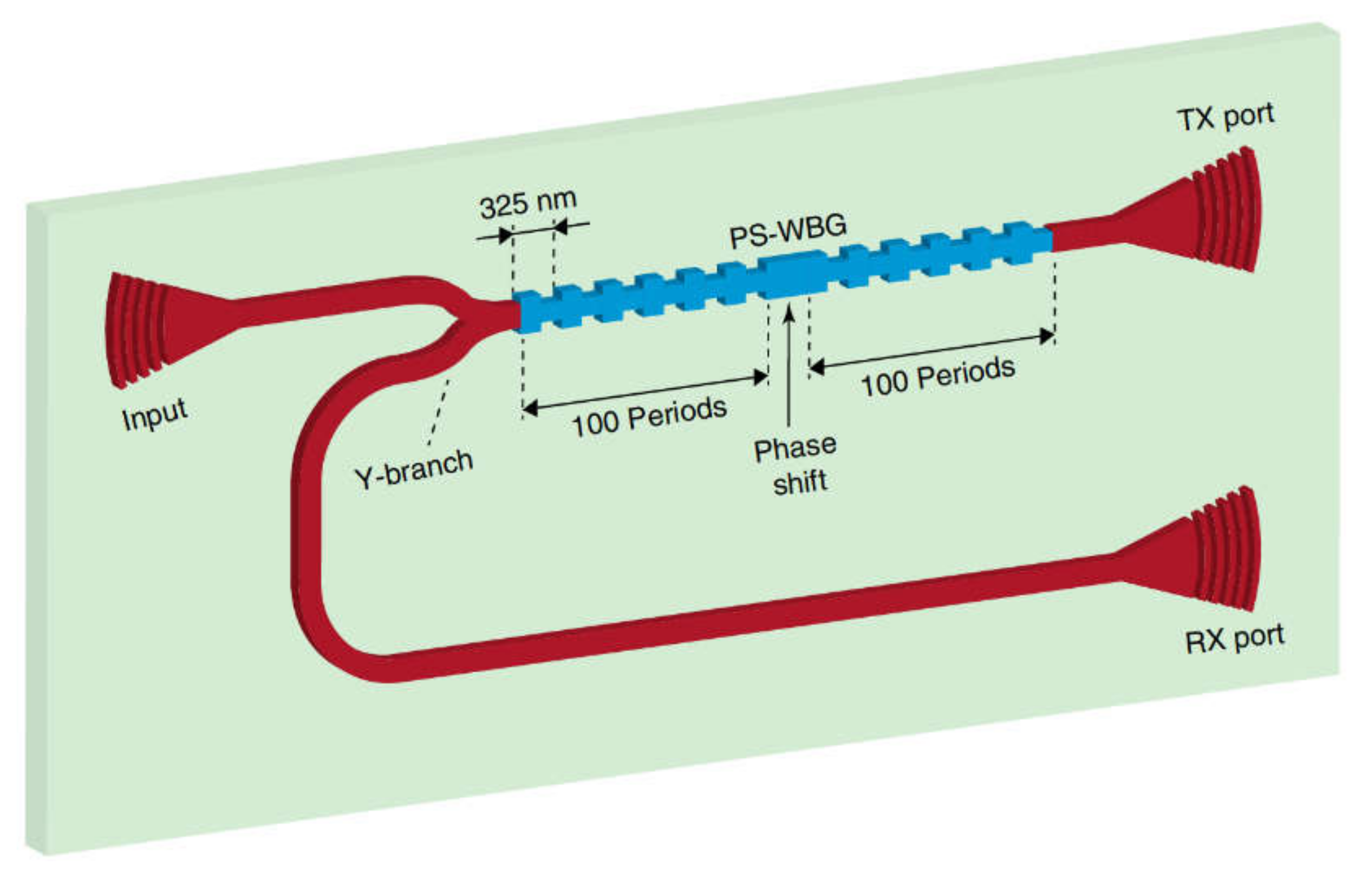
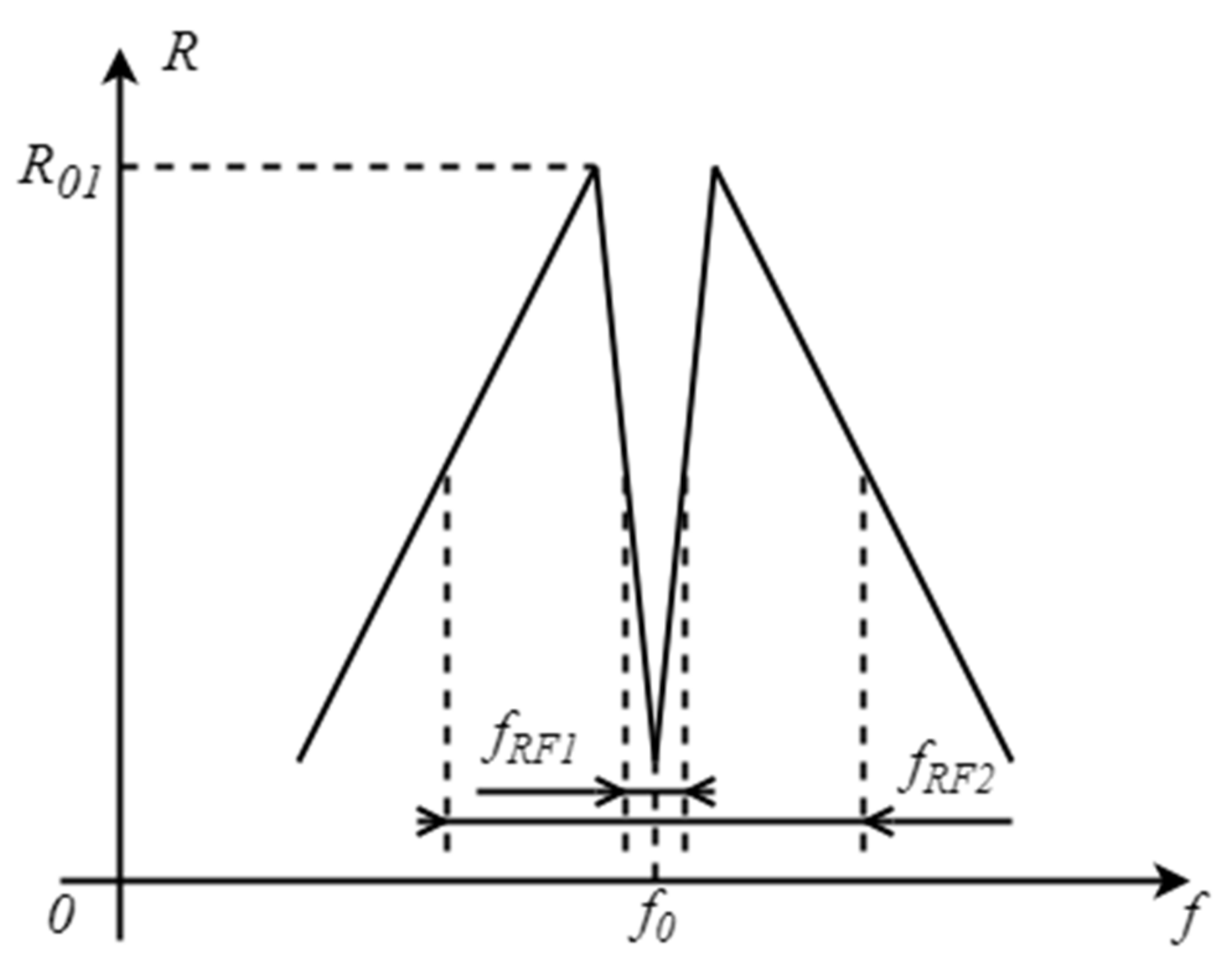
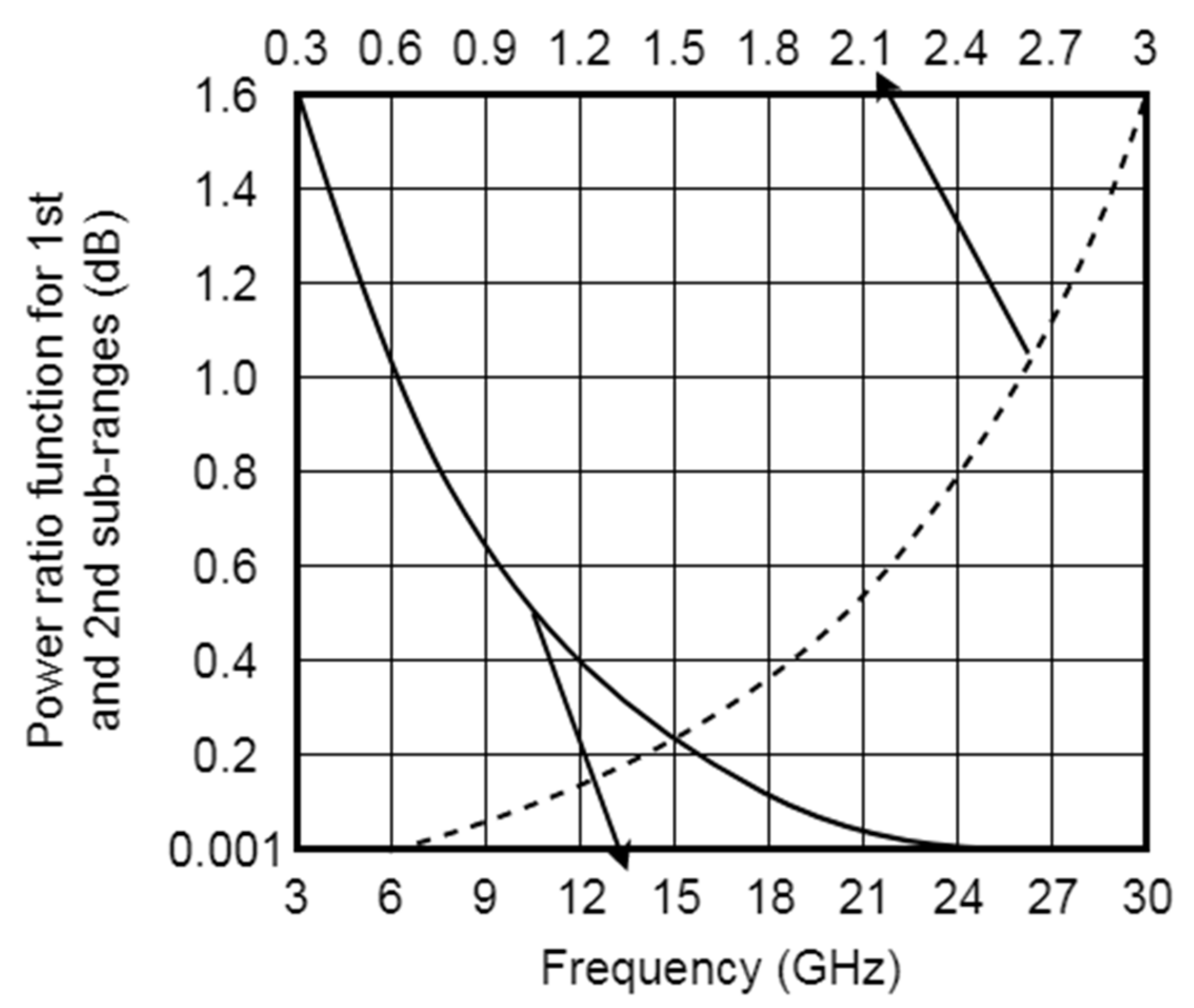
| Parameter | Photonics-Based Transceiver |
|---|---|
| Transmitter | |
| Carrier frequency | Flexible direct generation from 0.04 to 40 GHz |
| Signal-to-noise ratio | >73 dB MHz |
| Spurious-free dynamic range | >70 dBc |
| Instantaneous bandwidth | 200 MHz, easily extendable with MLL at a higher repetition rate |
| Receiver | |
| Input carrier frequency | Up to 40 GHz, with direct RF under sampling |
| Instantaneous bandwidth | 200 MHz, easily extendable with MLL at a higher repetition rate |
| Spurious-free dynamic range | 50 dB |
| Effective number of bits | >7 for carrier frequencies up to 40 GHz |
| Reference | Technology/Method | Frequency Range, GHz | Measurement Error, GHz |
|---|---|---|---|
| [23] | FBG with concave profile | 1–10 | ±0.2 |
| [24] | Polarization maintaining FBG | 0–17.2 | ±0.12 |
| [25] | Gaussian FBG | 0–10 | ±0.08 |
| [26] | Gaussian FBG | 0.04–20 | n/a |
| [27] | Gaussian FBG | 3–18 | ±0.22 |
| Reference | Technology/Method | Frequency Range, GHz | Measurement Error, GHz |
|---|---|---|---|
| [28] | Suppressed carrier double sideband modulation | 0.3–30 | n/a |
| [29] | Based on two-port MZM, optical single sideband | 0–32 | ±0.755 |
| [30] | Double sideband modulation | 2–18 | n/a |
| [31] | Based on dual-parallel Mach–Zehnder modulator, double sideband modulation | 0–50 | ±0.618 |
| Reference | Technology/Method | Frequency Range, GHz | Measurement Error, GHz |
|---|---|---|---|
| [32] | Double sideband suppressed carrier modulation | 0.5–35 | ±0.024 |
| [33] | Double sideband suppressed carrier modulation | 2–34 | ±0.0185 |
| [34] | Double sideband modulation | Up to 32.5 | n/a |
| [35] | Phase modulation | 0.5–4 | ±0.0936 |
| [36] | Phase modulation | 0.5–12 | ±0.4 |
| Reference | Technology/Method | Frequency Range, GHz | Measurement Error, GHz |
|---|---|---|---|
| [4] | Single sideband modulation | 0.5–28 | ±0.02 |
| [37] | Double sideband suppressed carrier modulation | 9–38 | ±0.001 |
| [38] | Double sideband suppressed carrier modulation | 0.47–23 | ±0.008 |
| [39] | Double sideband suppressed carrier modulation | 1–9 | ±0.03 |
| [40] | Single sideband suppressed carrier modulation | Up to 40 | ±0.001 |
| Reference | Technology/Method | Frequency Range, GHz | Measurement Error, GHz |
|---|---|---|---|
| [41] | Double sideband suppressed carrier modulation | 0–15 | ±0.5 |
| Reference | IFM Technique | Technology/Method | Frequency Range, GHz | Measurement Error, GHz |
|---|---|---|---|---|
| [24] | FBG | Single sideband modulation | 0.5–28 | ±0.02 |
| [31] | PS-FBG | Double sideband suppressed carrier modulation | 9–38 | ±0.001 |
| [33] | MRR | Double sideband suppressed carrier modulation | 0.47–23 | ±0.008 |
| [40] | SBS | Double sideband suppressed carrier modulation | 1–9 | ±0.03 |
| [41] | Fano | Single sideband suppressed carrier modulation | Up to 40 | ±0.001 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ivanov, A.; Morozov, O.; Sakhabutdinov, A.; Kuznetsov, A.; Nureev, I. Photonic-Assisted Receivers for Instantaneous Microwave Frequency Measurement Based on Discriminators of Resonance Type. Photonics 2022, 9, 754. https://doi.org/10.3390/photonics9100754
Ivanov A, Morozov O, Sakhabutdinov A, Kuznetsov A, Nureev I. Photonic-Assisted Receivers for Instantaneous Microwave Frequency Measurement Based on Discriminators of Resonance Type. Photonics. 2022; 9(10):754. https://doi.org/10.3390/photonics9100754
Chicago/Turabian StyleIvanov, Alexander, Oleg Morozov, Airat Sakhabutdinov, Artem Kuznetsov, and Ilnur Nureev. 2022. "Photonic-Assisted Receivers for Instantaneous Microwave Frequency Measurement Based on Discriminators of Resonance Type" Photonics 9, no. 10: 754. https://doi.org/10.3390/photonics9100754
APA StyleIvanov, A., Morozov, O., Sakhabutdinov, A., Kuznetsov, A., & Nureev, I. (2022). Photonic-Assisted Receivers for Instantaneous Microwave Frequency Measurement Based on Discriminators of Resonance Type. Photonics, 9(10), 754. https://doi.org/10.3390/photonics9100754







