Phase-Inserted Fiber Gratings and Their Applications to Optical Filtering, Optical Signal Processing, and Optical Sensing: Review
Abstract
1. Introduction
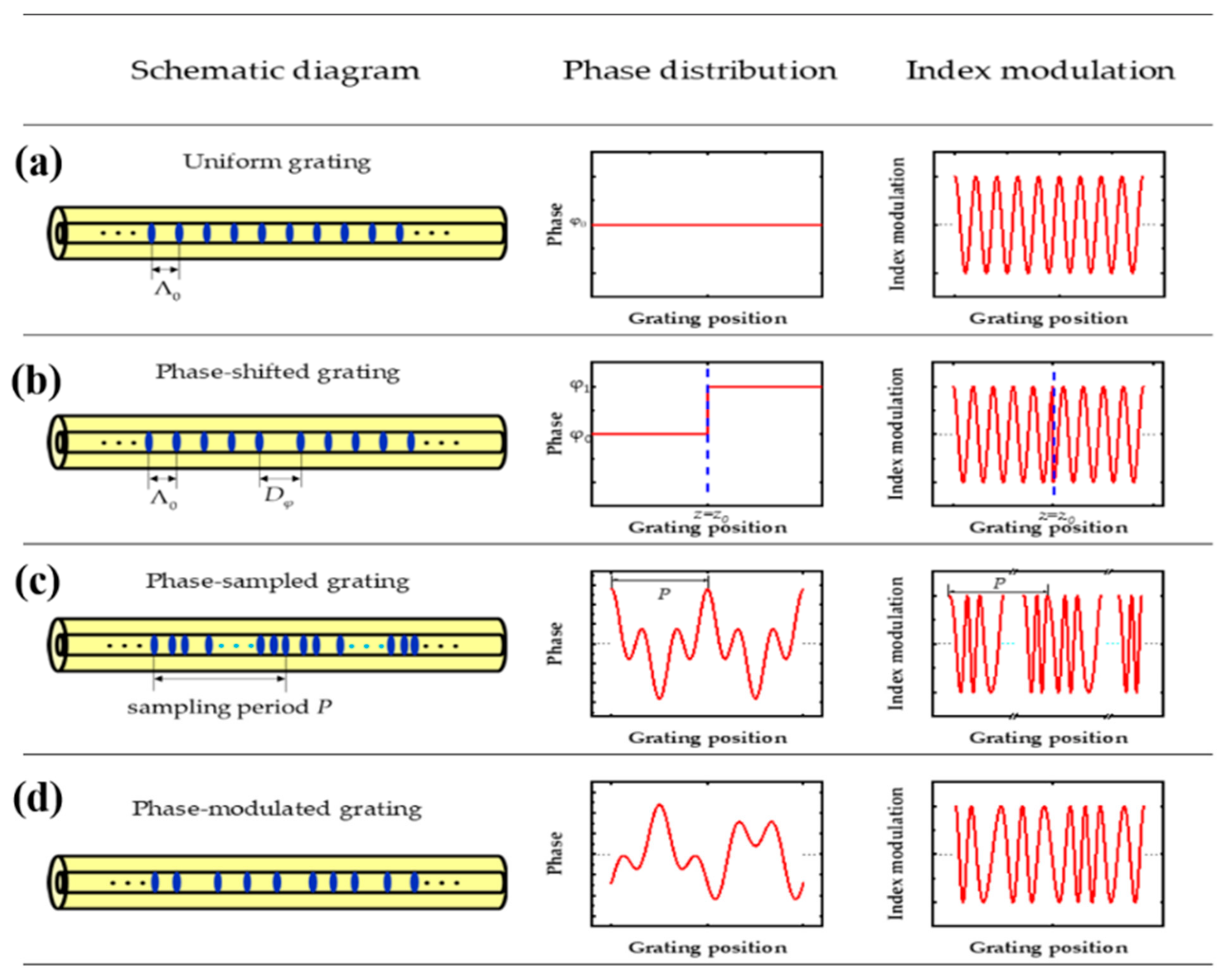
2. Phase-Inserted Fiber Bragg Grating
2.1. Phase-Shifted FBGs (PS-FBGs) and Their Applications
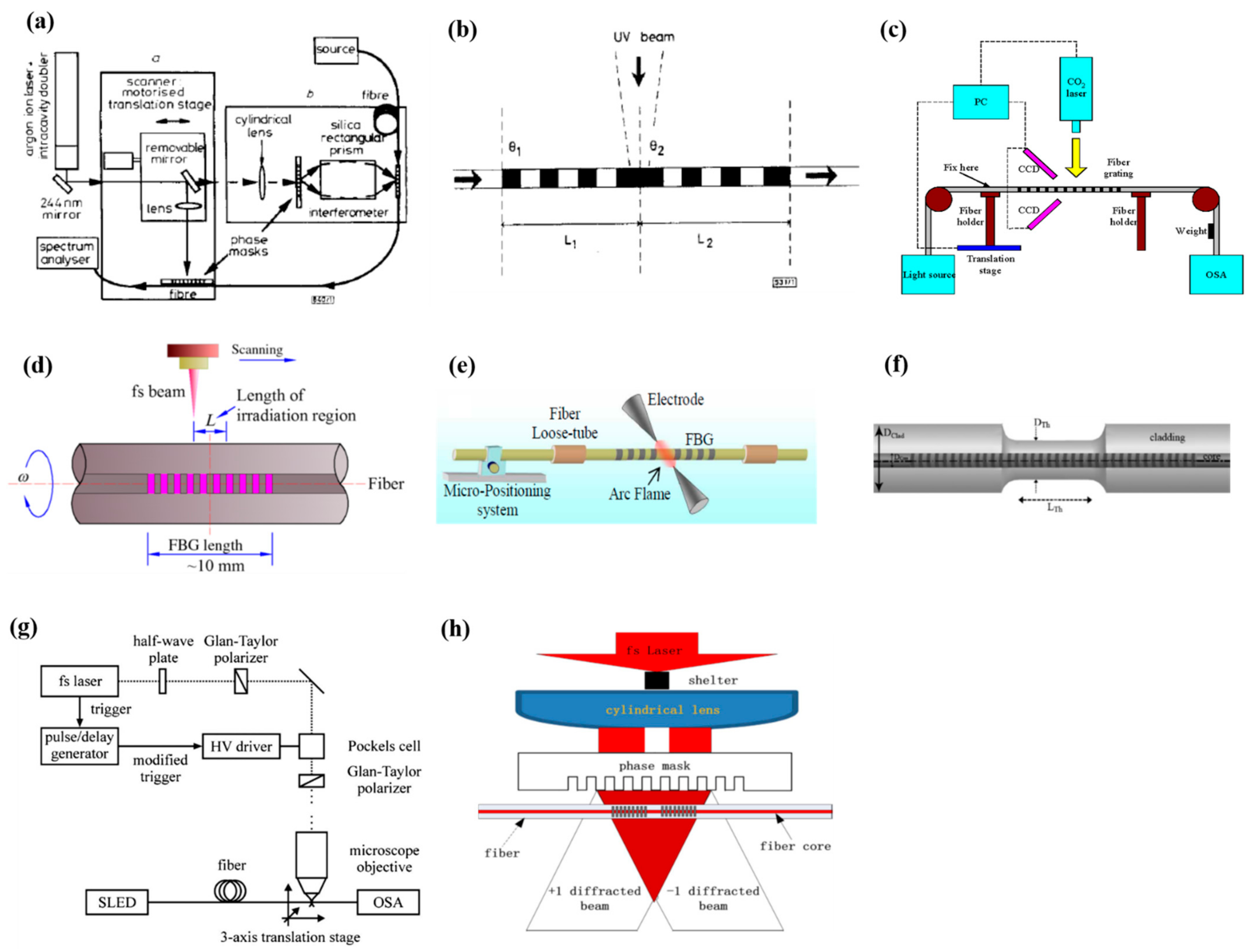
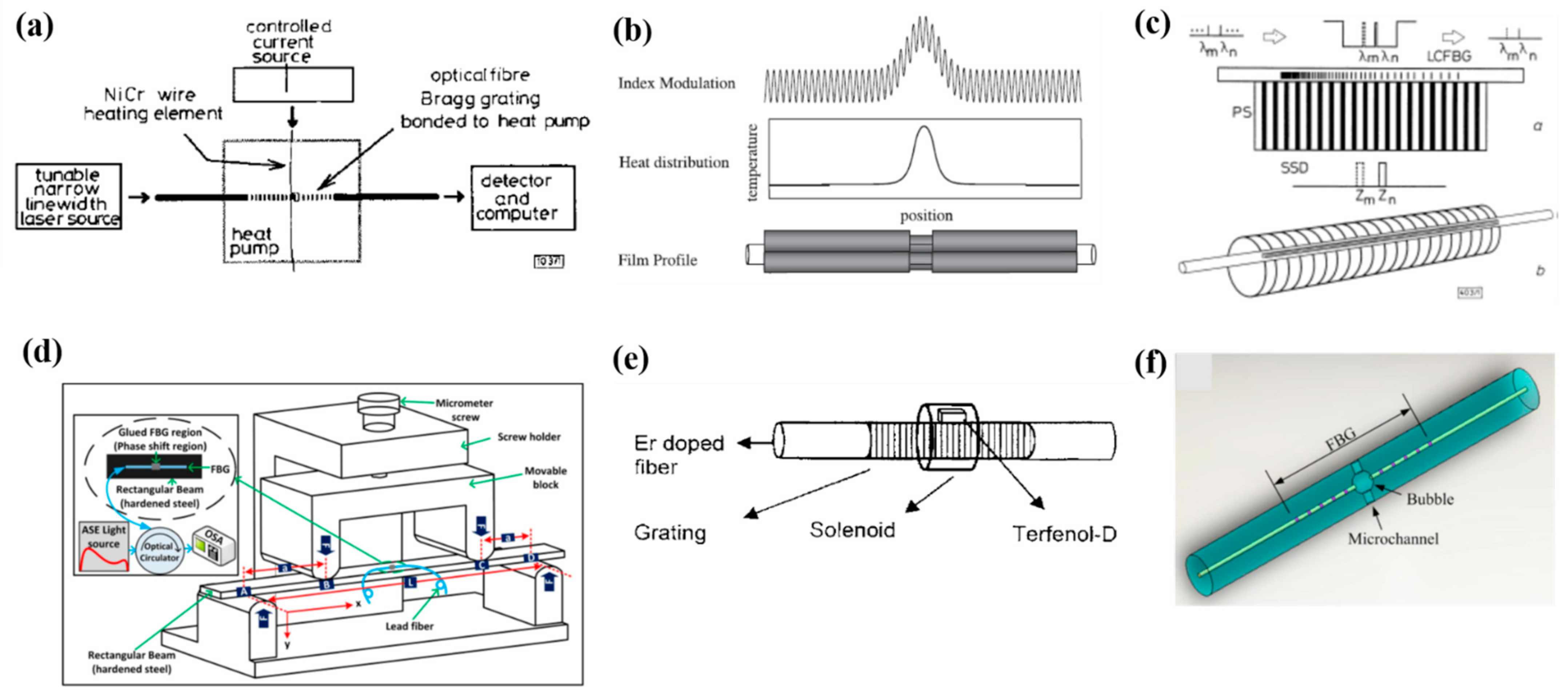
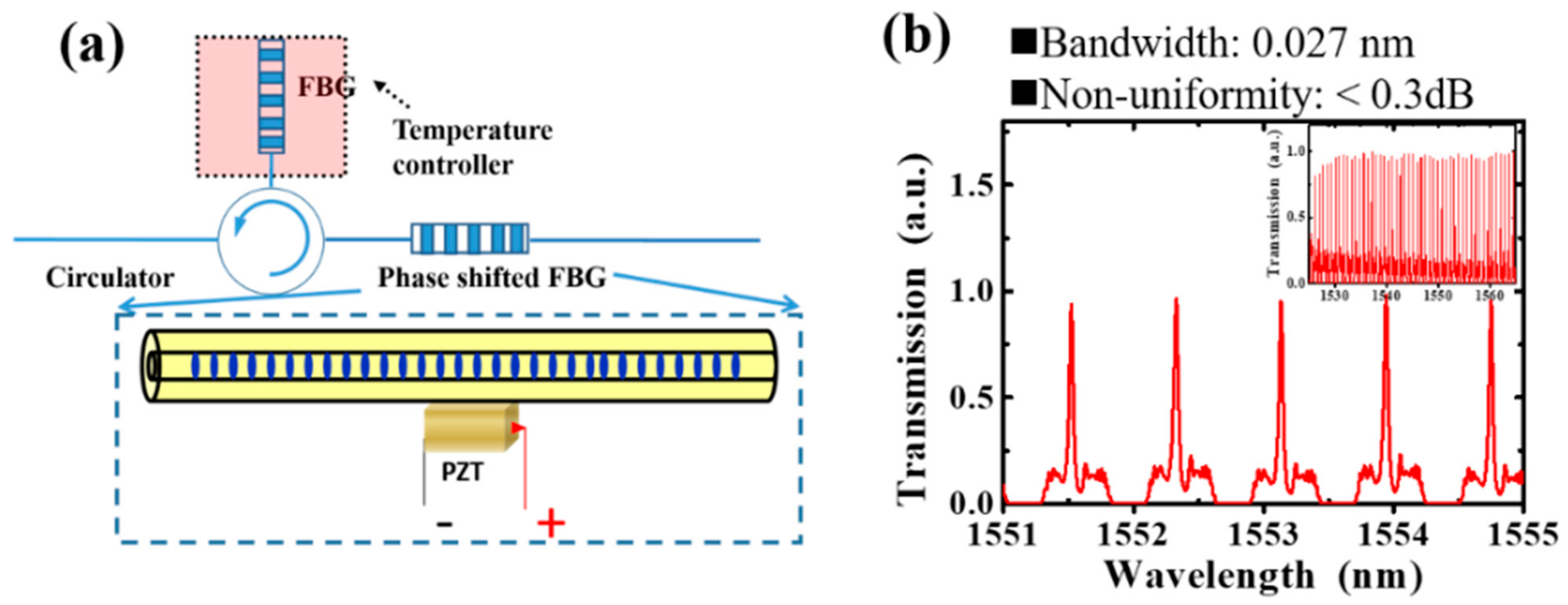
2.1.1. Principle and Fabrication Methods of the PS-FBG
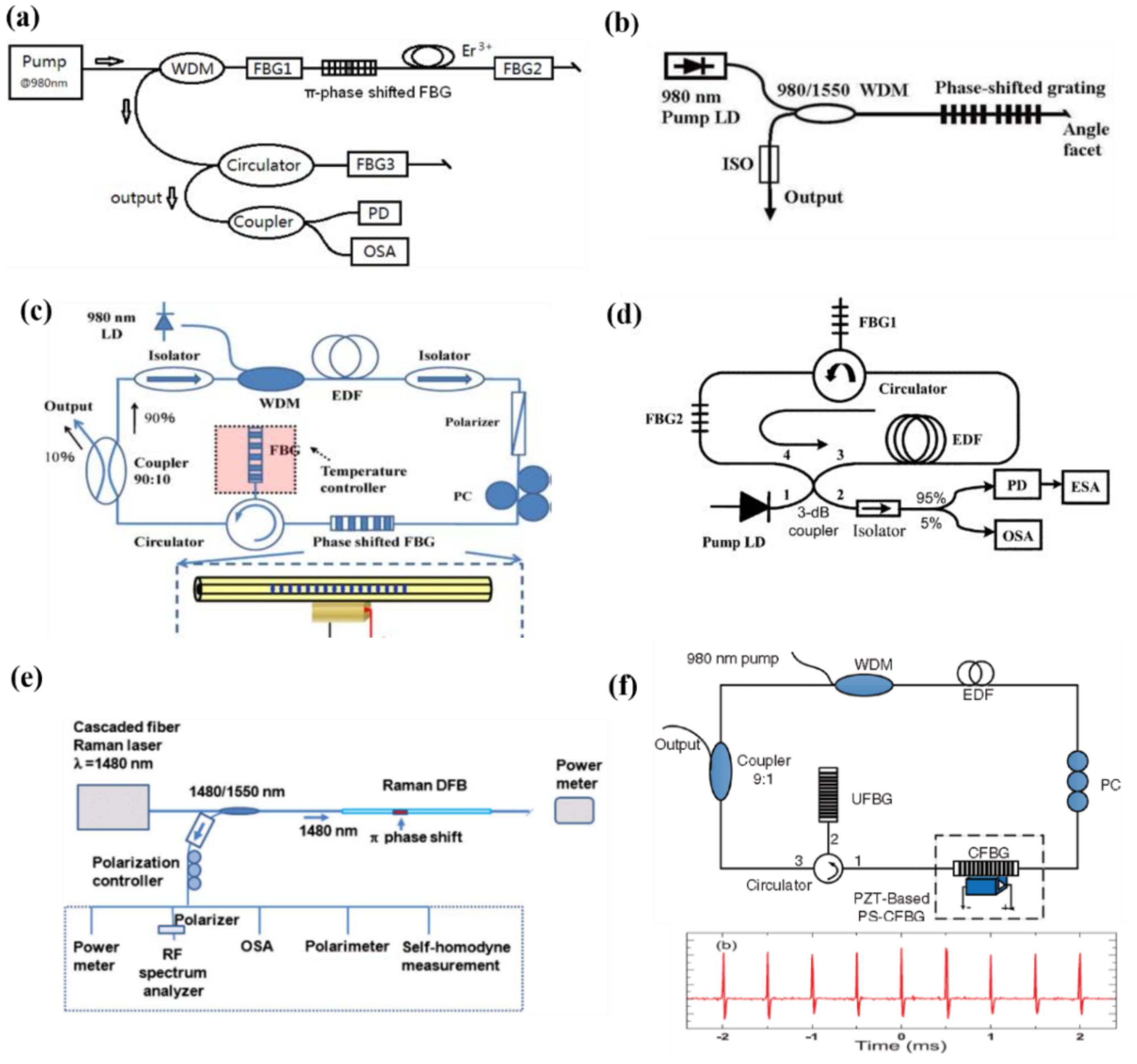
2.1.2. Applications of the Phase-Shifted FBG to Fiber Lasers
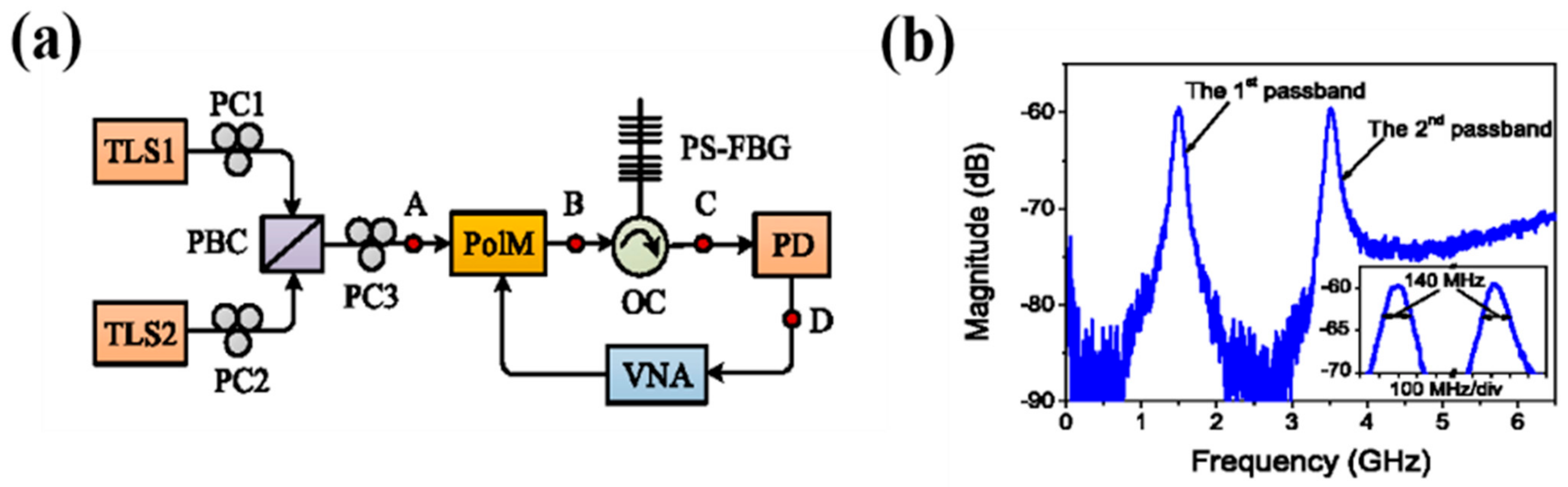
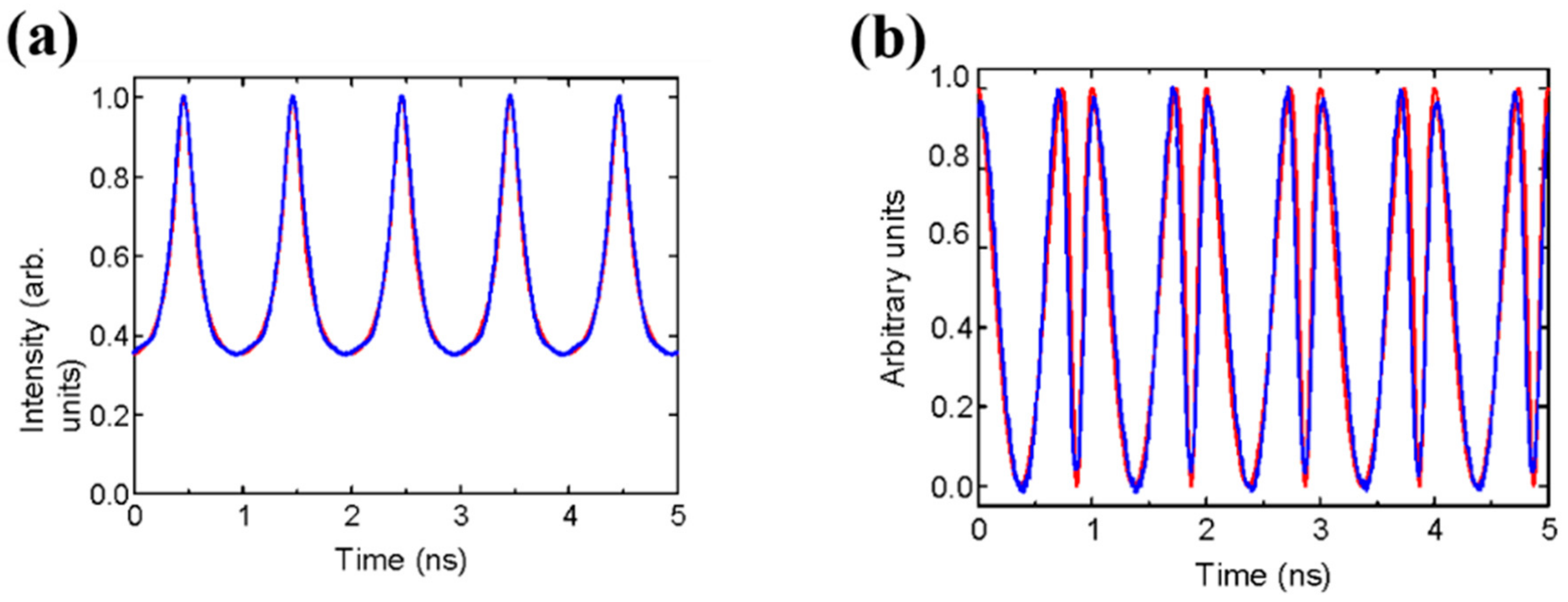
2.1.3. Applications of the PS-FBGs to Microwave Photonics
2.1.4. Applications of the PS-FBGs to WDM and Comb Filters
2.1.5. Applications of the PS-FBGs to Optical Switching
2.1.6. Applications of the PS-FBGs to All-Optical Computing Devices
2.1.7. Applications of the PS-FBGs to Fiber Sensors
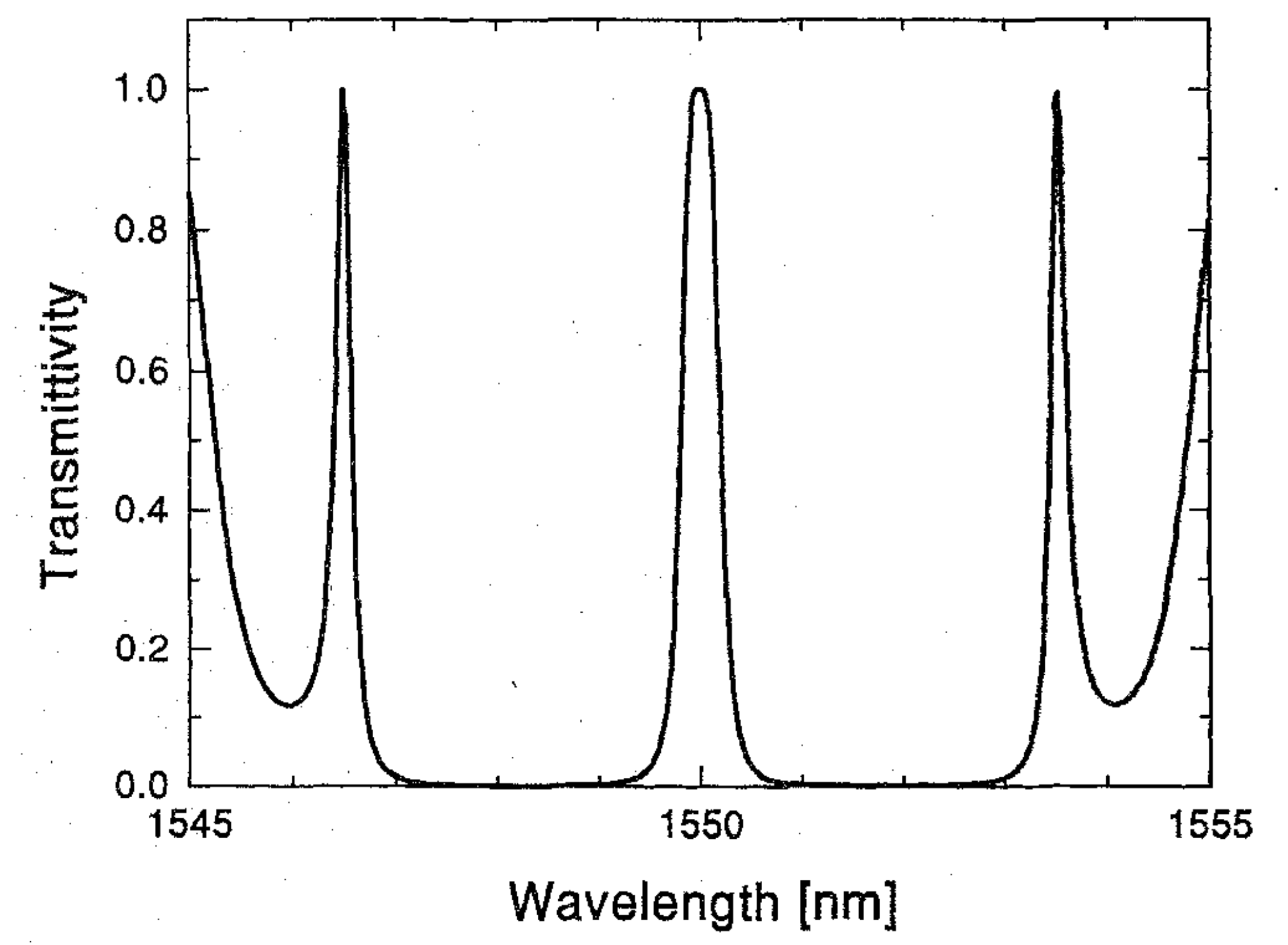
2.2. Phase-Only Sampled FBGs and Their Applications
2.2.1. Principle and Optimization of the Phase-Only Sampled FBG
2.2.2. Fabrication and Experimental Results for the Designed Multi-Channel FBG
2.2.3. Applications of the Phase-Only Sampled FBG in the Fields of Optical Communications and Optical Signal Processing
2.3. Phase-Modulated FBGs and Their Applications
3. Phase-Inserted Long-Period Fiber Grating
3.1. Phase-Shifted LPGs and Their Applications
3.1.1. Spectral Characteristics and Fabrication Techniques for the Phase-Shifted LPG
3.1.2. Applications of the PS-LPGs to All-Fiber Optical Filters and All-Optical Computing Devices
3.1.3. Applications of the PS-LPGs to Fiber Sensors
3.2. Phase-Only Sampled LPGs/HLPGs and Their Applications
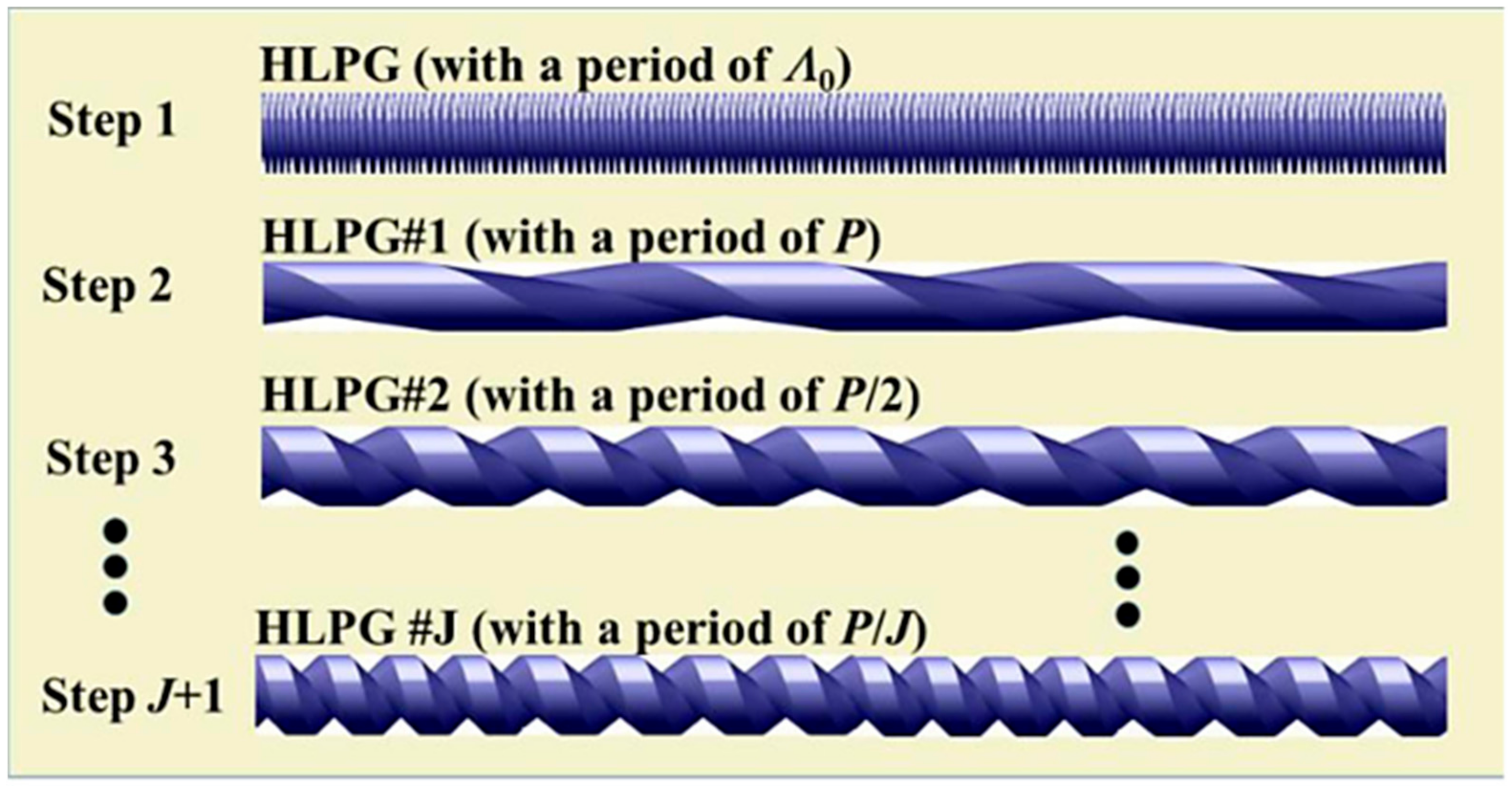
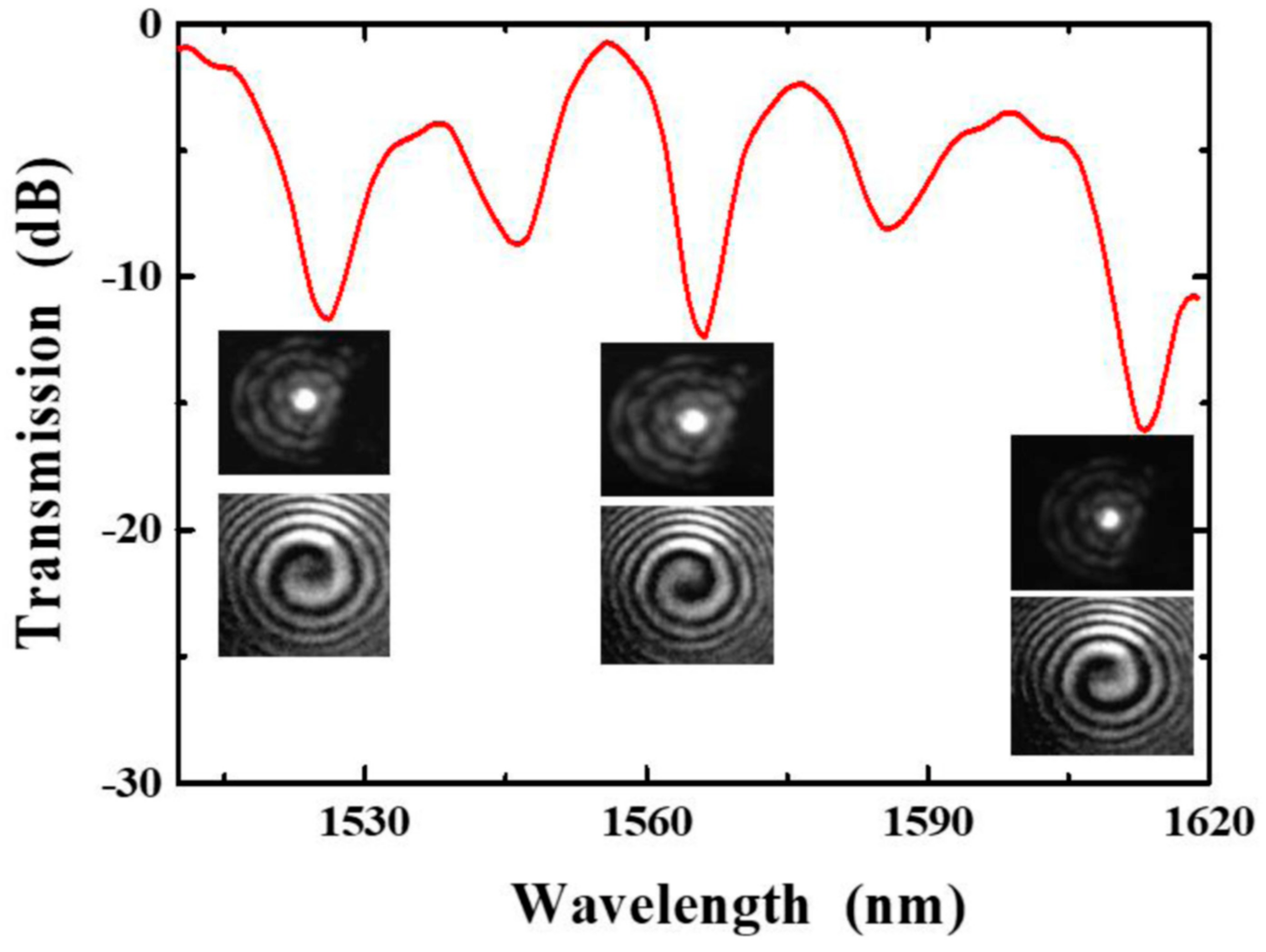
3.2.1. Design Principle and Fabrication Results for the Phase-Only Sampled HLPG
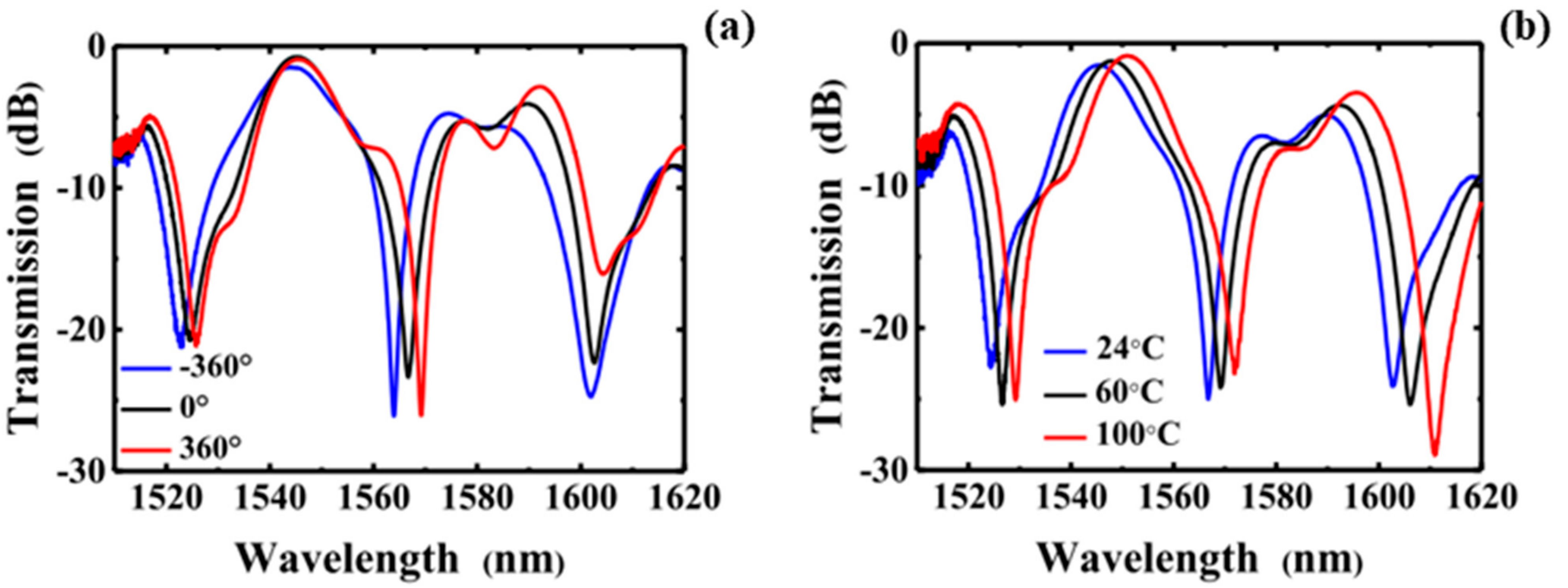
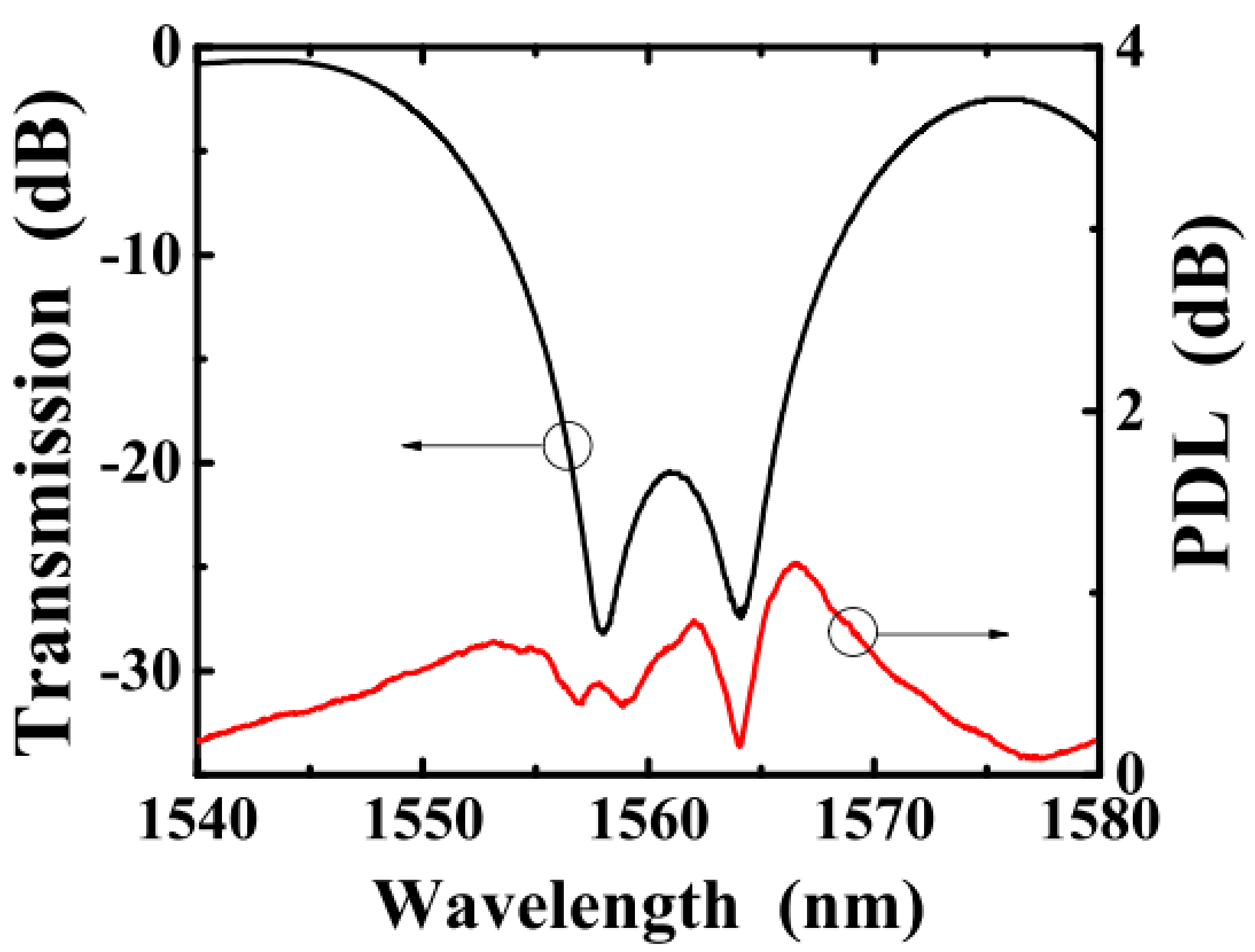
3.2.2. Applications of the Phase-Only Sampled HLPG/LPG
3.3. Phase-Modulated HLPGs and Their Applications
4. Conclusions and Prospects
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hill, K.O.; Fujii, Y.; Johnson, D.C.; Kawasaki, B.S. Photosensitivity in optical fiber waveguides: Applications to reflection filter fabrication. Appl. Phys. Lett. 1978, 32, 647–649. [Google Scholar] [CrossRef]
- Meltz, G.; Morey, W.W.; Glenn, W.H. Formation of Bragg gratings in optical fibers by a transverse holographic method. Opt. Lett. 1989, 14, 823–825. [Google Scholar] [CrossRef] [PubMed]
- Hill, K.O.; Meltz, G. Fiber Bragg grating technology fundamentals and overview. J. Lightw. Technol. 1997, 15, 1263–1276. [Google Scholar] [CrossRef]
- Giles, C.R. Lightwave applications of fiber Bragg gratings. J. Lightw. Technol. 1997, 15, 1391–1404. [Google Scholar] [CrossRef]
- Erdogan, T. Fiber Grating Spectra. J. Lightw. Technol. 1997, 15, 1277–1294. [Google Scholar] [CrossRef]
- Agrawal, G.P.; Radic, S. Phase-shifted fiber Bragg gratings and their application for wavelength demultiplexing. IEEE Photon. Technol. Lett. 1994, 6, 995–997. [Google Scholar] [CrossRef]
- Zengerle, R.; Leminger, O. Phase-Shifted Bragg-grating filters with improved transmission characteristics. J. Lightw. Technol. 1995, 13, 2354–2358. [Google Scholar] [CrossRef]
- Legoubin, S.; Fertein, E.; Douay, M.; Bernage, P.; Niay, P.; Bayon, F.; Georges, T. Formation of moire grating in core of Germanosilicate fibre by transverse holographic double exposure method. Electron. Lett. 1991, 27, 1945–1946. [Google Scholar] [CrossRef]
- Kashyap, R.; Mckee, P.F.; Armes, D. UV written reflection grating structures in photosensitive optical fibres using phase-shifted phase masks. Electron. Lett. 1994, 30, 1977–1978. [Google Scholar] [CrossRef]
- Canning, J.; Sceats, M.G. π-phase-shifted periodic distributed structures in optical fibres by UV post-processing. Electron. Lett. 1994, 30, 1344–1345. [Google Scholar] [CrossRef]
- Li, X.; Ping, S.; Chao, L. Phase-shifted bandpass filter fabrication through CO2 laser irradiation. Opt. Exp. 2005, 13, 5878–5882. [Google Scholar]
- Zhou, X.; Dai, Y.; Karanja, J.M.; Liu, F.; Yang, M. Fabricating phase-shifted fiber Bragg grating by simple postprocessing using femtosecond laser. Opt. Eng. 2017, 56, 027108. [Google Scholar] [CrossRef]
- Cusano, A.; Iadicicco, A.; Paladino, D.; Campopiano, S.; Cutolo, A. Photonic band-gap engineering in UV fiber gratings by the arc discharge technique. Opt. Exp. 2008, 16, 15332–15342. [Google Scholar] [CrossRef]
- Iadicicco, A.; Campopiano, S.; Cutolo, A.; Giordano, M.; Cusano, A. Microstructured fibre Bragg gratings: Analysis and fabrication. Electron. Lett. 2005, 41, 466–468. [Google Scholar] [CrossRef]
- Cusano, A.; Iadicicco, A.; Paladino, D.; Campopiano, S.; Cutolo, A.; Giordano, M. Micro-structured fiber Bragg gratings. Part I: Spectral characteristics. Opt. Fiber Technol. 2007, 13, 281–290. [Google Scholar] [CrossRef]
- Burgmeier, J.; Waltermann, C.; Flachenecker, G.; Schade, W. Point-by-point inscription of phase-shifted fiber Bragg gratings with electro-optic amplitude modulated femtosecond laser pulses. Opt. Lett. 2014, 39, 540–543. [Google Scholar] [CrossRef]
- Huang, B.; Shu, X. Ultra-compact strain- and temperature-insensitive torsion sensor based on a line-by-line inscribed phase-shifted FBG. Opt. Exp. 2016, 24, 17670–17679. [Google Scholar] [CrossRef]
- Shamir, A.; Ishaaya, A.A. Femtosecond inscription of phase-shifted gratings by overlaid fiber Bragg gratings. Opt. Lett. 2016, 41, 2017–2020. [Google Scholar] [CrossRef]
- Du, Y.; Chen, T.; Zhang, Y.; Wang, R.; Cao, H.; Li, K. Fabrication of phase-shifted fiber Bragg grating by femtosecond laser shield method. IEEE Photon. Technol. Lett. 2017, 29, 2143–2146. [Google Scholar] [CrossRef]
- Zhou, J.; Guo, K.; He, J.; Hou, M.; Zhang, Z.; Liao, C.; Wang, Y.; Xu, G.; Wang, Y. Novel fabrication technique for phase-shifted fiber Bragg gratings using a variable-velocity scanning beam and a shielded phase mask. Opt. Exp. 2018, 26, 13311–13321. [Google Scholar] [CrossRef]
- Hnatovsky, C.; Grobnic, D.; Mihailov, S.J. High-temperature stable π-phase-shifted fiber Bragg gratings inscribed using infrared femtosecond pulses and a phase mask. Opt. Exp. 2018, 26, 23550–235641. [Google Scholar] [CrossRef]
- Kringlebotn, J.T.; Archambault, J.L.; Reekie, L.; Payne, D.N. Er3+:Yb3+-codoped fiber distributed-feedback laser. Opt. Lett. 1994, 19, 2101–2103. [Google Scholar] [CrossRef]
- Janos, M.; Canning, J. Permanent and transient resonances thermally induced in optical-fiber Bragg gratings. Electron. Lett. 1995, 31, 1007–1009. [Google Scholar] [CrossRef]
- Mokhtar, M.R.; Ibsen, M.; Teh, P.C.; Richardson, D.J. Reconfigurable multilevel phase-shift keying encoder-decoder for all-optical networks. IEEE Photon. Technol. Lett. 2003, 15, 431–433. [Google Scholar] [CrossRef]
- Li, S.Y.; Ngo, N.Q.; Tjin, S.C.; Shum, P.; Zhang, J. Thermally tunable narrow-bandpass filter based on a linearly chirped fiber Bragg grating. Opt. Lett. 2004, 29, 29–31. [Google Scholar] [CrossRef]
- Li, M.; Li, H.; Painchaud, Y. Multi-channel notch filter based on a phase-shift phase-only-sampled fiber Bragg grating. Opt. Exp. 2008, 16, 19388–19394. [Google Scholar] [CrossRef] [PubMed]
- Ahuja, A.K.; Steinvurzel, P.E.; Eggleton, B.J.; Rogers, J.A. Tunable single phase-shifted and superstructure gratings using microfabricated on-fiber thin film heaters. Opt. Commun. 2000, 184, 119–125. [Google Scholar] [CrossRef]
- Xu, M.G.; Alavie, A.T.; Maaskant, R.; Ohn, M.M. Tunable fibre bandpass filter based on a linearly chirped fibre Bragg grating for wavelength demultiplexing. Electron. Lett. 1996, 32, 1918–1919. [Google Scholar] [CrossRef]
- Ohn, M.M.; Alavie, A.T.; Maaskant, R.; Xu, M.G. Dispersion variable fibre Bragg grating using a piezoelectric stack. Electron. Lett. 1996, 32, 2000–2001. [Google Scholar] [CrossRef]
- Pacheco, M.; Mendez, A.; Santoyo, F.M.; Zenteno, L.A. Analysis of the spectral characteristics of piezoelectrically driven dual and triple-period optical fibre Bragg gratings. Opt. Commun. 1999, 167, 89–94. [Google Scholar] [CrossRef]
- Chen, X.; Painchaud, Y.; Ogusu, K.; Li, H. Phase shifts induced by the piezoelectric transducers attached to a linearly chirped fiber Bragg grating. J. Lightw. Technol. 2010, 28, 2017–2022. [Google Scholar] [CrossRef]
- Wu, L.; Pei, L.; Wang, J.; Li, J.; Ning, T.; Liu, S. Q-switched erbium-doped fiber ring laser with piezoelectric transducer-based PS-CFBG. Laser Phys. Lett. 2016, 13, 95101. [Google Scholar] [CrossRef]
- Hamarsheh, M.M.N.; Falah, A.A.S.; Mokhtar, M.R. Tunable fiber Bragg grating phase shift by simple pressure packaging. Opt. Eng. 2015, 54, 016105. [Google Scholar] [CrossRef]
- Falah, A.A.S.; Mokhtar, M.R.; Yusoff, Z.; Ibsen, M. Reconfigurable phase shifted fiber Bragg grating using localized micro-strain. IEEE Photon. Technol. Lett. 2016, 28, 951–954. [Google Scholar] [CrossRef]
- Perez-Millan, P.; Cruz, J.L.; Andres, M.V. Active Q-switched distributed feedback erbium-doped fiber lasers. Appl. Phys. Lett. 2005, 87, 011104. [Google Scholar] [CrossRef]
- Gonzalez-Segura, A.; Perez-Millan, P.; Cruz, J.L.; Andres, M.V. Fiber ring laser operated by dynamic local phase shifting of a chirped grating. IEEE Photon. Technol. Lett. 2009, 21, 417–419. [Google Scholar] [CrossRef]
- Liao, C.; Xu, L.; Wang, C.; Wang, D.N.; Wang, Y.; Wang, Q.; Yang, K.; Li, Z.; Zhong, X.; Zhou, J.; et al. Tunable phase-shifted fiber Bragg grating based on femtosecond laser fabricated in-grating bubble. Opt. Lett. 2013, 38, 4473–4476. [Google Scholar] [CrossRef]
- Zeng, L.; Sun, X.; Chang, Z.; Hu, Y.; Duan, J. Tunable phase-shifted fiber Bragg grating based on a microchannel fabricated by a femtosecond laser. Chin. Opt. Lett. 2021, 19, 030602. [Google Scholar] [CrossRef]
- Dai, Y.; Chen, X.; Jiang, D.; Xie, S.; Fan, C. Equivalent phase shift in a fiber Bragg grating achieved by changing the sampling period. IEEE Photon. Technol. Lett. 2004, 16, 2284–2286. [Google Scholar] [CrossRef]
- Othonos, A. Fiber Bragg gratings. Rev. Sci. Instrum. 1997, 68, 4309–4341. [Google Scholar] [CrossRef]
- Liu, X. A novel ultra-narrow transmission-band fiber Bragg grating and its application in a single-longitudinal-mode fiber laser with improved efficiency. Opt. Commun. 2007, 280, 147–152. [Google Scholar] [CrossRef]
- Zhao, Y.; Chang, J.; Wang, Q.; Ni, J.; Song, Z.; Qi, H.; Wang, C.; Wang, P.; Gao, L.; Sun, Z.; et al. Research on a novel composite structure Er3+-doped DBR fiber laser with a pi-phase shifted FBG. Opt. Exp. 2013, 21, 22515–22522. [Google Scholar] [CrossRef]
- Zha, Y.; Xu, Z.; Xiao, P.; Feng, F.; Ran, Y.; Guan, B. Phase-shifted type-IIa fiber Bragg gratings for high-temperature laser applications. Opt. Exp. 2019, 27, 4346–4353. [Google Scholar] [CrossRef]
- Asseh, A.; Storoy, H.; Kringlebotn, J.T.; Margulis, W.; Sahlgren, B.; Sandgren, S.; Stubbe, R.; Edwall, G. 10 cm Yb3+ DFB fibre laser with permanent phase shifted grating. Electron. Lett. 1995, 31, 969–970. [Google Scholar] [CrossRef]
- Loh, W.H.; Laming, R.I. 1.55 μm phase-shifted distributed-feedback fiber laser. Electron. Lett. 1995, 31, 1440–1442. [Google Scholar] [CrossRef]
- Qi, H.; Song, Z.; Li, S.; Guo, J.; Wang, C.; Peng, G. Apodized distributed feedback fiber laser with asymmetrical outputs for multiplexed sensing applications. Opt. Exp. 2013, 21, 11309–11314. [Google Scholar] [CrossRef]
- Jiang, M.; Zhou, P.; Gu, X. Ultralong π-phase shift fiber Bragg grating empowered single-longitudinal mode DFB phosphate fiber laser with low-threshold and high-efficiency. Sci. Rep. 2018, 8, 13131. [Google Scholar] [CrossRef]
- Li, S.Y.; Ngo, N.Q.; Zhang, Z.R. Tunable fiber laser with ultra-narrow linewidth using a tunable phase-shifted chirped fiber grating. IEEE Photon. Technol. Lett. 2008, 20, 1482–1484. [Google Scholar] [CrossRef]
- Xian, L.; Li, H. Calibration of a phase-shift formed in a linearly chirped fiber Bragg grating and its thermal effect. J. Lightw. Technol. 2013, 31, 1185–1190. [Google Scholar] [CrossRef]
- Rota-Rodrigo, S.; Rodriguez-Cobo, L.; Quintela, M.A.; Lopez-Higuera, J.M.; Lopez-Amo, M. Dual-wavelength single-longitudinal mode fiber laser using phase-shift Bragg gratings. IEEE J. Sel. Top. Quantum Electron. 2014, 20, 0900305. [Google Scholar] [CrossRef]
- Chen, X.; Yao, J.; Zeng, F.; Deng, Z. Single-longitudinal-mode fiber ring laser employing an equivalent phase-shifted fiber Bragg grating. IEEE Photon. Technol. Lett. 2005, 17, 1390–1392. [Google Scholar] [CrossRef]
- Sun, J.; Dai, Y.; Zhang, Y.; Chen, X.; Xie, S. Dual-wavelength DFB fiber laser based on unequalized phase shifts. IEEE Photon. Technol. Lett. 2006, 18, 2493–2495. [Google Scholar] [CrossRef]
- Westbrook, P.S.; Abedin, K.S.; Nicholson, J.W.; Kremp, T.; Porque, J. Raman fiber distributed feedback lasers. Opt. Lett. 2011, 36, 2895–2897. [Google Scholar] [CrossRef] [PubMed]
- Cheng, X.P.; Tse, C.H.; Shurn, P.; Wu, R.F.; Tang, M.; Tan, W.C.; Zhang, J. All-fiber Q-switched erbium-doped fiber ring laser using phase-shifted fiber Bragg grating. J. Lightw. Technol. 2008, 26, 945–951. [Google Scholar] [CrossRef]
- Kai, L.; Cheng, M.; Sun, J. Minute Wavelength shift detection of actively mode-locked fiber laser based on stimulated Brillouin scattering Effect. J. Lightw. Technol. 2021, 39, 4447–4452. [Google Scholar] [CrossRef]
- Yao, J. Microwave Photonics. J. Lightw. Technol. 2009, 27, 314–335. [Google Scholar] [CrossRef]
- Chen, X.; Deng, Z.; Yao, J. Photonic generation of microwave signal using a dual-wavelength single-longitudinal-mode fiber ring laser. IEEE Trans. Microw. Theory Techn. 2006, 54, 804–809. [Google Scholar] [CrossRef]
- Jiang, M.; Lin, B.; Shum, P.P.; Tjin, S.C.; Dong, X.; Sun, Q. Tunable microwave generation based on a dual-wavelength single-longitudinal-mode fiber laser using a phase-shifted grating on a triangular cantilever. Appl. Opt. 2011, 50, 1900–1904. [Google Scholar] [CrossRef]
- Lin, B.; Jiang, M.; Tjin, S.C.; Shum, P. Tunable microwave generation using a phase-shifted chirped fiber Bragg grating. IEEE Photon. Technol. Lett. 2011, 23, 1292–1294. [Google Scholar] [CrossRef]
- Li, W.; Kong, F.; Yao, J. Stable and frequency-hopping-free microwave generation based on a mutually injection-locked optoelectronic oscillator and a dual-wavelengthsingle-longitudinal-mode fiber laser. J. Lightw. Technol. 2014, 32, 4174–4179. [Google Scholar]
- Li, W.; Li, M.; Yao, J. A narrow-pass band and frequency-tunable microwave photonic filter based on phase-modulation to intensity-modulation conversion using a phase-shifted fiber Bragg grating. IEEE Trans. Microw. Theory Tech. 2012, 60, 1287–1296. [Google Scholar] [CrossRef]
- Gao, L.; Zhang, J.; Chen, X.; Yao, J. Microwave photonic filter with two independently tunable passbands using a phase modulator and an equivalent phase-shifted fiber Bragg grating. IEEE Trans. Microw. Theory Tech. 2014, 62, 380–387. [Google Scholar] [CrossRef]
- Han, X.; Xu, E.; Liu, W.; Yao, J. Tunable dual-passband microwave photonic filter using orthogonal polarization modulation. IEEE Photon. Technol. Lett. 2015, 27, 2209–2212. [Google Scholar] [CrossRef]
- Ma, Y.; Zhang, Z.; Yuan, J.; Zeng, Z.; Zhang, S.; Zhang, Y.; Zhang, Z.; Fu, D.; Wang, J.; Liu, Y. Optically tunable microwave frequency downconversion based on an optoelectronic oscillator employing a phase-shifted fiber Bragg grating. IEEE Photon. J. 2018, 10, 5501611. [Google Scholar] [CrossRef]
- Chen, X.; Xian, L.; Ogusu, K.; Li, H. Phase-shift induced in a high-channel-count fiber Bragg grating and its application to multiwavelength fiber ring laser. IEEE Photon. Technol. Lett. 2011, 23, 498–500. [Google Scholar] [CrossRef]
- Li, M.; Fujii, T.; Li, H. Multiplication of a multichannel notch filter based on a phase-shifted phase-only sampled fiber Bragg grating. IEEE Photon. Technol. Lett. 2009, 21, 926–928. [Google Scholar]
- Wei, L.; Lit, J.W.Y. Phase-shifted Bragg grating filters with symmetrical structures. J. Lightw. Technol. 1997, 15, 1405–1410. [Google Scholar] [CrossRef]
- Kulishov, M.; Laniel, J.M.; Bélanger, N.; Azaña, J.; Plant, D.V. Nonreciprocal waveguide Bragg gratings. Opt. Exp. 2005, 13, 3068–3078. [Google Scholar] [CrossRef]
- Lin, Z.; Ramezani, H.; Eichelkraut, T.; Kottos, T.; Cao, H.; Christodoulides, D.N. Unidirectional invisibility induced by PT-Symmetric periodic structures. Phys. Rev. Lett. 2011, 106, 213901. [Google Scholar] [CrossRef]
- Raja, S.V.; Govindarajan, A.; Mahalingam, A.; Lakshmanan, M. Phase-shifted PT-symmetric periodic structures. Phys. Rev. A 2020, 102, 013515. [Google Scholar] [CrossRef]
- Radic, S.; George, N.; Agrawal, G.P. Optical switching in λ/4-shifted nonlinear periodic structures. Opt. Lett. 1994, 19, 1789–1791. [Google Scholar] [CrossRef]
- Radic, S.; George, N.; Agrawal, G.P. Theory of low-threshold optical switching in nonlinear phase-shifted periodic structures. J. Opt. Soc. Am. B 1995, 12, 671–680. [Google Scholar] [CrossRef]
- Lee, H.; Agrawal, G.P. Nonlinear switching of optical pulses in fiber Bragg gratings. IEEE J. Quantum. Electron 2003, 39, 508–515. [Google Scholar]
- Kabakova, I.V.; Walsh, T.; de Sterke, C.M.; Eggleton, B.J. Performance of field-enhanced optical switching in fiber Bragg gratings. J. Opt. Soc. Am. B 2010, 27, 1343–1351. [Google Scholar] [CrossRef]
- Melloni, A.; Chinello, M.; Martinelli, M. All-optical switching in phase-shifted fiber Bragg grating. IEEE Photon. Technol. Lett. 2000, 12, 42–44. [Google Scholar] [CrossRef]
- Li, Q.; Song, J.; Chen, X.; Bi, M.; Hu, M.; Li, S. All-optical logic gates based on cross phase modulation effect in a phase-shifted grating. Appl. Opt. 2016, 55, 6880–6886. [Google Scholar] [CrossRef]
- Gan, X.; Wang, Y.; Zhang, F.; Zhao, C.; Jiang, B.; Fang, L.; Li, D.; Wu, H.; Ren, Z.; Zhao, J. Graphene-controlled fiber Bragg grating and enabled optical bistability. Opt. Lett. 2016, 41, 603–606. [Google Scholar] [CrossRef]
- Azaña, J.; Madsen, C.; Takiguchi, K.; Cincontti, G. Guest editorial-optical signal processing. J. Lightw. Technol. 2006, 24, 2484–2767. [Google Scholar] [CrossRef]
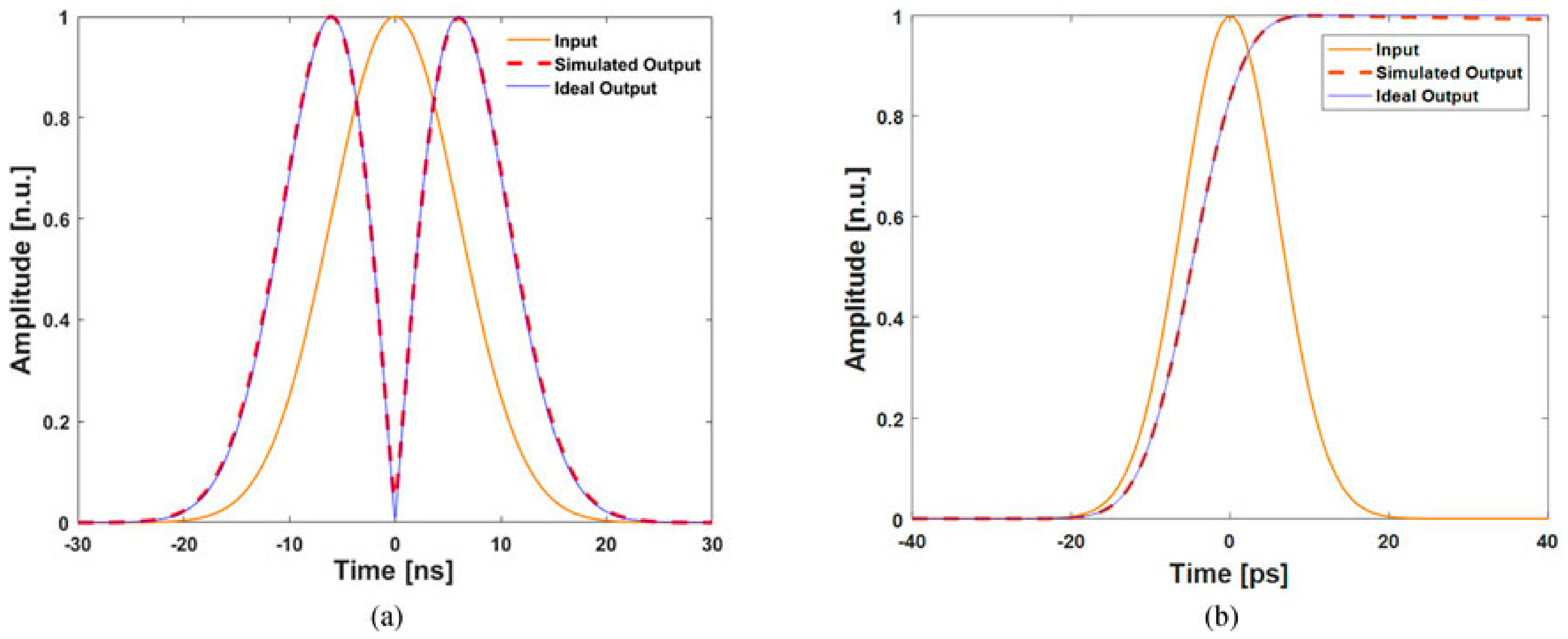
- Berger, N.K.; Levit, B.; Fischer, B.; Kulishov, M.; Plant, D.V.; Azana, J. Temporal differentiation of optical signals using a phase-shifted fiber Bragg grating. Opt. Exp. 2007, 15, 371–381. [Google Scholar] [CrossRef]
- Ngo, N.Q. Design of an optical temporal integrator based on a phase-shifted fiber Bragg grating in transmission. Opt. Lett. 2007, 32, 3020–3022. [Google Scholar]
- Asghari, M.H.; Azana, J. Design of all-optical high-order temporal integrators based on multiple-phase-shifted Bragg gratings. Opt. Exp. 2008, 16, 11459–11469. [Google Scholar] [CrossRef] [PubMed]
- Preciado, M.A.; Muriel, M.A. Design of an ultrafast all-optical differentiator based on a fiber Bragg grating in transmission. Opt. Lett. 2008, 33, 2458–2460. [Google Scholar] [CrossRef] [PubMed]
- Asghari, M.H.; Azana, J. All-optical Hilbert transformer based on a single phase-shifted fiber Bragg grating: Design and analysis. Opt. Lett. 2009, 34, 334–336. [Google Scholar] [CrossRef] [PubMed]
- Li, M.; Yao, J. All-fiber temporal photonic fractional Hilbert transformer based on a directly designed fiber Bragg grating. Opt. Lett. 2010, 35, 223–225. [Google Scholar] [CrossRef]
- Ge, J.; Wang, C.; Zhu, X. Fractional optical Hilbert transform using phase shifted fiber Bragg gratings. Opt. Commun. 2011, 284, 3251–3257. [Google Scholar] [CrossRef]
- Preciado, M.A.; Shu, X.; Harper, P.; Sugden, K. Experimental demonstration of an optical differentiator based on a fiber Bragg grating in transmission. Opt. Lett. 2013, 38, 917–919. [Google Scholar] [CrossRef][Green Version]
- Liu, X.; Shu, X.; Cao, H. Proposal of a Phase-shift fiber Bragg grating as an optical differentiator and an optical integrator simultaneously. IEEE Photon. J. 2018, 10, 7800907. [Google Scholar] [CrossRef]
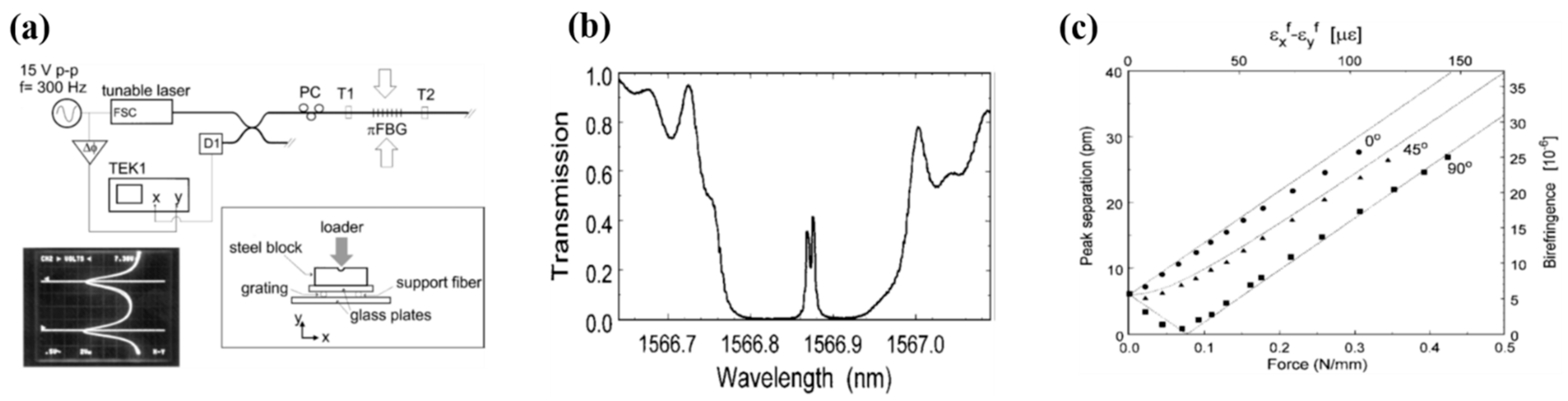
- LeBlanc, M.; Vohra, S.T.; Tsai, T.E.; Friebele, E.J. Transverse load sensing by use of pi-phase-shifted fiber Bragg gratings. Opt. Lett. 1999, 24, 1091–1093. [Google Scholar] [CrossRef]
- Fu, H.; Shu, X.; Mou, C.; Zhang, L.; He, S.; Bennion, I. Transversal loading sensor based on tunable beat frequency of a dual-wavelength fiber laser. IEEE Photon. Technol. Lett. 2009, 21, 987–989. [Google Scholar]
- Wang, Y.; Li, N.; Huang, X.; Wang, M. Fiber optic transverse load sensor based on polarization properties of phase-shifted fiber Bragg grating. Opt. Commun. 2015, 342, 152–156. [Google Scholar]
- Gatti, D.; Galzerano, G.; Janner, D.; Longhi, S.; Laporta, P. Fiber strain sensor based on a π-phase-shifted Bragg grating and the Pound–Drever–Hall technique. Opt. Exp. 2008, 16, 1945–1950. [Google Scholar] [CrossRef]
- Ghosh, B.; Mandal, S. Mathematical modeling of π-phase-shifted fiber Bragg grating and its application for strain measurement in epoxy resin cantilever beam. IEEE Sens. J. 2020, 20, 9856–9863. [Google Scholar] [CrossRef]
- Ouyang, Y.; Liu, J.; Xu, X.; Zhao, Y.; Zhou, A. Phase-shifted eccentric core fiber Bragg grating fabricated by electric arc discharge for directional bending measurement. Sensors 2018, 18, 1168. [Google Scholar] [CrossRef]
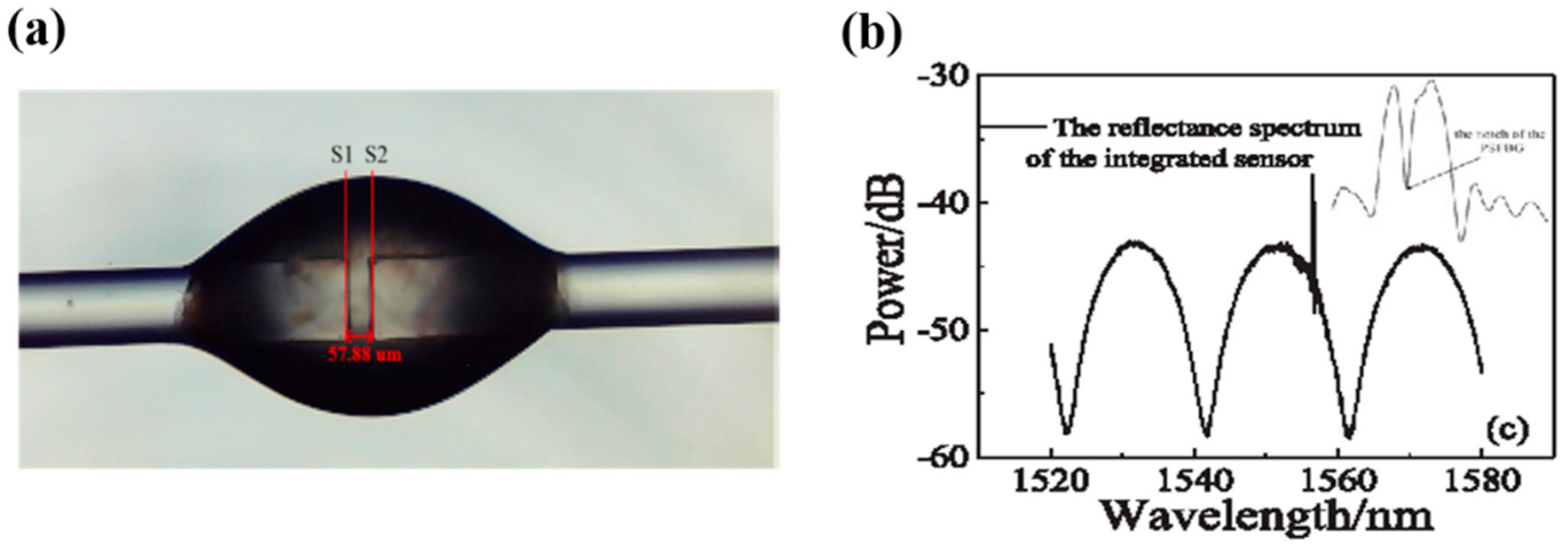
- Zhang, Q.; Liu, N.; Fink, T.; Li, H.; Peng, W.; Han, M. Fiber-optic pressure sensor based on π-phase-shifted fiber Bragg grating on side-hole fiber. IEEE Photon. Technol. Lett. 2012, 24, 1519–1522. [Google Scholar] [CrossRef]
- Yang, D.; Liu, Y.; Wang, Y.; Zhang, T.; Shao, M.; Yu, D.; Fu, H.; Jia, Z. Integrated optic-fiber sensor based on enclosed EFPI and structural phase-shift for discriminating measurement of temperature, pressure and RI. Opt. Laser Technol. 2020, 126, 106112. [Google Scholar] [CrossRef]
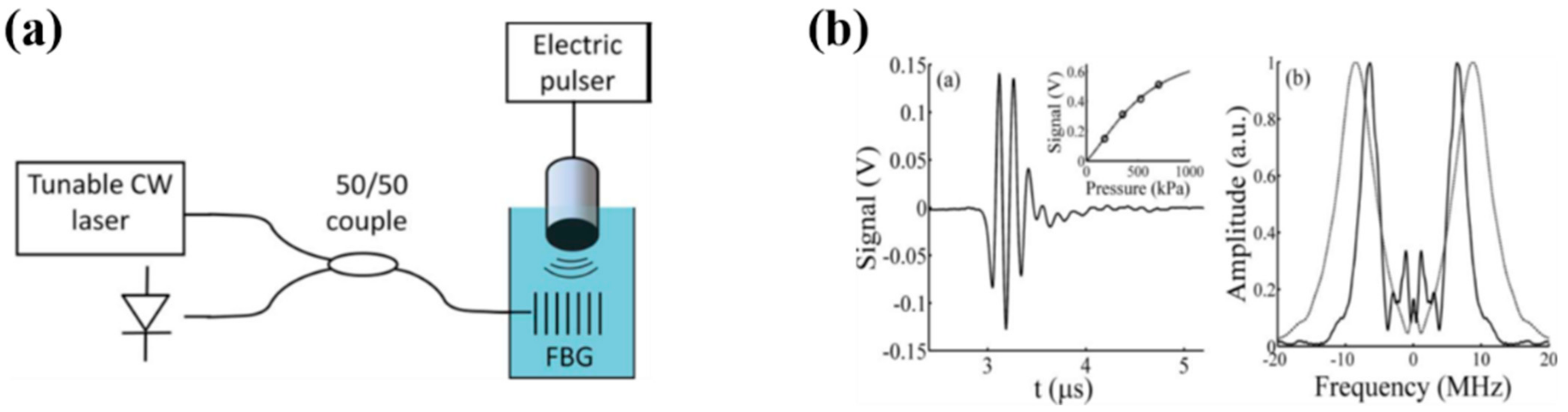
- Rosenthal, A.; Razansky, D.; Ntziachristos, V. High-sensitivity compact ultrasonic detector based on a pi-phase-shifted fiber Bragg grating. Opt. Lett. 2011, 36, 1833–1835. [Google Scholar] [CrossRef]
- Wu, Q.; Okabe, Y. Novel real-time acousto-ultrasonic sensors using two phase-shifted fiber Bragg gratings. J. Intell. Mater. Syst. Struct. 2014, 25, 640–646. [Google Scholar] [CrossRef]
- Guo, J.; Yang, C. Highly stabilized phase-shifted fiber Bragg grating sensing system for ultrasonic detection. IEEE Photon. Technol. Lett. 2015, 27, 848–851. [Google Scholar] [CrossRef]
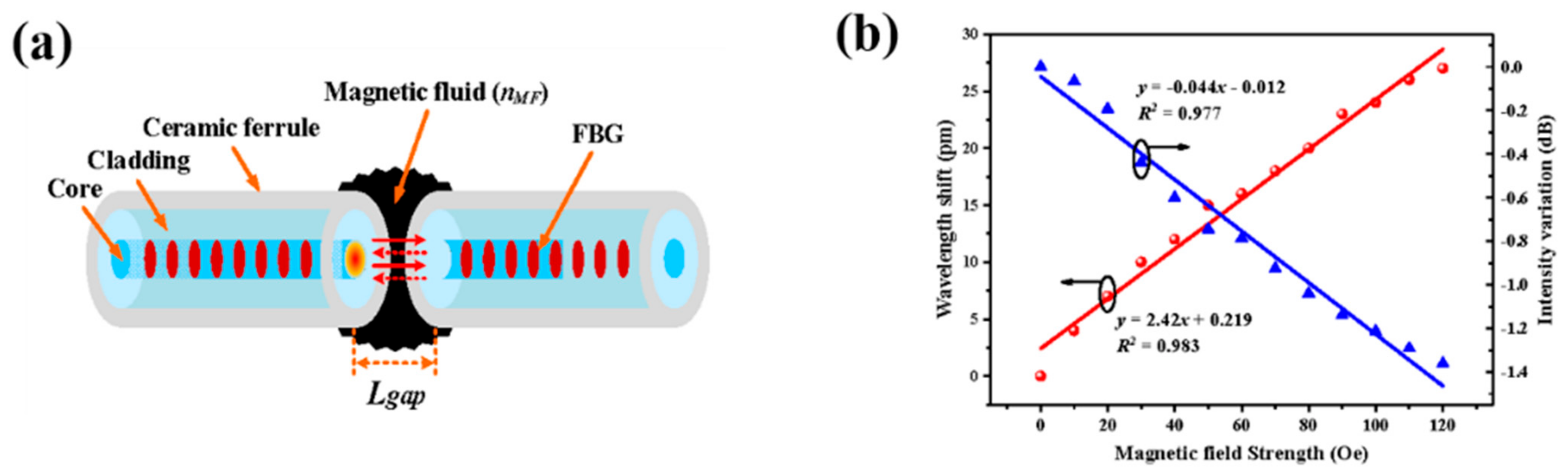
- Bao, L.; Dong, X.; Zhang, S.; Shen, C.; Shum, P. Magnetic field sensor based on magnetic fluid-infiltrated phase-shifted fiber Bragg grating. IEEE Sens. J. 2018, 18, 4008–4012. [Google Scholar] [CrossRef]
- Jackson, S.D.; Sabella, A.; Lancaster, D.G. Application and development of high-power and highly efficient silica-based fiber lasers operating at 2 μm. IEEE J. Sel. Top. Quantum Electron. 2007, 13, 567–572. [Google Scholar] [CrossRef]
- Zhang, L.; Yan, F.; Bai, Z.; Bai, Y.; Liu, S.; Zhou, H.; Hou, Y.; Zhang, N. Research on transmission characteristics of phase-shifted CFBG in 2 μm band. Opt. Fiber Technol. 2017, 36, 428–437. [Google Scholar] [CrossRef]
- Zhang, L.; Yan, F.; Han, W.; Bai, Z.; Cheng, D.; Zhou, H.; Suo, Y.; Feng, T. Transmission characteristics of sampled fiber Bragg grating and phase-shifted sampled fiber Bragg grating in the 2 μm band. Opt. Fiber Technol. 2019, 50, 263–270. [Google Scholar] [CrossRef]
- Jayaraman, V.; Chuang, Z.M.; Coldren, L.A. Theory, design, and performance of extended tuning range semiconductor lasers with sampled gratings. IEEE J. Quantum Electron. 1993, 29, 1824–1834. [Google Scholar] [CrossRef]
- Ouellette, F.; Krug, P.A.; Stephens, T.; Dhosi, G.; Eggleton, B. Broadband and WDM dispersion compensation using chirped sampled fibre Bragg gratings. Electron. Lett. 1995, 31, 899–901. [Google Scholar] [CrossRef]
- Ibsen, M.; Durkin, M.K.; Cole, M.J.; Laming, R.I. Sinc-sampled fiber Bragg gratings for identical multiple wavelength operation. IEEE Photon. Technol. Lett. 1998, 10, 842–844. [Google Scholar] [CrossRef]
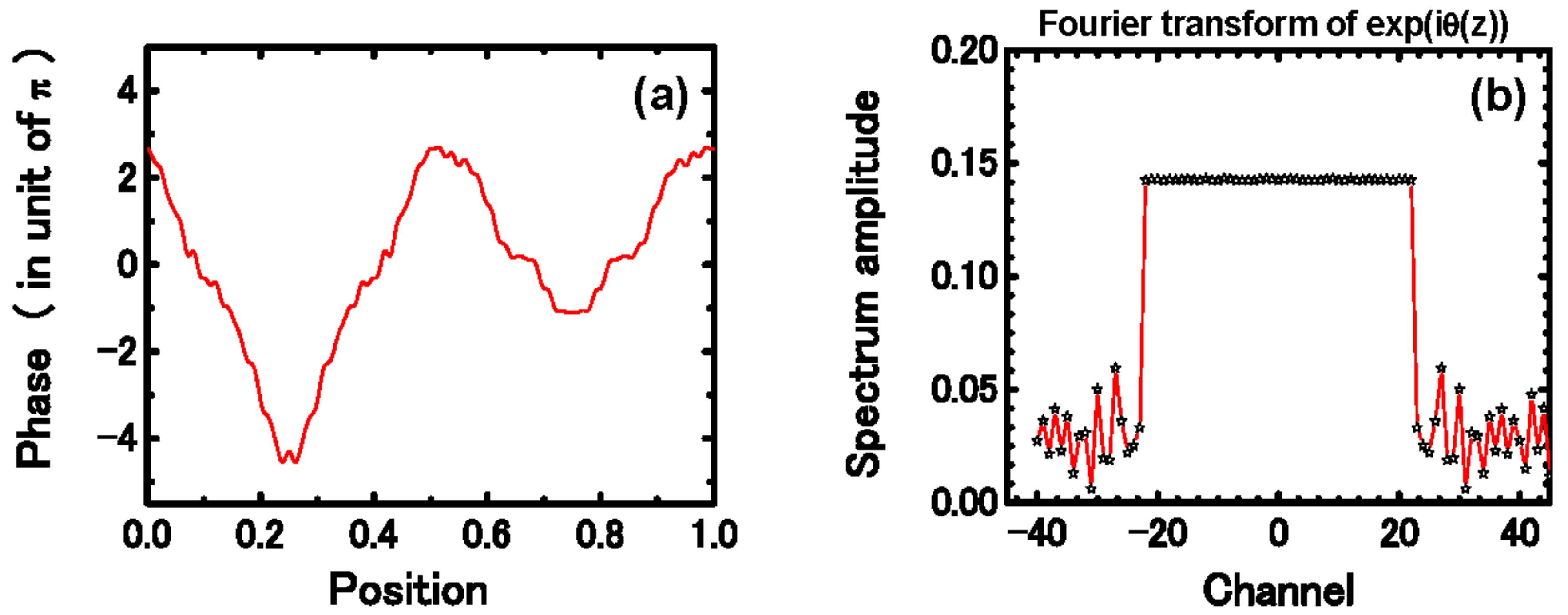
- Rothenberg, J.E.; Li, H.; Li, Y.; Popelek, J.; Sheng, Y.; Wang, Y.; Wilcox, R.B.; Zweiback, J. Dammann fiber Bragg gratings and phase-only sampling for high channel counts. IEEE Photon. Technol. Lett. 2002, 14, 1309–1311. [Google Scholar] [CrossRef]
- Li, H.; Sheng, Y.; Li, Y.; Rothenberg, J.E. Phased-only sampled fiber Bragg gratings for high-channel-count chromatic dispersion compensation. J. Lightw. Technol. 2003, 21, 2074–2083. [Google Scholar]
- Lee, H.; Agrawal, G.P. Purely phase-sampled fiber Bragg gratings for broad-band dispersion and dispersion slope compensation. IEEE Photon. Technol. Lett. 2003, 15, 1091–1093. [Google Scholar]
- Lee, H.; Agrawal, G.P. Bandwidth equalization of purely phase-sampled fiber Bragg gratings for broadband dispersion and dispersion slope compensation. Opt. Exp. 2004, 12, 5595–5602. [Google Scholar] [CrossRef]
- Nasu, Y.; Yamashita, S. Densification of sampled fiber Bragg gratings using multiple-phase-shift (MPS) technique. J. Lightw. Technol. 2005, 23, 1808–1817. [Google Scholar] [CrossRef]
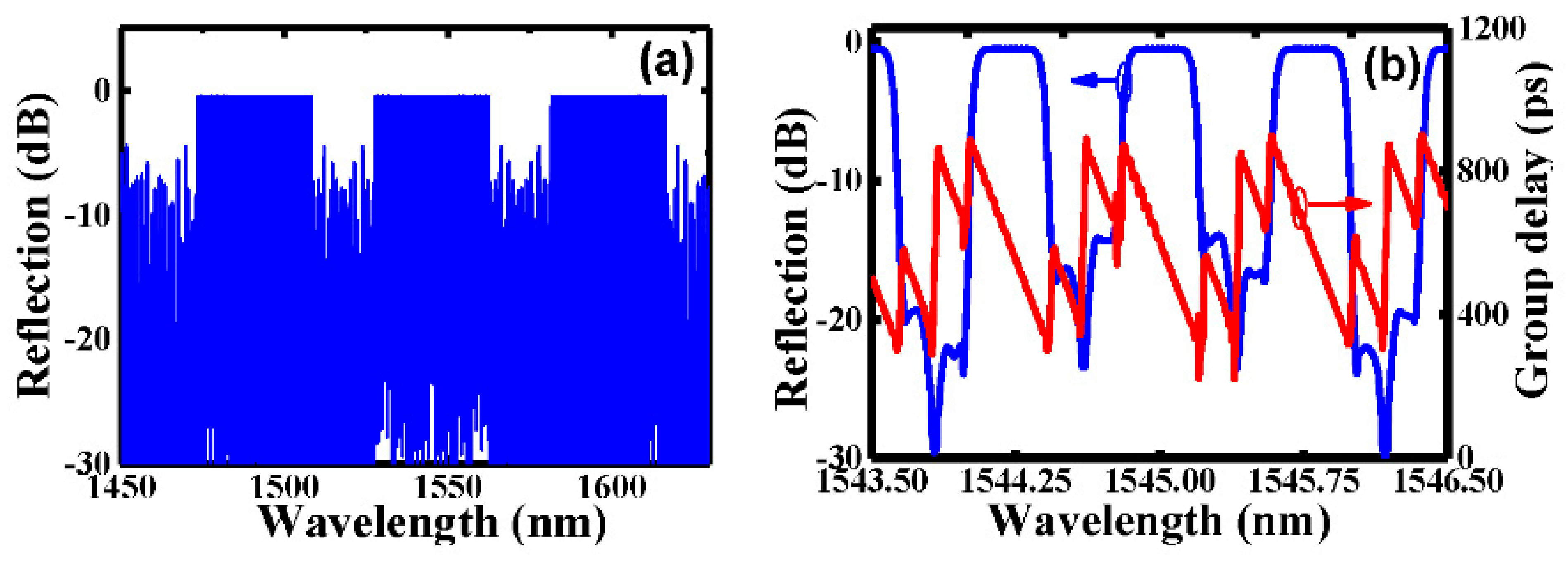
- Rothenberg, J.E.; Li, H.; Sheng, Y.; Popelek, J.; Zweiback, J. Phase-only sampled 45 channel fiber Bragg grating written with a diffraction-compensated phase mask. Opt. Lett. 2006, 31, 1199–1201. [Google Scholar] [CrossRef] [PubMed]
- Li, H.; Li, M.; Ogusu, K.; Sheng, Y.; Rothenberg, J.E. Optimization of a continuous phase-only sampling for high channel-count fiber Bragg gratings. Opt. Exp. 2006, 14, 3152–3160. [Google Scholar] [CrossRef] [PubMed]
- Dai, Y.; Chen, X.; Sun, J.; Xie, S. Wideband multichannel dispersion compensation based on a strongly chirped sampled Bragg grating and phase shifts. Opt. Lett. 2006, 31, 311–313. [Google Scholar] [CrossRef] [PubMed]
- Li, H.; Li, M.; Sheng, Y.; Rothenberg, J.E. Advances in the design and fabrication of high-channel-count fiber Bragg gratings. J. Lightw. Technol. 2007, 25, 2739–2750. [Google Scholar] [CrossRef]
- Li, M.; Li, H. Reflection equalization of the simultaneous dispersion and dispersion-slope compensator based on a phase-only sampled fiber Bragg grating. Opt. Exp. 2008, 16, 9821–9828. [Google Scholar] [CrossRef] [PubMed]
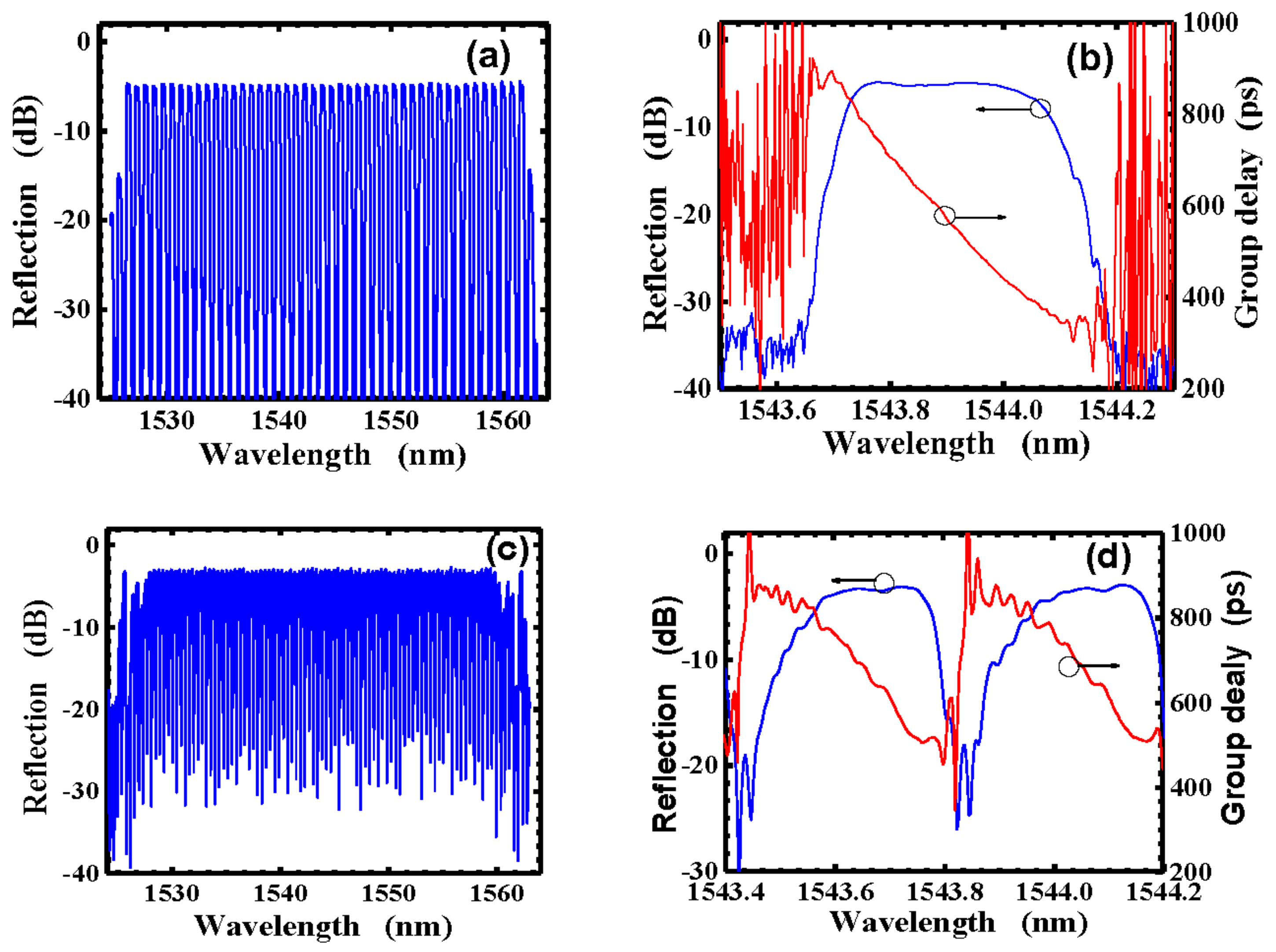
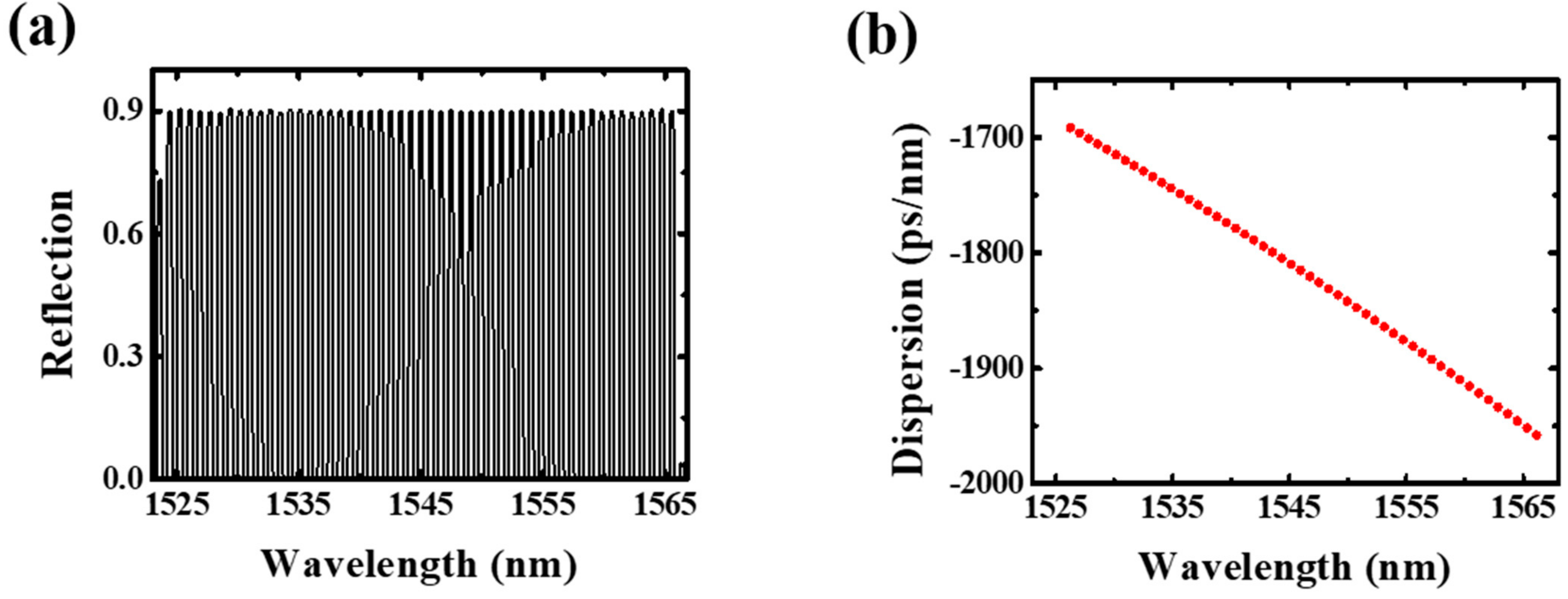
- Li, H.; Li, M.; Hayashi, J. Ultrahigh-channel-count phase-only sampled fiber Bragg grating covering the S, C, and L bands. Opt. Lett. 2009, 34, 938–940. [Google Scholar] [CrossRef]
- Li, M.; Chen, X.; Hayashi, J.; Li, H. Advanced design of the ultrahigh-channel-count fiber Bragg grating based on the double sampling method. Opt. Exp. 2009, 17, 8382–8394. [Google Scholar] [CrossRef]
- Chen, X.; Hayashi, J.; Li, H. Ultrahigh-channel-count fiber Bragg grating based on the triple sampling method. Opt. Commun. 2011, 284, 1842–1846. [Google Scholar] [CrossRef]
- Zhao, J.; Yu, Y.; Tang, Y. Design of high channel-count comb filter based on digital concatenated grating with multiple phase shifts (MPS-DCG). IEEE Photon. Technol. Lett. 2011, 23, 1814–1816. [Google Scholar] [CrossRef]
- Sheng, Y.; Rothenberg, J.E.; Li, H.; Wang, Y.; Zweiback, J. Split of phase shifts in a phase mask for fiber Bragg gratings. IEEE Photon. Technol. Lett. 2004, 16, 1316–1318. [Google Scholar] [CrossRef]
- Sheng, Y.; Sun, L. Near-field diffraction of irregular phase gratings with multiple phase-shifts. Opt. Exp. 2005, 13, 6111–6116. [Google Scholar] [CrossRef]
- Li, M.; Chen, X.; Fujii, T.; Kudo, Y.; Li, H.; Painchaud, Y. Multiwavelength fiber laser based on the utilization of a phase-shifted phase-only sampled fiber Bragg grating. Opt. Lett. 2009, 34, 1717–1719. [Google Scholar] [CrossRef]
- Cai, J.X.; Feng, K.M.; Willner, A.E.; Grubsky, V.; Starodubov, D.S.; Feinberg, J. Simultaneous tunable dispersion compensation of many WDM channels using a sampled nonlinearly chirped fiber Bragg grating. IEEE Photon. Technol. Lett. 1999, 11, 1455–1457. [Google Scholar] [CrossRef]
- Lee, H.; Agrawal, G.P. Add–drop multiplexers and interleavers with broad-band chromatic dispersion compensation based on purely phase-sampled fiber gratings. IEEE Photon. Technol. Lett. 2004, 16, 635–637. [Google Scholar] [CrossRef]
- Li, M.; Yao, J. Multichannel arbitrary-order photonic temporal differentiator for wavelength-division-multiplexed signal processing using a single fiber Bragg grating. J. Lightw. Technol. 2011, 29, 2506–2511. [Google Scholar] [CrossRef]
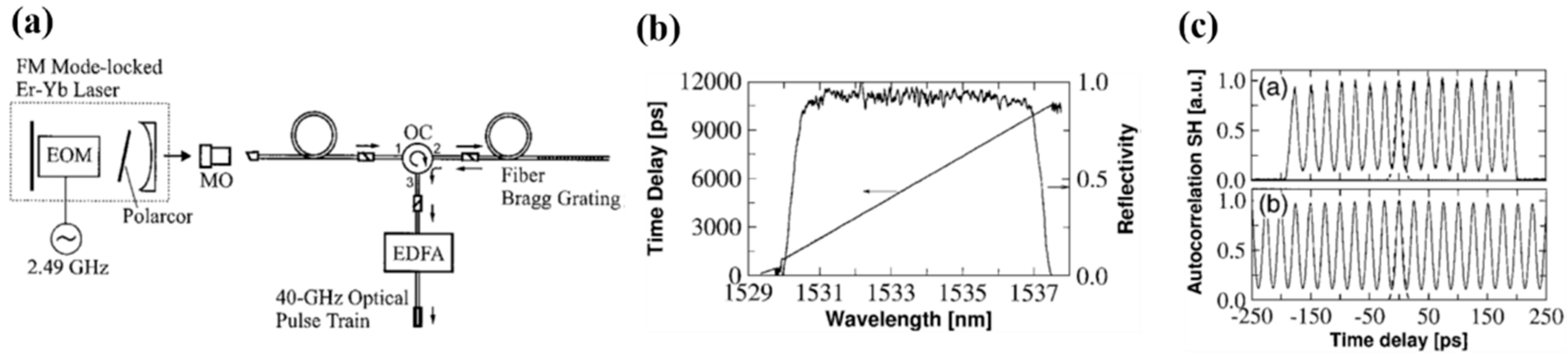
- Petropoulos, P.; Ibsen, M.; Zervas, M.N.; Richardson, D.J. Generation of a 40-GHz pulse stream by pulse multiplication with a sampled fiber Bragg grating. Opt. Lett. 2000, 25, 521–523. [Google Scholar] [CrossRef]
- Longhi, S.; Marano, M.; Laporta, P.; Svelto, O.; Belmonte, M.; Agogliati, B.; Arcangeli, L.; Pruneri, V.; Zervas, M.N.; Ibsen, M. 40-GHz pulse-train generation at 1.5 μm with a chirped fiber grating as a frequency multiplier. Opt. Lett. 2000, 25, 1481–1483. [Google Scholar] [CrossRef]
- Azaña, J.; Slavík, R.; Kockaert, P.; Chen, L.R.; LaRochelle, S. Generation of customized ultrahigh repetition rate pulse sequences using superimposed fiber Bragg gratings. J. Lightw. Technol. 2003, 21, 1490–1498. [Google Scholar] [CrossRef]
- Chen, X.; Li, H. Simultaneous optical pulse multiplication and shaping based on the amplitude-assisted phase-only filter utilizing a fiber Bragg grating. J. Lightw. Technol. 2009, 27, 5246–5252. [Google Scholar] [CrossRef]
- Wakabayashi, S.; Baba, A.; Itou, A.; Adachi, J. Design and fabrication of an apodization profile in linearly chirped fiber Bragg gratings for wideband >35 nm and compact tunable dispersion compensator. J. Opt. Soc. Am. B 2008, 25, 210–217. [Google Scholar] [CrossRef]
- Liu, X.; Shu, X. Design of arbitrary-order photonic temporal differentiators based on phase-modulated fiber Bragg gratings in transmission. J. Lightw. Technol. 2017, 35, 2926–2932. [Google Scholar] [CrossRef]
- Li, Y.; Liu, X.; Shu, X.; Zhang, L. Arbitrary-order photonic Hilbert transformers based on phase-modulated fiber Bragg gratings in transmission. Photonics. 2021, 8, 27. [Google Scholar] [CrossRef]
- Liu, X.; Xu, Z.; Preciado, M.A.; Gbadebo, A.; Zhang, L.; Xiong, J.; Yu, Y.; Cao, H.; Shu, X. Transmissive fiber Bragg grating-based delay line interferometer for RZ-OOK to NRZ-OOK format conversion. IEEE Access 2019, 7, 140300–140304. [Google Scholar] [CrossRef]
- Wang, C.; Yao, J. Photonic generation of chirped millimeter-wave pulses based on nonlinear frequency-to-time mapping in a nonlinearly chirped fiber Bragg grating. IEEE Trans. Microw. Theory Tech. 2008, 56, 542–552. [Google Scholar] [CrossRef]
- Tosi, D. Review of chirped fiber Bragg grating (CFBG) fiber-optic sensors and their applications. Sensors 2018, 18, 2147. [Google Scholar] [CrossRef]
- Vengsarkar, A.M.; Lemaire, P.J.; Judkins, J.B.; Bhatia, V.; Erdogan, T.; Sipe, J.E. Long-period fiber gratings as band rejection filters. J. Lightw. Technol. 1996, 14, 58–65. [Google Scholar] [CrossRef]
- Bakhti, F.; Sansonetti, P. Realization of low back-reflection, wideband fiber bandpass filters using phase-shifted long-period gratings. In Proceedings of the Optical Fiber Communication (OFC’97) Conference, Dallas, TX, USA, 16–21 February 1997. [Google Scholar]
- Ke, H.; Chiang, K.S.; Peng, J. Analysis of phase-shifted long-period fiber gratings. IEEE Photon. Technol. Lett. 1998, 10, 1596–1598. [Google Scholar] [CrossRef]
- Liu, Y.; Williams, J.A.R.; Zhang, L.; Bennion, I. Phase shifted and cascaded long-period fiber gratings. Opt. Commun. 1999, 164, 27–31. [Google Scholar] [CrossRef]
- Chen, L.R. Design of flat-top bandpass filter based on symmetric multiple phase-shifted long-period fiber gratings. Opt. Commun. 2002, 205, 271–276. [Google Scholar] [CrossRef]
- Zhang, J.; Shum, P.; Li, S.; Ngo, N.; Cheng, X.; Ng, J. Design and fabrication of flat-band long-period grating. IEEE Photon. Technol. Lett. 2003, 15, 1558–1560. [Google Scholar] [CrossRef]
- Chen, L.R. Phase-shifted long-period gratings by refractive index-shifting. Opt. Commun. 2001, 200, 187–191. [Google Scholar] [CrossRef]
- James, S.W.; Topliss, S.M.; Tatam, R.P. Properties of length-apodized phase-shifted LPGs operating at the phase matching turning point. J. Lightw. Technol. 2012, 30, 2203–2209. [Google Scholar] [CrossRef]
- Chen, H.; Gu, Z. Filtering characteristics of film-coated long-period fiber gratings operating at the phase-matching turning point. Optik 2014, 125, 6003–6009. [Google Scholar] [CrossRef]
- Zhu, Y.; Shum, P.; Lu, C.; Lacquet, B.; Swart, P.L.; Spammer, S.J. EDFA gain flattening using phase-shifted long-period grating. Microw. Opt. Technol. Lett. 2003, 37, 153–157. [Google Scholar] [CrossRef]
- Han, Y.G.; Lee, J.H.; Lee, S.B. Discrimination of bending and temperature sensitivities with phase-shifted long-period fiber gratings depending on initial coupling strength. Opt. Exp. 2004, 12, 3204–3208. [Google Scholar] [CrossRef]
- Chung, K.W.; Yin, S. Design of a phase-shifted long-period grating using the partial-etching technique. Microw. Opt. Techn. Let. 2005, 45, 18–21. [Google Scholar] [CrossRef]
- Del Villar, I.; Arregui, F.J.; Matias, I.R.; Cusano, A.; Paladino, D.; Cutolo, A. Fringe generation with nonuniformly coated long-period fiber gratings. Opt. Exp. 2007, 15, 9326–9340. [Google Scholar] [CrossRef]
- Li, X.; Zhang, W.; Chen, L.; Yan, T. Temperature-independent force sensor based on PSLPFG induced by electric-arc discharge. IEEE Photon. Technol. Lett. 2015, 27, 1946–1948. [Google Scholar] [CrossRef]
- Yang, W.; Geng, T.; Yang, J.; Zhou, A.; Liu, Z.; Geng, S.; Yuan, L. A phase-shifted long period fiber grating based on filament heating method for simultaneous measurement of strain and temperature. J. Opt. 2015, 17, 75801. [Google Scholar] [CrossRef]
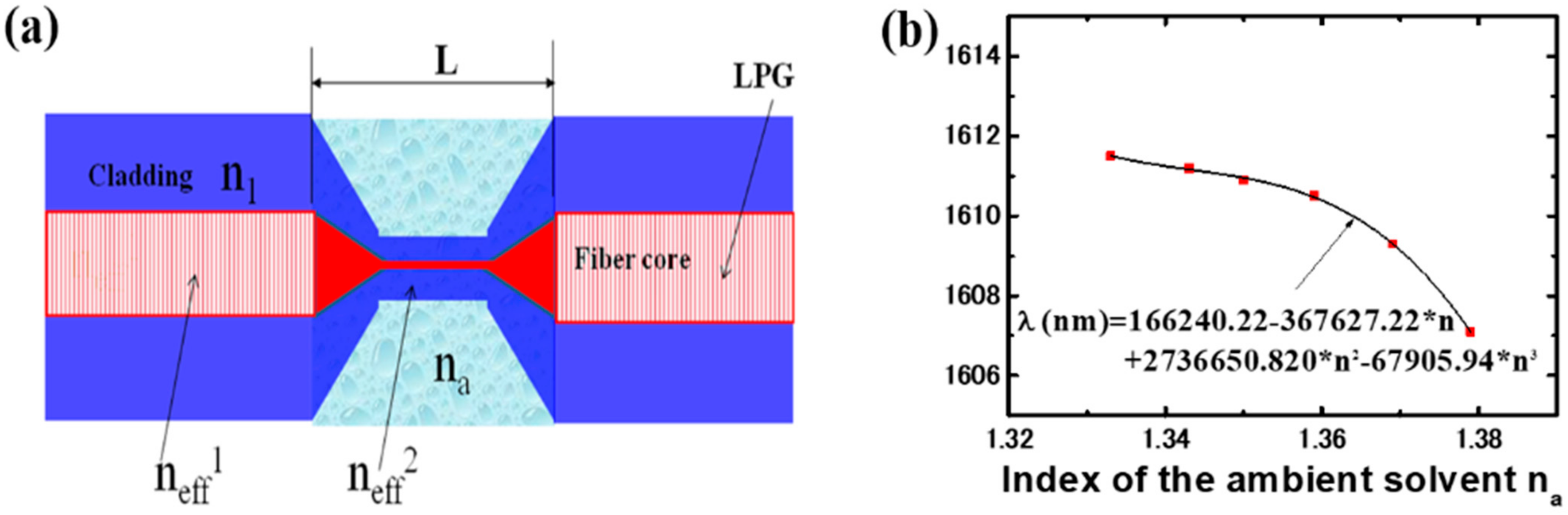
- Hishiki, K.; Li, H. Phase-shift formed in a long period fiber grating and its application to the measurements of temperature and refractive index. Opt. Exp. 2013, 21, 11901–11912. [Google Scholar] [CrossRef]
- Zhou, X.; Shi, S.; Zhang, Z.; Zou, J.; Liu, Y. Mechanically-induced π-shifted long-period fiber gratings. Opt. Exp. 2011, 19, 6253–6259. [Google Scholar] [CrossRef]
- Humbert, G.; Malki, A. High performance bandpass filters based on electric arc-induced π-shifted long-period fibre gratings. Electron. Lett. 2003, 39, 1506–1507. [Google Scholar] [CrossRef]
- Falate, R.; Frazão, O.; Rego, G.; Fabris, J.L.; Santos, J.L. Refractometric sensor based on a phase-shifted long-period fiber grating. Appl. Opt. 2006, 45, 5066–5072. [Google Scholar] [CrossRef]
- Zhu, Y.; Shum, P.; Chen, X.; Tan, C.H.; Lu, C. Resonance-temperature-insensitive phase-shifted long period fiber gratings induced by surface deformation with anomalous strain characteristics. Opt. Lett. 2005, 30, 1788–1790. [Google Scholar] [CrossRef]
- Gu, Y.; Chiang, K.S.; Rao, Y.J. Writing of apodized phase-shifted long-period fiber gratings with a computer-controlled CO2 laser. IEEE Photon. Technol. Lett. 2009, 21, 657–659. [Google Scholar]
- Cheng, B.; Lan, X.; Huang, J.; Fang, X.; Xiao, H. Flexible fabrication of long period fiber grating devices based on erasing effect by controlled CO2 laser pulse exposure. Microw. Opt. Technol. Lett. 2013, 55, 1735–1738. [Google Scholar] [CrossRef]
- Zheng, S.; Lei, X.; Zhu, Y. Temperature-insensitive compact phase-shifted long-period gratings induced by surface deformation in single-mode fiber. Appl. Phys. B 2015, 121, 259–263. [Google Scholar] [CrossRef]
- Wang, P.; Xian, L.; Li, H. Fabrication of phase-shifted long-period fiber grating and its application to strain measurement. IEEE Photon. Technol. Lett. 2015, 27, 557–560. [Google Scholar] [CrossRef]
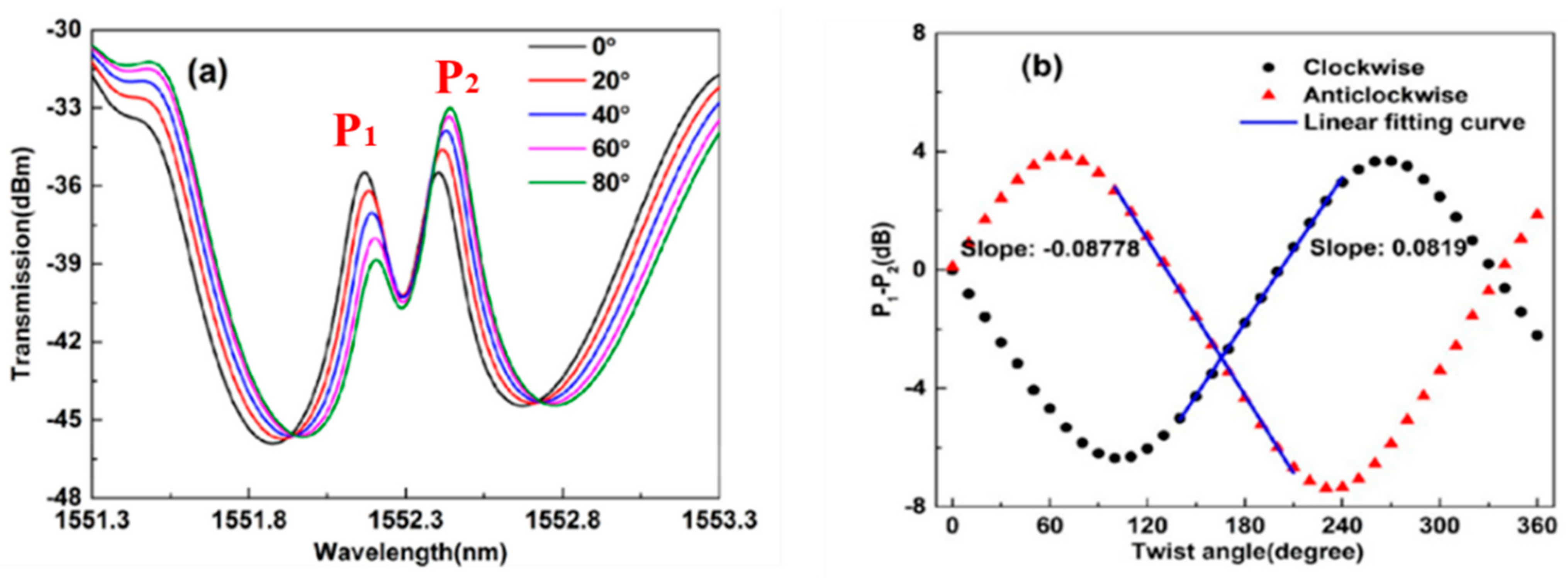
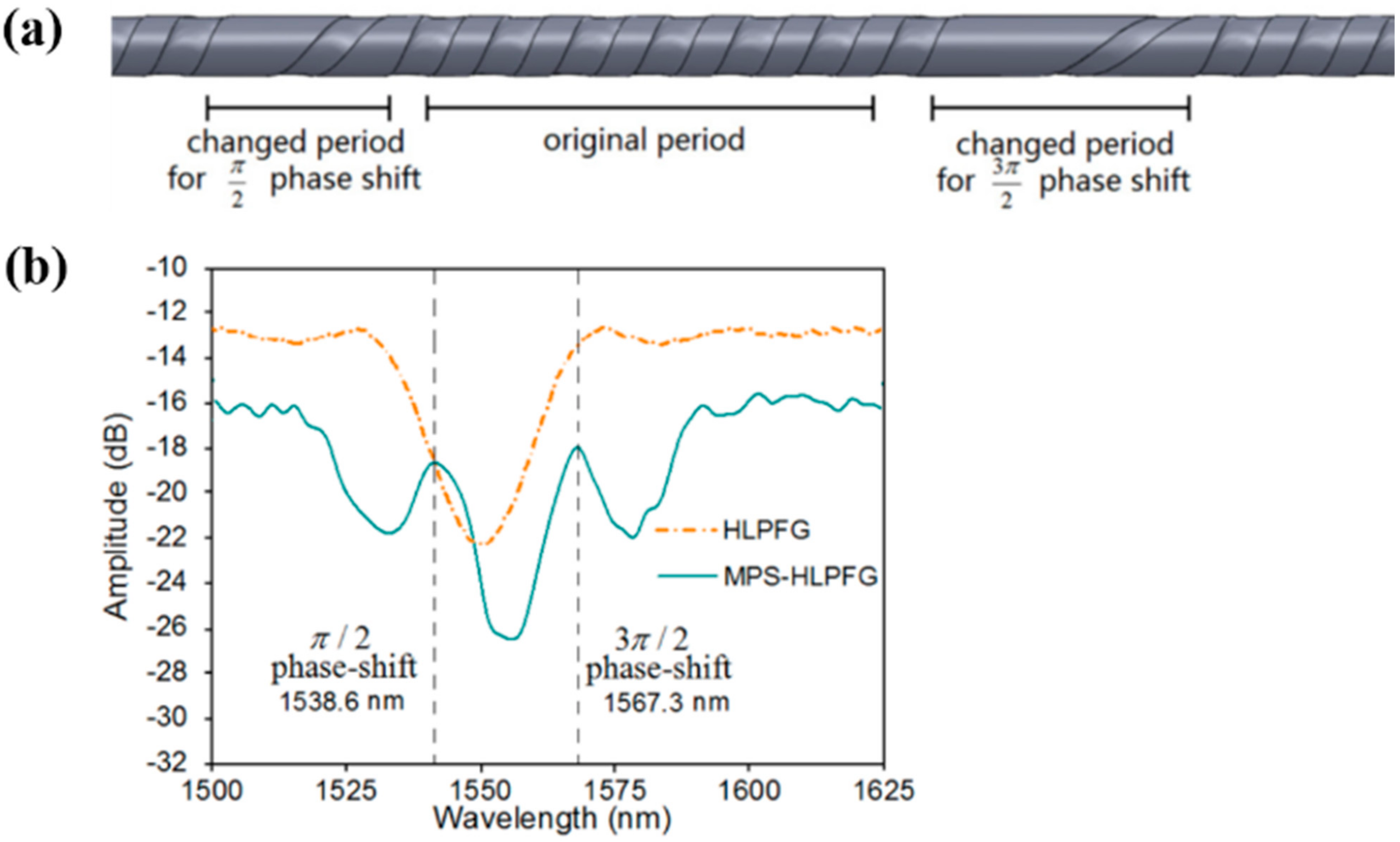
- Gao, R.; Jiang, Y.; Jiang, L. Multi-phase-shifted helical long period fiber grating based temperature-insensitive optical twist sensor. Opt. Exp. 2014, 22, 15697–15709. [Google Scholar] [CrossRef]
- Zheng, S.; Zhu, Y. Photonic crystal fiber π-phase-shifted long-period gratings with wide bandpass and temperature insensitivity. Opt. Eng. 2015, 54, 116101. [Google Scholar] [CrossRef]
- Inoue, G.; Wang, P.; Li, H. Flat-top band-rejection filter based on two successively-cascaded helical fiber gratings. Opt. Exp. 2016, 24, 5442–5447. [Google Scholar] [CrossRef]
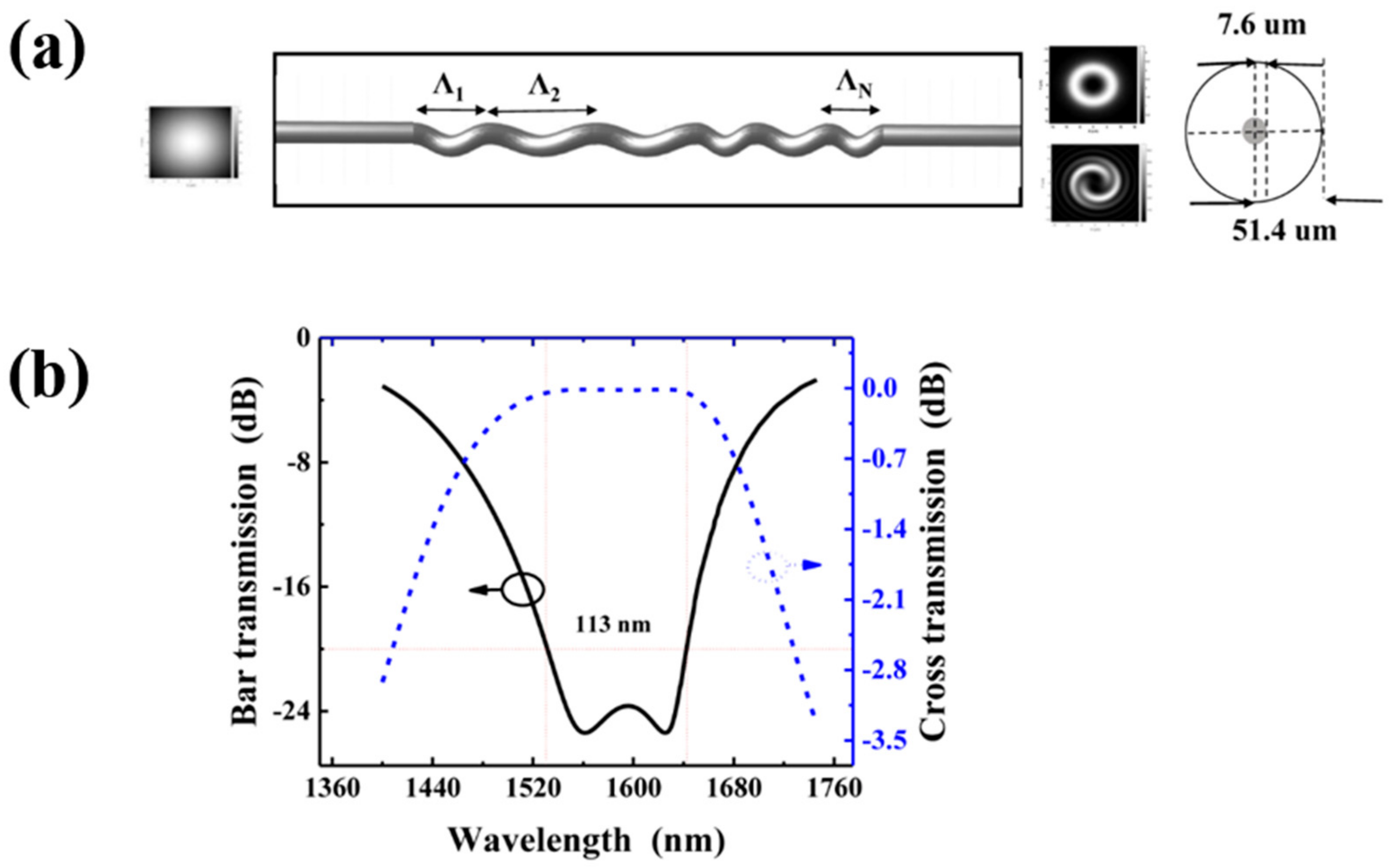
- Zhao, H.; Li, H. Advances on mode-coupling theories, fabrication techniques, and applications of the helical long-period fiber gratings: A review. Photonics 2021, 8, 8040106. [Google Scholar] [CrossRef]
- Xian, L.; Wang, P.; Li, H. Power-interrogated and simultaneous measurement of temperature and torsion using paired helical long period fiber gratings with opposite helicities. Opt. Exp. 2014, 22, 20260–20267. [Google Scholar] [CrossRef]
- Wang, P.; Subramanian, R.; Zhu, C.; Zhao, H.; Li, H. Phase-shifted helical long-period fiber grating and its characterization by using the microscopic imaging method. Opt. Exp. 2017, 25, 7402–7407. [Google Scholar] [CrossRef]
- Qian, J.R.; Chen, H.F. Gain flattening fiber filters using phase-shifted long period fiber gratings. Electron. Lett. 1998, 34, 1132–1133. [Google Scholar] [CrossRef]
- Harumoto, M.; Shigehara, M.; Suganuma, H. Gain-flattening filter using long-period fiber Gratings. J. Lightw. Technol. 2002, 20, 1027–1033. [Google Scholar] [CrossRef]
- Azana, J.; Kulishov, M. All-fibre ultrafast optical differentiator based on π phase-shifted long-period grating. Electron. Lett. 2005, 41, 1368–1369. [Google Scholar] [CrossRef]
- Kulishov, M.; Krcmarik, D.; Slavik, R. Design of terahertz-bandwidth arbitrary-order temporal differentiators based on long-period fiber gratings. Opt. Lett. 2007, 32, 2978–2980. [Google Scholar] [CrossRef]
- Ashrafi, R.; Li, M.; Azana, J. Coupling-strength-independent long-period grating designs for THz-bandwidth optical differentiators. IEEE Photon. J. 2013, 5, 7100311. [Google Scholar] [CrossRef]
- Liu, S.X.; Wang, C.H.; Zhu, X.J.; Bu, C.X.; Zhang, G.J. Arbitrarily switchable multi-wavelength Yb-doped fiber lasers with phase-shifted long-period fiber grating. Laser Phys. 2012, 22, 1260–1264. [Google Scholar] [CrossRef]
- Jiao, K.; Shen, H.; Yang, F.; Wu, X.; Bian, Y.; Zhu, R. Optimizing output spectral linewidth of fiber laser utilizing phase-shifted long-period fiber grating. Opt. Laser Technol. 2021, 142, 107221. [Google Scholar] [CrossRef]
- Wu, X.; Gao, S.; Tu, J.; Shen, L.; Hao, C.; Zhang, B.; Feng, Y.; Zhou, J.; Chen, S.; Liu, W.; et al. Multiple orbital angular momentum mode switching at multi-wavelength in few-mode fibers. Opt. Exp. 2020, 28, 36084–36094. [Google Scholar] [CrossRef] [PubMed]
- Sun, C.; Wang, R.; Jin, X.; Wang, Z.; Liu, W.; Zhang, S.; Ma, Y.; Lin, J.; Li, Y.; Geng, T.; et al. A new phase-shifted long-period fiber grating for simultaneous measurement of torsion and temperature. Chin. Opt. Lett. 2020, 18, 21203. [Google Scholar] [CrossRef]
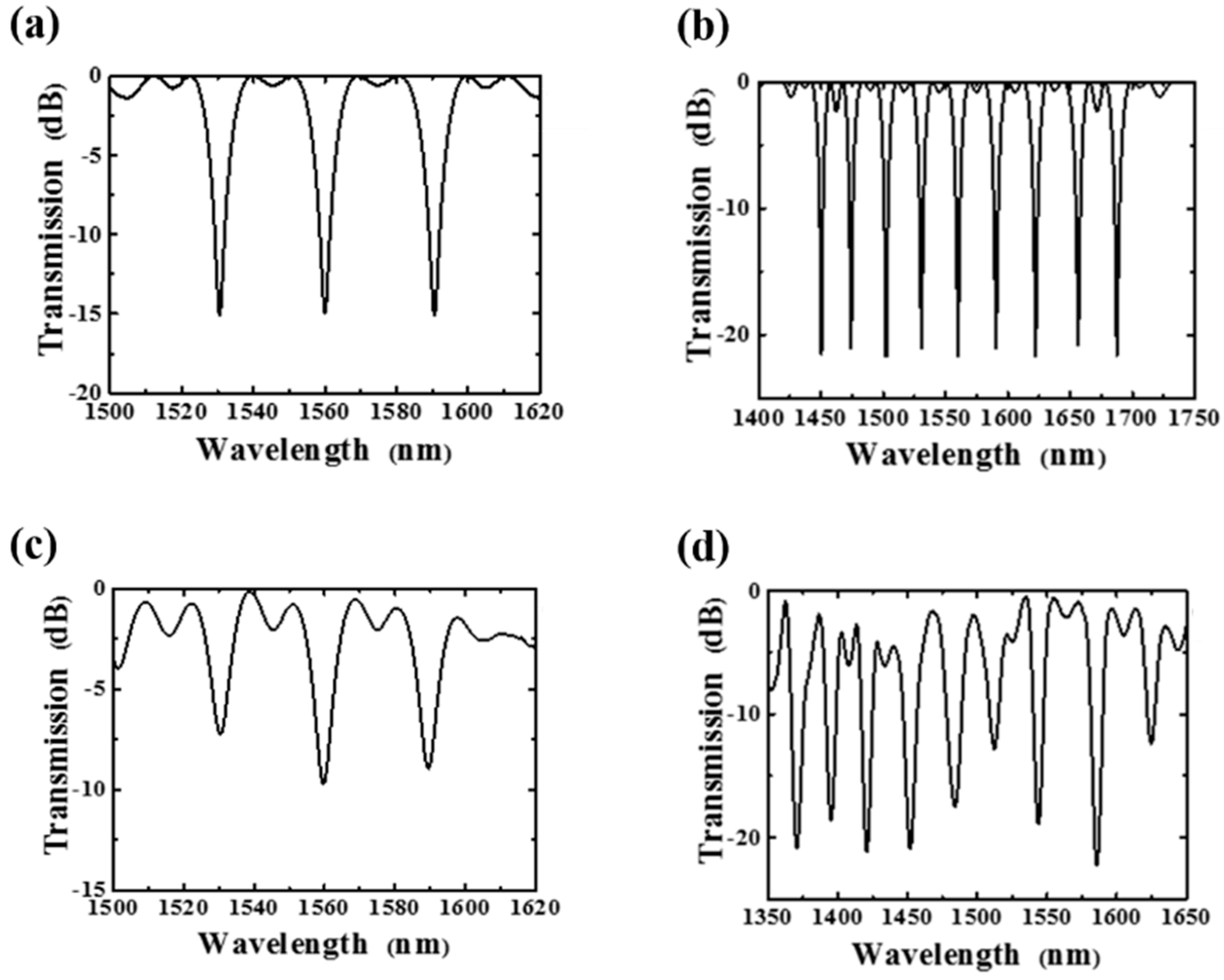
- Zhu, C.; Ishikami, S.; Wang, P.; Zhao, H.; Li, H. Optimal design and fabrication of multichannel helical long-period fiber gratings based on phase-only sampling method. Opt. Exp. 2019, 27, 2281–2291. [Google Scholar] [CrossRef]
- Zhu, C.; Ishikami, S.; Zhao, H.; Li, H. Multichannel long-period fiber grating realized by using the helical sampling approach. J. Lightw. Technol. 2019, 37, 2008–2013. [Google Scholar] [CrossRef]
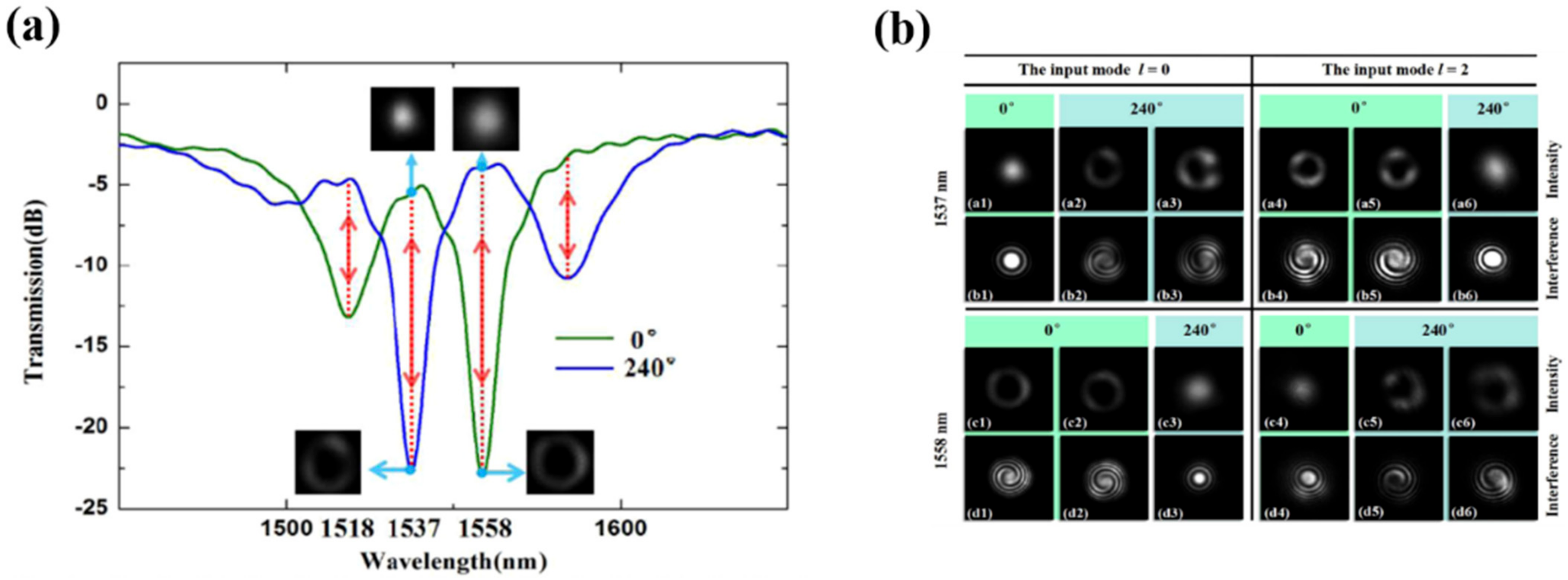
- Zhu, C.; Wang, P.; Zhao, H.; Mizushima, R.; Ishikami, S.; Li, H. DC-sampled helical fiber grating and its application to multi-channel OAM generator. IEEE Photon. Technol. Lett. 2019, 31, 1445–1448. [Google Scholar] [CrossRef]
- Mizushima, R.; Detani, T.; Zhu, C.; Wang, P.; Zhao, H.; Li, H. The superimposed multi-channel helical long-period fiber grating and its application to multi-channel OAM mode generator. J. Lightw. Technol. 2021, 39, 3269–3275. [Google Scholar] [CrossRef]
- Detani, T.; Zhao, H.; Wang, P.; Suzuki, T.; Li, H. Simultaneous generation of the second- and third-order OAM modes by using a high-order helical long-period fiber grating. Opt. Lett. 2021, 46, 949–952. [Google Scholar] [CrossRef]
- Zhu, C.; Zhao, Y.; Chen, M.; Tong, R.; Hu, S.; Li, H. Simultaneous measurement of directional torsion and temperature by using a DC-sampled helical long-period fiber grating. Opt. Laser Technol. 2021, 142, 107171. [Google Scholar] [CrossRef]
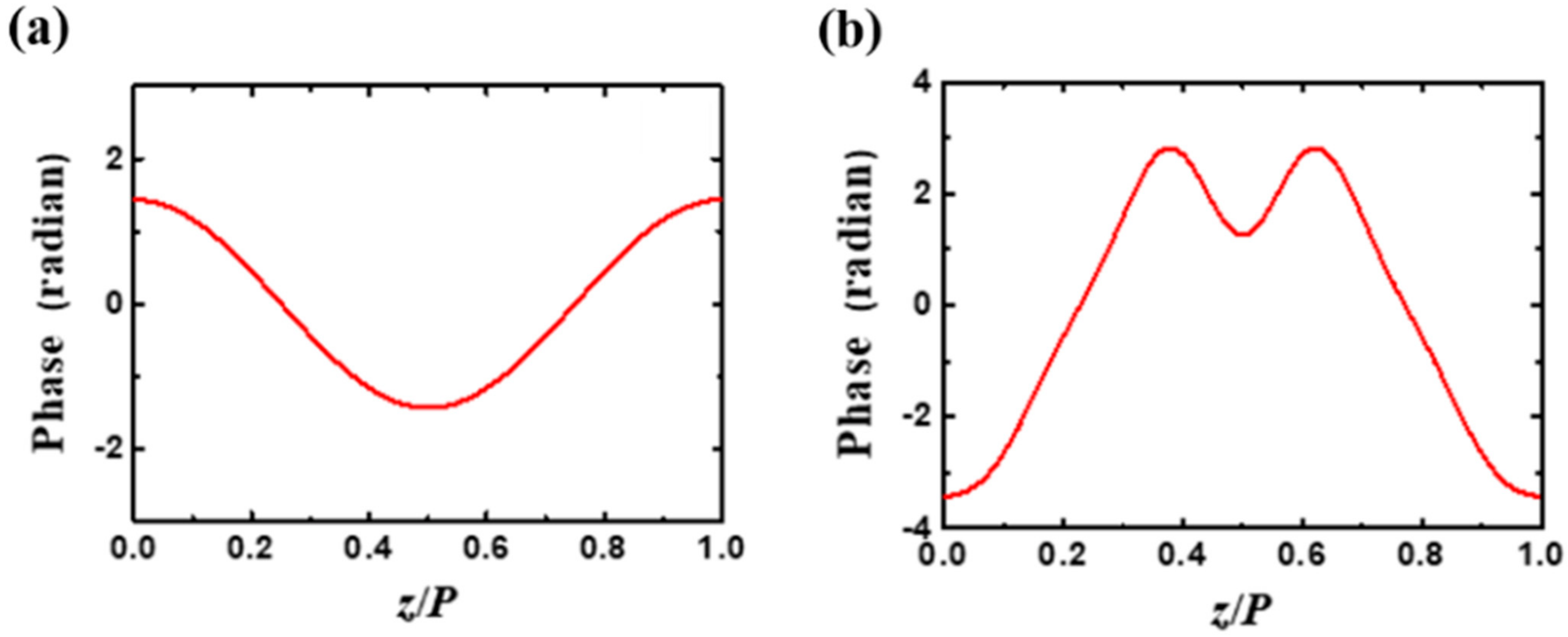
- Zhao, H.; Zhu, C.; Li, H. Design of an edge filter based on a phase-only modulated long-period fiber grating. IEEE Photon. J. 2018, 10, 1–9. [Google Scholar] [CrossRef]
- Wang, P.; Zhao, H.; Yamakawa, T.; Li, H. Polarization-independent flat-top band-rejection filter based on the phase-modulated HLPG. IEEE Photon. Technol. Lett. 2020, 32, 170–173. [Google Scholar] [CrossRef]
- Zhu, C.; Wang, L.; Bing, Z.; Tong, R.; Chen, M.; Hu, S.; Zhao, Y.; Li, H. Ultra-broadband OAM mode generator based on a phase-modulated helical grating working at a high radial-order of cladding mode. IEEE J. Quantum Electron. 2021, 57, 1–7. [Google Scholar] [CrossRef]
- Zhao, H.; Zhang, Z.; Zhang, M.; Hao, Y.; Wang, P.; Li, H.; Zhang, Z.; Zhang, M.; Hao, Y.; Wang, P.; et al. Broadband flat-top second-order OAM mode converter based on a phase-modulated helical long-period fiber grating. Opt. Exp. 2021, 29, 29518–29526. [Google Scholar] [CrossRef] [PubMed]
- Feng, L.; El-Ganainy, R.; Ge, L. Non-Hermitian photonics based on parity-time symmetry. Nat. Photon. 2017, 11, 752. [Google Scholar] [CrossRef]











Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, C.; Wang, L.; Li, H. Phase-Inserted Fiber Gratings and Their Applications to Optical Filtering, Optical Signal Processing, and Optical Sensing: Review. Photonics 2022, 9, 271. https://doi.org/10.3390/photonics9040271
Zhu C, Wang L, Li H. Phase-Inserted Fiber Gratings and Their Applications to Optical Filtering, Optical Signal Processing, and Optical Sensing: Review. Photonics. 2022; 9(4):271. https://doi.org/10.3390/photonics9040271
Chicago/Turabian StyleZhu, Chengliang, Lei Wang, and Hongpu Li. 2022. "Phase-Inserted Fiber Gratings and Their Applications to Optical Filtering, Optical Signal Processing, and Optical Sensing: Review" Photonics 9, no. 4: 271. https://doi.org/10.3390/photonics9040271
APA StyleZhu, C., Wang, L., & Li, H. (2022). Phase-Inserted Fiber Gratings and Their Applications to Optical Filtering, Optical Signal Processing, and Optical Sensing: Review. Photonics, 9(4), 271. https://doi.org/10.3390/photonics9040271





