Van Der Waals Materials for Subdiffractional Light Guidance
Abstract
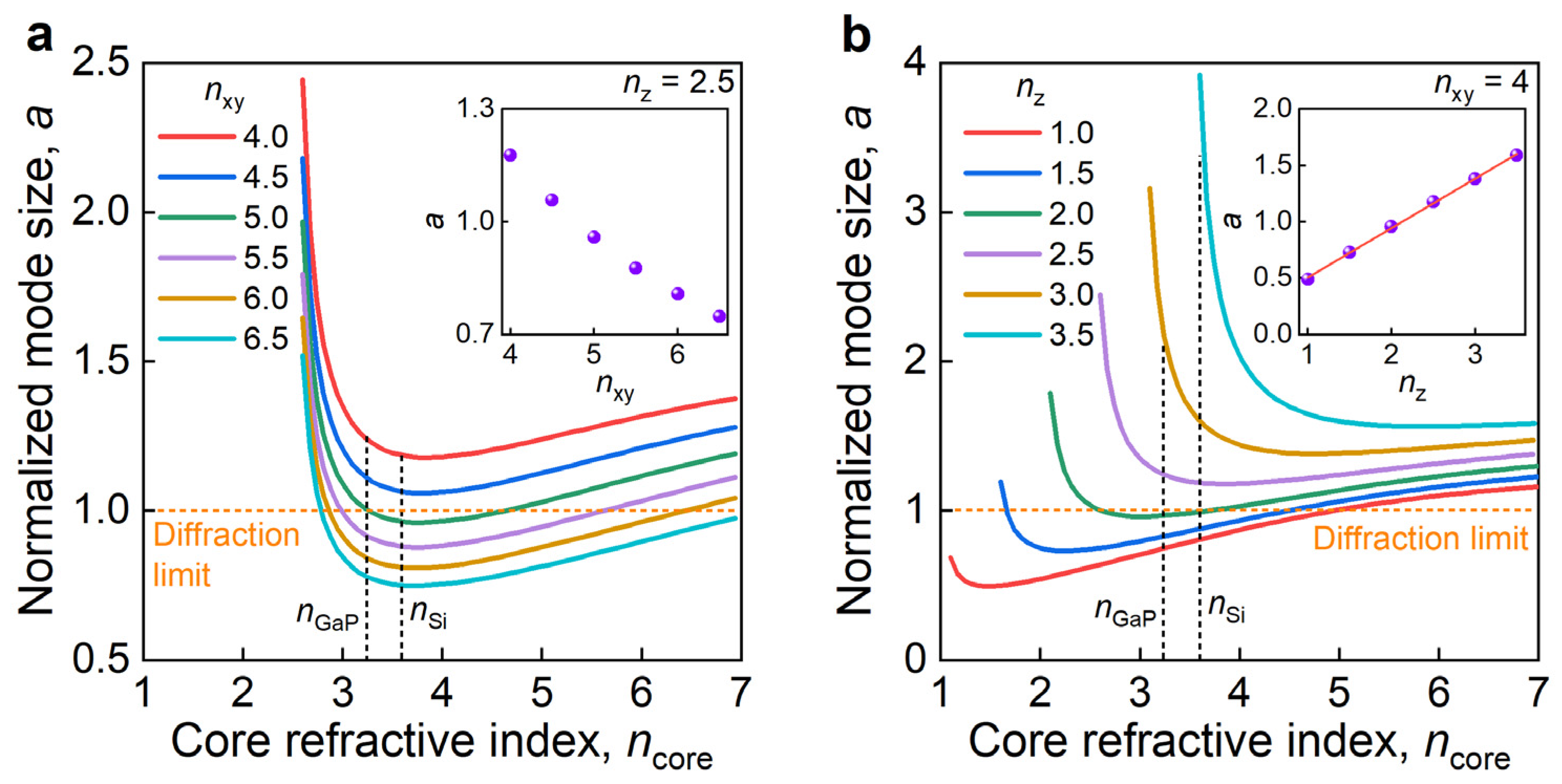
1. Introduction
2. Results
3. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A

References
- Jahani, S.; Jacob, Z. Transparent Subdiffraction Optics: Nanoscale Light Confinement without Metal. Optica 2014, 1, 96. [Google Scholar] [CrossRef]
- Jahani, S.; Kim, S.; Atkinson, J.; Wirth, J.C.; Kalhor, F.; Noman, A.A.; Newman, W.D.; Shekhar, P.; Han, K.; Van, V.; et al. Controlling Evanescent Waves Using Silicon Photonic All-Dielectric Metamaterials for Dense Integration. Nat. Commun. 2018, 9, 1893. [Google Scholar] [CrossRef]
- Mia, M.B.; Ahmed, S.Z.; Ahmed, I.; Lee, Y.J.; Qi, M.; Kim, S. Exceptional Coupling in Photonic Anisotropic Metamaterials for Extremely Low Waveguide Crosstalk. Optica 2020, 7, 881. [Google Scholar] [CrossRef]
- van Niekerk, M.; Jahani, S.; Bickford, J.; Cho, P.; Anderson, S.; Leake, G.; Coleman, D.; Fanto, M.L.; Tison, C.C.; Howland, G.A.; et al. Two-Dimensional Extreme Skin Depth Engineering for CMOS Photonics. J. Opt. Soc. Am. B 2021, 38, 1307. [Google Scholar] [CrossRef]
- Jahani, S.; Jacob, Z. All-Dielectric Metamaterials. Nat. Nanotechnol. 2016, 11, 23–36. [Google Scholar] [CrossRef] [PubMed]
- Ermolaev, G.A.; Grudinin, D.V.; Stebunov, Y.V.; Voronin, K.V.; Kravets, V.G.; Duan, J.; Mazitov, A.B.; Tselikov, G.I.; Bylinkin, A.; Yakubovsky, D.I.; et al. Giant Optical Anisotropy in Transition Metal Dichalcogenides for Next-Generation Photonics. Nat. Commun. 2021, 12, 854. [Google Scholar] [CrossRef]
- Bozhevolnyi, S.I.; Volkov, V.S.; Devaux, E.; Laluet, J.-Y.; Ebbesen, T.W. Channel Plasmon Subwavelength Waveguide Components Including Interferometers and Ring Resonators. Nature 2006, 440, 508–511. [Google Scholar] [CrossRef] [PubMed]
- Fedyanin, D.Y.; Yakubovsky, D.I.; Kirtaev, R.V.; Volkov, V.S. Ultralow-Loss CMOS Copper Plasmonic Waveguides. Nano Lett. 2016, 16, 362–366. [Google Scholar] [CrossRef]
- Thomaschewski, M.; Zenin, V.A.; Fiedler, S.; Wolff, C.; Bozhevolnyi, S.I. Plasmonic Lithium Niobate Mach–Zehnder Modulators. Nano Lett. 2022, 22, 6471–6475. [Google Scholar] [CrossRef]
- Minin, I.V.; Minin, O.V.; Glinskiy, I.A.; Khabibullin, R.A.; Malureanu, R.; Lavrinenko, A.; Yakubovsky, D.I.; Volkov, V.S.; Ponomarev, D.S. Experimental Verification of a Plasmonic Hook in a Dielectric Janus Particle. Appl. Phys. Lett. 2021, 118, 131107. [Google Scholar] [CrossRef]
- Hsu, C.W.; Zhen, B.; Lee, J.; Chua, S.-L.; Johnson, S.G.; Joannopoulos, J.D.; Soljačić, M. Observation of Trapped Light within the Radiation Continuum. Nature 2013, 499, 188–191. [Google Scholar] [CrossRef] [PubMed]
- Mekis, A.; Chen, J.C.; Kurland, I.; Fan, S.; Villeneuve, P.R.; Joannopoulos, J.D. High Transmission through Sharp Bends in Photonic Crystal Waveguides. Phys. Rev. Lett. 1996, 77, 3787–3790. [Google Scholar] [CrossRef] [PubMed]
- Dai, D.; Shi, Y.; He, S. Comparative Study of the Integration Density for Passive Linear Planar Light-Wave Circuits Based on Three Different Kinds of Nanophotonic Waveguide. Appl. Opt. 2007, 46, 1126. [Google Scholar] [CrossRef] [PubMed]
- Lebsir, Y.; Boroviks, S.; Thomaschewski, M.; Bozhevolnyi, S.I.; Zenin, V.A. Ultimate Limit for Optical Losses in Gold, Revealed by Quantitative Near-Field Microscopy. Nano Lett. 2022, 22, 5759–5764. [Google Scholar] [CrossRef] [PubMed]
- Hemmati, H.; Magnusson, R. Applicability of Rytov’s Full Effective-Medium Formalism to the Physical Description and Design of Resonant Metasurfaces. ACS Photonics 2020, 7, 3177–3187. [Google Scholar] [CrossRef]
- Rytov, S.M. Electromagnetic Properties of a Finely Stratified Medium. Sov. Phys. JETP 1956, 2, 446–475. [Google Scholar]
- Kats, M.A.; Genevet, P.; Aoust, G.; Yu, N.; Blanchard, R.; Aieta, F.; Gaburro, Z.; Capasso, F. Giant Birefringence in Optical Antenna Arrays with Widely Tailorable Optical Anisotropy. Proc. Natl. Acad. Sci. USA 2012, 109, 12364–12368. [Google Scholar] [CrossRef]
- Niu, S.; Joe, G.; Zhao, H.; Zhou, Y.; Orvis, T.; Huyan, H.; Salman, J.; Mahalingam, K.; Urwin, B.; Wu, J.; et al. Giant Optical Anisotropy in a Quasi-One-Dimensional Crystal. Nat. Photonics 2018, 12, 392–396. [Google Scholar] [CrossRef]
- Verre, R.; Baranov, D.G.; Munkhbat, B.; Cuadra, J.; Käll, M.; Shegai, T. Transition Metal Dichalcogenide Nanodisks as High-Index Dielectric Mie Nanoresonators. Nat. Nanotechnol. 2019, 14, 679–684. [Google Scholar] [CrossRef]
- Ermolaev, G.; Voronin, K.; Baranov, D.G.; Kravets, V.; Tselikov, G.; Stebunov, Y.; Yakubovsky, D.; Novikov, S.; Vyshnevyy, A.; Mazitov, A.; et al. Topological Phase Singularities in Atomically Thin High-Refractive-Index Materials. Nat. Commun. 2022, 13, 2049. [Google Scholar] [CrossRef]
- Ermolaev, G.A.; Stebunov, Y.V.; Vyshnevyy, A.A.; Tatarkin, D.E.; Yakubovsky, D.I.; Novikov, S.M.; Baranov, D.G.; Shegai, T.; Nikitin, A.Y.; Arsenin, A.V.; et al. Broadband Optical Properties of Monolayer and Bulk MoS2. npj 2D Mater. Appl. 2020, 4, 21. [Google Scholar] [CrossRef]
- Mounet, N.; Gibertini, M.; Schwaller, P.; Campi, D.; Merkys, A.; Marrazzo, A.; Sohier, T.; Castelli, I.E.; Cepellotti, A.; Pizzi, G.; et al. Two-Dimensional Materials from High-Throughput Computational Exfoliation of Experimentally Known Compounds. Nat. Nanotechnol. 2018, 13, 246–252. [Google Scholar] [CrossRef] [PubMed]
- Munkhbat, B.; Yankovich, A.B.; Baranov, D.G.; Verre, R.; Olsson, E.; Shegai, T.O. Transition Metal Dichalcogenide Metamaterials with Atomic Precision. Nat. Commun. 2020, 11, 4604. [Google Scholar] [CrossRef] [PubMed]
- Munkhbat, B.; Küçüköz, B.; Baranov, D.G.; Antosiewicz, T.J.; Shegai, T.O. Nanostructured Transition Metal Dichalcogenide Multilayers for Advanced Nanophotonics. arXiv 2022, arXiv:2202.04898. [Google Scholar]
- Tselikov, G.I.; Ermolaev, G.A.; Popov, A.A.; Tikhonowski, G.V.; Panova, D.A.; Taradin, A.S.; Vyshnevyy, A.A.; Syuy, A.V.; Klimentov, S.M.; Novikov, S.M.; et al. Transition Metal Dichalcogenide Nanospheres for High-Refractive-Index Nanophotonics and Biomedical Theranostics. Proc. Natl. Acad. Sci. USA 2022, 119, e2208830119. [Google Scholar] [CrossRef]
- Okamoto, K. Fundamentals of Optical Waveguides, 2nd ed.; Optics and Photonics Series; Elsevier: Amsterdam, The Netherlands, 2005; ISBN 0125250967. [Google Scholar]
- Oulton, R.F.; Bartal, G.; Pile, D.F.P.; Zhang, X. Confinement and Propagation Characteristics of Subwavelength Plasmonic Modes. New J. Phys. 2008, 10, 105018. [Google Scholar] [CrossRef]
- Khmelevskaia, D.; Markina, D.I.; Fedorov, V.V.; Ermolaev, G.A.; Arsenin, A.V.; Volkov, V.S.; Goltaev, A.S.; Zadiranov, Y.M.; Tzibizov, I.A.; Pushkarev, A.P.; et al. Directly Grown Crystalline Gallium Phosphide on Sapphire for Nonlinear All-Dielectric Nanophotonics. Appl. Phys. Lett. 2021, 118, 201101. [Google Scholar] [CrossRef]
- Novoselov, K.S.; Mishchenko, A.; Carvalho, A.; Castro Neto, A.H. 2D Materials and van Der Waals Heterostructures. Science 2016, 353, aac9439. [Google Scholar] [CrossRef]
- Taboada-Gutiérrez, J.; Álvarez-Pérez, G.; Duan, J.; Ma, W.; Crowley, K.; Prieto, I.; Bylinkin, A.; Autore, M.; Volkova, H.; Kimura, K.; et al. Broad Spectral Tuning of Ultra-Low-Loss Polaritons in a van Der Waals Crystal by Intercalation. Nat. Mater. 2020, 19, 964–968. [Google Scholar] [CrossRef]
- Duan, J.; Capote-Robayna, N.; Taboada-Gutiérrez, J.; Álvarez-Pérez, G.; Prieto, I.; Martín-Sánchez, J.; Nikitin, A.Y.; Alonso-González, P. Twisted Nano-Optics: Manipulating Light at the Nanoscale with Twisted Phonon Polaritonic Slabs. Nano Lett. 2020, 20, 5323–5329. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ermolaev, G.; Grudinin, D.; Voronin, K.; Vyshnevyy, A.; Arsenin, A.; Volkov, V. Van Der Waals Materials for Subdiffractional Light Guidance. Photonics 2022, 9, 744. https://doi.org/10.3390/photonics9100744
Ermolaev G, Grudinin D, Voronin K, Vyshnevyy A, Arsenin A, Volkov V. Van Der Waals Materials for Subdiffractional Light Guidance. Photonics. 2022; 9(10):744. https://doi.org/10.3390/photonics9100744
Chicago/Turabian StyleErmolaev, Georgy, Dmitriy Grudinin, Kirill Voronin, Andrey Vyshnevyy, Aleksey Arsenin, and Valentyn Volkov. 2022. "Van Der Waals Materials for Subdiffractional Light Guidance" Photonics 9, no. 10: 744. https://doi.org/10.3390/photonics9100744
APA StyleErmolaev, G., Grudinin, D., Voronin, K., Vyshnevyy, A., Arsenin, A., & Volkov, V. (2022). Van Der Waals Materials for Subdiffractional Light Guidance. Photonics, 9(10), 744. https://doi.org/10.3390/photonics9100744






