Phase-Controlled Planar Metalenses for High-Resolution Terahertz Focusing
Abstract
1. Introduction
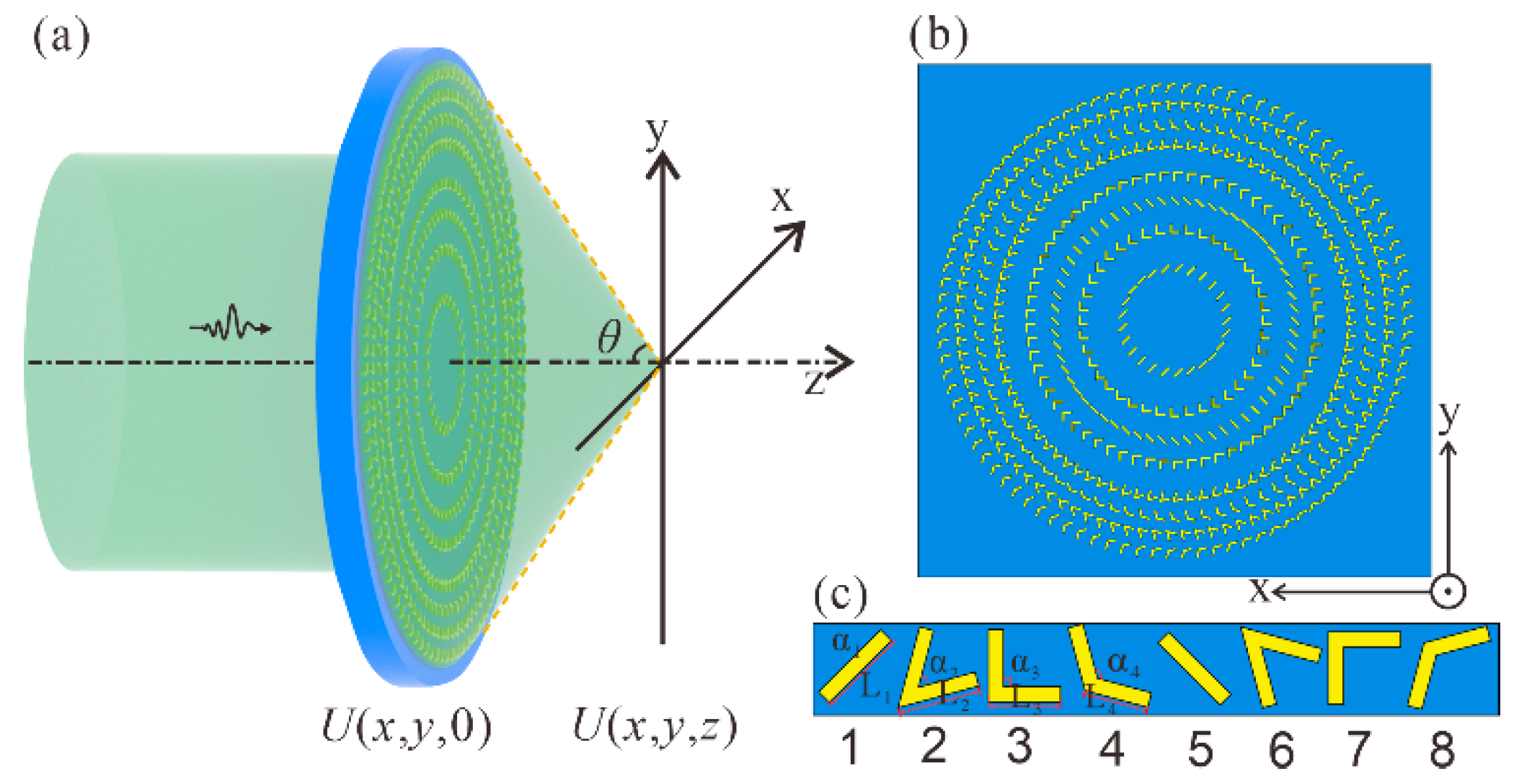
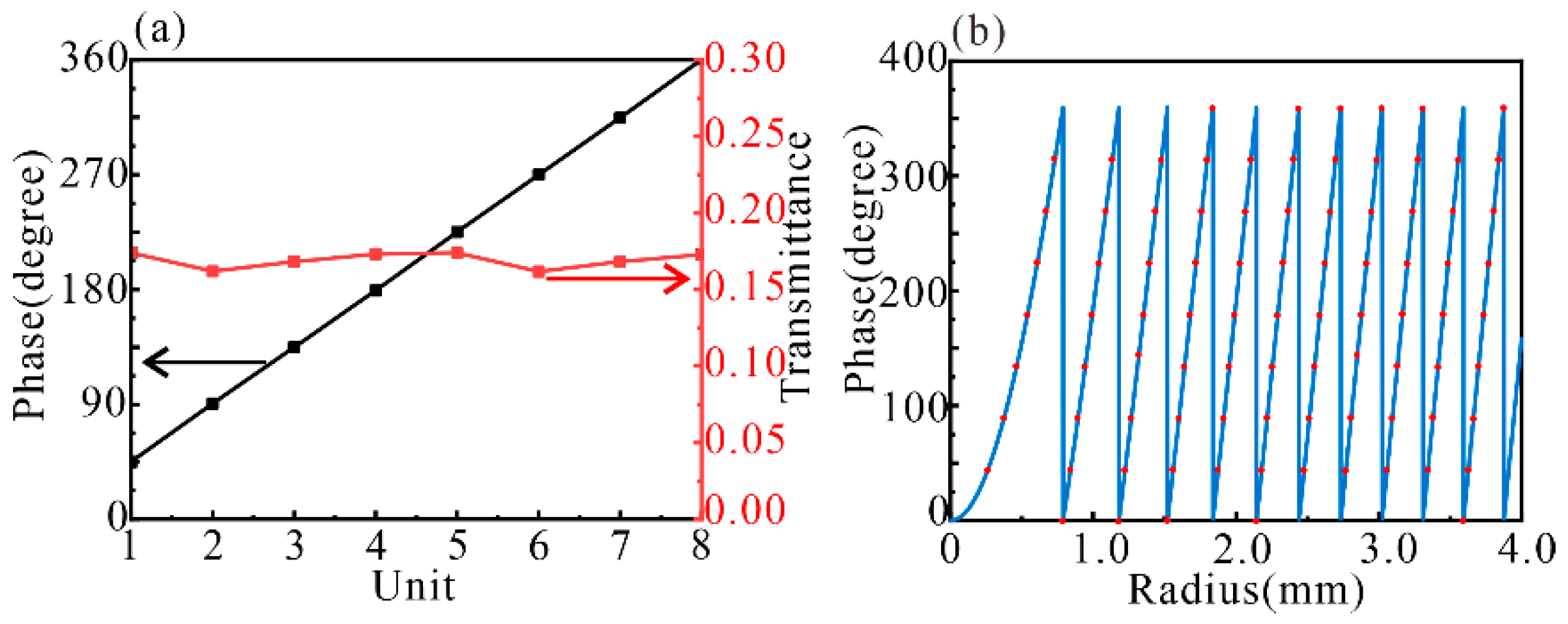
2. Design and Theory
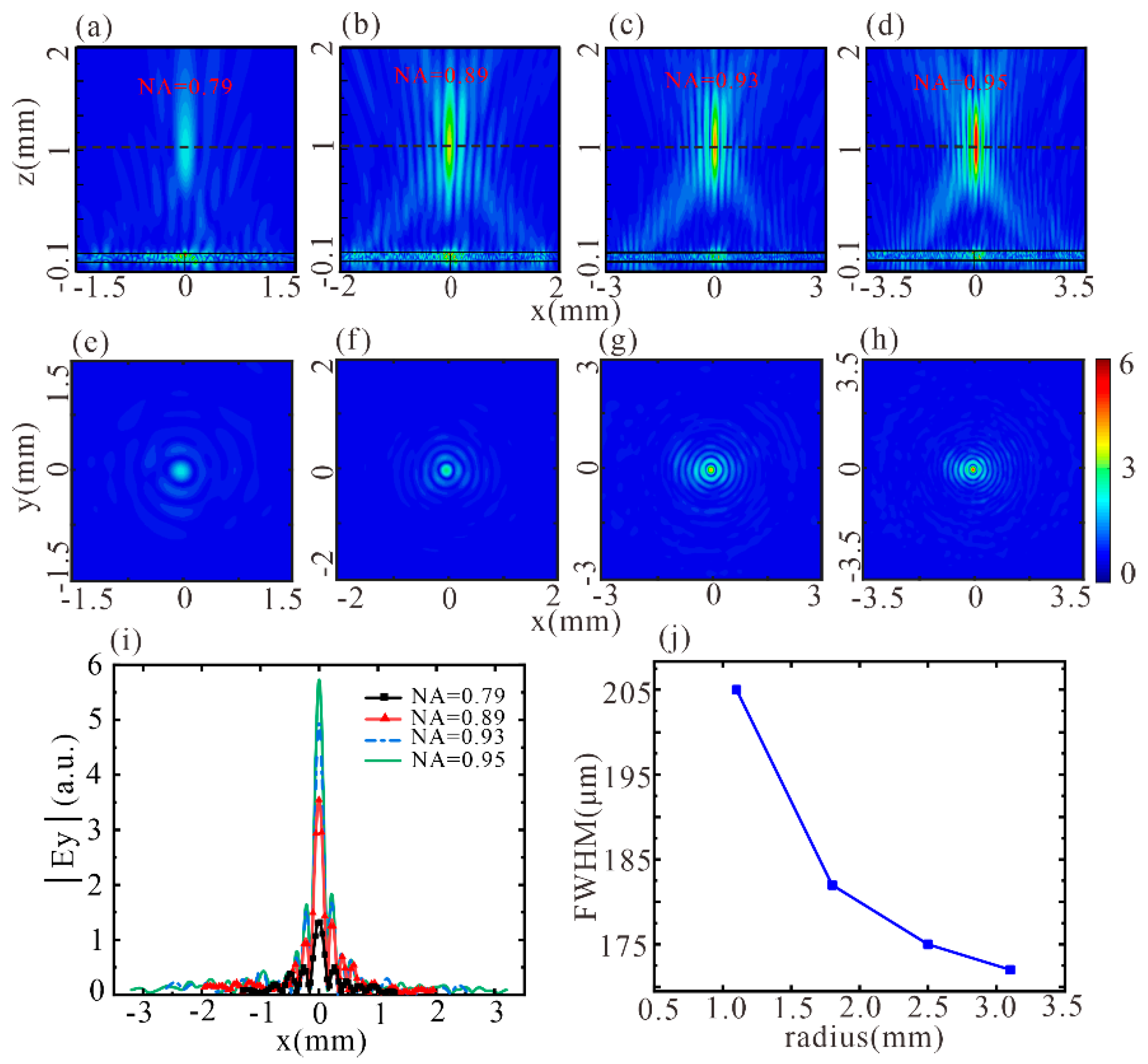
3. Simulation Results and Discussion
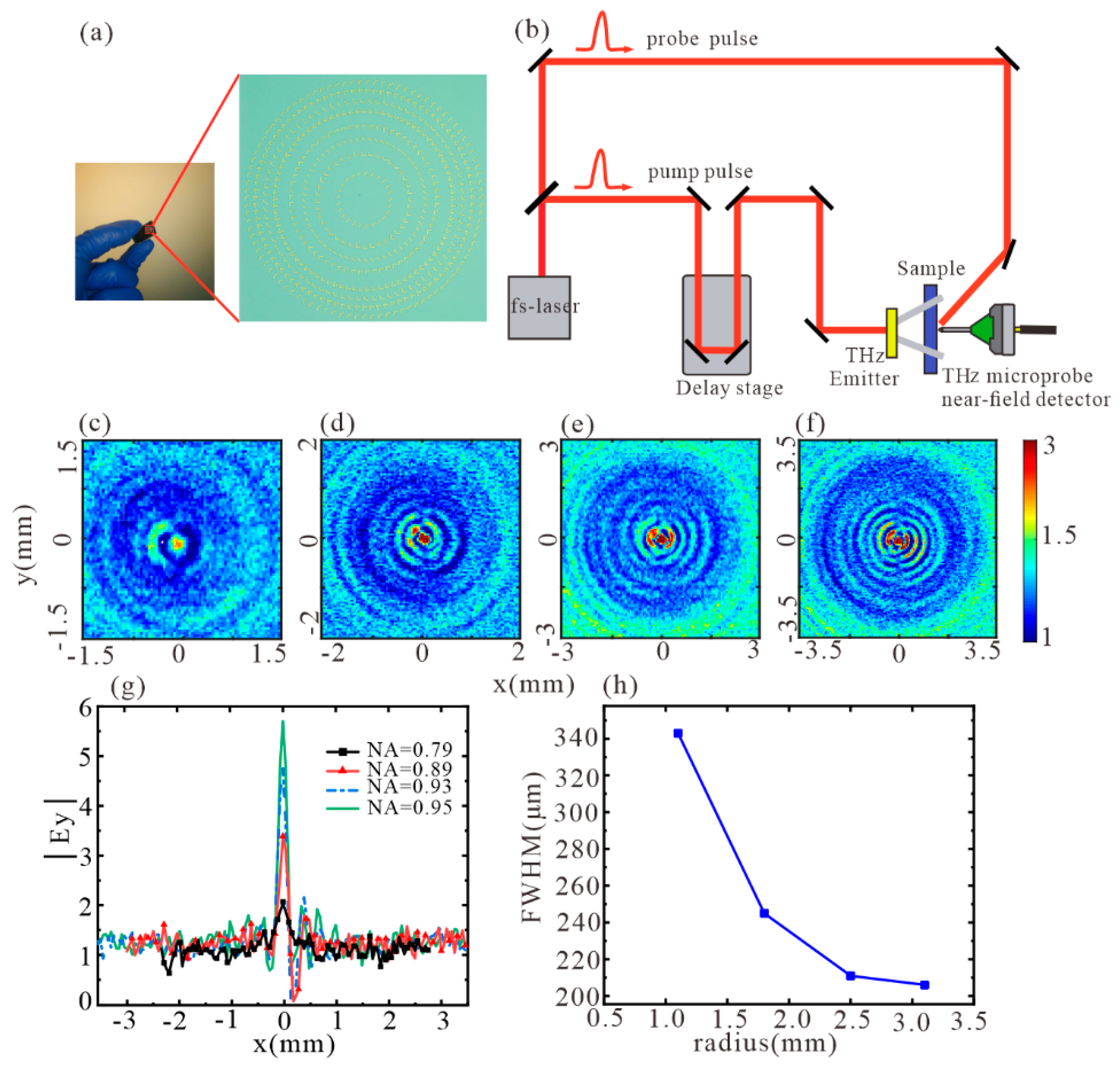
4. Experimental Results
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Chen, S.; Li, Z.; Zhang, Y.; Cheng, H.; Tian, J. Phase Manipulation of Electromagnetic Waves with Metasurfaces and Its Applications in Nanophotonics. Adv. Opt. Mater. 2018, 6, 1800104. [Google Scholar] [CrossRef]
- Grady, N.K.; Heyes, J.E.; Chowdhury, D.R.; Zeng, Y.; Reiten, M.T.; Azad, A.K.; Taylor, A.J.; Dalvit, D.A.; Chen, H.T. Terahertz metamaterials for linear polarization conversion and anomalous refraction. Science 2013, 340, 1304–1307. [Google Scholar] [CrossRef] [PubMed]
- Chen, X.; Huang, L.; Mühlenbernd, H.; Li, G.; Bai, B.; Tan, Q.; Jin, G.; Qiu, C.W.; Zhang, S.; Zentgraf, T. Dual-polarity plasmonicmetalens for visible light. Nat. Commun. 2012, 3, 1–6. [Google Scholar] [CrossRef]
- Ma, C.; Liu, Z. A super resolution metalens with phase compensation mechanism. Appl. Phys. Lett. 2010, 96, 183103. [Google Scholar] [CrossRef]
- Williams, C.; Montelongo, Y.; Wilkinson, T.D. PlasmonicMetalens for Narrowband Dual-Focus Imaging. Adv. Opt. Mater. 2017, 5, 1700811. [Google Scholar] [CrossRef]
- Ni, X.; Ishii, S.; Kildishev, A.V.; Shalaev, V.M. Ultra-thin, planar, Babinet-inverted plasmonicmetalenses. Light Sci. Appl. 2013, 2, e72. [Google Scholar] [CrossRef]
- Hu, D.; Wang, X.; Feng, S.; Ye, J.; Sun, W.; Kan, Q.; Klar, P.J.; Zhang, Y. Ultrathin Terahertz Planar Elements. Adv. Opt. Mater. 2013, 1, 186–191. [Google Scholar] [CrossRef]
- Liu, Z.; Lee, H.; Xiong, Y.; Sun, C.; Zhang, X. Far-field optical hyperlens magnifying sub-diffraction-limited objects. Science 2007, 315, 1686. [Google Scholar] [CrossRef]
- Fang, N.; Lee, H.; Sun, C.; Zhang, X. Sub-Diffraction-Limited Optical Imaging with a Silver Superlens. Science 2005, 5721, 534–537. [Google Scholar] [CrossRef] [PubMed]
- Berweger, S.; Atkin, J.M.; Olmon, R.L.; Raschke, M.B. Adiabatic Tip-Plasmon Focusing for Nano-Raman Spectroscopy. J. Phys. Chem. Lett. 2010, 1, 3427–3432. [Google Scholar] [CrossRef]
- Manikandan, E.; Sreeja, B.S.; Radha, S.; Bathe, R.N. Direct laser fabrication of five-band symmetric terahertz metamaterial with Fano resonance. Mater. Lett. 2018, 229, 320–323. [Google Scholar] [CrossRef]
- Yang, C.; Shen, Y.; Xie, Y.; Zhou, Q.; Deng, X.; Cao, J. Terahertz planar lenses based on plasmonicmetasurfaces. Phys. Lett. A 2019, 383, 789–792. [Google Scholar] [CrossRef]
- Zhang, F.; Yu, H.; Fang, J.; Zhang, M.; Chen, S.; Wang, J.; He, A.; Chen, J. Efficient generation and tight focusing of radially polarized beam from linearly polarized beam with all-dielectric metasurface. Opt. Express 2016, 24, 6656. [Google Scholar] [CrossRef] [PubMed]
- Fattal, D.; Li, J.; Peng, Z.; Fiorentino, M.; Beausoleil, R.G. Flat dielectric grating reflectors with focusing abilities. Nat. Photonics 2010, 4, 466–470. [Google Scholar] [CrossRef]
- Yu, N.; Genevet, P.; Kats, M.A.; Aieta, F.; Tetienne, J.P.; Capasso, F.; Gaburro, Z. Light Propagation with Phase Discontinuities: Generalized Laws of Reflection and Refraction. Science 2011, 334, 333–337. [Google Scholar] [CrossRef] [PubMed]
- Gao, H.; Pu, M.; Li, X.; Ma, X.; Zhao, Z.; Guo, Y.; Luo, X. Super-resolution imaging with a Bessel lens realized by a geometric metasurface. Opt. Express 2017, 25, 13933–13943. [Google Scholar] [CrossRef] [PubMed]
- Jiang, R.H.; Chen, C.; Lin, D.Z.; Chou, H.C.; Chu, J.Y.; Yen, T.J. Near-Field Plasmonic Probe with Super Resolution and High Throughput and Signal-to-Noise Ratio. Nano Lett. 2018, 18, 881–885. [Google Scholar] [CrossRef]
- Khorasaninejad, M.; Chen, W.T.; Oh, J.; Capasso, F. Super-Dispersive Off-Axis Meta-Lenses for Compact High Resolution Spectroscopy. Nano Lett. 2016, 16, 3732–3737. [Google Scholar] [CrossRef]
- Khorasaninejad, M.; Chen, W.T.; Devlin, R.C.; Oh, J.; Zhu, A.Y.; Capasso, F. Metalenses at visible wavelengths: Diffraction-limited focusing and subwavelength resolution imaging. Science 2016, 4, 352–1190. [Google Scholar] [CrossRef]
- Xie, Y.; Yang, C.; Wang, Y.; Shen, Y.; Deng, X.; Zhou, B.; Cao, J. Anomalous refraction and reflection characteristics of bend V-shaped antenna metasur faces. Sci. Rep. 2019, 9, 1–8. [Google Scholar] [CrossRef]
- Blanchard, R.; Aoust, G.; Genevet, P.; Yu, N.; Kats, M.A.; Gaburro, Z.; Capasso, F. Modeling nanoscale V-shaped antennas for the design of optical phased arrays. Phys. Rev. B 2012, 85, 155457. [Google Scholar] [CrossRef]
- Aieta, F.; Genevet, P.; Yu, N.; Kats, M.A.; Gaburro, Z.; Capasso, F. Out-of-Plane Reflection and Refraction of Light by Anisotropic Optical Antenna Metasurfaces with Phase Discontinuities. Nano Lett. 2012, 12, 1702–1706. [Google Scholar] [CrossRef]
- Wen, D.; Yue, F.; Kumar, S.; Ma, Y.; Chen, M.; Ren, X.; Kremer, P.E.; Gerardot, B.D.; Taghizadeh, M.R.; Buller, G.S.; et al. Metasurface for characterization of the polarization state of light. Opt. Express 2015, 23, 10272. [Google Scholar] [CrossRef] [PubMed]
- Fan, Q.; Huo, P.; Wang, D.; Liang, Y.; Yan, F.; Xu, T. Visible light focusing flat lenses based on hybrid dielectric-metal metasurface reflector-arrays. Sci. Rep. 2017, 7, 1–9. [Google Scholar] [CrossRef] [PubMed]
- Ding, P.; Li, Y.; Shao, L.; Tian, X.; Wang, J.; Fan, C. Graphene aperture-based metalens for dynamic focusing of terahertz waves. Opt. Express 2018, 26, 28038–28050. [Google Scholar] [CrossRef]
- Ramm, A.G. Does negative refraction make a perfect lens. Phys. Rev. Lett. 2008, 372, 6518–6520. [Google Scholar] [CrossRef][Green Version]
- Tan, Q.; Xu, Z.; Zhang, D.H.; Yu, T.; Zhang, S.; Luo, Y. Polarization-Controlled Plasmonic Structured Illumination. Nano Lett. 2020, 20, 2602–2608. [Google Scholar] [CrossRef] [PubMed]
- Richards, B.; Wolf, E. Electromagnetic Diffraction in Optical Systems. II. Structure of the Image Field in an Aplanatic System. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1959, 253, 358–379. [Google Scholar] [CrossRef]
- Huang, F.M.; Kao, T.S.; Fedotov, V.A.; Chen, Y.; Zheludev, N.I. Nanohole Array as a Lens. Nano Lett. 2008, 8, 2469–2472. [Google Scholar] [CrossRef] [PubMed]
- Ullah, N.; Liu, W.; Wang, G.; Wang, Z.; Khalid, A.U.R.; Hu, B.; Liu, J.; Zhang, Y. Gate-controlled terahertz focusing based on graphene-loaded metasurface. Opt. Express 2020, 28, 2789. [Google Scholar] [CrossRef] [PubMed]
- Cheng, Q.; Ma, M.; Yu, D.; Shen, Z.; Xie, J.; Wang, J.; Xu, N.; Guo, H.; Hu, W.; Wang, S.; et al. Broadband achromatic metalens in terahertz regime. Sci. Bull. 2019, 64, 1525–1531. [Google Scholar] [CrossRef]
- Cai, H.; Czaplewski, D.; Ogando, K.; Martinson, A.; Gosztola, D.; Stan, L.; López, D. Ultrathin transmissive metasurfaces for multi-wavelength optics in the visible. Appl. Phys. Lett. 2019, 114, 71106. [Google Scholar] [CrossRef]
- Dharmavarapu, R.; Izumi, K.I.; Katayama, I.; Ng, S.H.; Vongsvivut, J.; Tobin, M.J.; Kuchmizhak, A.; Nishijima, Y.; Bhattacharya, S.; Juodkazis, S. Dielectric cross-shaped-resonator-based metasurface for vortex beam generation at mid-IR and THz wavelengths. Nanophotonics 2019, 8, 1263–1270. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, X.; Shen, Y.; Dai, G.; Zou, L.; Zhang, T.; Deng, X. Phase-Controlled Planar Metalenses for High-Resolution Terahertz Focusing. Photonics 2021, 8, 143. https://doi.org/10.3390/photonics8050143
Yu X, Shen Y, Dai G, Zou L, Zhang T, Deng X. Phase-Controlled Planar Metalenses for High-Resolution Terahertz Focusing. Photonics. 2021; 8(5):143. https://doi.org/10.3390/photonics8050143
Chicago/Turabian StyleYu, Xin, Yun Shen, Guohong Dai, Liner Zou, Tailin Zhang, and Xiaohua Deng. 2021. "Phase-Controlled Planar Metalenses for High-Resolution Terahertz Focusing" Photonics 8, no. 5: 143. https://doi.org/10.3390/photonics8050143
APA StyleYu, X., Shen, Y., Dai, G., Zou, L., Zhang, T., & Deng, X. (2021). Phase-Controlled Planar Metalenses for High-Resolution Terahertz Focusing. Photonics, 8(5), 143. https://doi.org/10.3390/photonics8050143



